Applicability of Single-Borehole Dilution Tests in Aquifers with Vertical Flow
Abstract
1. Introduction
2. Hydrogeological Context
3. Materials and Methods
3.1. Single-Borehole Dilution Tests (SBDTs)
3.1.1. Field Procedure
3.1.2. Hydraulic Conductivity Calculation
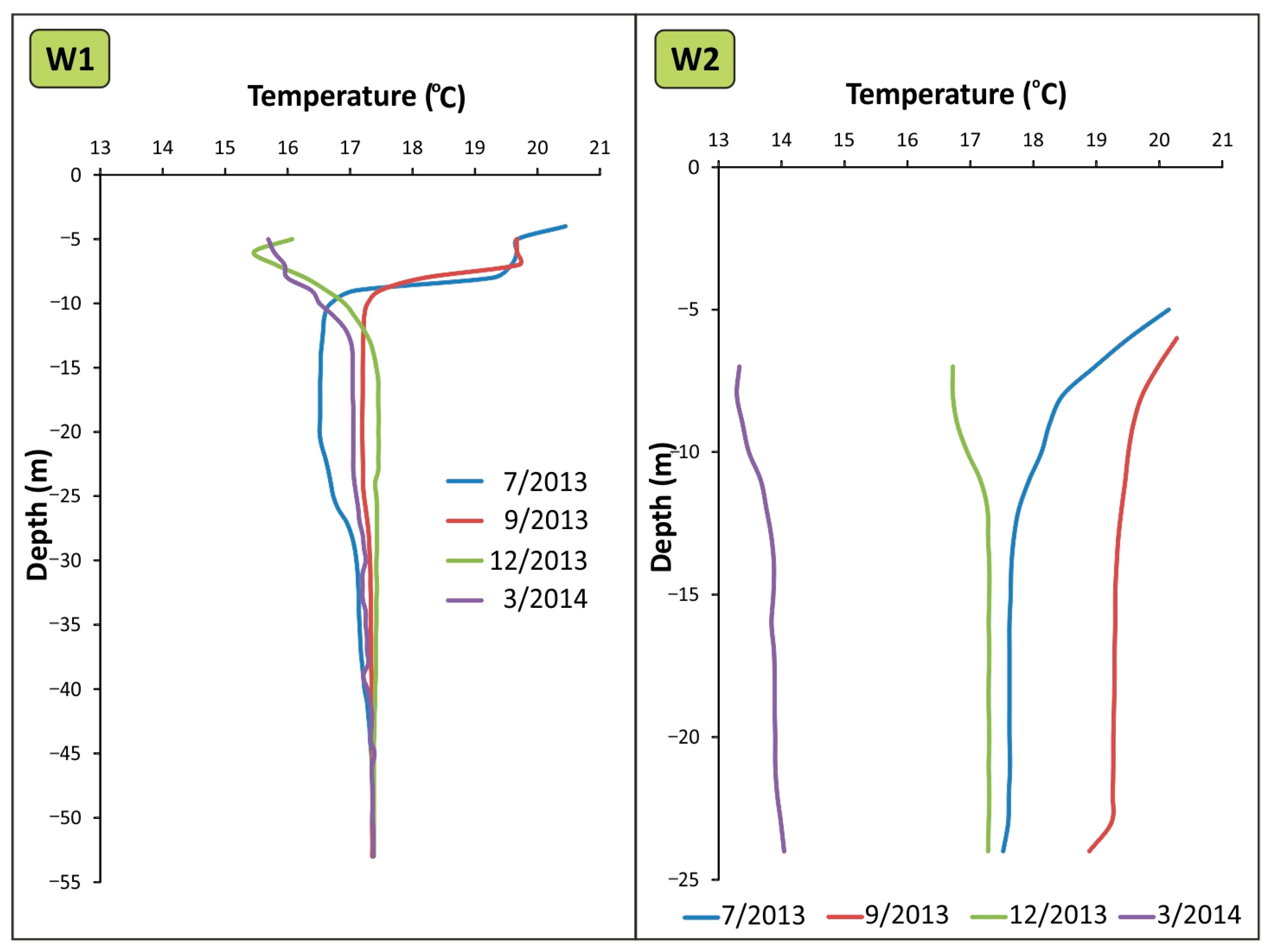
3.2. Temperature Logs
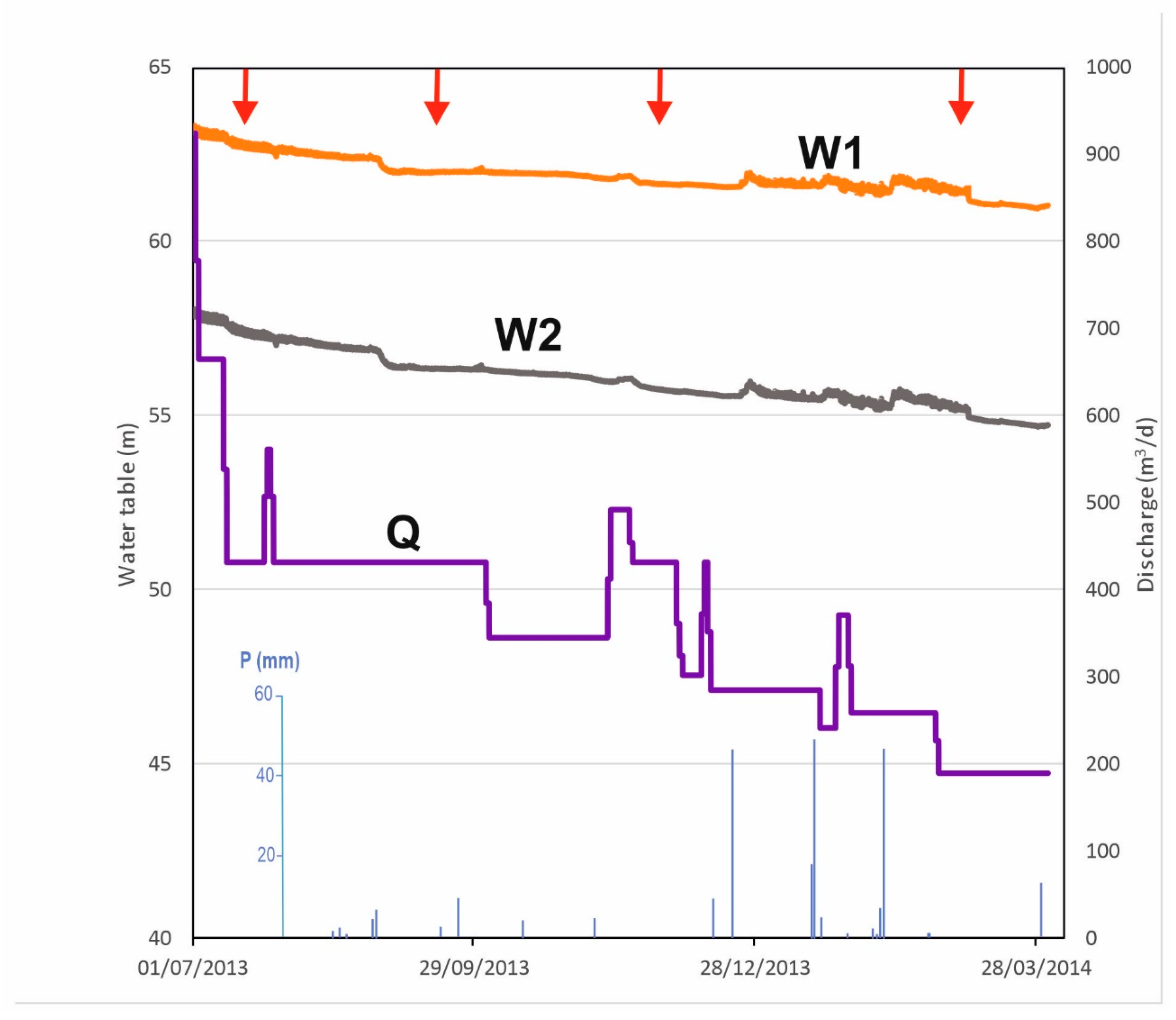
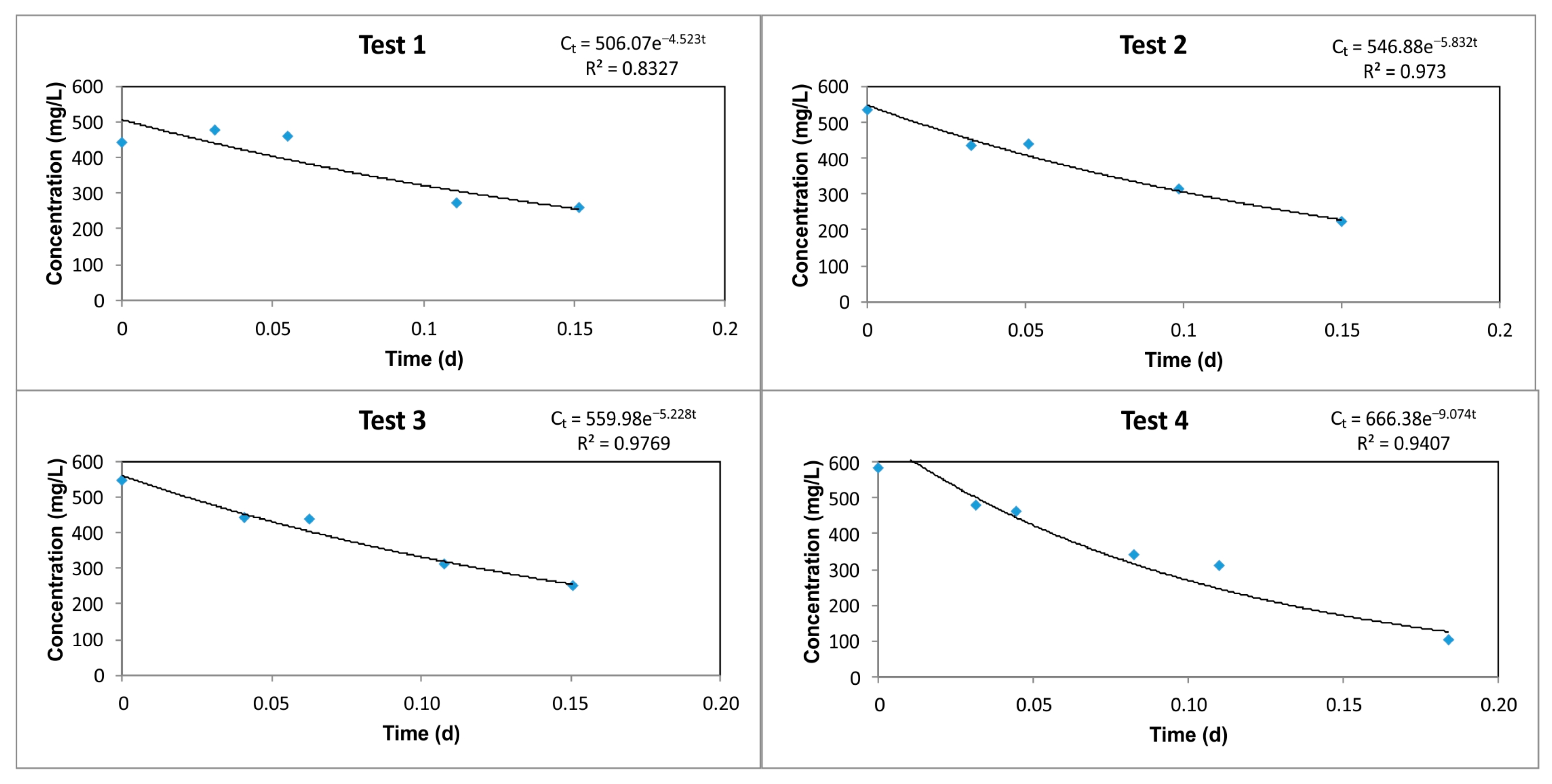
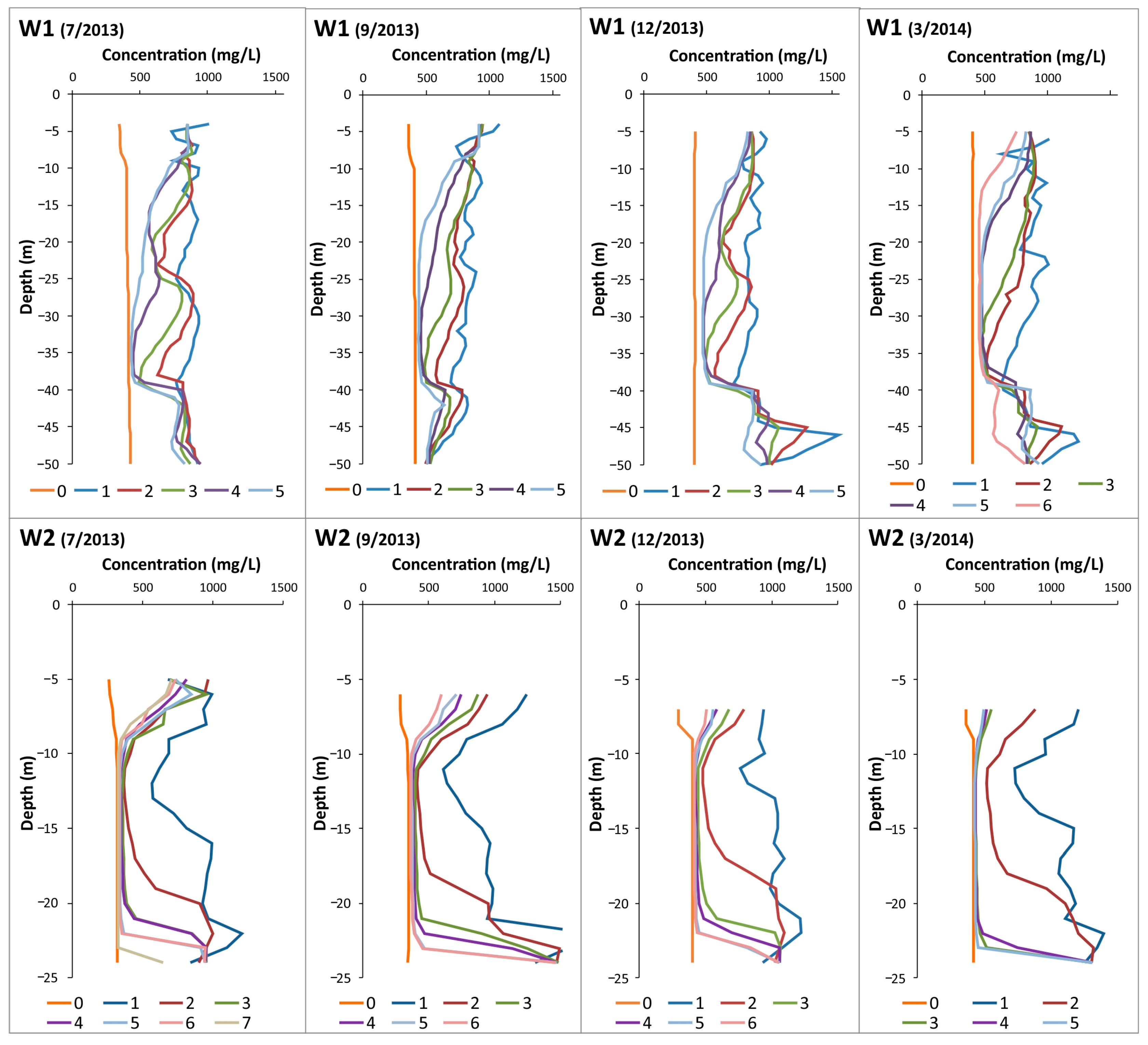
4. Results
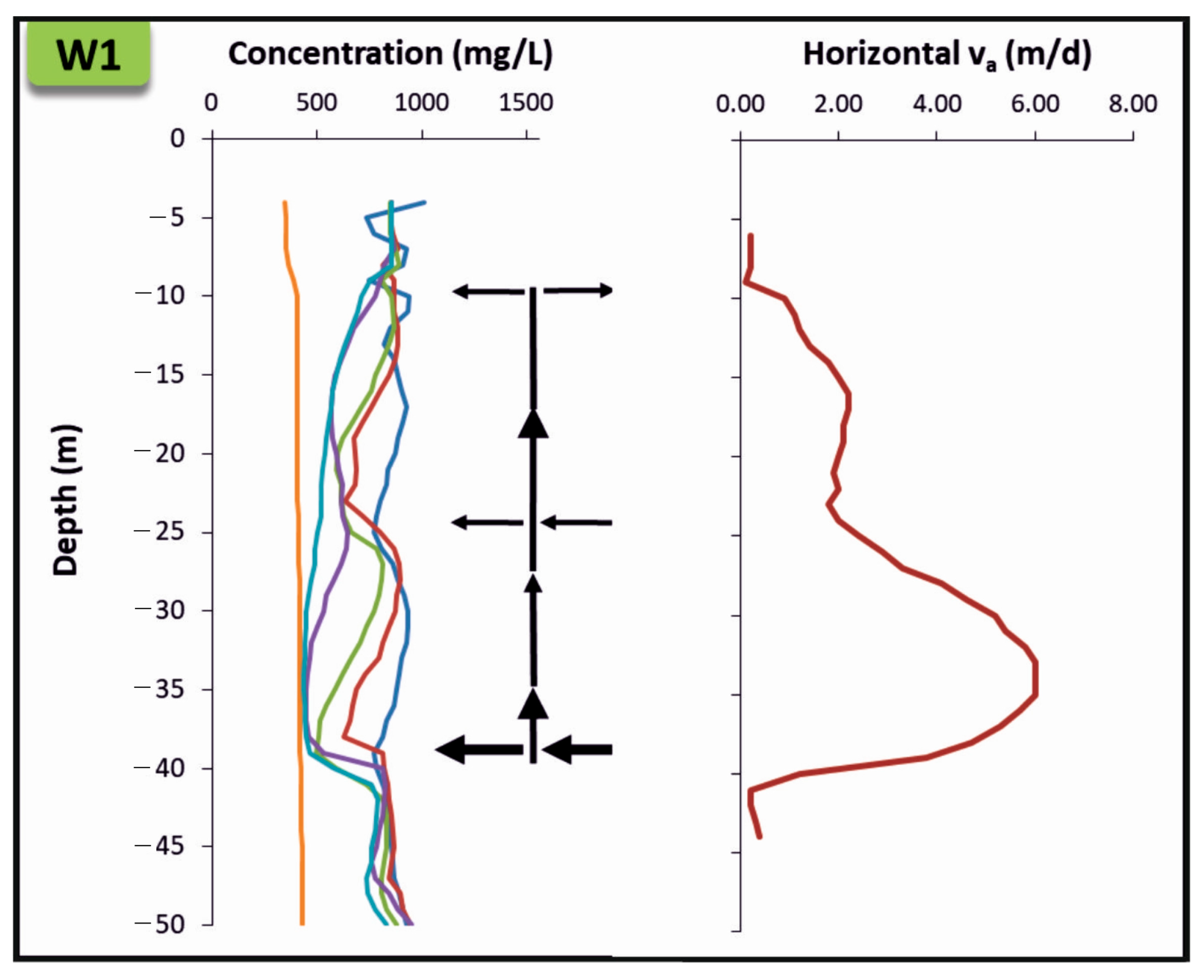
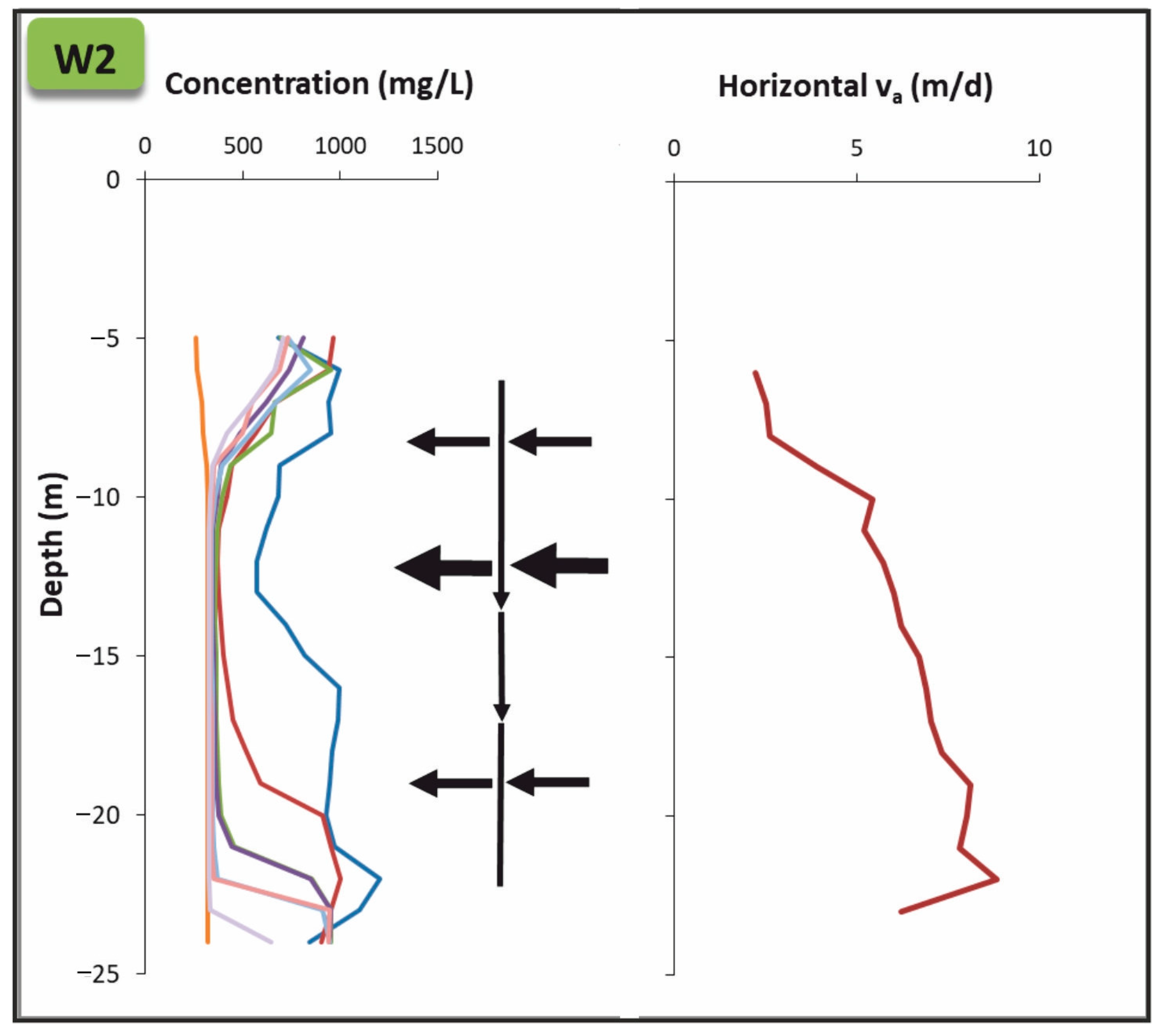
4.1. Concentration Logs
4.2. Darcy Velocity and Hydraulic Conductivity
4.3. Temperature Logs
5. Discussion
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ogilvi, N.A. Electrolytic method for determination of the ground water filtration velocity. Bull. Sci. Technol. News 1958, 4, 23–44. (In Russian) [Google Scholar]
- Halevy, E.; Moser, H.; Zellhofer, O.; Zuber, A. Borehole dilution techniques: A critical review. In Proceedings of the Symposium on Isotopes in Hydrology; IAEA: Vienna, Austria, 1967; pp. 531–564. [Google Scholar]
- Drost, W.; Klotz, D.; Koch, A.; Moser, H.; Neumaier, F.; Rauert, W. Point dilution methods of investigating ground water flow by means of radioisotopes. Water Resour. Res. 1968, 4, 125–146. [Google Scholar] [CrossRef]
- Grisak, G.E.; Merrit, W.F.; Williams, D.W. Fluoride borehole dilution apparatus for groundwater velocity measurements. Canadian Geotech. J. 1977, 14, 554–561. [Google Scholar] [CrossRef]
- Klotz, D.; Moser, H.; Trimborn, P. Single borehole techniques: Present status and examples of recent applications. In Proceedings of the Symposium on Isotopes in Hydrology; IAEA: Vienna, Austria, 1978; pp. 159–175. [Google Scholar]
- Lewis, D.C.; Kritz, G.J.; Burgy, R.H. Tracer dilution sampling technique to determine hydraulic conductivity of fractured rock. Water Resour. Res. 1966, 2, 533–542. [Google Scholar] [CrossRef]
- Michalski, A. Conductive slug tracing as a single-well test technique for heterogeneous and fractured formations. In Proceedings of the Conference on New Field Techniques for Quantifying the Physical and Chemical Properties, Dallas, TX, USA, 20–23 March 1989; pp. 247–263. [Google Scholar]
- Sottani, A.; Del Prà, A. Groundwater velocity measurements with the single point dilution method (SPDM) in a sample site of the High Venetian Plain (Galliera V.–northern Italy). In Proceedings of International Meeting of Young Researchers in Applied Geology; Politecnico di Torino: Turin, Italy, 1995. [Google Scholar]
- Pitrak, M.; Mares, S.; Kobr, M. A simple borehole dilution technique in measuring horizontal ground water flow. Ground Water 2007, 45, 89–92. [Google Scholar] [CrossRef] [PubMed]
- Williams, A.; Bloomfield, J.; Griffiths, K.; Butler, A. Characterising the vertical variations in hydraulic conductivity within the Chalk aquifer. J. Hydrol. 2006, 330, 53–62. [Google Scholar] [CrossRef]
- Bernstein, A.; Adar, E.; Yakirevich, A.; Nativ, R. Dilution tests in a low-permeability fractured aquifer: Matrix diffusion effect. Ground Water 2007, 45, 235–241. [Google Scholar] [CrossRef] [PubMed]
- Piccinini, L.; Fabbri, P.; Pola, M. Point dilution tests to calculate groundwater velocity: An example in a porous aquifer in northeast Italy. Hydrol. Sci. J. 2016, 61, 1512–1523. [Google Scholar] [CrossRef]
- Newcomer, D.R.; Hall, S.H.; Vermeul, V.R. Use of improved hydrologic testing and borehole geophysical logging methods for aquifer characterization. Winter 1996, 16, 67–72. [Google Scholar] [CrossRef]
- Rollinson, J.; Rees-White, T.; Beaven, R.B.; Barker, J.A. A single borehole dilution technique to measure the hydrogeological properties of a saturated landfilled waste. In Proceedings of the Waste 2010, Stratford-upon-Avon, UK, 27–28 September 2010. [Google Scholar]
- Maldaner, C.H.; Quinn, P.M.; Cherry, J.A.; Parker, B.L. Improving estimates of groundwater velocity in a fractured rock borehole using hydraulic and tracer dilution methods. J. Contam. Hydrol. 2018, 214, 75–86. [Google Scholar] [CrossRef]
- Fujinawa, K. Asymptotic solutions to the convection-dispersion equation and Powell’s optimization method for evaluating groundwater velocity and dispersion coefficients from observed data of single dilution tests. J. Hydrol. 1983, 62, 333–353. [Google Scholar] [CrossRef]
- Canul-Macario, C.; González-Herrera, R.; Sánchez-Pinto, I.; Graniel-Castro, E. Contribution to the evaluation of soute transport properties in a karstic aquifer (Yucatan, Mexico). Hydrogeol. J. 2019, 27, 1683–1691. [Google Scholar] [CrossRef]
- Doummar, J.; Margane, A.; Geyer, T.; Sauter, M. Monitoring transient groundwater fluxes using the Finite Volume Point Dilution Method. J. Contam. Hydrol. 2018, 218, 10–18. [Google Scholar]
- Gustafsson, E.; Andersson, P. Groundwater flow conditions in a low-angle fracture zone at Finnsjön, Sweden. J. Hydrol. 1991, 126, 79–111. [Google Scholar] [CrossRef]
- Gutiérrez, M.G.; Guimerà, J.; Yllera de Llano, A.; Hernández-Benitez, A.; Humm, J.; Saltink, M. Tracer test at El Berrocal site. J. Contam. Hydrol. 1997, 26, 179–188. [Google Scholar] [CrossRef]
- Sanford, W.E.; Moore, G.K. Measurement of specific discharge with point-dilution tests in the fractured rocks of Eastern Tennessee. In Proceedings of Extended Abstracts, American Water Resources Association 1994 Annual Spring Symposium in Nashville, Tennessee; A.A. Balkema Publishers: Cape Town, South Africa, 1994; pp. 449–453. [Google Scholar]
- Jardine, P.M.; Sanford, W.E.; Gwo, J.P.; Reedy, O.C.; Hicks, C.S.; Riggs, J.S.; Bailey, W.B. Quantifying diffusive mass transfer in fractured shale bedrock. Water Resour. Res. 1999, 35, 2015–2030. [Google Scholar] [CrossRef]
- Novakowski, K.S.; Lapcevic, P.A.; Voralek, J.; Bickerton, G. Preliminary interpretation of tracer experiments conducted in a discrete rock fracture under conditions of natural flow. Geophys. Res. Lett. 1995, 22, 1417–1420. [Google Scholar] [CrossRef]
- Moore, Y.H.; Stoesell, R.K.; Easley, D.H. Fresh-water/ sea-water relationship within a ground-water flow system, northern coast of the Yucatan Peninsula. Ground Water 1992, 30, 343–350. [Google Scholar] [CrossRef]
- Novakowski, K.; Bickerton, G.; Lapcevic, P.; Voralek, J.; Ross, N. Measurements of groundwater velocity in discrete rock fractures. J. Contam. Hydrol. 2006, 82, 44–60. [Google Scholar] [CrossRef]
- Riemann, K.; Van Tonder, G.; Dzanga, P. Interpretation of single-well tracer tests using fractional-flow dimensions. Part 2: A case study. Hydrogeol. J. 2002, 10, 357–367. [Google Scholar] [CrossRef]
- Hall, S.H. Single well tracer tests in aquifer characterization. Ground Water Monitor. Remed. 1993, 13, 118–124. [Google Scholar] [CrossRef]
- Ronen, D.; Magaritz, M.; Paldor, N.; Bachmat, Y. The behaviour of groundwater in the vicinity of the water table evidenced by specific discharge profiles. Water Resour. Res. 1986, 22, 1217–1224. [Google Scholar] [CrossRef]
- Ronen, D.; Magaritz, M.; Molz, F.J. Comparison between natural and forced gradient tests to determine the vertical distribution of horizontal transport properties of aquifers. Water Resour. Res. 1991, 27, 1309–1314. [Google Scholar] [CrossRef]
- Ronen, D.; Berkowitz, B.; Magaritz, M. Vertical heterogeneity in horizontal components of specific discharge: Case study analysis. Ground Water 1993, 31, 33–40. [Google Scholar] [CrossRef]
- Wurzel, P. Updated radioisotope studies in Zimbabwean ground waters. Ground Water 1983, 21, 597–605. [Google Scholar] [CrossRef]
- Flynn, R.M.; Schnegg, P.A.; Costa, R.; Mallen, G.; Zwahlen, F. Identification of zones of preferential groundwater tracer transport using a mobile downhole fluorometer. Hydrogeol. J. 2005, 13, 366–377. [Google Scholar] [CrossRef][Green Version]
- Hatfield, K.; Annable, M.; Cho, J.; Raom, P.S.C.; Klammler, H. A direct passive method for measuring water and contaminant fluxes in porous media. J. Contam. Hydrol. 2004, 75, 155–181. [Google Scholar] [CrossRef] [PubMed]
- West, L.J.; Odling, N.E. Characterization of a multilayer aquifer using open well dilution tests. Ground Water 2007, 45, 74–84. [Google Scholar] [CrossRef]
- Shafer, J.M.; Brantley, D.T.; Waddell, M.G. Variable-density flow and transport simulation of wellbore brine displacement. Ground Water 2010, 48, 122–130. [Google Scholar] [CrossRef]
- Tsang, C.F.; Hufschmied, P.; Hale, F.V. Determination of fracture inflow parameters with a borehole fluid conductivity logging method. Water Resour. Res. 1990, 26, 561–578. [Google Scholar] [CrossRef]
- Paillet, F.L.; Pedler, W.H. Integrated borehole logging methods for wellhead protection applications. Eng. Geol. 1996, 42, 155–165. [Google Scholar] [CrossRef]
- Maurice, L.; Barker, J.A.; Atkinson, T.C.; Williams, A.T.; Smart, P.L. A tracer methodology for identifying ambient flows in boreholes. Ground Water 2011, 49, 227–238. [Google Scholar] [CrossRef] [PubMed]
- Benavente, J.; Cardenal, J.; Cruz San Julián, J.J. Exploitation effects in the Guadalfeo alluvial aquifer (Escalate Canyon area, Granada, Southeastern Spain). In Proceedings of the XXIII Conference A.I.H “Aquifer Overexploitation” (Puerto de la Cruz), Canary Islands, Spain, April 1991; pp. 499–502. [Google Scholar]
- Duque, C.; Calvache, M.L.; Engesgaard, P. Investigating river-aquifer relations using water temperature in an anthropized environment (Motril-Salobreña aquifer). J. Hydrol. 2010, 381, 121–133. [Google Scholar] [CrossRef]
- Calvache, M.L.; Ibáñez, P.; Duque, C.; López-Chicano, M.; Martín-Rosales, W.; González-Ramón, A.; Rubio, J.C.; Viseras, C. Numerical modelling of the potential effects of a dam on a coastal aquifer in S. Spain. Hydrol. Process. 2009, 23, 1268–1281. [Google Scholar] [CrossRef]
- Duque, C.; Calvache, M.L.; Pedrera, A.; Martín-Rosales, W.; López-Chicano, M. Combined time domain electromagnetic soundings and gravimetry to determine marine intrusion in a detrital coastal aquifer (Southern Spain). J. Hydrol. 2008, 349, 536–547. [Google Scholar] [CrossRef]
- CHSE-IRYDA. Estudio de Viabilidad de la Ampliación de la Zona Regable de Motril-Salobreña Hasta la Cota 300, Unpublished. 1984.
- ITGE. Investigación Hidrogeológica para Apoyo a la Gestión Hidrológica en la Cuenca del río Guadalfeo (Cuenca Sur de España, Granada), 1988.
- García-Aróstegui, J.L.; Heredia, J.; Murillo, J.M.; Rubio Campos, J.C.; González-Ramón, A.; López-Geta, J.A. Contribución desde la modelización del flujo subterráneo al conocimiento del acuífero del río Verde (Granada. Proceedings of V Simposio sobre el agua en Andalucía, IGME, Almería 2001.
- Gaspar, E. Modern Trends in Tracer Hydrology; CRC Press: Boca Raton, FL, USA, 1987; Volume II. [Google Scholar]
- Cook, P.G.; Dighton, J.C. Inferring ground water flow in fractured rock from dissolved radon. Ground Water 1999, 37, 606–610. [Google Scholar] [CrossRef]
- Taniguchi, M.; Sharma, M.L. Determination of groundwater recharge using the change in soil temperature. J. Hydrol. 1993, 148, 219–229. [Google Scholar] [CrossRef]
- Calvache, M.L.; Duque, C.; Gomez-Fontalva, J.M.; Crespo, F. Processes affecting groundwater temperature patterns in a coastal aquifer. Int. J. Environ. Technol. 2011, 8, 223–236. [Google Scholar] [CrossRef]
- Freeze, R.A.; Cherry, J.A. Groundwater; Prentice Hall: Hoboken, NJ, USA, 1979. [Google Scholar]
- Taniguchi, M.; Sharma, M.L. Solute and heat transport experiments for estimating recharge rate. J. Hydrol. 1990, 119, 57–69. [Google Scholar] [CrossRef]

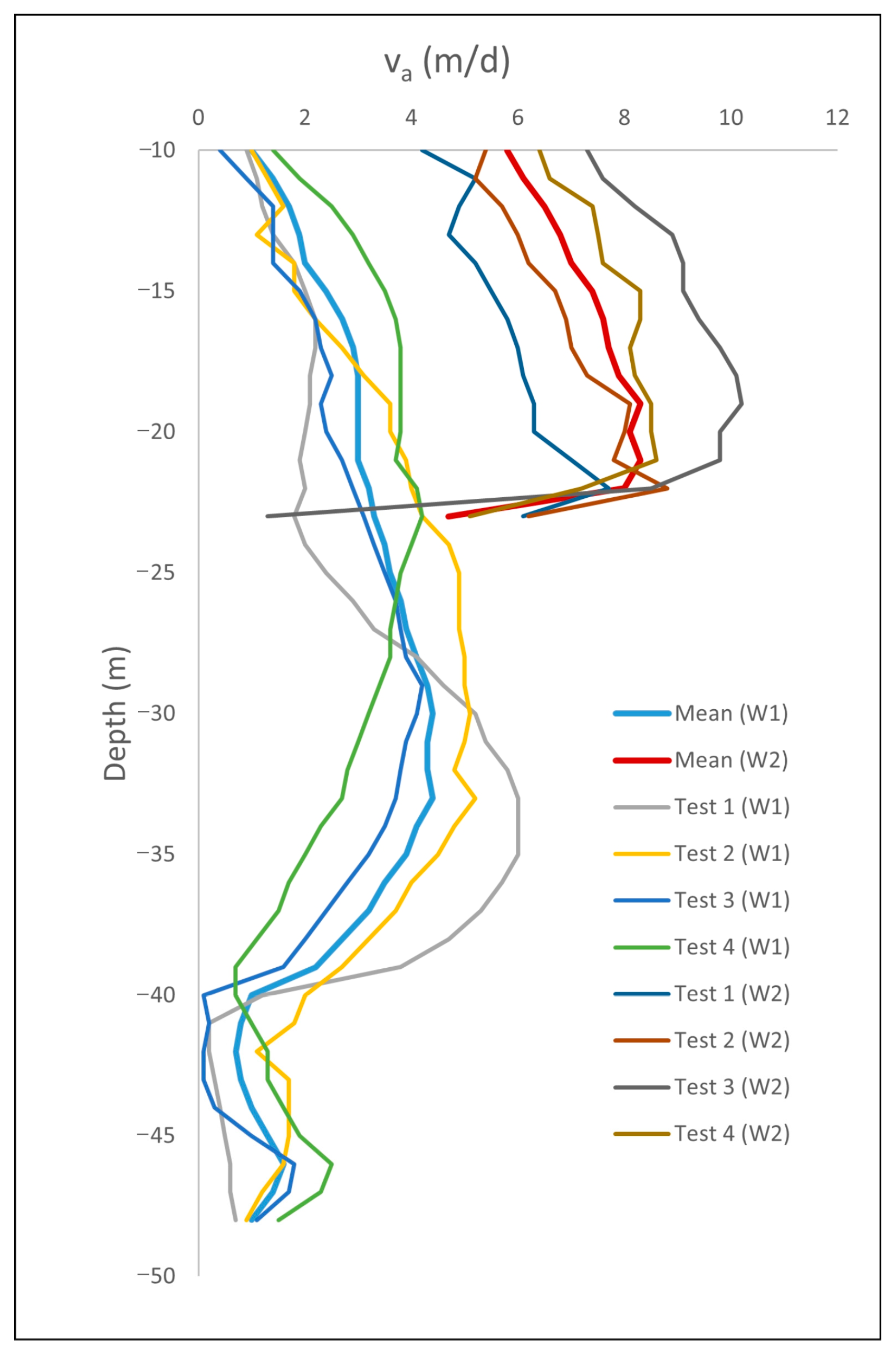
| W1 | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Test 1 | Test 2 | Test 3 | Test 4 | Mean | ||||||
| α = 2 i = 0.0099 | α = 2 i = 0.0103 | α = 2 i = 0.0108 | α = 2 i = 0.0114 | α = 2 i = 0.0106 | ||||||
| Depth (m) | (m/d) | K (m/d) | (m/d) | K (m/d) | (m/d) | K (m/d) | (m/d) | K (m/d) | (m/d) | K (m/d) |
| −10 | 0.9 | 48 | 1.0 | 47 | 0.4 | 18 | 1.4 | 61 | 1.0 | 47 |
| −11 | 1.1 | 58 | 1.3 | 64 | 0.9 | 41 | 1.9 | 86 | 1.4 | 66 |
| −12 | 1.2 | 63 | 1.6 | 78 | 1.4 | 67 | 2.5 | 110 | 1.7 | 80 |
| −13 | 1.4 | 73 | 1.1 | 81 | 1.4 | 67 | 2.9 | 128 | 1.9 | 89 |
| −14 | 1.8 | 90 | 1.8 | 86 | 1.4 | 66 | 3.2 | 140 | 2.0 | 94 |
| −15 | 2.0 | 102 | 1.8 | 86 | 1.9 | 88 | 3.5 | 155 | 2.4 | 113 |
| −16 | 2.2 | 110 | 2.2 | 107 | 2.2 | 101 | 3.7 | 166 | 2.7 | 127 |
| −17 | 2.2 | 111 | 2.7 | 131 | 2.3 | 106 | 3.8 | 169 | 2.9 | 137 |
| −18 | 2.1 | 10 | 3.1 | 155 | 2.5 | 118 | 3.8 | 169 | 3.0 | 141 |
| −19 | 2.1 | 104 | 3.6 | 174 | 2.3 | 109 | 3.8 | 169 | 3.0 | 141 |
| −20 | 2.0 | 100 | 3.6 | 173 | 2.4 | 111 | 3.8 | 167 | 3.0 | 141 |
| −21 | 1.9 | 98 | 3.9 | 189 | 2.7 | 126 | 3.7 | 165 | 3.0 | 141 |
| −22 | 2.0 | 101 | 4.0 | 192 | 2.9 | 136 | 4.1 | 182 | 3.2 | 151 |
| −23 | 1.8 | 92 | 4.2 | 206 | 3.1 | 146 | 4.2 | 186 | 3.3 | 156 |
| −24 | 2.0 | 102 | 4.7 | 228 | 3.3 | 151 | 4.0 | 175 | 3.5 | 165 |
| −25 | 2.4 | 123 | 4.9 | 237 | 3.5 | 162 | 3.8 | 167 | 3.6 | 170 |
| −26 | 2.9 | 149 | 4.9 | 238 | 3.7 | 170 | 3.7 | 163 | 3.8 | 179 |
| −27 | 3.3 | 169 | 4.9 | 243 | 3.8 | 176 | 3.6 | 158 | 3.9 | 184 |
| −28 | 4.1 | 206 | 5.0 | 244 | 3.9 | 182 | 3.6 | 159 | 4.1 | 193 |
| −29 | 4.6 | 234 | 5.0 | 24 | 4.2 | 193 | 3.4 | 151 | 4.3 | 203 |
| 30 | 5.2 | 261 | 5.1 | 246 | 4.1 | 189 | 3.2 | 141 | 4.4 | 207 |
| −31 | 5.4 | 275 | 5.0 | 243 | 3.9 | 183 | 3.0 | 132 | 4.3 | 203 |
| −32 | 5.8 | 292 | 4.8 | 232 | 3.8 | 177 | 2.8 | 124 | 4.3 | 203 |
| −33 | 6.0 | 302 | 5.2 | 252 | 3.7 | 173 | 2.7 | 118 | 4.4 | 207 |
| −34 | 6.0 | 302 | 4.8 | 231 | 3.5 | 163 | 2.3 | 104 | 4.1 | 193 |
| −35 | 6.0 | 301 | 4.5 | 218 | 3.2 | 147 | 2.0 | 90 | 3.9 | 184 |
| −36 | 5.7 | 287 | 4.0 | 196 | 2.8 | 128 | 1.7 | 77 | 3.5 | 165 |
| −37 | 5.3 | 270 | 3.7 | 178 | 2.4 | 111 | 1.5 | 66 | 3.2 | 151 |
| −38 | 4.7 | 238 | 3.2 | 154 | 2.0 | 92 | 1.1 | 51 | 2.7 | 127 |
| −39 | 3.8 | 193 | 2.7 | 131 | 1.6 | 73 | 0.7 | 30 | 2.2 | 104 |
| −40 | 1.2 | 60 | 2.0 | 100 | 0.1 | 3 | 0.7 | 33 | 1.0 | 47 |
| −41 | 0.2 | 12 | 1.8 | 87 | 0.2 | 10 | 1.0 | 46 | 0.8 | 38 |
| −42 | 0.2 | 12 | 1.1 | 55 | 0.1 | 7 | 1.3 | 58 | 0.7 | 33 |
| −43 | 0.3 | 15 | 1.7 | 83 | 0.1 | 3 | 1.3 | 60 | 0.8 | 38 |
| −44 | 0.4 | 20 | 1.7 | 83 | 0.3 | 15 | 1.6 | 70 | 1.0 | 47 |
| −45 | 0.5 | 26 | 1.7 | 85 | 1.0 | 48 | 1.9 | 86 | 1.3 | 61 |
| −46 | 0.6 | 28 | 1.6 | 77 | 1.8 | 82 | 2.5 | 112 | 1.6 | 75 |
| −47 | 0.6 | 32 | 1.2 | 56 | 1.7 | 80 | 2.3 | 103 | 1.4 | 66 |
| −48 | 0.7 | 34 | 0.9 | 46 | 1.1 | 50 | 1.5 | 68 | 1.0 | 47 |
| Mean | 2.6 | 133 | 3.1 | 153 | 2.2 | 104 | 2.6 | 118 | 2.6 | 126 |
| W2 | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Test 1 | Test 2 | Test 3 | Test 4 | Mean | ||||||
| α = 2 i = 0.0099 | α = 2 i = 0.0103 | α = 2 i = 0.0108 | α = 2 i = 0.0114 | α = 2 i = 0.0106 | ||||||
| Depth (m) | (m/d) | K (m/d) | (m/d) | K (m/d) | (m/d) | K (m/d) | (m/d) | K (m/d) | (m/d) | K (m/d) |
| −10 | 4.2 | 213 | 5.4 | 262 | 7.3 | 339 | 6.4 | 285 | 5.8 | 274 |
| −11 | 5.2 | 262 | 5.2 | 254 | 7.6 | 352 | 6.6 | 293 | 6.1 | 288 |
| −12 | 4.9 | 246 | 5.7 | 275 | 8.2 | 379 | 7.4 | 327 | 6.5 | 307 |
| −13 | 4.7 | 239 | 6.0 | 292 | 8.9 | 413 | 7.5 | 334 | 6.8 | 320 |
| −14 | 5.2 | 261 | 6.2 | 303 | 9.1 | 421 | 7.6 | 337 | 7.0 | 330 |
| −15 | 5.5 | 278 | 6.7 | 325 | 9.1 | 421 | 8.3 | 365 | 7.4 | 349 |
| −16 | 5.8 | 295 | 6.9 | 338 | 9.4 | 436 | 8.3 | 367 | 7.6 | 358 |
| −17 | 6.0 | 302 | 7.0 | 341 | 9.8 | 452 | 8.1 | 358 | 7.7 | 363 |
| −18 | 6.1 | 307 | 7.3 | 356 | 10.1 | 466 | 8.2 | 362 | 7.9 | 372 |
| −19 | 6.3 | 317 | 8.1 | 393 | 10.2 | 473 | 8.5 | 377 | 8.3 | 391 |
| −20 | 6.3 | 337 | 8.0 | 388 | 9.8 | 453 | 8.5 | 376 | 8.1 | 382 |
| −21 | 7.0 | 356 | 7.8 | 381 | 9.8 | 456 | 8.6 | 381 | 8.3 | 391 |
| −22 | 7.7 | 387 | 8.8 | 427 | 8.5 | 394 | 7.2 | 318 | 8.0 | 377 |
| −23 | 6.1 | 310 | 6.2 | 303 | 1.3 | 59 | 5.1 | 225 | 4.7 | 222 |
| Mean | 5.4 | 294 | 6.3 | 331 | 8.0 | 394 | 7.1 | 336 | 6.7 | 337 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Calvache, M.L.; López-Chicano, M.; Blanco-Coronas, A.M.; de la Torre, B.; Duque, C. Applicability of Single-Borehole Dilution Tests in Aquifers with Vertical Flow. Water 2024, 16, 1305. https://doi.org/10.3390/w16091305
Calvache ML, López-Chicano M, Blanco-Coronas AM, de la Torre B, Duque C. Applicability of Single-Borehole Dilution Tests in Aquifers with Vertical Flow. Water. 2024; 16(9):1305. https://doi.org/10.3390/w16091305
Chicago/Turabian StyleCalvache, Maria L., Manuel López-Chicano, Angela M. Blanco-Coronas, Beatriz de la Torre, and Carlos Duque. 2024. "Applicability of Single-Borehole Dilution Tests in Aquifers with Vertical Flow" Water 16, no. 9: 1305. https://doi.org/10.3390/w16091305
APA StyleCalvache, M. L., López-Chicano, M., Blanco-Coronas, A. M., de la Torre, B., & Duque, C. (2024). Applicability of Single-Borehole Dilution Tests in Aquifers with Vertical Flow. Water, 16(9), 1305. https://doi.org/10.3390/w16091305







