Slope Deformation Mechanisms and Stability Assessment under Varied Conditions in an Iron Mine Waste Dump
Abstract
1. Introduction
2. Experiments and Methods
2.1. Sampling Preparation and Pretesting
2.2. Experimental Procedure
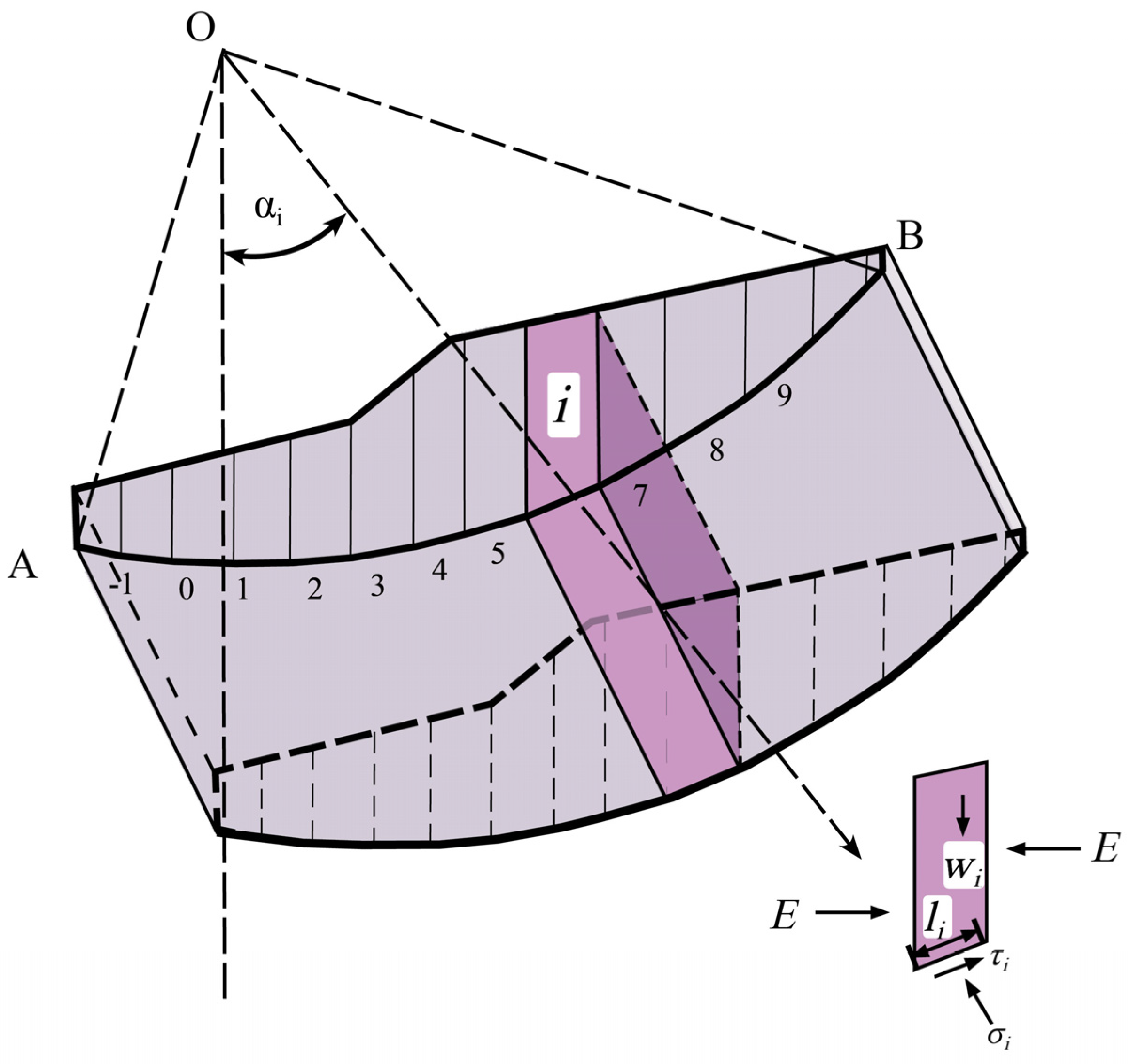
2.3. Theoretical Foundation
2.4. Software Selection
3. Results and Discussion
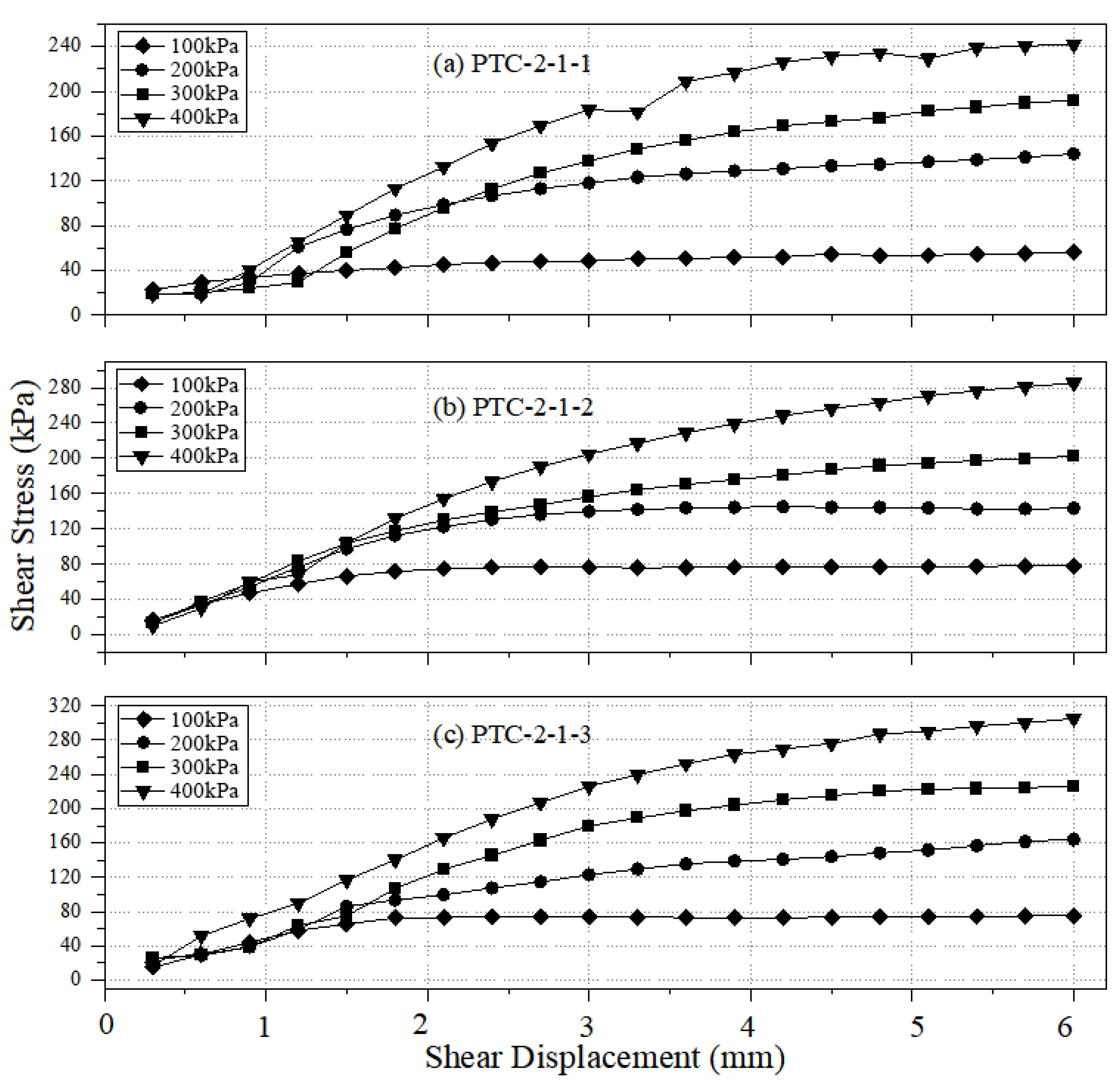
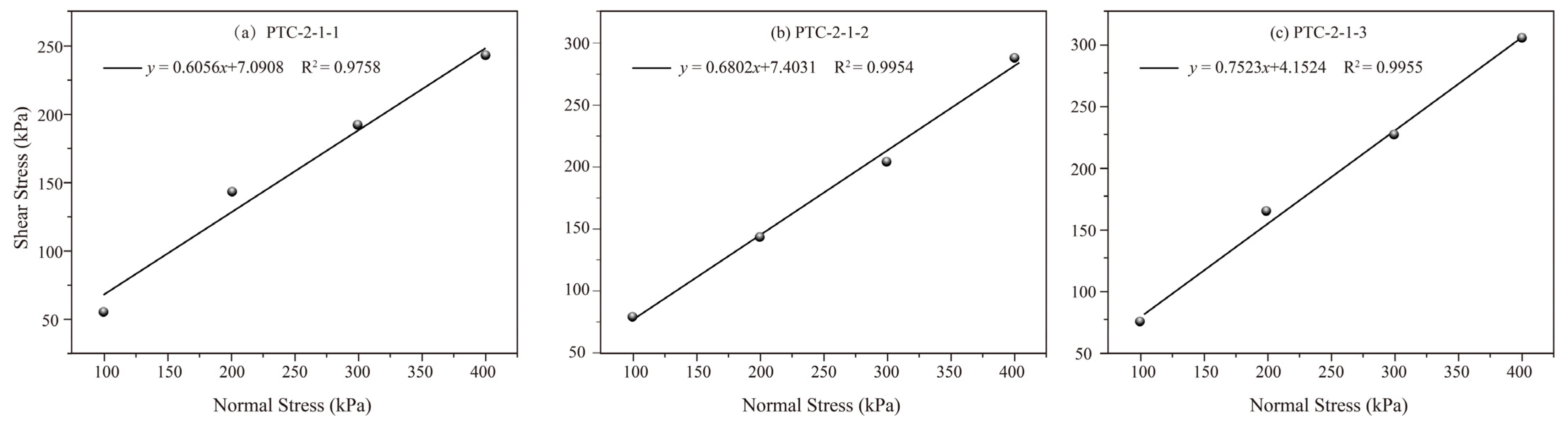
3.1. Direct Shear Test Results
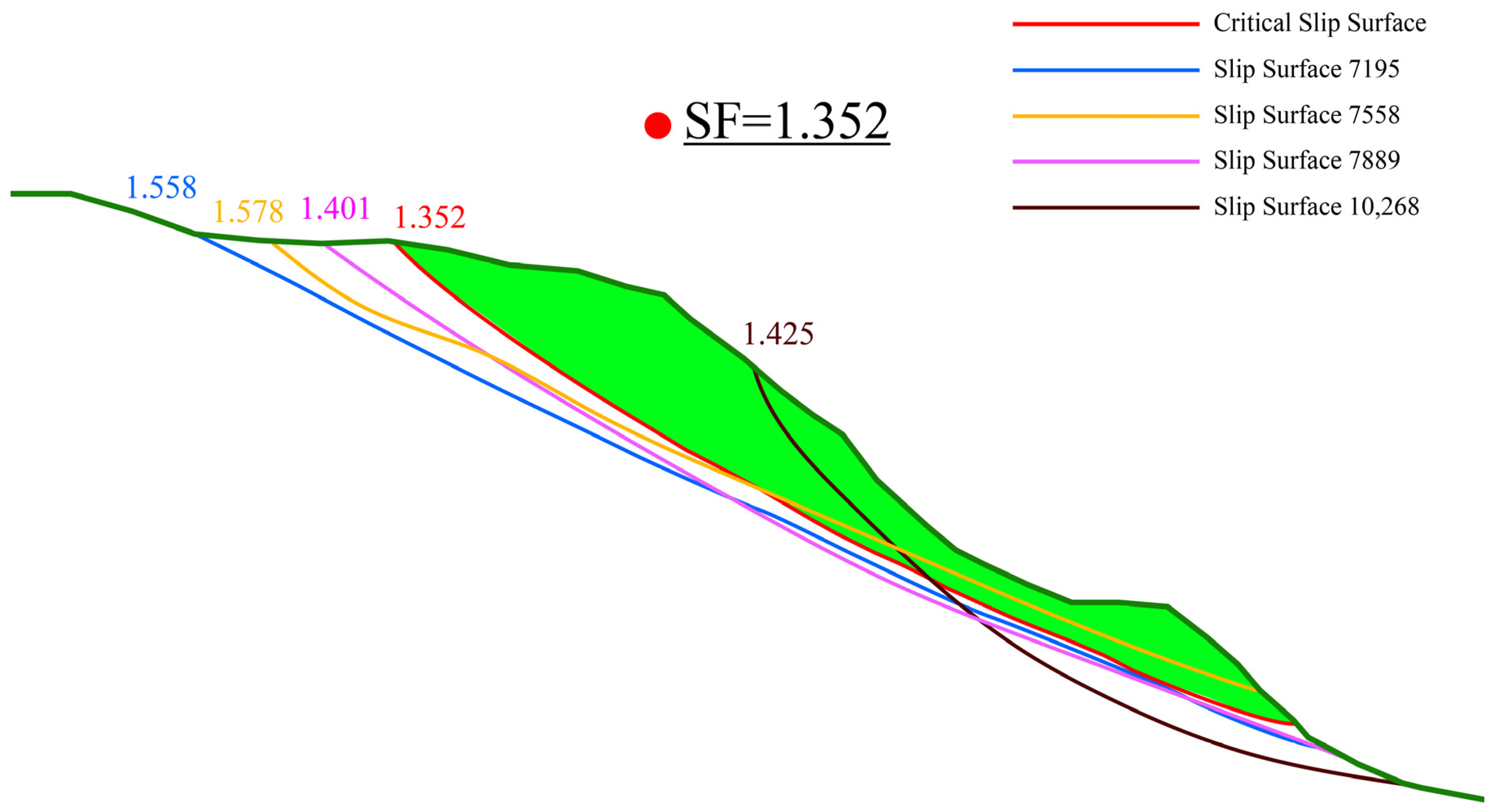
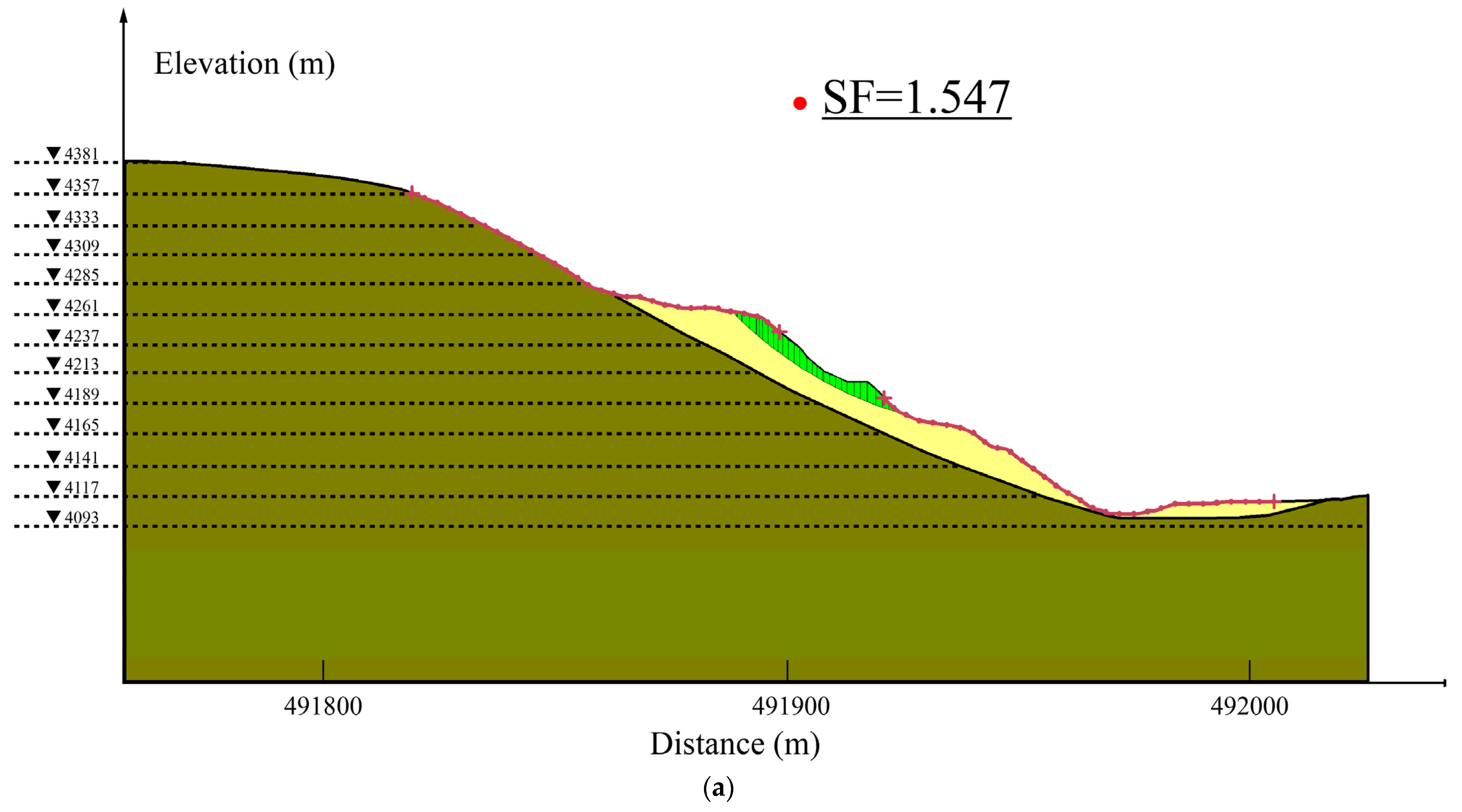
3.2. Evaluation of the Waste Dump Slope Stability
- The discharge material (in our case study, Ziluoyi Iron Mine, Waste Dump#2) is both homogenous and isotropic.
- No sizeable cracks exist in the body of the discharge material.
- In the mechanical modeling of the problem, the quartz schist medium underneath the discharge material is assumed to be rigid.
- The strength of the material and the mechanical behavior of the discharge material are mainly modeled based on the Mohr–Coulomb criterion (see Equation (4)).
- The failure surface fully occurs in the discharge material, which has a lower strength than the underlying quartz schist.
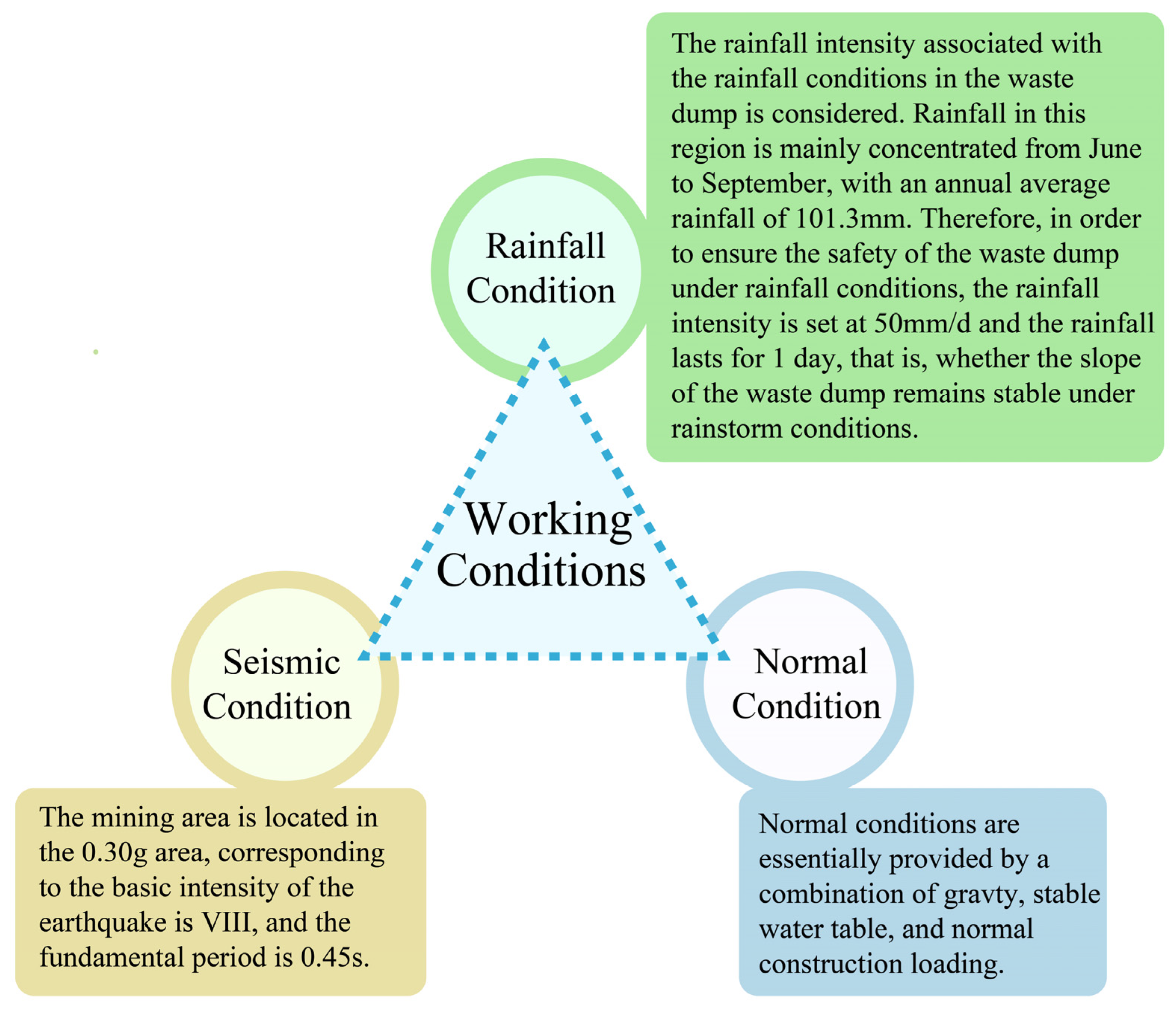
- Three distinct conditions (i.e., normal, rainfall, and seismic conditions) have been considered in the stability analysis of the understudied slope and their potential interactions have been not taken into account.
3.3. Slope Problems and Control Measures
3.3.1. Disaster Prevention and Control Measures of Waste Dump#2
- Timely mapping of the zone of sliding deformation cracks in the waste dump to master the extent of the deformation area is required. The monitoring points are set in the deformation zone to more effectively capture the deformation state of the slope. If sliding deformation appears to increase, the production operations in the deformation zone must be stopped immediately and personnel and equipment must be evacuated to a safe place.
- The top of the waste dump and safety platform is set with a 3% inward reverse slope to prevent the sloping roof from collapsing during the discharge process, which would cause personnel and vehicles to roll off. The design of the waste dump unloading platform is set up about 10 m away from the edge of the safety mine truck. The mining truck is made of piles of waste rock, and the height of the staff is 1/2 the height of the wheel platform of the vehicle.
- The cracks caused by subsidence and deformation of the waste dump should be filled and compacted in time to prevent the penetration of a large amount of rainwater and the stability of the slope. Low-lying areas formed by compaction or water should be filled in time to prevent rainwater accumulation and infiltration to form landslides.
3.3.2. Providing Instructions for Slope Displacement Monitoring of Waste Dump#2
4. Conclusions
- The direct shear tests on soil samples from Waste Dump#2 provided valuable visions into its geotechnical properties. The cohesion range was obtained in an interval of 4.15–7.40 kPa, with an internal friction angle between 31.20° and 36.95°. The average of these values, resulting in a cohesion of 6.215 kPa and an internal friction angle of 34.12°, were effectively employed in the complementary analyses.
- The slope stability assessment was carried out through numerical simulations involving various environmental conditions, including normal, rainfall, and seismic scenarios, using appropriate software and formulations in the existing literature. The results were reassuring because the actual slope stability factor of Waste Dump#2 acted upon by normal conditions was measured to be 1.547. In the presence of rainfall, this factor slightly decreased to 1.276, which is still within the safety standard. Even under seismic conditions, the slope remained stable with a safety factor of 1.352, indicating its resilience against external forces.
- Understanding the progressive failure behavior of waste dump slopes in open-pit coal mines and implementing appropriate control measures is also critical to ensure the safety of personnel, equipment, and the environment. The insights and recommendations presented in this study significantly help to improve risk management and prevent possible disasters in open-pit mining operations.
Author Contributions
Funding
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. A Discussion of the Failure Process

- Rainfall, excavation, embankment, or a combination of these factors that affect the k0 (coefficient of earth pressure at rest) of the soil [39] are incorporated into the stress concentration in the toe of the slope Zone 1, and the shear strength in the region of stress concentration alters from peak strength to residual strength. The occurrence of local deformation yields a change in the stress state, causing stress adjustment in the area adjacent to the geotechnical body, stress release, stress transfer, and stress redistribution in a certain range.
- Due to the discrepancies in the disturbances and differences in the structure and geological conditions of the slopes themselves, a specific direction in the change of the stresses in Zone 1 is configured. This fact leads to a difference in the degree and speed of the advance of the damage forward or backward, affecting the triggering stress concentration in the adjacent Zone 2. It should be also noticed that the relationship between the material state and slope stability is crucial, and it is also primarily governed by the fundamental factor k0. However, it is important to note that the stress state in the slope could also be affected by the slope geometry and the actions it undergoes, leading to a heterogeneity of stress states.
- Under the influence of the change of the stress state in Zone 1, Zone 2 also exceeds the shear strength and suffers damage, turns into residual strength, and again undergoes stress release, transfer, and redistribution, which in turn causes Zone 3 to reach maximum strength.
- Repetition of this process of stress transfer, triggering stress concentration, and the development of the plasticity surfaces leads to the gradual development of the slope failure zone, with the extension of the rupture surface, transfer, and progressive penetration along the potential sliding surface until it extends to Zone 4.
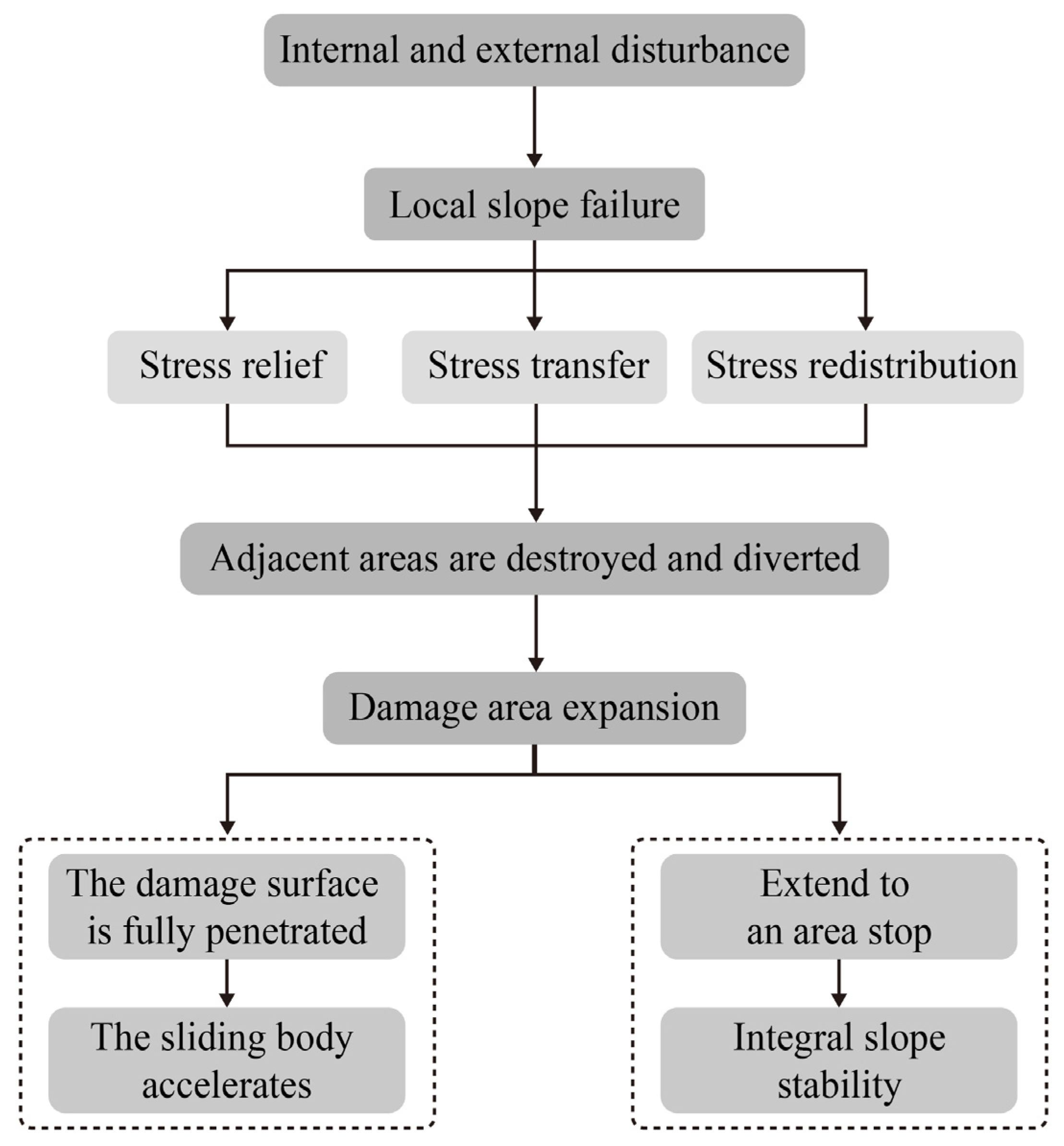
Appendix B. Temporal and Spatial Evolution of the Progressive Slope Failure
Appendix B.1. Time Evolution of the Progressive Slope Failure
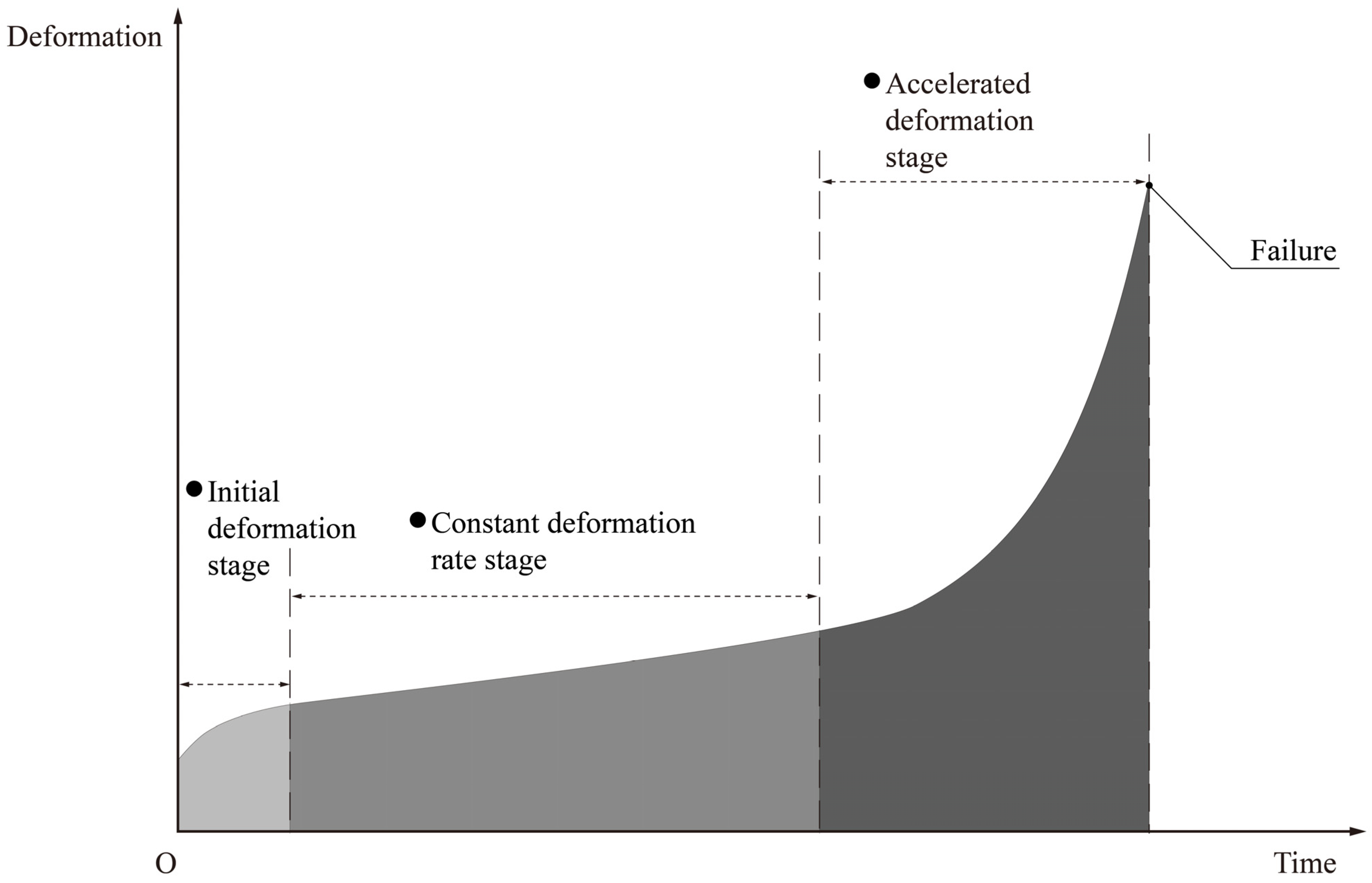
- Initial deformation stage
- 2.
- Constant deformation rate stage
- 3.
- Accelerated deformation stage
Appendix B.2. Spatial Evolution of the Progressive Slope Failure
- Integral compaction stage
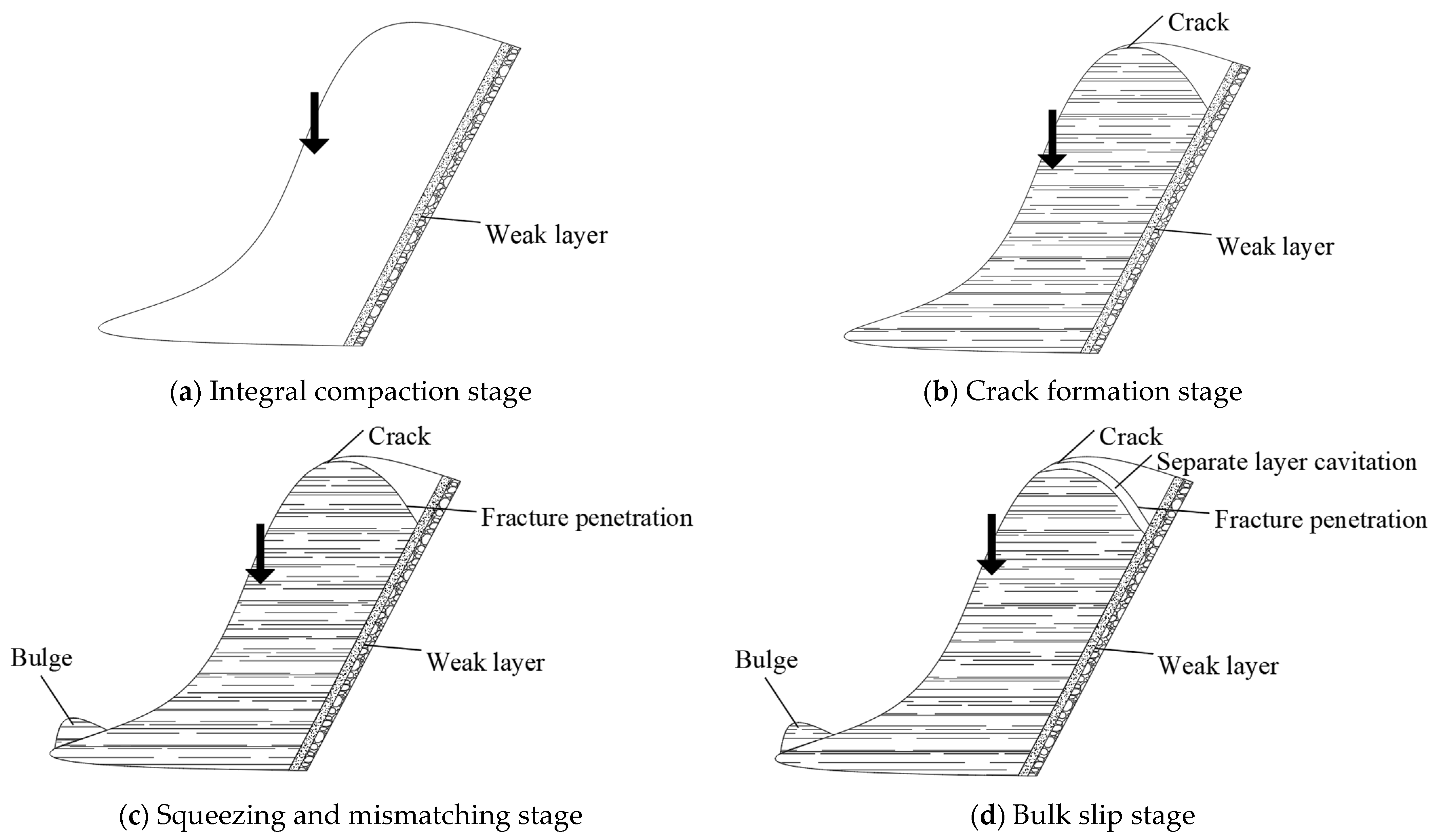
- 2.
- Crack formation stage
- 3.
- Squeezing and mismatching stage
- 4.
- Bulk slip stage
References
- Tian, Y.; Jiskani, I.M.; Lu, X.A.; Zhou, W.; Liu, F.M.; Zhang, C.W.; Cai, Q.X. Control mechanism of end-slope deformation in open pit mine based on discrete-continuous coupling method. Comput. Part. Mech. 2023, 10, 951–963. [Google Scholar] [CrossRef]
- Xu, C.Y.; Liu, Q.S.; Tang, X.H.; Sun, L.; Deng, P.H.; Liu, H. Dynamic stability analysis of jointed rock slopes using the combined finite-discrete element method (FDEM). Comput. Geotech. 2023, 160, 105556. [Google Scholar] [CrossRef]
- Luan, B.Y.; Zhou, W.; Jiskani, I.M.; Wang, Z.M. An improved machine learning approach for optimizing dust concentration estimation in open-pit mines. Int. J. Environ. Res. Public Health 2023, 20, 1353. [Google Scholar] [CrossRef]
- Wang, G.J.; Zhao, B.; Wu, B.S.; Zhang, C.; Liu, W.L. Intelligent prediction of slope stability based on visual exploratory data analysis of 77 in situ cases. Int. J. Min. Sci. Technol. 2023, 33, 47–59. [Google Scholar] [CrossRef]
- Sari, M. Evaluation of rock slopes susceptible to circular failures using logistic and multiple regression models. Arab. J. Geosci. 2020, 13, 61. [Google Scholar] [CrossRef]
- Tagarelli, V.; Cotecchia, F. Deep movements in clayey slopes relating to climate: Modeling for early warning system design. In Geotechnical Research for Land Protection and Development: Proceedings of CNRIG 2019; Springer: Berlin/Heidelberg, Germany, 2020; Volume 7, pp. 205–214. [Google Scholar]
- Ding, X.H.; Ao, Z.C.; Li, X.S.; Xiao, S.S.; Wu, M.; Xing, B.K.; Ge, R.H.; Zhang, D.H. The mechanism of plugging open-pit mine cannon holes and the modification of plugging materials. Sustainability 2023, 15, 4257. [Google Scholar] [CrossRef]
- Wang, Z.M.; Zhou, W.; Jiskani, I.M.; Ding, X.H.; Luo, H.T. Dust pollution in cold region surface mines and its prevention and control. Environ. Pollut. 2022, 292, 118293. [Google Scholar] [CrossRef] [PubMed]
- Sun, L.; Grasselli, G.; Liu, Q.S.; Tang, X.H.; Abdelaziz, A. The role of discontinuities in rock slope stability: Insights from a combined finite-discrete element simulation. Comput. Geotech. 2022, 147, 104788. [Google Scholar] [CrossRef]
- Sari, M. Stability analysis of cut slopes using empirical, kinematical, numerical, and limit equilibrium methods: Case of old Jeddah–Mecca Road (Saudi Arabia). Environ. Earth Sci. 2019, 78, 621. [Google Scholar] [CrossRef]
- Yuan, W.B.; Ye, C.M.; Yao, J.; Wang, L.D. Stability analysis of mountain slope based on finite element and discrete element. Appl. Mech. Mater. 2012, 170–173, 1087–1090. [Google Scholar] [CrossRef]
- Rui, P. Study on Stability Analysis Theory of Complicated Soil Slope and Computer Realization. In Proceedings of the 1st International Conference on Sustainable Construction and Risk Management, Chongqing, China, 12–14 June 2010; pp. 1390–1394. [Google Scholar]
- Yu, A.; Liu, J.; Yang, D.D.; Cao, H.F.; Yang, X.P. A road slope stability analysis. Adv. Mater. Res. 2012, 594–597, 642–646. [Google Scholar] [CrossRef]
- Sun, S.G.; Miao, Z.Z.; Dong, Y.F.; He, Y.Y. The slope stability evaluation of open-pit mine based on GIS. In Proceedings of the International Conference on Architectural, Civil and Hydraulics Engineering (ICACHE), Guangzhou, China, 28–29 November 2015; pp. 403–408. [Google Scholar]
- Yin, Y.J.; Xu, G.H.; Qian, Y.M. Analysis about Stability of a Slope. In Proceedings of the 4th International Conference on Civil Engineering, Architecture and Building Materials (CEABM), Haikou, China, 24–25 May 2014; pp. 152–155. [Google Scholar]
- Xie, X.F.; Liu, Y.L.; Han, J.Y.; Xing, L. 3D Finite element numerical analysis for stability calculation of high steep rock slope. Appl. Mech. Mater. 2014, 580–583, 532–538. [Google Scholar] [CrossRef]
- Guo, J.; Gan, D.Q.; Zhang, Y.; Zhang, W.H. Application of GM (1, N) model on slope stability analysis in open pit mine. Appl. Mech. Mater. 2011, 84–85, 729–732. [Google Scholar] [CrossRef]
- Guanhua, Q. Stability analysis of nonhomogeneous and anisotropic stepped slopes under the influence of earthquakes. Heliyon 2023, 9, e15057. [Google Scholar] [CrossRef]
- Li, Y.Q.; Goh, A.T.C.; Zhang, R.H.; Zhang, W.G. Stability charts for undrained clay slopes considering soil anisotropic characteristics. Bull. Eng. Geol. Environ. 2023, 82, 52. [Google Scholar] [CrossRef]
- Huang, A.P.; Zhu, Y.P.; Wang, L.; Ye, S.H.; Fang, G.W. Three-dimensional stability of unsaturated soil slopes reinforced by frame beam anchor plates. Int. J. Geomech. 2023, 23, 04023068. [Google Scholar] [CrossRef]
- Shu, S.; Ge, B.; Wu, Y.X.; Zhang, F. Probabilistic assessment on 3D stability and failure mechanism of undrained slopes based on the kinematic approach of limit analysis. Int. J. Geomech. 2023, 23, 06022037. [Google Scholar] [CrossRef]
- Li, B.; Hu, Y.R.; Zhang, W.H.; Chen, J.; Zhou, B. Safety distance analysis about electricity transmission tower set on the loess high slope. In Proceedings of the 2nd Global Conference on Civil, Structural and Environmental Engineering (GCCSEE 2013), Shenzhen, China, 28–29 September 2013; pp. 710–717. [Google Scholar]
- Ma, Z.Y.; Liao, H.J.; Yu, M.H. Slope stability analysis using unified strength theory. In Proceedings of the SREE Workshop on Applied Mechanics and Civil Engineering (AMCE), Macau, China, 17–18 December 2011; pp. 59–64. [Google Scholar]
- Zhang, Z.P.; Chang, C.Y.; Zhao, Z.Y. Influence of the slope shape on seismic stability of a slope. Adv. Civ. Eng. 2020, 2020, 1–8. [Google Scholar] [CrossRef]
- Gasmo, J.M.; Rahardjo, H.; Leong, E.C. Infiltration effects on stability of a residual soil slope. Comput. Geotech. 2000, 26, 145–165. [Google Scholar] [CrossRef]
- Wang, S.; Xie, X.Y.; Mei, G.D.; Lu, X.Q.; Li, K.; Wang, Y.L.; Publishing, I.O.P. Three-dimensional stability analysis of considering seepage effect on complex loose slope. In Proceedings of the 6th International Conference on Energy Materials and Environment Engineering (ICEMEE), Tianjin, China, 24–26 April 2020. [Google Scholar]
- Liu, X.R.; Liu, Y.Q.; Lu, Y.M.; Li, X.W.; Li, P. Numerical analysis of evaluation methods and influencing factors for dynamic stability of bedding rock slope. J. Vibroeng. 2017, 19, 1937–1961. [Google Scholar] [CrossRef]
- Jiang, P.; Zhang, M.S. Elastic parameters adjusted in soil slope stability and displacement analysis. In Proceedings of the International Conference on Sensors, Measurement and Intelligent Materials (ICSMIM 2012), Guilin, China, 26–27 December 2012; pp. 2889–2892. [Google Scholar]
- Xu, J.S.; Yang, X.L. Seismic and static stability analysis for 3D reinforced slope in nonhomogeneous and anisotropic soils. Int. J. Geomech. 2018, 18, 04018065. [Google Scholar] [CrossRef]
- Kang, K.; Fomenko, I.K.; Wang, J.; Nikolskaya, O.V. Probabilistic assessment of rock slope stability in open pit mine chaarat using the generalized hoek-brown criterion. J. Min. Sci. 2020, 56, 732–740. [Google Scholar] [CrossRef]
- Zhao, E.P.; Jia, X.B. Research on the slope stability under the condition of water level fluctuation. In Proceedings of the 2nd International Conference on Civil Engineering and Transportation (ICCET 2012), Guilin, China, 27–28 October 2012; pp. 350–353. [Google Scholar]
- Wang, W.T.; Song, T.; Liu, Z.R. Stability analysis of high filling slope affected by precipitation intensity. In Proceedings of the 2nd International Conference on Civil Engineering, Architecture and Building Materials (CEABM 2012), Yantai, China, 25–27 May 2012; pp. 1174–1178. [Google Scholar]
- Yang, P. Iop: Analysis of the influence of initial gravity field on the stability analysis of soil slope. In Proceedings of the International Conference on Civil, Architecture and Disaster Prevention, Hefei, China, 19 October–4 November 2018. [Google Scholar]
- Han, C.Y.; Chen, J.J.; Xia, X.H.; Wang, J.H. Three-dimensional stability analysis of anisotropic and non-homogeneous slopes using limit analysis. J. Cent. South Univ. 2014, 21, 1142–1147. [Google Scholar] [CrossRef]
- Xiao, Y.J.; Mao, X.S.; Li, J. The Slope shape of loose accumulation body effect analysis of subgrade slope stability. In Proceedings of the 5th International Conference on Frontiers of Manufacturing Science and Measuring Technology (FMSMT), Taiyuan, China, 24–25 June 2017; pp. 718–721. [Google Scholar]
- Sun, L.; Tang, X.H.; Abdelaziz, A.; Liu, Q.S.; Grasselli, G. Stability analysis of reservoir slopes under fluctuating water levels using the combined finite-discrete element method. Acta Geotech. 2023, 18, 5403–5426. [Google Scholar] [CrossRef]
- Rajabian, A.; Shukla, S.K. Stability analysis of anchor-reinforced soil slopes with Taylor’s stability chart. Int. J. Geomech. 2023, 23, 04022278. [Google Scholar] [CrossRef]
- Zou, J.Q.; Chen, H.; Jiang, Y.; Zhang, W.; Liu, A.H. An effective method for real-time estimation of slope stability with numerical back analysis based on particle swarm optimization. Appl. Rheol. 2023, 33, 20220143. [Google Scholar] [CrossRef]
- Tagarelli, V.; Cotecchia, F. The effects of slope initialization on the numerical model predictions of the slope-vegetation-atmosphere interaction. Geosciences 2020, 10, 85. [Google Scholar] [CrossRef]
- Wang, Z.M.; Zhou, W.; Jiskani, I.M.; Luo, H.T.; Ao, Z.C.; Mvula, E.M. Annual dust pollution characteristics and its prevention and control for environmental protection in surface mines. Sci. Total Environ. 2022, 825, 153949. [Google Scholar] [CrossRef] [PubMed]
- Shen, Z.Y.; Ao, Z.C.; Wang, Z.M.; Yang, Y.Q. Study on crust-shaped dust suppressant in non-disturbance area of open-pit coal mine-A Case Study. Int. J. Environ. Res. Public Health 2023, 20, 934. [Google Scholar] [CrossRef] [PubMed]
- GB50421-2018; Standard for Waste Dump Design of Nonferrous Metal Mines. China National Standard: Beijing, China, 2018.
- Das, B.M.; Sobhan, K. Principles of Geotechnical Engineering, 8th ed.; Cengage Learning Publisher: Stamford, CT, USA, 2013. [Google Scholar]
- Huang, W. Stability of Unsaturated Soil Slopes under Rainfall and Seismic Loading. Ph.D. Thesis, Nanyang Technological University, Singapore, 2018. [Google Scholar]
- GB18306-2001; China Earthquake Administration: Seismic Ground Motion Parameter Zonation Map of China. China Standards Press: Beijing, China, 2001.
- Kramer, S.L. Geotechnical Earthquake Engineering; Prentice-Hall, Inc.: Upper Saddle River, NJ, USA, 1996; pp. 434–437. [Google Scholar]
- Hynes-Griffin, M.E.; Franklin, A.G. Rationalizing the Seismic Coefficient Method; Miscellaneous Paper GL-84-13; U.S. Army Corps of Engineers Waterways Experiment Station: Vicksburg, MS, USA, 1984; 21p. [Google Scholar]
- Corps of Engineers. Slope Stability Manual EM-1110-2-1902; Department of the Army, Office of the Chief of Engineers: Washington, DC, USA, 1982. [Google Scholar]
- Marcuson, W.F.; Franklin, A.G. Seismic Design, Analysis, and Remedial Measures to Improve the Stability of Existing Earth Dams—Corps of Engineers Approach. In Seismic Design of Embankments and Caverns; Howard, T.R., Ed.; ASCE: New York, NY, USA, 1983. [Google Scholar]
- Seed, H.B. Considerations in the earthquake-resistant design of earth and rockfill dams. Géotechnique 1979, 29, 215–263. [Google Scholar] [CrossRef]
- Zhou, C.; Shi, Q.; He, D.; Tu, B.; Li, H.; Plaza, A. Spectral-spatial sequence characteristics-based convolutional transformer for hype spectral change detection. CAAI Trans. Intell. Technol. 2023, 8, 1237–1257. [Google Scholar] [CrossRef]
- Cao, H.; Wu, Y.; Bao, Y.; Feng, X.; Wan, S.; Qian, C. UTrans-Net: A model for short-term precipitation prediction. Artif. Intell. Appl. 2023, 1, 106–113. [Google Scholar] [CrossRef]
- Du, H.; Du, S.; Li, W. Probabilistic time series forecasting with deep non-linear state space models. CAAI Trans. Intell. Technol. 2023, 8, 3–13. [Google Scholar] [CrossRef]
- Luo, C. KELL: A kernel-embedded local learning for data-intensive modeling. Artif. Intell. Appl. 2024, 2, 38–44. [Google Scholar] [CrossRef]
- Luo, X.; Wen, X.; Li, Y.; Li, Q. Pruning method for dendritic neuron model based on dendrite layer significance constraints. CAAI Trans. Intell. Technol. 2023, 8, 308–318. [Google Scholar] [CrossRef]
- Yusuf, A.; Kiri, A.A.; Lawal, L.; Kiri, A.I. A hybrid conjugate gradient algorithm for nonlinear system of equations through conjugacy condition. Artif. Intell. Appl. 2023. [Google Scholar] [CrossRef]
- Tien Bui, D.; Moayedi, H.; Gör, M.; Jaafari, A.; Foong, L.K. Predicting slope stability failure through machine learning paradigms. ISPRS Int. J. Geo-Inf. 2019, 8, 395. [Google Scholar]
- Moayedi, H.; Tien Bui, D.; Kalantar, B.; Kok Foong, L. Machine-learning-based classification approaches toward recognizing slope stability failure. Appl. Sci. 2019, 9, 4638. [Google Scholar] [CrossRef]
- Lin, S.; Zheng, H.; Han, C.; Han, B.; Li, W. Evaluation and prediction of slope stability using machine learning approaches. Front. Struct. Civ. Eng. 2021, 15, 821–833. [Google Scholar] [CrossRef]
- Potts, D.M.; Kovacevic, N.; Vaughan, P.R. Delayed collapse of cut slopes in stiff clay. Géotechnique 1997, 47, 953–982. [Google Scholar] [CrossRef]
- Xu, Q.; Tang, M.G.; Xu, K.X.; Huang, X. Research on space-time evolution laws and early warning-prediction of landslides. Chin. J. Rock Mech. Eng. 2008, 27, 1104–1112. [Google Scholar]

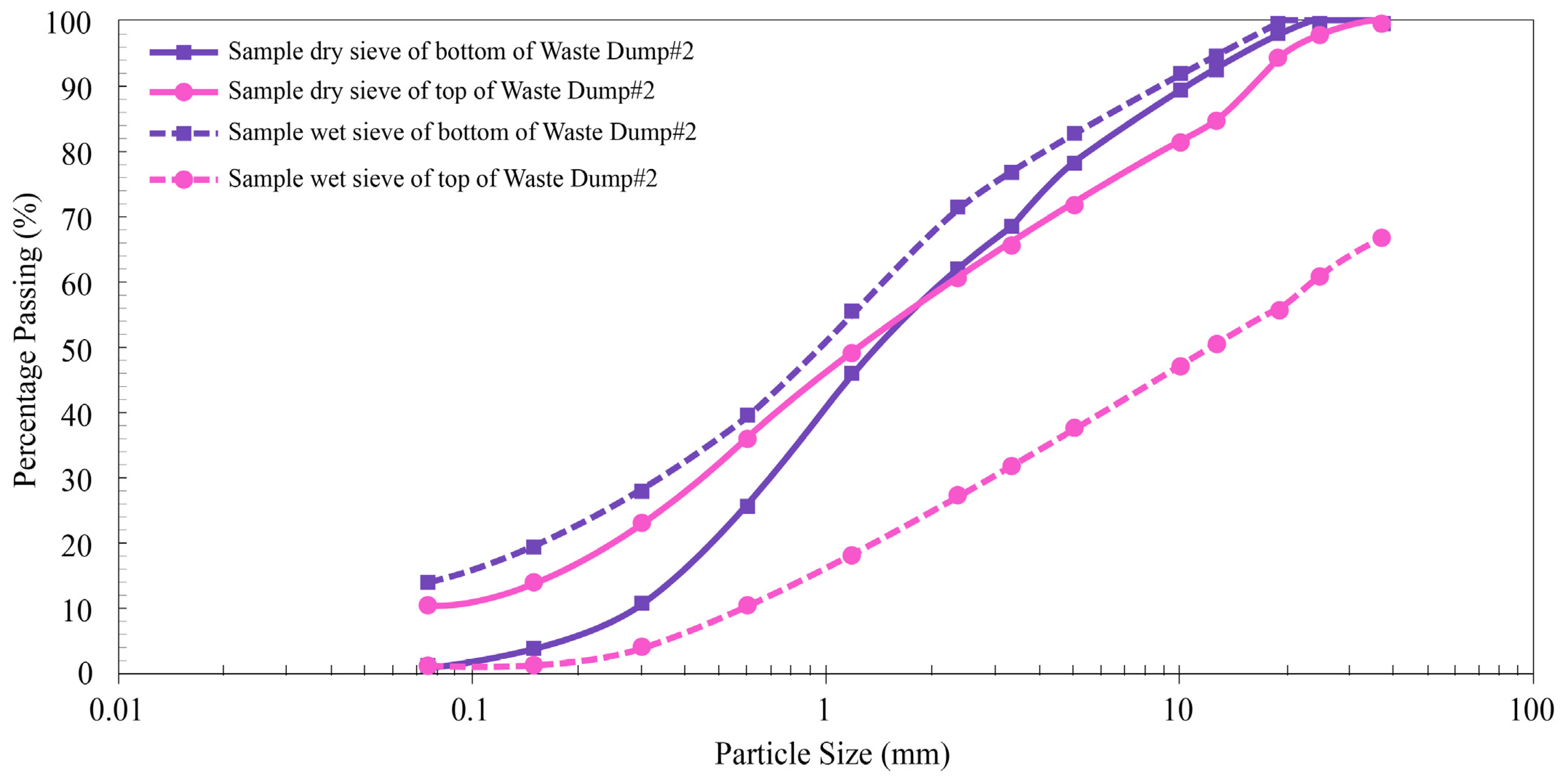




| Samples from Waste Dump#2 | Gravel (2 mm–76.2 mm) | Sand (0.075 mm–2 mm) | Silt and Clay (<0.075 mm) | |||
|---|---|---|---|---|---|---|
| Wash Sieving (%) | Dry Sieving (%) | Wash Sieving (%) | Dry Sieving (%) | Wash Sieving (%) | Dry Sieving (%) | |
| Bottom | 33.5 | 41.3 | 53.2 | 57.7 | 13.8 | 1.5 |
| Top | 43.7 | 75.8 | 46.3 | 24.7 | 10.7 | 0.8 |
| Instrument | Model and Main Parameter | Manufacturer |
|---|---|---|
| New Standard Soil Sieve | N.A. | China Beijing Zhongjiao Jianyi Technology Development Co., Ltd. |
| Electronic Balance | BSA224S | China Shenzhen Xinlangpu Electronic Technology Co., Ltd. |
| Strain-controlled Straight Shears (Quadruple Shears) | ZJ | China Nanjing Soil Instrument Factory Co., Ltd. |
| Waste Dump Grade | Total Volume of a Single Waste Dump (×104 m3) | Stacking Height (m) |
|---|---|---|
| Level 1 | V ≥ 10,000 | H ≥ 150 |
| Level 2 | 2000 ≤ V < 10,000 | 100 ≤ H < 150 |
| Level 3 | 500 ≤ V < 2000 | 50 ≤ H < 100 |
| Level 4 | V < 500 | H < 50 |
| Waste Dump Grade | Safety Factor Reserve Standard |
|---|---|
| Level 1 | 1.25~1.30 |
| Level 2 | 1.20~1.25 |
| Level 3 | 1.15~1.20 |
| Level 4 | 1.15 |
Notes:
| |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, Z.; Ding, X.; Liu, X.; Wahab, A.; Ao, Z.; Tian, Y.; Bang, V.S.; Long, Z.; Li, G.; Ma, P. Slope Deformation Mechanisms and Stability Assessment under Varied Conditions in an Iron Mine Waste Dump. Water 2024, 16, 846. https://doi.org/10.3390/w16060846
Yang Z, Ding X, Liu X, Wahab A, Ao Z, Tian Y, Bang VS, Long Z, Li G, Ma P. Slope Deformation Mechanisms and Stability Assessment under Varied Conditions in an Iron Mine Waste Dump. Water. 2024; 16(6):846. https://doi.org/10.3390/w16060846
Chicago/Turabian StyleYang, Zhongao, Xiaohua Ding, Xin Liu, Abdoul Wahab, Zhongchen Ao, Ya Tian, Van Son Bang, Zhaoxi Long, Guodong Li, and Penglin Ma. 2024. "Slope Deformation Mechanisms and Stability Assessment under Varied Conditions in an Iron Mine Waste Dump" Water 16, no. 6: 846. https://doi.org/10.3390/w16060846
APA StyleYang, Z., Ding, X., Liu, X., Wahab, A., Ao, Z., Tian, Y., Bang, V. S., Long, Z., Li, G., & Ma, P. (2024). Slope Deformation Mechanisms and Stability Assessment under Varied Conditions in an Iron Mine Waste Dump. Water, 16(6), 846. https://doi.org/10.3390/w16060846







