Study on the Hydrodynamic Performance of Swing-Type Flapping Hydrofoil Bionic Pumps Affected by Foil Camber
Abstract
1. Introduction
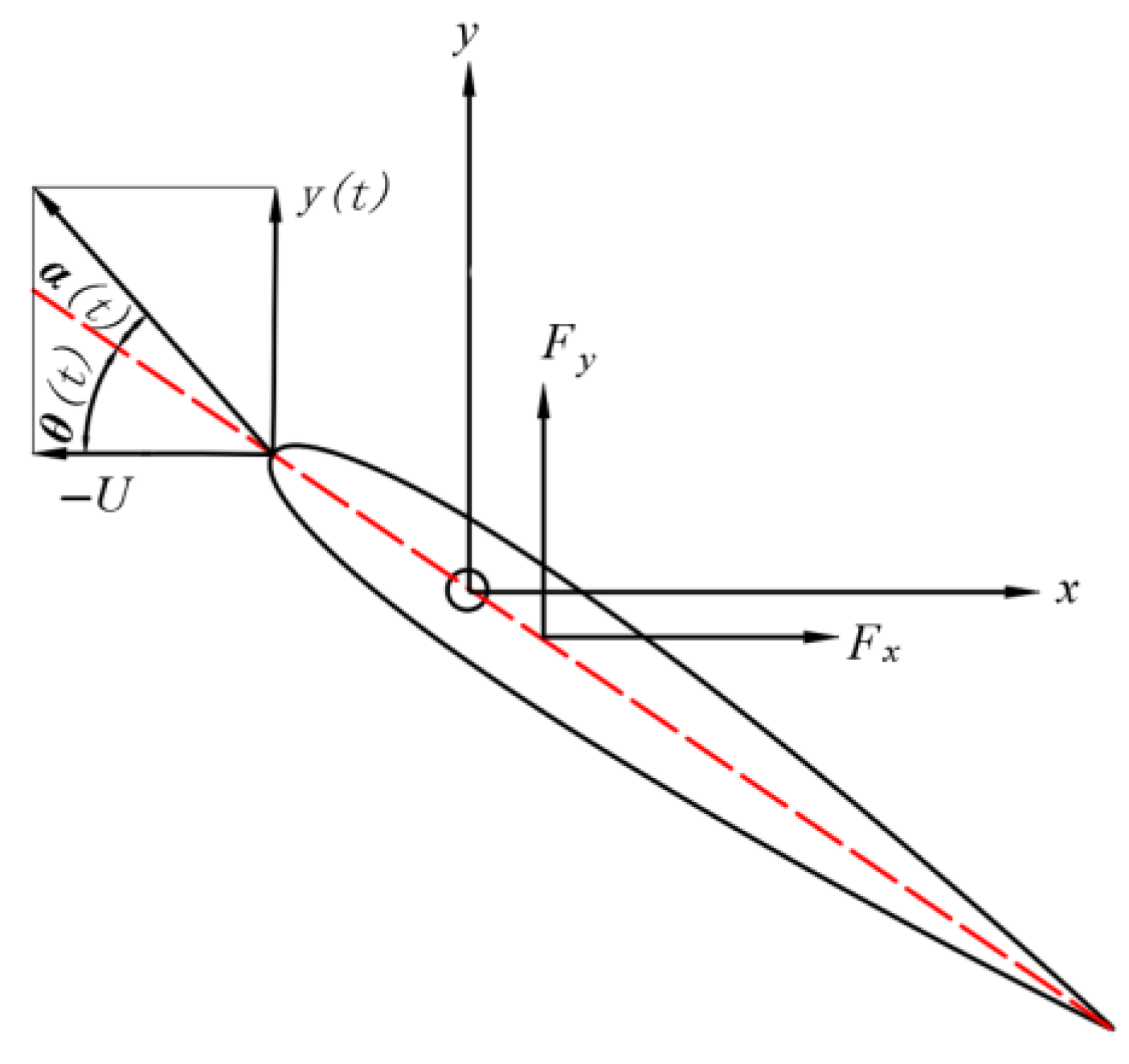
2. Motion Description and Mechanical Model
2.1. Motion Description
2.2. Mechanical Model and Pumping Indicators
3. Foil Geometry Parameter Model
4. Calculation Method
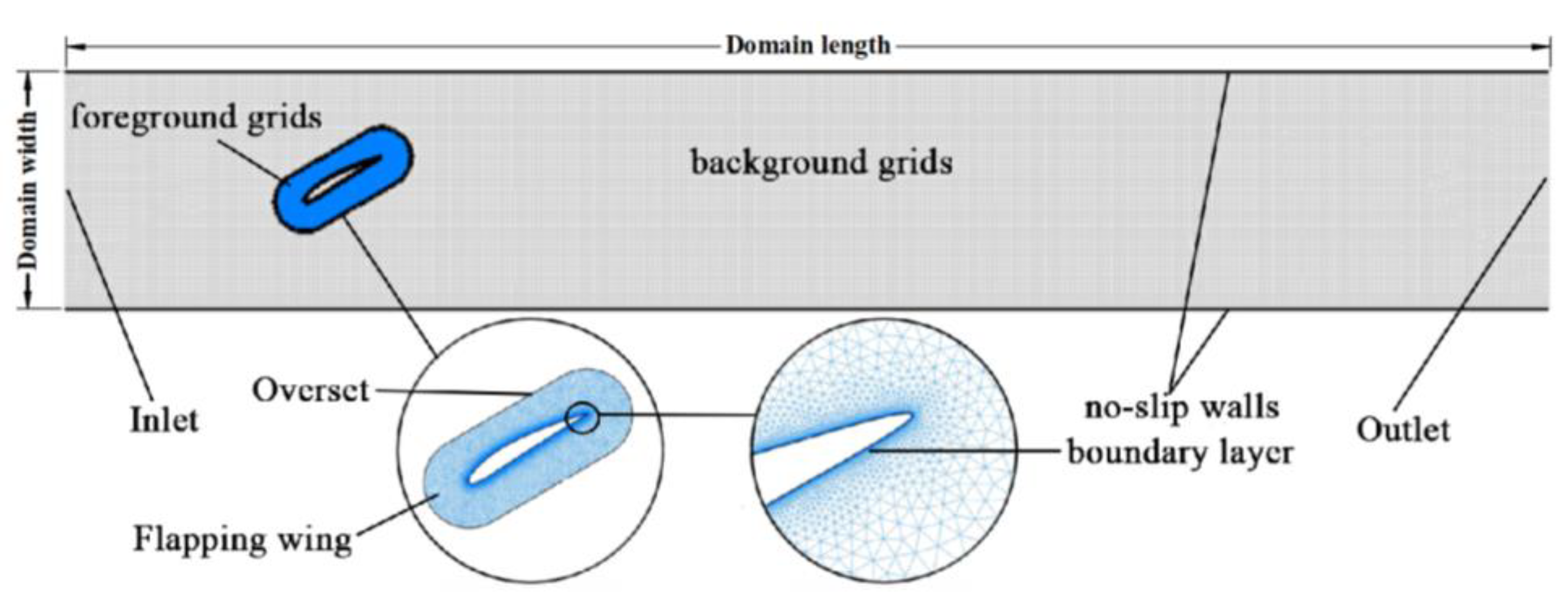
4.1. Mesh Generation and Solution Settings
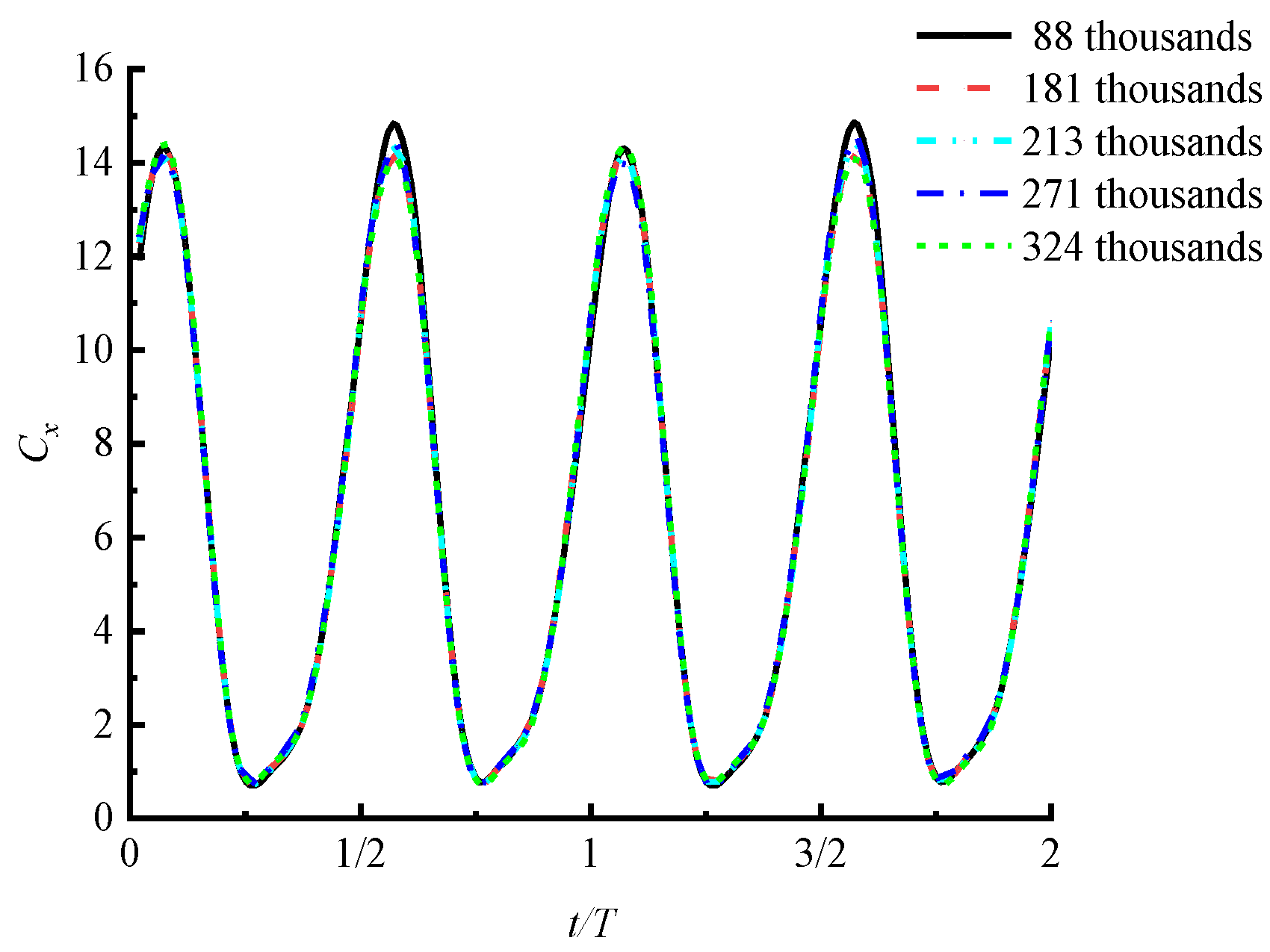
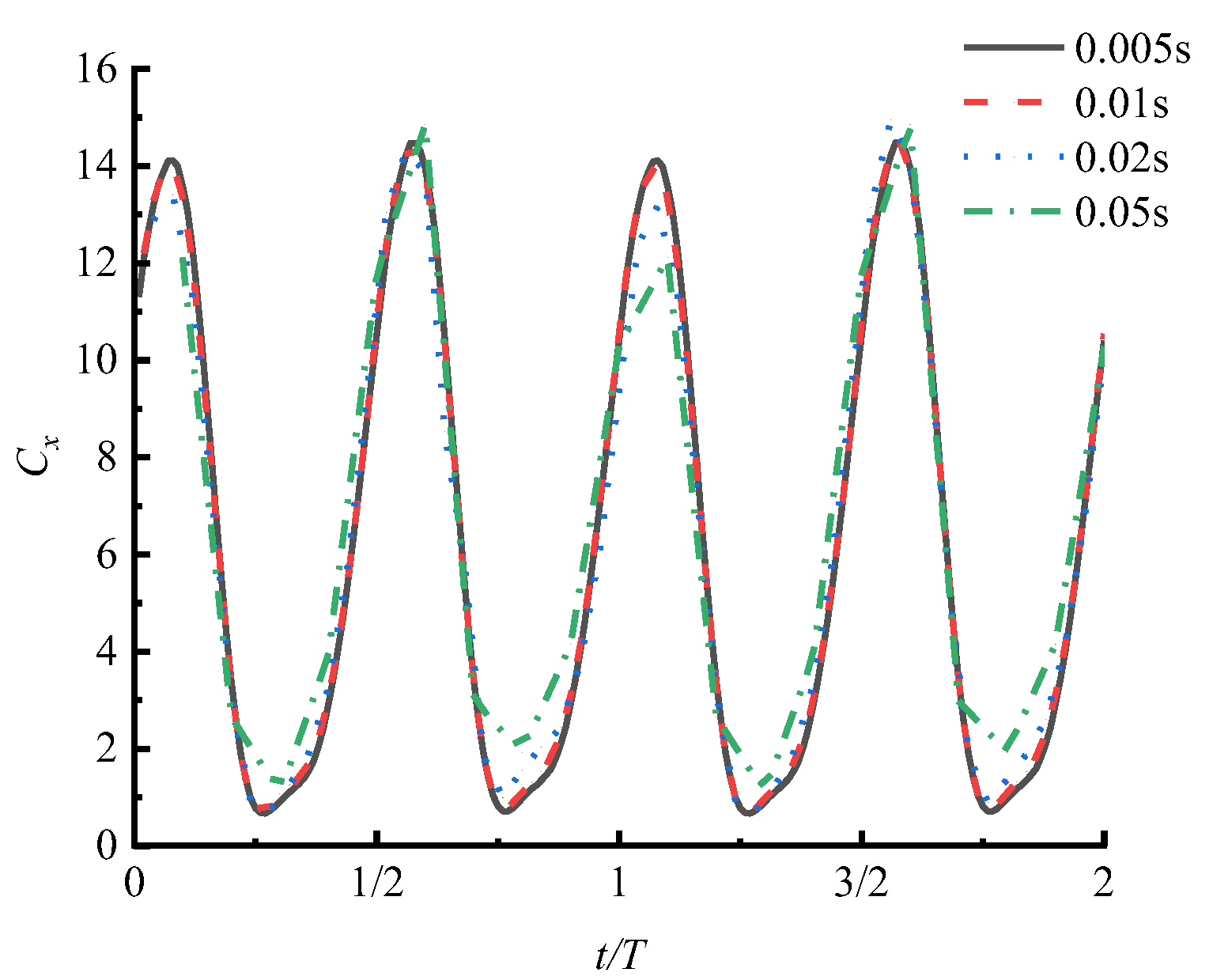
4.2. Mesh Independence and Time Step Insensitivity Verification
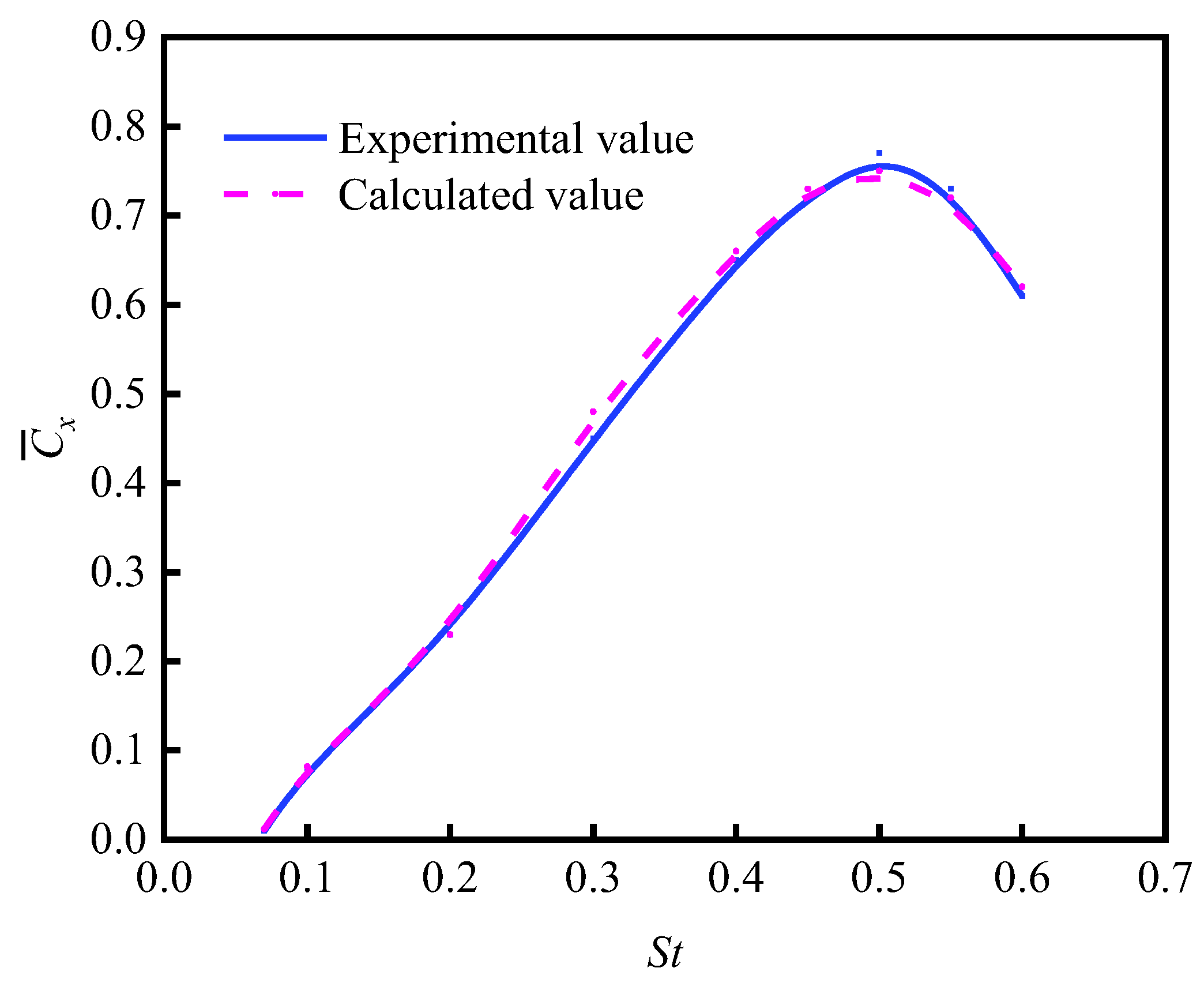
5. Numerical Method Validation
6. Results and Analyses
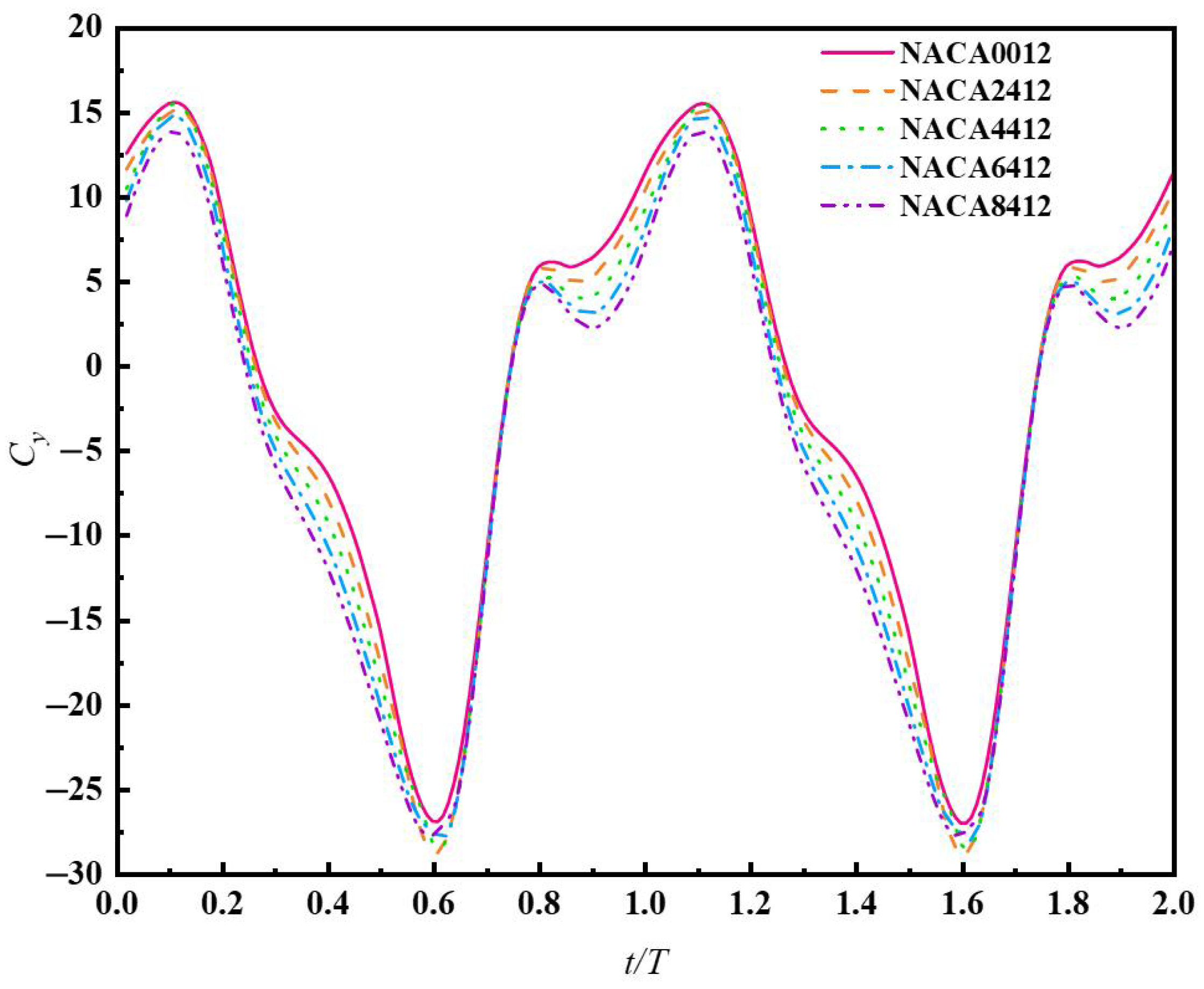
6.1. Analysis of the Influence of the Foil Camber on the Mechanical Performance of Flapping Hydrofoil Bionic Pumps
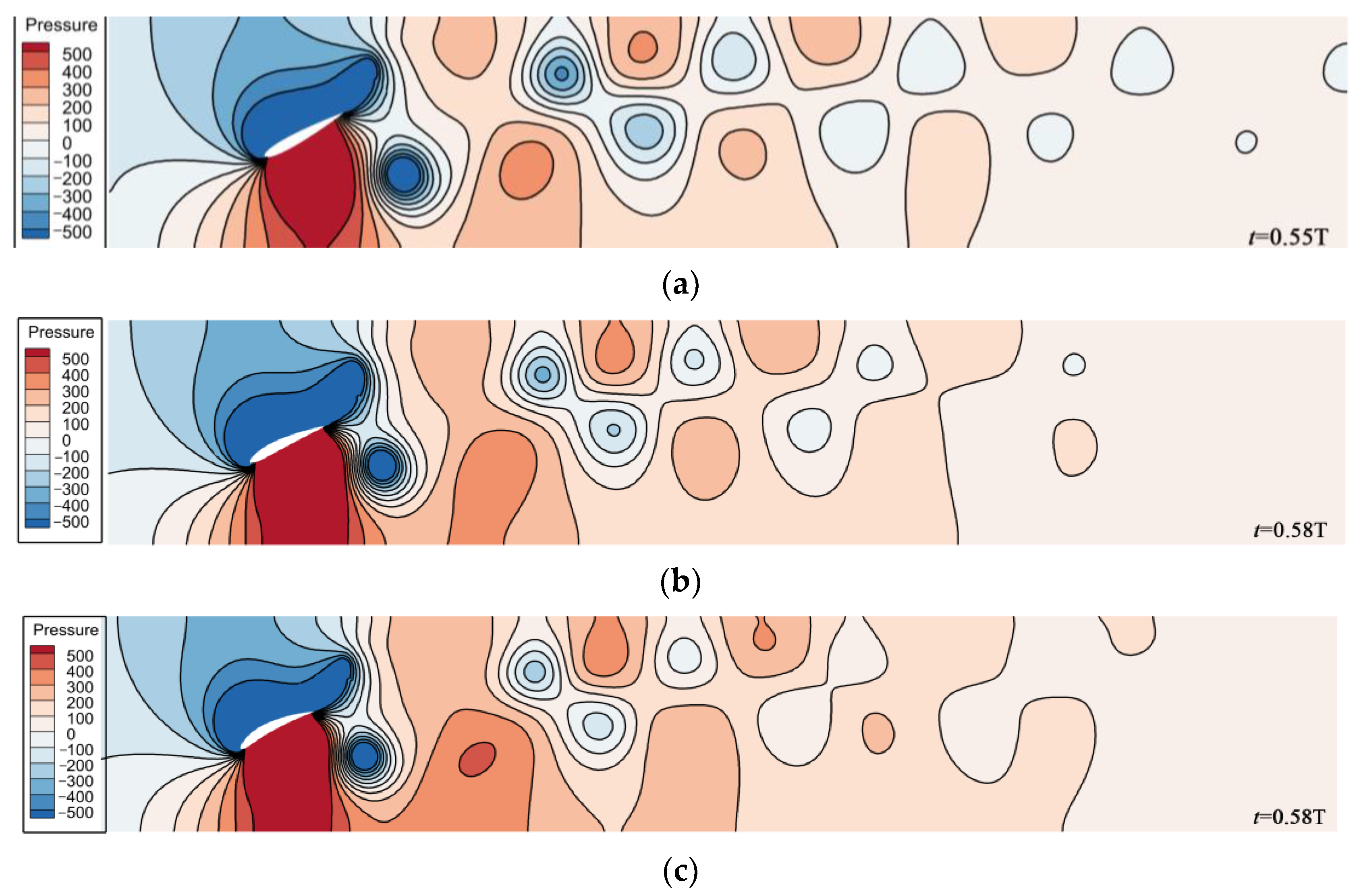
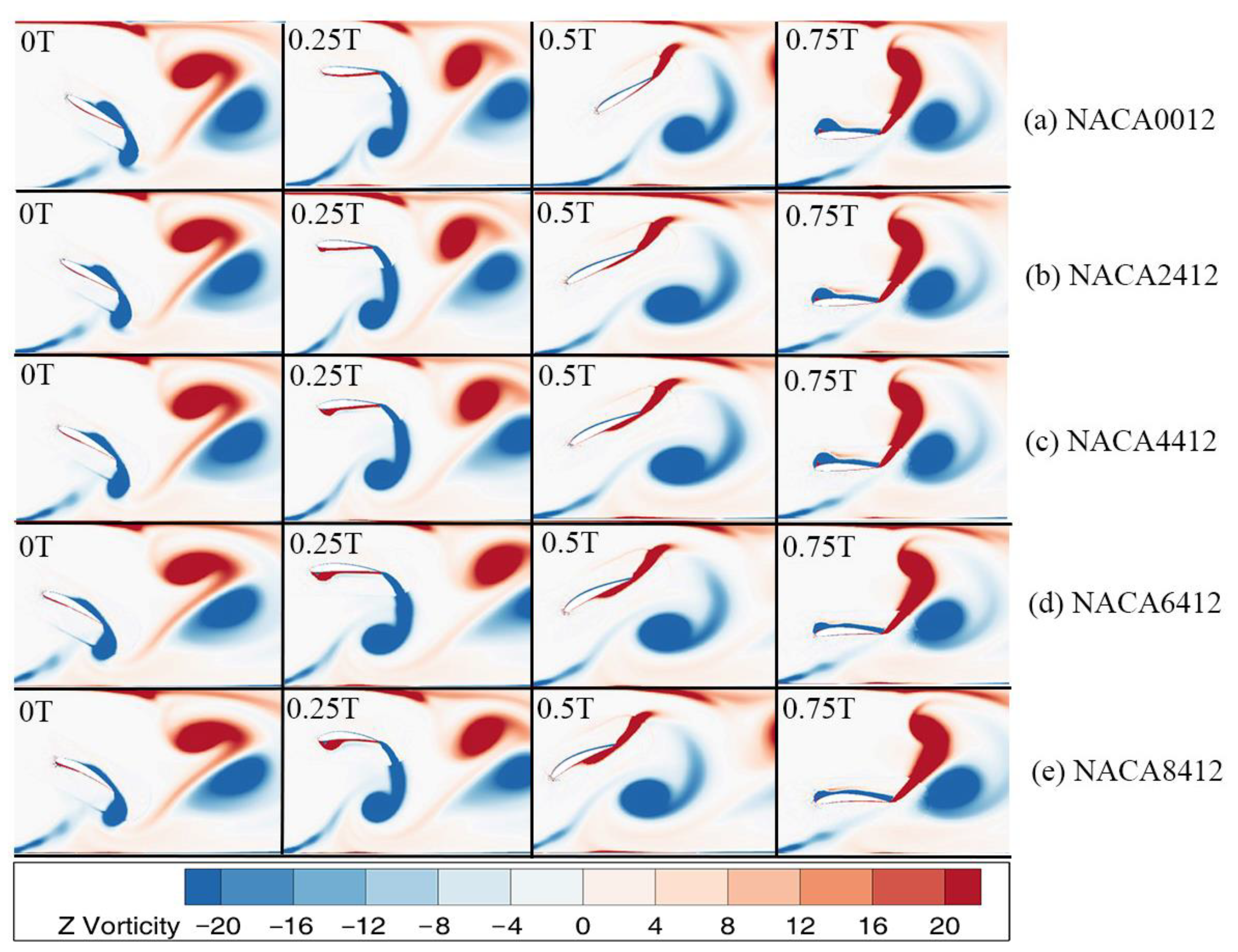
6.2. Analysis of the Influence of Different Foil Curvatures on the Vortex Structure of the Hydrofoil Tail
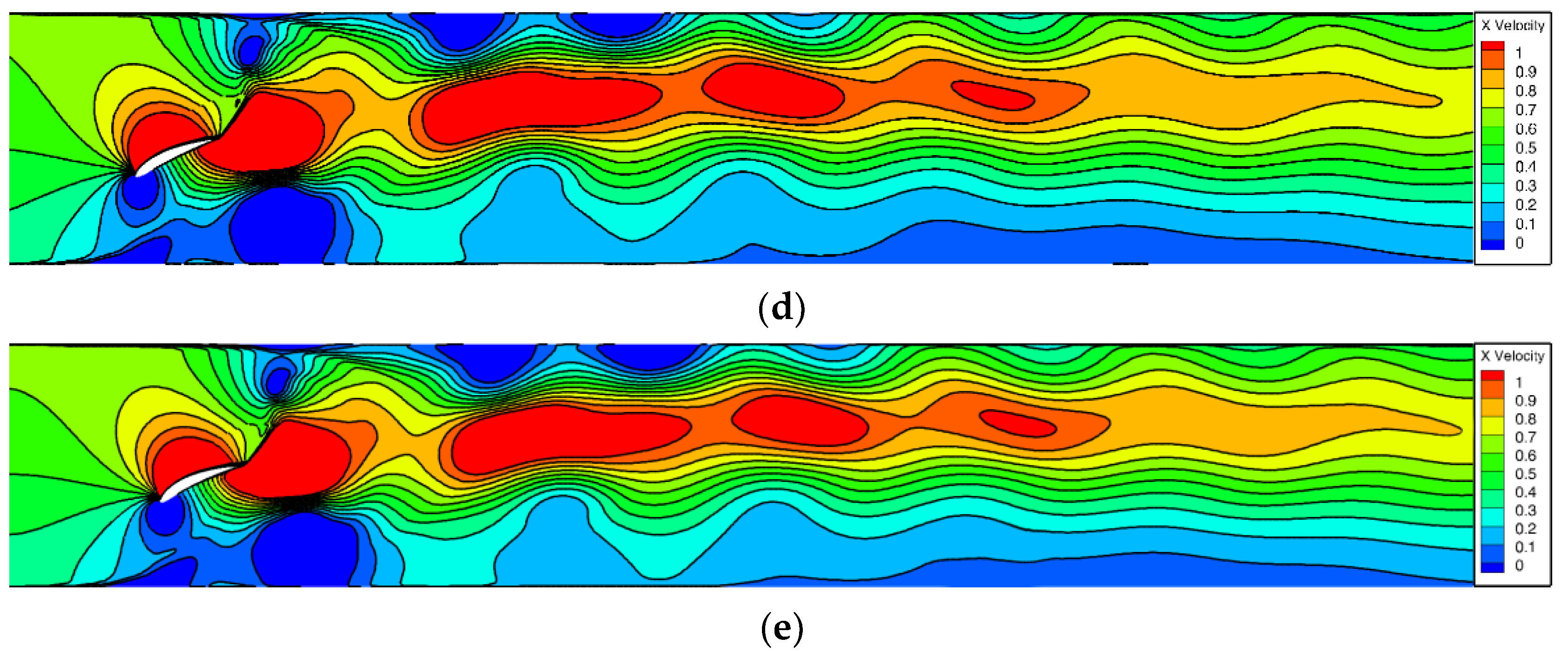
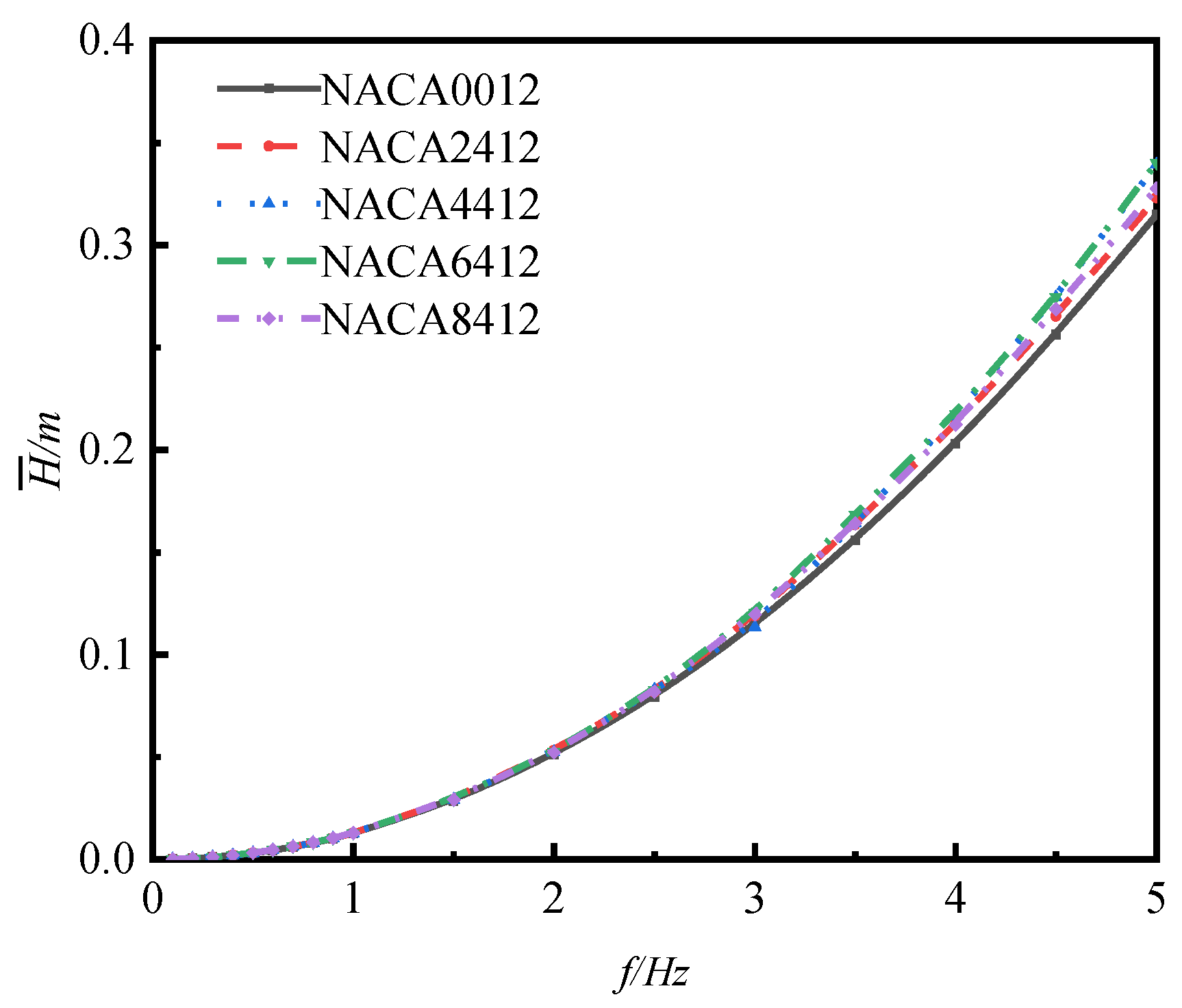
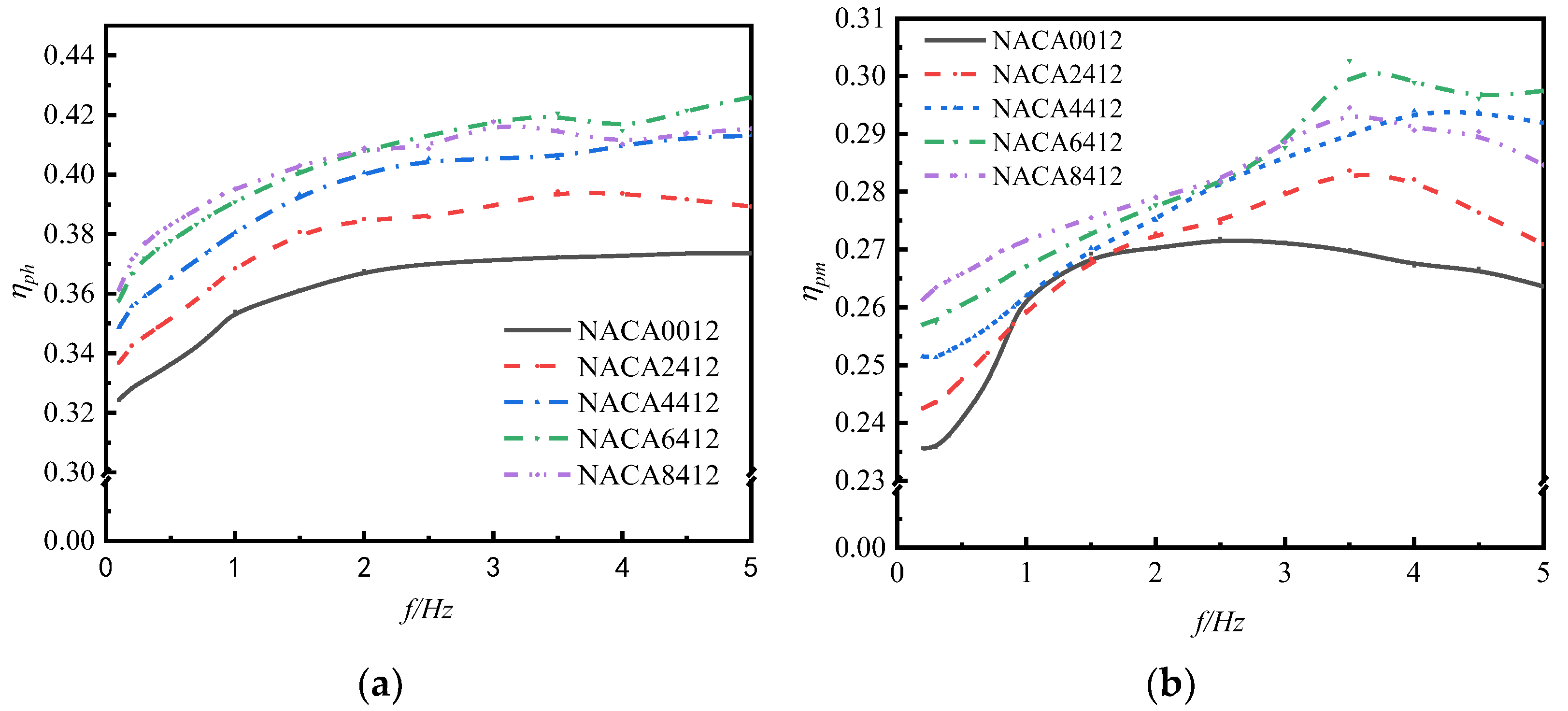
6.3. Impact Analysis of Different Foil Curvatures on the Pumping Performance of the Hydrofoil
7. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Dai, X.; Xu, G.; Ding, Y.; Zeng, S.; You, L.; Jiang, J.; Zhang, H. Online Storage Technology of the Separate Sewage System: Demonstration Study in a Typical Plain River Network City. Water 2022, 14, 3194. [Google Scholar] [CrossRef]
- Phillips, J.; Slattery, M. Downstream trends in discharge, slope, and stream power in a lower coastal plain river. J. Hydrol. 2007, 334, 290–303. [Google Scholar] [CrossRef]
- Eui, L.; Joong, K. Convertible Operation Techniques for Pump Stations Sharing Centralized Reservoirs for Improving Resilience in Urban Drainage Systems. Water 2017, 9, 843. [Google Scholar] [CrossRef]
- Yan, Y.; Yuan, S.; Lyu, S. Hydrodynamic reconstruction of Wennan river network in Shanghai City. J. Hohai Univ. Nat. Sci. 2021, 49, 329–334+365. [Google Scholar]
- Feng, H.; Wan, Y.; Fan, Z. Numerical investigation of turbulent cavitating flow in an axial flow pump using a new transport-based model. J. Mech. Sci. Technol. 2020, 34, 745–756. [Google Scholar] [CrossRef]
- Yan, J.; Chao, C.; Ji, P.; Wen, W. Experiment on Pressure Fluctuation of Axial Flow Pump System under Different Cavitation Conditions. Trans. Chin. Soc. Agric. Mach. 2018, 49, 158–164. [Google Scholar]
- Zhang, D.; Shi, L.; Shi, W.; Zhao, R.; Wang, H.; Van Esch, B.B. Numerical analysis of unsteady tip leakage vortex cavitation cloud and unstable suction-side-perpendicular cavitating vortices in an axial flow pump. Int. J. Multiph. Flow 2015, 77, 244–259. [Google Scholar] [CrossRef]
- Wu, D.; Bai, Y. Numerical Simulation of Flow-Induced Noise in Horizontal Axial Flow Pumps in Forward and Reverse Conditions. Water 2023, 15, 322. [Google Scholar] [CrossRef]
- Dai, C.; Zhang, Y.; Pan, Q.; Dong, L.; Liu, H. Study on Vibration Characteristics of Marine Centrifugal Pump Unit Excited by Different Excitation Sources. J. Mar. Sci. Eng. 2021, 9, 274. [Google Scholar] [CrossRef]
- Xie, L.; Wang, F.; He, C. Experimental investigation on hydrodynamic characteristics of a 15 degree slanted axial-flow pump system. J. Hydraul. Eng. 2019, 50, 798–805. [Google Scholar]
- Hua, E.; Qiu, L.; Xie, R.; Su, Z.; Zhu, W. Comparative Analysis of the Hydrodynamic Performance of Dual Flapping Foils with In-Phase and Out-of-Phase Oscillations. Water 2023, 15, 3275. [Google Scholar] [CrossRef]
- Ziemińska-Stolarska, A.; Kempa, M. Modeling and Monitoring of Hydrodynamics and Surface Water Quality in the Sulejów Dam Reservoir, Poland. Water 2021, 13, 296. [Google Scholar] [CrossRef]
- Keserwan, G.; Ayog, L.; Bau, D. Discontinuous Galerkin formulation for 2D hydrodynamic modelling: Trade-offs between theoretical complexity and practical convenience. Comput. Methods Appl. Mech. Eng. 2018, 342, 710–741. [Google Scholar] [CrossRef]
- Man, X.; Lei, C.; Carey, C.C.; Little, J.C. Relative Performance of 1-D Versus 3-D Hydrodynamic, Water-Quality Models for Predicting Water Temperature and Oxygen in a Shallow, Eutrophic, Managed Reservoir. Water 2021, 13, 88. [Google Scholar] [CrossRef]
- Martínez-Aranda, S.; Murillo, J. A 1D numerical model for the simulation of unsteady and highly erosive flows in rivers. Comput. Fluids 2019, 181, 8–34. [Google Scholar] [CrossRef]
- Betz, A. Ein Beitrag zur Erklaerung Segelfluges. Z Flugtech Mot. 1912, 3, 269–272. [Google Scholar]
- Lu, K.; Xie, Y.H.; Zhang, D. Numerical study of large amplitude, no sinusoidal motion and camber effects on pitching airfoil propulsion. J. Fluids Struct. 2013, 36, 184–194. [Google Scholar] [CrossRef]
- Read, D.A.; Hover, F.S.; Triantafyllou, M.S. Forces on oscillating foils for propulsion and maneuvering. J. Fluids Struct. 2003, 17, 163–183. [Google Scholar] [CrossRef]
- Schouveiler, L.; Hover, F.S.; Triantafyllou, M.S. Performance of flapping foil propulsion. J. Fluids Struct. 2005, 20, 949–959. [Google Scholar] [CrossRef]
- Yang, S.; Liu, C.; Wu, J. Effect of motion trajectory on the aerodynamic performance of a flapping airfoil. J. Fluids Struct. 2017, 75, 213–232. [Google Scholar] [CrossRef]
- Hua, E.; Zhu, W.; Xie, R.; Su, Z.; Luo, H.; Qiu, L. Comparative Analysis of the Hydrodynamic Performance of Arc and Linear Flapping Hydrofoils. Processes 2023, 11, 1579. [Google Scholar] [CrossRef]
- Lin, X.; Wu, J.; Zhang, T. Performance investigation of a self-propelled foil with combined oscillating motion in stationary fluid. Ocean Eng. 2019, 175, 33–49. [Google Scholar] [CrossRef]
- Sun, G.; Wang, Y.; Xie, Y.; Ma, P.; Zhang, Y. Hydrodynamic and energy extraction properties of oscillating hydrofoils with a trailing edge flap. Appl. Ocean Res. 2021, 110, 102530. [Google Scholar] [CrossRef]
- Ashraf, M.A.; Young, J.; Lai, C.S. Reynolds number, thickness and camber effects on flapping airfoil propulsion. J. Fluids Struct. 2011, 27, 145–160. [Google Scholar] [CrossRef]
- Zhou, J.; Yan, W.; Mei, L.; Shi, W. Performance of Semi-Active Flapping Hydrofoil with Arc Trajectory. Water 2023, 15, 269. [Google Scholar] [CrossRef]
- Zhang, S.; Yan, W.; Zhou, J.; Mei, L.; Yu, D. Influence of tip shapes on hydrodynamic performance of low-aspect-ratio oscillating foils. J. Ship Mech. 2022, 26, 629–644. [Google Scholar]
- Li, Y.; Zhang, X.; Pan, Z. Side wall effect on the propulsive performance of flapping foils—A numerical investigation. J. Ship Mech. 2020, 24, 570–577. [Google Scholar]
- Hua, E.; Luo, H.; Xie, R.; Chen, W. Investigation on the Influence of Flow Passage Structure on the Performance of Bionic Pumps. Processes 2022, 10, 2569. [Google Scholar] [CrossRef]
- Lighthill, M.J. Aquatic animal propulsion of high hydromechanical efficiency. J. Fluid Mech. 1970, 44, 265–301. [Google Scholar] [CrossRef]
- Hua, E.; Chen, W.; Tang, S. Water Pushing Flow Characteristics of Flapping Hydrofoil Device in Small Rive. Trans. Chin. Soc. Agric. Mach. 2022, 53, 154–162. [Google Scholar]
- Wang, F.J. Computational Fluid Dynamics Analysis—Principles and Applications of CFD Software; Tsinghua University Press: Beijing, China, 2004; pp. 124–126. [Google Scholar]
- Jit, S.; Kim, L.; Sunil, D. Influence of the Pivot Location on the Thrust and Propulsive Efficiency Performance of a Two-dimensional Flapping Elliptic Airfoil in a Forward Flight. Phys. Fluids 2021, 8, 163–183. [Google Scholar]


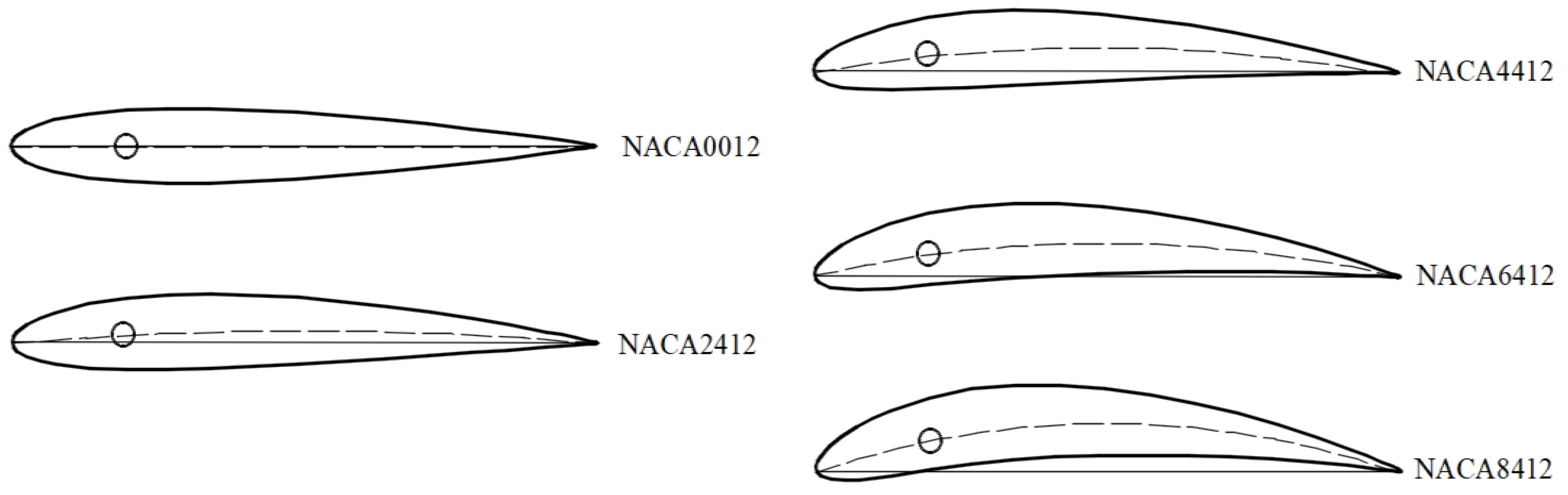


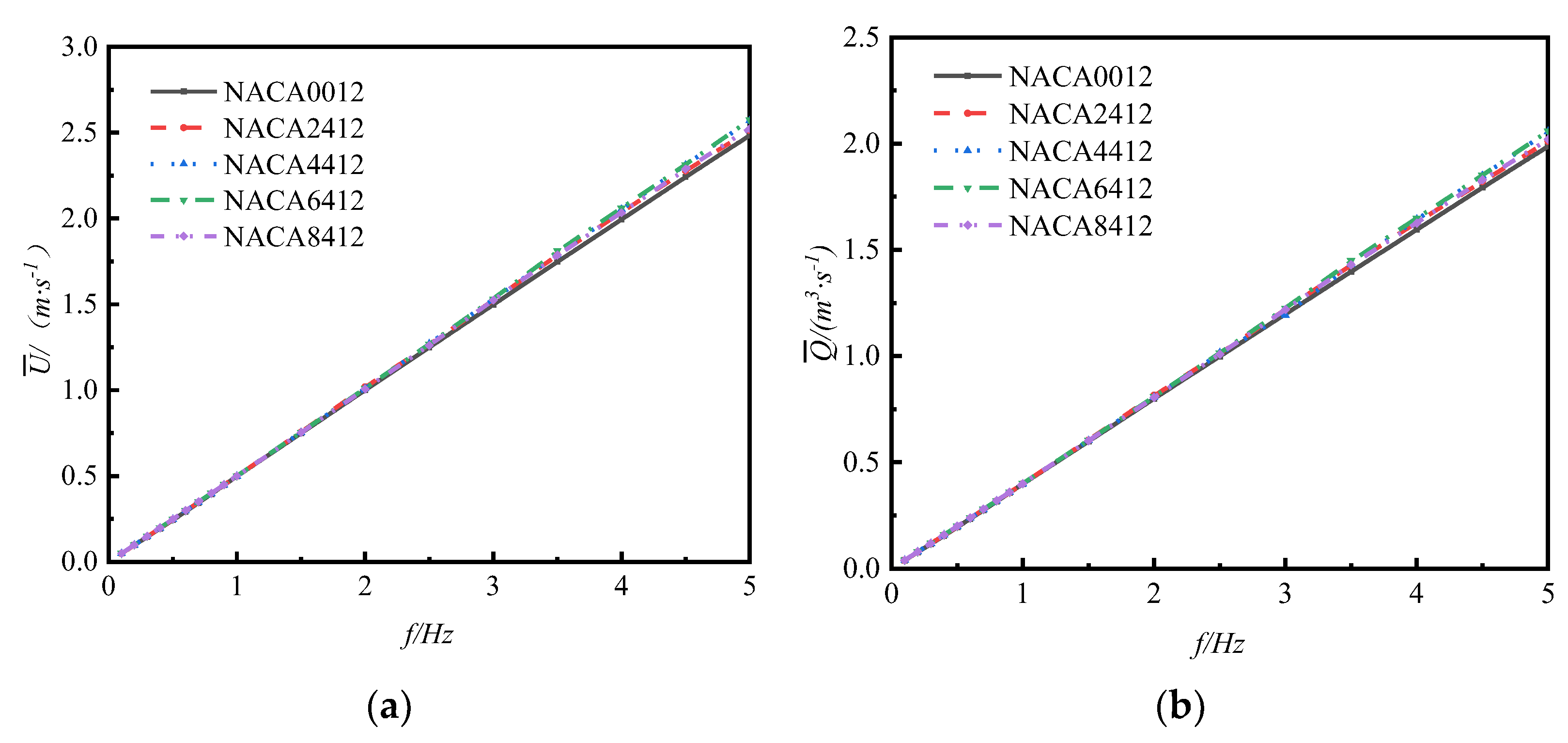
| Foil Name | Chord Length c (m) | Maximum Camber e | Maximum Camber Position |
|---|---|---|---|
| NACA0012 | 0.3 | 0%c | 0%c |
| NACA2412 | 2%c | 40%c | |
| NACA4412 | 4%c | 40%c | |
| NACA6414 | 6%c | 40%c | |
| NACA8424 | 8%c | 40%c |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sun, Q.; Hua, E.; Sun, L.; Qiu, L.; Song, Y.; Xiang, M. Study on the Hydrodynamic Performance of Swing-Type Flapping Hydrofoil Bionic Pumps Affected by Foil Camber. Water 2024, 16, 595. https://doi.org/10.3390/w16040595
Sun Q, Hua E, Sun L, Qiu L, Song Y, Xiang M. Study on the Hydrodynamic Performance of Swing-Type Flapping Hydrofoil Bionic Pumps Affected by Foil Camber. Water. 2024; 16(4):595. https://doi.org/10.3390/w16040595
Chicago/Turabian StyleSun, Qizong, Ertian Hua, Liying Sun, Linfeng Qiu, Yabo Song, and Mingwang Xiang. 2024. "Study on the Hydrodynamic Performance of Swing-Type Flapping Hydrofoil Bionic Pumps Affected by Foil Camber" Water 16, no. 4: 595. https://doi.org/10.3390/w16040595
APA StyleSun, Q., Hua, E., Sun, L., Qiu, L., Song, Y., & Xiang, M. (2024). Study on the Hydrodynamic Performance of Swing-Type Flapping Hydrofoil Bionic Pumps Affected by Foil Camber. Water, 16(4), 595. https://doi.org/10.3390/w16040595





