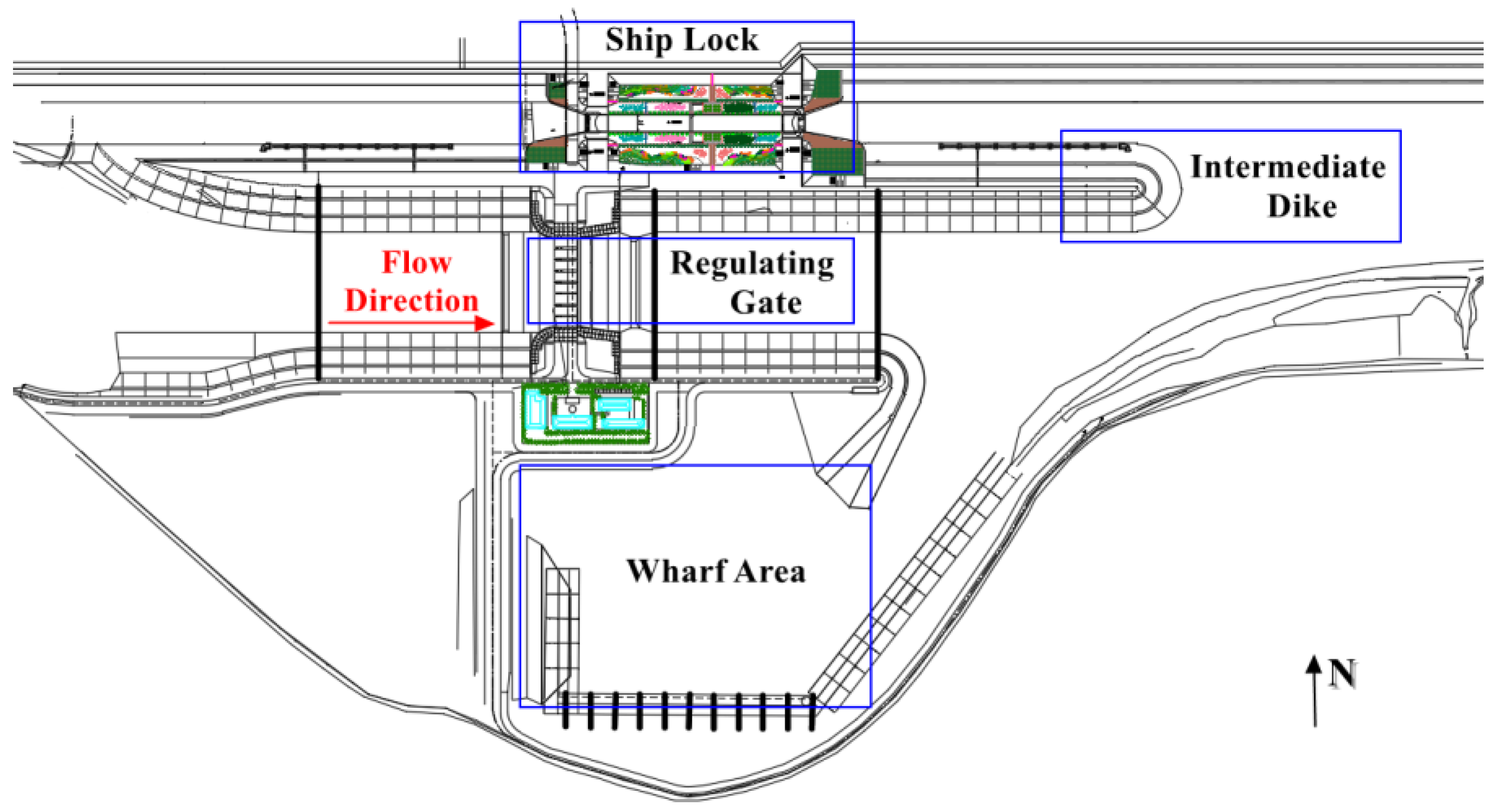
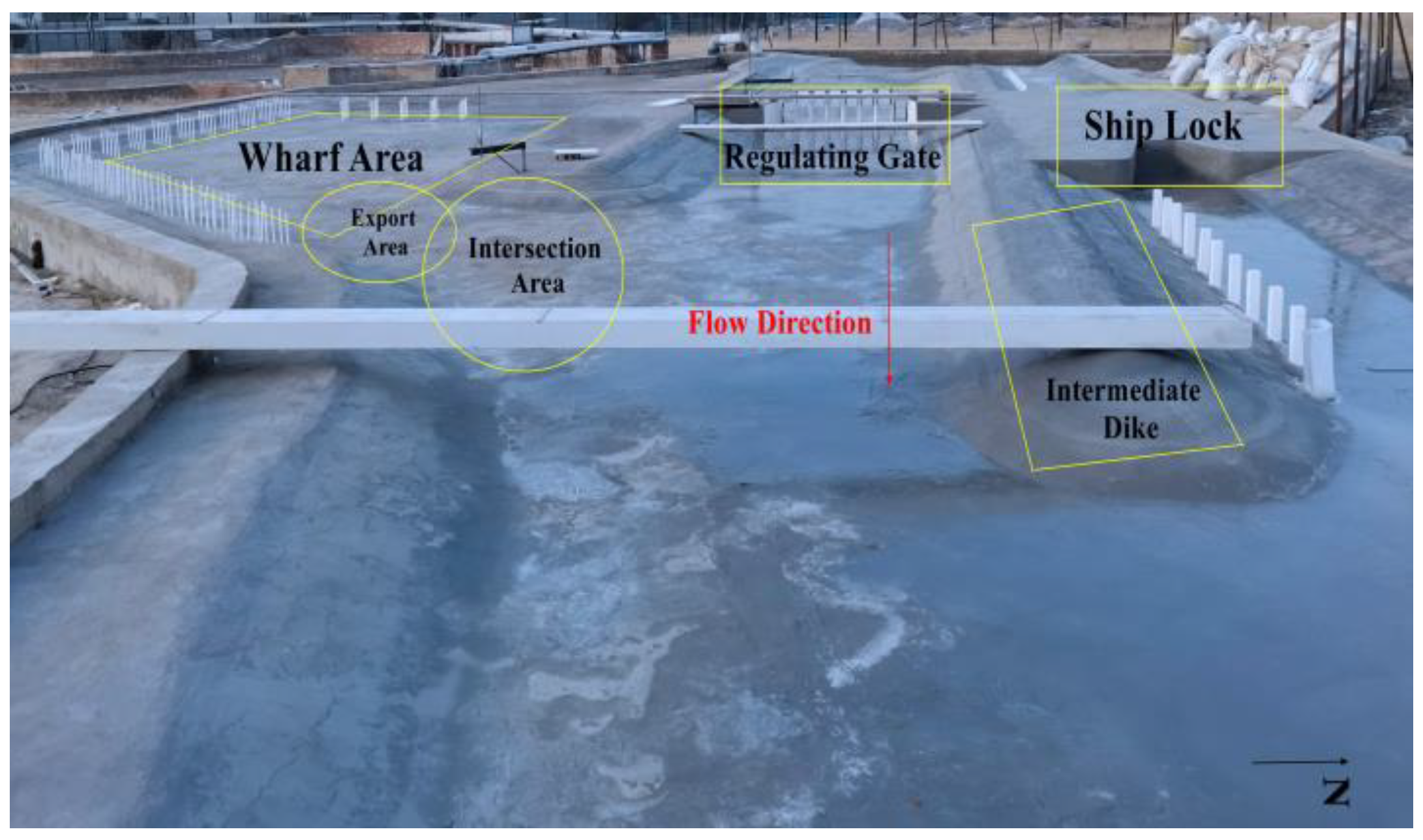
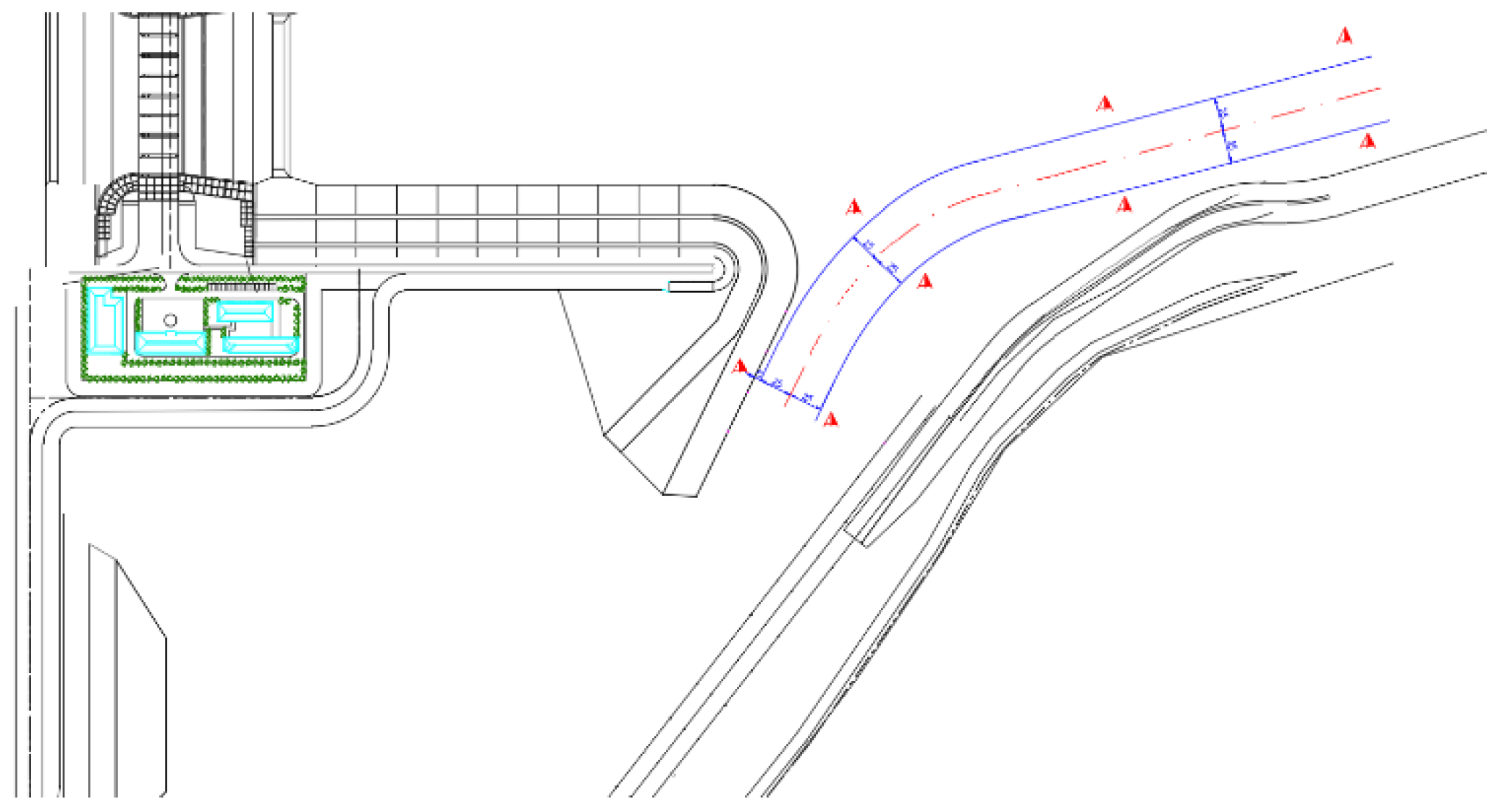
During the test, the physical model was divided into four main areas: the downstream area (of the regulating gate), the wharf area, the export area (of the wharf), and the intersection area (between the export area and the downstream of the regulating gate). The plan of the main study area is shown in
Figure 4. This paper primarily investigates the flow velocity and sedimentation in the wharf entrance area, which includes the export area and the intersection area.
The experimental model adopts a constant flow, gravity-driven circulation water supply system. The functions of the main measuring instruments are as follows: controlled using an E-MAG electromagnetic flow meter to regulate the upstream water flow in the channel; recorded using fixed water level needles and self-recording water level gauges to monitor changes in water levels at various control points along the channel; measured using an L-8 infrared multi-point rotating vane flowmeter and an ADV flowmeter to capture the distribution of flow velocities; assessed through underwater topographic instruments, total stations, and similar devices to measure changes in the riverbed; vibrations in gates detected using a DASP dynamic intelligent monitoring device; documented using a digital camera to record the entire process.
4.1. Experiment Scheme
According to the planning and operation conditions of the regulating gate and the combination of the design water level, the optimal operation scheme comparison test of the gate dispatching mode is carried out. The optimal dispatching operation mode of the gate given by the test results is shown in
Table 3. According to the six design conditions and the corresponding gate scheduling operation mode, the flow velocity test is carried out in the entrance area.
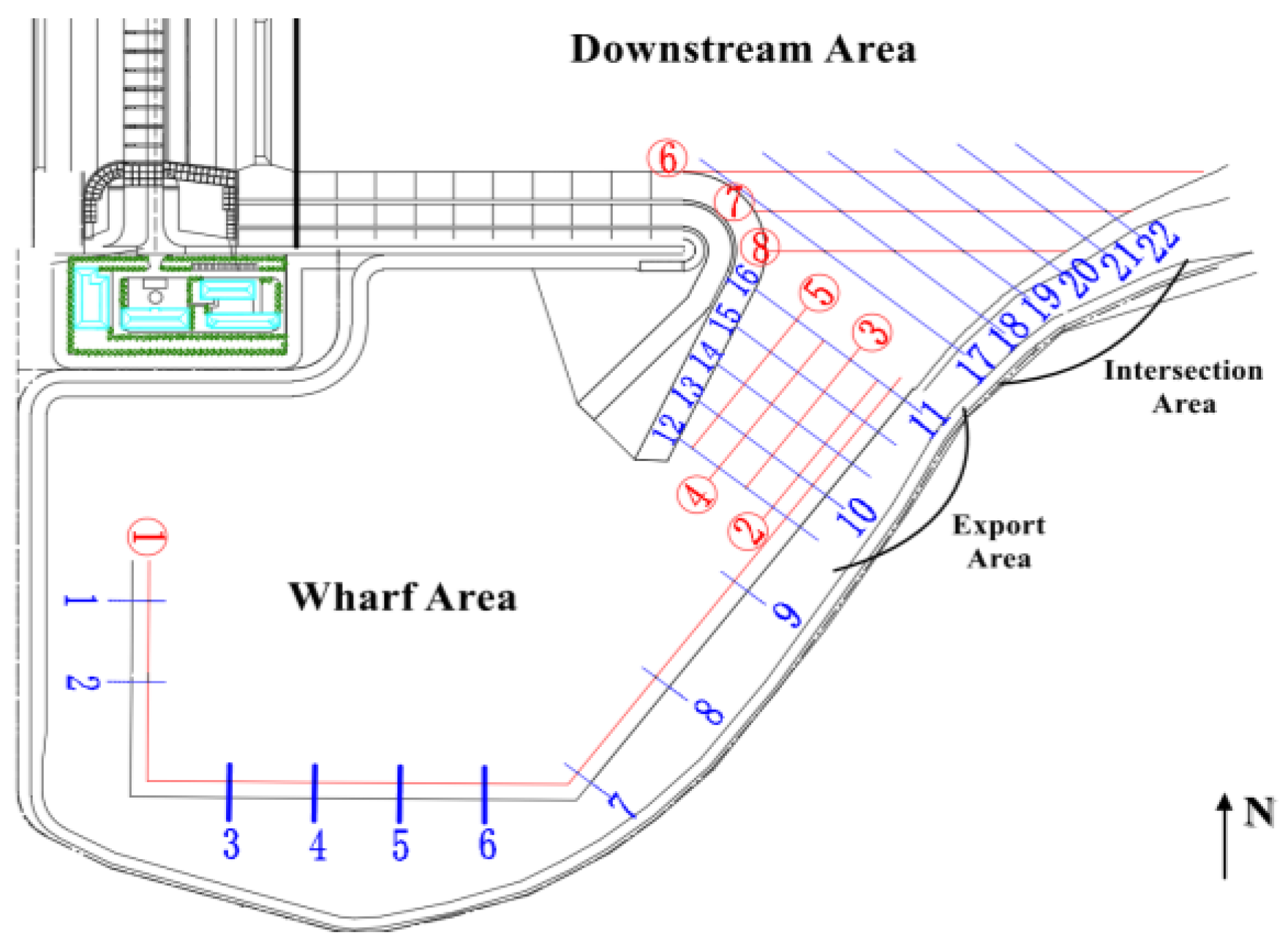
The main test sections and test points of the hydraulic elements collected in the experiment are shown in
Figure 5.
(1) Layout of the measurement points in the wharf area.
A survey section perpendicular to the wharf is made in the front of wharf 1# to wharf 11#, and the number is 1–11. Measuring line ➀ is parallel to the wharf and 13 m away from the front of the wharf. The intersection of each section and measuring line ➀ is the measurement point of the wharf—a total of 11 measurement points.
(2) Layout of the measuring points in the export area.
The export section is arranged in the area between the 10# wharf and the diversion dike. Taking section No. 10 as the reference, a section is set at a distance of 32.5 m, parallel to the direction of section No. 9, which is recorded as section No. 12. In the direction of section No. 11, three parallel sections are set at an interval of 32.5 m, which are sections 14–16 (section No. 13 coincides with section No. 10, section No. 16 coincides with section No. 11)—a total of five test sections. A total of four measuring lines are set up every 26 m parallel to the wharf and from the wharf front, which are recorded as measuring lines ➁–➄, and the intersection with the section is the measuring point, making a total of 20 measuring points.
(3) Layout of the measuring points in intersection area.
Based on section No. 11, a parallel section is set at an interval of 56 m along the exit direction, which is recorded as section No. 17. Then, taking section No. 17 as the basis, a parallel section is set every 32.5 m, making a total of five sections, numbered 18–22. Three measuring lines are arranged. The intersection line between the bank slope and the elevation of 26 m downstream of the regulating gate is set as measuring line ➅, and a parallel survey line is set up every 32.5 m in the direction of the wharf as measuring lines ➆–➇, with a total of 16 measuring points in the intersection area.
4.2. Experiment Result
The flow velocities of the cross-section of the wharf area, the export area, and the intersection area under each working condition are shown in
Table 4,
Table 5 and
Table 6.
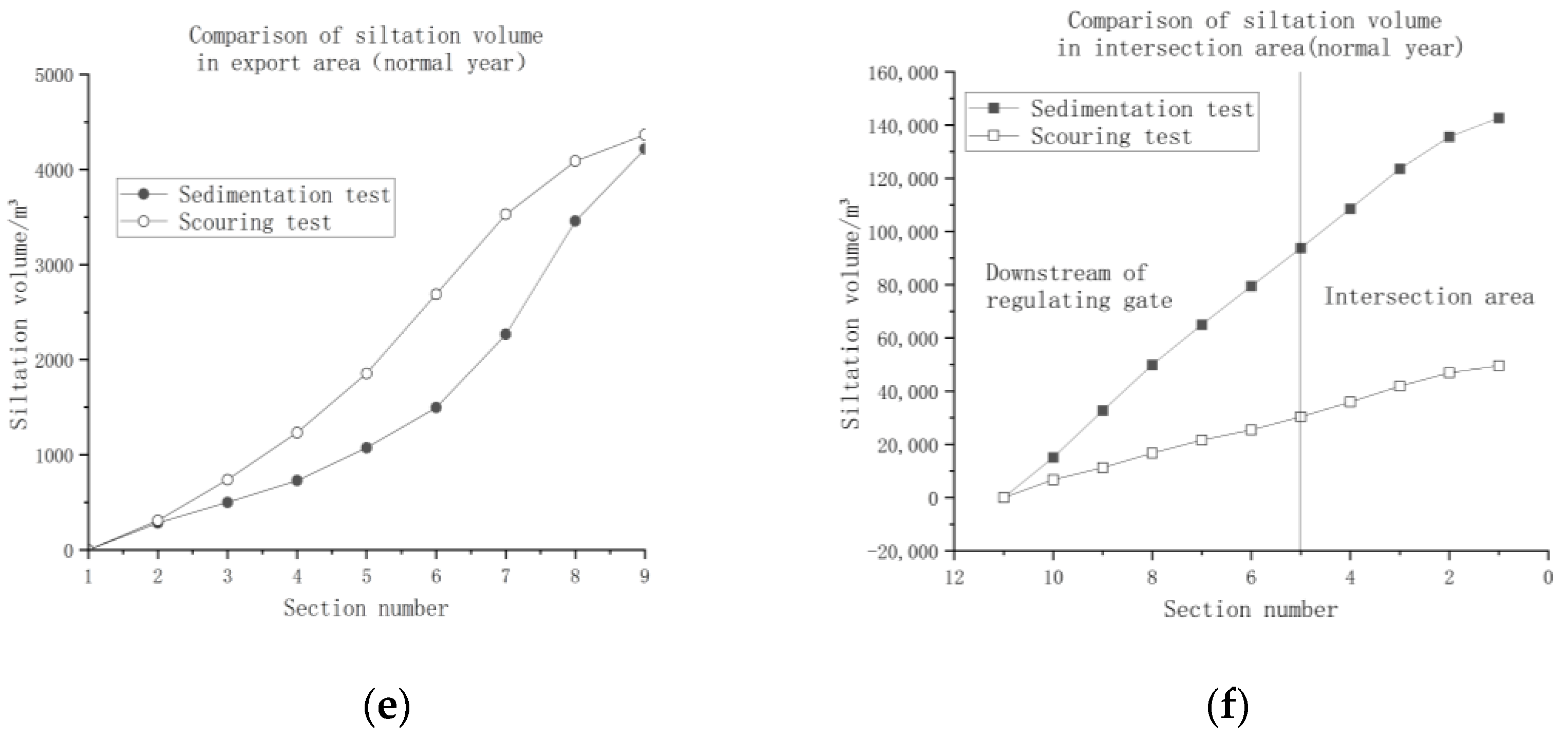
It can be seen from the test data in
Table 4 that the wharf area is fundamentally a still water area, and the overall flow rate is small. In the case of condition 5, the water depth in the wharf area is extremely shallow, and the data are difficult to measure. Under the other five conditions, the flow velocity is weak, and the flow velocity to the wharf export and intersection areas increases slightly. The experimental water level only exceeds the highest navigable water level under condition 1, and the whole wharf area should stop the ship entering and leaving. Under the other five working conditions, the water level is normal, and the wharf area can pass normally.
Under working condition 1, the flow velocity in the wharf area is relatively low, with a maximum of 0.32 m/s, and it is also low in the export area and the intersection area. The data covering 16 measuring points in the intersection area are shown in
Table 7. It can be seen that the flow velocity gradually increases in the direction of the mainstream of the discharge to the regulating gate. Under this working condition, the ship entering the intersection area is perpendicular to the flow velocity direction under the most unfavorable conditions, which can also meet the requirement that the transverse flow velocity of the ship is less than 0.3 m/s [
34]. Therefore, the ship entering the wharf is safe in this area.
The flow velocity at the outlet of the wharf under condition 2 and condition 3 is slightly higher than condition 1, but it is still small, with a maximum of only 0.24 m/s. The flow law of the intersection area is similar to condition 1, and the flow velocity is still larger in the mainstream area closer to the control gate. The angle between the ship entering the wharf and the flow direction is about 30°, and the transverse flow velocity is 0.28 m/s and 0.24 m/s, respectively, which still meets the requirements of the transverse flow velocity of the ship, and the ship entering the wharf is safe in this area.
The export area and the wharf area of condition 4 and condition 5 are similar, and the water depth is shallow. However, the flow velocity in the intersection area increases greatly. The maximum flow velocity of working condition 4 is measuring line ➅ close to the mainstream area; the flow velocity is 0.54 m/s, and the transverse flow velocity of the ship is about 0.24 m/s. The maximum flow velocity of measuring line ➅ in the intersection area of working condition 5 is 0.56 m/s, and the transverse flow velocity of the ship is about 0.28 m/s. Both of them still meet the requirements of transverse flow velocity.
The flow velocity in the intersection area of condition 6 is the largest in each case, as shown in
Table 8. The maximum flow velocity is measuring line ➅ close to the mainstream area, and the flow velocity is 0.97 m/s. Under this condition, the ship needs to reduce the angle with the incoming flow as much as possible to ensure the transverse flow velocity meets the requirements.
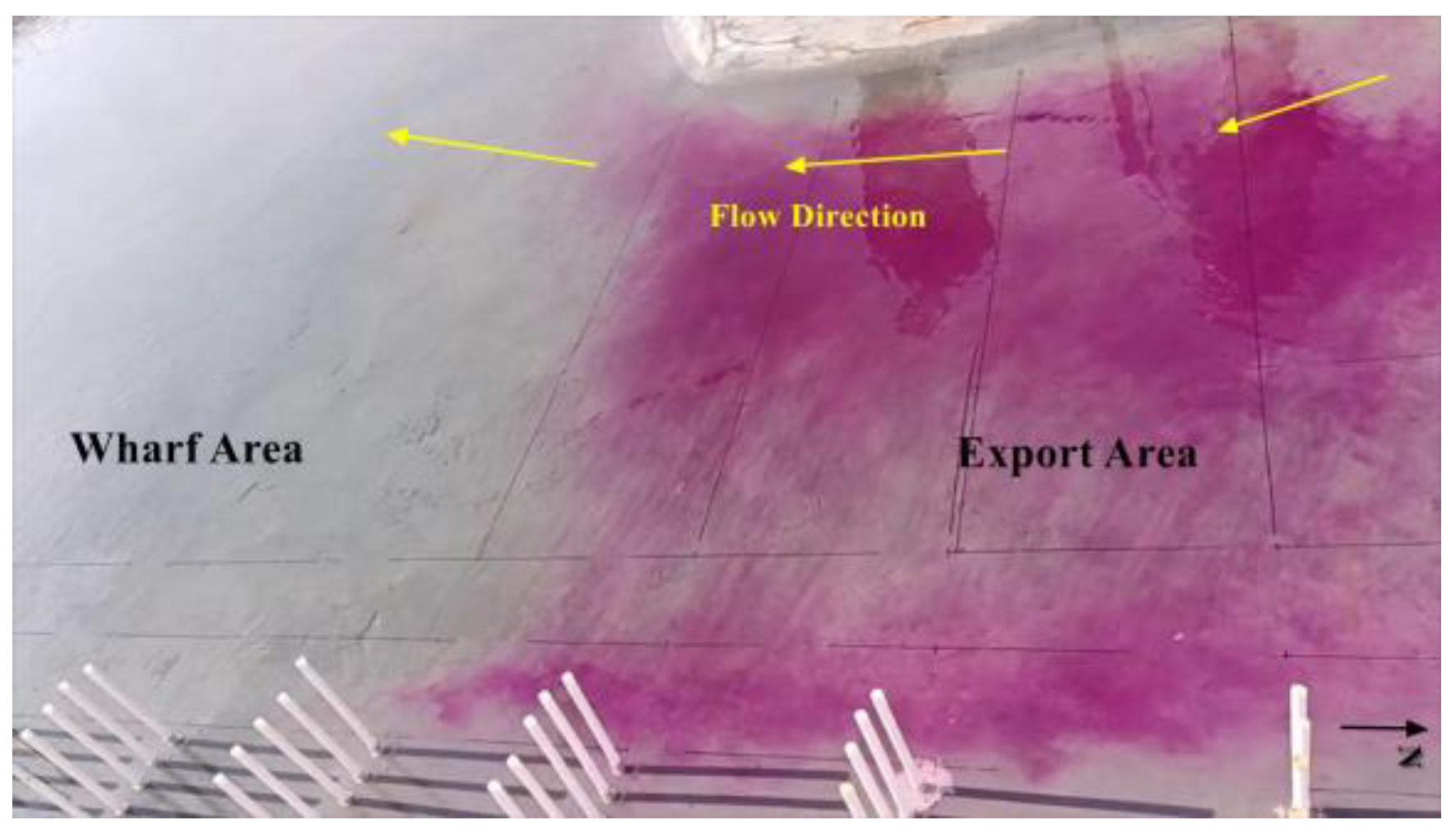
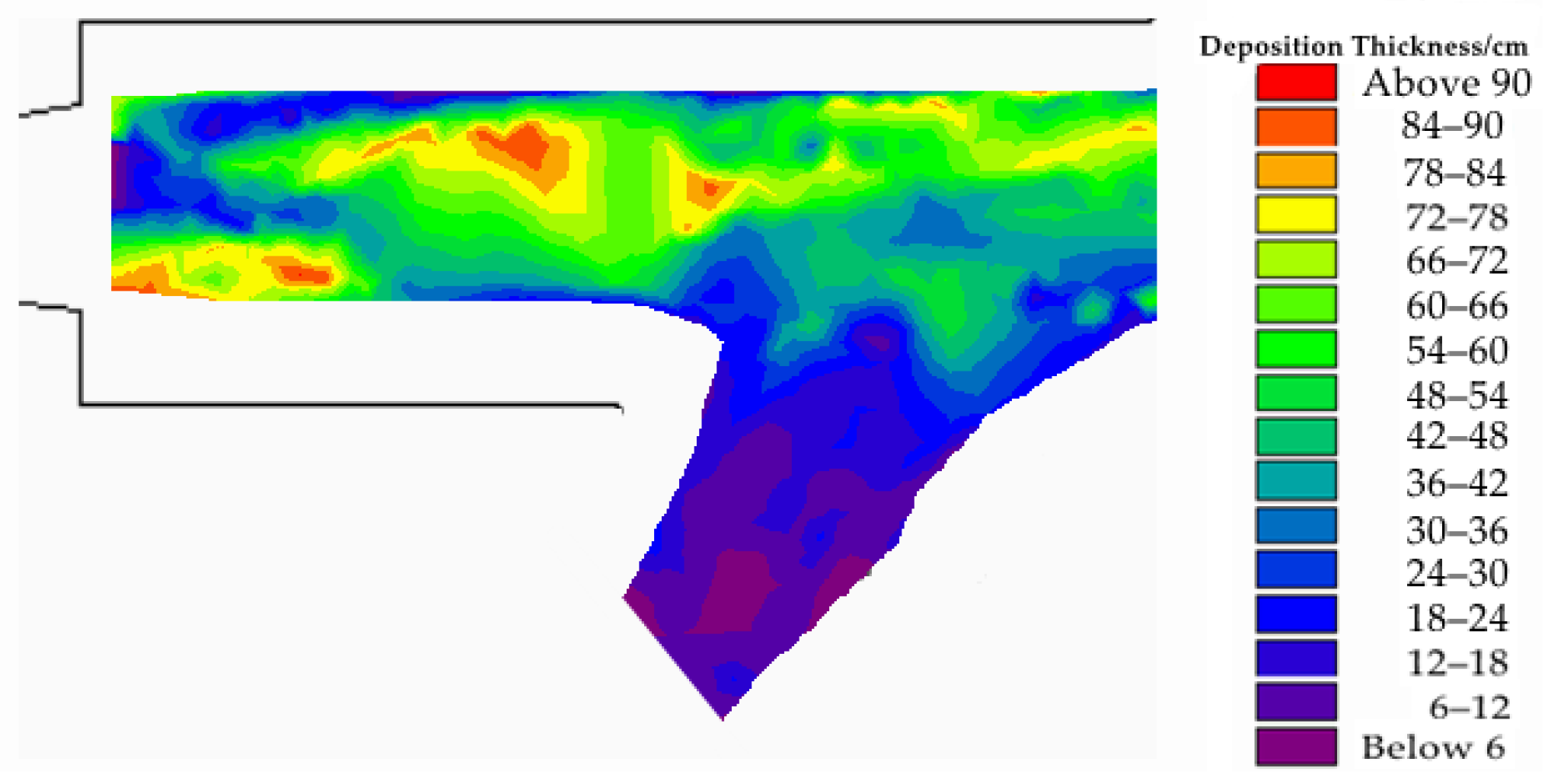
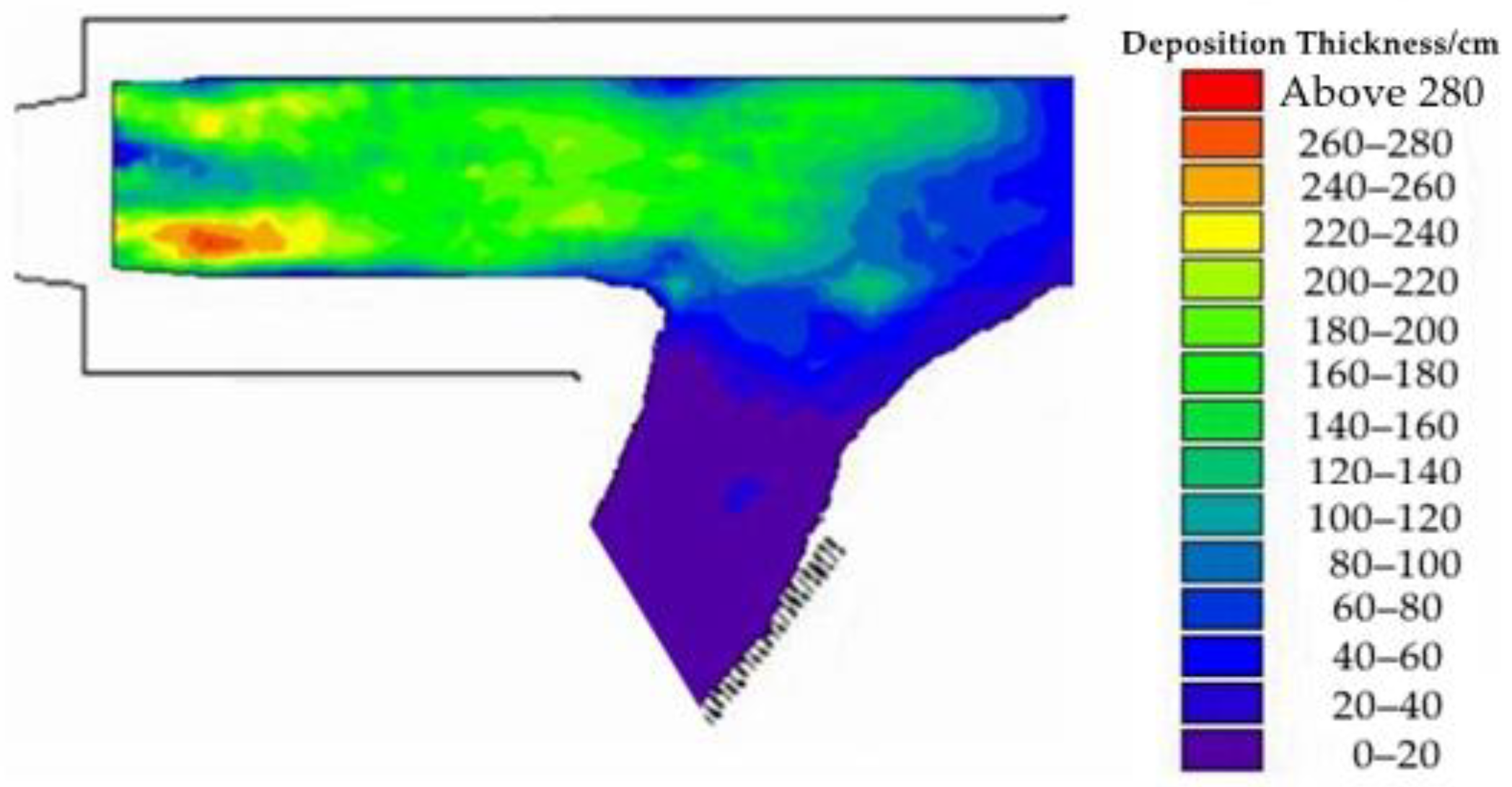
The wharf area and the intersection area are far away from the regulating gate, and the scheduling form of the gate has little effect on the flow velocity distribution in the entrance area to the wharf. From the experimental observation of each working condition, it can be found that there are obvious dynamic and static separation areas in the intersection area between the wharf outlet and the downstream of the regulating gate. The mainstream is distributed in the downstream section of the regulating gate and near the middle partition wall separated from the lock; while the flow velocity at the wharf outlet is small, and the flow velocity under working conditions 4 and 5 is close to 0, the water flow in the wharf area is mostly static. The flow pattern is shown in
Figure 6 and
Figure 7.
It can be seen from the flow pattern diagram that the main flow of the discharge flow from the regulating gate is close to the left side (the middle partition wall of the regulating gate and ship lock). It can be seen from the flow pattern diagram that the main flow of the discharge flow from the regulating gate is close to the left side (the middle partition wall of the control gate ship lock). The tracer near the outlet of the wharf shows that the dynamic of the main river channel and the static of the wharf area are obviously separated. Under each working condition, the wharf area essentially maintains a static water state, and the wharf outlet is close to the right bank, with a small flow rate.
Comprehensive analysis of the flow velocity distribution and flow pattern in each area in the test shows that the flow velocity from the wharf outlet to the downstream near the right bank is small, and the flow pattern is smooth. Under working condition 1, the water level has exceeded the maximum navigable water level, and the ship should be stopped from entering and leaving the wharf; under condition 2–condition 5, ships can meet the requirements of safe navigation in the entrance area of the wharf. Under condition 6, the maximum flow velocity in the intersection area is 0.97 m/s. Under this condition, the ship should increase the navigation distance in and out of the port, reduce the angle with the incoming flow, and sail at a small angle. When the ship is sailing, the lateral flow rate is required not to exceed 0.3 m/s, otherwise the lateral thrust is too large and safety accidents are prone to occur. According to
Table 8, it can be seen that the velocity of the three measuring points on measuring lines ➅➆➇ of section No. 17 is relatively small, so the planned route should be as close as possible to section No. 17. By calculating, whether the transverse velocity is less than 0.3 m/s, the angle between the route and the water flow direction should not be less than 20° when passing through line ➅, and not less than 39° when passing through line ➆. Therefore, the arrangement of the route is recommended as shown in
Figure 8. At the same time, the route planned according to condition 6 can also meet the navigation requirements of other conditions.