Approximate Solutions of the Boussinesq Equation for Horizontal Unconfined Aquifers During Pure Drainage Phase
Abstract
1. Introduction
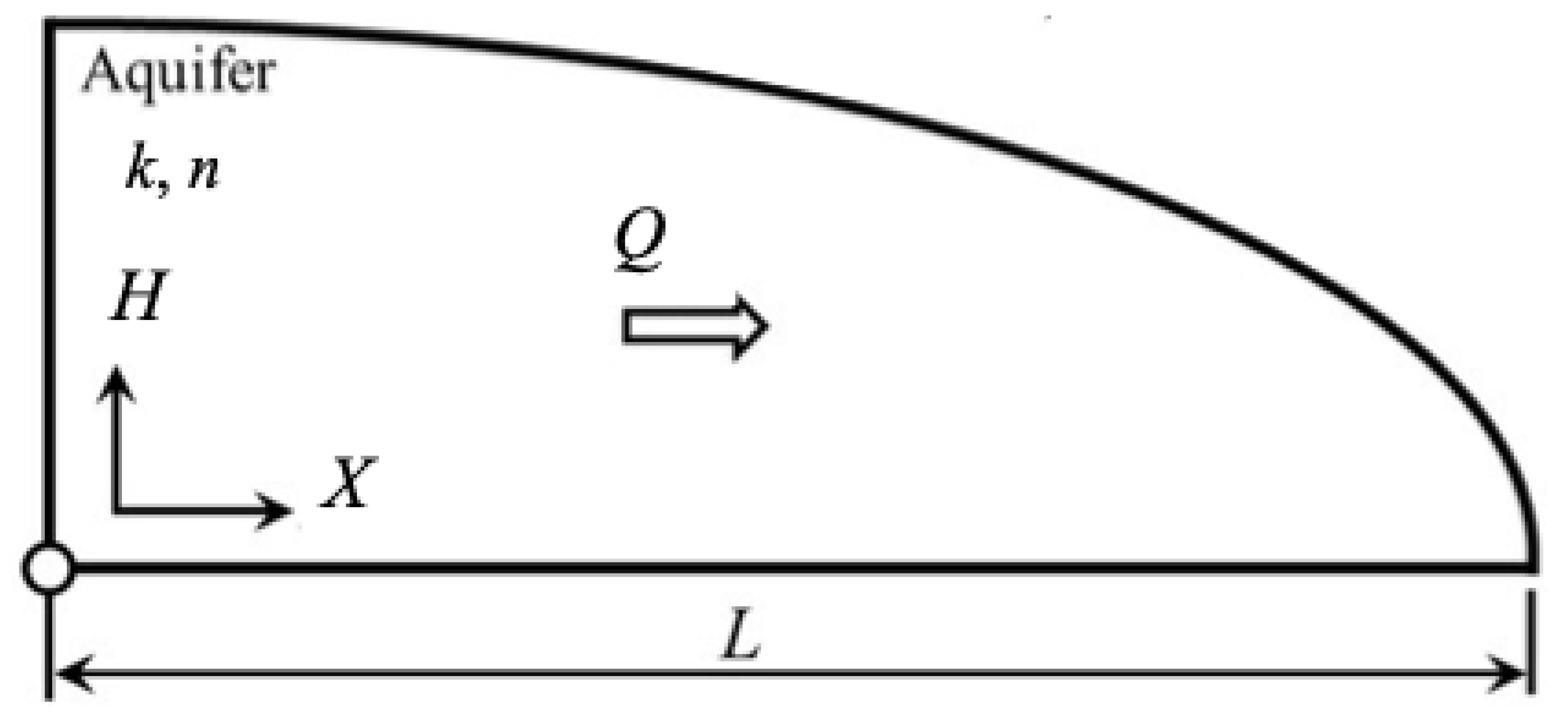
2. Pure Drainage Flow in Horizontal Unconfined Aquifers
2.1. Dimensionless Form of the Boussinesq Equation During Pure Drainage
2.2. Series Expansion and Late-Time Asymptotic Analysis of the Water Table During Pure Drainage
3. A New Model for the Drainage Phase of Horizontal Unconfined Aquifer
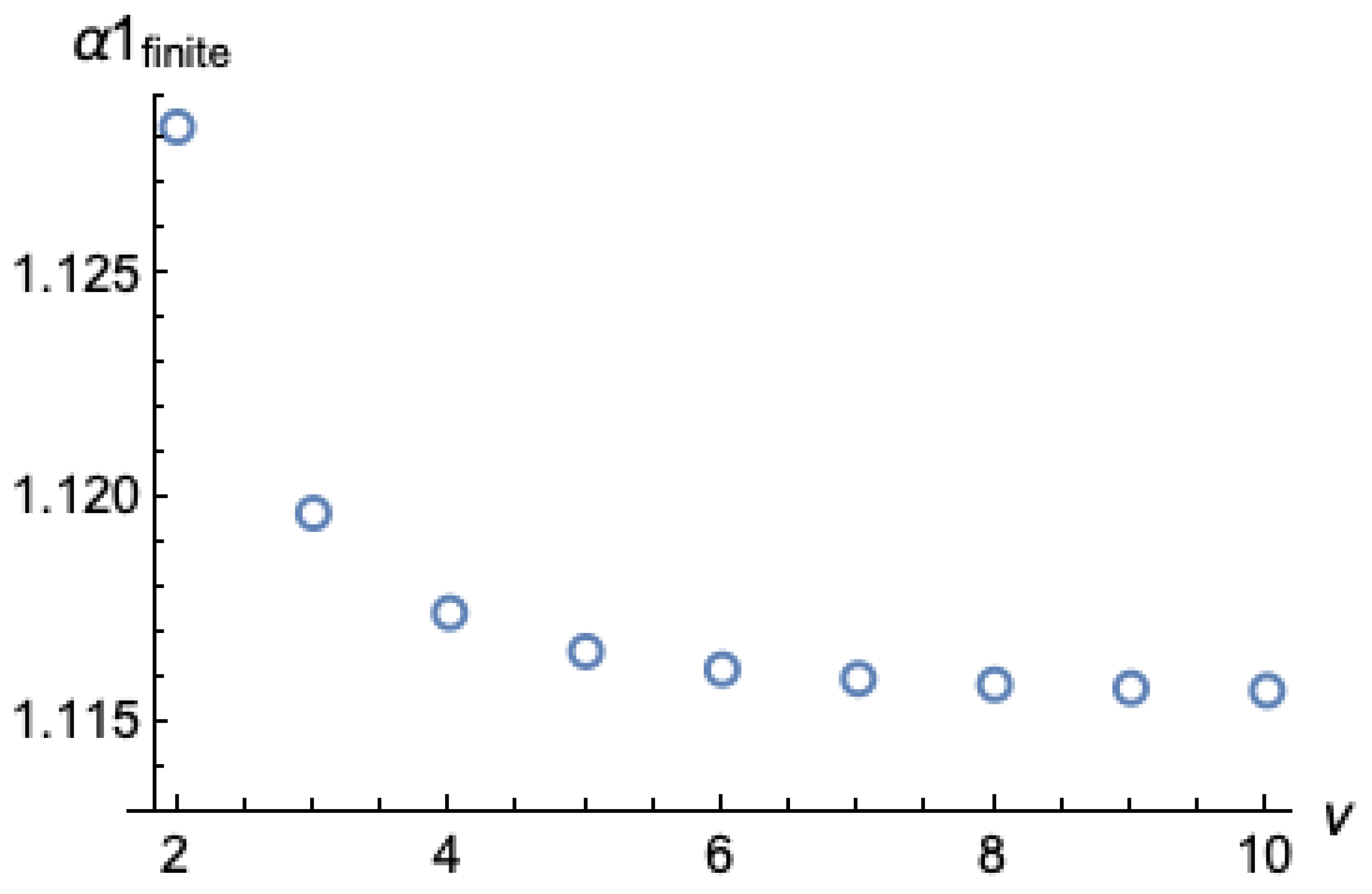
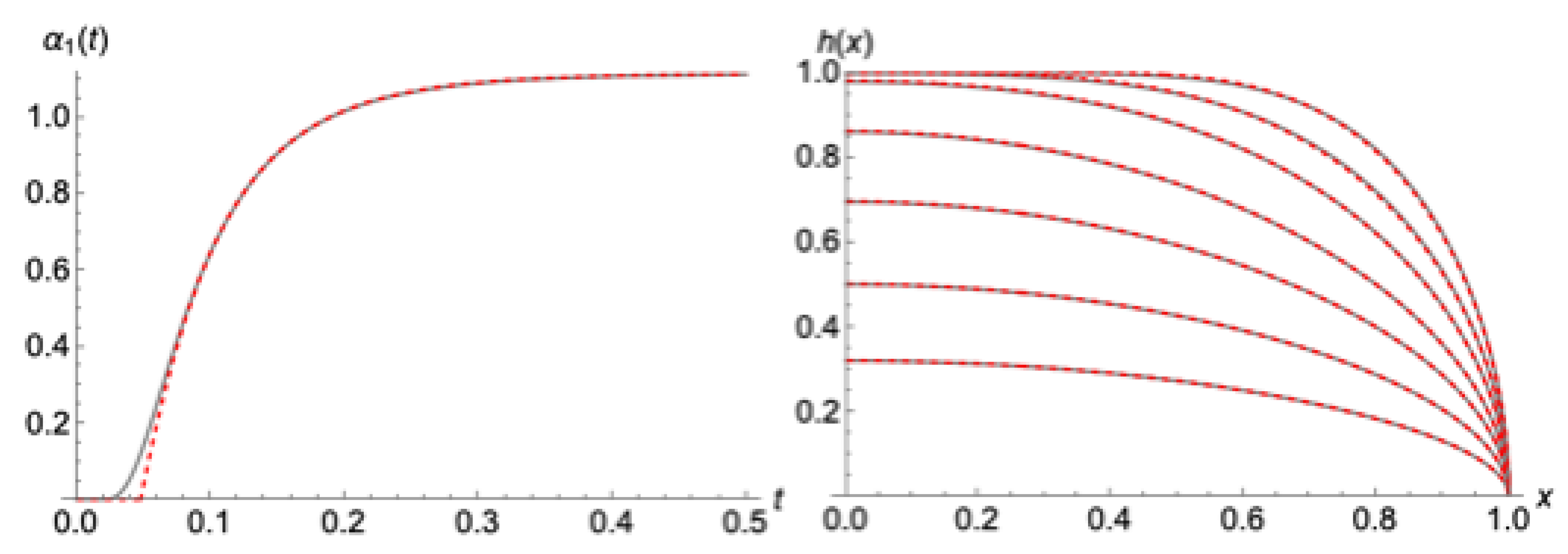
3.1. Analysis and Application of the New Model
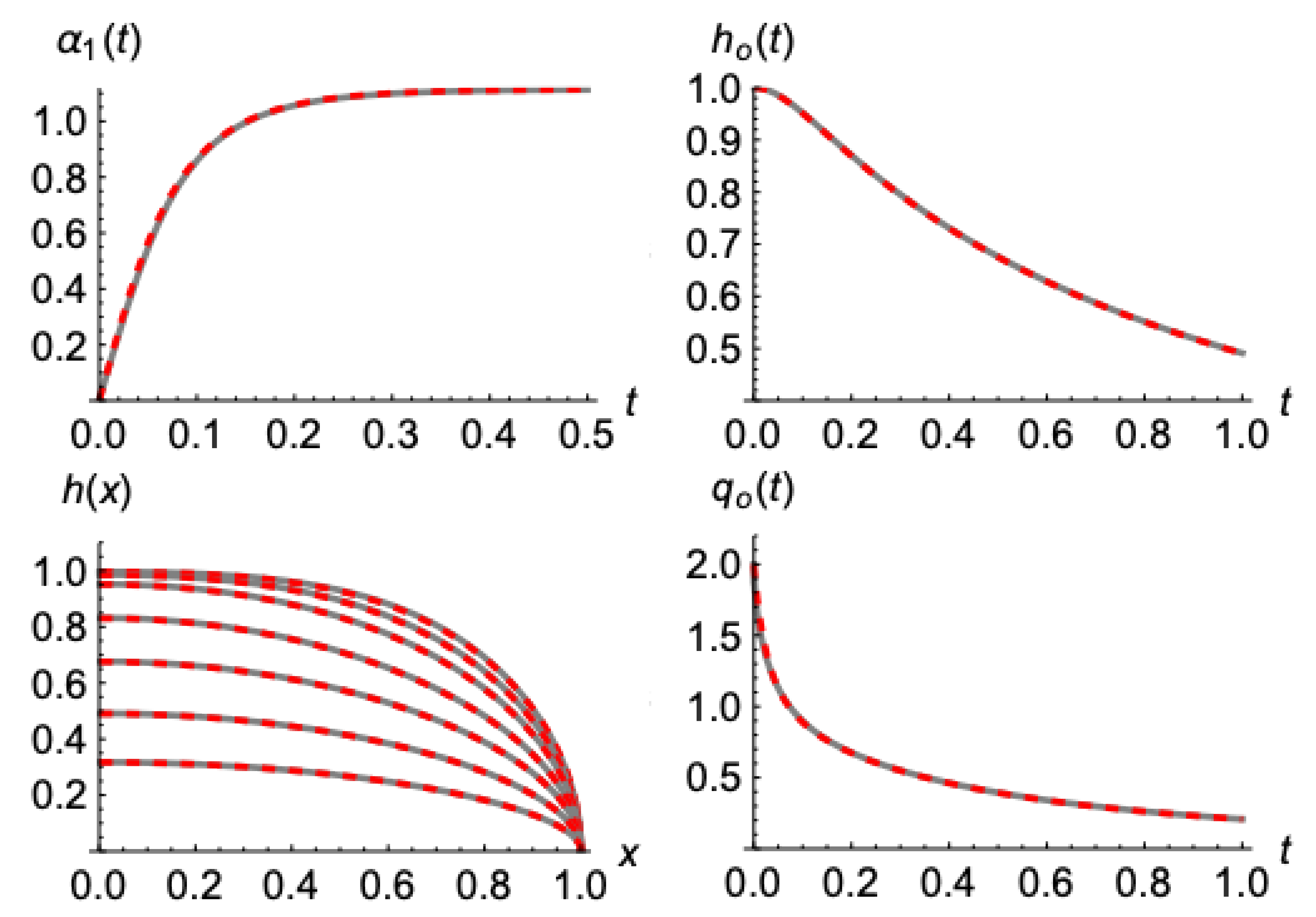
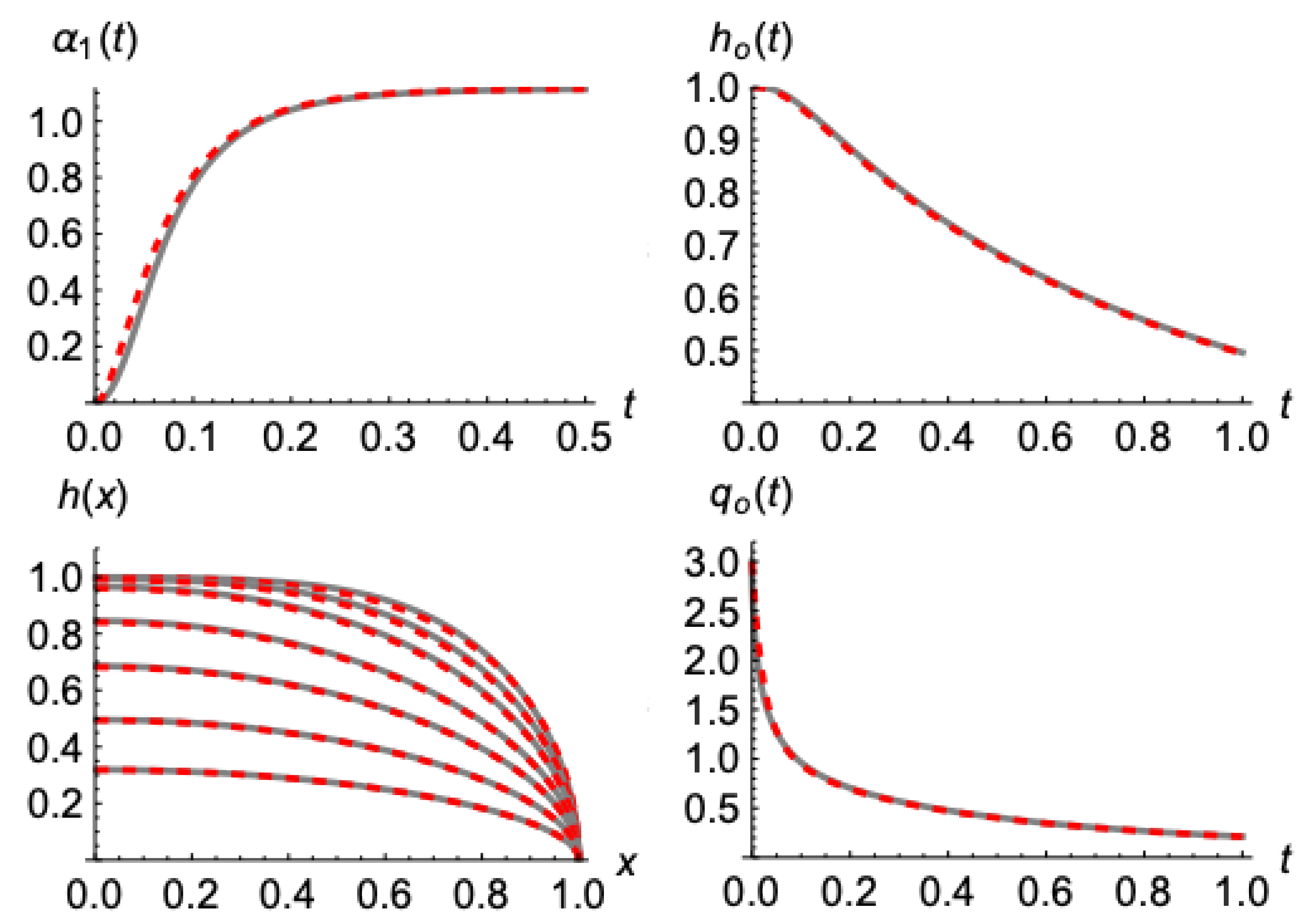
3.2. The Case of a Sudden Drawdown from an Initially Horizontal Water Table
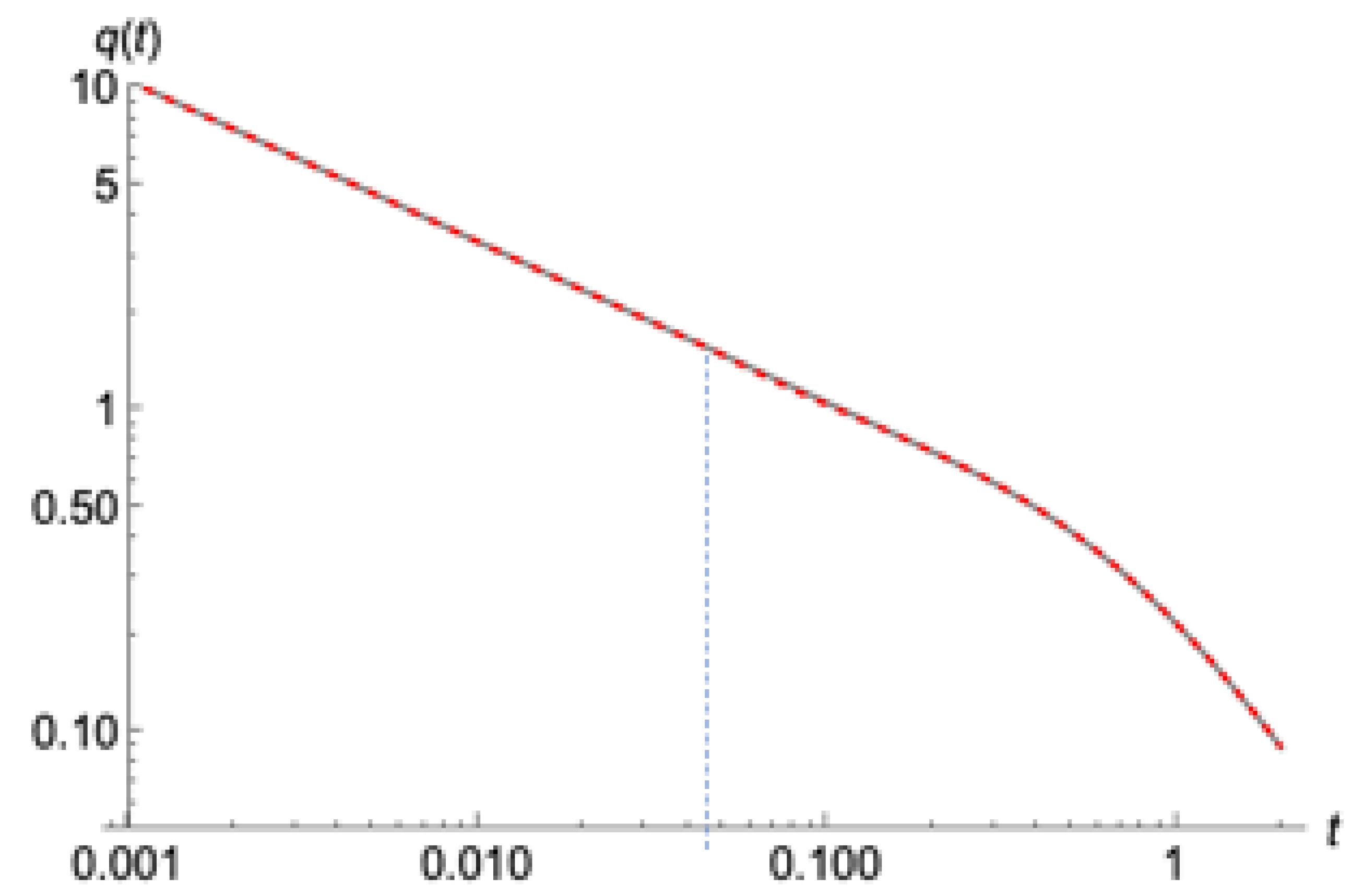
3.2.1. Early Time Approximation
3.2.2. Later Times
3.3. An Analytic Explicit Linear Approximation for the Solution of the New Model
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Boussinesq, J. Essai sur la theorie des eaux courantes du mouvement nonpermanent des eaux souterraines. Acad. Sci. Inst. Fr. 1877, 23, 252–260. [Google Scholar]
- Boussinesq, J. Recherches theoriques sur l’ecoulement des nappes d’eau infiltrees dans le sol et sur debit de sources. J. Math. Pures Appl. 1904, 10, 5–78. [Google Scholar]
- Dupuit, J. Etudes Theoriques et Practiques sur le Mouvement des Eaux dans les Canaux Decouverts et a Travers les Terrains Permeables, 2nd ed.; Dunod: Paris, France, 1863. [Google Scholar]
- Forchheimer, P. Über die Ergiebigkeit von Brunnen-Anlagen und Sickerschlitzen. Z. Architekt. Ing.-Ver. Hann. 1886, 32, 539–563. [Google Scholar]
- Wooding, R.A.; Chapman, T.G. Groundwater flow over a sloping impermeable layer: 1. Application of the Dupuit-Forchheimer assumption. J. Geophys. Res. 1966, 71, 2895–2902. [Google Scholar] [CrossRef]
- Barenblatt, G.I. On some unsteady fluid and gas motions in a porous medium. J. Appl. Math. Mech. 1952, 16, 67–78. [Google Scholar]
- Polubarinova-Kochina, P.Y. Theory of Ground Water Movement; Princeton University Press: Princeton, NJ, USA, 1962. [Google Scholar]
- Barenblatt, G.I.; Entov, V.M.; Ryzhik, V.M. Theory of Fluid Flows through Natural Rocks; Kluwer Academic Publishers: Dordrecht, The Netherlands, 1990. [Google Scholar]
- Chen, Z.X.; Bodvarsson, G.S.; Witherspoon, P.A.; Yortsos, Y.C. An integral equation formulation for the unconfined flow of groundwater with variable inlet conditions. Trans. Porous Media 1995, 18, 15–36. [Google Scholar] [CrossRef]
- Lockington, D.A.; Parlange, J.Y.; Parlange, M.B.; Selker, J. Similarity solution of the Boussinesq equation. Adv. Water Resour. 2000, 23, 725–729. [Google Scholar] [CrossRef][Green Version]
- Parlange, J.Y.; Hogarth, W.L.; Govindaraju, R.S.; Parlange, M.B.; Lockington, D. On an exact analytical solution of the Boussinesq equation. Trans. Porous Media 2000, 39, 339–345. [Google Scholar] [CrossRef]
- Telyakovskiy, A.S.; Braga, G.A.; Furtado, F. Approximate similarity solutions to the Boussinesq equation. Adv. Water Resour. 2002, 25, 191–194. [Google Scholar] [CrossRef]
- Pistiner, A. Similarity solution to unconfined flow in an aquifer. Trans. Porous Media 2008, 71, 265–272. [Google Scholar] [CrossRef]
- Moutsopoulos, N. Solutions of the Boussinesq equation subject to a nonlinear Robin boundary condition. Water Resour. Res. 2013, 49, 7–18. [Google Scholar] [CrossRef]
- Basha, H.A. Traveling wave solution of the Boussinesq equation for groundwater flow in horizontal aquifers. Water Resour. Res. 2013, 49, 1668–1679. [Google Scholar] [CrossRef]
- Basha, H.A. Perturbation solutions of the Boussinesq equation for horizontal flow in finite and semi-infinite aquifers. Adv. Water Resour. 2021, 155, 104016. [Google Scholar] [CrossRef]
- Chor, T.; Ruiz de Zarate, A.; Dias, N.L. A generalized series solution for the Boussinesq equation with constant boundary conditions. Water Resour. Res. 2019, 55, 3567–3575. [Google Scholar] [CrossRef]
- Chor, T.; Dias, N.L.; Ruiz de Zárate, A. An exact series and improved numerical and approximate solutions for the Boussinesq equation. Water Resour. Res. 2013, 49, 7380–7387. [Google Scholar] [CrossRef]
- Tzimopoulos, C.; Papadopoulos, K.; Papadopoulos, B.; Samarinas, N.; Evangelides, C. Fuzzy solution of nonlinear Boussinesq equation. J. Hydroinformatics 2022, 24, 1127–1147. [Google Scholar] [CrossRef]
- Hayek, M. A simple and accurate closed-form analytical solution to the Boussinesq equation for horizontal flow. Adv. Water Resour. 2024, 185, 104628. [Google Scholar] [CrossRef]
- Tzimopoulos, C.; Samarinas, N.; Papadopoulos, K.; Evangelides, C. Fuzzy Analytical Solution for the Case of a semi-Infinite Unconfined Aquifer. Environ. Sci. Proc. 2023, 25, 70. [Google Scholar] [CrossRef]
- Ceretani, A.; Falcini, F.; Garra, R. Exact solutions for the fractional nonlinear Boussinesq equation. In Proceedings of the INdAM Workshop on Fractional Differential Equations: Modeling, Discretization, and Numerical Solvers, Singapore, 8 March 2023; Springer Nature: Singapore, 2023. [Google Scholar]
- Animasaun, I.L.; Shah, N.A.; Wakif, A.; Mahanthesh, B.; Sivaraj, R.; Koriko, O.K. Ratio of Momentum Diffusivity to Thermal Diffusivity: Introduction, Meta-analysis, and Scrutinization; Chapman and Hall/CRC: New York, NY, USA, 2022; ISBN 13: 978-1032108520/10: 1032108525/9781003217374. [Google Scholar] [CrossRef]
- Akylas, E.; Gravanis, E.; Koussis, A.D. Quasi-steady flow in sloping aquifers. Water Resour. Res. 2015, 51, 9165–9181. [Google Scholar] [CrossRef][Green Version]
- Gravanis, E.; Akylas, E.; Sarris, E. Approximate Solutions for Horizontal Unconfined Aquifers in the Buildup Phase. Water 2024, 16, 1031. [Google Scholar] [CrossRef]
- Gravanis, E.; Akylas, E. Early-time solution of the horizontal unconfined aquifer in the buildup phase. Water Resour. Res. 2017, 53, 8310–8326. [Google Scholar] [CrossRef]
- Lockington, D.A. Response of unconfined aquifer to sudden change in boundary head. J. Irrig. Drain. Eng. 1997, 123, 24–27. [Google Scholar] [CrossRef]
- Parlange, J.Y.; Stagnitti, F.; Heilig, A.; Szilagyi, J.; Parlange, M.B.; Steenhuis, T.S.; Hogarth, W.L.; Barry, D.A.; Li, L. Sudden drawdown and drainage of a horizontal aquifer. Water Resour. Res. 2001, 37, 2097–2101. [Google Scholar] [CrossRef]
- Akylas, E.; Koussis, A.D. Response of sloping unconfined aquifer to stage changes in adjacent stream I. Theoretical analysis and derivation of system response functions. J. Hydrol. 2007, 338, 85–95. [Google Scholar] [CrossRef]
- Koussis, A.D.; Akylas, E.; Mazi, K. Response of sloping unconfined aquifer to stage changes in adjacent stream II. Applications. J. Hydrol. 2007, 338, 73–84. [Google Scholar] [CrossRef]
- Koussis, A.D.; Akylas, E. Slug Test in Confined Aquifers, the Over-Damped Case: Quasi-Steady Flow Analysis. Groundwater 2012, 50, 608–613. [Google Scholar] [CrossRef]
- Moutsopoulos, K.N. The analytical solution of the Boussinesq equation for flow induced by a step change of the water table elevation revisited. Trans. Porous Med. 2010, 85, 919–940. [Google Scholar] [CrossRef]
- Jiang, Q.; Tang, Y. A general approximate method for the groundwater response problem caused by water level variation. J. Hydrol. 2015, 529, 398–409. [Google Scholar] [CrossRef]



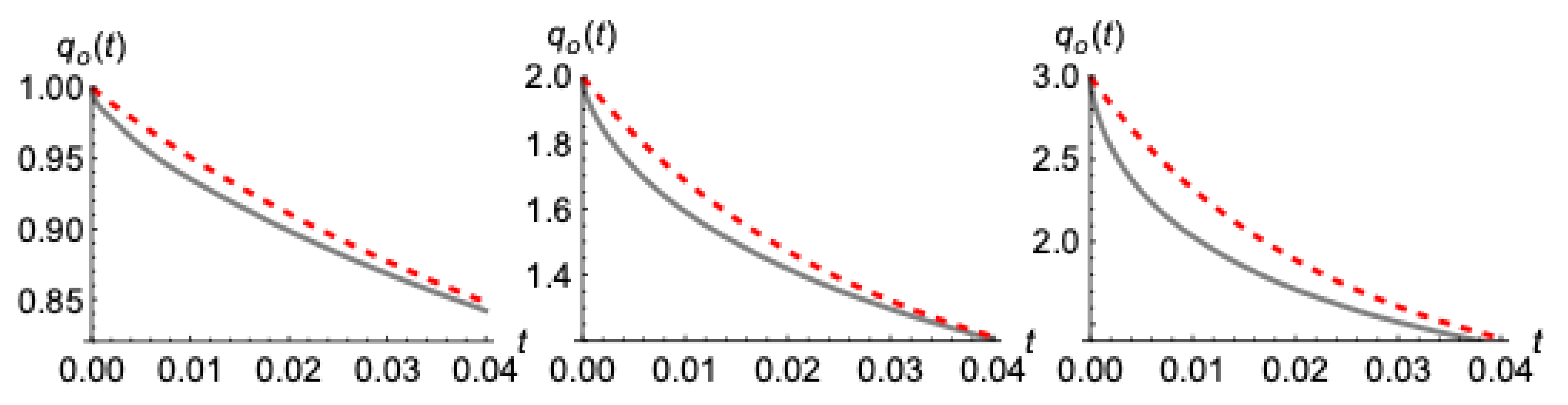

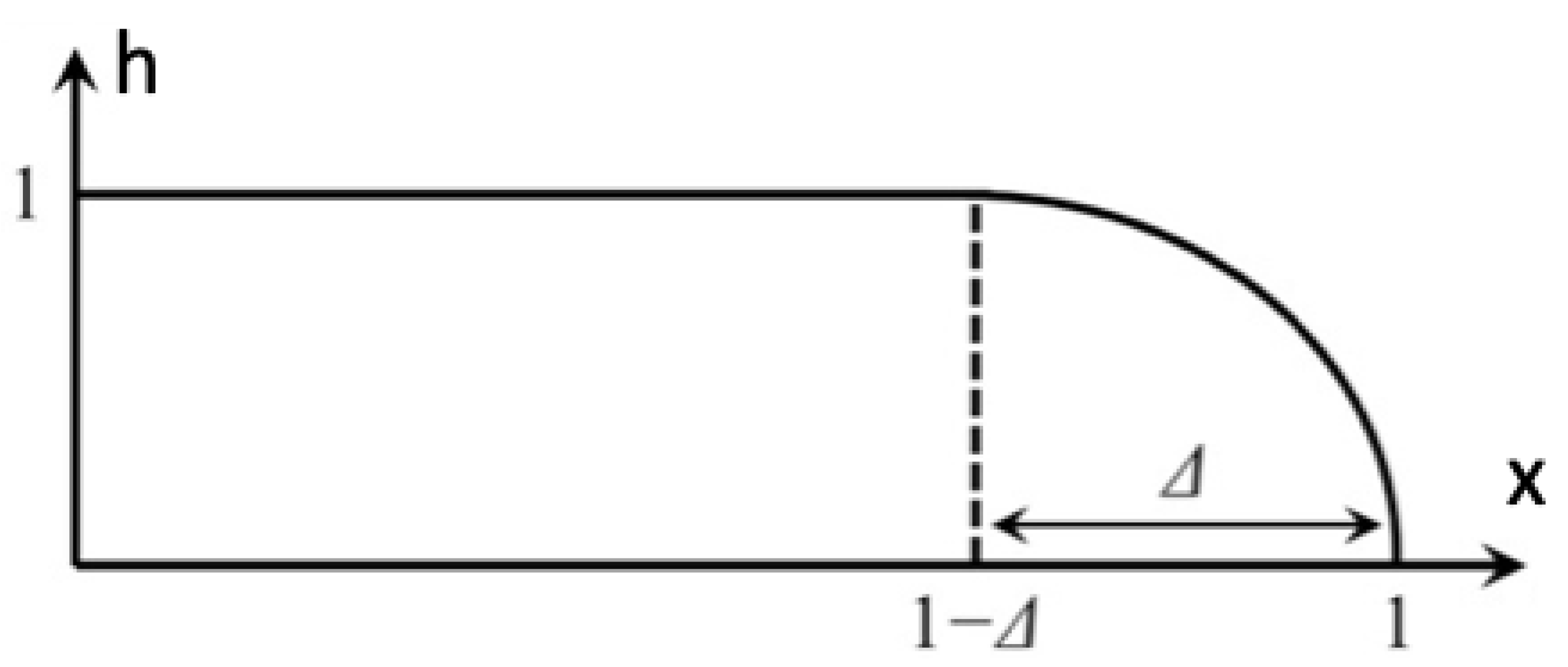
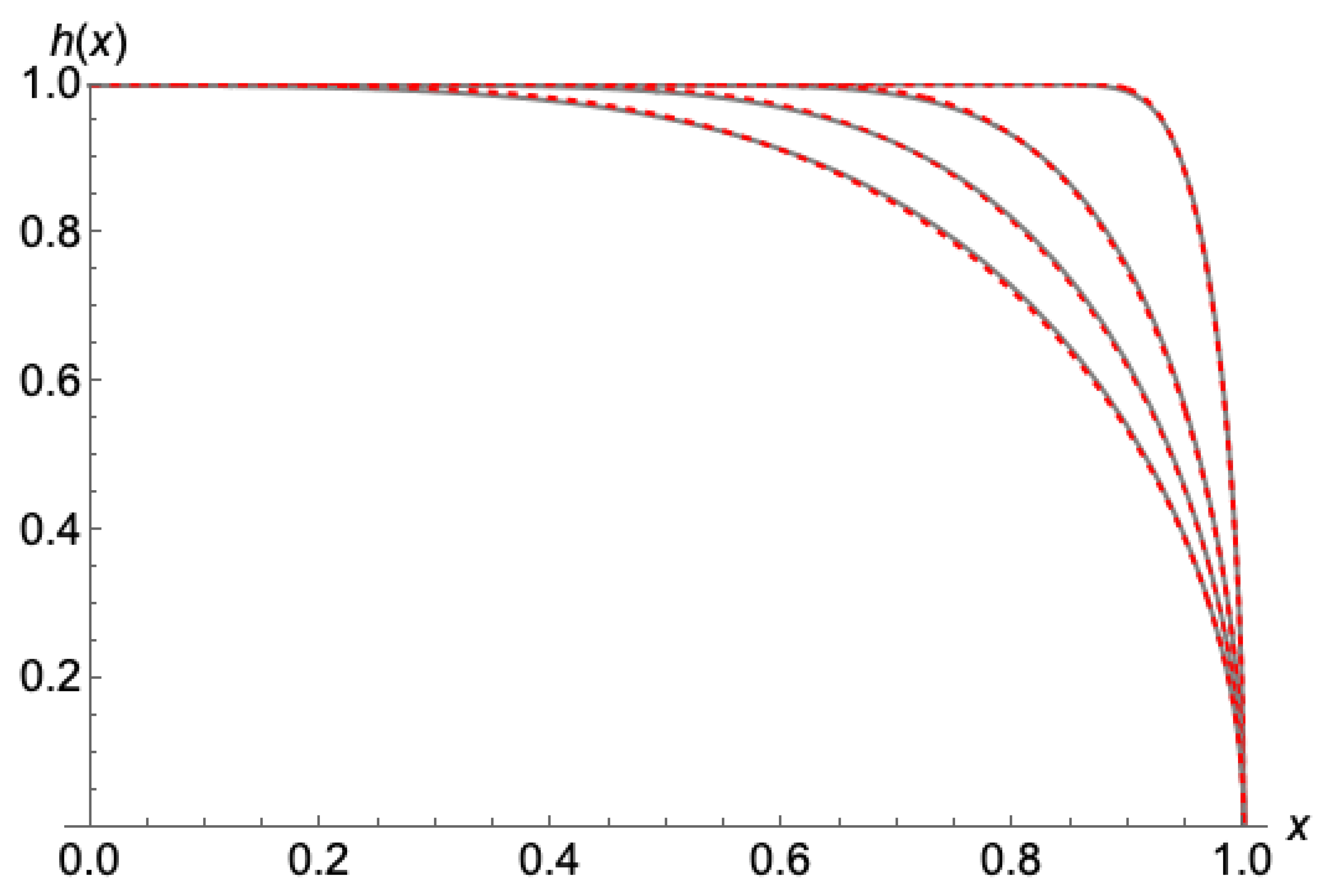
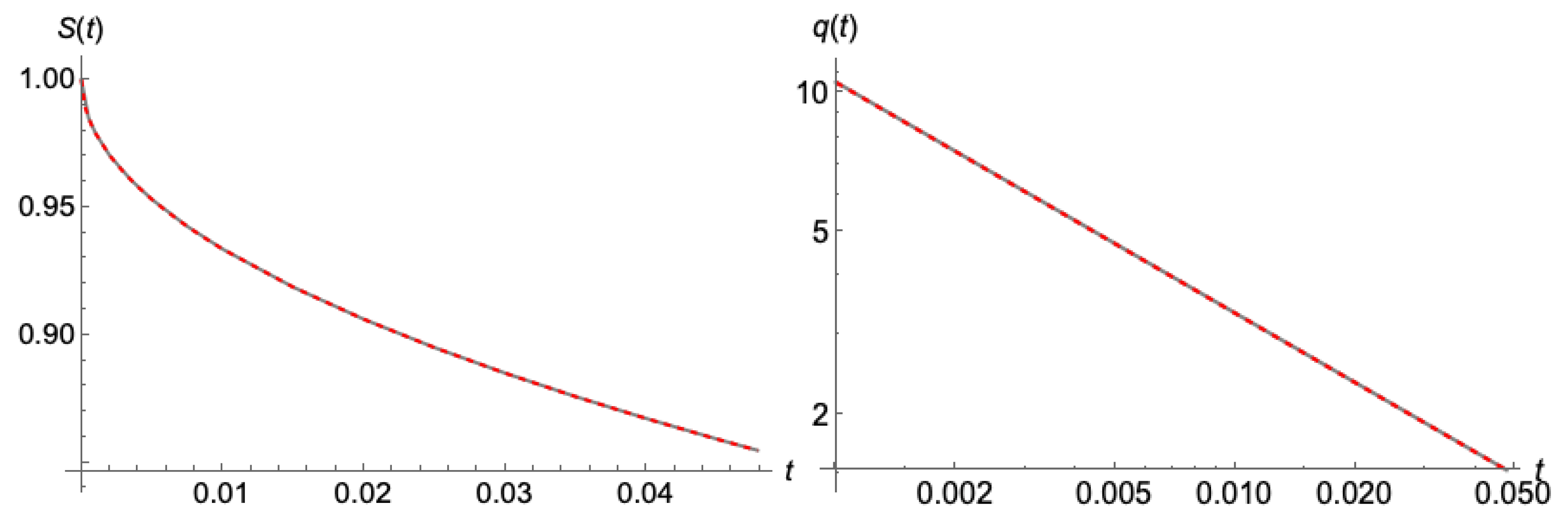

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Akylas, E.; Gravanis, E. Approximate Solutions of the Boussinesq Equation for Horizontal Unconfined Aquifers During Pure Drainage Phase. Water 2024, 16, 2984. https://doi.org/10.3390/w16202984
Akylas E, Gravanis E. Approximate Solutions of the Boussinesq Equation for Horizontal Unconfined Aquifers During Pure Drainage Phase. Water. 2024; 16(20):2984. https://doi.org/10.3390/w16202984
Chicago/Turabian StyleAkylas, Evangelos, and Elias Gravanis. 2024. "Approximate Solutions of the Boussinesq Equation for Horizontal Unconfined Aquifers During Pure Drainage Phase" Water 16, no. 20: 2984. https://doi.org/10.3390/w16202984
APA StyleAkylas, E., & Gravanis, E. (2024). Approximate Solutions of the Boussinesq Equation for Horizontal Unconfined Aquifers During Pure Drainage Phase. Water, 16(20), 2984. https://doi.org/10.3390/w16202984





