Ensuring the Safety of an Extraction Well from an Upgradient Point Source of Pollution in a Computationally Constrained Setting
Abstract
1. Introduction
2. Materials and Methods
2.1. Conceptual Framework
- Regional flow is steady, e.g., the direction and the magnitude of the regional flow do not vary seasonally or with precipitation;
- The shallow aquifer is homogeneous and isotropic in the x- and y-directions;
- Flow can be considered as two-dimensional (depth-integrated);
- The extraction rate of the well is constant over time;
- The rate at which the point source introduces water into the aquifer (which we will hereafter call the hydraulic loading rate) is constant over time.
2.2. Mathematical Model and Non-Dimensionalization
2.3. Exact Determination of for Specified Values of α and
2.4. Development of Approximate Analytical Equations = f () and ymin = f (xps)
- A simple-but-accurate approximate analytical solution will be deployable by constituencies that do not have adequate computer access to implement the exact solution;
- A suitably simple analytical equation explicitly articulates the dependence of on and α, whereas the “exact” procedure does not. Thus, the analytical equation may yield insights into the physics of the problem and/or may enable estimation of important conditions or criteria;
- As will be seen subsequently, an analytical equation for can be applied to a well-known problem in groundwater hydraulics, enabling a closed-form solution to a problem that previously relied on the solution of a transcendental equation.
3. Results
3.1. Exact Values of for Specified Values of α and
3.2. Closed-Form Approximation for
3.3. Dimensional Form of the Approximate Analytical Equation
4. Discussion
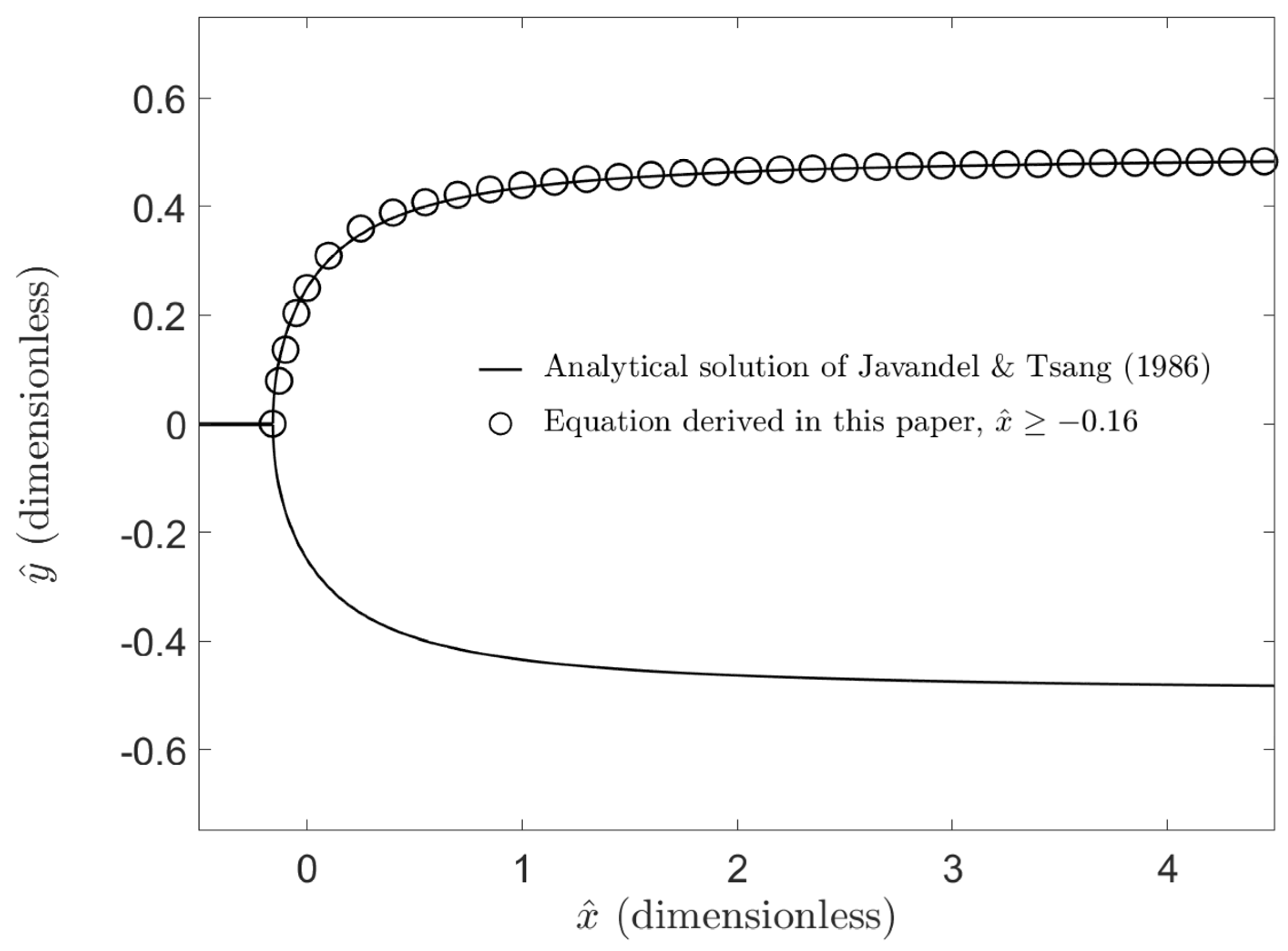
4.1. Application to the Results of Javandel and Tsang [19]
4.2. Comparison with the Results of Cunningham and Reinhard [22]
4.3. Comparison with the Results of Zhang and Wang [24]
4.4. Application of the Methodology and Equations Presented Herein
4.5. Limitations to This Analysis
- Application of Equation (9) or Equation (11) requires the user to have reliable estimates of the magnitude and direction of regional groundwater flow. This knowledge might not be readily available in some contexts;
- Equations are derived by assuming that both the magnitude and direction of the regional flow are steady over time, e.g., any seasonal variations in the regional flow must be small. In some situations, particularly for shallow unconfined aquifers such as those considered here, the direction of regional flow can vary significantly over time. In these situations, Equations (9) and (11) could still be applied to assess the risk of well contamination, but the equations would need to be applied for each of the most “extreme” possible flow directions to ensure that the well is protected regardless of the flow direction;
- The injection rate of the point source and the extraction rate of the well are assumed to be steady in time. This is probably not realistic. However, this limitation can be overcome by assuming that the point source and the well always operate at or near their maximum flow rates. That will result in a conservative estimate of ymin that is protective of health;
- The hydraulic conductivity in the vicinity of the well and the point source are assumed to be sufficiently homogeneous that heterogeneity has negligible effects on the flow field. In environments that exhibit high degrees of heterogeneity even at small scales, the equations derived here might not apply;
- The equations derived here account only for a single point source, a single extraction well, and regional groundwater flow. Therefore, any other features that affect the local flow field (e.g., recharge zones, physical barriers to flow, additional wells) would have to be sufficiently far away that they do not impact the groundwater flow in the vicinity of the point source and the extraction well.
5. Conclusions
- For constituencies with adequate computer resources, we have presented a computer-based method capable of finding ymin exactly (to within the tolerance of a computer-based root-finding algorithm);
- For constituencies lacking reliable access to computing resources, we have presented a simple approximate closed-form expression for ymin;
- The simple algebraic solution for ymin was shown to provide an excellent approximation to the exact solution. The average relative error of the approximate solution is less than 3% for the conditions we considered. For a subset of scenarios in which the point source is sufficiently far upgradient of the well (n = 77), the root mean square relative error of the approximate solution is only 0.52%;
- We found that ymin depends on the upgradient separation distance xps between the point source and the latrine, as well as a length parameter that we call L. The length parameter L is easily computed from the hydraulic loading rate of the point source, the extraction rate of the well, and the regional groundwater flow rate, as shown in Equation (12);
- If ymin > L/2, then the well and the point source are guaranteed to be hydraulically disconnected regardless of the upgradient separation distance xps;
- The methodology and equations presented here enable the siting of a proposed well or a proposed point source at a location that protects the quality of the water extracted by the well and hence protects the health of the users of the well.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A. MATLAB® Codes for Constituencies with Necessary Computer Access

Appendix B. Comparison of Computer-Based and Equation-Based Estimates of
| α = 0 | α = 0.1 | |||
|---|---|---|---|---|
| Exact (Computer-Based) Value of | Analytical Estimate of | Exact (Computer-Based) Value of | Analytical Estimate of | |
| 0 | 0.2500 | 0.2500 | 0.2906 | 0.2750 |
| 0.25 | 0.3489 | 0.3596 | 0.3836 | 0.3892 |
| 0.5 | 0.3938 | 0.4024 | 0.4298 | 0.4364 |
| 1 | 0.4347 | 0.4394 | 0.4742 | 0.4784 |
| 1.5 | 0.4533 | 0.4560 | 0.4951 | 0.4977 |
| 2 | 0.4637 | 0.4655 | 0.5071 | 0.5088 |
| 3 | 0.4750 | 0.4759 | 0.5202 | 0.5211 |
| 4 | 0.4810 | 0.4815 | 0.5272 | 0.5278 |
| 5 | 0.4845 | 0.4850 | 0.5316 | 0.5319 |
| 7 | 0.4889 | 0.4891 | 0.5367 | 0.5368 |
| 10 | 0.4913 | 0.4922 | 0.5406 | 0.5406 |
| α = 0.2 | α = 0.5 | |||
|---|---|---|---|---|
| Exact (Computer-Based) Value of | Analytical Estimate of | Exact (Computer-Based) Value of | Analytical Estimate of | |
| 0 | 0.3278 | 0.3000 | 0.4285 | 0.3750 |
| 0.25 | 0.4176 | 0.4183 | 0.5155 | 0.5034 |
| 0.5 | 0.4652 | 0.4697 | 0.5680 | 0.5663 |
| 1 | 0.5130 | 0.5168 | 0.6262 | 0.6284 |
| 1.5 | 0.5363 | 0.5389 | 0.6569 | 0.6591 |
| 2 | 0.5499 | 0.5517 | 0.6757 | 0.6774 |
| 3 | 0.565 | 0.5660 | 0.6972 | 0.6983 |
| 4 | 0.5732 | 0.5737 | 0.7091 | 0.7098 |
| 5 | 0.5782 | 0.5786 | 0.7167 | 0.7172 |
| 7 | 0.5842 | 0.5844 | 0.7257 | 0.7259 |
| 10 | 0.5888 | 0.5889 | 0.7327 | 0.7328 |
| α = 1 | α = 2 | |||
|---|---|---|---|---|
| Exact (Computer-Based) Value of | Analytical Estimate of | Exact (Computer-Based) Value of | Analytical Estimate of | |
| 0 | 0.5792 | 0.5000 | 0.8570 | 0.7500 |
| 0.25 | 0.6691 | 0.6404 | 0.9560 | 0.9050 |
| 0.5 | 0.7299 | 0.7193 | 1.0311 | 1.0068 |
| 1 | 0.8050 | 0.8049 | 1.1360 | 1.1327 |
| 1.5 | 0.8486 | 0.8505 | 1.2046 | 1.2073 |
| 2 | 0.8767 | 0.8788 | 1.2524 | 1.2568 |
| 3 | 0.9105 | 0.9121 | 1.3139 | 1.3182 |
| 4 | 0.9299 | 0.9310 | 1.3514 | 1.3548 |
| 5 | 0.9424 | 0.9433 | 1.3765 | 1.3792 |
| 7 | 0.9577 | 0.9581 | 1.4079 | 1.4095 |
| 10 | 0.9697 | 0.9699 | 1.4334 | 1.4343 |
| 15 | 0.9795 | 0.9795 | 1.4545 | 1.4549 |
| 20 | 1.4654 | 1.4656 | ||
| 25 | 1.4721 | 1.4723 | ||
| 30 | 1.4767 | 1.4767 | ||
| α = 5 | α = 10 | |||
|---|---|---|---|---|
| Exact (Computer-Based) Value of | Analytical Estimate of | Exact (Computer-Based) Value of | Analytical Estimate of | |
| 0 | 1.6392 | 1.5000 | 2.9064 | 2.7500 |
| 0.25 | 1.7568 | 1.6728 | 3.0383 | 2.9324 |
| 0.5 | 1.8577 | 1.8099 | 3.1585 | 3.0920 |
| 1 | 2.0211 | 2.0137 | 3.3698 | 3.3584 |
| 1.5 | 2.1469 | 2.1579 | 3.5491 | 3.5717 |
| 2 | 2.2462 | 2.2653 | 3.7030 | 3.7464 |
| 3 | 2.3917 | 2.4146 | 3.9526 | 4.0153 |
| 4 | 2.4922 | 2.5135 | 4.1455 | 4.2128 |
| 5 | 2.5652 | 2.5838 | 4.2984 | 4.3638 |
| 7 | 2.6636 | 2.6771 | 4.5236 | 4.5798 |
| 10 | 2.7497 | 2.7584 | 4.7420 | 4.7840 |
| 15 | 2.8251 | 2.8298 | 4.9510 | 4.9773 |
| 20 | 2.8658 | 2.8686 | 5.0709 | 5.0884 |
| 25 | 2.8912 | 2.8930 | 5.1482 | 5.1606 |
| 30 | 2.9085 | 2.9098 | 5.2021 | 5.2112 |
References
- United Nations World Water Development Report (UN WWDR). Groundwater: Making the Invisible Visible; United Nations Educational, Scientific, and Cultural Organization (UNESCO): Paris, France, 2022. [Google Scholar]
- Smits, S.; Sutton, S. Self Supply: The Case for Leveraging Greater Household Investment in Water Supply; IRC: The Hague, The Netherlands, 2015. [Google Scholar]
- Butterworth, J.; Sutton, S.; Mekonta, L. Self-supply as a complementary water services delivery model in Ethiopia. Water Altern. 2013, 6, 405–423. [Google Scholar]
- MacCarthy, M.F.; Annis, J.E.; Mihelcic, J.R. Unsubsidized self-supply in eastern Madagascar. Water Altern. 2013, 6, 424–438. [Google Scholar]
- Marshall, K.C. An evaluation of the water lifting limit of a manually operated suction pump: Model estimation and laboratory assessment. Master’s Thesis, University of South Florida, Tampa, FL, USA, 2017. [Google Scholar]
- Sorensen, J.P.R.; Lapworth, D.J.; Nkhuwa, D.C.W.; Stuart, M.E.; Goody, D.C.; Bell, R.A.; Chirwa, M.; Kabika, J.; Liemisa, M.; Chibesa, M.; et al. Emerging contaminants in urban groundwater sources in Africa. Water Res. 2015, 72, 51–63. [Google Scholar] [CrossRef] [PubMed]
- Ouedraogo, I.; Defourney, P.; Vanclooster, M. Mapping the groundwater vulnerability for pollution at the pan African scale. Sci. Total Environ. 2016, 544, 939–953. [Google Scholar] [CrossRef] [PubMed]
- Franceys, R.; Pickford, J.; Reed, R. A Guide to the Development of On-Site Sanitation; World Health Organization: Geneva, Switzerland, 1992. [Google Scholar]
- Orner, K.; Naughton, C.; Stenstrom, T.A. Pit toilets (latrines). In Sanitation and Disease in the 21st Century: Health and Microbiological Aspects of Excreta and Wastewater Management, Global Water Pathogen Project, Part Four, Management of Risk from Excreta and Wastewater; Rose, J.B., Jiménez-Cisneros, J., Eds.; UNESCO: East Lansing, MI, USA, 2018. [Google Scholar] [CrossRef]
- Dillon, K.S.; Corbett, D.R.; Chanton, J.P.; Burnett, W.C.; Kump, L. Bimodal transport of a waste water plume injected into saline ground water of the Florida Keys. Ground Water 2000, 38, 624–634. [Google Scholar] [CrossRef]
- Omrcen, B.; Loncaric, B.; Brkic, V. Waste disposal by injection into deep wells. In Proceedings of the ASME 2001 Engineering Technology Conference on Energy, Houston, TX, USA, 5–7 February 2001. [Google Scholar] [CrossRef]
- Tomiyama, S.; Igarashi, T. The potential threat of mine drainage to groundwater resources. Curr. Opin. Environ. Sci. Health 2022, 27, 100347. [Google Scholar] [CrossRef]
- Mezzacapo, M.; Donohue, M.J.; Smith, C.; El-Kadi, A.; Falinski, K.; Lerner, D.T. Hawaii’s cesspool problem: Review and recommendations for water resources and human health. J. Contemp. Wat. Res. Ed. 2020, 170, 35–75. [Google Scholar] [CrossRef]
- Vroblesky, D.A.; Rhodes, L.C.; Robertson, J.F.; Harrigan, J.A. Locating VOC contamination in a fractured-rock aquifer at the ground-water/surface-water interface using passive vapor collectors. Ground Water 1996, 34, 223–230. [Google Scholar] [CrossRef]
- Kanazawa, K.; Miyaji, N.; Kusaba, T.; Ban, K.; Hayakawa, Y.; Hatano, R. Groundwater pollution by cattle slurry stored in unlined lagoon. JARQ 1999, 33, 7–13. [Google Scholar]
- Nenninger, C.R.; Cunningham, J.A.; Mihelcic, J.R. A historical and critical review of latrine-siting guidelines. J. Water Sanit. Hyg. Dev. 2023, 13, 833–846. [Google Scholar] [CrossRef]
- Shan, C. Analytical solution for the capture zone of two arbitrarily located wells. J. Hydrol. 1999, 222, 123–128. [Google Scholar] [CrossRef]
- Haitjema, H. The role of hand calculations in ground water flow modeling. Ground Water 2006, 44, 786–791. [Google Scholar] [CrossRef] [PubMed]
- Javandel, I.; Tsang, C. Capture-zone type curves: A tool for aquifer cleanup. Ground Water 1986, 24, 616–625. [Google Scholar] [CrossRef]
- Grubb, S. Analytical model for estimation of steady-state capture zones of pumping wells in confined and unconfined aquifers. Ground Water 1993, 31, 27–32. [Google Scholar] [CrossRef]
- Christ, J.A.; Goltz, M.N. Hydraulic containment: Analytical and semi-analytical models for capture zone curve delineation. J. Hydrol. 2002, 262, 224–244. [Google Scholar] [CrossRef]
- Cunningham, J.A.; Reinhard, M. Injection-extraction treatment well pairs: An alternative to permeable reactive barriers. Ground Water 2002, 40, 599–607. [Google Scholar] [CrossRef]
- Cunningham, J.A.; Hoelen, T.P.; Hopkins, G.D.; Lebrón, C.A.; Reinhard, M. Hydraulics of recirculation well pairs for ground water remediation. Ground Water 2004, 42, 880–889. [Google Scholar] [CrossRef]
- Zhang, S.; Wang, X. Regional flow influenced recirculation zones of pump-and-treat systems for groundwater remediation with one or two injection wells: An analytical comparison. Water 2023, 15, 2852. [Google Scholar] [CrossRef]
- International Telecommunication Union (ITU) Development Sector. Connectivity in the Least Developed Countries Status Report 2021; ITU Publications: Geneva, Switzerland, 2021. [Google Scholar]
- World Health Organization and the United Nations International Children’s Emergency Fund (WHO/UNICEF). Joint Monitoring Programme (JMP). Available online: https://washdata.org/data/household#!/ (accessed on 3 March 2024).
- UNICEF, UNICEF Data. Available online: https://data.unicef.org/topic/water-and-sanitation/drinking-water/ (accessed on 17 July 2024).
- United Nations Children’s Fund (UNICEF) and World Health Organization (WHO). Progress on Sanitation and Hygiene in Africa 2000–2022; UNICEF and WHO: New York, NY, USA, 2023. [Google Scholar]
- Strack, O.D.L. Analytical Groundwater Mechanics; Cambridge University Press: Cambridge, UK, 2017. [Google Scholar]
- Strack, O.D.L. Groundwater Mechanics; Prentice Hall: Englewood Cliffs, NJ, USA, 1989. [Google Scholar]
- Haitjema, H.M. Analytic Element Modeling of Groundwater Flow; Academic Press Inc.: San Diego, CA, USA, 1995. [Google Scholar]
- Bakker, M.; Strack, O.D.L. Capture zone delineation in two-dimensional groundwater flow models. Water Resour. Res. 1996, 32, 1309–1315. [Google Scholar] [CrossRef]
- Fienen, M.N.; Luo, J.; Kitanidis, P.K. Semi-analytical homogenous anisotropic capture zone delineation. J. Hydrol. 2005, 312, 39–50. [Google Scholar] [CrossRef]
- Fitts, C.R. Modeling aquifer systems with analytic elements and subdomains. Water Resour. Res. 2010, 46, 1–10. [Google Scholar] [CrossRef]



| Conditions Considered | Value of
Found in This Paper | Value of
Found by Previous Researchers | Relative Difference between This Paper and Previous Results |
|---|---|---|---|
| α = 1, | 0.5792 | 0.579 [22] | 0.03% |
| α = 1, | 0.5792 | 0.573 [24] | 1.1% |
| α = 1, | 0.7736 | 0.788 [24] | 1.8% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nenninger, C.; Mihelcic, J.R.; Cunningham, J.A. Ensuring the Safety of an Extraction Well from an Upgradient Point Source of Pollution in a Computationally Constrained Setting. Water 2024, 16, 2645. https://doi.org/10.3390/w16182645
Nenninger C, Mihelcic JR, Cunningham JA. Ensuring the Safety of an Extraction Well from an Upgradient Point Source of Pollution in a Computationally Constrained Setting. Water. 2024; 16(18):2645. https://doi.org/10.3390/w16182645
Chicago/Turabian StyleNenninger, Christopher, James R. Mihelcic, and Jeffrey A. Cunningham. 2024. "Ensuring the Safety of an Extraction Well from an Upgradient Point Source of Pollution in a Computationally Constrained Setting" Water 16, no. 18: 2645. https://doi.org/10.3390/w16182645
APA StyleNenninger, C., Mihelcic, J. R., & Cunningham, J. A. (2024). Ensuring the Safety of an Extraction Well from an Upgradient Point Source of Pollution in a Computationally Constrained Setting. Water, 16(18), 2645. https://doi.org/10.3390/w16182645







