Comprehensive Assessment of Climate Change Impacts on River Water Availability for Irrigation, Wheat Crop Area Coverage, and Irrigation Canal Hydraulic Capacity of Large-Scale Irrigation Scheme in Nepal
Abstract
1. Introduction
2. Materials and Methods
2.1. Study Area
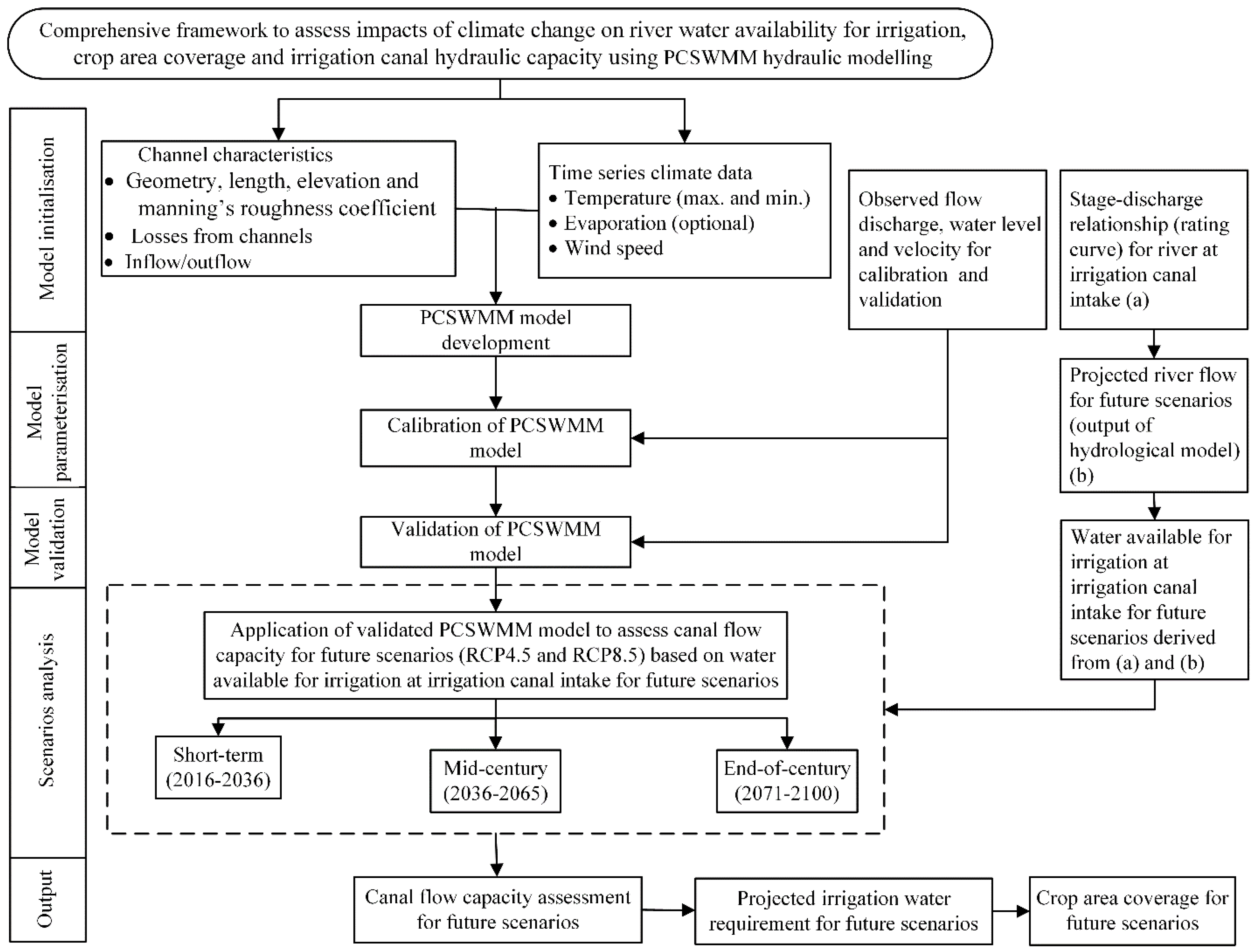
2.2. Assessment Methodology
2.2.1. PCSWMM Input Data Processing and Model Development (Model Initialisation)
2.2.2. Model Parameterisation
2.2.3. Model Validation
2.2.4. Scenario Analysis (Canal Capacity Assessment)
2.3. Personal Computer Storm Water Management Model (PCSWMM) Hydraulic Model
3. Application of Methodology for Case Study
3.1. PCSWMM Input Data and Model Initialisation
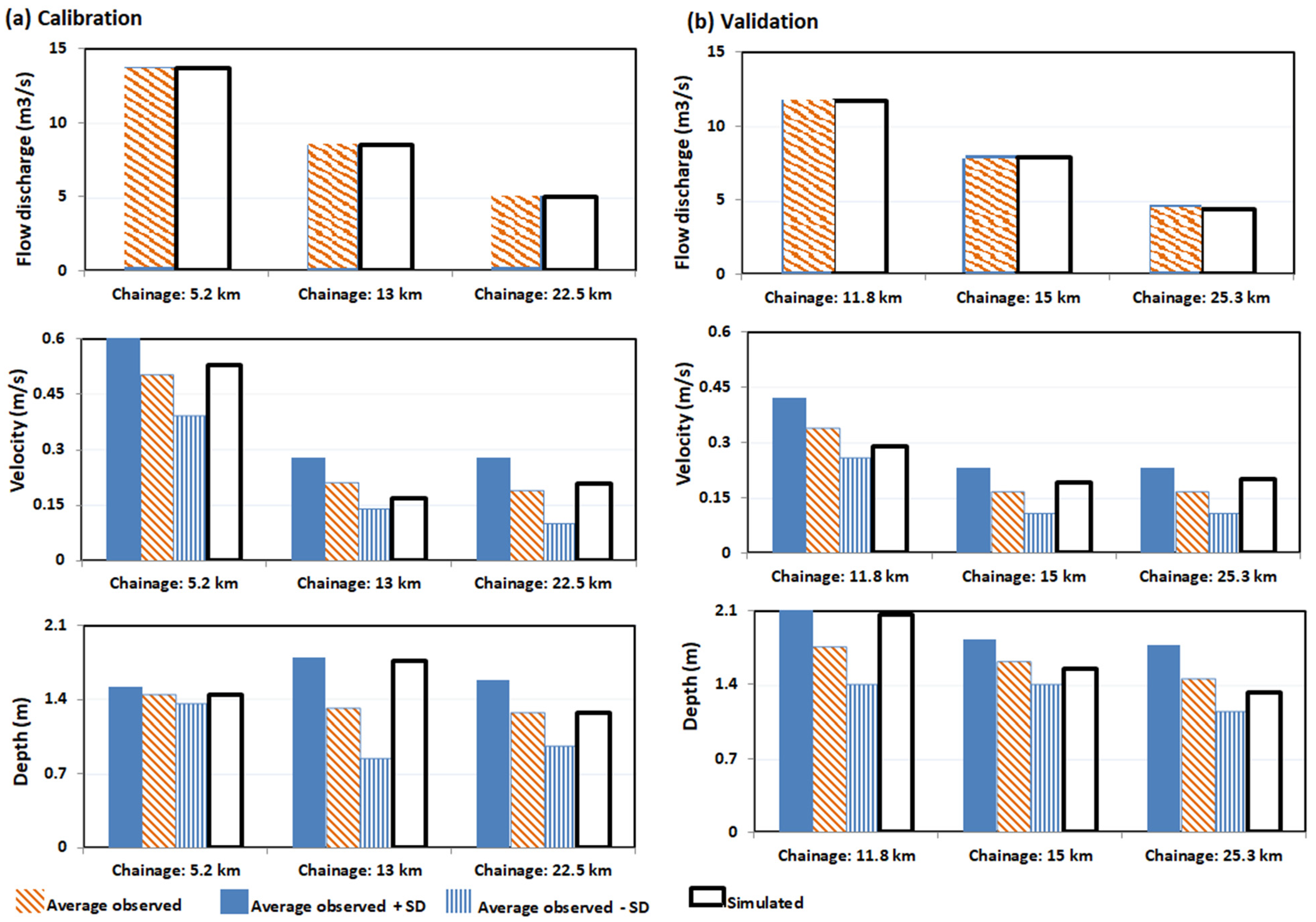
3.2. Model Parameterisation (Calibration)
3.3. Model Validation
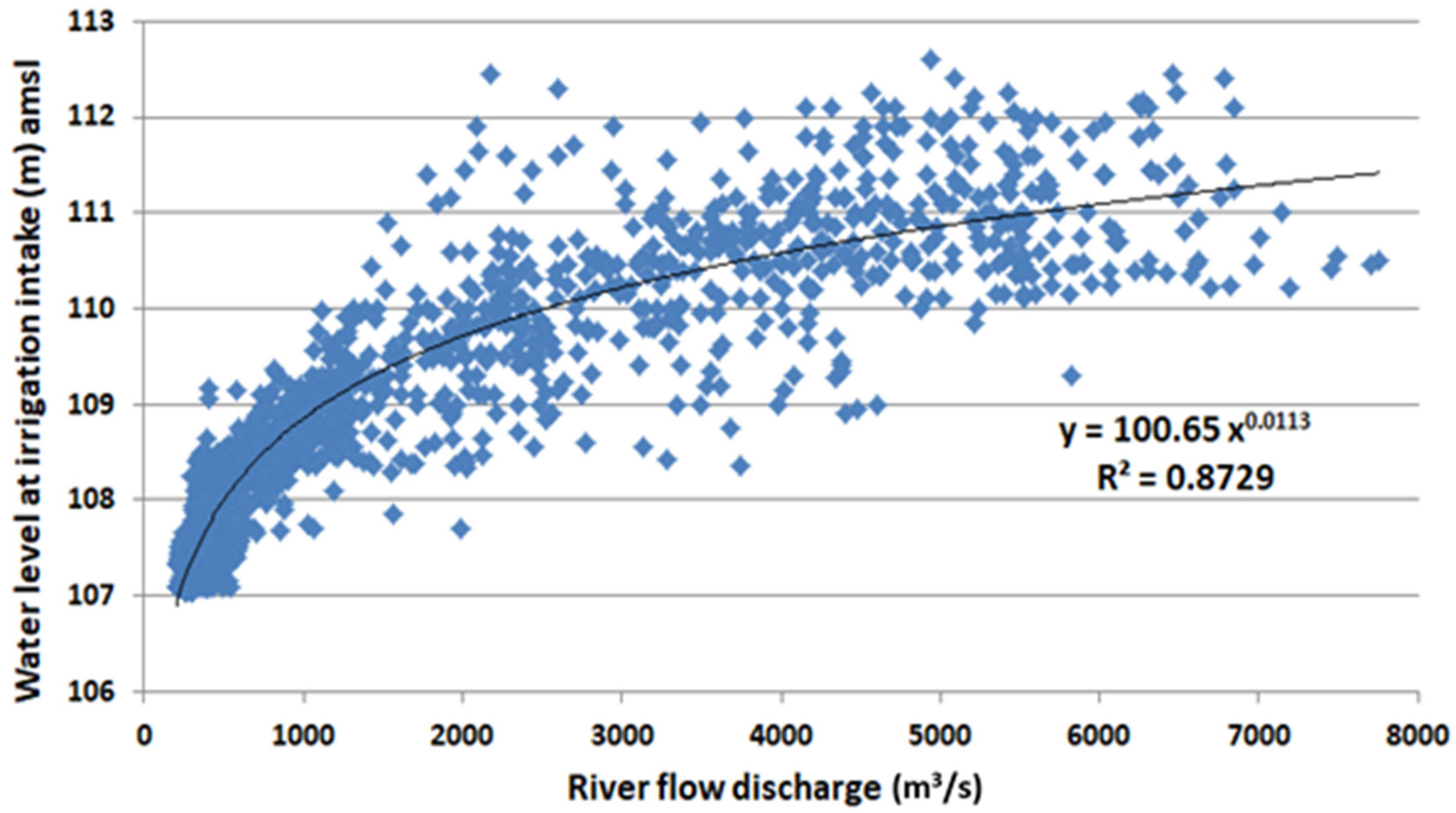
3.4. Canal Discharge Carrying Capacity Considering Water Availability under Future Climates (Scenario Analysis)
- (a)
- Development of a stage–discharge relationship (rating curve) for the Koshi River at irrigation canal intake.
- (b)
- Projected river flow for future climate scenarios, using the output of the Soil and Water Assessment Tool (SWAT) hydrological model [3].
- (c)
- Water availability for irrigation at the irrigation canal intake for future scenarios derived from (a) and (b) above.
4. Results and Discussion
4.1. Average Monthly Water Availability for Irrigation at the Irrigation Canal Intake for Dry Season during 1982–2010
4.2. Projected Average Monthly Minimum Flow Availability for Irrigation at Canal Intake
4.3. Implications of Climate Change for Potential Irrigated Cropping Area
4.4. Conveyance Losses at Canal
4.5. Canal Flow Capacity Assessment
5. Conclusions
- Water losses along the Sunsari Morang Irrigation main canal were 1.2 m3/m2/day, 0.77 m3/m2/day, and 0.60 m3/m2/day between the chainage 5 to 25 km, while average water loss in the main canal was 0.86 m3/m2/day. Similar water losses were also reported in other regions. Average water losses in the main canal were 5.78 m3/m2/day in Turkey [22], 1.21 m3/m2/day in Turkey [25], 1.08 m3/m2/day in Ethiopia [23], and 1.21 m3/m2/day in Iran [24].
- Average monthly water flows into the Sunsari Morang canal during the dry season months (December, January, February, March, April, and May) of 1982 to 2010 were 77 m3/s, 42 m3/s, 28 m3/s, 29 m3/s, 50 m3/s, and 125 m3/s, respectively. The inflow into the canal during January, February, March, and April was substantially less than the designed canal flow capacity of 60 m3/s, suggesting that engineering interventions enabling increased inflow rates (e.g., via river barrage, pumping, etc.) could be accommodated by the existing canal.
- Whilst future climate change projections indicate an increase in river water availability for irrigation extraction in the months of December, January, February, and March, the nominal maximum designed canal discharge capacity of 60 m3/s will still not be met in February and March, for all future time periods and climate change scenarios.
- Weed growth, silt deposition, and lack of regular maintenance have reduced the canal discharge carrying capacity by 12–31% (depending on chainage distance) with an average discharge reduction of 23%. Lining, relining, or piping sections of the canal and regular maintenance (silt and weed removal) could improve flow carrying capacity.
- The current amount of water available for irrigation was sufficient for irrigating 13,000 ha of wheat during 1982–2010 with no water deficit conditions, while the average wheat cropping area supported by the scheme during 2008–2016 was 26,000 ha. This shows that farmers are still practicing protective irrigation in the Sunsari Morang Irrigation Scheme command area.
- The irrigated broadacre cropping area could be increased by 3000–5000 ha in the short-term (2016–2045), 6000–7000 ha in the mid-century (2036–2065), and 3000–9000 ha in the end-of-century (2071–2100) periods with no water deficit occurring. However, given that climate projections are for more variable weather, and more extreme events [47], it is likely that the frequencies of drought in the region may change in the future. This deserves further attention in the future.
- As with all cereal crops, wheat grain yield is most sensitive to water stress in the flowering to grain filling stages, a period that includes the peak water demand period of March. Options could be explored that tap into alternative water supplies, or allow a certain yield reduction from partial water stress, thereby allowing the total area of wheat to be expanded. An increased area but with a lower average grain yield may increase the total yield from the whole SMIS. This water stress versus yield interaction could be efficiently explored in future research.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Kaini, S.; Nepal, S.; Pradhananga, S.; Gardner, T.; Sharma, A.K. Representative general circulation models selection and downscaling of climate data for the transboundary Koshi river basin in China and Nepal. Int. J. Clim. 2020, 40, 4131–4149. [Google Scholar] [CrossRef]
- Wester, P.; Mishra, A.; Mukherji, A.; Shrestha, A.B. The Hindu Kush Himalaya Assessment: Mountains, Climate Change, Sustainability and People; Springer Nature: Cham, Switzerland, 2019; p. 627. [Google Scholar]
- Kaini, S.; Nepal, S.; Pradhananga, S.; Gardner, T.; Sharma, A.K. Impacts of climate change on the flow of the transboundary Koshi River, with implications for local irrigation. Int. J. Water Resour. Dev. 2021, 37, 929–954. [Google Scholar] [CrossRef]
- Phelan, D.C.; Harrison, M.T.; McLean, G.; Cox, H.; Pembleton, K.G.; Dean, G.J.; Parsons, D.; do Amaral Richter, M.E.; Pengilley, G.; Hinton, S.J.; et al. Advancing a farmer decision support tool for agronomic decisions on rainfed and irrigated wheat cropping in Tasmania. Agric. Syst. 2018, 167, 113–124. [Google Scholar] [CrossRef]
- Liu, K.; Harrison, M.T.; Archontoulis, S.V.; Huth, N.; Yang, R.; Liu, D.L.; Yan, H.; Meinke, H.; Huber, I.; Feng, P.; et al. Climate change shifts forward flowering and reduces crop waterlogging stress. Environ. Res. Lett. 2021, 16, 094017. [Google Scholar] [CrossRef]
- Muleke, A.; Harrison, M.T.; De Voil, P.; Hunt, I.; Liu, K.; Yanotti, M.; Eisner, R. Earlier crop flowering caused by global warming alleviated by irrigation. Environ. Res. Lett. 2022, 17, 044032. [Google Scholar] [CrossRef]
- Schultz, B.; Tardieu, H.; Vidal, A. Role of water management for global food production and poverty alleviation. Irrig. Drain. 2009, 58, S3–S21. [Google Scholar] [CrossRef]
- Moreno-Pérez, M.F.; Roldán-Cañas, J. Assessment of irrigation water management in the Genil-Cabra (Córdoba, Spain) irrigation district using irrigation indicators. Agric. Water Manag. 2013, 120, 98–106. [Google Scholar] [CrossRef]
- FAO. Water Withdrawal by Sector, around 2010. 2017. Available online: http://www.fao.org/nr/Water/aquastat/tables/WorldData-Withdrawal_eng.pdf (accessed on 23 August 2017).
- Awulachew, S.B.; Ayana, M. Performance of irrigation: An assessment at different scales in Ethiopia. Exp. Agric. 2011, 47, 57–69. [Google Scholar] [CrossRef]
- Checkol, G.; Alamirew, T. Technical and Institutional Evaluation of Geray Irrigation Scheme in West Gojjam Zone, Amhara Region, Ethiopia. J. Spat. Hydrol. 2008, 8, 36–48. [Google Scholar]
- Asres, S.B. Evaluating and enhancing irrigation water management in the upper Blue Nile basin, Ethiopia: The case of Koga large scale irrigation scheme. Agric. Water Manag. 2016, 170, 26–35. [Google Scholar] [CrossRef]
- Kim, H.; Kim, J.; Nam, W.; Kim, S.; Choi, J.; Koh, B. Irrigation canal network flow analysis by a hydraulic model. Irrig. Drain. 2016, 65, 57–65. [Google Scholar] [CrossRef]
- Worstell, R.V. Estimating seepage losses from canal systems, In Proceedings of the American Society of Civil Engineers. J. Irrig. Drain. Div. 1976, 102, 137–147. [Google Scholar] [CrossRef]
- Monjardino, M.; Harrison, M.T.; DeVoil, P.; Rodriguez, D.; Sadras, V.O. Agronomic and on-farm infrastructure adaptations to manage economic risk in Australian irrigated broadacre systems: A case study. Agric. Water Manag. 2022, 269, 107740. [Google Scholar] [CrossRef]
- Swamee, P.K.; Mishra, G.C.; Chahar, B.R. Design of minimum water-loss canal sections. J. Hydraul. Res. 2002, 40, 215–220. [Google Scholar] [CrossRef]
- Memon, A.A.; Leghari, K.Q.; Pathan, A.F.; Khatri, K.L.; Shah, S.A.; Pinjani, K.K.; Soomro, R.; Ansari, K. Design and evaluation of Dadu canal lining for sustainable water saving. J. Water Resour. Prot. 2013, 5, 689–698. [Google Scholar] [CrossRef]
- IS:9452 (Part II)-1980; Indian Standard Code of Practice for Measurement of Seepage Losses from Canals Part II: Inflow-outflow Method. Indian Standards Institution: New Delhi, India, 1980.
- Moghazi, H.E.M.; Ismail, E.-S. A study of losses from field channels under arid region conditions. Irrig. Sci. 1997, 17, 105–110. [Google Scholar] [CrossRef]
- FAO/UNESCO. Irrigation, Drainage and Salinity: An International Source Book; The Camelot Press Ltd.: London, UK, 1973. [Google Scholar]
- Wilkinson, R.W. Plastic lining on riverton unit, Wyoming. J. Irrig. Drain. Eng. 1985, 111, 287–298. [Google Scholar] [CrossRef]
- Kilic, M.; Tuylu, G.I. Determination of water conveyance loss in the ahmetli regulator irrigation system in the lower Gediz Basin Turkey. Irrig. Drain. 2011, 60, 579–589. [Google Scholar] [CrossRef]
- Eshetu, B.D.; Alamirew, T. Estimation of seepage loss in irrigation canals of Tendaho sugar estate, Ethiopia. Irrig. Drain. Syst. Eng. 2018, 7. [Google Scholar] [CrossRef]
- Mohammadi, A.; Rizi, A.P.; Abbasi, N. Field measurement and analysis of water losses at the main and tertiary levels of irrigation canals: Varamin Irrigation Scheme, Iran. Glob. Ecol. Conserv. 2019, 18, e00646. [Google Scholar] [CrossRef]
- Akkuzu, E. Usefulness of empirical equations in assessing canal losses through seepage in concrete-lined canal. J. Irrig. Drain. Eng. 2012, 138, 455–460. [Google Scholar] [CrossRef]
- Kaini, S. Climate change impacts on the renewable energy sector in Nepal. In Nepalma Urja, 2024; Lamsal, R., Ed.; Research for Nepal: Kathmandu, Nepal, 2024; pp. 35–43. [Google Scholar]
- Gurung, A.; Adhikari, S.; Chauhan, R.; Thakuri, S.; Nakarmi, S.; Ghale, S.; Dongol, B.S.; Rijal, D. Water crises in a water-rich country: Case studies from rural watersheds of Nepal’s mid-hills. Water Policy 2019, 21, 826–847. [Google Scholar] [CrossRef]
- Kaini, S.; Harrison, M.T.; Gardner, T.; Nepal, S.; Sharma, A.K. The impacts of climate change on the irrigation water demand, grain yield, and biomass yield of wheat crop in Nepal. Water 2022, 14, 2728. [Google Scholar] [CrossRef]
- Mishra, Y.; Babel, M.S.; Nakamura, T.; Mishra, B. Impacts of climate change on irrigation water management in the Babai River Basin, Nepal. Hydrology 2021, 8, 85. [Google Scholar] [CrossRef]
- Ministry of Agriculture and Livestock Development. Statistical Information on Nepalese Agriculture 2021/22; Government of Nepal, Ministry of Agriculture and Livestock Development: Kathmandu, Nepal, 2023.
- Do, J.W.; Nam, W.H.; Lee, K.Y.; Ha, T.H.; Lee, S.Y.; Jung, E.Y. Optimal irrigation management system based on hydraulic analysis of irrigation canal. In Proceedings of the 2019 ASABE Annual International Meeting, Boston, MA, USA, 7–10 July 2019; American Society of Agricultural and Biological Engineers: St. Joseph, MI, USA, 2019; p. 1. [Google Scholar]
- Bang, N.K.; Nam, W.H.; An, H.U.; Ha, T.H.; Lee, K.Y. Optimal Operation of Irrigation Canal Network System Using SWMM. In Proceedings of the 3rd World Irrigation Forum (WIF3), Bali, Indonesia, 1–7 September 2019. [Google Scholar]
- Schoenfelder, C.; Kenner, S.; Hoyer, D. Hydraulic model of the belle fourche irrigation district using EPA SWMM 5.0. In Proceedings of the World Environmental and Water Resource Congress 2006: Examining the Confluence of Environmental and Water Concerns, Omaha, NE, USA, 21–25 May 2006; pp. 1–10. [Google Scholar]
- Banda, D.; Kasitu, R. Modeling of storm water runoff for Kitwe CBD drainage system using SWMM software. Rwanda J. Eng. Sci. Technol. Environ. 2018, 1, 1. [Google Scholar] [CrossRef]
- Holzworth, D.; Fainges, J.; Brown, H.; Zurcher, E.; Cichota, R.; Verrall, S.; Herrmann, N.; Zheng, B.; Snow, V. APSIM Next Generation: Overcoming challenges in modernising a farming systems model. Environ. Model. Softw. 2018, 103, 43–51. [Google Scholar] [CrossRef]
- Garg, S.K. Irrigation Engineering and Hydraulic Structures, 9th ed.; Khanna Publishers: Delhi, India, 2006. [Google Scholar]
- Department of Irrigation. Design Manuals for Irrigation Projects in Nepal, M3: Hydrology and Agro-Meteorology Manual; Department of Irrigation: Lalitpur, Nepal, 1990.
- Paudel, K.P. Role of Sediment in the Design and Management of Irrigation Canals. Ph.D. Thesis, UNESCO-IHE, Institute for Water Education, Delft, The Netherlands, 2010. [Google Scholar]
- Adhikari, B. Design of Water Distribution System: Appropriateness of Structured System in Large Irrigation Projects in Nepal. Hydro Nepal J. Water Energy Environ. 2016, 19, 25–30. [Google Scholar] [CrossRef]
- Renault, D.; Wahaj, R. MASSCOT: A Methodology to Modernize Irrigation Services and Operations in Canal Systems, Applications to Two Systems in Nepal Terai: Sunsari Morang Irrigation System and Narayani Irrigation System; FAO: Rome, Italy, 2006; pp. 1–42. [Google Scholar]
- Shahpari, S.; Allison, J.; Harrison, M.T.; Stanley, R. An integrated economic, environmental and social approach to agricultural land-use planning. Land 2021, 10, 364. [Google Scholar] [CrossRef]
- Jurriëns, M.; Mollinga, P.P.; Wester, P. Scarcity by Design: Protective Irrigation in India and Pakistan; International Institute for Land Relclamation and Improvement (ILRI): Wageningen, The Netherlands, 1996. [Google Scholar]
- Kaini, S.; Hayde, L.G.; Schultz, B.; Marence, M. Seepage analysis underneath the headwork of Chanda Mohana Irri-gation Scheme, Sunsari, Nepal. In Proceedings of the National Irrigation Seminar Micro to Mega: Irrigation For Prosperous Nepal, Kathmandu, Nepal, July 13–14 July 2011; Department of Irrigation/Nepal, International Network for Participatory Irrigation Management (INPIM)/Nepal and IWMI/Nepal: Lalitpur, Nepal, 2011; pp. 94–128. [Google Scholar]
- Kaini, S. Multiple use of irrigation water: Way towards sustainable irrigation management. In Proceedings of the National Irrigation Seminar on Irrigation Development and Management: Learning from the Past and Planning for the Future, Lalitpur, Nepal, 10–11 June 2016; Department of Irrigation: Lalitpur, Nepal, 2016; pp. 113–124. [Google Scholar]
- Kaini, S.; Gardner, T.; Sharma, A.K. Assessment of socio-economic factors impacting on the cropping intensity of an irrigation scheme in developing countries. Irrig. Drain. 2020, 69, 363–375. [Google Scholar] [CrossRef]
- Nepal, S.; Neupane, N.; Koirala, S.; Lautze, J.; Shrestha, R.N.; Bhatt, D.; Shrestha, N.; Adhikari, M.; Kaini, S.; Karki, S.; et al. Integrated assessment of irrigation and agriculture management challenges in Nepal: An interdisciplinary perspective. Heliyon 2024, 10, e29407. [Google Scholar] [CrossRef]
- Harrison, M.T. Climate change benefits negated by extreme heat. Nat. Food 2021, 2, 855–856. [Google Scholar] [CrossRef] [PubMed]



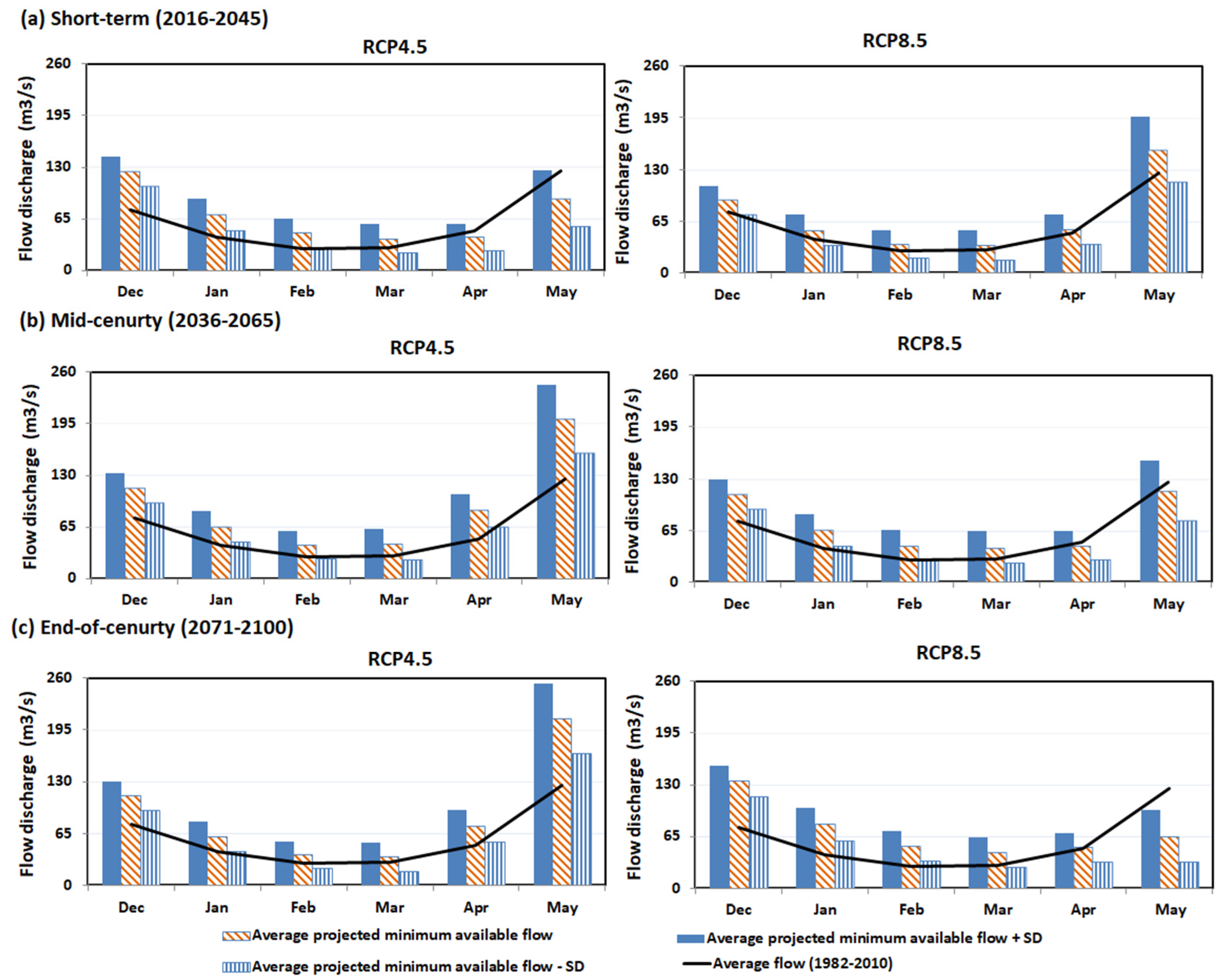
| Scenarios | Dec | Jan | Feb | Mar | Apr | May |
|---|---|---|---|---|---|---|
| Reference period (1982–2010) | 76.69 | 41.68 | 27.87 | 29.39 | 50.50 | 124.84 |
| Short-term (2016–2045)_RCP4.5 | 124.80 | 70.42 | 47.65 | 40.50 | 42.02 | 90.64 |
| Short-term (2016–2045)_RCP8.5 | 92.18 | 54.35 | 36.96 | 35.57 | 55.26 | 155.43 |
| Mid-century (2036–2065)_RCP4.5 | 114.41 | 65.15 | 42.80 | 43.46 | 85.89 | 200.22 |
| Mid-century (2036–2065)_RCP8.5 | 110.84 | 66.21 | 46.69 | 44.44 | 46.87 | 115.34 |
| End-of-century (2071–2100)_RCP4.5 | 112.03 | 60.87 | 37.93 | 35.57 | 73.78 | 209.31 |
| End-of-century (2071–2100)_RCP8.5 | 134.76 | 80.70 | 53.43 | 45.42 | 51.68 | 65.51 |
| Irrigation Water Requirement (mm) for a Wheat Crop at Field Level | |||||
|---|---|---|---|---|---|
| Scenarios | Dec | Jan | Feb | Mar | Apr |
| Reference period (1982–2010) | 3 | 44 | 118 | 198 | 66 |
| Short-term (2016–2045)_RCP4.5 | 4 | 48 | 125 | 195 | 67 |
| Short-term (2016–2045)_RCP8.5 | 4 | 50 | 130 | 197 | 58 |
| Mid-century (2036–2065)_RCP4.5 | 4 | 49 | 126 | 198 | 58 |
| Mid-century (2036–2065)_RCP8.5 | 5 | 53 | 127 | 192 | 50 |
| End-of-century (2071–2100)_RCP4.5 | 4 | 55 | 135 | 196 | 51 |
| End-of-century (2071–2100)_RCP8.5 | 4 | 52 | 125 | 183 | 29 |
| Irrigation Water Requirement (L/s/ha) for Winter Wheat at the Irrigation Canal Intake | |||||
|---|---|---|---|---|---|
| Scenarios | Dec | Jan | Feb | Mar | Apr |
| Reference period (1982–2010) | 0.03 | 0.5 | 1.35 | 2.26 | 0.75 |
| Short-term (2016–2045)_RCP4.5 | 0.05 | 0.55 | 1.43 | 2.23 | 0.77 |
| Short-term (2016–2045)_RCP8.5 | 0.05 | 0.57 | 1.49 | 2.25 | 0.66 |
| Mid-century (2036–2065)_RCP4.5 | 0.05 | 0.56 | 1.44 | 2.26 | 0.66 |
| Mid-century (2036–2065)_RCP8.5 | 0.06 | 0.61 | 1.45 | 2.19 | 0.57 |
| End-of-century (2071–2100)_RCP4.5 | 0.05 | 0.63 | 1.54 | 2.24 | 0.58 |
| End-of-century (2071–2100)_RCP8.5 | 0.05 | 0.59 | 1.43 | 2.09 | 0.33 |
| Scenarios | Dec | Jan | Feb | Mar | Apr |
|---|---|---|---|---|---|
| Reference period (1982–2010) | 2556.3 | 83.4 | 20.6 | 13.0 | 67.3 |
| Short-term (2016–2045)_RCP4.5 | 2496.0 | 128.0 | 33.3 | 18.2 | 54.6 |
| Short-term (2016–2045)_RCP8.5 | 1843.6 | 95.4 | 24.8 | 15.8 | 83.7 |
| Mid-century (2036–2065)_RCP4.5 | 2288.2 | 116.3 | 29.7 | 19.2 | 130.1 |
| Mid-century (2036–2065)_RCP8.5 | 1847.3 | 108.5 | 32.2 | 20.3 | 82.2 |
| End-of-century (2071–2100)_RCP4.5 | 2240.6 | 96.6 | 24.6 | 15.9 | 127.2 |
| End-of-century (2071–2100)_RCP8.5 | 2695.2 | 136.8 | 37.4 | 21.7 | 156.6 |
| Distance from Intake | Discharge (m3/s) | Wetted Perimeter (m) | Losses (m3/m2/day) |
|---|---|---|---|
| 5.2 km | 13.77 | 21.23 | 1.21 |
| 11.8 km | 11.81 | 20.92 | |
| 13 km | 8.53 | 29.72 | 0.77 |
| 15 km | 8.01 | 30.58 | |
| 22.6 km | 5.04 | 20.56 | 0.60 |
| 25.4 km | 4.65 | 19.73 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kaini, S.; Harrison, M.T.; Gardner, T.; Sharma, A.K. Comprehensive Assessment of Climate Change Impacts on River Water Availability for Irrigation, Wheat Crop Area Coverage, and Irrigation Canal Hydraulic Capacity of Large-Scale Irrigation Scheme in Nepal. Water 2024, 16, 2595. https://doi.org/10.3390/w16182595
Kaini S, Harrison MT, Gardner T, Sharma AK. Comprehensive Assessment of Climate Change Impacts on River Water Availability for Irrigation, Wheat Crop Area Coverage, and Irrigation Canal Hydraulic Capacity of Large-Scale Irrigation Scheme in Nepal. Water. 2024; 16(18):2595. https://doi.org/10.3390/w16182595
Chicago/Turabian StyleKaini, Santosh, Matthew Tom Harrison, Ted Gardner, and Ashok K. Sharma. 2024. "Comprehensive Assessment of Climate Change Impacts on River Water Availability for Irrigation, Wheat Crop Area Coverage, and Irrigation Canal Hydraulic Capacity of Large-Scale Irrigation Scheme in Nepal" Water 16, no. 18: 2595. https://doi.org/10.3390/w16182595
APA StyleKaini, S., Harrison, M. T., Gardner, T., & Sharma, A. K. (2024). Comprehensive Assessment of Climate Change Impacts on River Water Availability for Irrigation, Wheat Crop Area Coverage, and Irrigation Canal Hydraulic Capacity of Large-Scale Irrigation Scheme in Nepal. Water, 16(18), 2595. https://doi.org/10.3390/w16182595










