Research and Prevention of Harmful Gases in Special Structures of Urban Deep Drainage Systems
Abstract
1. Introduction
2. Mathematical and Experimental Models
2.1. Simulation Experiment Mathematical Model
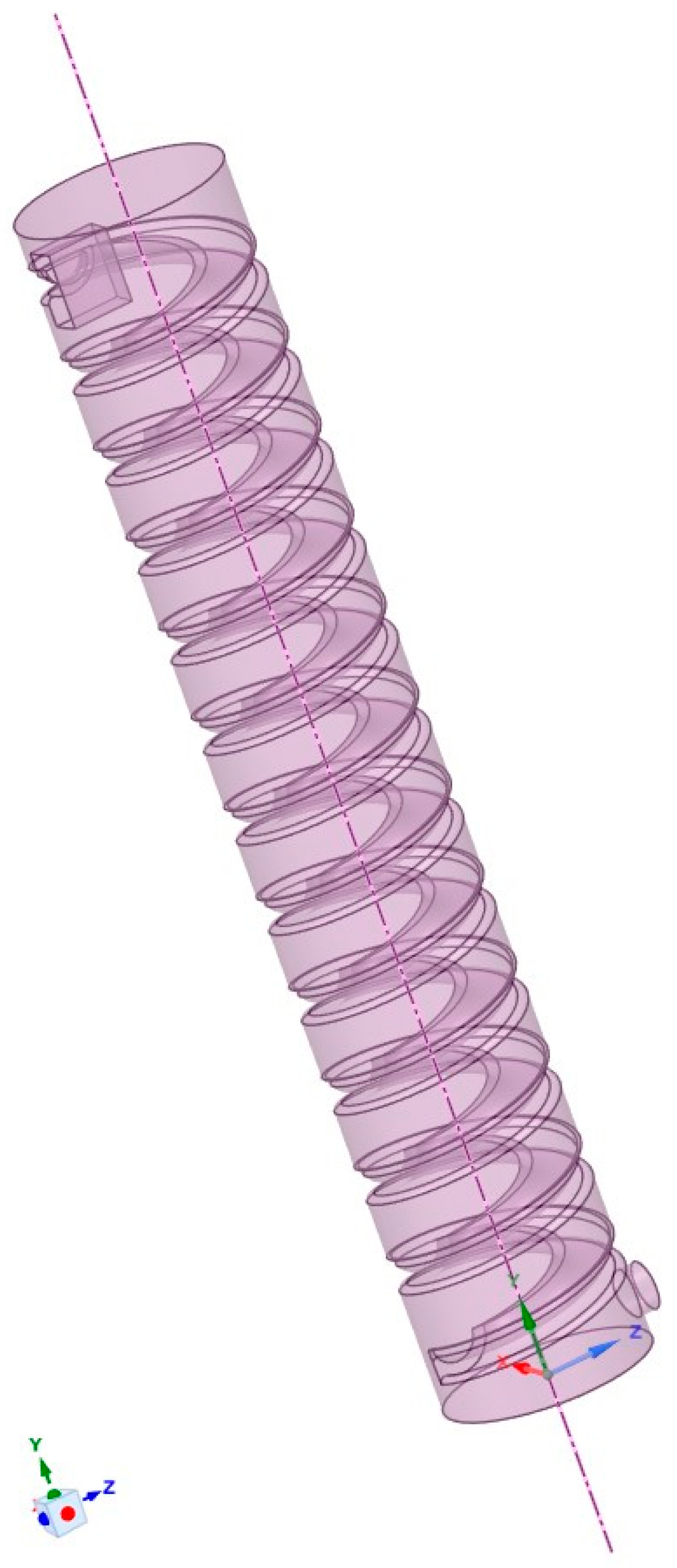
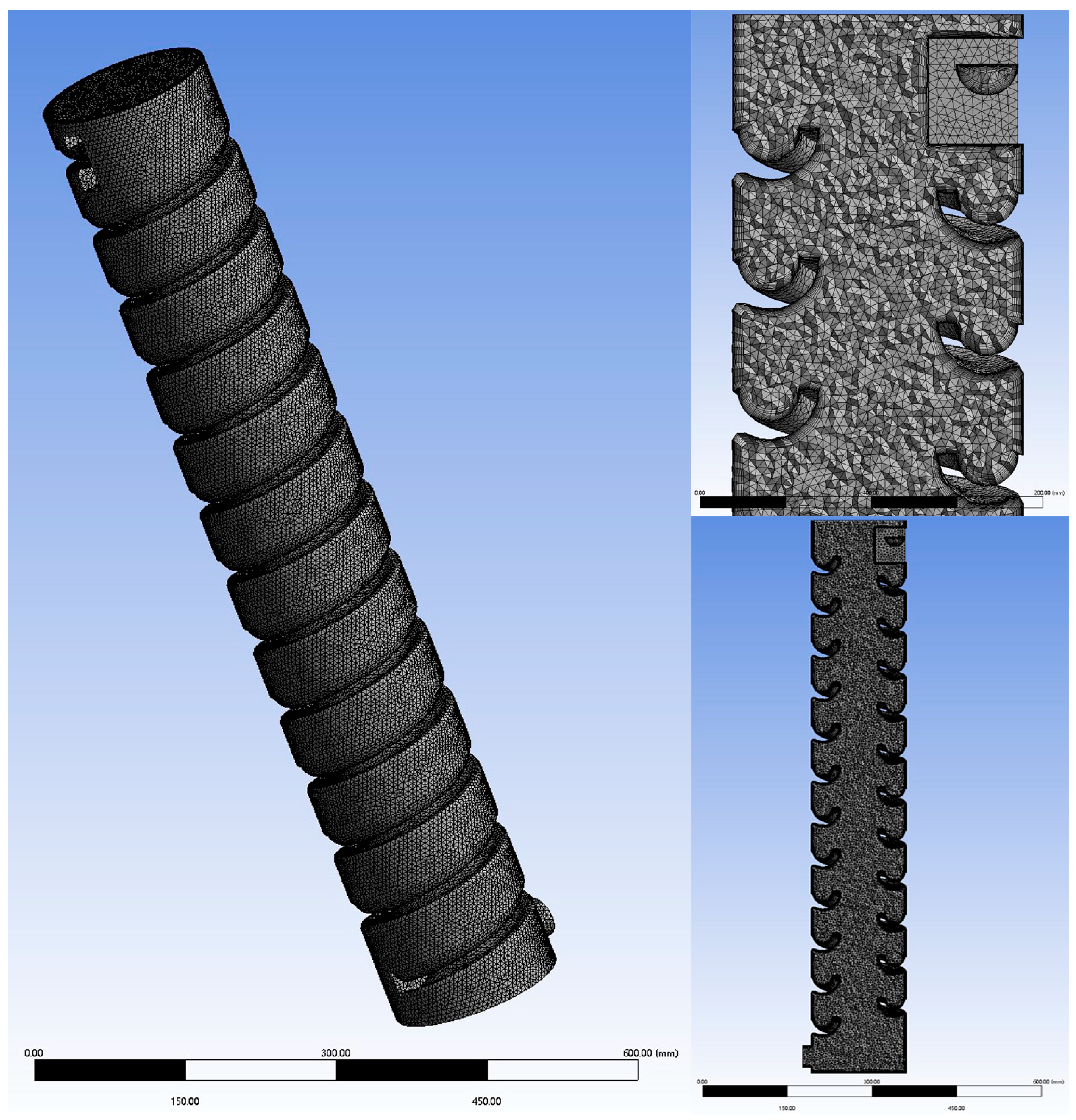
2.1.1. Calculation Area and Mesh Division
2.1.2. Boundary Conditions
2.1.3. Standard k-ε Turbulence Model
2.1.4. Mass Transport and Component Diffusion Equations
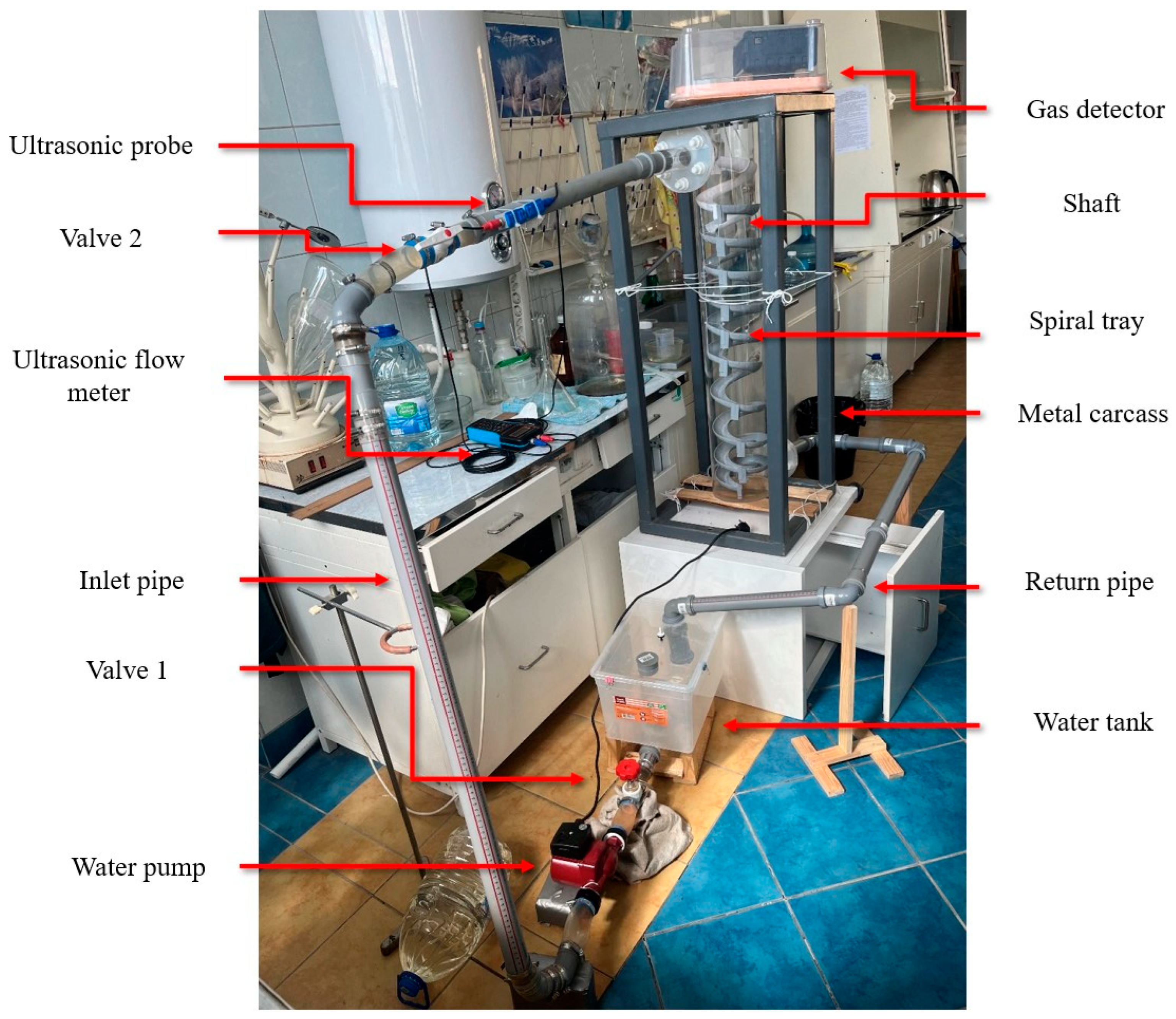
2.2. Experimental Model
2.2.1. Experimental Model Parameters
2.2.2. Experimental Water Parameters
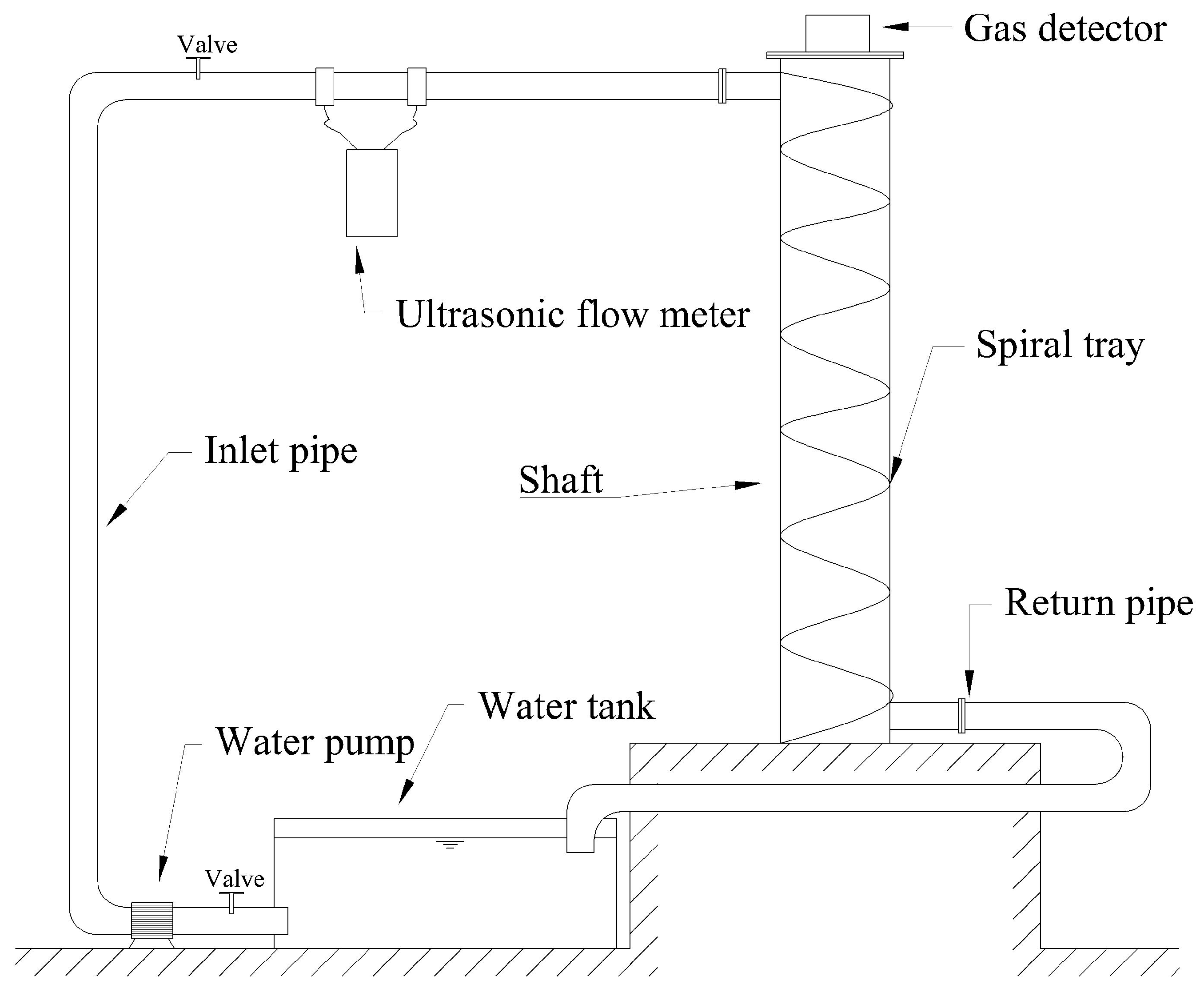
2.2.3. Experimental Circulation System
3. Research and Analysis of Results
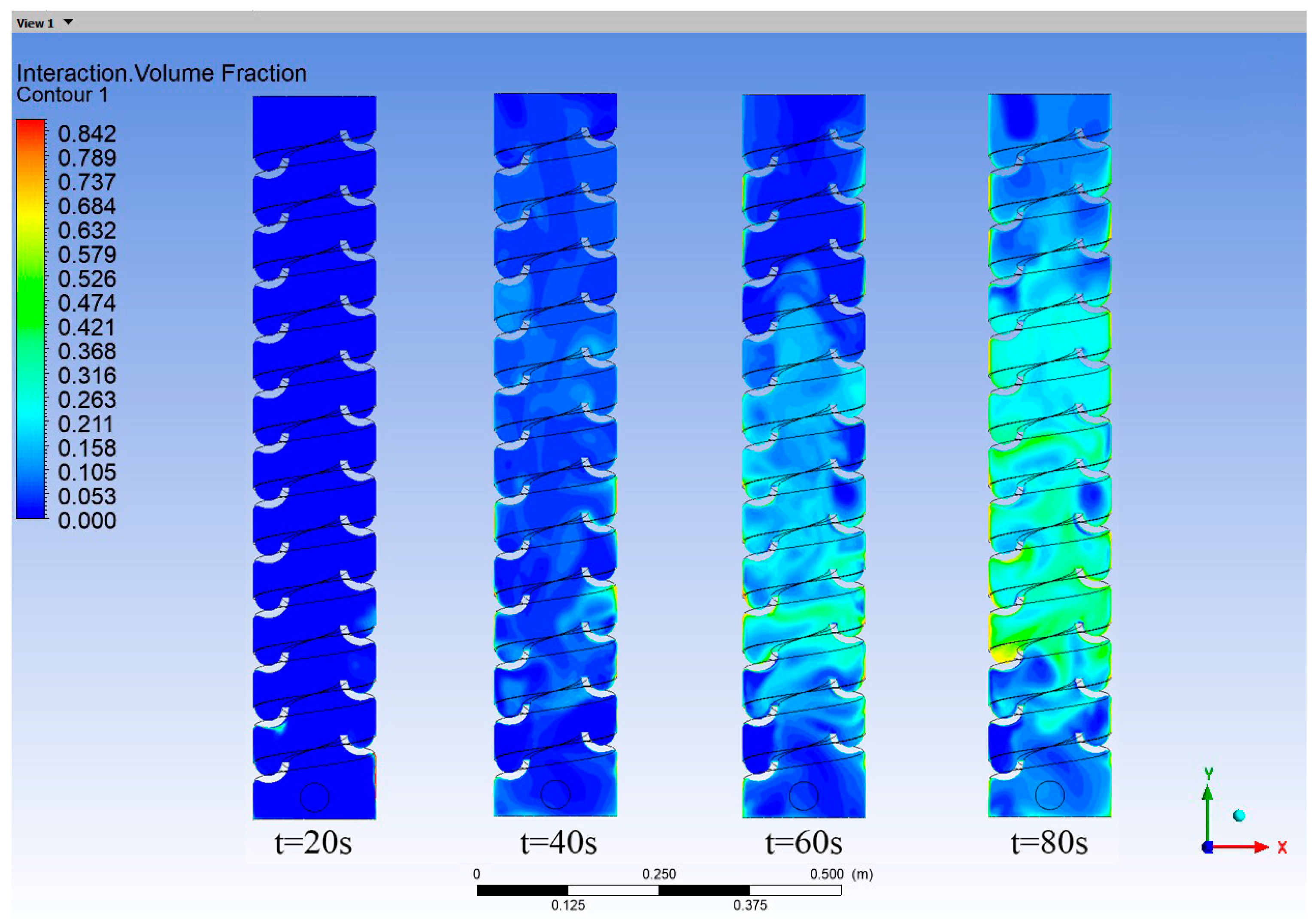
3.1. Analysis of Gas Flow within the Shaft
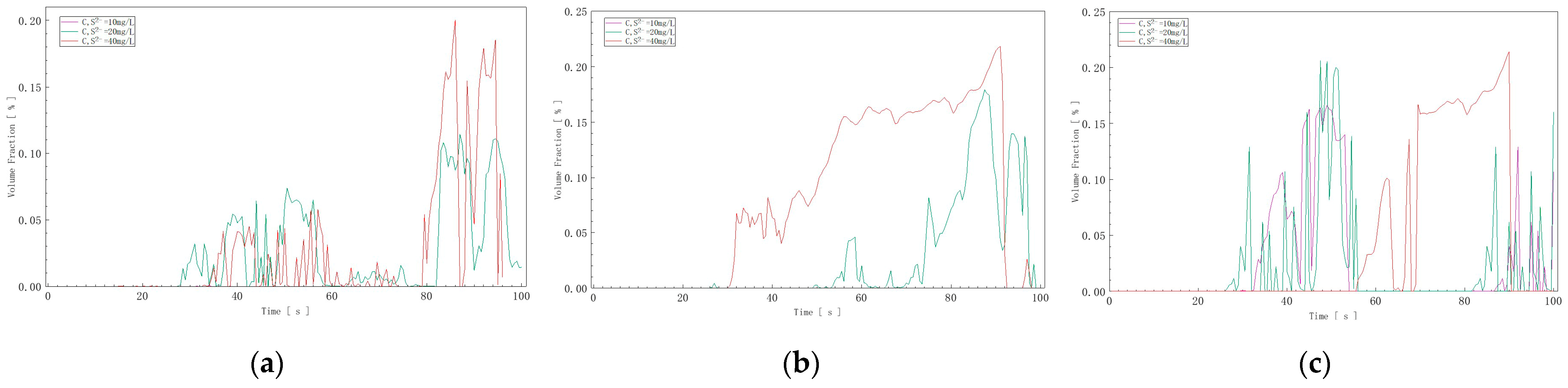
3.2. Simulation Analysis of Hydrogen Sulfide Release
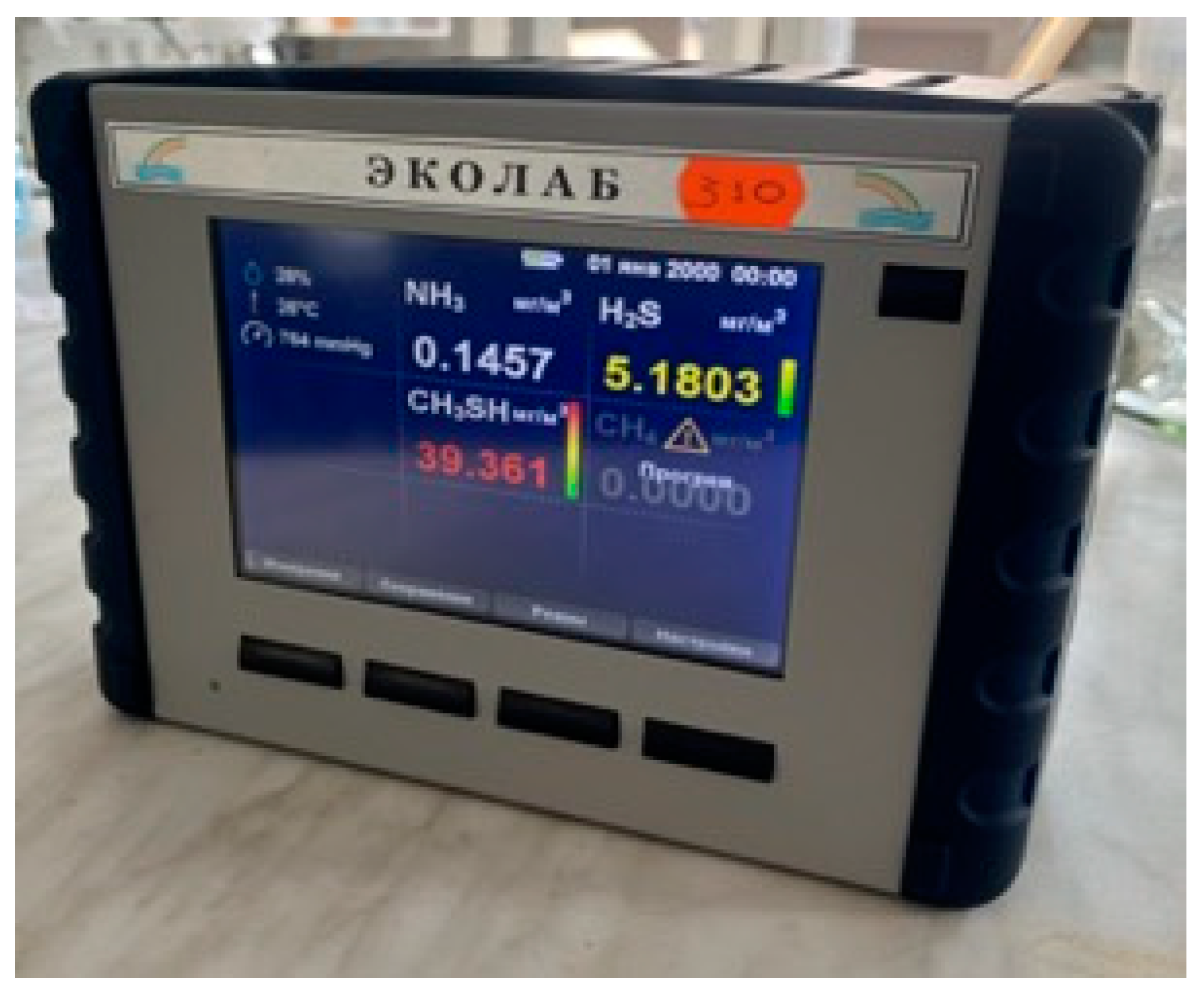
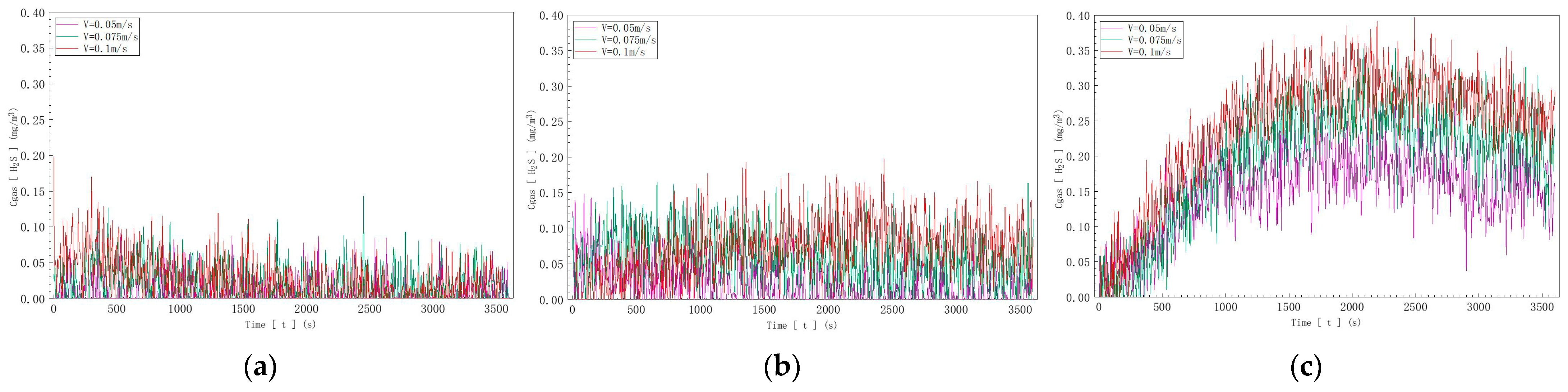
3.3. Experimental Analysis of Hydrogen Sulfide Gas Release
4. Conclusions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Xing, Y.; Cao, X.; Liu, T.; Xu, G.; Yang, C.; Lu, C.; He, J. Current Status, Problems, and Development Suggestions of Urban Drainage Systems in China. China Water Wastewater 2020, 36, 19–23. [Google Scholar]
- Li, H.; Zhang, L.; Tang, X.; Chen, W. Analysis of the Causes and Influencing Factors of Hydrogen Sulfide Gas Production in Urban Drainage Pipelines. Environ. Sci. Manag. 2012, 37, 95–97+107. [Google Scholar]
- Sun, H.; Hao, Y.; Long, T. Drainage Engineering (Volume I); China Architecture & Building Press: Beijing, China, 1999; pp. 1–3. [Google Scholar]
- Xu, W. Study on the Overflow Law of H_2S in Urban Sewage Drainage Systems. Master’s Thesis, Lanzhou University of Technology, Lanzhou, China, 2014. [Google Scholar]
- Su, S.; Yang, X.; Chen, S.; Peng, S. Study on Methane Explosion in Urban Sewers. Urban Gas 2021, 153–157. [Google Scholar]
- Hu, W.; Du, Y. Hazards and Detection Methods of Hydrogen Sulfide Gas. Materials Prot. 1996, 29, 17–18. [Google Scholar]
- Zhu, Y.; Wang, X.; Zhang, L.; Zhang, J. Hazards and Preventive Measures of Hydrogen Sulfide in Drainage Systems. China Water Wastewater 2000, 16, 45–47. [Google Scholar]
- Zhang, Y. Experimental Study on Methane Chlorination Reaction with Pulsed Corona Plasma. Master’s Thesis, Zhejiang University of Technology, Hangzhou, China, 2009. [Google Scholar]
- Robert, W.; Seabloom, P.E. Emeritus Professor of Civil and Environmental Engineering Dept. of Civil and Environmental Engineering. Septic Tank; Ted Loudon Professor of Agricultural Engineering Farall Hall.
- Li, H. Analysis of Key Points in the Design of Municipal Drainage Networks. Urban Archit. Space 2023, 30 (Suppl. S2), 197–198. [Google Scholar]
- He, J. Analysis of Construction and Operation Problems of Urban Drainage Networks. Urban Constr. Theory Res. (Electron. Ed.) 2022, 142–144. [Google Scholar]
- Chen, W.; Wu, Z.; Xu, C.; Bian, G.; Ma, Z.; Dai, L. CFD-Based Design and Energy Dissipation Analysis of Vertical Drop Shaft Structures. Software 2020, 41, 170–174. [Google Scholar]
- Lin, B. Planning and Optimization Design of Municipal Drainage Networks. Eng. Technol. Res. 2022, 7, 181–182. [Google Scholar]
- Chen, Z.; Wei, H.; Qin, J. Research on Large Drop Shaft Model Tests in Shanghai Drainage Projects. J. Northwest Inst. Archit. Eng. (Nat. Sci. Ed.) 2000, 69–75. [Google Scholar]
- Wu, H.; Ma, N. Model Test Research on Energy Dissipation Effect of Drop Shafts. J. Chongqing Univ. 2022, 45 (Suppl. S1), 111–114. [Google Scholar]
- Wang, L.; Guo, J.; Wei, J.; Liang, R. Application Research of Vertical Shaft Structures in Urban Drainage Systems. J. Lanzhou Petrochem. Vocat. Coll. 2022, 22, 23–26. [Google Scholar]
- Ma, Y. Research on Gas Entrainment and Energy Dissipation in Drop Structures of Drainage Systems. Ph.D. Thesis, Zhejiang University, Hangzhou, China, 2016. [Google Scholar]
- Ding, C. Research on the Movement and Distribution of Harmful Gases in Sewage Pipelines. Master’s Thesis, Xi’an University of Architecture and Technology, Xi’an, China, 2015. [Google Scholar]
- Lu, S.; Qiao, S. Optimization and Numerical Simulation of Pressure Characteristics of Vertical Drop Shaft Structures. Adv. Water Sci. Technol. 2023, 43, 24–29+43. [Google Scholar]
- Liu, C. Numerical Simulation of Energy Dissipation in Vertical Shaft Flood Discharge Tunnels Based on CFD. Hydraul. Sci. Cold Reg. Eng. 2022, 5, 10–13. [Google Scholar]
- Gao, M. Hydraulic Characteristics Simulation Analysis of Vertical Swirling Flood Discharge Shaft Based on FLUENT. Shaanxi Water Resour. 2022, 4–8. [Google Scholar] [CrossRef]
- Yao, Y. Experimental Study on Hydraulic Characteristics of Deflector Plate Type Deep Tunnel Vertical Shaft Energy Dissipation. Master’s Thesis, Southwest Jiaotong University, Chengdu, China, 2020. [Google Scholar]
- Vallet, A.; Burluka, A.A.; Borghi, R. Development of a Eulerian model for the “Atomization” of a liquid jet. At. Sprays 2001, 11, 619–642. [Google Scholar] [CrossRef]
- Wu, P. Numerical Simulation Study of a New Energy Dissipation Device for Horizontal Swirling Flow in Vertical Shafts. Master’s Thesis, Hefei University of Technology, Hefei, China, 2010. [Google Scholar]
- Lu, J.; Ding, C. Verification of a Mathematical Model for Ventilation in Sewage Pipelines Based on CFD. China Water Wastewater 2014, 30, 67–70. [Google Scholar]
- Li, L.; Xie, M.; Jia, M. Numerical Analysis of Different Turbulence Models for Sprays Using the New Euler Model. J. Intern. Combust. Engines 2018, 36, 76–82. [Google Scholar]
- Zhu, D.; Gu, P.; Chu, H.; Lin, F.; Lu, S. Numerical Study on the Layout and Optimization of Airflow Organization in the Grate Room of Sewage Pumping Stations. J. Nanjing Norm. Univ. (Eng. Technol. Ed.) 2019, 19, 43–49+81. [Google Scholar]
- Gao, W.; Ma, L.; Zhu, X.; Li, S.; Jiang, X. Non-Isothermal Thermal Decomposition Kinetics of Acrylic Artificial Stone Waste Powder/ABS Composites. Plastics 2024, 53, 52–57. [Google Scholar]
- Zheng, S.; Li, W.; Yang, H.; Chen, S.; Wei, Q. Research and Application Progress on the Modification of 3D Printed Polylactic Acid. Mater. Rev. 2024, 38, 256–265. [Google Scholar]
- Teng, Y. Dissolution Behavior of Hydrogen Sulfide in Water. Arid. Environ. Monit. 1994, 11–13+60. [Google Scholar]
- Zhang, L.; De Schryver, P.; De Gusseme, B.; De Muynck, W.; Boon, N.; Verstraete, W. Chemical and biological technologies for hydrogen sulfide emission in sewer systems: A review. Water Res. 2008, 42, 1–12. [Google Scholar] [CrossRef] [PubMed]





| Model Size | Spiral Pallet Dimensions | |||
|---|---|---|---|---|
| Height | Diameter | Screw pitch | Spiral number | Pallet width |
| 1000 mm | 170 mm | 76 mm | 12 | 40 mm |
| Model Size | Spiral Pallet Dimensions | |||
|---|---|---|---|---|
| Height | Diameter | Screw pitch | Spiral number | Pallet width |
| 1000 mm | 170 mm | 76 mm | 10 | 40 mm |
| Preparation of Aqueous Solution of 5 L S2− | ||
|---|---|---|
| Concentration | Dosage of Na2S·9H2O | Take the Dosage from a Solution with a Concentration of 5g/L |
| 5 g/L | 187.231 g | - |
| 10 mg/L | 0.374 g | 10 mL |
| 20 mg/L | 0.749 g | 20 mL |
| 40 mg/L | 1.498 g | 40 mL |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, H. Research and Prevention of Harmful Gases in Special Structures of Urban Deep Drainage Systems. Water 2024, 16, 2481. https://doi.org/10.3390/w16172481
Liu H. Research and Prevention of Harmful Gases in Special Structures of Urban Deep Drainage Systems. Water. 2024; 16(17):2481. https://doi.org/10.3390/w16172481
Chicago/Turabian StyleLiu, Hao. 2024. "Research and Prevention of Harmful Gases in Special Structures of Urban Deep Drainage Systems" Water 16, no. 17: 2481. https://doi.org/10.3390/w16172481
APA StyleLiu, H. (2024). Research and Prevention of Harmful Gases in Special Structures of Urban Deep Drainage Systems. Water, 16(17), 2481. https://doi.org/10.3390/w16172481






