2DH Numerical Study of Solitary Wave Processes around an Idealized Reef-Fringed Island
Abstract
1. Introduction
2. Model and Validation
2.1. Introduction of the Numerical Method
2.2. Model Validation
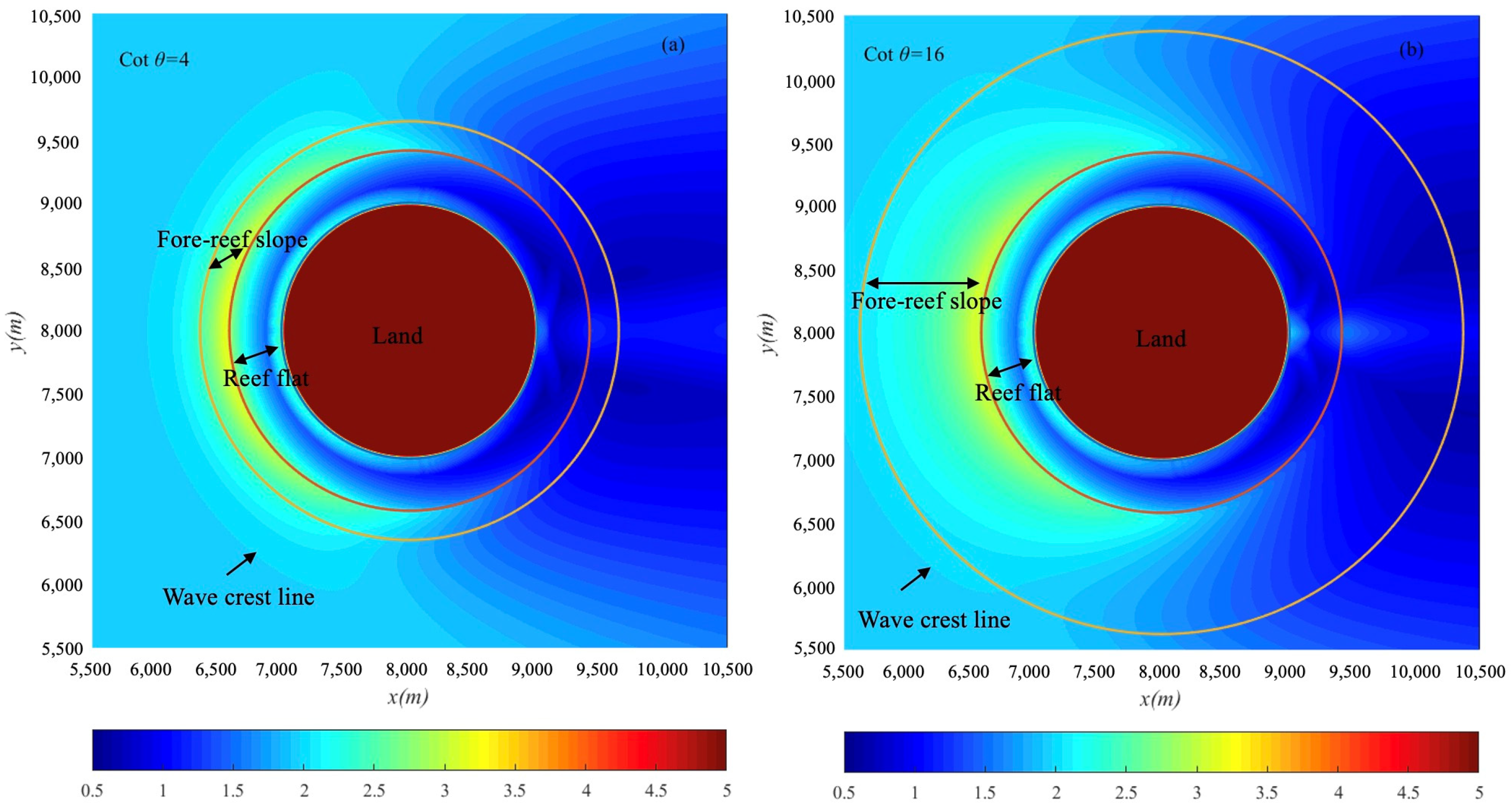
3. Typical Propagation Process around a Reef-Fringed Island
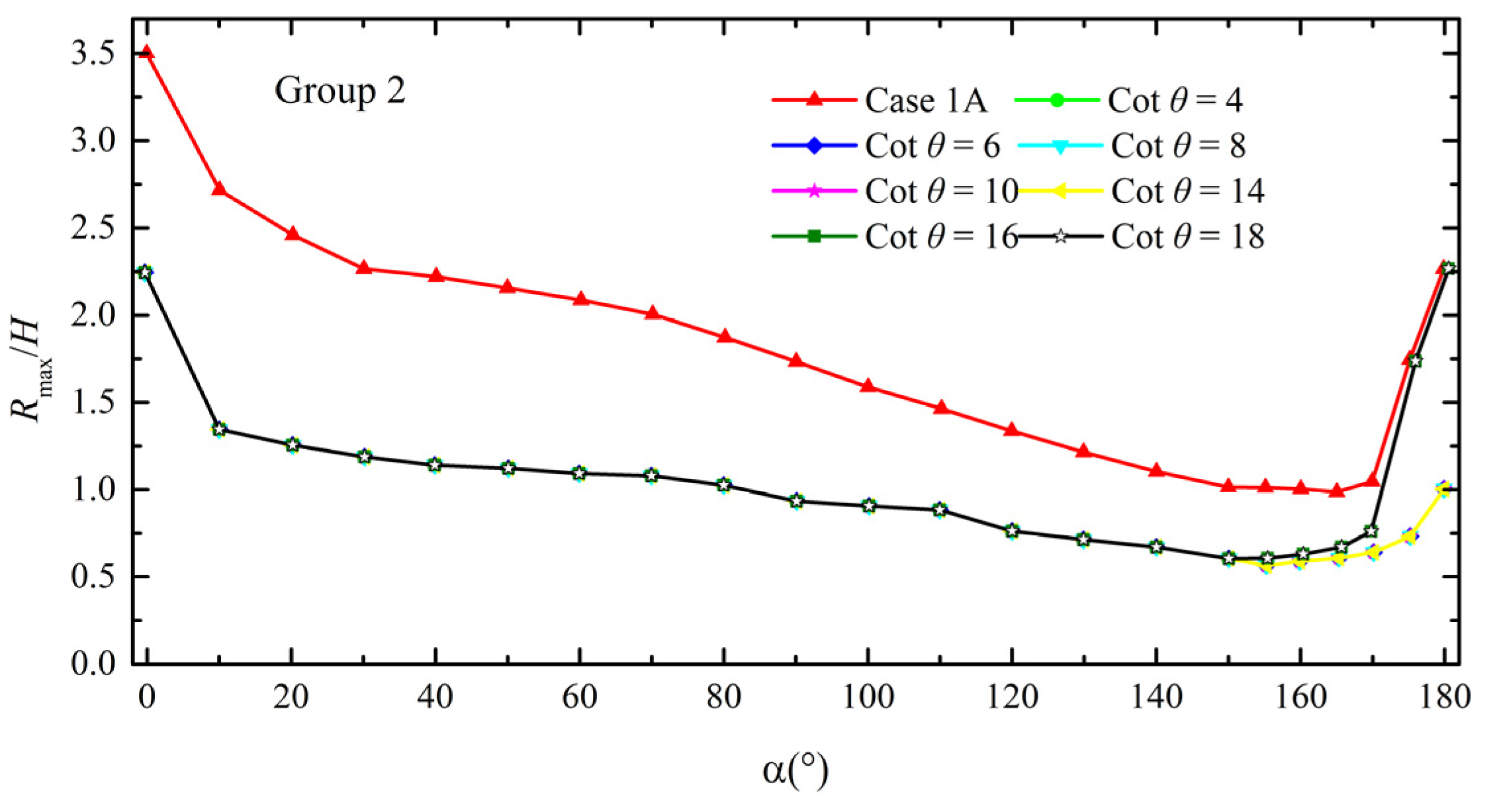
4. Effects of Morphological and Hydrodynamic Parameters
5. Conclusions
- (1)
- The presence of coral reefs effectively reduces the maximum run-up heights around an island. Run-up enhancement at the back of the reef-fringed island is induced by collisions of trapped waves.
- (2)
- Maximum run-up heights around the reef-fringed island and the area of run-up enhancement on the lee side increase with an increase in reef flat water depth. Sea-level rise associated with climate change will cause coral reefs to lose their protective capability on the lee side of the island.
- (3)
- The maximum run-up heights around the reef-fringed island decrease as the reef flat width increases. When the reef flat width is very narrow, the presence of coral reefs may even enhance the tsunami hazards around the island. Similarly, the maximum run-up heights around the reef-fringed island decrease as the reef surface roughness increases. Coral bleaching associated with climate change will not only reduce the defensive effect of coral reefs against tsunami hazards but also exacerbate the degree of tsunami disasters around the island.
- (4)
- The fore-reef slope has little impact on the maximum run-up heights around the reef-fringed island. But when the fore-reef slope gets mild to a certain extent, a larger body of water will be trapped by the reef-fringed island and increase the run-up heights around the back of the island.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Synolakis, C.E. The runup of solitary waves. J. Fluid Mech. 1987, 185, 523–545. [Google Scholar] [CrossRef]
- Briggs, M.J.; Synolakis, C.E.; Harkins, G.S.; Green, D.R. Laboratory experiments of tsunami runup on a circular island. Pure Appl. Geophys. 1995, 144, 569–593. [Google Scholar] [CrossRef]
- Maiti, S.; Sen, D. Computation of solitary waves during propagation and runup on a slope. Ocean Eng. 1999, 26, 1063–1083. [Google Scholar] [CrossRef]
- Lin, P. A numerical study of solitary wave interaction with rectangular obstacles. Coast. Eng. 2004, 51, 35–51. [Google Scholar] [CrossRef]
- Chang, Y.-H.; Hwang, K.-S.; Hwung, H.-H. Large-scale laboratory measurements of solitary wave inundation on a 1:20 slope. Coast. Eng. 2009, 56, 1022–1034. [Google Scholar] [CrossRef]
- Shakeel, M.; Attaullah; Shah, N.A.; Chung, J.D. Modified Exp-Function Method to Find Exact Solutions of Microtubules Nonlinear Dynamics Models. Symmetry 2023, 15, 360. [Google Scholar] [CrossRef]
- Shakeel, M.; Attaullah; El-Zahar, E.R.; Shah, N.A.; Chung, J.D. Generalized Exp-Function Method to Find Closed Form Solutions of Nonlinear Dispersive Modified Benjamin–Bona–Mahony Equation Defined by Seismic Sea Waves. Mathematics 2022, 10, 1026. [Google Scholar] [CrossRef]
- Li, Y.; Tang, B.; Geng, B.; Jiao, S. Fractional Order Fuzzy Dispersion Entropy and Its Application in Bearing Fault Diagnosis. Fractal Fract. 2022, 6, 544. [Google Scholar] [CrossRef]
- Attaullah; Shakeel, M.; Ahmad, B.; Shah, N.A.; Chung, J.D. Solitons Solution of Riemann Wave Equation via Modified Exp Function Method. Symmetry 2022, 14, 2574. [Google Scholar] [CrossRef]
- Shakeel, M.; Attaullah; Kbiri Alaoui, M.; Zidan, A.M.; Shah, N.A.; Weera, W. Closed-Form Solutions in a Magneto-Electro-Elastic Circular Rod via Generalized Exp-Function Method. Mathematics 2022, 10, 3400. [Google Scholar] [CrossRef]
- Danielsen, F.; Sørensen, M.K.; Olwig, M.F.; Selvam, V.; Parish, F.; Burgess, N.D.; Hiraishi, T.; Karunagaran, V.M.; Rasmussen, M.S.; Hansen, L.B.; et al. The Asian Tsunami: A Protective Role for Coastal Vegetation. Science 2005, 310, 643. [Google Scholar] [CrossRef] [PubMed]
- Yanagisawa, H.; Koshimura, S.; Goto, K.; Miyagi, T.; Imamura, F.; Ruangrassamee, A.; Tanavud, C. The reduction effects of mangrove forest on a tsunami based on field surveys at Pakarang Cape, Thailand and numerical analysis. Estuar. Coast. Shelf Sci. 2009, 81, 27–37. [Google Scholar] [CrossRef]
- Adger, W.N.; Hughes, T.P.; Folke, C.; Carpenter, S.R.; Rockström, J. Social-Ecological Resilience to Coastal Disasters. Science 2005, 309, 1036. [Google Scholar] [CrossRef] [PubMed]
- Baird, A.H.; Campbell, S.J.; Anggoro, A.W.; Ardiwijaya, R.L.; Fadli, N.; Herdiana, Y.; Kartawijaya, T.; Mahyiddin, D.; Mukminin, A.; Pardede, S.T.; et al. Acehnese Reefs in the Wake of the Asian Tsunami. Curr. Biol. 2005, 15, 1926–1930. [Google Scholar] [CrossRef] [PubMed]
- Fernando, H.J.S.; McCulley, J.L.; Mendis, S.G.; Perera, K. Coral poaching worsens tsunami destruction in Sri Lanka. Eos Trans. Am. Geophys. Union 2005, 86, 301–304. [Google Scholar] [CrossRef]
- Goff, J.; Liu, P.L.F.; Higman, B.; Morton, R.; Jaffe, B.E.; Fernando, H.; Lynett, P.; Fritz, H.; Synolakis, C.; Fernando, S. Sri Lanka Field Survey after the December 2004 Indian Ocean Tsunami. Earthq. Spectra 2006, 22, 155–172. [Google Scholar] [CrossRef]
- Fernando, H.J.S.; Samarawickrama, S.P.; Balasubramanian, S.; Hettiarachchi, S.S.L.; Voropayev, S. Effects of porous barriers such as coral reefs on coastal wave propagation. J. Hydro-Environ. Res. 2008, 1, 187–194. [Google Scholar] [CrossRef]
- Quiroga, P.D.; Cheung, K.F. Laboratory study of solitary-wave transformation over bed-form roughness on fringing reefs. Coast. Eng. 2013, 80, 35–48. [Google Scholar] [CrossRef]
- Yao, Y.; He, F.; Tang, Z.; Liu, Z. A study of tsunami-like solitary wave transformation and run-up over fringing reefs. Ocean Eng. 2018, 149, 142–155. [Google Scholar] [CrossRef]
- Kunkel, C.M.; Hallberg, R.W.; Oppenheimer, M. Coral reefs reduce tsunami impact in model simulations. Geophys. Res. Lett. 2006, 33, L23612. [Google Scholar] [CrossRef]
- Yao, Y.; Huang, Z.; Monismith, S.G.; Lo, E.Y.M. 1DH Boussinesq modeling of wave transformation over fringing reefs. Ocean Eng. 2012, 47, 30–42. [Google Scholar] [CrossRef]
- Nwogu, O.; Demirbilek, Z. Infragravity Wave Motions and Runup over Shallow Fringing Reefs. J. Waterw. Port Coast. Ocean Eng. 2010, 136, 295–305. [Google Scholar] [CrossRef]
- Roeber, V.; Cheung, K. Boussinesq-type model for energetic breaking waves in fringing reef environments. Coast. Eng. 2012, 70, 1–20. [Google Scholar] [CrossRef]
- Fang, K.; Liu, Z.; Zou, Z. Fully nonlinear modeling wave transformation over fringing reefs using shock-capturing Boussinesq model. J. Coast. Res. 2016, 32, 164–171. [Google Scholar] [CrossRef]
- Ning, Y.; Liu, W.; Zhao, X.; Zhang, Y.; Sun, Z. Study of irregular wave run-up over fringing reefs based on a shock-capturing Boussinesq model. Appl. Ocean Res. 2019, 84, 216–224. [Google Scholar] [CrossRef]
- Liu, W.; Ning, Y. Numerical simulation of wave propagation over coral reef bathymetries based on fully-dispersive Boussinesq equations. J. Chang. Univ. Sci. Technol. (Nat. Sci.) 2023, 20, 47–54. [Google Scholar] [CrossRef]
- Zhou, Q.; Zhan, J.-M.; Li, Y.S. Parametric Investigation of Breaking Solitary Wave Over Fringing Reef Based on Shock-Capturing Boussinesq Model. Coast. Eng. J. 2016, 58, 1650007. [Google Scholar] [CrossRef]
- Ning, Y.; Liu, W.; Sun, Z.; Zhao, X.; Zhang, Y. Parametric study of solitary wave propagation and runup over fringing reefs based on a Boussinesq wave model. J. Mar. Sci. Technol. 2019, 24, 512–525. [Google Scholar] [CrossRef]
- Liu, W.; Shao, K.; Ning, Y. A Study of the Maximum Momentum Flux in the Solitary Wave Run-Up Zone over Back-Reef Slopes Based on a Boussinesq Model. J. Mar. Sci. Eng. 2019, 7, 109. [Google Scholar] [CrossRef]
- Qu, K.; Liu, T.W.; Chen, L.; Yao, Y.; Kraatz, S.; Huang, J.X.; Lan, G.Y.; Jiang, C.B. Study on transformation and runup processes of tsunami-like wave over permeable fringing reef using a nonhydrostatic numerical wave model. Ocean Eng. 2022, 243, 110228. [Google Scholar] [CrossRef]
- Su, S.-F.; Ma, G. Modeling two-dimensional infragravity motions on a fringing reef. Ocean Eng. 2018, 153, 256–267. [Google Scholar] [CrossRef]
- Briggs, M.J.; Cho, Y.-S.; Kanoglu, U.; Liu, P.L.F.; Synolakis, C.E. Runup of solitary waves on a circular Island. J. Fluid Mech. 1995, 302, 259–285. [Google Scholar] [CrossRef]
- Shi, F.; Kirby, J.T.; Harris, J.C.; Geiman, J.D.; Grilli, S.T. A high-order adaptive time-stepping TVD solver for Boussinesq modeling of breaking waves and coastal inundation. Ocean Model. 2012, 43–44, 36–51. [Google Scholar] [CrossRef]
- Kirby, J.T.; Wei, G.; Chen, Q.; Kennedy, A.B.; Dalrymple, R.A. FUNWAVE 1.0. Fully Nonlinear Boussinesq Wave Model. Documentation and User’s Manual; Center for Applied Coastal Research, Department of Civil and Environmental Engineering, University of Delaware: Newark, DE, USA, 1998; Available online: www.udel.edu/kirby/papers/kirby-etal-cacr-98-06.pdf (accessed on 1 July 2024).
- Chen, Q. Fully nonlinear Boussinesq-type equations for waves and currents over porous beds. J. Eng. Mech. 2006, 132, 220–230. [Google Scholar] [CrossRef]
- Kennedy, A.B.; Chen, Q.; Kirby, J.T.; Dalrymple, R.A. Boussinesq modeling of wave transformation, breaking, and runup. I: 1D. J. Waterw. Port Coast. Ocean Eng. 2000, 126, 39–47. [Google Scholar] [CrossRef]
- Gelfenbaum, G.; Apotsos, A.; Stevens, A.W.; Jaffe, B. Effects of fringing reefs on tsunami inundation: American Samoa. Earth-Sci. Rev. 2011, 107, 12–22. [Google Scholar] [CrossRef]
- Choi, Y.-K.; Shi, F.; Malej, M.; Smith, J.M. Performance of various shock-capturing-type reconstruction schemes in the Boussinesq wave model, FUNWAVE-TVD. Ocean Model. 2018, 131, 86–100. [Google Scholar] [CrossRef]
- Tonelli, M.; Petti, M. Hybrid finite volume—Finite difference scheme for 2DH improved Boussinesq equations. Coast. Eng. 2009, 56, 609–620. [Google Scholar] [CrossRef]
- Shi, F.; Malej, M.; Smith, J.M.; Kirby, J.T. Breaking of ship bores in a Boussinesq-type ship-wake model. Coast. Eng. 2018, 132, 1–12. [Google Scholar] [CrossRef]
- Liu, W.; Liu, Y.; Zhao, X. Numerical study of Bragg reflection of regular water waves over fringing reefs based on a Boussinesq model. Ocean Eng. 2019, 190, 106415. [Google Scholar] [CrossRef]
- Chakrabarti, A.; Brandt, S.R.; Chen, Q.; Shi, F. Boussinesq modeling of wave-induced hydrodynamics in coastal wetlands. J. Geophys. Res. Ocean 2017, 122, 3861–3883. [Google Scholar] [CrossRef]
- Su, S.-F.; Ma, G.; Hsu, T.-W. Boussinesq modeling of spatial variability of infragravity waves on fringing reefs. Ocean Eng. 2015, 101, 78–92. [Google Scholar] [CrossRef]
- Shao, K.; Liu, W.; Gao, Y.; Ning, Y. The influence of climate change on tsunami-like solitary wave inundation over fringing reefs. J. Integr. Environ. Sci. 2019, 16, 71–88. [Google Scholar] [CrossRef]
- Wei, G.; Kirby, J.T. Time-Dependent Numerical Code for Extended Boussinesq Equations. J. Waterw. Port Coast. Ocean Eng. 1995, 121, 251–261. [Google Scholar] [CrossRef]
- Chen, Q.; Kirby James, T.; Dalrymple Robert, A.; Kennedy Andrew, B.; Chawla, A. Boussinesq Modeling of Wave Transformation, Breaking, and Runup. II: 2D. J. Waterw. Port Coast. Ocean Eng. 2000, 126, 48–56. [Google Scholar] [CrossRef]
- Quataert, E.; Storlazzi, C.; Rooijen, A.; Cheriton, O.; Van Dongeren, A. The influence of coral reefs and climate change on wave-driven flooding of tropical coastlines. Geophys. Res. Lett. 2015, 42, 6407–6415. [Google Scholar] [CrossRef]
- Vermeer, M.; Rahmstorf, S. Global sea level linked to global temperature. Proc. Natl. Acad. Sci. USA 2009, 106, 21527. [Google Scholar] [CrossRef]
- Grinsted, A.; Moore, J.C.; Jevrejeva, S. Reconstructing sea level from paleo and projected temperatures 200 to 2100 ad. Clim. Dyn. 2010, 34, 461–472. [Google Scholar] [CrossRef]
- Michael, S. The Great Barrier Reef: A catastrophe laid bare. The Guardian, 7 June 2016. [Google Scholar]

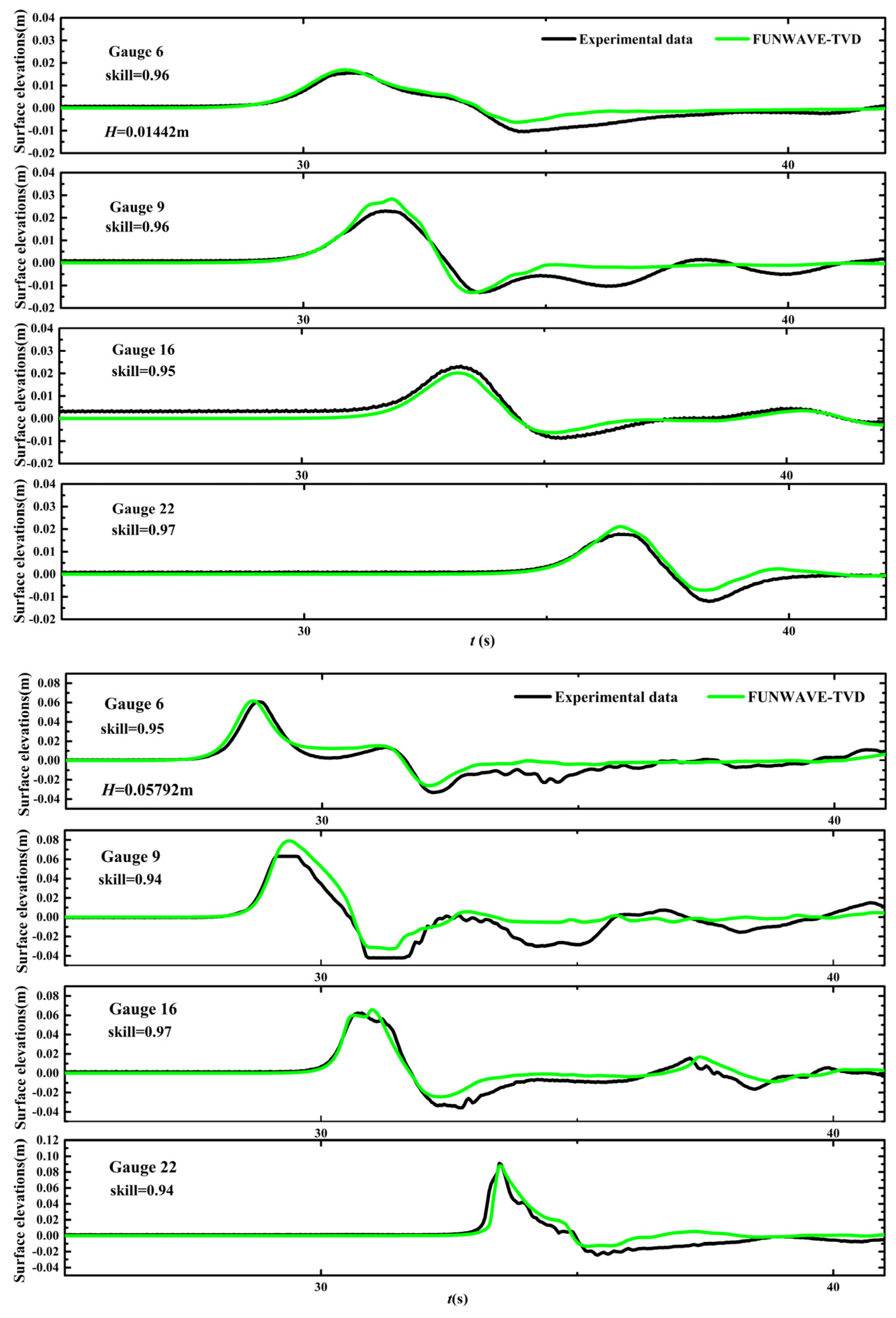
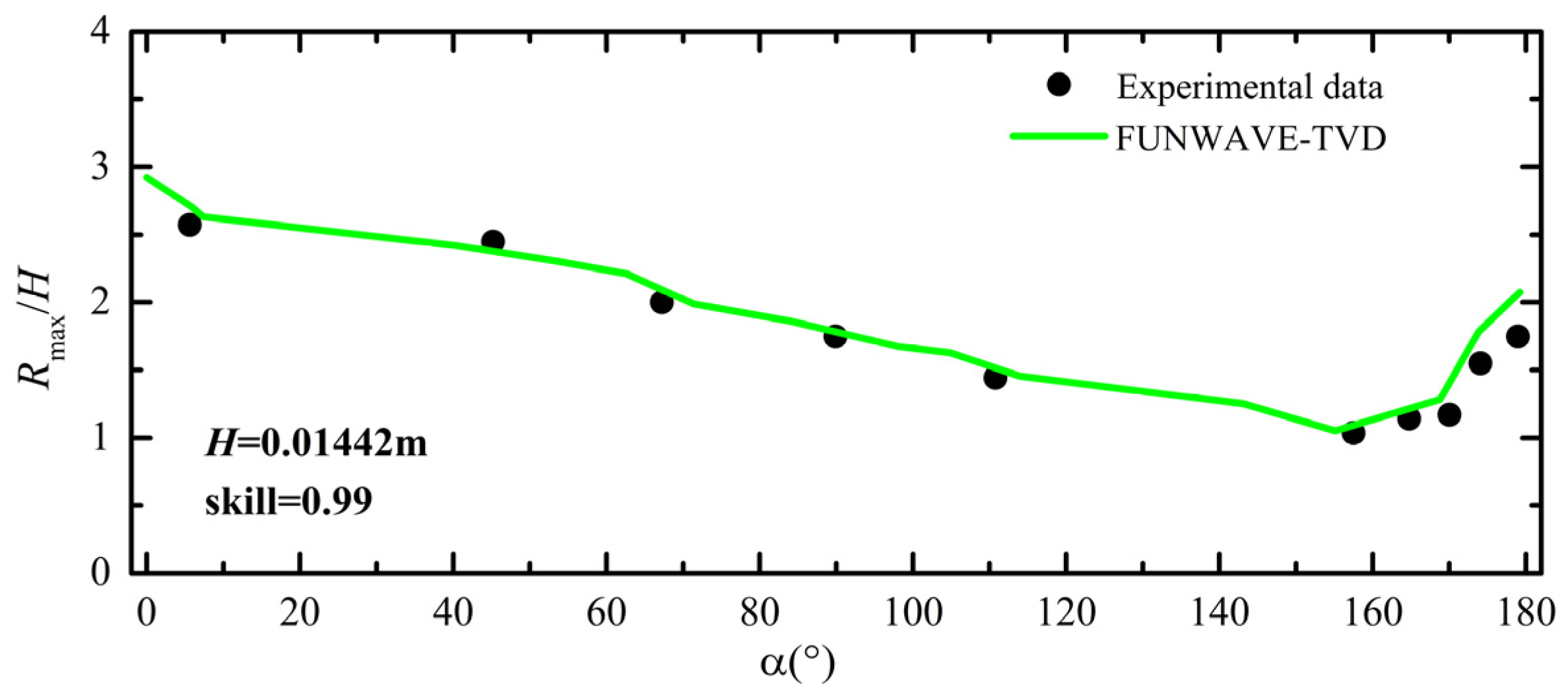
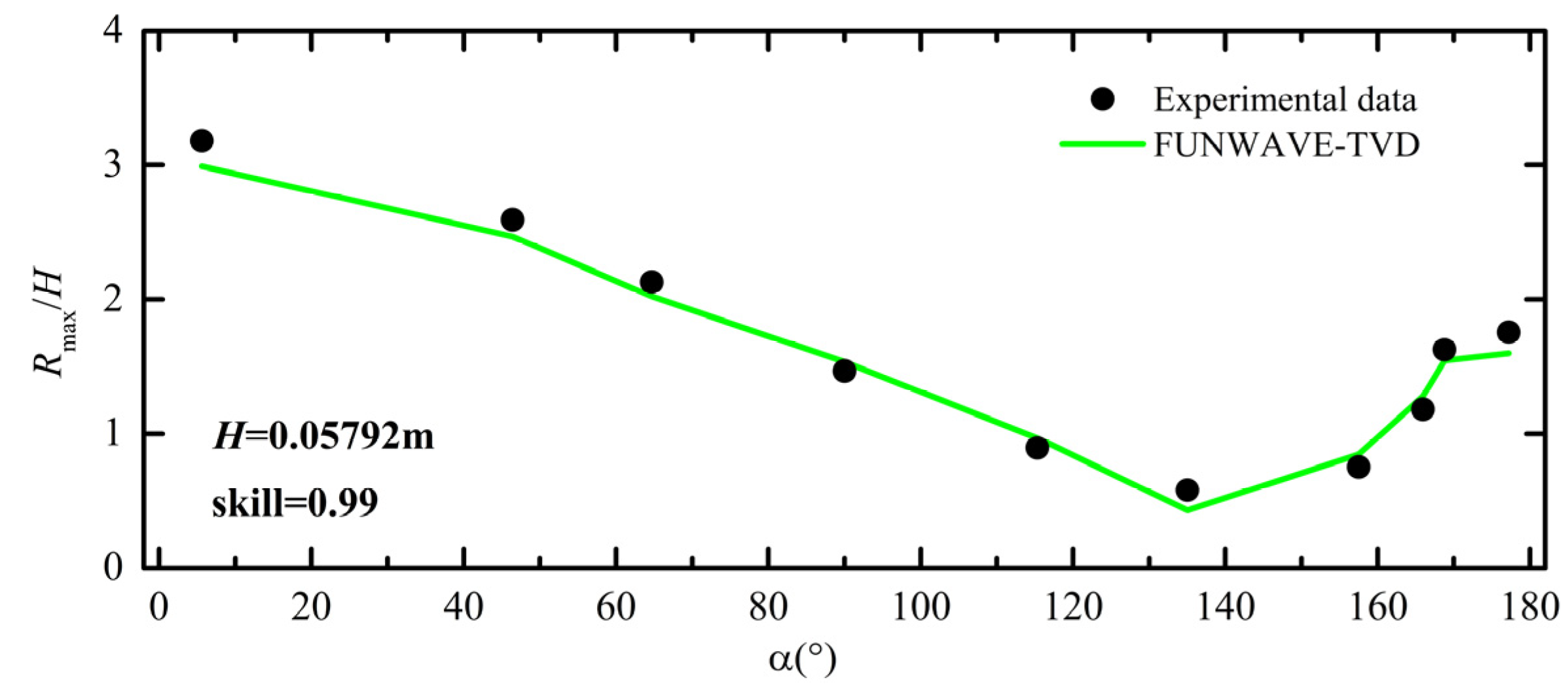

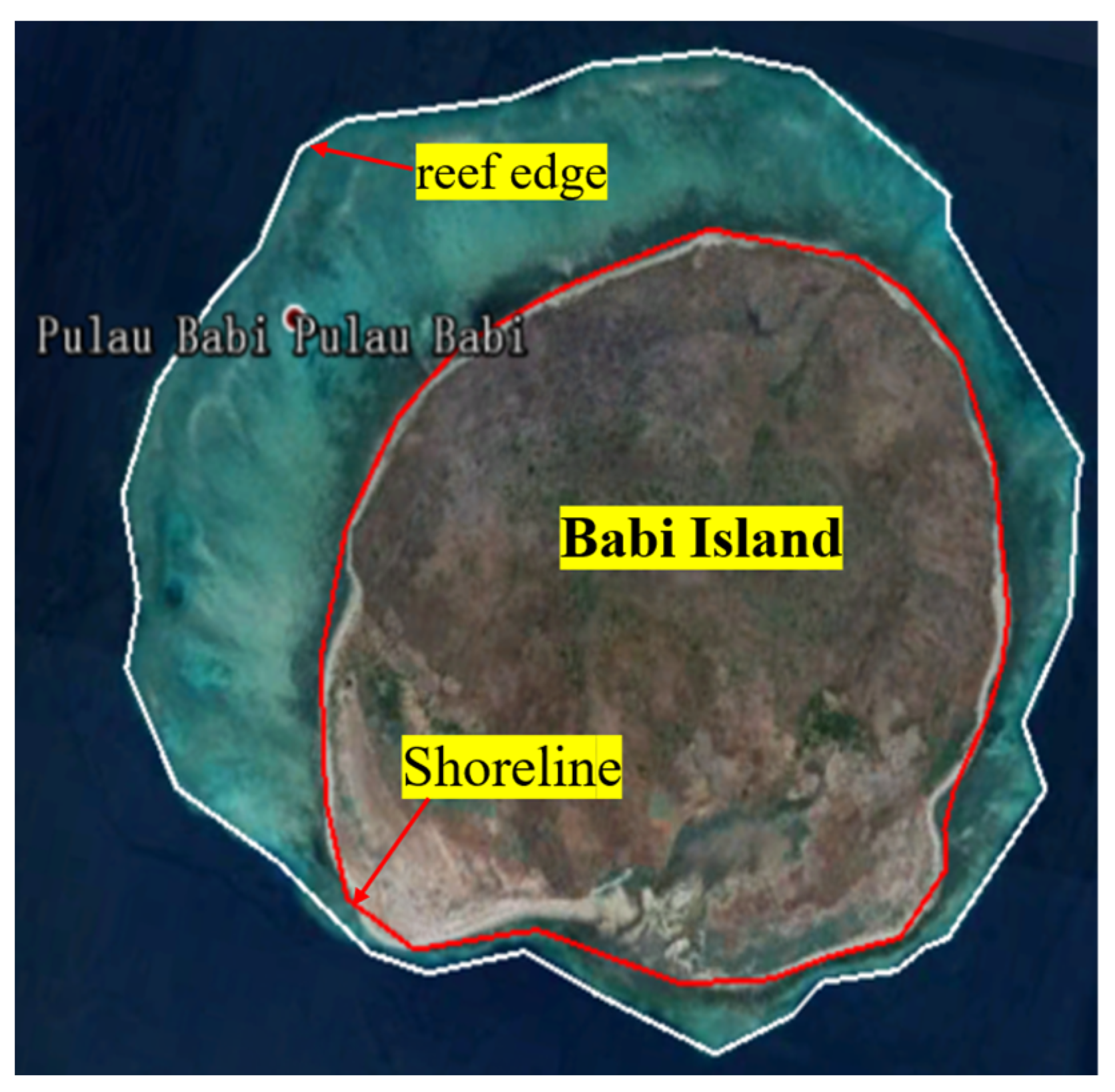
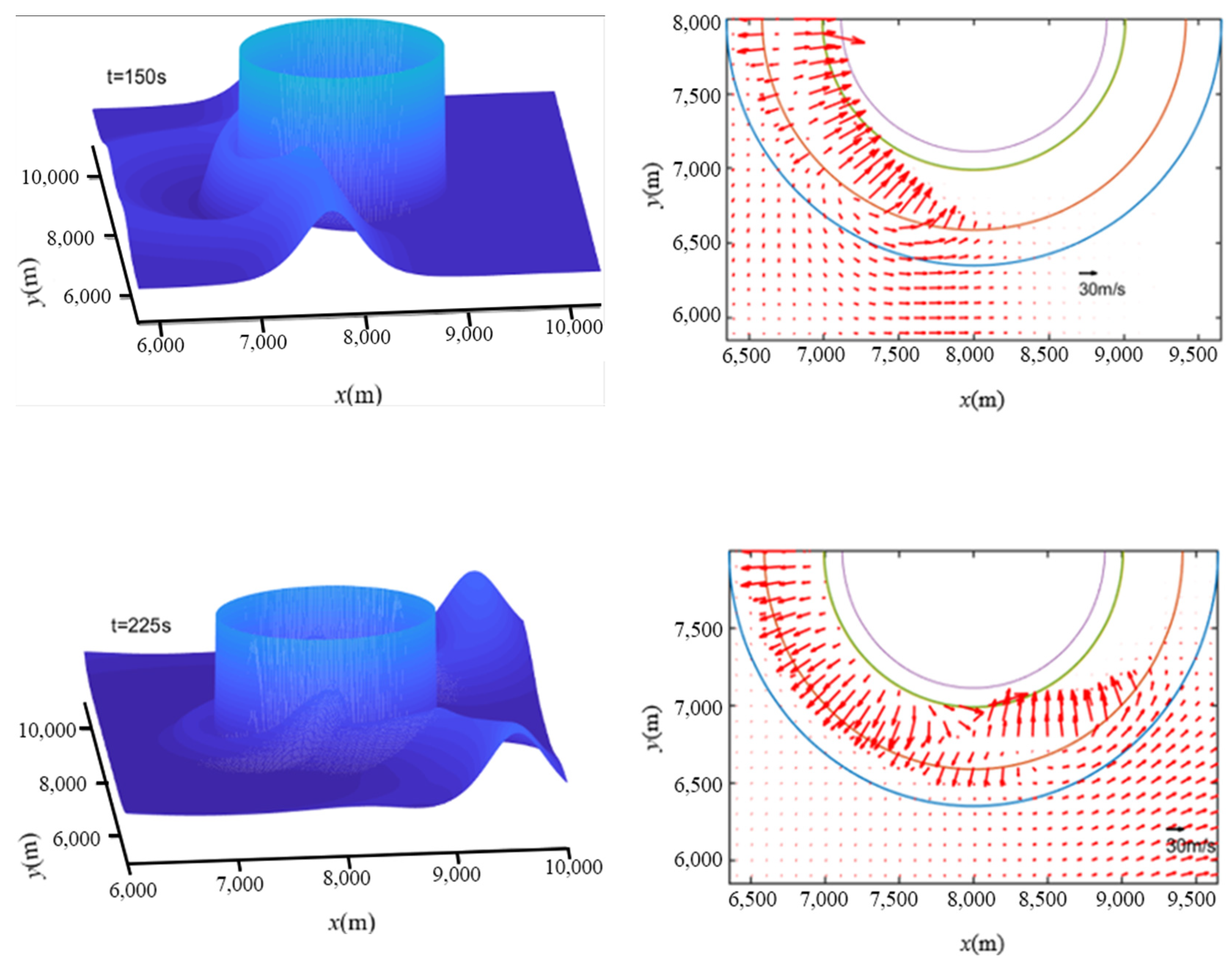
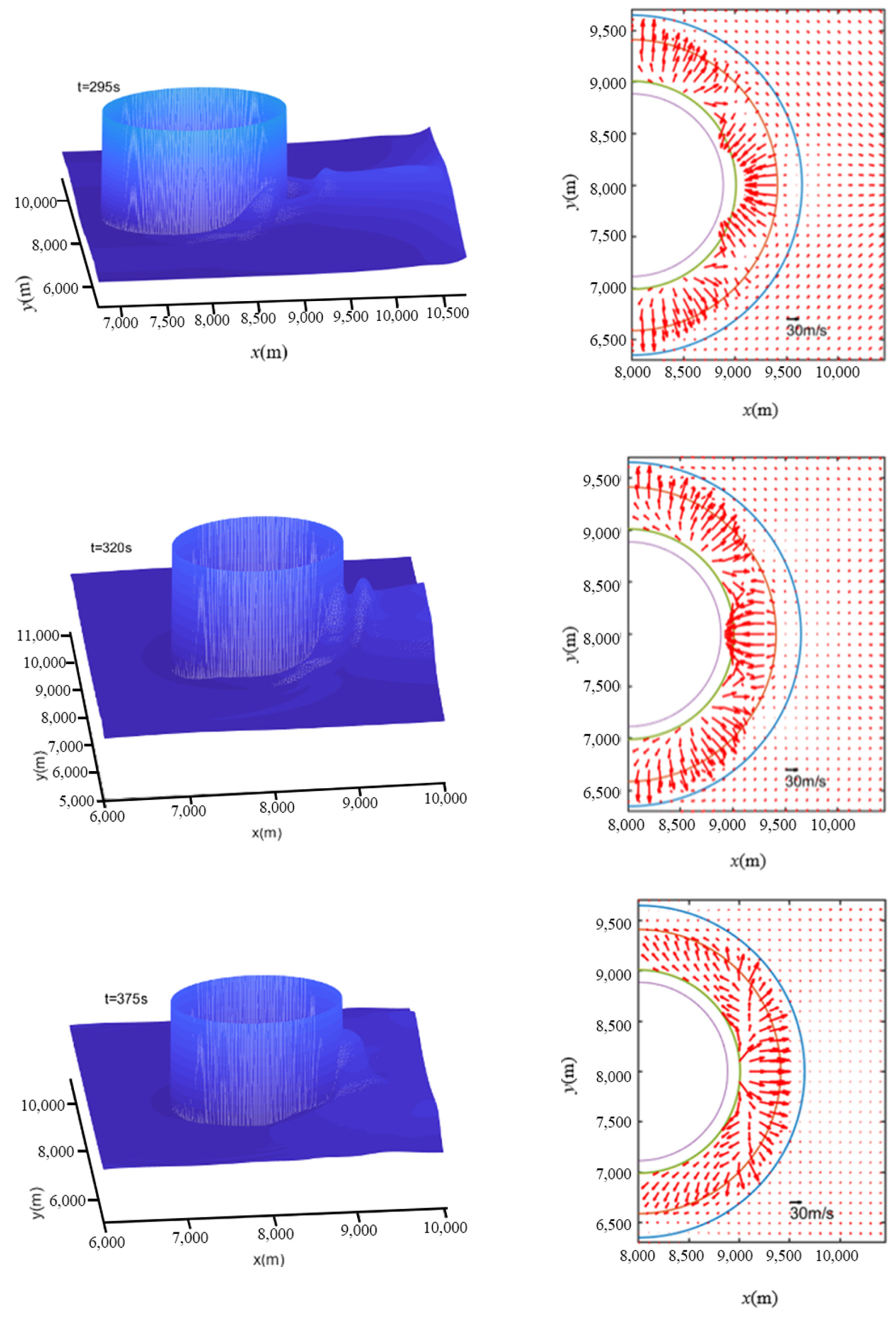
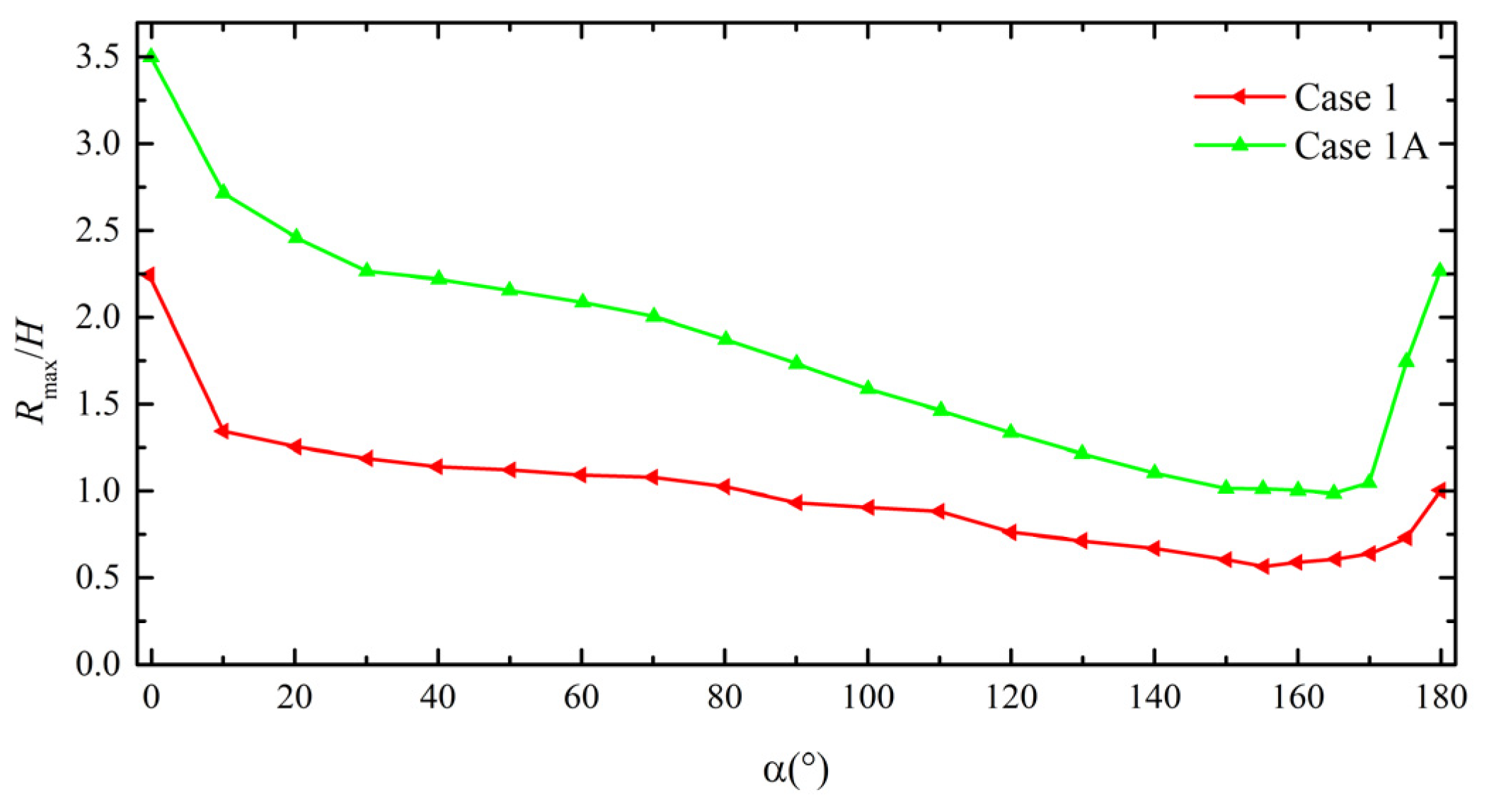
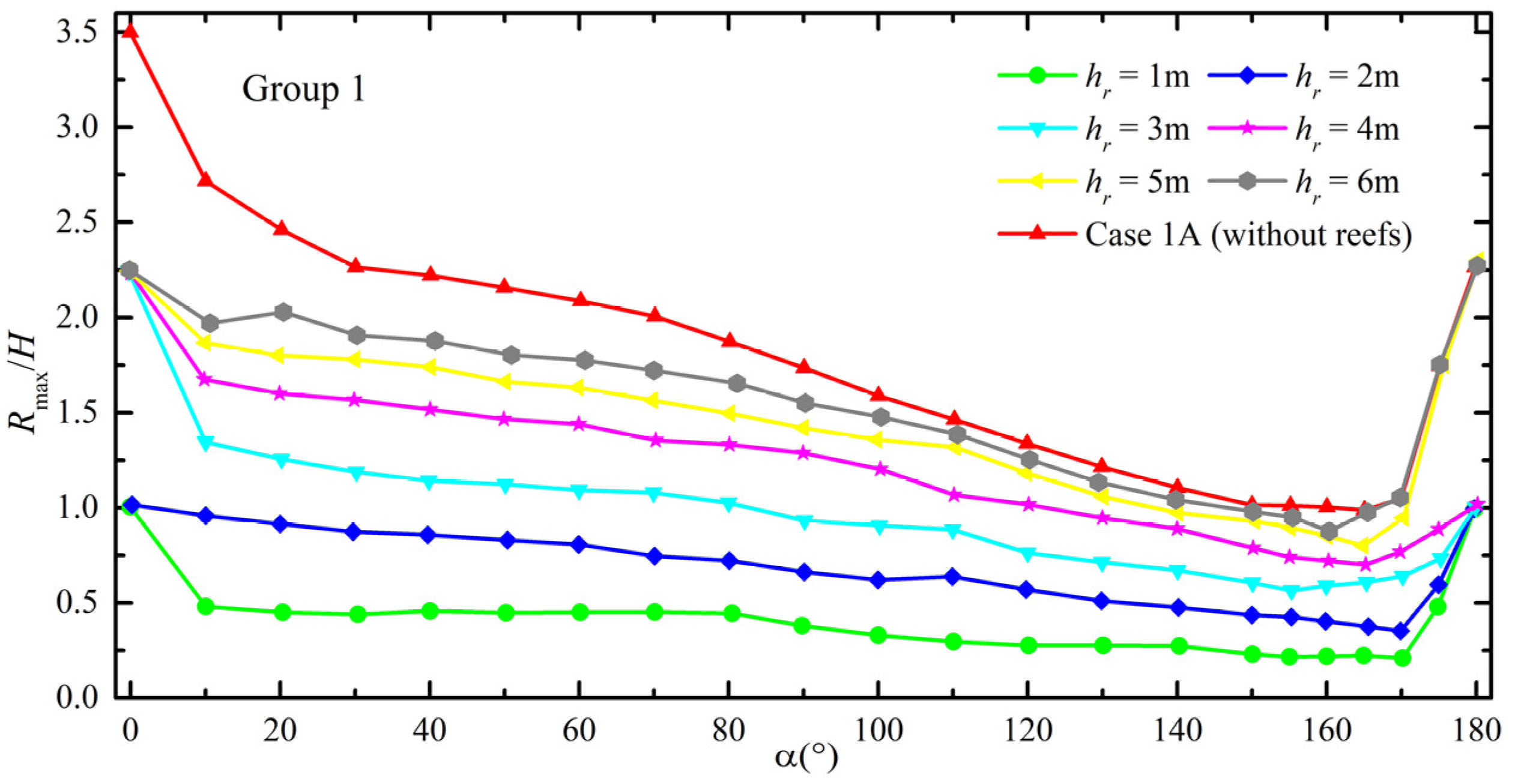




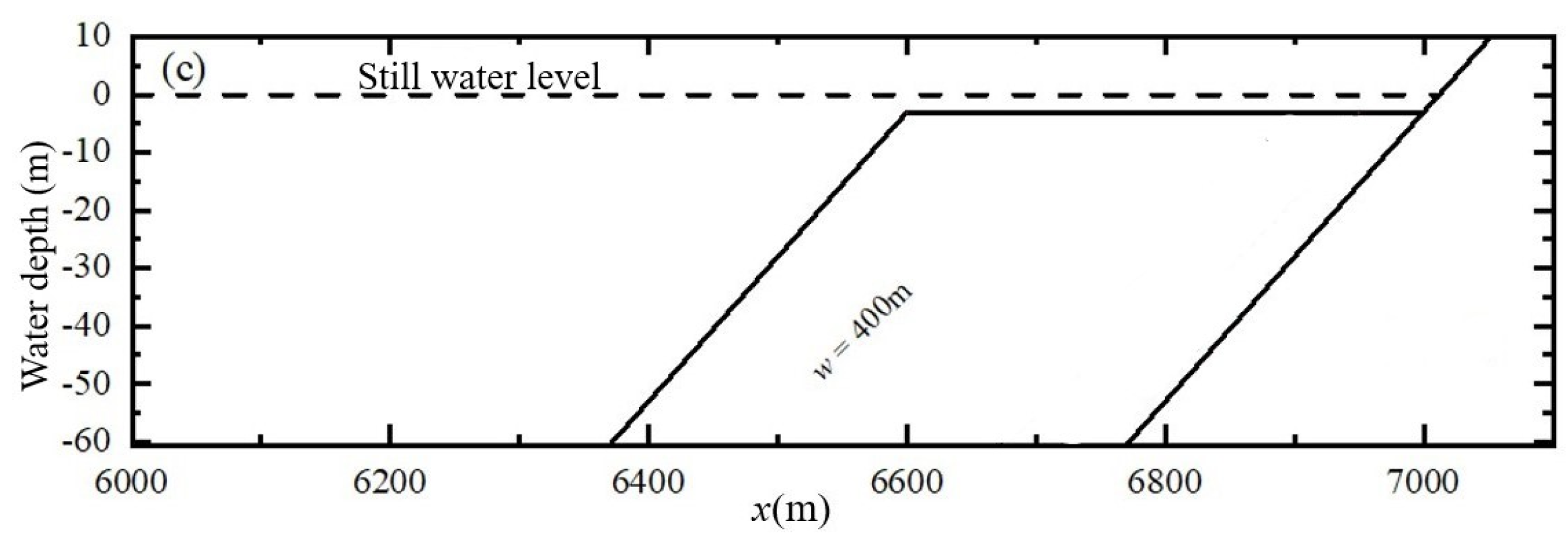
| Case No. | H (m) | hd (m) | w (m) | hr (m) | cot θ | cot β | n2 | Di (m) |
|---|---|---|---|---|---|---|---|---|
| Case 1 | 2 | 60.5 | 400 | 3 | 4 | 4 | 0.09 | 2016 |
| Case 1A | 2 | 60.5 | - | - | - | 4 | - | 2016 |
| Group No. | H (m) | hd (m) | w (m) | hr (m) | cot θ | cot β | n2 | Di (m) |
|---|---|---|---|---|---|---|---|---|
| Group 1 | 2 | 60.5 | 400 | 1~6 | 4 | 4 | 0.09 | 2016 |
| Group 2 | 2 | 60.5 | 400 | 3 | 4~18 | 4 | 0.09 | 2016 |
| Group 3 | 2 | 60.5 | 50~800 | 3 | 4 | 4 | 0.09 | 2016 |
| Group 4 | 2 | 60.5 | 400 | 3 | 4 | 4 | 0.02~0.09 | 2016 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, W.; Qian, F.; Ning, Y.; Cheng, R. 2DH Numerical Study of Solitary Wave Processes around an Idealized Reef-Fringed Island. Water 2024, 16, 1991. https://doi.org/10.3390/w16141991
Liu W, Qian F, Ning Y, Cheng R. 2DH Numerical Study of Solitary Wave Processes around an Idealized Reef-Fringed Island. Water. 2024; 16(14):1991. https://doi.org/10.3390/w16141991
Chicago/Turabian StyleLiu, Weijie, Feng Qian, Yue Ning, and Rongliang Cheng. 2024. "2DH Numerical Study of Solitary Wave Processes around an Idealized Reef-Fringed Island" Water 16, no. 14: 1991. https://doi.org/10.3390/w16141991
APA StyleLiu, W., Qian, F., Ning, Y., & Cheng, R. (2024). 2DH Numerical Study of Solitary Wave Processes around an Idealized Reef-Fringed Island. Water, 16(14), 1991. https://doi.org/10.3390/w16141991







