Monitoring of the Icing Process and Simulation of Its Formation Mechanism in the Cut Slope of Beihei Highway
Abstract
1. Introduction
2. Materials and Methods
2.1. Study Area
2.1.1. Geographic Location and Permafrost Distribution
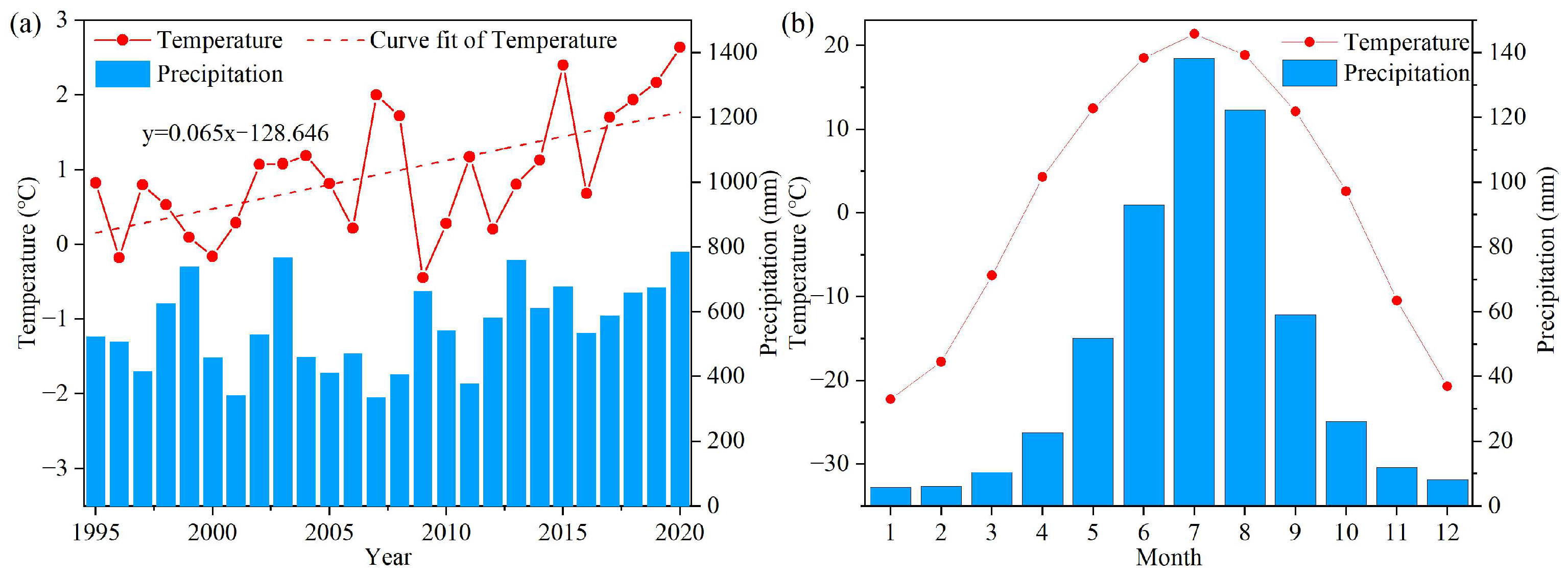
2.1.2. Climate
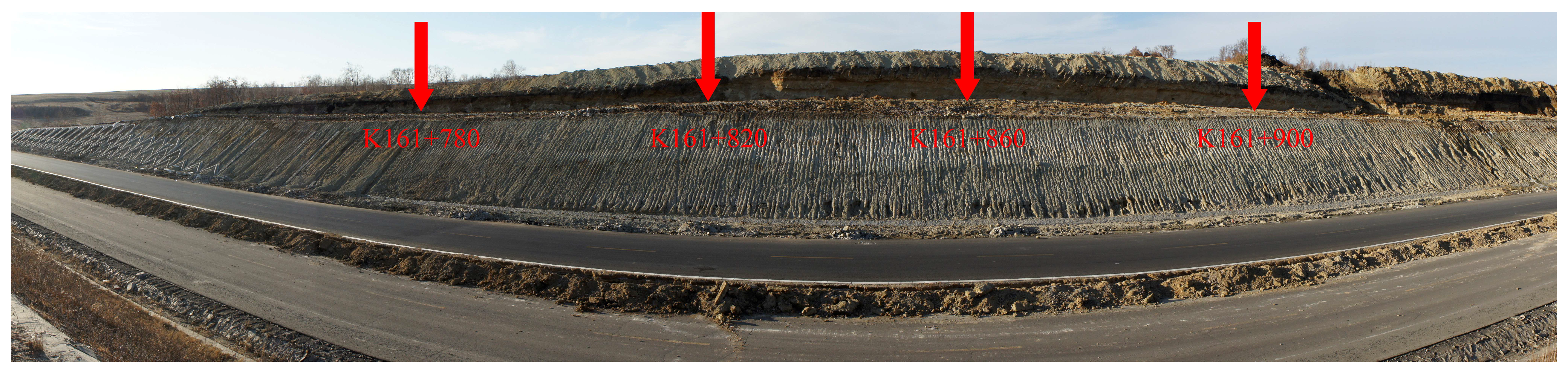
2.1.3. Geologic Environment
2.2. Analysis Methods
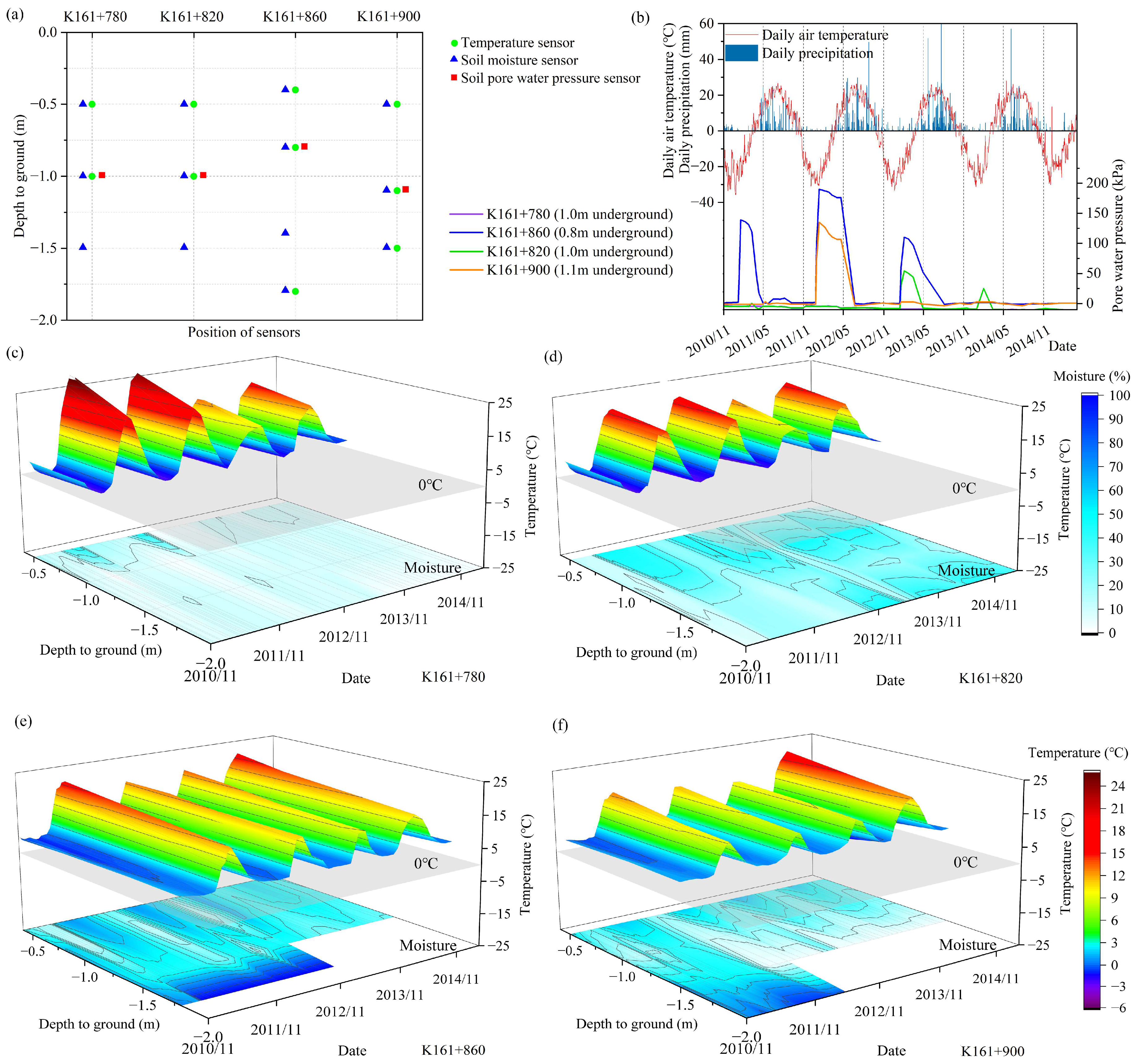
2.2.1. Position of Sensors
2.2.2. Numerical Simulation
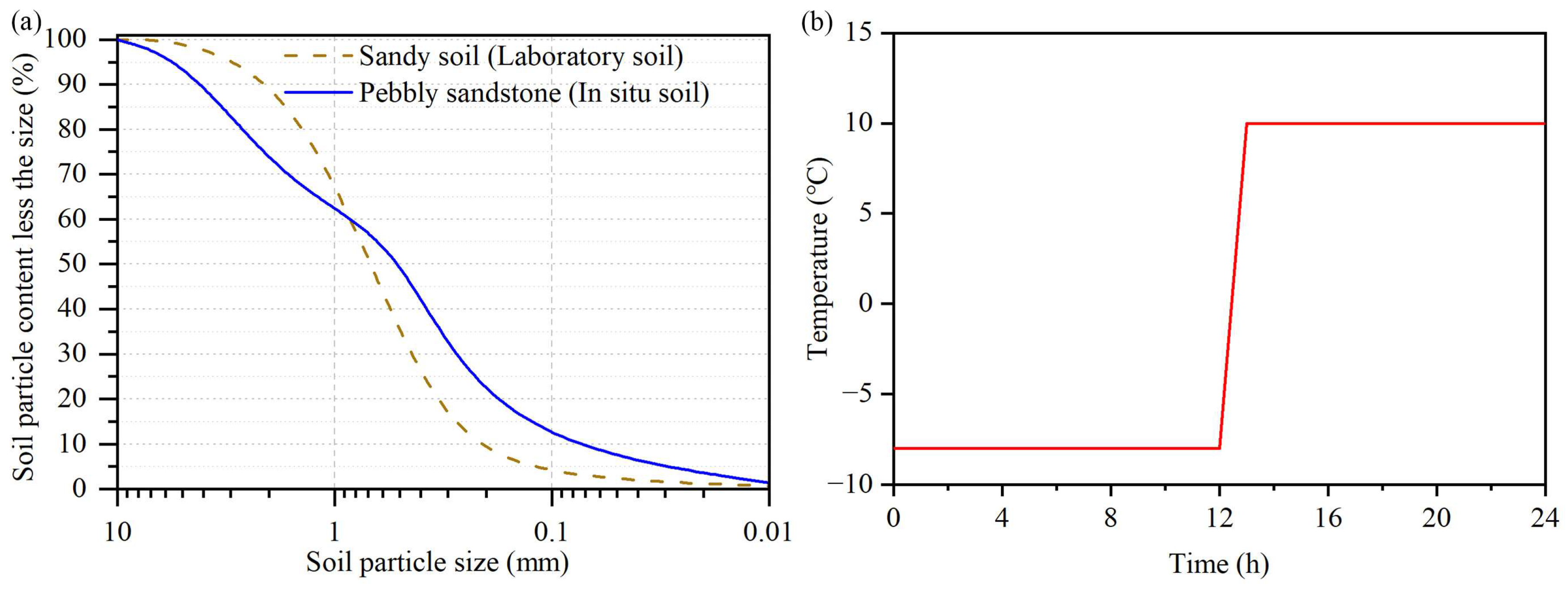
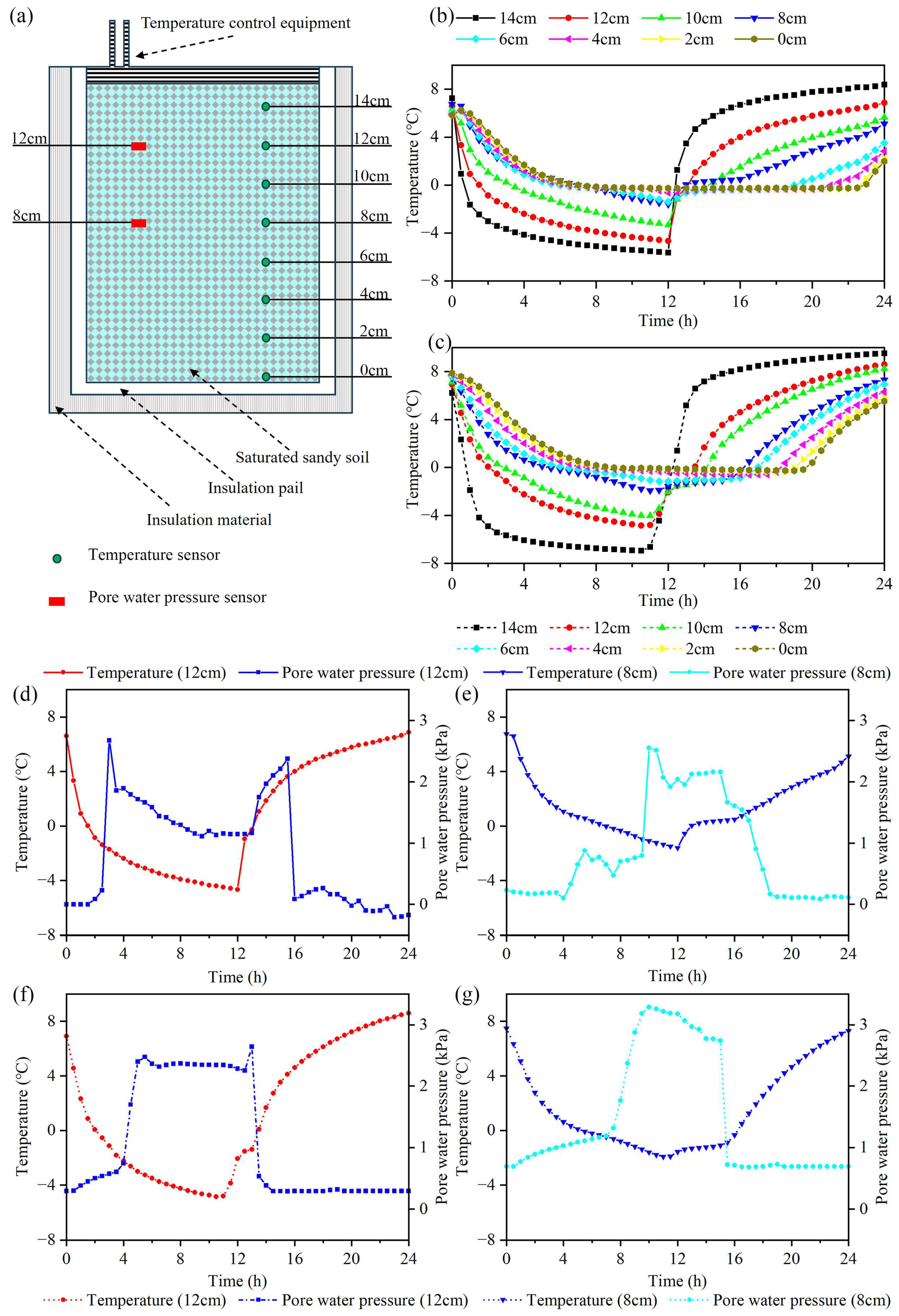
2.2.3. Controlled Laboratory Experiments
2.2.4. Climate Data
3. Results
3.1. Analysis of the Monitoring Data
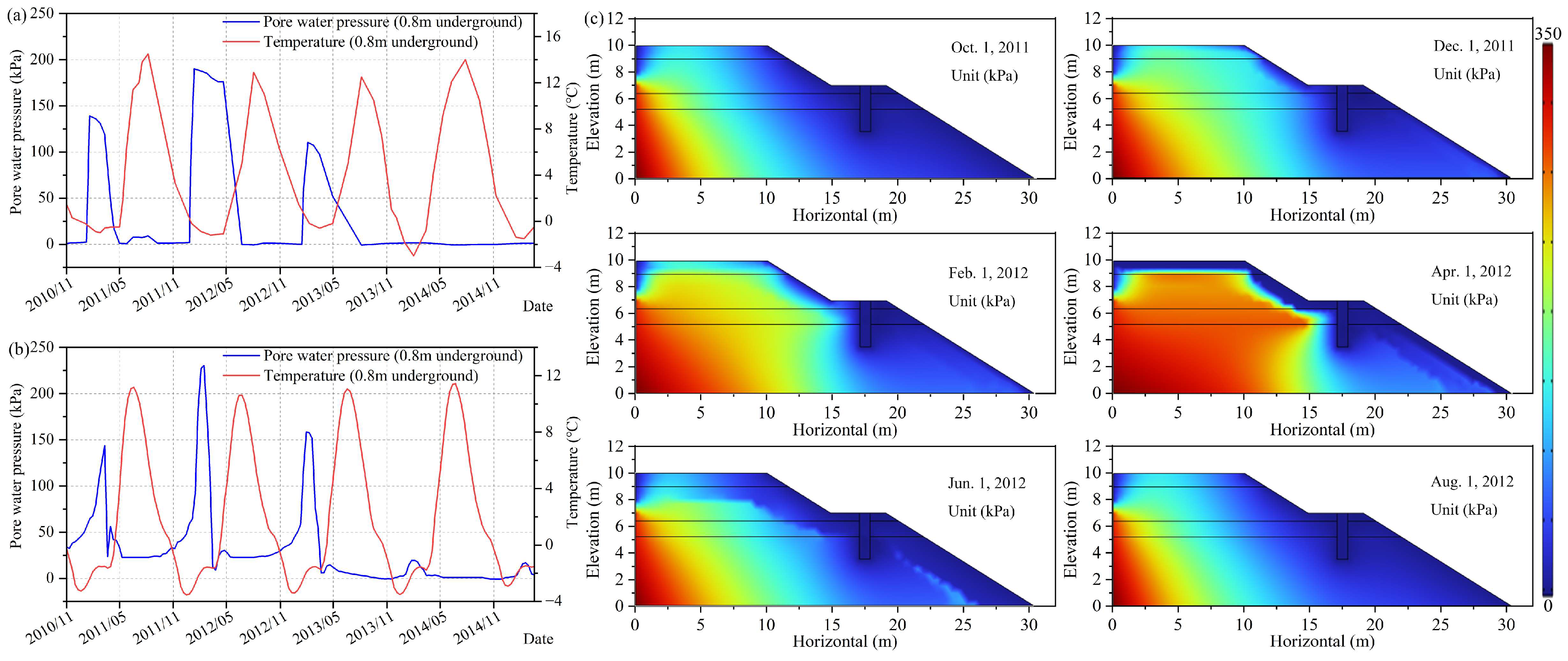
3.1.1. Analysis of the Monitoring Data of the K162 Section
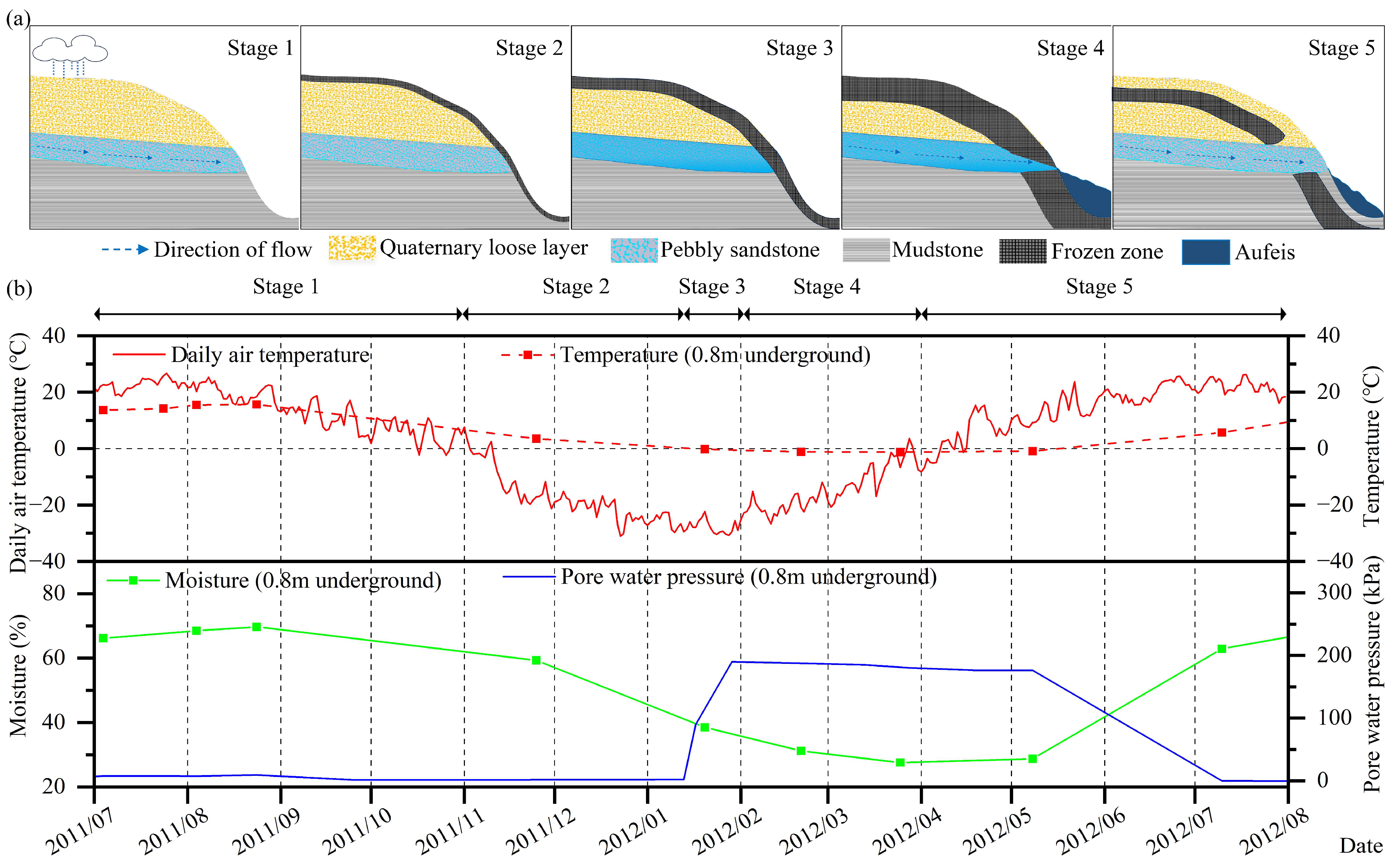
3.1.2. Analysis of Monitoring Data of the K161+860 Section
3.2. Numerical Simulation of Icing in Cut Slopes
3.2.1. Numerical Model
3.2.2. Soil Parameters and Boundary Conditions
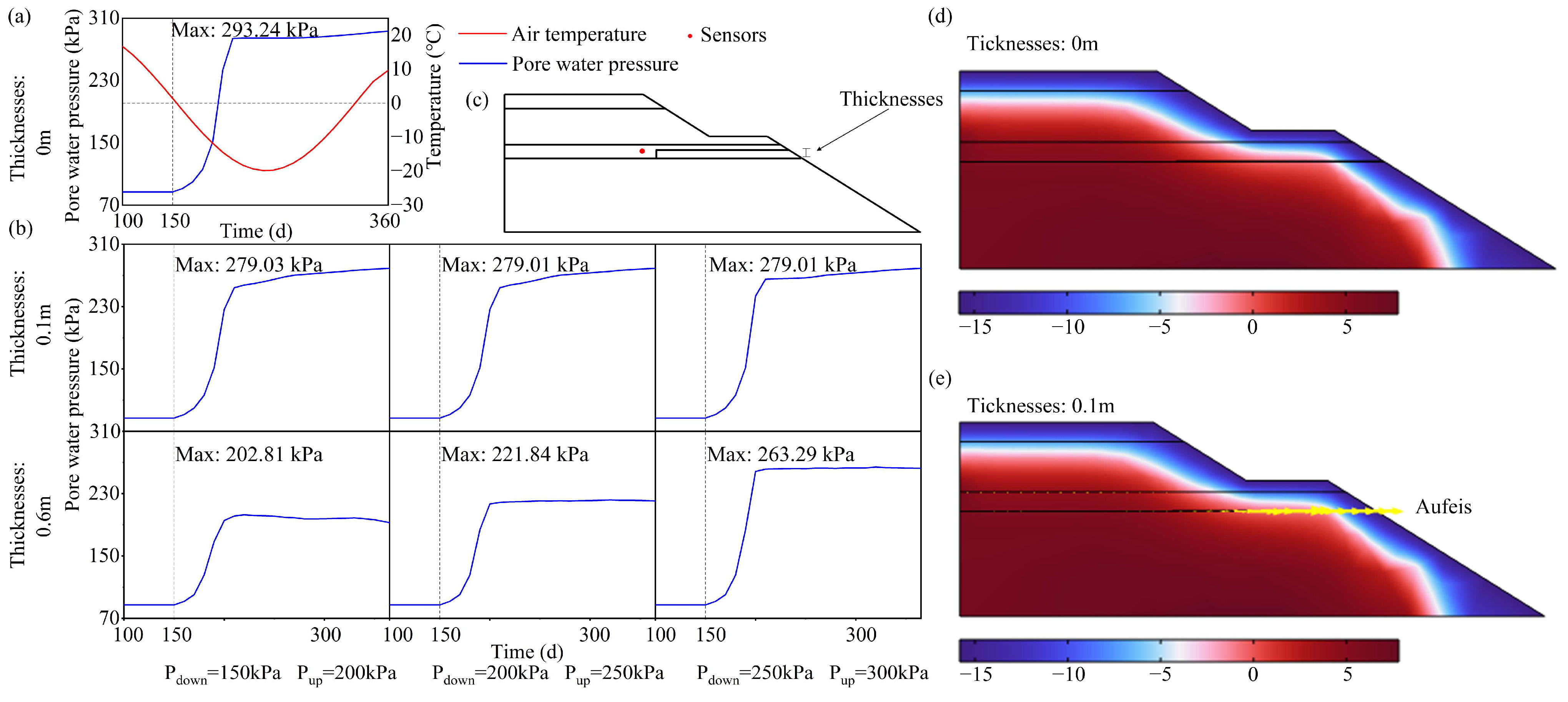
3.2.3. Results of the Numerical Simulation
3.3. Unidirectional Freeze–Thaw Laboratory Experiments
3.3.1. The Process of Laboratory Experiments
3.3.2. Results of the Experiment and Numerical Simulation
4. Discussion
4.1. Pressurized Groundwater and Icing
4.2. Dynamic Pressure and Icing
4.3. Mudstone–Sandstone Combination Stratigraphy and Icing
4.4. Variation of Hydrostatic Pressure in the Sandy Soil
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Ensom, T.; Makarieva, O.; Morse, P.; Kane, D.; Alekseev, V.; Marsh, P. The Distribution and Dynamics of Aufeis in Permafrost Regions. Permafr. Periglac. Process. 2020, 31, 383–395. [Google Scholar] [CrossRef]
- Wankiewicz, A. Analysis of Winter Heat Flow in an Ice-Covered Arctic Stream (Caribou Creek, NWT). Can. J. Civ. Eng. 2011, 11, 430–443. [Google Scholar] [CrossRef]
- Kane, D.L.; Yoshikawa, K.; McNamara, J.P. Regional Groundwater Flow in an Area Mapped as Continuous Permafrost, NE Alaska (USA). Hydrogeol. J. 2013, 21, 41–52. [Google Scholar] [CrossRef]
- Wanty, R.B.; Wang, B.; Vohden, J.; Day, W.C.; Gough, L.P. Aufeis Accumulations in Stream Bottoms in Arctic and Subarctic Environments as a Possible Indicator of Geologic Structure: Chapter F in Recent U.S. Geological Survey Studies in the Tintina Gold Province, Alaska, United States, and Yukon, Canada—Results of a 5-Year Project; U.S. Geological Survey: Reston, VA, USA, 2007. [CrossRef]
- Olenchenko, V.; Zemlianskova, A.; Makarieva, O.; Potapov, V. Geocryological Structure of a Giant Spring Aufeis Glade at the Anmangynda River (Northeastern Russia). Geosciences 2023, 13, 328. [Google Scholar] [CrossRef]
- Gagarin, L.; Wu, Q.; Cao, W.; Jiang, G. Icings of the Kunlun Mountains on the Northern Margin of the Qinghai-Tibet Plateau, Western China: Origins, Hydrology and Distribution. Water 2022, 14, 2396. [Google Scholar] [CrossRef]
- Morse, P.D.; Wolfe, S.A. Geological and Meteorological Controls on Icing (Aufeis) Dynamics (1985 to 2014) in Subarctic Canada. J. Geophys. Res. Earth Surf. 2015, 120, 1670–1686. [Google Scholar] [CrossRef]
- Yoshikawa, K.; Hinzman, L.D.; Kane, D.L. Spring and Aufeis (Icing) Hydrology in Brooks Range, Alaska. J. Geophys. Res. Biogeosci. 2007, 112, G04S43. [Google Scholar] [CrossRef]
- Zhao, T. Investigation and Analysis on the Origin of Salivating Ice Flow in Harbin Liming Road Tunnel. Ph.D. Dissertation, Heilongjiang University, Harbin, China, 2023. [Google Scholar]
- Yu, W.; Han, F.; Yi, X.; Liu, W.; Hu, D. Cut-Slope Icing Prevention: Case Study of the Seasonal Frozen Area of Western China. J. Cold Reg. Eng. 2016, 30, 05016001. [Google Scholar] [CrossRef]
- Hu, X.; Pollard, W.H. The Hydrologic Analysis and Modelling of River Icing Growth, North Fork Pass, Yukon Territory, Canada. Permafr. Periglac. Process. 1997, 8, 279–294. [Google Scholar] [CrossRef]
- Yu, W.B.; Lai, Y.M.; Bai, W.L.; Zhang, X.F.; Zhuang, D.S.; Li, Q.H.; Wang, J.W. Icing Problems on Road in Da Hinggangling Forest Region and Prevention Measures. Cold Reg. Sci. Technol. 2005, 42, 79–88. [Google Scholar] [CrossRef]
- Kane, D.L. Physical Mechanics of Aufeis Growth. Can. J. Civ. Eng. 1981, 8, 186–195. [Google Scholar] [CrossRef]
- Guo, Y.; Shan, W.; Hu, Z.; Jiang, H. Cut Slope Icing Formation Mechanism and Its Influence on Slope Stability in Periglacial Area. In Advancing Culture of Living with Landslides; Mikoš, M., Vilímek, V., Yin, Y., Sassa, K., Eds.; Springer International Publishing: Cham, Switzerland, 2017; pp. 183–189. [Google Scholar]
- Xu, S.; Zhang, H.; Wang, M.; Ren, H. Study on Indoor and Field Tests of Salivary Icing on Tonghuang Highway and Novel Control Measures. HIGHWAY 2017, 62, 253–260. [Google Scholar]
- Zhang, H. Study on Formation Mechanism and Control Techniques of Extruded Ice for Highway. Ph.D. Dissertation, Chang’an University, Xi’an, China, 2017. [Google Scholar]
- Crites, H.; Kokelj, S.V.; Lacelle, D. Icings and Groundwater Conditions in Permafrost Catchments of Northwestern Canada. Sci. Rep. 2020, 10, 3283. [Google Scholar] [CrossRef] [PubMed]
- Li, L.; Gao, P.; An, G. Formation of icing and its management. Commun. Sci. Technol. Heilongjiang 2004, 11, 35–36. [Google Scholar] [CrossRef]
- Zhou, Z.; Lei, J.; Zhu, S.; Qiao, S.; Zhang, H. The Formation Mechanism and Influence Factors of Highway Waterfall Ice: A Preliminary Study. Sustainability 2019, 11, 4059. [Google Scholar] [CrossRef]
- Guo, Y.; Qi, Y.; Shan, W. Research progress on investigation and control of highway salivary ice disease in China. J. Nat. Disasters 2022, 31, 15–26. [Google Scholar] [CrossRef]
- Sloan, C.E.; Zenone, C.; Mayo, L.R. Icings along the Trans-Alaska Pipeline Route; U.S. Government Printing Office: Washington, DC, USA, 1976. [CrossRef]
- Makarieva, O.; Nesterova, N.; Shikhov, A.; Zemlianskova, A.; Luo, D.; Ostashov, A.; Alexeev, V. Giant Aufeis—Unknown Glaciation in North-Eastern Eurasia According to Landsat Images 2013–2019. Remote Sens. 2022, 14, 4248. [Google Scholar] [CrossRef]
- Schmitt, T.; Brombierstäudl, D.; Schmidt, S.; Nüsser, M. Giant Aufeis in the Pangong Tso Basin: Inventory of a Neglected Cryospheric Component in Eastern Ladakh and Western Tibet. Atmosphere 2024, 15, 263. [Google Scholar] [CrossRef]
- Yu, M. Study on the Formation Mechanism of Groundwater Overflow into Ice in Alpine Area. Ph.D. Dissertation, Heilongjiang University, Harbin, China, 2021. [Google Scholar]
- Yang, P. Analysis of Disease Causes and Influence Main Factors for Highway Extruded Ice. Ph.D. Dissertation, Chang’an University, Xi’an, China, 2016. [Google Scholar]
- Wang, G. Study on the Mechanism and Prevention Measures of Extruded Ice for The Sichuan-Tibet Railway. Ph.D. Dissertation, Southwest Jiaotong University, Chengdu, China, 2023. [Google Scholar]
- Hu, Z. The Characteristics of Permafrost Degradation in Lesser Khingan Mountains of China and Its Effect on Road Subgrade Stability. Ph.D. Dissertation, Northeast Forestry University, Harbin, China, 2019. [Google Scholar]
- Jiang, Z. Beihei Highway—The Flying Dragon. Commun. Sci. Technol. Heilongjiang 2011, 34, 72. [Google Scholar] [CrossRef]
- Wang, S. Permafrost Degradation Characteristics and Foundation Settlement Prediction along Bei-Hei Expressway. Ph.D. Dissertation, Northeast Forestry University, Harbin, China, 2021. [Google Scholar]
- Shan, W.; Zhang, C.; Guo, Y.; Qiu, L.; Xu, Z.; Wang, Y. Spatial Distribution and Variation Characteristics of Permafrost Temperature in Northeast China. Sustainability 2022, 14, 8178. [Google Scholar] [CrossRef]
- Shan, W.; Xu, G.; Wang, Y.; Qiu, L.; Guo, Y.; Zhang, C. Response of Alpine Timberline to Permafrost Degradation on Changbai Mountain. Sustainability 2023, 15, 16768. [Google Scholar] [CrossRef]
- Shan, W.; Hou, P.; Wang, Y.; Qiu, L.; Guo, Y.; Zhang, C. Response of the Alpine Timberline to Residual Permafrost Degradation in Mount Wutai. Forests 2024, 15, 651. [Google Scholar] [CrossRef]
- Zhang, C. Spatio Temporal Evolution and Linear Engineering Response of Permafrost in Northeast China. Ph.D. Dissertation, Northeast Forestry University, Harbin, China, 2023. [Google Scholar]
- Walvoord, M.A.; Striegl, R.G. Increased Groundwater to Stream Discharge from Permafrost Thawing in the Yukon River Basin: Potential Impacts on Lateral Export of Carbon and Nitrogen. Geophys. Res. Lett. 2007, 34, L12402. [Google Scholar] [CrossRef]
- Jiang, H. Formation Law of the Landslide and Its Effect on the Subgrade Stability in Permafrost Degradation Region. Ph.D. Dissertation, Northeast Forestry University, Harbin, China, 2016. [Google Scholar]
- Grenier, C.; Anbergen, H.; Bense, V.; Chanzy, Q.; Coon, E.; Collier, N.; Costard, F.; Ferry, M.; Frampton, A.; Frederick, J.; et al. Groundwater Flow and Heat Transport for Systems Undergoing Freeze-Thaw: Intercomparison of Numerical Simulators for 2D Test Cases. Adv. Water Resour. 2018, 114, 196–218. [Google Scholar] [CrossRef]
- Zhang, C.; Ma, M.; Shan, W.; Guo, Y. Process and Numerical Simulation of Landslide Sliding Caused by Permafrost Degradation and Seasonal Precipitation. Nat. Hazards 2024, 120, 5429–5458. [Google Scholar] [CrossRef]
- Li, Z. The Analysis of Numerical Simulation and Theoretical Research for Highway Saliva Ice. Ph.D. Dissertation, Chang’an University, Xi’an, China, 2017. [Google Scholar]


| Layer | Category | Types | Thickness (m) | Permeability Coefficient (cm/s) |
|---|---|---|---|---|
| 1 | Quaternary loose layer | Silty clay | 0.8~1.0 | 3.84 × 10−8 |
| 2 | Tertiary loose layer | Muddy sandstone | 2.5~3.0 | 6.49 × 10−6 |
| 3 | Tertiary sandstone | Pebbly sandstone | 1.0~1.2 | 2.11 × 10−5 |
| 4 | Cretaceous mudstone | Mudstone | >5 | 2.09 × 10−8 |
| Types | W/(mK) | W/(mK) | J/m3K | J/m3K | kg/m3 | n |
|---|---|---|---|---|---|---|
| Silty clay | 1.229 | 1.105 | 2.011 × 106 | 2.968 × 106 | 1799 | 0.491 |
| Muddy sandstone | 1.651 | 1.550 | 1.011 × 106 | 1.612 × 106 | 2210 | 0.346 |
| Pebbly sandstone | 1.875 | 1.689 | 2.221 × 106 | 3.406 × 106 | 1842 | 0.512 |
| Mudstone | 1.976 | 1.836 | 2.062 × 106 | 2.821 × 106 | 1811 | 0.313 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shan, W.; Hou, P.; Xu, G.; Du, H.; Guo, Y.; Zhang, C. Monitoring of the Icing Process and Simulation of Its Formation Mechanism in the Cut Slope of Beihei Highway. Water 2024, 16, 1851. https://doi.org/10.3390/w16131851
Shan W, Hou P, Xu G, Du H, Guo Y, Zhang C. Monitoring of the Icing Process and Simulation of Its Formation Mechanism in the Cut Slope of Beihei Highway. Water. 2024; 16(13):1851. https://doi.org/10.3390/w16131851
Chicago/Turabian StyleShan, Wei, Peijie Hou, Guangchao Xu, Helong Du, Ying Guo, and Chengcheng Zhang. 2024. "Monitoring of the Icing Process and Simulation of Its Formation Mechanism in the Cut Slope of Beihei Highway" Water 16, no. 13: 1851. https://doi.org/10.3390/w16131851
APA StyleShan, W., Hou, P., Xu, G., Du, H., Guo, Y., & Zhang, C. (2024). Monitoring of the Icing Process and Simulation of Its Formation Mechanism in the Cut Slope of Beihei Highway. Water, 16(13), 1851. https://doi.org/10.3390/w16131851







