Modeling the Hydraulic Fracturing Processes in Shale Formations Using a Meshless Method
Abstract
1. Introduction
2. Numerical Treatments of Hydraulic Fracturing in SPH
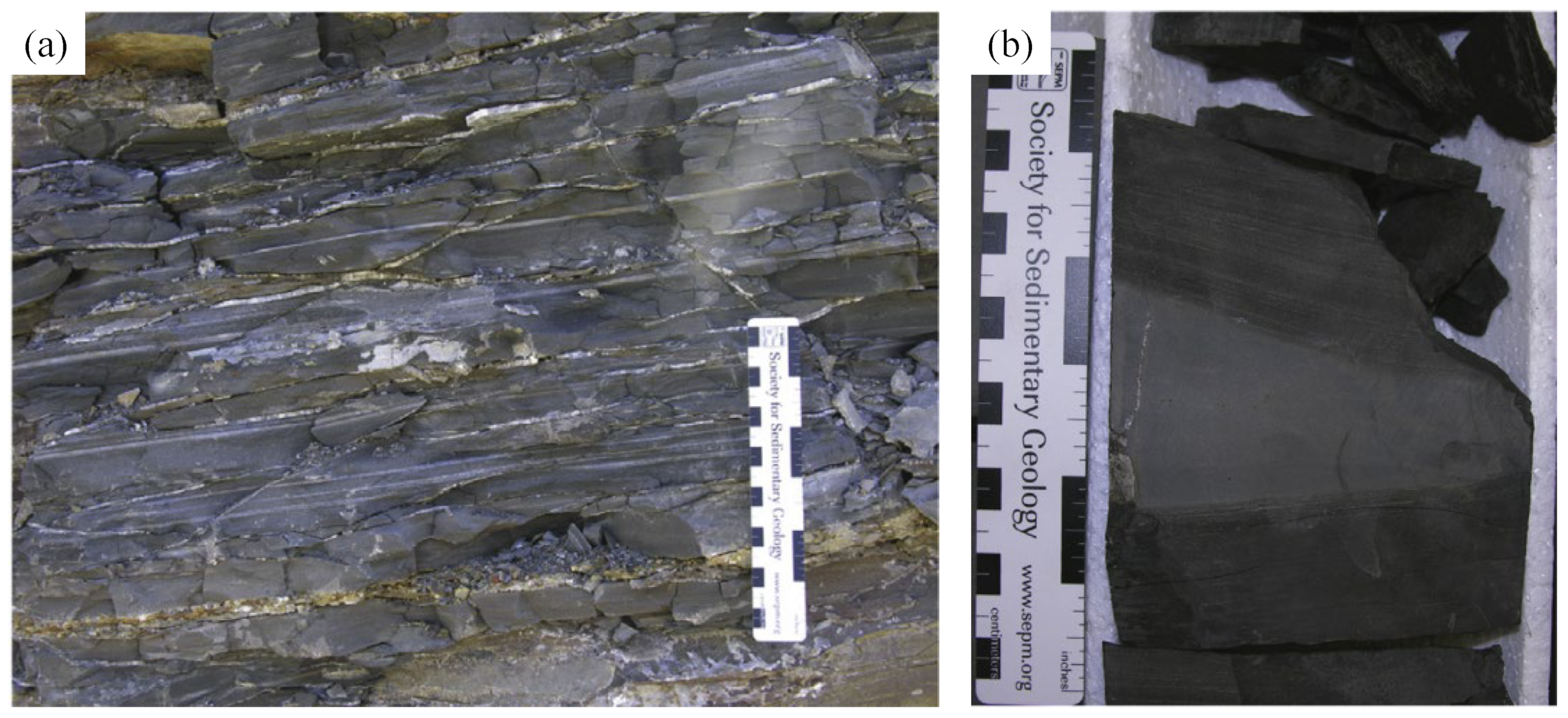
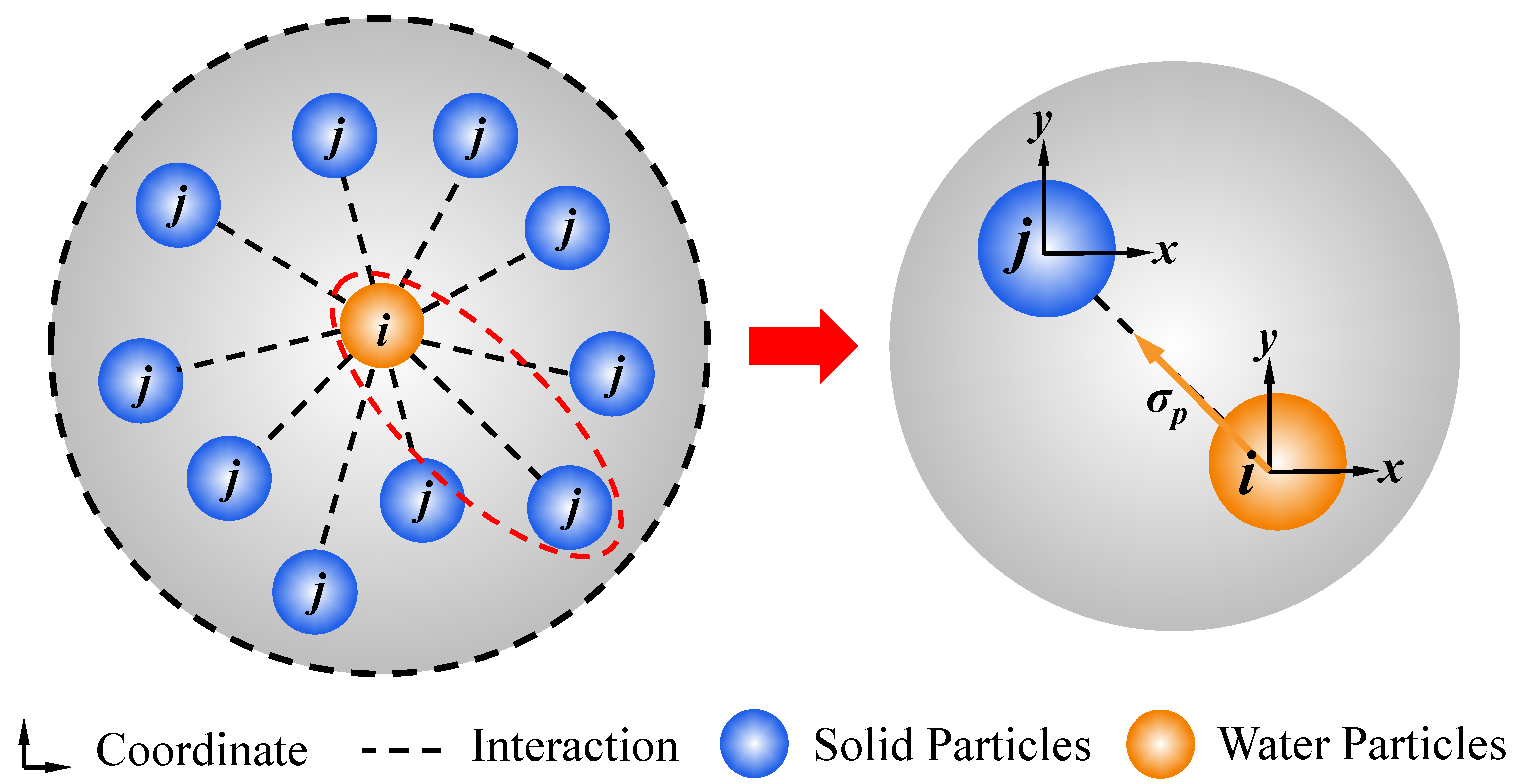
2.1. Liquid—Solid Particle Interaction Modes
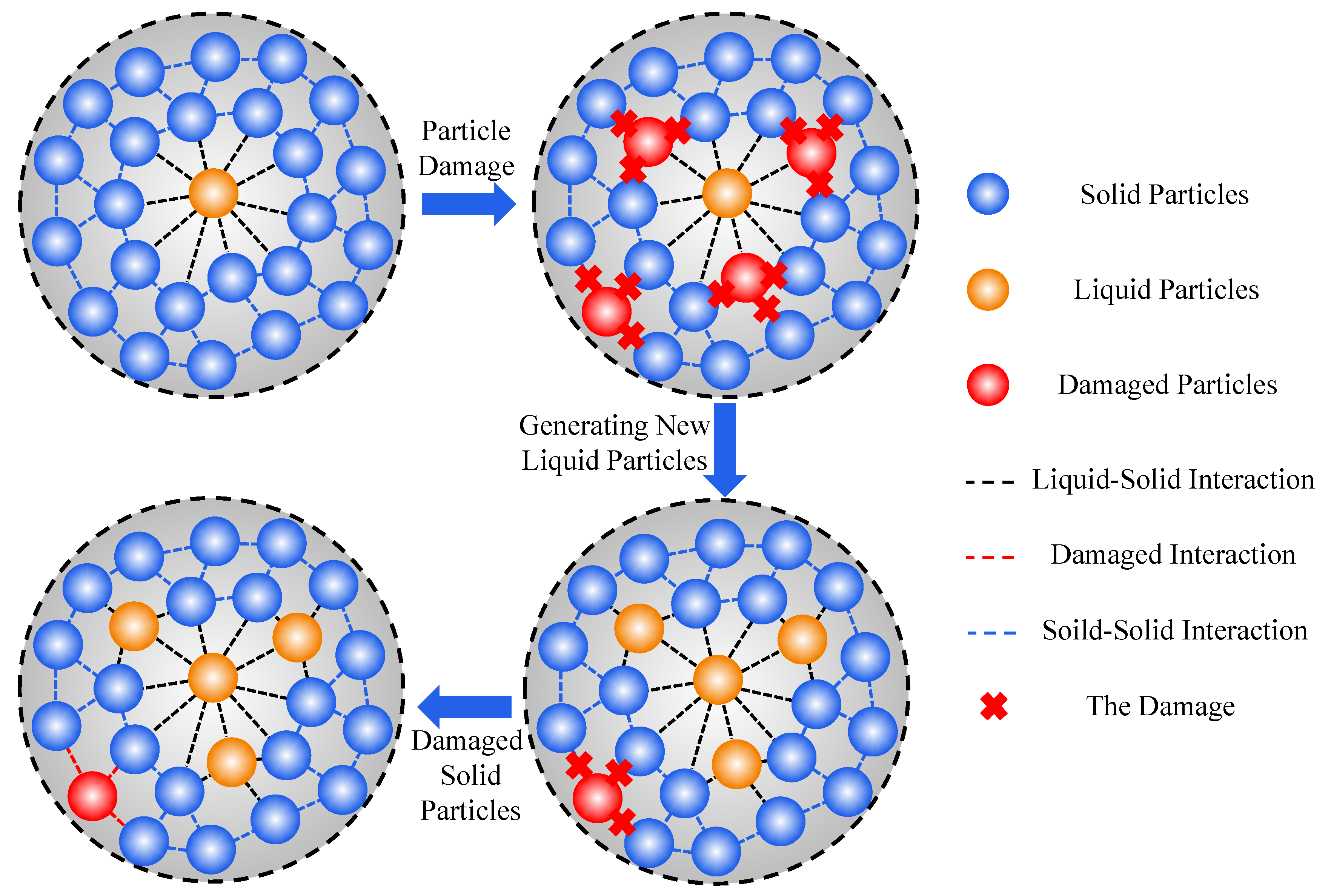
2.2. Fracture Treatments under Liquid–Solid Interactions
2.2.1. Failure Criterion
2.2.2. Fracture Treatments of Solid Particles
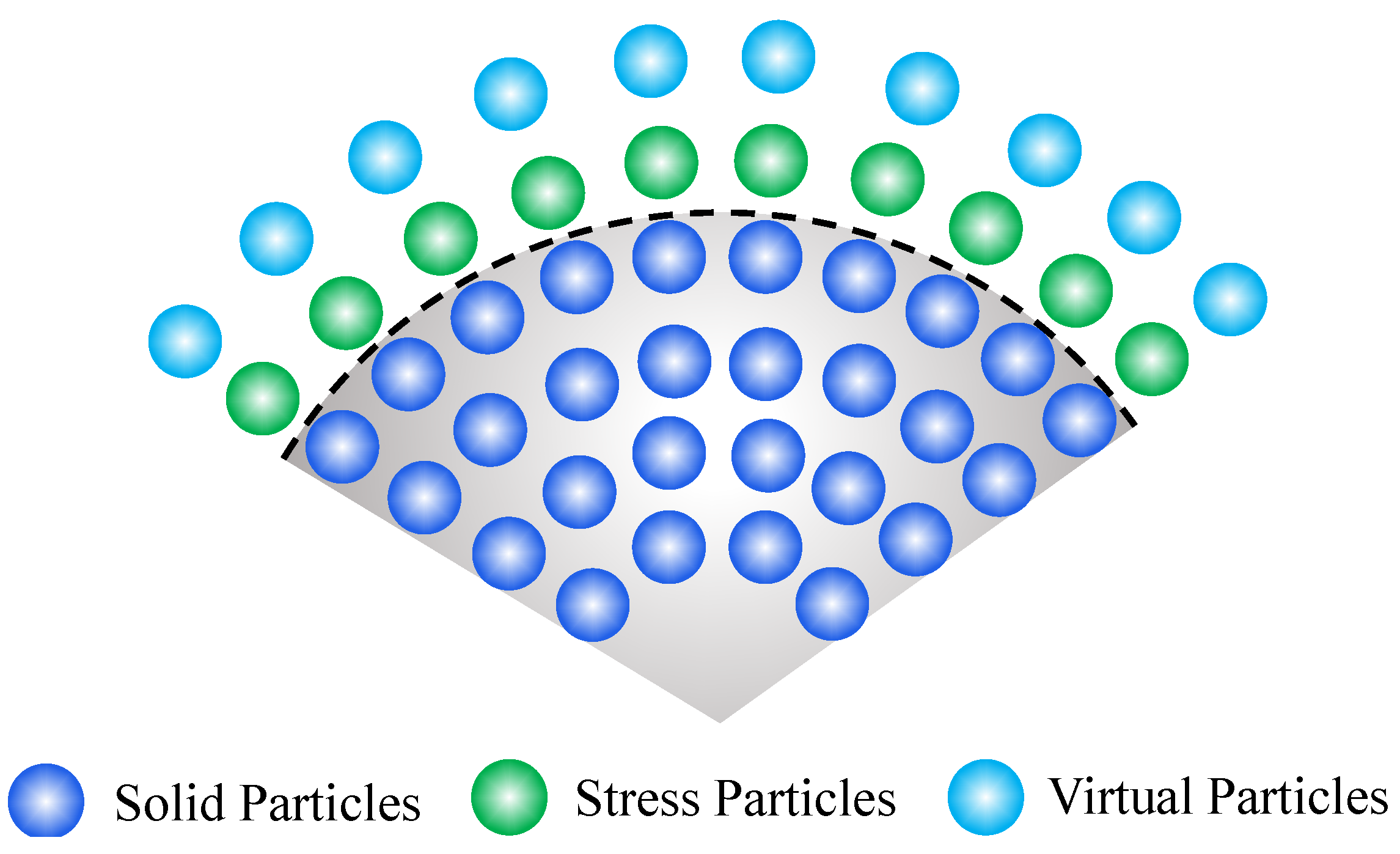
2.3. Application of Stress Boundaries in SPH
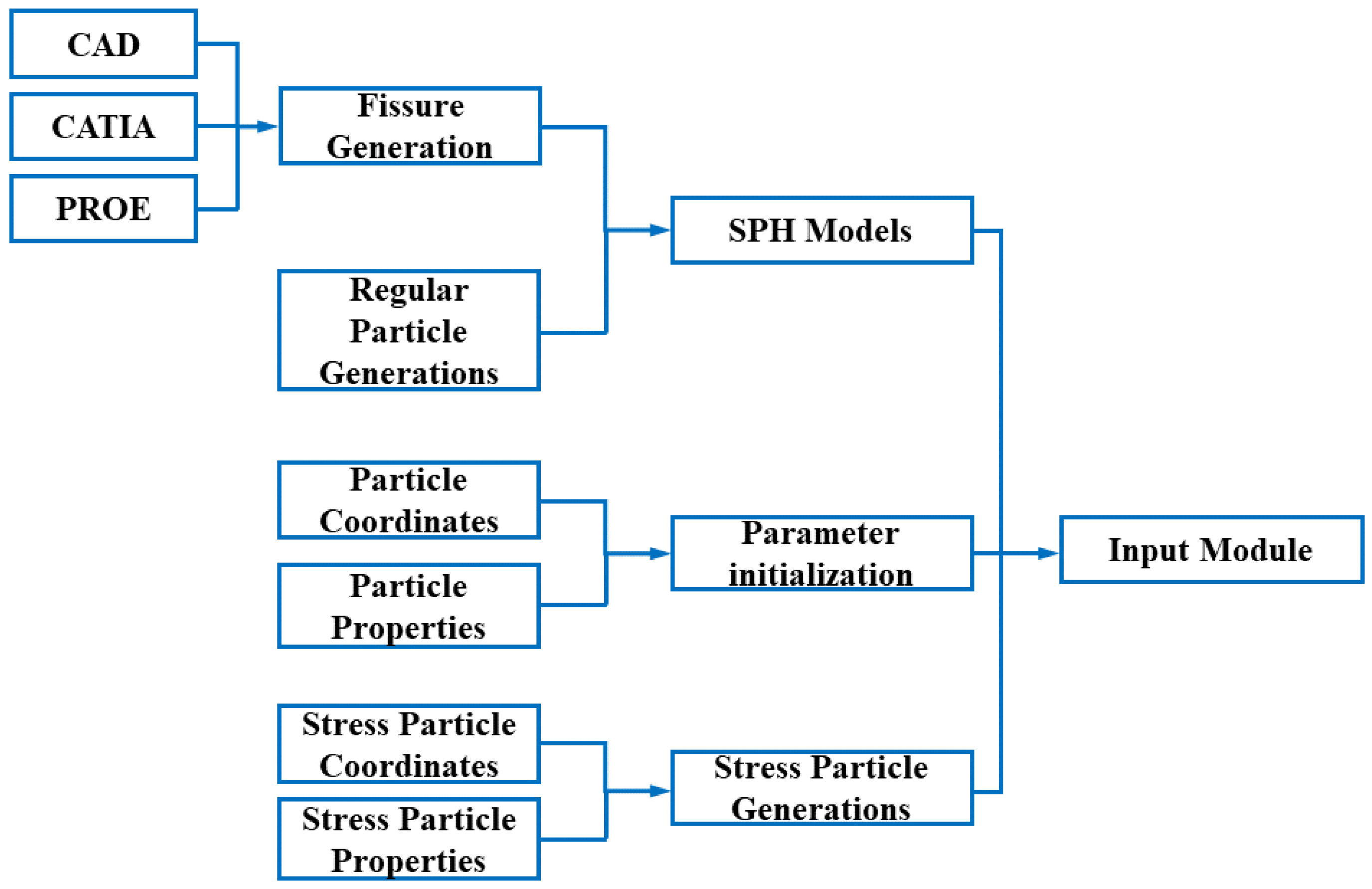
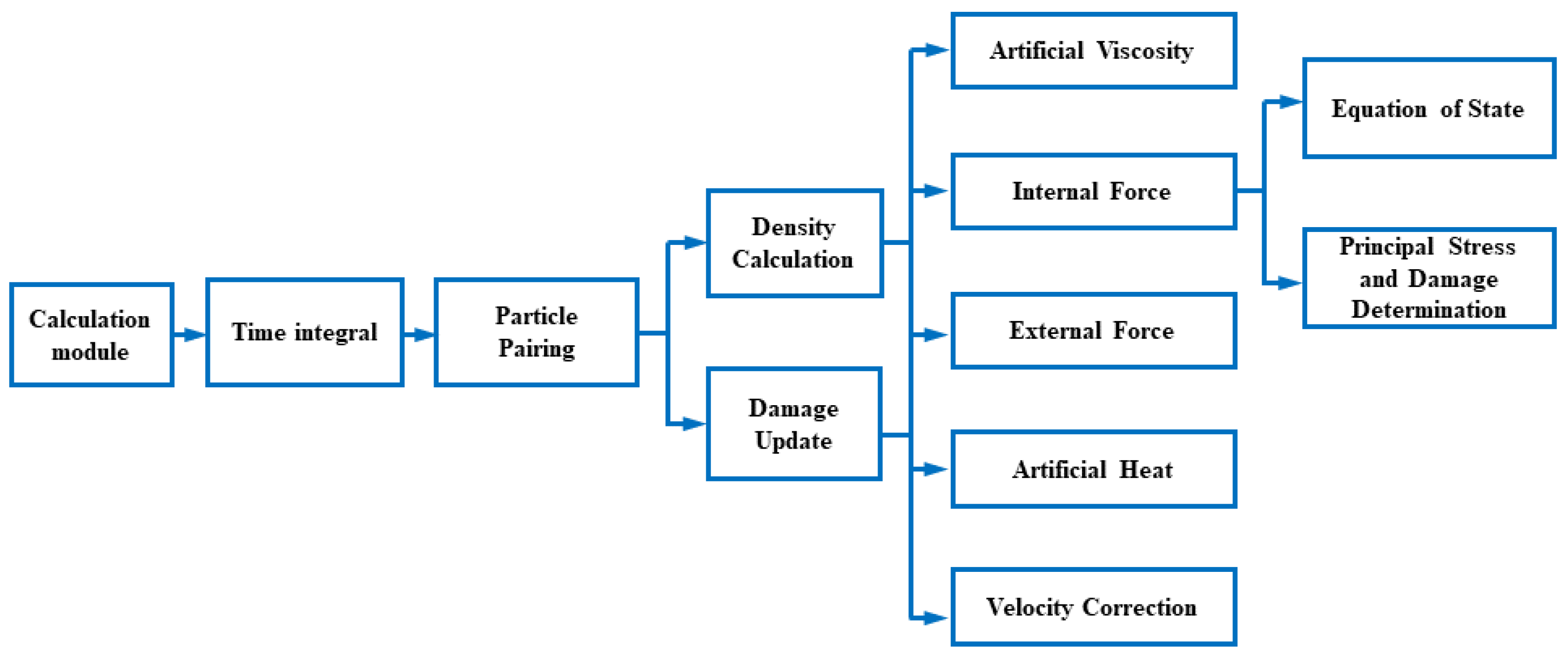
2.4. Solutions of SPH Method
3. Validation of Improved SPH Method
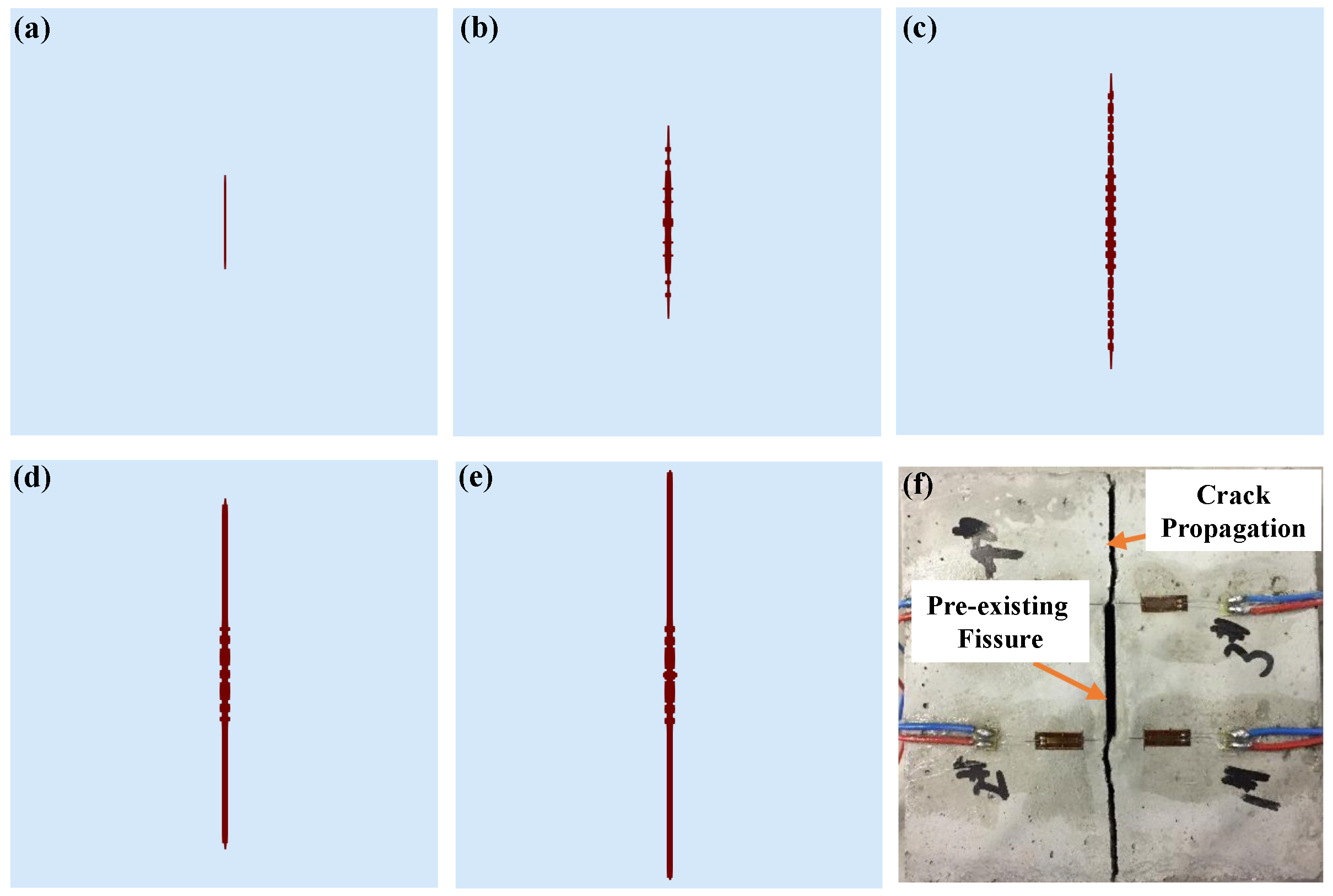
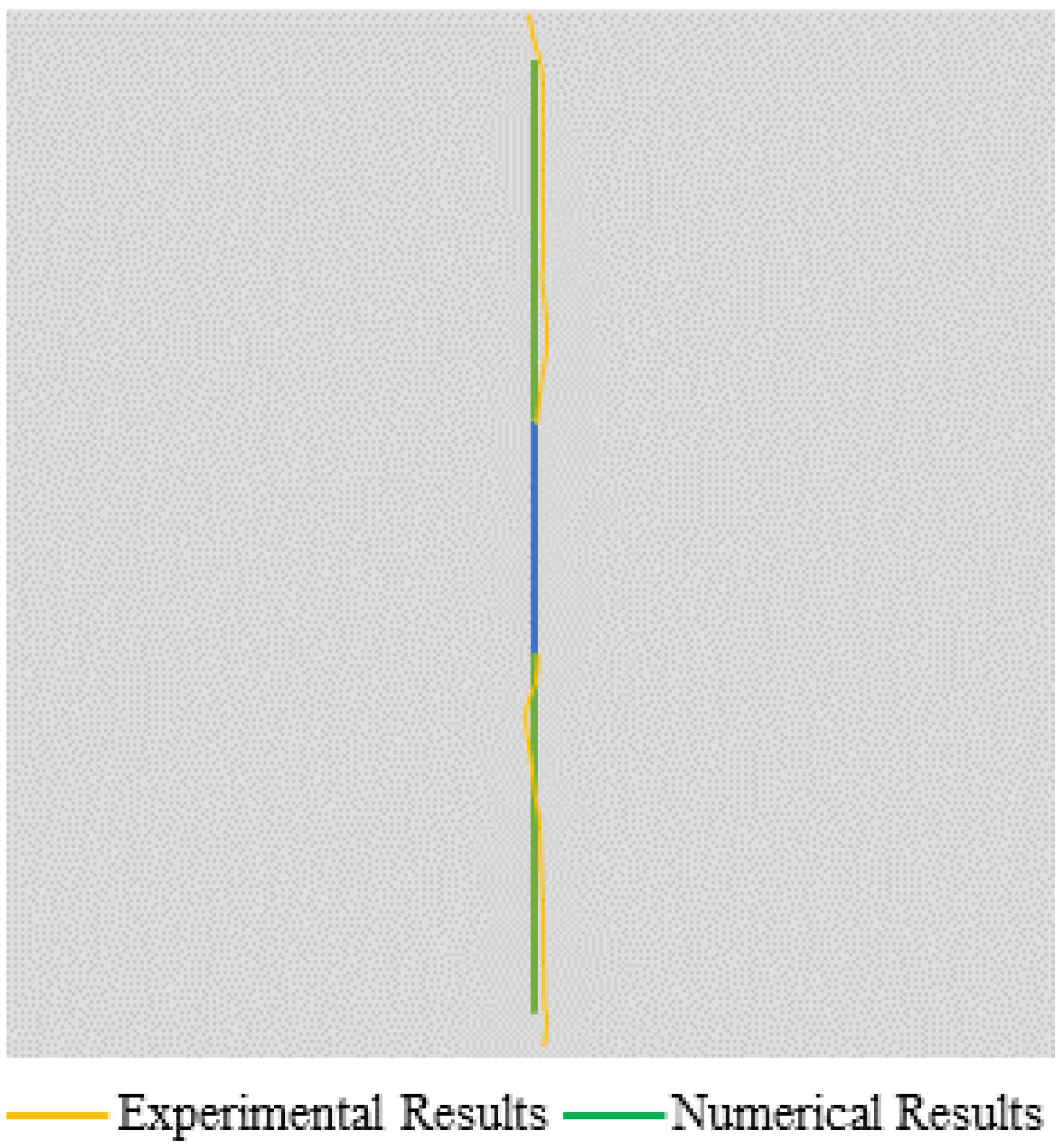
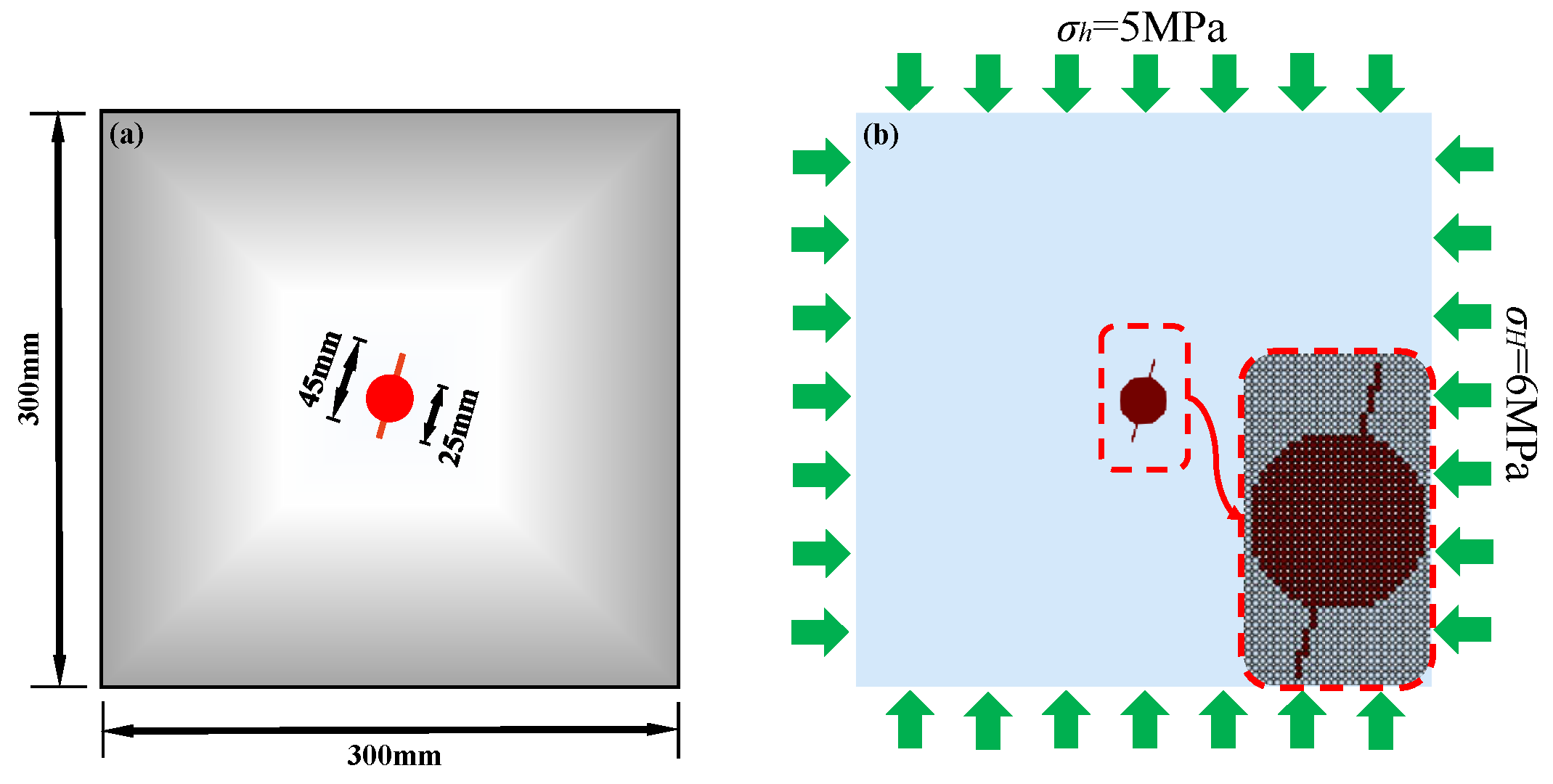
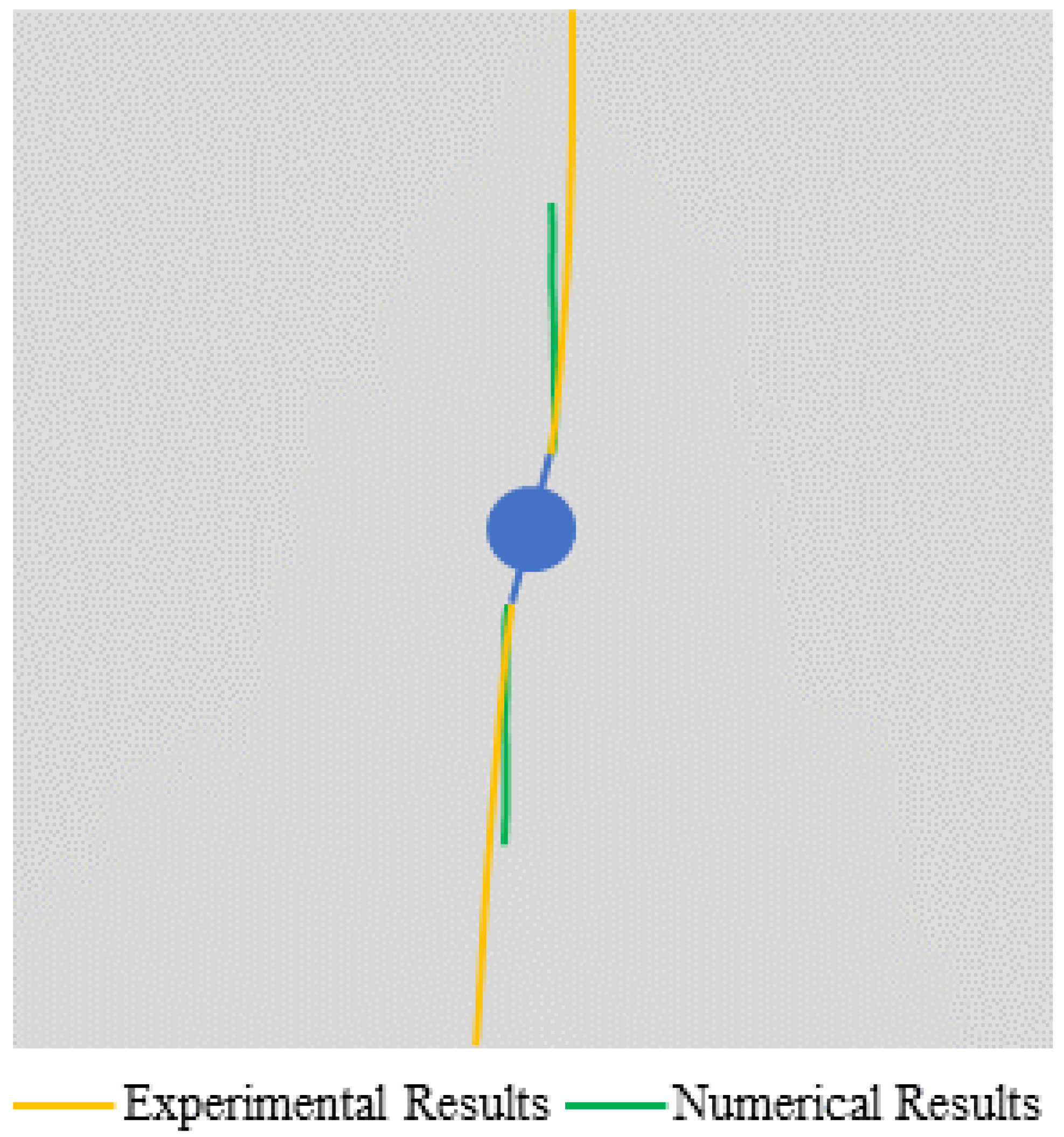
3.1. One Vertical Fissure without Lateral Pressure
3.2. Directional Hydraulic Fracturing with Confining Pressure
4. Numerical Simulation of Bedding Shale Hydraulic Fracturing
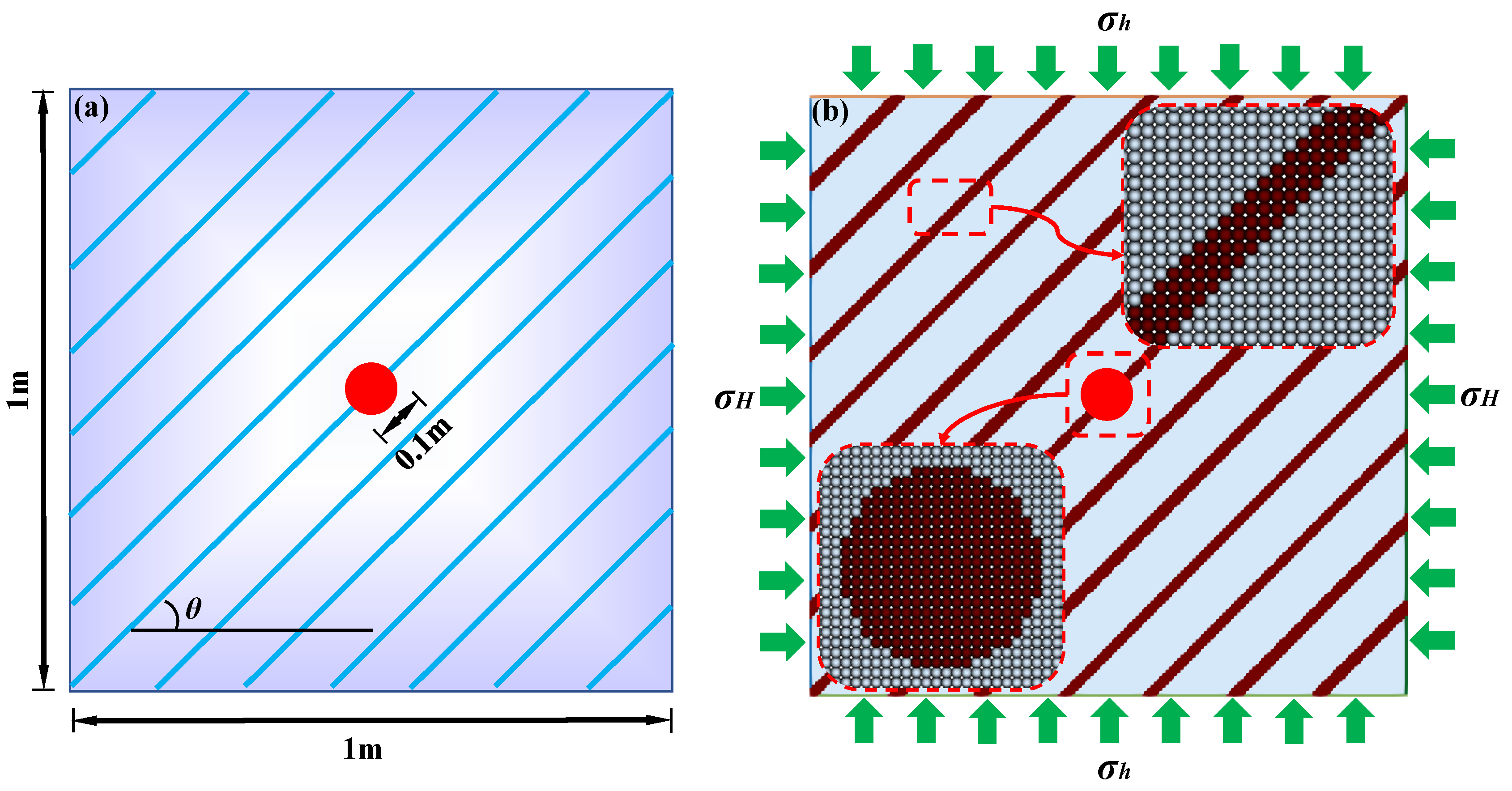
4.1. Numerical Model and Calculation Parameters
4.2. Effects of Bedding Dip Angles on Particle Failure Counts under Hydraulic Fracturing
4.3. Effects of Bedding Dip Angles on Formations of Hydraulic Fracturing Networks
5. Conclusions
- (1)
- The interaction modes between liquid particles and solid particles have been introduced and the traditional kernel function in the SPH method has been improved by introducing the fracture mark ξ, which can realize the hydraulic fracturing simulations of particles.
- (2)
- The application of the stress boundary of SPH is realized by stress mapping. The feasibility of the improved numerical method is validated by two numerical examples, and the correctness of the method is verified by comparisons with previous experimental results.
- (3)
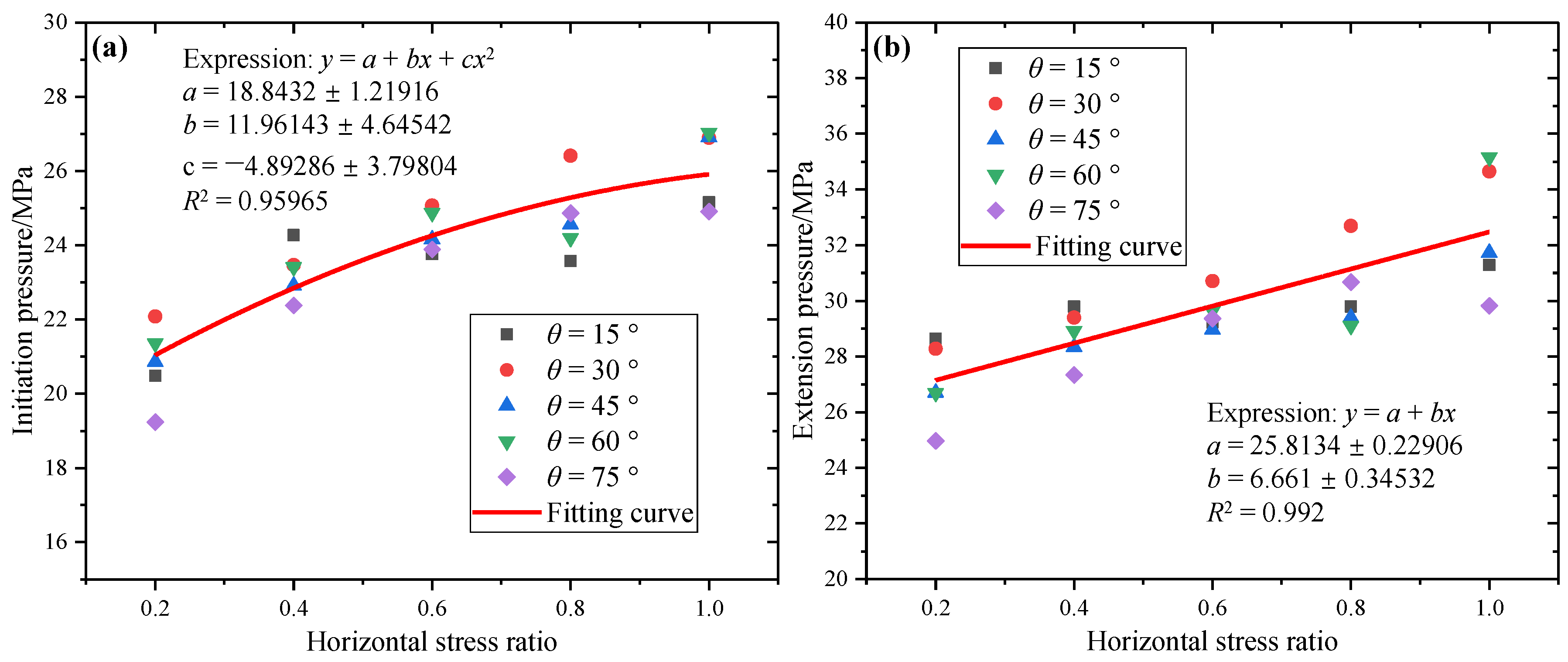
- The hydraulic fracturing mesh-less numerical model containing bedding properties has been established, and the numerical simulations are carried out on the progressive fracture propagations under different horizontal stress ratios. With the increase of horizontal stress ratio, the total number of damaged particles decreases, but the initiation and extension pressure increase gradually. The initiation stress of small bedding dip angles (θ < 45°) is larger than that of big bedding dip angles (θ > 45°).
- (4)
- The morphology of hydraulic fracturing networks is greatly affected by bedding dip angles and the horizontal stress ratio. The range of hydraulic fracture propagation at low horizontal stress ratio is wider and the fracture propagation is along the direction of maximum principal stress, while the range of hydraulic fracture propagation at a high horizontal stress ratio is limited to perforation. The hydraulic fracture will propagate through the bedding with small dip angles. However, when the bedding dip angle is larger, the hydraulic fracture will propagate along the bedding direction.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Li, Q.; Liu, J.; Wang, S. Numerical insights into factors affecting collapse behavior of horizontal wellbore in clayey silt hydrate-bearing sediments and the accompanying control strategy. Ocean Eng. 2024, 297, 117029. [Google Scholar] [CrossRef]
- Li, Q.; Li, Y.; Cheng, Y. Numerical simulation of fracture reorientation during hydraulic fracturing in perforated horizontal well in shale reservoirs. Energy Sources Part A Recovery Util. Environ. Eff. 2018, 40, 1807–1813. [Google Scholar] [CrossRef]
- Li, Q.; Zhang, C.; Yang, Y. Preliminary experimental investigation on long-term fracture conductivity for evaluating the feasibility and efficiency of fracturing operation in offshore hydrate-bearing sediments. Ocean Eng. 2023, 281, 114949. [Google Scholar] [CrossRef]
- Li, Q.; Zhao, D.; Yin, J. Sediment Instability Caused by Gas Production from Hydrate-bearing Sediment in Northern South China Sea by Horizontal Wellbore: Evolution and Mechanism. Nat. Resour. Res. 2023, 32, 1595–1620. [Google Scholar] [CrossRef]
- Tan, P.; Jin, Y.; Wang, Y. Hydraulic fracture vertical propagation behavior in transversely isotropic layered shale formation with transition zone using XFEM-based CZM method. Eng. Fract. Mech. 2021, 248, 107707. [Google Scholar] [CrossRef]
- Zheng, H.; Pu, C.; Xu, E. Numerical investigation on the effect of well interference on hydraulic fracture propagation in shale formation. Eng. Fract. Mech. 2020, 228, 106932. [Google Scholar] [CrossRef]
- Gasparrini, M.; Sassi, W.; Gale, J.F.W. Natural sealed fractures in mudrocks: A case study tied to burial history from the Barnett Shale, Fort Worth Basin, Texas, USA. Mar. Pet. Geol. 2014, 55, 122–141. [Google Scholar] [CrossRef]
- Warpinski, N.R.; Teufel, L.W. Determination of the effective-stress law for permeability and deformation in low-permeability rocks. SPE Form. Eval. 1992, 7, 123–131. [Google Scholar] [CrossRef]
- Janszen, M.; Bakker, T.; Zitha, P. Hydraulic Fracturing in the Dutch Posedonia Shale. In Proceedings of the SPE European Formation Damage Conference and Exhibition. Society of Petroleum Engineers, Budapest, Hungary, 3–5 June 2015. [Google Scholar]
- Wang, H.; Jiang, Y.; Bian, X. Optimization and field test of deep shale fracturing process. Pet. Drill. Technol. 2016, 44, 76–81. [Google Scholar]
- Sun, K.; Zhang, S.; Xin, L. Effect of shale gas reservoir bedding direction on hydraulic fracturing crack propagation. Nat. Gas Ind. 2016, 36, 45–51. [Google Scholar]
- Liu, Z.; Feng, Q.; Wang, Y. Fracture height prediction model and operation optimization method considering bedding influence in Weiyuan shale gas reservoir. Logging Technol. 2022, 46, 114–121. [Google Scholar]
- Wang, C.; Zhang, H.; Zhao, J. Study on hydraulic fracturing fracture propagation in elastic-plastic reservoir. Pet. Mach. 2022, 50, 80–88. [Google Scholar]
- Cai, X. Complex fracture propagation model for supercritical CO2 fracturing in unconventional reservoirs. Fault-Block Field 2022, 29, 107–110+123. [Google Scholar]
- Branco, R.; Antunes, F.V.; Costa, J.D. A review on 3D-FE adaptive remeshing techniques for crack growth modelling. Eng. Fract. Mech. 2015, 141, 170–195. [Google Scholar] [CrossRef]
- Xu, X.P.; Needleman, A. Numerical simulations of fast crack growth in brittle solids. J. Mech. Phys. Solids 1994, 42, 1397–1434. [Google Scholar] [CrossRef]
- Zhang, P.; Zhang, L.; Baxevanakis, K.P. Modelling short crack propagation in a single crystal nickel-based superalloy using crystal plasticity and XFEM. Int. J. Fatigue 2020, 136, 105594. [Google Scholar] [CrossRef]
- Zhao, E.; Li, B. Evaluation Method for Cohesive Crack Propagation in Fragile Locations of RCC Dam Using XFEM. Water 2020, 13, 58. [Google Scholar] [CrossRef]
- Teimouri, F.; Heidari-Rarani, M.; Aboutalebi, F. Experimental characterization and cyclic cohesive zone modeling of mode I delamination growth in glass/epoxy composite laminates under high cycle fatigue. Eng. Fract. Mech. 2024, 9, 109853. [Google Scholar] [CrossRef]
- Alberto, A.; Valente, S. Asymptotic fields at the tip of a cohesive frictional crack growing at the bi-material interface between a dam and the foundation rock. Eng. Fract. Mech. 2013, 108, 152–161. [Google Scholar] [CrossRef]
- Zhang, L.; Du, H.; Yu, W. String-based cohesive zone model: Implicit integration scheme and calibration method. Int. J. Fract. 2020, 222, 53–74. [Google Scholar] [CrossRef]
- Li, Z.; Rao, Q.H. Quantitative determination of PFC3D microscopic parameters. J. Cent. South Univ. 2021, 28, 911–925. [Google Scholar] [CrossRef]
- He, Y. Dynamic Response of Rock-like Materials Based on SHPB Pulse Waveform Characteristics. Materials 2021, 15, 210. [Google Scholar] [CrossRef]
- Yang, Y.; Xia, Y.; Zheng, H. Investigation of rock slope stability using a 3D nonlinear strength-reduction numerical manifold method. Eng. Geol. 2021, 292, 106285. [Google Scholar] [CrossRef]
- Xl, A.; Cheng, H.A.; Ql, B. Grout penetration process simulation and grouting parameters analysis in fractured rock mass using numerical manifold method—ScienceDirect. Eng. Anal. Bound. Elem. 2021, 123, 93–106. [Google Scholar]
- Jiang, W. Numerical Analysis of The Damping Vibration Effect of Pre-splitting Crack Based on DDA. Int. J. Adv. Comput. Technol. 2011, 3, 188–196. [Google Scholar]
- Wang, C.; Wang, S.; Chen, G. Implementation of a J-integral Based Maximum Circumferential Tensile Stress Theory in DDA for Simulating Crack Propagation. Eng. Fract. Mech. 2021, 246, 107621. [Google Scholar] [CrossRef]
- Zhou, X.; Du, E.; Wang, Y. Thermo-hydro-chemo-mechanical coupling peridynamic model of fractured rock mass and its application in geothermal extraction. Comput. Geotech. 2022, 148, 104837. [Google Scholar] [CrossRef]
- Tian, D.; Zhou, X. A viscoelastic model of geometry-constraint-based non-ordinary state-based peridynamics with progressive damage. Comput. Geotech. 2022, 69, 1413–1441. [Google Scholar] [CrossRef]
- Spetz, A.; Denzer, R.; Tudisco, E. Phase-field fracture modelling of crack nucleation and propagation in porous rock. Int. J. Fract. 2020, 224, 31–46. [Google Scholar] [CrossRef]
- Zhou, S.; Zhuang, X.; Zhou, J. Phase Field Characterization of Rock Fractures in Brazilian Splitting Test Specimens Containing Voids and Inclusions. Int. J. Geomech. 2021, 21, 04021006. [Google Scholar] [CrossRef]
- Gnanasekaran, B.; Liu, G.R.; Fu, Y. A Smoothed Particle Hydrodynamics (SPH) procedure for simulating cold spray process—A study using particles. Surf. Coat. Technol. 2019, 377, 124812. [Google Scholar] [CrossRef]
- Bi, J.; Zhou, X.P. A Novel Numerical Algorithm for Simulation of Initiation, Propagation and Coalescence of Flaws Subject to Internal Fluid Pressure and Vertical Stress in the Framework of General Particle Dynamics. Rock Mech. Rock Eng. 2017, 50, 1833–1849. [Google Scholar] [CrossRef]
- Bi, J.; Zhou, X.P.; Qian, Q.H. The 3D Numerical Simulation for the Propagation Process of Multiple Pre-existing Flaws in Rock-Like Materials Subjected to Biaxial Compressive Loads. Rock Mech. Rock Eng. 2016, 49, 1611–1627. [Google Scholar] [CrossRef]
- Zhou, X.P.; Bi, J.; Qian, Q.H. Numerical Simulation of Crack Growth and Coalescence in Rock-Like Materials Containing Multiple Pre-existing Flaws. Rock Mech. Rock Eng. 2015, 48, 1097–1114. [Google Scholar] [CrossRef]
- Zhou, X.P.; Bi, J. 3D Numerical Study on the Growth and Coalescence of Pre-existing Flaws in Rocklike Materials Subjected to Uniaxial Compression. Int. J. Geomech. 2016, 16, 04015096. [Google Scholar] [CrossRef]
- Zhou, X.P.; Zhao, Y.; Qian, Q.H. A novel meshless numerical method for modeling progressive failure processes of slopes. Eng. Geol. 2015, 192, 139–153. [Google Scholar] [CrossRef]
- Zhao, Y.; Zhou, X.P.; Qian, Q.H. Progressive failure processes of reinforced slopes based on general particle dynamic method. J. Cent. South Univ. (Sci. Technol.) 2015, 22, 4049–4055. [Google Scholar] [CrossRef]
- Zhou, X.P.; Zhao, Y.; Qian, Q.H. Numerical simulation of rock failure process in uniaxial compression using smoothed particle hydrodynamics. Chin. J. Rock Mech. Eng. 2015, 34, 2647–2658. [Google Scholar]
- Zhou, X.P.; Yang, H.Q. Multiscale numerical modeling of propagation and coalescence of multiple cracks in rock masses. Int. J. Rock Mech. Min. Sci. 2012, 55, 15–27. [Google Scholar] [CrossRef]
- Yang, S.; Ren, X.; Zhang, J. Hydraulic fracturing of gravity dam based on numerical manifold method. Rock Soil Mech. 2018, 39, 3055–3060+3070. [Google Scholar]
- Liu, D. Experimental Study on Fracture and Hydraulic Fracturing Characteristics of Cement Mortar under Chemical Erosion. Ph.D. Thesis, Hohai University, Nanjing, China, 2019. [Google Scholar]
- Yang, S. Study on Initiation and Propagation of Prefabricated Fractures in Sandstone by Directional Fracturing. Ph.D. Thesis, Taiyuan University of Technology, Taiyuan, China, 2017. [Google Scholar]
- Cl, A.; Jgwa, B. Peridynamic simulation on hydraulic fracture propagation in shale formation. Eng. Fract. Mech. 2021, 258, 108095. [Google Scholar]




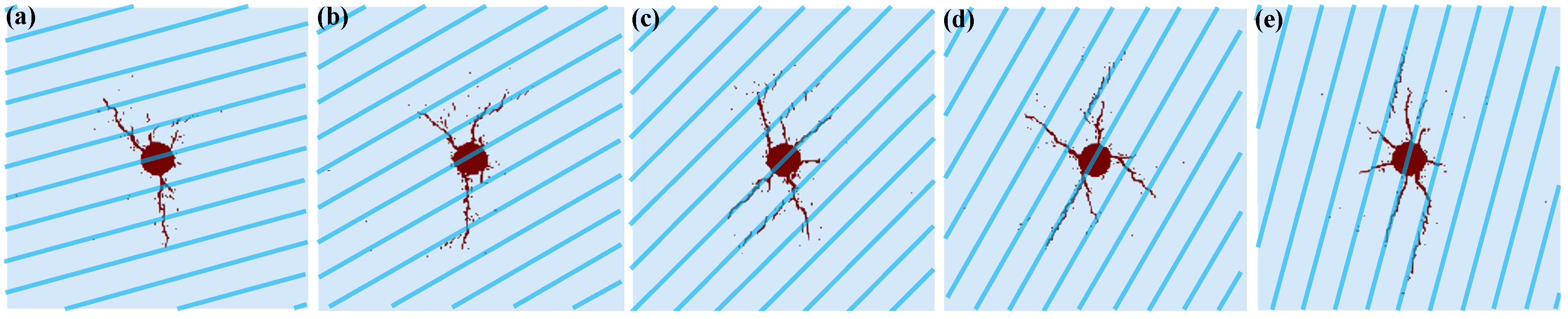
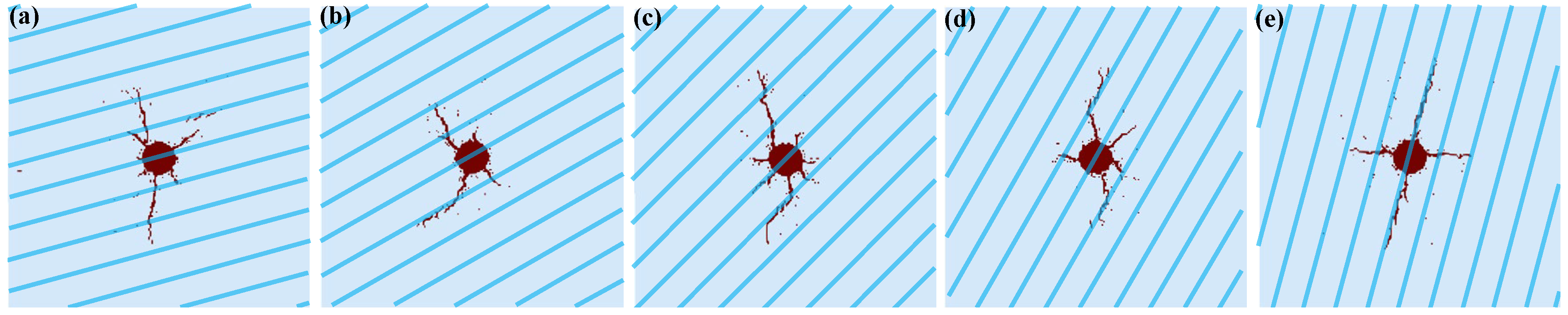
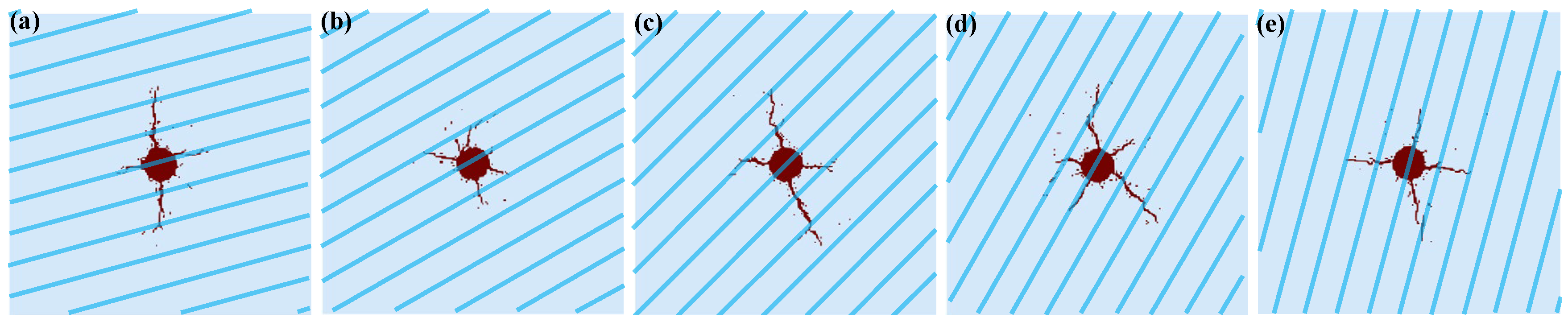
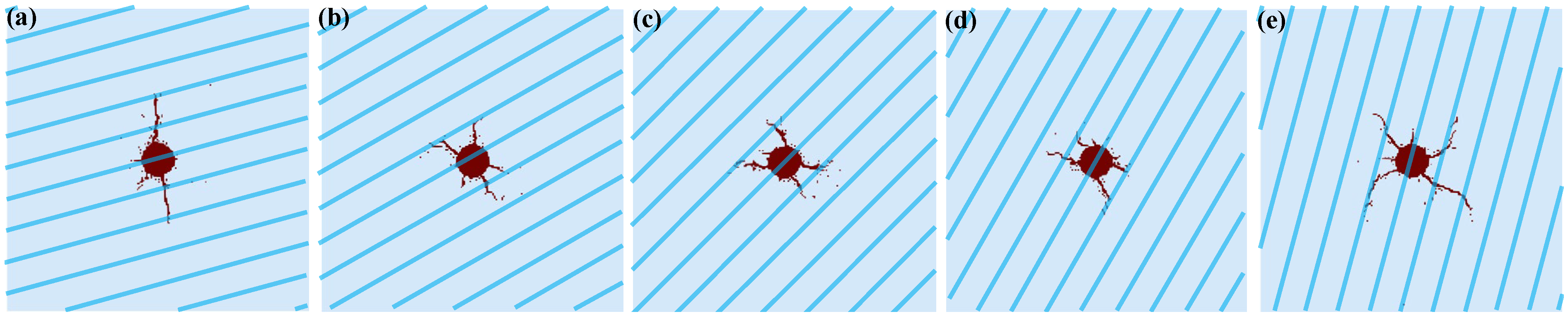
| Bedding Dip Angle θ/° | Horizontal Stress σH/MPa | Vertical Stress σh/MPa |
|---|---|---|
| 15 | 2, 4, 6, 8, 10 | 10 |
| 30 | 2, 4, 6, 8, 10 | 10 |
| 45 | 2, 4, 6, 8, 10 | 10 |
| 60 | 2, 4, 6, 8, 10 | 10 |
| 75 | 2, 4, 6, 8, 10 | 10 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xiang, Z.; Yu, S.; Wang, X. Modeling the Hydraulic Fracturing Processes in Shale Formations Using a Meshless Method. Water 2024, 16, 1855. https://doi.org/10.3390/w16131855
Xiang Z, Yu S, Wang X. Modeling the Hydraulic Fracturing Processes in Shale Formations Using a Meshless Method. Water. 2024; 16(13):1855. https://doi.org/10.3390/w16131855
Chicago/Turabian StyleXiang, Ziru, Shuyang Yu, and Xiangyu Wang. 2024. "Modeling the Hydraulic Fracturing Processes in Shale Formations Using a Meshless Method" Water 16, no. 13: 1855. https://doi.org/10.3390/w16131855
APA StyleXiang, Z., Yu, S., & Wang, X. (2024). Modeling the Hydraulic Fracturing Processes in Shale Formations Using a Meshless Method. Water, 16(13), 1855. https://doi.org/10.3390/w16131855






