Physical Model Test of Deformation Self-Adaptive Mechanism of Landslide Mass
Abstract
1. Introduction
2. Test Protocol
2.1. Principle of Experiment
- (1)
- Geometric similarity
- (2)
- Hydrodynamic characteristics similarity
- (3)
- Material similarity
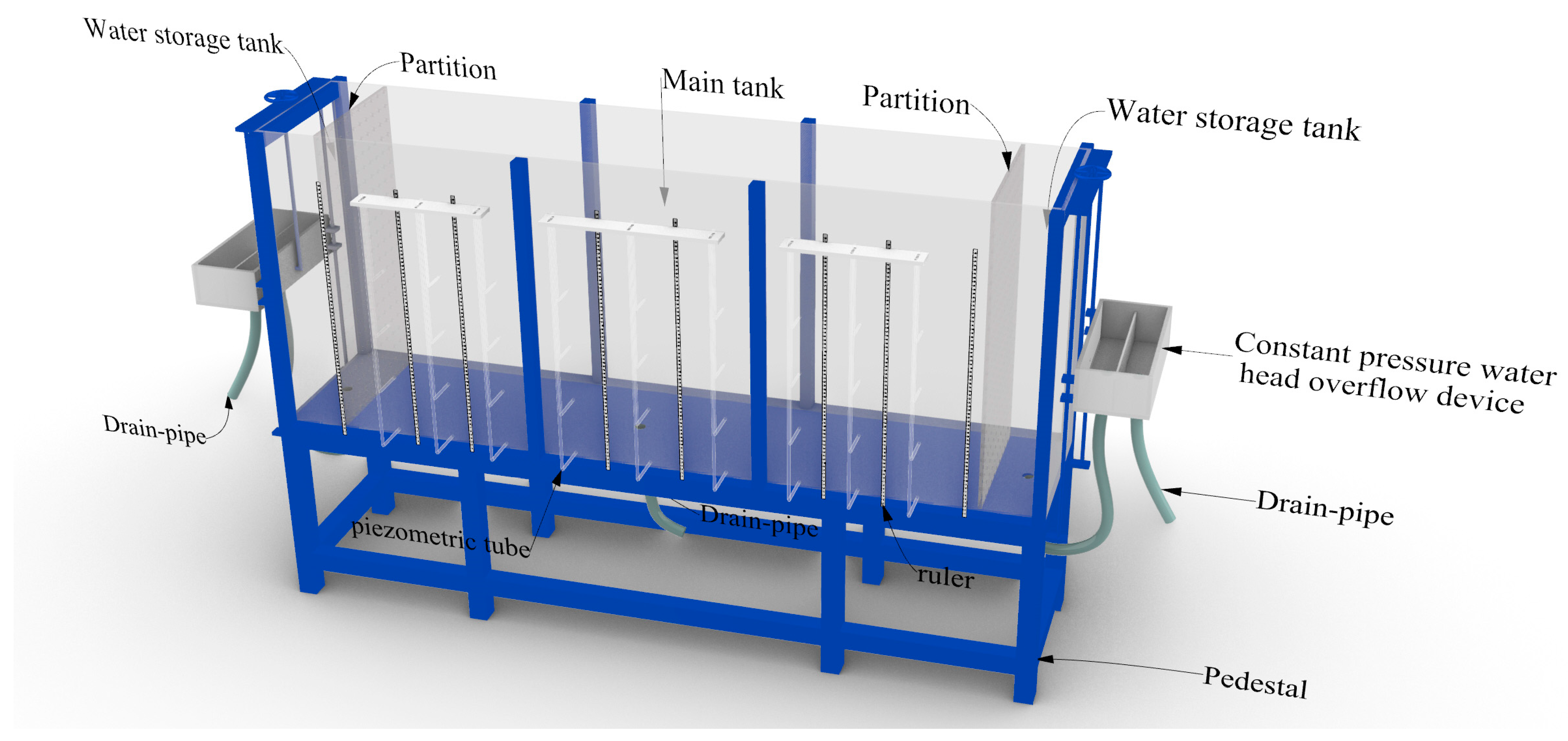
2.2. Test Model Box
2.3. Physical Model and Parameters
2.4. Working Conditions
3. Test Results
3.1. Variation Characteristics of Seepage Field and Permeability
3.1.1. Variation Characteristics of Seepage Field
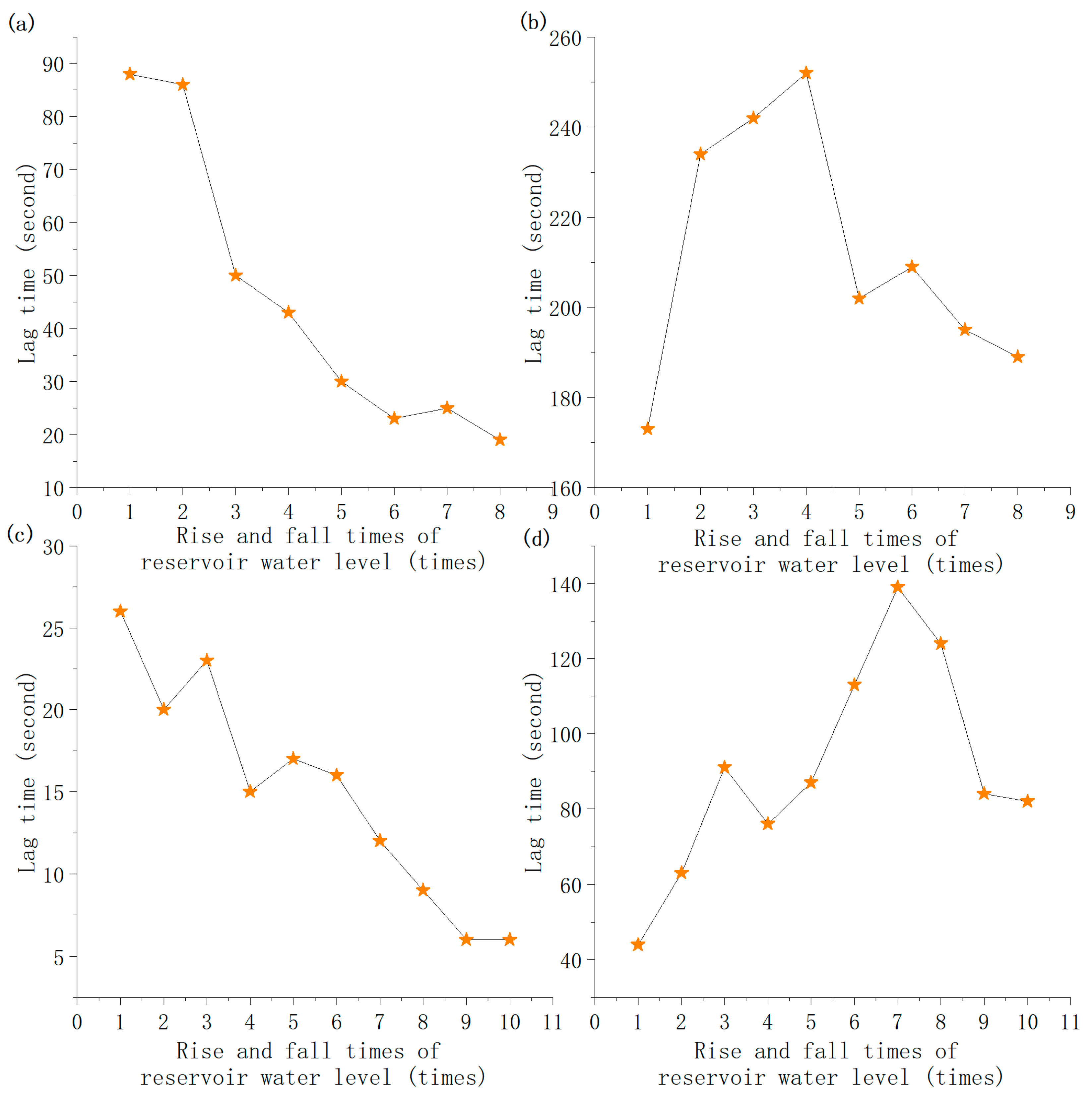
3.1.2. Quantitative Analysis of Permeability Change
3.1.3. Qualitative Analysis of Permeability Change
3.2. Characteristics of Deformation and Stability
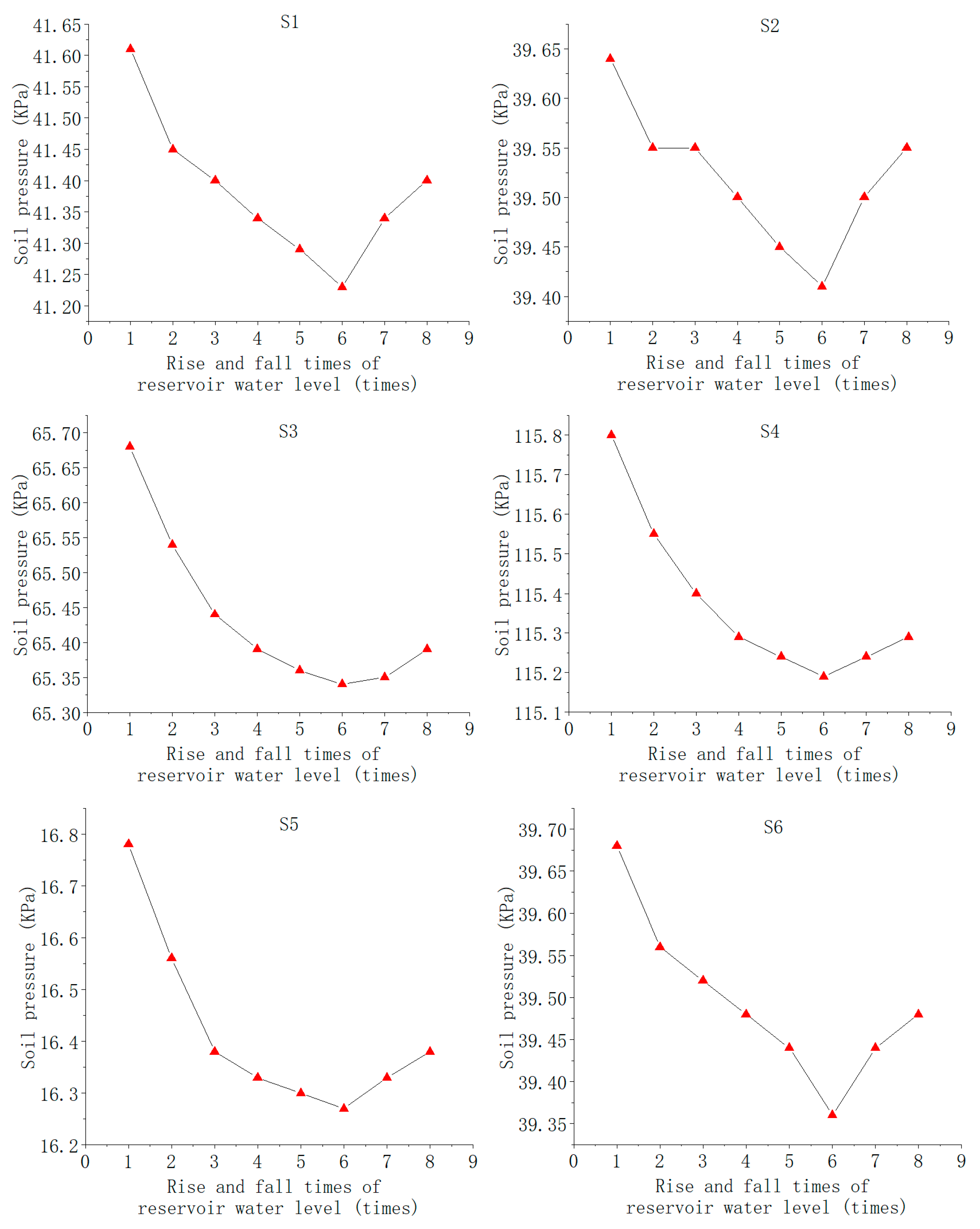
3.2.1. Changes in Earth Pressure
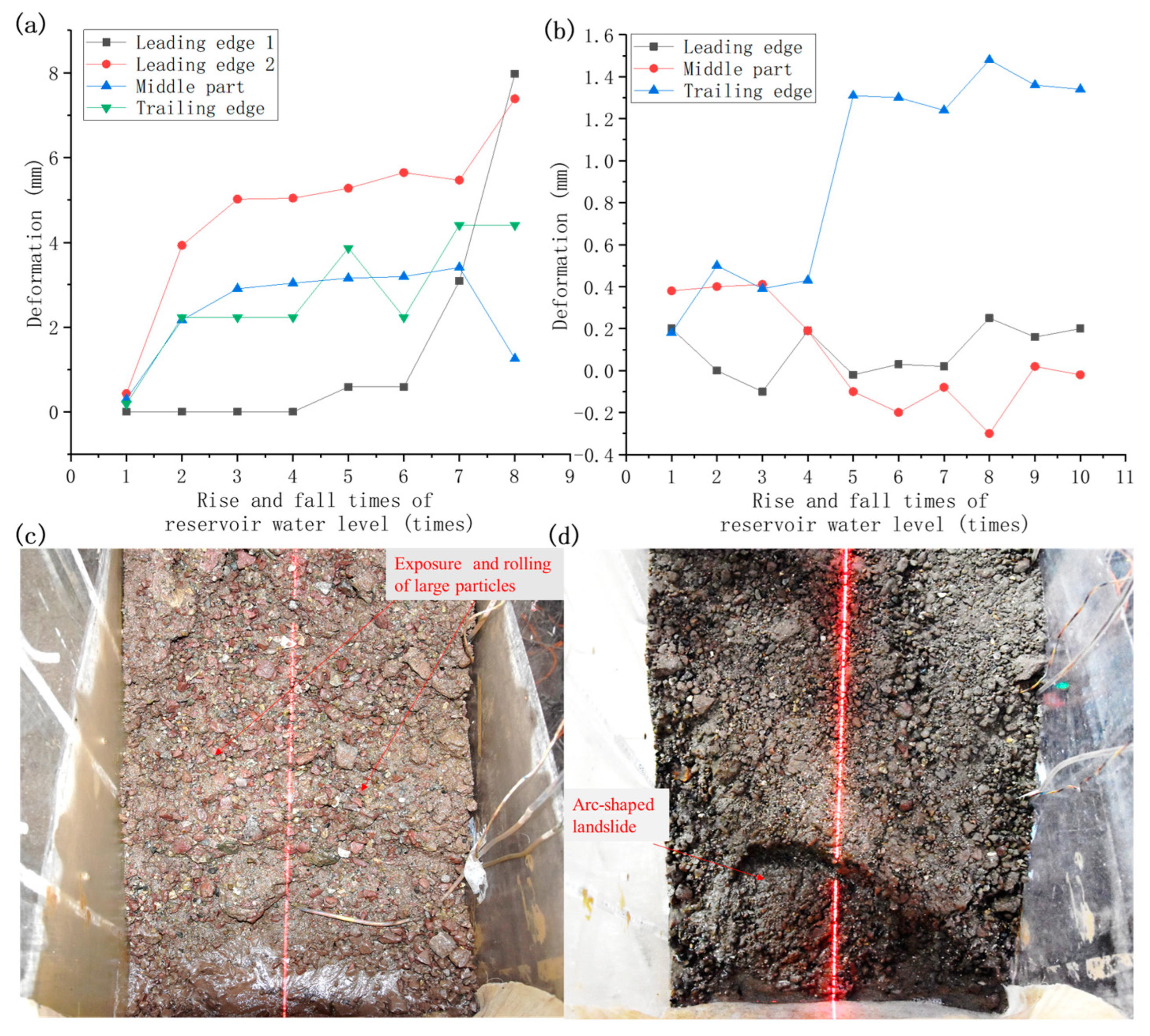
3.2.2. Changes in Surface Displacement
4. Discussion
4.1. Mechanism Analysis of Permeability Change
4.1.1. Effect of Deformation
4.1.2. Effect of Seepage Force
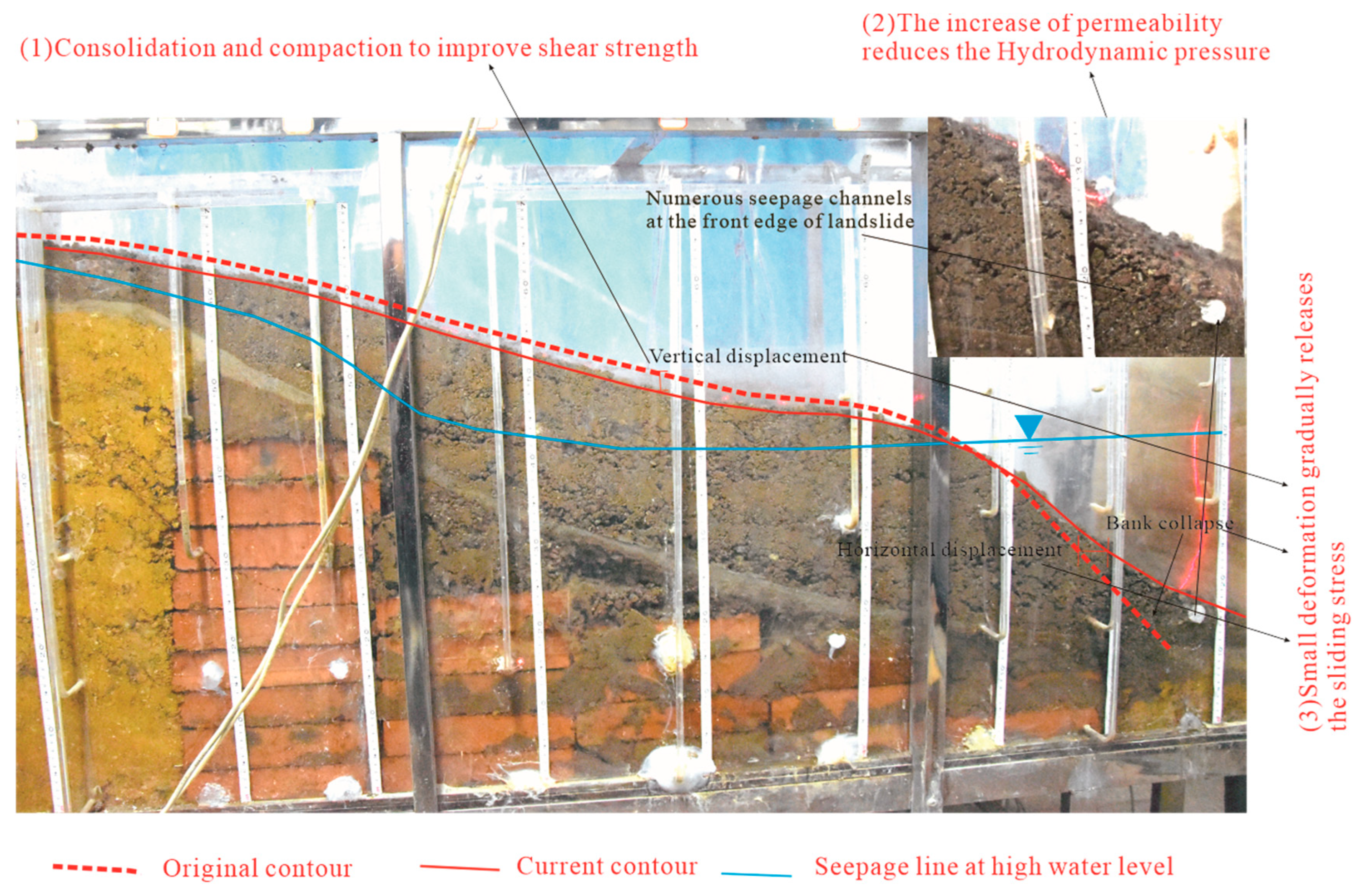
4.2. Mechanism Analysis of Deformation Self-Adaptation
- (1)
- Consolidation and compaction
- (2)
- Increased permeability
- (3)
- Release of sliding stress
5. Conclusions
- (1)
- Due to the rise of the impounded water level and the subsequent 12 years of operation during which the reservoir water level rose and fell, the permeability of the landslide accumulation in the Three Gorges Reservoir area changed. The permeability first decreased and then increased, and the permeability of the leading edge increased greatly. The main factors affecting the permeability change were collapsible compaction, seepage, front bank collapse, internal deformation, and the rate of reservoir water level rise and fall.
- (2)
- When the landslide underwent small deformation, the permeability of the slope above the groundwater level increased. However, the seepage channels of the slope below the groundwater level may have been blocked. After many cycles of the reservoir water level rising and falling, new and relatively stable seepage channels (pipeline seepage) formed in the slope, composing the network seepage.
- (3)
- The model test results showed that the landslide mass deformation was self-adaptive. The main reasons for the self-adaptive characteristics were as follows: (1) the long-term consolidation compaction caused the shear strength of the landslide body to increase gradually; (2) the small deformation caused the sliding stress to release, and the sliding force decreased; and (3) the permeability increased and caused the seepage force to decrease. The interactions of these three factors improved the stability of the landslide.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Van Asch, T.W.J.; Malet, J.P.; Bogaard, T.A. The effect of groundwater fluctuations on the velocity pattern of slow-moving landslides. Nat. Hazards Earth Syst. Sci. 2009, 9, 739–749. [Google Scholar] [CrossRef]
- Jiao, Y.Y.; Song, L.; Tang, H.M.; Li, Y.A. Material Weakening of Slip Zone Soils Induced by Water Level Fluctuation in the Ancient Landslides of Three Gorges Reservoir. Adv. Mater. Sci. Eng. 2014, 2014, 202340. [Google Scholar] [CrossRef]
- Yin, Y.; Huang, B.; Wang, W.; Wei, Y.; Ma, X.; Ma, F.; Zhao, C. Reservoir-induced landslides and risk control in Three Gorges Project on Yangtze River, China. J. Rock Mech. Geotech. Eng. 2016, 8, 577–595. [Google Scholar] [CrossRef]
- Zhao, N.; Hu, B.; Yi, Q.; Yao, W.; Ma, C. The Coupling Effect of Rainfall and Reservoir Water Level Decline on the Baijiabao Landslide in the Three Gorges Reservoir Area, China. Geofluids 2017, 2017, 3724867. [Google Scholar] [CrossRef]
- Yang, H.; Tang, M.G.; Xu, Q.; Xiao, X.X.; Li, H.J. Modification of phreatic line calculation model for landslide accumulation in the Three Gorges Reservoir area. Bull. Eng. Geol. Environ. 2022, 81, 175. [Google Scholar] [CrossRef]
- Gibo, S.; Chen, H.H.; Egashira, K.; Hayashi, Y.; Zhou, Y. Residual and Recovery Strength Characteristics of Soil from the Reactivated Landslide Occurred at the National Road across the Middle Part of Taiwan. Landslides 1997, 34, 50–56_1. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Gibo, S.; Egashira, K.; Ohtsubo, M.; Nakamura, S. Strength recovery from residual state in reactivated landslides. Géotechnique 2002, 52, 683–686. [Google Scholar] [CrossRef]
- Bhat, D.; Bhandary, N.; Yatabe, R. Experimental Study of Strength Recovery from Residual Strength on Kaolin Clay. Int. J. Civ. Environ. Eng. 2013, 7, 76–82. [Google Scholar]
- Doglioni, A.; Simeone, V. Recovery of Strength along Shear Surfaces in Clay Soils. Landslide Sci. Pract. 2013, 3, 183–188. [Google Scholar]
- Li, Y. Study on the Response and Genetic Mechanism of Reactivation Deformation of Landslide to the Variation of Reservoir Water Level in the Three Gorges Reservoir Area. Master’s Thesis, Chengdu University of Technology, Chengdu, China, 2018. [Google Scholar]
- Nian, T.K.; Feng, Z.K.; Yu, P.C.; Wu, H.J. Strength behavior of slip-zone soils of landslide subject to the change of water content. Nat. Hazards 2013, 68, 711–721. [Google Scholar] [CrossRef]
- Julina, M.; Thyagaraj, T. Combined effects of wet-dry cycles and interacting fluid on desiccation cracks and hydraulic conductivity of compacted clay. Eng. Geol. 2020, 267, 105505. [Google Scholar] [CrossRef]
- Chen, H.; Ma, W.; Yuan, X.; Niu, C.; Shi, B.; Tian, G. Influence of stress conditions on shear behavior of slip zone soil in ring shear test: An experimental study and numerical simulation. Nat. Hazards 2022, 111, 1179–1197. [Google Scholar] [CrossRef]
- Yan, F. Study on Mechanism of Reservoir Landslide Reactivation and Prediction of Its Development Trend. Ph.D. Thesis, Institute of Geology and Geophysics, Chinese Academy of Science, Beijing, China, 2004. [Google Scholar]
- Cho, S.E. Probabilistic stability analysis of rainfall-induced landslides considering spatial variability of permeability. Eng. Geol. 2014, 171, 11–20. [Google Scholar] [CrossRef]
- Chang, K.-T.; Cheng, M.-C. Estimation of the shear strength of gravel deposits based on field investigated geological factors. Eng. Geol. 2014, 171, 70–80. [Google Scholar] [CrossRef]
- Xu, X.; Zhang, J.; Ji, E.; Wang, L.; Huang, P.; Wang, X. A Laboratory Simulation Experiment to Assess Permeability and Shear Strength of a Gravel Soil Colluvium. Water 2023, 15, 3089. [Google Scholar] [CrossRef]
- Yao, M.; Wang, Q.; Yu, Q.; Wu, J.; Li, H.; Dong, J.; Xia, W.; Han, Y.; Huang, X. Mechanism Study of Differential Permeability Evolution and Microscopic Pore Characteristics of Soft Clay under Saturated Seepage: A Case Study in Chongming East Shoal. Water 2023, 15, 968. [Google Scholar] [CrossRef]
- Wang, K.; Guo, Y.; Xu, H.; Dong, H.; Du, F.; Huang, Q. Deformation and permeability evolution of coal during axial stress cyclic loading and unloading: An experimental study. Geomech. Eng. 2021, 24, 519–529. [Google Scholar]
- Ivoke, J.; Khan, M.S.; Nobahar, M. Unsaturated Hydraulic Conductivity Variation of Expansive Yazoo Clay with Wet-Dry Cycles. Transp. Res. Rec. 2021, 2675, 629–641. [Google Scholar] [CrossRef]
- Okeefe, S. The Recovery of Soils after Compaction: A Laboratory Investigation into the Effect of Wet/Dry Cycles on Bulk Density and Soil Hydraulic Functions. Ph.D. Thesis, Cranfield University, Cranfield, UK, 2009. [Google Scholar]
- Li, S. Study on the Deformation Response and Self-Adaptability of Landslide in the Three Gorges Reservoir Area to the Variation of Reservoir Water Level. Ph.D. Thesis, Chengdu University of Technology, Chengdu, China, 2020. [Google Scholar]
- Xiao, X.X.; Gutiérrez, F.; Guerrero, J. The impact of groundwater drawdown and vacuum pressure on sinkhole development. Physical laboratory models. Eng. Geol. 2020, 279, 105894. [Google Scholar] [CrossRef]
- Kasama, K.; Zen, K.; Nakagawa, Y.; Furukawa, Z. Instability evaluation of rubble mound for breakwaters subjected to tsunami-induced overflow and seepage flow. Soils Found. 2020, 60, 1532–1545. [Google Scholar] [CrossRef]
- Wang, H.K.; Qian, H.; Gao, Y.Y. Non-darcy flow in loess at low hydraulic gradient. Eng. Geol. 2020, 267, 105483. [Google Scholar] [CrossRef]
- Xu, Q.; Yang, H.; Tang, M.G.; Li, S.L.; Cai, G.J. Variability of permeability and seepage characteristics in soil landslides: A tests case in the three gorges reservoir area, China. Water Supply 2019, 19, 2453–2463. [Google Scholar] [CrossRef]
- Shen, H.; Huang, Y.; Tang, Y.; Qiu, H.; Wang, P. Impact Analysis of Karst Reservoir Construction on the Surrounding Environment: A Case Study for the Southwest of China. Water 2019, 11, 2327. [Google Scholar] [CrossRef]
- Zhang, W.; Pan, X.; Liang, J.; Zeng, J.; Song, C. Study on the Hydrogeological Structure of a Karst Subterranean River and Seepage of a Karst Reservoir: A Case Study of the Yibasan Reservoir in Yunnan Province, China. Water 2024, 16, 92. [Google Scholar] [CrossRef]
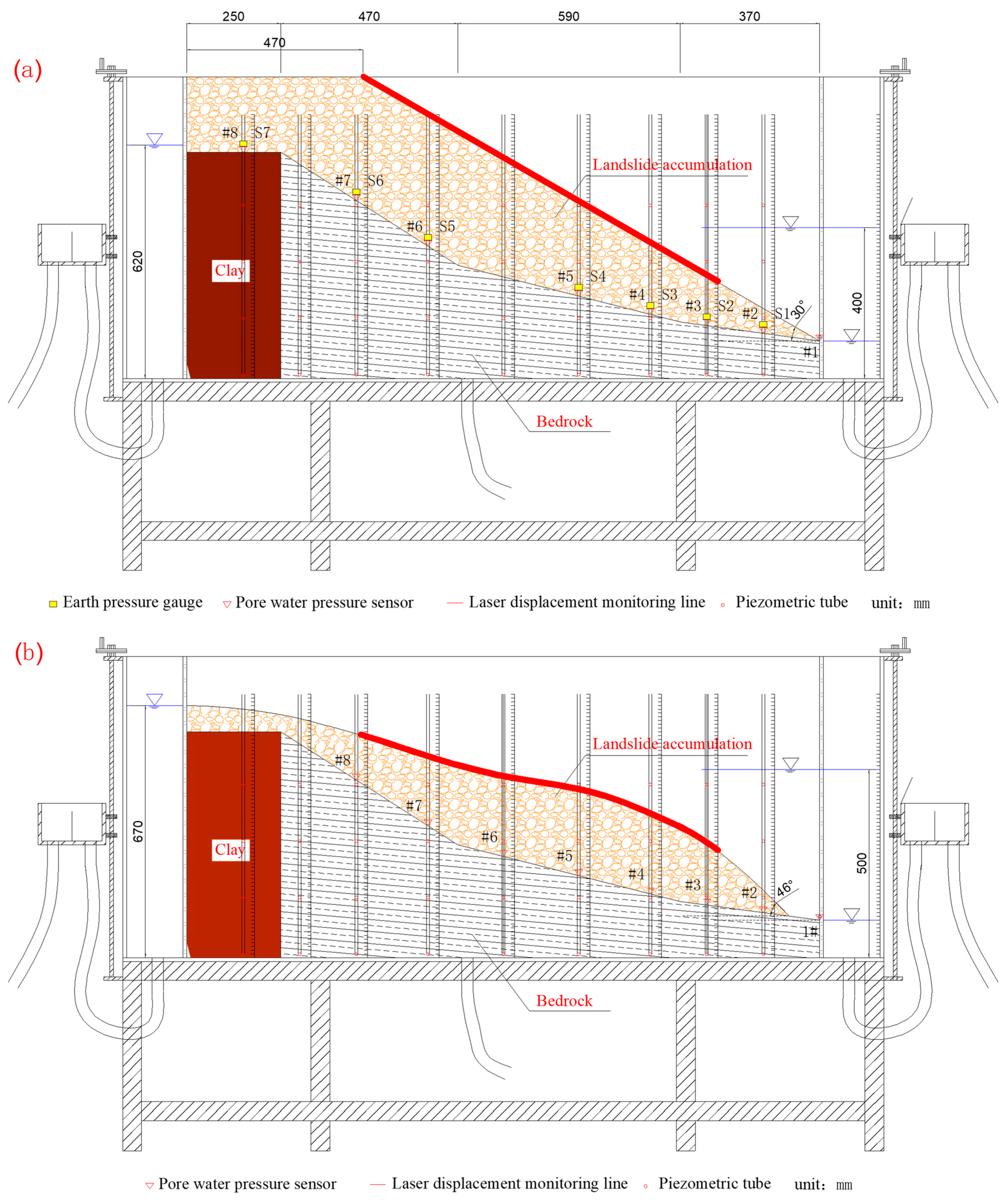
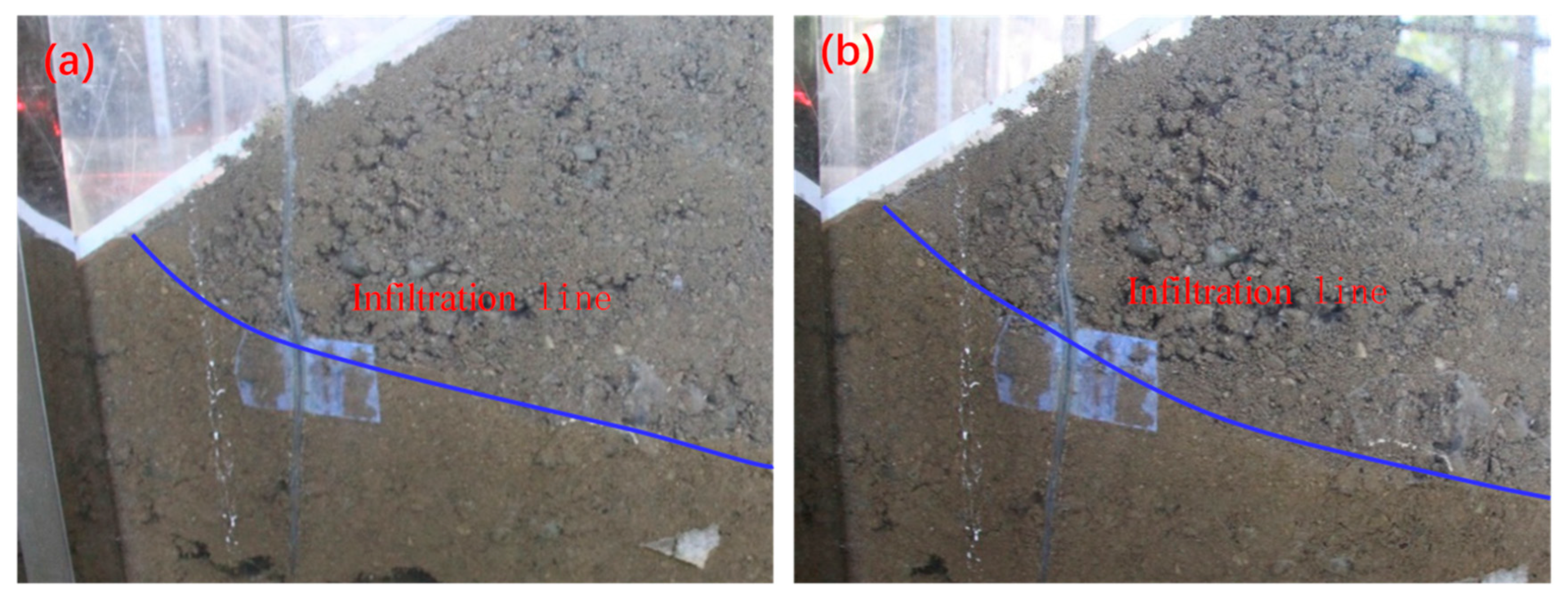
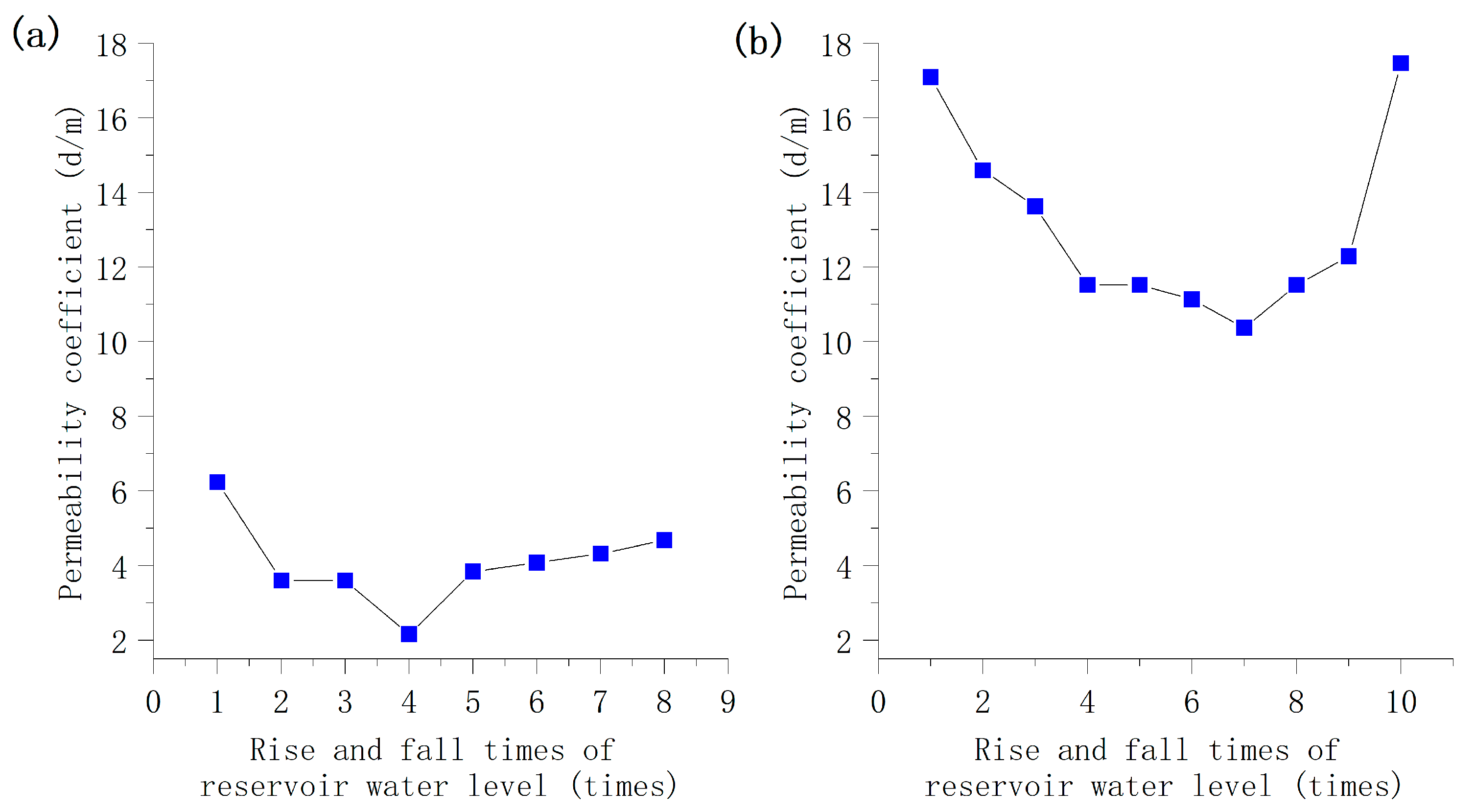

| Condition No. | Gravel Particle Content above 2 mm | Trailing Edge Water Head (cm) | Leading Edge Starting Water Level (cm) | Rise and Fall of Reservoir Water Level (cm) | Rate of Rise and Fall |
|---|---|---|---|---|---|
| Model 1 | 53% | 62 | 10 | 10–40–10 | The rate of rise and fall of the reservoir water level was 10 cm/5 min in the 1st to the 6th cycle and 30 cm/time in the 7th and 8th cycles |
| Model 2 | 76% | 67 | 10 | 10–50–10 | To increase the hydraulic gradient, the rate of water level rise and fall was 40 cm/time |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, H.; Tang, M.; Xiao, X.; Cai, G.; Wei, Y.; Li, S.; Li, H.; Xie, J. Physical Model Test of Deformation Self-Adaptive Mechanism of Landslide Mass. Water 2024, 16, 1720. https://doi.org/10.3390/w16121720
Yang H, Tang M, Xiao X, Cai G, Wei Y, Li S, Li H, Xie J. Physical Model Test of Deformation Self-Adaptive Mechanism of Landslide Mass. Water. 2024; 16(12):1720. https://doi.org/10.3390/w16121720
Chicago/Turabian StyleYang, He, Minggao Tang, Xianxuan Xiao, Guojun Cai, Yong Wei, Songlin Li, Huajin Li, and Jingwei Xie. 2024. "Physical Model Test of Deformation Self-Adaptive Mechanism of Landslide Mass" Water 16, no. 12: 1720. https://doi.org/10.3390/w16121720
APA StyleYang, H., Tang, M., Xiao, X., Cai, G., Wei, Y., Li, S., Li, H., & Xie, J. (2024). Physical Model Test of Deformation Self-Adaptive Mechanism of Landslide Mass. Water, 16(12), 1720. https://doi.org/10.3390/w16121720







