Development and Application of a New Open-Source Integrated Surface–Subsurface Flow Model in Plain Farmland
Abstract
1. Introduction
2. Materials and Methods
2.1. Study Area
2.2. FullSWOF-Plain Model Structure
2.2.1. 2D Overland Flow Module
2.2.2. 1D Subsurface Flow Module
2.2.3. Integrated Method
2.3. Modeling Cases
3. Results
3.1. Validation of the Surface Flow Module
3.1.1. One-Dimensional Outflow Case
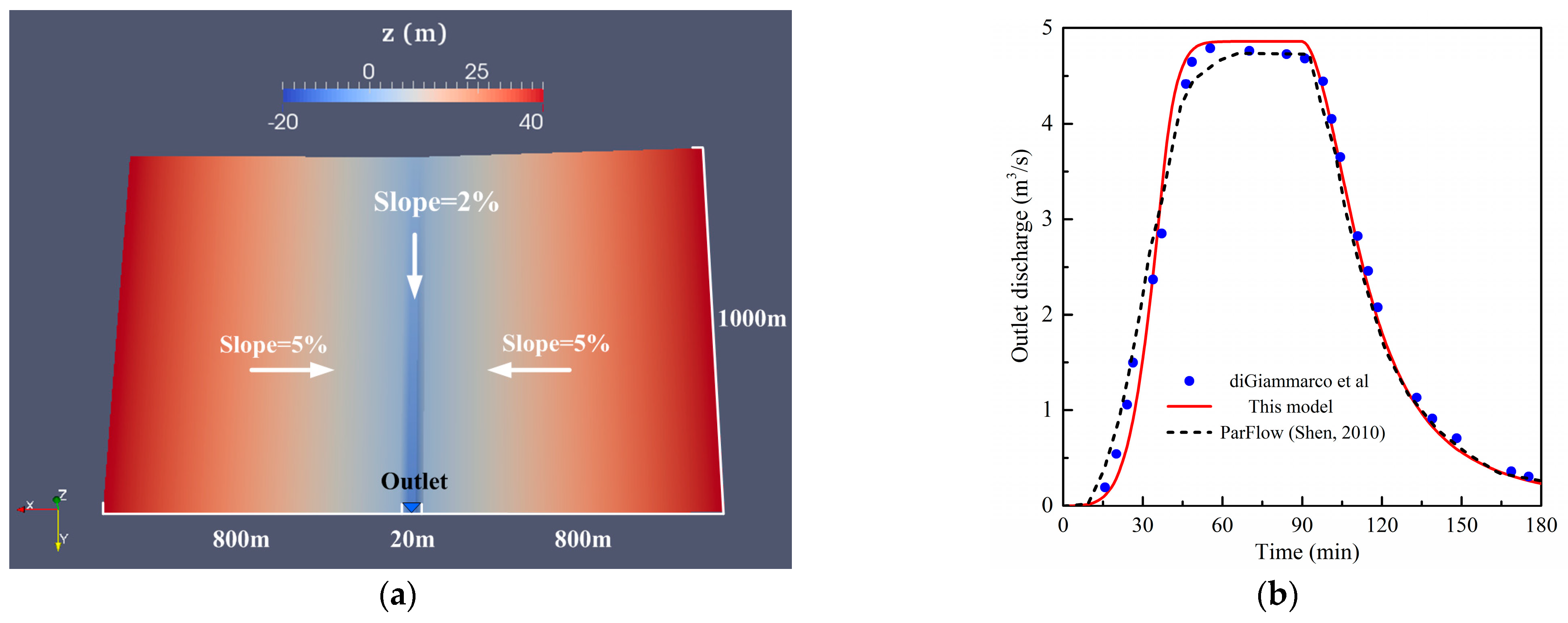
3.1.2. Two-Dimensional Outflow Case
3.2. Validation of the Subsurface Flow Module
3.2.1. A Classical One-Dimensional Infiltration Case
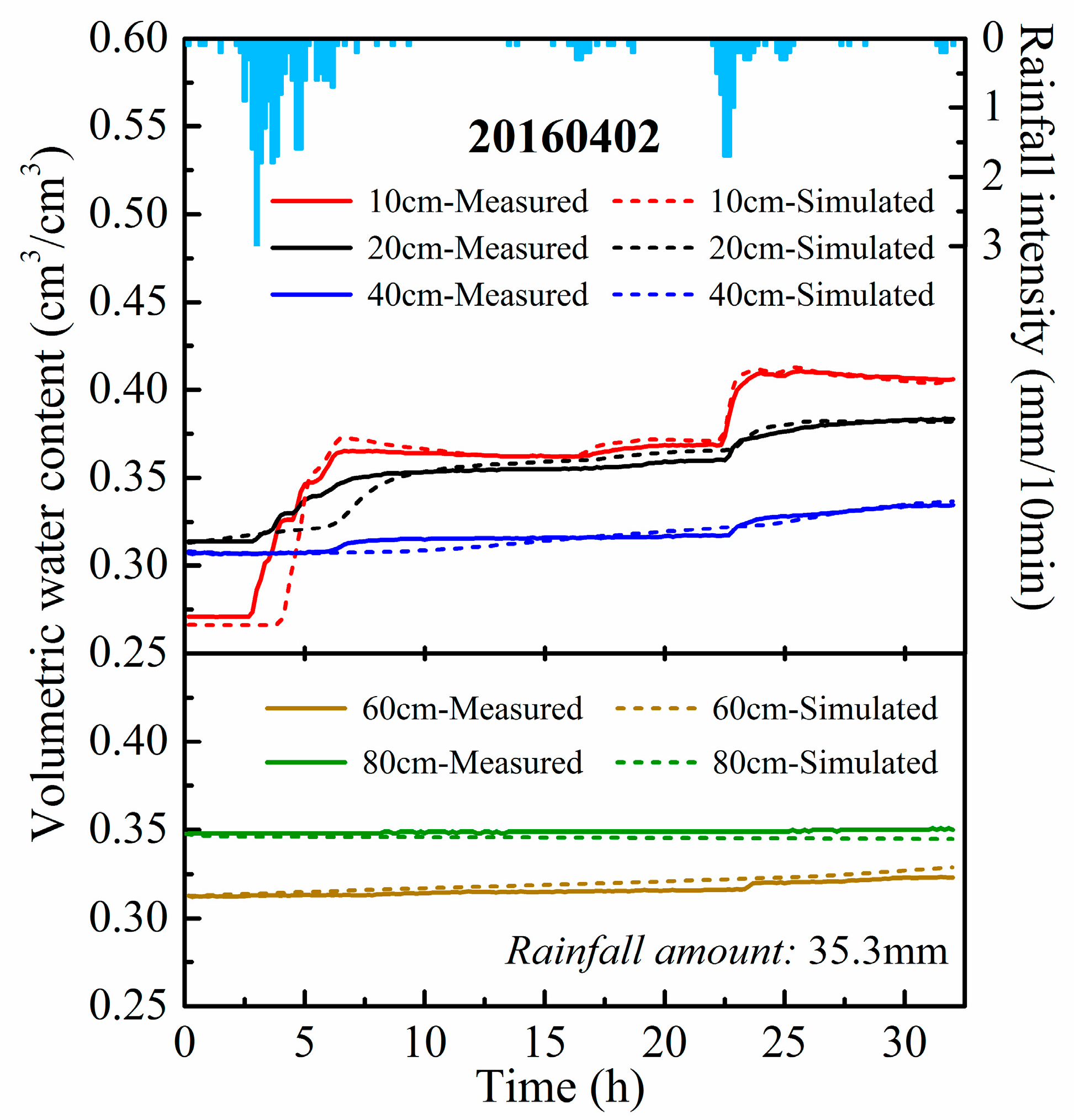
3.2.2. One-Dimensional Infiltration Case at the Experimental Field
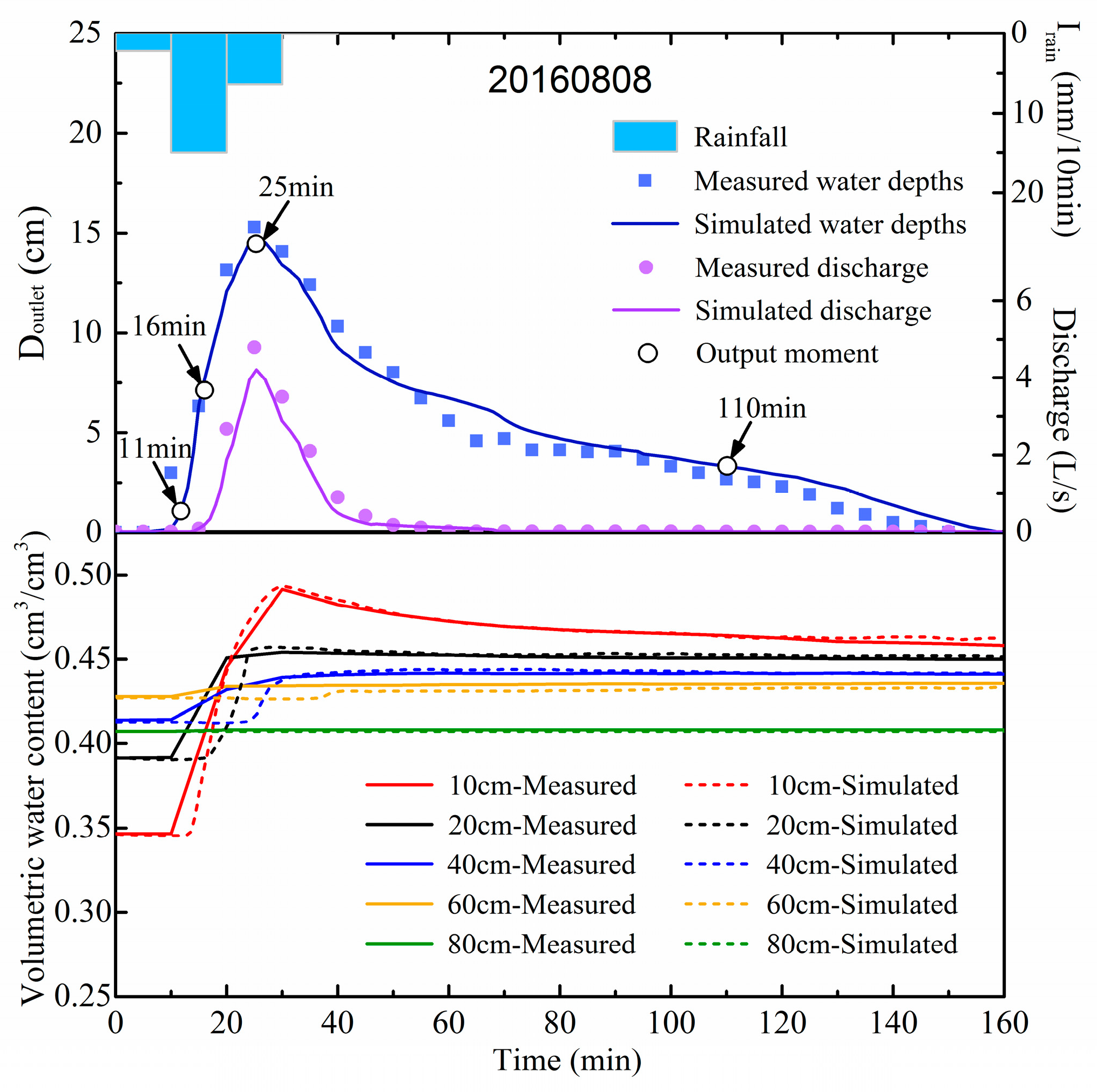
3.3. Validation of FullSWOF-Plain Model at Experimental Field
4. Discussion
5. Conclusions
- (1)
- The simulated results of this proposed model were accurate. The NSE of the simulated discharge exceeded 0.90 in the experimental field case, and the RMSE values for soil moisture at the five depths were consistently below 0.03 cm3/cm3. However, the simulated response time of soil moisture lagged due to the neglect of preferential flow.
- (2)
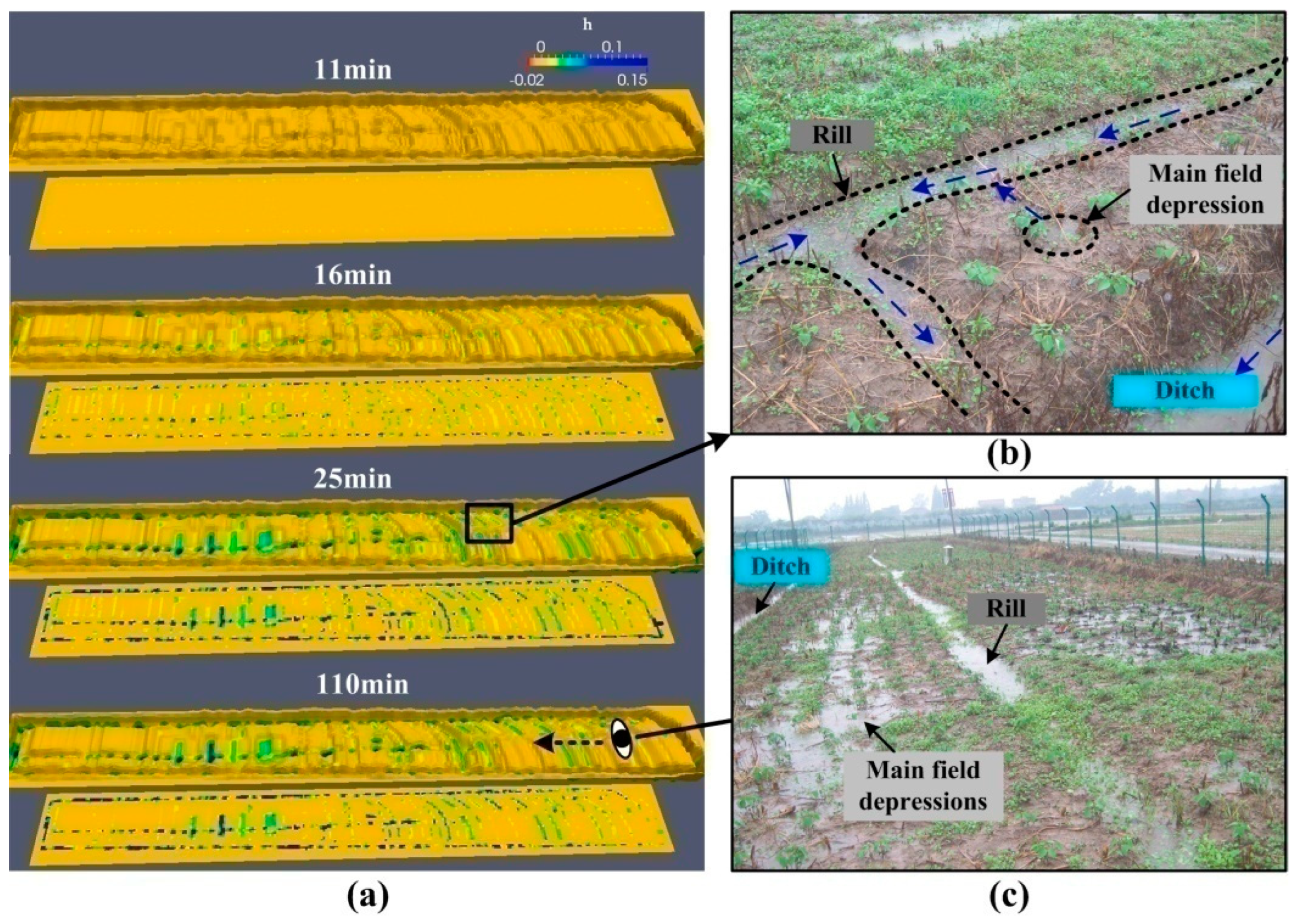
- Micro-topography at multiple scales greatly affected the ponding time and ponding areas. Lower local terrain normally experienced earlier surface ponding. Scattered surface ponding water initially occurred in the ditch and subsequently in the relatively low areas in the main field.
- (3)
- The concentration process of surface runoff exhibited hierarchical characteristics. The drainage ditch outflowed first, contributing the majority of the discharge during the early stage. As some adjacent scattered puddles in the main field connected, the excess surface water in these puddles drained into the ditch through rills.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Yan, R.; Li, L.; Gao, J. Framework for quantifying rural NPS pollution of a humid lowland catchment in Taihu Basin, Eastern China. Sci. Total Environ. 2019, 688, 983–993. [Google Scholar] [CrossRef] [PubMed]
- Appels, W.M.; Bogaart, P.W.; van der Zee, S.E.A.T.M. Feedbacks Between Shallow Groundwater Dynamics and Surface Topography on Runoff Generation in Flat Fields. Water Resour. Res. 2017, 53, 10336–10353. [Google Scholar] [CrossRef]
- Van der Ploeg, M.J.; Appels, W.M.; Cirkel, D.G.; Oosterwoud, M.R.; Witte, J.-P.M.; van der Zee, S.E.A.T.M. Microtopography as a Driving Mechanism for Ecohydrological Processes in Shallow Groundwater Systems. Vadose Zone J. 2012, 11, 811–822. [Google Scholar] [CrossRef]
- Planchon, O.; Esteves, M.; Silvera, N.; Lapetite, J.-M. Microrelief induced by tillage: Measurement and modelling of Surface Storage Capacity. Catena 2001, 46, 141–157. [Google Scholar] [CrossRef]
- Frei, S.; Fleckenstein, J.H. Representing effects of micro-topography on runoff generation and sub-surface flow patterns by using superficial rill/depression storage height variations. Environ. Model. Softw. 2014, 52, 5–18. [Google Scholar] [CrossRef]
- Habtezion, N.; Nasab, M.T.; Chu, X. How Does DEM Resolution Affect Microtopographic Characteristics, Hydrologic Connectivity, and Modeling of Hydrologic Processes? Hydrol. Process. 2016, 30, 4870–4892. [Google Scholar] [CrossRef]
- Appels, W.M.; Bogaart, P.W.; van der Zee, S.E.A.T.M. Influence of spatial variations of microtopography and infiltration on surface runoff and field scale hydrological connectivity. Adv. Water Resour. 2011, 34, 303–313. [Google Scholar] [CrossRef]
- Carvajal, F.; Aguilar, M.A.; Agüera, F.; Aguilar, F.J.; Giráldez, J.V. Maximum Depression Storage and Surface Drainage Network in Uneven Agricultural Landforms. Biosyst. Eng. 2006, 95, 281–293. [Google Scholar] [CrossRef]
- Hansen, B. Estimation of surface runoff and water-covered area during filling of surface microrelief depressions. Hydrol. Process. 2000, 14, 1235–1243. [Google Scholar] [CrossRef]
- Chu, X.; Yang, J.; Chi, Y.; Zhang, J. Dynamic puddle delineation and modeling of puddle-to-puddle filling-spilling-merging-splitting overland flow processes. Water Resour. Res. 2013, 49, 3825–3829. [Google Scholar] [CrossRef]
- Appels, W.M.; Bogaart, P.W.; van der Zee, S.E.A.T.M. Surface runoff in flat terrain: How field topography and runoff generating processes control hydrological connectivity. J. Hydrol. 2016, 534, 493–504. [Google Scholar] [CrossRef]
- Frei, S.; Lischeid, G.; Fleckenstein, J.H. Effects of micro-topography on surface–subsurface exchange and runoff generation in a virtual riparian wetland—A modeling study. Adv. Water Resour. 2010, 33, 1388–1401. [Google Scholar] [CrossRef]
- Freeze, R.A.; Harlan, R.L. Blueprint for a physically-based, digitally-simulated hydrologic response model. J. Hydrol. 1969, 9, 237–258. [Google Scholar] [CrossRef]
- Ebel, B.A.; Loague, K. Physics-based hydrologic-response simulation: Seeing through the fog of equifinality. Hydrol. Process. 2006, 20, 2887–2900. [Google Scholar] [CrossRef]
- Paniconi, C.; Putti, M. Physically based modeling in catchment hydrology at 50: Survey and outlook. Water Resour. Res. 2015, 51, 7090–7129. [Google Scholar] [CrossRef]
- Loague, K.; VanderKwaak, J.E. Physics-based hydrologic response simulation: Platinum bridge, 1958 Edsel, or useful tool. Hydrol. Process. 2004, 18, 2949–2956. [Google Scholar] [CrossRef]
- Kampf, S.K.; Burges, S.J. A framework for classifying and comparing distributed hillslope and catchment hydrologic models. Water Resour. Res. 2007, 43, W05423. [Google Scholar] [CrossRef]
- Furman, A. Modeling coupled surface-subsurface flow processes: A review. Vadose Zone J. 2008, 7, 741–756. [Google Scholar] [CrossRef]
- Sebben, M.L.; Werner, A.D.; Liggett, J.E.; Partington, D.; Simmons, C.T. On the testing of fully integrated surface–subsurface hydrological models. Hydrol. Process. 2013, 27, 1276–1285. [Google Scholar] [CrossRef]
- Refsgaard, J.C.; Stisen, S.; Koch, J. Hydrological process knowledge in catchment modelling—Lessons and perspectives from 60 years development. Hydrol. Process. 2022, 36, e14463. [Google Scholar] [CrossRef]
- Ebel, B.A.; Loague, K.; Vanderkwaak, J.E.; Dietrich, W.E.; Montgomery, D.R.; Torres, R.; Anderson, S.P. Near-surface hydrologic response for a steep, unchanneled catchment near Coos Bay, Oregon: 2. Physics-based simulations. Am. J. Sci. 2007, 307, 709–748. [Google Scholar] [CrossRef]
- Mirus, B.B.; Loague, K.; VanderKwaak, J.E.; Kampf, S.K.; Burges, S.J. A hypothetical reality of Tarrawarra-like hydrologic response. Hydrol. Process. 2009, 23, 1093–1103. [Google Scholar] [CrossRef]
- Abbott, M.B.; Bathurst, J.C.; Cunge, J.A.; O’Connell, P.E.; Rasmussen, J. An introduction to the European Hydrological System—Systeme Hydrologique Europeen, “SHE”, 1: History and philosophy of a physically-based, distributed modelling system. J. Hydrol. 1986, 87, 45–59. [Google Scholar] [CrossRef]
- Abbott, M.B.; Bathurst, J.C.; Cunge, J.A.; O’Connell, P.E.; Rasmussen, J. An introduction to the European Hydrological System—Systeme Hydrologique Europeen, “SHE”, 2: Structure of a physically-based, distributed modelling system. J. Hydrol. 1986, 87, 61–77. [Google Scholar] [CrossRef]
- VanderKwaak, J.E. Numerical Simulation of Flow and Chemical Transport in Integrated Surface-Subsurface Hydrologic Systems; University of Waterloo: Waterloo, ON, Canada, 1999. [Google Scholar]
- Morita, M.; Chie Yen, B. Numerical methods for conjunctive two-dimensional surface and three-dimensional sub-surface flows. Int. J. Numer. Methods Fluids 2000, 32, 921–957. [Google Scholar] [CrossRef]
- Morita, M.; Yen, B.C. Modeling of conjunctive two-dimensional surface-three-dimensional subsurface flows. J. Hydraul. Eng. 2002, 128, 184–200. [Google Scholar] [CrossRef]
- Panday, S.; Huyakorn, P.S. A fully coupled physically-based spatially-distributed model for evaluating surface/subsurface flow. Adv. Water Resour. 2004, 27, 361–382. [Google Scholar] [CrossRef]
- Yeh, G.T.; Huang, G.B.; Zhang, F.; Lin, H.C.; Edris, E.; Richards, D. A Numerical Model of Flow, Heat transfer, and Salinity, Sediment, and Water Quality Transport in Watershed Systems of 1-D Stream-River Network, 2-D Overland Regime, and 3D Subsurface Media (WASH123D: Version 2.0); U.S. Army Corps of Eng: Vicksburg, MI, USA, 2004. [Google Scholar]
- Therrien, R.; McLaren, R.G.; Sudicky, E.A.; Panday, S.M. HydroGeoSphere-A Three-Dimensional Numerical Model Describing Fully-Integrated Subsurface and Surface Flow and Solute Transport; Groundwater Simulations Group: Waterloo, ON, Canada, 2010. [Google Scholar]
- Kollet, S.J.; Maxwell, R.M. Integrated surface–groundwater flow modeling: A free-surface overland flow boundary condition in a parallel groundwater flow model. Adv. Water Resour. 2006, 29, 945–958. [Google Scholar] [CrossRef]
- Qu, Y.; Duffy, C.J. A semidiscrete finite volume formulation for multiprocess watershed simulation. Water Resour. Res. 2007, 43, W08419. [Google Scholar] [CrossRef]
- He, Z.; Wu, W.; Wang, S.S. Coupled Finite-Volume Model for 2D Surface and 3D Subsurface Flows. J. Hydrol. Eng. 2008, 13, 835–845. [Google Scholar] [CrossRef]
- Kumar, M.; Duffy, C.J.; Salvage, K.M. A Second-Order Accurate, Finite Volume–Based, Integrated Hydrologic Modeling (FIHM) Framework for Simulation of Surface and Subsurface Flow. Vadose Zone J. 2009, 8, 873–890. [Google Scholar] [CrossRef]
- Kolditz, O.; Bauer, S.; Bilke, L.; Böttcher, N.; Delfs, J.O.; Fischer, T.; Görke, U.J.; Kalbacher, T.; Kosakowski, G.; McDermott, C.I.; et al. OpenGeoSys: An open-source initiative for numerical simulation of thermo-hydro-mechanical/chemical (THM/C) processes in porous media. Environ. Earth Sci. 2012, 67, 589–599. [Google Scholar] [CrossRef]
- Warsta, L.; Taskinen, A.; Koivusalo, H.; Paasonen-Kivekäs, M.; Karvonen, T. Modelling soil erosion in a clayey, subsurface-drained agricultural field with a three-dimensional FLUSH model. J. Hydrol. 2013, 498, 132–143. [Google Scholar] [CrossRef]
- Shen, C.; Phanikumar, M.S. A process-based, distributed hydrologic model based on a large-scale method for surface–subsurface coupling. Adv. Water Resour. 2010, 33, 1524–1541. [Google Scholar] [CrossRef]
- Shokri, A.; Bardsley, W.E. Development, testing and application of DrainFlow: A fully distributed integrated surface-subsurface flow model for drainage study. Adv. Water Resour. 2016, 92, 299–315. [Google Scholar] [CrossRef]
- De Maet, T.; Cornaton, F.; Hanert, E. A scalable coupled surface–subsurface flow model. Comput. Fluids 2015, 116, 74–87. [Google Scholar] [CrossRef]
- Tian, D.f.; Zheng, H.; Liu, D.f. A 2D integrated FEM model for surface water–groundwater flow of slopes under rainfall condition. Landslides 2017, 14, 577–593. [Google Scholar] [CrossRef]
- Giammarco, P.D.; Todini, E.; Lamberti, P. A conservative finite elements approach to overland flow: The control volume finite element formulation. J. Hydrol. 1996, 175, 267–291. [Google Scholar] [CrossRef]
- Lighthill, M.J.; Whitham, G.B. On Kinematic Waves. I. Flood Movement in Long Rivers. Proc. R. Soc. Lond. A Math. Phys. Eng. Sci. 1955, 229, 281–316. [Google Scholar]
- Costabile, P.; Costanzo, C.; Macchione, F. Comparative analysis of overland flow models using finite volume schemes. J. Hydroinformatics 2012, 14, 122–135. [Google Scholar] [CrossRef]
- Yeh, G.-T.; Shih, D.-S.; Cheng, J.-R.C. An integrated media, integrated processes watershed model. Comput. Fluids 2011, 45, 2–13. [Google Scholar] [CrossRef]
- Zhou, Q.Y.; Shimada, J.; Sato, A. Temporal variations of the three-dimensional rainfall infiltration process in heterogeneous soil. Water Resour. Res. 2002, 38, 1-1–1-15. [Google Scholar] [CrossRef]
- Singh, J.; Altinakar, M.S.; Ding, Y. Numerical Modeling of Rainfall-Generated Overland Flow Using Nonlinear Shallow-Water Equations. J. Hydrol. Eng. 2015, 20, 04014089. [Google Scholar] [CrossRef]
- Simons, F.; Busse, T.; Hou, J.; Özgen, I.; Hinkelmann, R. A model for overland flow and associated processes within the Hydroinformatics Modelling System. J. Hydroinformatics 2014, 16, 375–391. [Google Scholar] [CrossRef]
- Fiedler, F.R.; Ramirez, J.A. A numerical method for simulating discontinuous shallow flow over an infiltrating surface. Int. J. Numer. Methods Fluids 2000, 32, 219–239. [Google Scholar] [CrossRef]
- Esteves, M.; Faucher, X.; Galle, S.; Vauclin, M. Overland flow and infiltration modelling for small plots during unsteady rain: Numerical results versus observed values. J. Hydrol. 2000, 228, 265–282. [Google Scholar] [CrossRef]
- Delestre, O.; Darboux, F.; James, F.; Lucas, C.; Laguerre, C.; Cordier, S. FullSWOF: A free software package for the simulation of shallow water flows. arXiv 2014, arXiv:1401.4125. [Google Scholar]
- Fernández-Pato, J.; Caviedes-Voullième, D.; García-Navarro, P. Rainfall/runoff simulation with 2D full shallow water equations: Sensitivity analysis and calibration of infiltration parameters. J. Hydrol. 2016, 536, 496–513. [Google Scholar] [CrossRef]
- Caviedes-Voullième, D.; García-Navarro, P.; Murillo, J. Influence of mesh structure on 2D full shallow water equations and SCS Curve Number simulation of rainfall/runoff events. J. Hydrol. 2012, 448-449, 39–59. [Google Scholar] [CrossRef]
- Delestre, O. Rain Water Overland Flow on Agricultural Fields Simulation; Université d’Orléans: Orléans, France, 2010. [Google Scholar]
- Unterweger, K.; Wittmann, R.; Neumann, P.; Weinzierl, T.; Bungartz, H.-J. Integration of FULLSWOF2D and PeanoClaw: Adaptivity and Local Time-Stepping for Complex Overland Flows. In Recent Trends in Computational Engineering—CE2014: Optimization, Uncertainty, Parallel Algorithms, Coupled and Complex Problems; Mehl, M., Bischoff, M., Schäfer, M., Eds.; Springer International Publishing: Cham, Switzerland, 2015; pp. 181–195. [Google Scholar]
- Nurritasari, F.A.; Sudibyakto, S.; Jetten, V.G. OpenLISEM Flash Flood Modelling Application in Logung Sub-Catchment, Central Java. Indones. J. Geogr. 2015, 47, 132–141. [Google Scholar] [CrossRef]
- Li, X.; Wang, C.; Chen, G.; Wang, Q.; Hu, Z.; Wu, J.; Wang, S.; Fang, X. Evaluating Efficiency Improvement of Deep-Cut Curb Inlets for Road-Bioretention Stripes. Water 2020, 12, 3368. [Google Scholar] [CrossRef]
- Hao, X.; Li, Y.; Liu, S. Comparison of dynamic flow interaction methods between pipe system and overland in urban flood analysis. Sci. Rep. 2021, 11, 12079. [Google Scholar] [CrossRef] [PubMed]
- Genuchten, B.V. HYDRUS-1D code for simulating the one-dimensional movement of water, heat, and multiple solutes in variably saturated media. In Proceedings of the International Ground Water Modeling Center, Colorado School of Mines, Golden, CO, USA, 4–8 October 1998. [Google Scholar]
- Simunek, J.; Van Genuchten, M.T.; Sejna, M. The HYDRUS-1D Software Package for Simulating the One-Dimensional Movement of Water, Heat, and Multiple Solutes in Variably-Saturated Media; U.S. Salinity Laboratory Agricultural Research Service, U.S. Department of Agriculture: Riverside, CA, USA, 2005; Volume 3, pp. 1–240. [Google Scholar]
- Šimůnek, J.; van Genuchten, M.T.; Šejna, M. Recent Developments and Applications of the HYDRUS Computer Software Packages. Vadose Zone J. 2016, 15, vzj2016.04.0033. [Google Scholar] [CrossRef]
- Tatard, L.; Planchon, O.; Wainwright, J.; Nord, G.; Favis-Mortlock, D.; Silvera, N.; Ribolzi, O.; Esteves, M.; Huang, C.H. Measurement and modelling of high-resolution flow-velocity data under simulated rainfall on a low-slope sandy soil. J. Hydrol. 2008, 348, 1–12. [Google Scholar] [CrossRef]
- Richards, L.A. Capillary conduction of liquids through porous mediums. J. Appl. Phys. 1931, 1, 318–333. [Google Scholar] [CrossRef]
- van Genuchten, M.T. A Closed-form Equation for Predicting the Hydraulic Conductivity of Unsaturated Soils. Soil Sci. Soc. Am. J. 1980, 44, 892–898. [Google Scholar] [CrossRef]
- Huang, G.; Yeh, G.-T. Comparative Study of Coupling Approaches for Surface Water and Subsurface Interactions. J. Hydrol. Eng. 2009, 14, 453–462. [Google Scholar] [CrossRef]
- Morita, M.; Yen, B.C. Study on interaction between surface and subsurface flows using conjunctive flow model. In Ground Water Updates; Sato, K., Iwasa, Y., Eds.; Springer: Berlin/Heidelberg, Germany, 2000; pp. 265–270. [Google Scholar]
- Brown, D.L. An Analysis of Transient Flow in Upland Watersheds: Interactions between Structure and Process; University of California: Berkeley, CA, USA, 1995. [Google Scholar]
- Kirstetter, G.; Hu, J.; Delestre, O.; Darboux, F.; Lagrée, P.Y.; Popinet, S.; Fullana, J.M.; Josserand, C. Modeling rain-driven overland flow: Empirical versus analytical friction terms in the shallow water approximation. J. Hydrol. 2016, 536, 1–9. [Google Scholar] [CrossRef]
- Nash, J.E.; Sutcliffe, J.V. RiverRiver flow forecasting through conceptual models part I—A discussion of principles. J. Hydrol. 1970, 10, 282–290. [Google Scholar] [CrossRef]
- Bradford, S.F.; Katopodes, N.D. Hydrodynamics of Turbid Underflows.I: Formulation and Numerical Analysis. J. Hydraul. Eng. 1999, 125, 1006–1015. [Google Scholar] [CrossRef]
- Sanders, B.F. High-resolution and non-oscillatory solution of the St. Venant equations in non-rectangular and non-prismatic channels. J. Hydraul. Res. 2001, 39, 321–330. [Google Scholar] [CrossRef]
- Shen, C. A Process-Based Distributed Hydrologic Model and Its Application to a Michigan Watershed; Michigan State University: East Lansing, MI, USA, 2009. [Google Scholar]
- Weill, S.; Mouche, E.; Patin, J. A generalized Richards equation for surface/subsurface flow modelling. J. Hydrol. 2009, 366, 9–20. [Google Scholar] [CrossRef]
- Tian, D.; Liu, D. A new integrated surface and subsurface flows model and its verification. Appl. Math. Model. 2011, 35, 3574–3586. [Google Scholar] [CrossRef]
- Huyakorn, P.S.; Springer, E.P.; Guvanasen, V.; Wadsworth, T.D. A three-dimensional finite-element model for simulating water flow in variably saturated porous media. Water Resour. Res. 1986, 22, 1790–1808. [Google Scholar] [CrossRef]
- Tan, X.; Shao, D.; Gu, W.; Liu, H. Field analysis of water and nitrogen fate in lowland paddy fields under different water managements using HYDRUS-1D. Agr. Water Manag. 2015, 150, 67–80. [Google Scholar] [CrossRef]
- Carsel, R.F.; Parrish, R.S. Developing joint probability distributions of soil water retention characteristics. Water Resour. Res. 1988, 24, 755–769. [Google Scholar] [CrossRef]
- Hofer, M.; Lehmann, P.; Biemelt, D.; Stähli, M.; Krafczyk, M. Modelling subsurface drainage pathways in an artificial catchment. Phys. Chem. Earth Parts A/B/C 2011, 36, 101–112. [Google Scholar] [CrossRef]
- Chow, V.T. Open-Channel Hydraulics; MaGraw-Hill: Tokyo, Japan, 1959; p. 680. [Google Scholar]
- Yang, J.; Chu, X. Quantification of the spatio-temporal variations in hydrologic connectivity of small-scale topographic surfaces under various rainfall conditions. J. Hydrol. 2013, 505, 65–77. [Google Scholar] [CrossRef]
- Yang, H.; Jiang, Y.; Zhou, Q.; Yang, H.; Ma, Q.; Zhang, C.; Wang, C. Effects of Microtopography on Runoff Generation in Plain Farmland: New Insights from an Event-Based Rainfall–Runoff Model. Water 2022, 14, 2686. [Google Scholar] [CrossRef]
- Chen, L.; Sela, S.; Svoray, T.; Assouline, S. The role of soil-surface sealing, microtopography, and vegetation patches in rainfall-runoff processes in semiarid areas. Water Resour. Res. 2013, 49, 5585–5599. [Google Scholar] [CrossRef]
- Buttinger-Kreuzhuber, A.; Konev, A.; Horváth, Z.; Cornel, D.; Schwerdorf, I.; Blöschl, G.; Waser, J. An integrated GPU-accelerated modeling framework for high-resolution simulations of rural and urban flash floods. Env. Model. Softw. 2022, 156, 105480. [Google Scholar] [CrossRef]

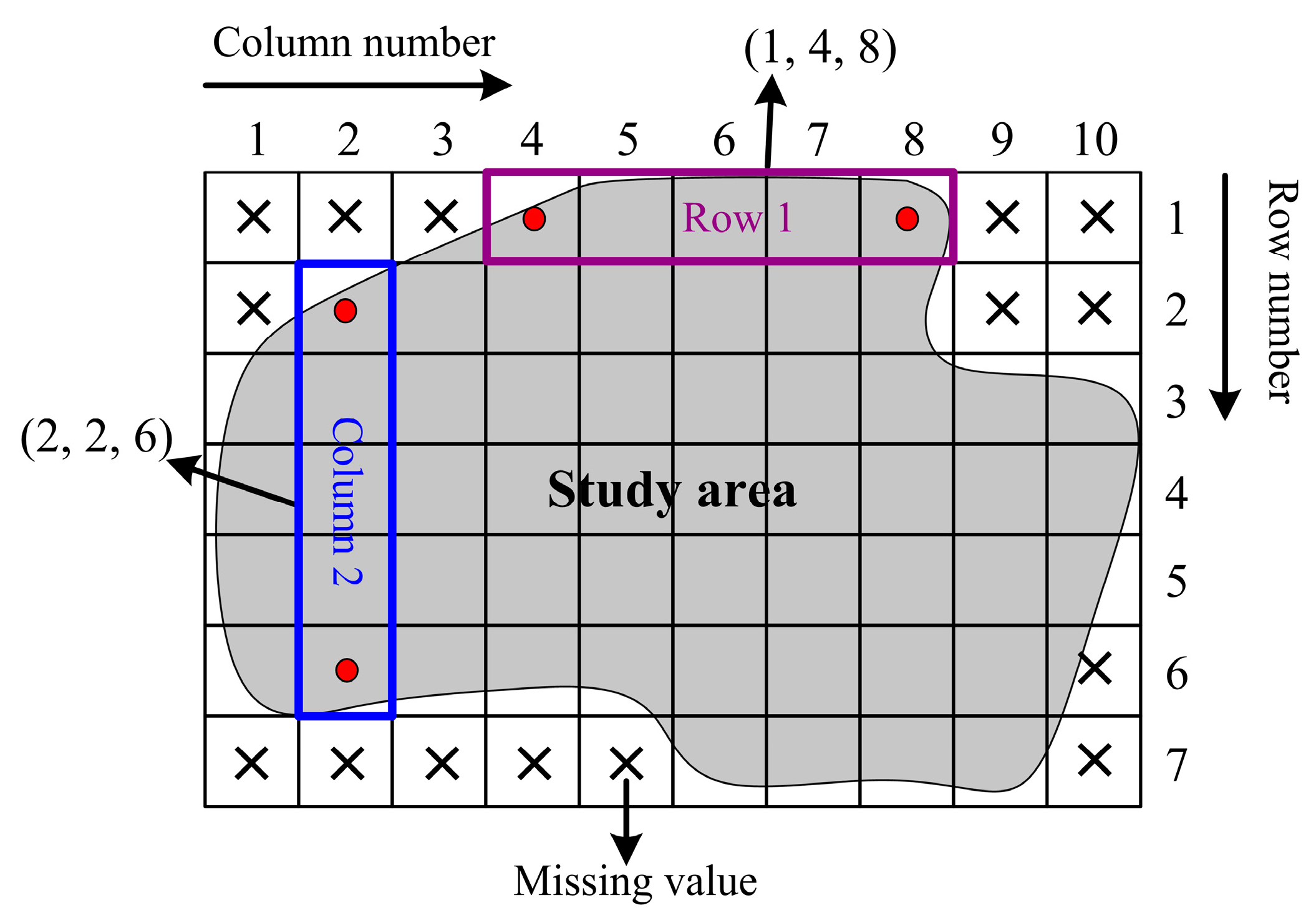


| Depth (cm) | Clay (-) | Silt (-) | (g/cm3) | (-) | (cm/min) |
|---|---|---|---|---|---|
| 0–10 | 0.31 (0.27–0.34) | 0.57 (0.53–0.62) | 1.20 (1.10–1.28) | 0.55 (0.52–0.58) | 0.07 (0.02–0.25) |
| 10–20 | 0.29 (0.26–0.34) | 0.61 (0.58–0.64) | 1.23 (1.05–1.50) | 0.50 (0.43–0.60) | 0.08 (0.01–0.22) |
| 20–40 | 0.29 (0.26–0.32) | 0.63 (0.61–0.67) | 1.47 (1.41–1.50) | 0.45 (0.43–0.47) | 0.07 (0.02–0.13) |
| 40–60 | 0.33 (0.30–0.35) | 0.61 (0.58–0.64) | 1.46 (1.35–1.53) | 0.45 (0.42–0.49) | 0.11 (0.04–0.18) |
| 60–80 | 0.41 (0.38–0.43) | 0.54 (0.53–0.58) | 1.46 (1.41–1.50) | 0.45 (0.42–0.47) | 0.03 (0.01–0.05) |
| Case Number | Rainfall Intensity Irain (mm/h) | Slope So (%) |
|---|---|---|
| 1 | 50 | 2 |
| 2 | 25 | 2 |
| 3 | 25 | 5 |
| Depth (cm) | (cm3/cm3) | (cm3/cm3) | (cm−1) | (-) | |
|---|---|---|---|---|---|
| 0–13 | 0.495 | 0.090 | 0.010 | 1.25 | 0.05 |
| 13–22 | 0.455 | 0.100 | 0.008 | 1.15 | 0.03 |
| 22–47 | 0.445 | 0.090 | 0.010 | 1.20 | 0.03 |
| 47–75 | 0.435 | 0.070 | 0.011 | 1.20 | 0.03 |
| 75–150 | 0.405 | 0.070 | 0.011 | 1.15 | 0.02 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, H.; Zhou, Q.; Jiang, Y.; Hou, L.; Yang, H.; Qi, Q. Development and Application of a New Open-Source Integrated Surface–Subsurface Flow Model in Plain Farmland. Water 2024, 16, 1528. https://doi.org/10.3390/w16111528
Yang H, Zhou Q, Jiang Y, Hou L, Yang H, Qi Q. Development and Application of a New Open-Source Integrated Surface–Subsurface Flow Model in Plain Farmland. Water. 2024; 16(11):1528. https://doi.org/10.3390/w16111528
Chicago/Turabian StyleYang, Hai, Quanping Zhou, Yuehua Jiang, Lili Hou, Hui Yang, and Qiuju Qi. 2024. "Development and Application of a New Open-Source Integrated Surface–Subsurface Flow Model in Plain Farmland" Water 16, no. 11: 1528. https://doi.org/10.3390/w16111528
APA StyleYang, H., Zhou, Q., Jiang, Y., Hou, L., Yang, H., & Qi, Q. (2024). Development and Application of a New Open-Source Integrated Surface–Subsurface Flow Model in Plain Farmland. Water, 16(11), 1528. https://doi.org/10.3390/w16111528








