Optimization of Elbow Draft Tubes for Variable Speed Propeller Turbine
Abstract
1. Introduction
2. Paper Overview
- An approach to the shape parameterisation of the elbow draft tube—changing the cross-section area along the centreline.
- EDT optimisation for the variable speed propeller turbine.
- Relationships between pressure regeneration efficiency and flow inhomogeneity.
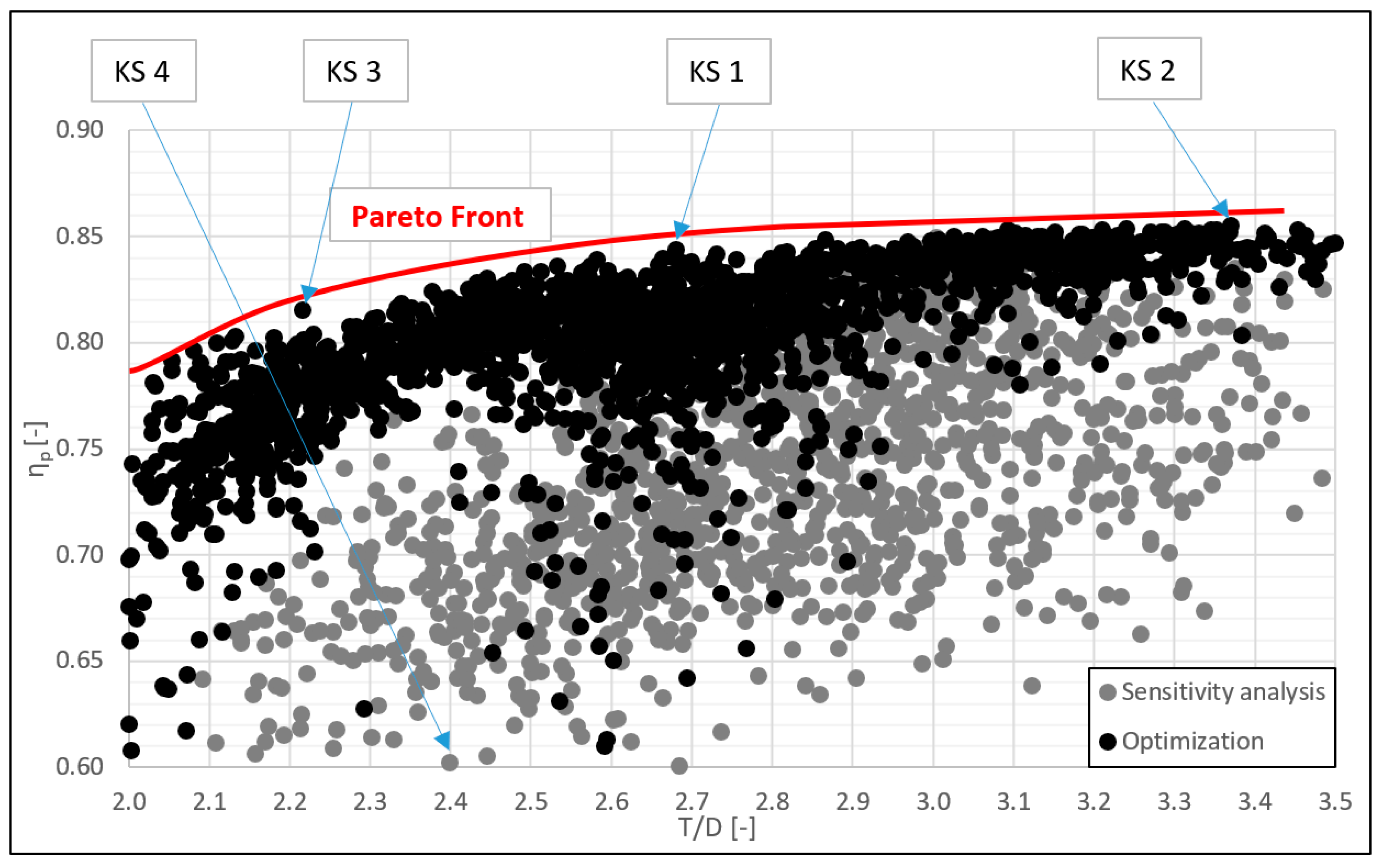
- The resulting set of candidates (Pareto front) with the highest pressure regeneration efficiency and different elbow draft tube heights.
- Performance comparison of the turbine with different elbow draft tubes and with straight draft tube.
3. Variable Speed Propeller Specifics
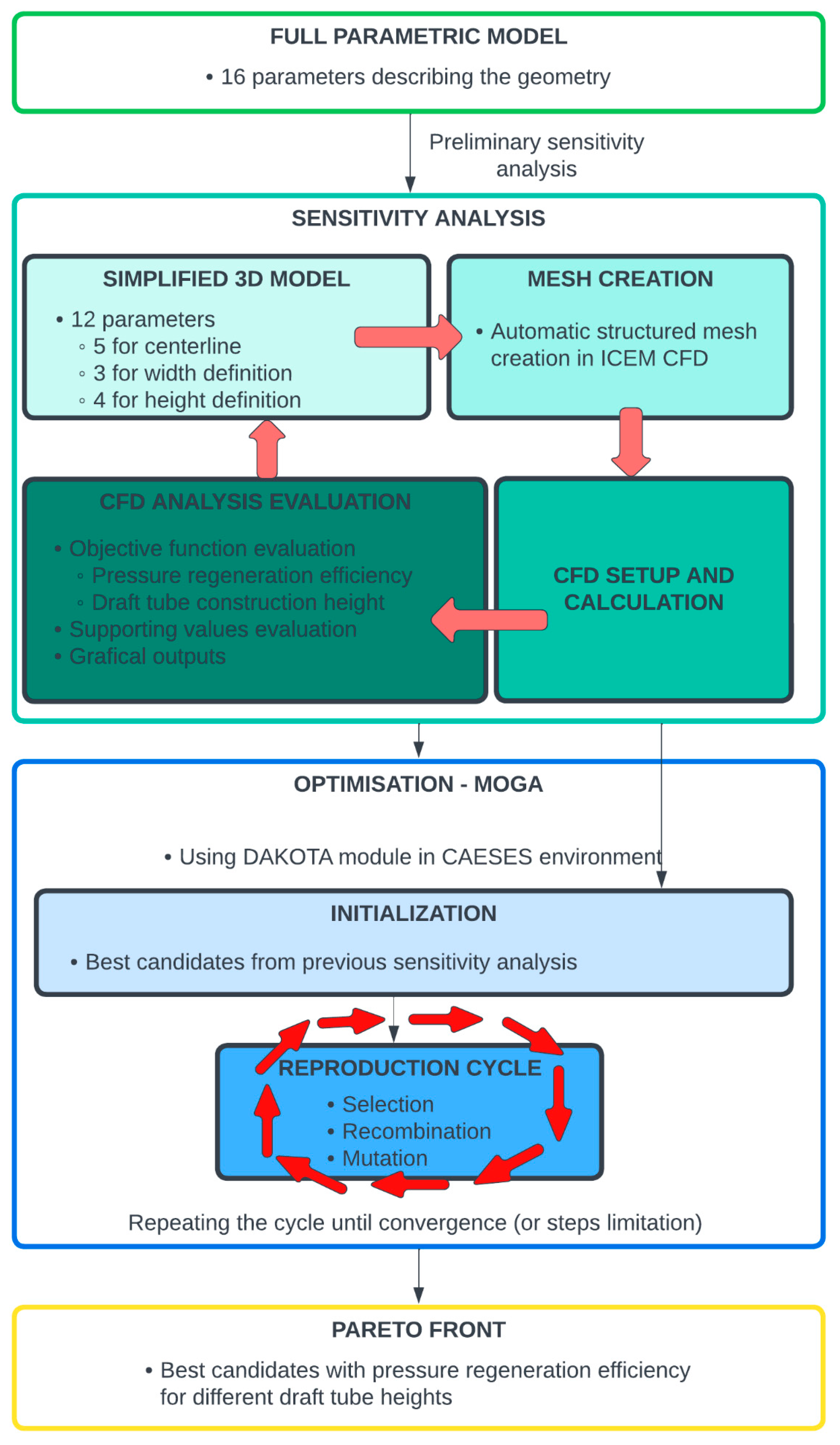
4. Optimisation Process
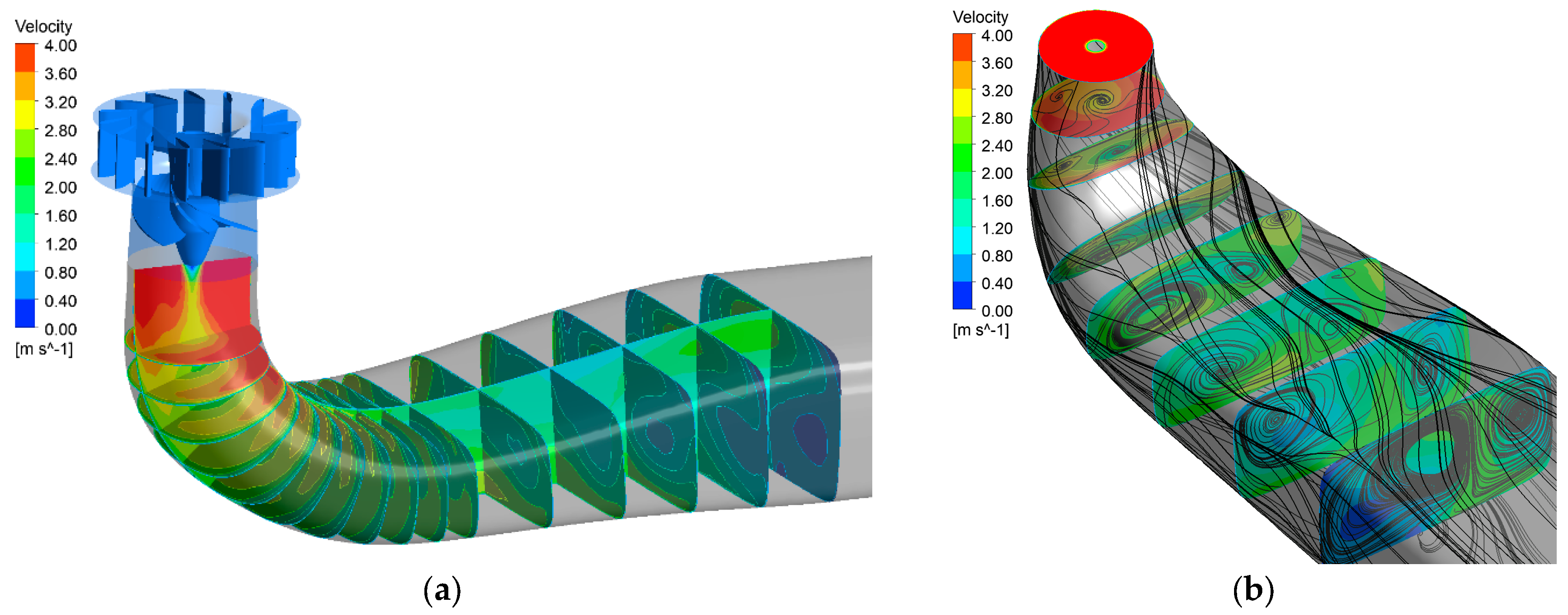
4.1. Methodology for Draft Tube Assesment
4.2. Geometry Shape Parametrisation
- The fixed inlet profile of EDT (outlet profile of runner chamber)
- The fixed shape of the outlet profile (rectangle with rounded corners; rectangle aspect ratio is 2:1). The position of the outlet profile can change.
- The shape of EDT is defined by the centreline. The height and width change along the length of the centreline.
- The symmetry of EDT—centreline lies in the vertical plane.
4.3. Grid Scaling Test
4.4. Numerical Setup
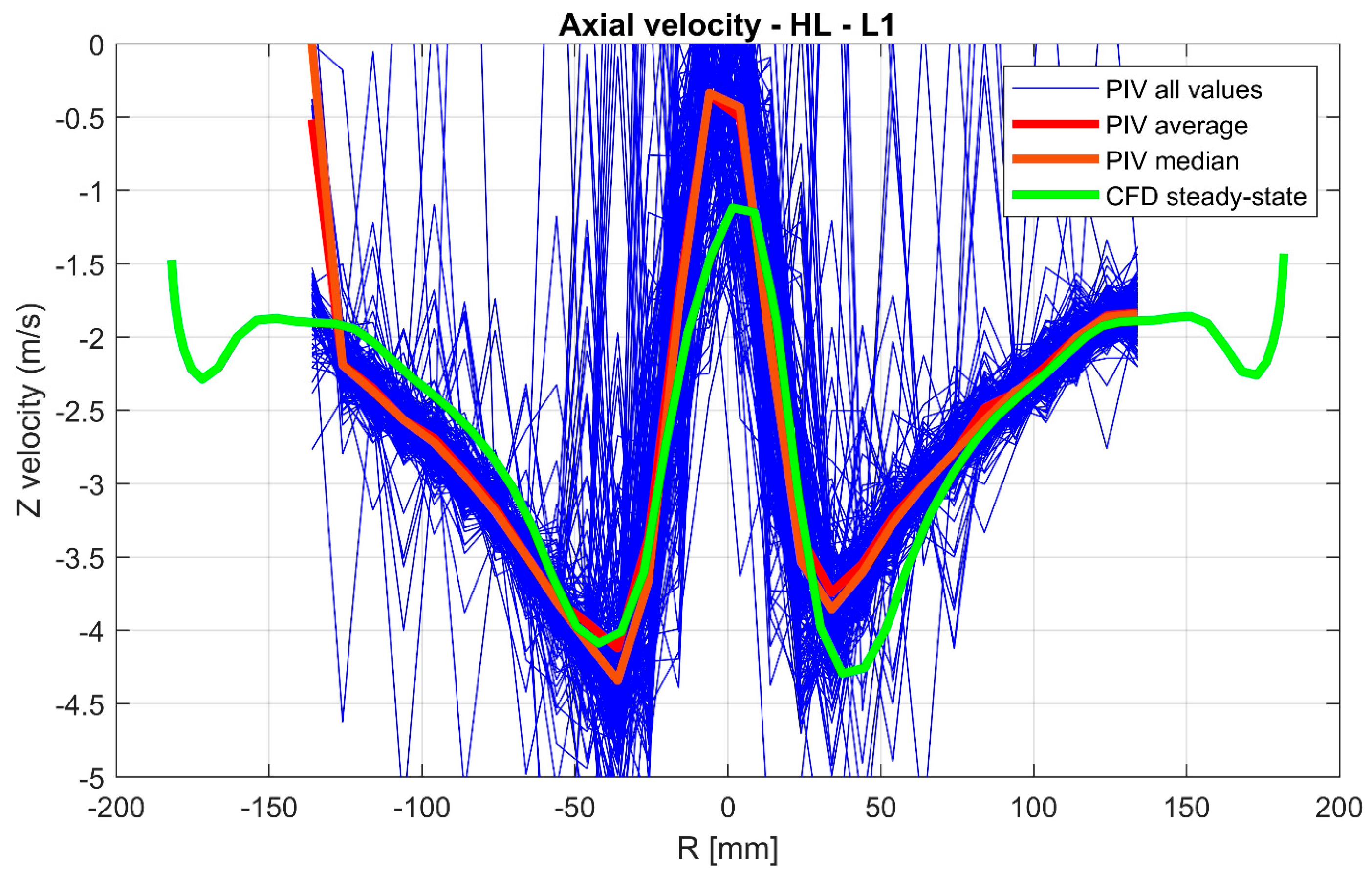
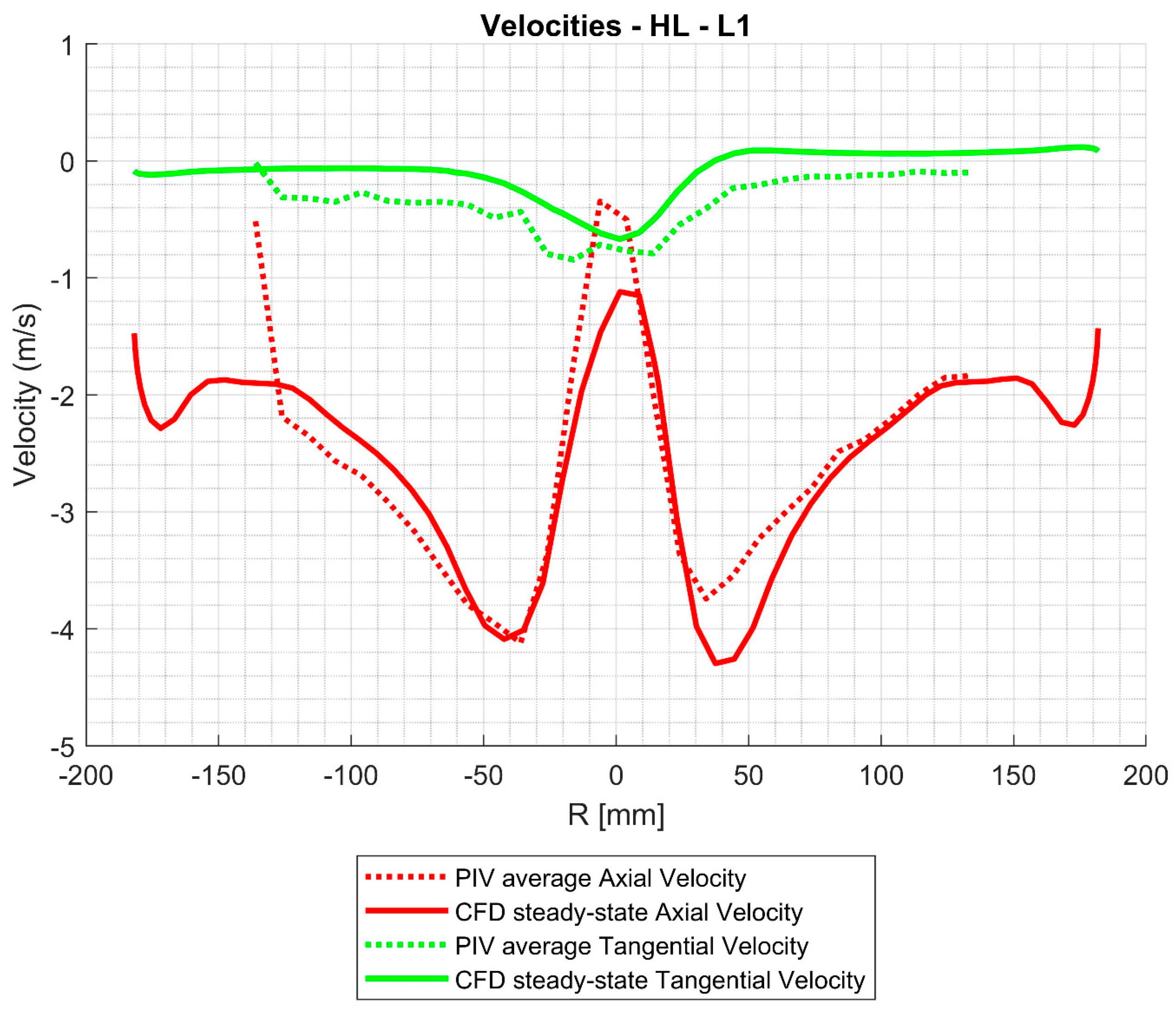
4.5. Validation of Numerical Model
4.6. Sensitivity Analysis and Optimisation
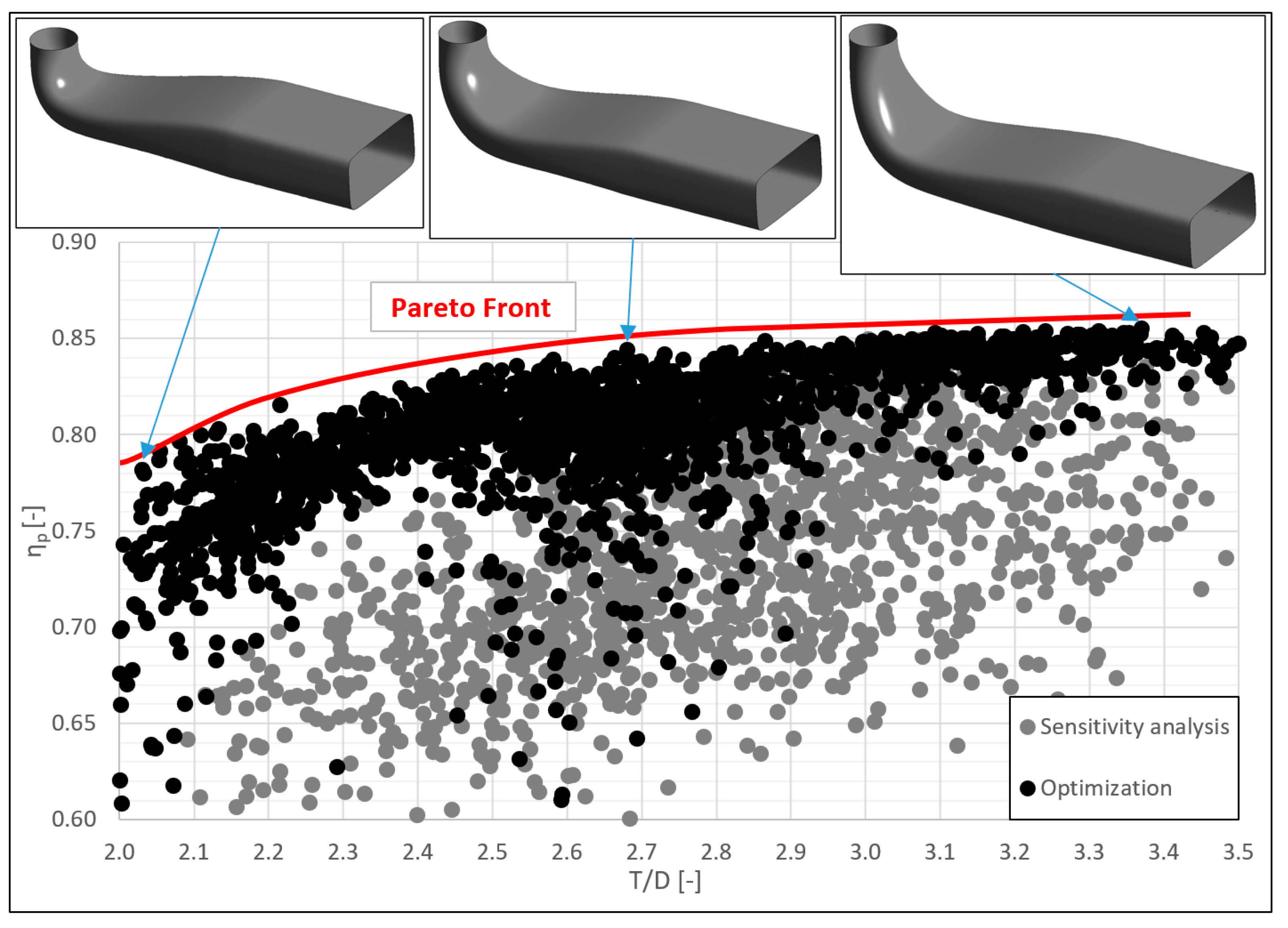
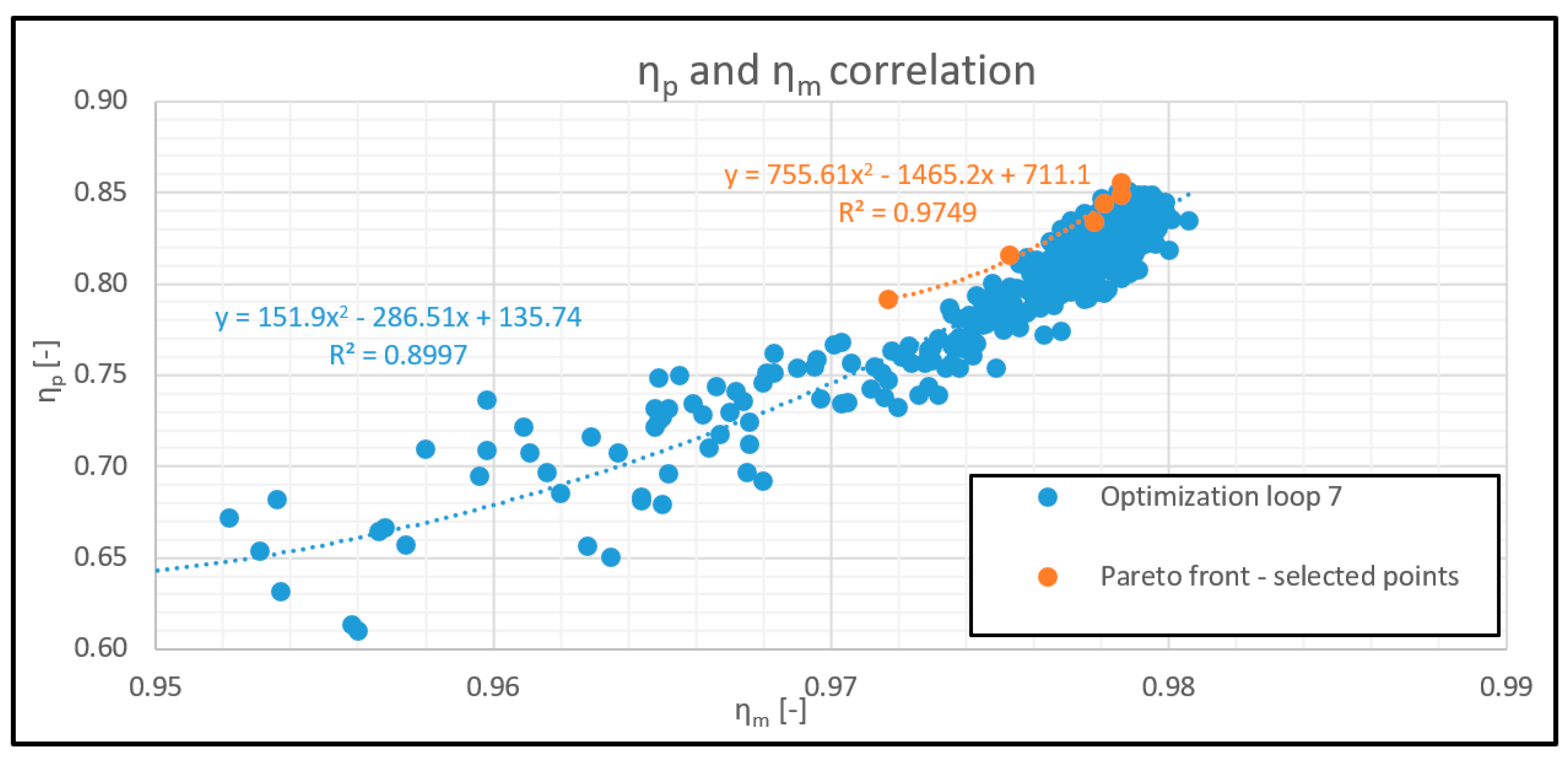
5. Optimisation Results
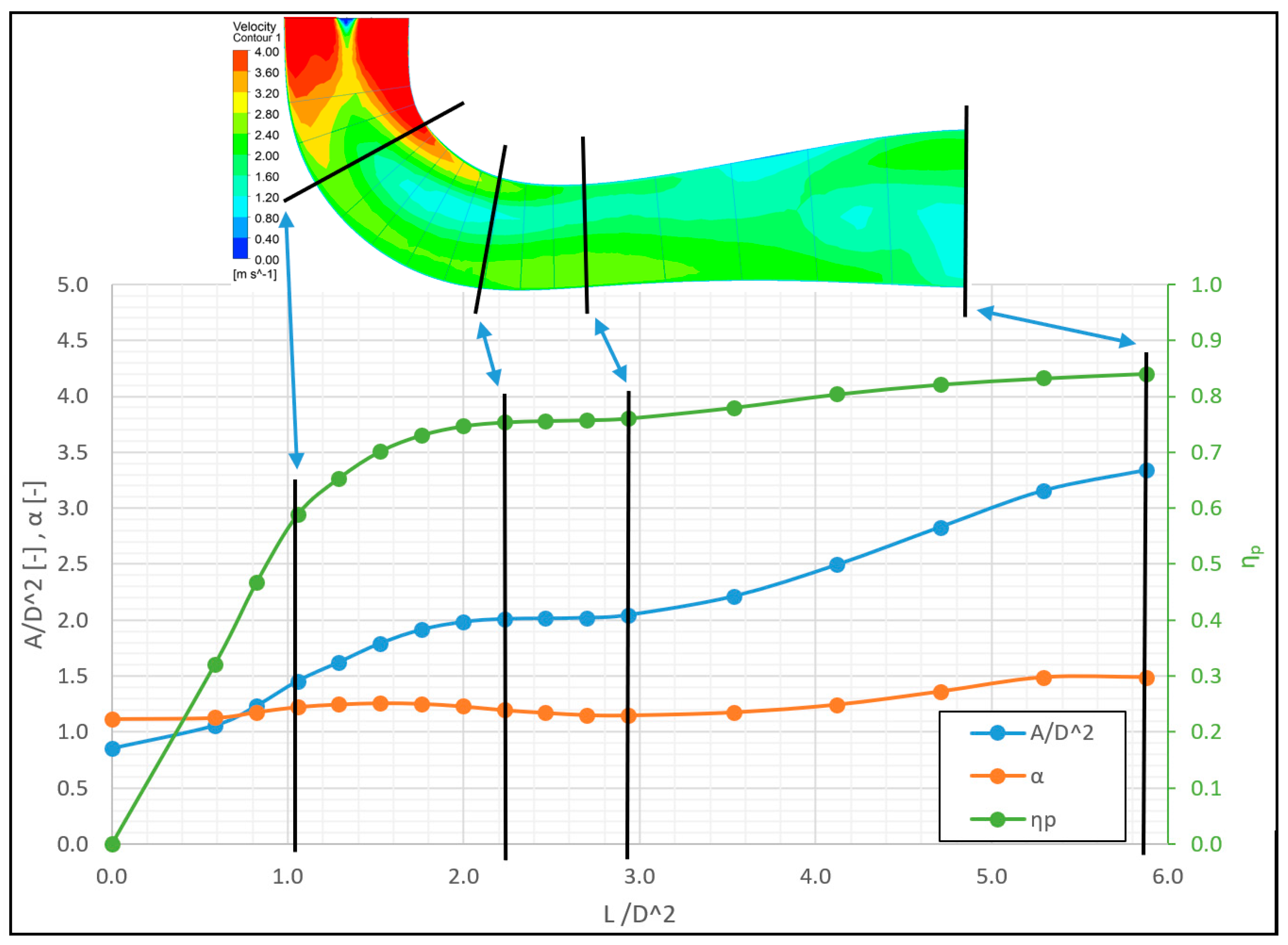
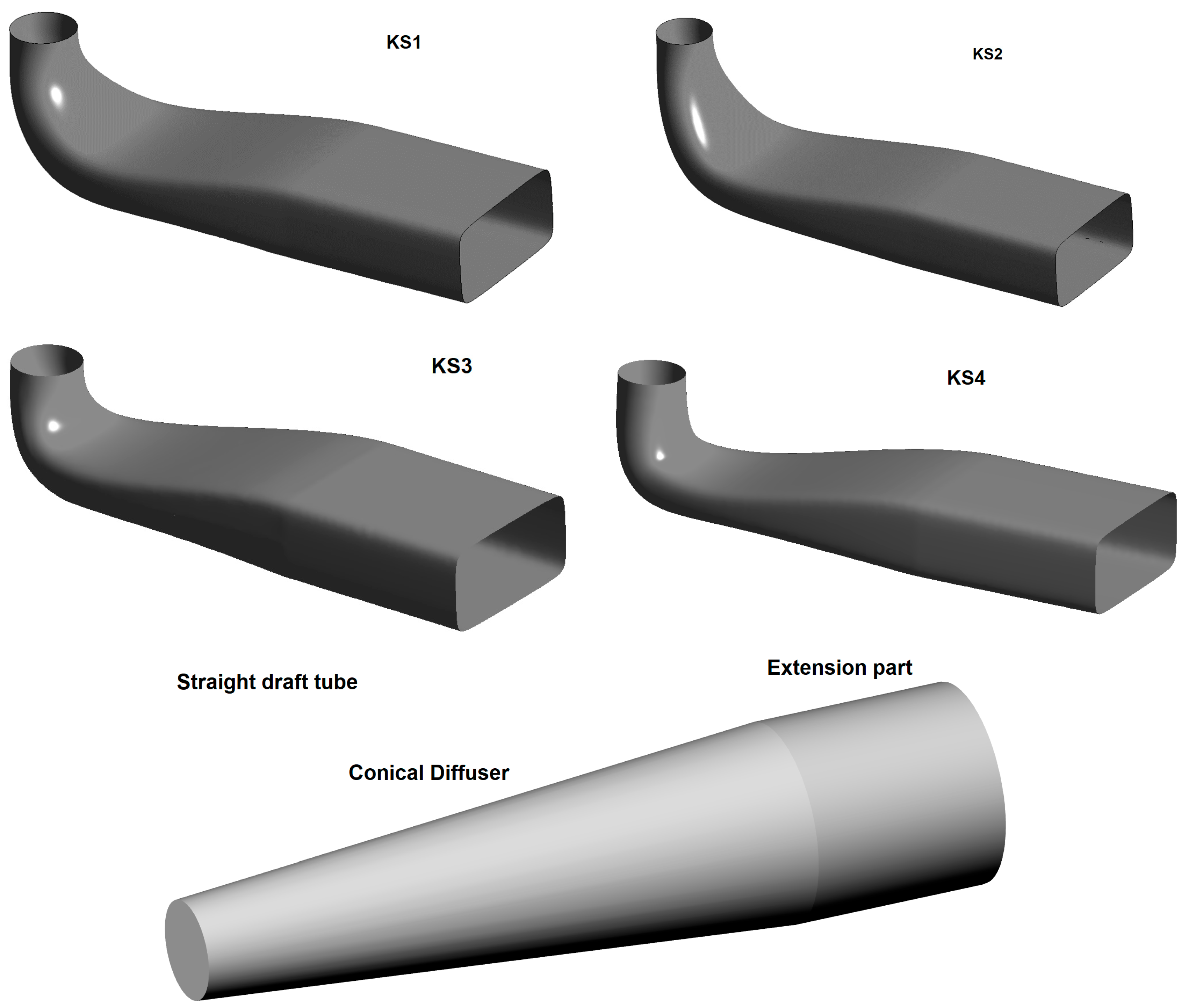
6. Characteristics of Chosen Candidate
7. Comparison of Different Draft Tubes
- The area of the output profile of the SDT is identical to KS1.
- The length of the centreline of the SDT is identical to that of the KS1 which results in a half angle θ equal to 4.55°.
- Computational grid is as similar as possible (number of elements and overall characteristics of the grid).
- CFD calculation settings are identical.
- After checking the convergence of CFD calculations, export the numerical results of the quantities of interest.
- Processing variables in MATLAB [34].
- Surface fitting of data points with the aim of achieving the highest possible values of the coefficient of determination R2. The polynomial fitting method (poly44) was used to fit the surfaces. This will give us 3D surfaces (and contours in 2D) for a more comprehensive display of the machine’s characteristics.
- Creating the ideal coupling of our variable speed turbine—maximum efficiency for individual unit discharges Q11.
- Display or compare the variables of interest in 3D (surfaces), the same in a 2D plan view (contours), and mutually compare the progress for the actual operation of turbines with variable speeds in ideal connections.
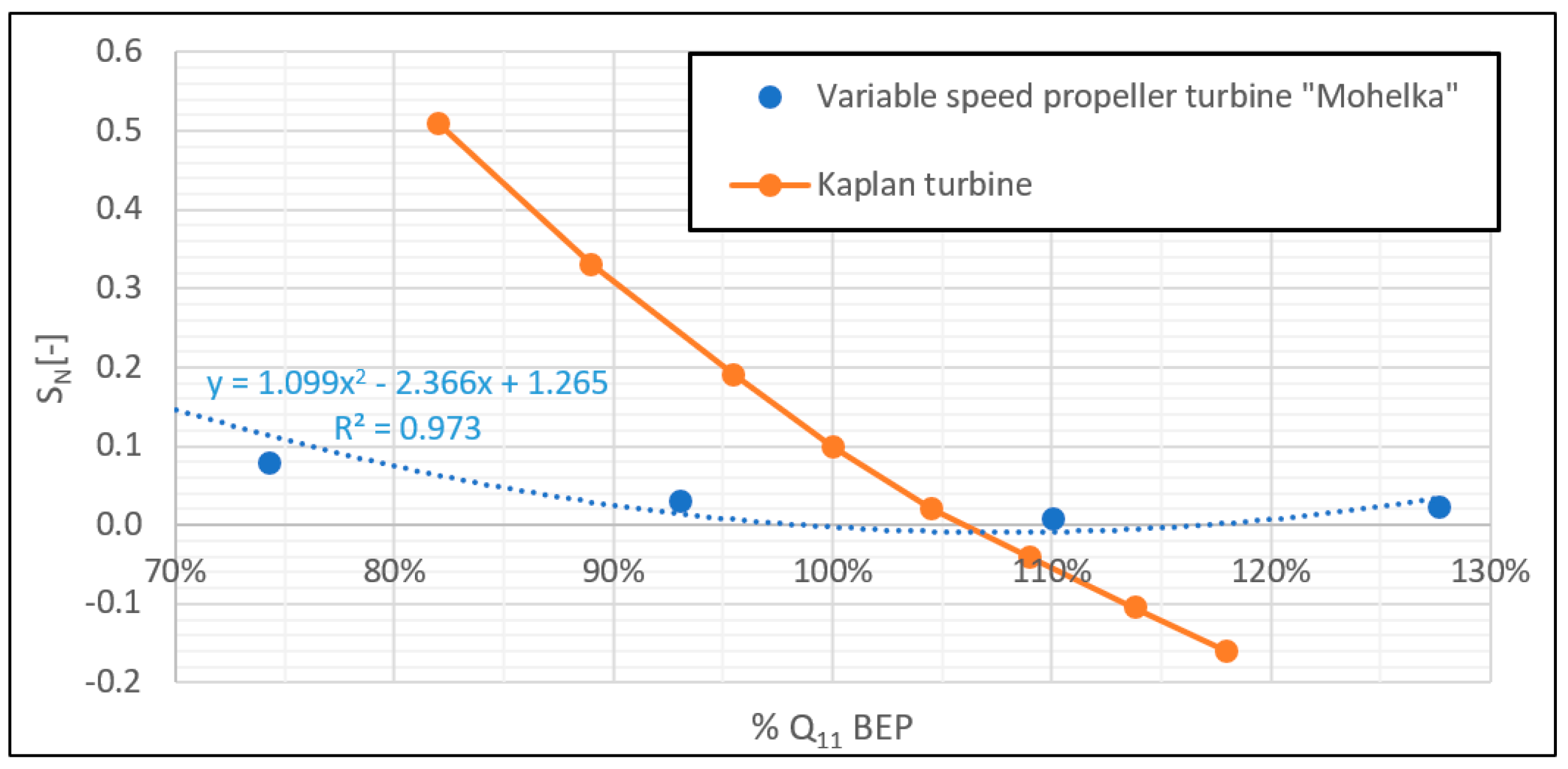
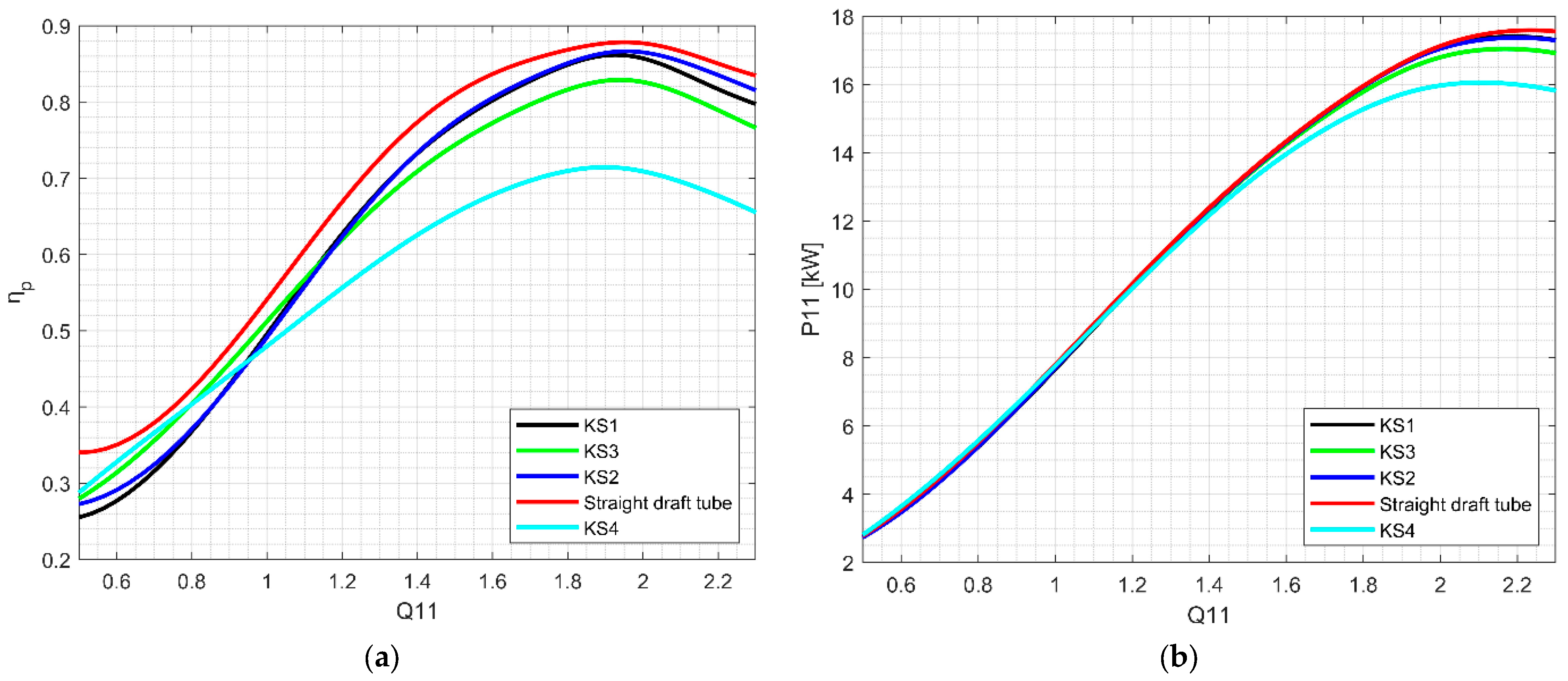
- For large flow rates (Q11 > 2 m3.s−1), the highest draft tube (KS2) has the highest efficiency, followed by the medium height draft tube (KS1), then the elbow draft tube with low height (KS3), and finally the inappropriately shaped draft tube (KS4).
- Surprisingly, for low flow rates (approx. Q11 < 1 m3.s−1), the situation is quite different, and draft tubes with a larger height paradoxically have a lower efficiency of pressure regeneration ηp than elbow draft tubes with a lower height (and even inappropriately shaped elbow draft tube KS4). We believe that the optimisation for one selected point can cause this situation (Q11 approx. 2.0 m3.s−1), and that the numerical model in general is not able to describe the inappropriate flow in these draft tube (especially KS3 and KS4) well enough.
8. Conclusions and Future Work
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| Acronyms: | |
| BEP | Best efficiency point |
| CFD | Computational Fluid Dynamics |
| CNC | Computer numerical control |
| DES | Detached Eddy Simulation turbulence model |
| DT | Draft tube |
| EDT | Elbow draft tube |
| GCI | Grid convergence index |
| LES | Large Eddy simulation turbulence model |
| MOGA | Multi-objective genetic algorithms |
| PIV | Particle image velocimetry |
| RANS | Reynolds-averaged Navier-Stokes equation |
| RMS | Root-mean squared |
| SAS | Scale-Adaptive Simulation turbulence model |
| SDT | Straight draft tube |
| Symbols: | |
| area-average dynamic pressure [Pa] | |
| area-average static pressure [Pa] | |
| area-average total pressure (overall energy) [Pa] | |
| A | cross section [m2] |
| cp | pressure recovery factor [-] or [%] |
| D | runner diameter [m] |
| g | gravitational acceleration [m.s−2] |
| H | net head [m] |
| k | turbulent kinetic energy [m2.s−2] |
| n11 | unit runner speed |
| P | turbine power output [kW] |
| Q | volumetric discharge [m3.s−1] |
| Q11 | unit discharge |
| Qmax | maximum turbine discharge [m3.s−1] |
| r | radius [m] |
| R | inlet profile radius [m] |
| Sn | swirl number [-] |
| T/D | ratio of the runner diameter to EDT construction height [-] |
| u | normal component of the point velocity [m.s−1] |
| v | absolute velocity [m.s−1] |
| vax | axial velocity [m.s−1] |
| vtan | tangential velocity [m.s−1] |
| y+ | dimensionless wall distance [-] |
| α | Coriolis number [-] |
| β | kinetic head ratio [-] or [%] |
| ε | turbulence eddy dissipation [m2.s−3] |
| ηm | draft tube hydraulic efficiency [-] or [%] |
| ηp | pressure regeneration efficiency [-] or [%] |
| θ | half wall angle [°] |
| ρ | water density [kg.m−3] |
References
- Ko, P.; Matsumoto, K.; Ohtake, N.; Ding, H. Design of a Kaplan turbine for a wide range of operating head—Curved draft tube design and model test verification. IOP Conf. Ser. Earth Environ. Sci. 2016, 49, 10. [Google Scholar] [CrossRef]
- Lyutov, A. Coupled Multipoint Shape Optimization of Runner and Draft Tube of Hydraulic Turbines. J. Fluids Eng. 2015, 137, 111302. [Google Scholar]
- Bílková, E.; Souček, J.; Kantor, M.; Kubíček, R.; Nowak, P. Variable-Speed Propeller Turbine for Small Hydropower Applications. Energies 2023, 16, 3811. [Google Scholar] [CrossRef]
- Kantor, M.; Chalupa, M.; Souček, J.; Bílkova, E.; Nowak, P. Application of genetic algorithm methods for water turbine blade shape optimization. Manuf. Technol. 2020, 20, 453–458. [Google Scholar] [CrossRef]
- Wilhelm, S.; Balarac, G.; Métais, O.; Ségoufin, C. Analysis of Head Losses in a Turbine Draft Tube by Means of 3D Unsteady Simulations. Flow Turbul. Combust. 2016, 97, 1255–1280. [Google Scholar] [CrossRef]
- Mosonyi, E. Low-Head Power Plants, 3rd ed.; Akadémiai Kiadó: Budapest, Hungary, 1987; ISBN 963054271. [Google Scholar]
- Schiffer, J.; Benigni, H.; Jaberg, H. An analysis of the impact of draft tube modifications on the performance of a Kaplan turbine by means of computational fluid dynamics. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2018, 2018, 1937–1952. [Google Scholar] [CrossRef]
- Pasche, S.; Avellan, F.; Gallaire, F. Part Load Vortex Rope as a Global Unstable Mode. J. Fluids Eng. 2017, 139, 11. [Google Scholar] [CrossRef]
- Daniels, S.; Rahat, A. Shape optimisation of the sharp-heeled Kaplan draft tube: Performance evaluation using Computotaional Fluid Dynamics. Renew. Energy 2020, 160, 112–116. [Google Scholar] [CrossRef]
- Favrel, A.; Lee, N.; Irie, T.; Miyagawa, K. Design of Experiments Applied to Francis Turbine Draft Tube to Minimize Pressure Pulsations and Energy Losses in Off-Design Conditions. Energies 2021, 14, 3894. [Google Scholar] [CrossRef]
- Lučin, I.; Sikirica, A.; Šiško Kuliš, M.; Čarija, Z. Investigation of Efficient Optimization Approach to the Modernization of Francis Turbine Draft Tube Geometry. Mathematics 2022, 10, 4050. [Google Scholar] [CrossRef]
- Orso, R.; Benini, E.; Minozzo, M.; Bergamin, R.; Magrini, A. Two-Objective Optimization of a Kaplan Turbine Draft Tube Using a Response Surface Methodology. Energies 2020, 13, 4899. [Google Scholar] [CrossRef]
- Nam, M.; Dechun, B.; Xiangji, Y.; Mingri, J. Design optimization of hydraulic turbine draft tube based on CFD and DOE method. IOP Conf. Ser. Earth Environ. Sci. 2018, 136, 9. [Google Scholar] [CrossRef]
- McNabb, J.; Devals, C.; Kyriacou, S.; Murry, N.; Mullins, B. CFD based draft tube hydraulic design optimization. IOP Conf. Ser. Earth Environ. Sci. 2014, 22, 012023. [Google Scholar] [CrossRef]
- Ciocan, T.; Susan-Resiga, R.; Muntean, S. Improving Draft Tube Hydrodynamics over a Wide Operating Range. Proc. Rom. Acad. 2014, 15, 9. [Google Scholar]
- Štefan, D.; Rudolf, P.; Skoták, A.; Motyčák, L. Energy transformation and flow topology in an elbow draft tube. Appl. Comput. Mech. 2012, 6, 14. [Google Scholar]
- Marjavaara, D. CFD Driven Optimization of Hydraulic Turbine Draft Tubes Using Surrogate Models. Ph.D. Thesis, Luleå Tekniska Universitet, Luleå, Sweden, 2006. [Google Scholar]
- Demirel, G.; Acar, E.; Celebioglu, K.; Aradag, S. CFD-driven surrogate-based multi-objective shape optimization of an elbow type draft tube. Int. J. Hydrogen Energy 2017, 42, 17601–17610. [Google Scholar] [CrossRef]
- Sikirica, A.; Lučin, I.; Alvir, M.; Kranjčević, L.; Čarija, Z. Computationally efficient optimisation of elbow-type draft tube using neural network surrogates. Alex. Eng. J. 2024, 90, 129–152. [Google Scholar] [CrossRef]
- Susan-Resiga, R.; Dan Ciocan, G.; Anton, I.; Avellan, F. Analysis of the Swirling Flow Downstream a Francis Turbine Runner. J. Fluids Eng. 2006, 128, 177–189. [Google Scholar] [CrossRef]
- Dauhlhaug, O. A Study of Swirl Flow in Draft Tubes. Master’s Thesis, Norwegian University of Science and Technology, Trondheim, Norway, 1997. [Google Scholar]
- IEC 62006:2010; Hydraulic Machines—Acceptance Tests of Small Hydroelectric Installation. IEC: Geneva, Switzerland, 2010.
- De Siervo, F.; de Leva, F. Modern trends in selecting and designing Kaplan turbines. Int. Water Power Dam Constr. 1978, 30, 52–58. [Google Scholar]
- Gubin, M. Draft Tubes of Hydro-Electric Station; Translated from Russian; Amerind Publishing Co.: New Delhi, India, 1973; ISBN 621.244-225.14. [Google Scholar]
- CAESES Software. Available online: https://www.caeses.com/ (accessed on 10 August 2023).
- ANSYS Software. Available online: https://www.ansys.com/ (accessed on 10 August 2023).
- Devals, C.; Vu, T.; Zhang, Y.; Dompierre, J.; Guibault, F. Mesh convergence study for hydraulic turbine draft-tube. IOP Conf. Ser. Earth Environ. Sci. 2016, 49, 082021. [Google Scholar] [CrossRef]
- Celik, I.B.; Ghia, U.; Roache, P.J.; Freitas, C.J. Procedure for Estimation and Reporting of Uncertainty Due to Discretization in CFD Applications. J. Fluids Eng. 2008, 130, 4. [Google Scholar] [CrossRef]
- Menter, F. Two-equation eddy-viscosity turbulence models for engineering applications. AIAA J. 1994, 32, 1598–1605. [Google Scholar] [CrossRef]
- Fleischli, B.; Del Rio, A.; Casartelli, E.; Mangani, L.; Mullins, B.; Devals, C.; Melot, M. Application of a General Discrete Adjoint Method for Draft Tube Optimization. IOP Conf. Ser. Earth Environ. Sci. 2021, 774, 11. [Google Scholar] [CrossRef]
- Trivedi, C.; Cervantes, M.; Gandhi, B.; Dahlhaug, O. Experimental and Numerical Studies for a High Head Francis Turbine at Several Operating Points. J. Fluids Eng. 2013, 135, 17. [Google Scholar] [CrossRef]
- Francis-99: Second Workshop; Norwegian Hydropower Centre: Trondheim, Norway, 2016.
- Nakamura, K.; Kurosawa, S. Design Optimization of a High Specific Speed Francis Turbine Using Multi-Objective Genetic Algorithm. Int. J. Fluid Mach. Syst. 2009, 2, 102–109. [Google Scholar] [CrossRef]
- MATLAB Software. Available online: https://www.mathworks.com/ (accessed on 10 August 2023).

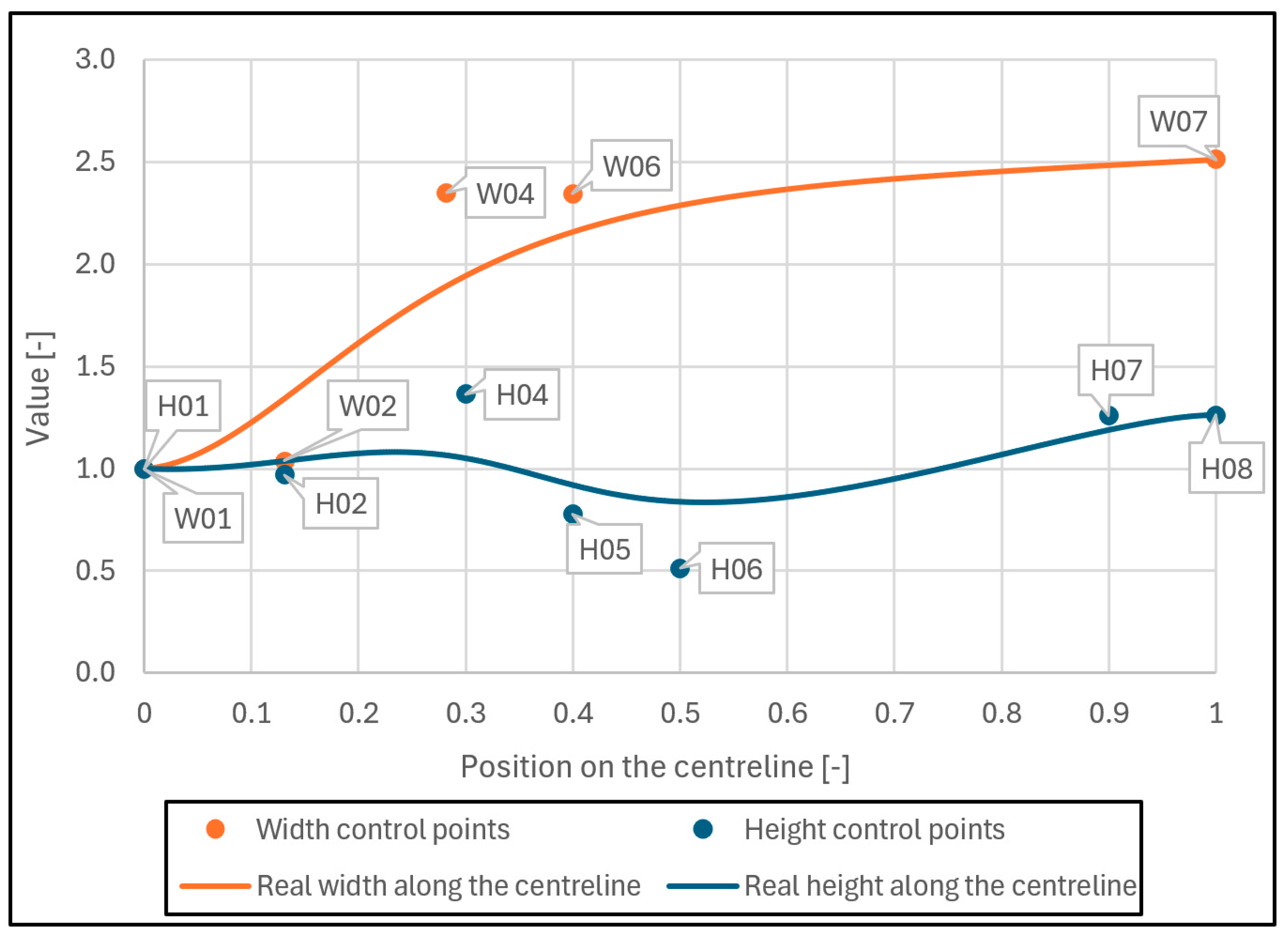



| Table of Geometry Parameters | Height Definition | Width Definition | Centreline Definition | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| H02 | H04 | H05 | H06 | W02 | W04 | W06 | P2_Z | P3_Z | P4_4 | P5_Z | P5_X | |
| [m] | [m] | [m] | [m] | [m] | [m] | [m] | [m] | [m] | [m] | [m] | [m] | |
| Minimum | 0.900 | 0.601 | 0.500 | 0.300 | 0.900 | 2.000 | 2.200 | 0.050 | −0.350 | −0.400 | −0.479 | −1.000 |
| Maximum | 1.100 | 1.499 | 0.900 | 0.750 | 1.100 | 2.500 | 2.650 | 0.469 | 0.200 | 0.550 | −0.051 | −1.999 |
| Grid no.1 | N1 (mil.) | 5.15 |
| Grid no.2 | N2 (mil.) | 1.07 |
| Grid no.3 | N3 (mil.) | 0.47 |
| Grid refinement factor 12 | r21 | 1.69 |
| Grid refinement factor 23 | r32 | 1.32 |
| ηp grid no.1 | ηp1 | 80.85% |
| ηp grid no.2 | ηp2 | 81.92% |
| ηp grid no.3 | ηp3 | 83.07% |
| Apparent order | p | 1.854 |
| Extrapolated value | η21p ext (-) | 80.20% |
| Approximate relative error | ea21 | 0.0133 |
| Extrapolated relative error | eext21 | 0.0080 |
| Grid convergence index grid 1 and 2 | GCI21 fine | 0.0101 |
| Grid convergence index grid 2 and 3 | GCI32 medium | 0.0269 |
| PL | BEP | HL | |
|---|---|---|---|
| [%] | [%] | [%] | |
| Francis 99 | 90.1 | 92.4 | 91.7 |
| Our CFD sim. | 93.6 | 94.2 | 93.0 |
| Difference | 3.5 | 1.8 | 1.3 |
| cp | ηp | α | T/D |
|---|---|---|---|
| [%] | [%] | [-] | [-] |
| 94.0 | 84.1 | 1.49 | 2.68 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Souček, J.; Nowak, P. Optimization of Elbow Draft Tubes for Variable Speed Propeller Turbine. Water 2024, 16, 1457. https://doi.org/10.3390/w16101457
Souček J, Nowak P. Optimization of Elbow Draft Tubes for Variable Speed Propeller Turbine. Water. 2024; 16(10):1457. https://doi.org/10.3390/w16101457
Chicago/Turabian StyleSouček, Jiří, and Petr Nowak. 2024. "Optimization of Elbow Draft Tubes for Variable Speed Propeller Turbine" Water 16, no. 10: 1457. https://doi.org/10.3390/w16101457
APA StyleSouček, J., & Nowak, P. (2024). Optimization of Elbow Draft Tubes for Variable Speed Propeller Turbine. Water, 16(10), 1457. https://doi.org/10.3390/w16101457






