Numerical Simulation of Cavitation Bubble Collapse inside an Inclined V-Shape Corner by Thermal Lattice Boltzmann Method
Abstract
:1. Introduction
2. Numerical Methods
2.1. Pseudo-Potential MRT-LBM Model
2.2. Thermal MRT-LBM Model
3. Collapse of a Single Cavitation Bubble inside an Inclined V-Shaped Corner
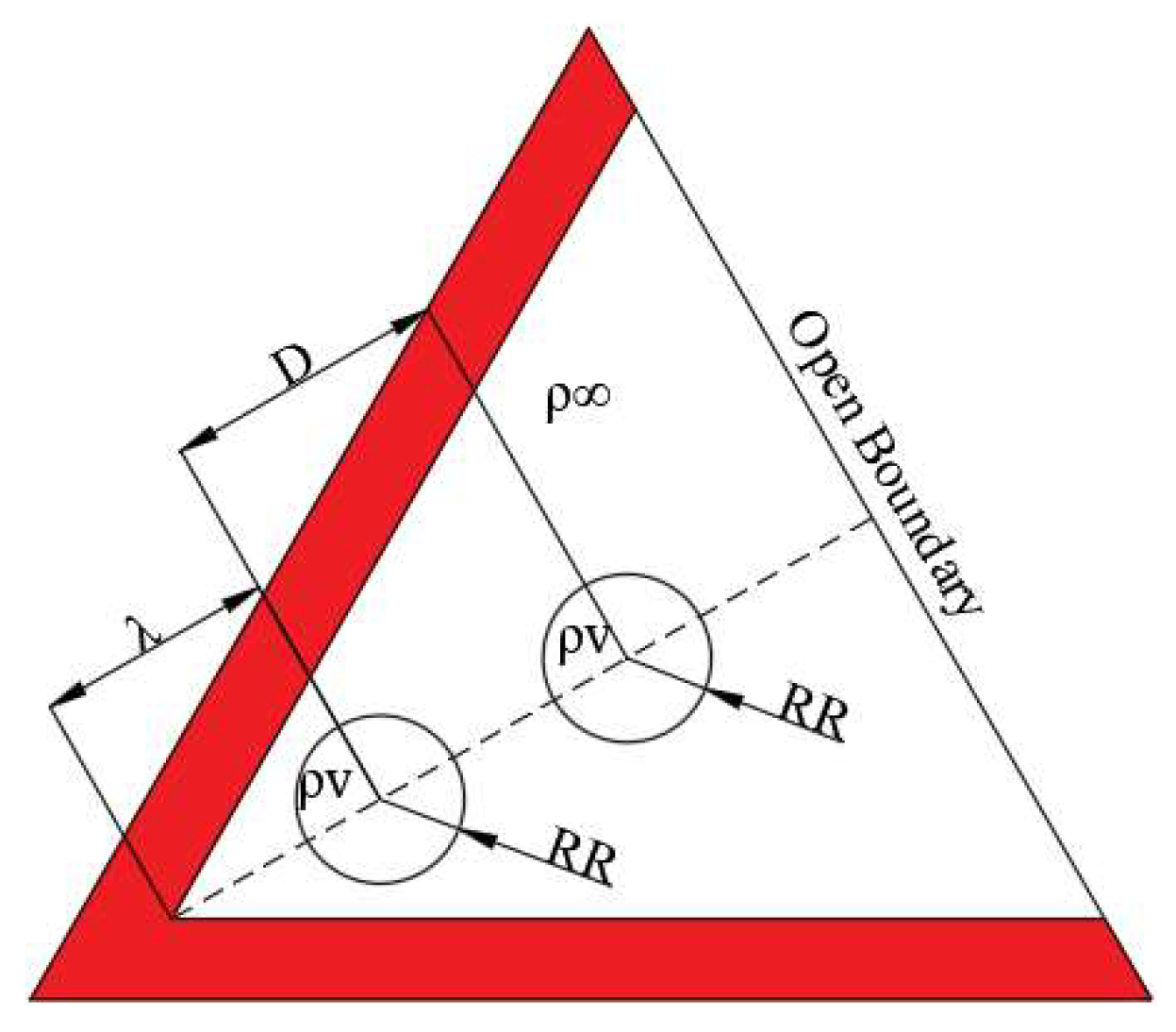
3.1. Numerical Model
3.2. Collapse of Single Bubble inside an Inclined V-Shaped Corner with Different λ
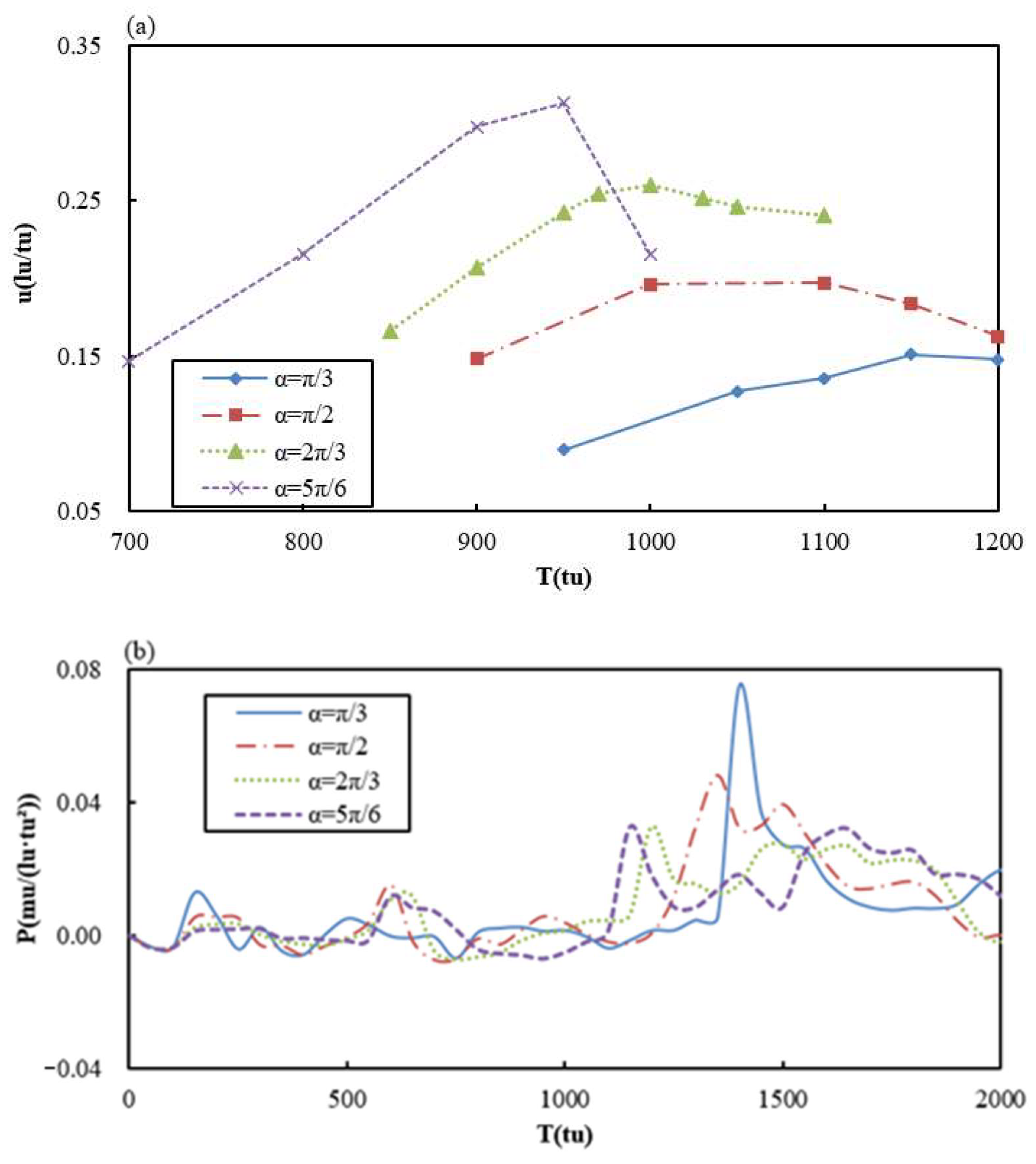
3.3. Collapse of Single Bubble inside an Inclined V-Shaped Wall with Different α
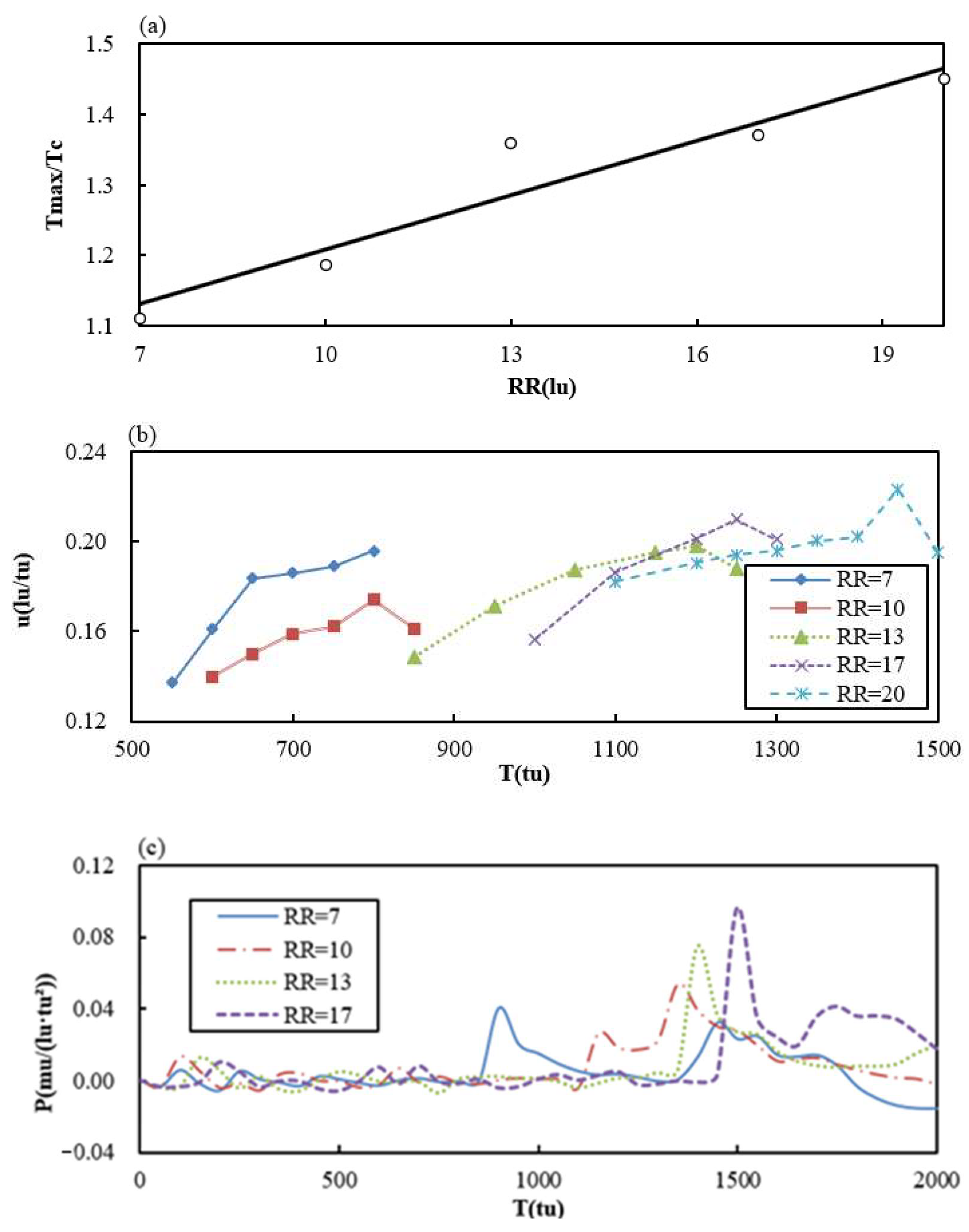
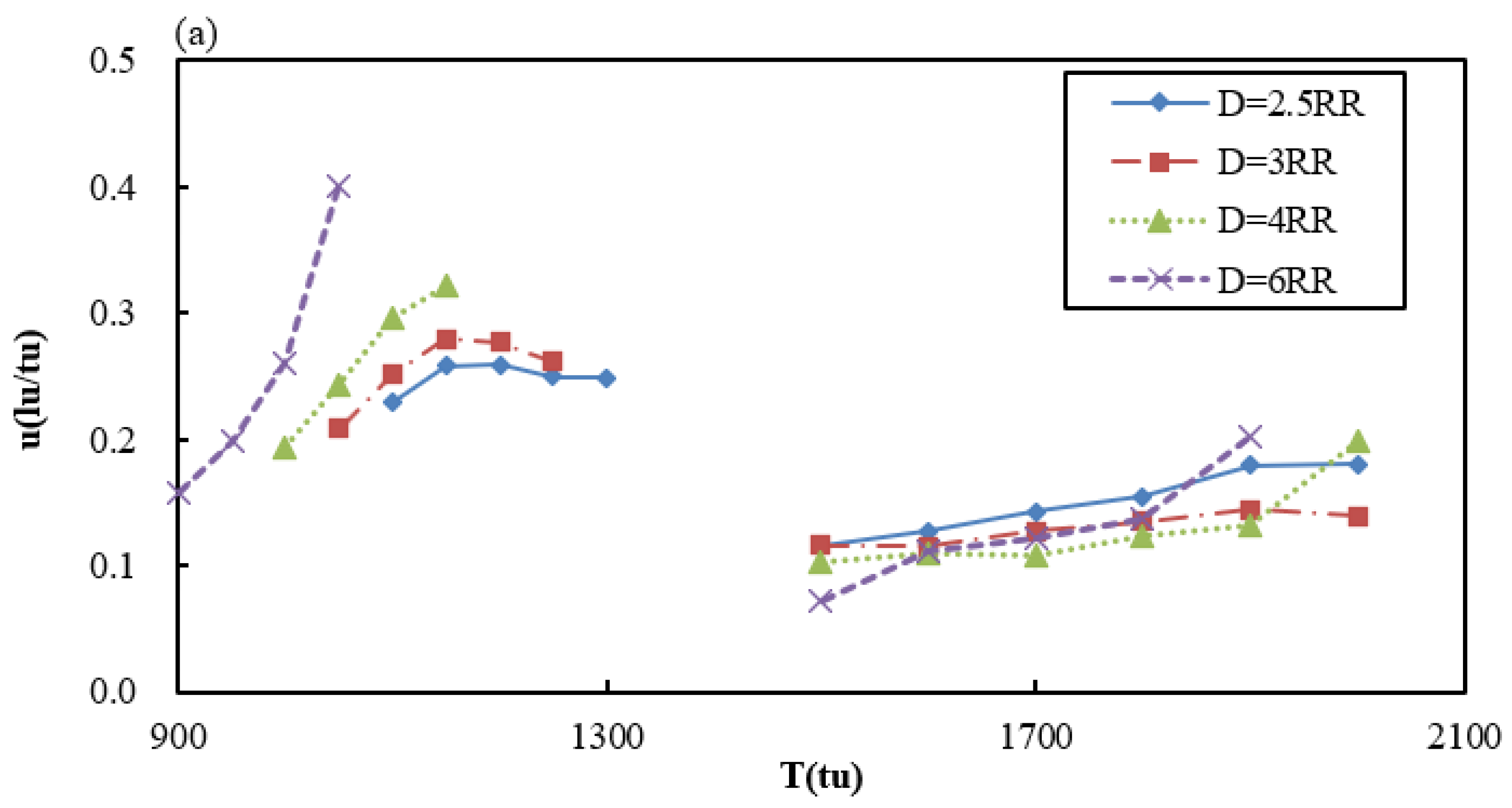
3.4. Collapse of Single Bubble inside an Inclined V-Shaped Wall with Different RR
4. Collapse of Double Cavitation Bubbles inside an Inclined V-Shaped Wall
4.1. Computational Layout
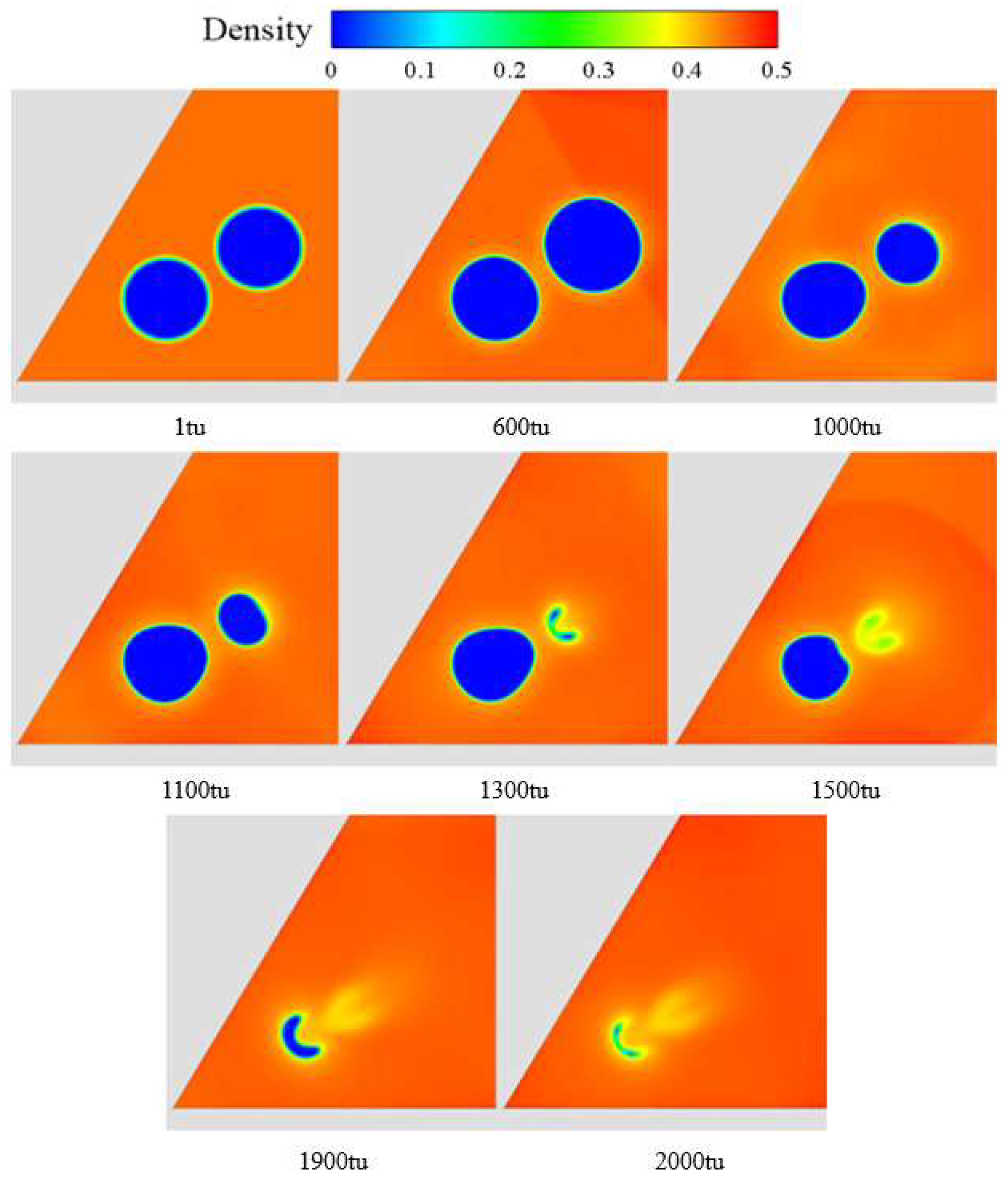
4.2. Numerical Simulation of Double Bubble Collapse inside an Inclined V-Shaped Wall
5. Conclusions
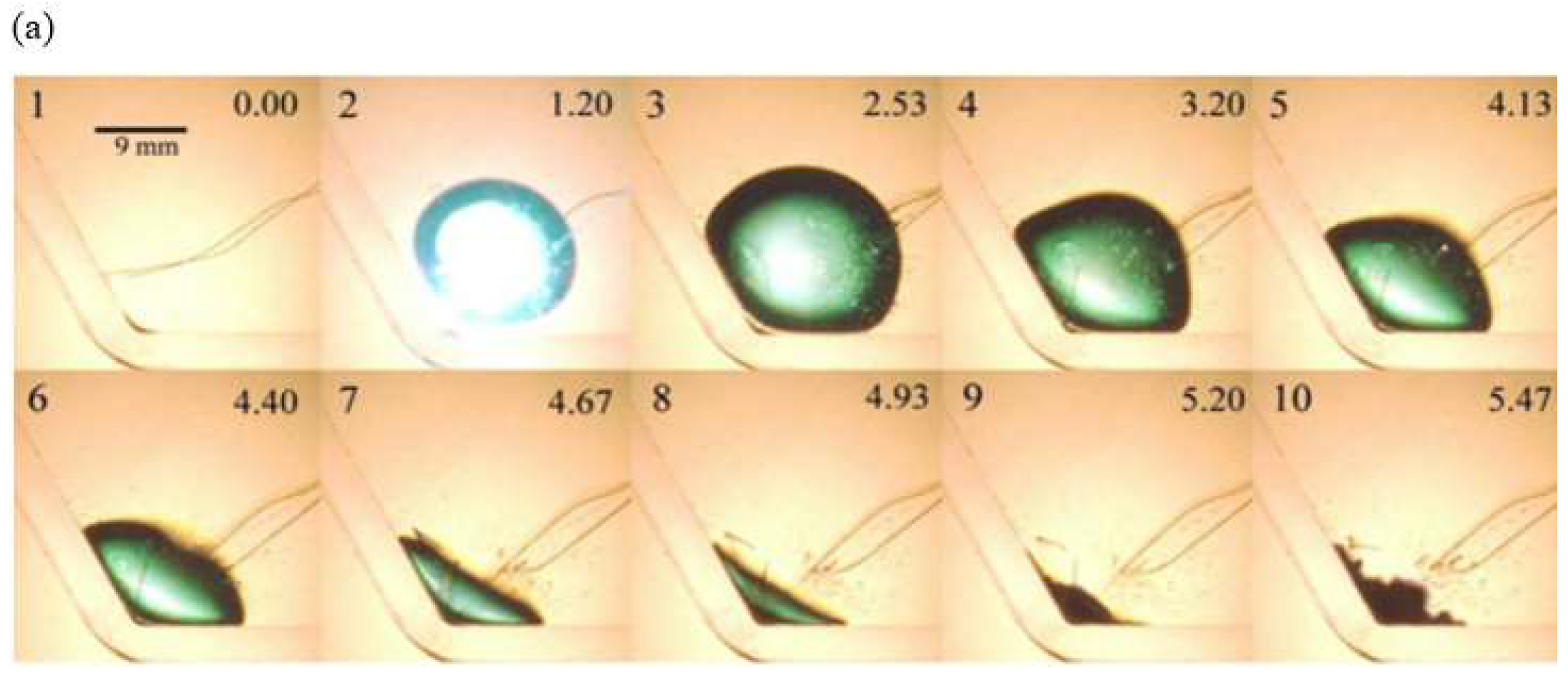
- The simulated density fields during the collapse process of a single cavitation bubble are compared with the experimental results and the agreement is acceptable. It is proved that the present pseudo-potential and thermal MRT-LBM models with double distribution function are reasonable to study the cavitation bubble collapse.
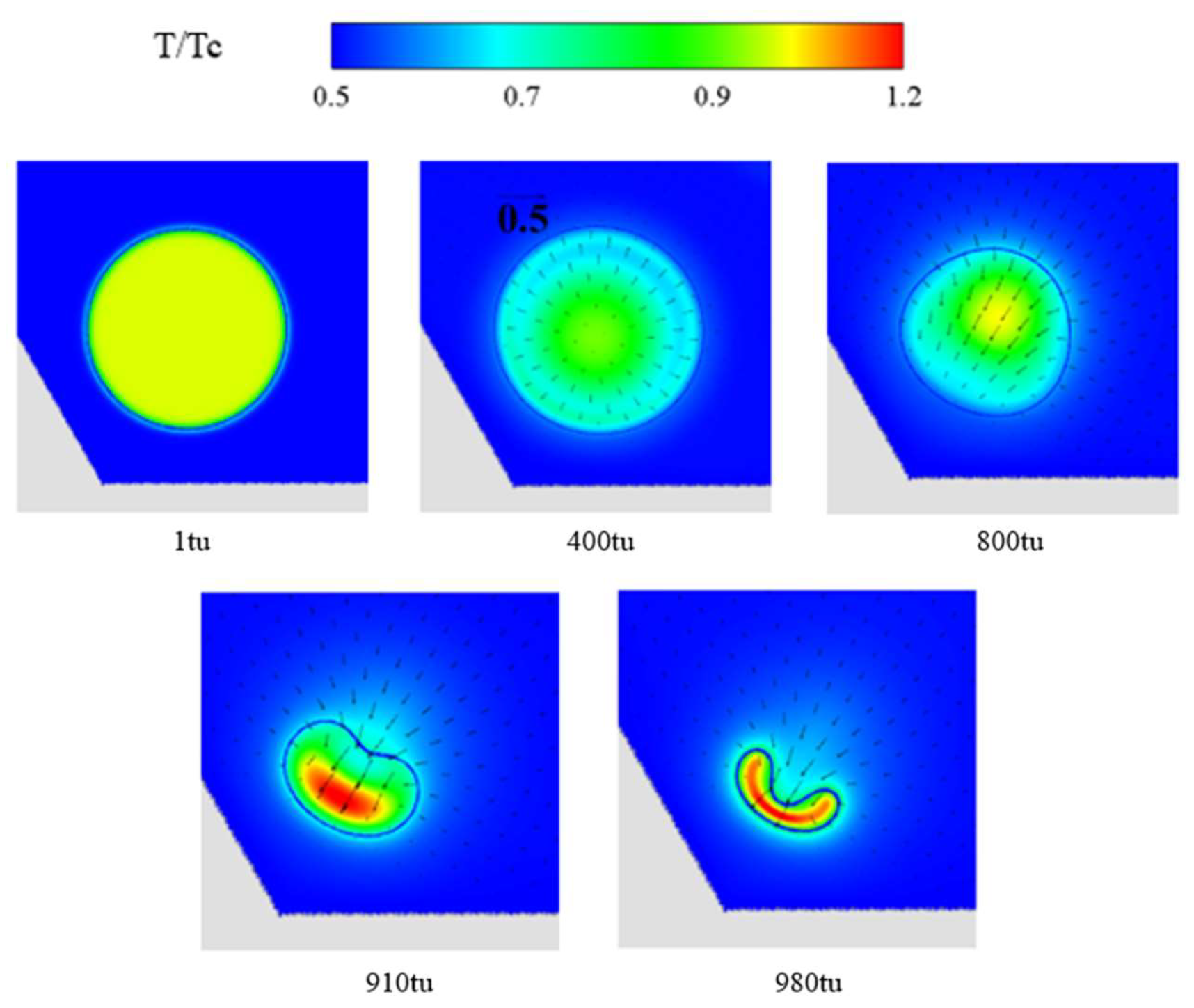
- The temperature field evolution during cavitation bubble collapse is similar. Firstly, the temperature inside the cavitation bubble gradually decreases in the process of bubble expansion. Then, the temperature will gradually increase in the contraction stage. When the bubble collapses, the temperature reaches the maximum. This law is also applicable to the double bubble collapse.
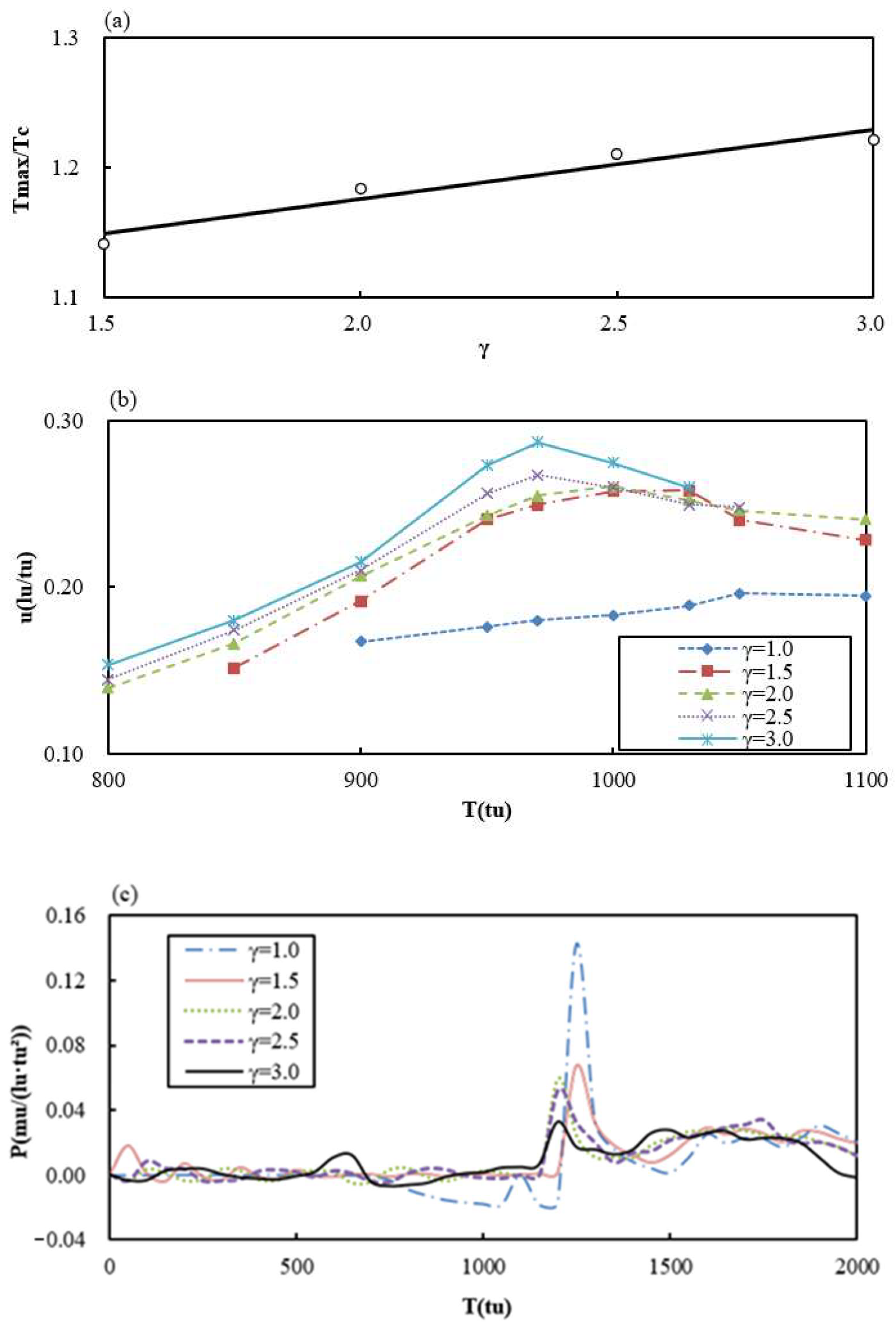
- It is found that the micro-jet velocity reaches the maximum in a period of time before bubble collapse and then decreases. With the rise of the distance between the single bubble center and the corner, the maximum temperature of bubble collapse increases approximately linearly, the velocity of micro-jet increases and its peak is more advanced and the pressure peak at the corner decreases. As the V-shaped angle increases, the velocity peak of micro-jet is larger and appears earlier, and the pressure peak at the corner is smaller and more advanced. With the increase in bubble radius, the maximum temperature of bubble collapse increases with an approximately linear relationship, the starting and ending of the micro-jet are delayed and the pressure peak at the corner is larger and also is delayed.
- In the double bubble simulation, the micro-jet velocity of the lower cavitation bubble is smaller than that of the upper one from formation to collapse. The maximum temperature of the upper bubble is higher than that of the lower one in all cases. Compared with the cases of a single bubble, the fluctuation of the pressure at the corner is much smaller before the collapse of the lower bubble in double bubble cases. With the increase in distance between two bubble centers, the maximum collapse temperature of each bubble increases, the velocity peak of the micro-jet of the upper bubble is higher, the time of the micro-jet appearing and ending is earlier and the corner pressure peak also increases.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Suo, D.J.; Jin, Z.Y.; Jiang, X.N.; Dayton, P.A.; Jing, Y. Microbubble mediated dual-frequency high intensity focused ultrasound thrombolysis: An In vitro study. Appl. Phys. Lett. 2017, 110, 023703. [Google Scholar] [CrossRef]
- Rivas, D.F.; Verhaagen, B. Preface to the Special Issue: Cleaning with bubbles. Ultrason. Sonochem. 2016, 29, 517–518. [Google Scholar] [CrossRef] [PubMed]
- Cui, P.; Zhang, A.M.; Wang, S.P.; Khoo, B.C. Ice breaking by a collapsing bubble. J. Fluid Mech. 2018, 841, 287–309. [Google Scholar] [CrossRef]
- Xu, W.L.; Bai, L.X.; Zhang, F.X. Interaction of a Cavitation Bubble and an Air Bubble with a rigid boundary. J. Hydrodyn. 2010, 22, 503–512. [Google Scholar] [CrossRef]
- Muller, M.; Hujer, J.; Kotek, M.; Zima, P. Identification of collapse patterns of cavitation bubbles close to a solid wall. EPJ Web Conf. 2013, 45, 01120. [Google Scholar] [CrossRef]
- Li, X.F.; Duan, Y.X.; Zhang, Y.N.; Tang, N.N. Retardant Effects of Collapsing Dynamics of a Laser-Induced Cavitation Bubble Near a Solid Wall. Symmetry 2019, 11, 1051. [Google Scholar] [CrossRef]
- Brujan, E.A.; Noda, T.; Ishigami, A.; Ogasawara, T.; Takahira, H. Dynamics of laser-induced cavitation bubbles near two perpendicular rigid walls. Fluid Mech. 2018, 841, 28–49. [Google Scholar] [CrossRef]
- Cui, J.; Chen, Z.P.; Wang, Q.; Zhou, T.R.; Corbett, C. Experimental studies of bubble dynamics inside a corner. Ultrason. Sonochem. 2020, 64, 104951. [Google Scholar] [CrossRef]
- Tomita, Y.; Robinson, P.B.; Tong, R.P.; Blake, J.R. Growth and collapse of cavitation bubbles near a curved rigid boundary. J. Fluid Mech. 2002, 466, 259–283. [Google Scholar] [CrossRef]
- Zhang, Y.N.; Qiu, X.; Zhang, X.Q.; Tang, N.N. Collapsing dynamics of a laser-induced cavitation bubble near the edge of a rigid wall. Ultrason. Sonochem. 2020, 67, 105157. [Google Scholar] [CrossRef]
- Kim, D.; Kim, D. Underwater bubble collapse on a ridge-patterned structure. Phys. Fluids 2020, 32, 053312. [Google Scholar] [CrossRef]
- Fukaya, M.; Tamura, Y.; Matsumoto, Y. Prediction of Cavitation Intensity and Erosion Area in Centrifugal Pump by Using Cavitating Flow Simulation with Bubble Flow Model. J. Fluid Sci. Technol. 2010, 5, 305–316. [Google Scholar] [CrossRef]
- Han, R.; Tao, L.B.; Zhang, A.M.; Li, S. A three-dimensional modeling for coalescence of multiple cavitation bubbles near a rigid wall. Phys. Fluids 2019, 31, 062107. [Google Scholar] [CrossRef]
- Li, B.B.; Jia, W.; Zhang, H.C.; Lu, J. Investigation on the collapse behavior of a cavitation bubble near a conical rigid boundary. Shock Waves 2014, 24, 317–324. [Google Scholar] [CrossRef]
- Lyu, F.X.; Zhang, X.T.; Yuan, H.X.; Han, S.Y.; Tang, M. Research on the collapse characteristics of single cavitation bubble near solid particle by the VOF method. Heliyon 2023, 9, e21855. [Google Scholar] [CrossRef]
- Li, S.; Zhang, A.M.; Han, R. 3D model for inertial cavitation bubble dynamics in binary immiscible fluids. J. Comput. Phys. 2023, 494, 112508. [Google Scholar] [CrossRef]
- Duy, T.N.; Nguyen, V.T.; Phan, T.H.; Nguyen, Q.T.; Park, S.H.; Park, W.G. Numerical study of bubble dynamics near a solid wall with a gas-entrapping hole. Ocean. Eng. 2023, 285, 115344. [Google Scholar] [CrossRef]
- Zhang, J.Y.; Du, Y.X.; Liu, J.Q.; Sun, Y.R.; Yao, Z.F.; Zhong, Q. Experimental and numerical investigations of the collapse of a laser-induced cavitation bubble near a solid wall. J. Hydrodyn. 2022, 34, 189–199. [Google Scholar] [CrossRef]
- Zhang, C.; Yin, Z.Q.; Tu, C.X.; Huang, Z.M.; Chen, T.H.; Bao, F.B.; Lu, J.L.; Ge, X.F. Dynamic behavior of the cavitation bubbles collapsing between a rigid wall and an elastic wall. AIP Adv. 2021, 11, 065025. [Google Scholar] [CrossRef]
- Lü, M.; Ning, Z.; Sun, C.H.; Ning, Z. Numerical simulation of cavitation bubble collapse within a droplet. Comput. Fluids. 2017, 152, 157–163. [Google Scholar]
- Zhang, J.; Zhang, L.X.; Deng, J. Numerical Study of the Collapse of Multiple Bubbles and the Energy Conversion during Bubble Collapse. Water 2019, 11, 247. [Google Scholar] [CrossRef]
- Ye, J.C.; Zhang, J.; Huang, T.Y. Direct Numerical Simulation of Bubble Cluster Collapse: Shape Evolution and Energy Transfer Mechanisms. Processes 2023, 11, 2191. [Google Scholar] [CrossRef]
- Popinet, S.; Zaleski, S. Bubble collapse near a solid boundary a numerical study of the influence of viscosity. J. Fluid Mech. 2002, 464, 137–163. [Google Scholar] [CrossRef]
- Shan, X.W.; Chen, H.D. Lattice Boltzmann model for simulating flows with multiple phases and components. Phys. Rev. E 1993, 47, 1815–1819. [Google Scholar] [CrossRef] [PubMed]
- d’Humières, D. Generalized Lattice-Boltzmann Equations. In AIAA Rarefied Gas Dynamics: Theory and Applications; American Institute of Aeronautics and Astronautics: Reston, VA, USA, 1994. [Google Scholar]
- Li, Q.; Luo, K.H.; Li, X.J. Lattice Boltzmann modeling of multiphase flows at large density ratio with an improved pseudopotential model. Phys. Rev. E 2013, 87, 053301. [Google Scholar] [CrossRef] [PubMed]
- Sukop, M.C.; Or, D. Lattice Boltzmann method for homogeneous and heterogeneous cavitation. Phys. Rev. E 2005, 71, 046703. [Google Scholar] [CrossRef]
- Mishra, S.K.; Deymier, P.A.; Muralidharan, K.; Frantziskonis, G.; Pannala, S.; Simunovic, S. Modeling the coupling of reaction kinetics and hydrodynamics in a collapsing cavity. Ultrason. Sonochem. 2010, 17, 258–265. [Google Scholar] [CrossRef]
- Ezzatneshan, E.; Vaseghnia, H. Simulation of collapsing cavitation bubbles in various liquids by lattice Boltzmann model coupled with the Redlich-Kwong-Soave equation of state. Phys. Rev. E 2020, 102, 053309. [Google Scholar] [CrossRef]
- Mao, Y.F.; Peng, Y.; Zhang, J.M. Study of Cavitation Bubble near a wall by the modified Lattice Boltzmann Mothod. Water 2018, 10, 1439. [Google Scholar] [CrossRef]
- Peng, C.; Tian, S.C.; Li, G.S.; Sukop, M.C. Simulation of laser-produced single cavitation bubbles with hybrid thermal Lattice Boltzmann method. Int. J. Heat Mass Transf. 2020, 149, 119136. [Google Scholar] [CrossRef]
- Swift, M.R.; Orlandini, E.; Osborn, W.R.; Yeomans, J.M. Lattice Boltzmann simulations of liquid-gas and binary fluid systems. Physical Rev. E 1996, 54, 5041–5052. [Google Scholar] [CrossRef] [PubMed]
- Shan, X.W. Pressure tensor calculation in a class of nonideal gas lattice Boltzmann models. Physical Rev. E 2008, 77, 066702. [Google Scholar] [CrossRef] [PubMed]
- Gunstensen, A.K.; Rothman, D.H.; Zaleski, S.; Zanetti, G. Lattice Boltzmann model of immiscible fluids. Phys. Rev. A 1991, 43, 4320. [Google Scholar] [CrossRef] [PubMed]
- Zheng, R.Y.; Liu, H.H.; Sun, J.J.; Ba, Y. Droplet hysteresis investigation on non-wetting striped textured surfaces: A lattice Boltzmann study. Physica A 2014, 411, 53–62. [Google Scholar] [CrossRef]
- Shi, D.Y.; Wang, Z.K.; Zhang, A.M. Study on coupling characteristics between bubble and complex walls at the same scale. Acta Phys. Sin. 2014, 63, 174701. [Google Scholar]
- Ahmad, S.; Eze, C.; Liu, H.Q.; Zhao, J.Y. Lattice Boltzmann study of bubble dynamics and heat transfer on a hybrid rough surface with a cavity-pillar structure. Int. Commun. Heat Mass Transf. 2020, 119, 104896. [Google Scholar] [CrossRef]
- Dong, B.; Zhang, Y.J.; Zhou, X.; Chen, C.; Li, W.Z. Numerical simulation of bubble dynamics in subcooled boiling along inclined structured surface. J. Thermophys. Heat Transf. 2021, 35, 16–27. [Google Scholar] [CrossRef]
- Liu, Y.; Peng, Y. Study on the Collapse Process of Cavitation Bubbles Near the Concave Wall by Lattice Boltzmann Method Pseudo-Potential Model. Energies 2020, 13, 4398. [Google Scholar] [CrossRef]
- Xue, H.H.; Shan, F.; Guo, X.S.; Tu, J.; Zhang, D. Cavitation bubble collapse near a curved wall by the Multiple-Relaxation-Time Shan-Chen Lattice Boltzmann Model. Chin. Phys. Lett. 2017, 34, 084301. [Google Scholar] [CrossRef]
- Yang, Y.; Shan, M.L.; Kan, X.F.; Shangguan, Y.Q.; Han, Q.B. Thermodynamic of collapsing cavitation bubble investigated by pseudopotential and thermal MRT-LBM. Ultrason. Sonochem. 2020, 62, 104873. [Google Scholar] [CrossRef]
- Liu, Y.; Peng, Y. Study on the collapse process of cavitation bubbles including heat transfer by Lattice Boltzmann Method. J. Mar. Sci. Eng. 2021, 9, 219. [Google Scholar] [CrossRef]
- Yuan, H.; Zhang, J.B.; Zhou, J.Y.; Tan, J.W.; Wang, Z.B.; Gan, W.D. Study of wall wettability effects on cavitation bubble collapse using lattice Boltzmann method. AIP Adv. 2021, 11, 065011. [Google Scholar] [CrossRef]
- He, X.L.; Song, X.; Peng, H.N.; Yuan, H. A lattice Boltzmann study of the thermodynamics of an interaction between two cavitation bubbles. In Discrete and Continuous Dynamical Systems—S; AIMS: Pasadena, CA, USA, 2023. [Google Scholar] [CrossRef]
- Wang, Y.R.; Peng, H.N.; He, X.L.; Zhang, J.M. Cavitation bubbles with a tunable-surface-tension thermal lattice Boltzmann model. Phys. Fluids 2022, 34, 102008. [Google Scholar] [CrossRef]
- Huang, H.B.; Sukop, M.C.; Lu, X.Y. Multiphase Lattice Boltzmann Methods: Theory and Application; Wiley: Hoboken, NJ, USA, 2015. [Google Scholar]
- Mukherjee, S.; Abraham, J. A pressure-evolution-based multi-relaxation-time high-density-ratio two-phase lattice-Boltzmann model. Comput. Fluids 2007, 36, 1149–1158. [Google Scholar] [CrossRef]
- Lallemand, P.; Luo, L.S. Theory of the lattice Boltzmann method Dispersion, dissipation, isotropy, Galilean invariance, and stability. Phys. Rev. E 2000, 61, 6546–6562. [Google Scholar] [CrossRef] [PubMed]
- Li, Q.; He, Y.L.; Tang, G.H.; Tao, W.Q. Improved axisymmetric lattice Boltzmann scheme. Phys. Rev. E 2010, 81, 056707. [Google Scholar] [CrossRef] [PubMed]
- Mohamad, A.A. Lattice Boltzmann Method: Fundamentals and Engineering Applications with Computer Codes; Springer: London, UK, 2017. [Google Scholar]
- Chen, S.Y.; Doolen, G.D. lattice Boltzmann method for fluid flows. Ann. Rev. Fluid Mech. 1998, 30, 329–364. [Google Scholar] [CrossRef]
- Sbragaglia, M.; Benzi, R.; Biferale, L.; Succi, S.; Sugiyama, K.; Toschi, F. Generalized lattice Boltzmann method with multirange pseudopotential. Phys. Rev. E 2007, 75, 026702. [Google Scholar] [CrossRef]
- Li, Q.; Luo, K.H.; Kang, Q.J.; Chen, Q. Contact angles in the pseudopotential lattice Boltzmann modeling of wetting. Phys. Rev. E 2014, 90, 053301. [Google Scholar] [CrossRef]
- Yuan, P.; Schaefer, L. Equations of state in a lattice Boltzmann model. Phys. Fluids 2006, 18, 042101. [Google Scholar] [CrossRef]
- Guo, Z.L.; Shi, B.C.; Zheng, C.G. A coupled lattice BGK model for the Boussinesq equations. Int. J. Numer. Methods 2002, 39, 325–342. [Google Scholar] [CrossRef]
- Li, Q.; Zhou, P.; Yan, H.J. Improved thermal lattice Boltzmann model for simulation of liquid-vapor phase change. Phys. Rev. E 2017, 96, 063303. [Google Scholar] [CrossRef] [PubMed]
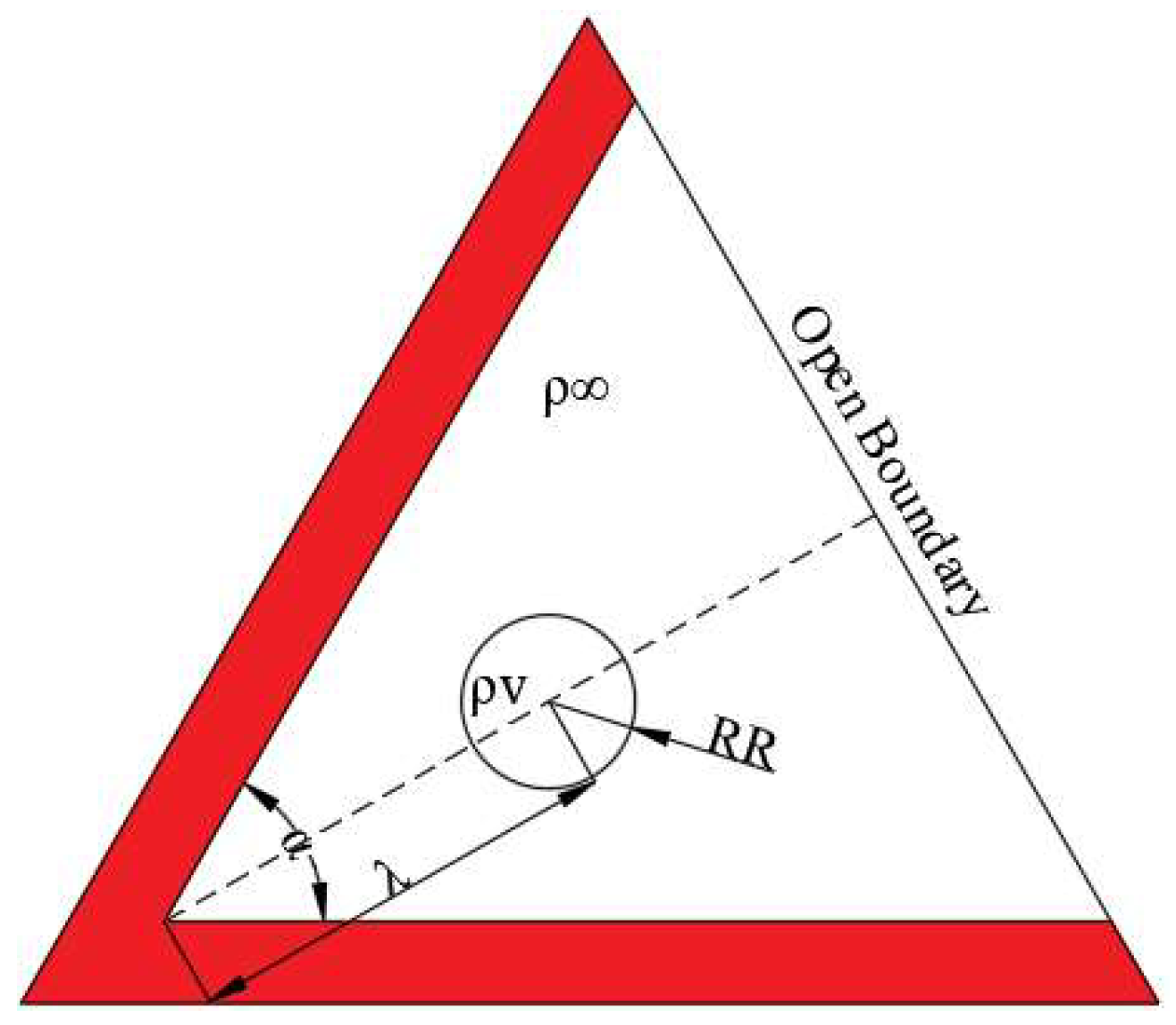





| Case | RR | α | λ |
|---|---|---|---|
| 1 | 13 | 2π/3 | 1 |
| 2 | 13 | 2π/3 | 1.5 |
| 3 | 13 | 2π/3 | 2 |
| 4 | 13 | 2π/3 | 2.5 |
| 5 | 13 | 2π/3 | 3 |
| 6 | 13 | π/3 | 2 |
| 7 | 13 | π/2 | 2 |
| 8 | 13 | 5π/6 | 2 |
| 9 | 7 | π/3 | 3 |
| 10 | 10 | π/3 | 3 |
| 11 | 13 | π/3 | 3 |
| 12 | 17 | π/3 | 3 |
| 13 | 20 | π/3 | 3 |
| Case | D | α | λ |
|---|---|---|---|
| 14 | 2.5RR | π/3 | 4RR |
| 15 | 3RR | π/3 | 4RR |
| 16 | 4RR | π/3 | 4RR |
| 17 | 6RR | π/3 | 4RR |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, Y.; Ouyang, J.; Peng, Y.; Liu, Y. Numerical Simulation of Cavitation Bubble Collapse inside an Inclined V-Shape Corner by Thermal Lattice Boltzmann Method. Water 2024, 16, 161. https://doi.org/10.3390/w16010161
Li Y, Ouyang J, Peng Y, Liu Y. Numerical Simulation of Cavitation Bubble Collapse inside an Inclined V-Shape Corner by Thermal Lattice Boltzmann Method. Water. 2024; 16(1):161. https://doi.org/10.3390/w16010161
Chicago/Turabian StyleLi, Yu, Jingyi Ouyang, Yong Peng, and Yang Liu. 2024. "Numerical Simulation of Cavitation Bubble Collapse inside an Inclined V-Shape Corner by Thermal Lattice Boltzmann Method" Water 16, no. 1: 161. https://doi.org/10.3390/w16010161
APA StyleLi, Y., Ouyang, J., Peng, Y., & Liu, Y. (2024). Numerical Simulation of Cavitation Bubble Collapse inside an Inclined V-Shape Corner by Thermal Lattice Boltzmann Method. Water, 16(1), 161. https://doi.org/10.3390/w16010161










