Integration of Hydrate-Based Desalination (HBD) into Multistage Flash (MSF) Desalination as a Precursor: An Alternative Solution to Enhance MSF Performance and Distillate Production
Abstract
1. Introduction
2. Methodology
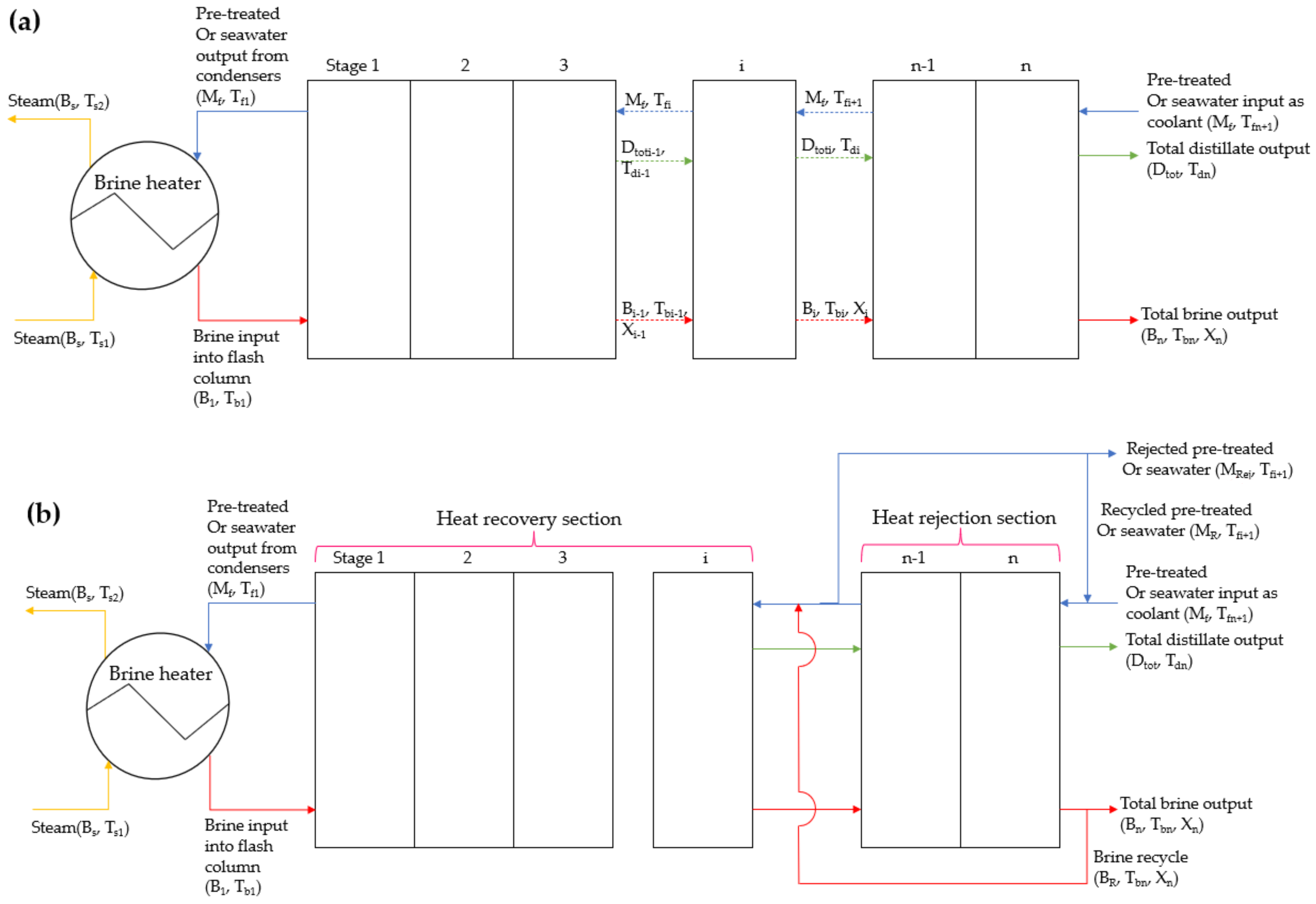
2.1. Multistage Flash Modelling
- Heat losses are negligible apart from the condenser tubes.
- The distillate produced from the MSF desalination is free of salt.
- Heat from mixing is negligible.
- Salinity changes in the coolant flowing in the condenser tubes are negligible
2.1.1. Applied Equations within the Stages
2.1.2. Applied Equations within the Condensers
2.1.3. Equations within the Brine Heater and Mixers
2.1.4. Generic Equations
2.2. Mechanism of Scale Formation
2.2.1. Model for Scale Formation
- I.
- Lumped distribution of scale formation along the condenser tubes.
- II.
- Any pressure drop between the inlet and outlets of the tubes was neglected.
- III.
- Fluctuations in velocity due to the narrowing of the tube’s cross-sectional area after scale formation was neglected.
- IV.
- The heat flux along the walls of tube bundles was neglected.
- V.
- All the ions are transported from the bulk to the heat transfer wall.
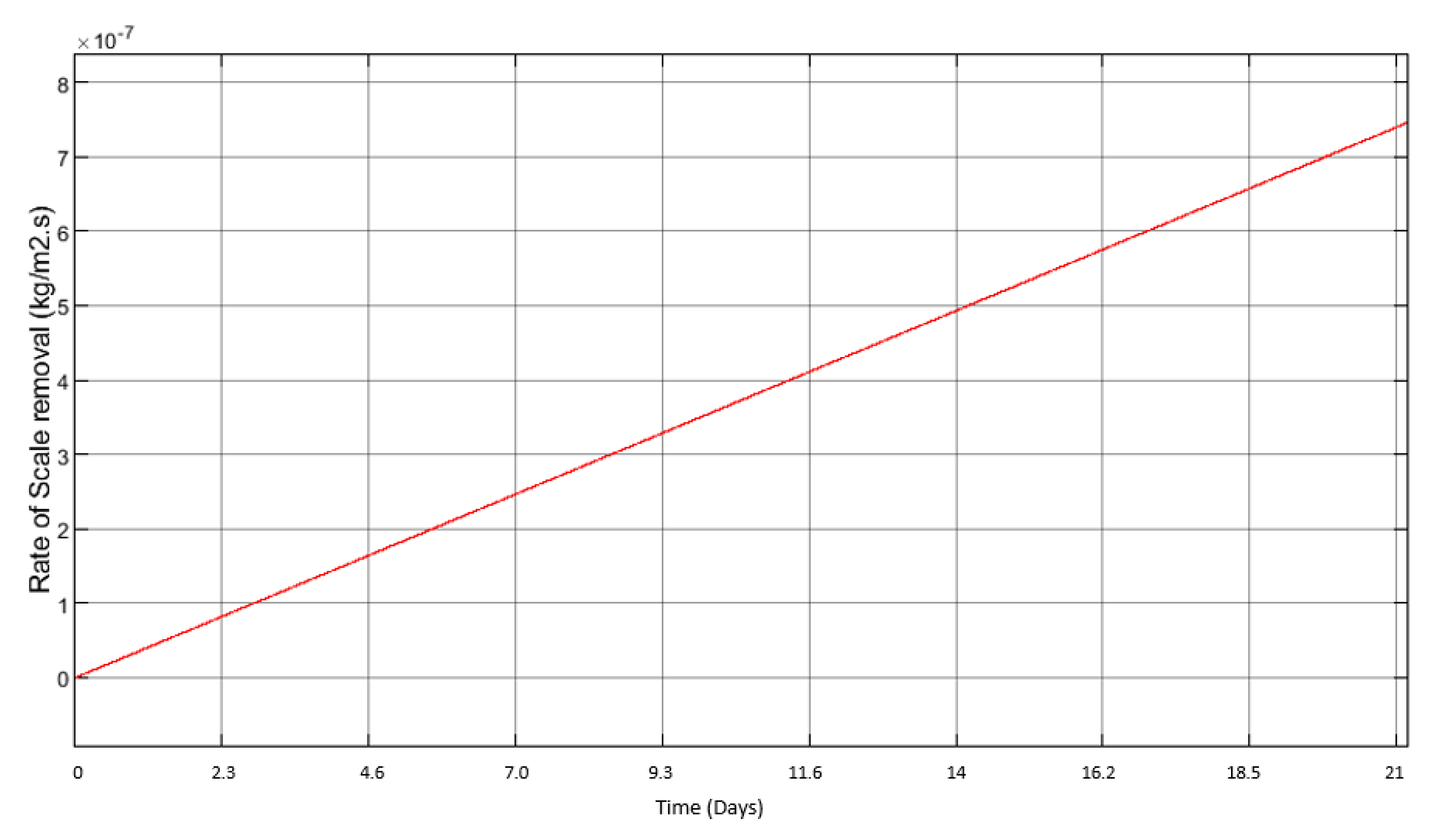
2.2.2. Calculation of Scale Deposition Rate
2.2.3. Calculation of Scale Removal Rate
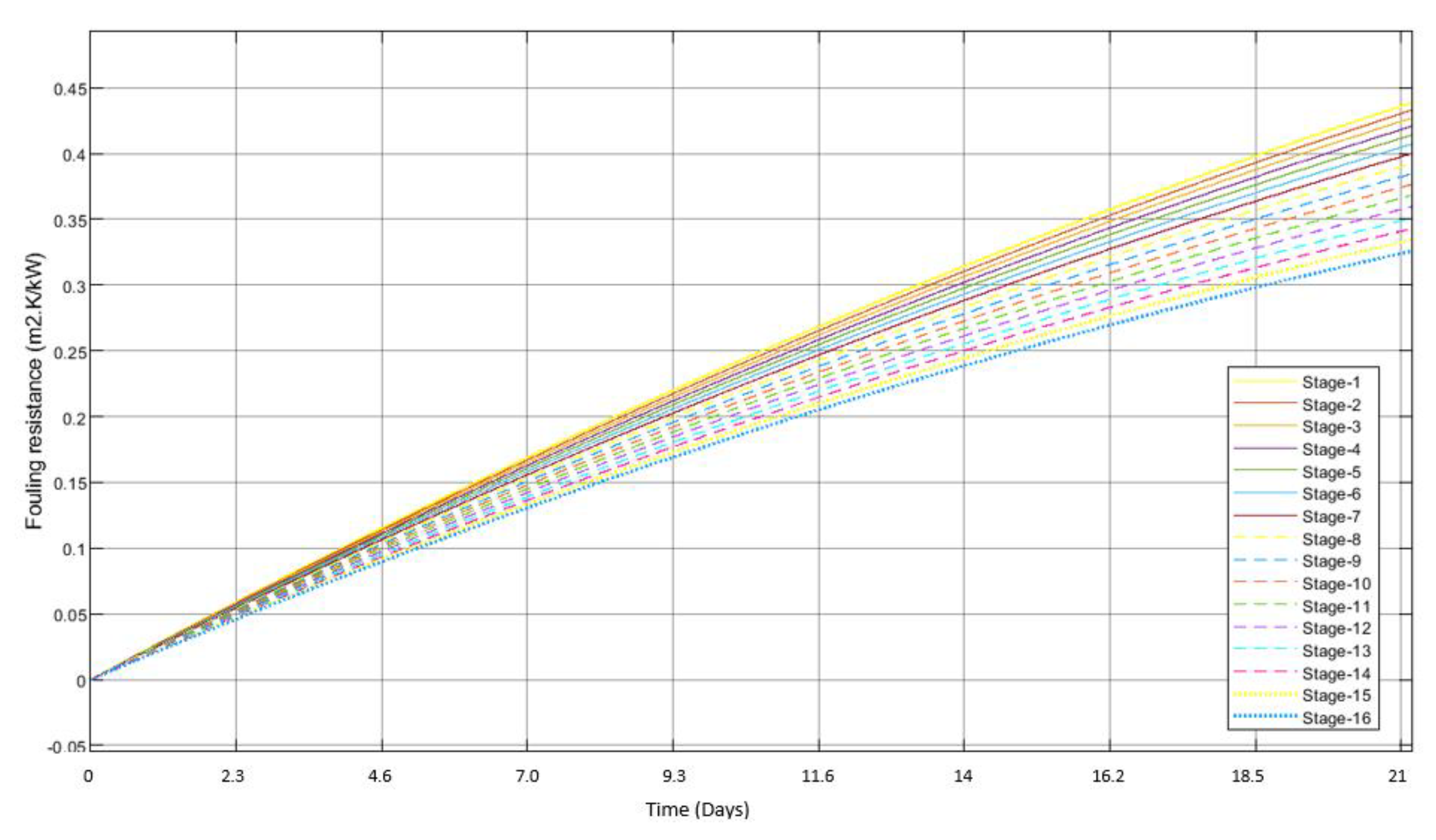
2.2.4. Calculation of Fouling Resistance
3. Results and Discussion
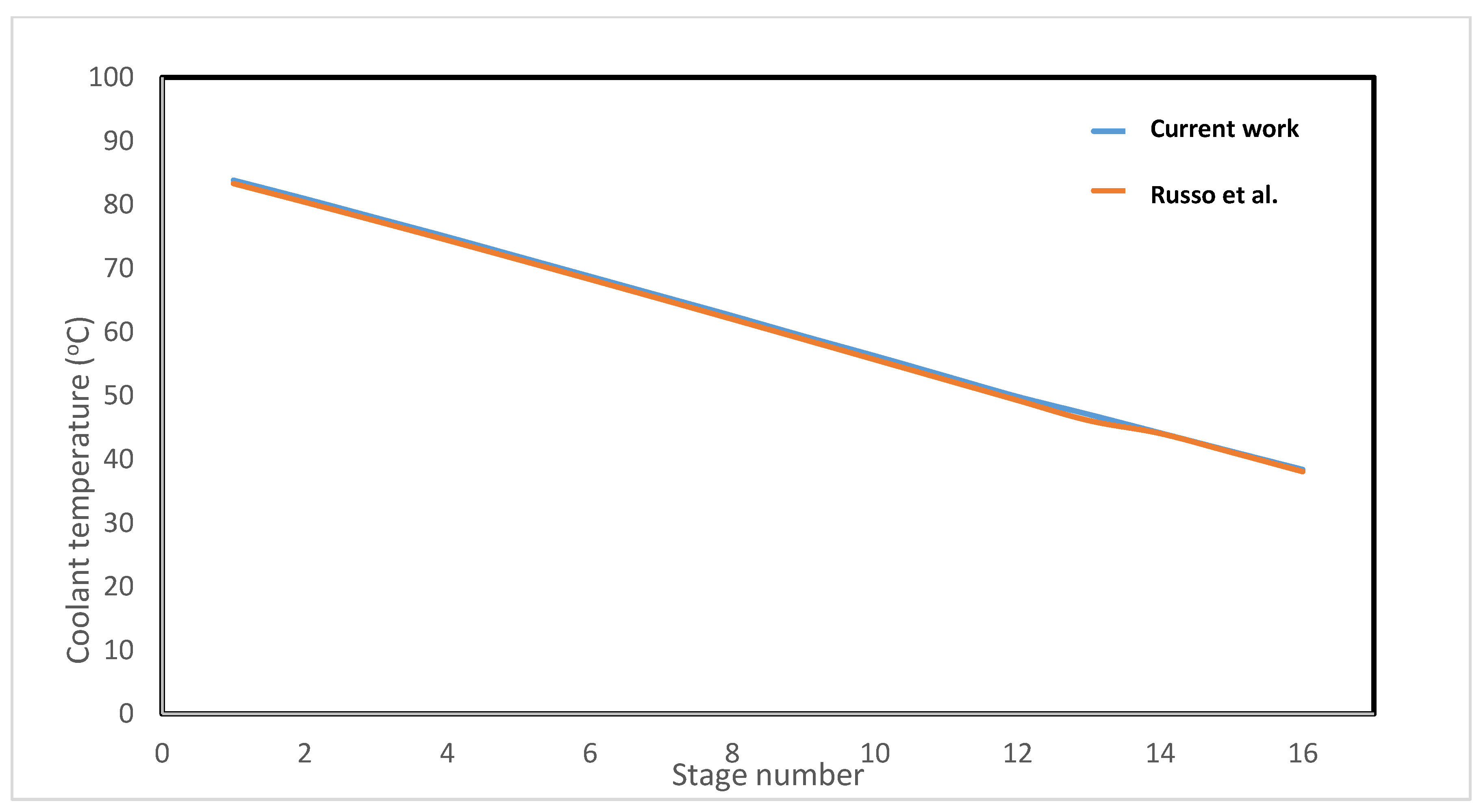
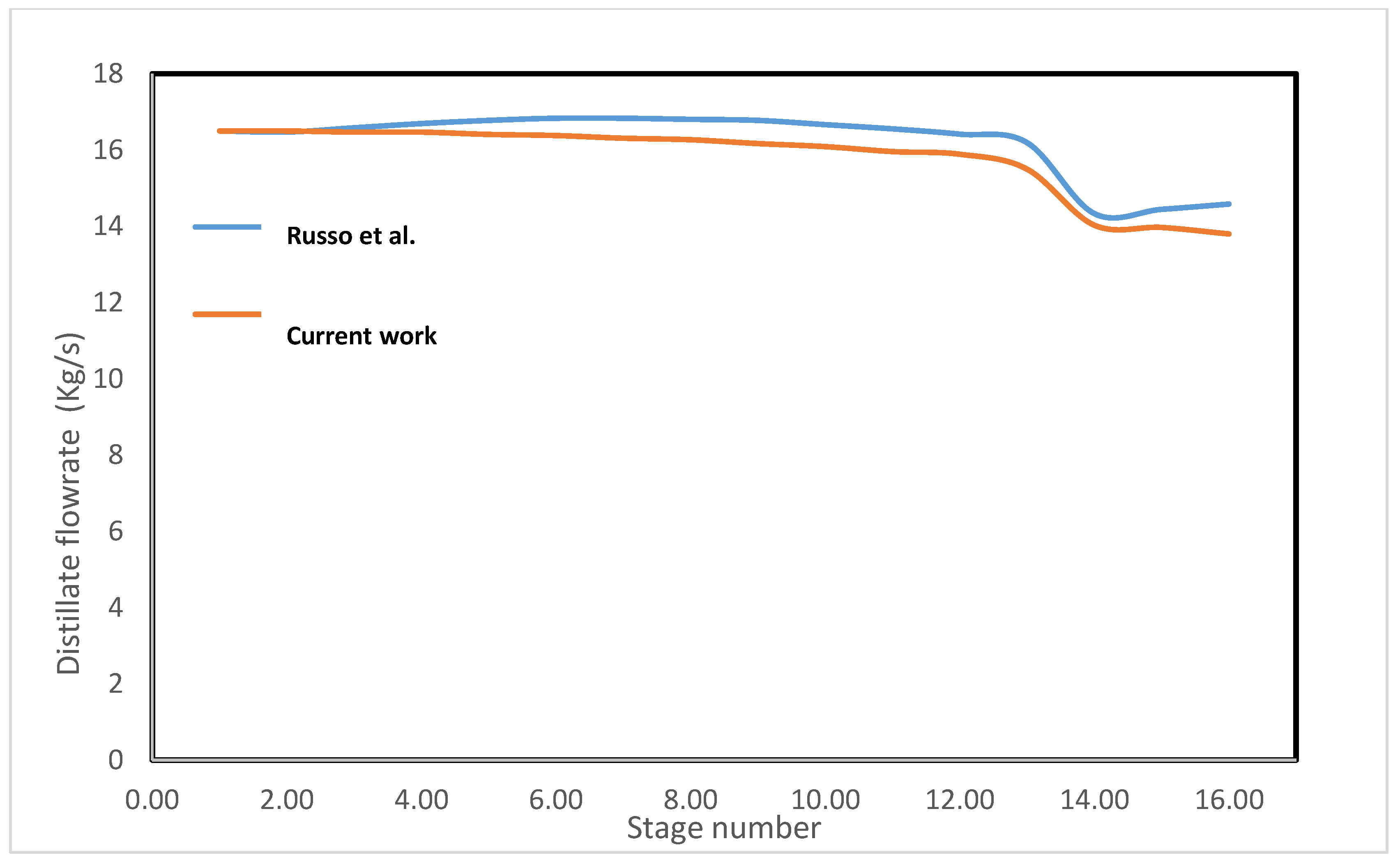
3.1. MSF Model and Validation
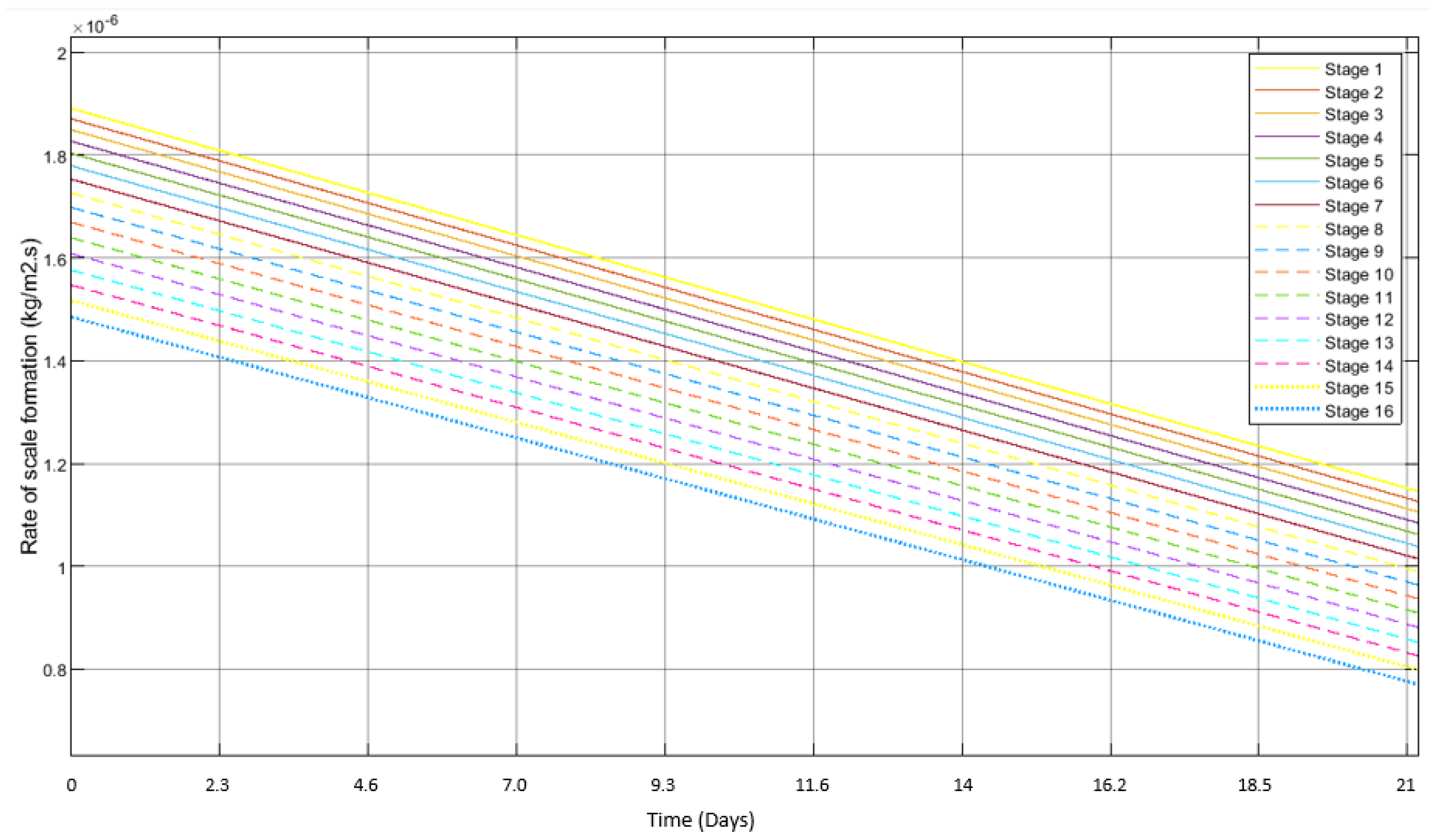
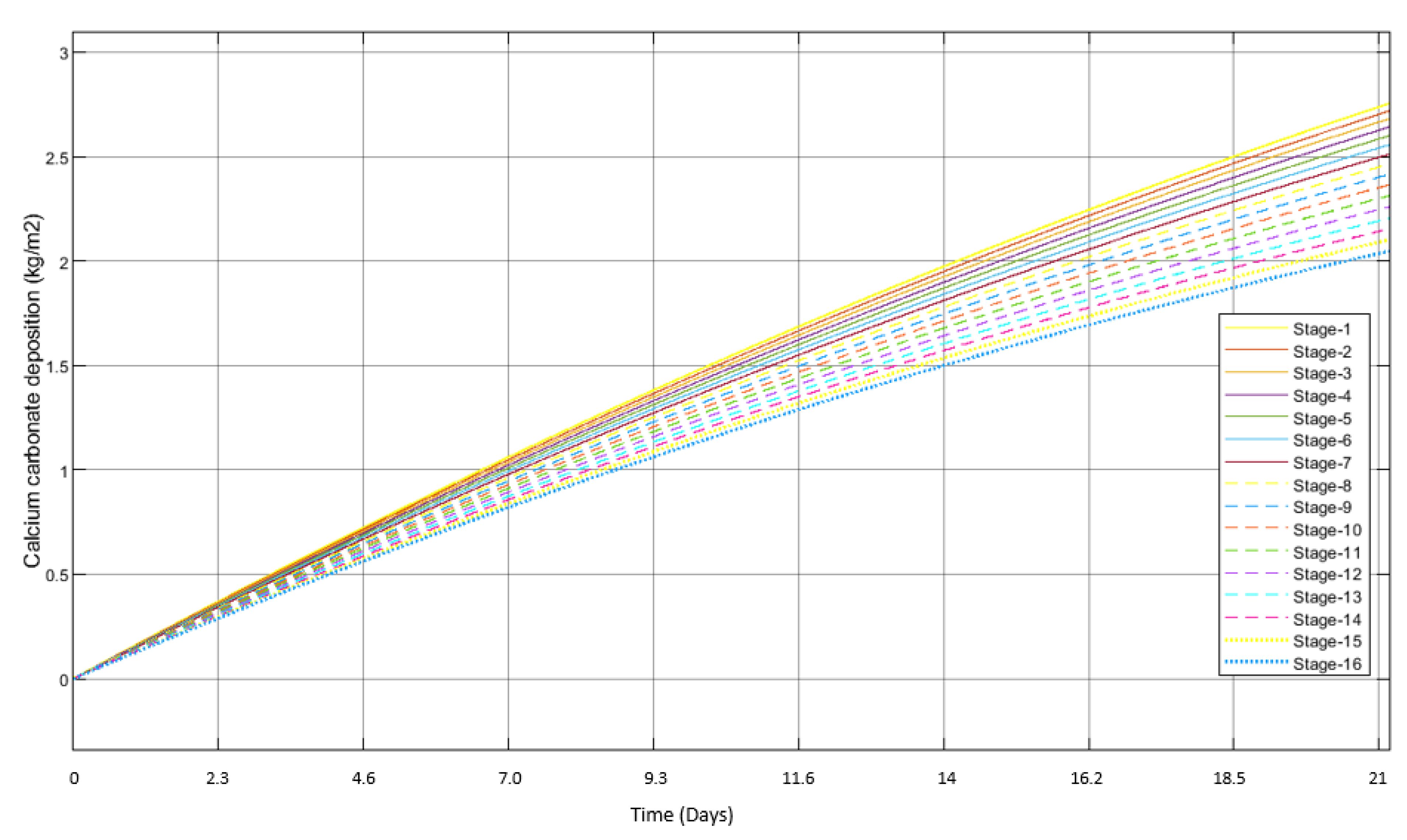
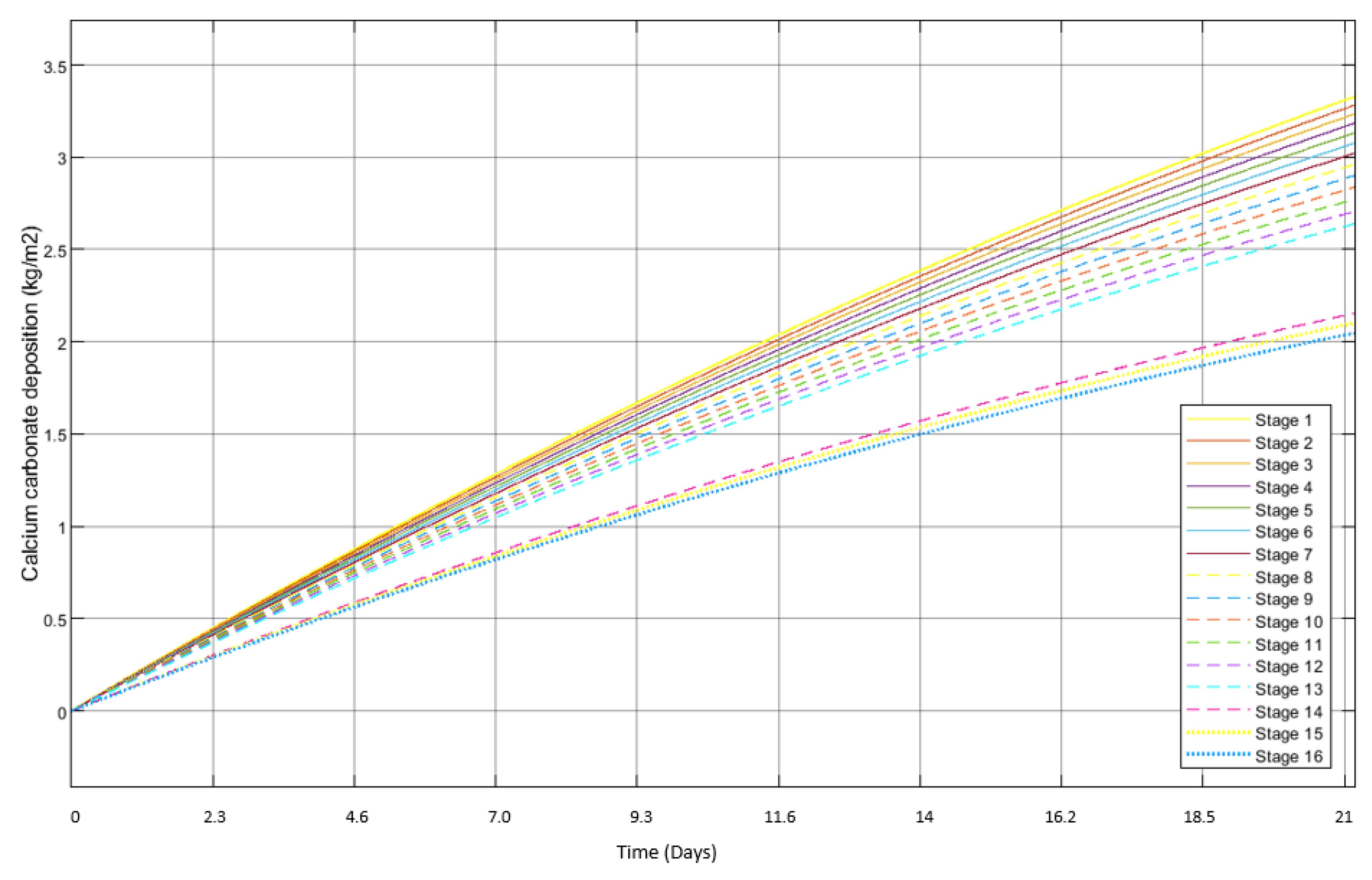
3.2. Scale Formation
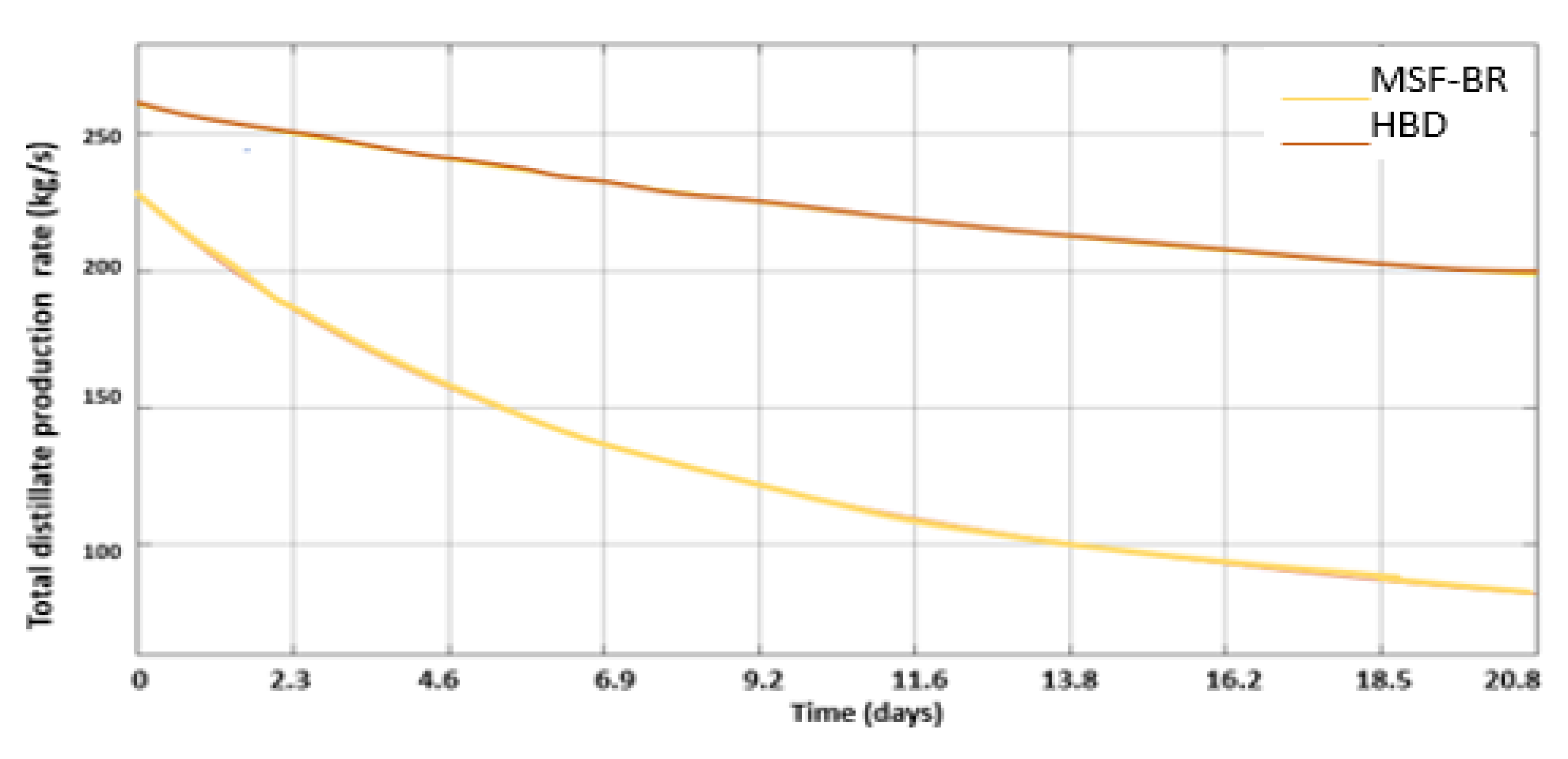
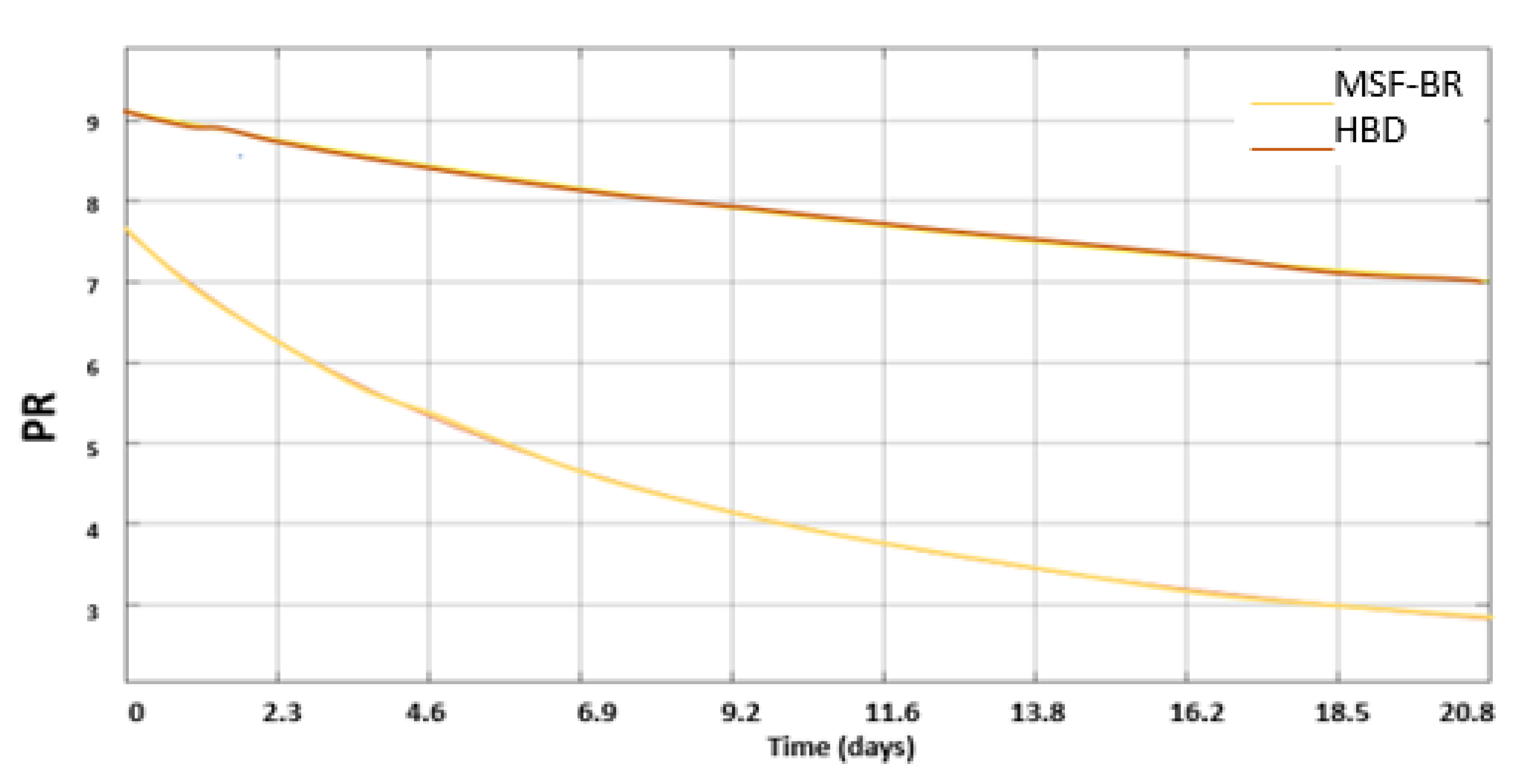
3.3. Introduction of HBD into MSF
- (a)
- The hydrate would form in the unstirred vessel, where the temporal collection of hydrate would occur. Therefore, HBD acts as a ’precursor’ thsat could, in turn, serve as the process of heat removal for MSF.
- (b)
- The liquid phase would consist of seawater along with 100 ppm of sodium dodecyl sulphate (SDS), which would be removed along with the brine remnant in HBD.
- (c)
- The hydrate forming guest gas would be a mixture of 95% CO2 and 5% CH4, where CH4 is considered to be the less hydrate-forming gaseous impurity.
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| β | Mass transfer coefficient reaction, m/s |
| µ | Brine viscosity, kg/m s |
| µR | Relative viscosity |
| µw | brine viscosity at wall, kg/m s |
| A | Heat transfer area, m2 |
| aa, ba | Debye–Huckel parameters specific to ion ‘a’ |
| Across | Tube cross-section area, m2 |
| ADH, BDH | Debye–Huckel constants |
| B | Brine flowrate, kg/s |
| BDH | Temperature dependent Debye–Huckel constant |
| Cb | Ion concentration in the bulk stream, kg/m3 |
| Ci | Ion concentration at the liquid–solid boundary layer, kg/m3 |
| Cp | Specific heat at constant pressure, kJ/kg °C |
| Cs | Saturation concentration, kg/m3 |
| D | Diffusion coefficient, m2/s |
| Dh | Hydraulic diameter of the tube, m |
| Di | Distillate flowrate, kg/s |
| di | Inner diameter of the condenser tube, m |
| do | Outer diameter of the condenser tube, m |
| Ea | Activation energy, kJ |
| f | Fanning friction factor |
| g | Gravitational acceleration, m/s2) |
| hin | Inside heat transfer coefficient, W/m2·K |
| hout | Outside heat transfer coefficient, W/m2·K |
| kD | Coefficient of mass transfer, m4/s·kg |
| kr | Reaction rate constant, m4/s·kg |
| krem | Removal rate constant, m3/s·kg |
| Ksp | Solubility product, mol2/kg2 |
| ktube | Thermal conductivity of the tube, kW/m·K |
| LMTD | Log mean temperature difference, °C |
| MCR | Recirculated coolant mass flowrate, kg/s |
| Mcw | Mass flow rate of colling water, kg/s |
| md | Progressive deposition rate, kg/s·m2 |
| Mf | Intake seawater, kg/s |
| mf | Net scale deposition, kg/s·m2 |
| mi | Molality of the dissolved gas, mol/kg |
| MR | Flow rates of recycled brine, kg/s |
| mr | Mass removal rate, kg/s·m2 |
| N | Number of defects in the fouling layer |
| P | Operational pressure, MPa |
| Re | Reynolds number, Equation (43) |
| Rf | Thermal resistance, °C/kJ |
| rfi | Heat transfer resistance on the inside of the condenser tube, m2·°C/kW |
| rfo | Heat transfer resistance on the outside of the condenser tube, m2·°C/kW |
| Sc | Schmidt number |
| Tb | Brine temperature |
| Tdi | Temperature of vapour after passing through the demisters, K |
| Tf | Temperature of the coolant, K |
| Ts | Surface temperature inside the tubes, K |
| Tv | Temperature of vapour, K |
| U | Overall heat transfer coefficient, kW/m2·°C |
| Uc | Overall heat transfer coefficient, kW/m2 °C |
| v | Friction velocity, m/s |
| Vel | Velocity of the coolant stream flowing inside the condenser tubes, m/s |
| X | Salt concentration, g/kg |
| xi | Mole fraction of dissolved gas in liquid |
| xf | Layer thickness, m |
| Z | Compressibility factor |
| Zi | Charge number of ion ‘i’ |
| Za | Ionic charge on the ion ‘a’ |
| λf | Thermal conductivity, kJ/m °C |
| λv | The latent heat of vapour, kJ/kg |
| λc | Latent heat of condensation |
| ɣi | Activity coefficient |
| ρf | Density, kg/m3 |
| σf | Shear strength of the fouling layer, N/m2 |
| δ | Linear expansion coefficient, °C−1 |
| Τf | Surface shear stress, N/m2 |
| υw | Viscosity of water, kg/m·s |
| ρw | Density of water, kg/m3 |
References
- El-Ghonemy, A. Performance test of a sea water multi-stage flash distillation plant: Case study. Alex. Eng. J. 2018, 57, 2401–2413. [Google Scholar] [CrossRef]
- Al-Sofi, M.A.K. Fouling phenomena in multi stage flash (MSF) distillers. Desalination 1999, 126, 61–76. [Google Scholar] [CrossRef]
- El Din AM, S.; Mohammed, R.A. The problem of alkaline scale formation from a study on Arabian Gulf water. Desalination 1989, 71, 313–324. [Google Scholar] [CrossRef]
- Shams El Din, A.; Mohammed, R. Contribution to the problem of vapour-side corrosion of copper-nickel tubes in MSF distillers. Desalination 1998, 115, 135–144. [Google Scholar] [CrossRef]
- Shams El Din, A.; El-Dahshan, M.; Mohammed, R. Scale formation in flash chambers of high-temperature MSF distillers. Desalination 2005, 177, 241–258. [Google Scholar] [CrossRef]
- Alsadaie, S.M. Design and operation of multistage flash (MSF) desalination: Advanced control strategies and impact of fouling. Ph.D. Thesis, University of Bradford, Bradford, UK, 2017. [Google Scholar]
- Hamed, O.A.; Al-Otaibi, H.A. Prospects of operation of MSF desalination plants at high TBT and low antiscalant dosing rate. Desalination 2010, 256, 181–189. [Google Scholar] [CrossRef]
- Steinhagen, R.; Müller-Steinhagen, H.; Maani, K. Problems and costs due to heat exchanger fouling in New Zealand industries. Heat Transf. Eng. 1993, 14, 19–30. [Google Scholar] [CrossRef]
- Al-Ahmad, M.; Aleem, F.A. Scale formation and fouling problems and their predicted reflection on the performance of desalination plants in Saudi Arabia. Desalination 1994, 96, 409–419. [Google Scholar] [CrossRef]
- Song, L.; Lobo, J.D.; Brons, G.B.; Chhotray, S.; Joshi, H.M.; Lutz, G.A. Mitigation of in-tube fouling in heat exchangers using controlled mechanical vibration. ExxonMobil Research and Engineering Co. U.S. Patent Number US7836941B2, 23 November 2010. [Google Scholar]
- Pogiatzis, T.; Ishiyama, E.M.; Paterson, W.R.; Vassiliadis, V.S.; Wilson, D.I. Identifying optimal cleaning cycles for heat exchangers subject to fouling and ageing. Appl. Energy 2012, 89, 60–66. [Google Scholar] [CrossRef]
- Müller-Steinhagen, H.; Malayeri, M.; Watkinson, A. Heat exchanger fouling: Mitigation and cleaning strategies. Heat Transf. Eng. 2011, 32, 189–196. [Google Scholar] [CrossRef]
- Rosso, M.; Beltramini, A.; Mazzotti, M.; Morbidelli, M. Modelling multistage flash desalination plants. Desalination 1997, 108, 365–374. [Google Scholar] [CrossRef]
- Ali, M.; Kairouani, L. Solving equations describing the steady-state model of MSF desalination process using Solver Optimization Tool of MATLAB software. Desalination Water Treat. 2014, 52, 7473–7483. [Google Scholar] [CrossRef]
- Thoutam, P.; Rezaei Gomari, S.; Chapoy, A.; Ahmad, F.; Islam, M. Study on CO2 hydrate formation kinetics in saline water in the presence of low concentrations of CH4. ACS Omega 2019, 4, 18210–18218. [Google Scholar] [CrossRef] [PubMed]
- Boublik, T.; Fried, V.; Hala, E. The Vapour Pressures of Pure Substances; OSTI, Office of Scientific and Technical Information: Oak Ridge, TN, USA, 1984. [Google Scholar]
- Alasfour, F.N.; Abdulrahim, H.K. Rigorous steady state modelling of MSF-BR desalination system. Desalination Water Treat. 2009, 1, 259–276. [Google Scholar] [CrossRef]
- El-Dessouky, H.; Bingulac, S. Solving equations simulating the steady-state behaviour of the multi-stage flash desalination process. Desalination 1996, 107, 171–193. [Google Scholar] [CrossRef]
- El-Dessouky, H.T.; Ettouney, H.M. Fundamentals of Saltwater Desalination; Elsevier Science: Amsterdam, The Netherlands, 2002. [Google Scholar]
- El Din AM, S.; El-Dahshan, M.E.; Mohammed, R.A. Inhibition of the thermal decomposition of HCO3: A novel approach to the problem of alkaline scale formation in seawater desalination plants. Desalination 2002, 142, 151–159. [Google Scholar] [CrossRef]
- Al-Anezi, K.; Hilal, N. Scale formation in desalination plants: Effect of carbon dioxide solubility. Desalination 2007, 204, 385–402. [Google Scholar] [CrossRef]
- Hasson, D.; Avriel, M.; Resnick, W.; Rozenman, T.; Windreich, S. Mechanism of calcium carbonate scale deposition on heat-transfer surfaces. Ind. Eng. Chem. Fundam. 1968, 7, 59–65. [Google Scholar] [CrossRef]
- Brahim, F.; Augustin, W.; Bohnet, M. Numerical simulation of the fouling process. Int. J. Therm. Sci. 2003, 42, 323–334. [Google Scholar] [CrossRef]
- Helalizadeh, A.; Müller-Steinhagen, H.; Jamialahmadi, M. Mixed salt crystallisation fouling. Chem. Eng. Process. Process Intensif. 2000, 39, 29–43. [Google Scholar] [CrossRef]
- Fahiminia, F.; Watkinson, A.P.; Epstein, N. Early events in the precipitation fouling of calcium sulphate dihydrate under sensible heating conditions. Can. J. Chem. Eng. 2007, 85, 679–691. [Google Scholar] [CrossRef]
- Najibi, S.H.; Müller-Steinhagen, H.; Jamialahmadi, M. Calcium sulphate scale formation during subcooled flow boiling. Chem. Eng. Sci. 1997, 52, 1265–1284. [Google Scholar] [CrossRef]
- Andritsos, N. Calcium carbonate deposit formation under isothermal conditions. Can. J. Chem. Eng. 1996, 74, 911–919. [Google Scholar] [CrossRef]
- Augustin, W.; Bohnet, M. Influence of the ratio of free hydrogen ions on crystallization fouling. Chem. Eng. Process. Process Intensif. 1995, 34, 79–85. [Google Scholar] [CrossRef]
- Pääkkönen, T.M.; Riihimäki, M.; Simonson, C.J.; Muurinen, E.; Keiski, R.L. Crystallization fouling of CaCO3: Analysis of experimental thermal resistance and its uncertainty. Int. J. Heat Mass Transf. 2012, 55, 6927–6937. [Google Scholar] [CrossRef]
- Hasson, D.; Sherman, H.; Biton, M. Prediction of calcium carbonate scaling rates. In Proceedings of the International Symposium on Freshwater from the Sea, Las Palmas, Spain, 9–12 November 1978; pp. 193–199. [Google Scholar]
- Bohnet, M. Fouling of heat transfer surfaces. Chem. Eng. Technol. 1987, 10, 113–125. [Google Scholar] [CrossRef]
- Helalizadeh, A.; Müller-Steinhagen, H.; Jamialahmadi, M. Mathematical modelling of mixed salt precipitation during convective heat transfer and sub-cooled flow boiling. Chem. Eng. Sci. 2005, 60, 5078–5088. [Google Scholar] [CrossRef]
- Pääkkönen, T.M.; Riihimäki, M.; Simonson, C.J.; Muurinen, E.; Keiski, R.L. Modelling CaCO3 crystallization fouling on a heat exchanger surface: Definition of fouling layer properties and model parameters. Int. J. Heat Mass Transf. 2015, 83, 84–98. [Google Scholar] [CrossRef]
- Segev, R.; Hasson, D.; Semiat, R. Rigorous modelling of the kinetics of calcium carbonate deposit formation. AIChE J. 2012, 58, 1222–1229. [Google Scholar] [CrossRef]
- Plummer, L.N.; Busenberg, E. The solubilities of calcite, aragonite and vaterite in CO2-H2O solutions between 0 and 90 C, and an evaluation of the aqueous model for the system CaCO3-CO2-H2O. Geochem. Et Cacochymical Acta 1982, 46, 1011–1040. [Google Scholar] [CrossRef]
- Krause, S. Fouling of heat transfer surfaces by crystallization and sedimentation. International Chemical Engineering, 33, Web. mitigation and cleaning strategies. Heat Transf. Eng. 1993, 32, 189–196. [Google Scholar]
- Zhang, F.; Xiao, J.; Chen, X.D. Towards predictive modelling of crystallization fouling: A pseudo-dynamic approach. Food Bioprod. Process. 2015, 93, 188–196. [Google Scholar] [CrossRef]
- Mwaba, M.G.; Golriz, M.R.; Gu, J. A semi-empirical correlation for crystallization fouling on heat exchange surfaces. Appl. Therm. Eng. 2006, 26, 440–447. [Google Scholar] [CrossRef]
- El Din, A.S.; Mohammed, R.A. Brine and scale chemistry in MSF distillers. Desalination 1994, 99, 73–111. [Google Scholar] [CrossRef]
- Zhao, J.; Wang, M.; Lababidi, H.M.; Al-Adwani, H.; Gleason, K.K. A review of heterogeneous nucleation of calcium carbonate and control strategies for scale formation in multi-stage flash (MSF) desalination plants. Desalination 2018, 442, 75–88. [Google Scholar] [CrossRef]
- Barsan, M.E. NIOSH Pocket Guide to Chemical Hazards; DHHS Publication: Washington, DC, USA, 2007. [Google Scholar]
- Horai, K. Thermal conductivity of rock-forming minerals. J. Geophys. Res. 1971, 76, 1278–1308. [Google Scholar] [CrossRef]
- Watkinson, A.; Martinez, O. Scaling of heat exchanger tubes by calcium carbonate. J. Heat Transf. 1975, 97, 504–508. [Google Scholar] [CrossRef]
- Veenman, A. Heat transfer in a multi-stage flash/fluidized bed evaporator (MSF/FBE). Desalination 1977, 22, 55–76. [Google Scholar] [CrossRef]
- Hawaidi, E.; Mujtaba, I. Simulation and optimization of MSF desalination process for fixed freshwater demand: Impact of brine heater fouling. Chem. Eng. J. 2010, 165, 545–553. [Google Scholar] [CrossRef]
- Alsadaie, S.; Mujtaba, I. Dynamic modelling of heat exchanger fouling in multistage flash (MSF) desalination. Desalination 2017, 409, 47–65. [Google Scholar] [CrossRef]
- Al-Saleh, S.; Khan, A. Evaluation of Belgard EV 2000 as antiscalant control additive in MSF plants. Desalination 1994, 97, 87–96. [Google Scholar] [CrossRef]
- Zhang, X.; Vijayamohan, P.; Hu, Y.; Sum, A.K.; Subramanian, S. Gas hydrates phase equilibria for brine blends: Measurements and comparison with prediction models. Fluid Phase Equilibria 2020, 521, 112688. [Google Scholar] [CrossRef]
- Hu, Y.; Makogon, T.Y.; Karanjkar, P.; Lee, K.-H.; Lee, B.R.; Sum, A.K. Gas hydrates phase equilibria and formation from high concentration NaCl brines up to 200 MPa. J. Chem. Eng. Data 2017, 62, 1910–1918. [Google Scholar] [CrossRef]
- Fakharian, H.; Ganji, H.; Naderifar, A. Saline produced water treatment using gas hydrates. J. Environ. Chem. Eng. 2017, 5, 4269–4273. [Google Scholar] [CrossRef]
- Thoutam, P.; Rezaei Gomari, S.; Ahmad, F.; Islam, M. Comparative analysis of hydrate nucleation for methane and carbon dioxide. Molecules 2019, 24, 1055. [Google Scholar] [CrossRef]
- Ali, M.B.; Kairouani, L. Multi-objective optimization of operating parameters of a MSF-BR desalination plant using solver optimization tool of Matlab software. Desalination 2016, 381, 71–83. [Google Scholar] [CrossRef]




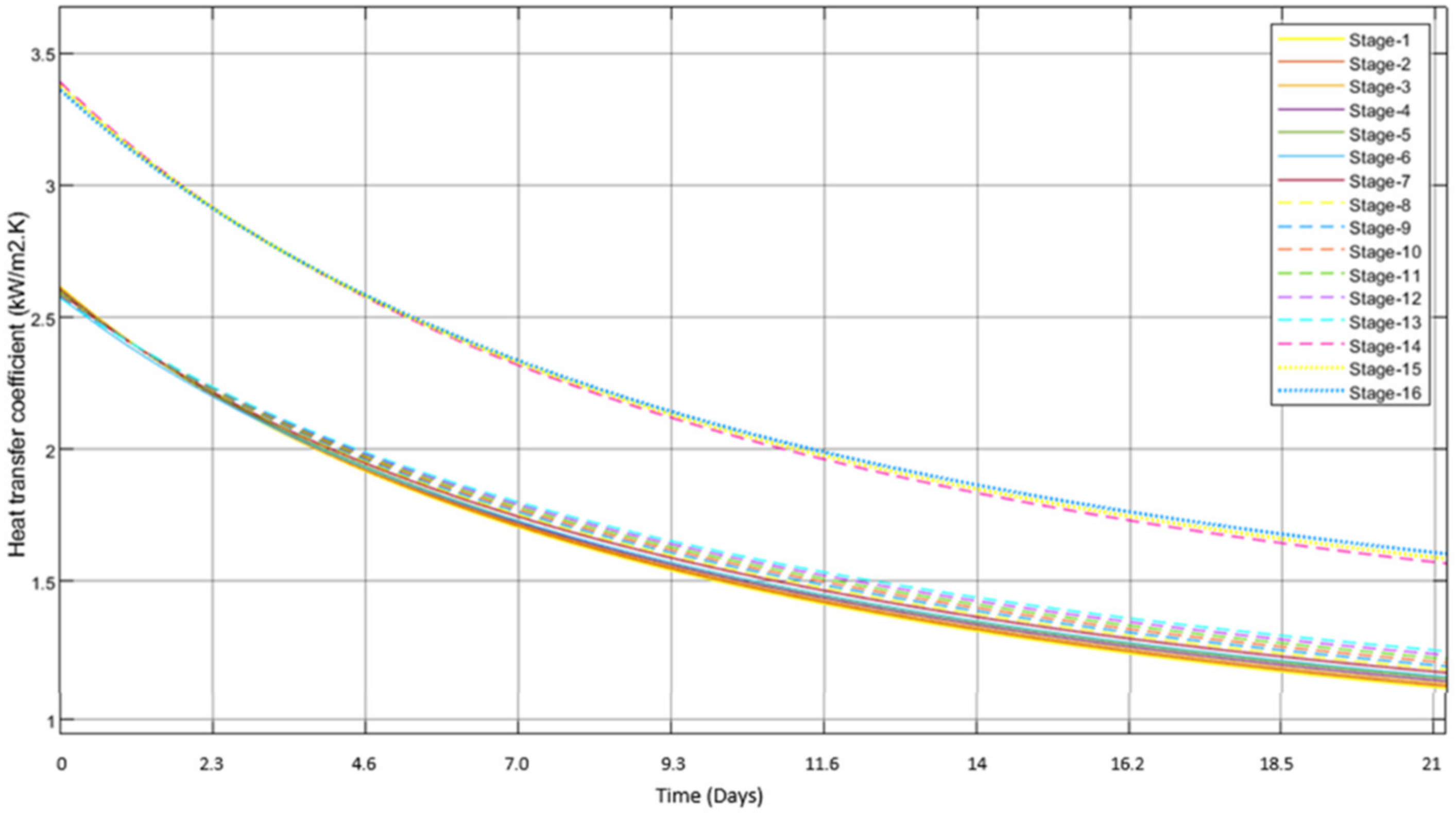
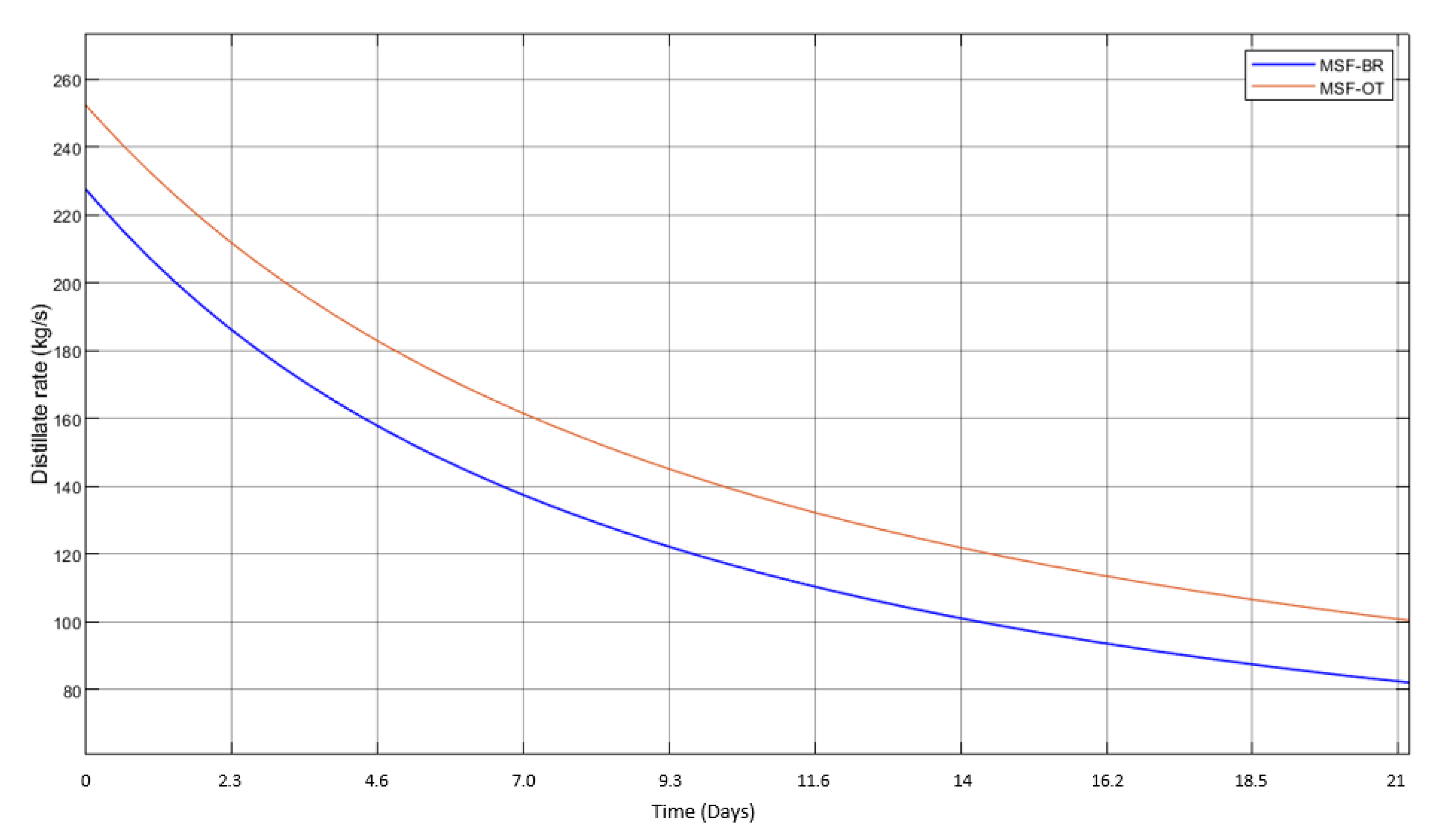
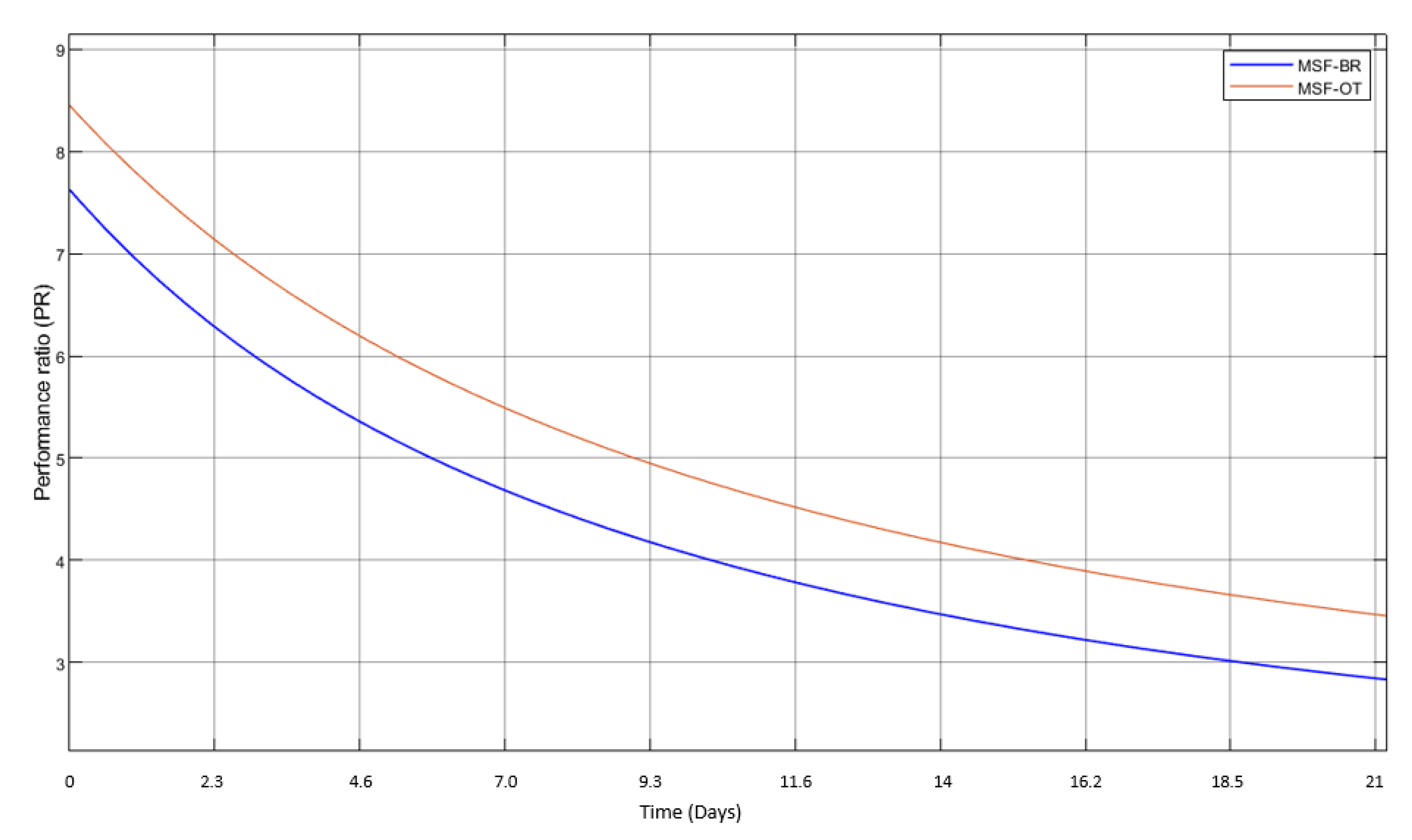
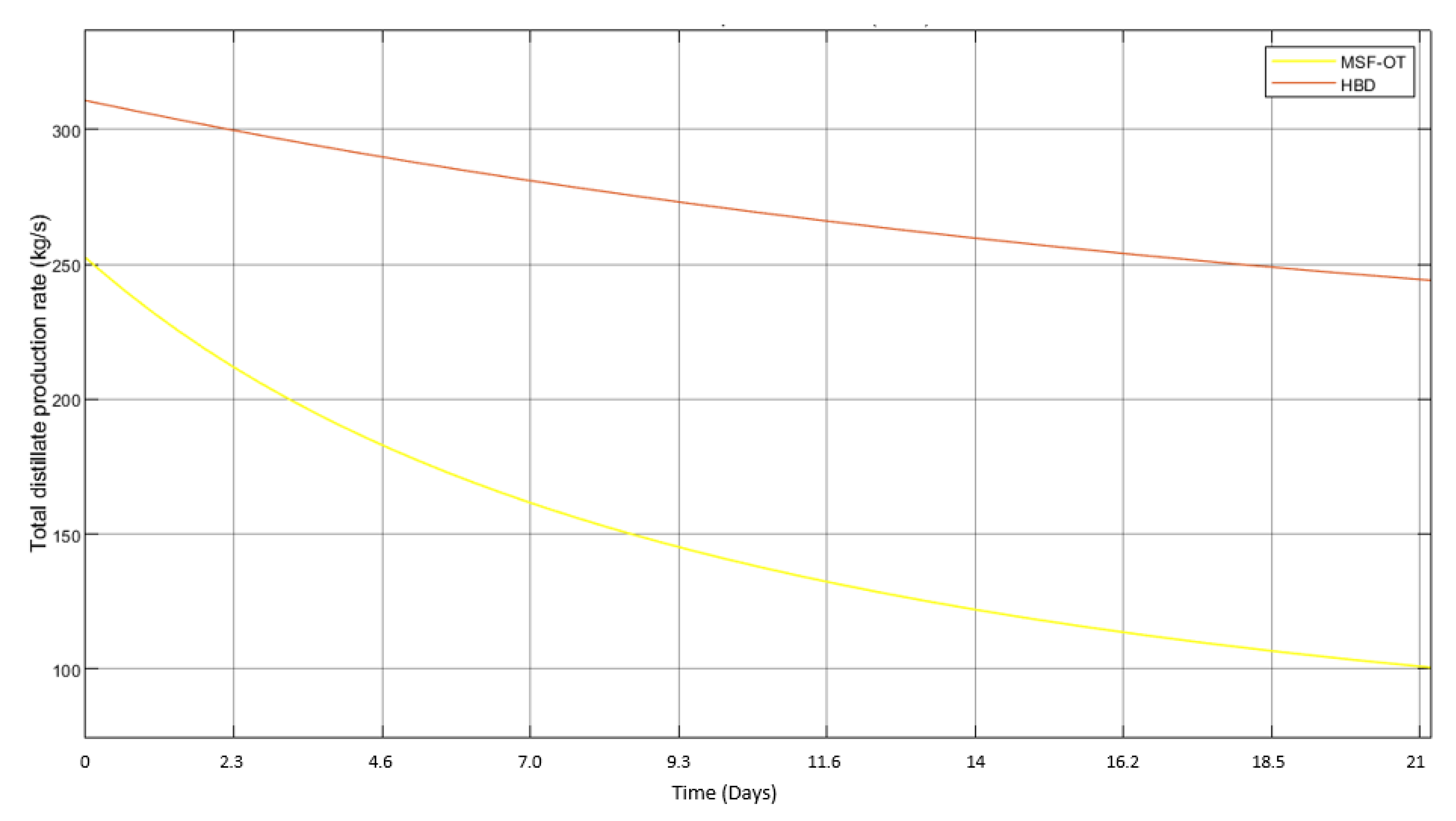



| # No. | Parameter | Value |
|---|---|---|
| 1 | No. of columns for OT | 16 |
| In the case of the BR model | ||
| 06 | |
| 13 | |
| 2 | Total seawater intake for OT | 3340 kg/s |
| In the case of the BR model | ||
| 1562 kg/s | |
| 1578 kg/s | |
| 203 kg/s | |
| 3 | Brine temperature in the flash column | 89 °C |
| 4 | Superheated steam temperature | 111 °C |
| 5 | Intake seawater salinity | 35,000 ppm |
| 6 | Intake seawater temperature | 37.7 °C |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Thoutam, P.; Ahmadi Sefiddashti, P.; Ahmad, F.; Abulkhair, H.; Ahmed, I.; Al-saiari, A.; Almatrafi, E.; Bamaga, O.; Rezaei Gomari, S. Integration of Hydrate-Based Desalination (HBD) into Multistage Flash (MSF) Desalination as a Precursor: An Alternative Solution to Enhance MSF Performance and Distillate Production. Water 2023, 15, 596. https://doi.org/10.3390/w15030596
Thoutam P, Ahmadi Sefiddashti P, Ahmad F, Abulkhair H, Ahmed I, Al-saiari A, Almatrafi E, Bamaga O, Rezaei Gomari S. Integration of Hydrate-Based Desalination (HBD) into Multistage Flash (MSF) Desalination as a Precursor: An Alternative Solution to Enhance MSF Performance and Distillate Production. Water. 2023; 15(3):596. https://doi.org/10.3390/w15030596
Chicago/Turabian StyleThoutam, Pranav, Parvin Ahmadi Sefiddashti, Faizan Ahmad, Hani Abulkhair, Iqbal Ahmed, Abdulmohsen Al-saiari, Eydhah Almatrafi, Omar Bamaga, and Sina Rezaei Gomari. 2023. "Integration of Hydrate-Based Desalination (HBD) into Multistage Flash (MSF) Desalination as a Precursor: An Alternative Solution to Enhance MSF Performance and Distillate Production" Water 15, no. 3: 596. https://doi.org/10.3390/w15030596
APA StyleThoutam, P., Ahmadi Sefiddashti, P., Ahmad, F., Abulkhair, H., Ahmed, I., Al-saiari, A., Almatrafi, E., Bamaga, O., & Rezaei Gomari, S. (2023). Integration of Hydrate-Based Desalination (HBD) into Multistage Flash (MSF) Desalination as a Precursor: An Alternative Solution to Enhance MSF Performance and Distillate Production. Water, 15(3), 596. https://doi.org/10.3390/w15030596








