A Model Development for Thermal and Solutal Transport Analysis of Non-Newtonian Nanofluid Flow over a Riga Surface Driven by a Waste Discharge Concentration

Abstract

Wastewater discharge plays a vital role in environmental management and various industries. Water pollution control and tracking are critical for conserving water resources and maintaining adherence to environmental standards. Therefore, the present analysis examines the impact of pollutant discharge concentration considering the non-Newtonian nanoliquids over a permeable Riga surface with thermal radiation. The analysis is made using two distinct kinds of non-Newtonian nanoliquids: second-grade and Walter’s liquid B. The governing equations are made using the applications of boundary layer techniques. Utilizing the suitable similarity variable reduces the formulated governing equations into an ordinary differential set of equations. The solutions will be obtained using an efficient numerical technique and the significance of various dimensionless constraints on their individual profiles will be presented using graphical illustrations. A comparative analysis is reported for second-grade and Walter’s liquid B fluids. The results show that the porous factor declines the velocity profile for both fluids. Radiation and external pollutant source variation constraints will improve thermal and concentration profiles. The rate of thermal distribution improved with the rise in radiation and solid volume factors. Further, essential engineering factors are analyzed. The outcomes of the present study will help in making decisions and putting efficient plans in place to reduce pollution and safeguard the environment.

1. Introduction

The Riga plate is a flat surface made of electrical and permanent magnetization. The Riga surface is an external factor used to minimize drag and control fluid flow. Because of the magnetoelectric fields this device generates, Lorentz forces allow fluid flow control. Flexible electronics, monitoring for earthquake disasters, reducing drag on ships, robots, smart energy networks, electromechanical devices, recuperating energy, thermal nuclear reactors, heat exchange systems, solar-powered equipment, and generators for electricity are some of the applications of Riga plates. Many academics have carried out studies on fluid flows in the area of the Riga surface. Asogwa et al. [1] scrutinized the character of Dufour, thermal production, and activation energy on the movement of the hyperbolic tangent nano-based liquid via the Riga body surface. Madhukesh et al. [2] scrutinized the consequence of thermophoretic particle decomposition of a Casson hybrid nanoliquid subjected to laminar, uniform flow generated by a Riga surface in the attendance of a porous media. Xu et al. [3] explored the consequence of the magnetic field, mixed convection, and thermal sink/source on a fourth-grade liquid circulation around a Riga body. Asgowa et al. [4] excavated the observed outcome of the heat sink and chemical reaction through a Riga surface of Casson nanofluids. Alshehri et al. [5] deliberated the thermal distribution rate, including the Cattaneo–Christov heat flux model, with the effect of fluid viscosity, magnetic field, and porous medium of hybrid nanofluids through a Riga surface. Prasad et al. [6] examined the impact of Brownian motion, viscosity, velocity slip, and first-order chemical reaction of thermal and mass distribution of nanoliquids through a Riga plate. Mandal and Pal [7] conducted a study on nanofluids having a mixture of convective and quadratic radiative movements over a decreasing Riga surface with convective boundary conditions and slip velocities

The term “Second-grade fluid” refers to a subtype of non-Newtonian fluid. And, the velocity sector displays up to two derivatives in the strain-and-stress tensor relationship. In contrast to Newtonian fluids, the equations controlling flow generally are significantly nonlinear. Non-Newtonian fluids have an inclusive array of industrial applications, together with plastic film illustration, fabricating fiberglass, creating paper, manufacturing plastic sheets, and several others. These factors make investigating non-Newtonian fluids a fascinating field for mathematical researchers, computer science researchers, and technologists. The convection of second-grade fluid in the appearance of a magnetic field along a flat plate using Prabakar fractional derivative was studied by Ali et al. [8]. Gowda et al. [9] examined the second-grade fluid along with significant impact of chemical reactions and activation energy over the Marangoni layer boundary conditions. Utilizing the Gear-generalized differential quadrature assessment of oscillating convective Taylor–Couette movement, second-grade fluid impacted by Lorentz and Darcy–Forchheimer quadratic drag forces was examined by Xia et al. [10]. The exact solution of the unsteady second-grade fluid model related to oscillating shear stress on the sphere is surveyed by Fetecau et al. [11]. Shah et al. [12] studied the impression of thermophoresis particle deposition of a second-grade fluid with the variation of viscosity, concentration diffusivity, and thermal conductivity with convective boundary conditions. Khan et al. [13] examined the impact of second-grade nanoliquid moving into two infinite plates while it reacts chemically. Over a nonlinear extending sheet, Hayat [14] investigated the magnetohydrodynamic motion of a second-grade nanofluid.

One of the momentous non-Newtonian liquid subsidiaries is Walter’s liquid B fluid. The formative equations in non-Newtonian liquids are more intricate. Hence, it is crucial to build more complicated equations. When dealing with the highly nonlinear Walter’s liquid B fluid model, it is quite complicated. One of the better models for describing the properties of a viscoelastic fluid is the Walter’s liquid B fluid model, which has a brief memory coefficient and limits viscosity at low shear rates. Elasticity features are helpful in comprehending non-Newtonian performance, and fundamental equations can frequently provide significant support in figuring out rheological qualities. Science has shown that this model is proficient in replicating the attributes of viscoelastic polymers, hydrocarbon molecules, paints, and other fluids in disparate fields. Non-Newtonian fluids are becoming increasingly significant in biology, petroleum-based substances, fluid dynamics, chemical fields, and material science. With the assistance of viscoelastic flow substances, noise reduction, mitigation of shock, and vibration isolation are performed. Walter’s liquid B fluid model through a sheet in the occurrence of porous medium with the impact of the Soret and Dufour local thermal nonequilibrium is studied by Kumar et al. [15]. Lorentz force, thermomigration, and random motion of small particles in radiative reactive Walter’s liquid B fluid brought on by mixed convection gyrotactic microorganisms are inspected by Wakif et al. [16]. Thermal stratifications have been utilized by Siddique et al. [17] to study the thermography of ferromagnetic Walter’s liquid B fluid. Qaiser et al. [18] scrutinized Walter’s liquid B fluid through an extending sheet with mass suction and magnetic field with Newtonian heating. Chu et al. [19] deliberated the interaction between Walter B nanoliquid movement across an extending sheet and TPD (thermophoresis particle deposition) with the influence of the pressure and buoyancy forces when there is a magnetic field.

Nanofluid is a base fluid combined with unique nanoparticles. In nanofluids, nanoparticles are formed of carbides, oxides, metals, etc. Water, oil, ethylene glycol, and other fundamental liquids are frequently considered base liquids. The term Nanofluid was first developed by Choi [20] in 1995. The basic liquid’s capacity to transmit heat is improved when nanoparticles are added. It has several practical uses in numerous sectors, especially the medicine, engineering, and chemical sciences. And, nanofluids have applications in industries, including biomedical, automotive, transportation, electrical, the distribution of drugs, real-time chemical monitoring of brain function, technology, and the removal of tumors. Researchers have also been more attentive to nanofluid applications of nanoliquids. Khan et al. [21] scrutinized the importance of Lorentz forces in a hybrid nanofluid movement caused by a stretched sheet contaminant. Gkountas et al. [22] examined how a nanofluid affected printed-circuit thermal transfer. Madhukesh et al. [23] deliberated the result of heat production and absorption on a nanoliquid flowing through a stretching surface in the incidence of activation energy. Haq et al. [24] examined the effect of heat sink/source, porous medium, and homogeneous and heterogeneous interaction on hybrid nanofluids of various geometries. Dogonchi et al. [25] studied the characteristics of nanofluids inside the irregular triangular enclosure in the appearance of a magnetism.

A phenomenon in which the transfer of thermal energy occurs is known as thermal radiation, and it disperses thermal energy through fluid particles. By accelerating thermal diffusivity, thermal radiation increases the temperature. Thermal radiation is frequently used in modern heat exchange systems that transport heat at exceedingly high temperatures. Thermal radiation also considerably impacts controlling the heat transfer mechanism in the polymer manufacturing industries. Fluid flow with thermal radiation is significant for applications in engineering fields, such as managing thermal distribution in the nuclear reactor and handling the thermal distribution in the polymer. The impression of thermal radiation on the space industry and high-temperature operations is well established. Gireesha et al. [26] investigated the three-dimensional Maxwell nanofluid motion with convective boundary conditions in addition to the occurrence of a magnetic field. Ramesh et al. [27] examined the movement of hybrid carbon nanotubes on a stagnation point around a spinning sphere when heat radiation and thermophoretic particle deposition were present. Taking magnetic force effects into account, Atashafrooz et al. [28] simulated the convective and radiative thermal transfer of a hybrid nanofluid flowing inside a trapezoidal container. Oke et al. [29] examined the effects of a magnetic field and thermal radiation on 3D hybrid nanofluid movement within the boundary layer. Alzahrani et al. [30] scrutinized the impact of thermal radiation on the transmission of heat in a Casson nanoliquid movement in a plane wall that exhibits suction under a slip boundary condition. Prasannakumara and Shashikumar [31] used numerical analysis to examine the boundary layer movement and transfer of heat of a tiny fluid material across a nonlinear stretched sheet in the existence of thermal radiation. Thumma et al. [32] examined the impact of Coriolis force on the movement of a nanoliquid in thermal radiation and heat generation/absorption. Hydrothermal behaviors of nanofluid flow in a trapezoid recess were analyzed using the second law of principles by Atashafrooz et al. [33].

Pollutant concentration describes the contaminants in water, air, or soil volume. Pollutants have a more significant impact on the health of people, animals, and living things. The transport of contaminants is challenging to forecast precisely. Certain research studies have produced initial conclusions on how external pollutant source characteristics affect the concentration of pollutants. Makinde et al. [34] studied pollutant transportation in rivers using partial differential equations. Cintolasi et al. [35] scrutinized the complicated connection between heat and inertial factors within the canyon, especially the effects on turbulence characteristics and pollution remediation techniques. Chinyoka and Makinde [36] examined the nonlinear spreading of a pollutant released by an external source through the laminar liquid circulation of an incompressible liquid in a channel. Southerland et al. [37] investigated the health risks of air pollution in towns. Chinyoka and Makinde [38] examined the dispersion of a polymeric impurity emanating from an external source into the laminar motion of a Newtonian solution moving via a rectangular channel.

Relative to the above-served literature, work has yet to be carried out to examine the impact of pollutant discharge concentration considering the non-Newtonian nanoliquids over a permeable Riga surface with thermal radiation. The governing expressions are made by taking the considerable impacts, and resultant equations are solved numerically using an efficient numerical scheme. The numerical outcomes are shown with the help of graphs, and the results are discussed in detail. The outcomes of this study will contribute to an enhanced understanding of waste management, temperature transportation, and distribution optimization, which increase the quality of decision-making and planning for environmental protection and environmentally friendly engineering.

The present investigation is carried out to find the answers to the following research insight questions:

• How does the modified Hartmann number impact the velocity profile in the presence of second-grade fluid and Walter’s liquid B fluid?
• What are the behavioral changes observed in the concentration profile when external pollutant source variation parameter are varied?
• How will the local pollutant external source parameter and solid volume fractions influence the mass transfer rate?

2. Mathematical Formulation

Consider a steady, two-dimensional, laminar flow of non-Newtonian second-grade fluid and Walter’s liquid B fluid circulating across a Riga surface. The Riga surface moves with a free stream velocity $\overline{u}=U_w=xa$, in $x$-direction ($a>0$) (see Figure 1). $T_w$, $T_{\infty }$, $C_w$ and $C_{\infty }$ terms represent the surface and ambient temperature of the Riga surface and surface and ambient concentration, respectively. The Riga plate is considered under the presence of electromagnetic force $F_m^{*}=\left(F_m^{*},0,0\right):\left[F_m^{*}=\exp \left(-\frac{\pi }{c}y\right)\frac{\pi j_0{M}_0}{8{\rho }_{nf}}\right]$. Further, thermal radiation and external pollutant concentrations are considered in the temperature and concentration equation.

Taking the above-stated assumptions, the governing equations are as follows (see [7,14,19,36,38]):

$$\frac{\partial \overline{u}}{\partial x}+\frac{\partial \overline{v}}{\partial y}=0,$$

(1)

$$\begin{array}{l}\overline{u}\frac{\partial \overline{u}}{\partial x}=\frac{{\mu }_{nf}}{{\rho }_{nf}}\frac{{\partial }^2\overline{u}}{\partial y^2}+\frac{\pi j_0{M}_0}{8{\rho }_{nf}}\exp \left(-\frac{\pi }{c}y\right)-\frac{{\nu }_{nf}}{K^{*}}\overline{u}-\overline{v}\frac{\partial \overline{u}}{\partial y}\\ \pm \frac{{\alpha }_1^{*}}{{\rho }_{nf}}\left(\frac{{\partial }^3\overline{u}}{\partial x\partial y^2}-\frac{{\partial }^3\overline{u}}{\partial y^2\partial x}+\overline{u}\frac{{\partial }^3\overline{u}}{\partial x\partial y^2}+\overline{v}\frac{{\partial }^3\overline{u}}{\partial y^3}\right)\\ \end{array},$$

(2)

$$\overline{u}\frac{\partial T^{*}}{\partial x}+\overline{v}\frac{\partial T^{*}}{\partial y}={\alpha }_{nf}\frac{{\partial }^2{T}^{*}}{\partial y^2}-\frac{1}{{\left(\rho C_p\right)}_{nf}}\frac{\partial q_r}{\partial y},$$

(3)

$$\overline{u}\frac{\partial C}{\partial x}-D_f\frac{{\partial }^{2}C}{\partial y^2}=Q^{*}\exp (b_3^{*}(C-C_{\infty }))-\overline{v}\frac{\partial C}{\partial y},$$

(4)

with the BCs

$$\begin{array}{l}y=0:\overline{u}=U_w,\overline{v}=0,T^{*}=T_w,C=C_w\\ y\to \infty :\overline{u}\to 0,\frac{\partial \overline{u}}{\partial y}\to 0,T^{*}\to T_{\infty },C\to C_{\infty }\end{array}\}.$$

(5)

The following set of similarity transformations are introduced:

$$\psi =x\sqrt{a{\nu }_f}f,\overline{u}=x{f}^{\prime }a,\overline{v}=-\sqrt{a{\nu }_f}f,\eta =\sqrt{\frac{1}{a^{-1}{\nu }_f}}y,\theta =\frac{T^{*}-T_{\infty }}{T_w-T_{\infty }},\chi =\frac{C-C_{\infty }}{C_w-C_{\infty }}.$$

(6)

From the overhead equations, the terms ${\alpha }_1^{*}$ denotes the material constant. ${\alpha }_1^{*}>0$ and ${\alpha }_1^{*}<0$ correspondingly denote second-grade fluid and Walter’s liquid B fluid, respectively. $x$ and $y$ are the directions. $\overline{u}$ and $\overline{v}$ are the velocity components. $\nu$ is the kinematic viscosity, $\rho$ is the density, and $j_0$ and $M_0$ are the applied current density in electrodes and magnetization of permanent magnets, $K^{*}$ is the permeability of the porous medium, $c$ is the width of the electrodes, $T^{*}$ is the temperature, $\alpha$ is the thermal diffusivity, $C_p$ is the heat capacitance, $k$ is the thermal conductivity, $D_f$ is the diffusivity, and $C$ is the concentration. $q_r$ is the radiation heat flux. $Q^{*}$ and $b_3^{*}$ denote pollutant external source variation parameters.

From Equation (3), the radiation heat flux term is defined (using Rosseland approximation (see [39])) as $q_r=-\frac{4{\sigma }^{*}}{3k^{*}}\left(\frac{\partial T^{*}{}^4}{\partial y}\right)$.

The term $T^{*}{}^4$ is a linear function of temperature. Expanding the term $T^{*}{}^4$ up to infinity, we get

$$T^{*}{}^4=T_{\infty }^4+4\left(T^{*}-T_{\infty }\right)T_{\infty }^3+6{\left(T^{*}-T_{\infty }\right)}^2{T}_{\infty }^2+\dots \dots \dots$$

Excluding the higher order components except $\left(T^{*}-T_{\infty }\right)$ in the above equation, we get $T^{*}{}^4=4T_{\infty }^3{T}^{*}-3T_{\infty }^4$; then, the expression $q_r$ takes the form

$$q_r=-\frac{16{\sigma }^{*}{T}_{\infty }^3}{3k^{*}}\left(\frac{\partial T^{*}}{\partial y}\right).$$

By substituting the above term, Equation (3) takes the following form

$$\overline{u}\frac{\partial T^{*}}{\partial x}+\overline{v}\frac{\partial T^{*}}{\partial y}=\left(\frac{k_{nf}}{{\left(\rho C_p\right)}_{nf}}+\frac{16{\sigma }^{*}{T}_{\infty }^3}{3k^{*}}\right)\frac{{\partial }^2{T}^{*}}{\partial y^2}.$$

(7)

By using Equation (6), Equations (1), (2), (4), (5) and (7) take the form

$$\frac{f^{‴}}{A_1^{*}{A}_2^{*}}\pm \frac{K_1^{*}}{A_2^{*}}\left[2f^{\prime }{f}^{‴}-f{f}^{⁗}-{(f^{″})}^2\right]+\frac{Q_1^{*}}{A_2^{*}}\exp (-\eta {\beta }_1^{*})-\frac{{\lambda }_1^{*}}{A_1^{*}{A}_2^{*}}{f}^{\prime }+f{f}^{″}-{(f^{\prime })}^2=0,$$

(8)

$$\left[\frac{k_{nf}}{k_f}+\frac{4}{3}R{d}_1^{*}\right]\frac{{\theta }^{″}}{\mathrm{Pr}{A}_3^{*}}+f{\theta }^{\prime }=0,$$

(9)

$$\frac{{\chi }^{″}}{S{c}_1^{*}}+f{\chi }^{\prime }+{\delta }_1^{*}\exp ({\gamma }_1^{*}\chi )=0,$$

(10)

with BCs

$$\begin{array}{l}f{(\eta )}_{\eta =0}=0,f^{\prime }{(\eta )}_{\eta =0}=1,\theta {(\eta )}_{\eta =0}=1,\chi {(\eta )}_{\eta =0}=1\\ f^{\prime }{(\eta )}_{\eta =\infty },f^{″}{(\eta )}_{\eta =\infty }=0,\theta {(\eta )}_{\eta =\infty }=0,\chi {(\eta )}_{\eta =\infty }=0\end{array}\}.$$

(11)

From the above Equations (8)–(10), the dimensionless constraints are listed in

Table 1. List of dimensionless constraints.

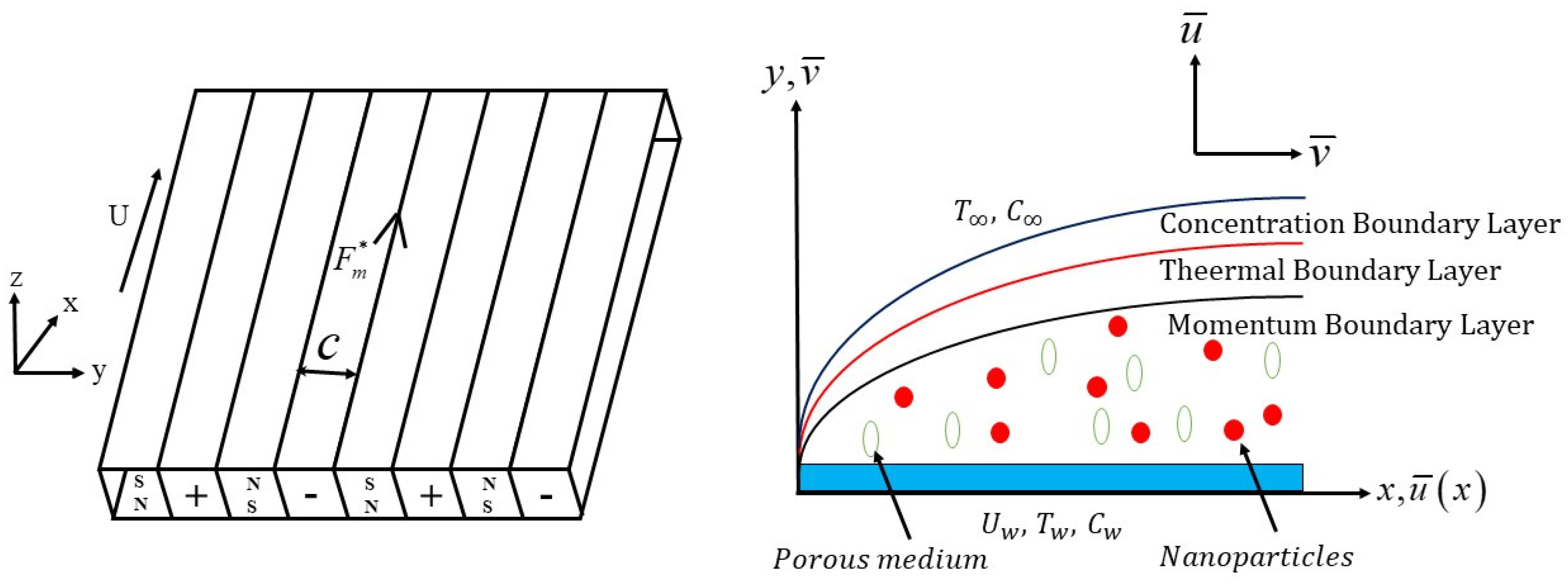
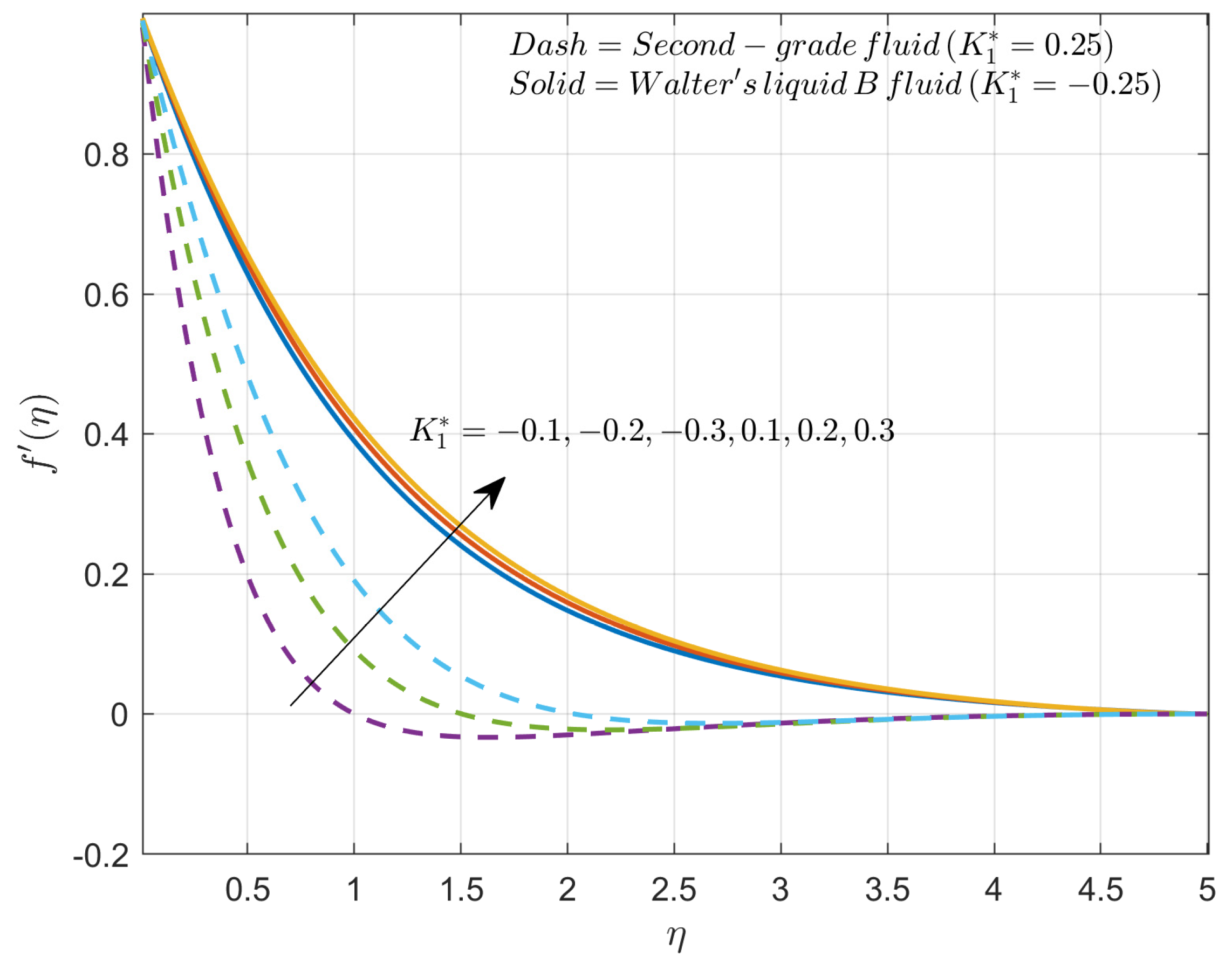
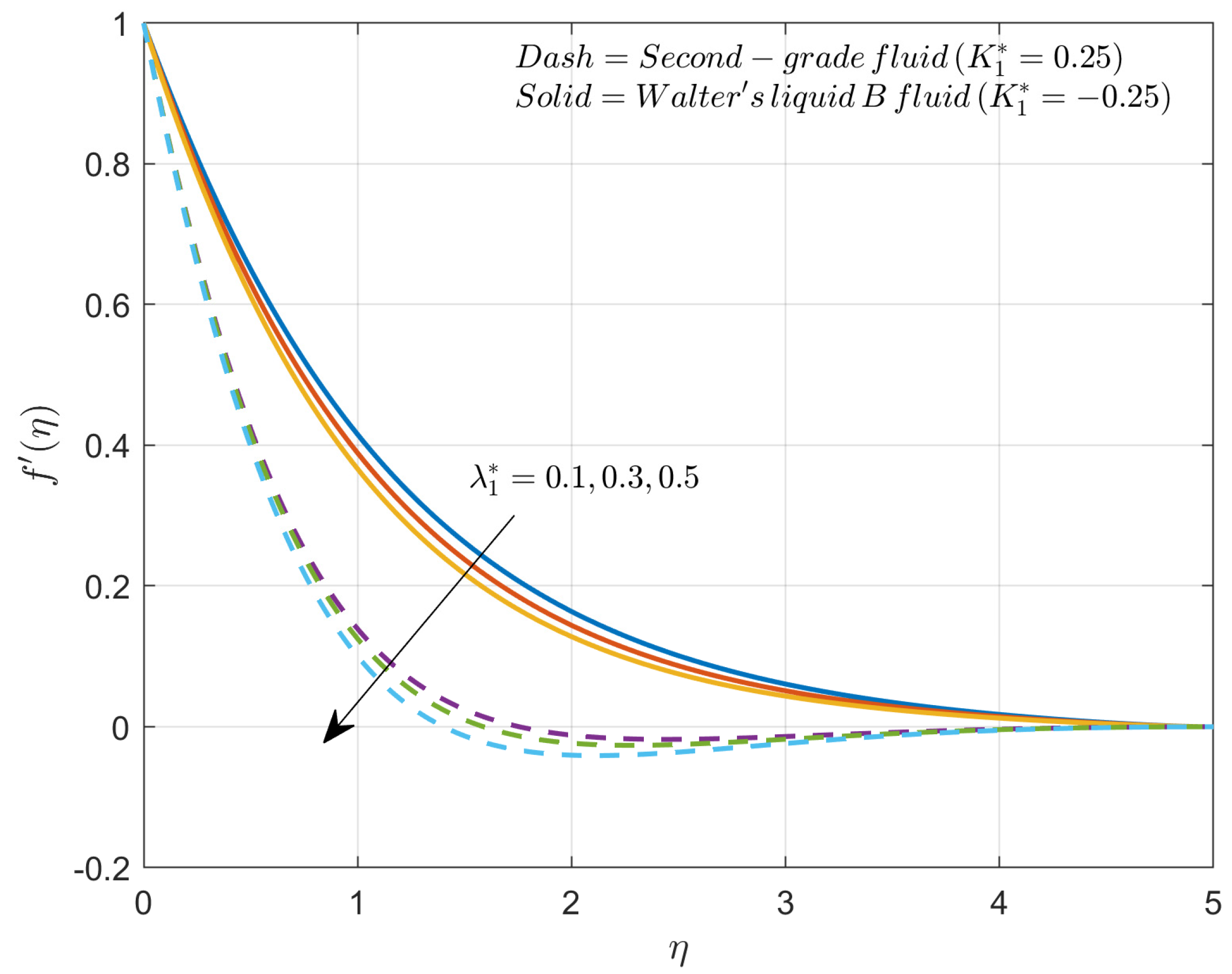
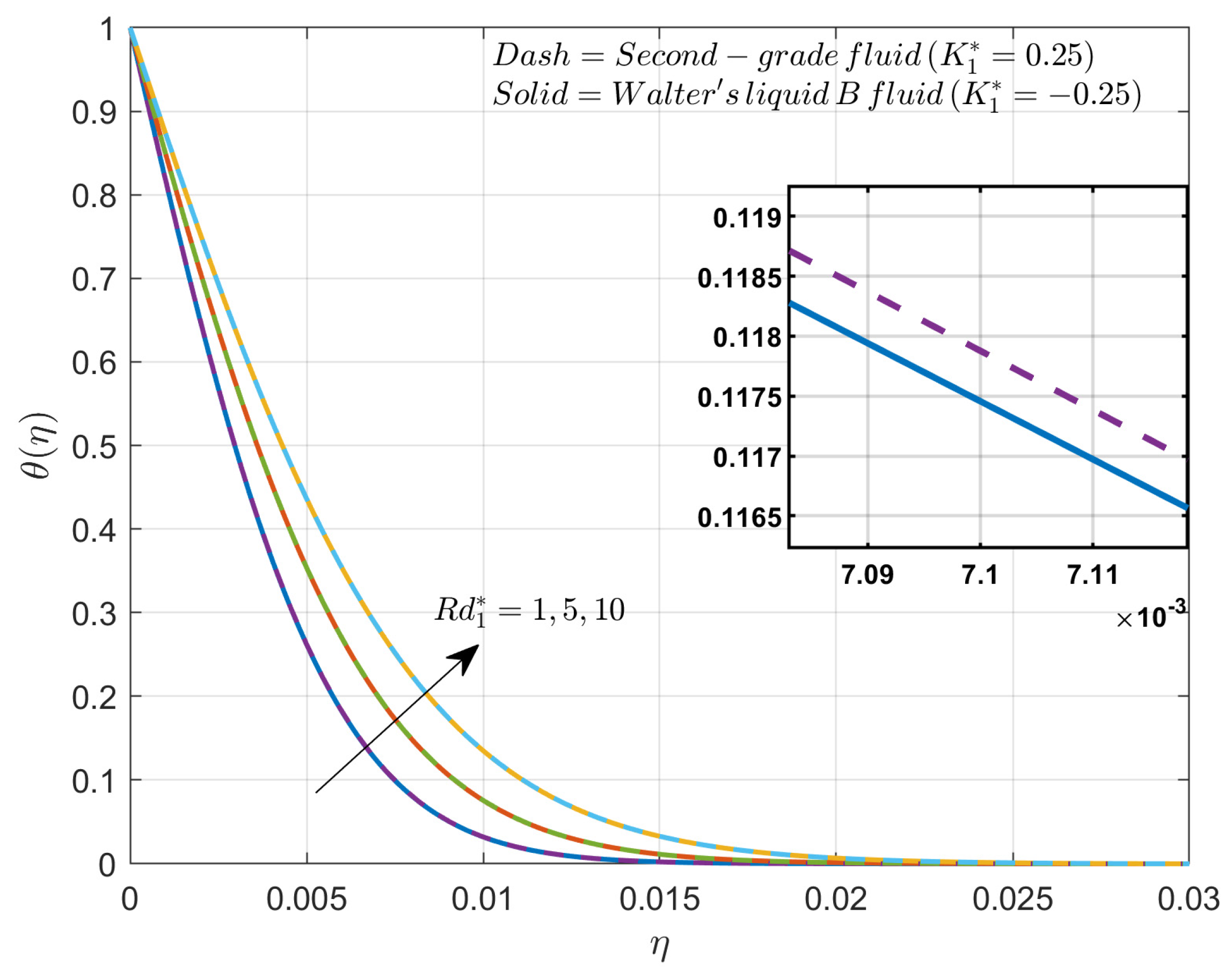
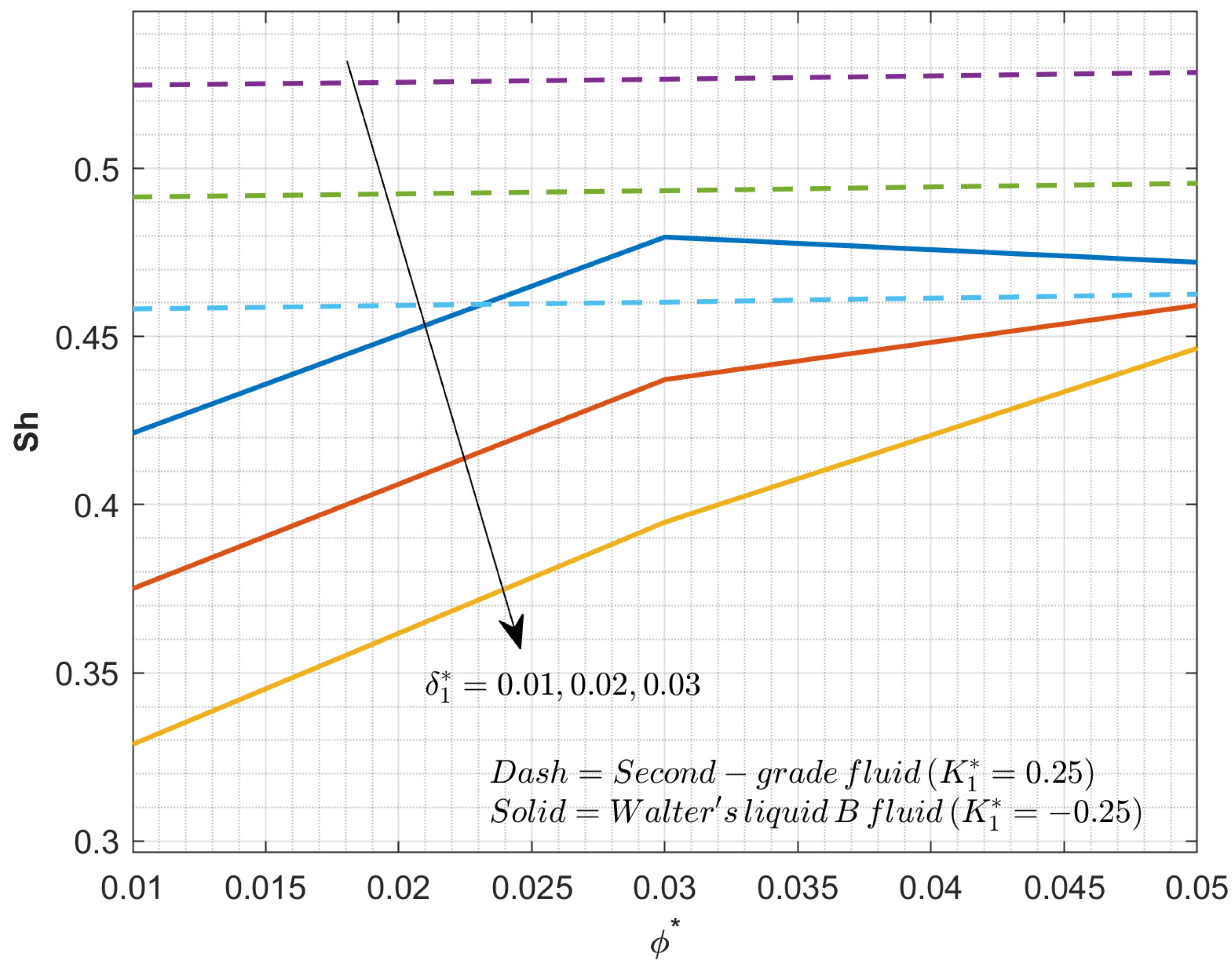
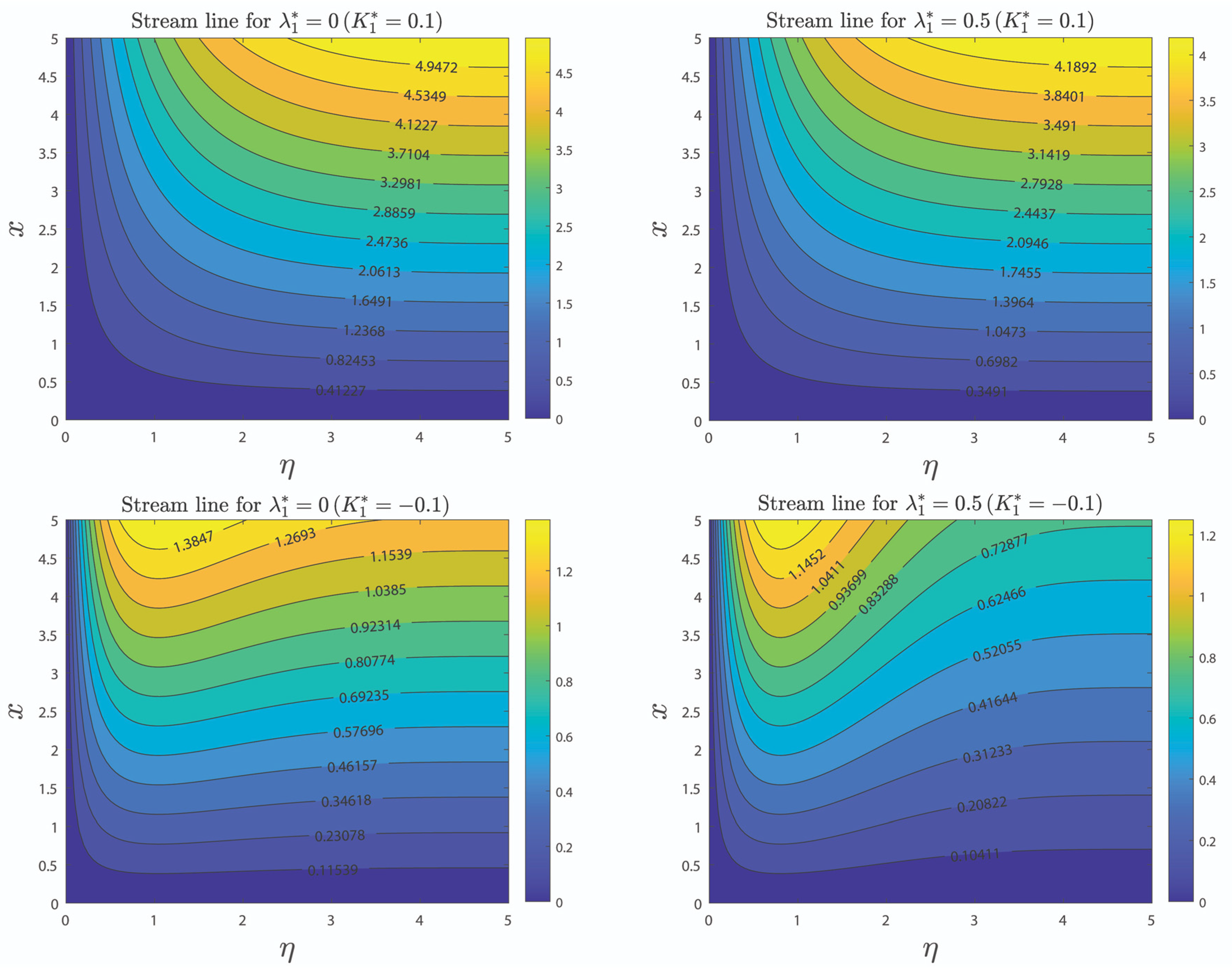
Sl. No	Parameter Definition	Parameter Name
01	$K_1^{*}=\frac{{\alpha }_1^{*}a}{{\rho }_f{\nu }_f}$	Viscoelastic constraint $K_1^{*}>0$ second-grade fluid $K_1^{*}<0$ Walter’s liquid B fluid
02	$Q_1^{*}=\frac{\pi j_0{M}_0}{8{\rho }_{f}a{U}_w}$	Modified Hartmann number
03	${\beta }_1^{*}=\sqrt{\frac{{\pi }^2{\nu }_f}{c^{2}a}}$	Parameter related to width and magnitude of electrodes
04	${\lambda }_1^{*}=\frac{{\nu }_f}{K^{*}a}$	Porous constraint
05	$R{d}_1^{*}=\frac{4{\sigma }^{*}{T}_{\infty }^3}{k^{*}{k}_f}$	Radiation constraint
06	$\mathrm{Pr}=\frac{{\nu }_f}{{\alpha }_f}$	Prandtl number
07	${\delta }_1^{*}=\frac{Q}{a(C_w-C_{\infty })}$	Parameter related to local pollutant external source
08	${\gamma }_1^{*}=b_3(C_w-C_{\infty })$	Parameter related to external pollutant source variation
09	$S{c}_1^{*}=\left(\frac{{\upsilon }_f}{D_f}\right)$	Schmidt number
10	$\mathrm{Re}=\frac{x^{2}a}{{\nu }_f}$	Local Reynolds number
11	$A_1^{*}={\left(1-{\varphi }^{*}\right)}^{2.5}$
12	$A_2^{*}=\left(1-{\varphi }^{*}+{\varphi }^{*}\frac{{\rho }_s}{{\rho }_f}\right)$
13	$A_3^{*}=\left(1-{\varphi }^{*}+{\varphi }^{*}\frac{{\left(\rho C_p\right)}_s}{{\left(\rho C_p\right)}_f}\right)$

.

The important engineering factor and its reduced form is provided as follows:

$$Cf=\frac{1}{{\rho }_f{U}_w^2}{\left({\mu }_{nf}\frac{\partial \overline{u}}{\partial y}\pm {\alpha }_1^{*}\left(\frac{{\partial }^2\overline{u}}{\partial x\partial y}2+\overline{v}\frac{{\partial }^2\overline{u}}{\partial y^2}+\frac{{\partial }^2\overline{u}}{\partial x\partial y}\overline{u}\right)\right)}_{y=0},$$

(12)

$$Nu=-\frac{x\left(k_{nf}+\frac{16{\sigma }^{*}{T}_{\infty }^3}{3k^{*}}\right){\left(\frac{\partial T^{*}}{\partial y}\right)}_{y=0}}{k_f\left(T_w-T_{\infty }\right)},$$

(13)

$$Sh=-\frac{x{D}_f{\left(\frac{\partial C}{\partial y}\right)}_{y=0}}{D_f\left(C_w-C_{\infty }\right)}.$$

(14)

Using the Equation (6) in (12)–(14) the following equations are attained:

$$Cf=-\frac{1}{\sqrt{\mathrm{Re}}}\left(f^{‴}(0)f(0)K_1^{*}-\left(\frac{1}{A_1}\pm 3K_1^{*}{f}^{\prime }(0)\right)f^{″}(0)\right),$$

(15)

$$Nu=-\left(\frac{k_{nf}}{k_f}+\frac{4}{3}R{d}_1^{*}\right){\theta }^{\prime }(0)\sqrt{\mathrm{Re}},$$

(16)

$$\frac{Sh}{\sqrt{\mathrm{Re}}}=-{\chi }^{\prime }(0).$$

(17)

3. Numerical Scheme

The transformed set of ODEs (8) to (10) and reduced boundaries, as stated in Equation (11), is challenging to obtain the analytical solution due to its high nonlinearity and two-point boundary nature. So, the solution of these equations is traced using the efficient numerical method. For this, we can convert the set of higher-order equations into first-order:

$$\begin{array}{l}\left\{f,f^{\prime },f^{″},f^{‴}\right\}=\left\{{\omega }_1,{\omega }_2,{\omega }_3,{\omega }_4\right\}\\ f^{⁗}=\frac{A_2^{*}}{K_1^{*}{\omega }_1}\left(\begin{array}{l}\frac{{\omega }_4}{A_1^{*}{A}_2^{*}}\pm \frac{K_1^{*}}{A_2^{*}}\left[2{\omega }_2{\omega }_4-{({\omega }_3)}^2\right]+\frac{Q_1^{*}}{A_2^{*}}\exp (-\eta {\beta }_1^{*})-\frac{{\lambda }_1^{*}}{A_1^{*}{A}_2^{*}}{\omega }_2\\ +{\omega }_1{\omega }_3-{({\omega }_2)}^2\end{array}\right)\end{array},$$

(18)

$$\begin{array}{l}\left\{\theta ,{\theta }^{\prime }\right\}=\left\{{\omega }_5,{\omega }_6\right\}\\ {\theta }^{″}=-\mathrm{Pr}{A}_3^{*}\frac{{\omega }_1{\omega }_6}{\left[\frac{k_{nf}}{k_f}+\frac{4}{3}R{d}_1^{*}\right]}\end{array},$$

(19)

$$\begin{array}{l}\left\{\chi ,{\chi }^{\prime }\right\}=\left\{{\omega }_7,{\omega }_8\right\}\\ {\chi }^{″}=-\left({\omega }_1{\omega }_8+{\delta }_1^{*}\exp ({\gamma }_1^{*}{\omega }_7)\right)S{c}_1^{*}\end{array},$$

(20)

and the BCs becomes

$$\begin{array}{l}{\omega }_1(0)=0,{\omega }_2(0)=1,{\omega }_3(0)={\epsilon }_1,\\ {\omega }_4(0)={\epsilon }_2,{\omega }_5(0)=1,{\omega }_6(0)={\epsilon }_3,\\ {\omega }_7(0)=1,{\omega }_8(0)={\epsilon }_4.\end{array}.$$

(21)

The above equations are obtained using RKF-45 (Runge Kutta Fehlberg’s 4th–5th order), and the missing boundary values in Equation (20) are obtained via the shooting procedure. The values of the parameters are set to $Q_1^{*}=0.01, {\beta }_1^{*}=0.1, {\lambda }_1^{*}=0.1, R{d}_1^{*}=0.1, {\gamma }_1^{*}={\delta }_1^{*}=0.01,$ and $S{c}_1^{*}=0.8$; using the thermophysical characteristics and properties stated in

Table 2. List of thermophysical characteristics of nanofluids.

Thermophysical Characteristics	Name
${\left(\rho C_p\right)}_{nf}=\left(\left(1-{\varphi }^{*}\right)+{\varphi }^{*}\frac{{\left(\rho C_p\right)}_s}{{\left(\rho C_p\right)}_f}\right){\left(\rho C_p\right)}_f$	Specific heat capacity
${\mu }_{nf}=\frac{{\mu }_f}{{\left(1-{\varphi }^{*}\right)}^{2.5}}$	Dynamic viscosity
$k_{nf}=\frac{\left(k_s+2{\varphi }^{*}{k}_s+2k_f(1-{\varphi }^{*})\right)k_f}{k_s-{\varphi }^{*}{k}_s+k_f(2+{\varphi }^{*})}$	Thermal conductivity
${\rho }_{nf}=\left(1-{\varphi }^{*}+{\varphi }^{*}\frac{{\rho }_s}{{\rho }_f}\right){\rho }_f$	Density

and

Table 3. List of thermophysical properties of nanofluids (see [43,44]).

Properties	$\mathbf{Pr}$	${\mathit{C}}_{\mathit{p}} \mathbf{\left(}\mathbf{J}\mathbf{k}{\mathbf{g}}^{\mathbf{-}\mathbf{1}}{\mathbf{K}}^{\mathbf{-}\mathbf{1}}\mathbf{\right)}$	$\mathit{k} \mathbf{\left(}\mathbf{k}\mathbf{g}\mathbf{m}{\mathbf{s}}^{\mathbf{-}\mathbf{3}}{\mathbf{K}}^{\mathbf{-}\mathbf{1}}\mathbf{\right)}$	$\mathit{\rho } \mathbf{\left(}\mathbf{k}\mathbf{g}{\mathbf{m}}^{\mathbf{-}\mathbf{3}}\mathbf{\right)}$
$SA\left({\mathrm{C}}_6{\mathrm{H}}_9\mathrm{N}\mathrm{a}{\mathrm{O}}_7\right)$	$6.45$	$4175$	$0.6376$	$989$
$\mathrm{T}\mathrm{i}{\mathrm{O}}_2$	-	$686.2$	$8.9528$	$4250$

, the numerical solutions are obtained using 0.01 as a step size and 10⁻⁶ set for tolerance of error. Further, our present numerical scheme is validated by the works of [40,41,42] for some limiting parameters and obtained good agreement (see

Table 4. Assessment of numerical values $-{\theta }^{\prime }{\left(\eta \right)}_{\eta =0}$ for variation in $\mathrm{Pr}$ with the absence of ${\lambda }_1^{*}$, $K_1^{*}$, $R{d}_1^{*}$, ${\varphi }^{*}$, and $Q_1^{*}$.

Pr	Ishak et al. [42]	Abolbashari et al. [43]	Das et al. [44]	Present Numerical Outcome
0.72	0.8086	0.80863135	0.80876122	0.80876153
1.0	1.0000	1.00000000	1.00000000	1.00000000
3.0	1.9237	1.92368259	1.92357431	1.92357446
7.0	3.0723	3.07225021	3.07314679	3.07314636
10	3.7207	3.72067390	3.72067390	3.72067335

).

The numerical flowchart of the present numerical scheme is presented in Figure 2.

4. Results and Discussion

The present section explains the influence of various dimensionless constraints on their profiles. The analysis is made using the comparison of two different kinds of non-Newtonian nanoliquids in the presence of various factors. Further, the important engineering factors are analyzed and deliberated in detail.

Figure 3 illustrates the impact of the $K_1^{*}$ (viscoelastic constraint) over $f^{\prime }$ (velocity) profile. The $K_1^{*}$ plays a significant role in identifying the behavior of the liquids, especially in second-grade fluid and Walter’s liquid B fluid. The positive values of $K_1^{*}$ will denotes the second-grade fluid. The improvement in positive $K_1^{*}$ values will significantly improve the $f^{\prime }$ profile. Second-grade fluid displayed shear-thinning behavior, which means the viscosity falls as the shear rate improves. As a result, increasing $K_1^{*}$ improves the liquid’s capacity to move more freely, leading to greater velocities. The negative values of $K_1^{*}$ signify the Walter’s liquid B fluid. The improvement in negative values $K_1^{*}$ will decline the velocity. Walter’s liquid B fluid has a more complicated behavior, with larger negative values of $K_1^{*}$ resulting in a greater viscosity and, thus, decreased velocity.

Figure 4 shows the variation in the velocity profile for improved values of the porous factor for both second-grade fluid and Walter’s liquid B fluid cases. The improved values of porous factor will reduce the fluid velocity in both the cases. The rise in porous factor will exhibit the resistance caused by the porous surface which acts as a barrier to the flow of liquid. Further, the porous medium will promote the interaction between the liquid and surface of the object which improves the thickness of the boundary layer. It is observed that velocity is more in second-grade fluid than Walter’s liquid B fluid in the presence of the porous factor.

Figure 5 illustrates the impact of the modified Hartmann number $Q_1^{*}$ over the $f^{\prime }$ profile for second-grade fluid and Walter’s liquid B fluid scenarios. The improvement in $Q_1^{*}$ will improve the velocity profile in the second-grade fluid case while the reverse behavior is seen in the case of Walter’s liquid B fluid. In case the of second-grade fluid, improved $Q_1^{*}$ will improve the external electrical impact over the liquid which improves the ability of the circulation, while in Walter’s liquid B fluid an improved $Q_1^{*}$ will improve the viscosity of the liquid due to an improved electrical field, making it more resistant to movement. In the presence of $Q_1^{*}$ the two liquid models behave differently over the velocity profile.

The variation in the thermal $\theta$ profile for changing values of the radiation $R{d}_1^{*}$ parameter is illustrated in Figure 6. The improved values of $R{d}_1^{*}$ will exhibit enhancement in thermal distribution. The adoption of radiation constraints reduces the $k^{*}$, which improves the radiative thermal flux coefficients and radiative heat transmission rates into the liquid. Because of the increased radiative thermal transmission, the extent of the thermal boundary layer is noticeable. For the given radiation, Rosseland’s diffusion manner raises the temperature of the fluid. It is observed that Walter’s liquid B fluid shows greater thermal distribution than second-grade fluid. The Walter’s liquid B fluid will exhibit higher thermal conductivity as well as thermal diffusivity when compared to second-grade fluid. These properties of Walter’s liquid B fluid result in more distribution of heat in the fluid system within the presence of $R{d}_1^{*}$.

The impact of external pollutant source variation factor ${\gamma }_1^{*}$ over the concentration $\chi$ profile is drawn in the Figure 7. The rise in ${\gamma }_1^{*}$ will enhances the concentration in both second-grade fluid and Walter’s liquid B fluid cases. As ${\gamma }_1^{*}$ improves, it releases more pollutants into the liquid. The enhancement in the impurities will intensify the concentration in the liquid. The Walter’s liquid B fluid shows a higher concentration curve than second-grade fluid. Walter’s liquid B fluid will exhibit higher viscosity, which decreases the dispersion, and slower dissolve of pollutants than second-grade fluid.

Figure 8 shows the impact of ${\varphi }^{*}$ on $Cf$ over various values of ${\lambda }_1^{*}$. The improvement in these two factors will decline $Cf$. The results show that Walter’s liquid B fluid exhibits less surface drag force than second-grade fluid in the presence of these two parameters. As ${\lambda }_1^{*}$ improves it will offer additional resistance to the liquid flow and acts as a barrier; the addition of ${\varphi }^{*}$ will enhance the thickness of the boundary layer. Due to its higher viscosity and higher tendency for the aggregation of particles, Walter’s liquid B fluid will experience lower $Cf$ than second-grade fluid.

The influence of ${\varphi }^{*}$ on $Nu$ over various values of $R{d}_1^{*}$ is shown in Figure 9. The rise in the values of ${\varphi }^{*}$ and $R{d}_1^{*}$ will enhance the thermal distribution rate. The Figure 9 shows second-grade fluid will exhibit a higher rate of thermal distribution than Walter’s liquid B fluid. $R{d}_1^{*}$ exchanges energy among the outermost layer and the surroundings via electromagnetic waves. As a result, an increase in $R{d}_1^{*}$ improves this heat exchange process, resulting in a faster rate of heat transmission. Furthermore, as ${\varphi }^{*}$ grows, additional impediments and disruptions in the fluid flow are introduced. This improved contact between liquid and solid particles improves convective heat transfer, resulting in a faster rate of heat transfer at the surface.

Figure 10 display the impact of ${\varphi }^{*}$ on $Sh$ over various values of ${\delta }_1^{*}$. The improvement in the values of ${\varphi }^{*}$ and ${\delta }_1^{*}$ will decline the rate of mass transfer. Surface mass transfer is affected by alterations in external pollution sources. Higher values of ${\delta }_1^{*}$ result in increasing surface-level pollution or more rapid change. Pollutant concentration distributions within the liquid become more pronounced, which slows down the mass transfer rate. More impediments and disruptions are introduced into the fluid flow as the solid volume percentage rises. Because of the increased contact between liquid and solid particles, the surface transfer of mass to contaminants is slowed down. Further from the diagram, it is clear that second-grade fluid will exhibit a lower rate of mass distribution than Walter’s liquid B fluid. Figure 11 shows the streamline pattern for second-grade fluid and Walter’s liquid B fluid in the presence and absence of ${\lambda }_1^{*}$.

Table 5. Comparison table of rate of mass transfer percentage for second-grade fluid and Walter’s liquid B fluid in the presence of ${\delta }_1^{*}$ and ${\gamma }_1^{*}$.

Parameter	Values	Second-Grade Fluid	Walter’s Liquid B Fluid
		$\left|\frac{\mathit{S}{\mathit{h}}_{{\mathit{\varphi }}^{*}=0.01}-\mathit{S}{\mathit{h}}_{{\mathit{\varphi }}^{*}=0}}{\mathit{S}{\mathit{h}}_{{\mathit{\varphi }}^{*}=0}}\right|\times 100$	$\left|\frac{\mathit{S}{\mathit{h}}_{{\mathit{\varphi }}^{*}=0.01}-\mathit{S}{\mathit{h}}_{{\mathit{\varphi }}^{*}=0}}{\mathit{S}{\mathit{h}}_{{\mathit{\varphi }}^{*}=0}}\right|\times 100$
${\delta }_1^{*}$	0.01	0.140264%	0.560122%
	0.02	0.140338%	0.570178%
	0.03	0.161919%	0.601998%
${\gamma }_1^{*}$	0.1	0.140853%	0.650966%
	0.2	0.122659%	0.631004%
	0.3	0.123269%	0.648976%

shows the percentagewise changes in the rate of mass transfer in both second-grade fluid and Walter’s liquid B fluid with respect to changes in the values of ${\delta }_1^{*}$ and ${\gamma }_1^{*}$. With the rise in the values of ${\delta }_1^{*}$ from 0.01 to 0.03, the rate of mass distribution in the second-grade fluid case will rise from 0.140264% to 0.161919%, while in Walter’s liquid B fluid it improves from 0.560122% to 0.601998%. In a similar manner, with improved values of ${\gamma }_1^{*}$, the rate of mass transfer percentage will decrease from 0.140853% to 0.123269% while in the Walter’s liquid B fluid case it is 0.650966% to 0.648976%. Comparing these two liquids, the rate of mass transfer percentage is more in the presence of the nanoparticle than in its absence, and Walter’s liquid B fluid shows a greater percentage of rate of mass transfer for these two constraints.

5. Final Remarks

The analysis and discussion of various dimensionless constraints and their influences on flow and thermal and mass transfer in the present investigation will provide valuable insights to the field of environmental protection and management and various engineering areas. The results focus on important factors like the viscoelastic parameter, porosity parameter, radiation, external pollutant source variation parameters, solid volume fraction, and modified Hartmann numbers on their respective profiles. Furthermore, a comparative analysis is made between the second-grade fluid and Walter’s liquid B fluid to exhibit their characteristics in the presence of these factors. The major outcomes reveal that an improved porous factor will decline the velocity in both of the fluid cases, while in the presence of the Hartmann number velocity improves in the second-grade fluid case while the opposite trend is observed in the Walter’s liquid B fluid case. Thermal distribution and concentration improve with rises in radiation and external pollutant source variation parameters. The addition of volume fraction and porosity will reduces the surface drag force. In the presence of the radiation factor, the rate of thermal distribution will rise. Local pollutant external source parameter and solid volume fractions will decline the rate of mass transfer. The rate of mass transfer percentage will increase by 0.140264% to 0.161919% for the case of second-grade fluid in the presence of nanoparticles. However, for the case of Walter’s liquid B fluid, it is upsurges at around 0.560122% to 0.601998% for the local pollutant external source parameter, but the reverse trend is observed for the external pollutant source variation parameter.

These findings help to improve knowledge of pollution management, temperature transportation, and the transfer of mass optimization, allowing for better decision-making and effective planning to safeguard the environment and sustainable engineering practices. The present work can be extended to examine the different non-Newtonian nanofluids with different geometries and physical aspects.