Numerical Simulation of Tidal Current and Sediment Movement in the Sea Area near Weifang Port
Abstract
:1. Introduction
2. Numerical Models
2.1. Hydrodynamic Model
2.2. Wave Model
2.3. Suspended-Sediment-Transport Model
2.3.1. Sediment-Settling Velocity
2.3.2. Bottom Reference Concentration
2.3.3. Sediment-Diffusion Coefficient
2.4. Model Coupling
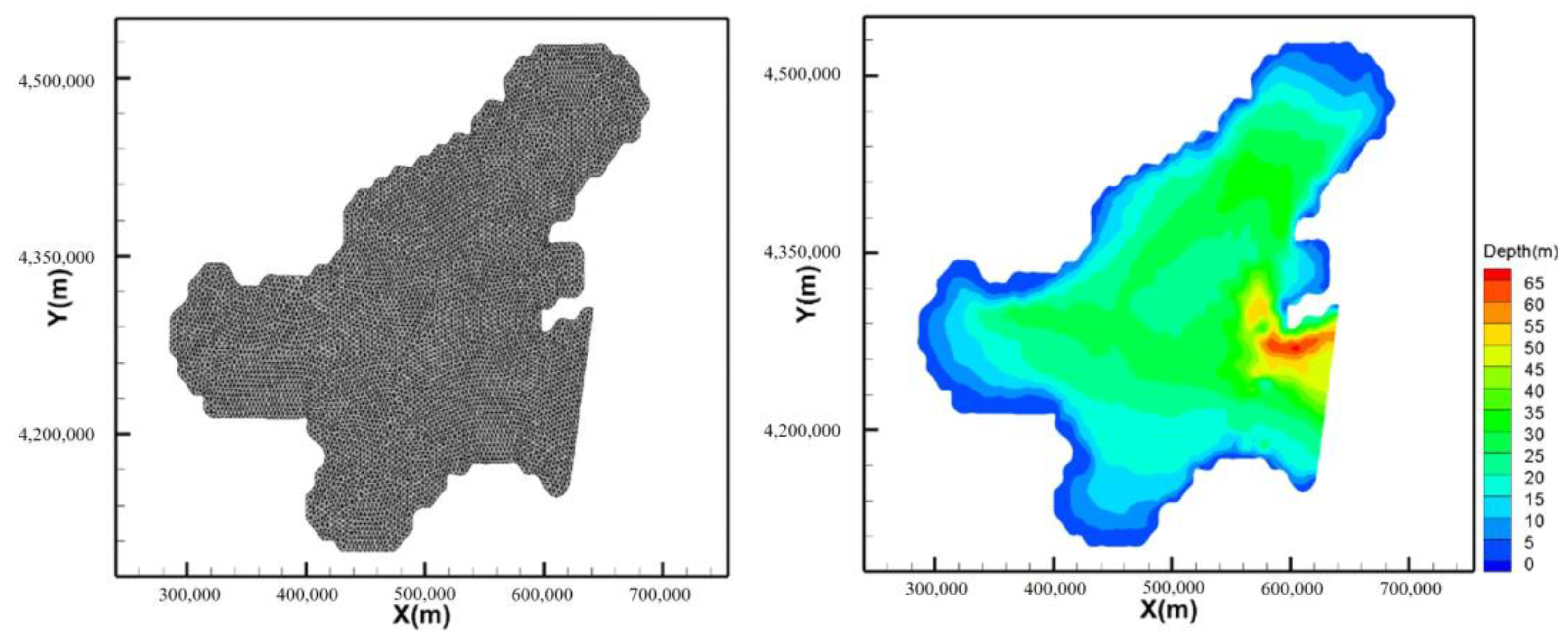
3. Study Area and Model Settings
3.1. Study Area
3.2. Model Settings
4. Model Results and Analysis
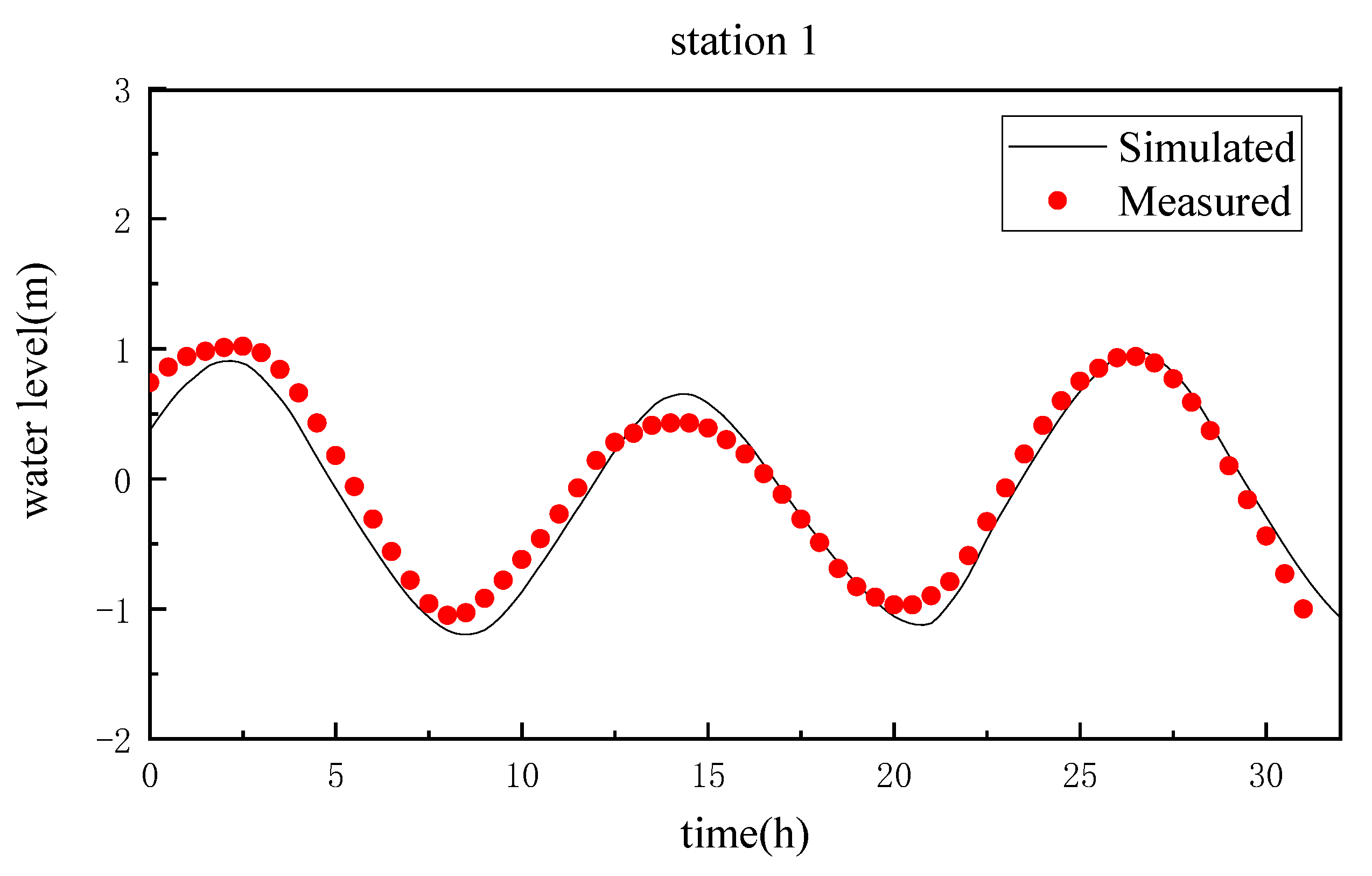
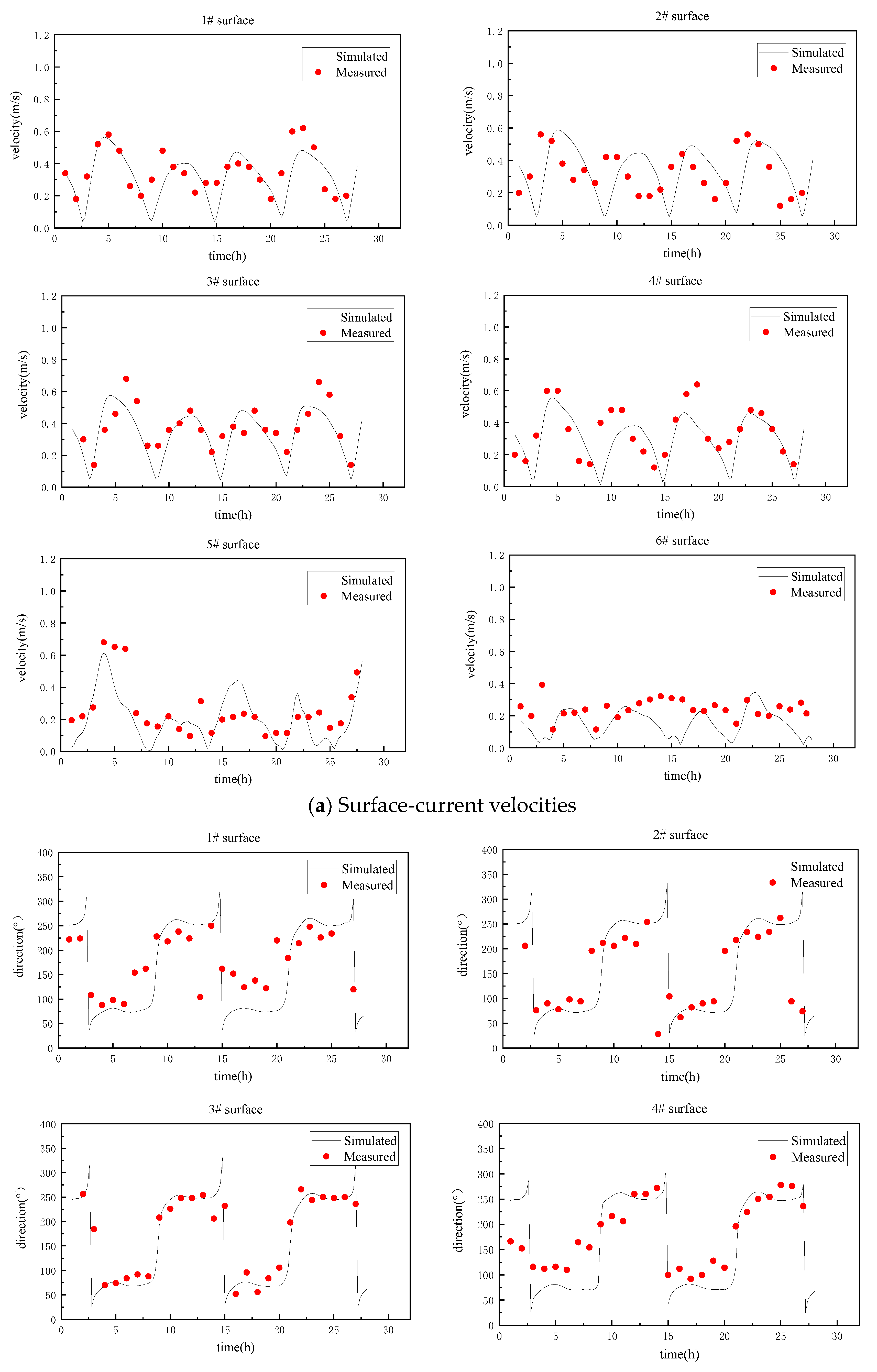
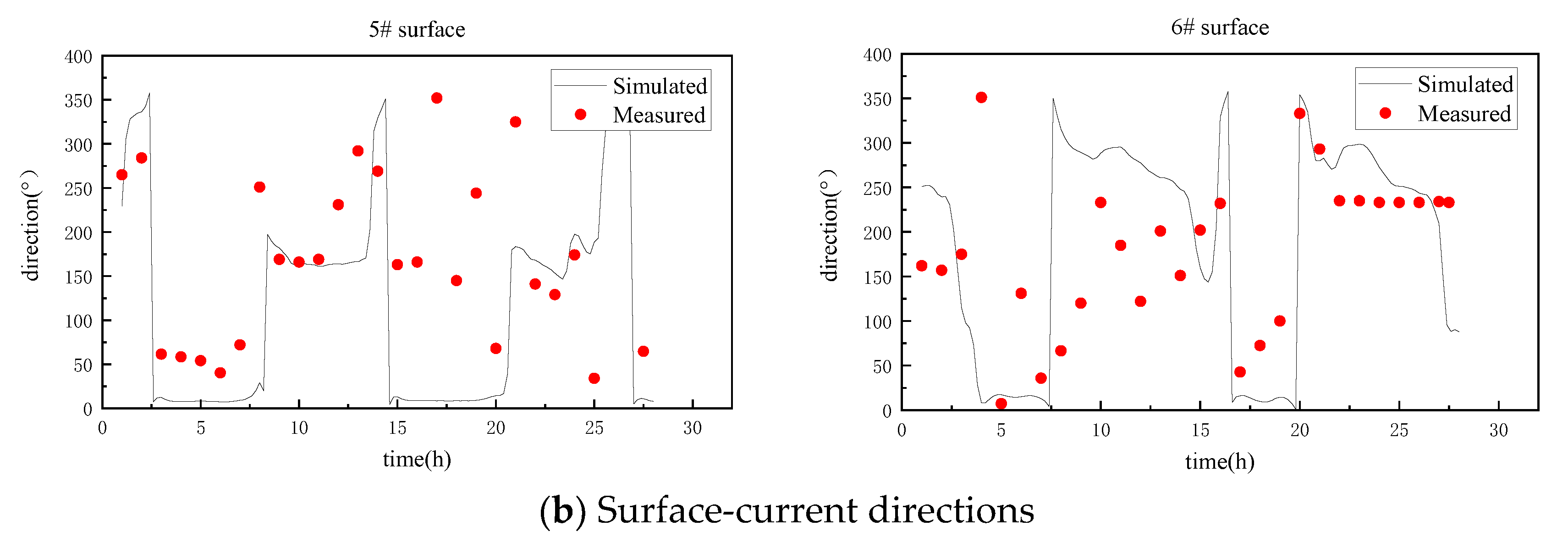
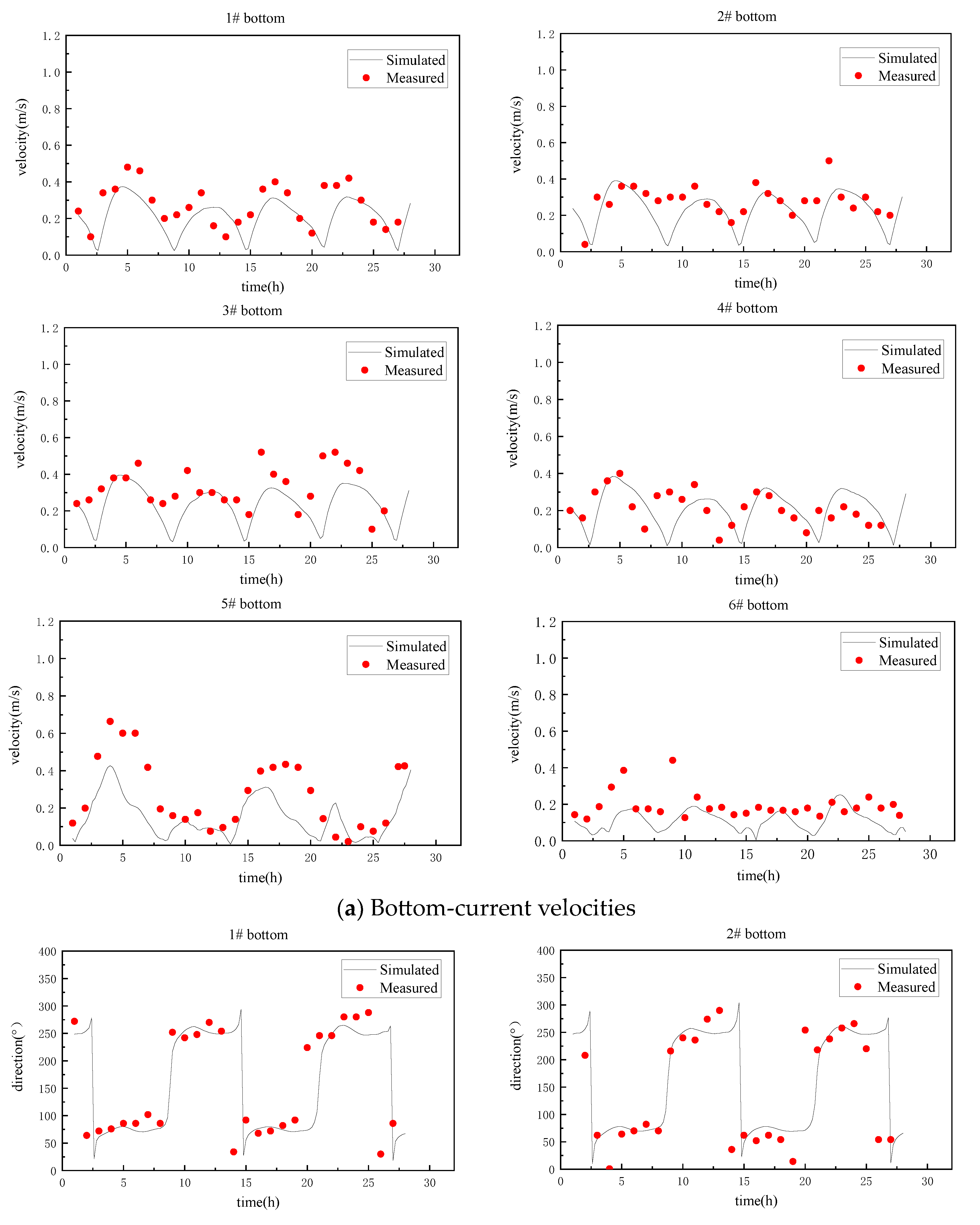
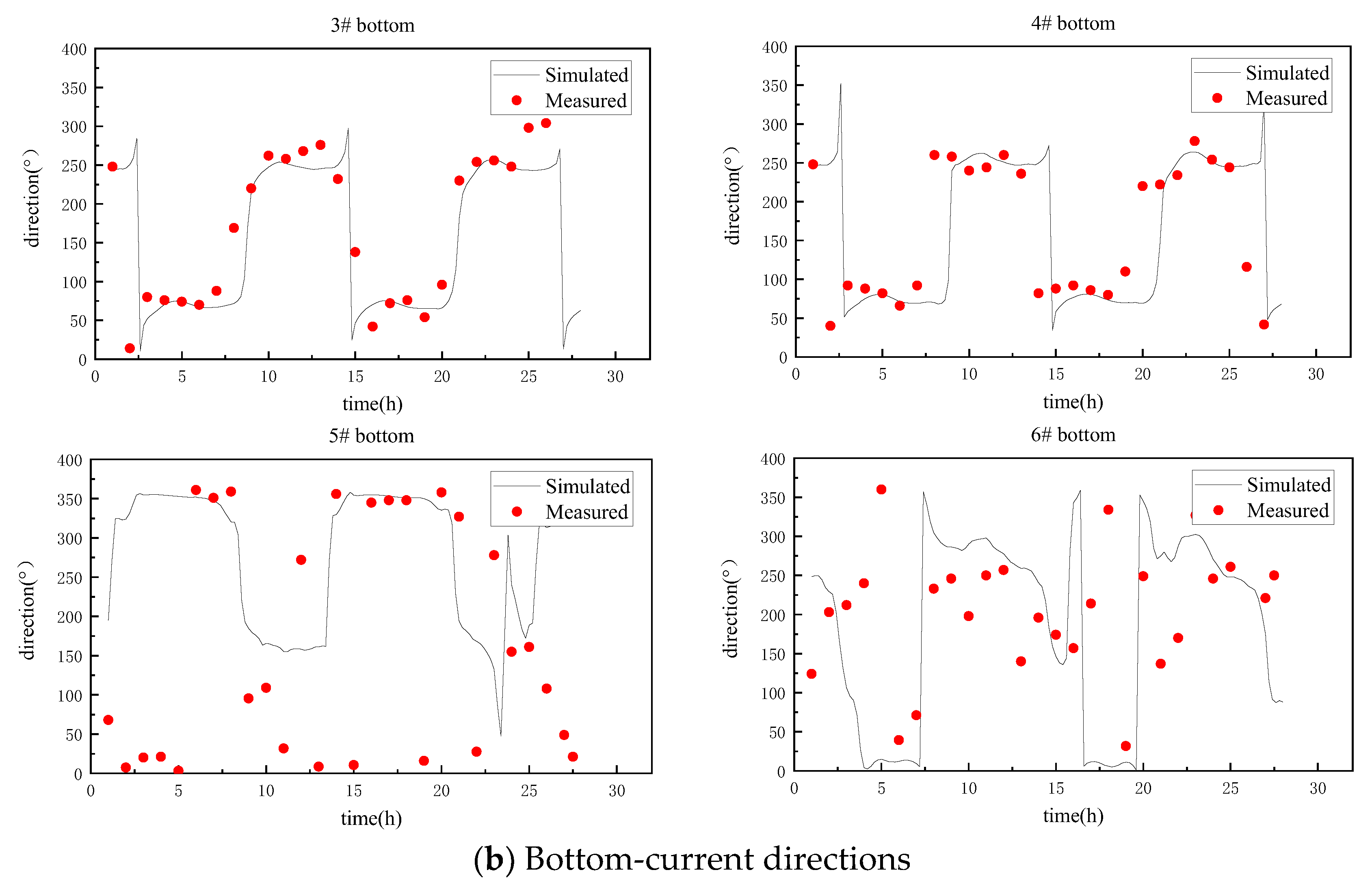
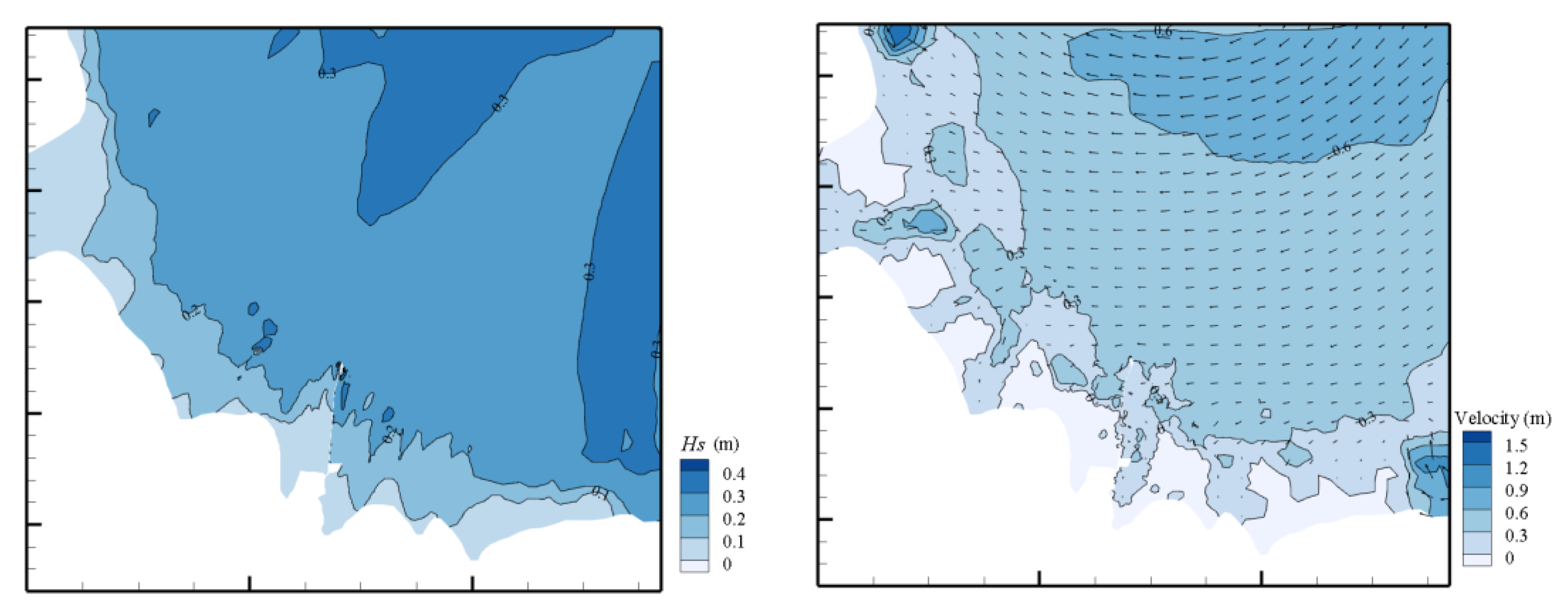
4.1. Tide Elevation and Tidal-Current Verification
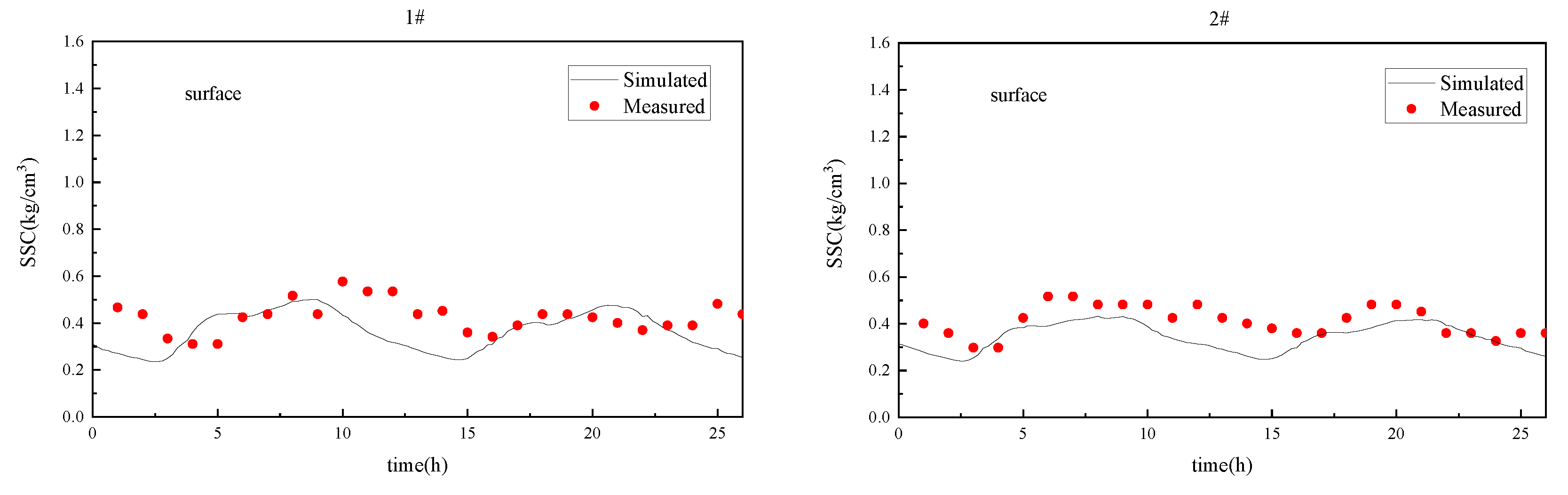
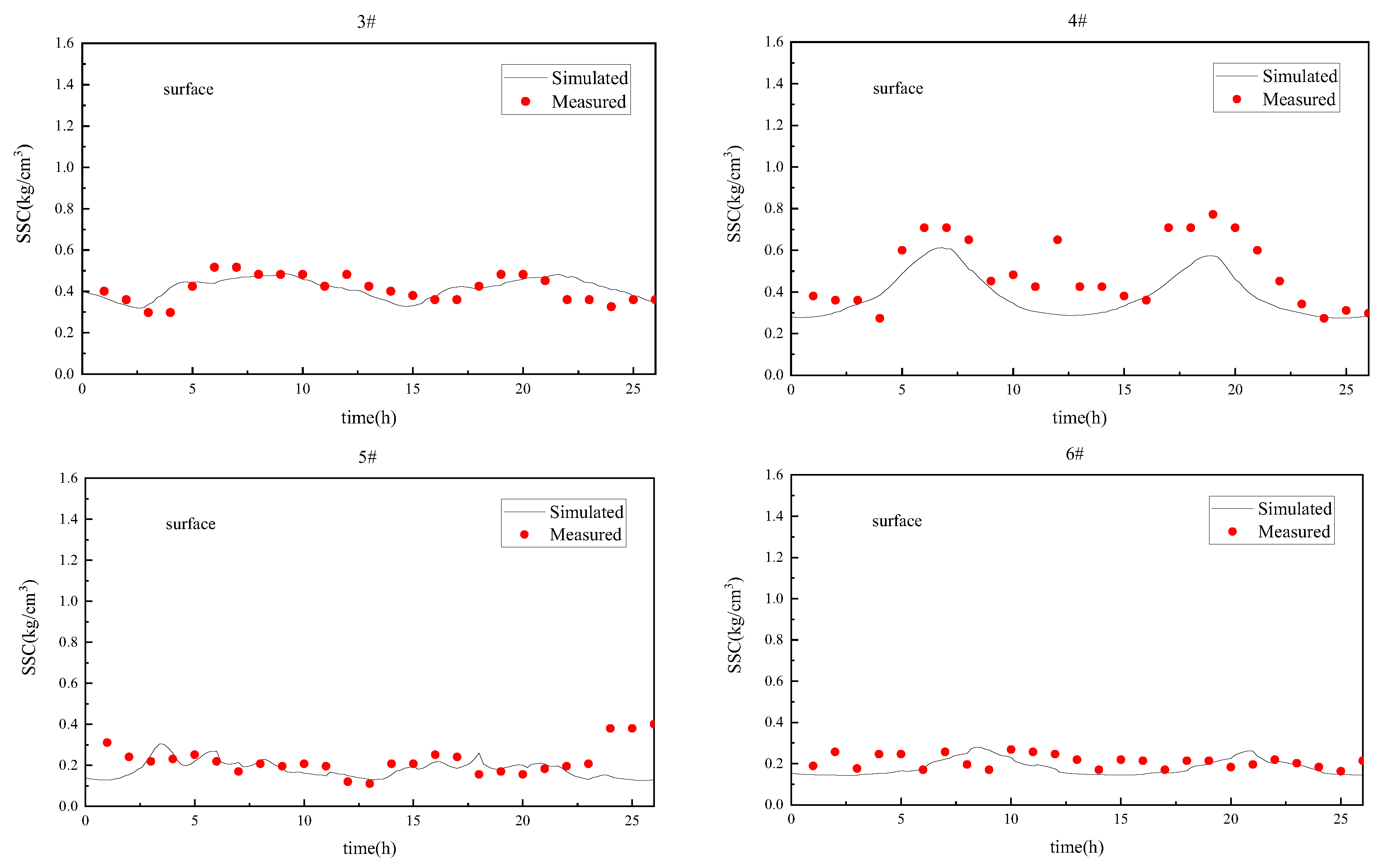
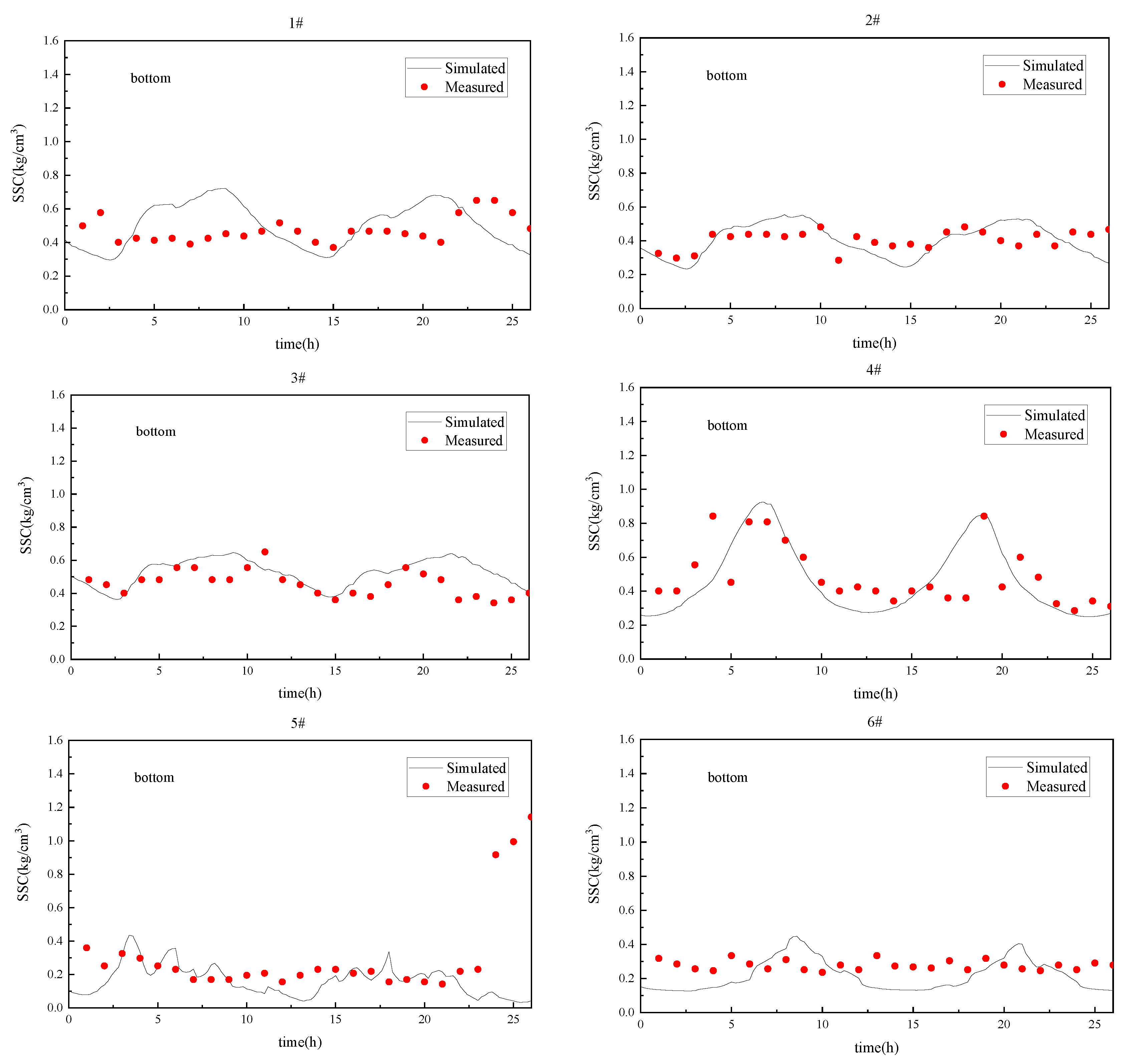
4.2. Suspended Sediment Concentration Verification
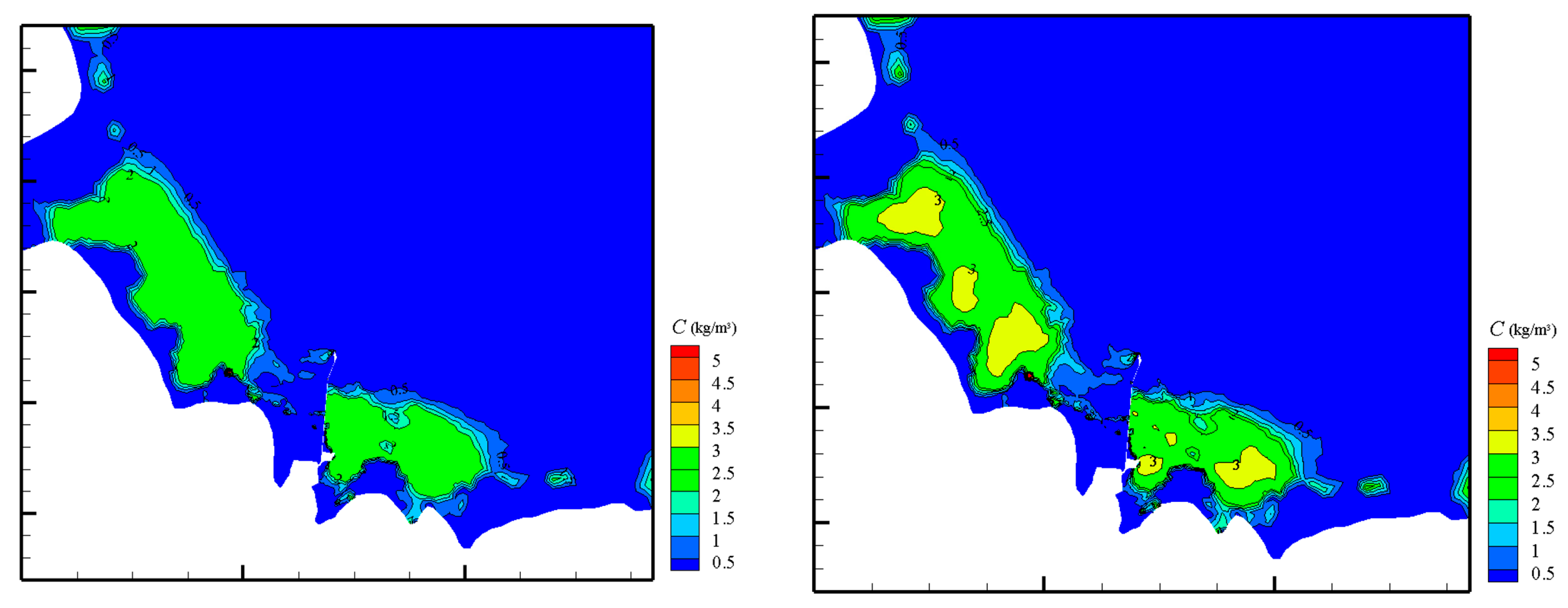
4.3. Sediment-Content Comparison
5. Conclusions
- (1)
- After introducing settling-velocity Formula (6), the overall settling velocity of the sediment decreased. The higher the sediment concentration is, the more the settling velocity is tempered. The sediment in the bottom water body was more highly concentrated than that in the surface water body. The SSC in the bottom layer was high and fluctuated more. After the introduction of settling-velocity Formula (6), the model fitted the measured data better. Hence, the model can effectively describe the sediment-movement process in the sea area near Weifang Port.
- (2)
- The SSCs simulated by settling-velocity Formula (6) were higher than those simulated by settling-velocity Formula (3), and the SSCs simulated by the two formulas differed more when the current velocity was faster. With settling-velocity Formula (6), the overall settling velocity of the sediment was slower than that simulated by settling-velocity Formula (3). When the current velocity was high, more sediment was suspended, the suspended sediment settled less easily, and the SSCs in the water body increased and fluctuated more.
- (3)
- For the SSC field in the sea area of Weifang Port, the nearshore SSCs calculated by settling-velocity Formula (6) were higher than those calculated by settling-velocity Formula (3). Specifically, the nearshore SSCs in the surface and bottom layers calculated by settling-velocity Formula (6) were approximately 1 kg/m3 and 2 kg/m3 higher, respectively than those calculated by settling-velocity Formula (3). In practical engineering applications, the SSCs calculated by a settling-velocity formula considering gradation will be even higher, so a construction scheme with a higher safety factor is recommended for the study area.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Warner, J.C.; Sherwood, C.R.; Signell, R.P.; Harris, C.K.; Arango, H.G. Development of a three-dimensional, regional, coupled wave, current, and sediment-transport model. Comput. Geosci. 2008, 34, 1284–1306. [Google Scholar] [CrossRef]
- Lesser, G.R.; Roelvink, J.A.; Kester, J.; Stelling, G.S. Development and validation of a three-dimensional morphological model. Coast. Eng. 2004, 51, 883–915. [Google Scholar] [CrossRef]
- Blumberg, A.F. A Primer for ECOMSED Version 1.3, Users Manual; HydroQual Inc.: Mahwah, NJ, USA, 2002. [Google Scholar]
- Sauvaget, P.; David, E.; Demmerle, D.; Lefort, P. Optimum design of large flood relief culverts under the A89 motorway in the Dordogne—Isle confluence plain. Hydrol. Process. 2000, 14, 2311–2329. [Google Scholar] [CrossRef]
- Zhang, Y.; Baptista, A.M. SELFE: A Semi-implicit Eulerian-Lagrangian Finite-Element model for cross-scale ocean circulation. Ocean. Model. 2008, 21, 71–96. [Google Scholar] [CrossRef]
- Chen, C.S.; Liu, H.; Beardsley, R.C. An Unstructured Grid, Finite-Volume, Three-Dimensional, Primitive Equations Ocean Model: Application to Coastal Ocean and Estuaries. J. Atmos. Ocean. Technol. 2003, 20, 159–186. [Google Scholar] [CrossRef]
- Booij, N.; Ris, R.C.; Holthuijsen, L.H. A third-generation wave model for coastal regions: 1. Model description and validation. J. Geophys. Res. 1999, 104, 7649–7666. [Google Scholar] [CrossRef] [Green Version]
- Tolman, H.L. User Manual and System Documentation of WAVEWATCH-III Version 3.14; NOAA/NWS/NCEP/MMAB Technical Note; U.S. Department of Commerce: Washington, DC, USA, 2009. [Google Scholar]
- Wang, J.H. Development and Application of a Three-Dimensional Unstructured Fully Coupled Wave-Current Model. Ph.D. Thesis, Dalian University of Technology, Dalian, China, 2011. [Google Scholar]
- Yang, J.S. Development and Application of a Dynamically Coupled Wave-Current Model. Master’s Thesis, Tianjin University, Tianjin, China, 2012. [Google Scholar]
- Dietrich, J.C.; Tanaka, S.; Westerink, J.J.; Dawson, C.N.; Luettich, R.A.; Zijlema, M.; Holthuijsen, L.H.; Smith, J.M.; Westerink, L.G.; Westerink, H.J. Performance of the Unstructured-Mesh, SWAN+ADCIRC Model in Computing Hurricane Waves and Surge. J. Sci. Comput. 2012, 468–497. [Google Scholar] [CrossRef]
- Luo, J. Numerical Modelling of Hydrodynamics, Sand Transport and Long-Term Morphology in Liverpool Bay. Ph.D. Thesis, Zhejiang University, Zhejiang, China, 2015. [Google Scholar]
- Priya, K.L.; Jegathambal, P.; James, E.J. On the factors affecting the settling velocity of fine suspended sediments in a shallow estuary. J. Oceanogr. 2015, 71, 163–175. [Google Scholar] [CrossRef]
- Wang, Q.; Pan, C. Three-dimensional modelling of sediment transport under tidal bores in the Qiantang estuary, China. J. Hydraul. Res. 2018, 56, 662–672. [Google Scholar] [CrossRef]
- Stokes, G.G. On the Effect of the Internal Friction of Fluids on the Motion of Pendulums, 2nd ed.; Johnson Reprint Corp.: New York, NY, USA, 1851. [Google Scholar]
- Oseen, C.W. Ueber die Stokes’ sche Formel, und uber eine verwandte Aufgabe in der Hydrodynamik. Ark. Math. Astronom. Fys. 1910, 6, 154–160. [Google Scholar]
- Krone, R.B. Flume Study of the Transport of Sediment in Estuarial Processes: Final Report; Hydraulic Engineering Laboratory and Sanitary Engineering Research Laboratory, University of California: Berkeley, CA, USA, 1962. [Google Scholar]
- van Rijn, L.C. Sediment Transport, Part II: Suspended Load Transport. J. Hydraul. Eng. 1984, 110, 1613–1641. [Google Scholar] [CrossRef]
- Soulsby, R.L.; Manning, A.J.; Spearman, J.; Whitehouse, R. Settling velocity and mass settling flux of flocculated estuarine sediments. Mar. Geol. 2013, 339, 1–12. [Google Scholar] [CrossRef] [Green Version]
- Cheng, N.S. Simplified Settling Velocity Formula for Sediment Particle. J. Hydraul. Eng. 1997, 123, 149–152. [Google Scholar] [CrossRef]
- Richardson, J.F.; Zaki, W.N. The sedimentation of a suspension of uniform spheres under conditions of viscous flow. Chem. Eng. Sci. 1954, 3, 65–73. [Google Scholar] [CrossRef]
- Camenen, B. Chapter 15 Settling velocity of sediments at high concentrations. Proc. Mar. Sci. 2008, 9, 211–226. [Google Scholar] [CrossRef]
- te Slaa, S.; Van Maren, D.S.; He, Q.; Winterwerp, J.C. Hindered Settling of Silt. J. Hydraul. Eng. 2015, 141, 04015020. [Google Scholar] [CrossRef]
- Fang, H.W.; Chen, M.H.; Chen, Q.H. One-dimensional numerical simulation of non-uniform sediment transport under unsteady flows. Int. J. Sediment. Res. 2008, 23, 316–328. [Google Scholar] [CrossRef]
- Molinas, A.; Wu, B. Effect of Size Gradation on Transport of Sediment Mixtures. J. Hydraul. Eng. 1998, 124, 786–793. [Google Scholar] [CrossRef]
- Wu, B.; Molinas, A.; Julien, P.Y. Bed-Material Load Computations for Nonuniform Sediments. J. Hydraul. Eng. 2004, 130, 1002–1012. [Google Scholar] [CrossRef]
- Smart, G.; Jaeggi, M.N.R. Sediment Transport on Steep Slopes; Eidgenössische Technische Hochschule Zürich: Zurich, Switzerland, 1983; p. 191. [Google Scholar]
- Shen, H.W.; Rao, C.X. Transport of Uniform and Nonuniform Sediment Sizes. In Proceedings of the 5th Federal Interagency Sedimentation Conference, Las Vegas, NV, USA, 18–21 March 1991; pp. 162–169. [Google Scholar]
- Sun, Z.L.; Zheng, H.L.; Xu, D.; Hu, C.H.; Zhang, C.F. Vertical concentration profile of nonuniform sediment. Int. J. Sediment. Res. 2021, 36, 120–126. [Google Scholar] [CrossRef]
- Ji, C. 3D Numerical Modelling of Sediment Transport and Morphological Evolution due to Coupled Wave-Current. Ph.D. Thesis, Tianjin University, Tianjin, China, 2019. [Google Scholar]
- Jing, Y.G. Study on Settling Characteristics of Silt and Its Application. Master’s Thesis, Tianjin University, Tianjin, China, 2023. [Google Scholar]
- van Rijn, L.C. Unified view of sediment transport by currents and waves suspended transport. J. Hydraul. Eng. 2007, 133, 668–689. [Google Scholar] [CrossRef]
- Yao, P.; Su, M.; Wang, Z.; Van Rijn, L.C.; Zhang, C.; Chen, Y.; Stive, M. Experiment inspired numerical modeling of sediment concentration over sand–silt mixtures. Coast. Eng. 2015, 105, 75–89. [Google Scholar] [CrossRef]
- Yang, G.Y. Vertical Distribution of Silt Suspension Concentration with Stratification Effect due to Combined Waves and Currents and Its Application. Master’s Thesis, Tianjin University, Tianjin, China, 2022. [Google Scholar]



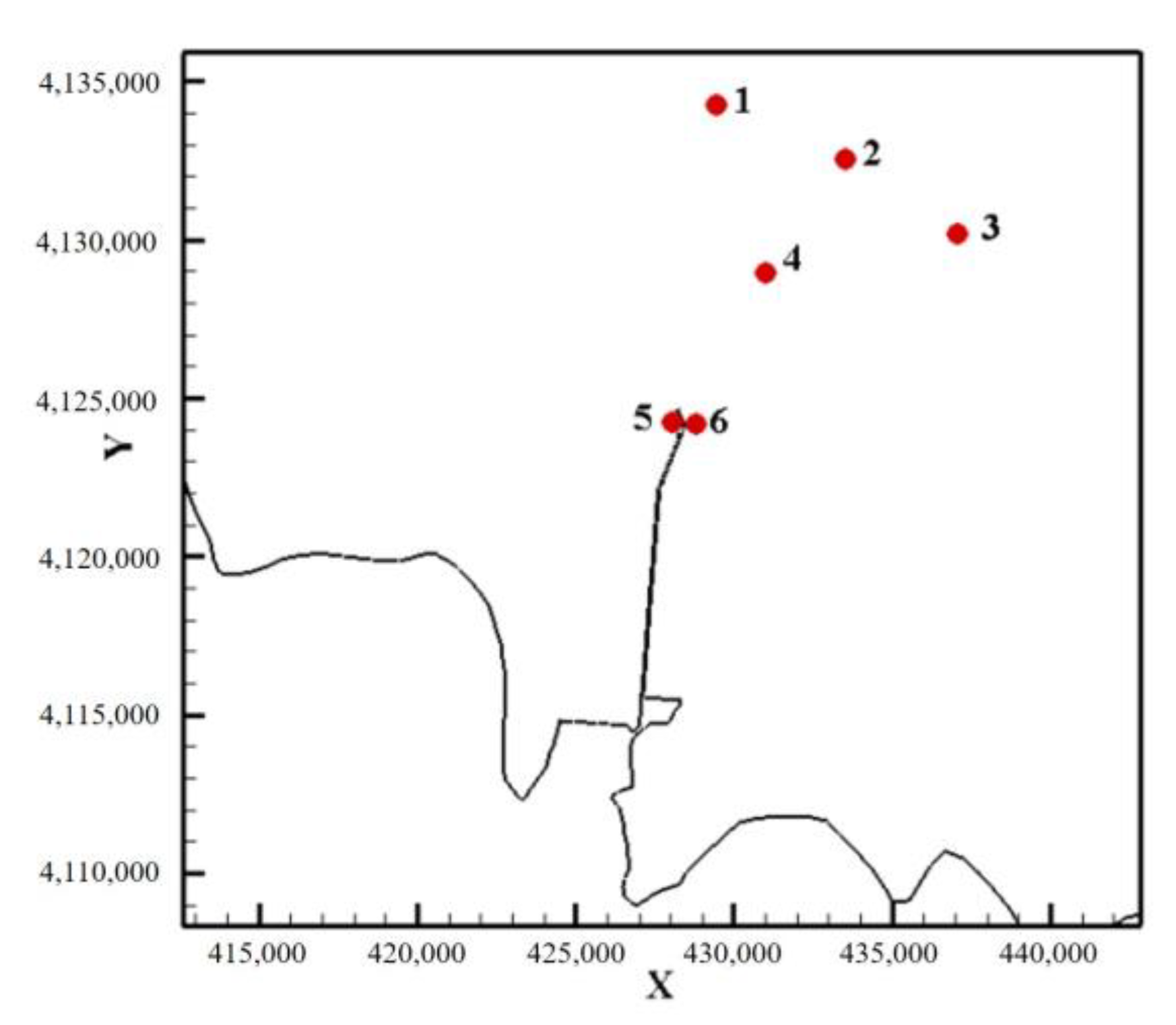


| Station Number | Beijing54 | WGS84 | ||
|---|---|---|---|---|
| x | y | E | N | |
| 1 | 429,431.5 | 4,134,252 | 119.2 | 37.34 |
| 2 | 433,521.9 | 4,132,544 | 119.25 | 37.32 |
| 3 | 437,045.2 | 4,130,220 | 119.29 | 37.30 |
| 4 | 431,003.2 | 4,128,988 | 119.22 | 37.29 |
| 5 | 428,060.5 | 4,124,240 | 119.19 | 37.25 |
| 6 | 428,809.7 | 4,124,215 | 119.20 | 37.25 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Qi, J.; Jing, Y.; Chen, C.; Zhang, J. Numerical Simulation of Tidal Current and Sediment Movement in the Sea Area near Weifang Port. Water 2023, 15, 2516. https://doi.org/10.3390/w15142516
Qi J, Jing Y, Chen C, Zhang J. Numerical Simulation of Tidal Current and Sediment Movement in the Sea Area near Weifang Port. Water. 2023; 15(14):2516. https://doi.org/10.3390/w15142516
Chicago/Turabian StyleQi, Jiarui, Yige Jing, Chao Chen, and Jinfeng Zhang. 2023. "Numerical Simulation of Tidal Current and Sediment Movement in the Sea Area near Weifang Port" Water 15, no. 14: 2516. https://doi.org/10.3390/w15142516




