Study on the Acoustic Emission Characteristics and Failure Precursors of Water-Rich Frozen Sandstone under Different Lateral Unloading Rates
Abstract
1. Introduction
2. Test Content
2.1. Sample Preparation
2.2. Experimental Design
2.3. Test Scheme
3. Test Results and Analysis
3.1. Effect of Temperature on the AE Characteristics of Sandstone under Lateral Unloading
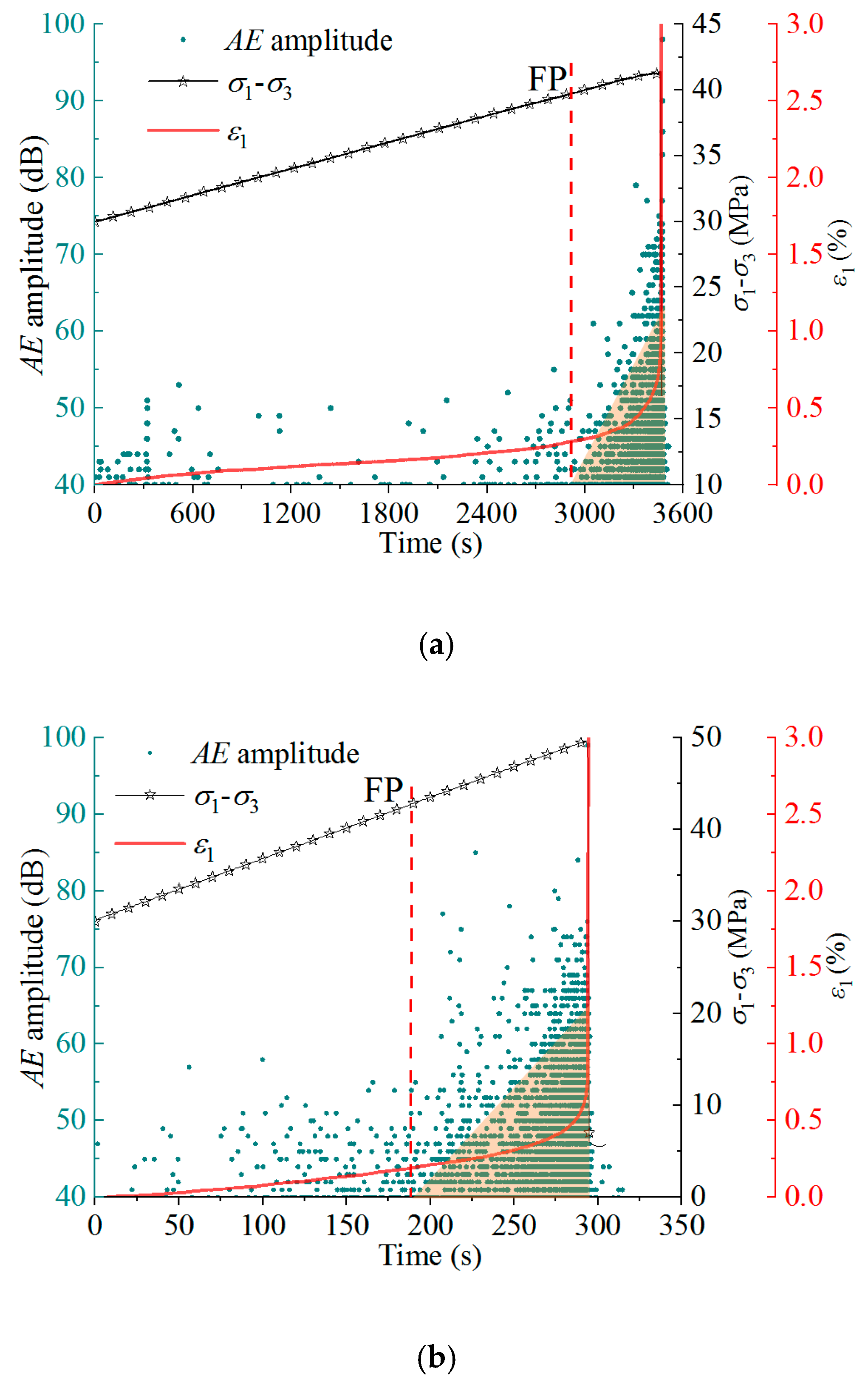
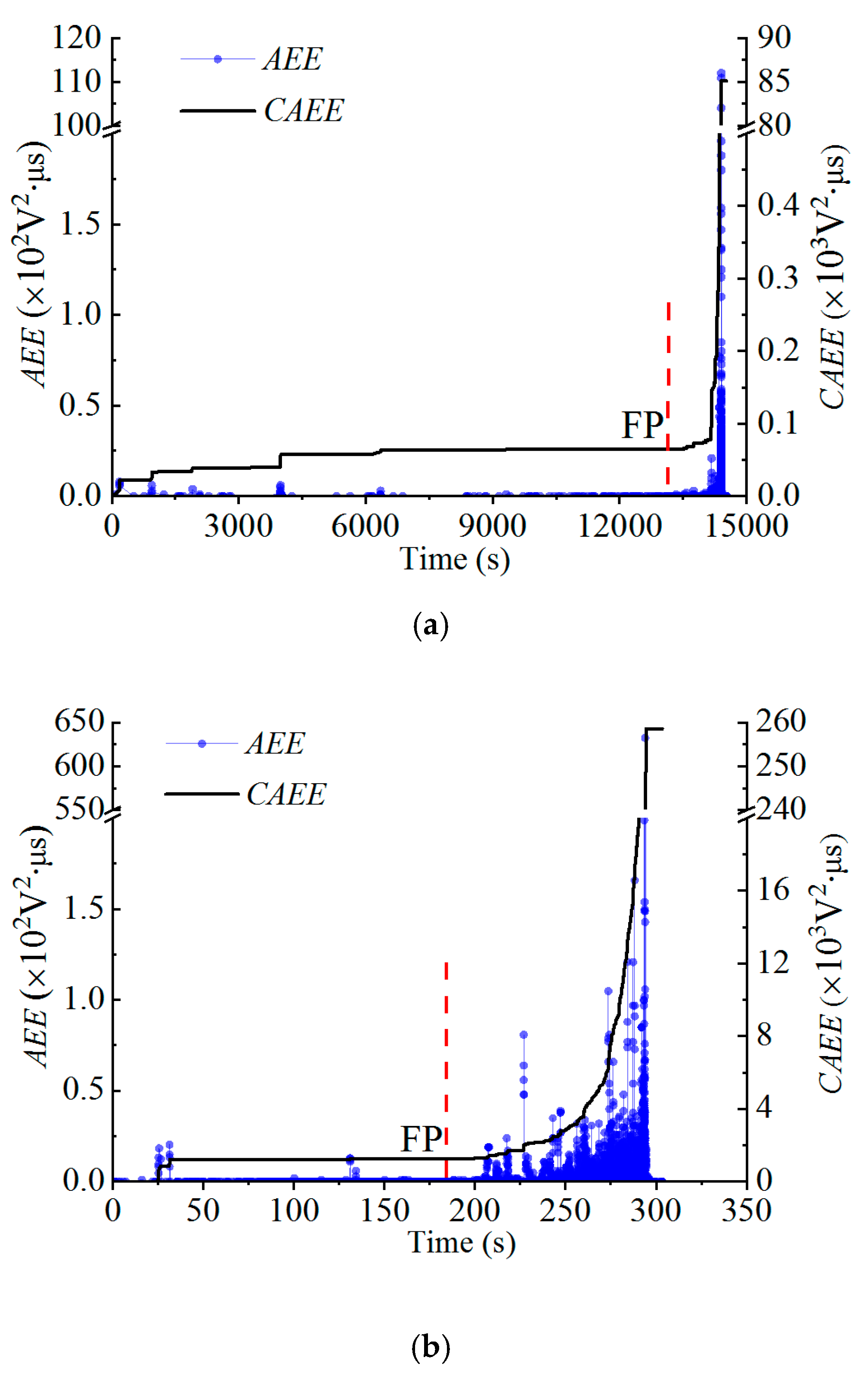
3.2. Influence of the Unloading Rate on the AE Characteristics of Frozen Sandstone
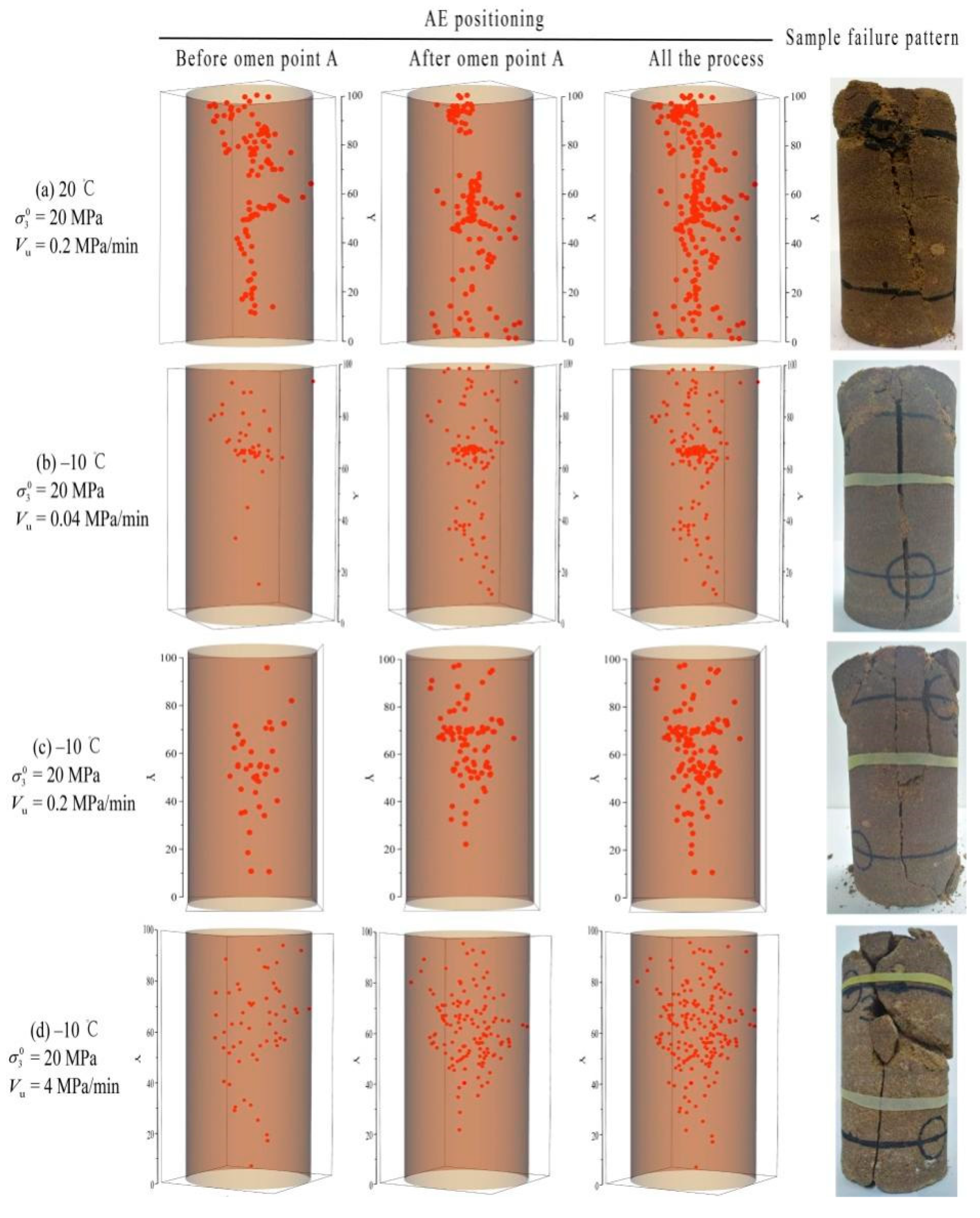
3.3. Comparative Analysis among the AE Event Locations and Failure Modes of Frozen Sandstone under Different Lateral Unloading Rates
- At an unloading rate of 0.2 MPa/min in the 20°C rock sample, a splitting crack forms in the axial direction but is slightly inclined, showing a certain degree of shear friction with a wide crack band and loose fragmentation.
- At unloading rates of 0.04 MPa/min and 0.2 MPa/min, the frozen rock sample is split vertically by an axial crack, and the section is clean with clearly defined fracture edges.
- At an unloading rate of 4 MPa/min, the frozen rock sample is vertically split by a main crack in the axial direction, and the right rock fragment is divided into two pieces from the middle by a transverse crack.
- As the confining pressure on frozen sandstone is unloaded, the deviator stress increases, the rock sample expands and deforms radially, and tensile stress appears in the centre of the rock sample, resulting in tensile cracks. After starting A, these tensile cracks extend and penetrate in the axial direction, forming a macroscopic splitting crack.
- As the axial stress is applied to a frozen rock sample by the upper indenter, the splitting cracks mostly start from the middle and upper parts of the rock sample, and the fragmentation at the upper end is more obvious.
- The frozen rock sample undergoes lateral swelling under a high unloading rate, and the surface expansion deformation is greater than the central expansion deformation in the short term. The inconsistency between the expansion and deformation of the surface and the centre part produces circumferential tensile cracks and crosscutting cracks.
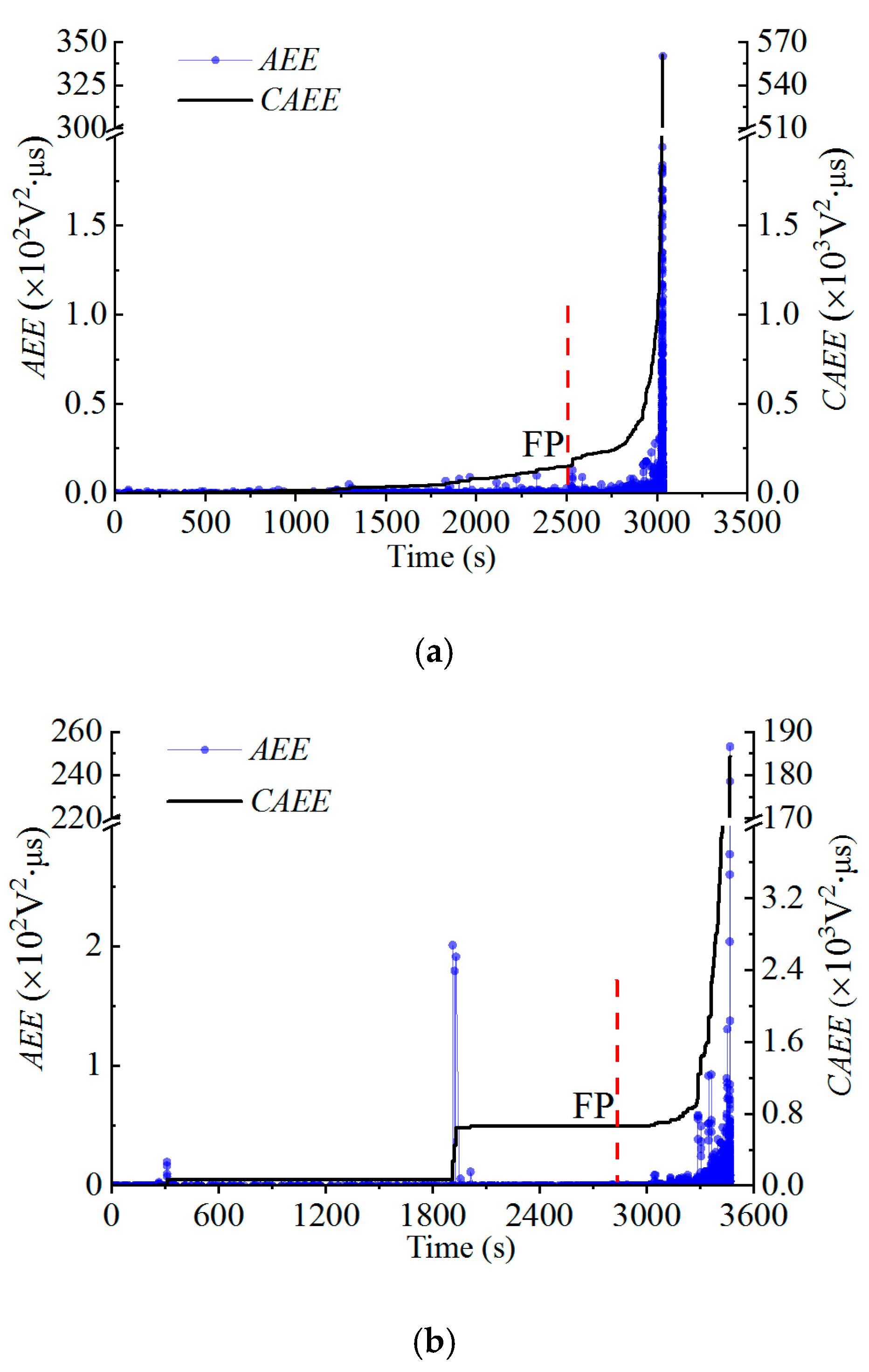
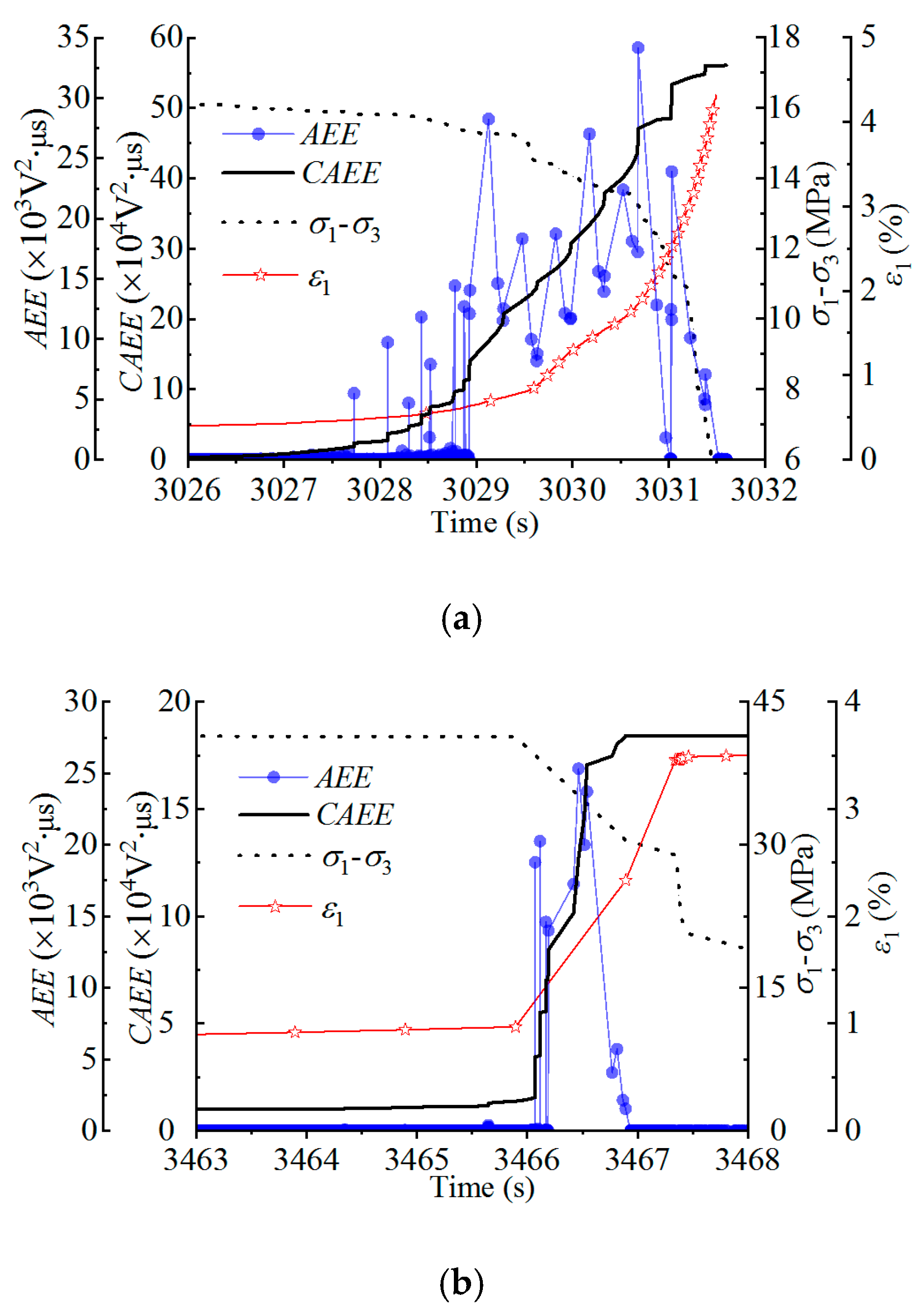
4. Picking the Acoustic Emission Key Energy
4.1. Picking Method
4.2. Picking Results
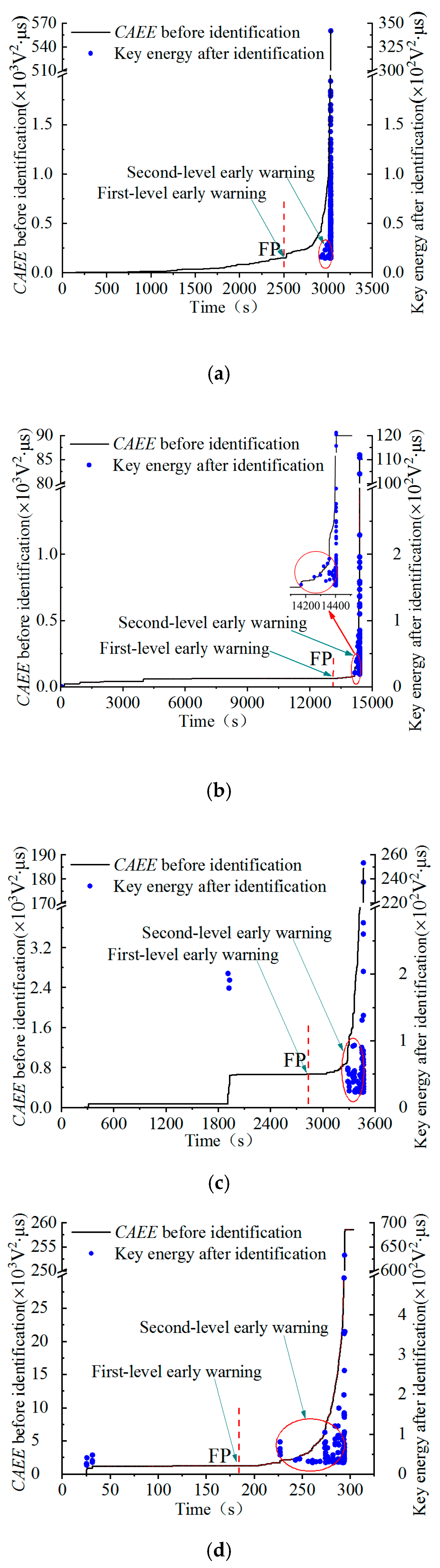
4.3. Early Warning Analysis of the AEE for Frozen Sandstone under Different Lateral Unloading Rates
5. Discussion
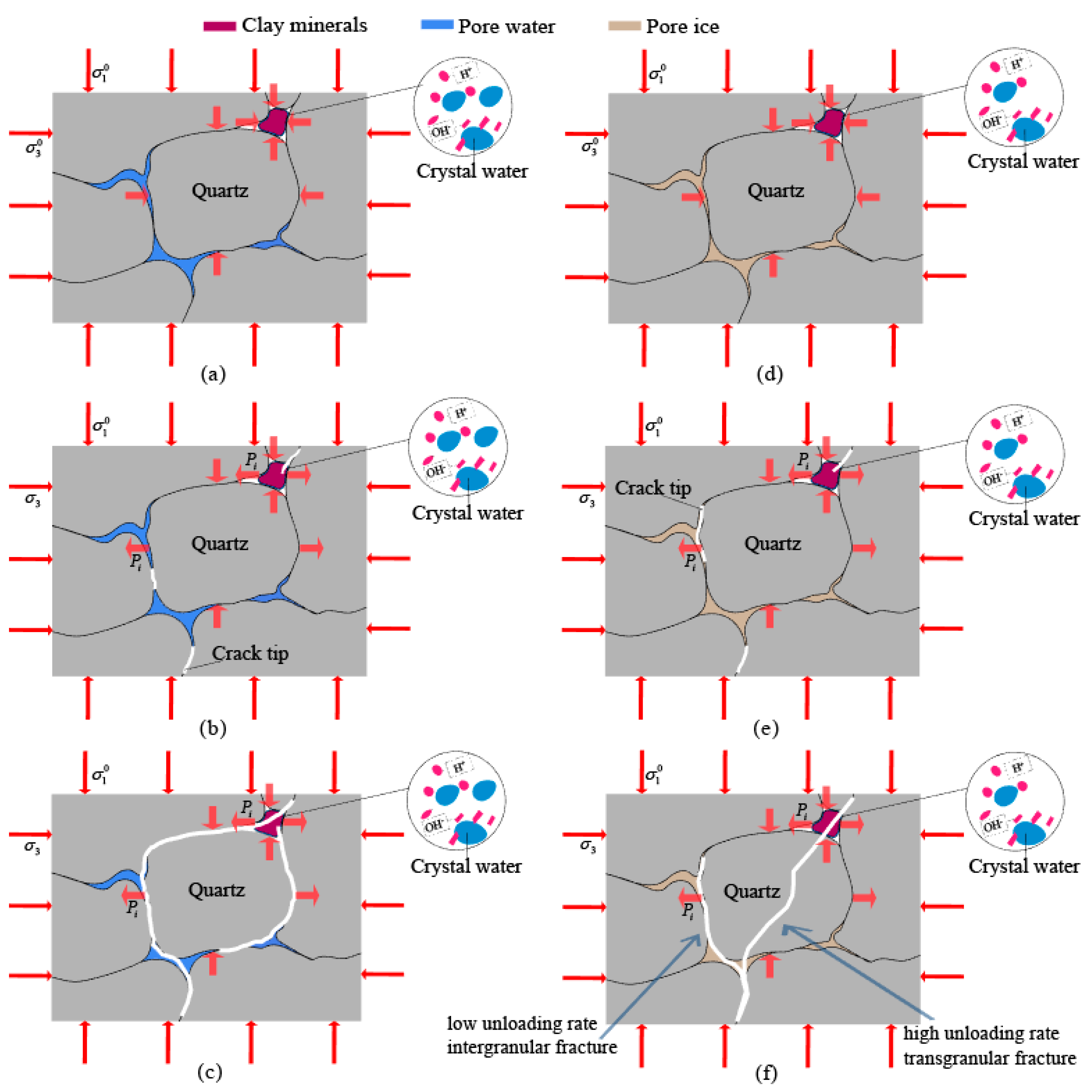
5.1. Effect of Freezing Action on Fracture Mechanism of Lateral Unloading Sandstone
5.2. Effect of Unloading Rate on Fracture Mechanism of Frozen Sandstone
6. Conclusions
- The peak lateral unloading stress of a frozen rock sample is approximately 2.5 times greater than that of a 20 °C rock sample under the same conditions. During the lateral unloading process of a rock sample, the AE amplitude data form a dense triangular area starting at point FP. Additionally, the CAEE of rock samples exhibit the same change trend over time: both increase slowly before point FP and increase exponentially after point FP. There is a cluster of AEE after point FP and before failure occurs. The triangular area and cluster area can be used as precursors signalling the lateral unloading failure of frozen sandstone.
- As the lateral unloading rate of a frozen rock sample increases, the peak stress increases, the axial strain decreases, and the number of AE amplitude datapoints increases significantly. As the CAEE of the rock sample at point FP increases, the peak AEE increases, the CAEE at the time of failure increases, and the damage-related sound becomes louder, signifying a more dangerous safety hazard.
- The lateral unloading failure pattern of frozen sandstone is consistent with the locations of the AE events recorded throughout the process. According to the AE event locations before point FP, the locations where the frozen surrounding rock needs support can be determined.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Maruvanchery, V.; Kim, E. Effects of water on rock fracture properties: Studies of mode I fracture toughness, crack propagation velocity, and consumed energy in calcite-cemented sandstone. Geomech. Eng. 2019, 17, 57–67. [Google Scholar] [CrossRef]
- Lin, G.; Li, M.; Chen, Y.L.; Zhang, J.Z.; Jiskani, I.M.; Doan, D.V.; Xu, L. Dynamic tensile mechanical properties and fracture characteristics of water-saturated sandstone under the freezing effect. Int. J. Geomech. 2021, 21, 04021044. [Google Scholar] [CrossRef]
- Li, Z.Y.; Yang, G.S. Constitutive model of frozen red sandstone based on ice-solid binary medium. Arab. J. Geosci. 2021, 14, 1616. [Google Scholar] [CrossRef]
- Cerfontaine, B.; Collin, F. Cyclic and fatigue behaviour of rock materials: Review, interpretation and research perspectives. Rock Mech. Rock Eng. 2018, 51, 391–414. [Google Scholar] [CrossRef]
- Rusin, Z.; Świercz, P. Frost resistance of rock materials. Constr. Build. Mater. 2017, 148, 704–714. [Google Scholar] [CrossRef]
- Zeng, Q. Discussion of freezing strain model for estimating the unfrozen water content of saturated rock under low temperature by Shibing Huang, Quansheng Liu, Yanzhang Liu, Zuyang Ye, and Aiping Cheng (Note). Int. J. Geomech. 2019, 19, 07019001. [Google Scholar] [CrossRef]
- Levin, L.Y.; Semin, M.A.; Parshakov, O.S. Mathematical Prediction of Frozen Wall Thickness in Shaft Sinking. J. Min. Sci. 2017, 53, 938–944. [Google Scholar] [CrossRef]
- Conver, J.L.; Yarwood, E.; Hetherington, L.D.; Swann, D.E. Nurse rock microclimates significantly buffer exposure to freezing temperature and moderate summer temperature. J. Arid Environ. 2020, 177, 104140. [Google Scholar] [CrossRef]
- Li, Z.Q.; Hu, F.; Qi, S.W.; Hu, R.L. Strain-softening failure mode after the post-peak as a unique mechanism of ruptures in a frozen soil-rock mixture. Eng. Geol. 2020, 274, 105725. [Google Scholar] [CrossRef]
- Zhang, D.M.; Yang, Y.S.; Chu, Y.P.; Zhang, X.; Xue, Y.G. Influence of loading and unloading velocity of confining pressure on strength and permeability characteristics of crystalline sandstone. Results Phys. 2018, 9, 1363–1370. [Google Scholar] [CrossRef]
- Xiao, T.L.; Huang, M.; Gao, M. Experimental study of the mechanical characteristics of a rock-like material containing a preexisting fissure under loading and unloading triaxial compression. Adv. Civ. Eng. 2020, 2020, 9078731. [Google Scholar] [CrossRef]
- Huang, X.; Liu, Q.S.; Liu, B.; Liu, X.W.; Pan, Y.C.; Liu, J.P. Experimental study on the dilatancy and fracturing behavior of soft rock under unloading conditions. Int. J. Civ. Eng. 2017, 15, 921–948. [Google Scholar] [CrossRef]
- Feng, F.; Chen, S.J.; Li, D.Y.; Huang, W.P.; Peng, K.; Zang, C.W. Excavation unloading-induced fracturing of hard rock containing different shapes of central holes affected by unloading rates and in situ stresses. Energy Sci. Eng. 2020, 8, 4–27. [Google Scholar] [CrossRef]
- Zhang, Y.; Zou, Y.; Zhang, Y.; Wang, L.; Liu, D.; Sun, J.; Ge, H.; Zhou, D. Experimental Study on Characteristics and Mechanisms of Matrix Pressure Transmission Near the Fracture Surface During Post-Fracturing Shut-In in Tight Oil Reservoirs. J. Pet. Sci. Eng. 2022, 219, 111133. [Google Scholar] [CrossRef]
- Meng, F.Z.; Louis, N.Y.W.; Zhou, H.; Wang, Z.Q.; Zhang, L.M. Asperity degradation characteristics of soft rock-like fractures under shearing based on acoustic emission monitoring. Eng. Geol. 2020, 266, 105392. [Google Scholar] [CrossRef]
- Luo, D.N.; Su, G.S.; Zhang, G.L. True-triaxial experimental study on mechanical behaviours and acoustic emission characteristics of dynamically induced rock failure. Rock Mech. Rock Eng. 2020, 53, 1205–1223. [Google Scholar] [CrossRef]
- Wei, J.; Zhu, W.C.; Guan, K.; Zhou, J.R.; Song, J.J. An acoustic emission data-driven model to simulate rock failure process. Rock Mech. Rock Eng. 2020, 53, 1605–1621. [Google Scholar] [CrossRef]
- Zha, E.S.; Zhang, R.; Ai, T.; Ren, L.; Zhang, Z.P.; Liu, Y.; Lou, C.D. Acoustic emission characteristics and damage evolution of rock under different loading modes. Energies 2020, 13, 3649. [Google Scholar] [CrossRef]
- Jing, H.W.; Yin, Q.; Yang, S.Q.; Chen, W.Q. Micro-mesoscopic creep damage evolution and failure mechanism of sandy mudstone. Int. J. Geomech. 2021, 21. [Google Scholar] [CrossRef]
- Kim, J.S.; Kim, G.Y.; Baik, M.H.; Finsterle, S.; Cho, G.C. A new approach for quantitative damage assessment of in-situ rock mass by acoustic emission. Geomech. Eng. 2019, 18, 11–20. [Google Scholar] [CrossRef]
- Li, S.C.; Li, D.Q.; Zhang, N. Multivariate prediction model of strength and acoustic emission energy considering parameter correlation of coal or rock. Adv. Mater. Sci. Eng. 2020, 2020, 8429652. [Google Scholar] [CrossRef]
- Yang, J.; Mu, Z.L.; Yang, S.Q. Experimental study of acoustic emission multi-parameter information characterizing rock crack development. Eng. Fract. Mech. 2020, 232, 107045. [Google Scholar] [CrossRef]
- Bost, M.; Pouya, A. Stress generated by the freeze–thaw process in open cracks of rock walls: Empirical model for tight limestone. Bull. Eng. Geol. Environ. 2017, 76, 1491–1505. [Google Scholar] [CrossRef]
- Lishman, B.; Marchenko, A.; Sammonds, P.; Murdza, A. Acoustic emissions from in situ compression and indentation experiments on sea ice. Cold Reg. Sci. Technol. 2020, 172, 102987. [Google Scholar] [CrossRef]
- Semin, M.A.; Levin, L.Y.; Zhelnin, M.S.; Plekhov, O.A. Natural Convection in Water-Saturated Rock Mass under Artificial Freezing. J. Min. Sci. 2020, 56, 297–308. [Google Scholar] [CrossRef]
- Shkuratnika, V.L.; Kravchenkoa, O.S.; Filimonovb, Y.L. Stresses and temperature affecting acoustic emission and rheological characteristics of rock salt. J. Min. Sci. 2019, 55, 531–537. [Google Scholar] [CrossRef]
- He, X.Q. Modern Statistical Analysis Methods and Applications; People’s University Publication House: Beijing, China, 2012. [Google Scholar]
- Ermolovich, E.A.; Ivannikov, A.L.; Khayrutdinov, M.M.; Kongar-Syuryun, C.B.; Tyulyaeva, Y.S. Creation of a Nanomodified Backfill Based on the Waste from Enrichment of Water-Soluble Ores. Materials 2022, 15, 3689. [Google Scholar] [CrossRef] [PubMed]
- Khayrutdinov, A.; Kongar-Syuryun, C.; Kowalik, T.; Faradzhov, V. Improvement of the backfilling characteristics by activation of halite enrichment waste for non-waste geotechnology. IOP Conf. Ser. Mater. Sci. Eng. 2020, 867, 012018. [Google Scholar] [CrossRef]
- Kongar-Syuryun, C.B.; Faradzhov, V.V.; Tyulyaeva, Y.S.; Khayrutdinov, A.M. Effect of activating treatment of halite flotation waste in backfill mixture preparation. Min. Inf. Anal. Bull. 2021, 2021, 43–57. [Google Scholar] [CrossRef]
- Jia, H.L.; Zi, F.; Yang, G.S.; Li, G.Y.; Shen, Y.J.; Sun, Q.; Yang, P.Y. Influence of pore water (ice) content on the strength and deformability of frozen argillaceous siltstone. Rock Mech. Rock Eng. 2020, 53, 967–974. [Google Scholar] [CrossRef]
- Jia, H.L.; Ding, S.; Wang, Y.; Zi, F.; Sun, Q.; Yang, G.S. An NMR-based investigation of pore water freezing process in sandstone. Cold Reg. Sci. Technol. 2019, 168, 102893. [Google Scholar] [CrossRef]
- Bai, Y.; Shan, R.L.; Ju, Y.; Wu, Y.X.; Sun, P.F.; Wang, Z.E. Study on the mechanical properties and damage constitutive model of frozen weakly cemented red sandstone. Cold Reg. Sci. Technol. 2020, 171, 102980. [Google Scholar] [CrossRef]
- Liu, S.; Yang, G.; Dong, X.; Shen, Y.; Liu, H. Mechanical characteristics of frozen sandstone under lateral unloading: An experimental study. Adv. Civ. Eng. 2021, 2021, 6653294. [Google Scholar] [CrossRef]
- Liu, S.; Yang, G.S.; Dong, X.H.; Shen, Y.J.; Liu, H. Energy characteristics and damage constitutive model of frozen sandstone under triaxial compression. J. Cold Reg. Eng. 2022, 36, 1–12. [Google Scholar] [CrossRef]
- Wang, C.; Zhang, K.; Zhao, Y.; Bi, J.; Ning, L.; Zhang, K. An adsorption model for cylindrical pore and its method to calculate pore size distribution of coal by combining NMR. Chem. Eng. J. 2022, 450, 138415. [Google Scholar] [CrossRef]
- Wang, C.; Zhao, Y.; Ning, L.; Bi, J. Permeability evolution of coal subjected to triaxial compression based on in-situ nuclear magnetic resonance. Int. J. Rock Mech. Min. 2022, 159, 105213. [Google Scholar] [CrossRef]
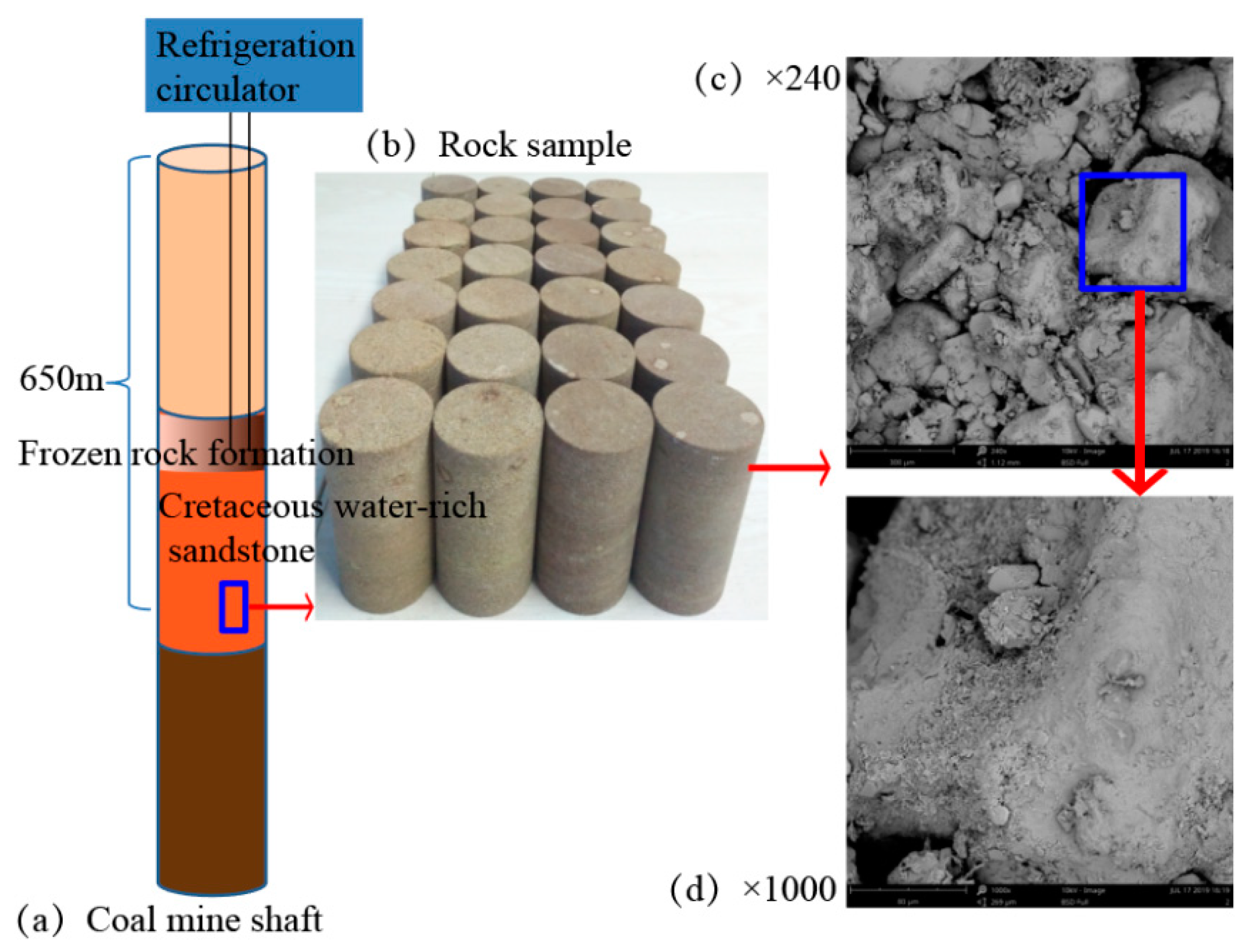




| Dry Density (g/cm3) | Saturation Density (g/cm3) | Saturated Water Capacity (%) | Porosity (%) | Longitudinal-Wave Velocity (m/s) |
|---|---|---|---|---|
| 2.18 | 2.35 | 7.58 | 16.56 | 2416 |
| Rock Sample | Temperature T (°C) | Initial Confining Pressure | Initial Axial Pressure | Unloading Rate |
|---|---|---|---|---|
| LUT-1-a LUT-1-b LUT-1-c | 20 | 20 | 26 | 0.2 |
| LUT-2-a LUT-2-b LUT-2-c | −10 | 20 | 50 | 0.04 |
| LUT-3-a LUT-3-b LUT-3-c | 50 | 0.2 | ||
| LUT-4-a LUT-4-b LUT-4-c | 50 | 4.0 |
| Number | X | Y | Z |
|---|---|---|---|
| 1 | −25 | 80 | 0 |
| 2 | 25 | 80 | 0 |
| 3 | 0 | 20 | −25 |
| 4 | 0 | 20 | 25 |
(MPa/min) | (MPa) | Early Warning Point FP | Emax (V2·μs) | Moment of Failure | ||||
|---|---|---|---|---|---|---|---|---|
| Time (s) | CAEE (V2·μs) | Time (s) | CAEE (V2·μs) | |||||
| 0.04 | 39.42 | 13,126 | 38.76 | 0.268 | 64 | 11,199 | 14,529 | 85,103 |
| 0.2 | 41.4 | 2830 | 39.42 | 0.266 | 664 | 25,322 | 3468 | 184,250 |
| 4 | 49.26 | 184 | 42.43 | 0.185 | 12,171 | 63,255 | 303 | 258,593 |
| Temperature T (°C) | Initial Confining Pressure | Unloading Rate | Picking Threshold | Energy before Picking (Number) | Energy after Picking (Number) | Percentage after Picking (%) |
|---|---|---|---|---|---|---|
| 20 | 20 | 0.2 | 14 | 18,344 | 517 | 2.81 |
| −10 | 20 | 0.04 | 18 | 14,316 | 213 | 1.49 |
| −10 | 20 | 0.2 | 22 | 17,233 | 128 | 0.74 |
| −10 | 20 | 4 | 28 | 25,056 | 175 | 0.7 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, S.; Yang, G.; Liu, H.; Dong, X.; Shen, Y. Study on the Acoustic Emission Characteristics and Failure Precursors of Water-Rich Frozen Sandstone under Different Lateral Unloading Rates. Water 2023, 15, 2297. https://doi.org/10.3390/w15122297
Liu S, Yang G, Liu H, Dong X, Shen Y. Study on the Acoustic Emission Characteristics and Failure Precursors of Water-Rich Frozen Sandstone under Different Lateral Unloading Rates. Water. 2023; 15(12):2297. https://doi.org/10.3390/w15122297
Chicago/Turabian StyleLiu, Shuai, Gengshe Yang, Hui Liu, Xihao Dong, and Yanjun Shen. 2023. "Study on the Acoustic Emission Characteristics and Failure Precursors of Water-Rich Frozen Sandstone under Different Lateral Unloading Rates" Water 15, no. 12: 2297. https://doi.org/10.3390/w15122297
APA StyleLiu, S., Yang, G., Liu, H., Dong, X., & Shen, Y. (2023). Study on the Acoustic Emission Characteristics and Failure Precursors of Water-Rich Frozen Sandstone under Different Lateral Unloading Rates. Water, 15(12), 2297. https://doi.org/10.3390/w15122297







