Effect of Fault Extension Relevant to Unconformity on Hydrothermal Fluid Flow, Mass Transport, and Uranium Deposition
Abstract
:1. Introduction
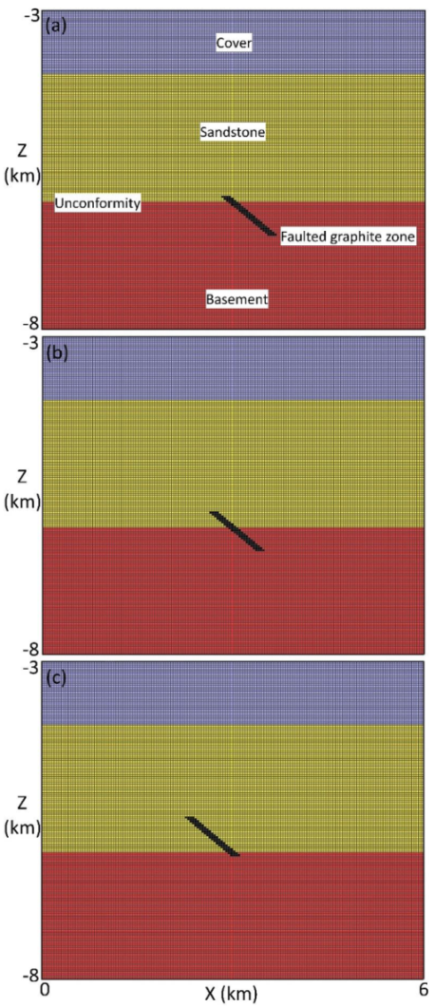
2. Model Development and Numerical Method
3. Results and Discussions
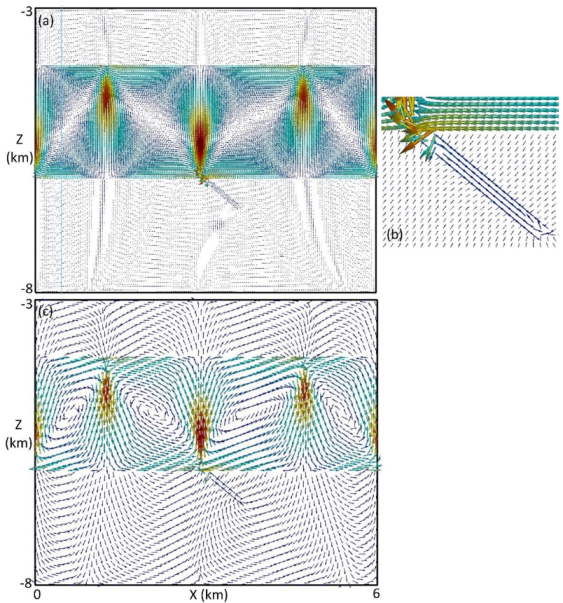
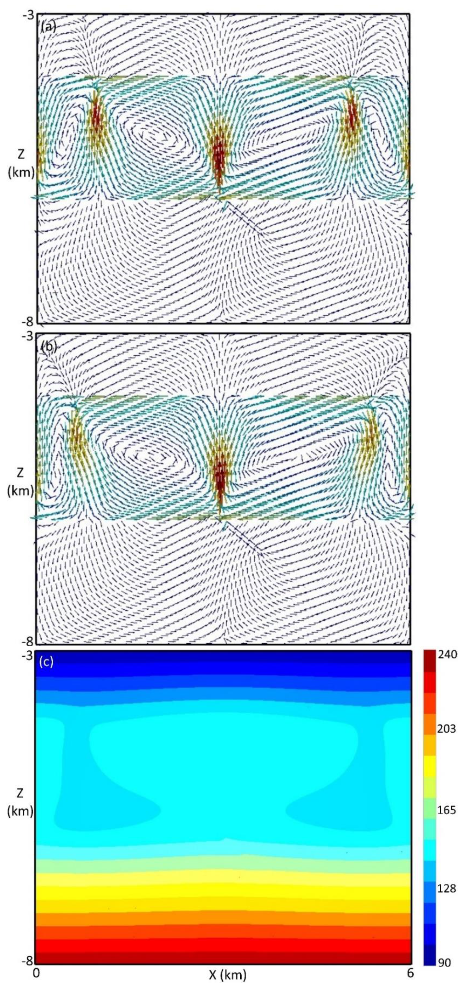
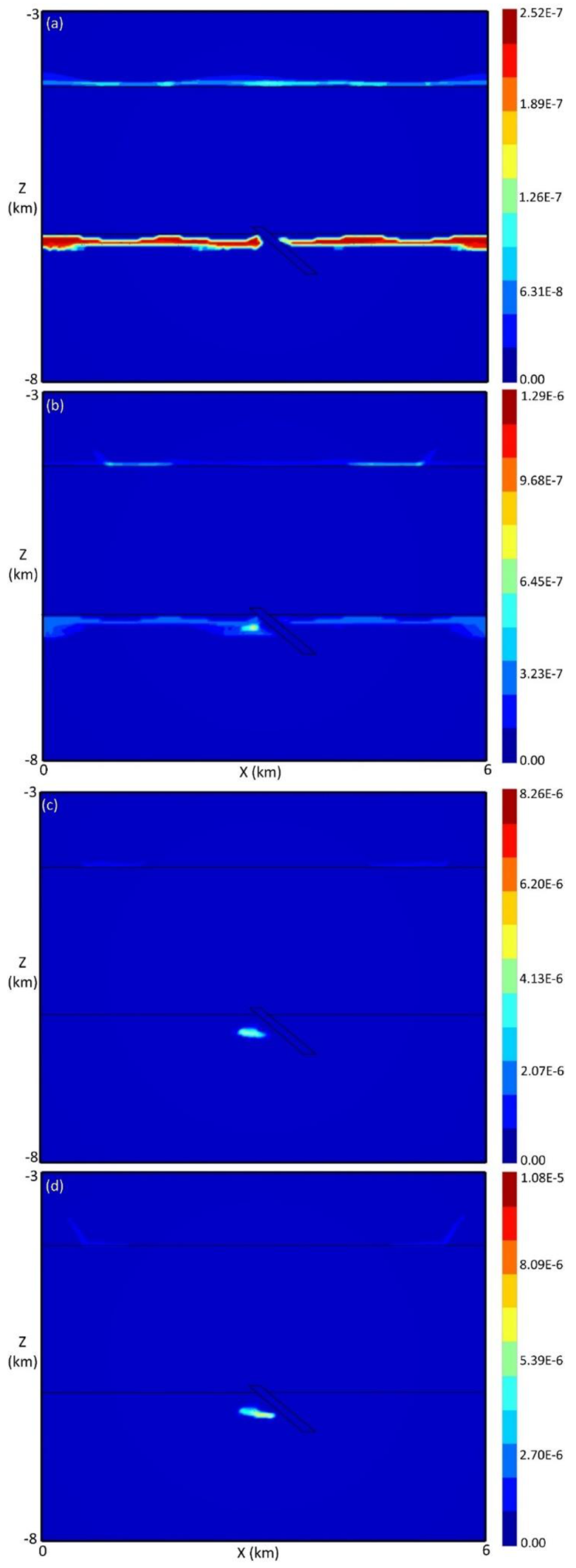
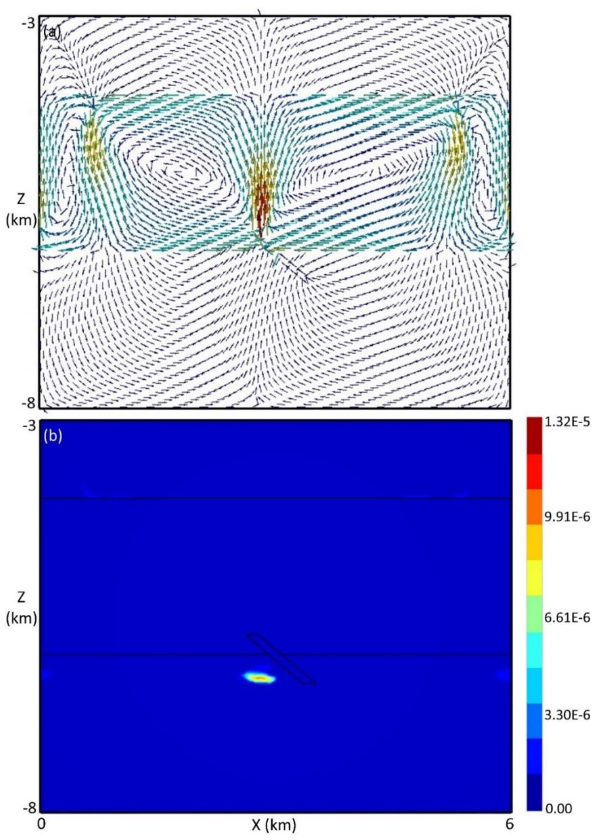
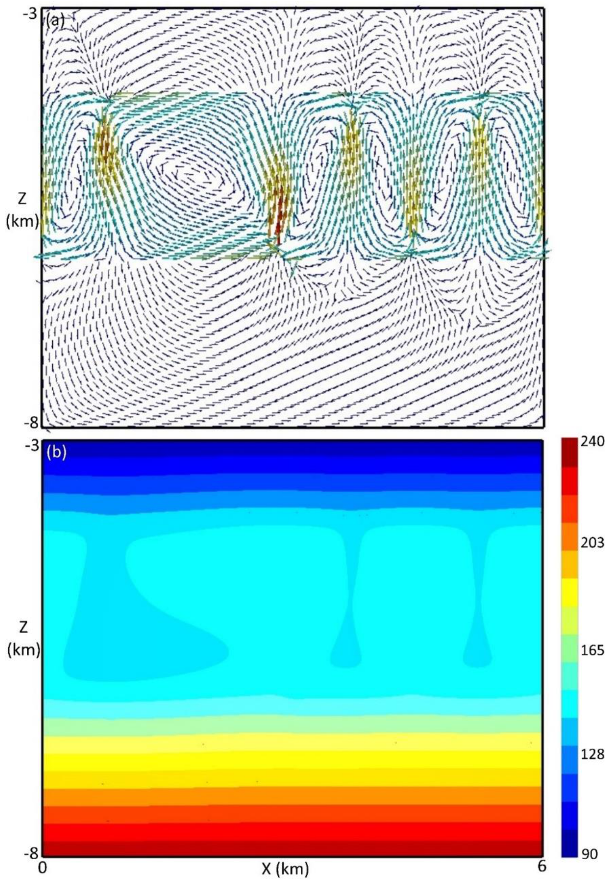
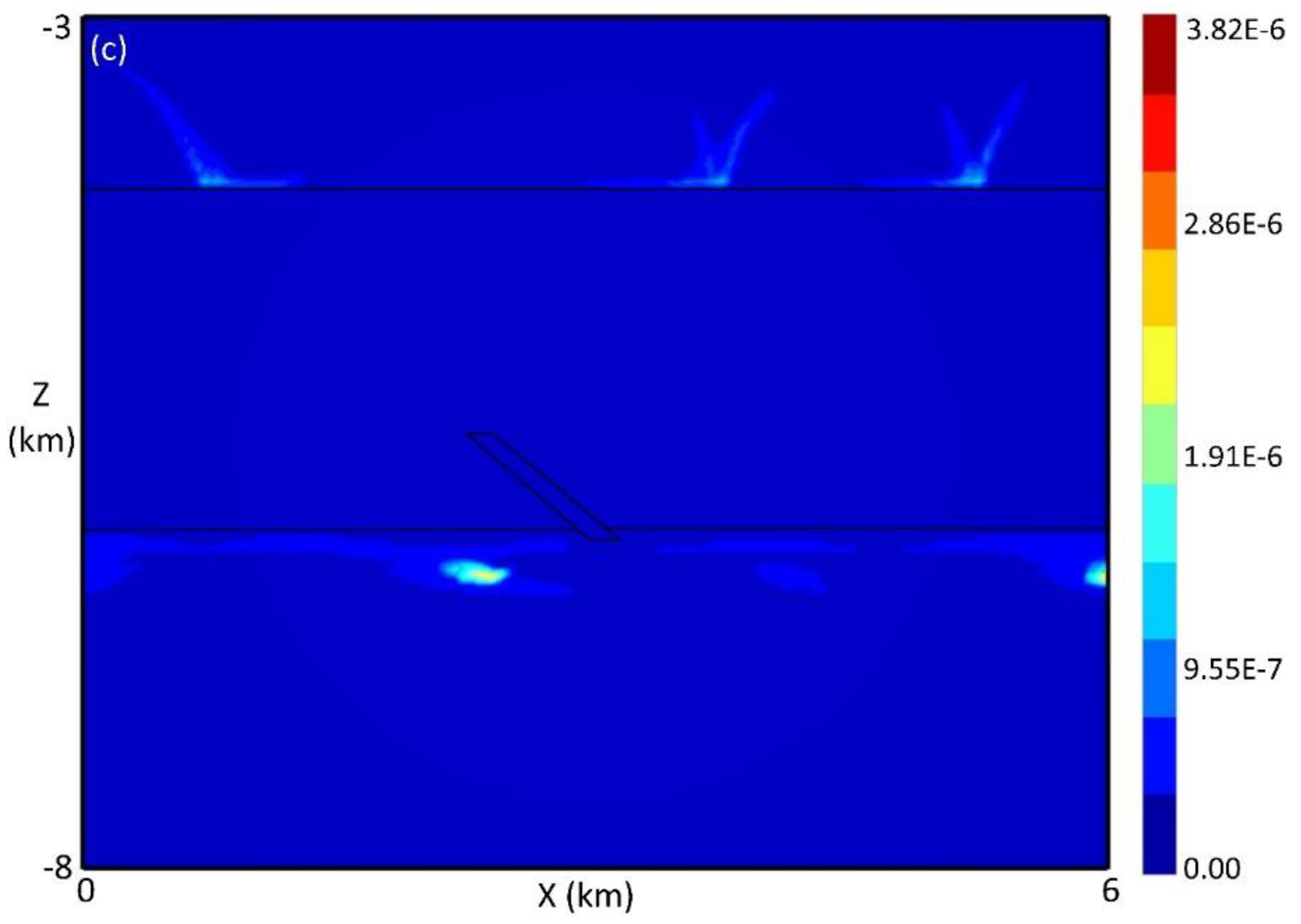
3.1. Scenario 1
3.2. Scenario 2
3.3. Scenario 3
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- International Atomic Energy Agency. Geological Classification of Uranium Deposits and Description of Selected Examples; IAEA-TECDOC-1842; International Atomic Energy Agency: Vienna, Austria, 2018; ISBN 978-92-0-101618-8. [Google Scholar]
- Cuney, M. The extreme diversity of uranium deposits. Miner. Depos. 2009, 44, 3–9. [Google Scholar] [CrossRef]
- Dahlkamp, F.J. Uranium Ore Deposits; Springer: Berlin, Germany, 2013; pp. 1–15. [Google Scholar]
- Fayek, M.; Kyser, T.K. Characterization of multiple fluid events and rare-earth-element mobility associated with formation of unconformity-type uranium deposits in the Athabasca Basin, Saskatchewan. Can. Miner. 1997, 35, 627–658. [Google Scholar]
- Jefferson, C.W.; Thomas, D.J.; Gandhi, S.S.; Ramaekers, P.; Delaney, G.; Brisbin, D.; Cutts, C.; Quirt, D.; Portella, P.; Olson, R.A. Unconformity associated uranium deposits of the Athabasca Basin, Saskatchewan and Alberta. In Mineral Deposits of Canada: A Synthesis of Major Deposit-Types, District Metallogeny, the Evolution of Geological Provinces, and Exploration Methods; Goodfellow, W.D., Ed.; Special Publication; Geological Association of Canada, Mineral Deposits Division: Ottawa, ON, Canada, 2007; Volume 5, pp. 273–305. [Google Scholar]
- Bruce, M.; Kreuzer, O.; Wilde, A.; Buckingham, A.; Butera, K.; Bierlein, F. Unconformity-type uranium systems: A comparative review and predictive modelling of critical genetic factors. Minerals 2020, 10, 738–793. [Google Scholar] [CrossRef]
- Ramaekers, P.; Jefferson, C.W.; Yeo, G.M.; Collier, B.; Long, D.G.F.; Drever, G.; Mchardy, S.; Jiricka, D.; Cutts, C.; Wheatley, K.; et al. Revised geological map and stratigraphy of the Athabasca Group, Saskatchewan and Alberta. In: EXTECH IV: Geology and Uranium Exploration Technology of the Proterozoic Athabasca Basin, Saskatchewan and Alberta. Geol. Surv. Can. Bull. 2007, 588, 155–192. [Google Scholar]
- Alexandre, P.; Kyser, K.; Thomas, D.; Polito, P.; Marlat, J. Geochronology of unconformity-related uranium deposits in the Athabasca Basin, Saskatchewan, Canada and their integration in the evolution of the basin. Miner. Depos. 2009, 44, 41–59. [Google Scholar] [CrossRef]
- Hoeve, J.; Rawsthorn, K.; Quirt, D. Uranium metallogenetic studies: Clay mineral stratigraphy and diagenesis in the Athabasca Group. Sask. Res. Counc. Publ. 1981, 22, 76–89. [Google Scholar]
- Halter, G. Zonality of Alterations in the Environment of Uranium Deposits Associated to Middle Proterozoic Unconformity (Saskatchewan, Canada). Ph.D. Thesis, Université Louis Pasteur, Strasbourg, France, 1988; 252p. [Google Scholar]
- Kotzer, T.G.; Kyser, T.K. Petrogenesis of the Proterozoic Athabasca Basin, northern Saskatchewan, Canada, and its relation to diagenesis, hydrothermal uranium mineralization and paleohydrology. Chem. Geol. 1995, 120, 45–89. [Google Scholar] [CrossRef]
- Renac, C.; Kyser, T.K.; Durocher, K.; Dreaver, G.; O’Connor, T. Comparison of diagenetic fluids in the Proterozoic Thelon and Athabasca Basins, Canada: Implications for protracted fluid histories in stable intracratonic basins. Can. J. Earth Sci. 2002, 39, 113–132. [Google Scholar] [CrossRef]
- Derome, D.; Cathelineau, M.; Cuney, M.; Fabre, C.; Lhomme, T.; Banks, D.A. Mixing of sodic and calcic brines and uranium deposition at McArthur River, Saskatchewan, Canada: A Raman and laser-induced breakdown spectroscopic study of fluid inclusions. Econ. Geol. 2005, 100, 1529–1545. [Google Scholar] [CrossRef]
- Hoeve, J.; Sibbald, T.I. On the genesis of Rabbit Lake and other unconformity-type uranium deposits in northern Saskatchewan, Canada. Econ. Geol. 1978, 73, 1450–1473. [Google Scholar] [CrossRef]
- Kyser, T.K.; Wilson, M.R.; Ruhrmann, G. Stable isotope constraints on the role of graphite in the genesis of unconformity-type uranium deposits. Can. J. Earth Sci. 1989, 26, 490–498. [Google Scholar] [CrossRef]
- Hoeve, J.; Quirt, D. Mineralization and host rock alteration in relation to clay mineral diagenesis and evolution of the middle-Proterozoic, Athabasca Basin, northern Saskatchewan, Canada. Sask. Res. Counc. Tech. Rep. 1984, 187, 187. [Google Scholar]
- Pascal, M.; Ansdell, K.M.; Annesley, I.R. Graphite-bearing and graphite-depleted basement rocks in the Dufferin Lake zone, south-central Athabasca Basin, Saskatchewan. In Targeted Geoscience Initiative 4: Unconformity-Related Uranium Systems; Potter, E.G., Wright, D.M., Eds.; Geological Survey of Canada Open File; Natural Resources Canada: Ottawa, ON, Canada, 2015; Volume 7791, pp. 83–92. [Google Scholar]
- Potter, E.G.; Wright, D.M. Targeted geoscience initiative 4: Unconformity-related uranium systems. In Geological Survey of Canada Open File; Natural Resources Canada: Ottawa, ON, Canada, 2015; Volume 7791, p. 126. [Google Scholar] [CrossRef]
- Bruneton, P. Geological environment of the Cigar Lake uranium deposit. Can. J. Earth Sci. 1993, 30, 653–673. [Google Scholar] [CrossRef]
- Hajnal, Z.; Györfi, I.; Annesley, I.R.; White, D.J.; Powell, B.; Koch, R. Seismic reflections outline complex structural setting of the uranium in the Athabasca Basin. In Uranium production and raw materials for the nuclear fuel cycle: Supply and demand, economics, the environment and energy security. Extended synopses, Report IAEA-CN-128. In Proceedings of the An International Symposium Held in Vienna, Vienna, Austria, 20–24 June 2005. [Google Scholar]
- Finch, W. Uranium provinces of North America: Their definition, distribution, and models. US Geol. Surv. Bull. 1996, 2141, 1–15. [Google Scholar]
- Bishop, C.; Mainville, A.; Yesnik, L. Cigar Lake Operation Northern Saskatchewan, Canada; Cameco Technical Report; National Instrument: Austin, TX, USA, 2016; pp. 1–164. [Google Scholar]
- McGill, B.; Marlatt, J.; Matthews, R.; Sopuck, V.; Homeniuk, L.; Hubregtse, J. The P2 North uranium deposit Saskatchewan, Canada. Explor. Min. Geol. 1993, 2, 321–331. [Google Scholar]
- Li, Z.; Chi, G.; Bethune, K. The effects of basement faults on thermal convection and implications for the formation of unconformity-related uranium deposits in the Athabasca Basin, Canada. Geofluids 2016, 16, 729–751. [Google Scholar] [CrossRef]
- Eldursi, K.; Chi, G.; Bethune, K.; Li, Z.; Ledru, P.; Quirt, D. New insights from 2- and 3-D numerical modelling on fluid flow mechanisms and geological factors responsible for the formation of the world-class Cigar Lake uranium deposit, eastern Athabasca Basin, Canada. Miner. Depos. 2020, 56, 1365–1388. [Google Scholar] [CrossRef]
- Caine, J.S.; Evans, J.P.; Forster, C.B. Fault zone architecture and permeability structure. Geology 1996, 24, 1025–1028. [Google Scholar] [CrossRef]
- Cui, T.; Yang, J.; Samson, I.M. Tectonic deformation and fluid flow: Implications for the formation of unconformity-related uranium deposits. Econ. Geol. 2012, 107, 147–163. [Google Scholar] [CrossRef]
- Cui, T.; Yang, J.; Samson, I.M. Solute transport across basement/cover interfaces by buoyancy-driven thermohaline convection: Implications for the formation of unconformity-related uranium deposits. Am. J. Sci. 2012, 312, 994–1027. [Google Scholar] [CrossRef]
- Pek, A.A.; Malkovsky, V.I. Linked thermal convection of the basement and basinal fluids in formation of the unconformity-related uranium deposits in the Athabasca Basin, Saskatchewan, Canada. Geofluids 2016, 16, 925–940. [Google Scholar] [CrossRef]
- Raffensperger, J.P.; Garven, G. The formation of unconformity-type uranium ore deposits 2. coupled hydrochemical modeling. Am. J. Sci. 1995, 295, 639–696. [Google Scholar] [CrossRef]
- Aghbelagh, Y.B.; Yang, J. Effect of graphite zone in the formation of unconformity-related uranium deposits: Insights from reactive mass transport modeling. J. Geochem. Explor. 2014, 144, 12–27. [Google Scholar] [CrossRef]
- Aghbelagh, Y.B.; Yang, J. Role of hydrodynamic factors in controlling the formation and location of unconformity-related uranium deposits: Insights from reactive-flow modeling. Hydrogeol. J. 2017, 25, 465–486. [Google Scholar] [CrossRef] [Green Version]
- Bear, J. Dynamics of Fluids in Porous Media; American Elsevier Publishing Company: Princeton, NJ, USA, 1972; p. 764. [Google Scholar]
- Xu, T.; Spycher, N.; Sonnenthal, E.; Zhang, G.; Zheng, L.; Pruess, K. TOUGHREACT Version 2.0: A simulator for subsurface reactive transport under non-isothermal multiphase flow conditions. Comput. Geosci. 2011, 37, 763–774. [Google Scholar] [CrossRef] [Green Version]
- Richard, A.; Rozsypal, C.; Mercadier, J.; Cuney, M.; Boiron, M.C.; Cathelineau, M.; Banks, D.A. Giant uranium deposits formed from exceptionally uranium-rich acidic brines. Nat. Geosci. 2012, 5, 142–146. [Google Scholar] [CrossRef]
- Richard, A.; Cathelineau, M.; Boiron, M.C.; Mercadier, J.; Banks, D.A.; Cuney, M. Metal-rich fluid inclusions provide new insights into unconformity-related U deposits (Athabasca Basin and Basement, Canada). Miner. Depos. 2016, 51, 249–270. [Google Scholar] [CrossRef] [Green Version]
- Chi, G.; Chu, H.; Petts, D.; Potter, E.; Jackson, S.; Williams-Jones, A. Uranium-rich diagenetic fluids provide the key to unconformity-related uranium mineralization in the Athabasca Basin. Sci. Rep. 2019, 9, 5530. [Google Scholar] [CrossRef]
| Parameter | Confining Cover | Sandstone Layer | Basement Unit | Faulted Graphite Zone |
|---|---|---|---|---|
| Permeability (m2) | 1.0 × 10−15 | 3.0 × 10−13 | 3.0 × 10−16 | 1.0 × 10−12 |
| Porosity | 0.15 | 0.2 | 0.1 | 0.2 |
| Density (kg/m3) | 2400 | 2500 | 2650 | 2400 |
| Thermal conductivity (W/(m·°C)) | 2.5 | 3.5 | 2.5 | 4.0 |
| Minerals | Volume Fraction |
|---|---|
| Calcite | 0.44 |
| Dolomite | 0.013 |
| Hematite | 0.0003 |
| Anhydrite | 0.002 |
| Kaolinite | 0.3 |
| Muscovite | 0.001 |
| Quartz | 0.095 |
| Minerals | Volume Fraction |
|---|---|
| Hematite | 0.01 |
| Anhydrite | 0.006 |
| K-feldspar | 0.01 |
| Chlorite | 0.0003 |
| Muscovite | 0.02 |
| Quartz | 0.75 |
| Minerals | Volume Fraction |
|---|---|
| Anhydrite | 0.003 |
| Chlorite | 0.006 |
| Hematite | 0.0005 |
| K-feldspar | 0.05 |
| Muscovite | 0.33 |
| Pyrite | 0.0001 |
| Quartz | 0.51 |
| Minerals | Volume Fraction |
|---|---|
| Graphite | 0.085 |
| Chlorite | 0.001 |
| Kaolinite | 0.0007 |
| Muscovite | 0.42 |
| Pyrite | 0.001 |
| Quartz | 0.29 |
| Cover | 0.1 | 2.8 × 10−2 | 0.1 | 0.1 | 7.3 × 10−15 | 0.4 | 0.022 | 0.0026 | 0.1 | 1.0 | 1.5 × 10−2 |
| Sandstone | 0.1 | 1.5 | 1.0 | 5.0 | 2.6 × 10−11 | 1.0 × 10−4 | 0.045 | 0.48 | 1.0 | 5.0 | 1.5 × 10−3 |
| Basement | 0.1 | 0.85 | 1.0 | 2.7 | 0.002 | 0.05 | 0.16 | 0.37 | 0.1 | 1.0 | 5.6 × 10−4 |
| Fault zone | 0.1 | 0.1 | 4.0 | 3.0 | 0.0005 | 1.2 × 10−4 | 0.038 | 0.88 | 1.0 | 0.5 | 1.0 × 10−3 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lin, H.; Xu, X.; Yang, J. Effect of Fault Extension Relevant to Unconformity on Hydrothermal Fluid Flow, Mass Transport, and Uranium Deposition. Water 2022, 14, 1097. https://doi.org/10.3390/w14071097
Lin H, Xu X, Yang J. Effect of Fault Extension Relevant to Unconformity on Hydrothermal Fluid Flow, Mass Transport, and Uranium Deposition. Water. 2022; 14(7):1097. https://doi.org/10.3390/w14071097
Chicago/Turabian StyleLin, Hua, Xu Xu, and Jianwen Yang. 2022. "Effect of Fault Extension Relevant to Unconformity on Hydrothermal Fluid Flow, Mass Transport, and Uranium Deposition" Water 14, no. 7: 1097. https://doi.org/10.3390/w14071097
APA StyleLin, H., Xu, X., & Yang, J. (2022). Effect of Fault Extension Relevant to Unconformity on Hydrothermal Fluid Flow, Mass Transport, and Uranium Deposition. Water, 14(7), 1097. https://doi.org/10.3390/w14071097





