1. Introduction
River ice is an integral component of the hydrological and hydraulic regimes of cold region rivers and affects an extensive portion of the global hydrologic system, particularly in the Northern Hemisphere [
1]. It regulates numerous hydrologic, geomorphic, biological, and chemical process in rivers, and provides important socio-economic and ecological benefits [
2]. However, river ice can also cause extensive damage to infrastructure, navigation, and hydroelectric production, and can result in detrimental effects to river ecology and water quality [
3].
The formation of river ice is a rather complex physical process [
4,
5], which involves the interaction of hydrodynamic, mechanical and thermal forces, and is also affected by meteorological and hydrological conditions as well as river topography features [
6]. There are four main types of river ice formation. In turbulent flow, water flows so fast that the flow can carry away the melting heat so quickly that ice can form at only the tiniest cooling temperature. Ice crystals (known as ice flowers) are generated as many tiny disks and continue to form ice [
7]. In calm water, the ice that first appears also takes the shape of a small disk, and the disk shape will develop into a dendritic shape when a critical size is reached. This dendritic ice develops rapidly across the surface of the water until an entire lake or pond is covered with it, often overnight. In addition, the surface is covered with an extremely thin but uniform layer of smooth and unbroken ice. The newly fallen snow is light and loose, with a density ranging from 0.1 to 0.3 kg/m
−3. The tension of edges of the crystalline and snow water vapor at the edge is higher than that inside, and the molecules evaporate towards the edges and condense inward, so the crystallization tends to be round [
8]. A solid fine substance, known as snow ice, is formed during the deformation, refreezing, recrystallization, and other processes. Recrystallization makes the space between particles more compact and tighter; the density is between 0.82~0.84 kg/m, and the air gap is squeezed out and becomes an impermeable object, i.e., the conversion of snow to ice. Affected by the gravity and centrifugal force, the surface water flows to the concave bank, and the bottom water flows to the convex bank at the curved reach of the river, forming a transverse circulation. The ice flowers floating on the water surface gather towards the concave bank under the action of transverse circulation. When the mechanical force caused by the flow turbulence is less than the bond force between the ice flowers, the ice flowers stick together and result in blockage, and such ice flowers fill the whole river, thereby giving rise to an iceberg [
9,
10].
River ice impacts local river hydraulics in many different ways [
11]. Similarly, in some small river systems, freezing of riverbeds may block groundwater inflows, which may also generate flooding through the aufeis [
12]. Ice jams can also change river channel properties and flow paths through ice scouring and erosion [
13]. Therefore, it is of great significance to study the blasting of river ice in order to open the river.
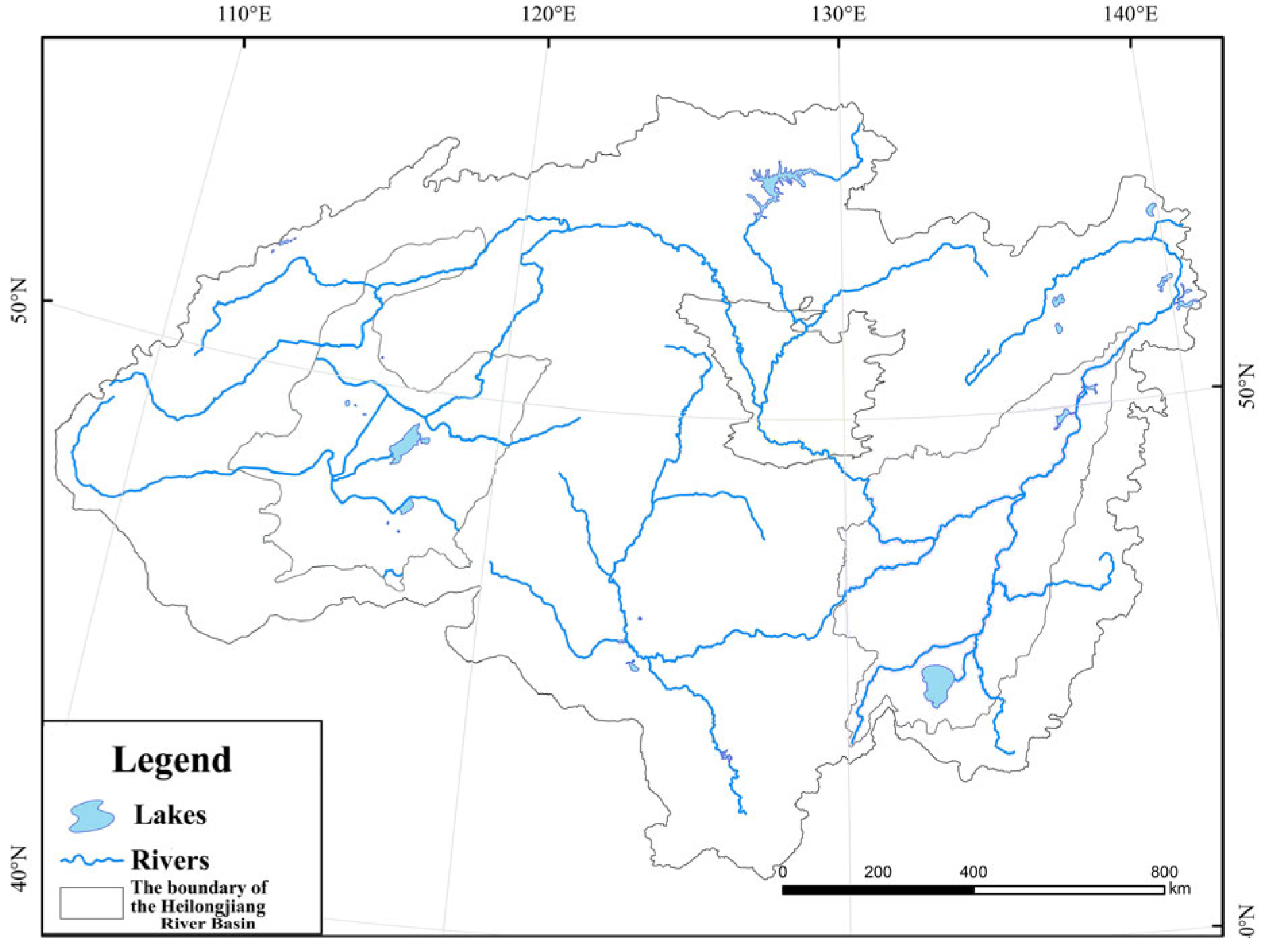
Heilongjiang is located in the northernmost part of China, as shown in
Figure 1, and its geographical location is 108°20′–141°20′ E and 42°00′–55°45′ N [
14]. This section belongs to the Sino–Russian Boundary River, where there is an ice dam of a certain size every 3 years on average, and where there are also serious melting ice floods. However, Heilongjiang is a remote province subject to underdeveloped transportation and communication, as well as backward observation equipment and technology, and relatively little research has been conducted on its ice jam flood disaster prevention and reduction. As the boundary river between China and Russia, the occurrence of ice disasters cannot be controlled, prevented or mitigated by engineering measures (such as the construction of hydraulic structures). Instead, only effective non-engineering measures can be used to prevent the occurrence of ice dams or smoothly break the ice dam [
15,
16]. Given that the measured ice sheet thickness can reach up to 1.8 m before the upper reach of the Heilongjiang River is opened up, that the ice sheet is of high strength, and that the snow cover on the ice sheet is thick, the measured data show the difficulty of achieving the destruction and movement of large ice structures by non-engineering measures (such as ice surface treatment, mechanical destruction of the ice sheet, cutting of the ice sheet, icebreaking by hovercraft, and river trepanning). In this case, exploding the ice sheet or ice dam using explosives has become the most effective way to prevent or alleviate ice disasters in the Heilongjiang River.
Although there was a record regarding icebreaking by explosives 100 years ago [
17], the research is limited by the ice conditions of the river and the surrounding environment of the river, and the measurement is difficult and risky, heavily impeding the research progress [
18,
19]. Mellor from the United States Army Corps of Engineers obtained a series of explosion data for more than 10 years from the infraglacial blasting experiment and explored the relationship among blasting pit radius, ice sheet thickness and explosive dosage using the regression analysis [
20,
21]. In China, He Bingshun et al. [
22] put forward the icebreaking principle of using the icebreaking and cannon firing technology by dropping a bomb with an aircraft. Based on the freezing layer with thick ice of the Yellow River and Inner Mongolia as the study object, Liang Xiangqian et al. [
23,
24] studied the vibration velocity of explosive blasting and the change in shock wave pressure in water under two icebreaking methods, i.e., ice contact energy-collected explosion and underwater explosion. Tong Zheng et al. [
25] investigated the crack and pressure distribution of ice blocks after blasting by simulating the field blasting and numerical situation of the Yellow River ice run. In terms of the blasting technique, Duan Yuansheng et al. [
26] summarized the parameter indexes (such as the arrangement form of explosive holes and the proper channel width of blasting the ice sheet for ice prevention) via engineering practice. Yan Huaitang et al. [
27] deduced the relationship between the length of extended explosive charge and the amount of explosives used for icebreaking via blasting under the ice sheet by studying the properties of the explosives. It can be observed from the above research results of infraglacial blasting in the river that the average thickness of the ice sheet is mainly 0.5 m and below, and that the measured maximum ice thickness of the Heilongjiang River can reach as high as 1.8 m. To this end, the field test of infraglacial blasting in the Mohe section in the upper reach of the Heilongjiang River was hereby studied pursuant to the characteristics of the Heilongjiang River (thick ice sheet, solid ice layer, and sub-glacial water depth) to obtain the effective methods and techniques of ice prevention blasting and establish the theoretical relationship between blasting pit radius as well as explosive dosage, ice sheet thickness, and water depth under ice, thereby providing a scientific basis and theoretical support for realizing efficient icebreaking by explosives. Therefore, it is of great significance to develop a blasting method with a good icebreaking effect to prevent the ice run in the Heilongjiang River. Additionally, the Huma section in the Heilongjiang River Basin is one of the main reaches where ice damage occurs, and ice damage of different degrees happens at the moment of closure and ice breakup every year. Therefore, the river at the Huma section of the Heilongjiang River main stream during the freeze-up period is hereby taken as the study object, and numerical simulation of ice explosions is hereby carried out for the river during the freeze-up period under different ice thicknesses and explosive charges, so as to provide the theoretical basis for flood control and disaster reduction, and develop a series of ice explosion methods in the Heilongjiang River Basin [
28].
3. Results
3.1. Analysis of the Influence of Ignition Location on Ice Sheet
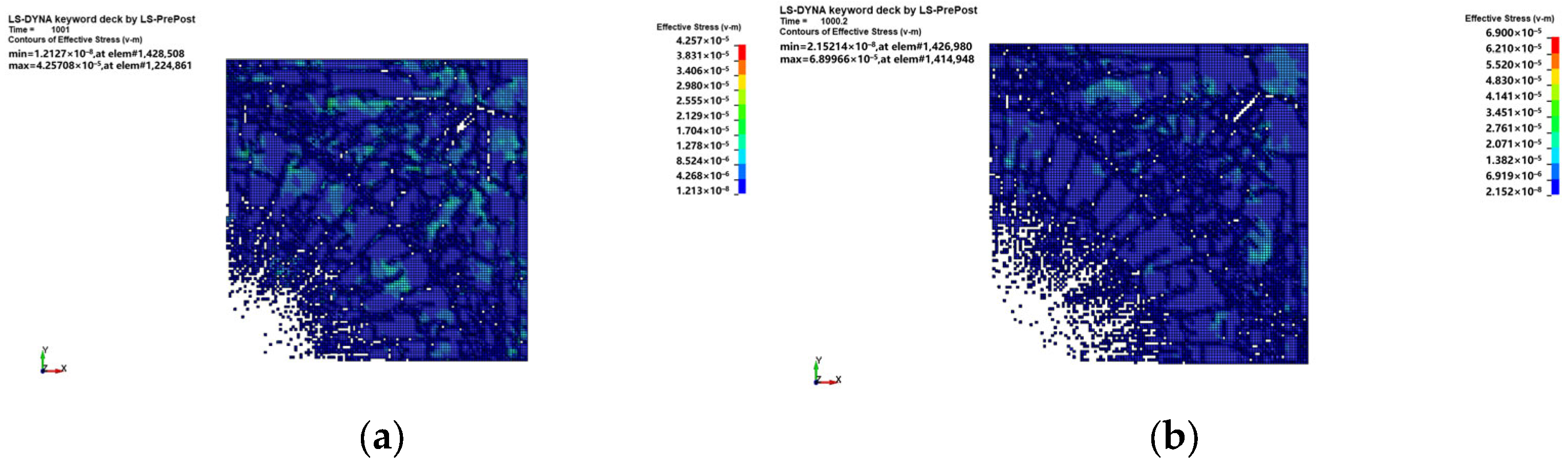
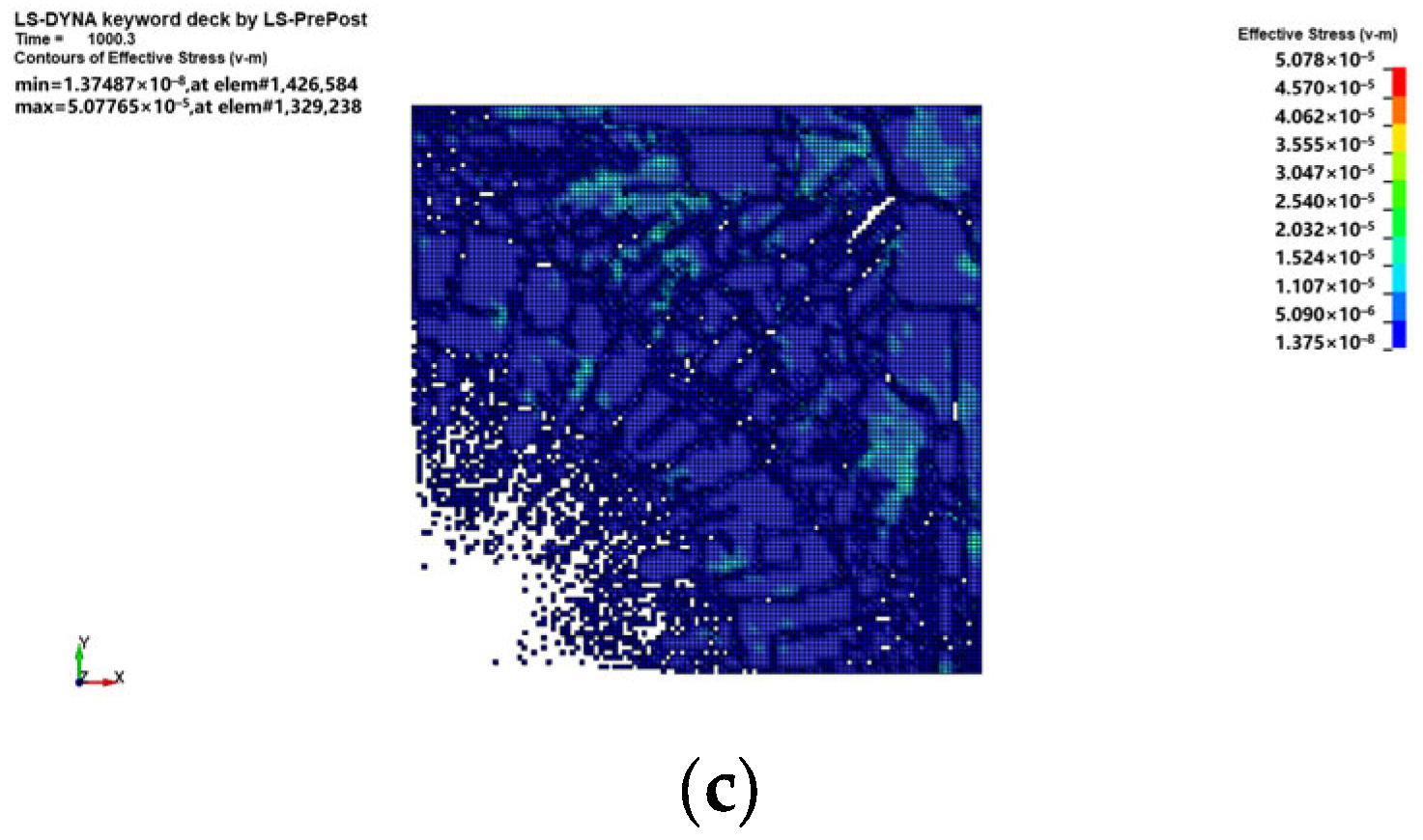
The established ice blasting model was used to analyze condition 1 regarding the impact of different ignition methods on the blast volume of ice bodies when the bottom was set as a non-reflective surface. The equivalent pressure distribution plot is shown in
Figure 3.
As can be seen from the figure, the maximum pressure of the equivalent pressure cloud at the center ignition was 6.89966 × 10−5 GPa, the maximum pressure of the bottom ignition was 5.07765 × 10−5 GPa, and the minimum pressure of the top ignition was 4.25708 × 10−5 GPa. In addition, analysis of its pressure distribution showed that the center ignition explosion was generated by the most concentrated pressure distribution, the top of the ignition explosion was generated by the most dispersed pressure distribution, and the bottom of the ignition explosion was generated by the pressure distribution between these. Furthermore, the analysis of pressure distribution showed that the center ignition pressure distribution was the most concentrated, the top ignition pressure distribution was the most dispersed, and the bottom ignition was between the two.
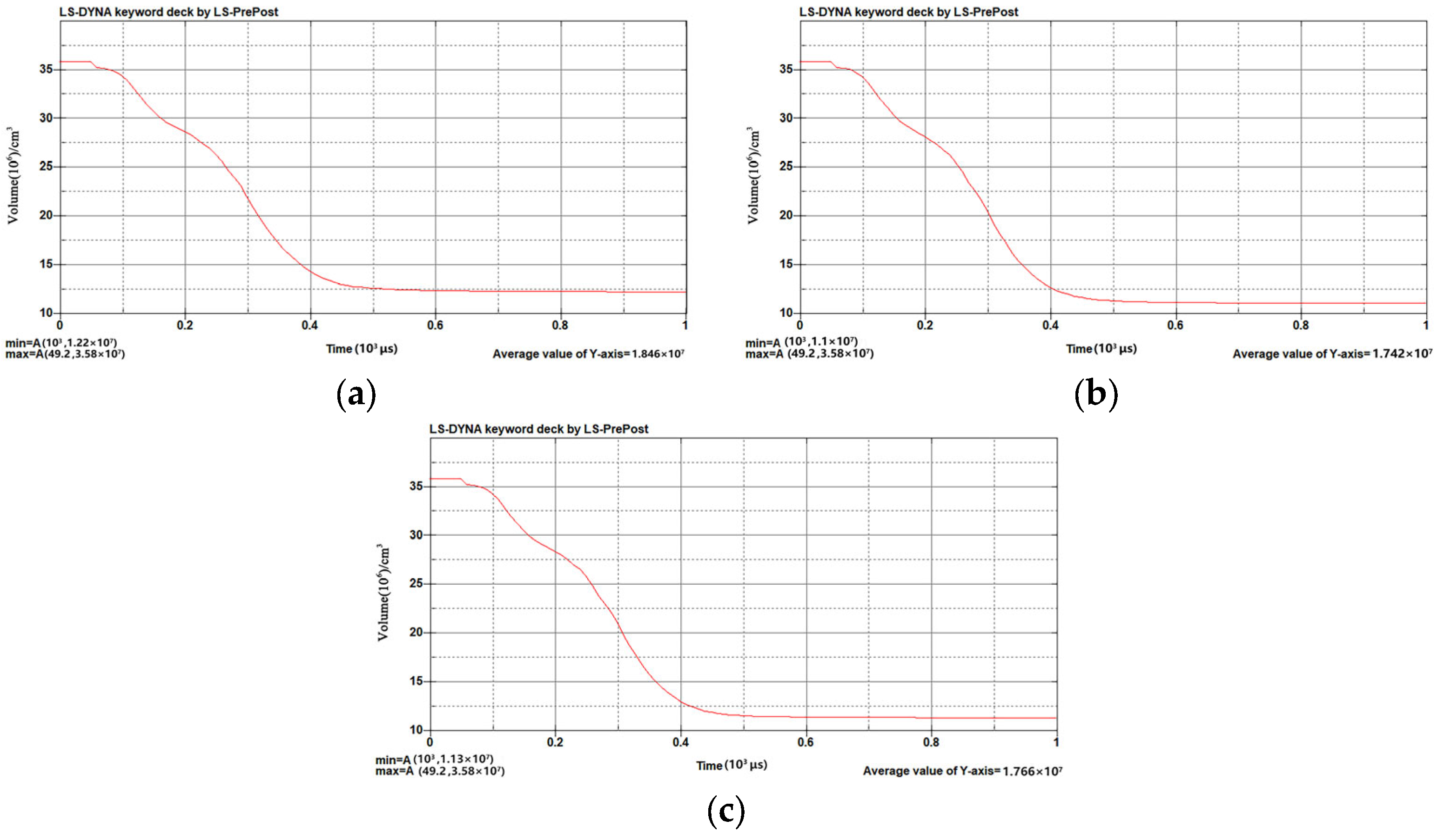
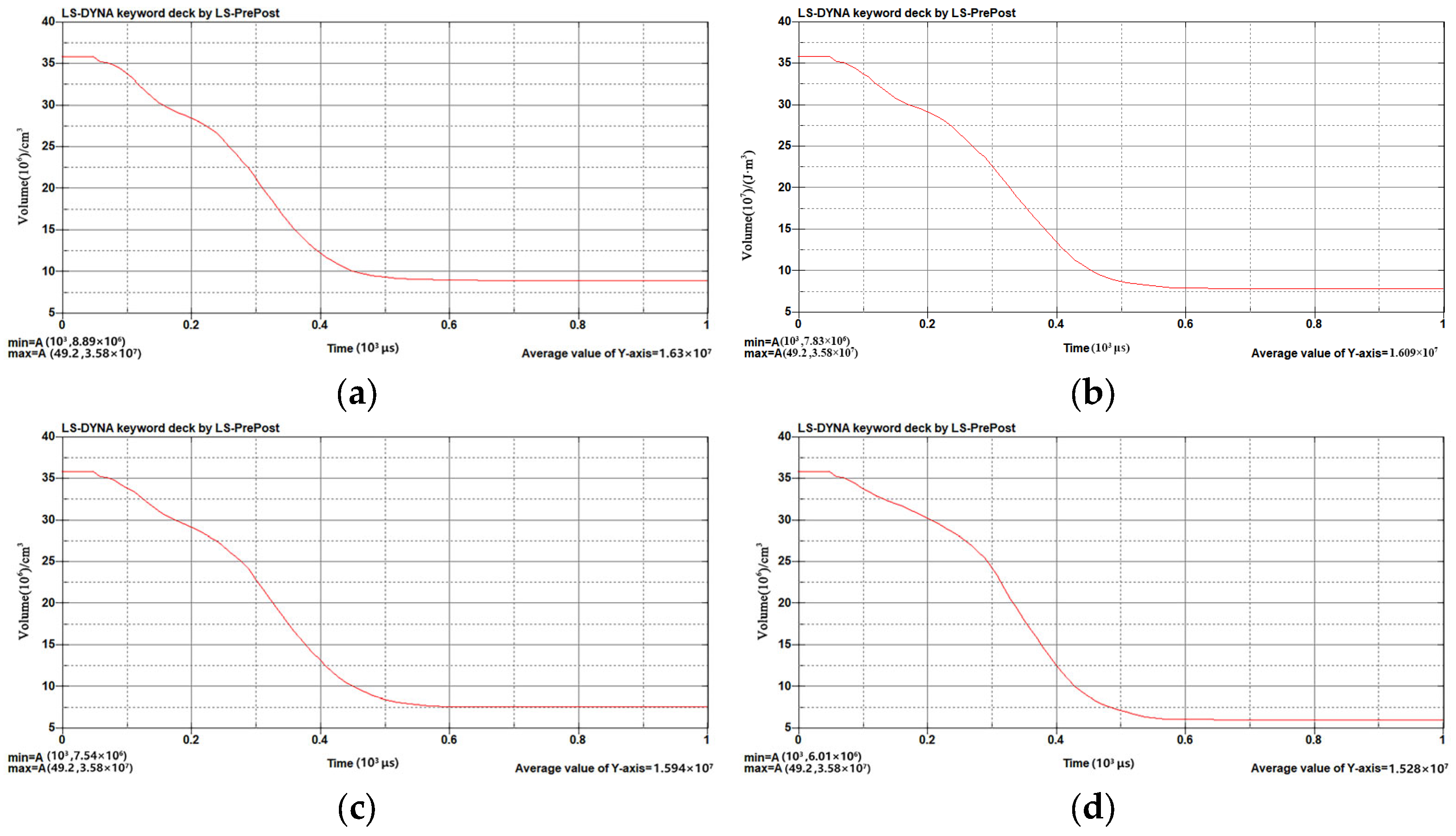
The time variation of ice blast volume for different ignition modes is shown in
Figure 4.
An analysis of the blast volume in time at different locations of ignition showed that there was no effect of ignition at different locations at the beginning of blasting, there was a significant volume change from 49.2 μs on, and the remaining volume after blasting was 1.1 × 107 cm3 at the center ignition, 1.13 × 107 cm3 at the bottom ignition, and 1.22 × 107 cm3 at the top ignition. The volume of blasting caused by the center ignition was the largest, followed by the bottom ignition, and the volume of blasting caused by the top ignition was the smallest.
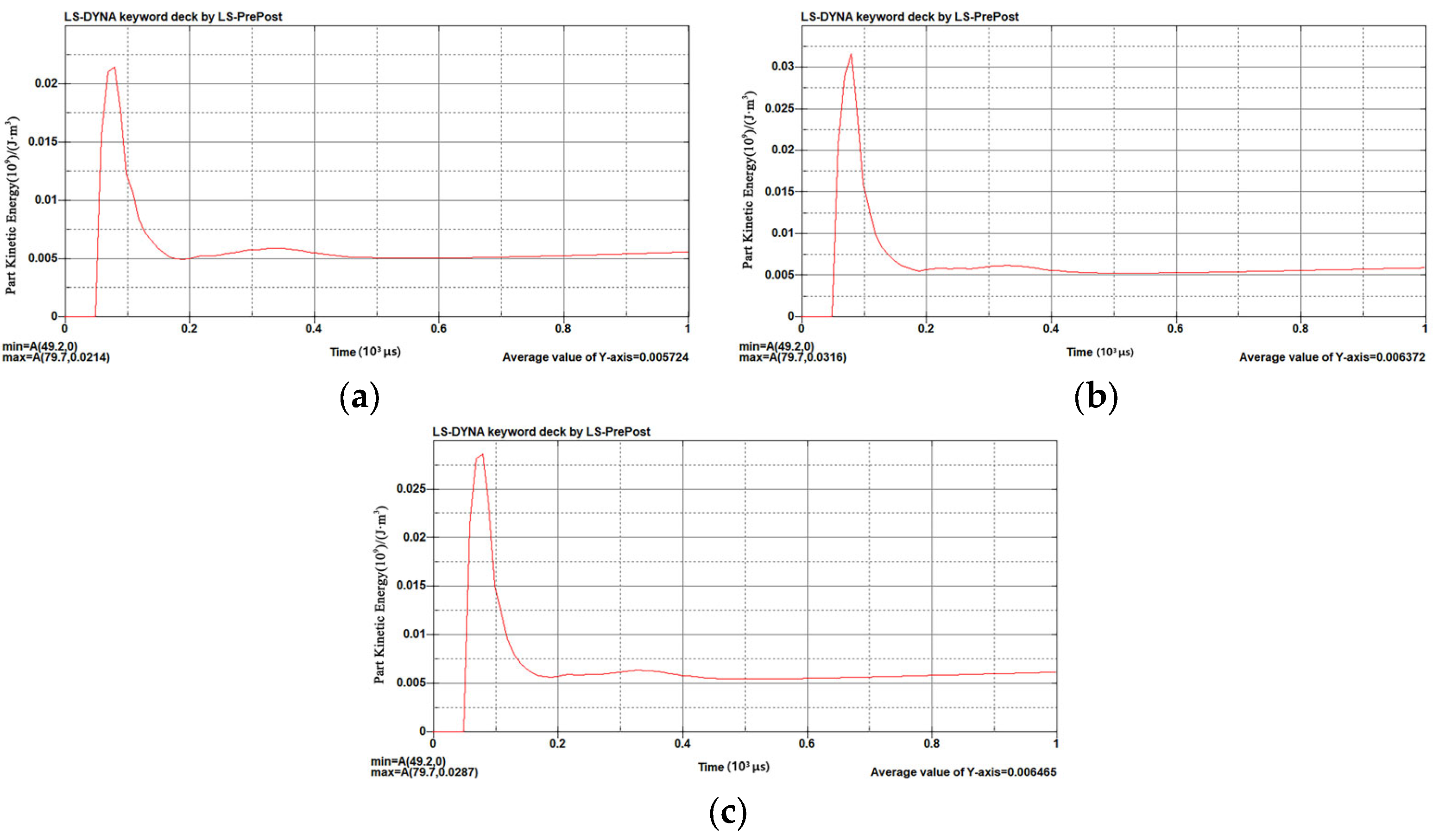
The change in kinetic energy at the moment of detonation for different ignition methods is shown in
Figure 5.
The figure shows that all three ignition methods resulted in a maximum kinetic energy at 79.7 μs following the maximum initial kinetic energy directly at 49.2 μs. The maximum initial kinetic energy was reached by wave transmission in a short time, and the maximum initial kinetic energy was 3.16 × 107 J/m−3 at the center ignition, 2.87 × 107 J/m−3 at the bottom ignition, and 2.14 × 107 J/m−3 at the top ignition.
The relationships between ignition position and ice blasting volume, final maximum pressure, and instantaneous kinetic energy are shown in
Table 5.
3.2. Analysis of the Influence of Explosive Masses on Ice Sheet
The established ice blasting model was used to analyze simulated condition 2 regarding the effect of different explosive masses on the ice blasting volume at center ignition. Equivalent pressure distributions are shown in
Figure 6.
From the figure, it can be seen that the maximum pressure of the equivalent pressure cloud was 6.89966 × 10−5 GPa when the explosive mass was 1.6 kg, the maximum pressure of the equivalent pressure cloud was 6.16525 × 10−5 GPa when the explosive mass was 3.2 kg, the maximum pressure of the equivalent pressure cloud was 7.77053 × 10−5 GPa when the explosive mass was 4.8 kg, the maximum pressure of the equivalent pressure cloud was 4.02354 × 10−5 GPa when the explosive mass was 6.4 kg, and the maximum pressure of the equivalent pressure cloud was 6.62249 × 10−5 GPa when the explosive mass was 8.0 kg. The maximum pressure of the equivalent pressure changes in waves.
The time variations of the ice blast volume for different explosive masses are shown in
Figure 7.
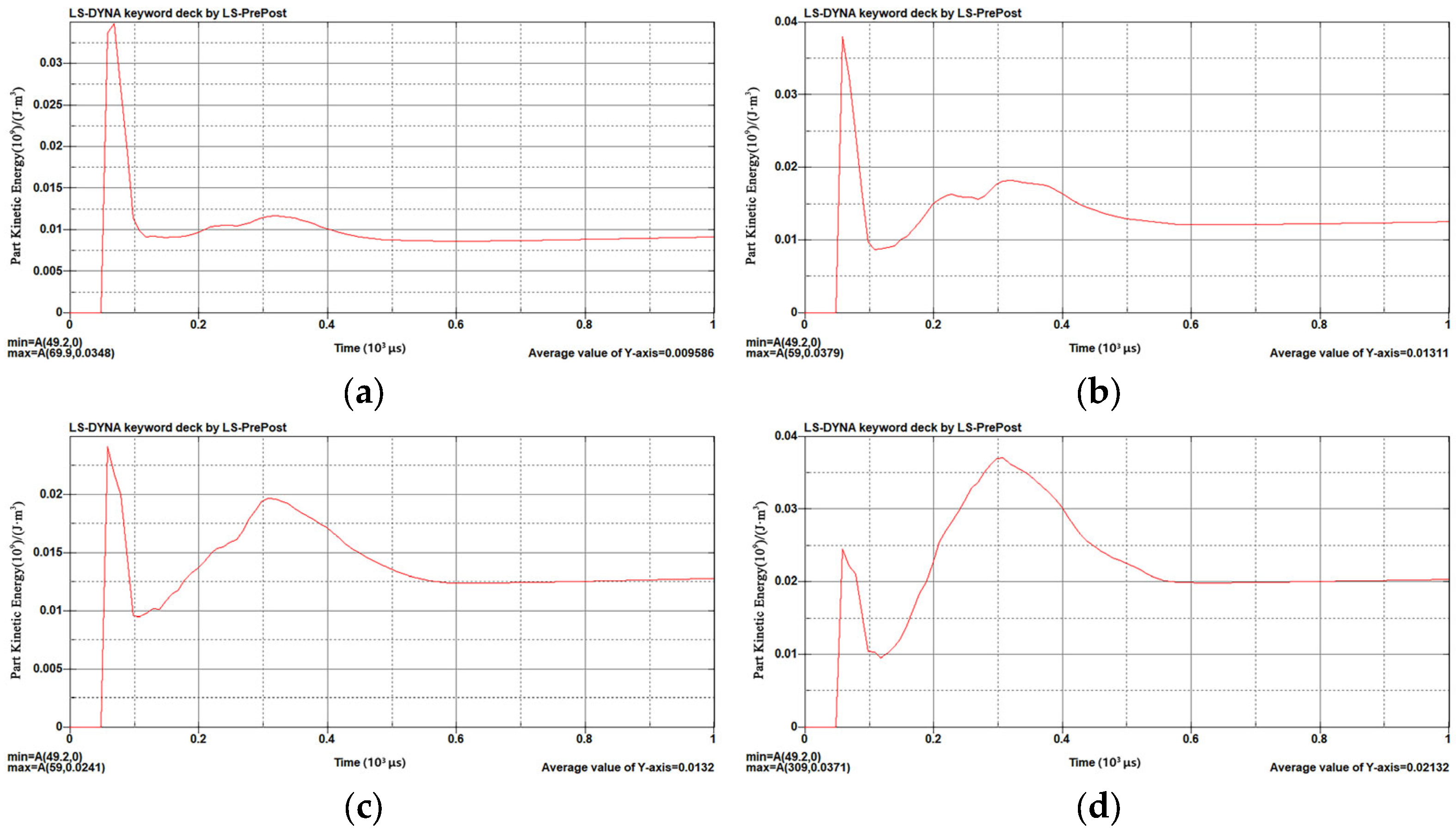
The instantaneous kinetic energy changes for different explosive masses are shown in
Figure 8.
The relationships between explosive mass and ice blasting volume, maximum pressure at the end, instantaneous kinetic energy, and average kinetic energy are shown in
Table 6.
From the above table, the volume of explosive blast is found to increase gradually as the mass of explosive increases. However, in the third column of the table, the blast volume is not found to increase linearly when the explosive mass increases from 1.6 kg to 6.4 kg. Here, the blast volume is found to increase gradually and more slowly, but when the explosive mass reaches 8 kg, a significant increase in blast volume can be observed. Therefore, an increase in blasting efficiency is assumed to be possible by increasing the number of explosive packages. However, as the number of individual explosive packages increases, the blasting effect will gradually approach its limit. This limit can only be exceeded by using a large quantity of explosives. When observing the maximum kinetic energy when blasting different masses of explosives, it was found that the maximum kinetic energy (3.16 × 107 J/m−3) was generated when the mass of explosives was 4.8 kg. As the explosive mass increased, the average kinetic energy of the ice layer was gradually increasing. However, the average kinetic energy generated by the explosion of 6.4 kg of explosives did not exhibit a significant change compared to the average kinetic energy generated by the explosion of 4.8 kg of explosives.
3.3. Analysis of the Effect of Explosive Location on Ice Blasting Model
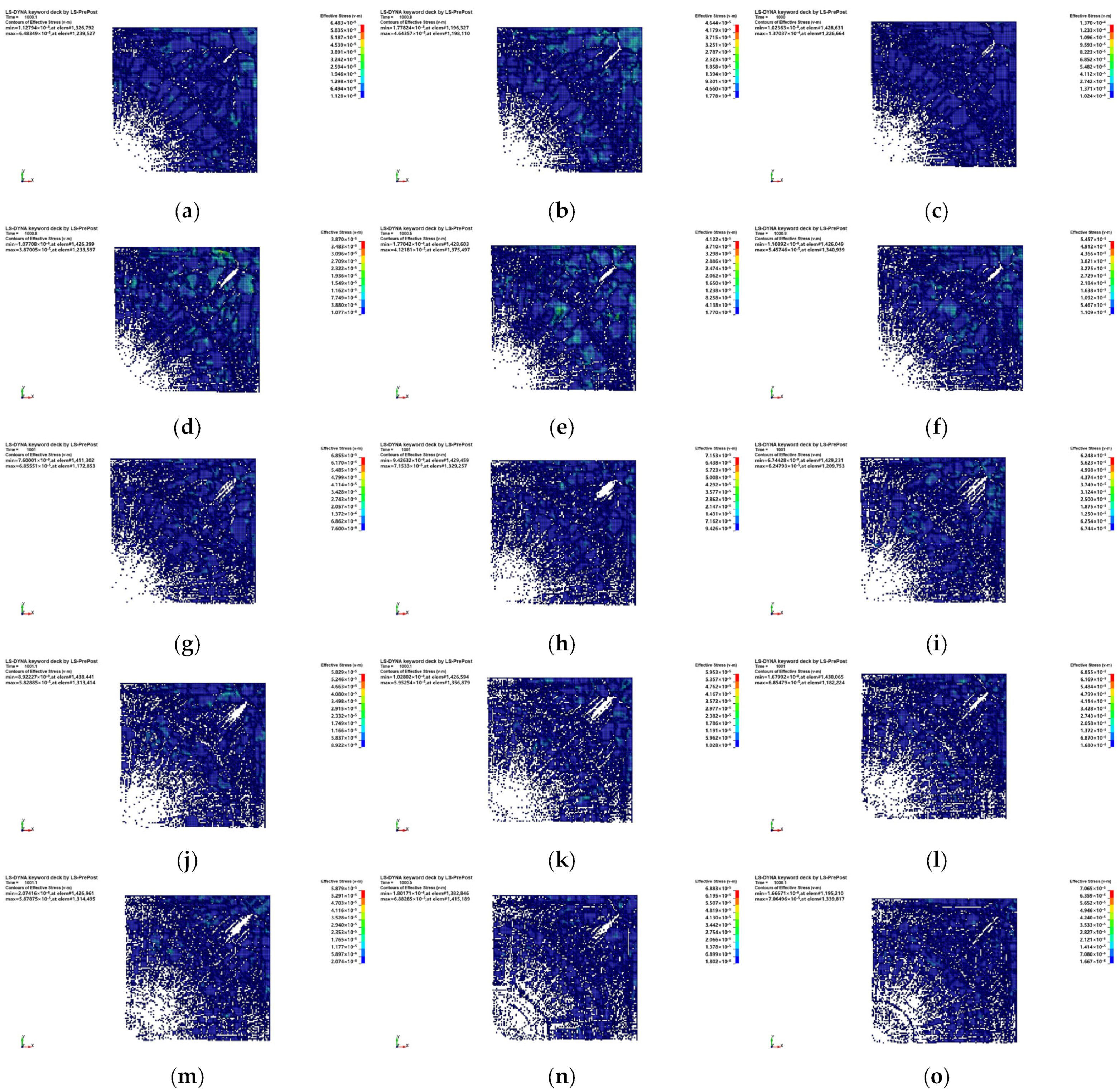
The established ice blasting model was used to analyze simulated working condition 3 regarding the effect of different positions of explosives on the blasting volume of the ice body at the center ignition and an ice thickness of 100 cm. The stress effects are shown in
Figure 9a–o.
The simulation results regarding the effects of different distances between explosives and the ice model on the ice blasting under working condition 3 are shown in
Table 7.
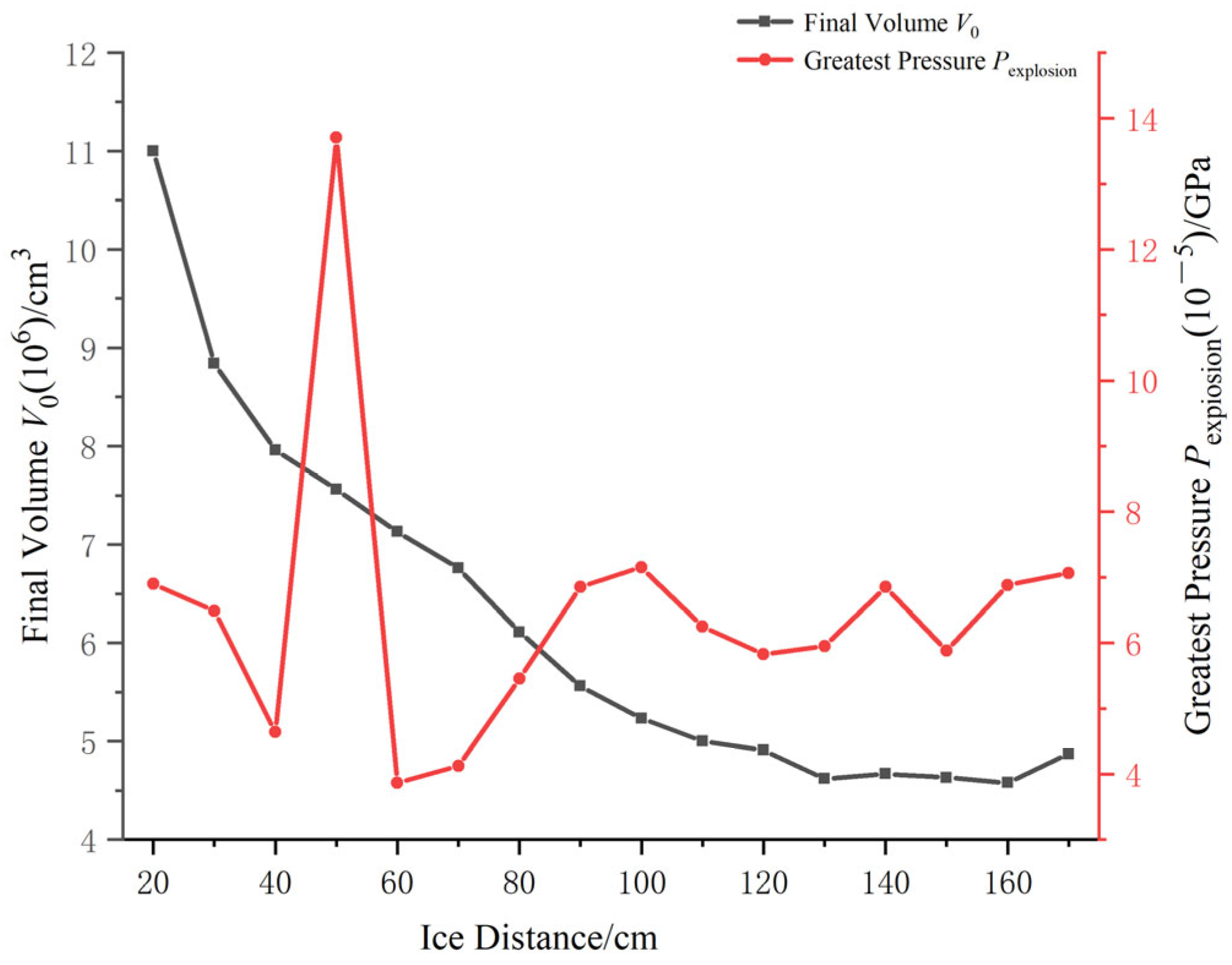
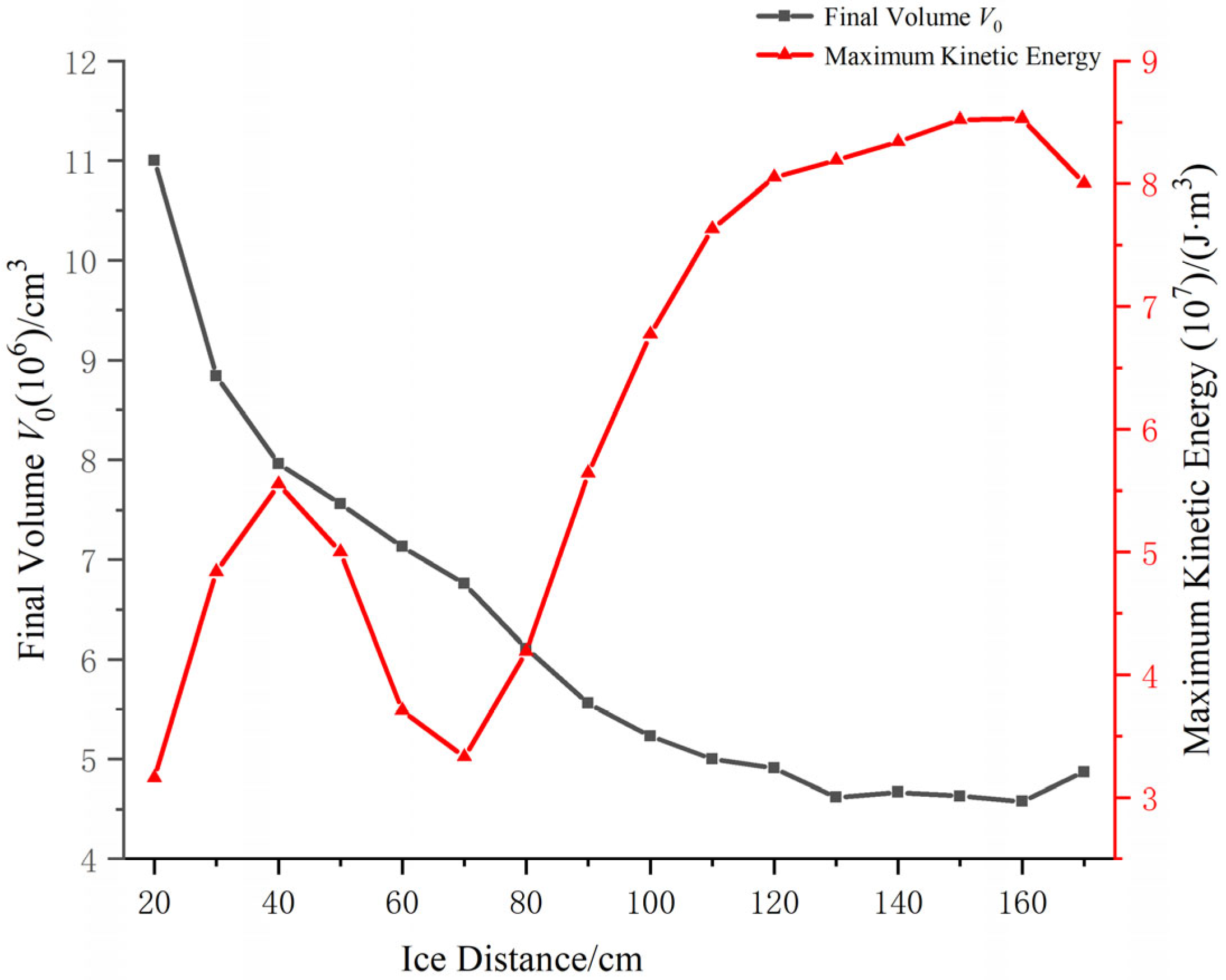
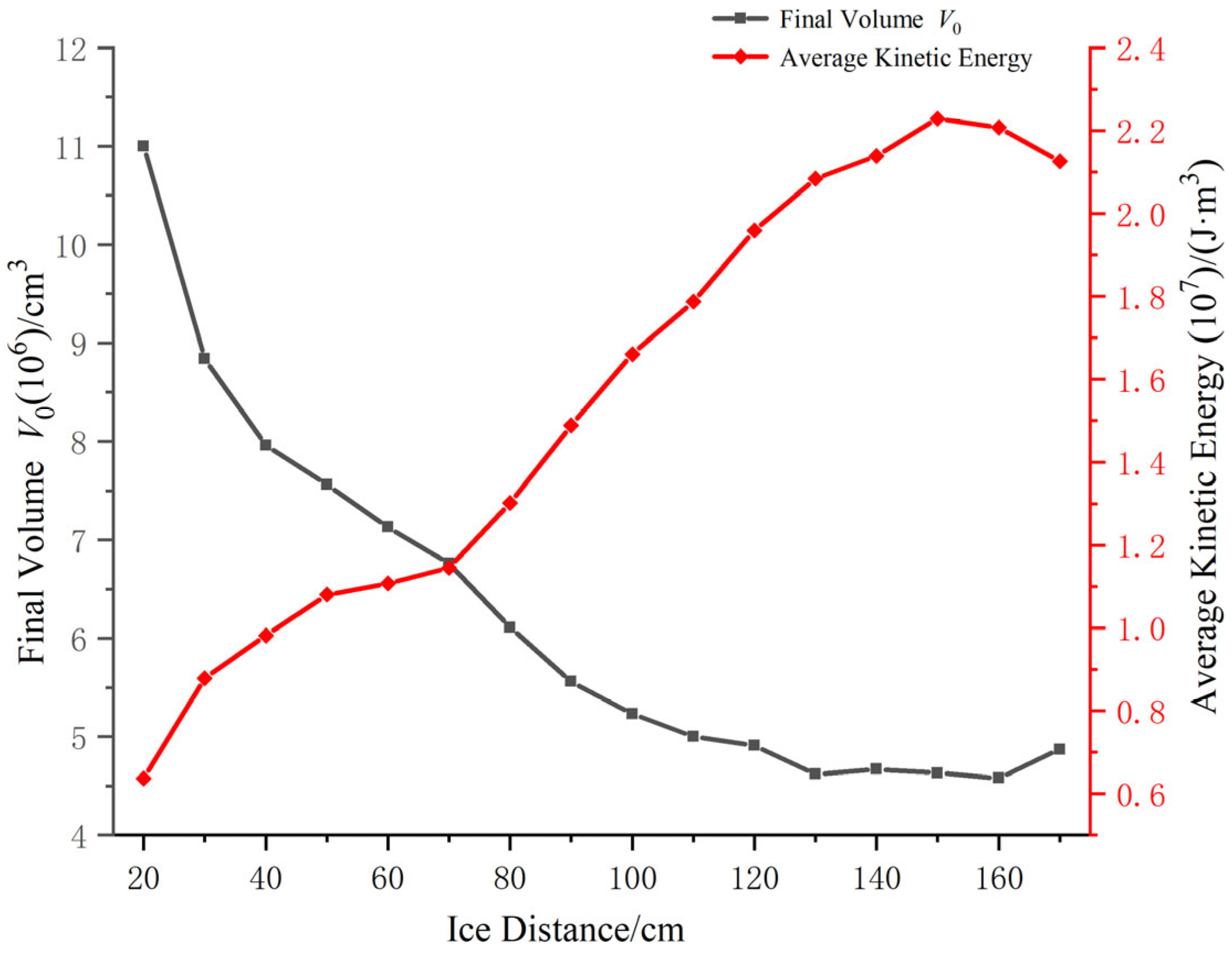
Table 7 shows that at different distances between the explosive and ice model, the blasting effects were also different.
Figure 10,
Figure 11 and
Figure 12 illustrate the relationships between different distances between the explosive and ice model and ice blasting volume, maximum pressure at the end, instantaneous kinetic energy, and average kinetic energy.
Figure 10 shows that when the distance between the dynamite and ice model was 20 to 120 cm, the larger the distance between the dynamite and ice model, the smaller the residual volume of the ice model after explosion and the more obvious the blasting effect. When the distance between the explosive and the ice model exceeded 160 cm, the residual volume of the ice model after explosion increased with the increase in the distance. As the distance between the dynamite and ice model increased, the maximum pressure at the end of the ice model changed in a wave-like manner, similar to the propagation of underwater explosion waves.
As the distance between the explosive and ice model increased, the maximum kinetic energy of the ice model reached a peak at 40 cm between the explosive and ice model. When the distance between the explosive and ice model was 70 to 160 cm, the maximum kinetic energy of the ice model increased with the increase in distance. When the distance between the explosive and ice model exceeded 160 cm, the maximum kinetic energy decreased with the increase in the distance.
Figure 12 shows that when the distance between the explosive and ice model was 20 to 150 cm, the average kinetic energy of the ice model increased with the increase in the distance; when the distance between the explosive and the ice model exceeded 150 cm, its average kinetic energy decreased with the increase in the distance.
4. Discussion
In the downstream section of Beiji Village, Mohe, between navigation mark 812 and 818, the ice thickness of approximately 100 cm was selected for the blasting experiments with three different ignition methods. The experimental results show that the central ignition blasting method produces the best explosion effect and the largest explosion radius, and the measured explosion radius is 387.5 cm, which is in line with the results obtained in condition 1 (working conditions of three different ignition methods), thus proving the reasonability of the simulation. In addition, after the explosion, it was found by observing the shape of the ice body that the explosion in the center produces a nearly circular blasting pit, but there are circular cracks in its outer ring, indicating that the ice explosion is not finished at one time, but carries on the energy transfer in the form of waves.
With the increase in the explosive mass, the blasting effect decreased gradually, and the impact could be increased by increasing the number of charge bags; however, as the number of individual charge bags increased, the blasting effect gradually approached the limit. Experimental verification indicated that when 1.6 kg TNT explosive explodes in a river section with an ice thickness of 1 m, the effective blasting area is a circular area with a radius of 8 m, thereby proving the reasonability of the simulation results in working condition 2.
In this case, it can be concluded that the explosive bags should be reasonably laid in a staggered arrangement in two rows of ice holes in the adjacent upstream and downstream sections along the direction of water flow, and the distance between the holes in the row and the distance of the adjacent row should be roughly equal. In addition, the effect of the broken ice sheet is the best in the case of an equal charge amount of each hole. The layout of explosive package hole distance is restricted by the water depth, ice thickness, and single hole charge, and the hole distance cannot be too large, or it cannot blast through the ice hole, and the icebreaking effect will be unfavorable as well, even if the single hole charge is large. If the blasting ice holes are arranged in the direction of the water flow along the river and the front and back rows are staggered, the blasting effect will be rather favorable and can effectively destroy the ice sheet and ensure that the scale of the ice is in line with the requirements of the river conditions for transporting the ice sheet.
Under the condition of an ice thickness of approximately 100 cm in the river section and an explosive mass of 1.6 kg, the blasting test was carried out in the ice area where the distance between the explosive and the ice was 20–200 cm (with 20 cm as the difference value). In order to facilitate the analysis, the explosion radius was measured, and it was found that the explosion effect on site is highly similar to the results simulated using the ANSYS model. The experimental results show that the blasting radius is the largest and the fracture effect of the ice body is the best in the case of a distance of 160 cm, which is in line with the results obtained in condition 3.
At the same time, the breakup of the ice of the main stream of the Heilongjiang River was performed after blasting for 4 days, during which the typical ice dam was observed near the 10 km overland route opening in the upper reach of the Mohe cross section at the blasting location during the period, since the ice blocks from the upper reach were accumulated and blocked. The field observation and theoretical reasoning results indicate a high probability that the ice dam will occur in the blasting river reach, and the resulting force will also be greater if the lower reach cross section of the ice dam is not blasted, without conditions for ice block transport in the blasting area. Since the slope of the river reach is small and has a great degree of curvature, it is considered the place where the water flow is mixed violently in the river. In this case, it is rather obvious that the river ice blasting plays a critical role in preventing an ice disaster in the Heilongjiang River.
5. Conclusions
The main conclusions drawn are as follows:
It can be concluded through model simulation and experimental comparison that the blasting effect in the center is the best, and the blasting volume and radius are the largest when the explosive is ignited under the ice;
With the increase in the explosive mass, the blasting effect will decrease gradually, and the impact can be increased by increasing the number of charge bags but will gradually approach the limit as the number of individual charge bags increases. Experimental verification indicates that 1.6 kg TNT can blast the river section with an ice thickness of 1 m, and the effective blasting area is a circular area with a radius of about 8 m;
The explosive bags should be reasonably laid, in staggered arrangement in two rows of ice holes in the adjacent upstream and downstream section along the direction of water flow, and the distance between the holes in the row and the distance of the adjacent row should be roughly equal;
In addition, the effect of the broken ice sheet is the best in the case of an equal charge amount of each hole. An ice blast is not finished at once, but experiences energy transfer in the form of waves. When the distance between the explosive and the ice is about L = 150 cm, the blasting effect is the best.
However, the simulation of the model needs to be improved in some areas. In the simulation, the temperature was not set, but the effect of temperature on the strength of the ice sheet is indeed the main factor. In general, the ice sheet intensity decreases with the decrease in temperature, and the blasting effect of river ice should be different at different temperatures. According to the experimental and field observation data of the blasting observation, ice sheet blasting can be carried out in the case of a stable and positive maximum temperature of the day, when the ice sheet is still hard in texture and high in efficiency. Breaking ice will break too early and will refreeze, and even destroy all previous efforts, resulting in waste. In this case, it is advisable to carry out ice blast 3–5 days before the opening of the river. During the opening period, the existence time of river ice is short, making it difficult to grasp the timing of the blasting simulation and field experiment, and the number of experiments is rather limited. Considering the influence of river hydraulic and thermal factors, the numerical simulation is combined under hydraulic and thermal conditions, and it is more difficult to simulate under complex hydraulic conditions. To this end, the following solutions to close the gap are hereby proposed: (i) to improve the cross application of multiple disciplines; (ii) to fully combine the method with various coupling factors, consider turbulent conditions of hydrothermal conditions, and carry out a deeper theoretical analysis of the model under the boundary treatment and scene setting; and (iii) to combine with practice, perform more field tests, provide data for laboratory tests, and create similarity analyses at the same time.