A Novel Method of Design Flood Hydrographs Estimation for Flood Hazard Mapping
Abstract
1. Introduction
2. Materials and Methods
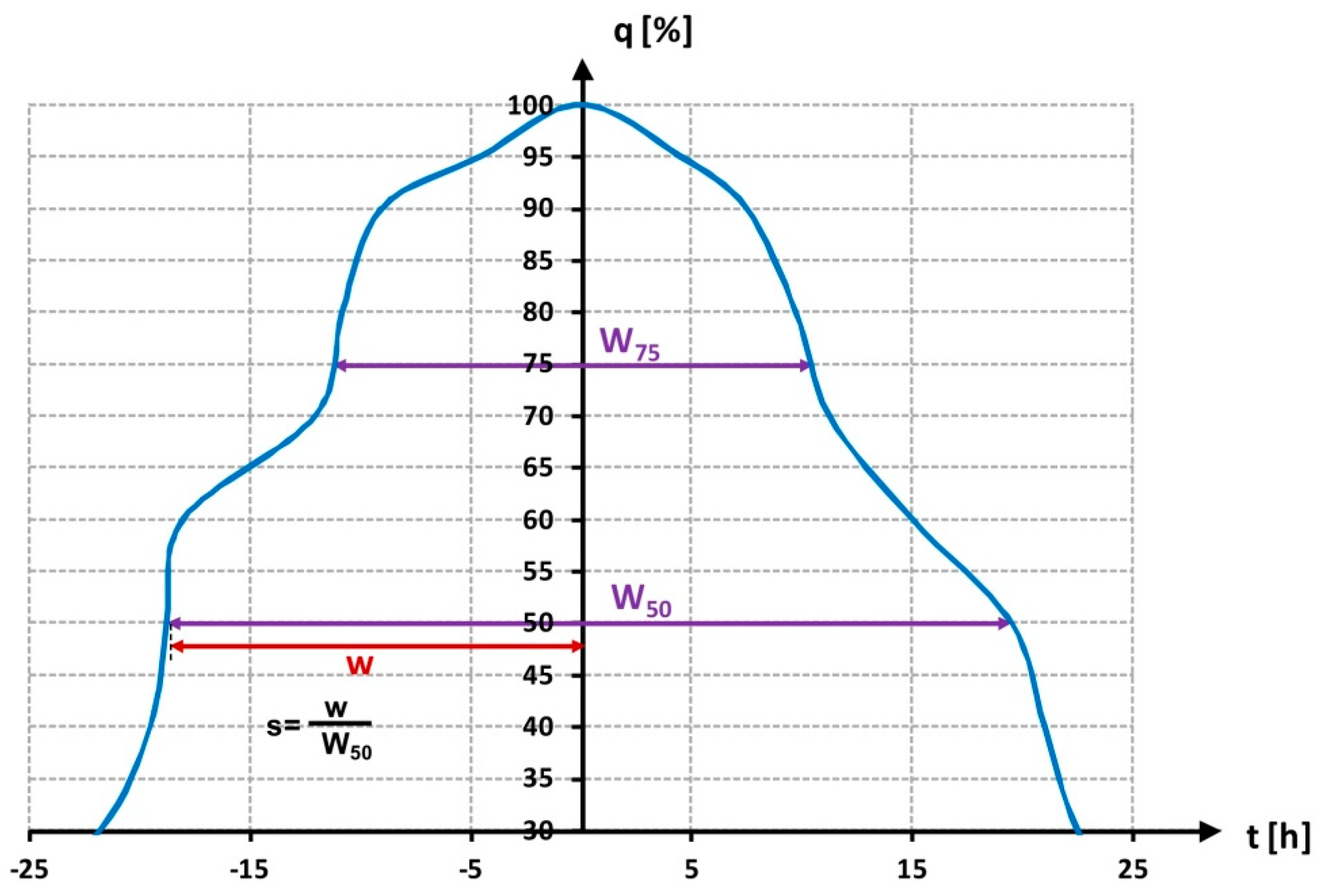
2.1. Nonparametric DFH Estimation
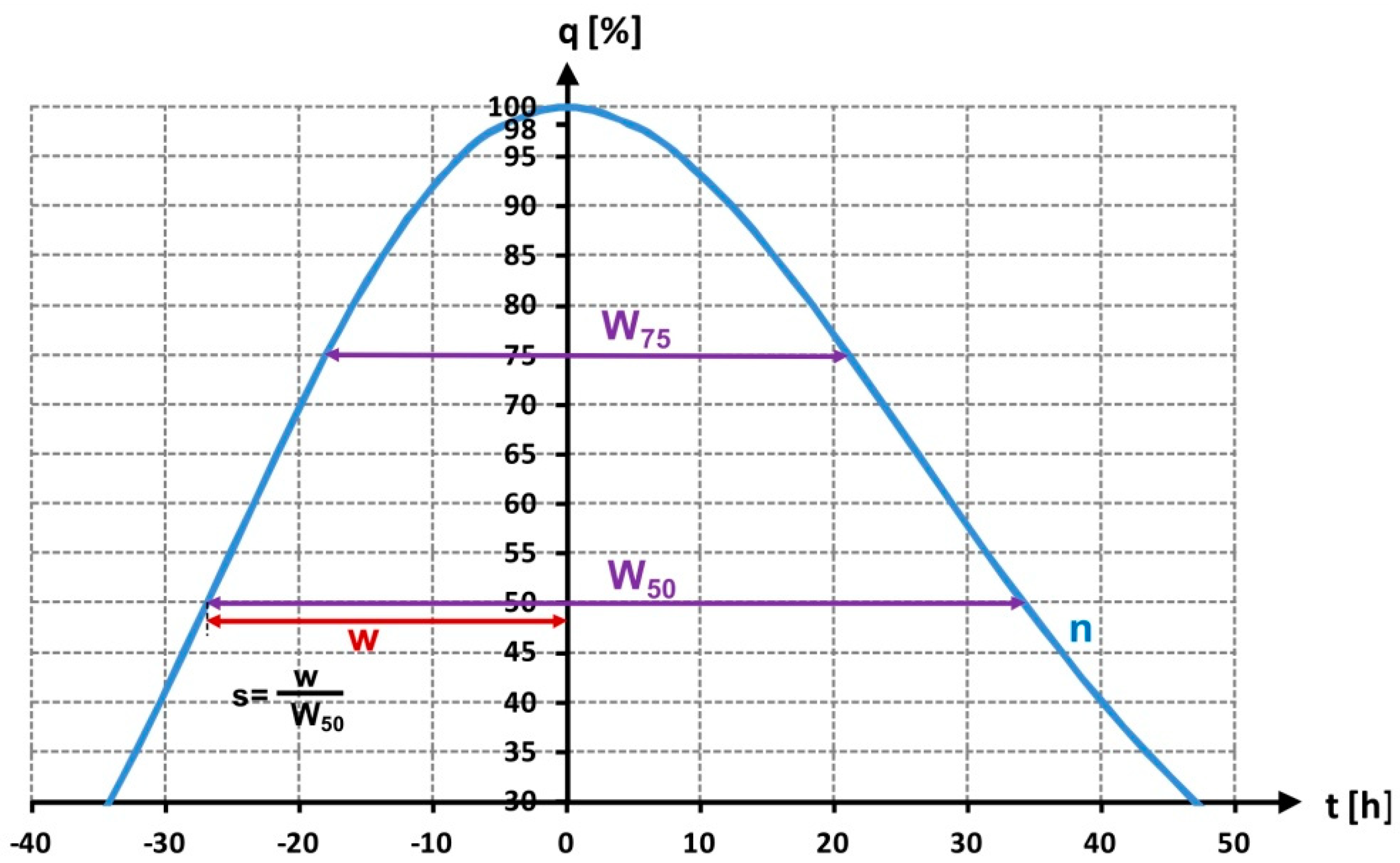
2.2. Parametric DFH Estimation
2.3. Hybrid Nonparametric and Parametric Approach
- Construction of nonparametric DFH using the Archer method based on long-term flow measurements for selected study catchments.
- Estimation of flood wave properties from developed nonparametric DFH: hydrograph width W50 at percentage p = 50% of peak flow, W75 at percentage p = 75% of the peak flow and a skewness coefficient s, representing catchment characteristics referred to as hydrograph descriptors.
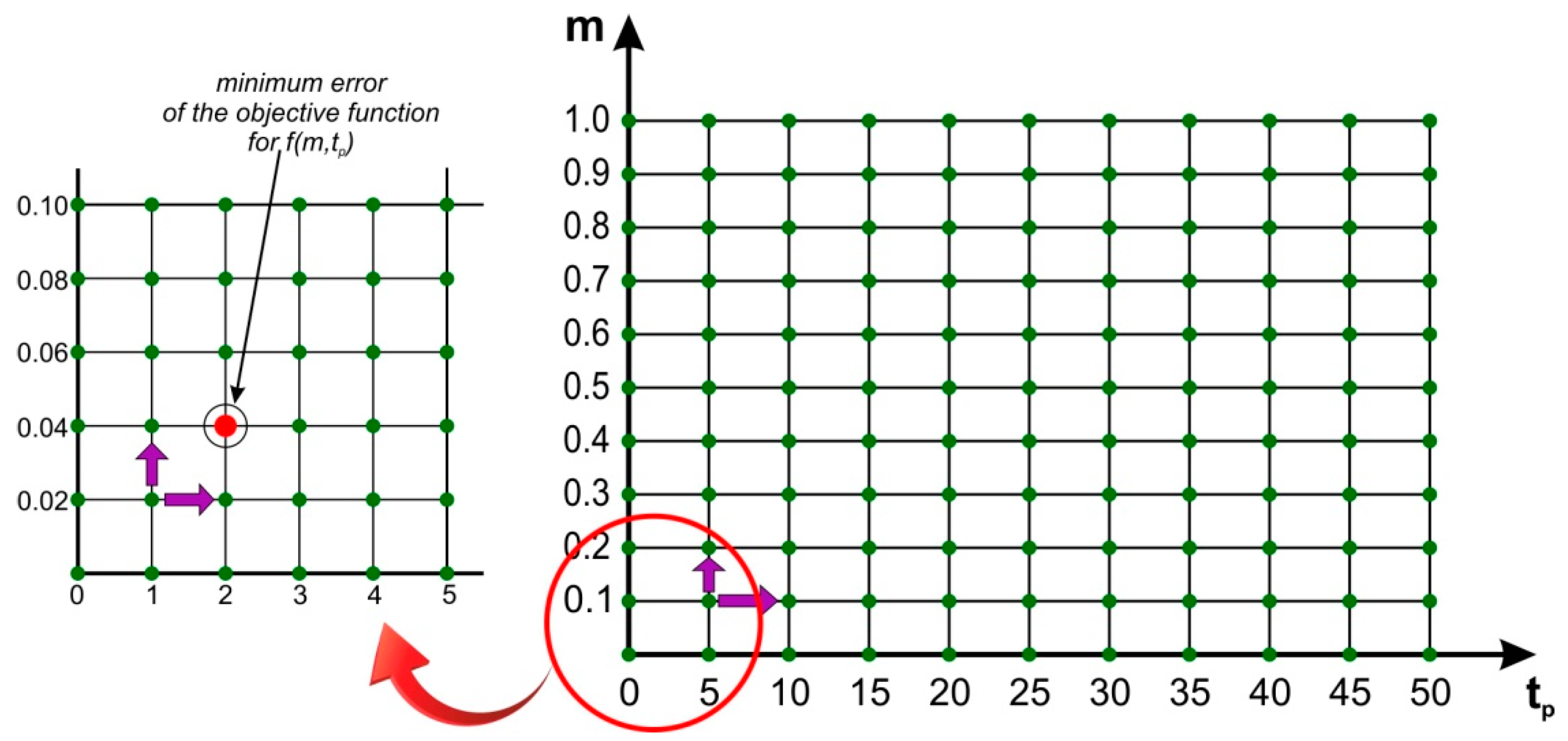
- Based on the developed hydrograph descriptors (step b), estimation of the parameters of the parametric DFH.
- Validation of the correspondence between nonparametric DFH and parametric DFHs to recommend the parametric distribution model that best reproduces analyzed catchments’ flood wave properties.
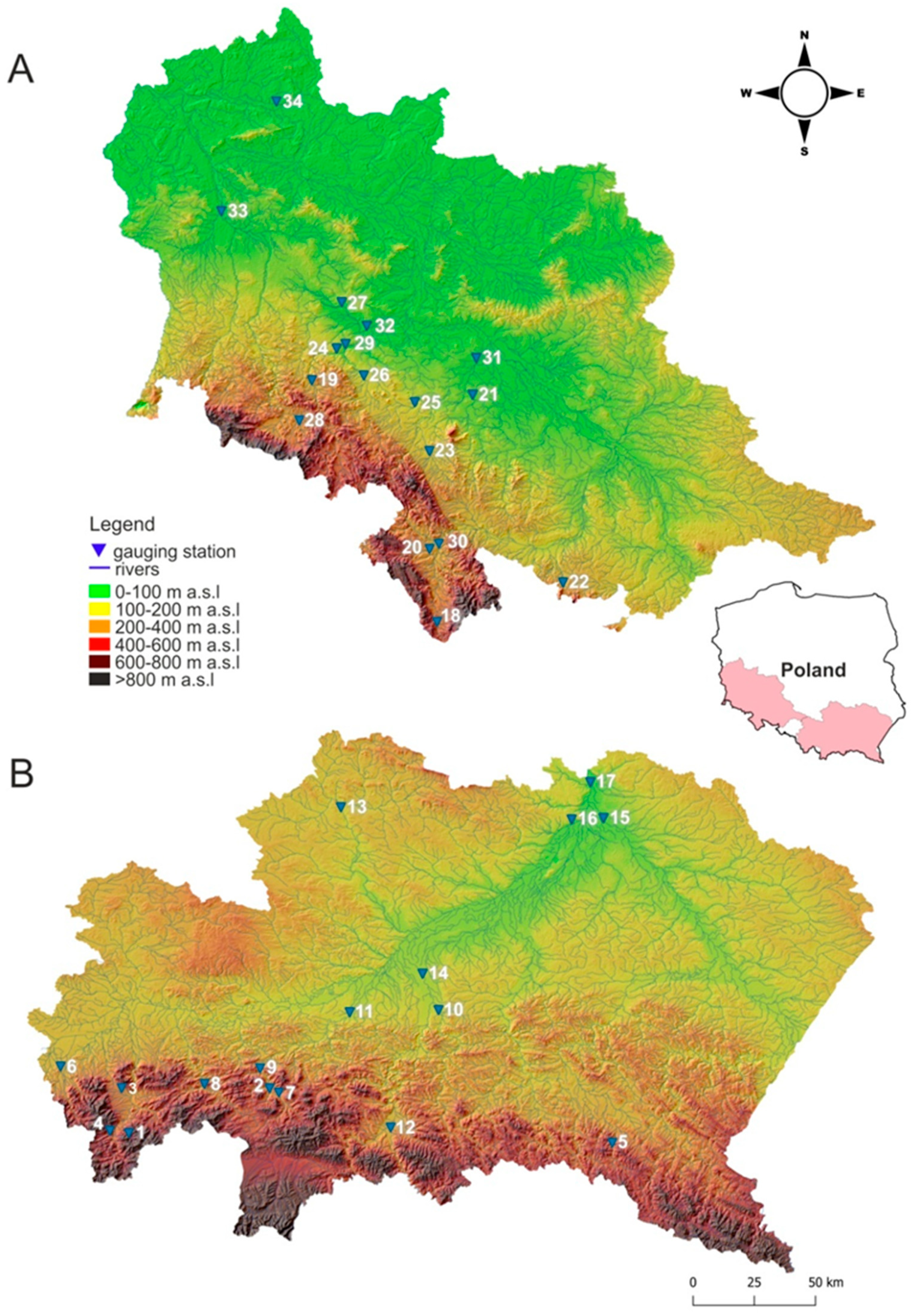
2.4. Study Area
3. Results
4. Conclusions and Discussion
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Shreevastav, B.B.; Tiwari, K.R.; Mandal, R.A.; Nepal, A. Assessing Flood Vulnerability on Livelihood of the Local Community: A Case from Southern Bagmati Corridor of Nepal. Prog. Disaster Sci. 2021, 12, 100199. [Google Scholar] [CrossRef]
- Vrijling, J.K.; van Hengel, W.; Houben, R.J. Acceptable Risk as a Basis for Design. Reliab. Eng. Syst. Saf. 1998, 59, 141–150. [Google Scholar] [CrossRef]
- Apel, H.; Thieken, A.H.; Merz, B.; Blöschl, G. A Probabilistic Modelling System for Assessing Flood Risks. Nat. Hazards 2006, 38, 79–100. [Google Scholar] [CrossRef]
- Criss, R.E.; Winston, W.E. Properties of a Diffusive Hydrograph and the Interpretation of Its Single Parameter. Math. Geosci. 2008, 40, 313–325. [Google Scholar] [CrossRef]
- Serinaldi, F.; Grimaldi, S. Synthetic Design Hydrographs Based on Distribution Functions with Finite Support. J. Hydrol. Eng. 2011, 16, 434–446. [Google Scholar] [CrossRef]
- Hattermann, F.F.; Kundzewicz, Z.W.; Huang, S.; Vetter, T.; Gerstengarbe, F.-W.; Werner, P. Climatological Drivers of Changes in Flood Hazard in Germany. Acta Geophys. 2013, 61, 463–477. [Google Scholar] [CrossRef]
- Alfieri, L.; Feyen, L.; Salamon, P.; Thielen, J.; Bianchi, A.; Dottori, F.; Burek, P. Modelling the Socio-Economic Impact of River Floods in Europe. Nat. Hazards Earth Syst. Sci. 2016, 16, 1401–1411. [Google Scholar] [CrossRef]
- Goswami, M. Generating Design Flood Hydrographs by Parameterizing the Characteristic Flood Hydrograph at a Site Using Only Flow Data. Hydrol. Sci. J. 2020, 1–19. [Google Scholar] [CrossRef]
- Jain, S.K. Engineering Hydrology: An Introduction to Processes, Analysis, and Modeling; McGraw-Hill Education: New York, NY, USA, 2019; ISBN 978-1-259-64197-8. [Google Scholar]
- Snyder, F.F. Synthetic Unit-Graphs. Trans. AGU 1938, 19, 447. [Google Scholar] [CrossRef]
- Chow, V.T.; Maidment, D.R.; Mays, L.W. Applied Hydrology, international ed.; McGraw-Hill series in water resources and environmental engineering; McGraw-Hill: New York, NY, USA, 1988; ISBN 978-0-07-100174-8. [Google Scholar]
- USDA Hydrographs, Part 630, Hydrology, Hydraulics and Hydrology-Technical References NRCS National Engineering Handbook; U.S. Department of Agriculture: Washington, DC, USA, 2007.
- Garrett, R.C.; Woolverton, A.H. The Unit Hydrograph-Its Construction and Uses; Texas State Board of Water Engineers: Austin, TX, USA, 1951. [Google Scholar]
- Ozga-Zielińska, M.; Gądek, W.; Książyński, K.; Nachlik, E. Mathematical Model of Rainfall-Runoff Transformation-WISTOO. In Mathematical Models of Large Watershed Hydrology; Singh, V.P., Frevert, D.K., Eds.; Water Resources Publications: Highlands Ranch, CO, USA, 2002; pp. 811–860. ISBN 978-1-887201-34-6. [Google Scholar]
- Wałęga, A. Application of HEC-HMS Programme for the Reconstruction of a Flood Event in an Uncontrolled Basin/Zastosowanie Programu HEC-HMS Do Odtworzenia Wezbrania Powodziowego w Zlewni Niekontrolowanej. J. Water Land Dev. 2013, 18, 13–20. [Google Scholar] [CrossRef][Green Version]
- Pietrusiewicz, I.; Cupak, A.; Wałęga, A.; Michalec, B. The Use of NRCS Synthetic Unit Hydrograph and Wackermann Conceptual Model in the Simulation of a Flood Wave in an Uncontrolled Catchment/Zastosowanie Syntetycznego Hydrogramu Jednostkowego NRCS Oraz Konceptualnego Modelu Wackermana Do Symulacji Fali Wezbraniowej w Zlewni Niekontrolowanej. J. Water Land Dev. 2014, 23, 53–59. [Google Scholar] [CrossRef]
- Gądek, W.; Bodziony, M. The Hydrological Model and Formula for Determining the Hypothetical Flood Wave Volume in Non-Gauged Basin. Meteorol. Hydrol. Water Manag. 2015, 3, 3–10. [Google Scholar] [CrossRef]
- Methods of Hydrological Computations for Water Projects: A Contribution to the International Hydrological Programme: Report; Eichert, B., Ed.; Studies and reports in hydrology; Unesco: Paris, France, 1982; ISBN 978-92-3-102005-6. [Google Scholar]
- Archer, D.; Foster, M.; Faulkner, D.; Mawdsley, J. The Synthesis of Design Flood Hydrographs; All Jeremy Benn Associates, Gillow House, Broughton Hall, Skipton: New York, NY, USA, 2000. [Google Scholar]
- Sauquet, E.; Ramos, M.-H.; Chapel, L.; Bernardara, P. Streamflow Scaling Properties: Investigating Characteristic Scales from Different Statistical Approaches. Hydrol. Processes 2008, 22, 3462–3475. [Google Scholar] [CrossRef]
- Gądek, W.; Baziak, B.; Tokarczyk, T. Nonparametric Design Hydrograph in the Gauged Cross Sections of the Vistula and Odra Basin. Meteorol. Hydrol. Water Manag. 2017, 5, 53–61. [Google Scholar] [CrossRef]
- Gądek, W.; Baziak, B.; Tokarczyk, T. Strupczewski Method for Parametric Design Hydrographs in Ungauged Cross-Sections. Arch. Hydro-Eng. Environ. Mech. 2017, 64, 49–67. [Google Scholar] [CrossRef]
- Merleau, J.; Perreault, L.; Angers, J.-F.; Favre, A.-C. Bayesian Modeling of Hydrographs. Water Resour. Res. 2007, 43. [Google Scholar] [CrossRef]
- Yue, S.; Ouarda, T.B.M.J.; Bobée, B.; Legendre, P.; Bruneau, P. Approach for Describing Statistical Properties of Flood Hydrograph. J. Hydrol. Eng. 2002, 7, 147–153. [Google Scholar] [CrossRef]
- Gądek, W.; Środula, A. The Evaluation of the Design Flood Hydrographs Determined with the Hydroproject Method in the Gauged Catchments. Infrastrukt. Ekol. Teren. Wiej./Infrastruct. Ecol. Rural. Areas 2014, IV/3, 1355–1366. [Google Scholar] [CrossRef]
- Gądek, W.; Tokarczyk, T. Determining Hypothetical Floods in the Odra Basin by Means of the Cracow Method and the Volume Formula. Infrastrukt. Ekol. Teren. Wiej. 2015, 1507–1519. [Google Scholar] [CrossRef]
- O’Connor, K.; Goswami, M.; Faulkner, D. Flood Studies Update Technical Research Report-Volume III-Hydrograph Analysis; NUI Galway and JBA Consulting, Office of Public Work: Dublin, Ireland, 2014.
- Qamar, M.U. Parametric and Non-Parametric Approaches for Runoff and Rainfall Regionalization. Ph.D. Thesis, Polytechnic University of Turin, Turin, Italy, 2015. [Google Scholar] [CrossRef]
- Brunner, M.I.; Viviroli, D.; Sikorska, A.E.; Vannier, O.; Favre, A.; Seibert, J. Flood Type Specific Construction of Synthetic Design Hydrographs. Water Resour. Res. 2017, 53, 1390–1406. [Google Scholar] [CrossRef]
- Debele, S.E.; Strupczewski, W.G.; Bogdanowicz, E. A Comparison of Three Approaches to Non-Stationary Flood Frequency Analysis. Acta Geophys. 2017, 65, 863–883. [Google Scholar] [CrossRef]
- Aranda, J.; García-Bartual, R. Synthetic Hydrographs Generation Downstream of a River Junction Using a Copula Approach for Hydrological Risk Assessment in Large Dams. Water 2018, 10, 1570. [Google Scholar] [CrossRef]
- McEnroe, B.M. Preliminary Sizing of Detention Reservoirs to Reduce Peak Discharges. J. Hydraul. Eng. 1992, 118, 1540–1549. [Google Scholar] [CrossRef]
- Baptista, M.; Michel, C. Influence des caracteristiques hydrauliques des bies sur la propagation des pointes de crue. La Houille Blanche 1990, 2, 141–148. [Google Scholar] [CrossRef]
- Baptista, M. Contribution à L’étude de la Propagation de Crues en Hydrologie; Ecole Nationale des Ponts et Chaussées, CEMAGREF Antony Cedex. 1990. Available online: https://pastel.archives-ouvertes.fr/pastel-00568722/document (accessed on 1 June 2022).
- Strupczewski, W.G. Equation of Flood Crest. Wiadomości Służby Hydrologicznej i Meteorologicznej 1964, 2, 35–58. [Google Scholar]
- Strupczewski, W.G.; Bogdanowicz, E.; Kochanek, K. Discussion of “Synthetic Design Hydrographs Based on Distribution Functions with Finite Support” by Francesco Serinaldi and Salvatore Grimaldi. J. Hydrol. Eng. 2013, 18, 121–126. [Google Scholar] [CrossRef]
- Reitz, W.; Kreps, H. Näherungsverfahren zur Berechnung des Erforderlichen Struraumes für Zwecke des Hochwasserschutzes; Deutsche Wasserwirtschaft: Hennef, Germany, 1945; Volume 1. [Google Scholar]
- Scarborough, J.B. Numerical Mathematical Analysis; Johns Hopkins Press: Baltimore, MD, USA, 1958. [Google Scholar]
- Reed, D.W.; Marshall, D.C. Defining a Design Hydrograph. In Flood Estimation Handbook; Institute of Hydrology, Centre for Ecology and Hydrology: Wallingford, UK, 1999; ISBN 978-1-906698-01-0. [Google Scholar]
- Nash, J.E. The Form of the Instantaneous Unit Hydrograph. In Proceedings of the International Association of Hydrological Sciences General Assembly, Toronto, ON, Canada; 1957; Volume 3, pp. 114–121. Available online: https://nora.nerc.ac.uk/id/eprint/508550/ (accessed on 1 June 2022).
- Reddyvaraprasad, C.; Patnaik, S.; Biswal, B. Recession Flow Prediction in Gauged and Ungauged Basins by Just Considering Past Discharge Information. Hydrol. Sci. J. 2020, 65, 21–32. [Google Scholar] [CrossRef]
- Cieplowski, A. Statistical Methods of Determining Typical Winter and Summer Hydrographs for Ungauged Watersheds; Louisiana State University: Baton Rouge, LA, USA, 1987; pp. 117–124. [Google Scholar]
- Cieplowski, A. Relationships between Selected Elements of the Flood Hydrographs in Rivers. J. Water Land Dev. 2001, 5, 89–105. [Google Scholar]
- Gądek, W.; Baziak, B.; Tokarczyk, T.; Bodziony, M. Flow Descriptors for Parametric Hydrographs Accounting for Afforestation of the Catchment. Meteorol. Hydrol. Water Manag. 2019, 36–45. [Google Scholar] [CrossRef]
- Bayliss, A.C. Catchment Descriptors Volume in Flood Estimation Handbook/Institute of Hydrology; Centre for Ecology and Hydrology: Wallingford, UK, 1999; ISBN 978-1-906698-01-0. [Google Scholar]
- Mills, P.; Nicholson, O.; Reed, D.W. Flood Studies Update Technical Research Report-Volume IV-Physical Catchment Descriptors; NUI Galway and JBA Consulting, Office of Public Work, 2014. Available online: https://data.gov.ie/dataset/flood-studies-update-fsu-physical-catchment-descriptors-ungauged (accessed on 1 June 2022).
- Deb, K. Optimization for Engineering Design: Algorithms and Examples; PHI Learning Private Limited: New Delhi, India, 2016; ISBN 978-81-203-4678-9. [Google Scholar]
- Parkinson, A.R.; Balling, R.; Hedengren, J.D. Optimization Methods for Engineering Design; Brigham Young University: Provo, UT, USA, 2013; Volume 5. [Google Scholar]
- Elshorbagy, A.; Simonovic, S.P.; Panu, U.S. Performance Evaluation of Artificial Neural Networks for Runoff Prediction. J. Hydrol. Eng. 2000, 5, 424–427. [Google Scholar] [CrossRef]
- Chai, T.; Draxler, R.R. Root Mean Square Error (RMSE) or Mean Absolute Error (MAE)?—Arguments against Avoiding RMSE in the Literature. Geosci. Model Dev. 2014, 7, 1247–1250. [Google Scholar] [CrossRef]
- Gądek, W.; Tokarczyk, T.; Środula, A. Estimation of Parametric Flood Hydrograph Determined by Means of Strupczewski Method in the Vistula and Odra Catchments. J. Water Land Dev. 2016, 31, 43–51. [Google Scholar] [CrossRef]
- Baziak, B.; Gądek, W. The Pearson Type IV Distribution Function Employed to Describe the Parametric Flow Hydrograph. Acta Geophys. 2019, 67, 1419–1433. [Google Scholar] [CrossRef]
- Ishiwatari, M.; Sasaki, D. Investing in Flood Protection in Asia: An Empirical Study Focusing on the Relationship between Investment and Damage. Prog. Disaster Sci. 2021, 12, 100197. [Google Scholar] [CrossRef]
- Hagen, J.S.; Cutler, A.; Trambauer, P.; Weerts, A.; Suarez, P.; Solomatine, D. Development and Evaluation of Flood Forecasting Models for Forecast-Based Financing Using a Novel Model Suitability Matrix. Prog. Disaster Sci. 2020, 6, 100076. [Google Scholar] [CrossRef]
- Uddin, K.; Matin, M.A. Potential Flood Hazard Zonation and Flood Shelter Suitability Mapping for Disaster Risk Mitigation in Bangladesh Using Geospatial Technology. Prog. Disaster Sci. 2021, 11, 100185. [Google Scholar] [CrossRef]
- Farhadi, H.; Esmaeily, A.; Najafzadeh, M. Flood Monitoring by Integration of Remote Sensing Technique and Multi-Criteria Decision Making Method. Comput. Geosci. 2022, 160, 105045. [Google Scholar] [CrossRef]
- Mioduszewski, W. Small Water Reservoirs–their function and construction I. J. Water Land Dev. 2012, 17, 45–52. [Google Scholar] [CrossRef]
- Mioduszewski, W. Small (Natural) Water Retention in Rural Areas/Mała (Naturalna) Retencja Wodna Na Obszarach Wiejskich. J. Water Land Dev. 2014, 20, 19–29. [Google Scholar] [CrossRef]
- WMO; GWP. Urban Flood Risk Management a Tool for Integrated Flood Management; Flood Management Tools Series; World Meteorological Organization: Geneva, Switzerland, 2008. [Google Scholar]
- Ernst, J.; Dewals, B.J.; Detrembleur, S.; Archambeau, P.; Erpicum, S.; Pirotton, M. Micro-Scale Flood Risk Analysis Based on Detailed 2D Hydraulic Modelling and High Resolution Geographic Data. Nat. Hazards 2010, 55, 181–209. [Google Scholar] [CrossRef]
- Zevenbergen, C.; Cashman, A.; Evelpidou, N.; Pasche, E.; Garvin, S.; Ashley, R. Urban Flood Management; CRC Press: Boca Raton, FL, USA, 2010; ISBN 978-0-429-10971-3. [Google Scholar]
- Berghuijs, W.R.; Aalbers, E.E.; Larsen, J.R.; Trancoso, R.; Woods, R.A. Recent Changes in Extreme Floods across Multiple Continents. Environ. Res. Lett. 2017, 12, 114035. [Google Scholar] [CrossRef]
- Hall, J.; Blöschl, G. Spatial Patterns and Characteristics of Flood Seasonality in Europe. Hydrol. Earth Syst. Sci. 2018, 22, 3883–3901. [Google Scholar] [CrossRef]
- Kundzewicz, Z.W.; Krysanova, V.; Dankers, R.; Hirabayashi, Y.; Kanae, S.; Hattermann, F.F.; Huang, S.; Milly, P.C.D.; Stoffel, M.; Driessen, P.P.J.; et al. Differences in Flood Hazard Projections in Europe–Their Causes and Consequences for Decision Making. Hydrol. Sci. J. 2017, 62, 1–14. [Google Scholar] [CrossRef]
- Blöschl, G.; Hall, J.; Viglione, A.; Perdigão, R.A.P.; Parajka, J.; Merz, B.; Lun, D.; Arheimer, B.; Aronica, G.T.; Bilibashi, A.; et al. Changing Climate Both Increases and Decreases European River Floods. Nature 2019, 573, 108–111. [Google Scholar] [CrossRef] [PubMed]
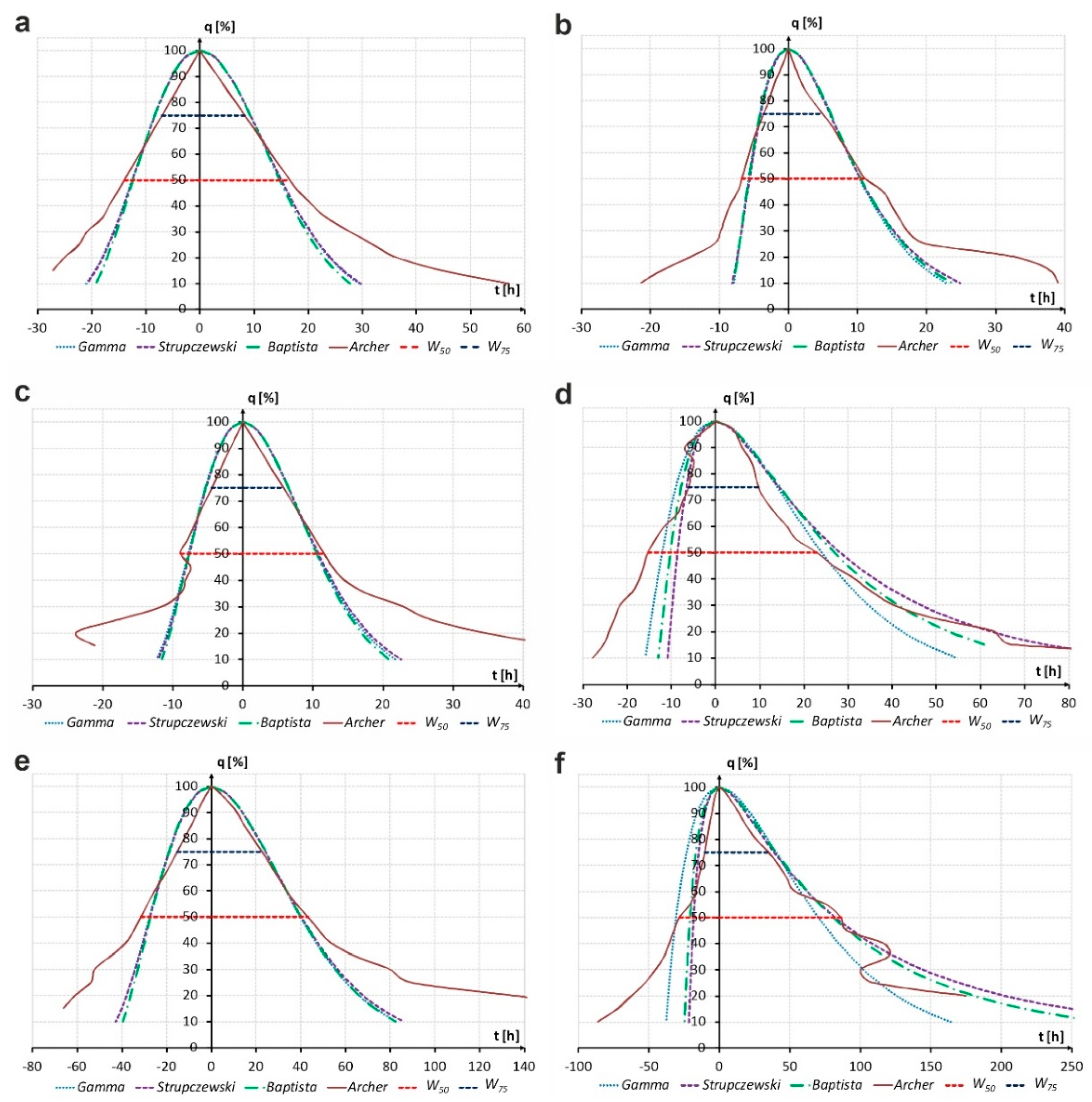
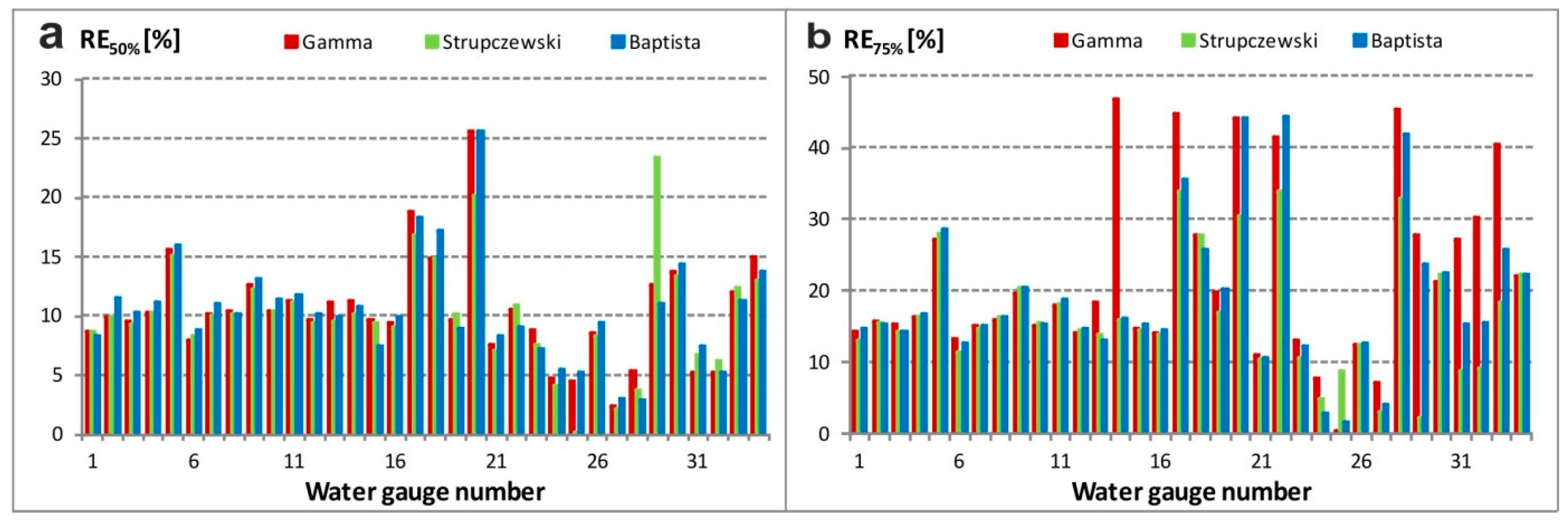
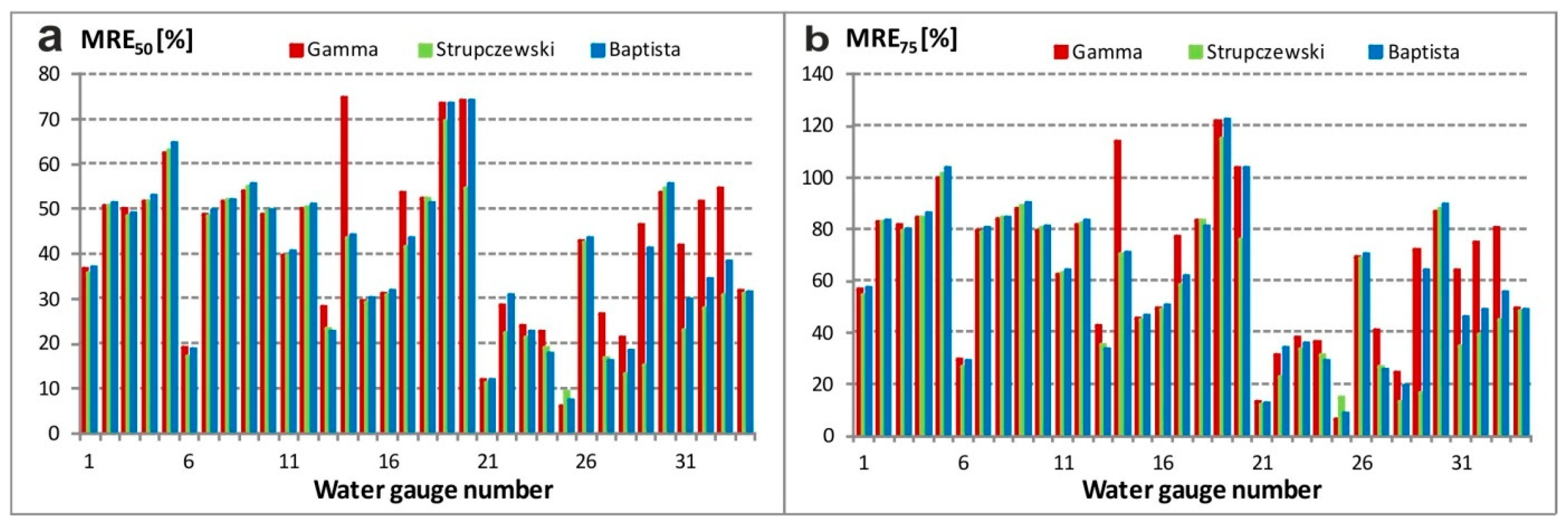
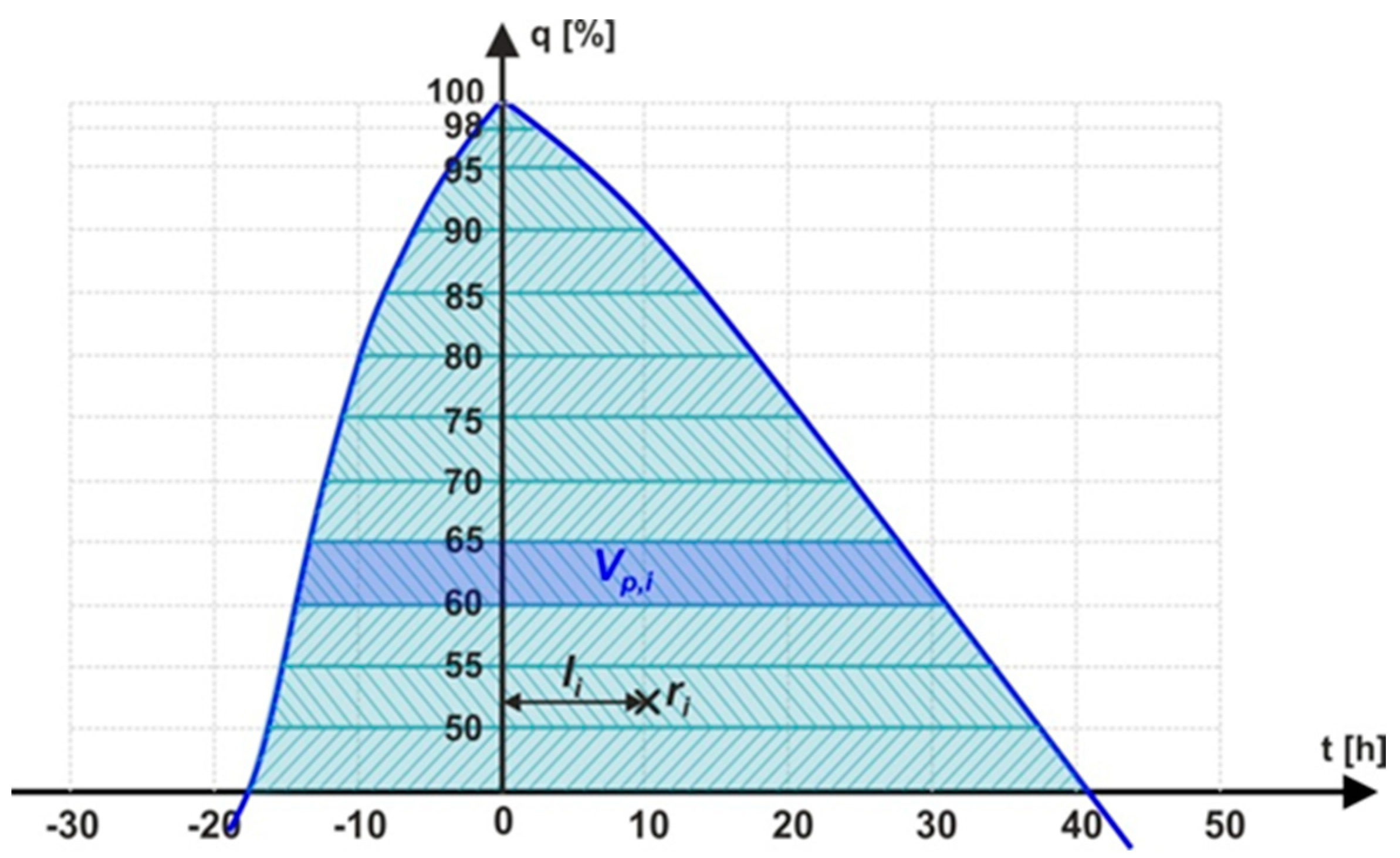
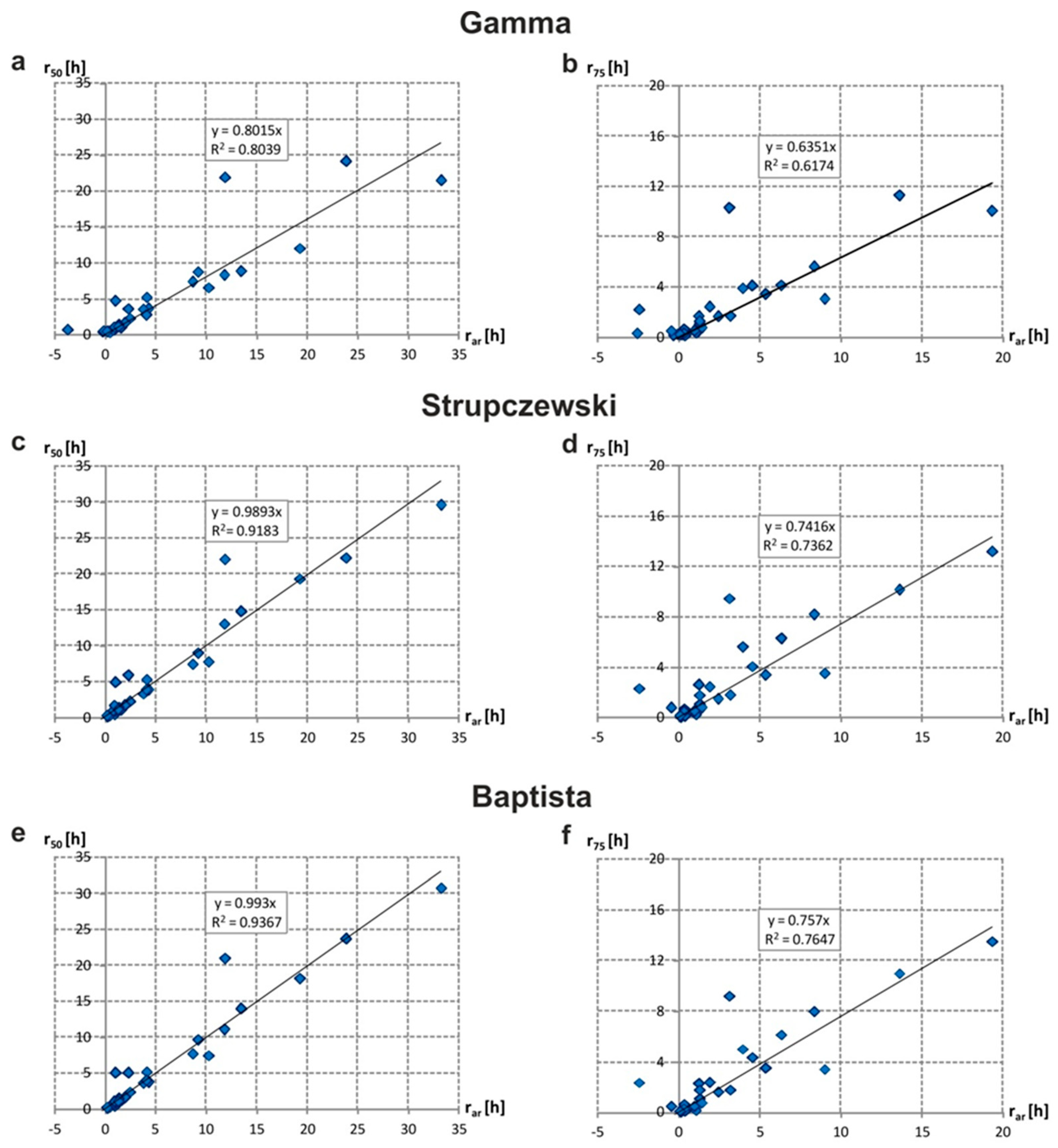
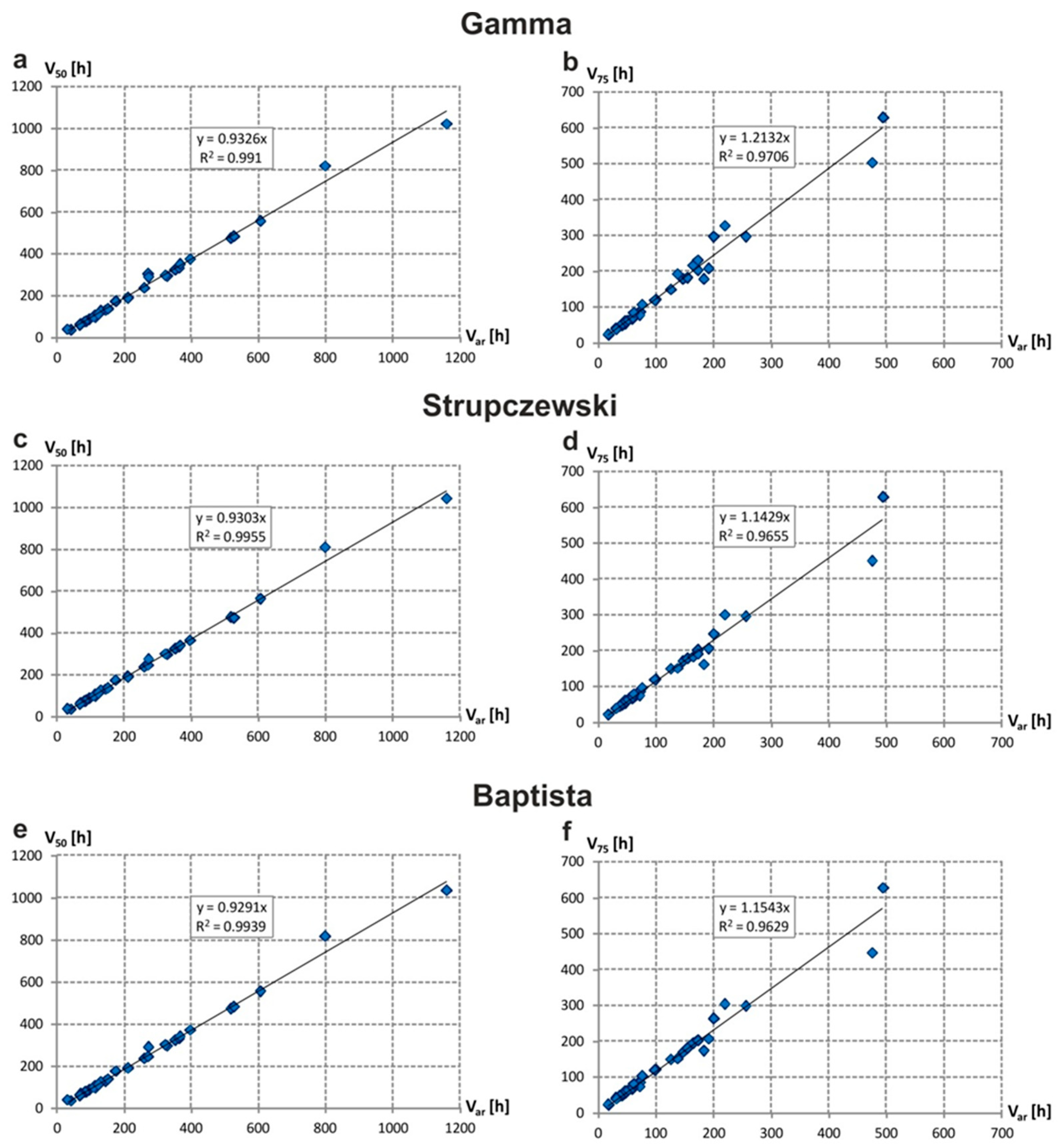
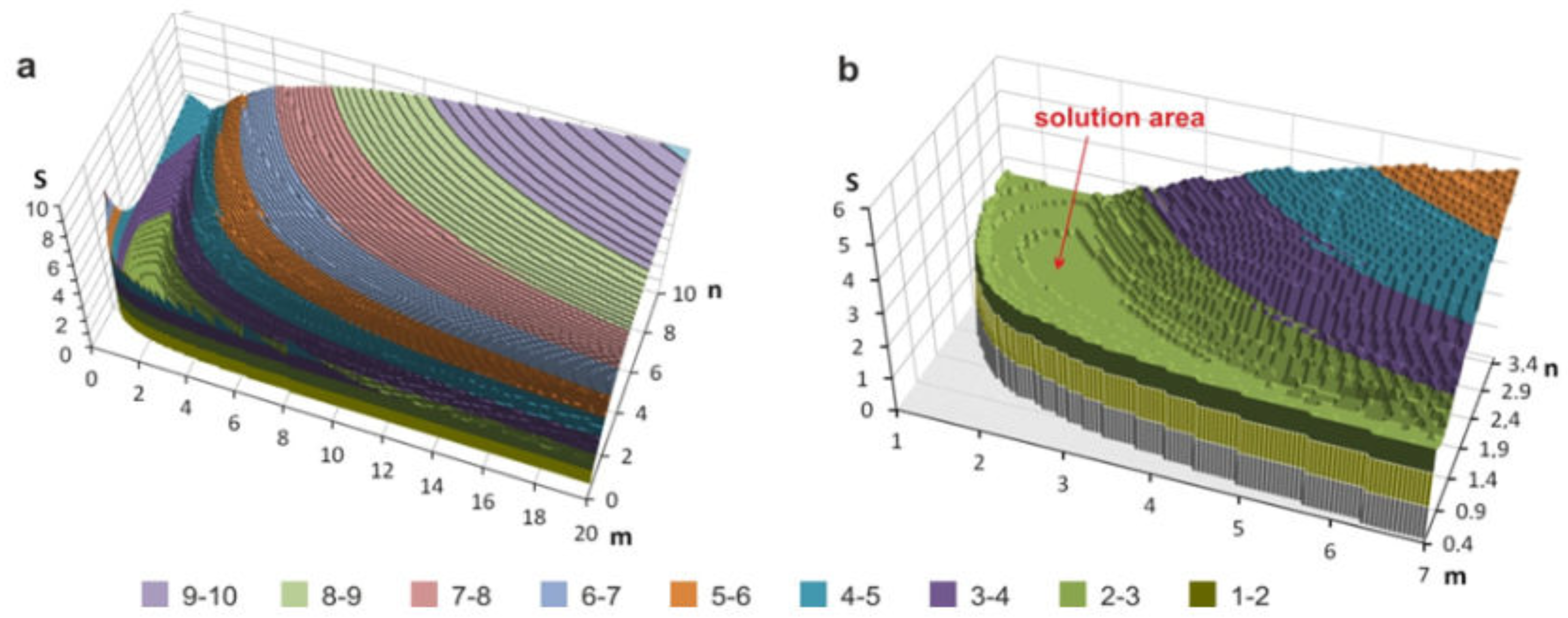
| No. | River | Gauging Station | Catchment Area (km2) | W50 (h) | W75 (h) | s (-) |
|---|---|---|---|---|---|---|
| Upper Vistula River water region | ||||||
| 1 | Żabniczanka | Żabnica | 22.8 | 17.5 | 8.9 | 0.40 |
| 2 | Lubieńka | Lubień | 46.9 | 30.5 | 15.3 | 0.46 |
| 3 | Żylica | Łodygowice | 47.8 | 32.2 | 16.1 | 0.39 |
| 4 | Bystra | Kamesznica | 48.2 | 44.8 | 22.2 | 0.47 |
| 5 | Wisłok | Puławy | 131 | 24.3 | 10.4 | 0.47 |
| 6 | Wisła | Skoczów | 296 | 24.6 | 12.8 | 0.39 |
| 7 | Raba | Kasinka Mała | 353 | 20.5 | 10.2 | 0.43 |
| 8 | Skawa | Sucha Beskidzka | 468 | 26.5 | 13.2 | 0.49 |
| 9 | Raba | Stróża | 644 | 23.8 | 11.2 | 0.50 |
| 10 | Biała | Koszyce Wielkie | 957 | 18.6 | 9.3 | 0.47 |
| 11 | Raba | Proszówki | 1 470 | 44.6 | 21.6 | 0.51 |
| 12 | Poprad | Stary Sącz | 2 071 | 54.8 | 27.9 | 0.51 |
| 13 | Nida | Brzegi | 3 359 | 69.2 | 32.3 | 0.30 |
| 14 | Dunajec | Żabno | 6 735 | 57.2 | 27.9 | 0.58 |
| 15 | San | Radomyśl | 16 824 | 109.4 | 55.2 | 0.43 |
| 16 | Wisła | Sandomierz | 31 847 | 74.6 | 37.9 | 0.42 |
| 17 | Wisła | Zawichost | 50 732 | 143.8 | 48.4 | 0.29 |
| Middle Odra River water region | ||||||
| 18 | Nysa Kłodzka | Międzylesie | 49.7 | 9.1 | 3.9 | 0.45 |
| 19 | Kaczawa | Świerzawa | 133 | 17.9 | 8.6 | 0.38 |
| 20 | Bystrzyca Dusznicka | Szalejów Dolny | 175 | 10.1 | 3.7 | 0.37 |
| 21 | Czarna Woda | Gniechowice | 251 | 75.3 | 39.9 | 0.39 |
| 22 | Biała Głuchołaska | Głuchołazy | 283 | 15.9 | 6.4 | 0.29 |
| 23 | Piława | Mościsko | 292 | 68.1 | 34.5 | 0.33 |
| 24 | Kaczawa | Rzymówka | 314 | 28.0 | 15.5 | 0.31 |
| 25 | Strzegomka | Łażany | 362 | 57.8 | 38.0 | 0.28 |
| 26 | Nysa Szalona | Jawor | 304 | 25.9 | 13.5 | 0.43 |
| 27 | Czarna Woda | Bukowna | 431 | 167.1 | 100.2 | 0.20 |
| 28 | Bóbr | Wojanów | 535 | 38.2 | 15.7 | 0.19 |
| 29 | Kaczawa | Dunino | 774 | 32.8 | 14.2 | 0.32 |
| 30 | Nysa Kłodzka | Kłodzko | 1 084 | 15.1 | 6.85 | 0.47 |
| 31 | Bystrzyca | Jarnołtów | 1 721 | 77.5 | 36.3 | 0.24 |
| 32 | Kaczawa | Piątnica | 1 807 | 82.7 | 37.9 | 0.22 |
| 33 | Bóbr | Żagań | 4 255 | 114.8 | 45.2 | 0.25 |
| 34 | Odra | Cigacice | 39 900 | 250.4 | 109.8 | 0.37 |
| Water Gauge No. | Gamma | Strupczewski | Baptista | ||||||
|---|---|---|---|---|---|---|---|---|---|
| W75 | W50 | s | W75 | W50 | s | W75 | W50 | s | |
| 1 | 10.2 | 16.0 | 0.39 | 10.1 | 16.0 | 0.39 | 10.2 | 16.0 | 0.38 |
| 2 | 17.7 | 27.5 | 0.45 | 17.7 | 27.5 | 0.45 | 17.6 | 27.0 | 0.45 |
| 3 | 18.5 | 29.1 | 0.37 | 18.4 | 29.2 | 0.37 | 18.4 | 28.9 | 0.36 |
| 4 | 25.8 | 40.1 | 0.47 | 25.8 | 40.1 | 0.46 | 25.9 | 39.8 | 0.47 |
| 5 | 13.2 | 20.5 | 0.44 | 13.3 | 20.6 | 0.46 | 13.3 | 20.4 | 0.47 |
| 6 | 14.5 | 22.7 | 0.38 | 14.2 | 22.6 | 0.37 | 14.4 | 22.5 | 0.38 |
| 7 | 11.8 | 18.4 | 0.42 | 11.8 | 18.4 | 0.41 | 11.8 | 18.2 | 0.42 |
| 8 | 15.3 | 23.7 | 0.47 | 15.3 | 23.8 | 0.49 | 15.3 | 23.8 | 0.49 |
| 9 | 13.4 | 20.8 | 0.46 | 13.5 | 20.9 | 0.50 | 13.5 | 20.7 | 0.51 |
| 10 | 10.7 | 16.7 | 0.45 | 10.8 | 16.7 | 0.47 | 10.7 | 16.5 | 0.46 |
| 11 | 25.5 | 39.5 | 0.48 | 25.5 | 39.6 | 0.51 | 25.6 | 39.3 | 0.51 |
| 12 | 31.9 | 49.5 | 0.48 | 32.0 | 49.6 | 0.51 | 32.0 | 49.2 | 0.52 |
| 13 | 38.2 | 61.5 | 0.27 | 36.8 | 62.6 | 0.26 | 36.5 | 62.4 | 0.24 |
| 14 | 41.0 | 63.7 | 0.48 | 32.4 | 51.4 | 0.60 | 32.4 | 51.0 | 0.60 |
| 15 | 63.3 | 98.7 | 0.42 | 63.2 | 99.0 | 0.42 | 63.7 | 98.3 | 0.41 |
| 16 | 43.3 | 67.5 | 0.41 | 43.2 | 67.8 | 0.41 | 43.4 | 67.2 | 0.41 |
| 17 | 70.1 | 116.8 | 0.20 | 64.8 | 119.5 | 0.20 | 65.6 | 117.4 | 0.21 |
| 18 | 4.9 | 7.8 | 0.44 | 4.9 | 7.8 | 0.44 | 4.9 | 7.6 | 0.43 |
| 19 | 10.2 | 16.1 | 0.36 | 10.0 | 16.1 | 0.35 | 10.3 | 16.3 | 0.35 |
| 20 | 5.4 | 8.5 | 0.36 | 4.9 | 8.1 | 0.30 | 5.4 | 8.5 | 0.36 |
| 21 | 44.4 | 69.5 | 0.38 | 44.1 | 69.9 | 0.37 | 44.2 | 69.0 | 0.38 |
| 22 | 9.0 | 14.2 | 0.37 | 8.6 | 14.2 | 0.30 | 9.2 | 14.4 | 0.38 |
| 23 | 39.0 | 62.1 | 0.30 | 38.2 | 62.9 | 0.30 | 38.8 | 63.2 | 0.30 |
| 24 | 16.7 | 26.7 | 0.28 | 16.2 | 26.9 | 0.30 | 15.9 | 26.5 | 0.27 |
| 25 | 38.1 | 60.4 | 0.32 | 34.7 | 57.9 | 0.28 | 37.3 | 60.8 | 0.30 |
| 26 | 15.1 | 23.6 | 0.42 | 15.1 | 23.7 | 0.42 | 15.2 | 23.4 | 0.42 |
| 27 | 107.3 | 171.2 | 0.29 | 97.0 | 170.6 | 0.22 | 96.2 | 172.2 | 0.21 |
| 28 | 22.9 | 36.2 | 0.33 | 20.9 | 36.8 | 0.23 | 22.3 | 37.1 | 0.27 |
| 29 | 18.1 | 28.6 | 0.34 | 17.1 | 28.7 | 0.28 | 17.5 | 29.2 | 0.27 |
| 30 | 8.3 | 13.0 | 0.44 | 8.4 | 13.0 | 0.46 | 8.4 | 12.9 | 0.47 |
| 31 | 46.2 | 73.4 | 0.31 | 39.5 | 72.2 | 0.20 | 41.9 | 71.7 | 0.24 |
| 32 | 49.3 | 78.3 | 0.31 | 41.4 | 77.6 | 0.18 | 43.7 | 78.3 | 0.21 |
| 33 | 63.5 | 101.0 | 0.30 | 53.5 | 100.5 | 0.18 | 56.8 | 101.8 | 0.21 |
| 34 | 134.1 | 212.8 | 0.31 | 134.3 | 217.7 | 0.33 | 134.2 | 215.7 | 0.32 |
| Gamma | Strupczewski | Baptista | |||||||
|---|---|---|---|---|---|---|---|---|---|
| Type of Statistic | W75 | W50 | s | W75 | W50 | s | W75 | W50 | s |
| Upper Vistula River water region | |||||||||
| r | 1.00 | 0.97 | 0.98 | 1.00 | 0.99 | 0.99 | 1.00 | 0.98 | 0.98 |
| RMSE | 8.8 | 7.0 | 0.1 | 7.5 | 5.3 | 0.0 | 7.6 | 6.7 | 0.0 |
| MAE | 6.6 | 4.0 | 0.1 | 5.4 | 4.0 | 0.0 | 5.7 | 4.4 | 0.0 |
| Middle Odra River water region | |||||||||
| r | 1.00 | 0.99 | 0.81 | 1.00 | 0.99 | 0.88 | 0.99 | 0.99 | 0.98 |
| RMSE | 12.1 | 8.1 | 0.1 | 9.2 | 6.8 | 0.0 | 9.6 | 7.6 | 0.0 |
| MAE | 7.1 | 5.4 | 0.1 | 5.2 | 4.2 | 0.0 | 5.7 | 4.9 | 0.0 |
| The whole area | |||||||||
| r | 1.00 | 0.99 | 0.82 | 1.00 | 0.99 | 0.95 | 0.99 | 0.98 | 0.98 |
| RMSE | 10.6 | 7.5 | 0.1 | 8.4 | 6.1 | 0.0 | 8.7 | 7.2 | 0.0 |
| MAE | 6.8 | 4.7 | 0.1 | 5.3 | 4.1 | 0.0 | 5.7 | 4.7 | 0.0 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gądek, W.; Baziak, B.; Tokarczyk, T.; Szalińska, W. A Novel Method of Design Flood Hydrographs Estimation for Flood Hazard Mapping. Water 2022, 14, 1856. https://doi.org/10.3390/w14121856
Gądek W, Baziak B, Tokarczyk T, Szalińska W. A Novel Method of Design Flood Hydrographs Estimation for Flood Hazard Mapping. Water. 2022; 14(12):1856. https://doi.org/10.3390/w14121856
Chicago/Turabian StyleGądek, Wiesław, Beata Baziak, Tamara Tokarczyk, and Wiwiana Szalińska. 2022. "A Novel Method of Design Flood Hydrographs Estimation for Flood Hazard Mapping" Water 14, no. 12: 1856. https://doi.org/10.3390/w14121856
APA StyleGądek, W., Baziak, B., Tokarczyk, T., & Szalińska, W. (2022). A Novel Method of Design Flood Hydrographs Estimation for Flood Hazard Mapping. Water, 14(12), 1856. https://doi.org/10.3390/w14121856








