Investigating the Result of Current Density, Temperature, and Electrolyte Concentration on COD: Subtraction of Petroleum Refinery Wastewater Using Response Surface Methodology
Abstract
1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Experimental Setup
2.3. Analytical Method
2.4. Experimental Design
3. Results and Discussion
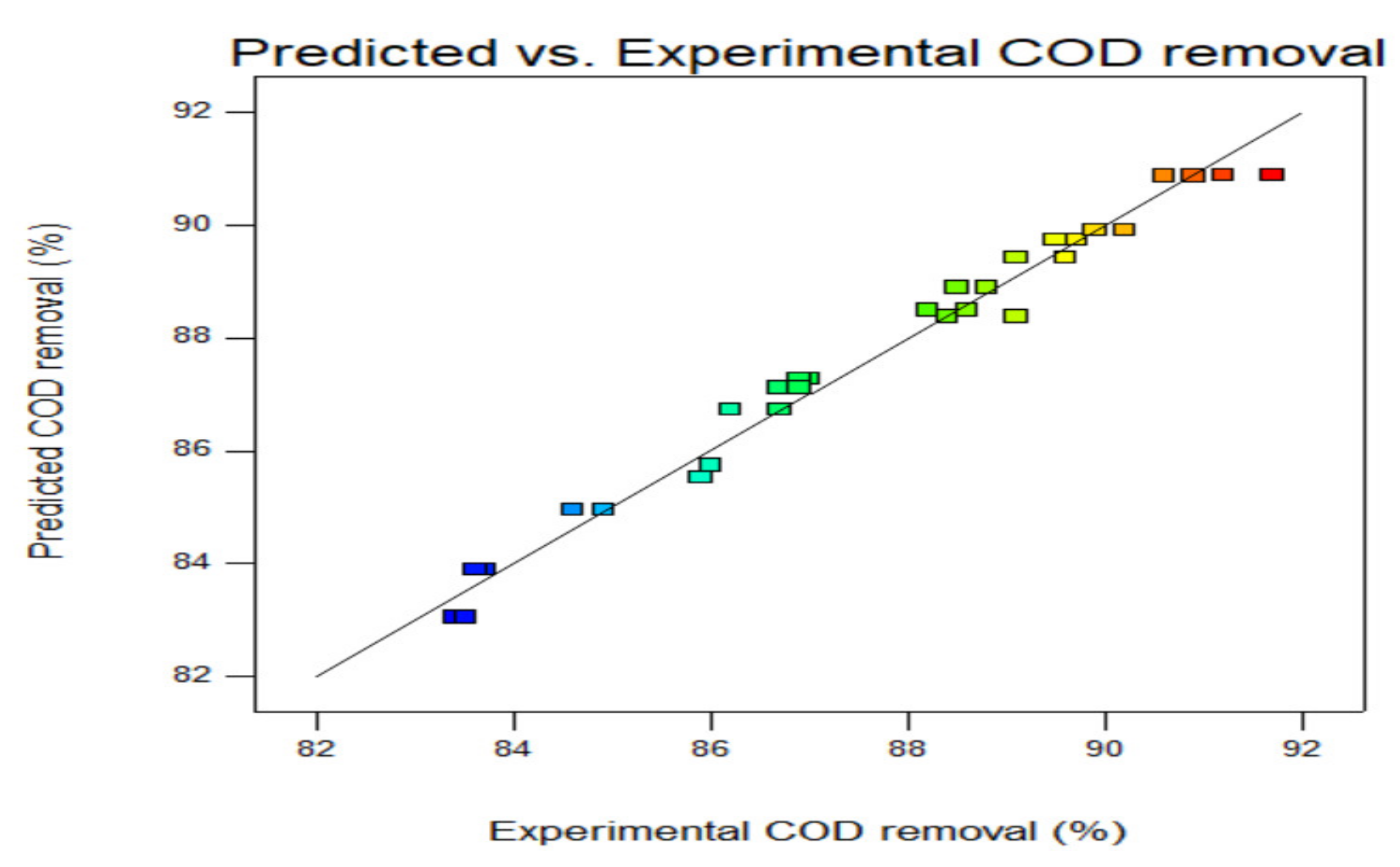
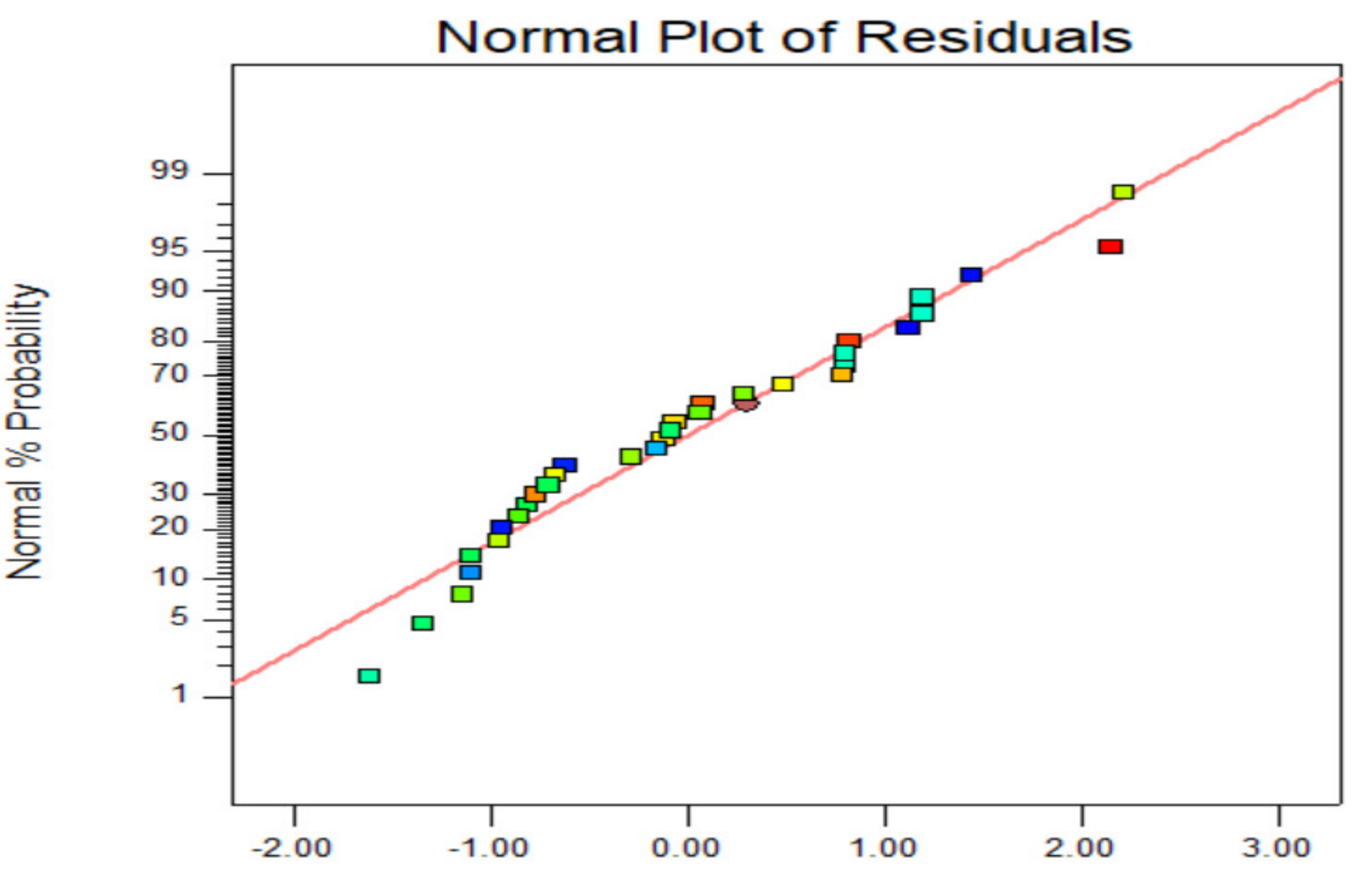
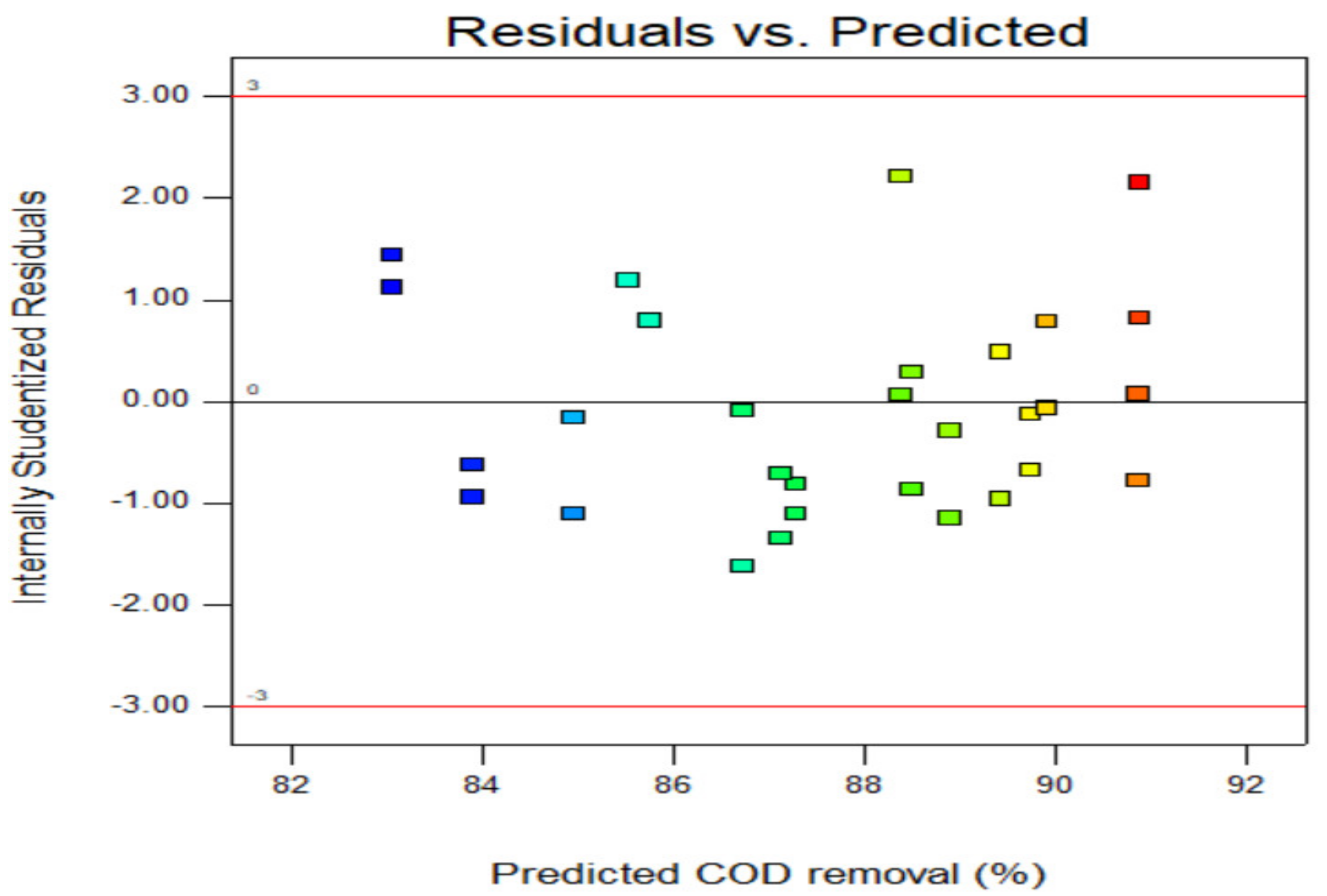
3.1. Model Fitting
3.2. Validation of Model
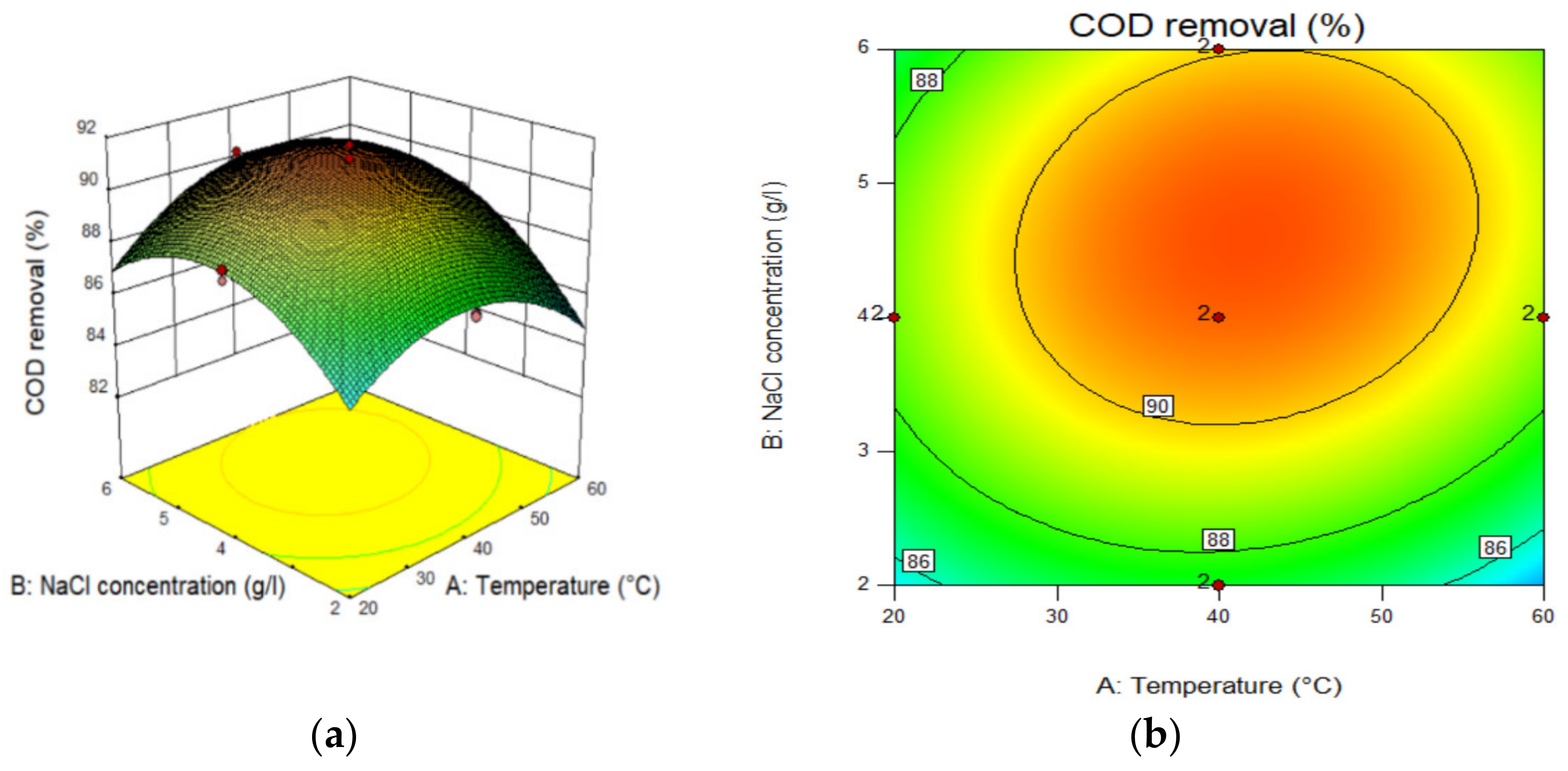
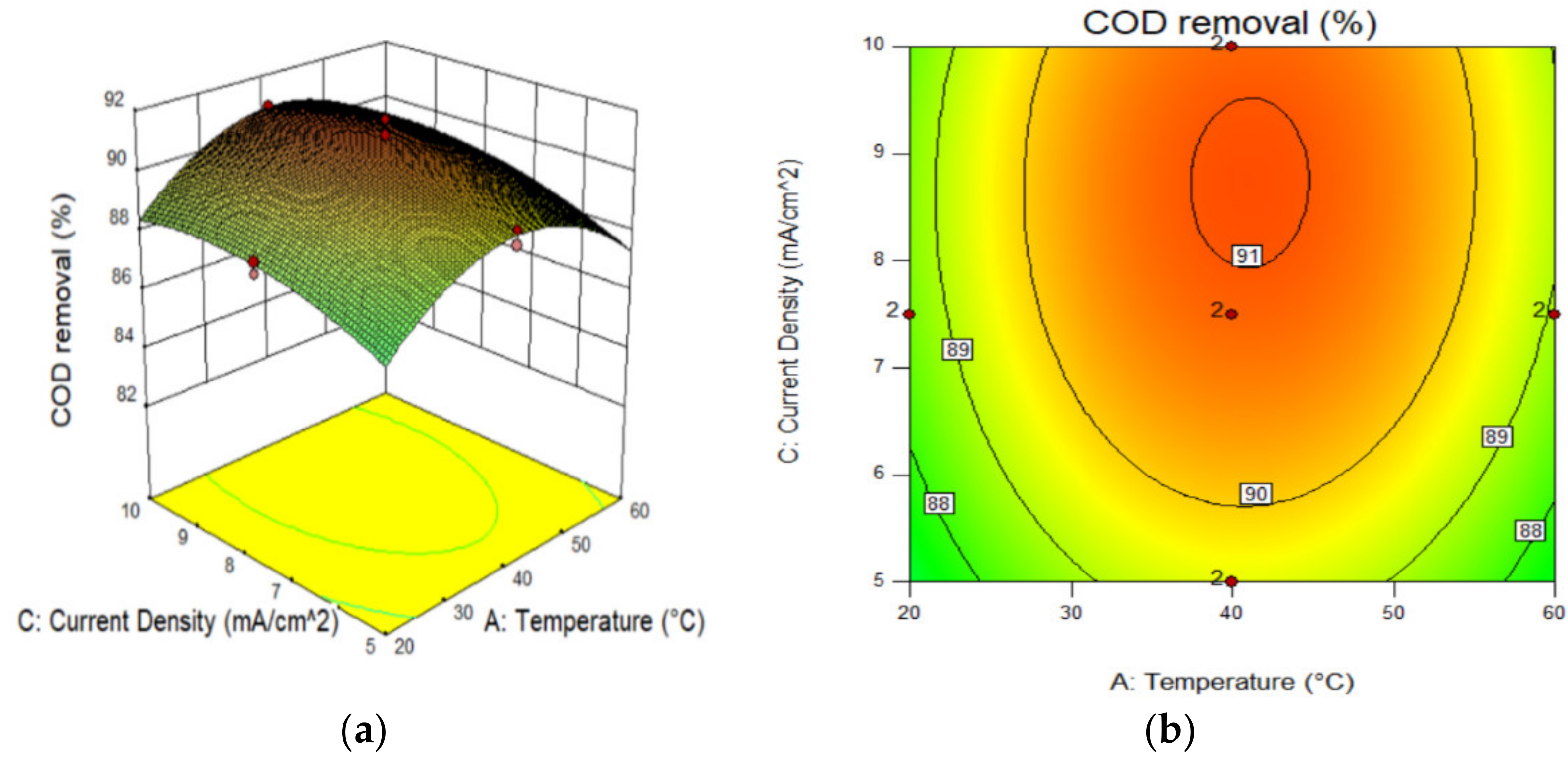
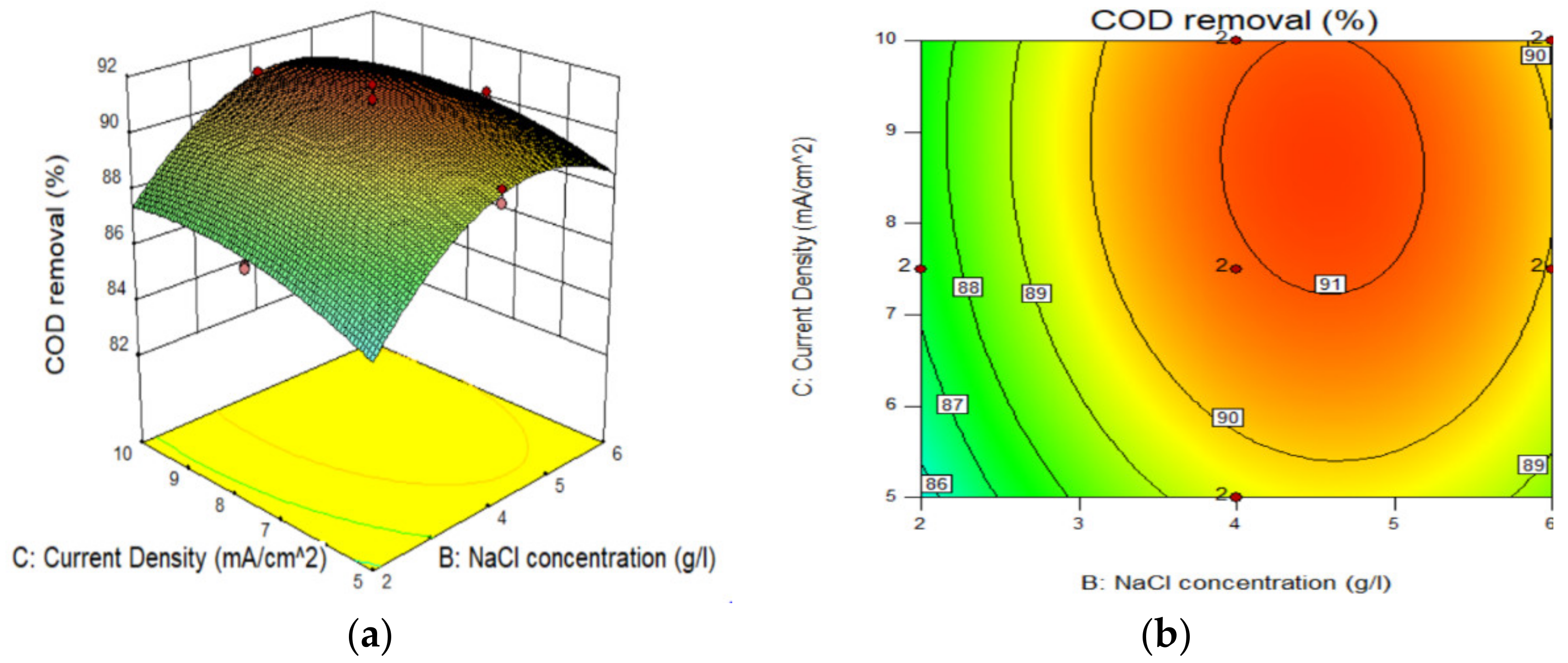
3.3. Analysis of Response
3.4. Economic Evaluation
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
References
- Coelho, A.; Castro, A.V.; Dezotti, M.; Sant’Anna, G.L.J. Treatment of petroleum refinery sourwater by advanced oxidation processes. J. Hazard. Mater. 2006, 137, 178–184. [Google Scholar] [CrossRef] [PubMed]
- Diya’uddeen, H.B.; Daud, W.M.A.W.; Aziz, A.R.A. Treatment technologies for petroleum refinery effluents: A review. Process Saf. Environ. Prot. 2010, 89, 95–105. [Google Scholar] [CrossRef]
- Ratshomo, K.; Nembahe, R. 2018 South African Energy Sector Report; Department of Energy: Pretoria, South Africa, 2018; ISBN 9781920435141.
- Bwapwa, J.K. Review on Main Issues Causing Deterioration of Water Quality and Water Scarcity: Case Study of South Africa. Environ. Manag. Sustain. Dev. 2018, 7, 14. [Google Scholar] [CrossRef]
- Gasim, H.A.; Kutty, S.R.M.; Isa, M.H.; Isa, M.P.M. Treatment of Petroleum Refinery Wastewater by using UASB Reactors. Int. J. Chem. Biol. Eng. 2012, 6, 174–177. [Google Scholar]
- Guo, S.; Li, G.; Qu, J.; Liu, X. Improvement of acidification on dewaterability of oily sludge from flotation. Chem. Eng. J. 2011, 168, 746–751. [Google Scholar] [CrossRef]
- Myburgh, D.P.; Aziz, M.; Roman, F.; Jardim, J.; Chakawa, S. Removal of COD from Industrial Biodiesel Wastewater Using an Integrated Process: Electrochemical-Oxidation with IrO2–Ta2O5/Ti Anodes and Chitosan Powder as an Adsorbent. Environ. Process. 2019, 6, 819–840. [Google Scholar] [CrossRef]
- Gao, J.; Yan, J.; Liu, Y.; Zhang, J.; Guo, Z. A novel electro-catalytic degradation method of phenol wastewater with Ti/IrO2-Ta2O5 anodes in high-gravity fields. Water Sci. Technol. 2017, 76, 662–670. [Google Scholar] [CrossRef] [PubMed]
- Park, H.; Bak, A.; Ahn, Y.Y.; Choi, J.; Hoffmannn, M.R. Photoelectrochemical performance of multi-layered BiO x-TiO 2/Ti electrodes for degradation of phenol and production of molecular hydrogen in water. J. Hazard. Mater. 2012, 211, 47–54. [Google Scholar] [CrossRef]
- Shestakova, M.; Bonete, P.; Gómez, R.; Sillanpää, M.; Tang, W.Z. Novel Ti/Ta2O5-SnO2 electrodes for water electrolysis and electro-catalytic oxidation of organics. Electrochim. Acta 2014, 120, 302–307. [Google Scholar] [CrossRef]
- Mukimin, A.; Vistanty, H.; Zen, N. Oxidation of textile wastewater using cylinder Ti/β-PbO2 electrode in electro-catalytic tube reactor. Chem. Eng. J. 2015, 259, 430–437. [Google Scholar] [CrossRef]
- Ani, I.J.; Akpan, U.G.; Olutoye, M.A.; Hameed, B.H. Photocatalytic degradation of pollutants in petroleum refinery wastewater by TiO2-and ZnO-based photocatalysts: Recent development. J. Clean. Prod. 2018, 205, 930–954. [Google Scholar] [CrossRef]
- Sillanpaa, M.; Shestakova, M. Electrochemical Water Treatment Methods-Fundamentals, Methods and Full Scale Application; Butterworth-Heinemann: Oxford, UK, 2017; ISBN 9780128114629. [Google Scholar]
- Feng, Y.; Yang, L.; Liu, J.; Logan, B.E. Electrochemical technologies for wastewater treatment and resource reclamation. Environ. Sci. Water Res. Technol. 2016, 2, 800–831. [Google Scholar] [CrossRef]
- Arslan, G.; Yazici, B.; Erbil, M. The effect of pH, temperature and concentration on electrooxidation of phenol. J. Hazard. Mater. 2003, 124, 37–43. [Google Scholar] [CrossRef]
- Yao, J.; Mei, Y.; Xia, G.; Lu, Y.; Xu, D.; Sun, N.; Wang, J.; Chen, J. Process optimisation of electrochemical oxidation of ammonia to nitrogen for actual dyeing wastewater treatment. Int. J. Environ. Res. Public Health 2019, 16, 2931. [Google Scholar] [CrossRef] [PubMed]
- Raju, G.B.; Karuppiah, M.T.; Latha, S.S.; Parvathy, S.; Prabhakar, S. Treatment of wastewater from synthetic textile industry by electrocoagulation-electrooxidation. Chem. Eng. J. 2008, 144, 51–58. [Google Scholar] [CrossRef]
- Delgarm, N.; Sajadi, B.; Azarbad, K.; Delgarm, S. Sensitivity analysis of building energy performance: A simulation-based approach using OFAT and variance-based sensitivity analysis methods. J. Build. Eng. 2018, 15, 181–193. [Google Scholar] [CrossRef]
- Ahmed, S.A.; Abdella, M.A.A.; El-Sherbiny, G.M.; Ibrahim, A.M.; El-Shamy, A.R.; Atalla, S.M.M. Application of one –factor- at-a-time and statistical designs to enhance α-amylase production by a newly isolate Bacillus subtilis strain-MK1. Biocatal. Agric. Biotechnol. 2019, 22, 101397. [Google Scholar] [CrossRef]
- Frey, D.D.; Sudarsanam, N. An adaptive one-factor-at-a-time method for robust parameter design: Comparison with crossed arrays via case studies. J. Mech. Des. Trans. Asme 2008, 130, 1–18. [Google Scholar] [CrossRef]
- Swati; Ghosh, P.; Thakur, I.S. Biodegradation of pyrene by Pseudomonas sp. ISTPY2 isolated from landfill soil: Process optimisation using Box-Behnken design model. Bioresour. Technol. Rep. 2019, 8, 100329. [Google Scholar] [CrossRef]
- Bas, D.; Boyaci, I.H. Modeling and optimisation I: Usability of response surface methodology. J. Food Eng. 2007, 78, 836–845. [Google Scholar] [CrossRef]
- Nor, N.M.; Mohamed, M.S.; Loh, T.C.; Foo, H.L.; Rahim, R.A.; Tan, J.S.; Mohamad, R. Comparative analyses on medium optimisation using one-factor-at-a-time, response surface methodology, and artificial neural network for lysine–methionine biosynthesis by Pediococcus pentosaceus RF-1. Biotechnol. Biotechnol. Equip. 2017, 31, 935–947. [Google Scholar] [CrossRef]
- Anderson, M.J.; Whitcomb, P.J. RSM Simplified: Optimising Processes Using Response Surface Methods for Design of Experiments, 2nd ed.; Productivity Press: New York, NY, USA, 2016. [Google Scholar]
- Fukuda, I.M.; Pinto, C.F.F.; Moreira, C.D.S.; Saviano, A.M.; Lourenço, F.R. Design of experiments (DoE) applied to pharmaceutical and analytical quality by design (QbD). Braz. J. Pharm. Sci. 2018, 54, 1–16. [Google Scholar] [CrossRef]
- Ahmadi, M.; Ghanbari, F. Optimizing COD removal from greywater by photoelectro-persulfate process using Box-Behnken design: Assessment of effluent quality and electrical energy consumption. Environ. Sci. Pollut. Res. 2016, 23, 19350–19361. [Google Scholar] [CrossRef]
- Ten Chan, Y.; Tan, M.C.; Chin, N.L. Application of Box-Behnken design in optimisation of ultrasound effect on apple pectin as sugar replacer. LWT Food Sci. Technol. 2019, 115, 108449. [Google Scholar] [CrossRef]
- Gulen, B.; Demircivi, P. Adsorption properties of flouroquinolone type antibiotic ciprofloxacin into 2:1 dioctahedral clay structure: Box-Behnken experimental design. J. Mol. Struct. 2020, 1206. [Google Scholar] [CrossRef]
- Scialdone, O.; Randazzo, S.; Galia, A.; Silvestri, G. Electrochemical oxidation of organics in water: Role of operative parameters in the absence and in the presence of NaCl. Water Res. 2009, 43, 2260–2272. [Google Scholar] [CrossRef] [PubMed]
- Sharma, S.; Aygun, A.; Simsek, H. Electrochemical treatment of sunflower oil refinery wastewater and optimisation of the parameters using response surface methodology. Chemosphere 2020, 249, 126511. [Google Scholar] [CrossRef]
- Samarghandi, M.R.; Nemattollahi, D.; Asgari, G.; Shokoohi, R.; Ansari, A.; Dargahi, A. Electrochemical process for 2,4-D herbicide removal from aqueous solutions using stainless steel 316 and graphite Anodes: Optimisation using response surface methodology. Sep. Sci. Technol. Phila. 2019, 54, 478–493. [Google Scholar] [CrossRef]
- Aziz, M.; Ojumu, T. Exclusion of estrogenic and androgenic steroid hormones from municipal membrane bioreactor wastewater using UF/NF/RO membranes for water reuse application. Membranes 2020, 10, 37. [Google Scholar] [CrossRef] [PubMed]
- Abbas, Z.N.; Abbar, A.H. Application of response surface methodology for optimisation of phenol removal from a simulated wastewater using rotating tubular packed bed electrochemical reactor. Egypt. J. Chem. 2020, 63, 3925–3936. [Google Scholar]
- Mukwevho, M.J.; Maharajh, D.; Chirwa, E.M.N. Evaluating the effect of ph, temperature, and hydraulic retention time on biological sulphate reduction using response surface methodology. Water 2020, 12, 2662. [Google Scholar] [CrossRef]
- Tian, S.; Hao, C.; Xu, G.; Yang, J.; Sun, R. Optimization conditions for extracting polysaccharide from Angelica sinensis and its antioxidant activities. J. Food Drug Anal. 2017, 25, 766–775. [Google Scholar] [CrossRef] [PubMed]
- Thangam, R.; Suresh, V.; Kannan, S. Optimised extraction of polysaccharides from Cymbopogon citratus and its biological activities. Int. J. Biol. Macromol. 2014, 65, 415–423. [Google Scholar] [CrossRef]
- Darvishmotevalli, M.; Zarei, A.; Moradnia, M.; Noorisepehr, M.; Mohammadi, H. Optimization of saline wastewater treatment using electrochemical oxidation process: Prediction by RSM method. MethodsX 2019, 6, 1101–1113. [Google Scholar] [CrossRef]
- Zhang, Y.J.; Li, Q.; Zhang, Y.X.; Wang, D.; Xing, J.M. Optimization of Succinic acid fermentation with Actinobacillus succinogenes by response surface methodology (RSM). J. Zhejiang Univ. Sci. B 2012, 13, 103–110. [Google Scholar] [CrossRef]
- Sales Solano, A.M.; Costa de Araújo, C.K.; Vieira de Melo, J.; Peralta-Hernandez, J.M.; Ribeiro da Silva, D.; Martínez-Huitle, C.A. Decontamination of real textile industrial effluent by strong oxidant species electrogenerated on diamond electrode: Viability and disadvantages of this electrochemical technology. Appl. Catal. B Environ. 2013, 130–131, 112–120. [Google Scholar] [CrossRef]
- Esfandyari, Y.; Mahdavi, Y.; Seyedsalehi, M.; Hoseini, M.; Safari, G.H.; Ghanbari, G.M.; Kamani, H.; Jaafari, J. Degradation and biodegradability improvement of the olive mill wastewater by peroxi-electrocoagulation/electrooxidation- electroflotation process with bipolar aluminum electrodes. Environ. Sci. Pollut. Res. 2015, 22, 6288–6297. [Google Scholar] [CrossRef]
- Sathian, S.; Rajasimman, M.; Radha, G.; Shanmugapriya, V.; Karthikeyan, C. Performance of SBR for the treatment of textile dye wastewater: Optimisation and kinetic studies. Alex. Eng. J. 2014, 53, 417–426. [Google Scholar] [CrossRef]
- Körbahti, B.K.; Turan, K.M. Evaluation of Energy Consumption in Electrochemical Oxidation of Acid Violet 7 Textile Dye using Pt/Ir Electrodes. J. Turk. Chem. Soc. Sect. A Chem. 2016, 3, 75. [Google Scholar] [CrossRef][Green Version]
- Martínez-Huitle, C.A.; Panizza, M. Electrochemical oxidation of organic pollutants for wastewater treatment. Curr. Opin. Electrochem. 2018, 11, 62–71. [Google Scholar] [CrossRef]
- Cañizares, P.; Paz, R.; Sáez, C.; Rodrigo, M.A. Costs of the electrochemical oxidation of wastewaters: A comparison with ozonation and Fenton oxidation processes. J. Environ. Manag. 2009, 90, 410–420. [Google Scholar] [CrossRef] [PubMed]
- Escudero, C.J.; Iglesias, O.; Dominguez, S.; Rivero, M.J.; Ortiz, I. Performance of electrochemical oxidation and photocatalysis in terms of kinetics and energy consumption. New insights into the p-cresol degradation. J. Environ. Manag. 2017, 195, 117–124. [Google Scholar] [CrossRef] [PubMed]
- Woisetschläger, D.; Humpl, B.; Koncar, M.; Siebenhofer, M. Electrochemical oxidation of wastewater-Opportunities and drawbacks. Water Sci. Technol. 2013, 68, 1173–1179. [Google Scholar] [CrossRef] [PubMed]
- Zöllig, H.; Remmele, A.; Morgenroth, E.; Udert, K.M. Removal rates and energy demand of the electrochemical oxidation of ammonia and organic substances in real stored urine. Environ. Sci. Water Res. Technol. 2017, 3, 480–491. [Google Scholar] [CrossRef]
- Ukundimana, Z.; Omwene, P.I.; Gengec, E.; Can, O.T.; Kobya, M. Electrooxidation as post treatment of ultrafiltration effluent in a landfill leachate MBR treatment plant: Effects of BDD, Pt and DSA anode types. Electrochim. Acta 2018, 286, 252–263. [Google Scholar] [CrossRef]
- Iskurt, C.; Keyikoglu, R.; Kobya, M.; Khataee, A. Treatment of coking wastewater by aeration assisted electrochemical oxidation process at controlled and uncontrolled initial pH conditions. Sep. Purif. Technol. 2020, 248, 117043. [Google Scholar] [CrossRef]

| Coded Levels | |||||
|---|---|---|---|---|---|
| Symbols | Independent Variables | Units | −1 | 0 | +1 |
| A | Temperature | °C | 20 | 40 | 60 |
| B | NaCl Concentration | g/L | 2 | 4 | 6 |
| C | Current Density | mA/cm2 | 5 | 7.5 | 10 |
| Run | Independent Variables | COD Removal (%) | |||
|---|---|---|---|---|---|
| A: Temperature (°C) | B: NaCl Concentration (g/L) | C: Current Density (mA/cm2) | Experimental Values | Predicted Values | |
| 1 | 40 | 2 | 7.5 | 87.00 | 87.28 |
| 2 | 40 | 4 | 7.5 | 91.70 | 90.89 |
| 3 | 40 | 6 | 7.5 | 90.20 | 89.92 |
| 4 | 60 | 2 | 10 | 84.90 | 84.95 |
| 5 | 20 | 4 | 7.5 | 88.20 | 88.50 |
| 6 | 20 | 6 | 10 | 86.20 | 86.73 |
| 7 | 40 | 4 | 10 | 90.60 | 90.87 |
| 8 | 20 | 2 | 10 | 85.90 | 85.52 |
| 9 | 40 | 4 | 5 | 89.10 | 89.43 |
| 10 | 20 | 2 | 5 | 83.70 | 83.90 |
| 11 | 20 | 6 | 5 | 86.00 | 85.75 |
| 12 | 60 | 6 | 10 | 89.10 | 88.38 |
| 13 | 40 | 6 | 10 | 89.70 | 89.74 |
| 14 | 60 | 2 | 5 | 83.40 | 83.05 |
| 15 | 60 | 4 | 7.5 | 88.50 | 88.90 |
| 16 | 60 | 6 | 5 | 86.70 | 87.12 |
| Source | Sum of Squares | Df | Mean Square | F-Value | p-Value |
|---|---|---|---|---|---|
| Model | 176.58 | 9 | 19.62 | 119.22 | <0.0001 1 |
| A-Temperature | 0.80 | 1 | 0.80 | 4.86 | 0.0382 1 |
| B-NaCl concentration | 37.02 | 1 | 37.02 | 224.96 | <0.0001 1 |
| C-Current Density | 11.02 | 1 | 11.02 | 66.98 | <0.0001 1 |
| AB | 4.95 | 1 | 4.95 | 30.08 | <0.0001 1 |
| AC | 0.076 | 1 | 0.076 | 0.46 | 0.5049 2 |
| BC | 0.45 | 1 | 0.45 | 2.75 | 0.1117 |
| A2 | 29.58 | 1 | 29.58 | 179.74 | <0.0001 |
| B2 | 28.09 | 1 | 28.09 | 170.66 | <0.0001 |
| C2 | 2.92 | 1 | 2.92 | 17.76 | 0.0004 |
| Residual | 3.62 | 22 | 0.16 | ||
| Lack of Fit | 2.69 | 6 | 0.45 | 7.66 | 0.0005 1 |
| Pure Error | 0.93 | 16 | 0.058 | ||
| Corr. Total | 180.20 | 31 | |||
| Std. Deviation | 0.41 | R-Squared | 0.9799 | ||
| Mean | 87.56 | Adjusted R2 | 0.9717 | ||
| Coefficient of variation % | 0.46 | Predicted R2 | 0.9564 |
| R2 | Adjusted R2 | Predicted R2 | |
|---|---|---|---|
| COD reduction efficiency % | 0.9799 | 0.9717 | 0.9564 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chakawa, S.; Aziz, M. Investigating the Result of Current Density, Temperature, and Electrolyte Concentration on COD: Subtraction of Petroleum Refinery Wastewater Using Response Surface Methodology. Water 2021, 13, 835. https://doi.org/10.3390/w13060835
Chakawa S, Aziz M. Investigating the Result of Current Density, Temperature, and Electrolyte Concentration on COD: Subtraction of Petroleum Refinery Wastewater Using Response Surface Methodology. Water. 2021; 13(6):835. https://doi.org/10.3390/w13060835
Chicago/Turabian StyleChakawa, Sharon, and Mujahid Aziz. 2021. "Investigating the Result of Current Density, Temperature, and Electrolyte Concentration on COD: Subtraction of Petroleum Refinery Wastewater Using Response Surface Methodology" Water 13, no. 6: 835. https://doi.org/10.3390/w13060835
APA StyleChakawa, S., & Aziz, M. (2021). Investigating the Result of Current Density, Temperature, and Electrolyte Concentration on COD: Subtraction of Petroleum Refinery Wastewater Using Response Surface Methodology. Water, 13(6), 835. https://doi.org/10.3390/w13060835







