Hydrostratigraphic Framework and Physicochemical Status of Groundwater in the Gioia Tauro Coastal Plain (Calabria—Southern Italy)
Abstract
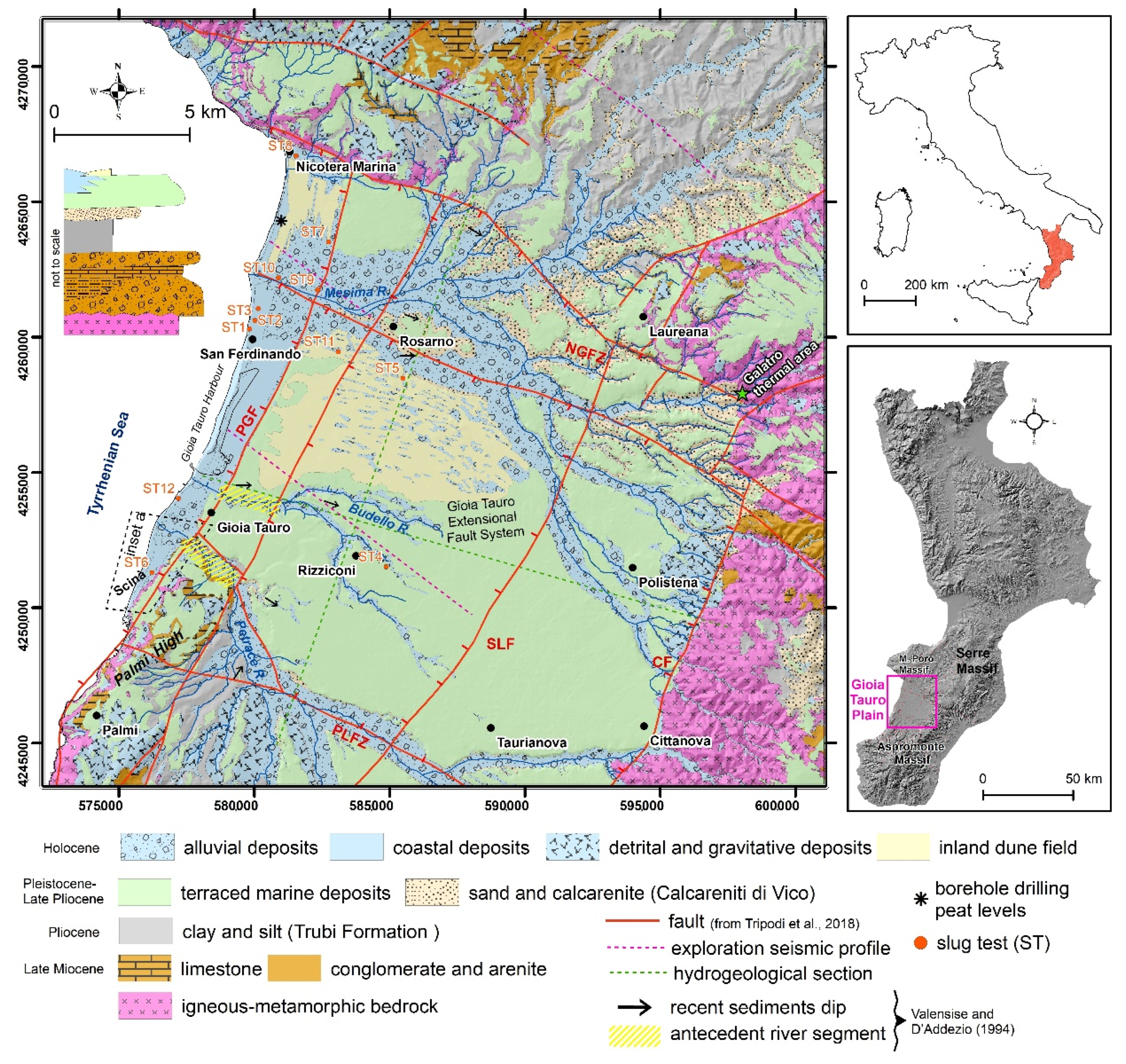
:1. Introduction
2. Geological and Hydrogeochemical Background
3. Methods
4. Results and Discussion
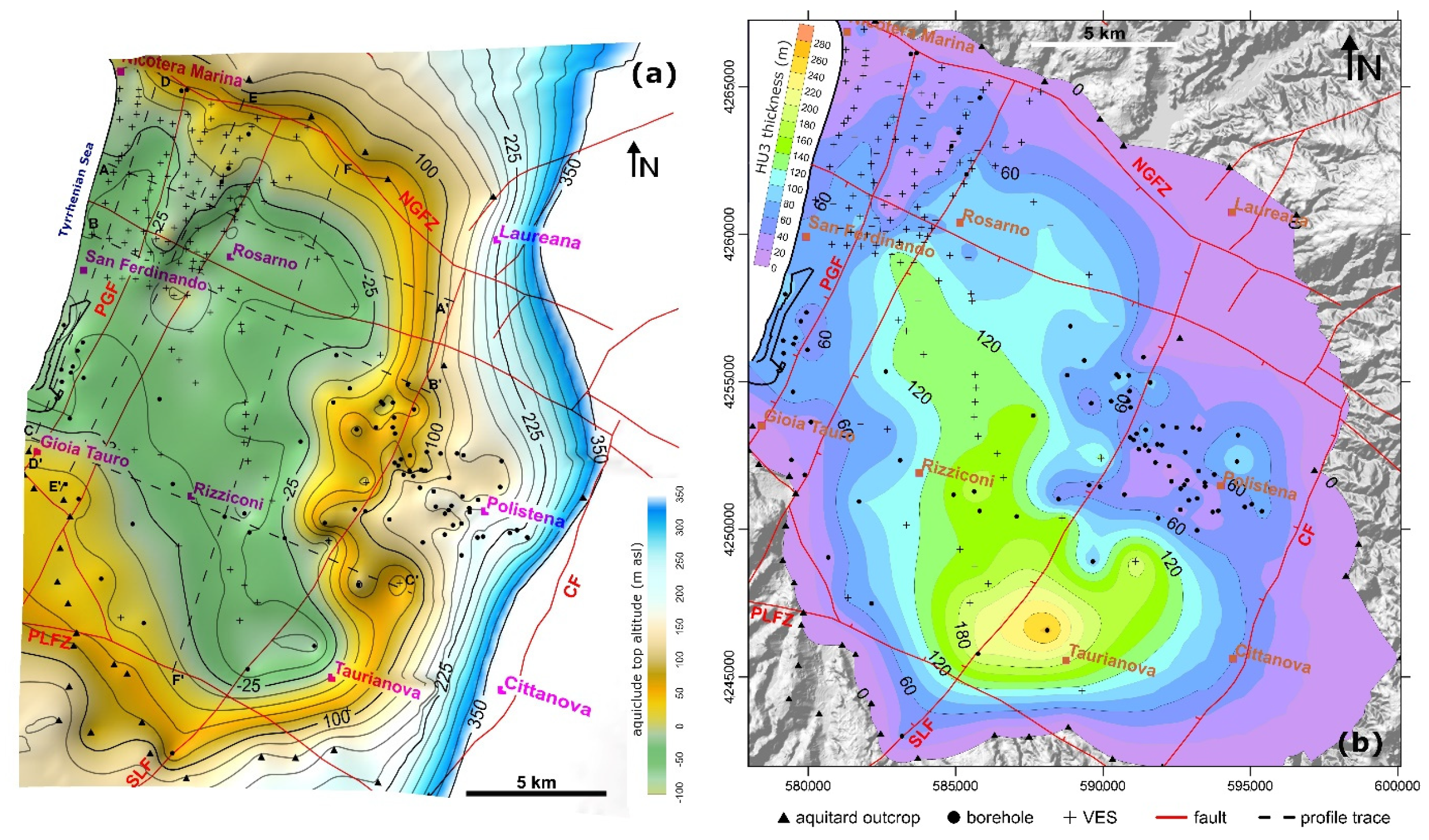
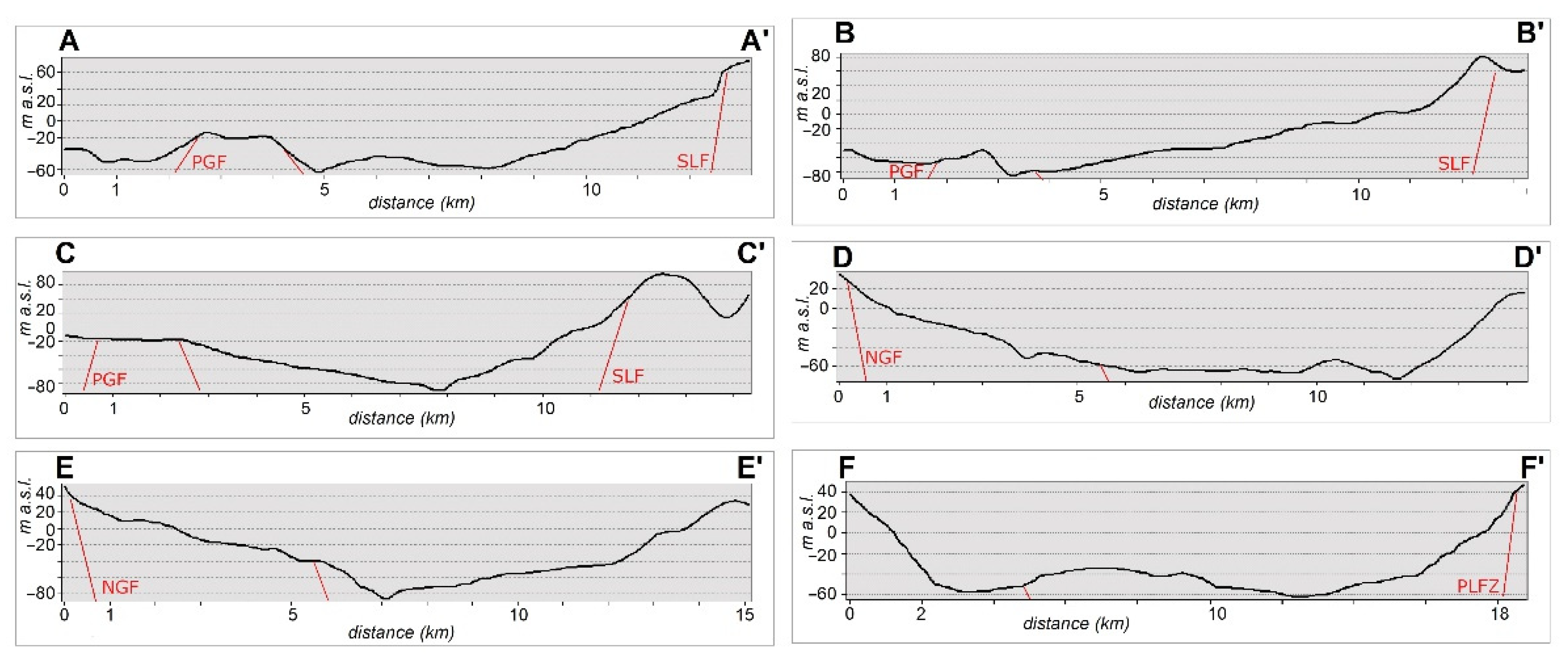
4.1. Lithology and Geometry of the Hydro-Stratigraphic Units
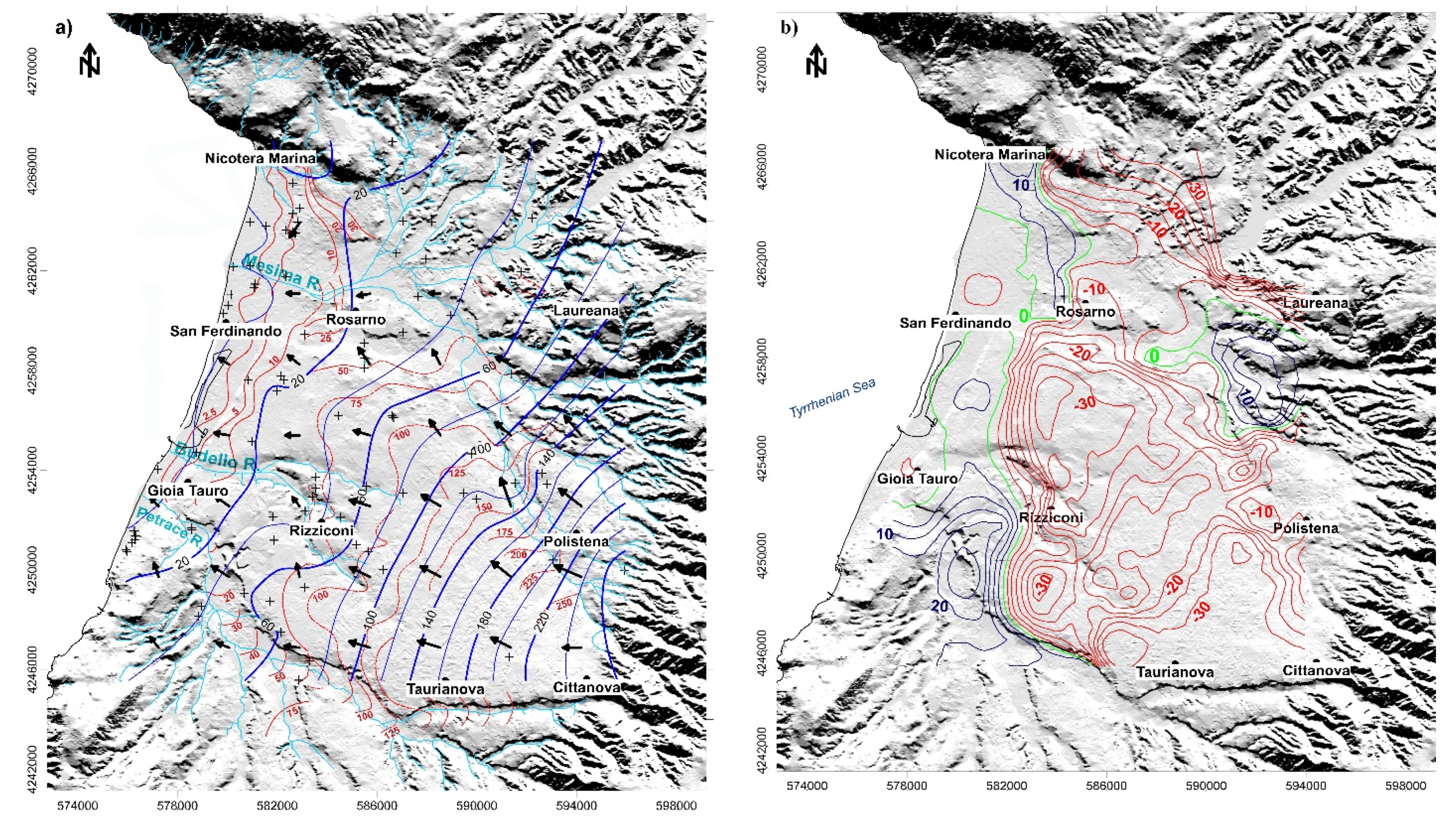
4.2. Hydrography and Groundwater Surface
4.3. Hydraulic Properties of the Hydrogeological Aquifer Units
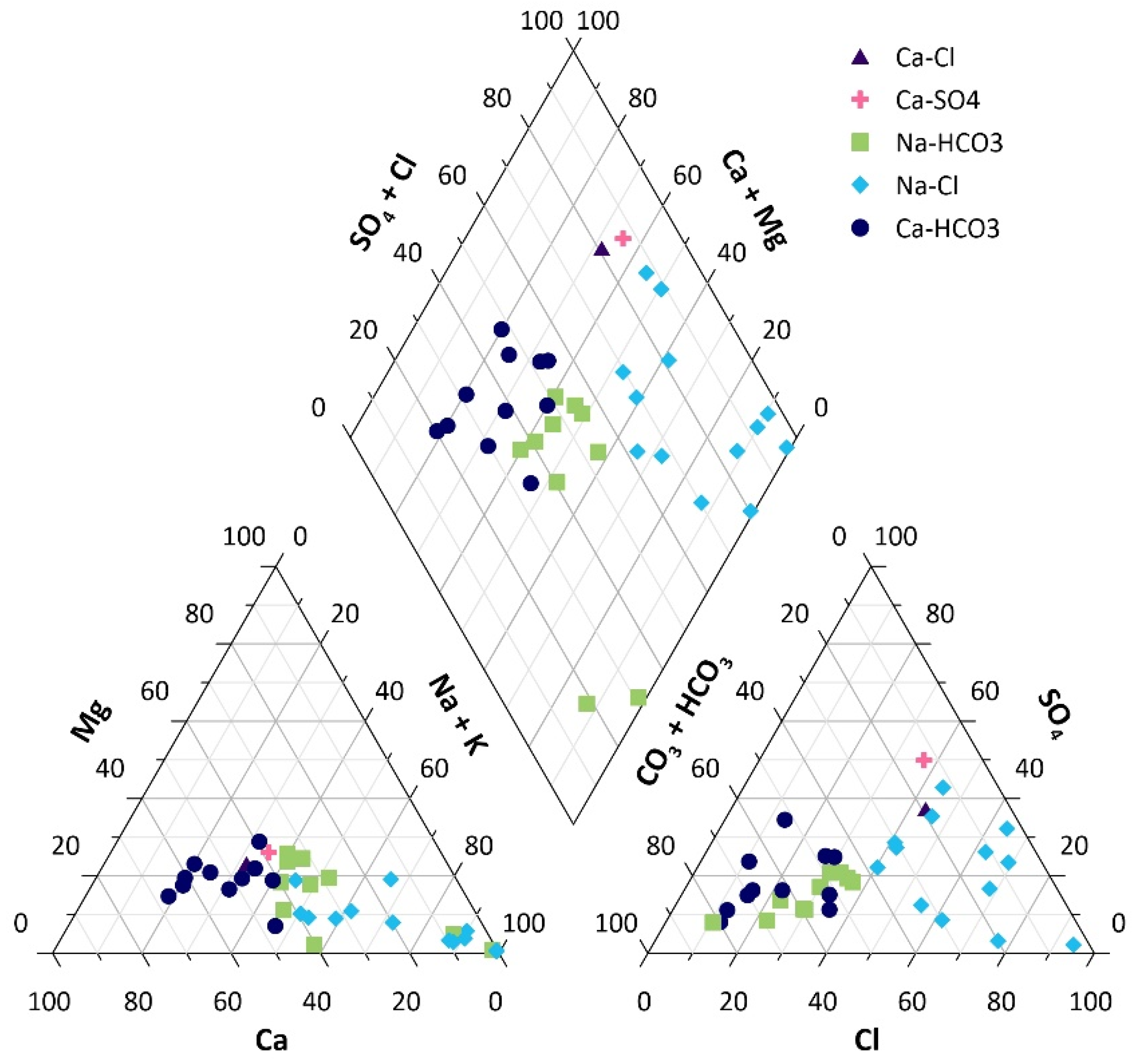
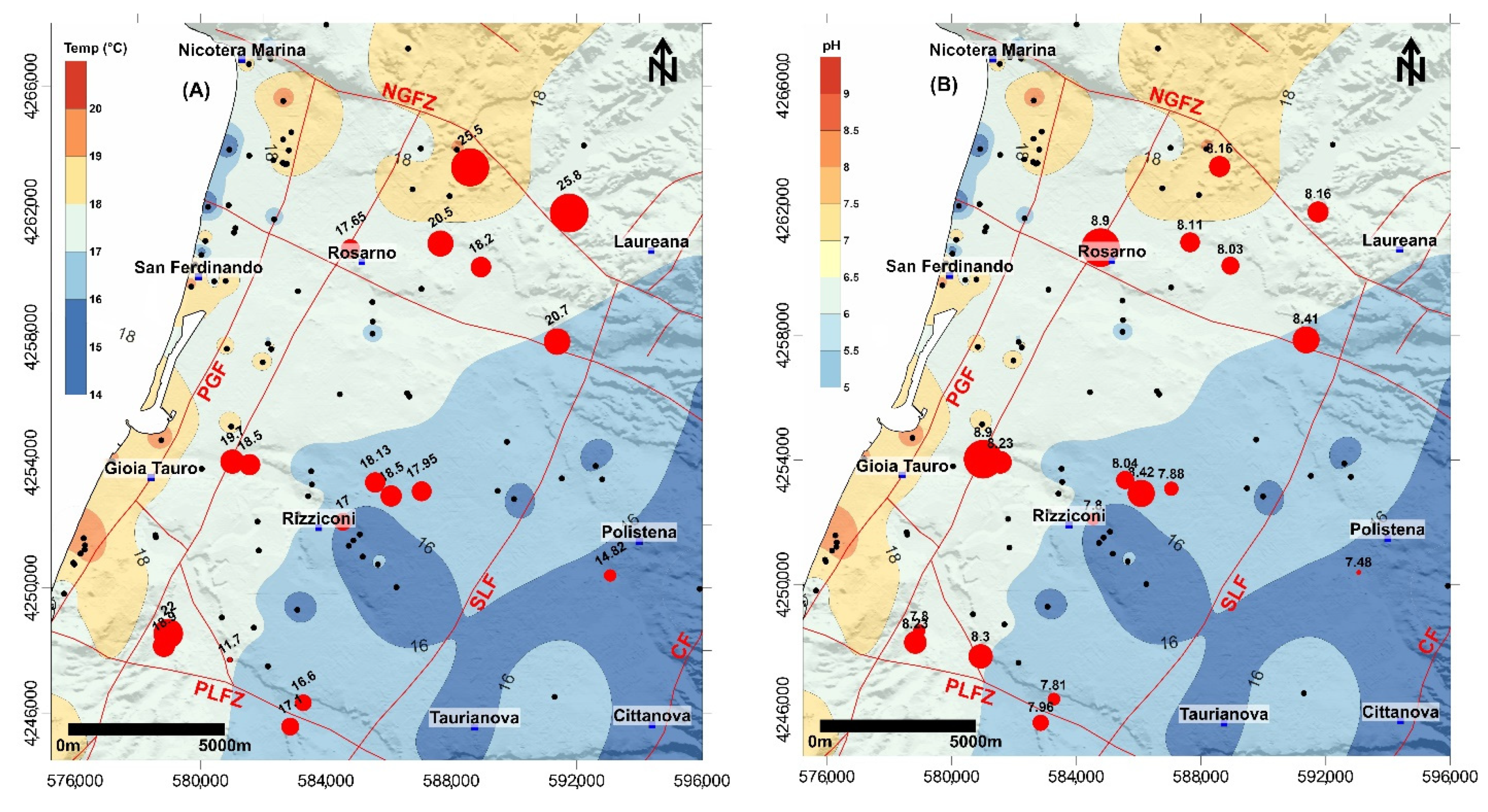
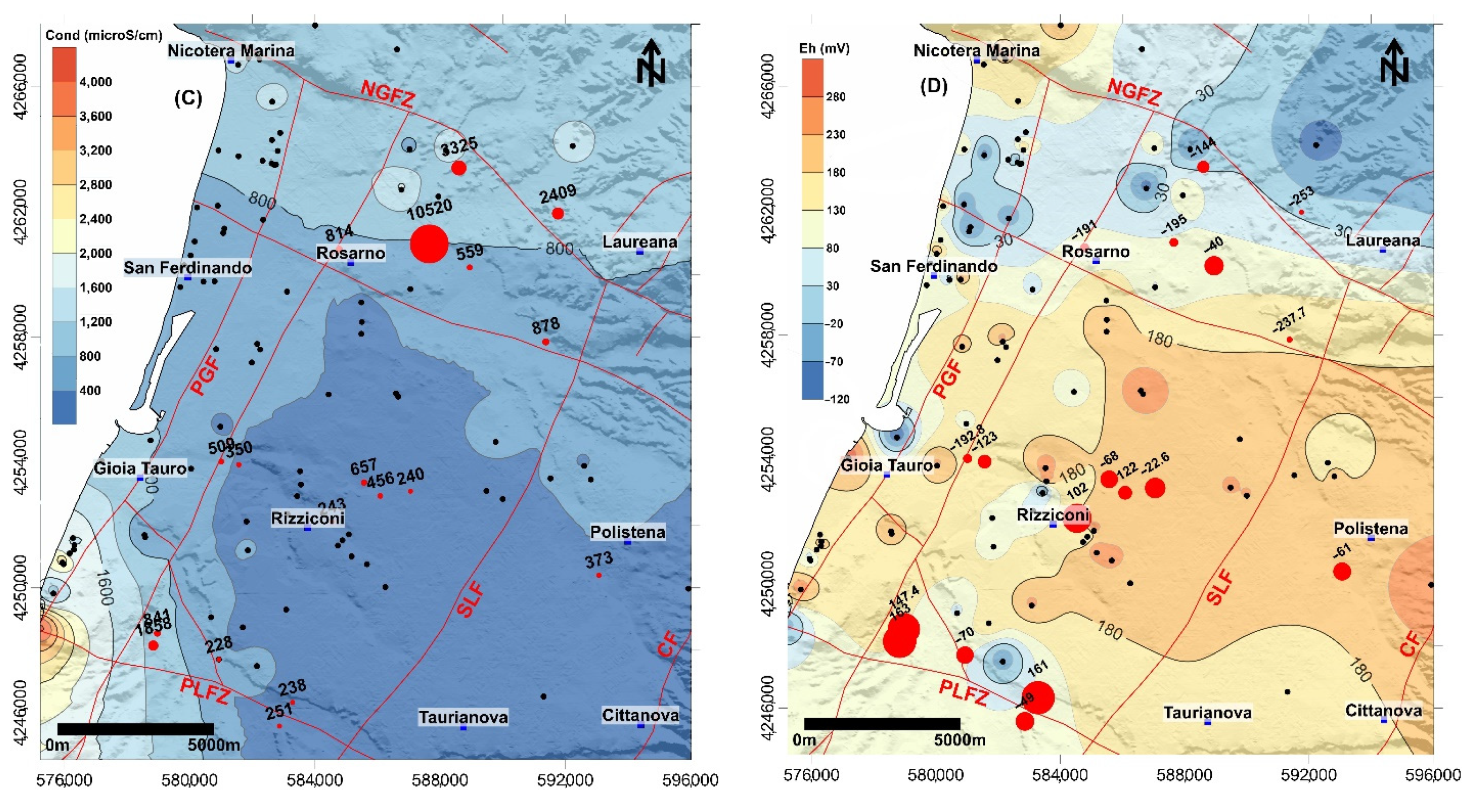
4.4. Groundwater Characterisation
5. Concluding Remarks
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Boughriba, M.; Jilali, A. Climate change and modeling of an unconfined aquifer: The Triffa plain, Morocco. Environ. Dev. Sustain. 2018, 20, 2009–2026. [Google Scholar] [CrossRef]
- Matiatos, I.; Paraskevopoulou, V.; Lazogiannis, K.; Botsou, F.; Dassenakis, M.; Ghionis, G.; Alexopoulos, J.D.; Poulos, S.E. Surface–ground water interactions and hydrogeochemical evolution in a fluvio-deltaic setting: The case study of the Pinios River delta. J. Hydrol. 2018, 561, 236–249. [Google Scholar] [CrossRef]
- Gkiougkis, I.; Pouliaris, C.; Pliakas, F.; Diamantis, I.; Kallioras, A. Conceptual and Mathematical Modeling of a Coastal Aquifer in Eastern Delta of R. Nestos (N. Greece). Hydrology 2021, 8, 23. [Google Scholar] [CrossRef]
- Mastrocicco, M.; Colombani, N. The Issue of Groundwater Salinization in Coastal Areas of the Mediterranean Region: A Review. Water 2021, 13, 90. [Google Scholar] [CrossRef]
- Vousdoukas, M.I.; Mentaschi, L.; Voukouvalas, E.; Verlaan, M.; Feyen, L. Extreme Sea levels on the rise along Europe’s coasts. Earth’s Future 2017, 5, 304–323. [Google Scholar] [CrossRef]
- Petrucci, O.; Polemio, M. Flood risk mitigation and anthropogenic modifications of a coastal plain in southern Italy: Combined effects over the past 150 years. Nat. Hazards Earth Syst. Sci. 2007, 7, 361–373. [Google Scholar] [CrossRef] [Green Version]
- Sanford, W.; Langevin, C.; Polemio, M.; Povinec, P. A new focus on groundwater seawater interactions. In A New Focus on Groundwater-Seawater Interactions; Sanford, W., Langevin, C., Polemio, M., Povinec, P., Eds.; IAHS Press: Wallingford, UK, 2007; Volume 312, pp. V–VI. [Google Scholar]
- De Montety, V.; Radakovitch, O.; Vallet-Coulomb, C.; Blavoux, B.; Hermitte, D.; Valles, V. Origin of groundwater salinity and hydrogeochemical processes in a confined coastal aquifer: Case of the Rhone delta (Southern France). Appl. Geochem. 2008, 23, 2337–2349. [Google Scholar] [CrossRef]
- Haruyama, S. Introduction-overview of natural disasters and coastal landforms. In Natural Disaster and Coastal Geomorphology; Haruyama, S., Sugai, T., Eds.; Springer: Cham, Switzerland, 2016; pp. 1–13. [Google Scholar] [CrossRef]
- Cianflone, G.; Dominici, R.; Viscomi, A. Potential recharge estimation of the Sibari Plain aquifers (southern Italy) through a new GIS procedure. Geographia Techica 2015, 10, 8–18. [Google Scholar]
- Cianflone, G.; Tolomei, C.; Brunori, C.A.; Dominici, R. InSAR time series analysis of natural and anthropogenic coastal plain subsidence: The case of Sibari (Southern Italy). Remote. Sens. 2015, 7, 16004–16023. [Google Scholar] [CrossRef] [Green Version]
- Polemio, M.; Zuffianò, L.E. Review of Utilization Management of Groundwater at Risk of Salinization. J. Water Resour. Plan. Manag. 2020, 146, 20. [Google Scholar] [CrossRef]
- Polemio, M.; Petrucci, O. The occurrence of floods and the role of climate variations from 1880 in Calabria (Southern Italy). Nat. Hazards Earth Syst. Sci. 2012, 12, 129–142. [Google Scholar] [CrossRef] [Green Version]
- Polemio, M. Monitoring and management of karstic coastal groundwater in a changing environment (Southern Italy): A review of a regional experience. Water 2016, 8, 148. [Google Scholar] [CrossRef] [Green Version]
- Vespasiano, G.; Apollaro, C.; Marini, L.; Dominici, R.; Cianflone, G.; Romanazzi, A.; Polemio, M.; De Rosa, R. Hydrogeological and isotopic study of the multi-aquifer system of the Sibari Plain (Calabria, Southern Italy). Rend. Online Soc. Geol. It. 2015, 39, 134–137. [Google Scholar] [CrossRef]
- Vespasiano, G.; Cianflone, G.; Cannata, C.B.; Apollaro, C.; Dominici, R.; De Rosa, R. Analysis of groundwater pollution in the Sant’Eufemia Plain (Calabria—South Italy). Ital. J. Eng. Geol. Environ. 2016, 2, 5–15. [Google Scholar] [CrossRef]
- Vespasiano, G.; Cianflone, G.; Romanazzi, A.; Apollaro, C.; Dominici, R.; Polemio, M.; De Rosa, R. A multidisciplinary approach for sustainable management of a complex coastal plain: The case of Sibari Plain (Southern Italy). Mar. Pet. Geol. 2019, 109, 710–759. [Google Scholar] [CrossRef]
- ISTAT. Available online: http://demo.istat.it/bilmens/index02.php?anno=2020&lingua=ita (accessed on 2 September 2021).
- Pizzino, L.; Burrato, P.; Quattrocchi, F.; Valensise, G. Geochemical signatures of large active faults: The example of the 5 February 1783, Calabrian earthquake (southern Italy). J. Seismol. 2004, 8, 363–380. [Google Scholar] [CrossRef]
- Italiano, F.; Bonfanti, P.; Pizzino, L.; Quattrocchi, F. Geochemistry of fluids discharged over the seismic area of the Southern Apennines (Calabria region, Southern Italy): Implications for fluid-fault relationships. Appl. Geochem. 2010, 25, 540–554. [Google Scholar] [CrossRef]
- Monaco, C.; Tortorici, L. Active faulting in the Calabrian arc and eastern Sicily. J. Geodyn. 2000, 29, 407–424. [Google Scholar] [CrossRef]
- Tripodi, V.; Muto, F.; Brutto, F.; Perri, F.; Critelli, S. NeogenQuaternary evolution of the forearc and backarc regions between the Serre and Aspromonte Massifs, Calabria (southern Italy). Mar. Pet. Geol. 2018, 95, 328–343. [Google Scholar] [CrossRef]
- Jacques, E.; Monaco, C.; Tapponnier, P.; Tortorici, L.; Winter, T. Faulting and earthquake triggering during the 1783 Calabria seismic sequence. Geophys. J. Int. 2001, 147, 499–516. [Google Scholar] [CrossRef] [Green Version]
- Galli, P.; Bosi, V. Paleoseismology along the Cittanova fault: Implications for seismotectonics and earthquake recurrence in Calabria (southern Italy). J. Geophys. Res. 2002, 107, 2044. [Google Scholar] [CrossRef]
- Galli, P.; Peronace, E. Low slip rates and multimillennial return times for Mw 7 earthquake faults in southern Calabria (Italy). Geophys. Res. Lett. 2015, 42, 5258–5265. [Google Scholar] [CrossRef] [Green Version]
- Valensise, G.; D’Addezio, G. Il contributo della geologia di superficie all’identificazione delle strutture sismogenetiche della Piana di Gioia Tauro. In Internal Rep. 559; Ist. Naz. di Geofis.: Bologna, Italy, 1994; p. 21. [Google Scholar]
- ISPRA. Foglio 590-Taurianova. In Carta Geologica d’Italia Alla Scala 1:50.000; Istituto Superiore per la Protezione e la Ricerca Ambientale: Roma, Italy, 2016. [Google Scholar]
- De Rosa, R.; Dominici, R.; Donato, P.; Barca, D. Widespread syn-eruptive volcaniclastic deposits in the Pleistocenic basins of South-Western Calabria. J. Volcanol. Geotherm. Res. 2008, 177, 155–169. [Google Scholar] [CrossRef]
- Longhitano, S.G.; Chiarella, D.; Di Stefano, A.; Messina, C.; Sabato, L.; Tropeano, M. Tidal signatures in Neogene to Quaternary mixed deposits of southern Italy straits and bays. Sediment. Geol. 2012, 279, 74–96. [Google Scholar] [CrossRef]
- Borrelli, L.; Antronico, L.; Le Pera, E.; Pisano, B.; Sorriso-Valvo, M. Morphology, properties, and source of windblown sediments of the coastal dune field in the Gioia Tauro Plain, Calabria, southern Italy. Catena 2021, 201, 105193. [Google Scholar] [CrossRef]
- ISPRA-Indagini nel Sottosuolo (L.464/84). Available online: http://sgi.isprambiente.it/GMV2/index.html (accessed on 27 August 2021).
- Facciorusso, J.; Vannucchi, G. Liquefaction hazard maps of the harbour area of Gioia Tauro (Italy) by geo-statistical methods. In Proceedings of the 4th International Conference of Earthquake Engineering and Seismology, Tehran, Iran, 12–14 May 2003. [Google Scholar]
- Anderson, M.P.; Woessner, W.W. Applied Groundwater Modelling: Simulation of Flow and Advective Transport; Academic Press: London, UK, 1992. [Google Scholar]
- Bersezio, R.; Giudici, M.; Mele, M. Combining sedimentological and geophysical data for high-resolution 3-D mapping of fluvial architectural elements in the Quaternary Po plain (Italy). Sediment. Geol. 2007, 202, 230–248. [Google Scholar] [CrossRef]
- Cianflone, G.; Cavuoto, G.; Punzo, M.; Dominici, R.; Sonnino, M.; Di Fiore, V.; Pelosi, N.; Tarallo, D.; Lirer, F.; Marsella, E.; et al. Late quaternary stratigraphic setting of the Sibari Plain (southern Italy): Hydrogeological implications. Mar. Pet. Geol. 2018, 97, 422–436. [Google Scholar] [CrossRef]
- Casmez (Cassa Speciale per il Mezzogiorno). Progetto Speciale 26; CMP: Roma, Italy, 1987. [Google Scholar]
- Logan, J. Estimating transmissibility from routine production tests of waterwalls. Groundwater 1964, 2, 35–37. [Google Scholar] [CrossRef]
- Hvorslev, M.J. Time Lag and Soil Permeability in Ground-Water Observations. In Bull. No. 36 Waterways Exper. Sta. Corps of Engineers; U.S. Army: Vicksburg, MI, USA, 1951; pp. 1–50. [Google Scholar]
- Apollaro, C.; Vespasiano, G.; De Rosa, R.; Marini, L. Use of mean residence time and flowrate of thermal waters to evaluate the volume of reservoir water contributing to the natural discharge and the related geothermal reservoir volume. Appl. North. Thail. Hot Springs Geotherm. 2015, 58, 62–74. [Google Scholar]
- Vespasiano, G.; Marini, L.; Muto, F.; Auqué, L.F.; Cipriani, M.; De Rosa, R.; Critelli, S.; Gimeno, M.J.; Blasco, M.; Dotsika, E.; et al. Chemical, isotopic and geotectonic relations of the warm and cold waters of the Cotronei (Ponte Coniglio), Bruciarello and Repole thermal areas, (Calabria—Southern Italy). Geothermics 2021, 96. [Google Scholar] [CrossRef]
- Nordstrom, D.K. Thermochemical redox equilibria of ZoBell’s solution. Geochim. Cosmochim. Acta 1997, 41, 1835–1841. [Google Scholar] [CrossRef]
- Carrozzo, M.T.; Luzio, C.; Margotta, C.; Quarta, T. Gravity Map of Italy. Scale 1:500 000. In Progetto Finalizzato Geodinamica; Consiglio Nazionale delle Ricerche: Rome, Italy, 1986. [Google Scholar]
- Domenico, P.A.; Schwartz, F.W. Physical and Chemical Hydrogeology; John Wiley & Sons: New York, NY, USA, 1990; 824p. [Google Scholar]
- Phocaides, A. Water quality for irrigation. In Technical Handbook on Pressurized Irrigation Techniques, Ch. 7; FAO: Roma, Italy, 2000; pp. 79–97. [Google Scholar]
- Savage, D.; Edmunds, W.M. Geochemical characteristics of groundwater in granites and related crystalline rocks. In Applied Groundwater Hydrology; Downing, R.A., Wilkinson, W.B., Eds.; Oxford University: Oxford, UK, 1991. [Google Scholar]
- Stober, I.; Zhong, J.; Zhang, L.; Bucher, K. Deep hydrothermal fluid–rock interaction: The thermal springs of Da Qaidam, China. Geofluids 2016, 16, 711–728. [Google Scholar] [CrossRef]
- Curewitz, D.; Karson, J.A. Structural settings of hydrothermal outflow: Fracture permeability maintained by fault propagation and interaction. J. Volc. Geoth. Res. 1997, 79, 149–168. [Google Scholar] [CrossRef]
- Apollaro, C.; Tripodi, V.; Vespasiano, G.; De Rosa, R.; Dotsika, E.; Fuoco, I.; Critelli, S.; Muto, F. Chemical, isotopic and geotectonic relations of the warm and cold waters of the Galatro and Antonimina thermal areas, southern Calabria, Italy. Mar. Pet. Geol. 2019, 109, 469–483. [Google Scholar] [CrossRef]
- Bense, V.F.; Gleeson, T.; Loveless, S.E.; Bour, O.; Scibek, J. Fault zone hydrogeology. Earth-Sci. Rev. 2013, 127, 171–192. [Google Scholar] [CrossRef]
- Medici, G.; Smeraglia, L.; Torabi, A.; Botter, C. Review of modeling approaches to groundwater flow in deformed carbonate aquifers. Groundwater 2021, 59, 334–351. [Google Scholar] [CrossRef] [PubMed]

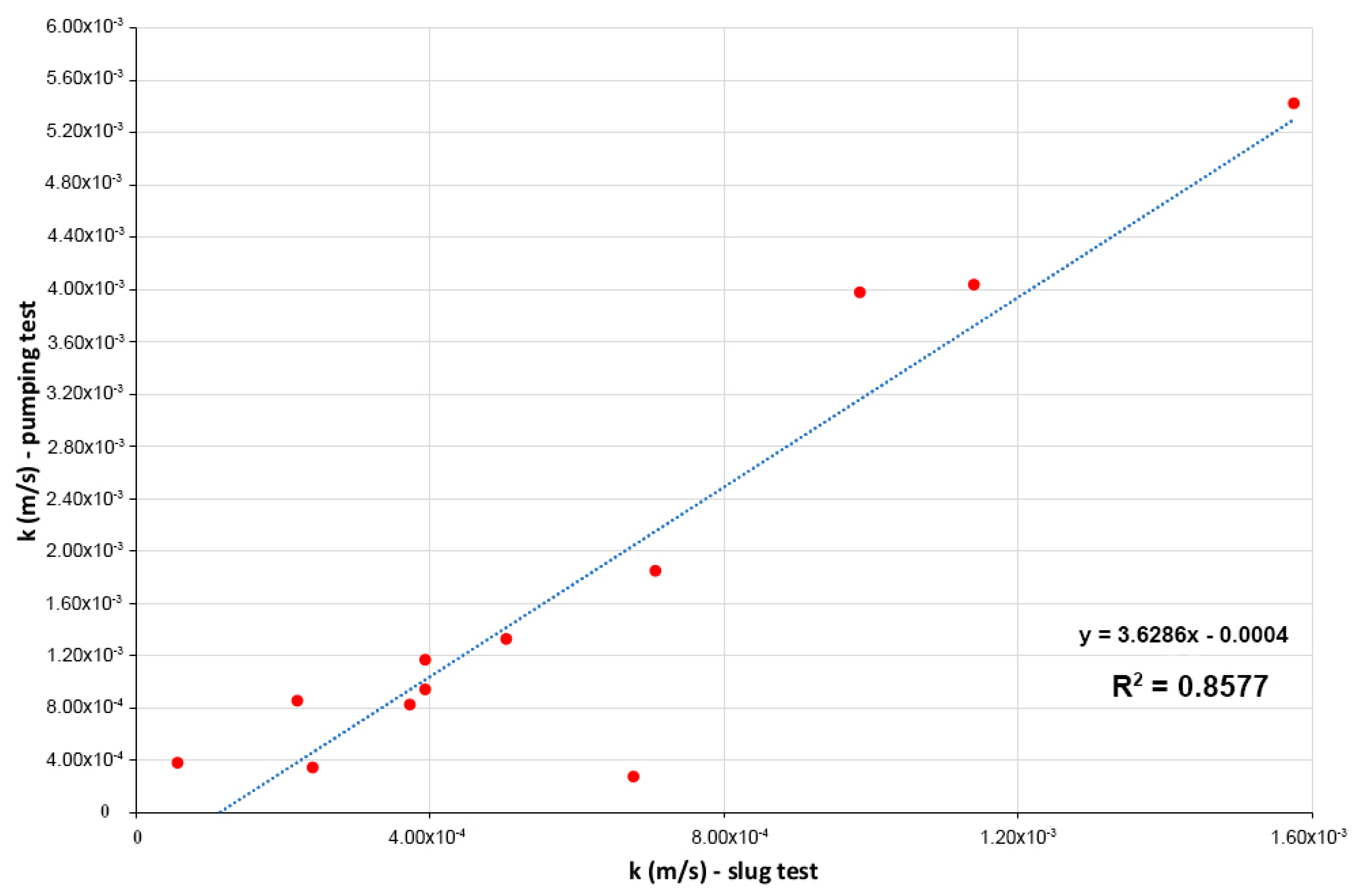
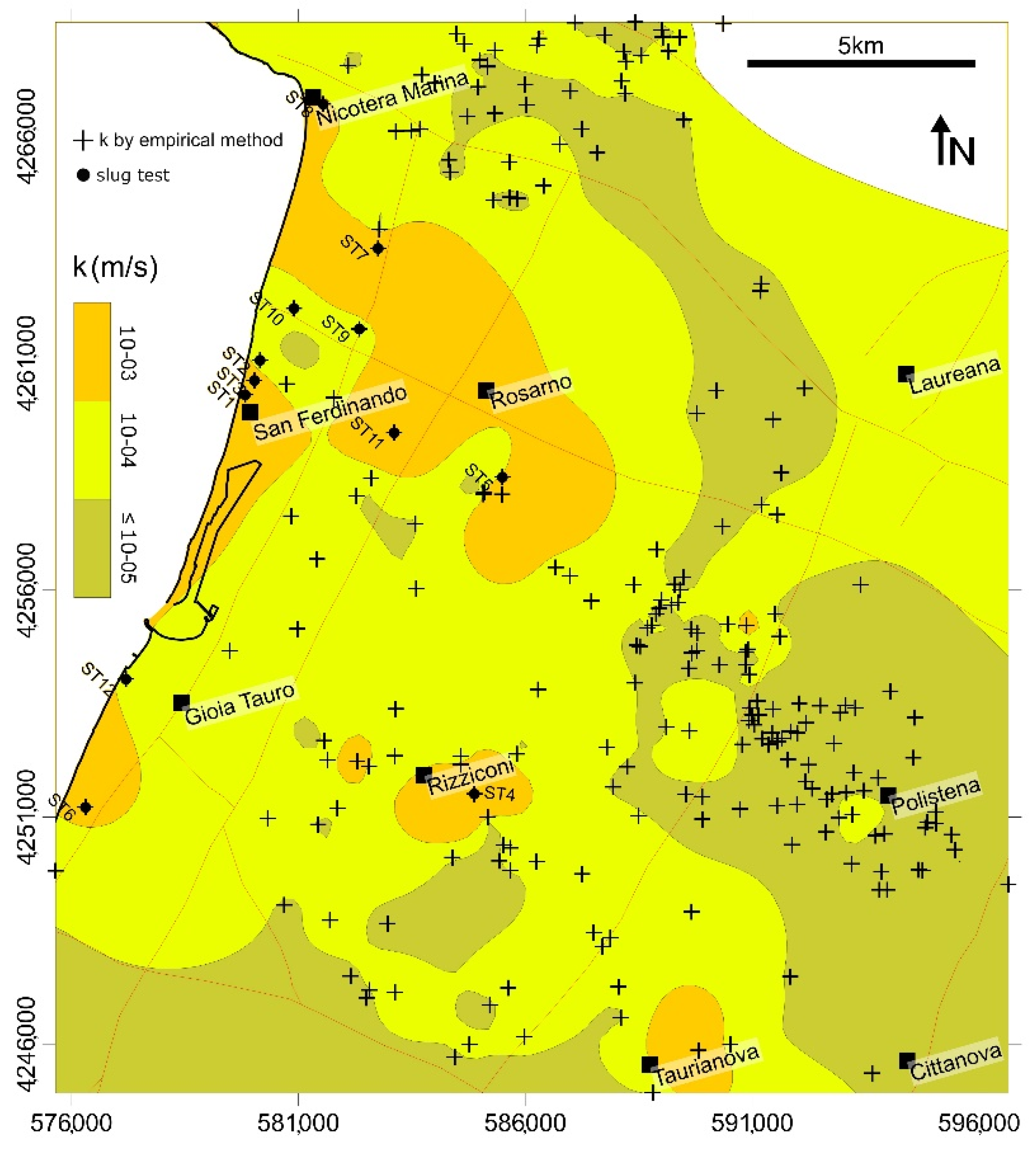

| Slug Test | k (m/s) | k (m/s) Pumping Test |
|---|---|---|
| ST1 | 9.85 × 10−4 | 3.98 × 10−3 |
| ST2 | 3.73 × 10−4 | 8.27 × 10−4 |
| ST3 | 7.07 × 10−4 | 1.85 × 10−3 |
| ST4 | 5.70 × 10−5 | 3.82 × 10−4 |
| ST5 | 2.20 × 10−4 | 8.56 × 10−4 |
| ST6 | 5.04 × 10−4 | 1.33 × 10−3 |
| ST7 | 1.58 × 10−3 | 5.43 × 10−3 |
| ST8 | 3.94 × 10−4 | 1.17 × 10−3 |
| ST9 | 2.41 × 10−4 | 3.46 × 10−4 |
| ST10 | 6.77 × 10−4 | 2.76 × 10−4 |
| ST11 | 1.14 × 10−3 | 4.04 × 10−3 |
| ST12 | 3.94 × 10−4 | 9.43 × 10−4 |
| T | pH | EC (25 °C) | Eh | TDS | Salinity | ||
|---|---|---|---|---|---|---|---|
| (°C) | μS/cm | mV | ppm | p.s.u. | |||
| HU1 | Min | 14.8 | 7.5 | 230 | −253 | 115 | 0.1 |
| Max | 25.8 | 8.9 | 10,520 | 238 | 5264 | 5.9 | |
| Median | 18.2 | 8.16 | 509 | −68 | 254 | 0.25 | |
| Mean | 19.1 | 8.2 | 1375 | −49.3 | 686 | 0.7 | |
| St. Dev. | 2.9 | 0.4 | 2436 | 152.1 | 1219 | 1.4 | |
| HU3 | Min | 11.7 | 5.9 | 228 | −106 | 114 | 0.1 |
| Max | 19.7 | 8.9 | 4049 | 303 | 2025 | 2.2 | |
| Median | 17.55 | 7.04 | 509.5 | 153.6 | 254.5 | 0.25 | |
| Mean | 17.3 | 7.1 | 736 | 126 | 371 | 0.4 | |
| St. Dev. | 1.4 | 0.5 | 595 | 112 | 298 | 0.3 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cianflone, G.; Vespasiano, G.; De Rosa, R.; Dominici, R.; Apollaro, C.; Vaselli, O.; Pizzino, L.; Tolomei, C.; Capecchiacci, F.; Polemio, M. Hydrostratigraphic Framework and Physicochemical Status of Groundwater in the Gioia Tauro Coastal Plain (Calabria—Southern Italy). Water 2021, 13, 3279. https://doi.org/10.3390/w13223279
Cianflone G, Vespasiano G, De Rosa R, Dominici R, Apollaro C, Vaselli O, Pizzino L, Tolomei C, Capecchiacci F, Polemio M. Hydrostratigraphic Framework and Physicochemical Status of Groundwater in the Gioia Tauro Coastal Plain (Calabria—Southern Italy). Water. 2021; 13(22):3279. https://doi.org/10.3390/w13223279
Chicago/Turabian StyleCianflone, Giuseppe, Giovanni Vespasiano, Rosanna De Rosa, Rocco Dominici, Carmine Apollaro, Orlando Vaselli, Luca Pizzino, Cristiano Tolomei, Francesco Capecchiacci, and Maurizio Polemio. 2021. "Hydrostratigraphic Framework and Physicochemical Status of Groundwater in the Gioia Tauro Coastal Plain (Calabria—Southern Italy)" Water 13, no. 22: 3279. https://doi.org/10.3390/w13223279
APA StyleCianflone, G., Vespasiano, G., De Rosa, R., Dominici, R., Apollaro, C., Vaselli, O., Pizzino, L., Tolomei, C., Capecchiacci, F., & Polemio, M. (2021). Hydrostratigraphic Framework and Physicochemical Status of Groundwater in the Gioia Tauro Coastal Plain (Calabria—Southern Italy). Water, 13(22), 3279. https://doi.org/10.3390/w13223279













