Rehabilitation of an Industrial Water Main Using Multicriteria Decision Analysis
Abstract
1. Introduction
2. Data and Methods
2.1. Case Study Description
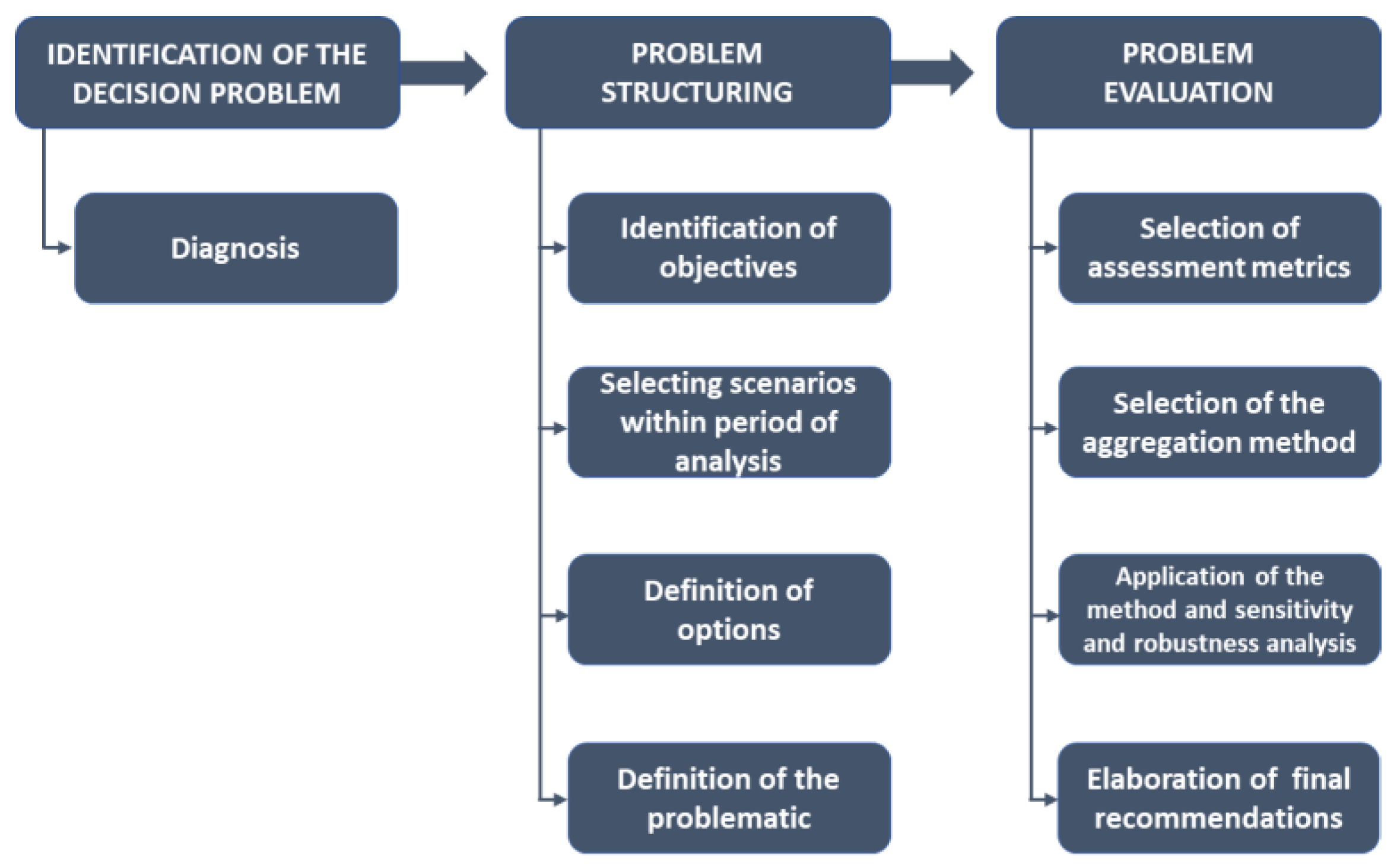
2.2. Structure of the Methodology
2.3. Identification of the Decision Problem
- From the hydraulic point-of-view, this pipe has a maximum flow rate of 40 × 106 m3/year., which is insufficient for satisfying the water demand of new industries that may settle in the industrial park.
- From the structural point of view, the current condition is unknown, as it has been in operation for over 30 years and has never been taken out of service and inspected. However, there are records of some failures that never caused water supply disruptions, such as non-operating valves and small localized bursts in the steel sections of the water main inside the valve chambers. The pipe has signs that indicate an unsatisfactory condition in some areas, mainly where high vegetation growth is observed, indicating the existence of leakage.
- Regarding the satisfaction of the water distribution network located downstream, it is necessary to increase storage capacity.
2.4. Problem Structuring
2.4.1. Identification of Objectives
- Ensure infrastructural sustainability and integrity.
- Ensure compliance with regulatory and contractual requirements related to water supply failures.
- Ensure the economic and financial sustainability of the water utility service.
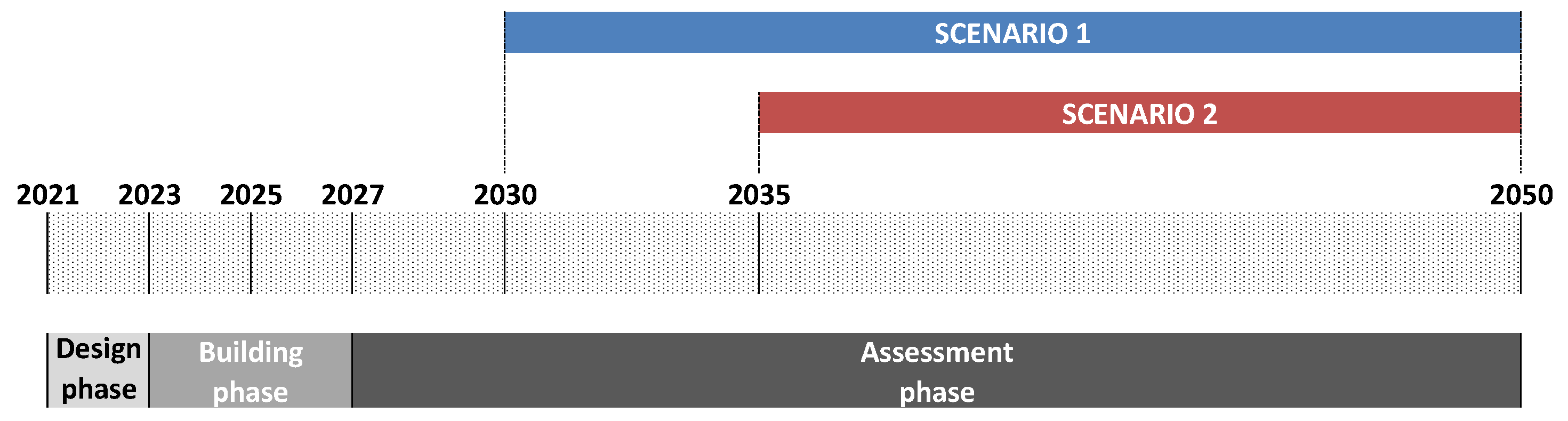
2.4.2. Selecting Scenarios within the Period of Analysis
2.4.3. Definition of Options
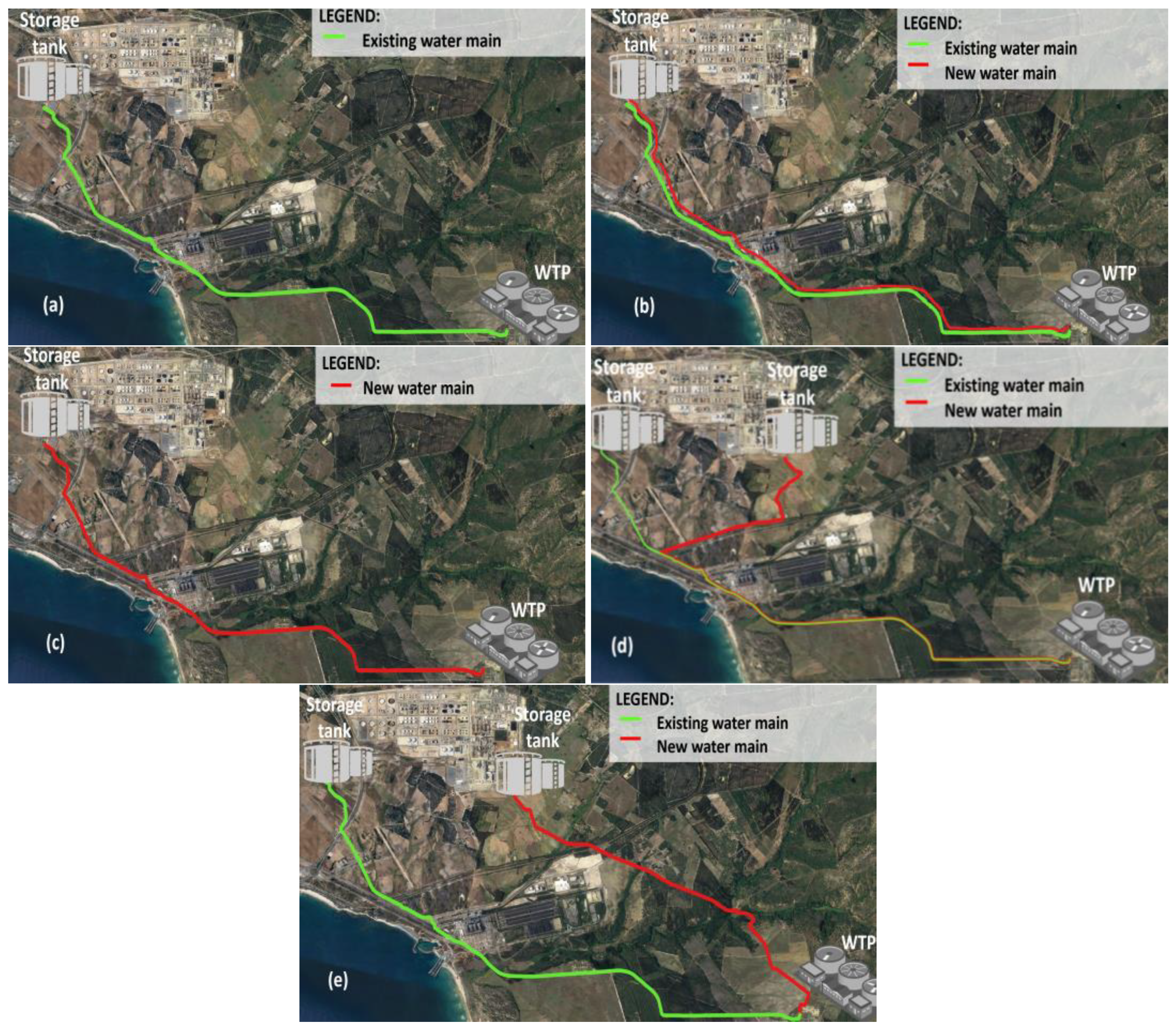
- A0: keeping the status quo situation, in which no rehabilitation interventions are carried out in the water main, maintaining the current O&M practices, and maintaining the maximum hydraulic capacity of the system (ca. 40 × 106 m3/year.).
- A1: the installation of a new pipe in parallel with the existing one, designed to convey ca. 20 × 106 m3/year., and the rehabilitation of the existing pipe.
- A2: the installation of a new pipe in parallel with the existing one, designed to convey ca. 50 × 106 m3/year., and the deactivation of the existing pipe.
- A3: the installation of a new pipe with a different layout, designed to convey ca. 20 × 106 m3/year., the construction of a new storage tank in a new location with a total capacity of 25,000 m3, and the rehabilitation of the existing pipe.
- A4: the same as A3 but considering a total capacity of only 5000 m3 for the new storage tank.
- A5: the same as A3 but considering a new pipe with a different layout.
- A6: the same as A4 but considering a new pipe with a different layout.
Rehabilitation of the Existing Pipe
2.4.4. Definition of the Problematic
2.5. Problem Evaluation
2.5.1. Evaluation Phases
2.5.2. Selection of Assessment Metrics
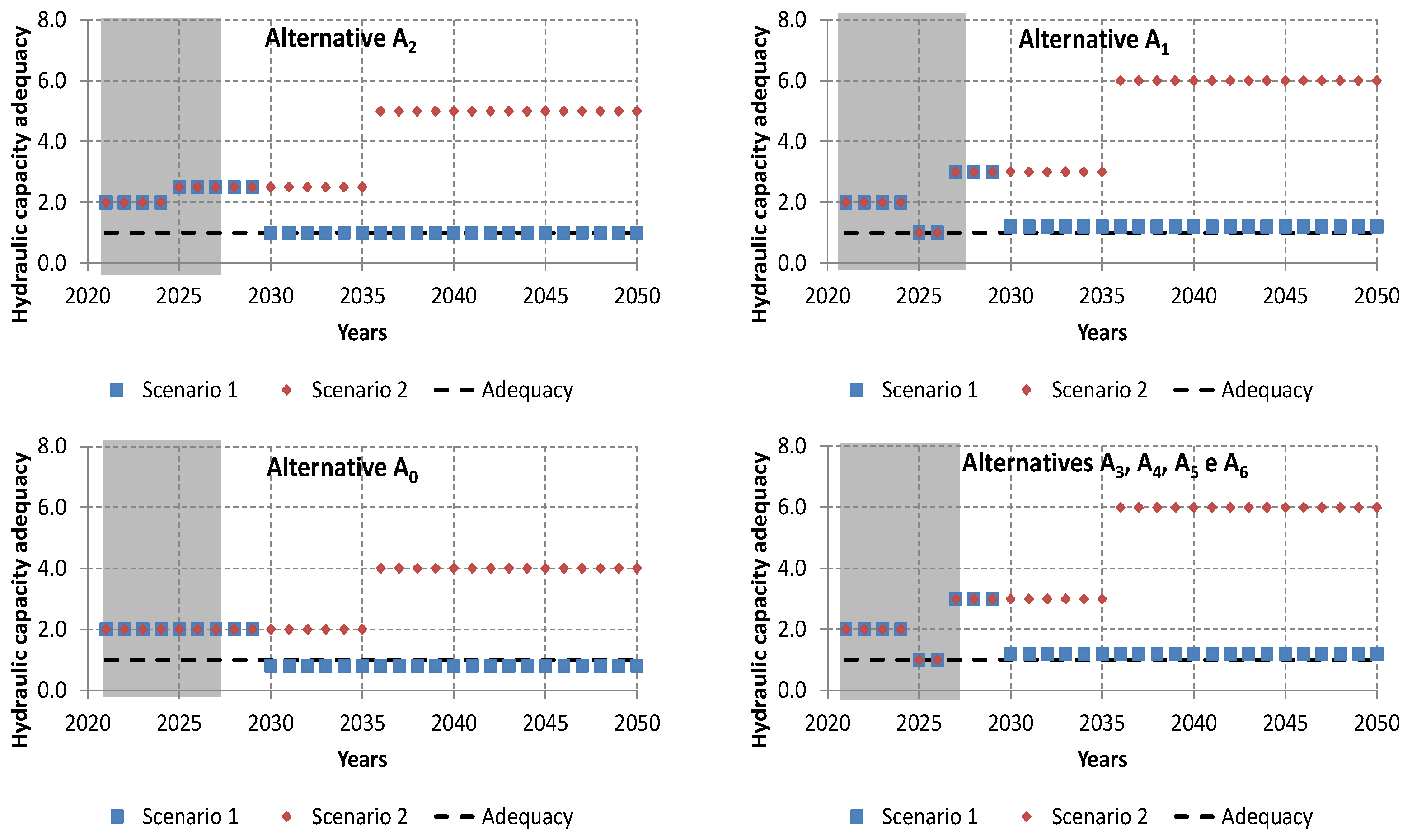
Hydraulic Capacity Adequacy
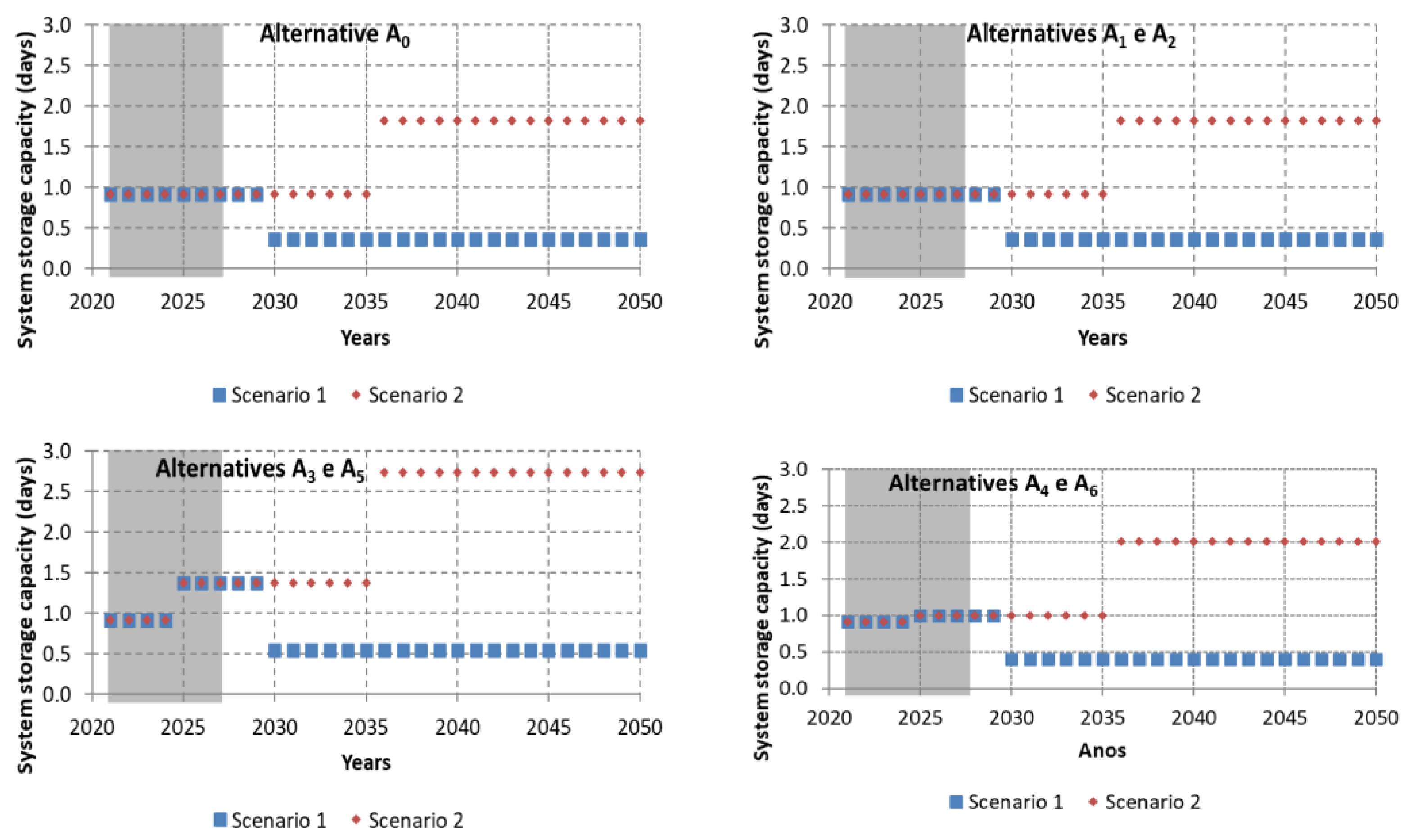
System Storage Capacity
Infrastructure Value Index
Real Water Losses
Structural Condition
Probability of Service Disruption
Risk of Pipe Burst
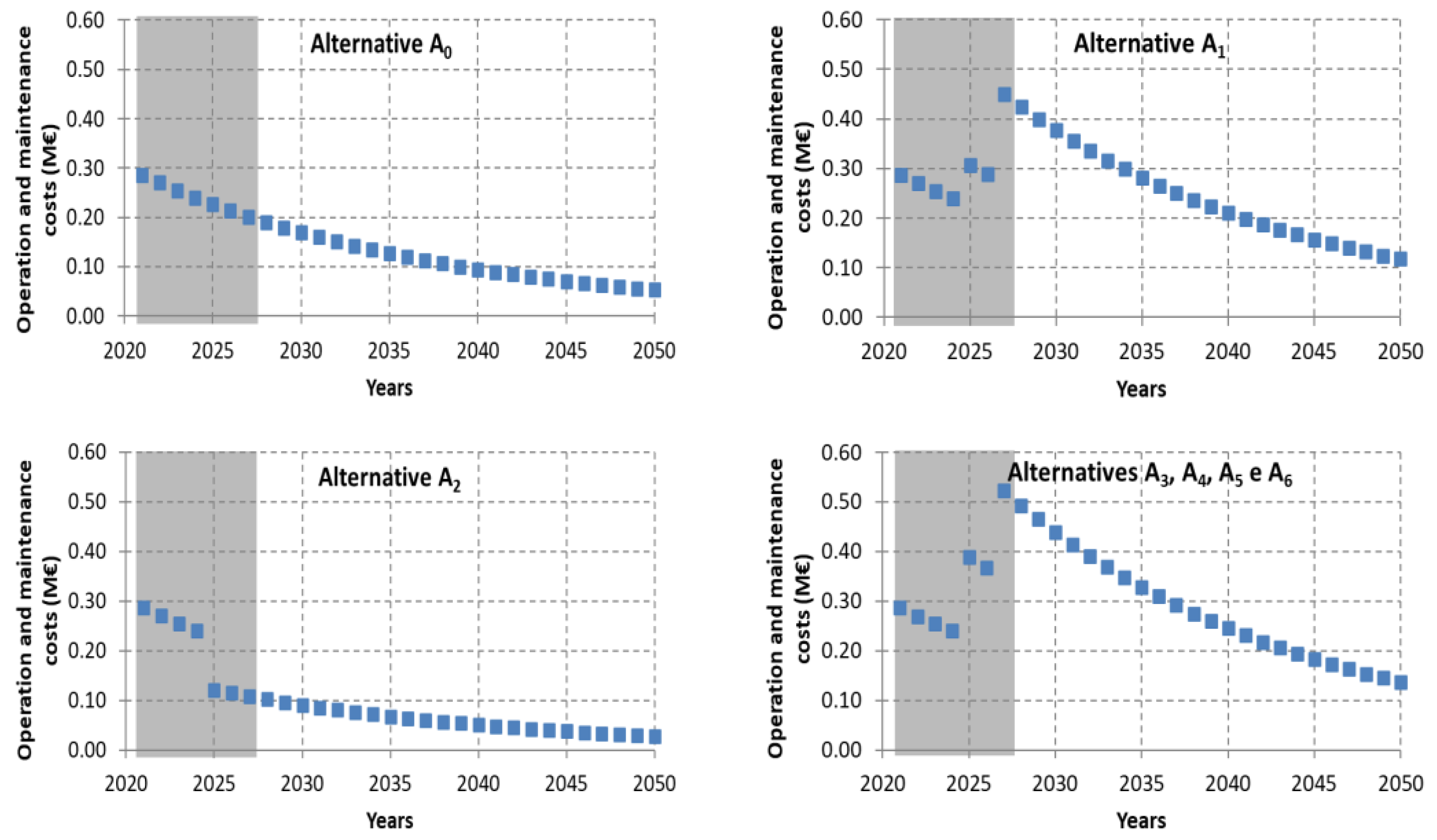
Operation and Maintenance Costs
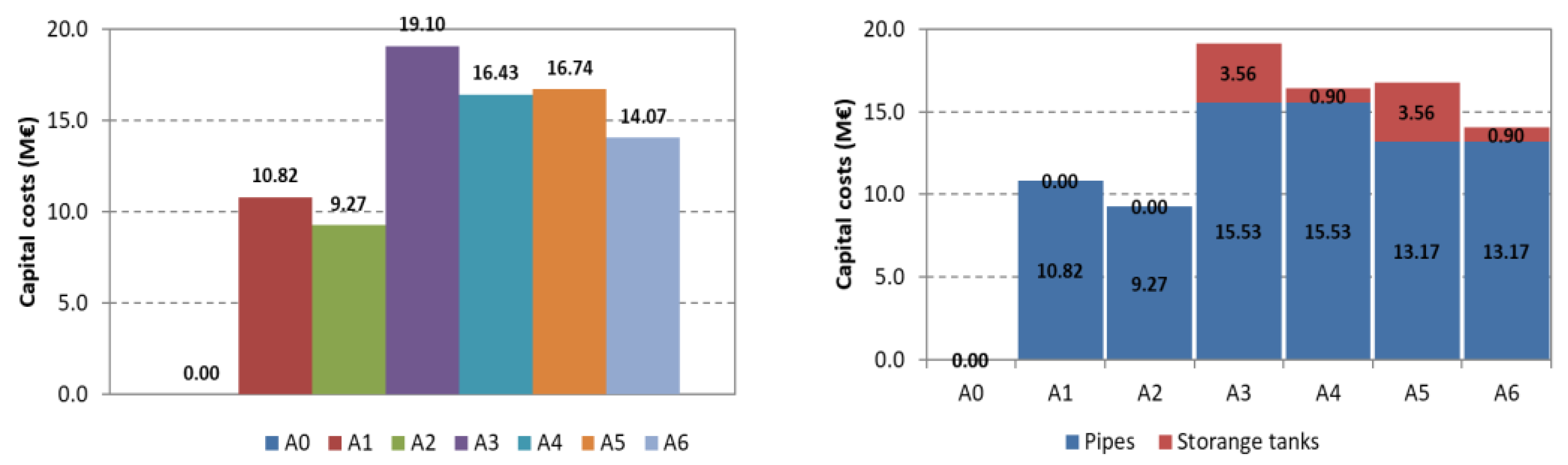
Capital Costs
2.5.3. Selection of the Aggregation Method
The Additive Model
ELECTRE III
Weights
2.5.4. Application of the Aggregation Methods and Elaboration of Final Recommendations
3. Results
3.1. Outcomes of Each Assessment Metric
3.1.1. Hydraulic Capacity Adequacy
3.1.2. System Storage Capacity
3.1.3. Infrastructure Value Index
3.1.4. Real Water Losses
3.1.5. Structural Condition
3.1.6. Probability of Service Disruption
3.1.7. Risk of Pipe Burst
3.1.8. Operation and Maintenance Costs
3.1.9. Capital Costs
3.2. Application of the Aggregation Method and Sensitivity and Robustness Analysis
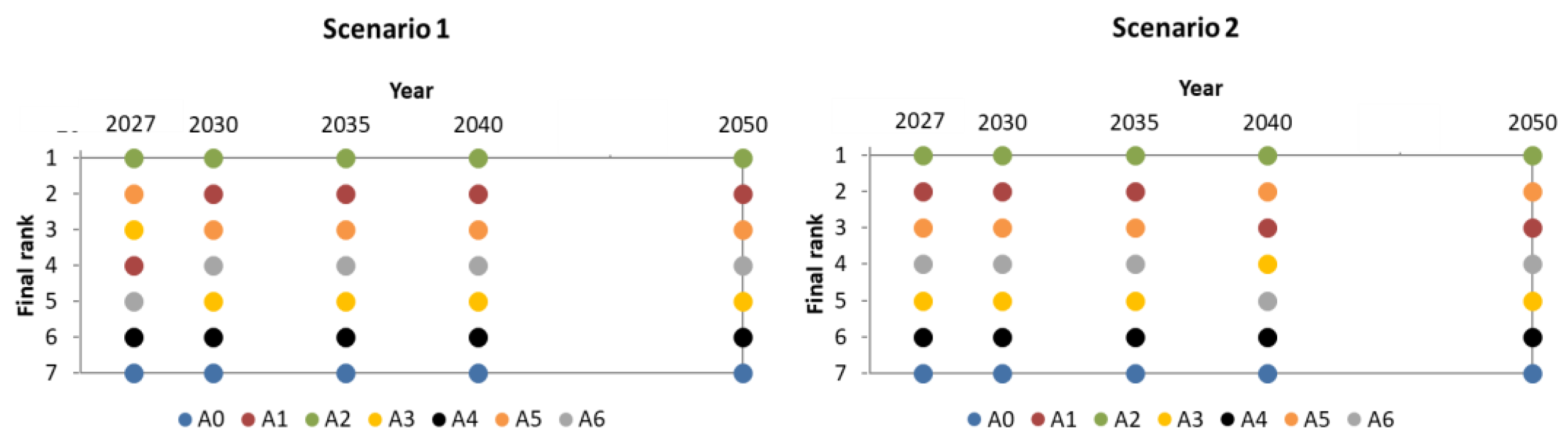
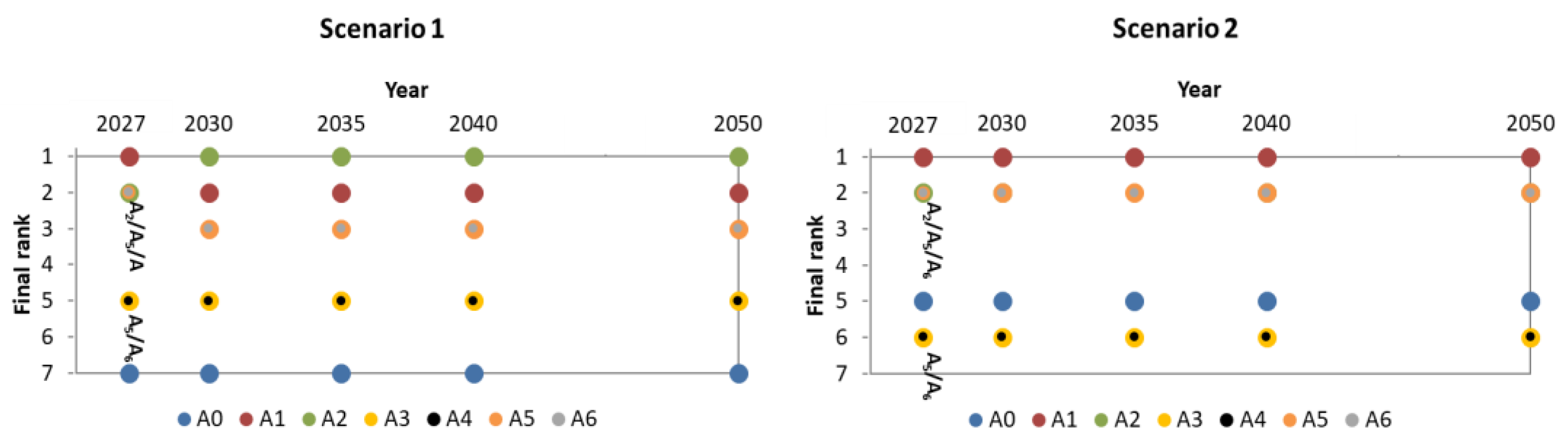
3.2.1. Additive Model
3.2.2. ELECTRE III
4. Conclusions and Further Research
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Carriço, N.; Covas, D.; Almeida, M.d.C. Multi-Criteria Decision Analysis in Urban Water Asset Management. Urban Water J. 2021, 18, 558–569. [Google Scholar] [CrossRef]
- Carriço, N.J.G.; Covas, D.I.C.; Almeida, M.C.; Leitão, J.P.; Alegre, H. Prioritization of Rehabilitation Interventions for Urban Water Assets Using Multiple Criteria Decision-Aid. Water Sci. Technol. 2012, 66, 1007–1014. [Google Scholar] [CrossRef]
- Cardoso, M.A.; Poças, A.; Silva, M.S.; Ribeiro, R.; Almeida, M.C.; Brito, R.S.; Coelho, S.T.; Alegre, H. Innovation Results of IAM Planning in Urban Water Services. Water Sci. Technol. 2016, 74, 1518–1526. [Google Scholar] [CrossRef][Green Version]
- Cardoso, M.A.; Brito, R.; Ribeiro, R.; Alegre, H. Infrastructure Asset Management—Maturity Assessment of Water Utilities Based on International Standards ISO 55000. In Proceedings of the LESAM 2017—Leading Edge Conference for Strategic Asset Management, Trondheim, Norway, 20–22 June 2017. [Google Scholar]
- Alegre, H.; Almeida, M.C.; Covas, D.I.C.; Cardoso, M.A.; Coelho, S.T. Integrated Approach for Infrastructure Asset Management of Urban Water Systems. In Proceedings of the LESAM 2011–Leading Edge Conference for Strategic Asset Management, Mülheim an der Ruhr, Germany, 27–30 September 2011. [Google Scholar]
- Rogers, C.D.F.; Hao, T.; Costello, S.B.; Burrow, M.P.N.; Metje, N.; Chapman, D.N.; Parker, J.; Armitage, R.J.; Anspach, J.H.; Muggleton, J.M.; et al. Condition Assessment of the Surface and Buried Infrastructure—A Proposal for Integration. Tunn. Undergr. Space Technol. 2012, 28, 202–211. [Google Scholar] [CrossRef]
- AWWA. Condition Assessment of Water Mains—Manual M77; American Water Works Association: Denver, CO, USA, 2019; ISBN 978-1-61300-503-3. [Google Scholar]
- St. Clair, A.M.; Sinha, S. State-of-the-Technology Review on Water Pipe Condition, Deterioration and Failure Rate Prediction Models! Urban Water J. 2012, 9, 85–112. [Google Scholar] [CrossRef]
- Liu, Z.; Kleiner, Y. State of the Art Review of Inspection Technologies for Condition Assessment of Water Pipes. Measurement 2013, 46, 1–15. [Google Scholar] [CrossRef]
- Dawood, T.; Elwakil, E.; Novoa, H.M.; Delgado, J.F.G. Artificial Intelligence for the Modeling of Water Pipes Deterioration Mechanisms. Autom. Constr. 2020, 120, 103398. [Google Scholar] [CrossRef]
- Fitchett, J.C.; Karadimitriou, K.; West, Z.; Hughes, D.M. Machine Learning for Pipe Condition Assessments. J. Am. Water Work. Assoc. 2020, 112, 50–55. [Google Scholar] [CrossRef]
- Scholten, L.; Scheidegger, A.; Reichert, P.; Mauer, M.; Lienert, J. Strategic Rehabilitation Planning of Piped Water Networks Using Multi-Criteria Decision Analysis. Water Res. 2014, 49, 124–143. [Google Scholar] [CrossRef] [PubMed]
- Bouyssou, D.; Marchant, T.; Pirlot, M.; Tsoukiàs, A.; Vincke, P. Evaluation and Decision Models with Multiple Criteria: Stepping Stones for the Analyst; Springer Science + Business Media, Inc.: Boston, MA, USA, 2006; ISBN 978-0-387-31098-5. [Google Scholar]
- Alegre, H.; Vitorino, D.; Coelho, S. Infrastructure Value Index: A Powerful Modelling Tool for Combined Long-Term Planning of Linear and Vertical Assets; Elsevier Ltd.: Amsterdam, The Netherlands, 2014; Volume 89, pp. 1428–1436. [Google Scholar]
- Amaral, R.; Alegre, H.; Matos, J.S. A Service-Oriented Approach to Assessing the Infrastructure Value Index. Water Sci. Technol. 2016, 74, 542–548. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Cabral, M.; Loureiro, D.; Covas, D. Using Economic Asset Valuation to Meet Rehabilitation Priority Needs in the Water Sector. Urban Water J. 2019, 16, 205–214. [Google Scholar] [CrossRef]
- Lai, C.-D.; Xie, M. (Eds.) Mean Residual Life—Concepts and Applications in Reliability Analysis. In Stochastic Ageing and Dependence for Reliability; Springer: New York, NY, USA, 2006; pp. 109–138. ISBN 978-0-387-34232-0. [Google Scholar]
- Laven, K.; Lambert, A.O. What Do We Know about Real Losses on Transmission Mains? In Proceedings of the IWA Water Loss Conference, Manila, Philippines, 22–25 January 2012. [Google Scholar]
- Rajani, B.; Kleiner, Y. Comprehensive Review of Structural Deterioration of Water Mains: Physically Based Models. Urban Water 2001, 3, 151–164. [Google Scholar] [CrossRef]
- Kleiner, Y.; Rajani, B. Water Main Assets: From Deterioration to Renewal. In Proceedings of the AWWA Annual Conference, Anaheim, CA, USA, 15–19 June 2003; pp. 1–12. [Google Scholar]
- IPWEA; NAMS. Condition Assessment and Asset Performance Guidelines; IPWEA: Sydney, Australia, 2012; p. 26. [Google Scholar]
- WRc. Sewerage Rehabiltation Manual, 4th ed.; Water Research Centre: Wiltshire, UK, 2001. [Google Scholar]
- IPWEA; NAMS. International Infrastructure Management Manual, 5th ed.; IPWEA: Sydney, Australia; NAMS Group: Wellington, New Zealand, 2015; ISBN 978-0-473-10685-0. [Google Scholar]
- Liu, Z.; Kleiner, Y.; Rajani, B. Condition Assessment Technologies for Water Transmission and Distribution Systems; U.S. Environmental Protection Agency (EPA): Cincinnati, OH, USA, 2012.
- Shamir, U.; Howard, C.D.D. An Analytic Approach to Scheduling Pipe Replacement. J. AWWA 1979, 71, 248–258. [Google Scholar] [CrossRef]
- Al-Barqawi, H.; Zayed, T. Condition Rating Model for Underground Infrastructure Sustainable Water Mains. J. Perform. Constr. Facil. 2006, 20, 126–135. [Google Scholar] [CrossRef]
- Assis, R. Apoio à Decisão Em Manutenção Na Gestão de Activos Físicos; Lidel—edições técnicas, Lda.: Lisboa, Portugal, 2010; ISBN 978-972-757-605-0. [Google Scholar]
- Almeida, A.B. Incertezas e Riscos: Conceptualização Operacional; Água, Ciência e Sociedade; APRH, Ed.; Esfera do Caos: Lisboa, Portugal, 2011; ISBN 978-989-680-044-4. [Google Scholar]
- ERSAR. Water and Waste Services Quality Assessment Guide: 2nd Generation of the Assessment System; ERSAR: Lisbon, Portugal, 2017; ISBN 978-989-8360-11-. [Google Scholar]
- Aven, T. What is a Risk Analysis? In Risk Analysis; John Wiley & Sons, Ltd.: Hoboken, NJ, USA, 2008; pp. 3–15. ISBN 978-0-470-69443-5. [Google Scholar]
- Aven, T.; Kørte, J. On the Use of Risk and Decision Analysis to Support Decision-Making. Reliab. Eng. Syst. Saf. 2003, 79, 289–299. [Google Scholar] [CrossRef]
- Mays, L.W. Urban Water Supply Handbook; McGraw-Hill Education: New York, NY, USA, 2002; ISBN 978-0-07-137160-5. [Google Scholar]
- Marchionni, V.; Cabral, M.; Amado, C.; Covas, D. Estimating Water Supply Infrastructure Cost Using Regression Techniques. J. Water Resour. Plan. Manag. 2016, 142, 04016003. [Google Scholar] [CrossRef]
- Podvezko, V. The Comparative Analysis of MCDA Methods SAW and COPRAS. Eng. Econ. 2011, 22, 134–146. [Google Scholar] [CrossRef]
- de Almeida, A.T.; Cavalcante, C.A.V.; Alencar, M.H.; Ferreira, R.J.P.; de Almeida-Filho, A.T.; Garcez, T.V. Multicriteria and Multiobjective Models for Risk, Reliability and Maintenance Decision Analysis, 1st ed.; Zhu, J., Ed.; Springer International Publishing: Cham, Switzerland, 2015; Volume 231, ISBN 978-3-319-17968-1. [Google Scholar]
- Figueira, J.; Greco, S.; Roy, B.; Slowinski, R.; Pardalos, P.M.; Hearn, D. ELECTRE Methods: Main Features and Recent Developments. In Handbook of Multicriteria Analysis; Zopounidis, C., Pardalos, P.M., Eds.; Springer: Berlin/Heidelberg, Germany, 2010; pp. 51–89. ISBN 978-3-540-92828-7. [Google Scholar]
- Almeida-Dias, J.; Figueira, J.R.; Roy, B. The Software ELECTRE III-IV: Methodology and User Manual (Version 3.x); Lamsade-Universite Paris Dauphine: Paris, France, 2006. [Google Scholar]
- Figueira, J.; Mousseau, V.; Roy, B.; Hillier, F.S. Electre Methods. In Multiple Criteria Decision Analysis: State of the Art Surveys; Figueira, J., Greco, S., Ehrgott, M., Eds.; Springer Science + Business Media, Inc.: Berlin/Heidelberg, Germany, 2005; pp. 133–153. ISBN 0-387-23067-X. [Google Scholar]
- Roy, B. The Outranking Approach and the Foundations of Electre Methods. Theory Decis. 1991, 31, 49–73. [Google Scholar] [CrossRef]
- Roy, B.; Vanderpooten, D. The European School of MCDA: Emergence, Basic Features and Current Works. J. Multi-Criteria Decis. Anal. 1996, 99, 22–38. [Google Scholar] [CrossRef]
- Rogers, M.; Bruen, M. Using ELECTRE III to Choose Route for Dublin Port Motorway. J. Transp. Eng. 2000, 126, 313–323. [Google Scholar] [CrossRef]
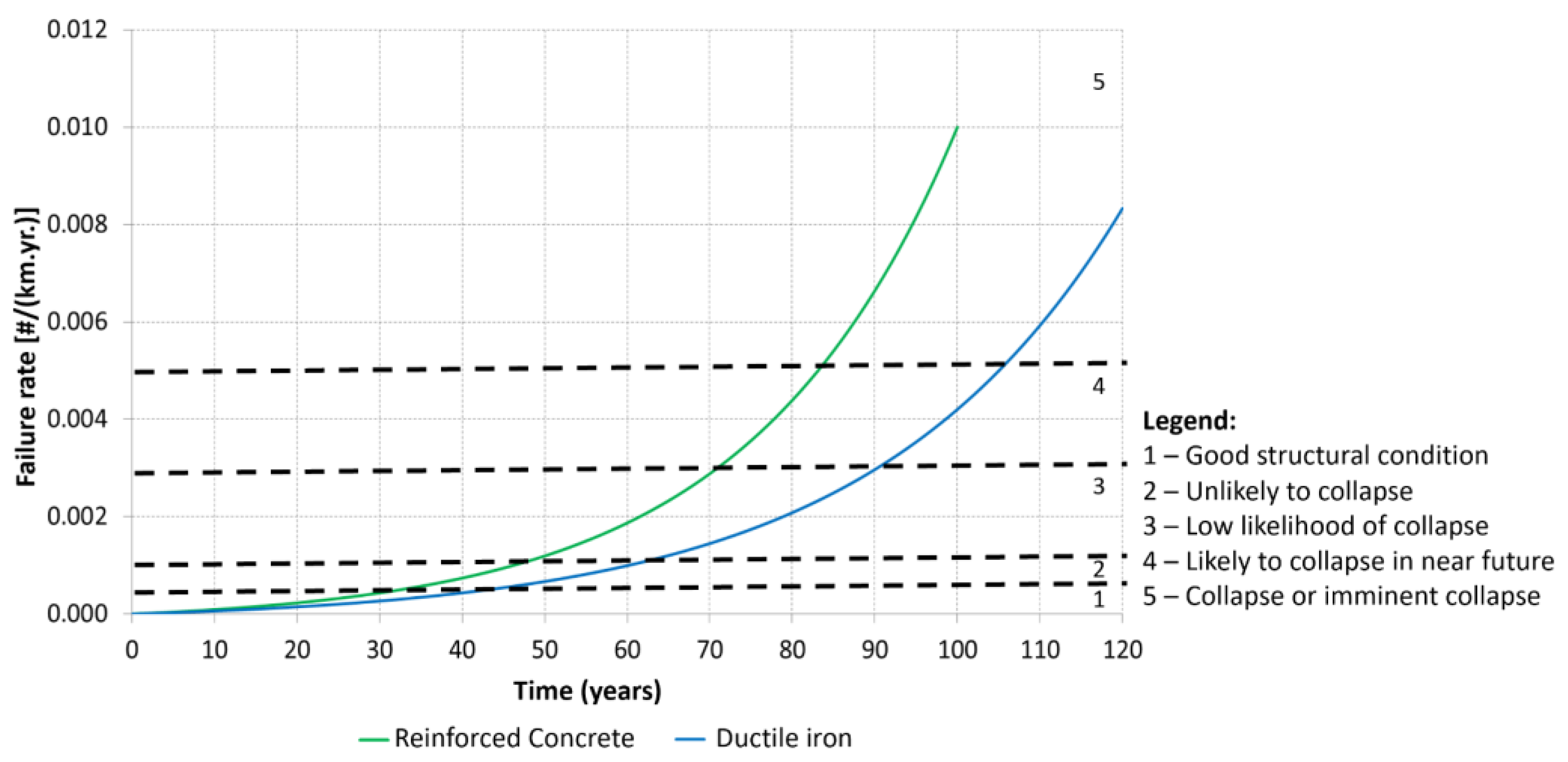
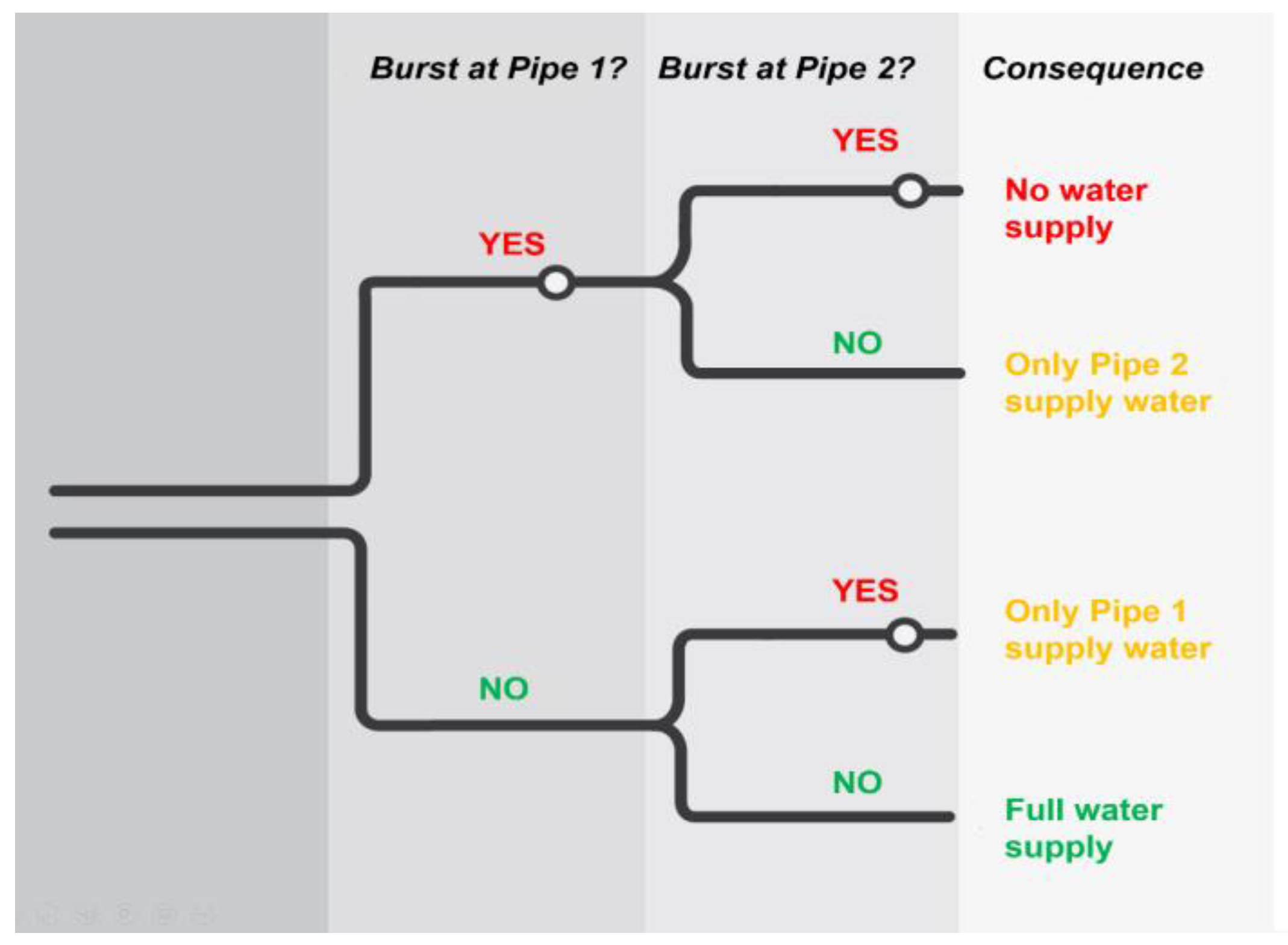
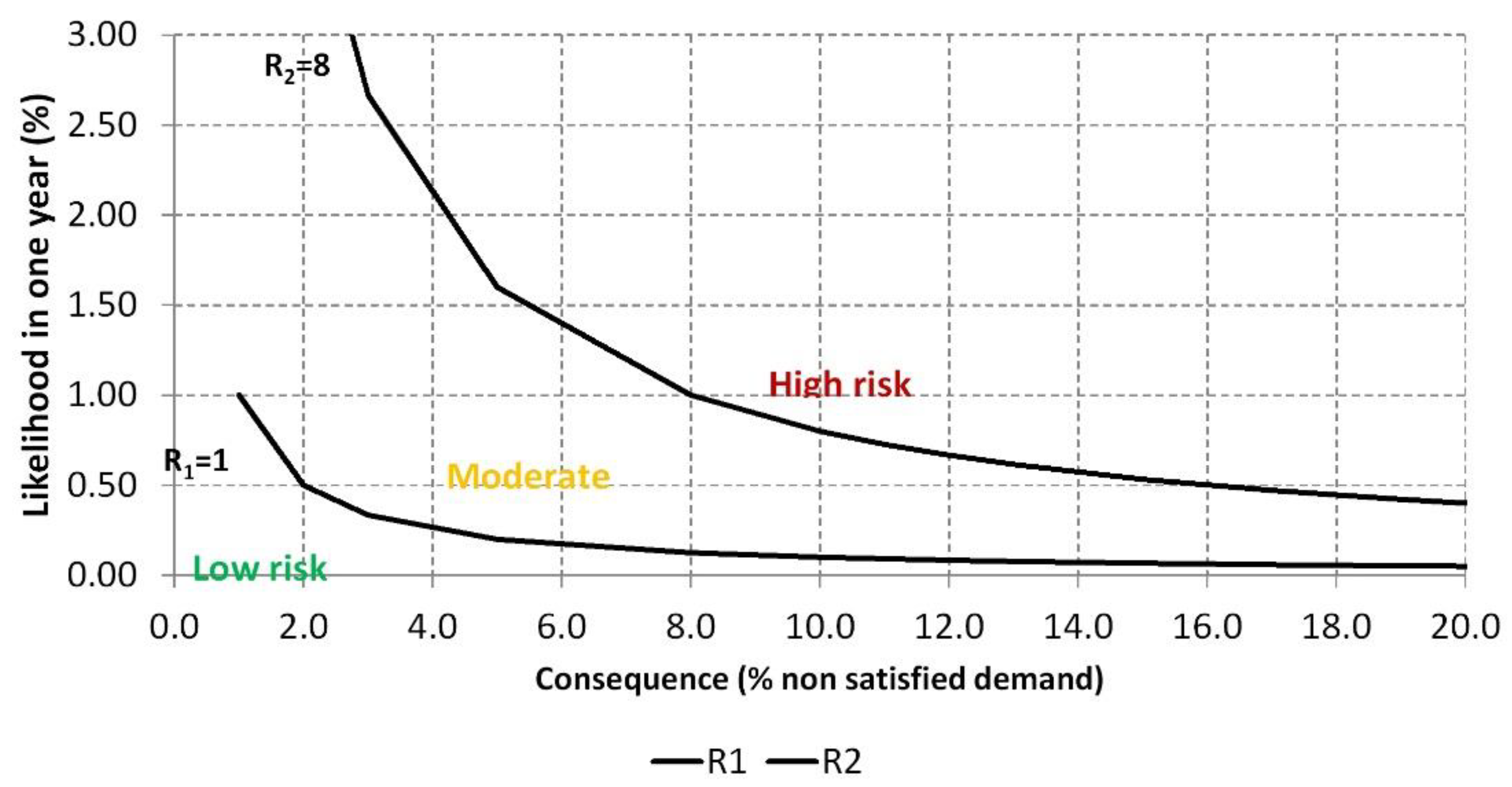
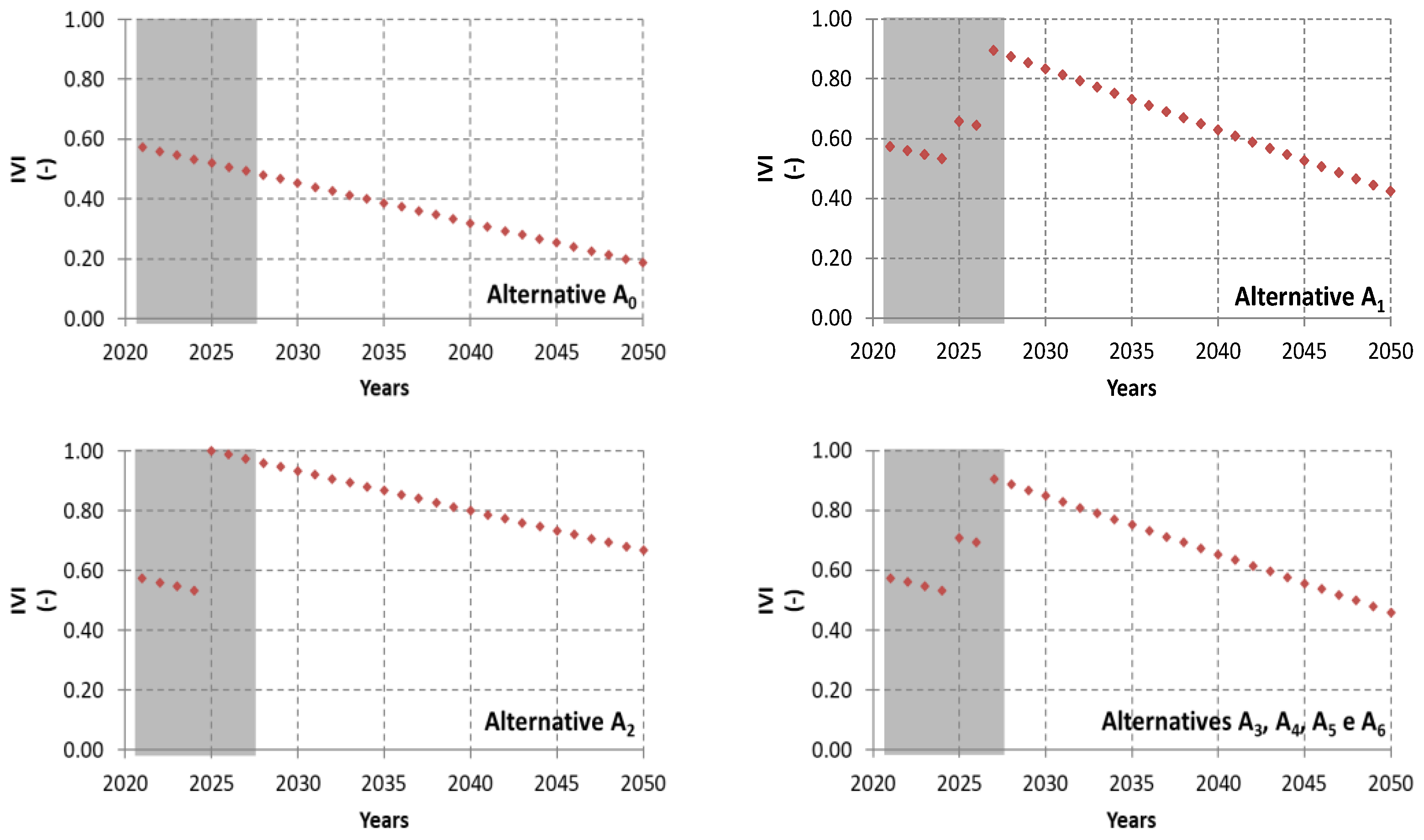
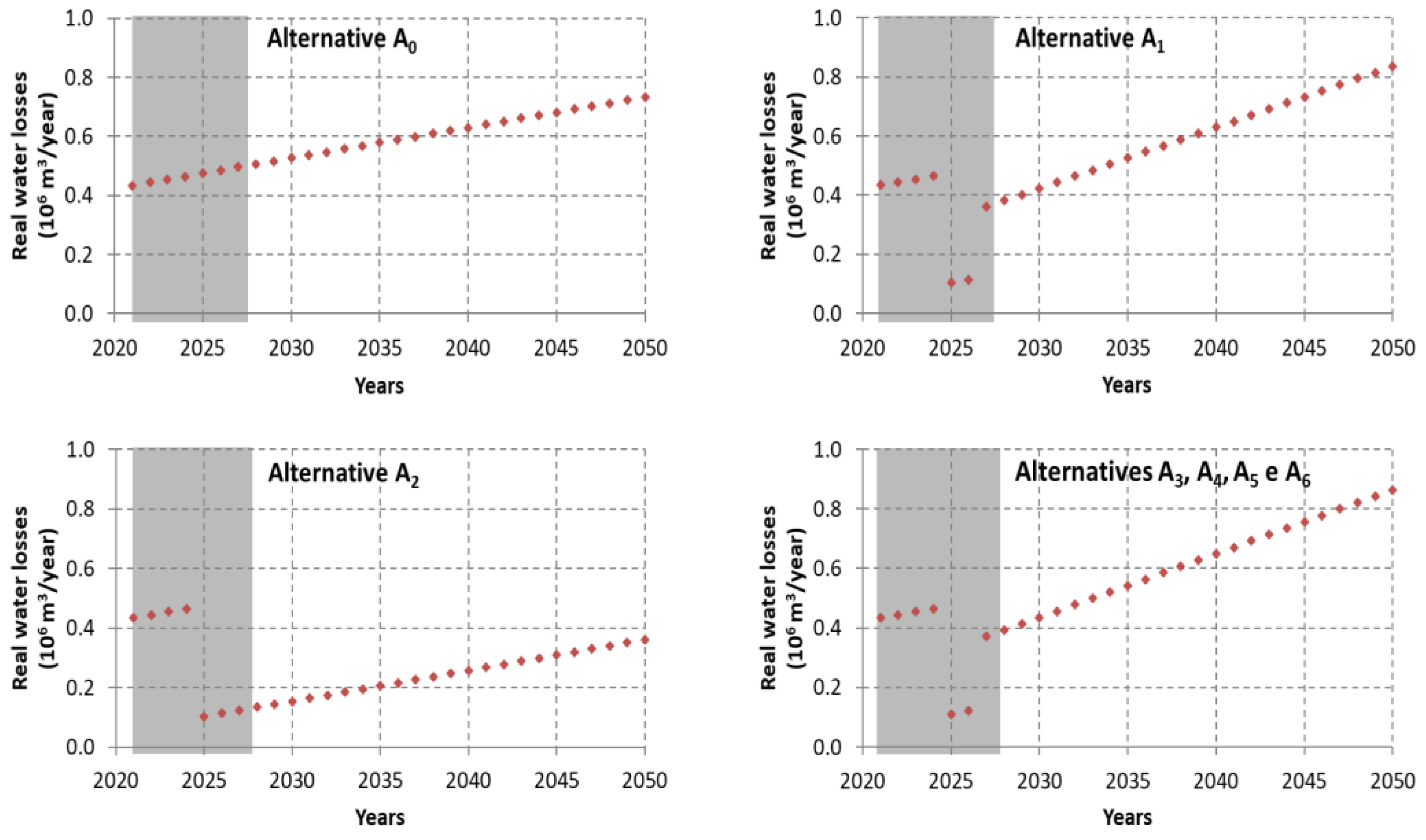
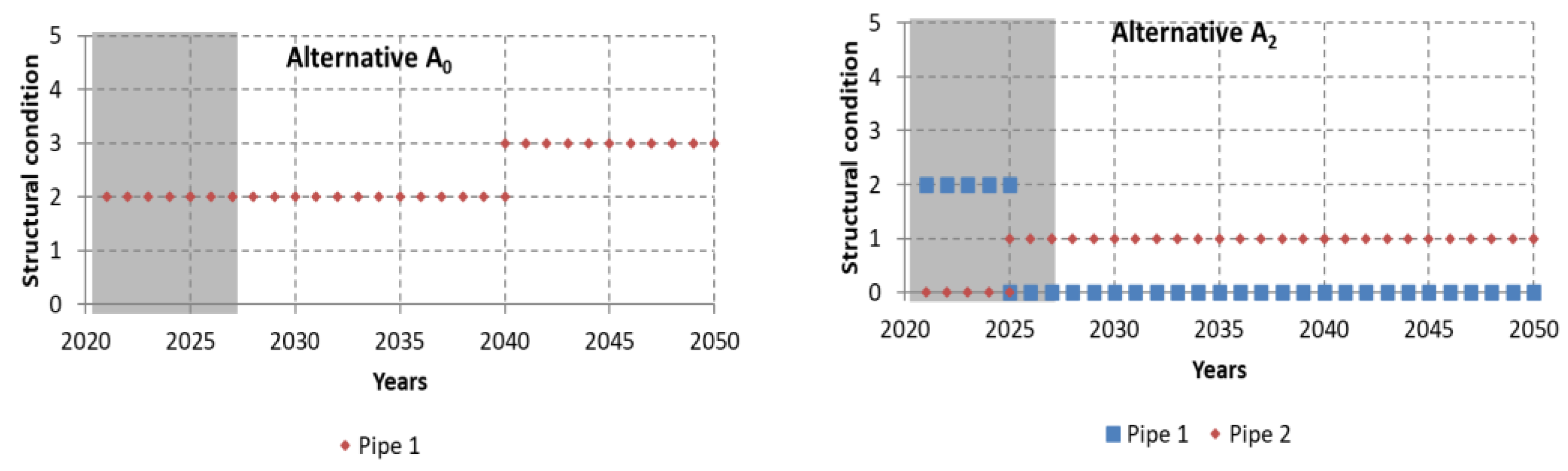
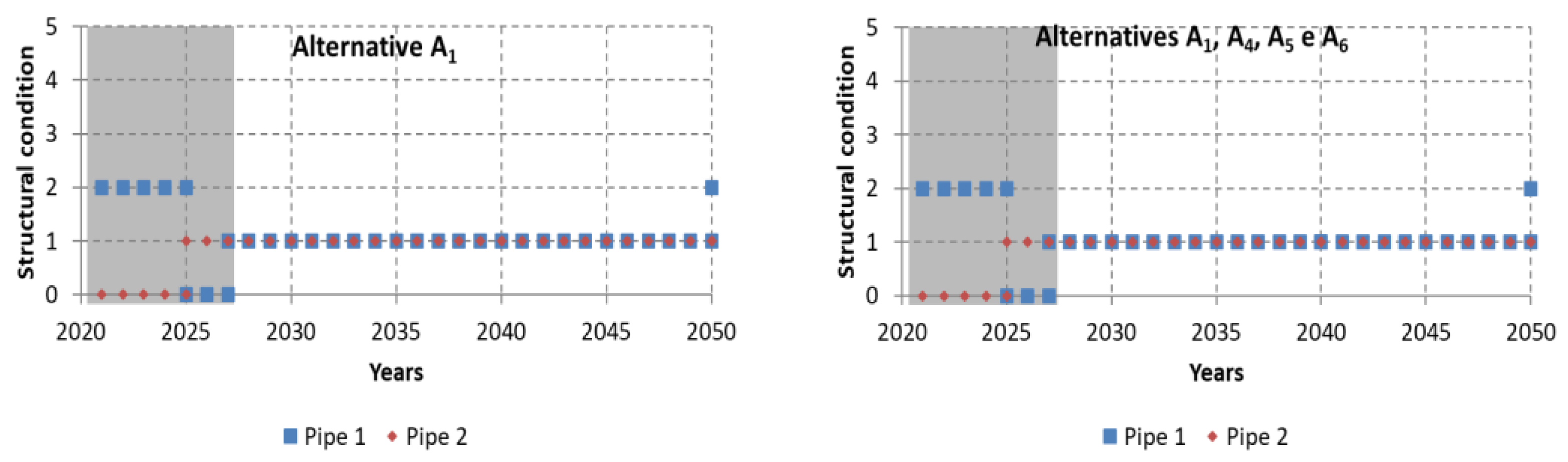



| Structural Condition | Failure Rate (#/(km.year.)) | Description |
|---|---|---|
| 1 | <0.0003 | Good structural condition |
| 2 | 0.0003–0.001 | Unlikely to collapse |
| 3 | 0.001–0.003 | Low likelihood of collapse |
| 4 | 0.003–0.005 | Likely to collapse in near future |
| 5 | >0.005 | Collapse or imminent collapse |
| Structural Condition | Failure Rate (#/(km.year.)) | Probability in a Year (%) |
|---|---|---|
| 1 | <0.0003 | <0.02 |
| 2 | 0.0003–0.001 | 0.02–0.15 |
| 3 | 0.001–0.003 | 0.15–0.35 |
| 4 | 0.003–0.005 | 0.35–0.50 |
| 5 | >0.005 | >0.50 |
| Metric | Good | Satisfactory | Unsatisfactory |
|---|---|---|---|
| Hydraulic capacity adequacy (-) | [1.0; 1.25] | [1.25; 1.5] | [1.5; 2.0] |
| System storage capacity (days) | [2.0; 1.0] | [1.0; 0.5] | [0.5; 0] |
| Infrastructure value index (-) | [1; 0.55] | [0.55; 0.45] | [0.45; 0] |
| Real water losses ((m3/day)/km) | [0.00; 0.20] | [0.20; 0.40] | [0.40; 0.60] |
| Structural condition (-) | [1; 3] | [3; 4] | [4; 5] |
| Probability of service disruption (%) | [0; 0.25] | [0.25; 0.50] | [0.50; 1] |
| Risk of pipe burst (-) | [0; 1] | [1; 8] | [8; 10] |
| Operation and Maintenance costs (M EUR) | [1.0; 3.0] | [3.0; 6.0] | [6.0; 10.0] |
| Capital costs (M EUR) | [0; 7.5] | [7.5; 12.5] | [12.5; 20] |
| Metric | Weight | Preference Direction |
|---|---|---|
| Hydraulic capacity adequacy (-) | 4 | Max (↑) |
| System storage capacity (days) | 4 | Max (↑) |
| Infrastructure value index (-) | 2 | Max (↑) |
| Real water losses ((m3/day)/km) | 4 | Min (↓) |
| Structural condition (-) | 6 | Max (↑) |
| Probability of service disruption (%) | 10 | Min (↓) |
| Risk of pipe burst (-) | 8 | Min (↓) |
| Operation and maintenance costs (M EUR) | 4 | Min (↓) |
| Capital costs (M EUR) | 10 | Min (↓) |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Carriço, N.; Covas, D.; Almeida, M.d.C. Rehabilitation of an Industrial Water Main Using Multicriteria Decision Analysis. Water 2021, 13, 3180. https://doi.org/10.3390/w13223180
Carriço N, Covas D, Almeida MdC. Rehabilitation of an Industrial Water Main Using Multicriteria Decision Analysis. Water. 2021; 13(22):3180. https://doi.org/10.3390/w13223180
Chicago/Turabian StyleCarriço, Nelson, Dídia Covas, and Maria do Céu Almeida. 2021. "Rehabilitation of an Industrial Water Main Using Multicriteria Decision Analysis" Water 13, no. 22: 3180. https://doi.org/10.3390/w13223180
APA StyleCarriço, N., Covas, D., & Almeida, M. d. C. (2021). Rehabilitation of an Industrial Water Main Using Multicriteria Decision Analysis. Water, 13(22), 3180. https://doi.org/10.3390/w13223180







