Hydrodynamics, Hydrochemistry, and Stable Isotope Geochemistry to Assess Temporal Behavior of Seawater Intrusion in the La Yarada Aquifer in the Vicinity of Atacama Desert, Tacna, Peru
Abstract
:1. Introduction
2. Study Area
2.1. Location and Climate
2.2. Hydrogeological Settings
3. Materials and Methods
3.1. Monitoring Network and Sampling
3.2. Analysis and Data Quality
3.3. Statistical Interpretation Procedure
3.4. Groundwater and Seawater Mixing Model
4. Results and Discussion
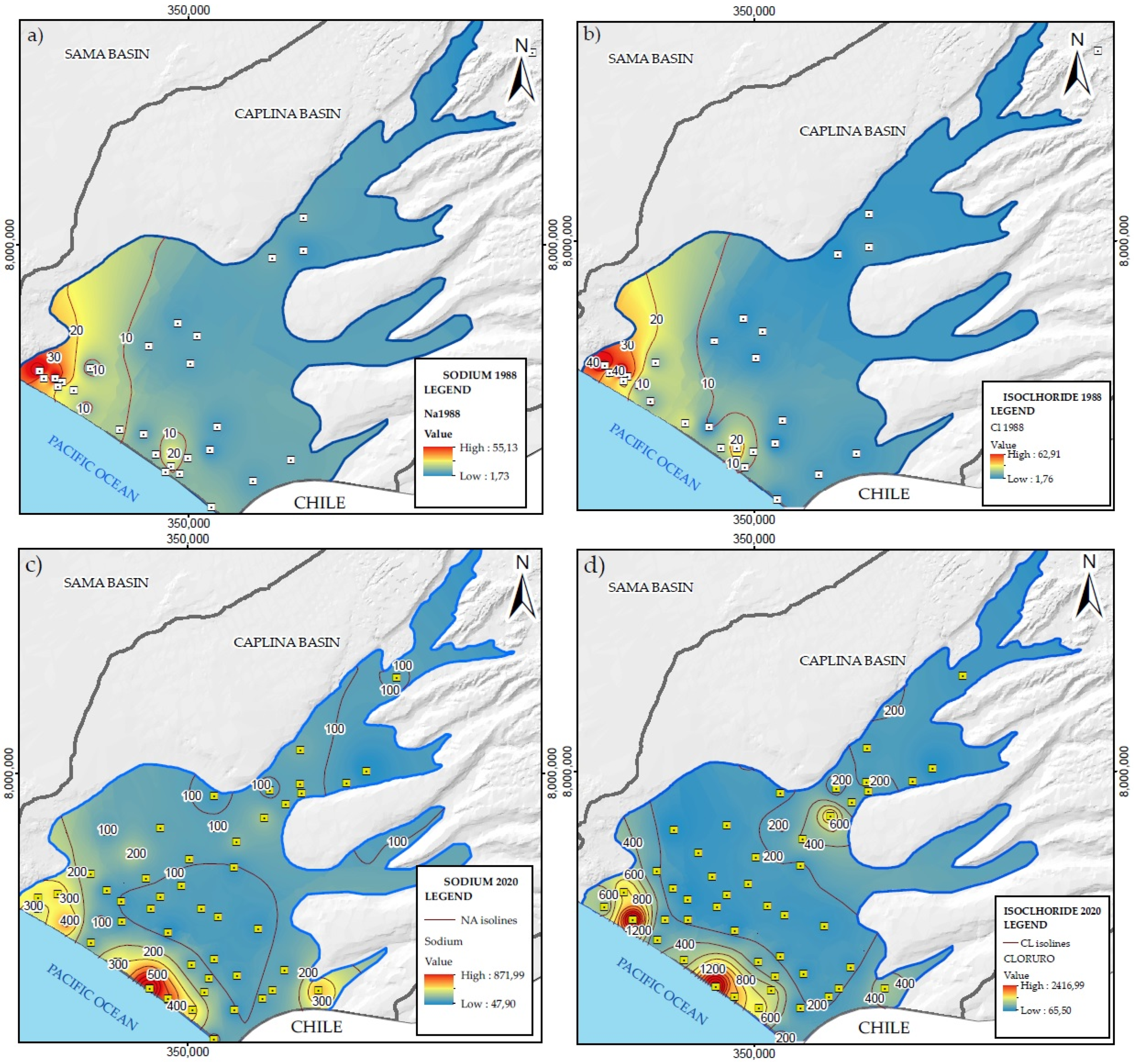
4.1. Spatial and Temporal Univariate Trends in Groundwater Chemistry
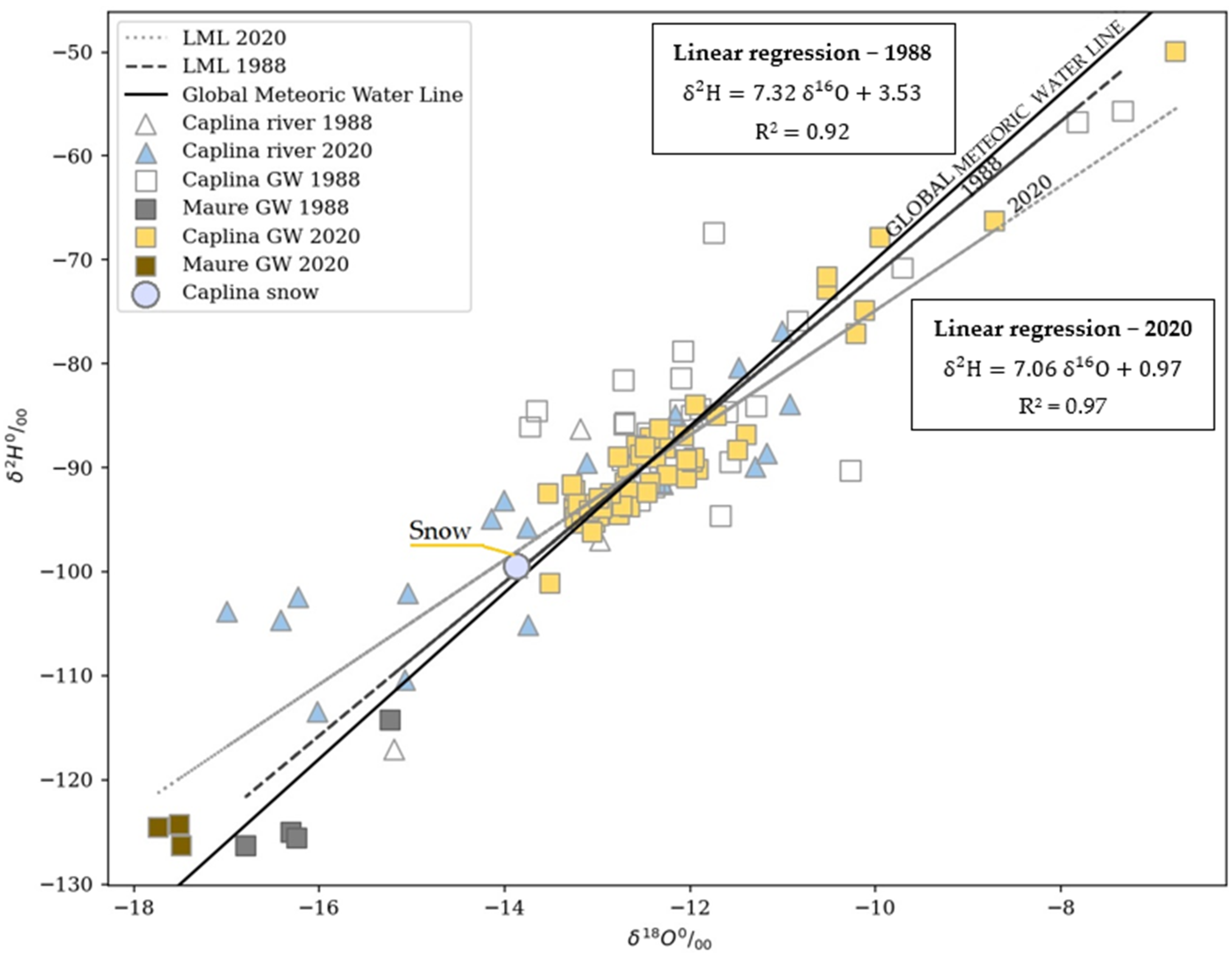
4.1.1. Stable Isotope of Caplina Basin
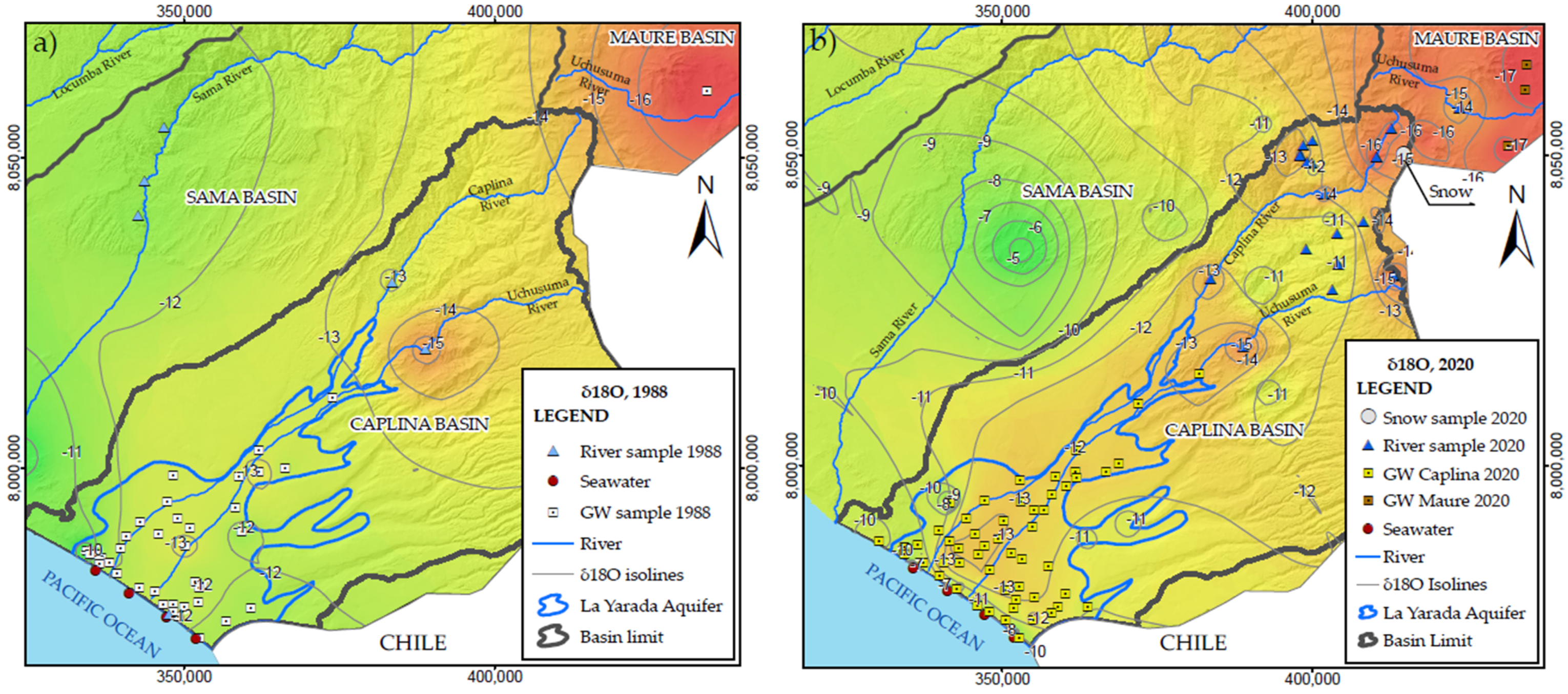
4.1.2. Isotopic Composition Interpolation
4.2. Statistical Correlation
4.3. Multivariate Analysis
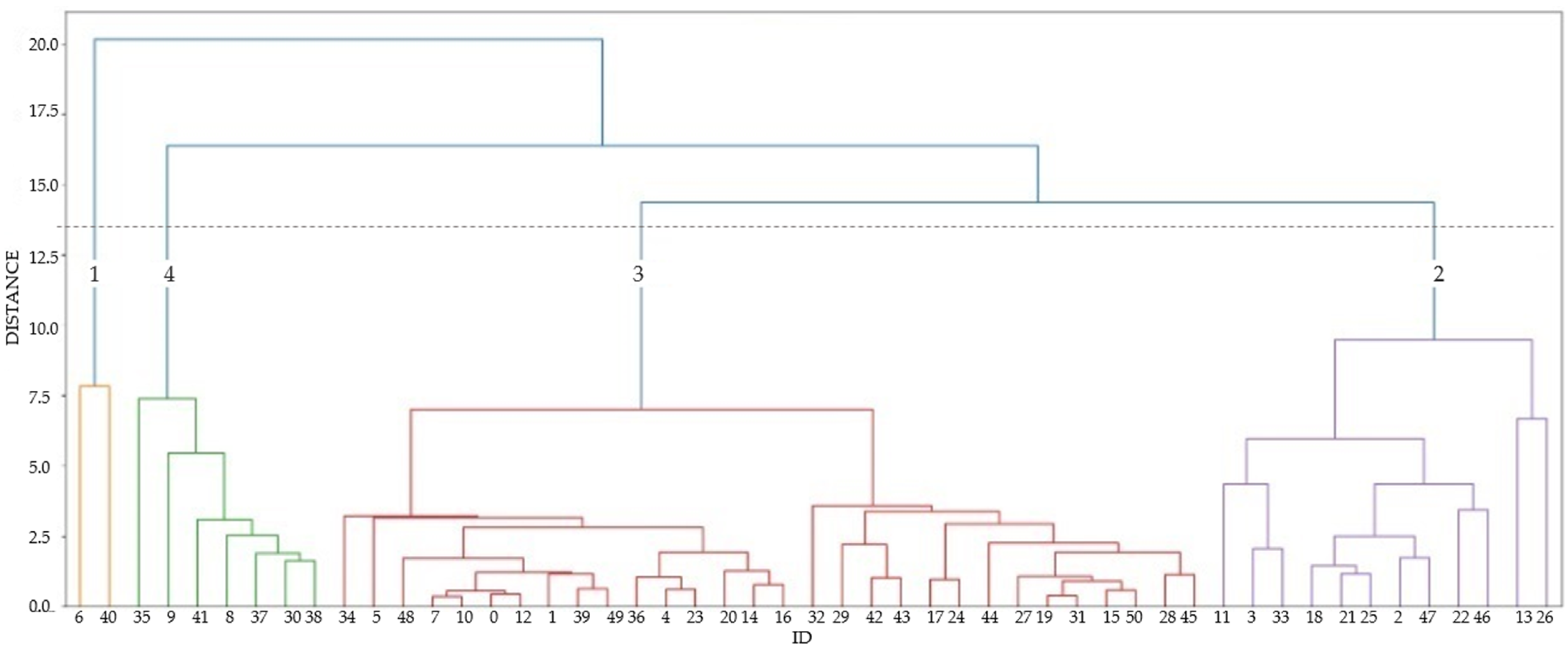
4.3.1. Cluster Analysis
4.3.2. Factorial Analysis
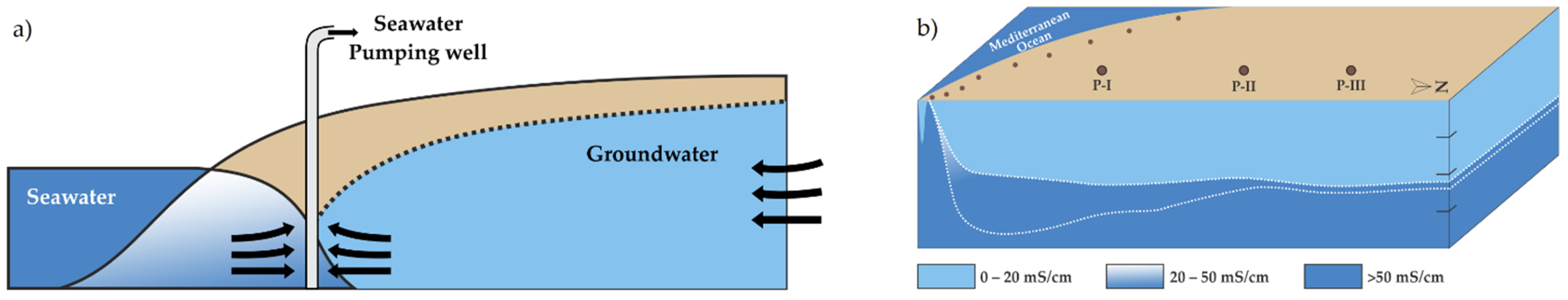
4.4. Conceptual Model for Seawater Intrusion in Groundwater Salinization
4.4.1. Sama Basin Underground Connection
4.4.2. Rock Dissolution
4.5. Seawater Salinization Processes
5. Conclusions
- No change in the chemical and isotopic analysis of groundwater of two samplings, performed in the dry (August 2020) and wet (November 2020), indicate practically no direct influence of precipitation on the La Yarada aquifer characteristics.
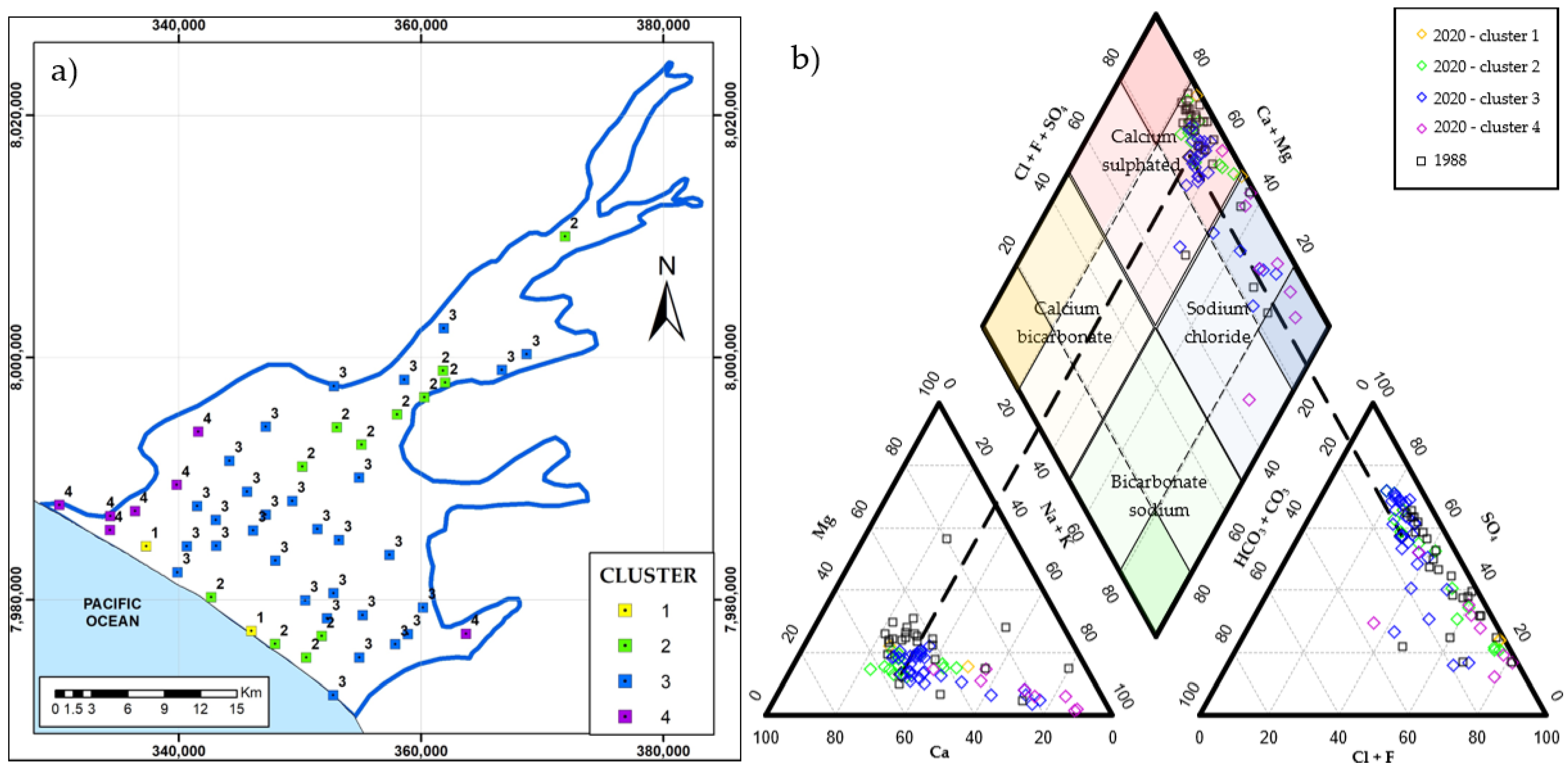
- The hydrochemical and isotopic mixing model supports the formation of a wedge with 20% seawater intrusion. The heterogeneity of piezometric map isolines corroborates the wedge formation associated with the groundwater movement.
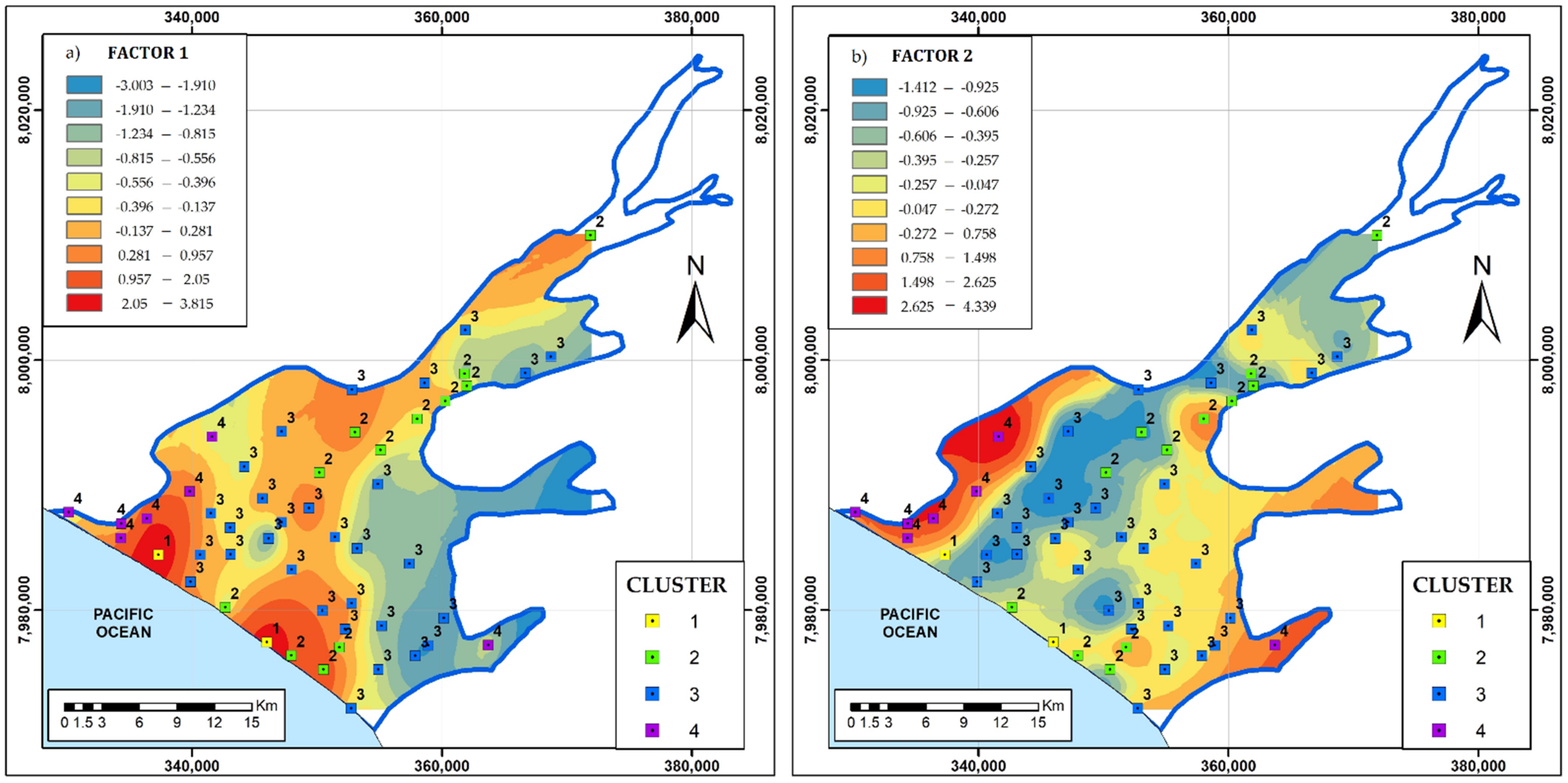
- The factor analysis reduces the chemical and isotopic parameters into the FA1 seawater chemical front and the FA2 seawater isotopic front.
- In the La Yarada aquifer, the seawater and freshwater mixing is dominant at the SW of the aquifer; however, the chemical species migration is along the sea-land boundary. The cluster 4 samples are in the region corresponding to the isotopic mixing process represented by the FA2, while cluster 4 describes the chemical diffusion process represented by the FA2.
- The intrusion of the mixing zone into groundwater behaves differently in the unconfined zone due to the variability of exploitation volumes. In the area proximal to the coastline, the extracted volumes do not exceed 0.5 Hm3. In contrast, more wells than those registered exist to pump 2.5 Hm3 water from the Caplina aquifers nearby the wedge formation.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Datta, P.S. Water Harvesting for Groundwater Management: Issues, Perspectives, Scope, and Challenges; John Wiley & Sons Ltd: Hoboken, NJ, USA, 2019. [Google Scholar]
- Chen, J.; Wu, H.; Qian, H.; Li, X. Challenges and Prospects of Sustainable Groundwater Management in an Agricultural Plain along the Silk Road Economic Belt, North-West China. Int. J. Water Resour. Dev. 2018, 34, 354–368. [Google Scholar] [CrossRef]
- Grosjean, M.; Veit, H. Water Resources in the Arid Mountains of the Atacama Desert (Northern Chile): Past Climate Changes and Modern Conflicts. In Global Change and Mountain Regions; Springer: Dordrecht, The Netherlands, 2005; pp. 93–104. [Google Scholar] [CrossRef]
- Jordan, T.E.; Herrera, L.C.; Godfrey, L.V.; Colucci, S.J.; Gamboa, P.C.; Urrutia, M.J.; González, L.G.; Paul, J.F.; Jordan, T.E.; Herrera, L.C.; et al. Características Isotópicas e Implicaciones Paleoclimáticas Del Evento de Precipitación Extrema de Marzo de 2015 En El Norte de Chile. Andean Geol. 2019, 46, 1–31. [Google Scholar] [CrossRef]
- Naciones Unidas La Agenda 2030 y Los Objetivos de Desarrollo Sostenible: Una Oportunidad Para América Latina y El Caribe; CEPAL: Santigo, Chile, 2018.
- Pino, V.E.; Chávarri, V.E.; Ramos, F.L. Crisis de Gobernanza y Gobernabilidad y Sus Implicancias En El Uso Inadecuado Del Agua Subterránea, Caso Acuífero Costero de La Yarada, Tacna, Perú. Idesia 2018, 36, 77–85. [Google Scholar] [CrossRef] [Green Version]
- Post, V.E.A. Fresh and Saline Groundwater Interaction in Coastal Aquifers: Is Our Technology Ready for the Problems Ahead? Hydrogeol. J. 2005, 13, 120–123. [Google Scholar] [CrossRef]
- Mahlknecht, J.; Merchán, D.; Rosner, M.; Meixner, A.; Ledesma-Ruiz, R. Assessing Seawater Intrusion in an Arid Coastal Aquifer under High Anthropogenic Influence Using Major Constituents, Sr and B Isotopes in Groundwater. Sci. Total Environ. 2017, 587–588, 282–295. [Google Scholar] [CrossRef] [PubMed]
- Cheng, A.H.-D.; Ouazar, D.; Bear, J.; Sorek, S.; Herrera, I. (Eds.) Seawater Intrusion in Coastal Aquifers-Concepts, Methods and Practices; Springer Science & Business Media: Dordrecht, The Netherlands, 1999; Volume XXXIII. [Google Scholar]
- Chai, Y.; Xiao, C.; Li, M.; Liang, X. Hydrogeochemical Characteristics and Groundwater Quality Evaluation Based on Multivariate Statistical Analysis. Water 2020, 12, 2792. [Google Scholar] [CrossRef]
- Güler, C.; Thyne, G.D.; McCray, J.E.; Turner, K.A. Evaluation of Graphical and Multivariate Statistical Methods for Classification of Water Chemistry Data. Hydrogeol. J. 2002, 10, 455–474. [Google Scholar] [CrossRef]
- Menció, A.; Mas-Pla, J. Assessment by Multivariate Analysis of Groundwater-Surface Water Interactions in Urbanized Mediterranean Streams. J. Hydrol. 2008, 352, 355–366. [Google Scholar] [CrossRef]
- Belkhiri, L.; Boudoukha, A.; Mouni, L. A Multivariate Statistical Analysis of Groundwater Chemistry Data. Int. J. Environ. Res. 2011, 5, 537–544. [Google Scholar]
- Villegas, P.; Paredes, V.; Betancur, T.; Ribeiro, L. Assessing the Hydrochemistry of the Urabá Aquifer, Colombia by Principal Component Analysis. J. Geochem. Explor. 2013, 123, 120–129. [Google Scholar] [CrossRef]
- Blake, S.; Henry, T.; Murray, J.; Flood, R.; Muller, M.R.; Jones, A.G.; Rath, V. Compositional Multivariate Statistical Analysis of Thermal Groundwater Provenance: A Hydrogeochemical Case Study from Ireland. Appl. Geochem. 2016, 75, 171–188. [Google Scholar] [CrossRef] [Green Version]
- Werner, A.D.; Simmons, C.T. Impact of Sea-Level Rise on Sea Water Intrusion in Coastal Aquifers. Ground Water 2009, 47, 197–204. [Google Scholar] [CrossRef]
- Binda, G.; Pozzi, A.; Livio, F. An Integrated Interdisciplinary Approach to Evaluate Potentially Toxic Element Sources in a Mountainous Watershed. Environ. Geochem. Health 2020, 42, 1255–1272. [Google Scholar] [CrossRef] [PubMed]
- Gabrielli, P.; Cozzi, G.; Torcini, S.; Cescon, P.; Barbante, C. Trace Elements in Winter Snow of the Dolomites (Italy): A Statistical Study of Natural and Anthropogenic Contributions. Chemosphere 2008, 72, 1504–1509. [Google Scholar] [CrossRef] [PubMed]
- Ritter, B.; Wennrich, V.; Medialdea, A.; Brill, D.; King, G.; Schneiderwind, S.; Niemann, K.; Fernández-Galego, E.; Diederich, J.; Rolf, C.; et al. Climatic Fluctuations in the Hyperarid Core of the Atacama Desert during the Past 215 Ka. Sci. Rep. 2019, 9, 1–13. [Google Scholar] [CrossRef] [Green Version]
- Pino, V.E.; Tacora, P.; Steenken, A.; Alfaro, L.; Valle, A.; Chavarri, E.; Ascencios Templo, D.R.; Mejía, J. Efecto de Las Características Ambientales y Geológicas Sobre La Calidad Del Agua En La Cuenca Del Río Caplina, Tacna, Perú. Tecnología y Ciencias del Agua 2017, 8, 77–99. [Google Scholar] [CrossRef]
- Pino, V.E.; Ramos, F.L.; Mejía, M.J.; Chávarri, V.E.; Ascensios, T.D. Medidas de Mitigación Para El Acuífero Costero La Yarada, Un Sistema Sobreexplotado En Zonas Áridas. Idesia 2020, 38, 21–31. [Google Scholar] [CrossRef]
- Ahmed, I.; Umar, R. Groundwater Flow Modelling of Yamuna-Krishni Interstream, a Part of Central Ganga Plain Uttar Pradesh. J. Earth Syst. Sci. 2009, 118, 507–523. [Google Scholar] [CrossRef]
- Pino, E.; Ramos, L.; Avalos, O.; Tacora, P.; Chávarri, E.; Angulo, O.; Ascensios, D.; Mejía, J. Factors Affecting Depletion and Pollution by Marine Intrusion in the La Yarada’s Coastal Aquifer, Tacna, Peru. Tecnol. Y Cienc. Del Agua 2019, 10, 177–213. [Google Scholar] [CrossRef]
- ANA. Autoridad Nacional del Agua Caracterización Hidrogeológica Del Acuífero Del Valle Del Caplina-La Yarada; ANA: Lima, Perú, 2009. [Google Scholar]
- ANA. Autoridad Nacional del Agua Caracterización Hidrogeoquímica Del Acuífero Caplina; ANA: Lima, Perú, 2010. [Google Scholar]
- Narvaez-Montoya, C.; Torres-Martínez, J.A.; Pino-Vargas, E.; Cabrera-Olivera, F.; Loge, F.J.; Mahlknecht, J. Predicting Adverse Scenarios for a Transboundary Coastal Aquifer System in the Atacama Desert (Peru/Chile). Sci. Total. Environ. 2022, 806, 150386. [Google Scholar] [CrossRef]
- Aranyossy, J. Estudio Isótoico Del Aquifero La Yarada (Perú). Informe de Interpretación; Proyecto PER/8/005; PET-INADE: Dakara, Senegal, 1988. [Google Scholar]
- Valenzuela, L.; Ramírez-Hernández, J.; Palomares, R.B. Composición Isotópica Del Agua Subterránea y Su Relación Con La Salinidad En El Valle de San Luis Río Colorado En Sonora, México. Inf. Tecnológica 2013, 24, 57–66. [Google Scholar] [CrossRef]
- Cruz Falcón, A.; Troyo Diéguez, E.; Murillo Jiménez, J.M.; García Hernández, J.L.; Murillo Amador, B. Groundwater Types and Total Dissolved Solids Distribution in the Aquifer of La Paz Baja California Sur, México. Terra Latinoam. 2018, 36, 31–38. [Google Scholar] [CrossRef] [Green Version]
- Henley, R.W.; Truesdell, A.H.; Barton, P.B. Fluid-Mineral Equilibria in Hydrothermal Systems; Society of Economic Geologists, Inc.: Littleton, CO, USA, 1984. [Google Scholar]
- Pool, M.; Carrera, J. Dynamics of Negative Hydraulic Barriers to Prevent Seawater Intrusion. Hydrogeol. J. 2010, 18, 95–105. [Google Scholar] [CrossRef]
- Jorreto, S.; Sola, F.; Vallejos, Á.; Sánchez-Martos, F.; Gisbert, J.; Molina, L.; Rigol, J.P.; Pulido-Bosch, A. Evolución de La Geometría de La Interfase Agua Dulce-Agua de Mar En Un Acuífero Costero Sometido a Un Intenso Bombeo de Agua de Mar. Geogaceta 2017, 62, 87–90. [Google Scholar]
- Pino, E.; Chávarri, E. Evidence of Climate Change in the Hyper-Arid Region of the Southern Coast of Peru, Head of the Atacama Desert. Tecnol. Cienc. Agua 2022, 12. in press. [Google Scholar]
- Craig, H. Isotopic Variations in Meteoric Waters. Science 1961, 133, 1702–1703. [Google Scholar] [CrossRef]
- Arslan, H. Application of Multivariate Statistical Techniques in the Assessment of Groundwater Quality in Seawater Intrusion Area in Bafra Plain, Turkey. Environ. Monit. Assess. 2013, 185, 2439–2452. [Google Scholar] [CrossRef]
- Moujabber, M.E.; Samra, B.B.; Darwish, T.; Atallah, T. Comparison of Different Indicators for Groundwater Contamination by Seawater Intrusion on the Lebanese Coast. Water Resour. Manag. 2006, 20, 161–180. [Google Scholar] [CrossRef]
- Liu, C.W.; Lin, K.H.; Kuo, Y.M. Application of Factor Analysis in the Assessment of Groundwater Quality in a Blackfoot Disease Area in Taiwan. Sci. Total Environ. 2003, 313, 77–89. [Google Scholar] [CrossRef]
- Appelo, C.A.J.; Postma, D. Groundwater Geochemistry. In A Practical Guide to Modelling of Natural and Contaminated Aquatic Systems; Cambridge University Press: Cambridge, UK, 1993; Volume 536, ISBN 978-84-9732-487-8. [Google Scholar]
- Acosta, H.; Alván, A.; Cutipa, M.; Mamani, M.; Rodríguez, J. Geología de los Cuadrángulos de La Yarada, Tacna y Huaylillas, Hojas 37-u, 37-v y 37-x, Escala 1: 50,000–[Boletín A 145]; INGEMMET: Lima, Peru, 2012. [Google Scholar]
- Acosta, H.; Alván, A.; Cutipa, M.; Mamani, M.; Rodríguez, J. Geología, de los Cuadrángulos de Pachía y Palca, hojas 36-v y 36-x, Escala 1: 50,000; Serie A: C.; INGEMMET: Lima, Peru, 2011. [Google Scholar]
- Robinson, W.O.; Edginton, G. Fluorine in soils. Soil Sci. 1946, 61, 341–354. [Google Scholar] [CrossRef]
- Arif, M.; Hussain, I.; Hussain, J.; Sharma, M.K.; Kumar, S.; Bhati, G. GIS-Based Inverse Distance Weighting Spatial Interpolation Technique for Fluoride Distribution in South West Part of Nagaur District, Rajasthan. Cogent Environ. Sci. 2015, 1, 1038944. Available online: http://www.editorialmanager.com/cogentenv (accessed on 4 October 2021). [CrossRef]
- Motevalli, A.; Moradi, H.R.; Javadi, S. A Comprehensive Evaluation of Groundwater Vulnerability to Saltwater Up-Coning and Sea Water Intrusion in a Coastal Aquifer (Case Study: Ghaemshahr-Juybar Aquifer). J. Hydrol. 2018, 557, 753–773. [Google Scholar] [CrossRef]


| Statistics | CE | pH | TDS | Ca2+ | Mg2+ | Na+ | K+ | HCO3− | SO42− | Cl− | NO3− | F− | ||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| μS/cm | mg/L | ‰ | ||||||||||||
| Dry Season (August 2020) | ||||||||||||||
| Mean | 1772.8 | 7.6 | 1248.6 | 164.9 | 34.3 | 161.8 | 17.4 | 87.8 | 410.3 | 362.1 | 3.6 | 0.2 | −12.1 | −88.0 |
| Minimum | 25.5 | 5.8 | 63.5 | 10.7 | 0.8 | 47.9 | 1.5 | 53.4 | 45.4 | 65.5 | 0.02 | 0.1 | −13.5 | −96.2 |
| Maximum | 8190.0 | 8.7 | 5594.0 | 874.0 | 223.0 | 872.0 | 51.7 | 196.0 | 1030.0 | 2417.0 | 20.4 | 0.6 | −6.7 | −49.9 |
| Wet Season (November 2020) | ||||||||||||||
| Mean | 2075.1 | 7.6 | 1381.1 | 185.6 | 35.2 | 166.5 | 15.1 | 86.6 | 394.7 | 369.9 | 4.1 | 0.2 | −12.2 | −86.4 |
| Minimum | 653.0 | 7.0 | 425.0 | 12.1 | 0.9 | 51.8 | 2.8 | 46.2 | 42.8 | 70.2 | 0.6 | 0.1 | −13.8 | −95.8 |
| Maximum | 8290.0 | 8.3 | 5918.0 | 899.6 | 209.1 | 871.0 | 44.3 | 170.0 | 969.0 | 2266.0 | 24.1 | 0.6 | −4.7 | −31.2 |
| EC | pH | TDS | Ca2+ | Mg2+ | Na+ | K+ | HCO3− | SO42− | Cl− | NO3− | F− | |||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| EC | 1.00 | −0.40 | 0.70 | 0.78 | 0.73 | 0.45 | 0.65 | 0.25 | 0.50 | 0.66 | 0.35 | 0.31 | 0.10 | 0.12 |
| pH | −0.40 | 1.00 | −0.52 | −0.62 | −0.55 | −0.29 | −0.30 | −0.47 | −0.52 | −0.47 | −0.51 | 0.22 | 0.46 | 0.48 |
| TDS | 0.70 | −0.52 | 1.00 | 0.92 | 0.93 | 0.86 | 0.84 | 0.19 | 0.71 | 0.98 | 0.43 | 0.27 | 0.03 | 0.08 |
| Ca2+ | 0.78 | −0.62 | 0.92 | 1.00 | 0.97 | 0.60 | 0.76 | 0.24 | 0.72 | 0.88 | 0.48 | 0.14 | −0.11 | −0.09 |
| Mg2+ | 0.73 | −0.55 | 0.93 | 0.97 | 1.00 | 0.66 | 0.82 | 0.14 | 0.77 | 0.89 | 0.33 | 0.11 | −0.13 | −0.07 |
| Na+ | 0.45 | −0.29 | 0.86 | 0.60 | 0.66 | 1.00 | 0.78 | 0.16 | 0.52 | 0.87 | 0.30 | 0.43 | 0.24 | 0.32 |
| K+ | 0.65 | −0.30 | 0.84 | 0.76 | 0.82 | 0.78 | 1.00 | 0.06 | 0.70 | 0.80 | 0.23 | 0.28 | 0.10 | 0.21 |
| HCO3− | 0.25 | −0.47 | 0.19 | 0.24 | 0.14 | 0.16 | 0.06 | 1.00 | 0.28 | 0.13 | 0.53 | 0.03 | −0.06 | −0.13 |
| SO42− | 0.50 | −0.52 | 0.71 | 0.72 | 0.77 | 0.52 | 0.70 | 0.28 | 1.00 | 0.58 | 0.27 | −0.16 | −0.40 | −0.31 |
| Cl− | 0.66 | −0.47 | 0.98 | 0.88 | 0.89 | 0.87 | 0.80 | 0.13 | 0.58 | 1.00 | 0.40 | 0.35 | 0.12 | 0.17 |
| NO3− | 0.35 | −0.51 | 0.43 | 0.48 | 0.33 | 0.30 | 0.23 | 0.53 | 0.27 | 0.40 | 1.00 | 0.15 | −0.02 | −0.11 |
| F− | 0.31 | 0.22 | 0.27 | 0.14 | 0.11 | 0.43 | 0.28 | 0.03 | −0.16 | 0.35 | 0.15 | 1.00 | 0.74 | 0.75 |
| 0.10 | 0.46 | 0.03 | −0.11 | −0.13 | 0.24 | 0.10 | −0.06 | −0.40 | 0.12 | −0.02 | 0.74 | 1.00 | 0.96 | |
| 0.12 | 0.48 | 0.08 | −0.09 | −0.07 | 0.32 | 0.21 | −0.13 | −0.31 | 0.17 | −0.11 | 0.75 | 0.96 | 1.00 |
| Cluster | Relative Abundance | ||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 1 | Cl− | >> | SO42− | >> | Ca2+ | > | Na+ | >> | Mg2+ | > | HCO3− | > | K+ | > | NO3− |
| 2 | Cl− | >> | Na+ | > | SO42− | >> | Ca2+ | > | HCO3− | >> | K+ | > | Mg2+ | > | NO3− |
| 3 | SO42− | >> | Cl− | > | Ca2+ | > | Na+ | > | HCO3− | > | Mg2+ | > | K+ | > | NO3− |
| 4 | SO42− | ≥ | Cl− | >> | Ca2+ | > | Na+ | > | HCO3− | >> | Mg2+ | > | K+ | > | NO3− |
| FA1 | FA2 | FA3 | Communality | |
|---|---|---|---|---|
| EC | 0.676 | 0.124 | 0.282 | 0.551 |
| pH | −0.431 | 0.423 | −0.591 | 0.715 |
| TDS | 0.969 | 0.079 | 0.219 | 0.993 |
| Ca2+ | 0.895 | −0.085 | 0.319 | 0.910 |
| Mg2+ | 0.963 | −0.113 | 0.134 | 0.957 |
| Na+ | 0.766 | 0.301 | 0.116 | 0.690 |
| K+ | 0.896 | 0.135 | −0.013 | 0.821 |
| HCO3− | 0.064 | −0.039 | 0.702 | 0.499 |
| SO42− | 0.736 | −0.358 | 0.165 | 0.696 |
| Cl− | 0.925 | 0.176 | 0.187 | 0.922 |
| NO3− | 0.260 | 0.028 | 0.725 | 0.594 |
| F− | 0.204 | 0.794 | 0.084 | 0.678 |
| δ18O | −0.035 | 0.971 | −0.044 | 0.946 |
| δ2H | 0.062 | 0.964 | −0.178 | 0.964 |
| Var | 6.213 | 2.991 | 1.733 | |
| %Var | 0.444 | 0.214 | 0.124 | |
| Accum | 0.444 | 0.657 | 0.781 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Vera, A.; Pino-Vargas, E.; Verma, M.P.; Chucuya, S.; Chávarri, E.; Canales, M.; Torres-Martínez, J.A.; Mora, A.; Mahlknecht, J. Hydrodynamics, Hydrochemistry, and Stable Isotope Geochemistry to Assess Temporal Behavior of Seawater Intrusion in the La Yarada Aquifer in the Vicinity of Atacama Desert, Tacna, Peru. Water 2021, 13, 3161. https://doi.org/10.3390/w13223161
Vera A, Pino-Vargas E, Verma MP, Chucuya S, Chávarri E, Canales M, Torres-Martínez JA, Mora A, Mahlknecht J. Hydrodynamics, Hydrochemistry, and Stable Isotope Geochemistry to Assess Temporal Behavior of Seawater Intrusion in the La Yarada Aquifer in the Vicinity of Atacama Desert, Tacna, Peru. Water. 2021; 13(22):3161. https://doi.org/10.3390/w13223161
Chicago/Turabian StyleVera, Alissa, Edwin Pino-Vargas, Mahendra P. Verma, Samuel Chucuya, Eduardo Chávarri, Miguel Canales, Juan Antonio Torres-Martínez, Abrahan Mora, and Jürgen Mahlknecht. 2021. "Hydrodynamics, Hydrochemistry, and Stable Isotope Geochemistry to Assess Temporal Behavior of Seawater Intrusion in the La Yarada Aquifer in the Vicinity of Atacama Desert, Tacna, Peru" Water 13, no. 22: 3161. https://doi.org/10.3390/w13223161
APA StyleVera, A., Pino-Vargas, E., Verma, M. P., Chucuya, S., Chávarri, E., Canales, M., Torres-Martínez, J. A., Mora, A., & Mahlknecht, J. (2021). Hydrodynamics, Hydrochemistry, and Stable Isotope Geochemistry to Assess Temporal Behavior of Seawater Intrusion in the La Yarada Aquifer in the Vicinity of Atacama Desert, Tacna, Peru. Water, 13(22), 3161. https://doi.org/10.3390/w13223161








