Optimal Sensor Placement in Hydraulic Conduit Networks: A State-Space Approach
Abstract
:1. Introduction
2. Materials and Methods
3. Results and Discussion
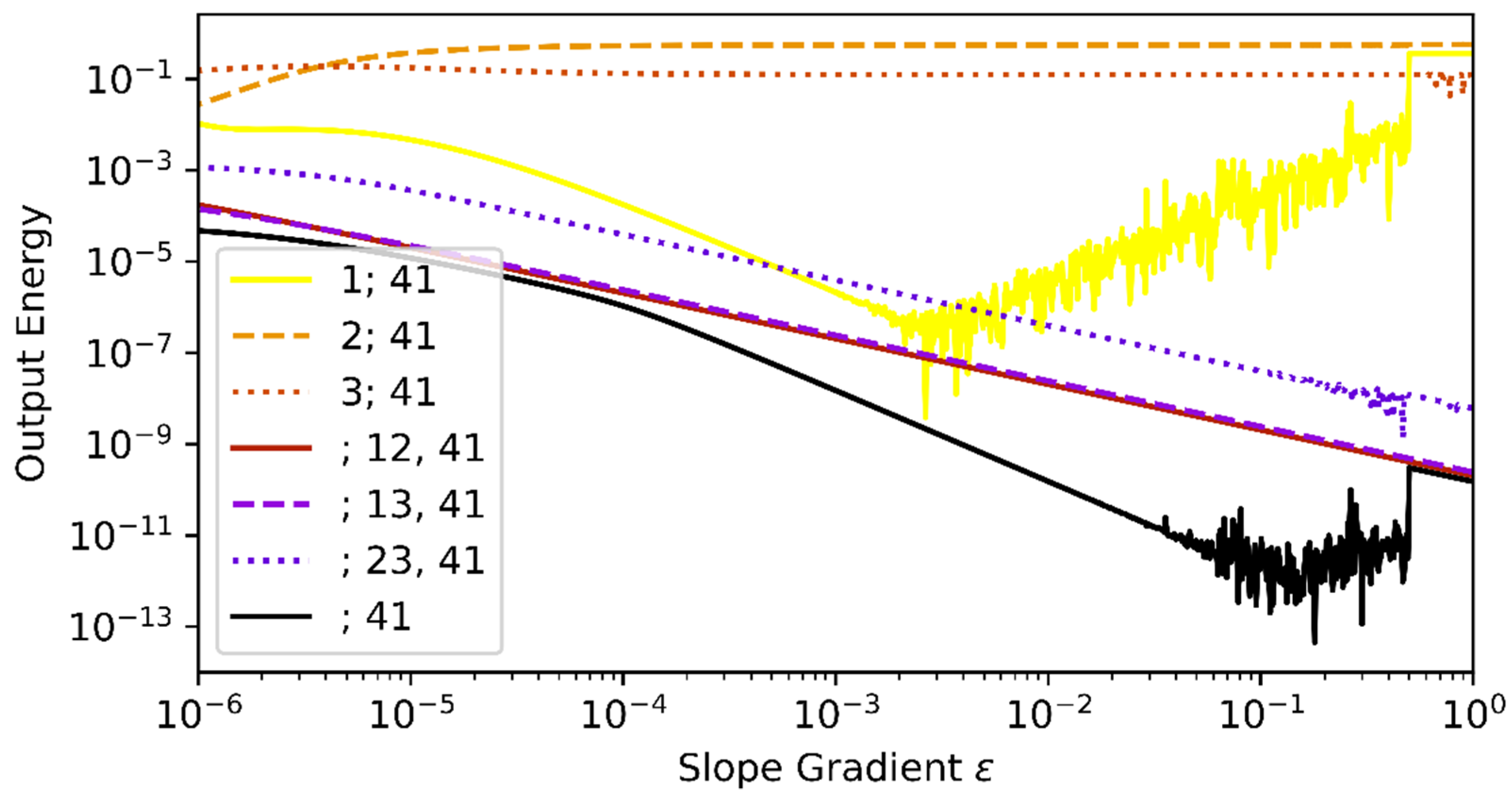
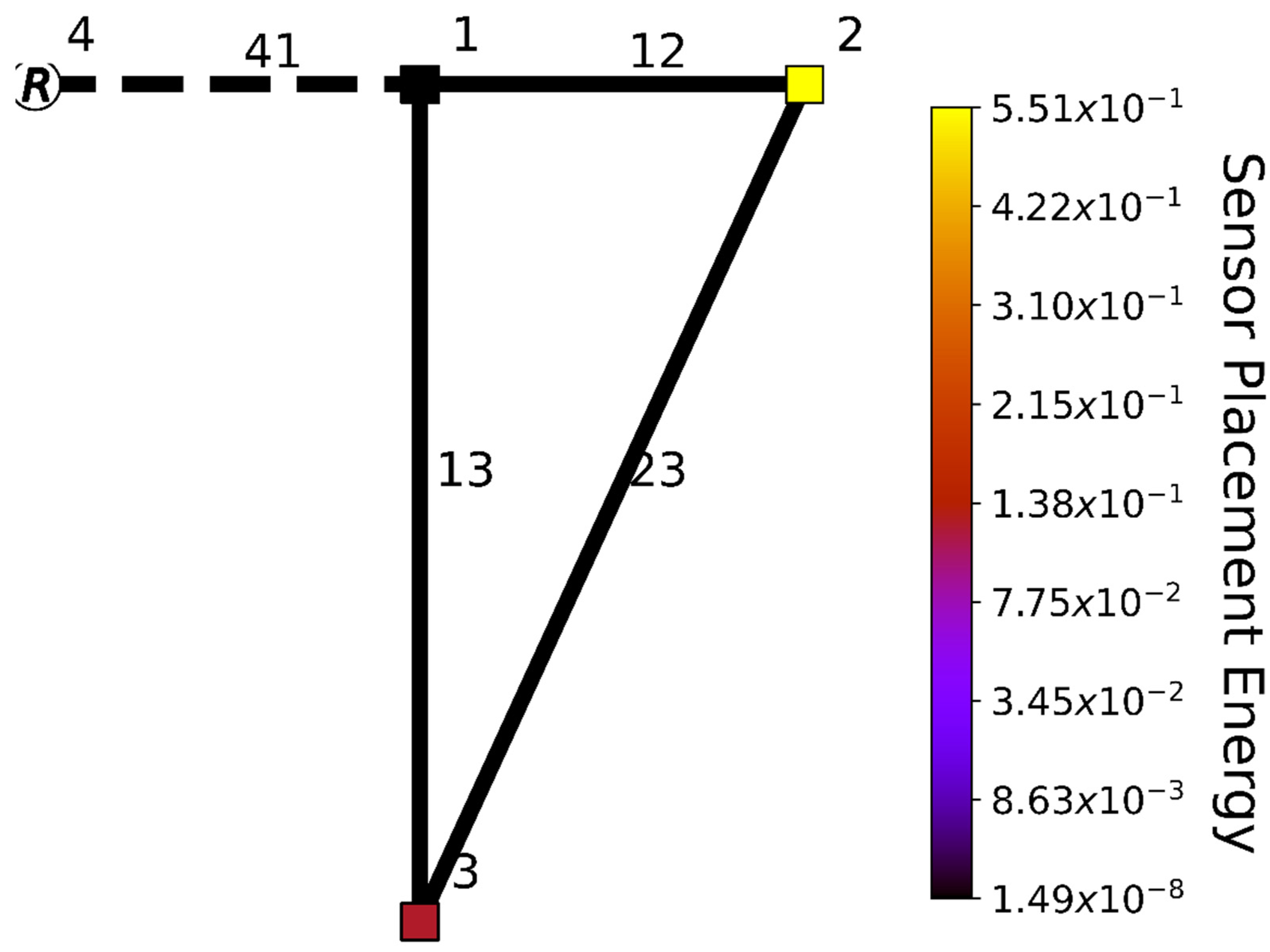
3.1. Example 1: Triangular Network
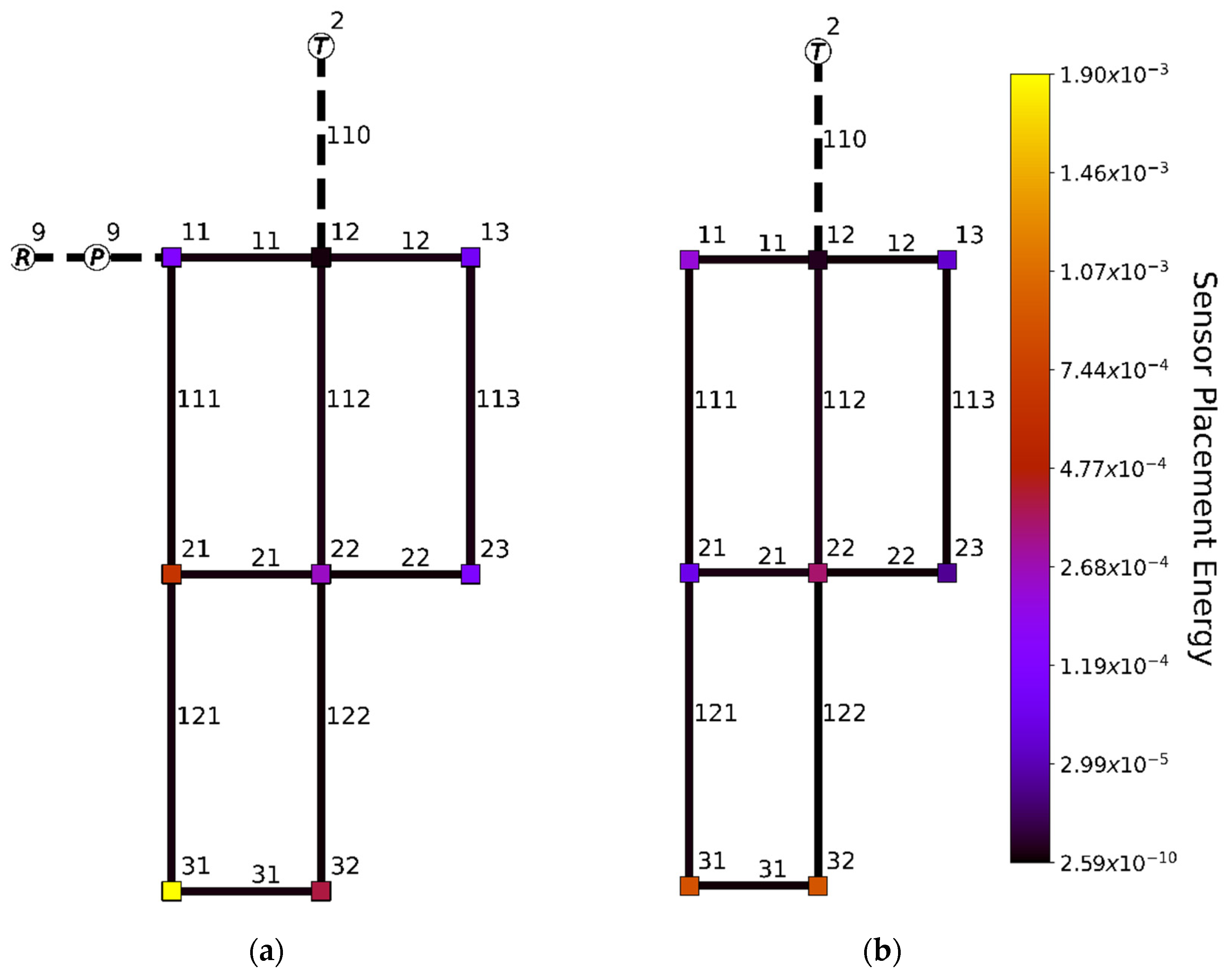
3.2. Example 2: Net1 Case Study
3.3. Hanoi Network
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Notation
| Pressure | ||
| Flow velocity | ||
| Mass density of transported fluid | ||
| Elastic wave velocity | ||
| Gravitational acceleration | ||
| Angle of conduit versus horizontal | ||
| Darcy–Weisbach friction factor | ||
| Inside diameter of conduit | ||
| Elevation | ||
| Cross-sectional area of conduit | ||
| Piezometric head | ||
| Volumetric flowrate | ||
| Hazen–Williams roughness coefficient | ||
| Relative flow gradient | ||
| Observability matrix | ||
| observability Gramian | ||
| Eigenvalue optimality output energy | ||
| Eigenvalues of the observability Gramian | ||
| State vector | ||
| Output vector | ||
| Output vector associated with the optimal sensor configuration | ||
| Input vector | ||
| State matrix | ||
| Input matrix | ||
| Output matrix | ||
| Feedthrough matrix | ||
| Network junction | ||
| Network conduit connecting junction and | ||
| Total number of states | ||
| Number of junction head states | ||
| Number of conduit flow states | ||
| Total number of states whose corresponding asset contains a sensor |
Appendix A
Appendix B
Appendix C
| Link 12 | Link 13 | Link 23 | Link 41 | Node 1 | Node 2 | Node 3 | |
|---|---|---|---|---|---|---|---|
| −0.003 + 0.112j | −0 + 0.001j | 0.001 − 0.001j | 0 − 0.037j | −0.001 − 0.001j | −0.018 + 0.046j | 0.709 | −0.702 − 0.032j |
| −0.003 − 0.112j | −0 − 0.001j | 0.001 + 0.001j | 0 + 0.037j | −0.001 + 0.001j | −0.018 − 0.046j | 0.709 | −0.702 + 0.032j |
| −0.025 + 0.08j | 0.001 − 0.003j | 0.001 − 0.001j | −0.002 − 0.002j | −0.004 + 0.028j | 0.971 | −0.116 − 0.073j | −0.191 − 0.029j |
| −0.025 − 0.08j | 0.001 + 0.003j | 0.001 + 0.001j | −0.002 + 0.002j | −0.004 − 0.028j | 0.971 | −0.116 + 0.073j | −0.191 + 0.029j |
| −0.091 | 0.004 | −0.014 | 0.019 | −0.023 | −0.534 | 0.23 | 0.813 |
| −0.025 + 0.003j | −0.005 + 0.001j | −0.001 | −0.003 + 0.001j | −0.019 + 0.002j | 0.102 + 0.012j | 0.716 | 0.69 + 0.008j |
| −0.025−0.003j | −0.005 − 0.001j | −0.001 | −0.003 − 0.001j | −0.019 − 0.002j | 0.102 − 0.012j | 0.716 | 0.69 − 0.008j |
References
- Chaudhry, M.H. Applied Hydraulic Transients, 3rd ed.; Springer: New York, NY, USA, 2014. [Google Scholar]
- Díaz, S.; González, J.; Mínguez, R. Observability Analysis in Water Transport Networks: Algebraic Approach. J. Water Resour. Plan. Manag. 2016, 142, 04015071. [Google Scholar] [CrossRef]
- di Nardo, A.; di Natale, M.; di Mauro, A.; Santonastaso, G.F.; Palomba, A.; Locoratolo, S. Calibration of a water distribution network with limited field measures: The case study of Castellammare di Stabia (Naples, Italy). In Learning and Intelligent Optimization; LION 12 2018: Lecture Notes in Computer Science; Springer: Cham, Switzerland, 2019; Volume 11353, pp. 433–436. [Google Scholar] [CrossRef]
- Sophocleous, S.; Savić, D.A.; Kapelan, Z.; Giustolisi, O. A Two-stage Calibration for Detection of Leakage Hotspots in a Real Water Distribution Network. Procedia Eng. 2017, 186, 168–176. [Google Scholar] [CrossRef]
- Fuertes, P.C.; Alzamora, F.M.; Carot, M.H.; Campos, J.C.A. Building and exploiting a Digital Twin for the management of drinking water distribution networks. Urban Water J. 2020, 17, 704–713. [Google Scholar] [CrossRef]
- Qi, Z.; Zheng, F.; Guo, D.; Maier, H.R.; Zhang, T.; Yu, T.; Shao, Y. Better Understanding of the Capacity of Pressure Sensor Systems to Detect Pipe Burst within Water Distribution Networks. J. Water Resour. Plan. Manag. 2018, 144, 04018035. [Google Scholar] [CrossRef]
- Steffelbauer, D.B.; Fuchs-Hanusch, D. Efficient Sensor Placement for Leak Localization Considering Uncertainties. Water Resour. Manag. 2016, 30, 5517–5533. [Google Scholar] [CrossRef] [Green Version]
- Sarrate, R.; Nejjari, F.; Rosich, A. Sensor placement for fault diagnosis performance maximization in Distribution Networks. In Proceedings of the 2012 20th Mediterranean Conference on Control & Automation (MED), Barcelona, Spain, 3–6 July 2012; pp. 110–115. [Google Scholar] [CrossRef] [Green Version]
- Farley, B.; Mounce, S.R.; Boxall, J.B. Field testing of an optimal sensor placement methodology for event detection in an urban water distribution network. Urban Water J. 2010, 7, 345–356. [Google Scholar] [CrossRef]
- Bonada, E.; Meseguer, J.; Tur, J.M.M. Practical-Oriented Pressure Sensor Placement for Model-Based Leakage Location in Water Distribution Networks. In Proceedings of the International Conference on Hydroinformatics, New York, NY, USA, 17–21 August 2014. [Google Scholar]
- Boatwright, S.; Romano, M.; Mounce, S.; Woodward, K.; Boxall, J. Optimal Sensor Placement and Leak/Burst Localisation in a Water Distribution System Using Spatially-Constrained Inverse-Distance Weighted Interpolation. In Proceedings of the 13th International Conference on Hydroinformatics, Palermo, Italy, 1–6 July 2018; Volume 3, pp. 282–289. [Google Scholar]
- Nagar, A.K.; Powell, R.S. Observability analysis of water distribution systems under parametric and measurement uncertainty. In Proceedings of the Joint Conference on Water Resource Engineering and Water Resources Planning and Management, Minneapolis, MN, USA, 30 July–2 August 2000; Volume 104, p. 55. [Google Scholar] [CrossRef]
- Marchi, A.; Dandy, G.C.; Boccelli, D.L.; Rana, S.M.M. Assessing the Observability of Demand Pattern Multipliers in Water Distribution Systems Using Algebraic and Numerical Derivatives. J. Water Resour. Plan. Manag. 2018, 144, 04018014. [Google Scholar] [CrossRef]
- Sarrate, R.; Blesa, J.; Nejjari, F.; Quevedo, J. Sensor placement for leak detection and location in water distribution networks. Water Sci. Technol. Water Supply 2014, 14, 795–803. [Google Scholar] [CrossRef] [Green Version]
- Quintiliani, C.; Vertommen, I.; van Laarhoven, K.; van der Vliet, J.; Van Thienen, P. Optimal Pressure Sensor Locations for Leak Detection in a Dutch Water Distribution Network. Environ. Sci. Proc. 2020, 2, 40. [Google Scholar] [CrossRef]
- Pudar, R.S.; Liggett, J.A. Leaks in Pipe Networks. J. Hydraul. Eng. 1992, 118, 1031–1046. [Google Scholar] [CrossRef]
- Cugueró-Escofet, M.; Puig, V.; Quevedo, J. Optimal pressure sensor placement and assessment for leak location using a relaxed isolation index: Application to the Barcelona water network. Control Eng. Pract. 2017, 63, 1–12. [Google Scholar] [CrossRef] [Green Version]
- Qi, J.; Sun, K.; Kang, W. Optimal PMU Placement for Power System Dynamic State Estimation by Using Empirical Observability Gramian. IEEE Trans. Power Syst. 2015, 30, 2041–2054. [Google Scholar] [CrossRef] [Green Version]
- Xu, B.; Abur, A. Observability analysis and measurement placement for systems with PMUs. In Proceedings of the IEEE PES Power Systems Conference and Exposition, New York, NY, USA, 10–13 October 2004; Volume 2, pp. 943–946. [Google Scholar] [CrossRef] [Green Version]
- Johnson, T.; Moger, T. A critical review of methods for optimal placement of phasor measurement units. Int. Trans. Electr. Energy Syst. 2021, 31, e12698. [Google Scholar] [CrossRef]
- Quiñones-Grueiro, M.; Bernal-De-Lázaro, J.M.; Verde, C.; Prieto-Moreno, A.; Llanes-Santiago, O. Comparison of Classifiers for Leak Location in Water Distribution Networks. IFAC-PapersOnLine 2018, 51, 407–413. [Google Scholar] [CrossRef]
- Casillas, M.V.; Puig, V.; Garza-Castañón, L.E.; Rosich, A. Optimal sensor placement for leak location in water distribution networks using genetic algorithms. Sensors 2013, 13, 14984–15005. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Giustolisi, O.; Savic, D.; Kapelan, Z. Pressure-Driven Demand and Leakage Simulation for Water Distribution Networks. J. Hydraul. Eng. 2008, 134, 626–635. [Google Scholar] [CrossRef] [Green Version]
- Kwakernaak, H.; Sivan, R. Linear Optimal Control Systems; Wiley-Interscience: New York, NY, USA, 1972; Volume 1. [Google Scholar]
- Kalman, R.E. Mathematical Description of Linear Dynamical Systems. J. Soc. Ind. Appl. Math. Ser. A Control 1963, 1, 152–192. [Google Scholar] [CrossRef]
- Georges, D. Use of observability and controllability gramians or functions for optimal sensor and actuator location in finite-dimensional systems. In Proceedings of the IEEE Conference on Decision and Control, New Orleans, LA, USA, 13–15 December 1995; Volume 4. [Google Scholar] [CrossRef]
- Rossman, L.A. EPANET 2; U.S. Environmental Protection Agency: Washington, DC, USA, 2000. [Google Scholar]
- Watters, G.Z. Analysis and Control of Unsteady Flow in Pipelines, 2nd ed.; Butterworths: Waltham, MA, USA, 1984. [Google Scholar]
- Dager, R.; Zuazua, E. Wave propagation, observation and control in 1-d flexible multi-structures. Math. Appl. 2006, 50, 227. [Google Scholar]
- Izquierdo, J.; Pérez, R.; Iglesias, P.L. Mathematical models and methods in the water industry. Math. Comput. Model. 2004, 39, 1353–1374. [Google Scholar] [CrossRef]
- Ramos, H.; Covas, D.; Borga, A.; Loureiro, D. Surge damping analysis in pipe systems: Modelling and experiments. J. Hydraul. Res. 2004, 42, 413–425. [Google Scholar] [CrossRef]
- Zhang, Z. Hydraulic Transients and Computations; Springer: Cham, Switzerland, 2020. [Google Scholar]
- Grubben, N.L.M.; Keesman, K.J. Controllability and observability of 2D thermal flow in bulk storage facilities using sensitivity fields. Int. J. Control 2018, 91, 1554–1566. [Google Scholar] [CrossRef] [Green Version]
- Keesman, K.J. Sytem Identification, an Introduction, Advanced Textbooks in Control and Signal Processing; Springer: Cham, Switzerland, 2011. [Google Scholar]
- Pronzato, L.; Pázman, A. Design of Experiments in Nonlinear Models: Asymptotic Normality, Optimality Criteria and Small-Sample Properties. Linear Notes Stat. 2013, 212, 404. [Google Scholar]
- Wald, A. On the Efficient Design of Statistical Investigations. Ann. Math. Stat. 1943, 14, 134–140. [Google Scholar] [CrossRef]
- Fujiwara, O.; Khang, D.B. A two-phase decomposition method for optimal design of looped water distribution networks. Water Resour. Res. 1990, 26, 539–549. [Google Scholar] [CrossRef]
- Bi, W.; Dandy, G.C.; Maier, H.R. Improved genetic algorithm optimization of water distribution system design by incorporating domain knowledge. Environ. Model. Softw. 2015, 69, 370–381. [Google Scholar] [CrossRef]


| Conduit | Length [m] | Diameter [m] | Roughness | Flow [m3/s] | |||
|---|---|---|---|---|---|---|---|
| 12 | 1524 | 0.2032 | 120 | 2.50 × 10−2 | 4.53 × 103 | 2.09 × 10−4 | −4.85 × 10−2 |
| 13 | 914.4 | 0.1524 | 80 | 1.10 × 10−2 | 8.05 × 103 | 1.96 × 10−4 | −1.10 × 10−1 |
| 23 | 243.8 | 0.3048 | 200 | −1.48 × 10−3 | 2.01 × 103 | 2.93 × 10−3 | −5.29 × 10-4 |
| 41 | 304.8 | 0.3048 | 100 | 4.86 × 10−2 | 2.01 × 103 | 2.35 × 10−3 | −3.74 × 10−2 |
| State | Conduit | Junction | ||||||
|---|---|---|---|---|---|---|---|---|
| State | 12 | 13 | 23 | 41 | 1 | 2 | 3 | |
| Conduit | 12 | −4.85 × 10−2 | 0 | 0 | 0 | 2.09 × 10−4 | −2.09 × 10−4 | 0 |
| 13 | 0 | −1.00 × 10−1 | 0 | 0 | 1.96 × 10−4 | 0 | −1.96 × 10−4 | |
| 23 | 0 | 0 | −5.29 × 10−4 | 0 | 0 | 2.93 × 10−3 | −2.93 × 10−3 | |
| 41 | 0 | 0 | 0 | −3.74 × 10−2 | −2.35 × 10−3 | 0 | 0 | |
| Junction | 1 | −4.53 × 103 | −8.05 × 103 | 0 | 2.01 × 103 | 0 | 0 | 0 |
| 2 | 4.53 × 103 | 0 | −2.01 × 103 | 0 | 0 | 0 | 0 | |
| 3 | 0 | 8.05 × 103 | 2.01 × 103 | 0 | 0 | 0 | 0 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Geelen, C.V.C.; Yntema, D.R.; Molenaar, J.; Keesman, K.J. Optimal Sensor Placement in Hydraulic Conduit Networks: A State-Space Approach. Water 2021, 13, 3105. https://doi.org/10.3390/w13213105
Geelen CVC, Yntema DR, Molenaar J, Keesman KJ. Optimal Sensor Placement in Hydraulic Conduit Networks: A State-Space Approach. Water. 2021; 13(21):3105. https://doi.org/10.3390/w13213105
Chicago/Turabian StyleGeelen, Caspar V. C., Doekle R. Yntema, Jaap Molenaar, and Karel J. Keesman. 2021. "Optimal Sensor Placement in Hydraulic Conduit Networks: A State-Space Approach" Water 13, no. 21: 3105. https://doi.org/10.3390/w13213105
APA StyleGeelen, C. V. C., Yntema, D. R., Molenaar, J., & Keesman, K. J. (2021). Optimal Sensor Placement in Hydraulic Conduit Networks: A State-Space Approach. Water, 13(21), 3105. https://doi.org/10.3390/w13213105






