An Integrated Aquifer Management Approach for Aridification-Affected Agricultural Area, Shengeldy-Kazakhstan
Abstract
:1. Introduction
2. Materials and Methods
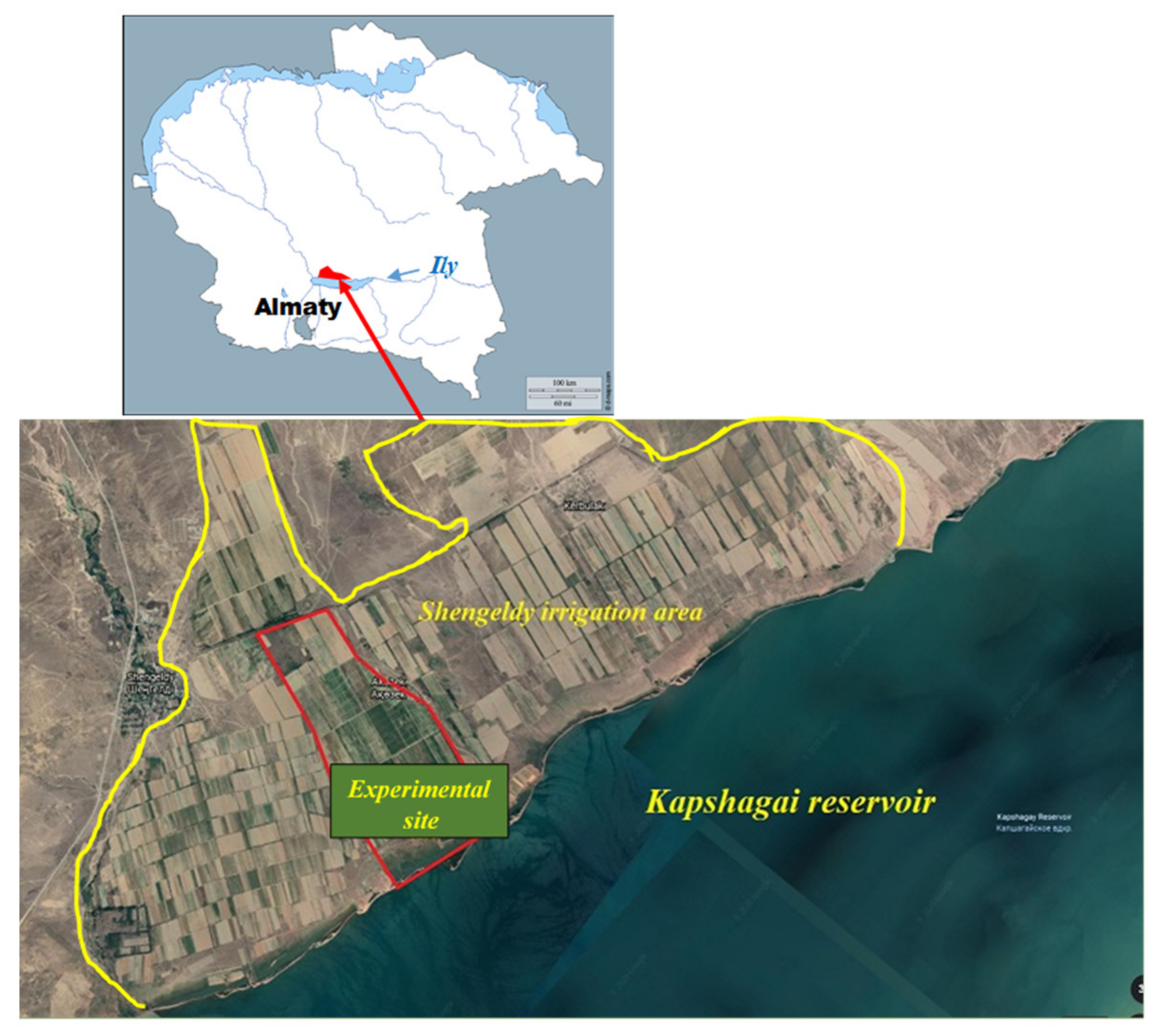
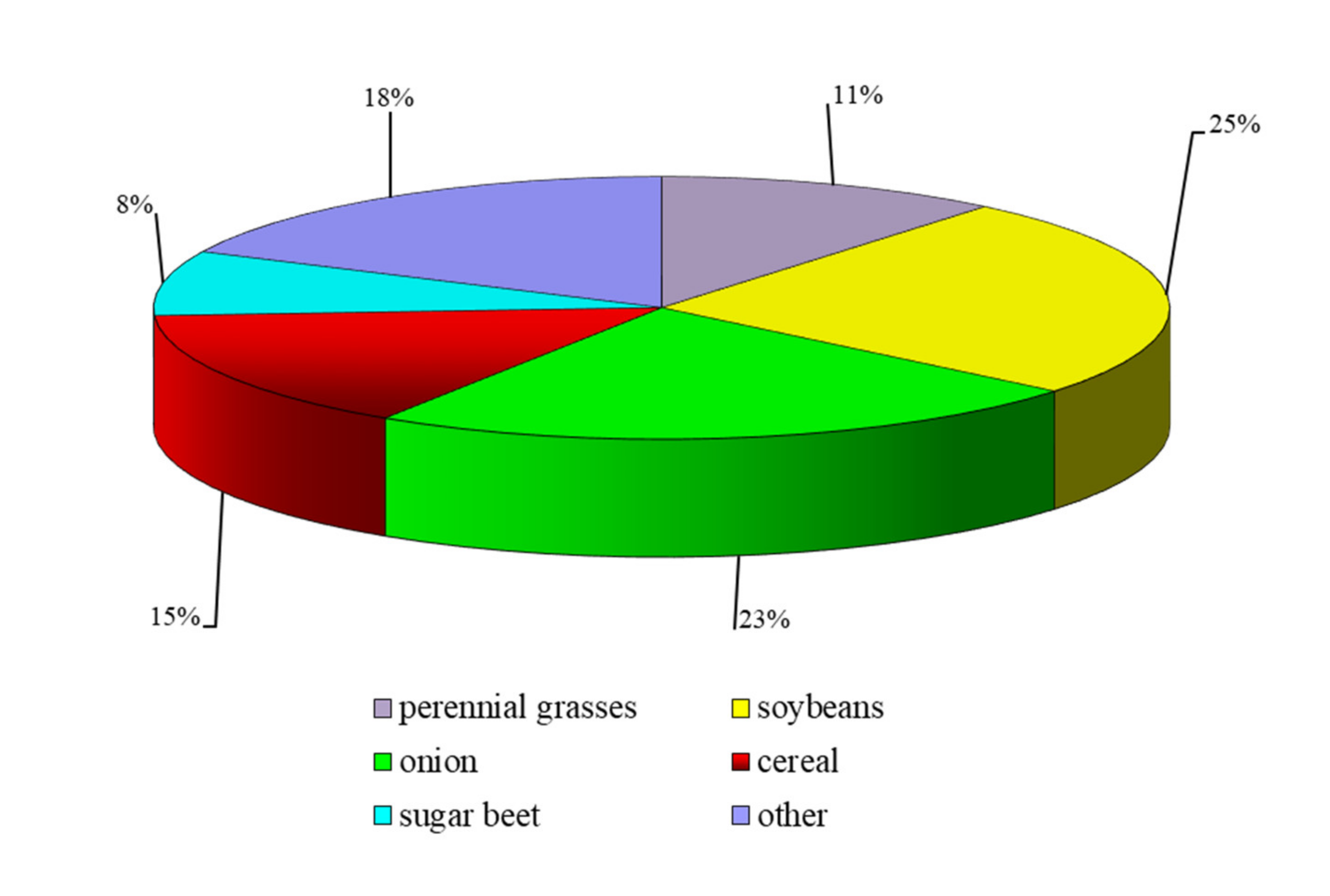
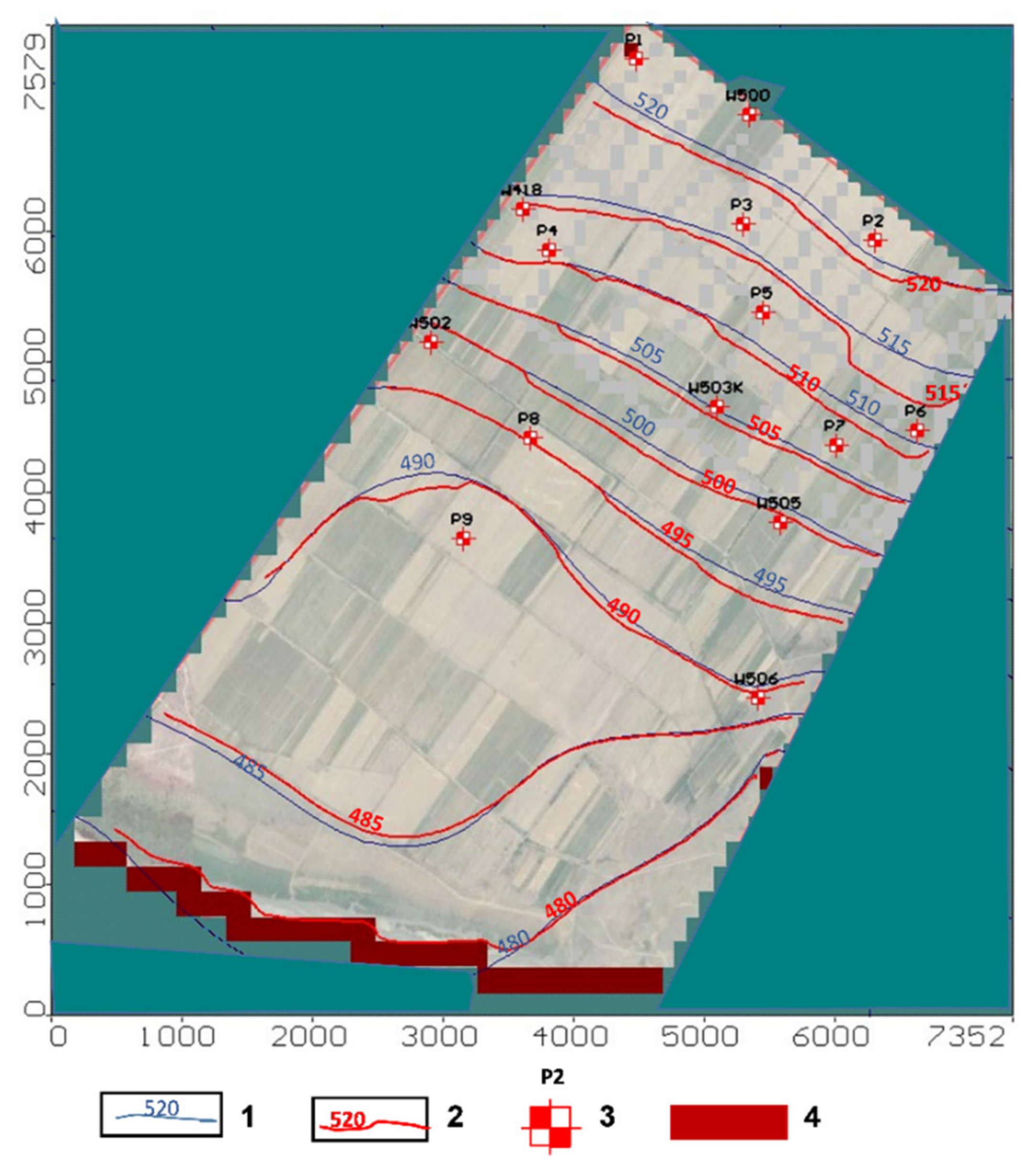
2.1. The Research Site Geographical and Hydrogeological Conditions
2.2. Modeling Setup
2.2.1. Hydrogeological Survey
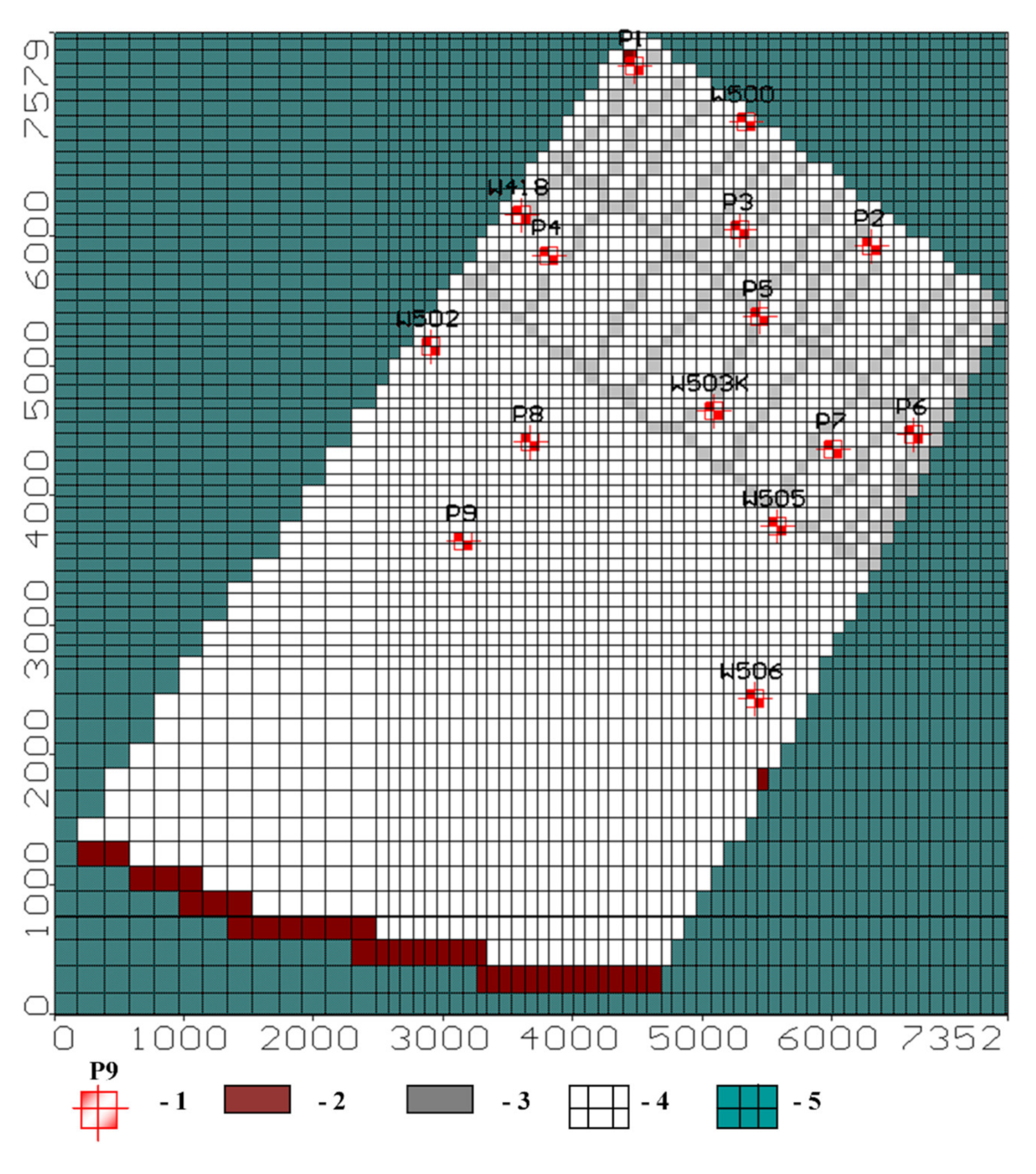
- Drilling and installation of nine observation piezometers (indicated by the letter Pin Figure 2), each 7 m deep. All piezometers were equipped with a slit filter and gravel filling surrounding them at a depth interval of 3 to 5 m;
- To study the sediment cover and the unsaturated zone hydrophysical and mechano-physical properties, eight test sites consisting of shallow observation wells and equipped with nitrometers and moisture meters were prepared;
- Groundwater level and salinity monitoring in the six monitoring wells (indicated by the letter W in Figure 2) were performed monthly from January to April and from September to December. From May to August, every 10 days in the first and third quarters (April, May, and October), as well as four times a month from June to September; monitoring was done in observation piezometers,
- The groundwater hydro-chemical regime and irrigation effect were studied by water solution chemical analyses, including pH, Ca, Mg, Na + K, Cl, SO4, and HCO3 concentrations; total dissolved solids (TDS); and the sodium adsorption ratio (SAR). Water sampling was done in May, August, and October (i.e., in the beginning, closer to the end of the growing season, and during the opening of the damper on the K-2 collector);
- The field measurement database included rainfall and irrigation regimes, drainage water discharge, and evaporation potential. The database was compiled in 2016 and based on reported data of the Zonal Hydrogeological-Ameliorative Center of the Ministry of Agriculture, Republic of Kazakhstan, beginning from 2012 [5].
2.2.2. The Site Mathematical Computer Modeling Structure
2.2.3. Model Illustration
- The groundwater flow rate to the aquifer through the northern border, as recharge in the boundary blocks of the model;
- The existing subsurface drainage;
- Irrigation water infiltration during the irrigation period, as recharge in the corresponding blocks of the model;
- Evapotranspiration;
- The water level in the Kapshagai reservoir;
- Rain precipitation from April to November 2017.
2.2.4. Model Calibration and Validation
2.2.5. Model Predictions
3. Results
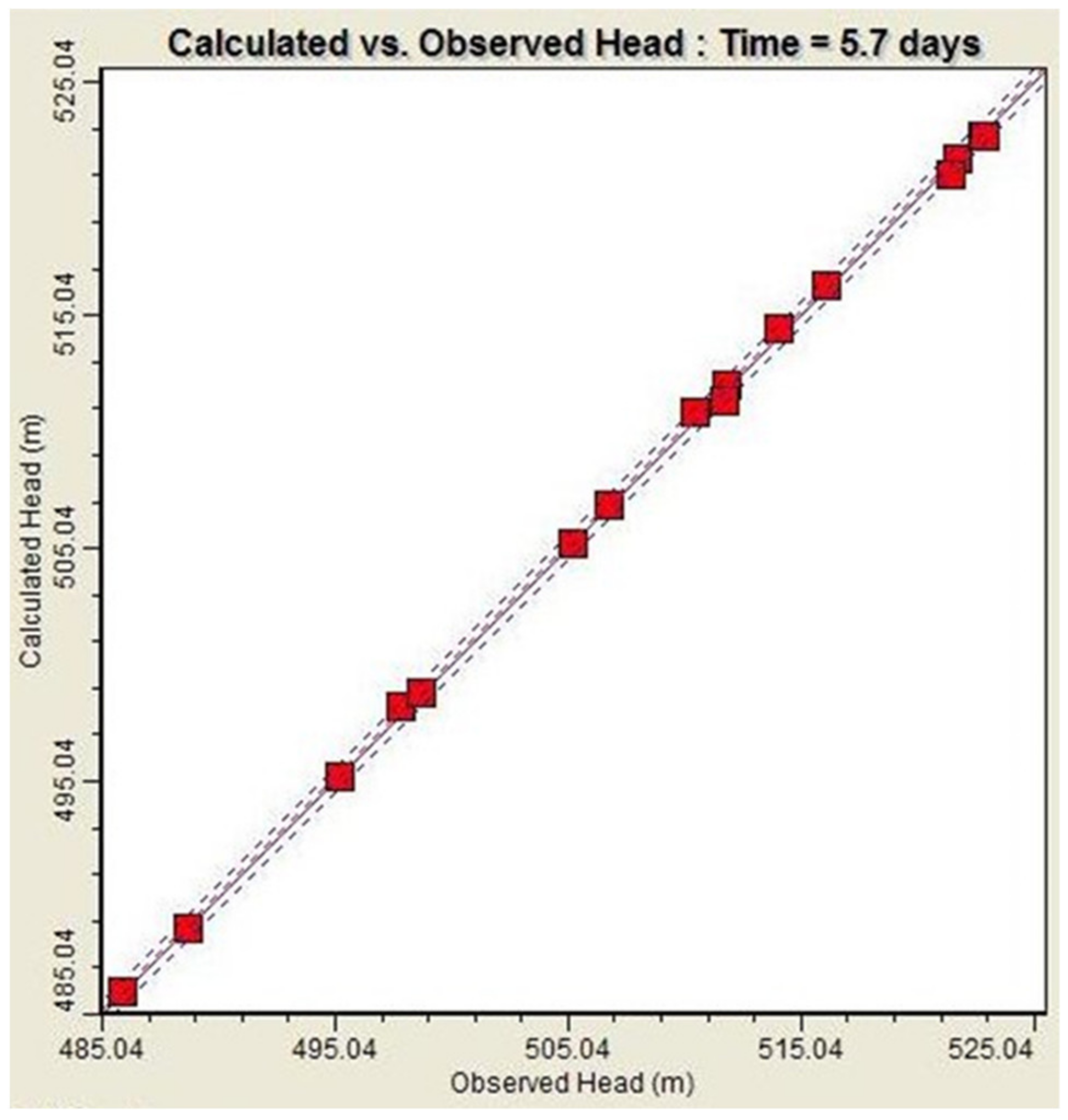
3.1. Model Calibration
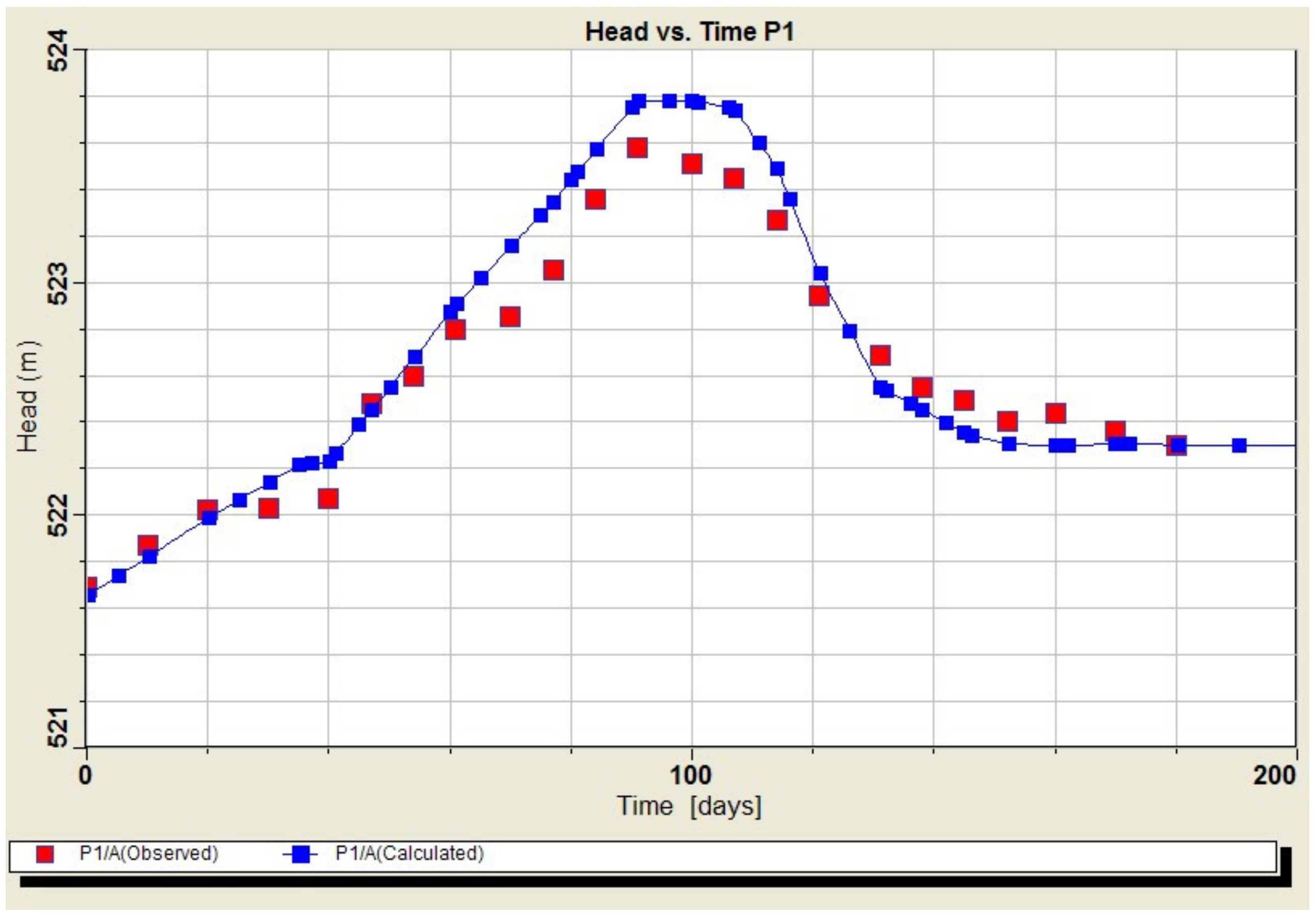
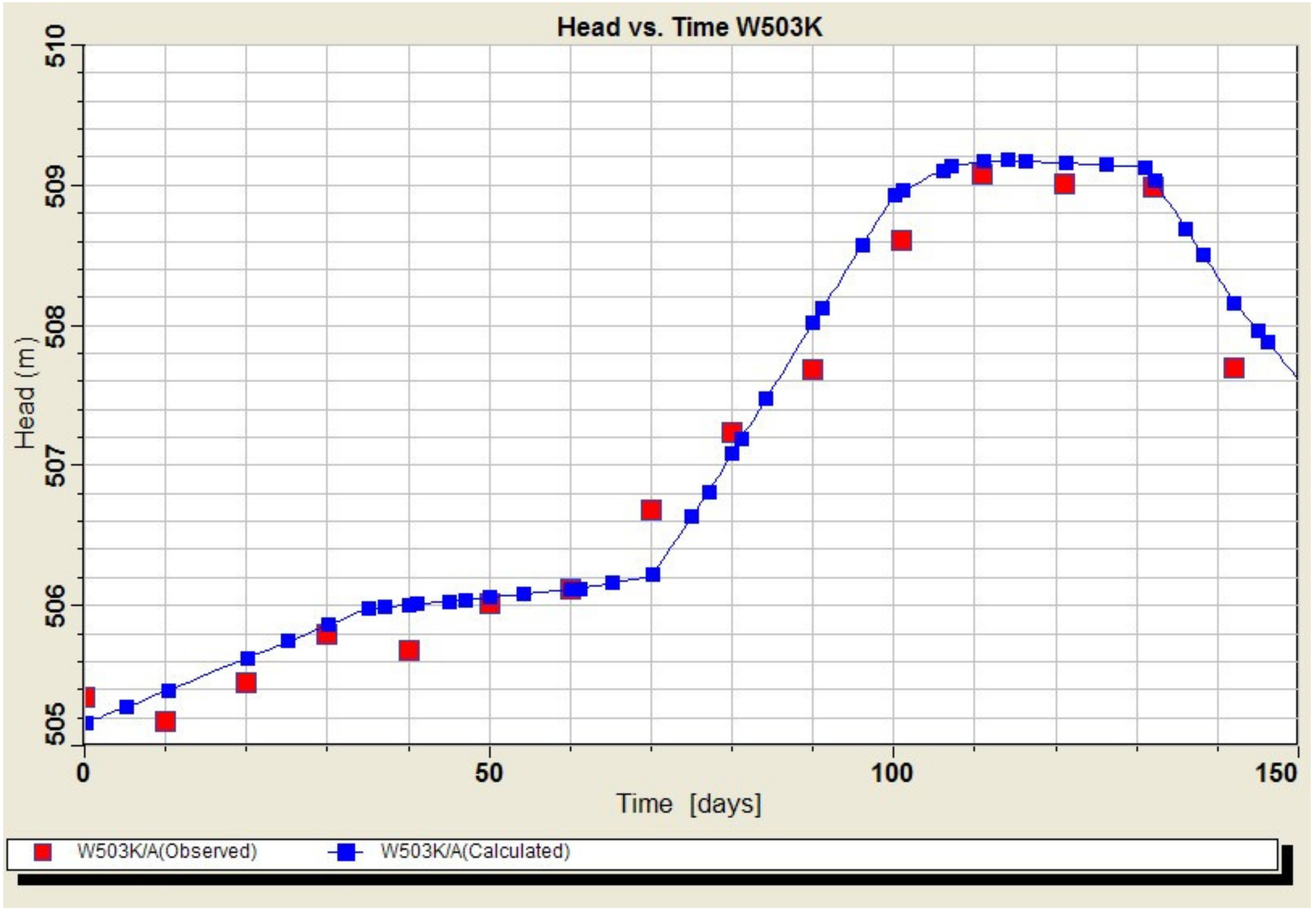
3.2. Model Validation
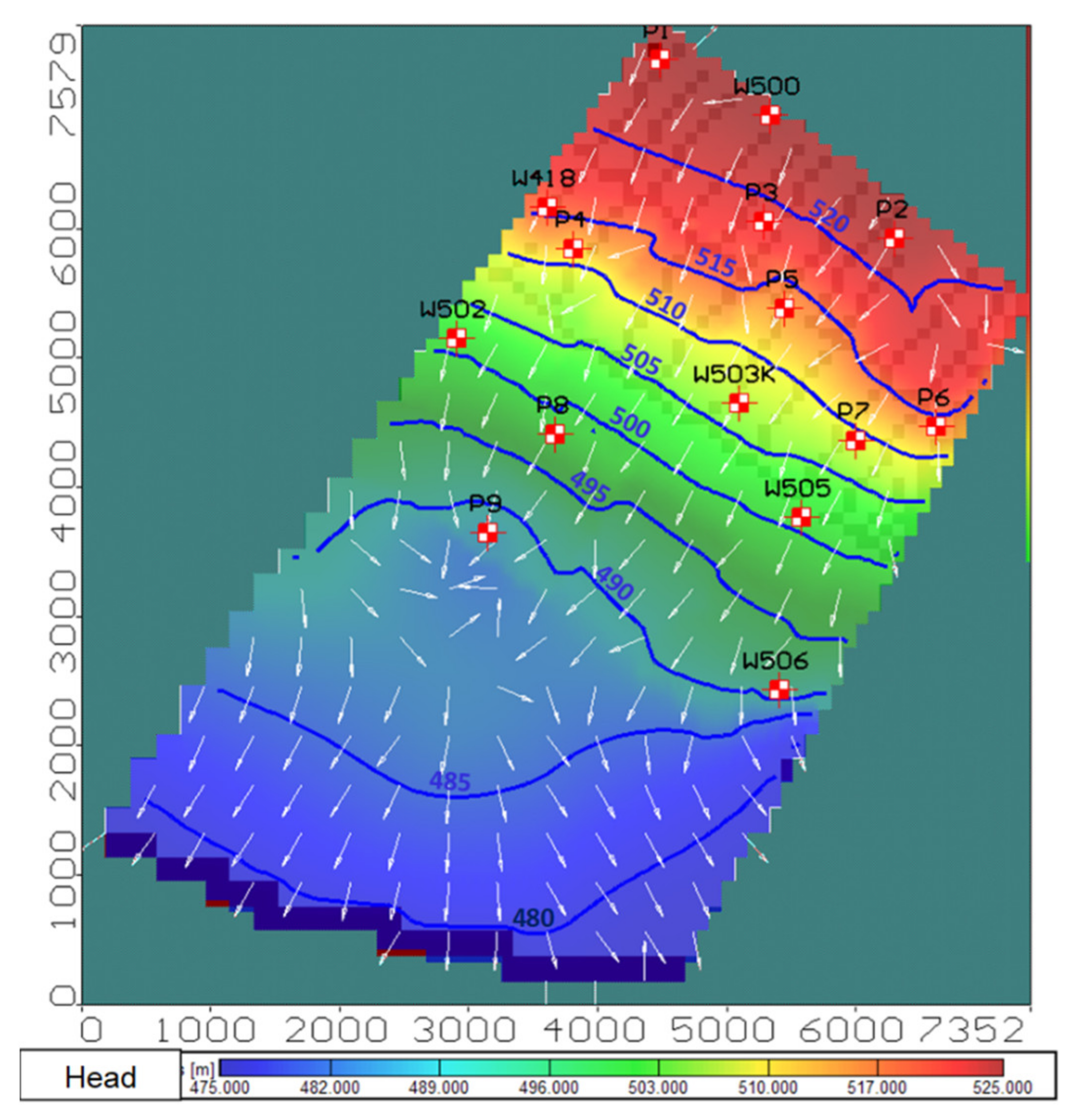
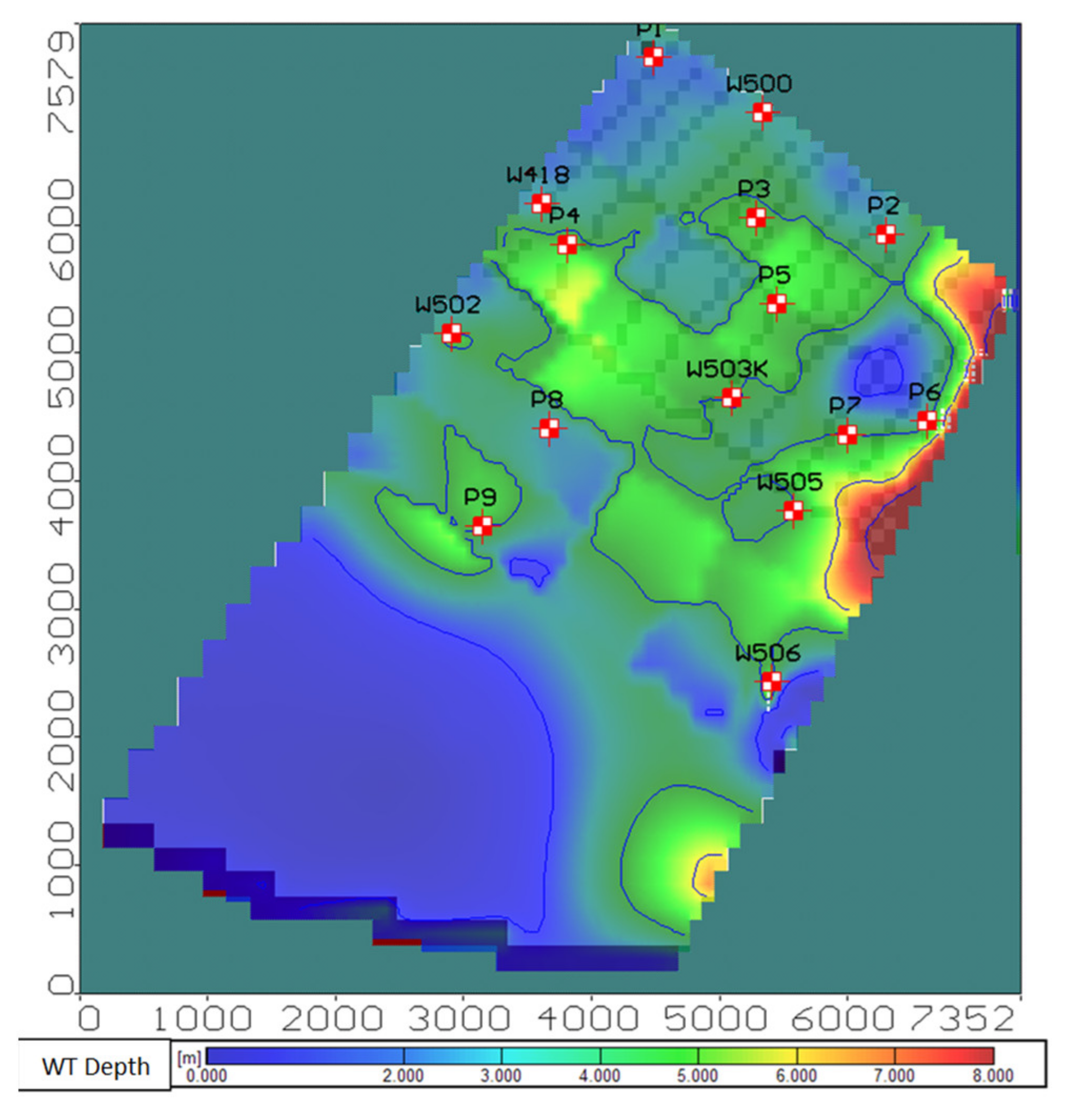
3.3. Model Prediction
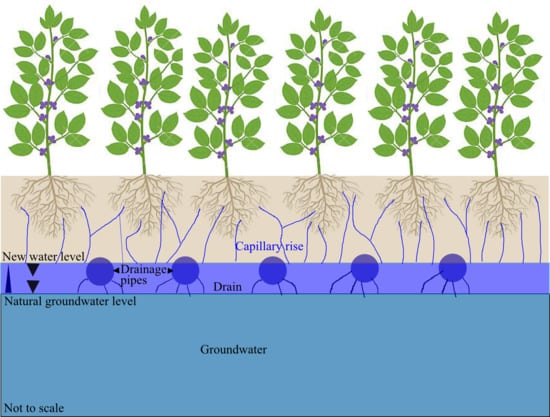
3.4. Assessment of Additional Drainage Water Irrigation Source for Crop Root Zone Moisturizing
4. Discussion
5. Conclusions
- The MODFLOW spatial distribution groundwater flow model provided reliable information about artificial groundwater recharge from existing drainage in complex hydrogeological and intensive farming conditions. The hydrogeological and ameliorative conditions of the geo-filtration model for the experimental site of Kazakhstan’s Shengeldy agricultural area were based on and validated by field study results and met the specified model solution accuracy and reliability requirements;
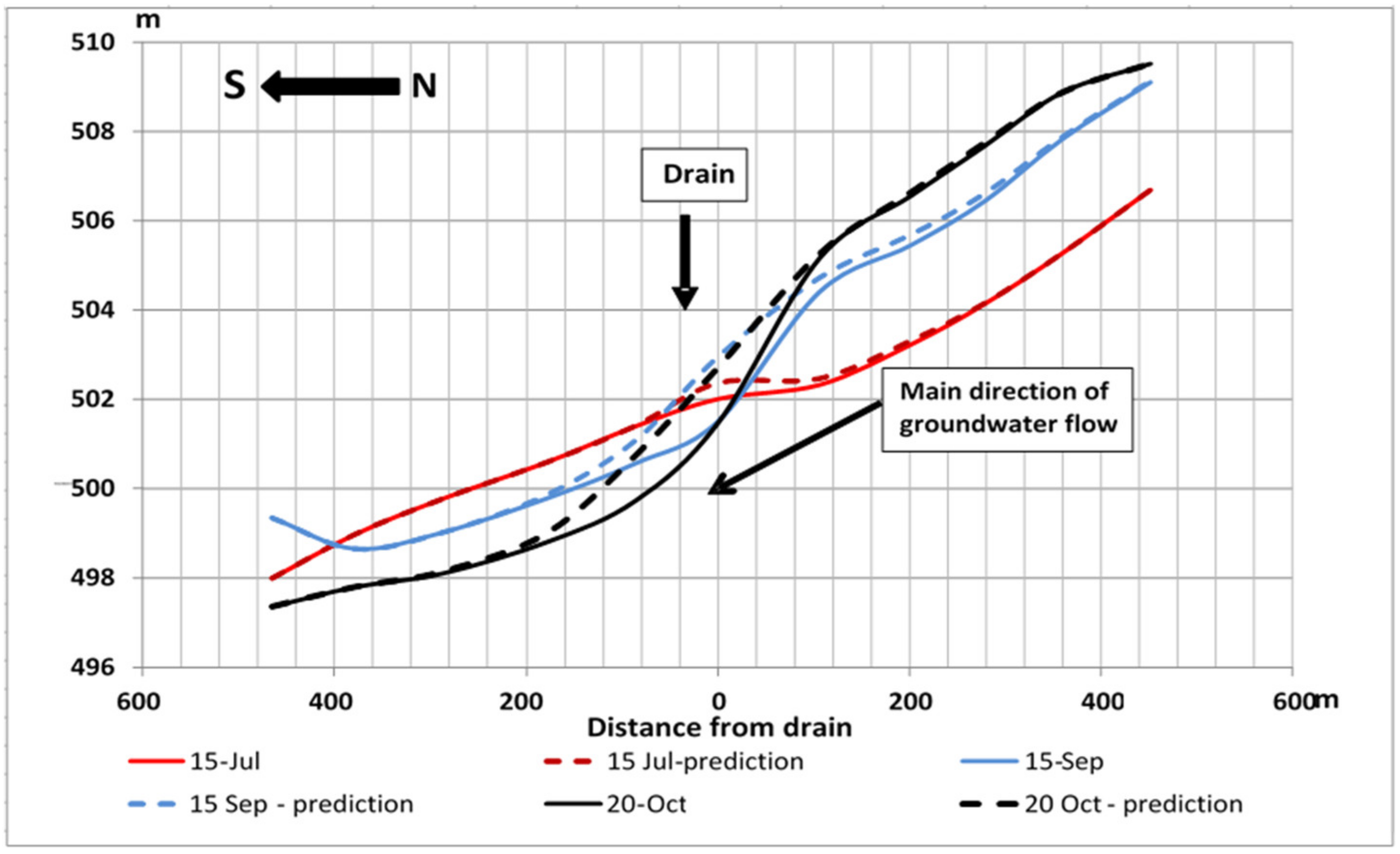
- The model’s additional groundwater recharge prediction due to existing drainage infiltration under the current irrigation conditions was validated against experimental data obtained at the site in 2017. It was found that at the end of the growing season, the groundwater table’s additional elevation from drainage infiltration would be about 1.5 m below the drains, and the additional groundwater recharge influence zone would spread to up to 350 m from the drains.
- Based on the simulation results, detailed groundwater head contour maps and flow paths, as well as groundwater table depths from the soil surface, were made for typical periods of irrigation. The groundwater flow balances that were calculated in the model for the same periods made it possible to quantify and characterize the temporal change in the groundwater flow balance. The calculated irrigation water infiltration loss reached 40.5% of the irrigation water supply.
- The experimental study and field monitoring results confirmed the MODFLOW simulation, showing that under certain conditions drainage can be an additional source of irrigation. Furthermore, through the artificial use of groundwater capillary rise to the lower root system boundary, crop nourishing and moisturizing can be obtained during the growing season.
- Agriculture is an important sector in the economy of Central Asia that is restricted by water scarcity [17]. The new capillary fringe irrigation approach presented in this paper can more than double the arable land in Kazakhstan and neighboring countries. Seasonal undulation in the groundwater level is necessary to prevent accumulation of salts in the soil section [37].
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Rhoades, J.D.; Bingham, F.; Letey, J.; Hoffman, G.; Dedrick, A.; Pinter, P.; Replogle, J. Use of saline drainage water for irrigation: Imperial Valley study. Agric. Water Manag. 1989, 16, 25–36. [Google Scholar] [CrossRef]
- Aljenaby, A.Z.A.; Aljenaby, Z.A.Z. Usage of drainage water in irrigation: Applications on the eastern euphrates in Babylon province. Int. J. Agric. Stat. Sci. 2018, 14, 331–338. [Google Scholar]
- Nindwani, B.A.; Lashari, B.K.; Memon, A.A.; Laghari, K.Q.; Hammad, H.M.; Farhad, W. Impact of drainage water use for crop irrigation. Sci. Int. 2014, 26, 273–278. [Google Scholar]
- Valipour, M. Effect of Drainage Parameters Change on Amount of Drain Discharge in Subsurface Drainage Systems. IOSR J. Agric. Vet. Sci. 2012, 1, 10–18. [Google Scholar] [CrossRef]
- Kulagin, V.; Umbetaliev, D.; Auelkhan, Y.; Makyzhanova, A.; Karataev, D. Groundwater water-salt balance of shengeldy irrigated lands using water-saving technologies. News Natl. Acad. Sci. Repub. Kazakhstan, Ser. Geol. Tech. Sci. 2017, 3, 161–174. [Google Scholar]
- Sanford, W. Recharge and groundwater models: An overview. Hydrogeol. J. 2002, 10, 110–120. [Google Scholar] [CrossRef]
- Hashemi, H.; Berndtsson, R.; Persson, M. Artificial recharge by floodwater spreading estimated by water balances and groundwater modeling. Hydrol. Sci. J. 2015, 60, 336–350. [Google Scholar] [CrossRef]
- Rejani, R.; Jha, M.K.; Panda, S.N.; Mull, R. Simulation modeling for efficient groundwater management in balasore coastal basin, India. Water Resour. Manag. 2008, 22, 23–50. [Google Scholar] [CrossRef]
- Mirlas, V.; Antonenko, V.; Kulagin, V.; Kuldeeva, E. Assessing artificial groundwater recharge on irrigated land using the MODFLOW model. Earth Sci. Res. 2015, 4, 16. [Google Scholar] [CrossRef]
- Mirlas, V. MODFLOW Modeling to Solve Drainage Problems in the Argaman Date Palm Orchard, Jordan Valley, Israel. J. Irrig. Drain. Eng. 2013, 139, 612–624. [Google Scholar] [CrossRef]
- Sarwar, F.; Feddes, R.A. Evaluating drainage design parameters for the fourth drainage project, Pakistan by using SWAP model: Part II- Modeling results. Irrig. Drain. Syst. 2000, 14, 281–299. [Google Scholar] [CrossRef]
- Gates, T.K.; Burkhalter, J.P.; Labadie, J.W.; Valliant, J.C.; Broner, I. Monitoring and modeling flow and salt transport in salinity-threatened irrigated valley. J. Irrig. Drain. Eng. 2002, 128, 87–99. [Google Scholar] [CrossRef]
- Kurien, V.M.; Cooke, R.A.; Hirshi, M.C.; Mitchell, J.K. Estimating drain spacing of incomplete drainage system. Trans. ASAE 1997, 40, 377–382. [Google Scholar] [CrossRef]
- Singh, R.; Helmers, M.J.; Zhiming, Q. Calibration and validation of DRAINMOD to design subsurface drainage system for Iowa’s tile landscapes. Agric. Water Manag. 2006, 65, 221–232. [Google Scholar] [CrossRef]
- Bradford, R.B.; Acreman, M.C. Applying MODFLOW to wet grassland in-field habitats: A case study from the Pevensey Levels, UK. Hydrol. Earth Syst. Sci. 2003, 7, 43–55. [Google Scholar] [CrossRef] [Green Version]
- Sagin, J.; Adenova, D.; Tolepbayeva, A.; Poryadin, V. Underground water resources in Kazakhstan. Int. J. Environ. Stud. 2017, 74, 386–398. [Google Scholar] [CrossRef]
- Ostrovsky, V.N. Comparative analysis of groundwater formation in arid and super-arid deserts (with examples from central Asia and northeastern Arabian Peninsula). Hydrogeol. J. 2007, 15, 759–771. [Google Scholar] [CrossRef]
- Zhupankhan, A.; Tussupova, K.; Berndtsson, R. Water in Kazakhstan, a key in Central Asian water management. Hydrol. Sci. J. 2018, 63, 752–762. [Google Scholar] [CrossRef] [Green Version]
- Mukhamedzhanov, M.; Makyzhanova, A.; Kulagin, V. The rationale and definition of prospects by the use of groundwater for irrigation, forage production and pastures irrigation of Kazakhstan. News Nat. Acad. Sci. Rep. 2017, 3, 72–83. [Google Scholar]
- Ring, I.; Sandström, C. Chapter 6: Options for governance and decision-making across scales and sectors. Regional and Subregional Assessments of Biodiversity and Ecosystem Services: Regional and Subregional Assessment for Europe and Central Asia; Bonn, Germany, 2018; Volume 9, pp. 1–587. Available online: https://jyx.jyu.fi/handle/123456789/62146 (accessed on 10 August 2021).
- Belitz, K.; Phillips, S.P. Alternative to Agricultural Drains in California’s San Joaquin Valley: Results of a Regional-Scale Hydrogeologic Approach. Water Resour. Res. 1995, 31, 1845–1862. [Google Scholar] [CrossRef]
- Pueppke, S.G.; Nurtazin, S.T.; Graham, N.A.; Qi, J. Central Asia’s Ili River ecosystem as a wicked problem: Unraveling complex interrelationships at the interface of water, energy, and food. Water 2018, 10, 541. [Google Scholar] [CrossRef] [Green Version]
- Wu, H.; Wu, J.; Song, F.; Abuduwaili, J.; Saparov, A.S.; Chen, X.; Shen, B. Spatial distribution and controlling factors of surface water stable isotope values (δ 18 O and δ 2 H) across Kazakhstan, Central Asia. Sci. Total Environ. 2019, 678, 53–61. [Google Scholar] [CrossRef]
- Makyzhanova, A. Groundwater Resources of Kazakhstan and Water Supply Tasks for Agrarian Sector of the Economy (State, Prospects); Kazakh National Research Technical University: Almaty, Kazahstan, 2018. [Google Scholar]
- Thevs, N.; Nurtazin, S.; Beckmann, V.; Salmyrzauli, R.; Khalil, A. Water consumption of agriculture and natural ecosystems along the Ili river in china and kazakhstan. Water 2017, 9, 15. [Google Scholar] [CrossRef] [Green Version]
- Dzhetimov, M.; Andasbayev, E.; Esengabylov, I.; Koyanbekova, S.; Tokpanov, E. Physical and Chemical Research of Processes of Salt Formation in the Water of Balkhash Lake. In Proceedings of the CBU International Conference Proceedings, Prague, Czech Republic, 30 June 2013; Volume 1, pp. 400–411. [Google Scholar]
- Gulnura, I.; Abuduwaili, J.; Oleg, S. Deflation processes and their role in desertification of the southern Pre-Balkhash deserts. Arab. J. Geosci. 2014, 7, 4513–4521. [Google Scholar] [CrossRef]
- Ding, Y.K.; Li, Y.P.; Liu, Y.R. Spatial-temporal assessment of agricultural virtual water and uncertainty analysis: The case of Kazakhstan (2000–2016). Sci. Total Environ. 2020, 724, 138155. [Google Scholar] [CrossRef] [PubMed]
- Wang, Z.; Fan, B.; Guo, L. Soil salinization after long-term mulched drip irrigation poses a potential risk to agricultural sustainability. Eur. J. Soil Sci. 2019, 70, 20–24. [Google Scholar] [CrossRef] [Green Version]
- Alemie, T.C.; Tilahun, S.A.; Ochoa-Tocachi, B.F.; Schmitter, P.; Buytaert, W.; Parlange, J.; Steenhuis, T.S. Predicting Shallow Groundwater Tables for Sloping Highland Aquifers. Water Resour. Res. 2019, 55, 11088–11100. [Google Scholar] [CrossRef]
- Kruseman, G.P.; de Ridder, N. Analysis and Evalution of Pumping Test Data; International Institute for Land Reclamation and Improvement: Wageningen, The Netherlands, 1994. [Google Scholar]
- Schlumberger Water Services. Visual MODFLOW 2011.1 User’s Manual; Schlumberger Water Services: Kitchener, ON, Canada, 2011. [Google Scholar]
- Zipper, S.C.; Dallemagne, T.; Gleeson, T.; Boerman, T.C.; Hartmann, A. Groundwater Pumping Impacts on Real Stream Networks: Testing the Performance of Simple Management Tools. Water Resour. Res. 2018, 54, 5471–5486. [Google Scholar] [CrossRef] [Green Version]
- Kaspar, T.C.; Stanley, C.D.; Taylor, H.M. Soybean Root Growth during the Reproductive Stages of Development 1. Agron. J. 1978, 70, 1105–1107. [Google Scholar] [CrossRef]
- Stone, R. For China and Kazakhstan, no meeting of the minds on water. Science 2012, 337, 405–407. [Google Scholar] [CrossRef]
- Ramazanova, E.; Lee, S.H.; Lee, W. Stochastic risk assessment of urban soils contaminated by heavy metals in Kazakhstan. Sci. Total Environ. 2021, 750, 141535. [Google Scholar] [CrossRef] [PubMed]
- Pandit, R.; Parrota, J.; Anker, Y.; Coudel, E.; Diaz Morejón, C.F.; Harris, J.; Karlen, D.L.; Kertész, Á.; Mariño De Posada, J.L.; Ntshotsho Simelane, P.; et al. Chapter 6: Responses to Halt Land. In IPBES. The IPBES Assessment Report on Land Degradation and Restoration; Montanarella, L., Scholes, R., Brainich, A., Eds.; Secretariat of the Intergovernmental Science-Policy Platform on Biodiversity and Ecosystem Services: Bonn, Germany, 2018; pp. 435–528. [Google Scholar]


| Date | 25-April | 15-July | 5-October | 15-November | |
|---|---|---|---|---|---|
| Input | Storage recharge | 16 | 10 | 111 | 76 |
| Infiltration from storage pond | 0.02 | 0.001 | 0.03 | 0 | |
| Infiltration of irrigation water and precipitation | 58.1 | 117 | 9.6 | 3.1 | |
| Total input | 74.5 | 126.6 | 120.9 | 78.7 | |
| Output | Storage discharge | 56.9 | 115.6 | 6.6 | 2.5 |
| Outflow to Kapshagai reservoir | 2.3 | 1.1 | 0.031 | −1 | |
| Areal discharge | 2.5 | 0 | 108.3 | 71.1 | |
| Evapotranspiration | 12 | 9.1 | 3.7 | 0.7 | |
| Drainage outflow | 0.9 | 0.6 | 1.6 | 0.4 | |
| Total output | 74.6 | 126.4 | 120.2 | 73.7 | |
| Input–output | −0.1 | 0.2 | 0.7 | 5 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mirlas, V.; Makyzhanova, A.; Kulagin, V.; Kuldeev, E.; Anker, Y. An Integrated Aquifer Management Approach for Aridification-Affected Agricultural Area, Shengeldy-Kazakhstan. Water 2021, 13, 2357. https://doi.org/10.3390/w13172357
Mirlas V, Makyzhanova A, Kulagin V, Kuldeev E, Anker Y. An Integrated Aquifer Management Approach for Aridification-Affected Agricultural Area, Shengeldy-Kazakhstan. Water. 2021; 13(17):2357. https://doi.org/10.3390/w13172357
Chicago/Turabian StyleMirlas, Vladimir, Assyl Makyzhanova, Vitaly Kulagin, Erghan Kuldeev, and Yaakov Anker. 2021. "An Integrated Aquifer Management Approach for Aridification-Affected Agricultural Area, Shengeldy-Kazakhstan" Water 13, no. 17: 2357. https://doi.org/10.3390/w13172357
APA StyleMirlas, V., Makyzhanova, A., Kulagin, V., Kuldeev, E., & Anker, Y. (2021). An Integrated Aquifer Management Approach for Aridification-Affected Agricultural Area, Shengeldy-Kazakhstan. Water, 13(17), 2357. https://doi.org/10.3390/w13172357