Comparison Between Two Hydrodynamic Models in Simulating Physical Processes of a Reservoir with Complex Morphology: Maroon Reservoir
Abstract
1. Introduction
2. Study Site, Data and Methods
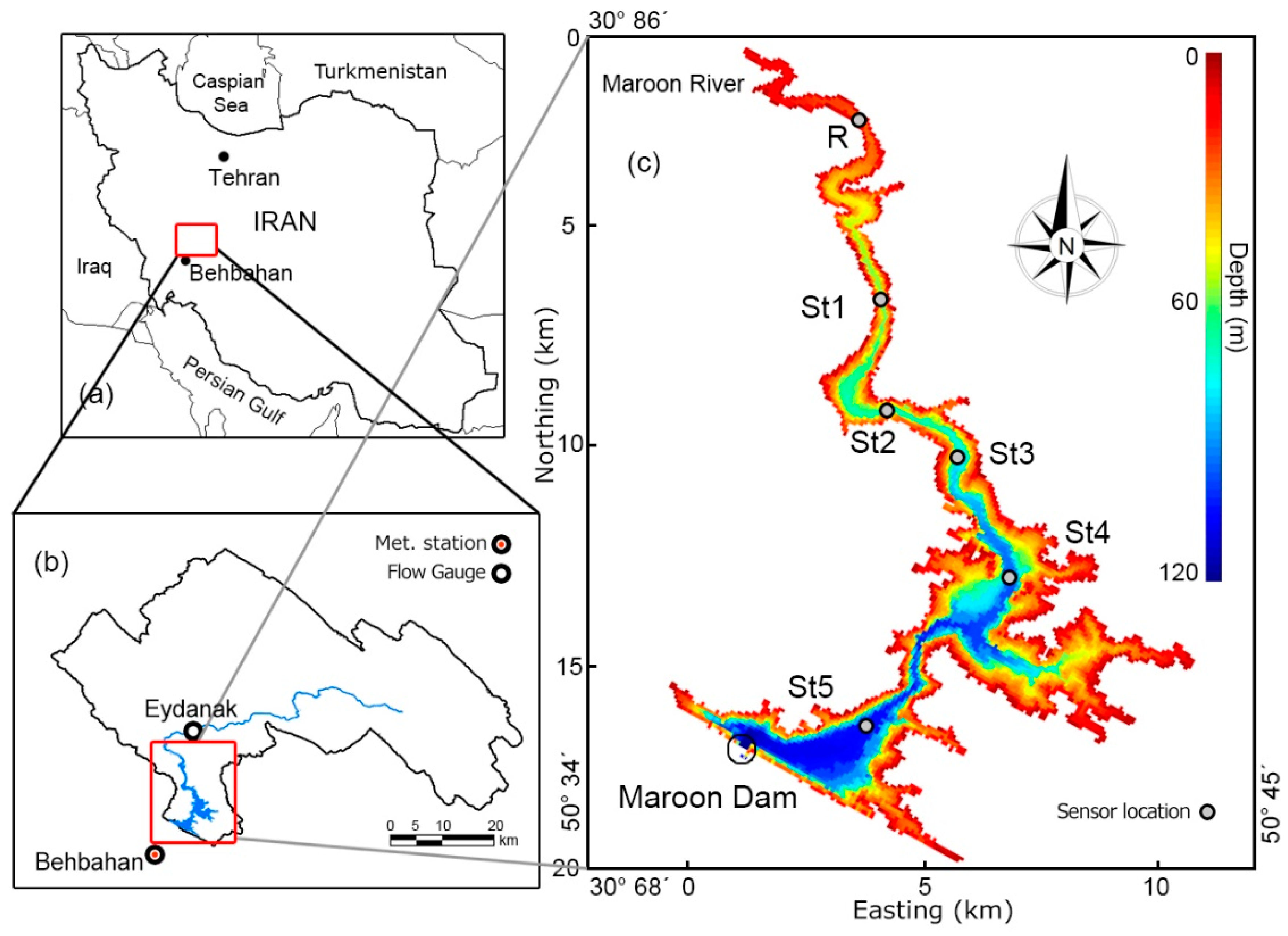
2.1. Study Site
2.2. Data
2.3. Computational Resources
2.4. AEM3D Model
2.4.1. Model Description
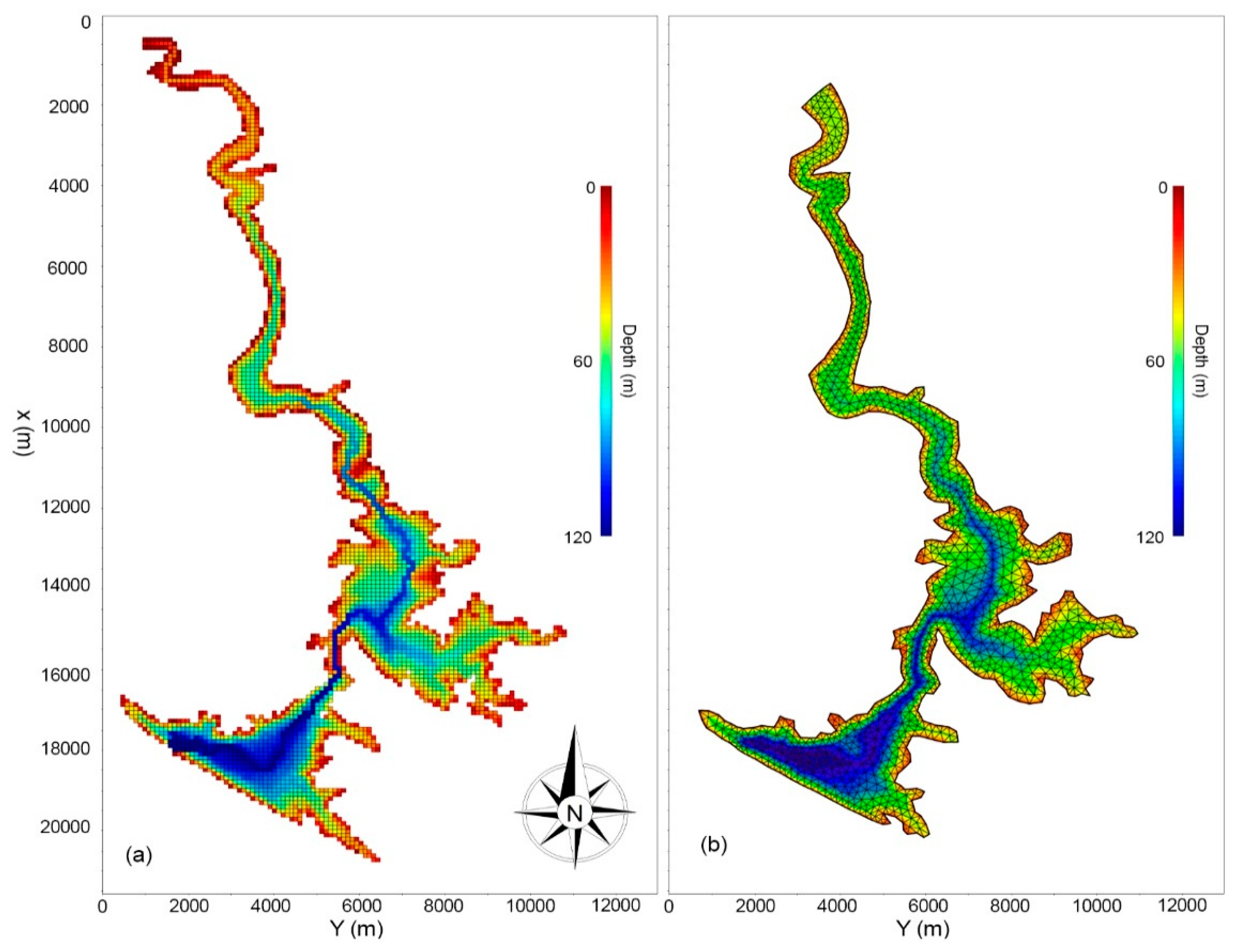
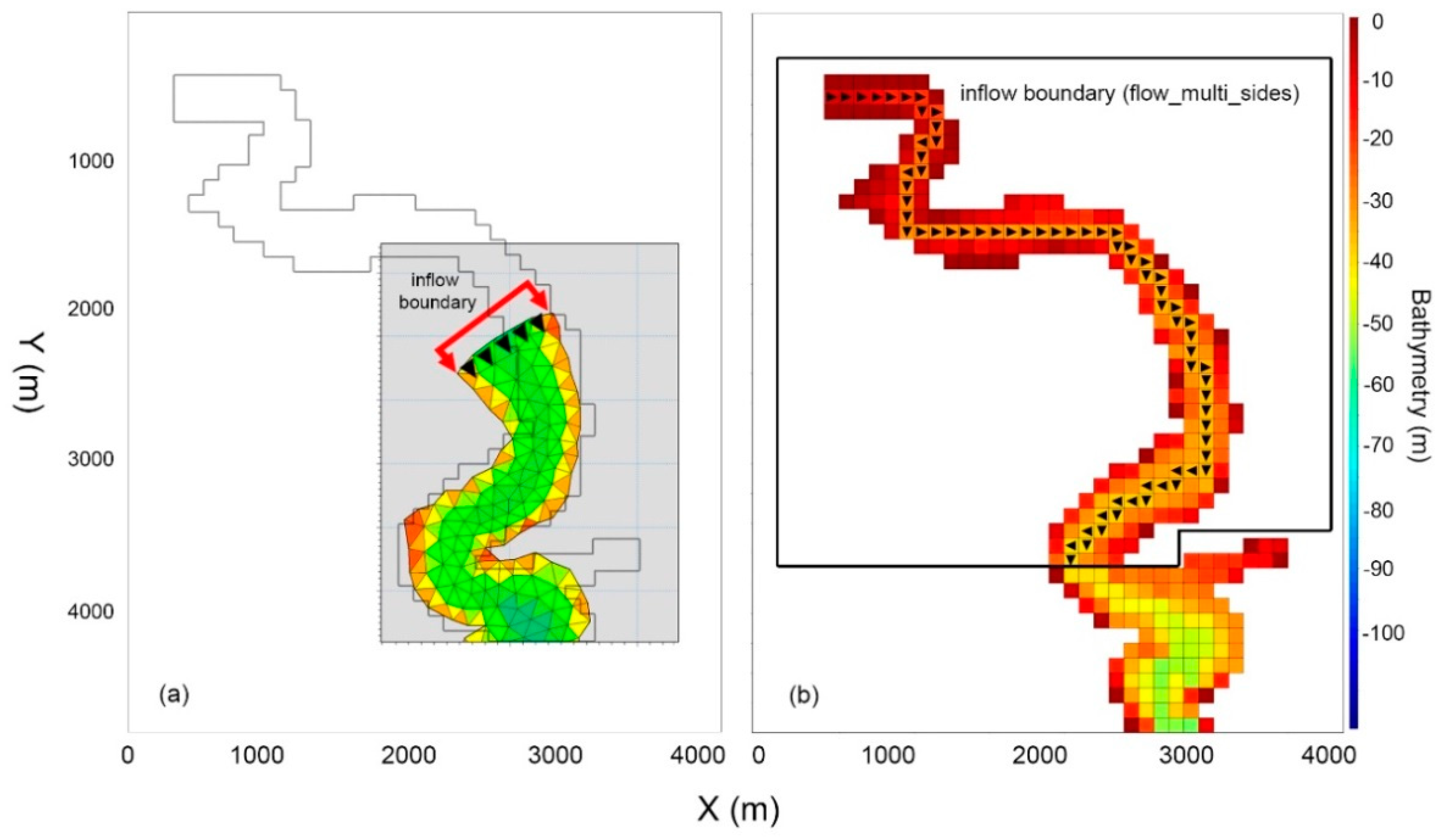
2.4.2. AEM3D Model Preparation
2.5. MIKE3 Model
2.5.1. Model Description
2.5.2. MIKE3 Model Preparation
2.6. Models’ Parameters
2.7. Methodology and Models’ Evaluation
3. Results and Discussion
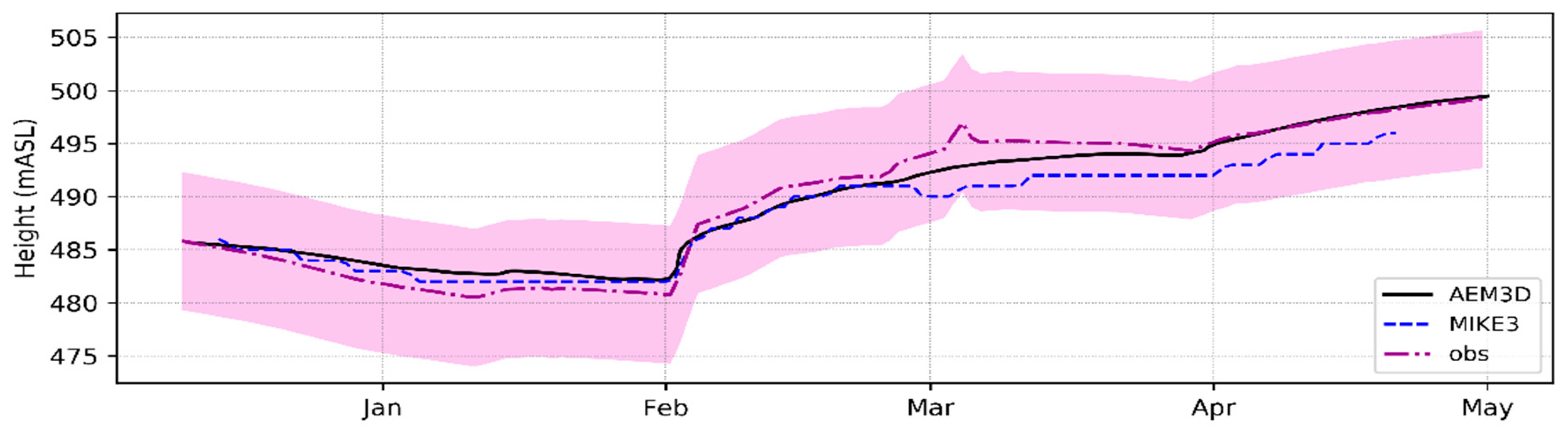
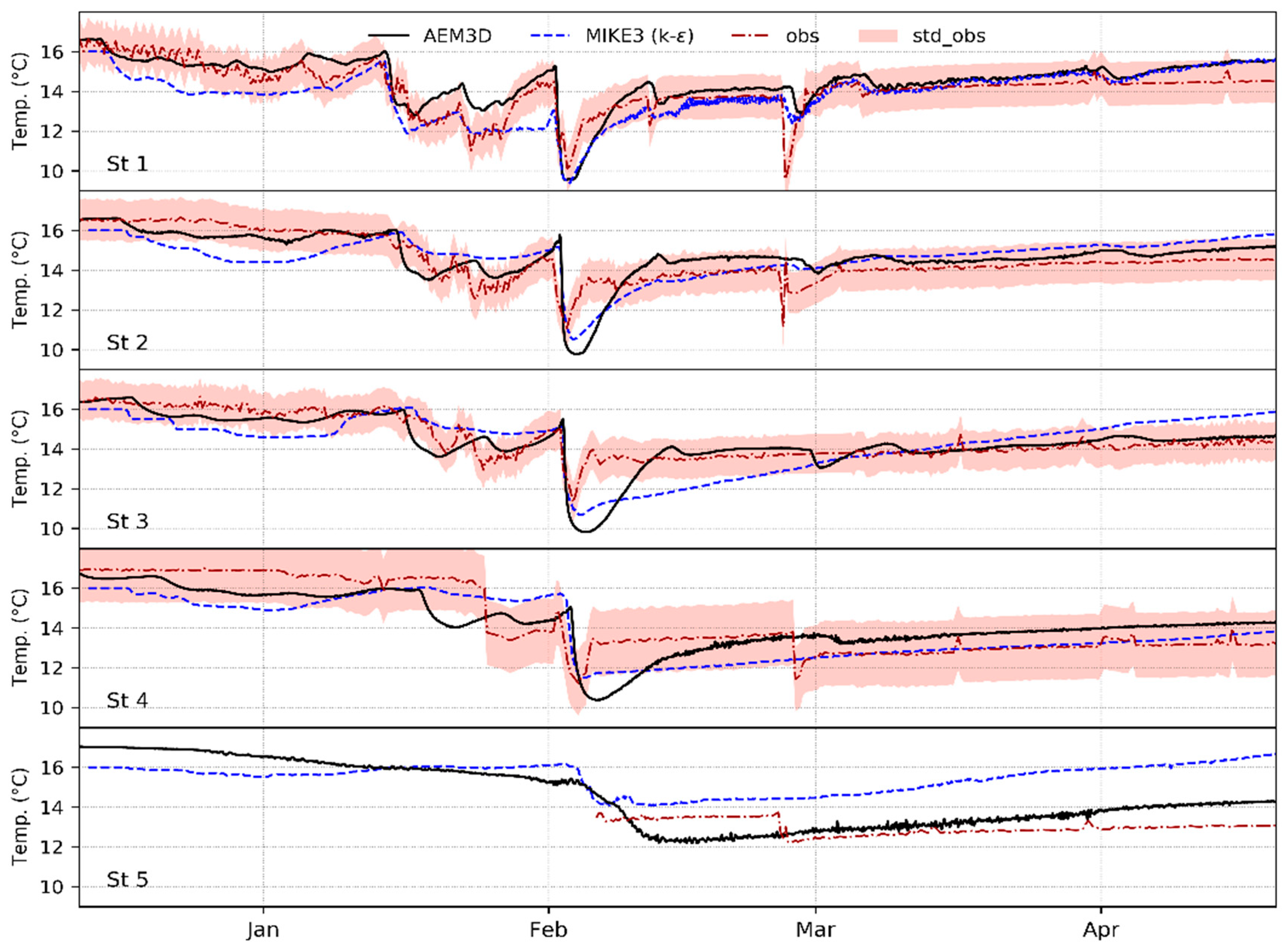
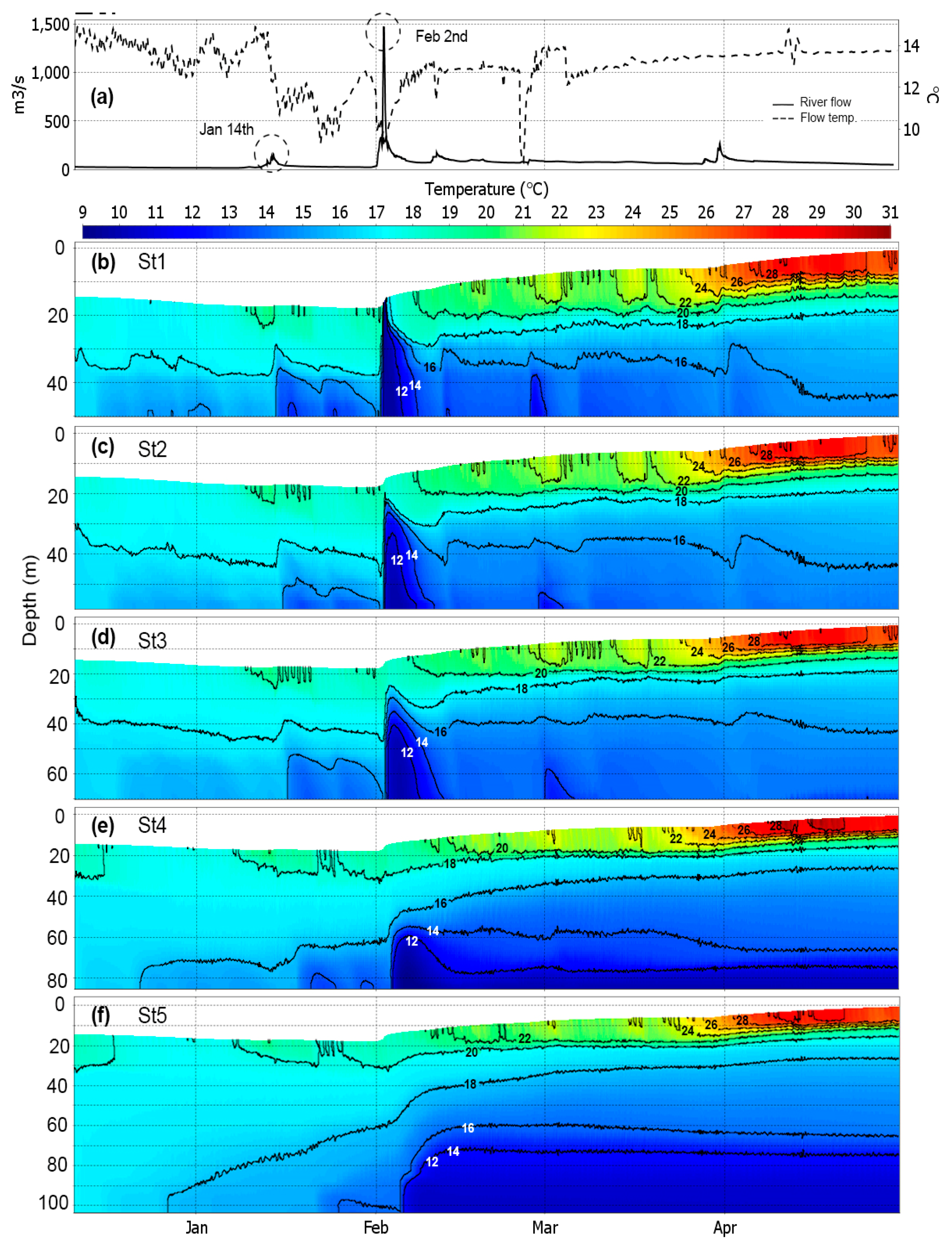
3.1. General Results of Hydrodynamics
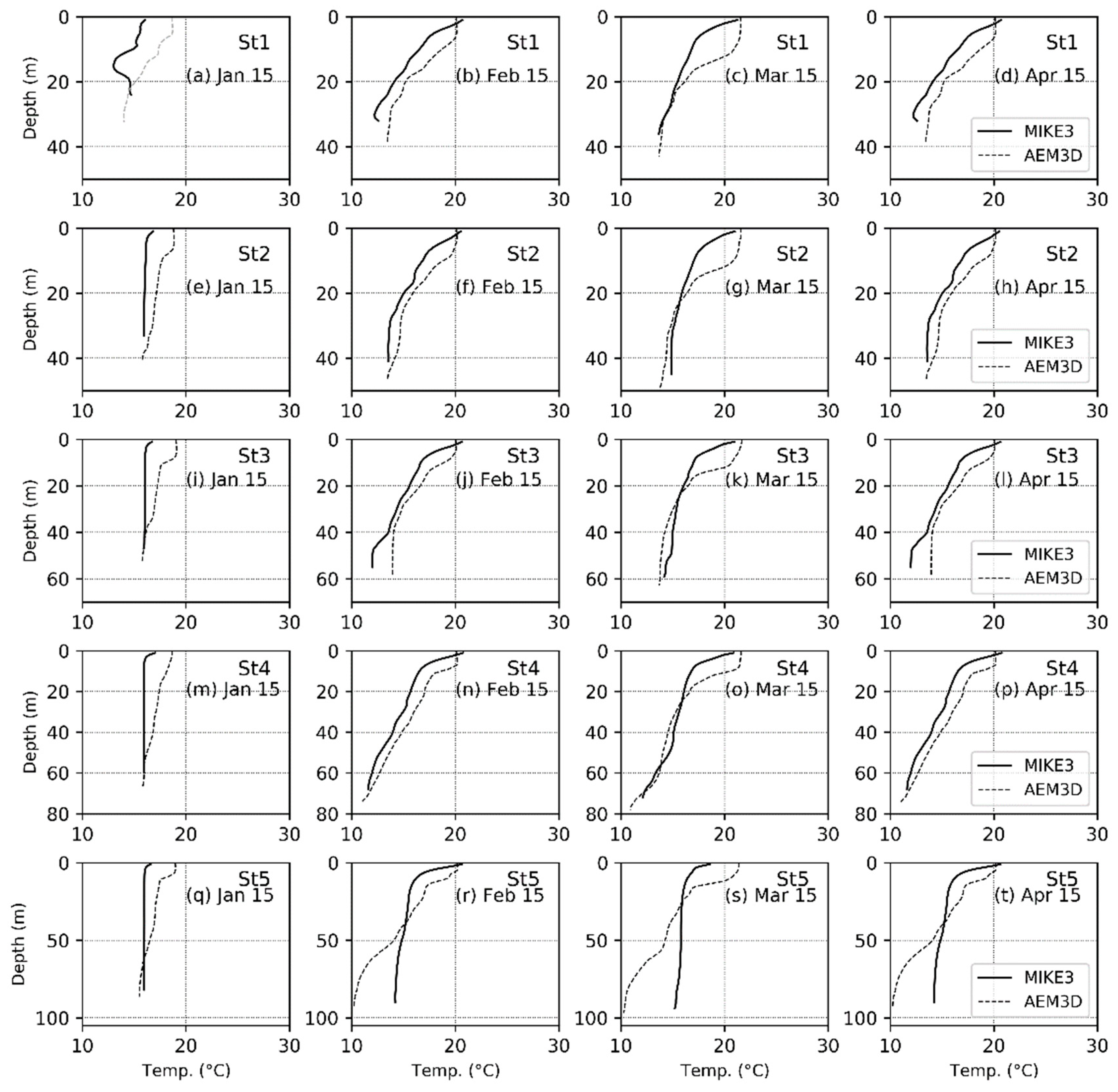
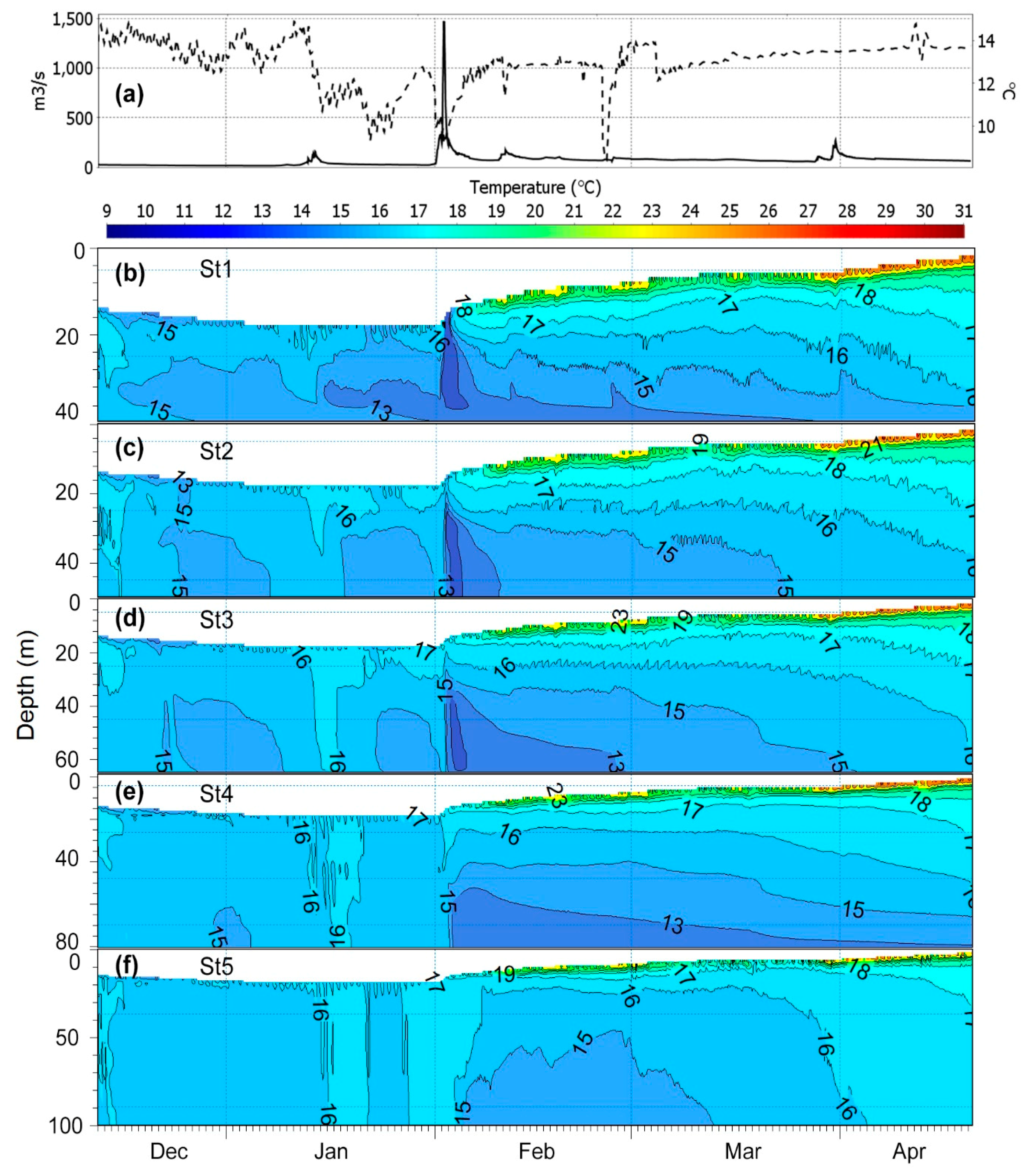
3.2. Water Column Profiles
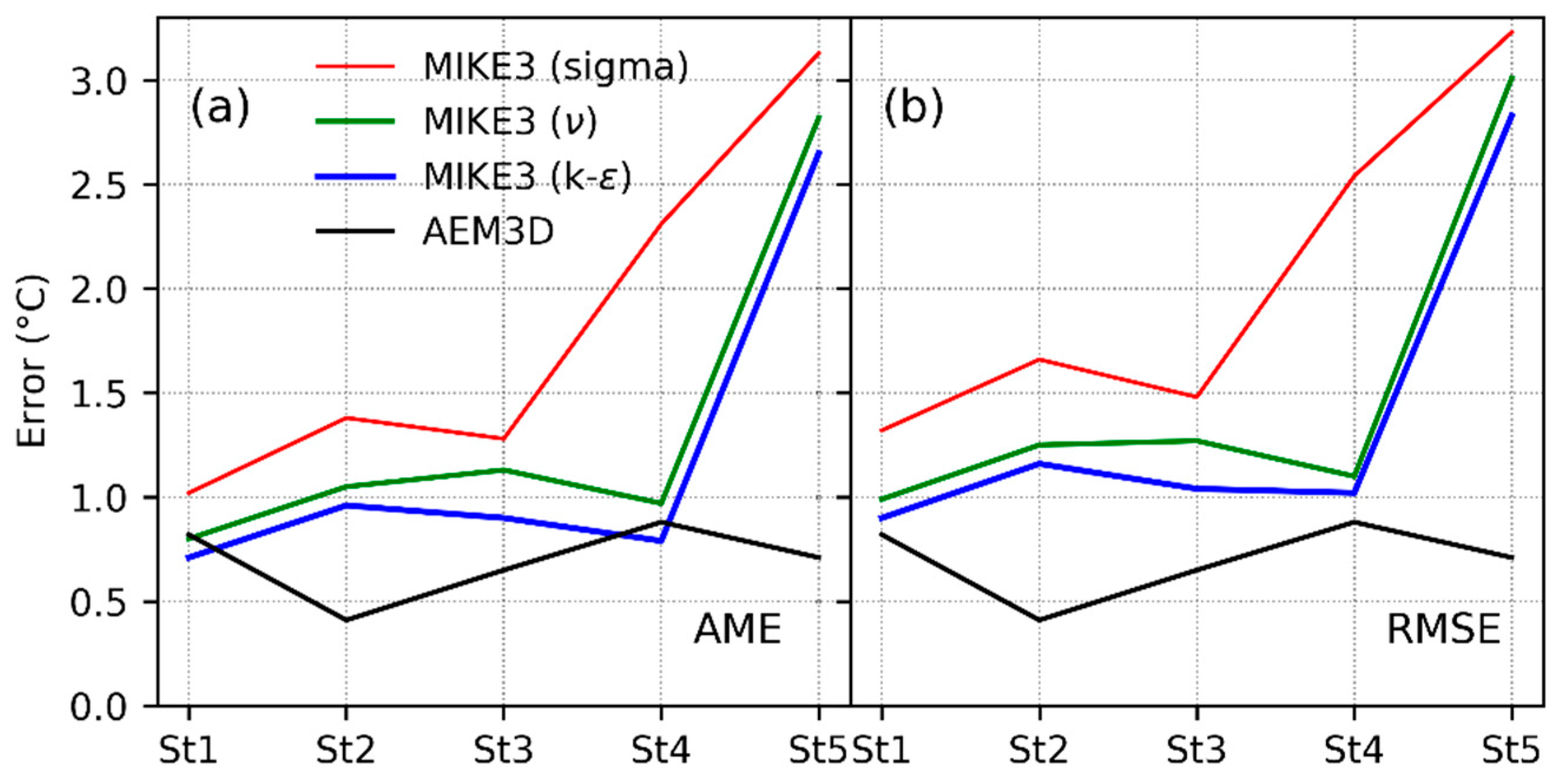
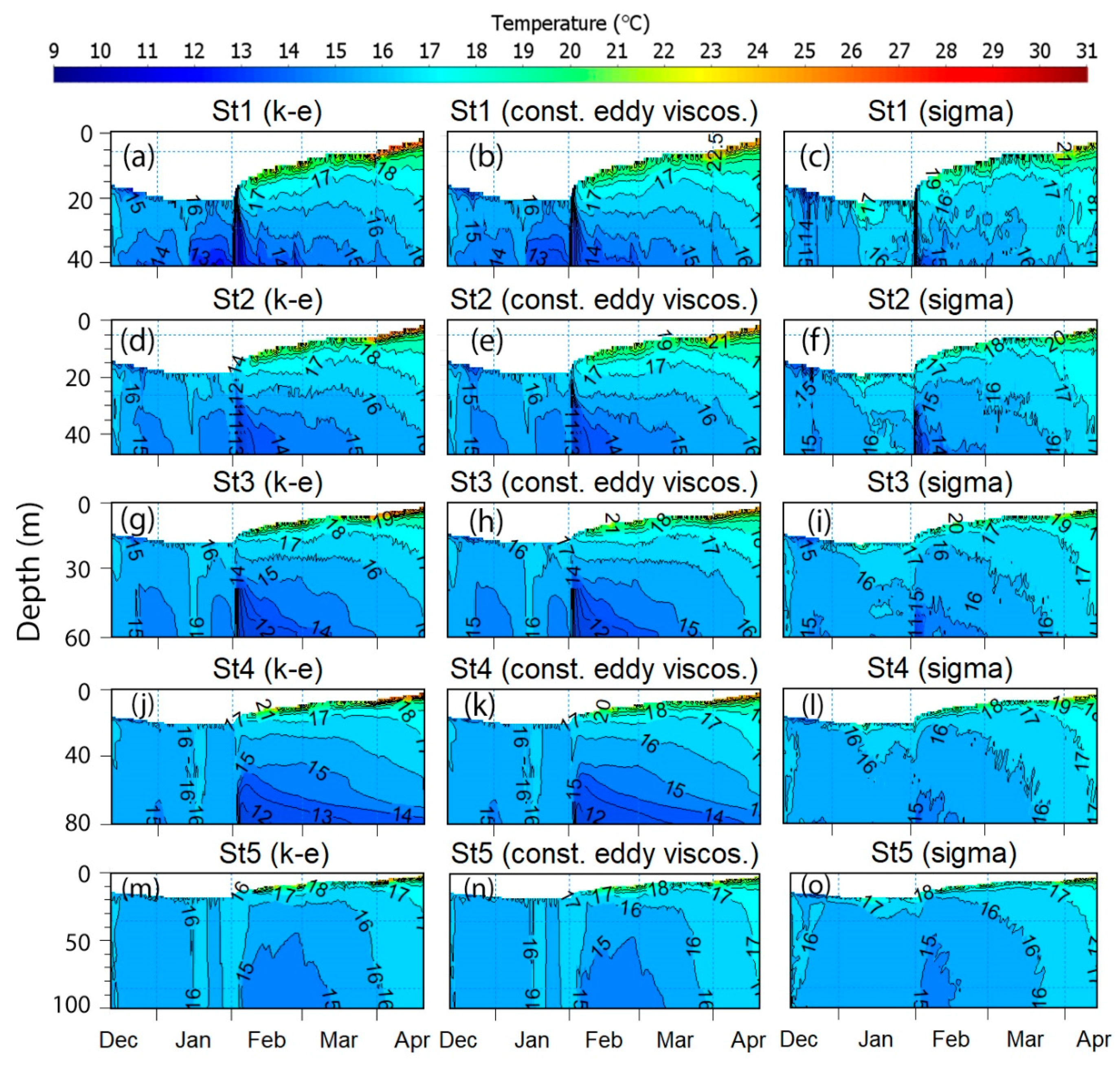
3.3. General Error Discussion
3.3.1. Effect of Poor Grid-Size Resolution
3.3.2. Effect of Hydrostatic Model Assumption
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- USACE. Reservoir Water Quality Analysis, Engineer Manual; U.S. Army Corps of Engineers: Washington, DC, USA, 1987. [Google Scholar]
- Hamilton, D.P.; Schladow, S.G. Prediction of water quality in lakes and reservoirs: Part I-Model description. Ecol. Modell. 1997, 96, 91–110. [Google Scholar] [CrossRef]
- Schladow, S.G.; Hamilton, D.P. Prediction of water quality in lakes and reservoirs: Part II-Model calibration, sensitivity analysis and application. Ecol. Modell. 1997, 96, 111–123. [Google Scholar] [CrossRef]
- Löffler, H. The origin of lake basins. In The Lakes Handbook, 2nd ed.; O’Sullivan, P.E., Reynolds, C.S., Eds.; Blackwell Publishing: Oxford, UK, 2004; Volume 1, pp. 8–60. [Google Scholar]
- Ji, Z.-G. Hydrodynamics and Water Quality: Modeling Rivers, Lakes, and Estuaries, 1st ed.; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2008. [Google Scholar]
- Valerio, G.; Pilotti, M.; Marti, C.L.; Imberger, J. The structure of basin-scale internal waves in a stratified lake in response to Lake Bathymetry and wind spatial and temporal distribution: Lake Iseo, Italy. Limnol. Oceanogr. 2012, 57, 772–786. [Google Scholar] [CrossRef]
- Boegman, L.; Imberger, J.; Ivey, G.N.; Antenucci, J.P. High-frequency internal waves in large stratified lakes. Limnol. Oceanogr. 2003, 48, 895–919. [Google Scholar] [CrossRef]
- Marti, C.L.; Imberger, J. Dynamics of the benthic boundary layer in a strongly forced stratified lake. J. Hydrobiol. 2006, 568, 217. [Google Scholar] [CrossRef]
- Hodges, B.R.; Imberger, J.; Saggio, A.; Winters, K.B. Modeling basin-scale internal waves in a stratified lake. Limnol. Oceanogr. 2000, 45, 1603–1620. [Google Scholar] [CrossRef]
- Laval, B.; Imberger, J.; Hodges, B.R.; Stocker, R. Modeling circulation in lakes: Spatial and temporal variations. Limnol. Oceanogr. 2003, 48, 983–994. [Google Scholar] [CrossRef]
- Zamani, B.; Koch, M.; Hodges, B.R.; Fakheri-Fard, A. Pre-impoundment assessment of the limnological processes and eutrophication in a reservoir using three-dimensional modeling: Abolabbas reservoir, Iran. J. Appl. Water Eng. Res. 2018, 6, 48–61. [Google Scholar] [CrossRef]
- Pacanowski, R.C.; Dixon, K.W.; Rosati, A.J. The GFDL Modular Ocean Model Users Guide (Technical Report); GFDL Ocean Group NOAA/Geophysical Fluid Dynamics Laboratory: Princeton, NJ, USA, 1991.
- Cassulli, V.; Cheng, R.T. Semi-implicit finite difference methods for three-dimensional shallow water flow. Int. J. Numer. Methods 1992, 15, 629–648. [Google Scholar] [CrossRef]
- Hodges, B.R.; Dallimore, C. Aquatic Ecosystem Model: AEM3D, User Manual; Hydronumerics: Docklands, Australia, 2016. [Google Scholar]
- Phillips, N.A. A coordinate system having some special advantage for numerical forecasting. J. Meteorol. 1957, 14, 184–185. [Google Scholar] [CrossRef]
- Blumberg, A.F.; Mellor, G.L. A description of a three-dimensional coastal ocean circulation model. In Coastal Estuarine Science. 4. Three Dimensional Coastal Ocean Models; Heaps, N., Ed.; American Geophysical Union: Washington, DC, USA, 1987; pp. 1–16. [Google Scholar]
- Haidvogel, D.B.; Wilkin, J.L.; Young, R.E. A semi-spectral primitive equation ocean circulation model using vertical sigma and orthogonal curvilinear horizontal co-ordinates. J. Comput. Phys. 1991, 94, 151–185. [Google Scholar] [CrossRef]
- Hamrick, J.M. A Three-Dimensional Environmental Fluid Dynamics Computer Code: Theoretical and Computational Aspects. Special Report. No. 317; The College of William and Mary, Virginia Institute of Marine Science: Gloucester Point, VA, USA, 1991. [Google Scholar]
- DHI. MIKE3 Flow Model FM Hydrodynamic and Transport Module, Scientific Documentation; DHI Danish Hydraulic Institute: Hørsholm, Denmark, 2017. [Google Scholar]
- Pierrzak, J.; Jakobson, J.B.; Burchard, H.; Vested, H.J.; Petersen, O. A three-dimensional hydrostatic model for coastal and ocean modelling using a generalised topography following co-ordinate system. Ocean Modell. 2002, 4, 173–205. [Google Scholar] [CrossRef]
- Gerdes, R. A primitive equation ocean circulation model using a general vertical coordinate transformation: 2. Application to an overflow problem. J. Geophys. Res. 1993, 98, 14703–14726. [Google Scholar] [CrossRef]
- Bell, M.J. Vortex stretching and bottom torques in the Bryan-Cox ocean circulation model. J. Geophys. Res. 1999, 104, 23545–23563. [Google Scholar] [CrossRef]
- Bleck, R.; Boudra, D. Initial testing of a numerical ocean circulation model using a hybrid (quasi-isopycnic) vertical coordinate. J. Phys. Oceanogr. 1981, 11, 755–770. [Google Scholar] [CrossRef]
- Bleck, R.; Benjamin, S. Regional weather prediction with a model combining terrain-following and isentropic coordinates. Part I: Model description. Mon. Weather Rev. 1993, 121, 1770–1785. [Google Scholar] [CrossRef]
- Mellor, G.L.; Häkkinen, S.; Ezer, T.; Patchen, R. A generalization of a sigma coordinate ocean model and an intercomparison of model vertical grids. In Ocean Forecasting: Theory and Practice; Pinardi, N., Ed.; Springer: Berlin, Germany, 1999. [Google Scholar]
- Burchard, H.; Petersen, O. Hybridization between σ- and z-co-ordinates for improving the internal pressure gradient calculation in marine models with steep bottom slopes. Int. J. Numer. Methods Fluids 1997, 25, 1003–1023. [Google Scholar] [CrossRef]
- Zhang, Y.J.; Ateljevich, E.; Yu, H.; Wu, C.H.; Yu, J.C.S. A new vertical coordinate system for a 3D unstructured-grid model. Ocean Modell. 2015, 85, 16–31. [Google Scholar] [CrossRef]
- Craig, P.M.; Chung, D.H.; Lam, N.T.; Son, P.H.; Tinh, N.X. Sigma-zed: A computationally efficient approach to reduce the horizontal gradient error in the EFDC’s vertical sigma grid. In Proceedings of the 11th International Conference on Hydrodynamics (ICHD 2014), Singapore, 19–24 October 2014. [Google Scholar]
- Ford, D.E.; Johnson, L.S. An Assessment of Reservoir Mixing Processes, Technical Report for US Army Corps of Engineers; Ford Thornton Norton and associates Ltd.: Little Rock, AR, USA, 1986. [Google Scholar]
- Hutchinson, G.E.; Löffler, H. The thermal classification of lakes. Proc. Natl. Acad. Sci. USA 1956, 42, 84–86. [Google Scholar] [CrossRef]
- Wüest, A.; Lorke, A. Small-scale hydrodynamics in lakes. Annu. Rev. Fluid Mech. 2003, 35, 373–412. [Google Scholar] [CrossRef]
- Karandish, F.; Porhemmat, J.; Ebrahimi, K. Determination of the main snowmelt parameters in estimating flood hydrograph in Karun basin. Iran Water Res. J. 2016, 10, 133–142. [Google Scholar]
- Allen, R.G.; Pereira, L.S.; Raes, D.; Smith, M. Crop Evapotranspiration – Guidelines for Computing Crop Water Requirements. FAO Irrigation and Drainage Paper 56; Food and Agriculture Organization of the United Nations (FAO): Rome, Italy, 1998. [Google Scholar]
- Kamali, G.; Moradi, E. Solar Radiation (Principles and Applications in Agriculture and New Energies); Atmospheric Science and Meteorological Research Center: Tehran, Iran, 2006. (In Persian) [Google Scholar]
- Alizadeh, A.; Khalili, N. Estimation of Angstrom coefficient and developing a regression equation for solar radiation estimation (case study: Mashhad). J. Water Soil 2009, 23, 229–238. (In Persian) [Google Scholar]
- Yang, K.; Koike, T. A general model to estimate hourly and daily solar radiation for hydrological studies. Water Resour. Res. 2005, 41, W10403. [Google Scholar] [CrossRef]
- Hajjam, S.; Jamei, M. Estimation of solar radiation parameter (sunshine hours) and Angstrom coefficients in Khuzestan Province. In Proceedings of the 10th National Conference of Irrigation and Evaporation Reduction, Kerman, Iran, 8 February 2009. (In Persian). [Google Scholar]
- Mehrabani, R.; Makvandi, A.; Nekouyanfar, M.; Hassounizadeh, H. Tracing and measurement of turbidity current in maroon dam. In Proceedings of the 9th International River Engineering Conference, Ahwaz, Iran, 13–15 February 2013. [Google Scholar]
- Hodges, B.R. Numerical Tecniques in CWR-ELCOM, Technical Report; Center for Water Research, The Univerity of Western Australia: Nedlands, Australia, 2000. [Google Scholar]
- Hipsey, M.R.; Romero, J.R.; Antenucci, J.J.; Hamilton, D. Computational Aquatic Ecosystem Dynamics Model: CAEDYM v3.2, Science Manual; Centre for Water Research, University of Western Australia: Nedlands, Australia, 2006. [Google Scholar]
- Arakawa, A.; Lamb, V.R. Computational design of the basic dynamical processes of the UCLA general circulation model In General Circulation Models of the Atmosphere. Methods Comput. Phys. 1977, 17, 173–265. [Google Scholar]
- Imberger, J.; Patterson, J.C. A dynamic reservoir simulation model—DYRESM: 5. In Transport Models for Inland and Coastal Waters; Fischer, H.B., Ed.; Academic Press: Cambridge, MA, USA, 1981; pp. 310–361. [Google Scholar]
- Imberger, J.; Patterson, J.C. Physical limnology. Adv. Appl. Mech. 1990, 27, 303–475. [Google Scholar]
- Spigel, R.H.; Imberger, J. The classification of mixed layer dynamics in lakes of small to medium size. J. Phys. Oceanogr. 1980, 10, 1104–1121. [Google Scholar] [CrossRef]
- Haney, R.L. On the pressure gradient force over steep topography in sigma co-ordinate ocean models. J. Phys. Oceanogr. 1991, 21, 610–619. [Google Scholar] [CrossRef]
- Mellor, G.L.; Ezer, T.; Oey, L. The Pressure Gradient Conundrum of Sigma Coordinate Ocean Models. J. Atmos. Ocean. Technol. 1994, 11, 1126–1134. [Google Scholar] [CrossRef]
- Rodi, W. Turbulence Models and Their Applications in Hydraulics. IAHR Monograph, 2nd ed.; CRC Press: Boca Raton, FL, USA, 1984. [Google Scholar]
- Lange, X. Numerical Simulations of Estuarine Circulation in a Non-Tidal Estuary. Master’s Thesis, University of Rostock, Rostock, Germany, 2015. [Google Scholar]
- Sikirić, M.D.; Janeković, I.; Kuzmić, M. A new approach to bathymetry smoothing in sigma-coordinate ocean models. Ocean Modell. 2009, 29, 128–136. [Google Scholar] [CrossRef]
- Chung, S.W.; Hipsey, M.R.; Imberger, J. Modelling the propagation of turbid density inflows into a stratified lake: Daecheong Reservoir, Korea. Environ. Modell. Softw. 2009, 24, 1467–1482. [Google Scholar] [CrossRef]
- Marti, C.L.; Imberger, J.; Garibaldi, L.; Leoni, B. Using time scales to characterize phytoplankton assemblages in a deep subalpine lake during the thermal stratification period: Lake Iseo, Italy. Water Resour. Res. 2016, 52, 1762–1780. [Google Scholar] [CrossRef]
- Lee, H.W.; Kim, E.J.; Park, S.S.; Choi, J.H. Effects of Climate Change on the Movement of Turbidity Flow in a Stratified Reservoir. Water Resour. Manag. 2015, 29, 4095–4110. [Google Scholar] [CrossRef]
- Hodges, B.R.; Laval, B.; Wadzuk, B.M. Numerical error assessment and a temporal horizon for internal waves in a hydrostatic model. Ocean Modell. 2006, 13, 44–64. [Google Scholar] [CrossRef]
- Lindim Fontes, C. Modelling of Water Quality in the Alqueva Reservoir, Portugal. Ph.D. Thesis, University of Minho, Braga, Portugal, 2010. [Google Scholar]
- Ji, Z.G.; Hu, G.; Shen, J.; Wan, Y. Three-dimensional modeling of hydrodynamic processes in the St. Lucie Estuary. Estuar. Coast. Shelf Sci. 2007, 73, 188–200. [Google Scholar] [CrossRef]
- Launder, B.; Spalding, D. Mathematical Models of Turbulence; Academic Press: Cambridge, MA, USA, 1972. [Google Scholar]
- Gary, J.M. Estimate of truncation error in transformed co-ordinate, primitive equation atmospheric models. J. Atmos. Sci. 1973, 30, 223–233. [Google Scholar] [CrossRef][Green Version]
- Salimi-Nasab, S.; Espandar, R.; Zandi, R. Assessment of thermal stratification and oxygen distribution in Karkheh dam reservoir. In Proceedings of the 2nd Iranian National Conference on Dams and Hydropower, Tehran, Iran, 14 May 2008. [Google Scholar]
- Paturi, S.; Boegman, L.; Yerubandi, R.R. Hydrodynamics of eastern Lake Ontario and the upper St. Lawrence River. J. Great Lakes Res. 2012, 38 (Suppl. 4), 194–204. [Google Scholar] [CrossRef]
- Martin, P.J. Simulation of the mixed layer at OWS November and Papa with several models. J. Geophys. Res. 1985, 90, 903–916. [Google Scholar] [CrossRef]


| Parameter | Description | Unit | Value |
|---|---|---|---|
| CD | Bottom drag coefficient | - | 0.05 |
| λPAR | Light extinction coefficient | m−1 | 0.25 |
| αsw | Mean albedo for short-wave radiation | - | 0.08 |
| αlw | Mean albedo for long-wave radiation | - | 0.03 |
| Cd | Wind stress coefficient | - | 0.0013 |
| CH/S | Bulk-transfer constant for surface scalar fluxes | - | 0.013 |
| Parameter | Description | Unit | Value |
|---|---|---|---|
| Vertical eddy viscosity of k-ε model | m2 s−1 | 1 × 10−6 to 1 × 10−5 | |
| Horizontal eddy viscosity | m2 s−1 | 0.002 | |
| Wind stress coefficient | - | 0.0013 | |
| Bottom drag coefficient (bed resistance) | - | 0.055 | |
| Horizontal diffusion coefficients | - | 0.002, 0.0015 | |
| Vertical diffusion coefficient | - | 1.1 × 10−6 |
| Model | Station | Mean (obs) (°C) | Mean (sim) (°C) | AME (°C) | RMSE (°C) |
|---|---|---|---|---|---|
| AEM3D | St1 | 14.27 | 14.96 | 0.82 | 0.97 |
| St2 | 14.57 | 14.48 | 0.41 | 0.71 | |
| St3 | 14.59 | 14.97 | 0.65 | 0.81 | |
| St4 | 14.19 | 13.98 | 0.88 | 1.07 | |
| St5 | 13.05 | 13.08 | 0.71 | 0.91 | |
| Mean | 0.70 | 0.89 | |||
| MIKE3 z-sigma k-ε | St1 | 14.27 | 14.20 | 0.71 | 0.90 |
| St2 | 14.57 | 14.60 | 0.96 | 1.16 | |
| St3 | 14.59 | 14.85 | 0.90 | 1.04 | |
| St4 | 14.19 | 13.87 | 0.79 | 1.02 | |
| St5 | 13.05 | 15.20 | 2.65 | 2.83 | |
| Mean | 1.20 | 1.39 | |||
| MIKE3 z-sigma eddy viscosity | St1 | 14.27 | 14.43 | 0.80 | 0.99 |
| St2 | 14.57 | 15.09 | 1.05 | 1.25 | |
| St3 | 14.59 | 15.22 | 1.13 | 1.27 | |
| St4 | 14.19 | 14.10 | 0.97 | 1.10 | |
| St5 | 13.05 | 15.32 | 2.82 | 3.01 | |
| Mean | 1.35 | 1.52 | |||
| MIKE3 sigma k-ε | St1 | 14.27 | 15.29 | 1.02 | 1.32 |
| St2 | 14.57 | 15.59 | 1.38 | 1.66 | |
| St3 | 14.59 | 15.21 | 1.28 | 1.48 | |
| St4 | 14.19 | 15.74 | 2.31 | 2.54 | |
| St5 | 13.05 | 15.79 | 3.13 | 3.23 | |
| Mean | 1.83 | 2.05 |
| Station | AEM3D | MIKE3 z-sigma k-ε | MIKE3 z-sigma eddy viscosity | MIKE3 sigma k-ε |
|---|---|---|---|---|
| St1 | 0.90 | 0.88 | 0.88 | 0.86 |
| St2 | 0.79 | 0.72 | 0.76 | 0.84 |
| St3 | 0.79 | 0.80 | 0.81 | 0.77 |
| St4 | 0.72 | 0.68 | 0.70 | 0.64 |
| St5 | 0.62 | 0.59 | 0.57 | 0.29 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zamani, B.; Koch, M. Comparison Between Two Hydrodynamic Models in Simulating Physical Processes of a Reservoir with Complex Morphology: Maroon Reservoir. Water 2020, 12, 814. https://doi.org/10.3390/w12030814
Zamani B, Koch M. Comparison Between Two Hydrodynamic Models in Simulating Physical Processes of a Reservoir with Complex Morphology: Maroon Reservoir. Water. 2020; 12(3):814. https://doi.org/10.3390/w12030814
Chicago/Turabian StyleZamani, Behnam, and Manfred Koch. 2020. "Comparison Between Two Hydrodynamic Models in Simulating Physical Processes of a Reservoir with Complex Morphology: Maroon Reservoir" Water 12, no. 3: 814. https://doi.org/10.3390/w12030814
APA StyleZamani, B., & Koch, M. (2020). Comparison Between Two Hydrodynamic Models in Simulating Physical Processes of a Reservoir with Complex Morphology: Maroon Reservoir. Water, 12(3), 814. https://doi.org/10.3390/w12030814





