Fish Response to Turbulence Generated Using Multiple Randomly Actuated Synthetic Jet Arrays
Abstract
1. Introduction
1.1. Background
1.2. Randomly Actuated Synthetic Jet Arrays
1.3. Aims and Objectives
2. Methods
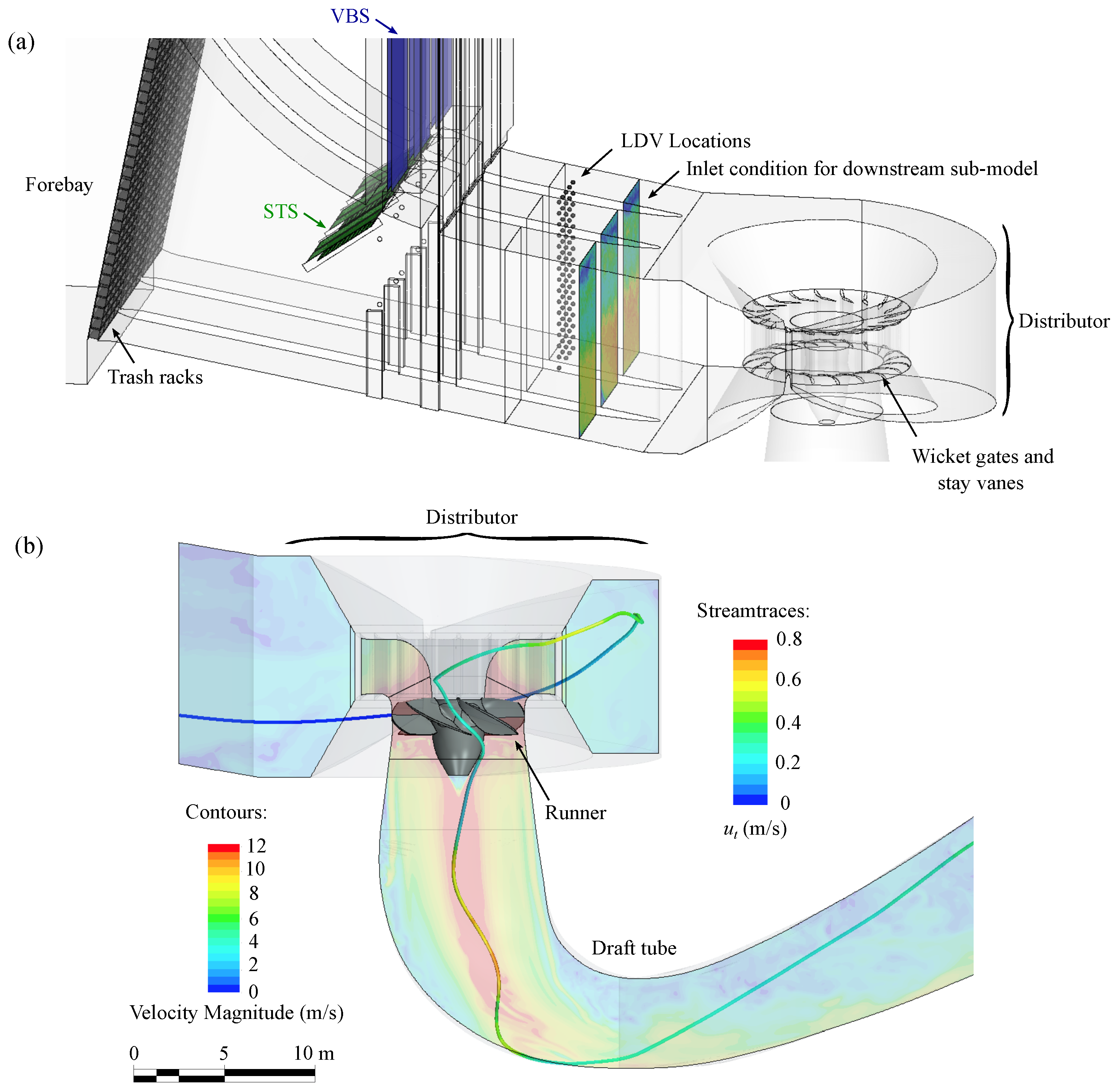
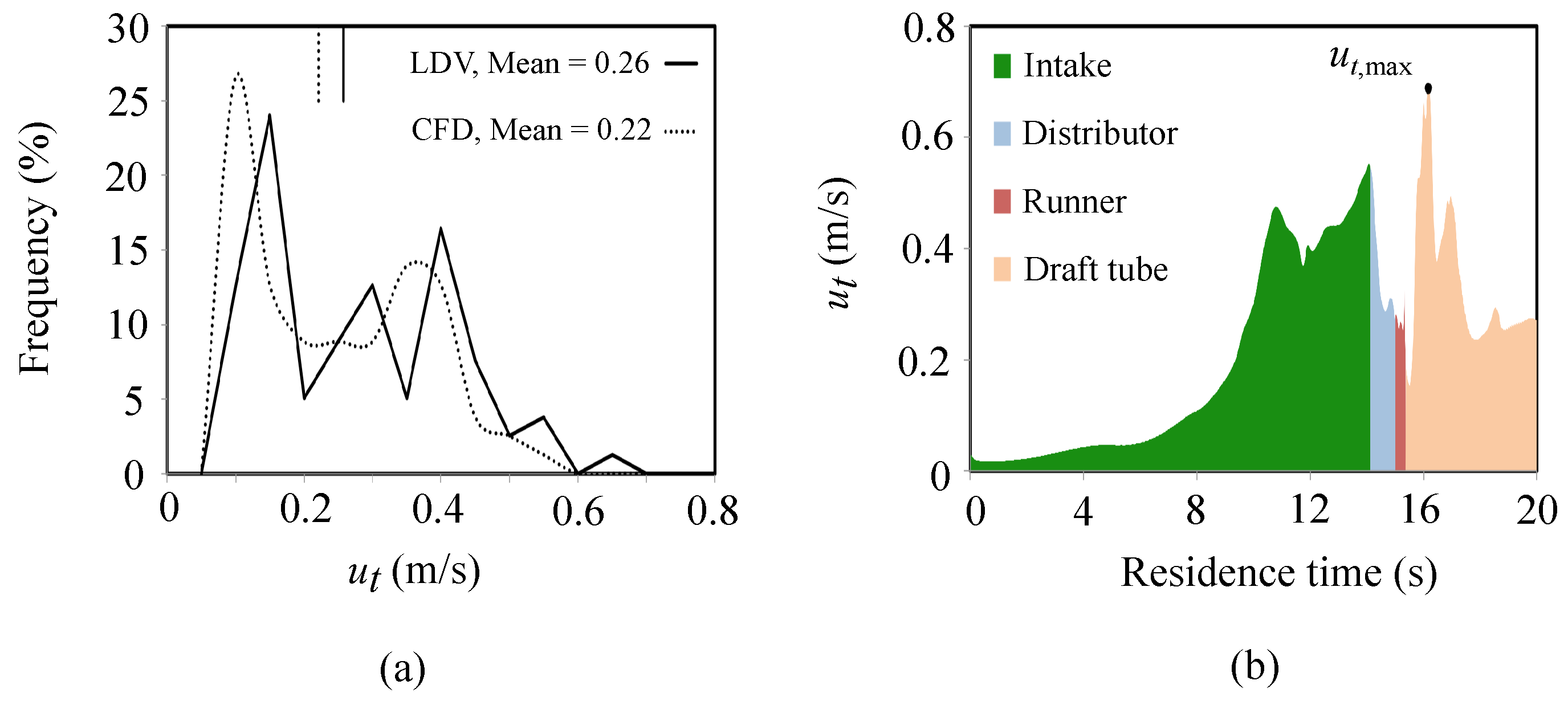
2.1. Estimation of Fish Exposure to Turbulence During Dam Passage
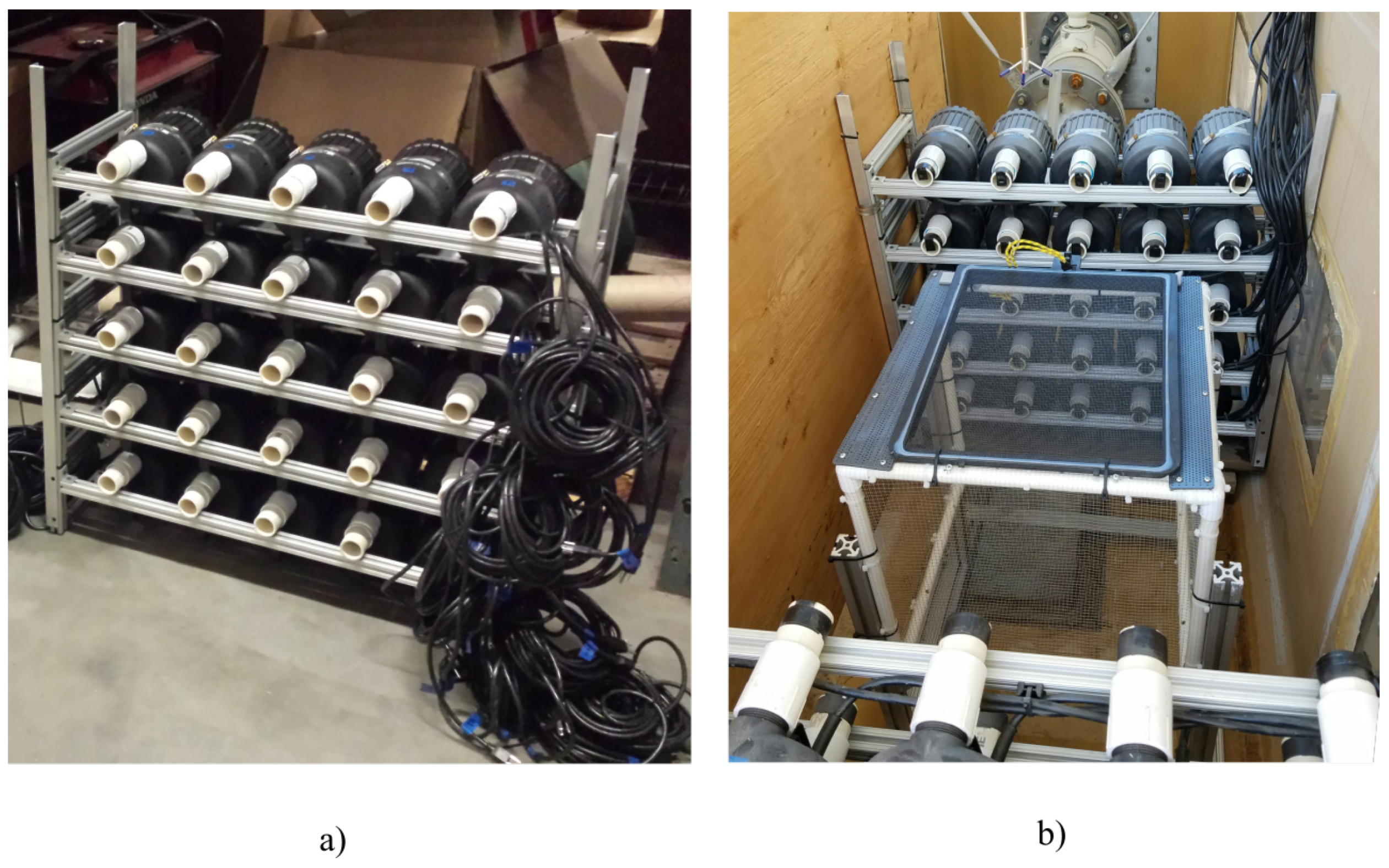
2.2. Development of the RASJA Test Facility
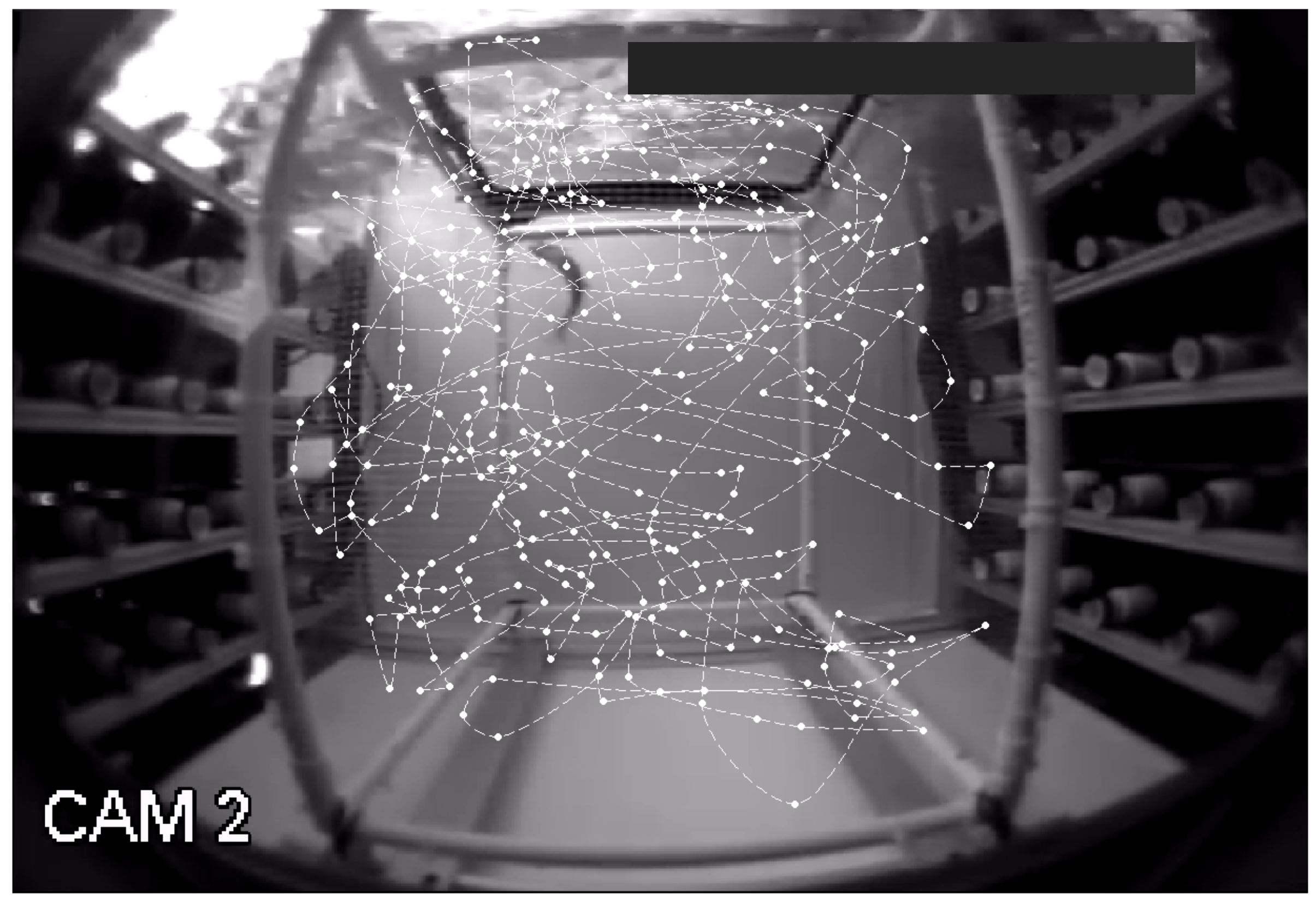
2.3. Fish Testing
3. Results
3.1. Velocity Characterization
3.1.1. Effects of Pump Utilization
3.1.2. Spatial Characterization of Selected Pump Settings
3.2. Biological Response
4. Discussion
5. Future Work
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Abbreviations
| ADV | acoustic Doppler velocimeter |
| ARL | Aquatic Research Laboratory |
| CFD | computational fluid dynamics |
| cfs | cubic feet per second |
| hp | horse-power |
| L | liter(s) |
| LDV | laser Doppler velocimeter |
| MW | megawatt(s) |
| PNNL | Pacific Northwest National Laboratory |
| PVC | polyvinyl chloride |
| RASJA | randomly actuated synthetic jet array |
| RMS | root-mean square |
| STS | submersible traveling screen |
| USACE | U.S. Army Corps of Engineers |
| VBS | vertical barrier screen |
Appendix A. Computational Estimate of Turbulence Exposure During Dam Passage
References
- Therrien, J.; Bourgeois, G. Fish Passage at at Small Hydro Sites; Technical Report; Genivar Consulting Group for CANMET Energy Technology Centre: Ottawa, ON, Canada, 2000. [Google Scholar]
- Čada, G.F. The development of Advanced Hydroelectric Turbines to Improve Fish Passage Survival. Fisheries 2001, 26, 14–23. [Google Scholar] [CrossRef]
- Lacey, R.; Neary, V.S.; Liao, J.C.; Enders, E.; Tritico, H.M. The IPOS framework: Linking fish swimming performance in altered flows from laboratory experiments to rivers. River Res. Appl. 2012, 28, 429–443. [Google Scholar] [CrossRef]
- Odeh, M.; Noreika, J.F.; Haro, A.; Maynard, A.; Castro-Santos, T.; Cada, G.F. Evaluation of the Effects of Turbulence on the Behaviour of Migratory Fish; Final Report 2002, Report to Bonneville Power Administration, Contact No. 00000022, Project No. 200005700; Oak Ridge National Laboratory (ORNL) and US Geological Survey (USGS): Oak Ridge, TN, USA; Reston, VA, USA, 2002; p. 55.
- Neitzel, D.A.; Dauble, D.D.; Cada, G.F.; Richmond, M.C.; Guensch, G.R.; Mueller, R.R.; Abernethy, C.S.; Amidan, B. Survival estimates for juvenile fish subjected to a laboratory-generated shear environment. Trans. Am. Fish. Soc. 2004, 133, 447–454. [Google Scholar] [CrossRef]
- Silva, A.; Santos, J.; Ferreira, M.; Pinheiro, A.; Katopodis, C. Effects of water velocity and turbulence on the behaviour of Iberian Barbel (Luciobarbus bocagei, Steindachner 1864) in an experimental pool-type fishway. River Res. Appl. 2011, 27, 360–373. [Google Scholar] [CrossRef]
- Trinci, G.; Harvey, G.L.; Henshaw, A.J.; Bertoldi, W.; Hölker, F. Life in turbulent flows: Interactions between hydrodynamics and aquatic organisms in rivers. Wiley Interdiscip. Rev. Water 2017, 4, 1–16. [Google Scholar] [CrossRef]
- Ryon, M.G.; Cada, G.F.; Smith, J.G. Further Tests of Changes in Fish Escape Behavior Resulting from Sublethal Stresses Associated with Hydroelectric Turbine Passage; Technical Report; Oakridge National Laboratory: Oak Ridge, TN, USA, 2004. [CrossRef]
- Roach, P. The generation of nearly isotropic turbulence by means of grids. Int. J. Heat Fluid Flow 1987, 8, 82–92. [Google Scholar] [CrossRef]
- Cheng, N.; Law, A. Measurement of turbulence generated by oscillating grid. J. Hydraul. Eng. 2001, 127, 201–208. [Google Scholar] [CrossRef]
- Srdic, A.; Fernando, H.; Montenegro, L. Generation of nearly isotropic turbulence using two oscillating grids. Exp. Fluids 1996, 20, 395–397. [Google Scholar] [CrossRef]
- Stiansen, J.; Sundby, S. Improved methods for generating and estimating turbulence in tanks suitable for fish larvae experiments. Sci. Mar. 2001, 65, 151–167. [Google Scholar] [CrossRef]
- Knebel, P.; Kittel, A.; Peinke, J. Atmospheric wind field conditions generated by active grids. Exp. Fluids 2011, 51, 471–481. [Google Scholar] [CrossRef]
- Makita, H. Realization of a large-scale turbulence field in a small wind tunnel. Fluid Dyn. Res. 1991, 8, 53–64. [Google Scholar]
- Mydlarski, L.; Warhaft, Z. On the onset of high-Reynolds-number grid-generated wind tunnel turbulence. J. Fluid Mech. 1996, 320, 31–68. [Google Scholar] [CrossRef]
- Maia, A.; Sheltzer, A.; Tytell, E. Streamwise vortices destabilize swimming bluegill sunfish (Lepomis macrochirus). J. Exp. Biol. 2015, 218, 786–792. [Google Scholar] [CrossRef]
- Variano, E.A.; Bodenschatz, E.; Cowen, E.A. A random synthetic jet array driven turbulence tank. Exp. Fluids 2004, 37, 613–615. [Google Scholar] [CrossRef]
- Variano, E.A.; Cowen, E.A. A random-jet-stirred turbulence tank. J. Fluid Mech. 2008, 604, 1–32. [Google Scholar] [CrossRef]
- Bellani, G.; Variano, E.A. Homogeneity and isotropy in a laboratory turbulent flow. Exp. Fluids 2014, 55, 1646. [Google Scholar] [CrossRef]
- Romero-Gomez, P.; Richmond, M.C. Movement and collision of Lagrangian particles in hydro-turbine intakes: A case study. J. Hydraul. Res. 2017, 55, 706–720. [Google Scholar] [CrossRef]
- Harding, S.; Romero-Gomez, P.; Richmond, M. Performance of Virtual Current Meters in Hydroelectric Turbine Intakes; Technical Report; Pacific Northwest National Laboratory: Richland, WA, USA, 2016.
- CD-adapco. User Guide, STAR-CCM+ Version 10.06; CD-adapco; Siemens PLM Software: Plano, TX, USA, 2015. [Google Scholar]
- Hurther, D.; Lemmin, U. A correction method for turbulence measurements with a 3D acoustic Doppler velocity profiler. J. Atmos. Ocean. Technol. 2001, 18, 446–458. [Google Scholar] [CrossRef]
- Nortek, A.S. Vector Current Meter User Manual; Technical Report August; Nortek AS: Rud, Norway, 2005. [Google Scholar]
- Rusello, P.; Lohrmann, A.; Siegel, E.; Maddux, T. Improvements in acoustic Doppler velocimetery. In Proceedings of the 7th International Conference in Hydroscience and Engineering (ICHE 2006), Philadelphia, PA, USA, 10–13 September 2006. [Google Scholar]
- Mori, N. MACE Toolbox. Available online: http://www.oceanwave.jp/softwares/mace (accessed on 19 August 2017).
- Mori, N.; Suzuki, T.; Kakuno, S. Noise of acoustic Doppler velocimeter data in bubbly flow. ASCE J. Eng. Mech. 2007, 133, 122–125. [Google Scholar] [CrossRef]
- Cotal, A.; Webb, P.; Tritico, H. Do brown trout choose locations with reduced turbulence? Trans. Am. Fish. Soc. 2006, 135, 610–619. [Google Scholar] [CrossRef]
- Higham, T.; Steward, W.; Wainwright, P. Turbulence, temperature, and turbidity: The ecomechanics of predator-prey interactions in fishes. Integr. Comp. Biol. 2015, 55, 6–20. [Google Scholar] [CrossRef]
- Lupandin, A. Effect of flow turbulence on swimming speed of fish. Biol. Bull. 2005, 32, 461–466. [Google Scholar] [CrossRef]
- Deng, Z.; Carlson, T.J.; Duncan, J.P.; Richmond, M.C. Six-degree-of-freedom sensor fish design and instrumentation. Sensors 2007, 7, 3399–3415. [Google Scholar] [CrossRef]
- Carlson, T.; Duncan, J.; Deng, Z. Data Overview for Sensor Fish Samples Acquired at Ice Harbor, John Day, and Bonneville II Dams in 2005, 2006, and 2007; Technical Report; Pacific Northwest National Laboratory: Richland, WA, USA, 2008.
- Romero-Gomez, P.; Harding, S.; Richmond, M. The effects of sampling location and turbulence on discharge estimates in short converging turbine intakes. Eng. Appl. Comput. Fluid Mech. 2017, 11, 513–525. [Google Scholar] [CrossRef]
- Spalart, P. Detached-Eddy simulation. Annu. Rev. Fluid Mech. 2009, 41, 181–202. [Google Scholar] [CrossRef]
- Coutant, C.C.; Whitney, R.R. Fish behavior in relation to passage through hydropower turbines: A review. Trans. Am. Fish. Soc. 2000, 129, 351–380. [Google Scholar] [CrossRef]
- Moursund, R.; Carlson, T. Turbine Imaging Technology Assessment; Technical Report; Pacific Northwest National Laboratory: Richland, WA, USA, 2004.
- Weiland, M.; Mueller, R.; Carlson, T.; Deng, Z.; McKinstry, C. Characterization of Bead Trajectories through the Draft Tube of a Turbine Physical Model; Technical Report; Pacific Northwest National Laboratory: Richland, WA, USA, 2005.
- Puckett, K.; Dill, L. Cost of sustained and burst swimming to juvenile Coho Salmon (Oncorhynchus kisutch). Can. J. Fish. Aquat. Sci. 1984, 41, 1546–1551. [Google Scholar] [CrossRef]
- Brown, M.L.; Parsheh, M.; Aidun, C.K. Turbulent flow in a converging channel: Effect of contraction and return to isotropy. J. Fluid Mech. 2006, 560, 437–448. [Google Scholar] [CrossRef]


| Filter | Threshold | % of Data Rejected |
|---|---|---|
| Correlation threshold | 70% | 33.3 |
| Signal-to-noise ratio threshold | 20 dB | 0 |
| Phase space filtering | - | 0.34 |
| Centroid | Survey Mean | Survey STD | Survey Range | |
|---|---|---|---|---|
| () | () | () | () | |
| U (mm/s) | 151.6 | 438 | ||
| V (mm/s) | 0.8 | 44.1 | 192 | |
| W (mm/s) | 31.3 | 4.3 | 31.0 | 126 |
| (mm/s) | 341.5 | 335.5 | 26.7 | 105 |
| (mm/s) | 183.7 | 190.0 | 8.6 | 35 |
| (mm/s) | 155.2 | 167.0 | 6.9 | 31 |
| (mm/s) | 241.1 | 242.8 | 12.7 | 50 |
| k (m2/s2) | 0.0872 | 0.0887 | 0.0091 | 0.036 |
| Exposure Duration | 2 min | 2 min | 10 min | 10 min |
|---|---|---|---|---|
| Exposure Condition | Turbulence | Control | Turbulence | Control |
| Sample size | 50 | 50 | 49 | 50 |
| Median fork length (mm) | 77 | 74 | 75 | 74 |
| Mean fish mass (g) | 4.9 | 4.6 | 4.9 | 4.7 |
| Time to Reorient in Steady Flow (s): | ||||
| <5 | 45 (90%) | 50 (100%) | 36 (74%) | 47 (94%) |
| 5 | 2 (4%) | 0 (0%) | 7 (14%) | 0 (0%) |
| 10 | 3 (6%) | 0 (0%) | 5 (10%) | 3 (6%) |
| 15 | 0 (0%) | 0 (0%) | 1 (2%) | 0 (0%) |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Harding, S.F.; Mueller, R.P.; Richmond, M.C.; Romero-Gomez, P.; Colotelo, A.H. Fish Response to Turbulence Generated Using Multiple Randomly Actuated Synthetic Jet Arrays. Water 2019, 11, 1715. https://doi.org/10.3390/w11081715
Harding SF, Mueller RP, Richmond MC, Romero-Gomez P, Colotelo AH. Fish Response to Turbulence Generated Using Multiple Randomly Actuated Synthetic Jet Arrays. Water. 2019; 11(8):1715. https://doi.org/10.3390/w11081715
Chicago/Turabian StyleHarding, Samuel F., Robert P. Mueller, Marshall C. Richmond, Pedro Romero-Gomez, and Alison H. Colotelo. 2019. "Fish Response to Turbulence Generated Using Multiple Randomly Actuated Synthetic Jet Arrays" Water 11, no. 8: 1715. https://doi.org/10.3390/w11081715
APA StyleHarding, S. F., Mueller, R. P., Richmond, M. C., Romero-Gomez, P., & Colotelo, A. H. (2019). Fish Response to Turbulence Generated Using Multiple Randomly Actuated Synthetic Jet Arrays. Water, 11(8), 1715. https://doi.org/10.3390/w11081715





