Hydraulic Parameters for Sediment Transport and Prediction of Suspended Sediment for Kali Gandaki River Basin, Himalaya, Nepal
Abstract
1. Introduction
2. Materials and Methods
2.1. Study Site Description
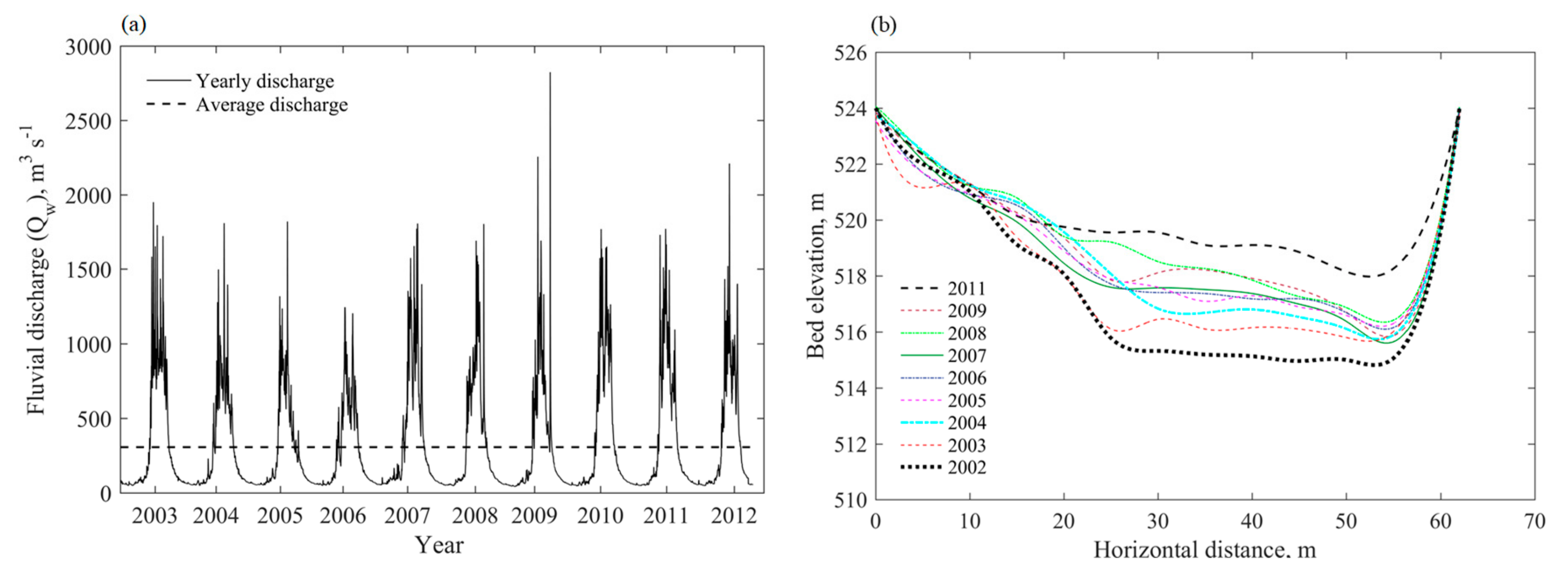
2.2. Data Collection and Acquisition
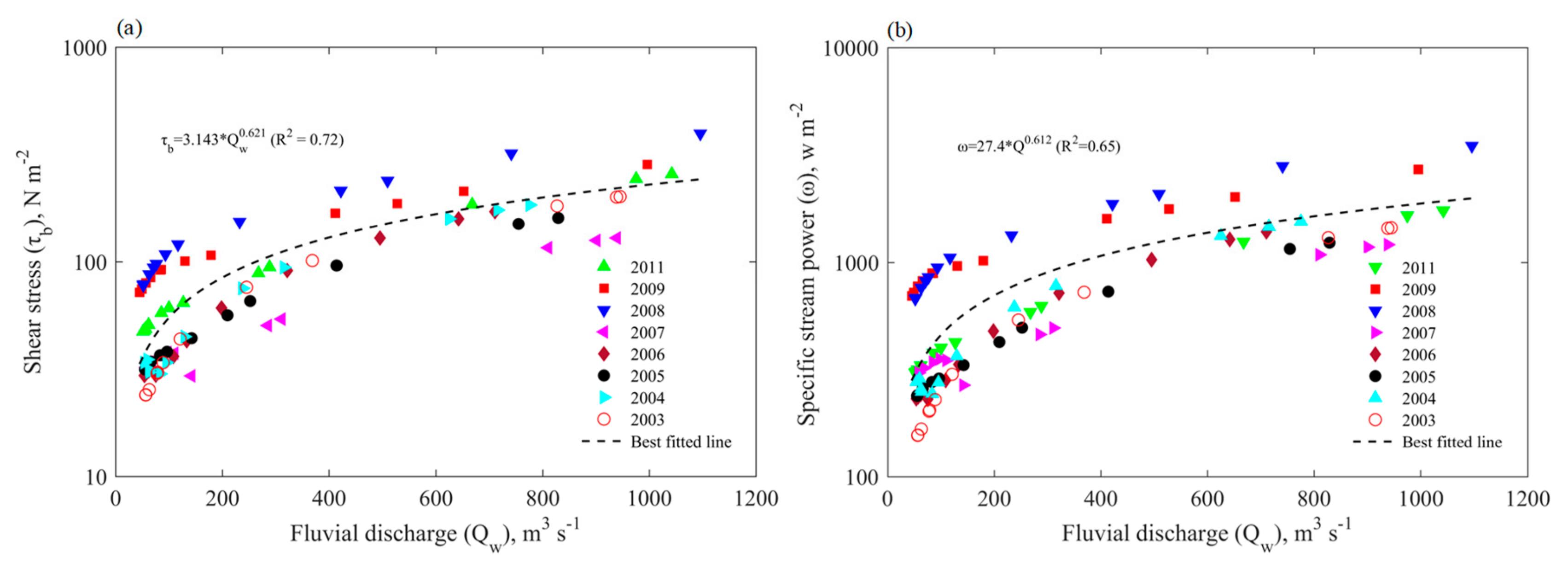
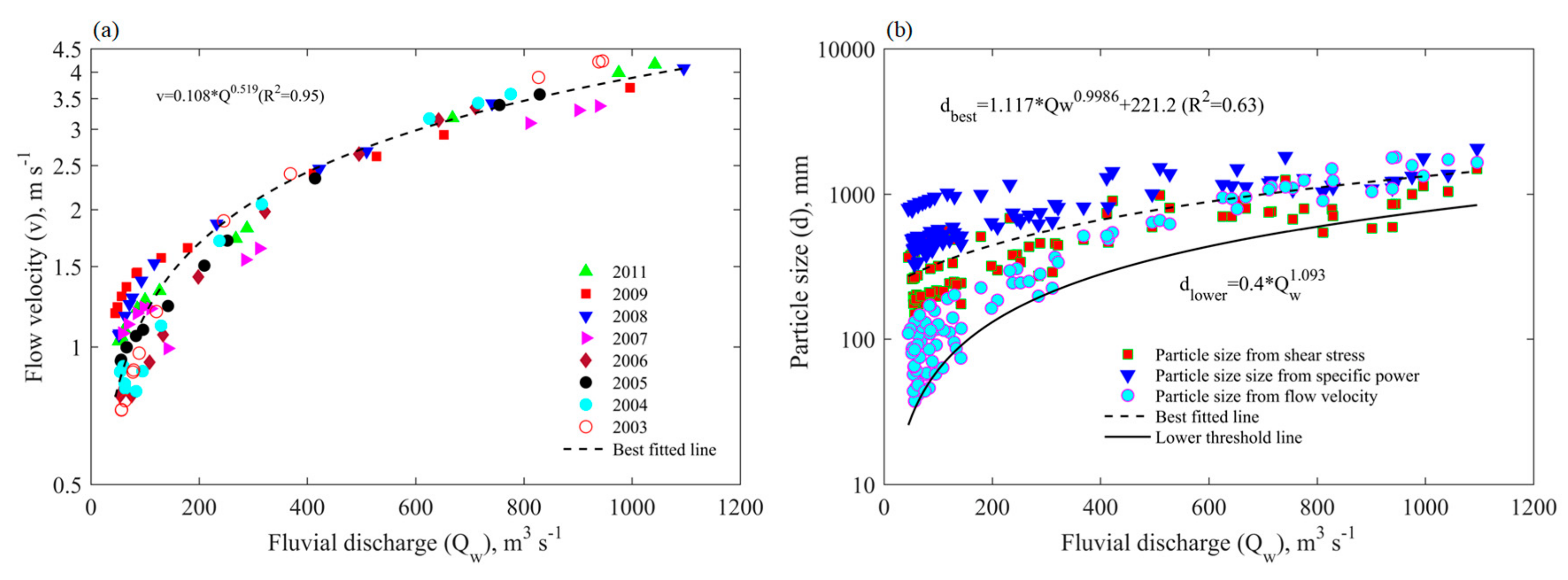
2.3. Analysis of Shear Stress, Specific Power, and Flow Velocity
2.4. Development of Different Models for Suspended Sediment Predictions
2.4.1. Multiple Linear Regression
2.4.2. Nonlinear Multiple Regression
2.4.3. Sediment Rating Curve
2.4.4. Artificial Neural Networks
2.5. Model Performance
3. Results and Discussion
3.1. Relationship of Shear Stress, Specific Stream Power, and Flow Velocity with Discharge
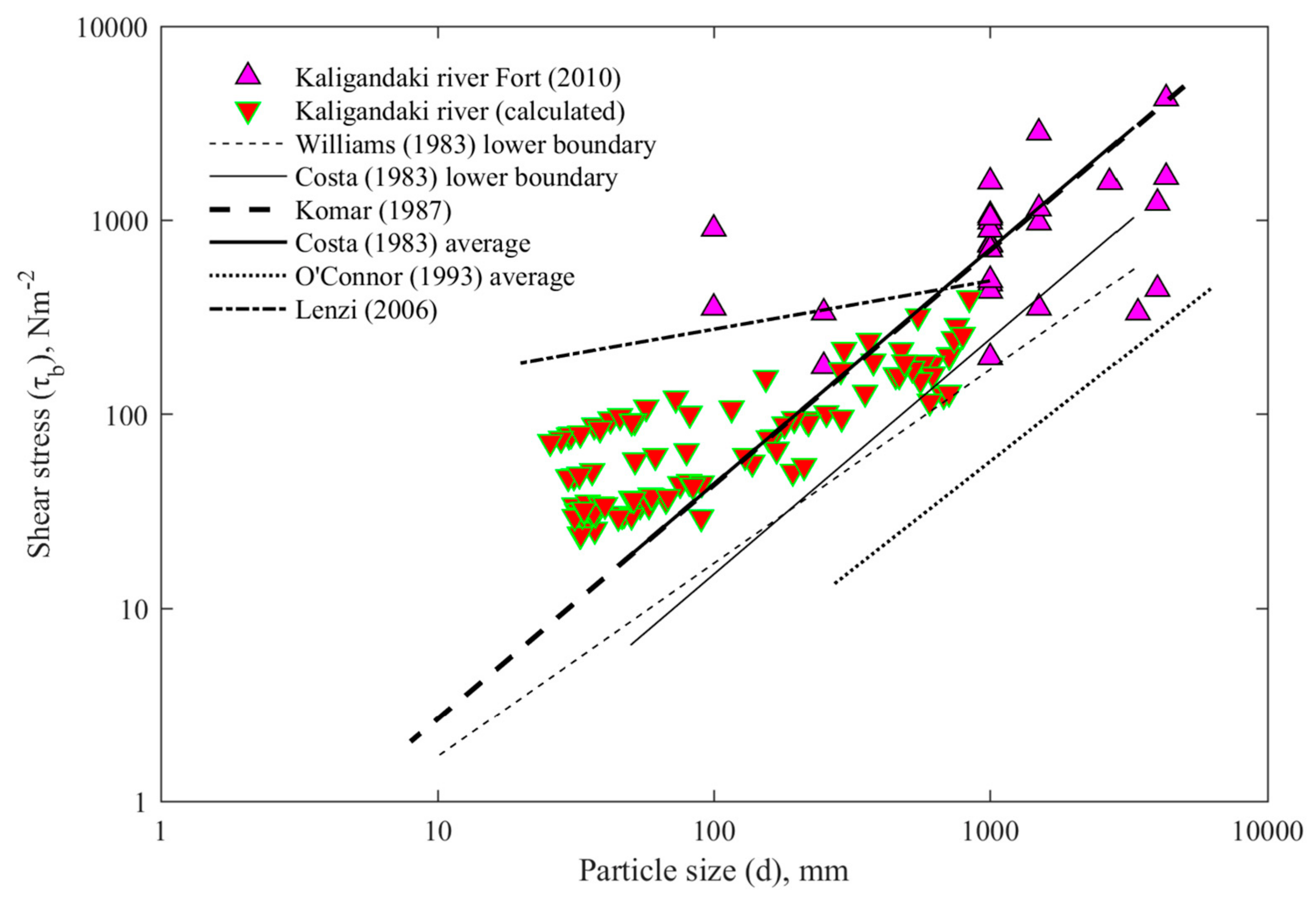
3.2. Relationship of Particle Sizes and Fluvial Discharge
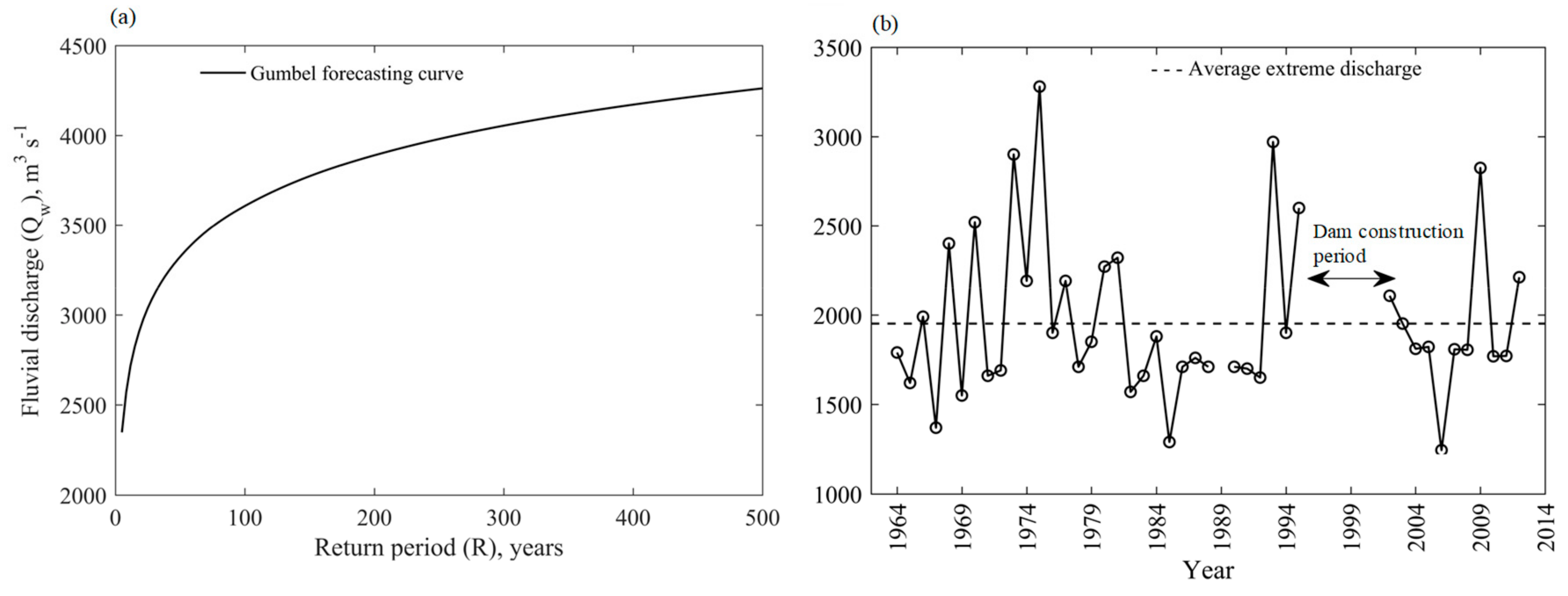
3.3. Estimation of the Return Period by Gumbel’s Distribution
3.4. Boulder Movement Mechanisms in the Himalayas
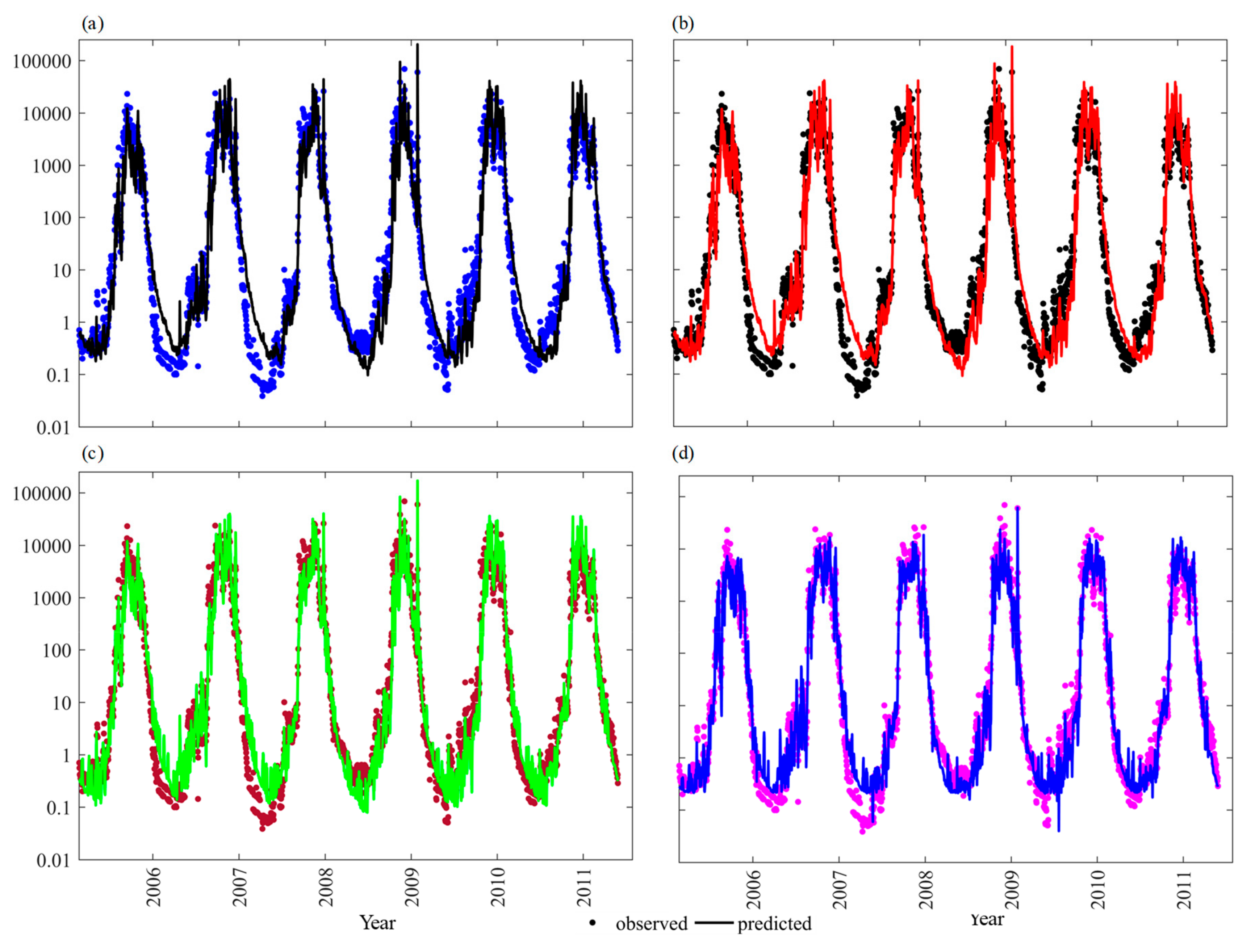
3.5. Quantification and Prediction of the Suspended Sediment
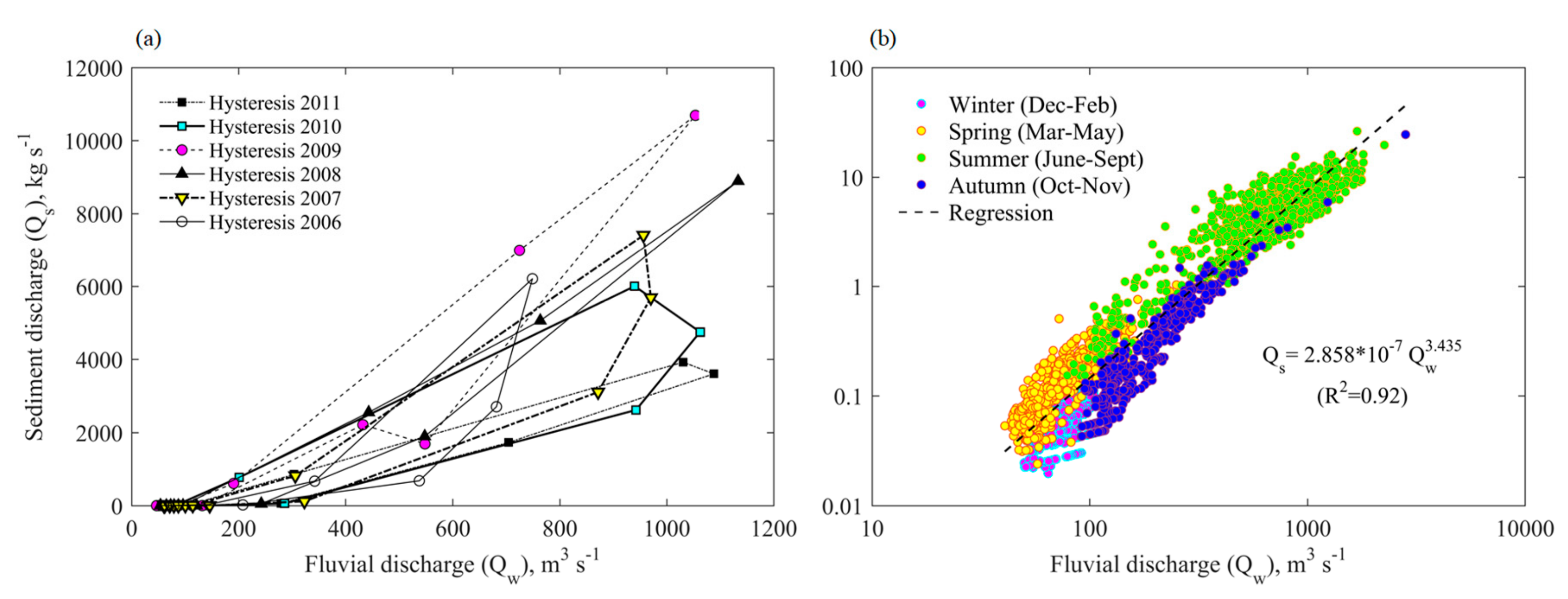
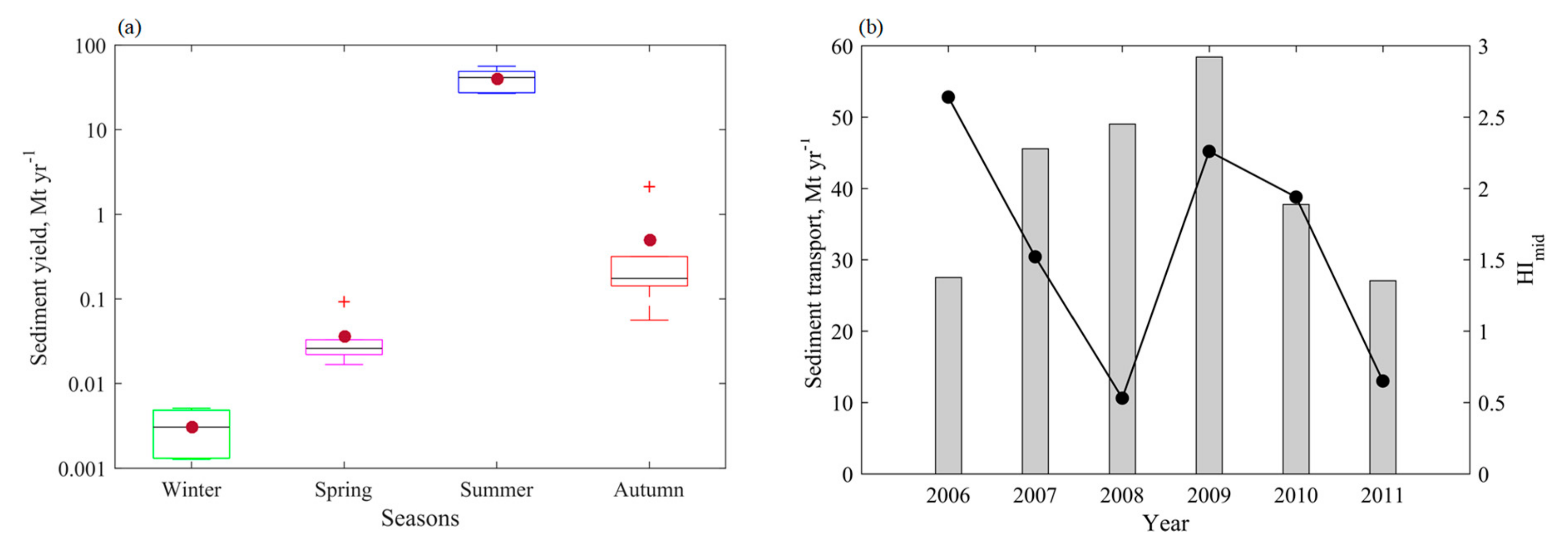
3.5.1. Hysteresis Curve and Hysteresis Index (HImid) Analysis
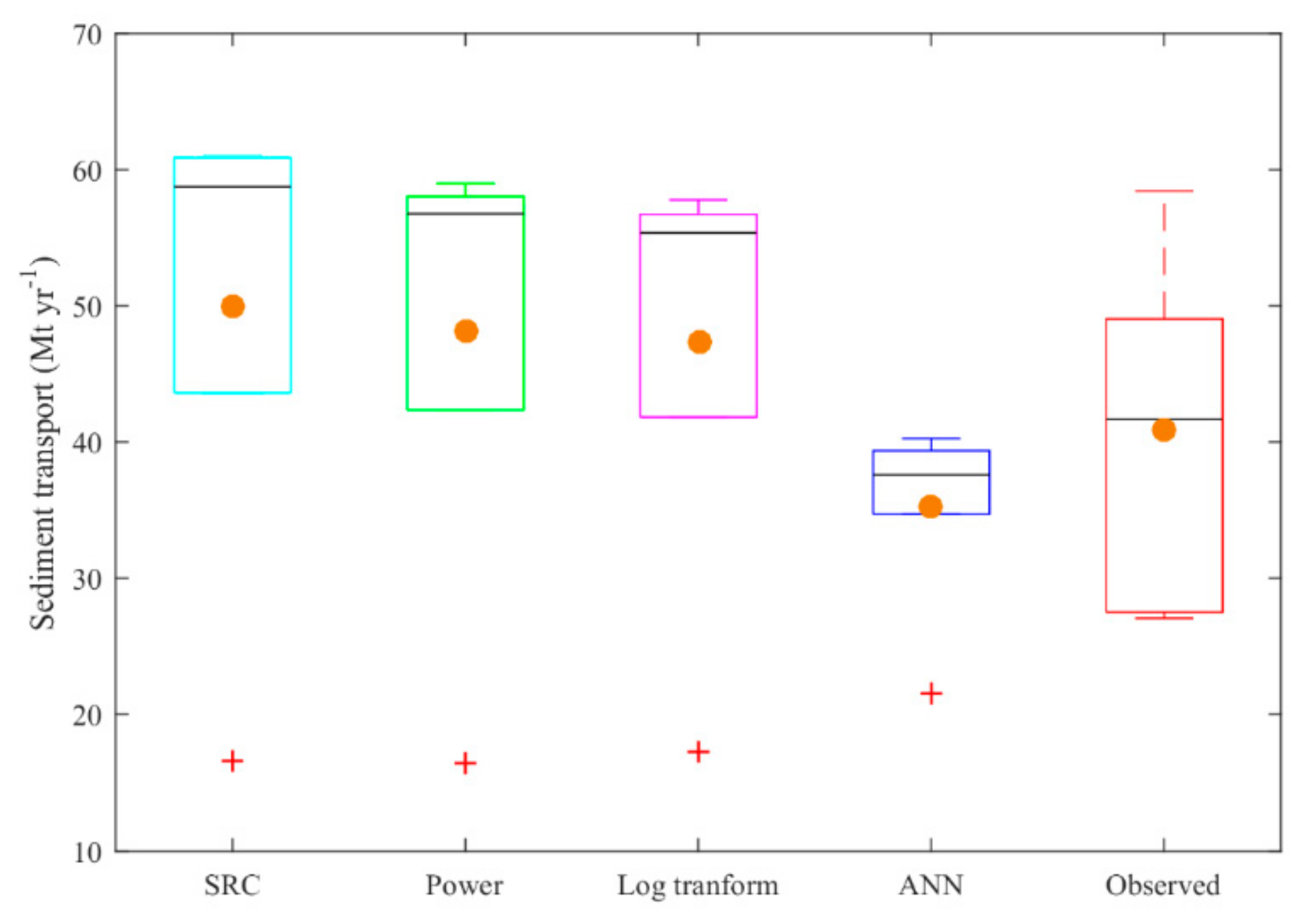
3.5.2. Yearly Suspended Sediment Yield and Prediction by Different Models
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Gudino-Elizondo, N.; Biggs, T.W.; Bingner, R.L.; Langendoen, E.J.; Kretzschmar, T.; Taguas, E.V.; Taniguchi-Quan, K.T.; Liden, D.; Yuan, Y. Modelling Runoff and Sediment Loads in a Developing Coastal Watershed of the US-Mexico Border. Water 2019, 11, 1024. [Google Scholar] [CrossRef]
- Fort, M. Sedimentary fluxes in Himalaya. In Source-to-Sink Fluxes in Undisturbed Cold Environments; Beylich, A.A., Dixon, J.C., Zwolinski, Z., Eds.; Cambridge University Press: Cambridge, UK, 2016; pp. 326–350. [Google Scholar]
- Fort, M.; Cossart, E. Erosion assessment in the middle Kali Gandaki (Nepal): A sediment budget approach. J. Nepal Geol. Soc. 2013, 46, 25–40. [Google Scholar]
- Mishra, B.; Babel, M.S.; Tripathi, N.K. Analysis of climatic variability and snow cover in the Kaligandaki River Basin, Himalaya, Nepal. Theor. Appl. Climatol. 2014, 116, 681–694. [Google Scholar] [CrossRef]
- Dahal, R.K.; Hasegawa, S. Representative rainfall thresholds for landslides in the Nepal Himalaya. Geomorphology 2008, 100, 429–443. [Google Scholar] [CrossRef]
- Fort, M.; Cossart, E.; Arnaud-Fassetta, G. Hillslope-channel coupling in the Nepal Himalayas and threat to man-made structures: The middle Kali Gandaki valley. Geomorphology 2010, 124, 178–199. [Google Scholar] [CrossRef]
- Lenzi, M.A.; D’Agostino, V.; Billi, P. Bedload transport in the instrumented catchment of the Rio Cordon: Part I: Analysis of bedload records, conditions and threshold of bedload entrainment. Catena 1999, 36, 171–190. [Google Scholar] [CrossRef]
- Struck, M.; Andermann, C.; Hovius, N.; Korup, O.; Turowski, J.M.; Bista, R.; Pandit, H.P.; Dahal, R.K. Monsoonal hillslope processes determine grain size-specific suspended sediment fluxes in a trans-Himalayan river. Geophys. Res. Lett. 2015, 42, 2302–2308. [Google Scholar] [CrossRef]
- Asaeda, T.; Baniya, M.B.; Rashid, M.H. Effect of floods on the growth of Phragmites japonica on the sediment bar of regulated rivers: A modelling approach. Int. J. River Basin Manag. 2011, 9, 211–220. [Google Scholar] [CrossRef]
- Guillén-Ludeña, S.; Manso, P.; Schleiss, A. Multidecadal Sediment Balance Modelling of a Cascade of Alpine Reservoirs and Perspectives Based on Climate Warming. Water 2018, 10, 1759. [Google Scholar] [CrossRef]
- Megnounif, A.; Terfous, A.; Ouillon, S. A graphical method to study suspended sediment dynamics during flood events in the Wadi Sebdou, NW Algeria (1973–2004). J. Hydrol. 2013, 497, 24–36. [Google Scholar] [CrossRef]
- Fort, M. Sporadic morphogenesis in a continental subduction setting: An example from the Annapurna Range, Nepal Himalaya. Z. Geomorphol. 1987, 63, 36. [Google Scholar]
- Costa, J.E.; Schuster, R.L. The formation and failure of natural dams. Geol. Soc. Am. Bull. 1988, 100, 1054–1068. [Google Scholar] [CrossRef]
- O’Connor, J.E.; Costa, J.E. Geologic and hydrologic hazards in glacierized basins in North America resulting from 19th and 20th century global warming. Nat. Hazards 1993, 8, 121–140. [Google Scholar] [CrossRef]
- Zhang, G.; Liu, Y.; Han, Y.; Zhang, X.C. Sediment Transport and Soil Detachment on Steep Slopes: I. Transport Capacity Estimation. Soil Sci. Soc. Am. J. 2009, 73, 1291. [Google Scholar] [CrossRef]
- Ali, M.; Sterk, G.; Seeger, M.; Boersema, M.; Peters, P. Effect of hydraulic parameters on sediment transport capacity in overland flow over erodible beds. Hydrol. Earth Syst. Sci. 2012, 16, 591–601. [Google Scholar] [CrossRef]
- Baker, V.R.; Ritter, D.F. Competence of rivers to transport coarse bedload material. Geol. Soc. Am. Bull. 1975, 86, 975–978. [Google Scholar] [CrossRef]
- Lotsari, E.; Wang, Y.; Kaartinen, H.; Jaakkola, A.; Kukko, A.; Vaaja, M.; Hyyppä, H.; Hyyppä, J.; Alho, P. Geomorphology Gravel transport by ice in a subarctic river from accurate laser scanning. Geomorphology 2015, 246, 113–122. [Google Scholar] [CrossRef]
- Komar, P.D.; Carling, P.A. Grain sorting in gravel-bed streams and the choice of particle sizes for flow-competence evaluations. Sedimentology 1991, 38, 489–502. [Google Scholar] [CrossRef]
- Costa, J.E. Paleohydraulic reconstruction of flash-flood peaks from boulder deposits in the Colorado Front Range. Geol. Soc. Am. Bull. 1983, 94, 986–1004. [Google Scholar] [CrossRef]
- Komar, P.D. Selective Gravel Entrainment and the Empirical Evaluation of Flow Competence. Sedimentology 1987, 34, 1165–1176. [Google Scholar] [CrossRef]
- Lenzi, M.A.; Mao, L.; Comiti, F. When does bedload transport begin in steep boulder-bed streams? Hydrol. Process. 2006, 20, 3517–3533. [Google Scholar] [CrossRef]
- O’Connor, J.E. Hydrology, Hydraulics, and Geomorphology of the Bonneville Flood; Geological Society of America: Boulder, CO, USA, 1993; Volume 274, ISBN 0813722748.
- Williams, G.P. Paleohydrological methods and some examples from Swedish fluvial environments: I cobble and boulder deposits. Geogr. Ann. Ser. A Phys. Geogr. 1983, 65, 227–243. [Google Scholar] [CrossRef]
- Bradley, W.C.; Mears, A.I. Calculations of flows needed to transport coarse fraction of Boulder Creek alluvium at Boulder, Colorado. Geol. Soc. Am. Bull. 1980, 91, 1057–1090. [Google Scholar] [CrossRef]
- Helley, E.J. Field Measurement of the Initiation of Large Bed Particle Motion in Blue Creek Near Klamath, California; US Government Printing Office: Washington, DC, USA, 1969.
- Bhusal, J.K.; Subedi, B.P. Effect of climate change on suspended sediment load in the Himalayan basin: A case study of Upper Kaligandaki River. J. Hydrol. 2015, 54, 1–10. [Google Scholar]
- Kale, V.S.; Hire, P.S. Effectiveness of monsoon floods on the Tapi River, India: Role of channel geometry and hydrologic regime. Geomorphology 2004, 57, 275–291. [Google Scholar] [CrossRef]
- Mao, L.; Uyttendaele, G.P.; Iroumé, A.; Lenzi, M.A. Field based analysis of sediment entrainment in two high gradient streams located in Alpine and Andine environments. Geomorphology 2008, 93, 368–383. [Google Scholar] [CrossRef]
- Wicher-Dysarz, J. Analysis of Shear Stress and Stream Power Spatial Distributions for Detection of Operational Problems in the Stare Miasto Reservoir. Water 2019, 11, 691. [Google Scholar] [CrossRef]
- Jarrett, R.D. Hydraulics of high-gradient streams. J. Hydraul. Eng. 1984, 110, 1519–1539. [Google Scholar] [CrossRef]
- Uca; Toriman, E.; Jaafar, O.; Maru, R.; Arfan, A.; Ahmar, A.S. Daily Suspended Sediment Discharge Prediction Using Multiple Linear Regression and Artificial Neural Network. J. Phys. Conf. Ser. 2018, 954, 012030. [Google Scholar]
- Ulke, A.; Tayfur, G.; Ozkul, S. Predicting suspended sediment loads and missing data for Gediz River, Turkey. J. Hydrol. Eng. 2009, 14, 954–965. [Google Scholar] [CrossRef]
- Glysson, G.D. Sediment-Transport Curves; Open-File Report 87-218; US Geological Survey: Reston, VA, USA, 1987.
- Moriasi, D.N.; Arnold, J.G.; Van Liew, M.W.; Bingner, R.L.; Harmel, R.D.; Veith, T.L. Model evaluation guidelines for systematic quantification of accuracy in watershed simulations. Trans. ASABE 2007, 50, 885–900. [Google Scholar] [CrossRef]
- Pandey, M.; Zakwan, M.; Sharma, P.K.; Ahmad, Z. Multiple linear regression and genetic algorithm approaches to predict temporal scour depth near circular pier in non-cohesive sediment. ISH J. Hydraul. Eng. 2018. [Google Scholar] [CrossRef]
- Bajracharya, A.R.; Bajracharya, S.R.; Shrestha, A.B.; Maharjan, S.B. Climate change impact assessment on the hydrological regime of the Kaligandaki Basin, Nepal. Sci. Total Environ. 2018, 625, 837–848. [Google Scholar] [CrossRef] [PubMed]
- Onen, F.; Bagatur, T. Prediction of Flood Frequency Factor for Gumbel Distribution Using Regression and GEP Model. Arab. J. Sci. Eng. 2017, 42, 3895–3906. [Google Scholar] [CrossRef]
- Grant, G.E.; Swanson, F.J.; Wolman, M.G. Pattern and origin of stepped-bed morphology in high-gradient streams, Western Cascades, Oregon. Bull. Geol. Soc. Am. 1990, 102, 340–352. [Google Scholar] [CrossRef]
- Montgomery, D.R.; Buffington, J.M. Channel-reach morphology in mountain drainage basins. Bull. Geol. Soc. Am. 1997, 109, 596–611. [Google Scholar] [CrossRef]
- Marahatta, S. Earthquake and Ramche Landslide Dam in Kali Gandaki. Post April 25, 2015. Available online: http://coramnepal.org/wp-content/uploads/2017/09/Kali-Gandaki-Landslide-Dam-Outburst-Flood.pdf (accessed on 18 May 2019).
- Bricker, J.D.; Schwanghart, W.; Adhikari, B.R.; Moriguchi, S.; Roeber, V.; Giri, S. Performance of Models for Flash Flood Warning and Hazard Assessment: The 2015 Kali Gandaki Landslide Dam Breach in Nepal. Mt. Res. Dev. 2017, 37, 5–15. [Google Scholar] [CrossRef]
- Griffiths, G.A. Origin and transport of large boulders in mountain streams. J. Hydrol. 1977, 16, 1–6. [Google Scholar]
- Williams, G.P. Sediment concentration versus water discharge during single hydrologic events in rivers. J. Hydrol. 1989, 111, 89–106. [Google Scholar] [CrossRef]
- Bača, P. Hysteresis effect in suspended sediment concentration in the Rybárik basin, Slovakia/Effet d’hystérèse dans la concentration des sédiments en suspension dans le bassin versant de Rybárik (Slovaquie). Hydrol. Sci. J. 2008, 53, 224–235. [Google Scholar] [CrossRef]
- Lloyd, C.E.M.; Freer, J.E.; Johnes, P.J.; Collins, A.L. Using hysteresis analysis of high-resolution water quality monitoring data, including uncertainty, to infer controls on nutrient and sediment transfer in catchments. Sci. Total Environ. 2016, 543, 388–404. [Google Scholar] [CrossRef] [PubMed]
- Lawler, D.M.; Petts, G.E.; Foster, I.D.L.; Harper, S. Turbidity dynamics during spring storm events in an urban headwater river system: The Upper Tame, West Midlands, UK. Sci. Total Environ. 2006, 360, 109–126. [Google Scholar] [CrossRef] [PubMed]
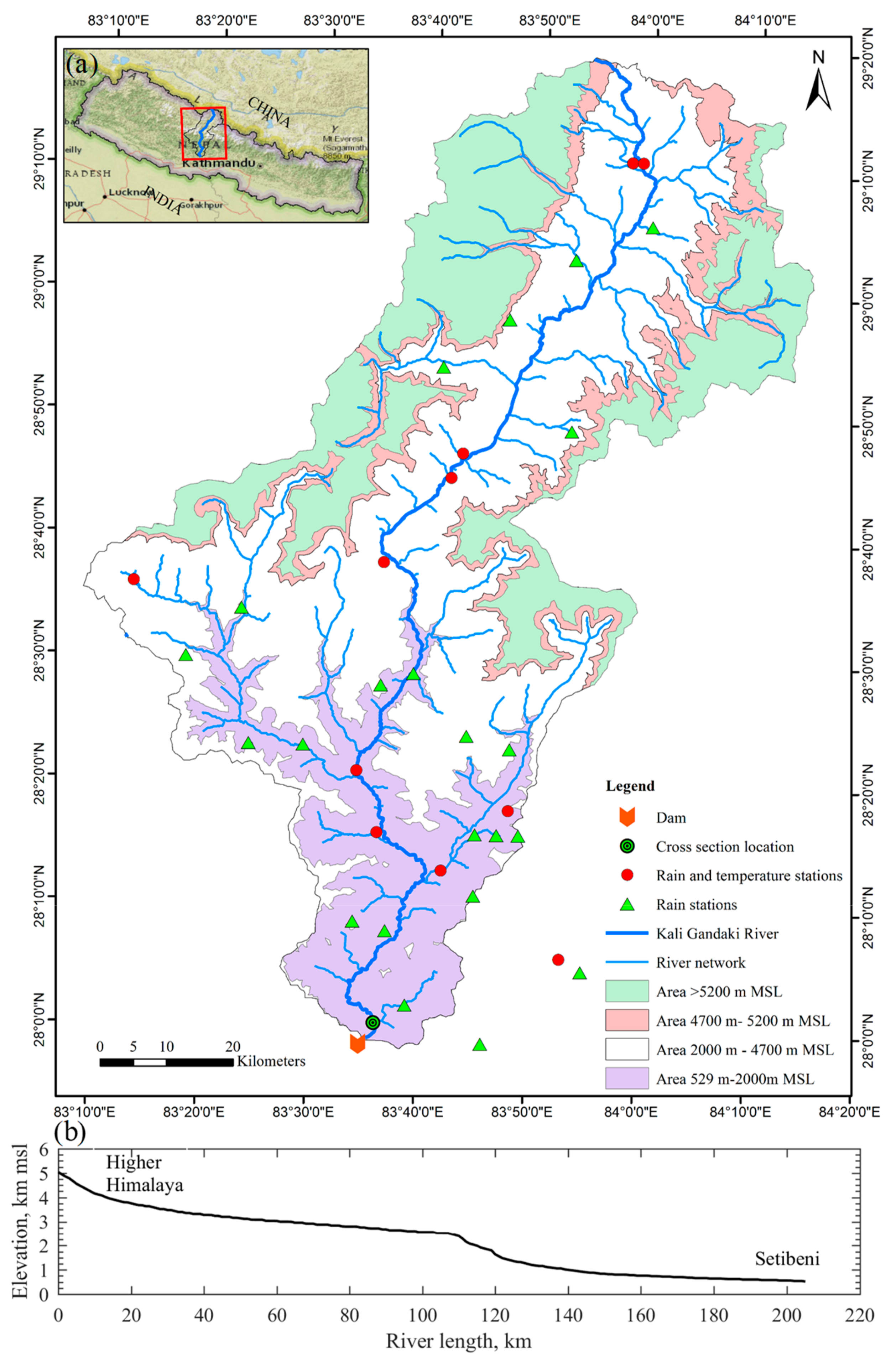



| Parameters | |
|---|---|
| Catchment area | 7060 km2 |
| Length of river up to dam | 210 km |
| Mean gradient of river | 2.20% |
| Extreme discharge | 3280 m3 s−1 in 1975, 2824.5 m3 s−1 in 2009 |
| Elevation ranges | 529 m MSL–8143 m MSL |
| Precipitation | Tibetan plateau <500 mm year−1, monsoon dominated Himalayas~2000 mm year−1 |
| Model Scenario | RMSE (kg·s−1) | PBIAS | RSR | R2 | NSE | Model Equation |
|---|---|---|---|---|---|---|
| 2498 | +0.47 | 0.66 | 0.53 | +0.56 | ||
| 2729 | +0.34 | 0.73 | 0.44 | +0.47 | ||
| 2442 | +0.22 | 0.64 | 0.55 | +0.59 | ||
| 2494 | +0.35 | 0.66 | 0.53 | +0.56 | ||
| 2339 | +0.29 | 0.59 | 0.59 | +0.65 |
| Model Scenario | RMSE (kg·s−1) | PBIAS | RSR | R2 | NSE | Model Equation |
|---|---|---|---|---|---|---|
| 2314 | +0.33 | 0.57 | 0.59 | +0.67 | ||
| 2697 | +0.66 | 0.71 | 0.46 | +0.49 | ||
| 2280 | +0.15 | 0.56 | 0.61 | +0.68 | ||
| 2303 | +0.32 | 0.57 | 0.59 | +0.67 | ||
| 2250 | +0.43 | 0.55 | 0.62 | +0.69 |
| Model Scenario | RMSE (kg·s−1) | PBIAS | RSR | R2 | NSE | Model Equation |
|---|---|---|---|---|---|---|
| General power model 1 | 2039 | +3.81 | 0.56 | 0.67 | +0.68 | |
| General power model 2 | 2039 | +0.22 | 0.56 | 0.67 | +0.68 |
| Model Scenario | RMSE (kg·s−1) | PBIAS | RSR | R2 | NSE | Model Equation |
|---|---|---|---|---|---|---|
| Linear model (SRC) | 4451 | −21.65 | 1.23 | 0.59 | −0.51 | |
| General power model 2 | 4039 | −17.50 | 1.12 | 0.59 | −0.25 | |
| Linear model | 3715 | −15.47 | 1.03 | 0.61 | −0.05 |
| Model Scenario | RMSE (kg·s−1) | PBIAS | RSR | R2 | NSE | Model Equation |
|---|---|---|---|---|---|---|
| 2768 | +54.07 | 0.77 | 0.45 | +0.41 | Levenberg-Marguardt | |
| 2070 | +14.91 | 0.57 | 0.67 | +0.66 | Levenberg-Marguardt | |
| 2052 | +15.99 | 0.56 | 0.71 | +0.68 | Levenberg-Marguardt | |
| 2123 | +22.95 | 0.59 | 0.69 | +0.66 | Levenberg-Marguardt | |
| 1982 | +14.26 | 0.55 | 0.71 | +0.70 | Levenberg-Marguardt |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Baniya, M.B.; Asaeda, T.; K.C., S.; Jayashanka, S.M.D.H. Hydraulic Parameters for Sediment Transport and Prediction of Suspended Sediment for Kali Gandaki River Basin, Himalaya, Nepal. Water 2019, 11, 1229. https://doi.org/10.3390/w11061229
Baniya MB, Asaeda T, K.C. S, Jayashanka SMDH. Hydraulic Parameters for Sediment Transport and Prediction of Suspended Sediment for Kali Gandaki River Basin, Himalaya, Nepal. Water. 2019; 11(6):1229. https://doi.org/10.3390/w11061229
Chicago/Turabian StyleBaniya, Mahendra B., Takashi Asaeda, Shivaram K.C., and Senavirathna M.D.H. Jayashanka. 2019. "Hydraulic Parameters for Sediment Transport and Prediction of Suspended Sediment for Kali Gandaki River Basin, Himalaya, Nepal" Water 11, no. 6: 1229. https://doi.org/10.3390/w11061229
APA StyleBaniya, M. B., Asaeda, T., K.C., S., & Jayashanka, S. M. D. H. (2019). Hydraulic Parameters for Sediment Transport and Prediction of Suspended Sediment for Kali Gandaki River Basin, Himalaya, Nepal. Water, 11(6), 1229. https://doi.org/10.3390/w11061229





