The Role of Winter Rain in the Glacial System on Svalbard
Abstract
:1. Introduction
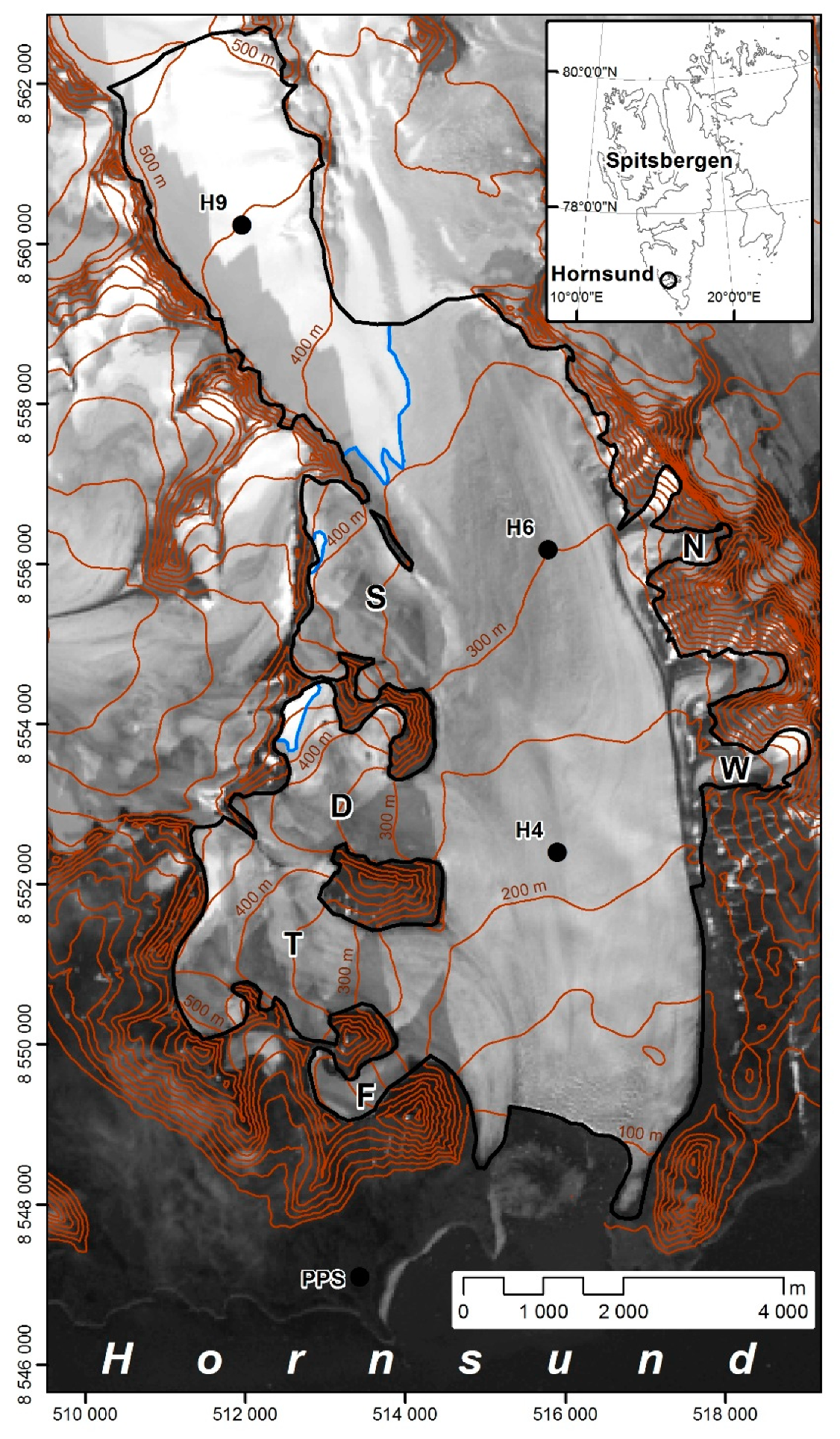
2. Study Area
3. Materials and Methods
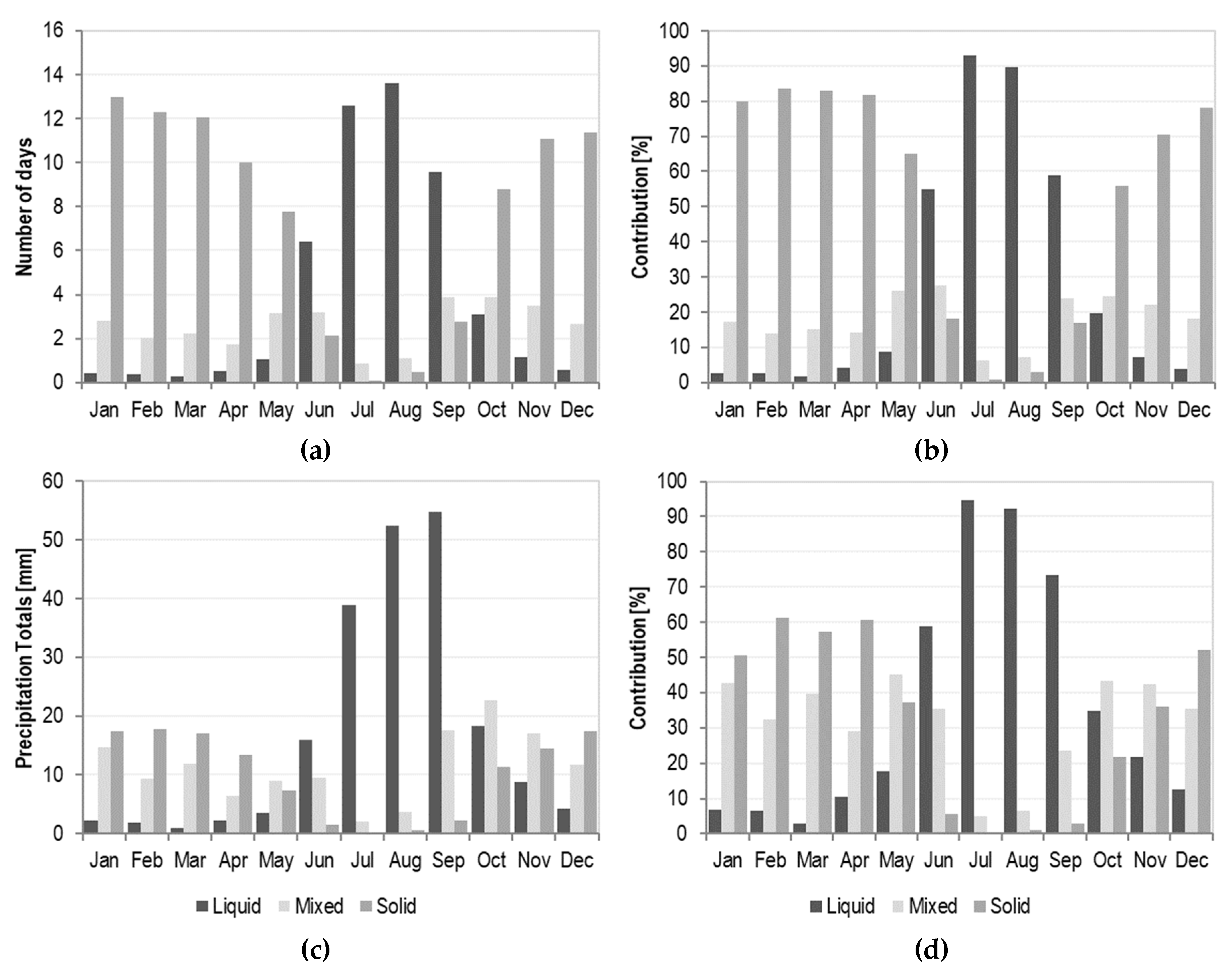
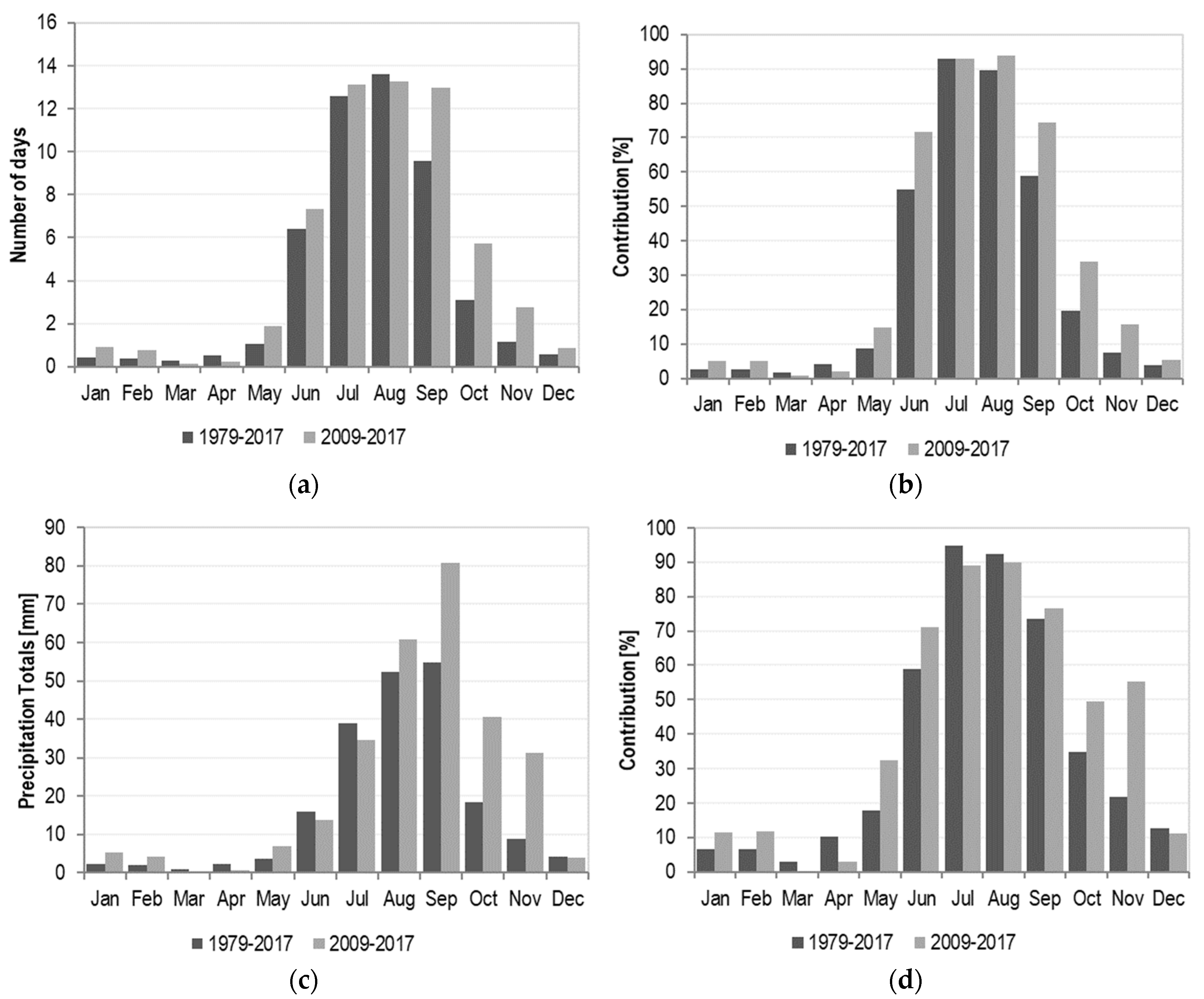
4. Winter Rainfall
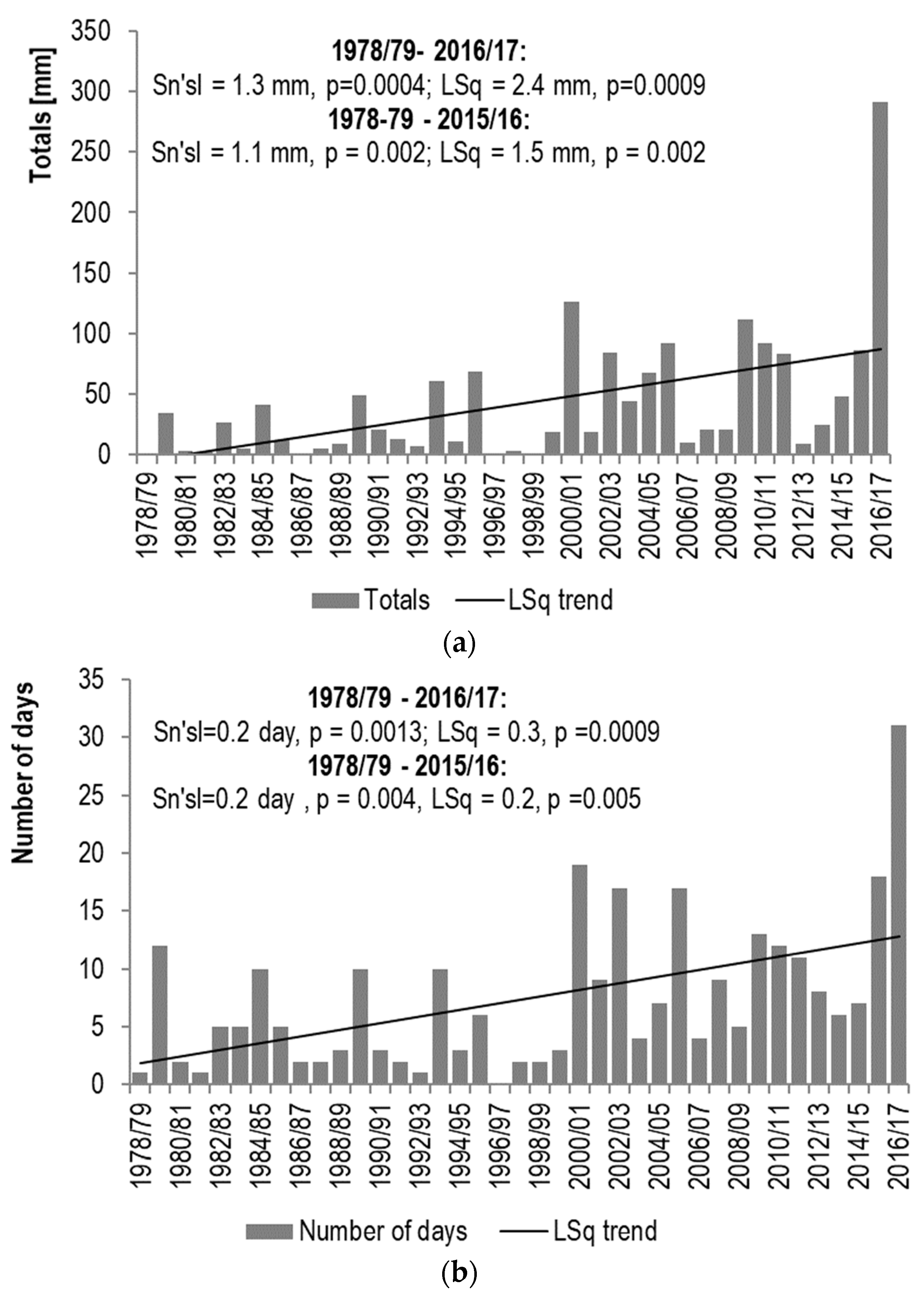
4.1. Climatology and Changes in Winter Rain
4.2. Episodes of Winter Rain in the Seasons 2009/2010 to 2016/2017
4.3. Examples of Acceleration of Glacier Velocity after Rainfalls
4.4. Traces of Rain in the Snow-Cover Structure
5. Estimation of the Extent of the Rain Field on Hansbreen
6. Discussion
6.1. Contribution of Winter Rain to Glacier Mass Balance
6.2. Winter Rain and Dynamic Behavior of the Glacier
6.3. Winter Rain Events Reflected in the Snowpack Structure
7. Conclusions
- The frequency of winter rainfall (Oct‒May) increased in the southern part of Svalbard between 1978 and 2017, particularly in October and November.
- Data on the occurrence of winter rainfall at Polish Polar Station Hornsund proved useful for identifying the liquid precipitation phase (winter rainfall) on the glacier, provided supporting measurements on the glacier were available.
- The contribution of rainfall to the winter mass balance of Hansbreen during the period 2009–2016 ranged from 0 to 21%, with an average contribution of 9%.
- The maximum total of winter rainfall and the greatest contribution of rainwater to the winter mass balance occurred on the glacier’s surface area between 250–400 m a.s.l. as a combined effect of both precipitation gradient and temperature lapse rate.
- Changes in winter rainfall totals on the glacier depended on both altitude and lapse rate. The positive gradient of winter rainfall totals, due to a general altitudinal increase in precipitation rate, was found to reach the altitude of the 0 °C isotherm (ca 250–400 m a.s.l.). The negative gradient was found above the approximate location of the 0 °C isotherm where temperatures were more frequently below the freezing point.
- The dynamic response of the glacier to winter rainfall varied, depending on the development of both the snow cover and the glacier drainage system. Early winter rain falling on an initially thin snow cover and a drainage system, which was squeezed following large discharges (i.e., inefficient) generally resulted in increased glacier motion. On the other hand, winter rainfall occurring after the formation of a sufficiently thick snow cover was effectively stored therein and caused no significant speed-ups.
- The increased frequency of winter rainfall and thaws contributed to a greater number of hard snow layers and consequently to greater bulk density of the snowpack. As both water sources (rainfall and melting) most often occur at the same time, their individual influence on the snowpack’s structure is difficult to distinguish.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Hodgkins, R. Glacier hydrology in Svalbard, Norwegian High Arctic. Quat. Sci. Rev. 1997, 16, 957–973. [Google Scholar] [CrossRef]
- Fountain, A.G.; Walder, J.S. Water flow through temperate glaciers. Rev. Geophys. 1998, 36, 299–328. [Google Scholar] [CrossRef]
- Jansson, P.; Hock, R.; Schneider, T. The concept of glacier storage: A review. J. Hydrol. 2003, 282, 116–129. [Google Scholar] [CrossRef]
- Irvine-Fynn, T.D.L.; Hodson, A.J.; Moorman, B.J.; Vatne, G.; Hubbard, A.L. Polythermal glacier hydrology: A review. Rev. Geophys. 2011, 49. [Google Scholar] [CrossRef]
- Shreve, R.L. Movement of water in glaciers. J. Glaciol. 1972, 11, 206–214. [Google Scholar] [CrossRef]
- Jania, J. Glacjologia. Nauka o lodowcach; PWN: Warsaw, Poland, 1997; p. 359. [Google Scholar]
- Hagen, J.O.; Kohler, J.; Melvold, K.; Winther, J.G. Glaciers in Svalbard: Mass balance, runoff and freshwater flux. Polar Res. 2003, 22, 145–159. [Google Scholar] [CrossRef]
- Majchrowska, E.; Ignatiuk, D.; Jania, J.; Marszałek, H.; Wąsik, M. Seasonal and interannual variability in runoff from the Werenskioldbreen catchment, Spitsbergen. Pol. Polar Res. 2015, 36, 197–224. [Google Scholar] [CrossRef]
- Hock, R. Modelling of Glacier Melt and Discharge. Ph.D. Dissertation, Zürcher Geographische Schriften Geographisches Institut, Zürich, Switzerland, 1998; p. 130. [Google Scholar]
- Willis, I.C. Intra-annual variations in glacier motion: A review. Prog. Phys. Geogr. 1995, 19, 61–106. [Google Scholar] [CrossRef]
- Van Pelt, W.; Kohler, J. Modelling the long-term mass balance and firn evolution of glaciers around Kongsfjorden, Svalbard. J. Glaciol. 2015, 61, 731–744. [Google Scholar] [CrossRef]
- Nowak, A.; Hodson, A. Hydrological response of a High-Arctic catchment to changing climate over past 35 years: A case study of Bayelva watershed, Svalbard. Polar Res. 2013, 32, 19691. [Google Scholar] [CrossRef]
- Vikhamar-Schuler, D.; Isaksen, K.; Haugen, J.E.; Tømmervik, H.; Luks, B.; Schuler, T.V.; Bjerke, J.W. Changes in winter warming events in the Nordic Arctic Region. J. Clim. 2016, 29, 6223–6244. [Google Scholar] [CrossRef]
- Fierz, C.; Armstrong, R.L.; Durand, Y.; Etchevers, P.; Greene, E.; Mcclung, D.M.; Nishimura, K.; Satyawali, P.K.; Sokratov, S.A. The International Classification for Seasonal Snow on the Ground; IACS Contribution N°1; UNESCO-IHP: Paris, France, 2009. [Google Scholar]
- Laska, M.; Luks, B.; Budzik, T. Influence of snowpack internal structure on snow metamorphism and melting intensity on Hansbreen, Svalbard. Pol. Polar Res. 2016, 37, 193–218. [Google Scholar] [CrossRef]
- Blatter, H.; Hutter, K. Polythermal conditions in Arctic glaciers. J. Glaciol. 1991, 37, 261–269. [Google Scholar] [CrossRef]
- Błaszczyk, M.; Jania, J.A.; Kolondra, L. Fluctuations of tidewater glaciers in Hornsund Fiord (Southern Svalbard) since the beginning of the 20th century. Pol. Polar Res. 2013, 34, 327–352. [Google Scholar] [CrossRef]
- Grabiec, M.; Jania, J.A.; Puczko, D.; Kolondra, L.; Budzik, T. Surface and bed morphology of Hansbreen, a tidewater glacier in Spitsbergen. Pol. Polar Res. 2012, 33, 111–138. [Google Scholar] [CrossRef]
- Grabiec, M.; Puczko, D.; Budzik, T.; Gajek, G. Snow distribution patterns on Svalbard glaciers derived from radio-echo soundings. Pol. Polar Res. 2011, 32, 393–421. [Google Scholar] [CrossRef]
- Grabiec, M. Stan i Współczesne Zmiany Systemów Lodowcowych Południowego Spitsbergenu w Świetle Badań Metodami Radarowymi; Prace Naukowe Uniwersytetu Śląskiego w Katowicach nr 3536; Wydawnictwo Uniwersytetu Śląskiego: Katowice, Poland, 2017; p. 328. ISBN 978-83-226-3015-0. [Google Scholar]
- Niedźwiedź, T. The atmospheric circulation. In Climate and Climate Change at Hornsund, Svalbard, 2nd ed.; Marsz, A., Styszyńska, A., Eds.; Gdynia Martitime University: Gdynia, Poland, 2013; pp. 57–74. [Google Scholar]
- Grabiec, M.; Leszkiewicz, J.; Głowacki, P.; Jania, J. Distribution of snow accumulation on some glaciers of Spitsbergen. Pol. Polar Res. 2006, 27, 309–326. [Google Scholar]
- Laska, M.; Grabiec, M.; Ignatiuk, D.; Budzik, T. Snow deposition patterns on southern Spitsbergen glaciers, Svalbard, in relation to recent meteorological conditions and local topography. Geogr. Ann. Ser. A Phys. Geogr. 2017, 99, 262–287. [Google Scholar] [CrossRef]
- Leszkiewicz, J.; Pulina, M. Snowfall phases in analysis of a snow cover in Hornsund, Spitsbergen. Pol. Polar Res. 1999, 20, 3–24. [Google Scholar]
- Laska, M.; Barzycka, B.; Luks, B. Melting Characteristics of Snow Cover on Tidewater Glaciers in Hornsund Fjord, Svalbard. Water 2017b, 9, 804. [Google Scholar] [CrossRef]
- Błaszczyk, M.; Jania, J.A.; Hagen, J.O. Tidewater glaciers of Svalbard: Recent changes and estimates of calving fluxes. Pol. Polar Res. 2009, 30, 85–142. [Google Scholar] [CrossRef]
- Vieli, A.; Jania, J.; Blatter, H.; Funk, M. Short-term velocity variations on Hansbreen, a tidewater glacier in Spitsbergen. J. Glaciol. 2004, 50, 389–398. [Google Scholar] [CrossRef]
- IMGW. Roczniki Meteorologiczne Hornsund (pod red. M Miętusa) 1982/1983–1999/2000; Instytut Meteorologii i Gospodarki Wodnej Oddział Morski: Gdynia, Poland, 2000–2001. [Google Scholar]
- IG PAS. Meteorological Conditions Hornsund, Spitsbergen 2000/2001. Publ. Inst. Geophys. Pol. Acad. Sci. 2001, D-57, 3–77. [Google Scholar]
- IG PAS. Meteorological conditions Hornsund, Spitsbergen 2001/2002. Publ. Inst. Geophys. Pol. Acad. Sci. 2003, D-60, 3–84. [Google Scholar]
- Manual and Codes. Regional Codes and National Coding Practices; WMO-No. 306; World Meteorological Organisation: Geneva, Switzerland, 2014; p. 2.
- Mann, H.B. Nonparametric tests against trend. Econom. J. Econom. Soc. 1945, 13, 245–259. [Google Scholar] [CrossRef]
- Sen, P.K. Estimates of the regression coefficient based on Kendall’s tau. J. Am. Stat. Assoc. 1968, 63, 1379–1389. [Google Scholar] [CrossRef]
- Kendall, M. Multivariate Analysis; Charles Griffin & Company: London, UK, 1975. [Google Scholar]
- Sevruk, B. Methods of Correction for Systematic Error in Point Precipitation Measurement for Operational Use; WMO Hydrol. Rep. 21, WMO-No. 589; World Meteorological Organisation: Geneva, Switzerland, 1982; p. 91. [Google Scholar]
- Groisman, P.Y.; Legates, D.R. Documenting and Detecting Long-Term Precipitation Trends: Where We Are and What Should Be Done. Clim. Chang. 1995, 31, 601–622. [Google Scholar] [CrossRef]
- Allerup, P.; Madsen, H.; Vejen, F.A. Comprehensive Model to Correcting Point Precipitation. Nord. Hydrol. 1997, 28, 1–20. [Google Scholar] [CrossRef]
- Goodison, B.E.; Louie, P.Y.T.; Yang, D. WMO Solid Precipitation Measurement Intercomparison; World Meteorological Organization: Geneva, Switzerland, 1998; No. 872; p. 88. [Google Scholar]
- Førland, E.J.; Hanssen-Bauer, I. Increased precipitation in the Norwegian Arctic: True or false? Clim. Chang. 2000, 46, 485–509. [Google Scholar] [CrossRef]
- WGMS Fluctuations of Glaciers 2005–2010; ICSU(WDS)/IUGG(IACS)/UNEP/UNESCO/WMO; World Glacier Monitoring Service: Zürich, Switzerland, 2012; Volume X.
- WGMS Global Glacier Change Bulletin No. 1 (2012–2013); ICSU(WDS)/IUGG(IACS)/UNEP/UNESCO/WMO; World Glacier Monitoring Service: Zürich, Switzerland, 2015.
- Decaux, L.; Grabiec, M.; Ignatiuk, D.; Jania, J. Role of discrete recharge from the supraglacial drainage system for modelling of subglacial conduits pattern of Svalbard polythermal glaciers. Cryosphere 2018. [Google Scholar] [CrossRef]
- Wise, E.K. Spatiotemporal variability of the precipitation dipole transition zone in the western United States. Geophys. Res. Lett. 2010, 37, L07706. [Google Scholar] [CrossRef]
- Yu, Z.; Jiang, P.; Gautam, M.R.; Zhang, Y.; Acharya, K. Changes of seasonal storm properties in california and nevada from an ensemble of climate projections. J. Geophys. Res. Atmos. 2015, 120, 2676–2688. [Google Scholar] [CrossRef]
- Førlland, E.; Hanssen-Bauer, I. Climate variations and implications for precipitation types in the Norwegian Arctic. Norw. Meteorol. Inst. Rep. 2003, 24, 21. [Google Scholar]
- Førland, E.J.; Hanssen-Bauer, I. Past and future climate variations in the Norwegian Arctic: Overview and novel analyses. Polar Res. 2003, 22, 113–124. [Google Scholar] [CrossRef]
- Bintanja, R.F.; Selten, M. Future increases in Arctic precipitation linked to local evaporation and sea-ice retreat. Nature 2014, 509, 479–482. [Google Scholar] [CrossRef] [PubMed]
- Głowacki, P. Role of physical and chemical processes in the internal structure formation and mass circulation of Spitsbergen glaciers. Publ. Inst. Geophys. PAS 2007, M-30, 1–146. (In Polish) [Google Scholar]
- Winther, J.G.; Bruland, O.; Sand, K.; Gerland, S.; Marechal, D.; Ivanov, B.; Głowacki, P.; König, M. Snow research in Svalbard—An overview. Polar Res. 2003, 22, 125–144. [Google Scholar] [CrossRef]
- Migała, K.; Pereyma, J.; Sobik, M. Snow Accumulation in South Spitsbergen. In Wyprawy Polarne Uniwersytetu Śląskiego: 1980–1984; Uniwersytet Śląski: Katowice, Poland, 1988; pp. 48–63. [Google Scholar]
- Chapman, W.L.; Walsh, J.E. Recent variations of sea ice and air temperature in high latitudes. Bull. Am. Meteorol. Soc. 1993, 74, 33–47. [Google Scholar] [CrossRef]
- Overpeck, J.; Hughen, K.; Hardy, D.; Bradley, R.; Case, R.; Douglas, M.; Finney, B.; Gajewski, K.; Jacoby, G.; Jennings, A.; et al. Arctic environmental change of the last four centuries. Science 1997, 278, 1251–1256. [Google Scholar] [CrossRef]
- Serreze, M.C.; Walsh, J.E.; Chapin, F.S.; Osterkamp, T.; Dyurgerov, M.; Romanovsky, V.; Oechel, W.C.; Morison, J.; Zhang, T.; Barry, R.G. Observational evidence of recent change in the northern high-latitude environment. Clim. Chang. 2000, 46, 159–207. [Google Scholar] [CrossRef]
- Førland, E.J.; Hanssen-Bauer, I.; Jónsson, T.; Kern-Hansen, C.; Nordli, P.; Tveito, O.E.; Vaarby Laursen, E. Twentieth century variations in temperature and precipitation in the Nordic Arctic. Polar Rec. 2002, 38, 203–210. [Google Scholar] [CrossRef]
- Stieglitz, M.; Déry, S.J.; Romanovsky, V.E.; Osterkamp, T.E. The role of snow cover in the warming of Arctic permafrost. Geophys. Res. Lett. 2003, 30. [Google Scholar] [CrossRef]
- Førland, E.J.; Benestad, R.; Hanssen-Bauer, I.; Haugen, J.E.; Skaugen, T.E. Temperature and precipitation development at Svalbard 1900–2100. Adv. Meteorol. 2011, 2011, 1–14. [Google Scholar] [CrossRef]
- Pachauri, R.K.; Allen, M.R.; Barros, V.R.; Broome, J.; Cramer, W.; Christ, R.; Dubash, N.K. Climate Change 2014: Synthesis Report. Contribution of Working Groups I, II and III to the Fifth Assessment Report of the Intergovernmental Panel on Climate Change; IPCC: Geneva, Switzerland, 2014; p. 151. [Google Scholar]
- Kusunoki, S.; Mizuta, R.; Hosaka, M. Future changes in precipitation intensity over the Arctic projected by a global atmospheric model with a 60-km grid size. Polar Sci. 2015, 9, 277–292. [Google Scholar] [CrossRef]
- Cogley, J.G.; Hock, R.; Rasmussen, L.A.; Arendt, A.A.; Bauder, A.; Braithwaite, R.J.; Jansson, P.; Kaser, G.; Möller, M.; Nicholson, L.; et al. Glossary of Glacier Mass Balance and Related Terms IHP-VII; Technical Documents in Hydrology; IACS Contribution No. 2; UNESCO-IHP: Paris, France, 2011; Volume 86. [Google Scholar]
- Sundal, A.V.; Shepherd, A.; Nienow, P.; Hanna, E.; Palmer, S.; Huybrechts, P. Melt-induced speed-up of Greenland ice sheet offset by efficient subglacial drainage. Nature 2011, 469, 521–524. [Google Scholar] [CrossRef] [PubMed]
- Copland, L.; Sharp, M.J.; Nienow, P.W. Links between short-term velocity variations and the subglacial hydrology of a predominantly cold polythermal glacier. J. Glaciol. 2003, 49, 337–348. [Google Scholar] [CrossRef]
- Dunse, T.; Schuler, T.V.; Hagen, J.O.; Reijmer, C.H. Seasonal speed-up of two outlet glaciers of Austfonna, Svalbard, inferred from continuos GPS measurements. Cryosphere 2012, 6, 453–466. [Google Scholar] [CrossRef]
- Fudge, T.J.; Harper, J.T.; Humphrey, N.F.; Pfeffer, W.T. Rapid glacier sliding, reverse ice motion and subglacial water pressure during an autumn rainstorm. Ann. Glaciol. 2009, 50, 101–108. [Google Scholar] [CrossRef]
- Doyle, S.H.; Hubbard, A.; van de Wal, R.S.W.; Box, J.E.; van As, D.; Scharrer, K.; Meierbachtol, T.W.; Smeets, P.C.J.P.; Harper, J.T.; Johansson, E.; et al. Amplified melt and flow of the Greenland ice sheet driven by late-summer cyclonic rainfall. Nat. Geosci. 2015, 8, 647. [Google Scholar] [CrossRef]
- Horgan, H.J.; Anderson, B.; Alley, R.B.; Chamberlain, C.J.; Dykes, R.; Kehrl, L.M.; Townend, J. Glacier velocity variability due to rain-induced sliding and cavity formation. Earth Planet. Sci. Lett. 2015, 432, 273–282. [Google Scholar] [CrossRef]
- Purdie, H.; Brook, M.S.; Fuller, I.C. Seasonal Variation in Ablation and Surface Velocity on a Temperate Maritime Glacier: Fox Glacier, New Zealand. Arc. Antarct. Alp. Res. 2008, 40, 140–147. [Google Scholar] [CrossRef]
- Schellenberger, T.; Dunse, T.; Kääb, A.; Kohler, J.; Reijmer, C.H. Surface speed and frontal ablation of Kronebreen and Kongsbreen, NW Svalbard, from SAR offset tracking. Cryosphere 2015, 9, 2339–2355. [Google Scholar] [CrossRef]
- Palmer, S.; Shepard, A.; Nienow, P.; Joughin, I. Seasonal speedup of the Greenland Ice Sheet linked to routing of surface water. Earth Planet. Sci. Lett. 2011, 302, 423–428. [Google Scholar] [CrossRef]
- McBean, G.; Alekseev, G.; Chen, D.; Foerland, E.; Fyfe, J.; Groisman, P.Y.; King, R.; Melling, H.; Vose, R.; Whitfield, P.H. Chapter 2: Arctic Climate: Past and Present. In Arctic Climate Impact Assessment; Cambridge University Press: New York, NY, USA, 2005; pp. 22–60. [Google Scholar]
- Ye, H.; Yang, D.; Robinson, D. Winter rain on snow and its association with air temperature in northern Eurasia. Hydrol. Process. Int. J. 2008, 22, 2728–2736. [Google Scholar] [CrossRef]
- Westermann, S.; Boike, J.; Langer, M.; Schuler, T.V.; Etzelmüller, B. Modelling the impact of wintertime rain events on the thermal regime of permafrost. Cryosphere 2011, 5, 945–959. [Google Scholar] [CrossRef]
- Hansen, B.B.; Isaksen, K.; Benestad, R.E.; Kohler, J.; Pedersen, Å.Ø.; Loe, L.E.; Coulson, S.J.; Larsen, J.O.; Varpe, Ø. Warmer and wetter winters: Characteristics and implications of an extreme weather event in the High Arctic. Environ. Res. Lett. 2014, 9, 114021. [Google Scholar] [CrossRef]
- Cohen, J.; Ye, H.; Jones, J. Trends and variability in rain-on-snow events. Geophys. Res. Lett. 2015, 42, 7115–7122. [Google Scholar] [CrossRef]
- Forbes, B.C.; Kumpula, T.; Meschtyb, N.; Laptander, R.; Macias-Fauria, M.; Zetterberg, P.; Verdonen, M.; Skarin, A.; Kim, K.Y.; Boisvert, L.N.; et al. Sea ice, rain-on-snow and tundra reindeer nomadism in Arctic Russia. Biol. Lett. 2016, 12, 20160466. [Google Scholar] [CrossRef] [PubMed]
- Łupikasza, E.; Niedźwiedź, T.; Małarzewski, Ł. Występowanie dni z przejściem temperatury powietrza przez 0 °C na wybranych stacjach w atlantyckim sektorze Arktyki. Problemy Klimatologii Polarnej 2013, 23, 121–135. (In Polish) [Google Scholar]
- Winther, J.G.; Bruland, O.; Sand, K.; Killingtveit, Å.; Marechal, D. Snow accumulation distribution on Spitsbergen, Svalbard, in 1997. Polar Res. 1998, 17, 155–164. [Google Scholar] [CrossRef]
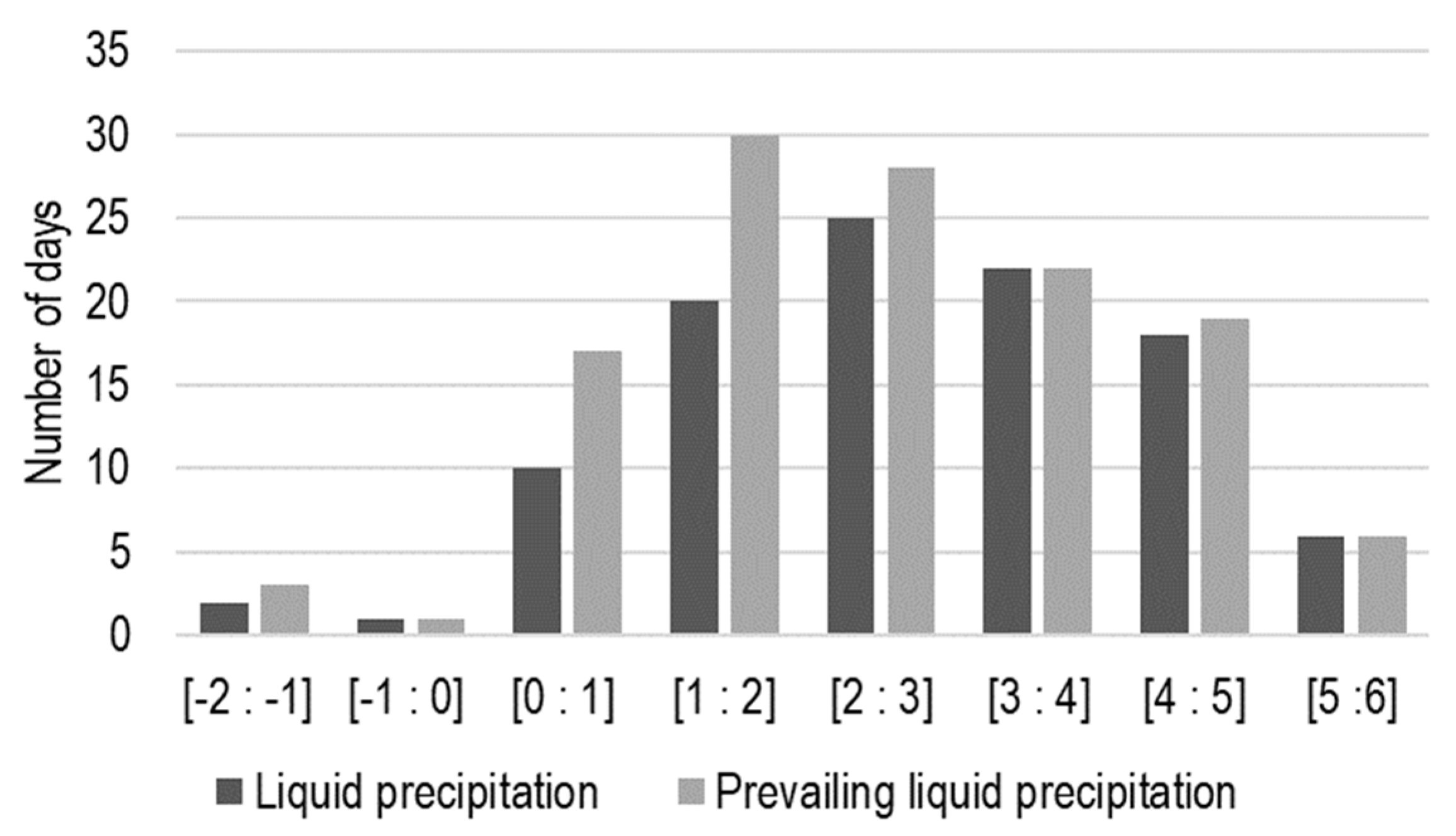

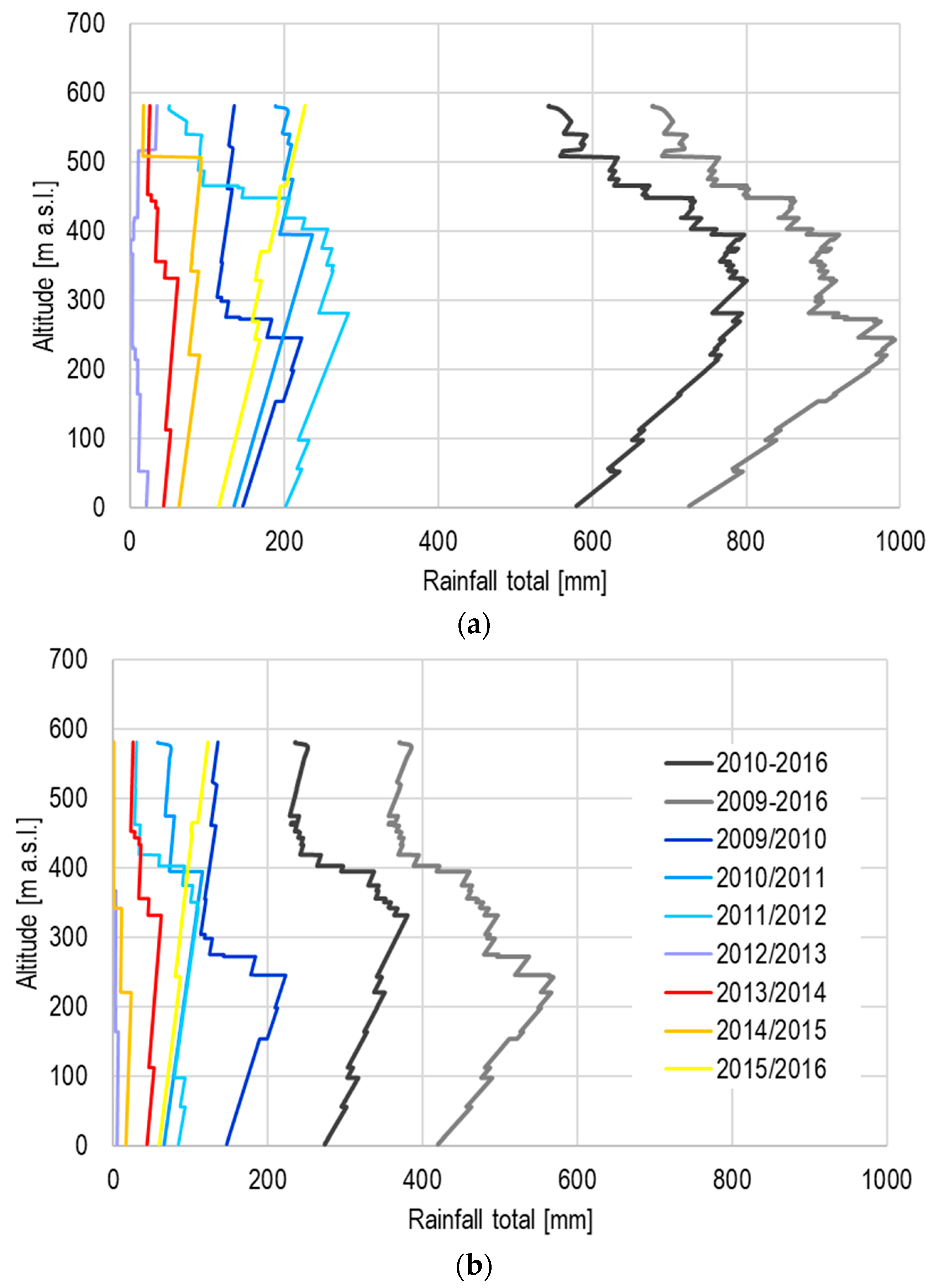

| Totals | ||||||||||||
| Jan | Feb | Mar | Apr | Jun | Jul | Aug | Sep | Oct | Nov | Dec | ||
| L | max | 24 | 23 | 12.3 | 42.7 | 43.1 | 59.7 | 127.6 | 147.4 | 203.8 | 130.3 | 138.2 |
| min | 0 | 0 | 0 | 0 | 0 | 0 | 1.4 | 7.4 | 0 | 0 | 0 | |
| S | max | 70.2 | 52.4 | 49 | 19 | 31.6 | 42.2 | 23.1 | 32.1 | 52.9 | 79.3 | 58.6 |
| min | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | |
| M | max | 41.7 | 54.1 | 40 | 83.8 | 18.9 | 8.4 | 3.1 | 8.9 | 10.3 | 38.3 | 44.9 |
| min | 3.4 | 0.3 | 1.8 | 2.1 | 0 | 0 | 0 | 0 | 0 | 0.3 | 3.1 | |
| Number of Days | ||||||||||||
| Jan | Feb | Mar | Apr | Jun | Jul | Aug | Sep | Oct | Nov | Dec | ||
| L | max | 4 | 5 | 2 | 3 | 5 | 16 | 23 | 25 | 20 | 19 | 8 |
| min | 0 | 0 | 0 | 0 | 0 | 0 | 1 | 4 | 0 | 0 | 0 | |
| S | max | 12 | 12 | 8 | 7 | 9 | 9 | 4 | 6 | 10 | 9 | 12 |
| min | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | |
| M | max | 22 | 19 | 21 | 15 | 16 | 9 | 1 | 4 | 10 | 16 | 19 |
| min | 5 | 2 | 4 | 5 | 0 | 0 | 0 | 0 | 0 | 1 | 4 | |
| Winter Seasons | NoDWRs Oct–May/Acc | WRsT (mm) Oct–May/Acc | IntWRs | AE (mm) | RE (%) |
|---|---|---|---|---|---|
| 2009/2010 | 14/14 | 221/221 | 16/16 | 26/26 | 12/12 |
| 2010/2011 | 15/6 | 207/100 | 14/17 | 27/10 | 27/10 |
| 2011/2012 | 18/10 | 295/119 | 16/12 | 33/18 | 11/15 |
| 2012/2013 | 9/6 | 28/6 | 3/1 | 13/6 | 46/100 |
| 2013/2014 | 8/7 | 61/51 | 8/9 | 14/14 | 22/27 |
| 2014/2015 | 8/3 | 95/22 | 12/7 | 15/5 | 16/23 |
| 2015/2016 | 21/12 | 177/91 | 8/8 | 38/21 | 21/23 |
| TOTAL | 93/58 | 1085/621 | 12/11 | 166/100 | 15/16 |
| Index | Unit | 2009/2010 | 2010/2011 | 2011/2012 | 2012/2013 | 2013/2014 | 2014/2015 | 2015/2016 |
|---|---|---|---|---|---|---|---|---|
| Bw | mm | 1063 | 1123 | 1088 | 669 | 936 | 1002 | 857 |
| bw H4 | mm | 720 | 760 | 728 | 584 | 740 | 660 | 544 |
| bw H6 | mm | 1028 | 1250 | 968 | 644 | 940 | 1040 | 828 |
| bw H9 | mm | 1652 | 1700 | 1640 | 976 | 1250 | 1384 | 1208 |
| NoDWRs | days | 14 | 6 | 10 | 6 | 7 | 3 | 12 |
| BwWRs | % | 21 | 9 | 11 | 1 | 7 | 2 | 11 |
| AWS | 2009/2010 | 2010/2011 | 2011/2012 | 2012/2013 | 2013/2014 | 2014/2015 | 2015/2016 |
|---|---|---|---|---|---|---|---|
| H4 | 29 | 12 | 12 | 0 | 7 | 3 | 15 |
| H6 | 12 | 8 | 10 | 0 | 6 | 1 | 10 |
| H9 | 8 | 4 | 2 | 0 | 3 | 0 | 8 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Łupikasza, E.B.; Ignatiuk, D.; Grabiec, M.; Cielecka-Nowak, K.; Laska, M.; Jania, J.; Luks, B.; Uszczyk, A.; Budzik, T. The Role of Winter Rain in the Glacial System on Svalbard. Water 2019, 11, 334. https://doi.org/10.3390/w11020334
Łupikasza EB, Ignatiuk D, Grabiec M, Cielecka-Nowak K, Laska M, Jania J, Luks B, Uszczyk A, Budzik T. The Role of Winter Rain in the Glacial System on Svalbard. Water. 2019; 11(2):334. https://doi.org/10.3390/w11020334
Chicago/Turabian StyleŁupikasza, Ewa B., Dariusz Ignatiuk, Mariusz Grabiec, Katarzyna Cielecka-Nowak, Michał Laska, Jacek Jania, Bartłomiej Luks, Aleksander Uszczyk, and Tomasz Budzik. 2019. "The Role of Winter Rain in the Glacial System on Svalbard" Water 11, no. 2: 334. https://doi.org/10.3390/w11020334
APA StyleŁupikasza, E. B., Ignatiuk, D., Grabiec, M., Cielecka-Nowak, K., Laska, M., Jania, J., Luks, B., Uszczyk, A., & Budzik, T. (2019). The Role of Winter Rain in the Glacial System on Svalbard. Water, 11(2), 334. https://doi.org/10.3390/w11020334






