Vulnerability Analysis of the Venetian Littoral and Adopted Mitigation Strategy
Abstract
1. Introduction
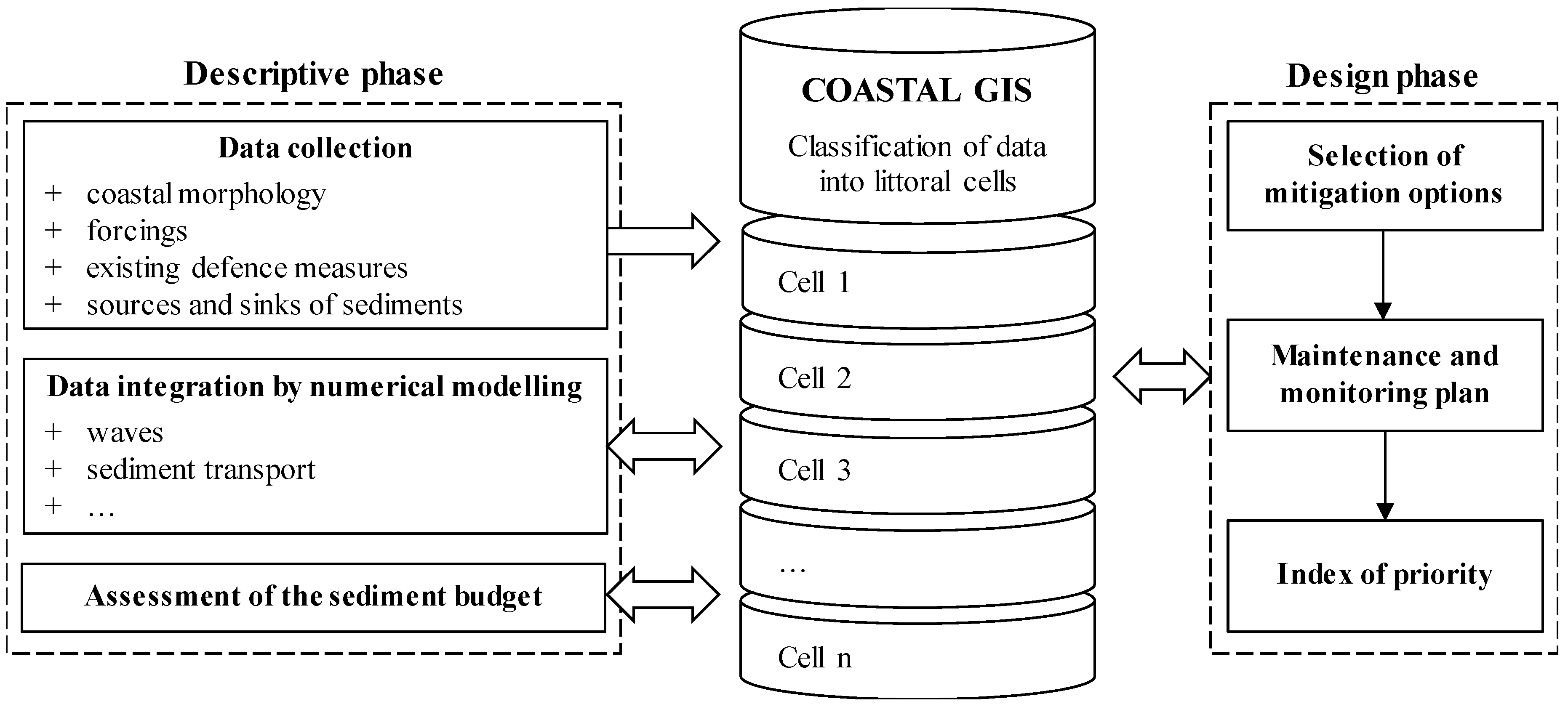
2. Methodology
2.1. Descriptive Phase
2.1.1. Data Collection and Harmonisation
2.1.2. Data Integration by Numerical Modelling
2.1.3. Assessment of Sediment Budget
2.2. Design Phase
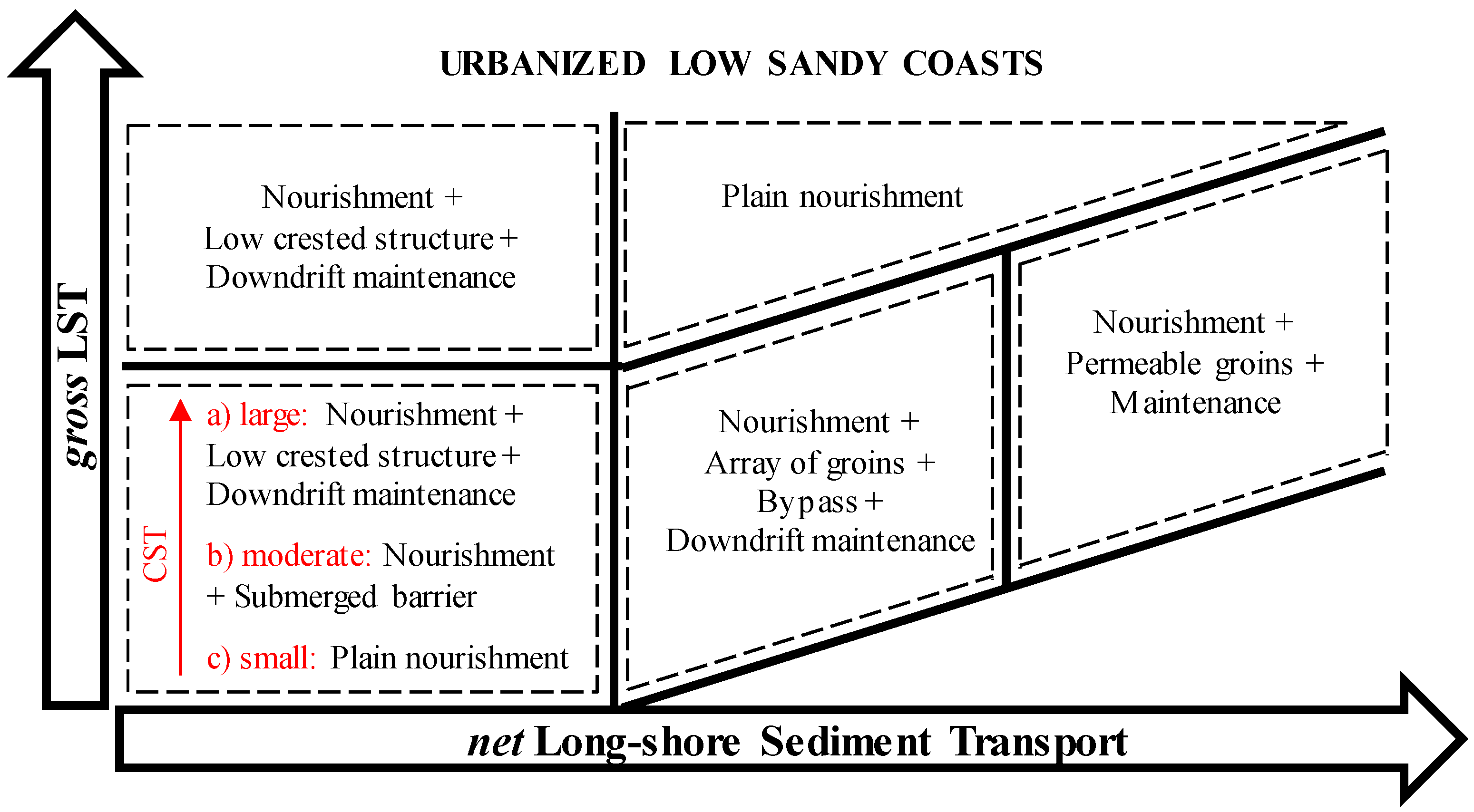
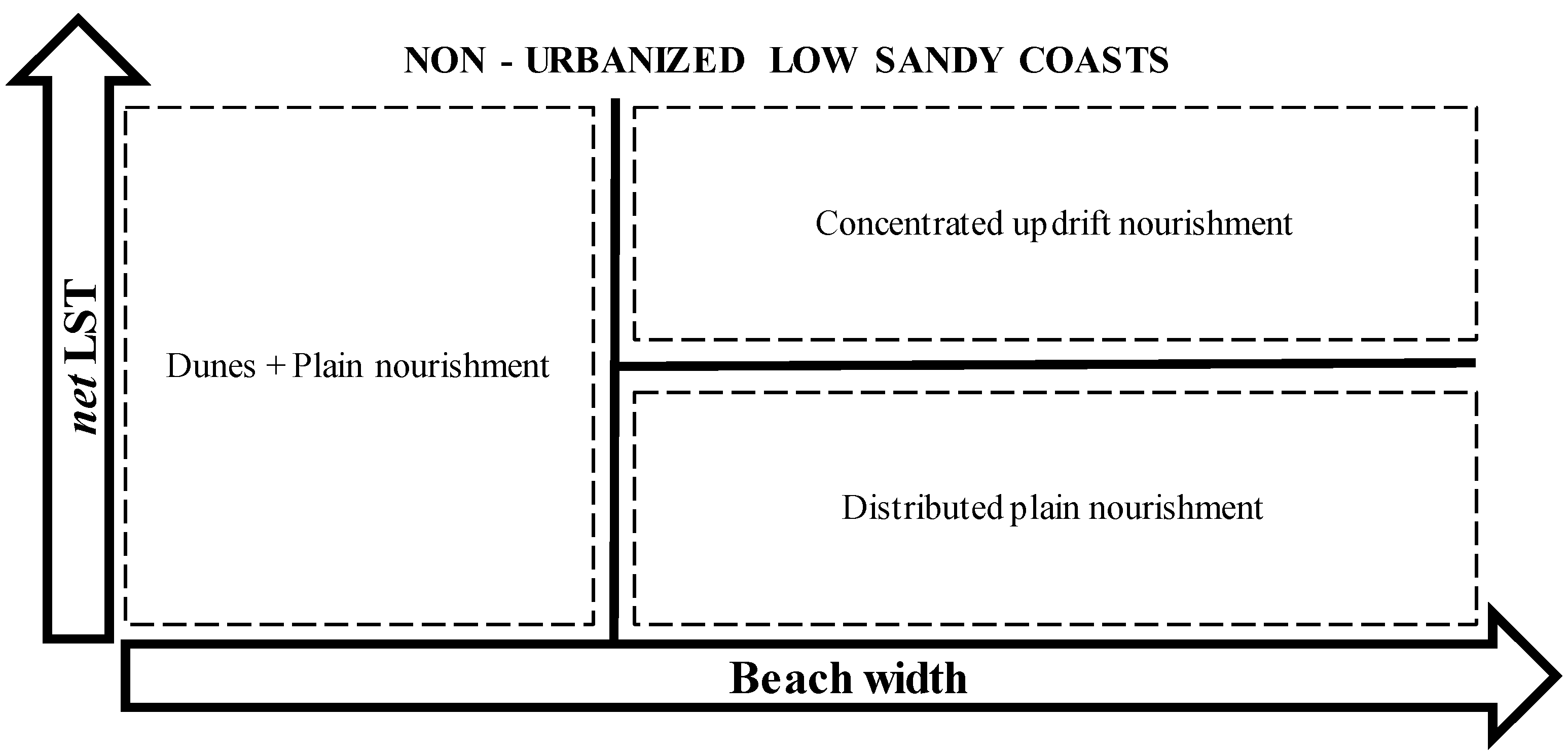
2.2.1. Mitigation Options and Selection Criteria
2.2.2. Maintenance and Monitoring Plan
- Co-ordinates and details of the methodology used to integrate information on waves, fluvial sediment transport, bathymetric profiles, etc. The plan must have a regional scale and be planned over a broad period, possibly using a common approach; it must also focus closely on the expected results.
- Results of monitoring. Each mitigation option is typically associated with a monitoring plan designed to check maintenance effectiveness and verify whether the option selected has achieved the objectives. It is highly advisable to plan an annual topo-bathymetric survey for the nourishment and additional (less frequent but specific) surveys for dunes, barriers and groins etc. These surveys should then be analysed and compared with maintenance plan predictions so that remedial action can be taken.
- Activities authorized by Coastal Authorities, such as dredging and nourishment, are to be recorded so that it is clear on which cell they were carried out, i.e., by subdividing the total dredged and nourished volumes according to the semi-cells shown in the plan. This is of great help for overall coastal management since it reduces uncertainty about total volumes.
- Any non-coastal-defence-related activities that may still contribute to the general knowledge framework.
2.2.3. Index of Priority
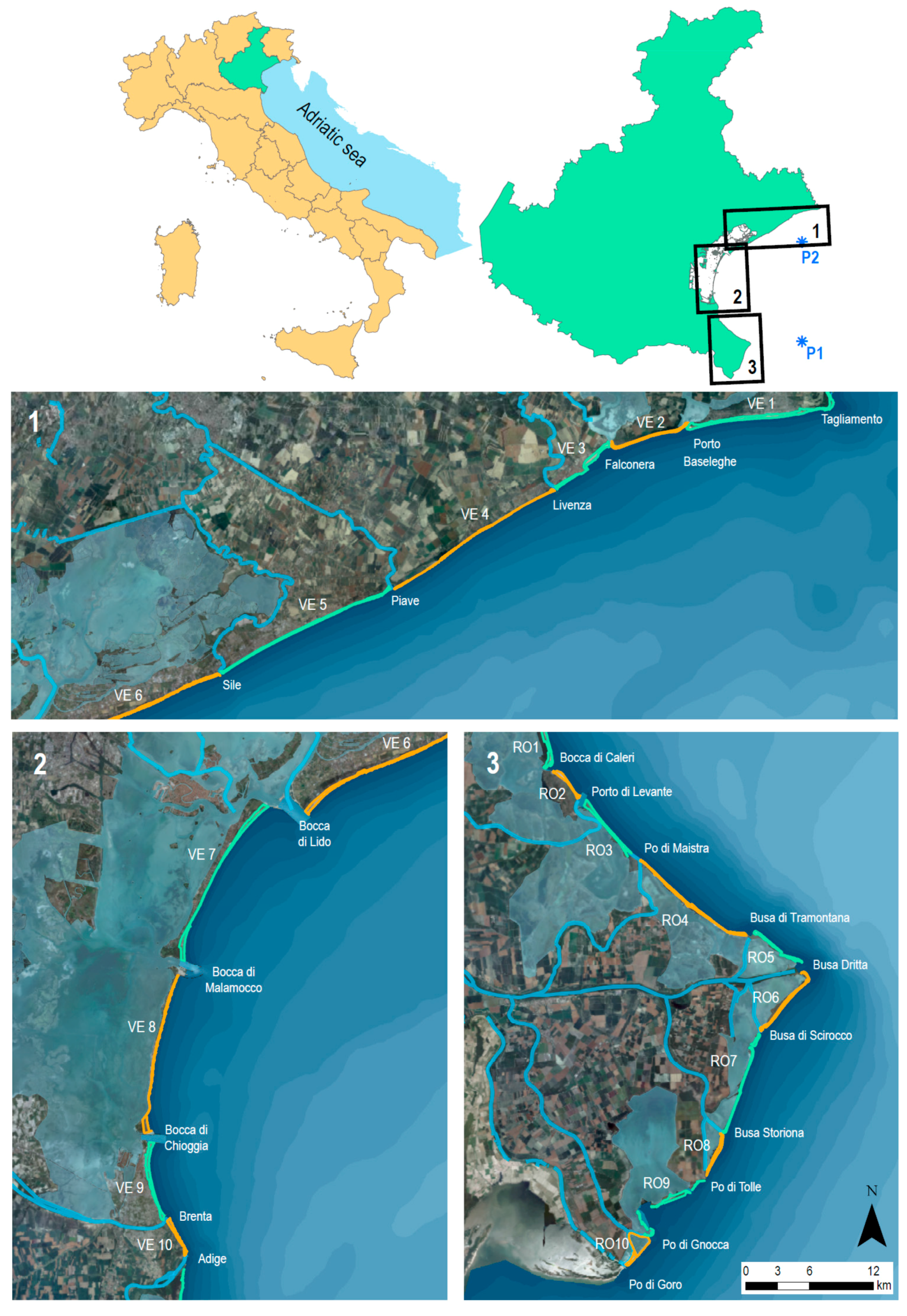
3. The Venetian Littoral
3.1. Description of the Area and Assessment of Sediment Budget
3.2. Design Phase
- Coast right of the mouth of the River Tagliamento (VE1): intensive erosive processes due to reduced river supply and a system of attached breakwaters that trap the long-shore sediment transport.
- VE4 cell (Porto Santa Margherita, Duna Verde and Eraclea-Venice): erosive processes due to a decreased river sediment transport supply and the presence of reflective structures along the coast.
- Coast right of the mouth of the River Piave (VE5): intensive erosive processes due to decreased river sediment supply.
- VE 8 cell (Pellestrina-Venice): huge nourishment was conducted in the late 1990s. The effectiveness of this measure is now reduced, and intensive erosive processes are affecting this littoral. This cell is a thin barrier/island separating the Venice lagoon from the sea.
- Scardovari spit (VE9), which confined the Scardovari lagoon (in the South of the Po Delta): this vulnerable sand formation is subject to erosion processes and to episodic overwashing, which endangers the lagoon environment.
3.3. Example for RO1 Littoral Cell
- Constructing a series of groins (min. 2) in the central zone in order to reduce erosion on this stretch of coast.
- Maintenance dredging the Caleri inlet (~70,000 m3/year) in order to nourish the northern and central areas and to ensure that boats can continue to navigate the lagoon inlet.
- A monitoring program.
4. Conclusions
Author Contributions
Acknowledgments
Conflicts of Interest
References
- French, J.; Payo, A.; Murray, B.; Orford, J.; Eliot, M.; Cowell, P. Appropriate complexity for the prediction of coastal and estuarine geomorphic behaviour at decadal to centennial scales. Geomorphology 2016, 256, 3–16. [Google Scholar] [CrossRef]
- Haasnoot, M.; Kwakkel, J.H.; Walker, W.E.; ter Maat, J. Dynamic adaptive policy pathways: A method for crafting robust decisions for a deeply uncertain world. Glob. Environ. Chang. 2013, 23, 485–498. [Google Scholar] [CrossRef]
- Van Maanen, B.; Nicholls, R.J.; French, J.R.; Barkwith, A.; Bonaldo, D.; Burningham, H.; Brad Murray, A.; Payo, A.; Sutherland, J.; Thornhill, G.; et al. Simulating mesoscale coastal evolution for decadal coastal management: A new framework integrating multiple, complementary modelling approaches. Geomorphology 2016, 256, 68–80. [Google Scholar] [CrossRef]
- Hinkel, J.; Lincke, D.; Vafeidis, A.T.; Perrette, M.; Nicholls, R.J.; Tol, R.S.J.; Marzeion, B.; Fettweis, X.; Ionescu, C.; Levermann, A. Coastal flood damage and adaptation costs under 21st century sea-level rise. Proc. Natl. Acad. Sci. USA 2014, 111, 3292–3297. [Google Scholar] [CrossRef] [PubMed]
- Weisse, R.; Bellafiore, D.; Menéndez, M.; Méndez, F.; Nicholls, R.J.; Umgiesser, G.; Willems, P. Changing extreme sea levels along european coasts. Coast. Eng. 2014, 87, 4–14. [Google Scholar] [CrossRef]
- Toimil, A.; Losada, I.J.; Camus, P.; Díaz-Simal, P. Managing coastal erosion under climate change at the regional scale. Coast. Eng. 2017, 128, 106–122. [Google Scholar] [CrossRef]
- Stocker, T.F.; Qin, D.; Plattner, G.-K.; Tignor, M.; Allen, S.K.; Boschung, J.; Nauels, A.; Xia, Y.; Bex, V.; Midgley, P.M. IPCC Climate Change 2013: The Physical Science Basis, WG1. In The Fifth Assessment Report of the Intergovernmental Panel on Climate Change; Cambridge University Press: Cambridge, UK, 2013. [Google Scholar]
- Antonioli, F.; Anzidei, M.; Amorosi, A.; Presti, V.L.; Mastronuzzi, G.; Deiana, G.; De Falco, G.; Fontana, A.; Fontolan, G.; Lisco, S.; et al. Sea-level rise and potential drowning of the Italian coastal plains: Flooding risk scenarios for 2100. Quat. Sci. Rev. 2017, 158, 29–43. [Google Scholar] [CrossRef]
- Bezzi, A.; Pillon, S.; Martinucci, D.; Fontolan, G. Inventory and conservation assessment for the management of coastal dunes, Veneto coasts, Italy. J. Coast. Conserv. 2018, 22, 503–518. [Google Scholar] [CrossRef]
- Montanari, R.; Marasmi, C. New Tools for Coastal Management in Emilia-Romagna; Technical Report: Bologna, Italy, 2012; Available online: http://www.coastance.eu (accessed on 4 April 2018).
- Zanuttigh, B.; Martinelli, L.; Lamberti, A.; Moschella, P.; Hawkins, S.; Marzetti, S.; Ceccherelli, V.U. Environmental design of coastal defence in lido di dante, italy. Coast. Eng. 2005, 52, 1089–1125. [Google Scholar] [CrossRef]
- Doddy, P.; Ferreria, M.; Lombardo, S.; Luicus, I.; Misdorp, R.; Niesing, H.; Smallegange, M. Living with Coastal Erosion in Europe–Sediment and Space for Sustainability; Results from the Eurossion Study; European Commission Publication: Luxembourg, Luxembourg, 2004. [Google Scholar]
- Zanuttigh, B. Coastal flood protection: What perspective in a changing climate? The theseus approach. Environ. Sci. Policy 2011, 14, 845–863. [Google Scholar] [CrossRef]
- Ciavola, P.; Ferreira, O.; Haerens, P.; Van Koningsveld, M.; Armaroli, C. Storm impacts along european coastlines. Part 2: Lessons learned from the MICORE project. Environ. Sci. Policy 2011, 14, 924–933. [Google Scholar] [CrossRef]
- Armaroli, C.; Duo, E. Validation of the coastal storm risk assessment framework along the Emilia-Romagna coast. Coast. Eng. 2018, 134, 159–167. [Google Scholar] [CrossRef]
- Zanuttigh, B.; Simcic, D.; Bagli, S.; Bozzeda, F.; Pietrantoni, L.; Zagonari, F.; Hoggart, S.; Nicholls, R.J. Theseus decision support system for coastal risk management. Coast. Eng. 2014, 87, 218–239. [Google Scholar] [CrossRef]
- Torresan, S.; Critto, A.; Rizzi, J.; Zabeo, A.; Furlan, E.; Marcomini, A. Desyco: A decision support system for the regional risk assessment of climate change impacts in coastal zones. Ocean Coast. Manag. 2016, 120, 49–63. [Google Scholar] [CrossRef]
- Vafeidis, A.T.; Nicholls, R.J.; McFadden, L.; Tol, R.S.J.; Hinkel, J.; Spencer, T.; Grashoff, P.S.; Boot, G.; Klein, R.J.T. A new global coastal database for impact and vulnerability analysis to sea-level rise. J. Coast. Res. 2008, 244, 917–924. [Google Scholar] [CrossRef]
- Van Dongeren, A.; Ciavola, P.; Viavattene, C.; de Kleermaeker, S.; Martinez, G.; Ferreira, O.; Costa, C.; McCall, R. Risc-kit: Resilience-increasing strategies for coasts-toolkit. J. Coast. Res. 2014, 70, 366–371. [Google Scholar] [CrossRef]
- Stelljes, N.; Martinez, G.; McGlade, K. Introduction to the RISC-KIT web based management guide for DRR in European coastal zones. Coast. Eng. 2018, 134, 73–80. [Google Scholar] [CrossRef]
- Preti, M. Stato del litorale Emiliano-Romagnolo All’anno 2007 e Piano Decennale di Gestione; Technical Report; I quaderni di ARPA Emilia Romagna: Bologna, Italy, 2008. [Google Scholar]
- Petrillo, A.F.; Bruno, M.F.; Francioso, R.; Nobile, B.; Tomasicchio, R.; D’alessandro, F.; Di Pace, P.; Gencarelli, R. Studi Propedeutici per la Predisposizione del Piano Stralcio della Dinamica delle Coste. AdB Puglia, Politecnico di Bari, Università del Salento, Allegato 3.1 & 3.2, 2010. Available online: old.regione.puglia.it/web/files/demaniomarittimo/06__All.3.2__Strutture_per_la_difesa_delle_coste.pdf; old.regione.puglia.it/web/files/demaniomarittimo/05__All.3.1__Strutture_di_mitigazione_del_rischio.pdf (accessed on 4 April 2018).
- Ruol, P.; Martinelli, L.; Favaretto, C. Gestione Integrata della zona Costiera. Studio e Monitoraggio per la Definizione degli Interventi di Difesa dei Litorali Dall’erosione nella Regione Veneto—Linee Guida; Edizioni Libreria Progetto: Padova, Italy, 2016. [Google Scholar]
- Simeoni, U.; Corbau, C. A review of the delta Po evolution (Italy) related to climatic changes and human impacts. Geomorphology 2009, 107, 64–71. [Google Scholar] [CrossRef]
- Carbognin, L.; Teatini, P.; Tosi, L. Eustacy and land subsidence in the Venice lagoon at the beginning of the new millennium. J. Mar. Syst. 2004, 51, 345–353. [Google Scholar] [CrossRef]
- Pomaro, A.; Cavaleri, L.; Lionello, P. Climatology and trends of the Adriatic Sea wind waves: Analysis of a 37-year long instrumental data set. Int. J. Climatol. 2017, 37, 4237–4250. [Google Scholar] [CrossRef]
- California Coastal Sediment Management Workgroup. California Coastal Sediment Master Plan Status Report; Draft for Public Review and Comment; California Geological Survey: Santa Rosa, CA, USA, 2006.
- Bartlett, D.; Smith, J.L. GIS for Coastal Zone Management; CRC Press: Boca Raton, FL, USA, 2004. [Google Scholar]
- Wright, D.; Bartlett, D. Marine and Coastal Geographical Information Systems; Taylor and Francis: London, UK, 2000. [Google Scholar]
- Williams, A.; Rangel-Buitrago, N.G.; Pranzini, E.; Anfuso, G. The management of coastal erosion. Ocean Coast. Manag. 2017. [Google Scholar] [CrossRef]
- Payo, A.; Favis-Mortlock, D.; Dickson, M.; Hall, J.W.; Hurst, M.D.; Walkden, M.J.A.; Townend, I.; Ives, M.C.; Nicholls, R.J.; Ellis, M.A. Coastal Modelling Environment version 1.0: A framework for integrating landform-specific component models in order to simulate decadal to centennial morphological changes on complex coasts. Geosci. Model Dev. 2017, 10, 2715–2740. [Google Scholar] [CrossRef]
- Cavaleri, L.; Abdalla, S.; Benetazzo, L.; Bertotti, J.-R.; Bidlot, Ø.; Breivik, S.; Carniel, R.E.; Jensen, J.; Portilla-Yandun, W.E.; Rogers, A.; et al. Wave modelling in coastal and inner seas. Prog. Oceanogr. 2018. [Google Scholar] [CrossRef]
- Roy, C.; Kovordányi, R. Tropical cyclone track forecasting techniques—A review. Atmos. Res. 2012, 104, 40–69. [Google Scholar] [CrossRef]
- Favaretto, C.; Martinelli, L.; Ruol, P. Raster Based Model of Inland Coastal Flooding Propagation Using Linearized Bottom Friction and Application to a Real Case Study in Caorle, Venice (IT). In Proceedings of the Twenty-Eight International Ocean and Polar Engineering Conference (ISOPE2018), Sapporo, Japan, 10–15 June 2018. [Google Scholar]
- Martinelli, L.; Zanuttigh, B.; Corbau, C. Assessment of coastal flooding hazard along the Emilia Romagna littoral, IT. Coast. Eng. 2010, 57, 1042–1058. [Google Scholar] [CrossRef]
- Samaras, A.G.; Koutitas, C.G. The impact of catchment management on coastal morphology. The case of Fourka in Greece. J. Coast. Res. 2009, 1686–1690. [Google Scholar]
- Samaras, A.G.; Koutitas, C.G. Comparison of three longshore sediment transport rate formulae in shoreline evolution modeling near stream mouths. Ocean Eng. 2014, 92, 255–266. [Google Scholar] [CrossRef]
- Mikhail, E.D.; Gracie, G. Analysis & Adjustment of Survey Measurements; Van Nostrand Reinhold: New York, NY, USA, 1981. [Google Scholar]
- Burcharth, H.F.; Zanuttigh, B.; Andersen, T.L.; Lara, J.L.; Steendam, G.J.; Ruol, P.; Sergent, P.; Ostrowski, R.; Silva, R.; Martinelli, L.; et al. Innovative Engineering Solutions and Best Practices to Mitigate Coastal Risk. Coast. Risk Manag. Chang. Clim. 2014, 55–170. [Google Scholar] [CrossRef]
- Ruol, P.; Martinelli, L.; Favaretto, C.; Scroccaro, D. Innovative Sand Groin Beach Nourishment with Environmental, Defense and Recreational Purposes. In Proceedings of the Twenty-Eight International ocean and polar engineering conference (ISOPE2018), Sapporo, Japan, 10–15 June 2018. [Google Scholar]
- Hoggart, S.; Hawkins, S.J.; Bohn, K.; Airoldi, L.; van Belzen, J.; Bichot, A.; Bilton, D.T.; Bouma, T.J.; Colangelo, M.A.; Davies, A.J.; et al. Ecological approaches to coastal risk mitigation. Coast. Risk Manag. Chang. Clim. 2015, 171–236. [Google Scholar] [CrossRef]
- Vanderlinden, J.P.; Baztan, J.; Coates, T.; Dávila, O.G.; Hissel, F.; Kane, I.O.; Koundouri, P.; McFadden, L.; Parker, D.; Penning-Rowsell, E.; et al. Nonstructural approaches to coastal risk mitigations. Coast. Risk Manag. Chang. Clim. 2015, 237–274. [Google Scholar] [CrossRef]
- Bergillos, R.J.; López-Ruiz, A.; Principal-Gómez, D.; Ortega-Sánchez, M. An integrated methodology to forecast the efficiency of nourishment strategies in eroding deltas. Sci. Total Environ. 2018, 613, 1175–1184. [Google Scholar] [CrossRef] [PubMed]
- Stronkhorst, J.; Huisman, B.; Giardino, A.; Santinelli, G.; Santos, F.D. Sand nourishment strategies to mitigate coastal erosion and sea level rise at the coasts of Holland (The Netherlands) and Aveiro (Portugal) in the 21st century. Ocean Coast. Manag. 2018, 156, 266–276. [Google Scholar] [CrossRef]
- Dean, R.G. Beach Nourishment: Theory and Practice; World Scientific Publishing Company: Toh Tuck Link, Singapore, 2003. [Google Scholar]
- Zanuttigh, B.; Martinelli, L.; Lamberti, A. Wave overtopping and piling-up at permeable low crested structures. Coast. Eng. 2008, 55, 484–498. [Google Scholar] [CrossRef]
- Buccino, M.; Calabrese, M. Conceptual approach for prediction of wave transmission at low-crested breakwaters. J. Waterw. Port Coast. Ocean Eng. 2007, 133, 213–224. [Google Scholar] [CrossRef]
- Martinelli, L.; Zanuttigh, B.; De Nigris, N.; Preti, M. Sand bag barriers for coastal protection along the emilia romagna littoral, northern adriatic sea, Italy. Geotext. Geomembr. 2011, 29, 370–380. [Google Scholar] [CrossRef]
- Hawkins, S.J.; Burcharth, H.F.; Zanuttigh, B.; Lamberti, A. Environmental Design Guidelines for Low Crested Coastal Structures; Elsevier: Oxford, UK, 2010. [Google Scholar]
- Basco, D.R.; Pope, J. Groin Functional Design Guidance from the Coastal Engineering Manual; Coastal Education & Research Foundation, Inc.: Collier County, FL, USA, 2004; pp. 121–130. [Google Scholar]
- Raudkivi, A.J. Permeable pile groins. J. Waterw. Port Coast Ocean Eng. 1996, 122, 267–272. [Google Scholar] [CrossRef]
- Kristensen, S.E.; Drønen, N.; Deigaard, R.; Fredsoe, J. Impact of groyne fields on the littoral drift: A hybrid morphological modelling study. Coast. Eng. 2016, 111, 13–22. [Google Scholar] [CrossRef]
- Van der Nat, A.; Vellinga, P.; Leemans, R.; Van Slobbe, E. Ranking coastal flood protection designs from engineered to nature-based. Ecol. Eng. 2016, 87, 80–90. [Google Scholar] [CrossRef]
- Stive, M.J.F.; de Schipper, M.A.; Luijendijk, A.P.; Aarninkhof, S.G.J.; van Gelder-Maas, C.; van Thiel de Vries, J.S.M.; de Vries, S.; Henriquez, M.; Marx, S.; Ranasinghe, R. A new alternative to saving our beaches from sea-level rise: The sand engine. J. Coast. Res. 2013, 290, 1001–1008. [Google Scholar] [CrossRef]
- Hanley, M.E.; Hoggart, S.P.G.; Simmonds, D.J.; Bichot, A.; Colangelo, M.A.; Bozzeda, F.; Heurtefeux, H.; Ondiviela, B.; Ostrowski, R.; Recio, M.; et al. Shifting sands? coastal protection by sand banks, beaches and dunes. Coast. Eng. 2014, 87, 136–146. [Google Scholar] [CrossRef]
- Gracia, C.A.; Rangel-Buitrago, N.; Oakley, J.A.; Williams, A. Use of ecosystems in coastal erosion management. Ocean Coast. Manag. 2018, 156, 277–289. [Google Scholar] [CrossRef]
- Villatoro, M.; Silva, R.; Méndez, F.J.; Zanuttigh, B.; Pan, S.; Trifonova, E.; Losada, I.J.; Izaguirre, C.; Simmonds, D.; Reeve, D.E.; et al. An approach to assess flooding and erosion risk for open beaches in a changing climate. Coast. Eng. 2014, 87, 50–76. [Google Scholar] [CrossRef]
- McCabe, M.V.; Stansby, P.K.; Apsley, D.D. Random wave runup and overtopping a steep sea wall: Shallow-water and boussinesq modelling with generalised breaking and wall impact algorithms validated against laboratory and field measurements. Coast. Eng. 2013, 74, 33–49. [Google Scholar] [CrossRef]
- Castellino, M.; Sammarco, P.; Romano, A.; Martinelli, L.; Ruol, P.; Franco, L.; De Girolamo, P. Large impulsive forces on recurved parapets under non-breaking waves. A numerical study. Coast. Eng. 2018, 136, 1–15. [Google Scholar] [CrossRef]
- Damiani, L.; Aristodemo, F.; Saponieri, A.; Verbeni, B.; Veltri, P.; Vicinanza, D. Full-scale experiments on a beach drainage system: Hydrodynamic effects inside beach. J. Hydraul. Res. 2011, 49, 44–54. [Google Scholar] [CrossRef]
- Damiani, L.; Vicinanza, D.; Aristodemo, F.; Saponieri, A.; Corvaro, S. Experimental investigation on wave set-up and nearshore velocity field in presence of a BDS. J. Coast. Res. 2011, SI 64, 55–59. [Google Scholar]
- Ruol, P.; Zanuttigh, B.; Martinelli, L.; Kofoed, P.; Frigaard, P. Near-shore floating wave energy converters: Applications for coastal protection. Coast. Eng. Proc. 2011, 1. [Google Scholar] [CrossRef]
- Mendoza, E.; Silva, R.; Zanuttigh, B.; Angelelli, E.; Lykke Andersen, T.; Martinelli, L.; Nørgaard, J.Q.H.; Ruol, P. Beach response to wave energy converter farms acting as coastal defence. Coast. Eng. 2014, 87, 97–111. [Google Scholar] [CrossRef]
- Benassai, G.; Chirico, F.; Corsini, S. Una metodologia sperimentale per la definizione del rischio da inondazione costiera. Stud. Costieri 2009, 16, 51–72. [Google Scholar]
- Canestrelli, P.; Mandich, M.; Pirazzoli, P.A.; Tomasin, A. Wind, depression and seiches: Tidal perturbations in Venice (1951–2000). Centro Previsioni e Segnalazioni Maree. Comune Venezia 2001, 1–104. [Google Scholar]
- Booij, N.; Ris, R.C.; Holthuijsen, L.H. A third-generation wave model for coastal regions: 1. Model description and validation. J. Geophys. Res. Ocean. 1999, 104, 7649–7666. [Google Scholar] [CrossRef]
- Dean, R.G. Equilibrium Beach Profiles: US Atlantic and Gulf Coasts; Department of Civil Engineering and College of Marine Studies; Technical Report 12; University of Delaware: Newark, DE, USA, 1977. [Google Scholar]
- Hanson, H.; Kraus, N.C. GENESIS: Generalized Model for Simulating Shoreline Change; Report 1. Technical Reference; Technical Report CERC-89-19; Coastal Engineering Research Center: Vicksburg, MS, USA, 1989. [Google Scholar]
- Lanzoni, S.; Luchi, R.; Bolla Pittaluga, M. Modeling the morphodynamic equilibrium of an intermediate reach of the po river (Italy). Adv. Water Resour. 2015, 81, 95–102. [Google Scholar] [CrossRef]
- Bijker, E.W. Some Considerations about Scales for Coastal Models with Movable Bed. Ph.D. Thesis, Delft Hydraulics Laboratory Publication, Delft, The Netherlands, 1967. [Google Scholar]
- Bayram, A.; Larson, M.; Miller, H.C.; Kraus, N.C. Cross-shore distribution of longshore sediment transport: Comparison between predictive formulas and field measurements. Coast. Eng. 2001, 44, 79–99. [Google Scholar] [CrossRef]
- Schoonees, J.S.; Theron, A.K. Evaluation of 10 cross-shore sediment transport/morphological models. Oceanogr. Lit. Rev. 1996, 2, 136. [Google Scholar] [CrossRef]
- Ferretti, A.; Prati, C.; Rocca, F. Permanent scatterers in sar interferometry. IEEE Trans. Geosci. Remote. Sens. 2001, 39, 8–20. [Google Scholar] [CrossRef]
- Lanari, R.; Mora, O.; Manunta, M.; Mallorqui, J.J.; Berardino, P.; Sansosti, E. A small-baseline approach for investigating deformations on full-resolution differential sar interferograms. IEEE Trans. Geosci. Remote Sens. 2004, 42, 1377–1386. [Google Scholar] [CrossRef]
- Fontolan, G.; Bezzi, A.; Martinucci, D.; Pillon, S.; Popesso, C.; Rizzetto, F. Sediment budget and management of the Veneto beaches, Italy: An application of the modified Littoral Cells Management System (SICELL). Proceedings of Coastal and Maritime Mediterranean Conference, Ferrare, Italy, 25–27 November 2015. [Google Scholar]


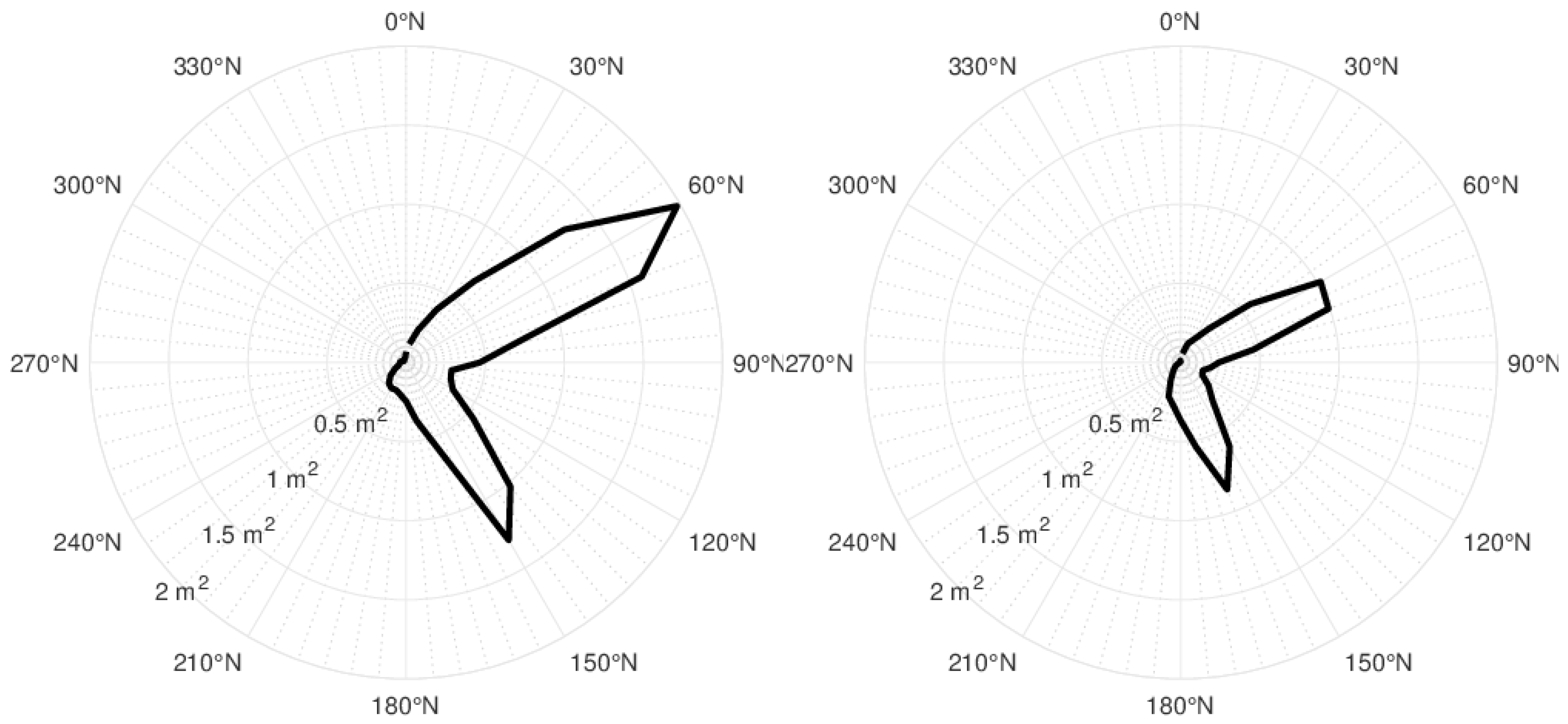
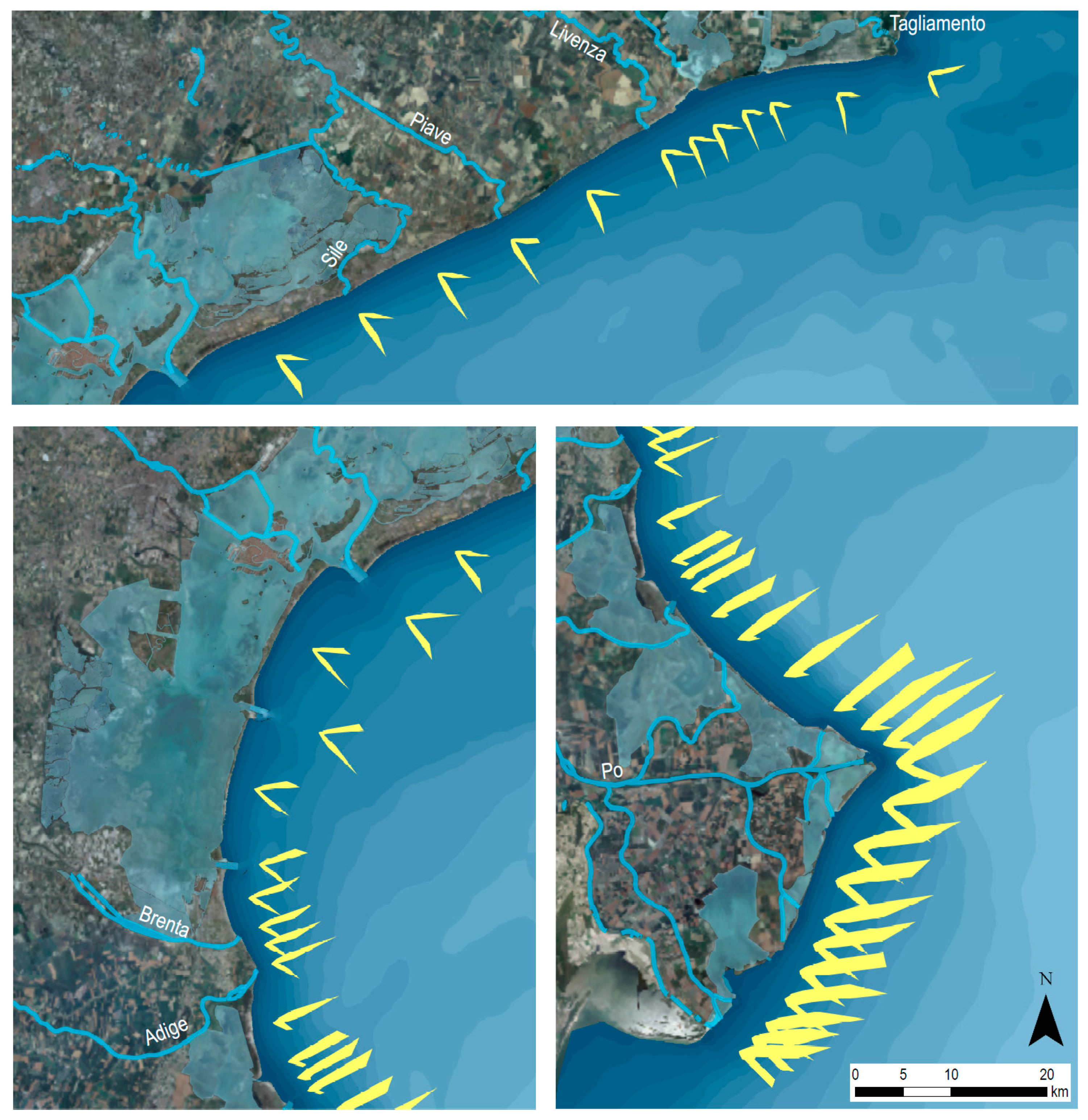

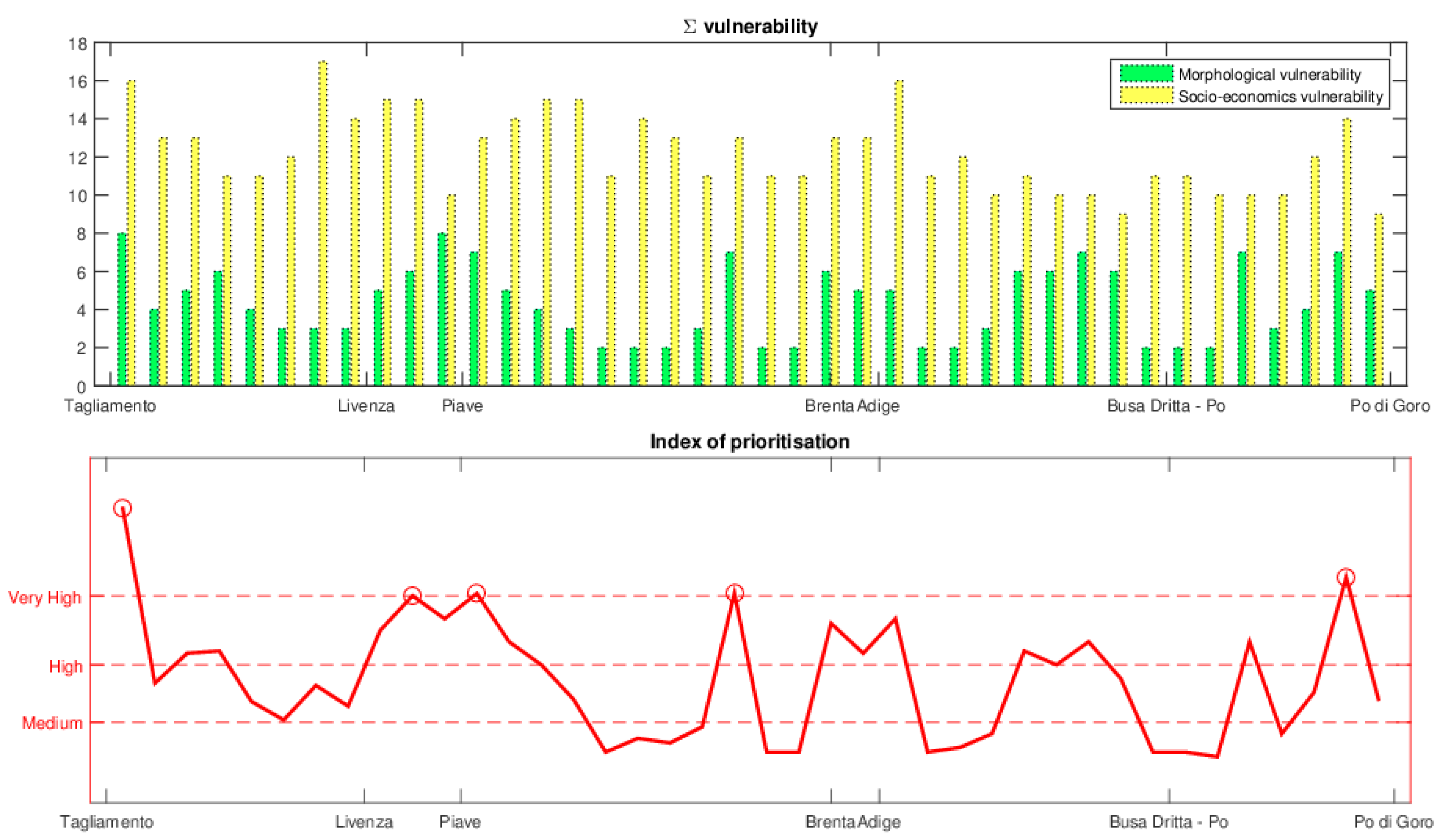
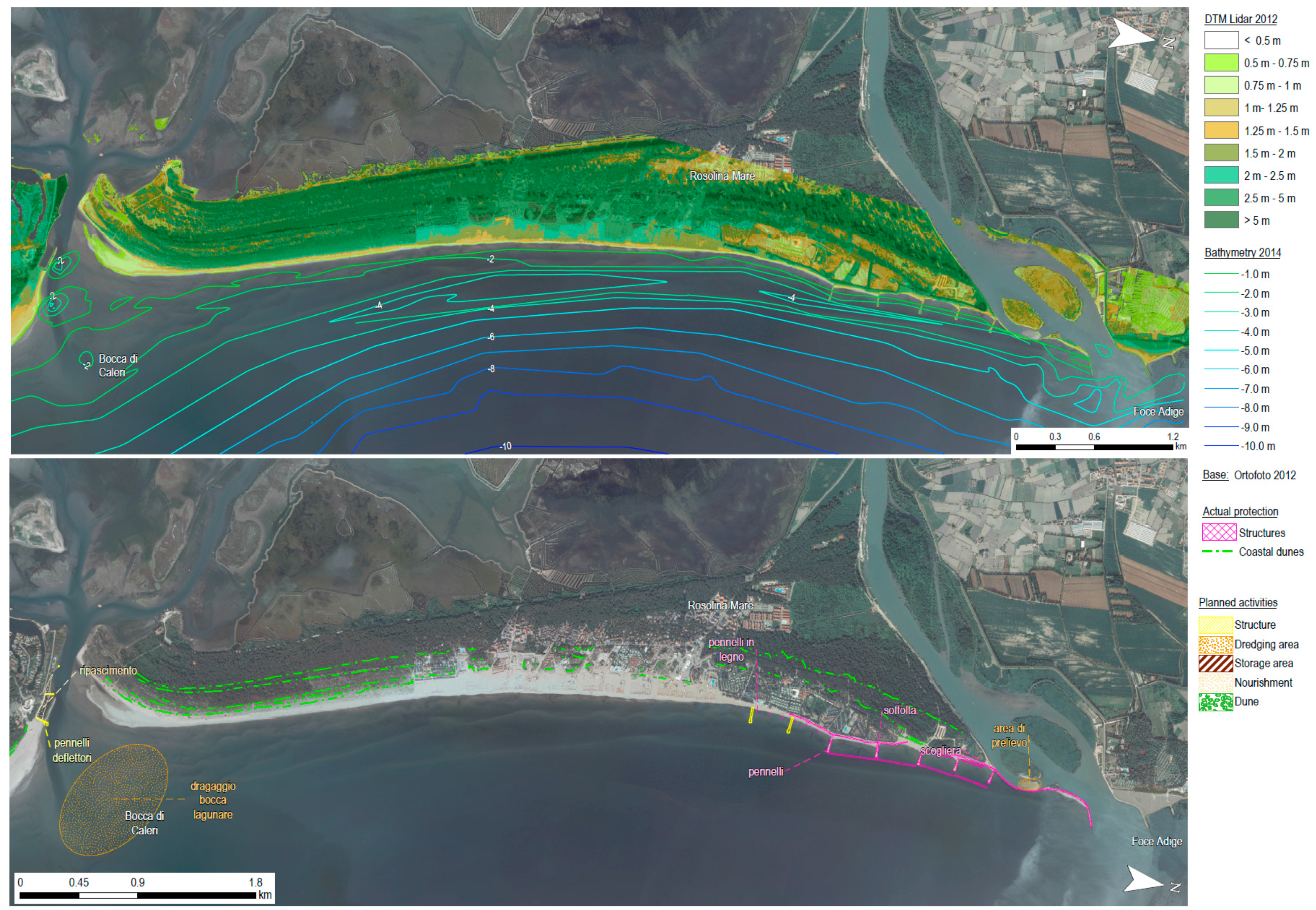
| Morphological Vulnerability (VM) | Socioeconomic Vulnerability (VSE) |
|---|---|
| Erosive Trend Coastal Flooding Risk | Value of coastal defence action (frequency and financial investments) Environmental relevance (presence of natural areas, Natura2000 sites, etc.) Tourist pressure (number of tourist/year, bathing facilities, tourist facilities, etc.) Urbanization (presence of cities, type of inland, etc.) Production activities (fishing, mussel/clam farming, etc.) Cultural heritage (monuments, archaeological sites, etc.) |
| Cell | North Bound | South Bound | d50 0 m/−2 m | d50 −2 m/−4 m | d50 −4 m/−6 m | d50 <−6 m | dEQ |
|---|---|---|---|---|---|---|---|
| VE1 | Tagliamento | Porto Baseleghe | 0.323 | - | - | - | 0.14 |
| VE2 | Porto Baseleghe | Falconera | 0.173 | - | - | - | 0.13 |
| VE3 | Falconera | Livenza | 0.175 | 0.235 | 0.118 | - | 0.16 |
| VE4 | Livenza | Piave | 0.205 | 0.173 | 0.143 | - | 0.16 |
| VE5 | Piave | Sile | 0.153 | 0.268 | 0.118 | - | 0.15 |
| VE6 | Sile | Bocca di Lido | - | 0.235 | 0.213 | - | 0.14 |
| VE7 | Bocca di Lido | Bocca di Malamocco | 0.181 | - | 0.186 | - | 0.12 |
| VE8 | Bocca di Malamocco | Bocca di Chioggia | - | - | - | - | 0.20 |
| VE9 | Bocca di Chioggia | Foce Brenta | - | 0.144 | 0.168 | - | 0.15 |
| VE10 | Brenta | Adige | - | - | - | - | 0.24 |
| RO1 | Adige | Bocca di Caleri | - | 0.190 | 0.191 | 0.129 | 0.18 |
| RO2 | Bocca di Caleri | Porto di Levante | - | 0.139 | 0.126 | 0.110 | 0.11 |
| RO3 | Porto di Levante | Po di Maistra | - | 0.143 | 0.185 | 0.142 | 0.11 |
| RO4 | Po di Maistra | Busa di Tramontana | - | 0.173 | 0.208 | 0.133 | 0.13 |
| RO5 | Busa di Tramontana | Busa Dritta | - | 0.234 | 0.230 | 0.101 | 0.17 |
| RO6 | Busa Dritta | Busa di Scirocco | - | 0.273 | 0.258 | 0.207 | 0.17 |
| RO7 | Busa di Scirocco | Busa Storiona | - | 0.167 | 0.148 | 0.132 | 0.17 |
| RO8 | Busa Storiona | Po di Tolle | - | 0.068 | 0.197 | 0.100 | 0.15 |
| RO9 | Po di Tolle | Po di Gnocca | - | 0.144 | 0.146 | - | 0.10 |
| RO10 | Po di Gnocca | Po di Goro | - | - | - | - | 0.13 |
| Cell | Part | QLS (1) Long-shore | QLS (2) Long-shore | QCR Cross-shore | QF Fluvial | QN Nourished | QD Dredged | ∂V |
|---|---|---|---|---|---|---|---|---|
| VE1 | N | −30.9 | −46.1 | 22.7 | 11.0 | 51.0 | 49.0 | −24.9 |
| VE1 | S | −46.1 | −48.9 | 7.1 | 65.9 | - | - | 55.9 |
| VE2 | N | −67.9 | −48.0 | 4.4 | - | - | - | 15.6 |
| VE2 | S | −48.0 | −36.4 | 3.0 | - | 22.8 | 17.2 | 14.1 |
| VE3 | N | −20.3 | −12.9 | 4.6 | - | 24.0 | 0.0 | 26.9 |
| VE3 | S | −12.9 | −10.0 | 3.8 | - | 22.4 | 5.1 | 16.3 |
| VE4 | N | −10.0 | −32.7 | 8.9 | - | 64.4 | - | 32.8 |
| VE4 | S | −32.7 | −57.3 | 79.8 | 13.0 | 29.9 | - | −61.5 |
| VE5 | N | −62.6 | −55.7 | 183.7 | 117.3 | 40.0 | 40.0 | −59.6 |
| VE5 | S | −55.7 | −91.2 | 19.7 | - | 35.6 | - | −19.6 |
| VE6 | N | −75.6 | −91.5 | 22.1 | - | 20.1 | - | −17.9 |
| VE6 | S | −91.5 | −24.3 | 17.2 | - | - | 20.2 | 29.8 |
| VE7 | N | −0.7 | 4.9 | 9.3 | - | 10.0 | - | 6.4 |
| VE7 | S | 4.9 | 2.1 | 9.9 | - | 10.0 | - | −2.7 |
| VE8 | N | 2.0 | 0.1 | 18.8 | - | - | - | −20.7 |
| VE8 | S | 0.1 | 2.3 | 18.0 | - | - | - | −15.8 |
| VE9 | N | −0.6 | 27.2 | 4.6 | - | - | - | 23.2 |
| VE9 | S | 27.2 | 16.2 | 48.0 | 19.8 | 39.9 | 20.0 | −19.3 |
| VE10 | N | 48.2 | 49.2 | 43.6 | 8.3 | 34.9 | 20.0 | −19.3 |
| VE10 | S | 49.2 | 58.0 | 93.2 | 62.5 | 35.0 | - | 13.1 |
| RO1 | N | −64.9 | −87.9 | 100.1 | 80.4 | 30.0 | - | −12.6 |
| RO1 | S | −87.9 | −65.0 | 6.0 | - | - | - | 16.8 |
| RO2 | N | 79.2 | 74.7 | 2.8 | - | - | - | −7.2 |
| RO2 | S | 74.7 | 62.9 | 3.6 | - | - | - | −15.4 |
| RO3 | N | 75.4 | 118.3 | 7.9 | - | - | - | 35.0 |
| RO3 | S | 118.3 | 86.3 | 31.6 | 60.1 | - | - | −3.5 |
| RO4 | N | 86.3 | 81.4 | 39.1 | 71.4 | - | - | 27.4 |
| RO4 | S | 81.4 | 207.6 | 20.8 | - | - | 59.3 | 46.1 |
| RO5 | N | 195.6 | 211.9 | 6.7 | - | - | - | 9.6 |
| RO5 | S | 211.9 | 359.8 | 368.8 | 240.4 | - | - | 19.4 |
| RO6 | N | −409.6 | −393.0 | 370.5 | 534.0 | - | - | 180.2 |
| RO6 | S | −393.0 | −305.3 | 8.7 | - | - | - | 78.9 |
| RO7 | N | −234.7 | −210.1 | 9.4 | - | - | - | 15.2 |
| RO7 | S | −210.1 | −252.5 | 9.0 | - | - | - | −51.3 |
| RO8 | N | −230.5 | −128.0 | 6.2 | - | - | - | 96.3 |
| RO8 | S | −128.0 | −103.5 | 96.9 | 99.1 | - | - | 26.8 |
| RO9 | N | −172.9 | −139.7 | 97.3 | 64.4 | - | - | 0.3 |
| RO9 | S | −139.7 | −190.5 | 78.6 | 106.2 | - | - | −23.2 |
| RO10 | N | −95.8 | −142.4 | 5.0 | - | - | - | −51.6 |
| RO10 | S | −142.4 | −155.5 | 119.5 | 120.8 | - | - | −11.8 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ruol, P.; Martinelli, L.; Favaretto, C. Vulnerability Analysis of the Venetian Littoral and Adopted Mitigation Strategy. Water 2018, 10, 984. https://doi.org/10.3390/w10080984
Ruol P, Martinelli L, Favaretto C. Vulnerability Analysis of the Venetian Littoral and Adopted Mitigation Strategy. Water. 2018; 10(8):984. https://doi.org/10.3390/w10080984
Chicago/Turabian StyleRuol, Piero, Luca Martinelli, and Chiara Favaretto. 2018. "Vulnerability Analysis of the Venetian Littoral and Adopted Mitigation Strategy" Water 10, no. 8: 984. https://doi.org/10.3390/w10080984
APA StyleRuol, P., Martinelli, L., & Favaretto, C. (2018). Vulnerability Analysis of the Venetian Littoral and Adopted Mitigation Strategy. Water, 10(8), 984. https://doi.org/10.3390/w10080984







