1. Introduction
Lakes and wetlands are important components of the terrestrial hydrosphere and provide essential ecological, environmental, and economic services, such as regulating the regional water resources and climate, providing habitats for the ecosystem, supplying water for agricultural, industrial, and domestic uses, and serving for the fishery industry, transportation, hydropower generation, and tourism [
1]. Water in lakes or wetlands is mainly recharged by precipitation and river and groundwater inflow, and is discharged by evaporation, river and groundwater outflow, and artificial water diversion from lakes or wetlands. With increasing water diversion from lakes/wetlands and their source rivers in recent decades, decreased recharge and increased discharge had resulted in a continuous drop of the water level in many lakes and wetlands, such as the Aral Sea in central Asia [
2], lakes in the middle Yangtze region of China [
3], the Ebinur Lake in Northwest China [
4], and the Honghe Wetland in Northeast China [
5]. The shrinkage of lakes/wetlands may result in a series of ecological and environmental problems, such as the degradation of the lake/wetland ecosystem, the deterioration of lake/wetland water quality, and the intensification of wind erosion [
6]. Therefore, the assessment of lake level, especially the minimum ecological lake level (MELL), has become a key factor in ecosystem protection and water resources allocation in lake and wetland basins [
7].
The MELL is usually defined as a threshold lake level below which the lake ecosystem can be significantly influenced. Once the MELL is reached, no human activities should be carried out to further decrease the lake water level. Commonly used assessment methods for MELL include historical lake level methods, lake morphology analysis methods, habitat analysis methods, and species-environment models [
7,
8]. These methods assess the MELL from different perspectives of the relationship between the water level and the lake ecosystem, each of which have its own advantages and disadvantages [
9]. The historical lake level methods are simple, however the MELL, which is defined as a statistic of the historical lake level records [
7,
8], may have no direct connection to the lake ecosystem. The lake morphology analysis methods define MELL as the inflection point of a specified lake morphology curve [
8,
10], however these methods face the problems of selecting an appropriate lake morphology curve and the possible non-existence of the inflection point in this selected curve. Both the habitat analysis methods and the species–environment models consider more of the interactions between the environment and the lake ecosystem [
11,
12], however they require detailed in situ surveys that are time-consuming and costly, which restricts their wide use in practice.
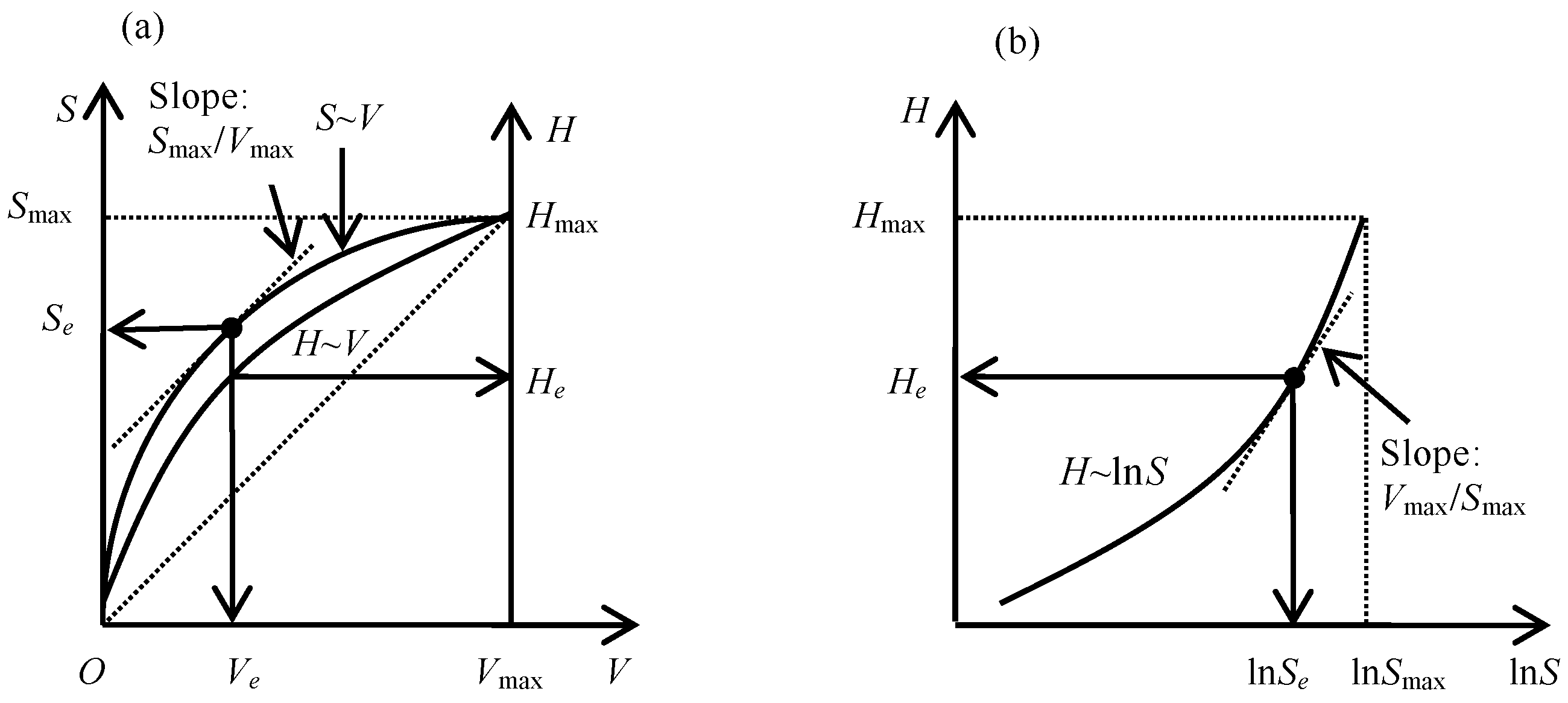
Through analyzing the characteristics and the existing problems of the widely used lake morphology analysis methods, Shang (2013) proposed the lake surface area method to define the MELL from the lake level-surface area-water storage curves [
9]. This method is an extension of the wetted perimeter method [
13] and the corresponding multi-objective assessment model [
14] for the minimum environmental flow of rivers. In the lake surface area method, the lake surface area and the water storage are taken as habitat and water indexes, respectively. The minimum ecological surface area is first determined from the critical point of the lake surface area-storage curve where the curve slope is equal to a specified value, which is similar to the wetted perimeter method. Then, the MELL corresponding to the minimum ecological surface area can be determined from the lake level-surface area curve. This method has been used to determine the MELL of several representative lakes in China [
9] and Greece [
15]. Compared with the traditional lake morphology analysis methods, the lake surface area is also applicable for cases when there is no inflection point of the lake morphology curve, and the MELL that is defined by the lake surface area method considers the tradeoff between the water that is used for the lake ecosystem and by humans. The lake surface area method can also avoid the error of the derivatives calculation from the scattered data that is required for the lake morphology analysis methods.
However, the lake surface area method requires both the lake surface area-storage curve and the lake level-surface area curve, and two stages in the calculation procedure can result in greater error in the numerical computation. To simplify the calculation procedure and to reduce the calculation error in the lake surface area method, the main objective of the present study was to propose a simplified lake surface area method that requires only the lake level-surface area curve to determine the MELL, which was applied in one freshwater lake, one saltwater lake, and one wetland in China.
2. Materials and Methods
2.1. Lake Surface Area Method and the Simplified Lake Surface Area Method
Lake sensitivity to desiccation is closely related with lake geometry or morphology [
16]. Water level (depth), area, and volume are important indexes of lake morphology. In the lake surface area method to determine the MELL [
9], the lake surface area was taken as a representative index for the ecosystem habitat, and the lake volume (water storage) was taken as a water index. Both the lake surface area (
S) and the lake water storage (
V) increased with the water level (
H), and the relationship between
S and
V can be expressed as
Generally, the lake surface area increases with the storage, while the increasing ratio (d
S/d
V) may increase or decrease with lake storage. Similar to the wetted perimeter method for the minimum environmental flow of rivers [
13], the lake surface area method defines the minimum ecological storage (
Ve) and area (
Se) as the lake storage and area corresponding to the point at which the slope of the
S-
V reaches a specified value, which is usually the ratio of maximum lake surface area to maximum lake storage (
Figure 1a) [
9], i.e.,
Then, the MELL,
He, can be determined from the
H-
S curve (
Figure 1a) [
9]. When the lake level is below the MELL, the lake surface area decreases rapidly with the decrease of the water level, which is unfavorable for lake ecosystem protection. While it is above the MELL, the lake surface area increases slowly with the increase of water level, which indicates that more water is required for a specified increase of the lake area. At the MELL, a relatively larger lake surface area can be reached at a relatively less water storage.
Therefore, both the
S-
V curve and the
H-
S curve are required to calculate the MELL using the lake surface area method. Moreover, the calculation procedure includes two stages, i.e., finding the breakpoint in the
S-
V curve with a specified slope and finding the corresponding lake level from the
H-
S curve. In practice, only scattered points of the
S-
V and
H-
S curves are available, which may result in significant errors in the derivative calculation and curve interpolation. To simplify the calculation procedure and to reduce the calculation error in the lake surface area method, a simplified lake surface area method was proposed considering the relationships among lake surface area, storage, and lake level. The relationship of these three lake morphology indexes can be described by
From Equations (2) and (3),
Therefore, the MELL can be determined directly from the
H-ln
S curve (
Figure 1b), where the curve has a slope of
Compared with the lake surface area method, the simplified lake surface area method requires only the H-lnS curve instead of both the S-V and H-S curves, which determines the MELL directly from the H-lnS curve. Therefore, the calculation of the simplified lake surface area method is simple, and the calculation error is generally less than that of the lake surface area method.
2.2. Calculation Method
Generally, the
H-ln
S curve of a lake is available as a table of scattered points from a field survey. If this curve can be approximated by a simple function, the MELL can be determined directly from Equation (5). However, the
H-ln
S curve of most lakes cannot be approximated by a simple function and the calculation accuracy of the curve slope from limited scatter points is usually low. To solve this problem, an equivalent multi-objective assessment model [
9,
17] can be used to determine the MELL, which is
where
h and
s(
h) are dimensionless water level and surface area, respectively,
H0 is the lowest lake level, and
Hmax is the highest water level in its natural state. The first objective in Equation (6) is a water use objective aiming at maximum water use for humans or minimum water use for the lake ecosystem, which achieves its minimum value of 0 as the lake drying out. The second objective is a habitat protection objective aiming at maximum lake surface area, which achieves its maximum value of 0 at the maximum lake level. Consequently, the ideal point for these two objectives are (0, 0), which cannot be achieved in practice due to the contradictoriness of these two objectives. As a whole, these two objectives aim at providing maximum possible habitat for the lake ecosystem with minimum possible lake water, which expresses the tradeoff between water that is reserved in the lake for ecosystem protection and water that is used by humans.
Using the weighted sum method or the ideal point method with the scaling coefficient of 1 [
18], the multi-objective optimization model (6) can be converted to the following single objective optimization model,
where
d(
H) is the evaluation function representing the Manhattan distance between the ideal point (0, 0) and a scenario in the objective space (
h, ln
s), and
w1 and
w2 are two non-negative weights with their sum of 1. The function
d(
H) reaches its minimum when
or
Considering Equation (3), Equations (2), (5), and (7) are equivalent for equal weights (w1 = w2 = 1/2) of the two objectives in Equation (6). It is more convenient to determine the MELL numerically from Equation (7). The determination of the MELL that is based on Equations (7) mainly includes the following steps:
(1) For all scattered points (Hi, Si), i = 1, 2, …, n, sorted in increasing order of Hi in the H-S curve, calculate the corresponding function value di, i = 1, 2, …, n, using Equation (7).
(2) Find the minimum value of
di,
i = 1, 2, …,
n, which is
(3) construct a parabola passing through points (
Hk−1,
dk−1), (
Hk,
dk), and (
Hk+1,
dk+1), the minimum point of which can be taken as an approximation of the minimum point of the evaluation function
d(
H) in Equation (7) based on the parabola interpolation method for univariate optimization. As a result, the MELL can be estimated numerically from
The above numerical method for the MELL can avoid the estimation of derivatives from scattered data and is consequently simpler and more accurate.
To compare the results of the different lakes and wetlands, the MELL was normalized with Equation (12).
where
he is the normalized MELL.
2.3. Case Study
The simplified lake surface area method was used to estimate the MELL for one freshwater lake (the Dongting Lake in Central China), one saltwater lake (the Ebinur Lake in Northwest China), and one wetland (the Honghe Wetland in Northeast China). Basic information about these two lakes and the one wetland is given in
Table 1. The lake geometry index (LGI), the absolute value of the fitted slope of the ln(
V)-
H curve [
16], was also calculated to classify these lakes and the wetland into different groups.
The Dongting Lake lies in the north of Hunan Province, Central China, and is an important lake in the middle and lower reaches of the Yangtze (Changjiang) River. The lake is mainly recharged from four source rivers (Xiangjiang, Zishui, Yuanjiang, and Lishui rivers) and four distributaries from the Yangtze River with the total drainage area of 2.57 × 10
5 km
2, and is discharged to the Yangtze River at Chenglingji. The Dongting Lake and the wetlands around the lake provide suitable habitats for aquatic and wetland ecosystems. However, the lake has been shrinking in the past several decades due to natural processes and human activities [
3,
19], which has resulted in the degradation of ecosystems and a decrease of the fishery resources. To protect the degrading lake ecosystem, it is urgent to study the MELL and the ecological water requirement for the Dongting Lake.
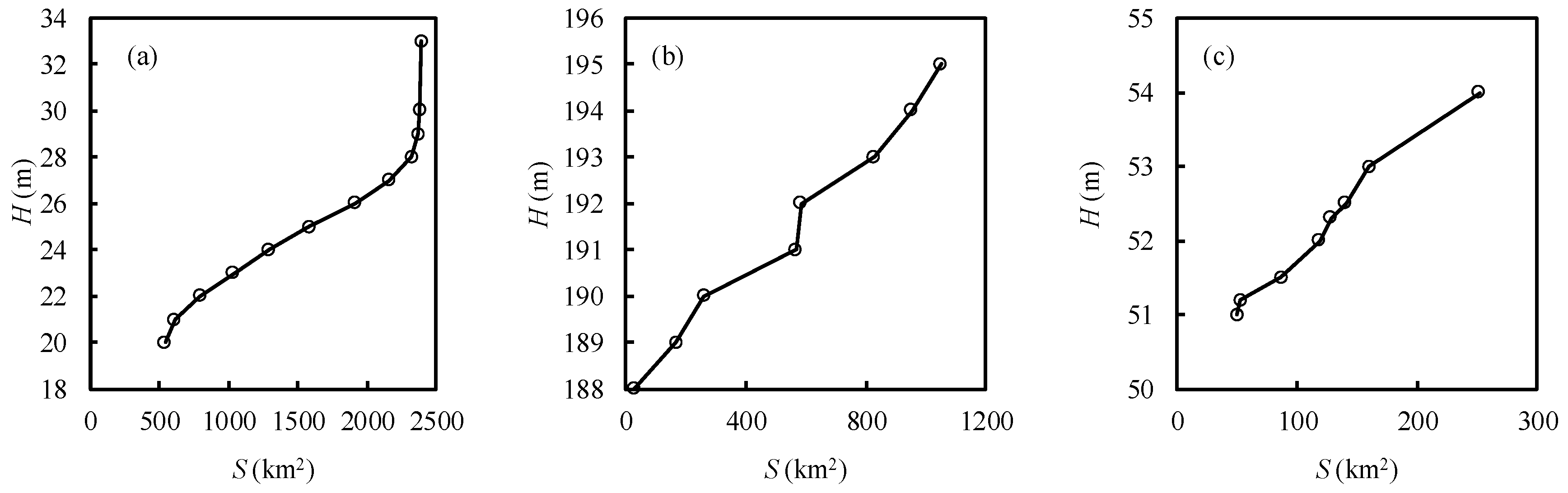
Figure 2a shows the Chenglingji water level (
H)-lake surface area (
S) curve that is based on data from [
19].
The Ebinur Lake lies in the Northwest of the Xinjiang Uygur Autonomous Region, Northwest China, and is the largest saltwater lake in Xinjiang and the largest lake in the Junggar Basin. The lake is an endorheic lake that is mainly recharged by the Bortala, Jing, and Kuitun rivers with the total drainage area of 5.06 × 10
4 km
2. The lake lies in an extremely arid region, with an annual precipitation of approximately 100 mm and a water surface evaporation of over 1500 mm. The lake ecosystem and the surrounding wetland ecosystem, which are fed by the lake water, are important for the local environment. However, lake shrinkage in the last several decades has led to severe ecological and environmental disasters, such as ecosystem degradation, desertification, and sandstorms [
20]. The
H-
S curve of the Ebinur Lake [
4] is shown in
Figure 2b.
The Honghe Wetland lies in the northeast of Heilongjiang Province, Northeast China, and has a total area of 281 km
2. It was listed as a national nature reserve in 1996 and in the Ramsar wetlands of international importance in 2002. The wetland provides suitable habitat for aquatic and terrestrial ecosystems, which includes some rare, threatened, and endangered species, such as Oriental Stork (
Ciconia boyciana), red-crowned crane (
Grus japonensis), white-naped crane (
Grus vipio), and whooper swan (
Cygnus cygnus). The water level in the wetland has dropped by over 1.0 m in recent decades, which has threatened the habitat for the wetland ecosystem. The
H-
S curve of the Honghe Wetland [
5] is shown in
Figure 2c.
3. Results and Discussion
From the calculated LGI in
Table 1, the Ebinur Lake and the Honghe Wetland fall into lake group 1 with the LGI from 0.5 to 2.5, while the Dongting Lake falls into group 2 with the LGI from 2.5 to 4.5 [
16]. These results are in agreement with [
16] that most natural lakes fall into groups 1 and 2.
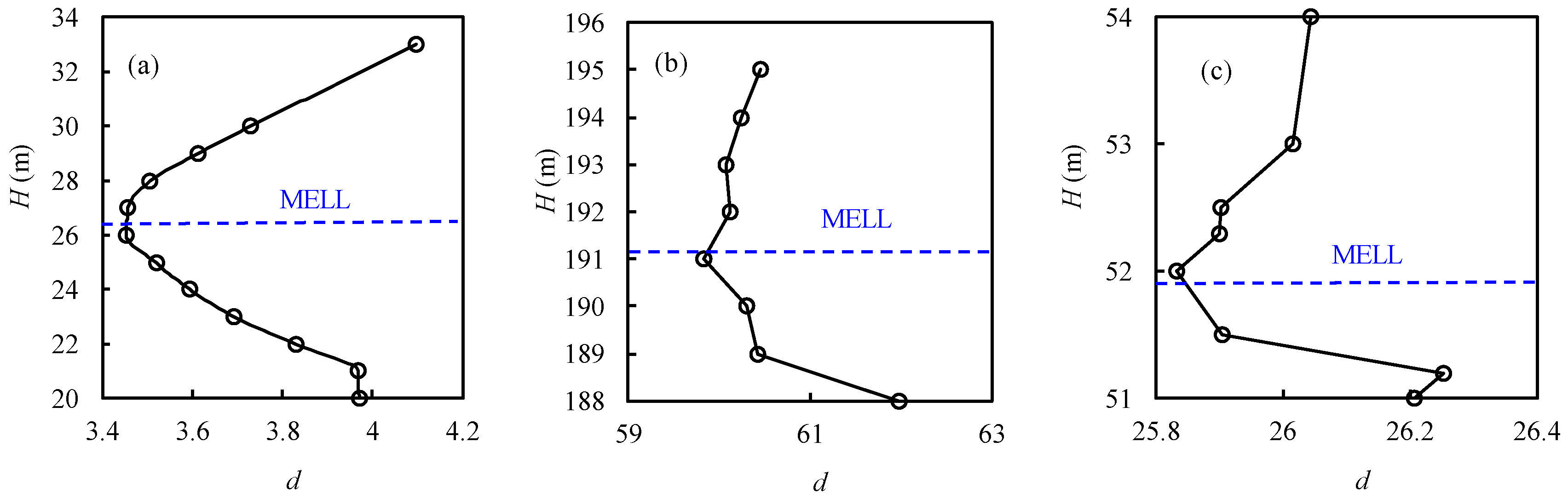
Based on the
H-
S curves for the two lakes and the one wetland in
Figure 2, the values of the evaluation function (
d) in each scatter point can be calculated using Equation (7) (
Figure 3), which was then used to estimate the MELL for each lake or wetland using the simplified lake surface area method. The results were further compared with the estimated MELL using other methods.
3.1. The Dongting Lake
For the Dongting Lake, the MELL can be approximated to be 26.4 m using the simplified lake surface area method, and the normalized MELL is 0.49. This result is close to the MELL of 26.7 m that was calculated with the lake surface area method [
9]. The present result of 26.4 m is 1.8 m higher than the MELL of 24.6 m that was defined as the lake level corresponding to the maximum value of Δ
S/Δ
V (59.0 km
2/10
8 m
3) [
21], and is 1.4 m higher than the MELL of 25.0 m that was defined as the critical lake level in the
H-
S curve [
22]. In fact, Δ
S/Δ
V is relatively large for lake level fluctuations under or above the latter MELL, which indicates that larger lake surface increments can be obtained at smaller water storage increments. For example, the values of Δ
S/Δ
V for the lake level increasing from 25.0 m to 26.0 m, 26.0 m to 27.0 m are both greater than 25% of the maximum. At the lake level of 24.6 m, 17% of the maximum lake storage provides 61% of the maximum lake surface area. While at the lake level of 26.4 m, 31% of the maximum storage provides 86% of the maximum lake surface area. When the lake level increases from 24.6 m to 26.4 m, the increase of the relative surface area (25%) is significantly greater than that of the relative lake storage (14%). When the lake level increases from 27.0 m to 28.0 m, an increase in the relative storage (9.6%) results in a smaller increase in the surface area (7.1%). From the view of marginal benefit, it is more appropriate to define the MELL as higher than the 24.6 m corresponding to the maximum value of Δ
S/Δ
V and lower than 27.0 m. The present value of 26.4 m lies in the interval of [24.6 m, 27.0 m], and is more appropriate to be taken as the MELL for the Dongting Lake.
3.2. The Ebinur Lake
For the Ebinur Lake, the MELL was estimated to be 191.1 m using the simplified lake surface area method, which is close to the MELL of 191.2 m that was calculated with the lake surface area method [
9]. The corresponding normalized MELL is 0.44. This result is slightly higher than the MELL of 190.9 m that was determined from the minimum habitat requirement for brine shrimp (
Artemia salina), which is an important biological resource in the lake, and the MELL of 191.0 m that was determined from the lake morphology analysis method [
4]. The smaller differences among the MELL that were determined by the present method, the minimum habitat requirement method, and the lake morphology analysis method indicate that the present result is appropriate.
3.3. The Honghe Wetland
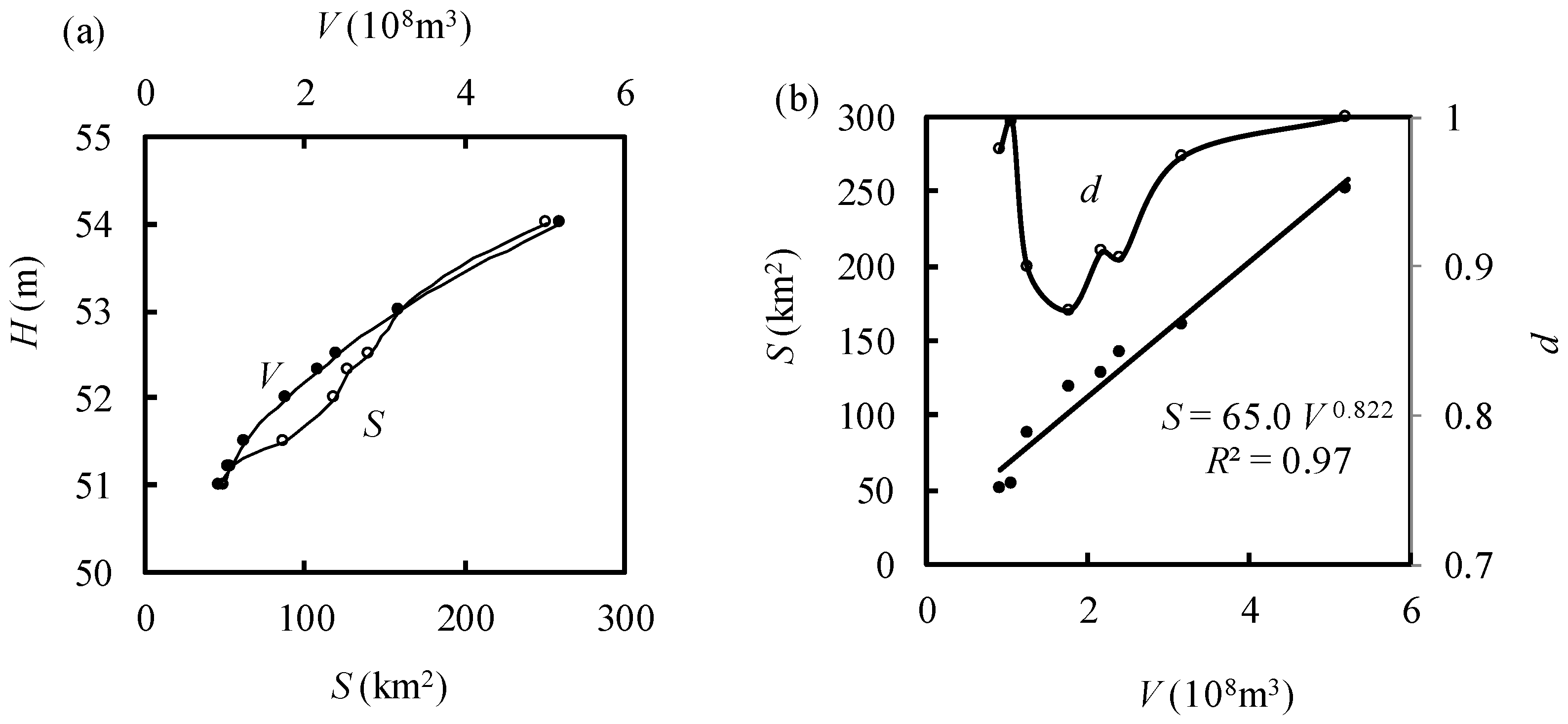
For the Honghe Wetland, the MELL was estimated to be 51.9 m using the simplified lake (water) surface area method, and the corresponding normalized MELL was 0.30. Using the lake surface area method [
9], the minimum ecological lake storage can be estimated analytically from the fitted
S-V curve of
S = 65.0
V0.822 (
Figure 4), which is 1.78 × 10
8 m
3, and the corresponding MELL is 52.0 m. Similar results can also be obtained from the numerical method for the lake surface area method (
Figure 4). Therefore, the MELL that was determined by the simplified lake surface area method is only slightly lower than that estimated by the lake surface area method.
The MELL was also estimated to be 51.5 m, corresponding the maximum value of ΔS/ΔV using the lake morphology analysis method, which is 0.4 m lower than the present result. However, the values of ΔS/ΔV are relatively greater for the water level from 51.5 m to 52.0 m, indicating that more increments of wetland water surface (13% of the maximum wetland surface area) can be achieved with less increments of water storage (10% of the maximum storage). However, when the water level increases from 52.0 m to 52.3 m, the relative increment of water surface area (3.8%) is significantly smaller than that of the water storage (7.7%). From the view of marginal benefit, it is appropriate to define the MELL in the range of 51.5 m and 52.0 m, where the present MELL of 51.9 m falls.
3.4. More Discussion on the Method and Results
The simplified lake surface area method was an extension of the lake surface area method, and they define the MELL from a critical point in the H-lnS and S-V curves, respectively. For the two lakes and the one wetland, the MELL that was determined by the simplified lake surface area method was slightly lower than that estimated by the lake surface area method. The simplified lake surface area method requires only the H-lnS curve or the H-S curve and its calculation is simpler than the lake surface area method, which generally results in a smaller calculation error. Therefore, the simplified lake surface area method is more applicable for lake water management than the lake surface area method.
Meanwhile, the values of MELL for these two lakes and the Honghe Wetland that were determined by the simplified lake surface area method are all higher than those that were estimated by lake morphology analysis methods. Considering that ΔS/ΔV is relatively large for lake level fluctuations under or above the maximum ΔS/ΔV, we argue that it is more appropriate to define the MELL as a lake level greater than the lake level corresponding to the maximum value of ΔS/ΔV. From the view of marginal benefit, the MELL can be defined from the water level with the same relative marginal output (relative increase of surface area) and relative marginal input (relative increase of water volume). The present results of MELL are all in the reasonable ranges for the MELL.
For the Ebinur Lake, the MELL values that were determined by the present method, the minimum habitat requirement method, and the lake morphology analysis method were all very close, indicating that the present result is also appropriate.
The values of the normalized MELL show that these two lakes have greater normalized MELL (0.49 and 0.44) than the wetland (0.30), and that the Dongting Lake, with a higher LGI, also has a greater normalized MELL (0.49) than the Ebinur Lake (0.44). These results imply that the normalized MELL may be related with LGI and lake/wetland types. However, more case studies are needed to find the possible links between LGI and normalized MELL in further studies.
4. Conclusions
Based on the lake surface area method for the minimum ecological lake level [
9], the simplified lake surface area method was proposed. The present method requires only the water level-logarithm of the water surface area curve and defines the minimum ecological lake/wetland level from the water level where the slope of the curve is equal to the ratio of maximum water storage to maximum water surface area. When the water level-water surface area curve can be expressed by a simple analytical function, the minimum ecological lake/wetland level can be calculated analytically. Otherwise, it can be calculated numerically from a corresponding multi-objective optimization model which represents the tradeoff between the lake’s ecological water use and the human water use. Compared with the lake surface area method, the simplified lake surface area method requires less data and simpler computation, which is more applicable for lake water management.
The simplified lake surface area method was used to determine the minimum ecological lake/wetland level for two representative lakes and one wetland in China. The calculated minimum ecological lake/wetland level for the Dongting Lake (freshwater lake), Ebinur Lake (saltwater lake), and the Honghe Wetland in China were 26.4 m, 191.1 m, and 51.9 m, respectively. These results indicate that the present MELL for these lakes and the wetland are all slightly lower than those that were determined by the lake surface area method and were higher than those that were determined by the lake morphology analysis method. Comparisons with results of other methods indicate that the present results are within reasonable ranges. These results can be used in lake ecosystem protection and water resources utilization planning.