Numerical Modeling of Long-Term Biogeochemical Processes and Its Application to Sedimentary Bed Formation in Tokyo Bay
Abstract
1. Introduction
2. Model Description
2.1. Integrated Model Framework
2.2. Hydrodynamic Model
2.3. Wave Hindcasting Model
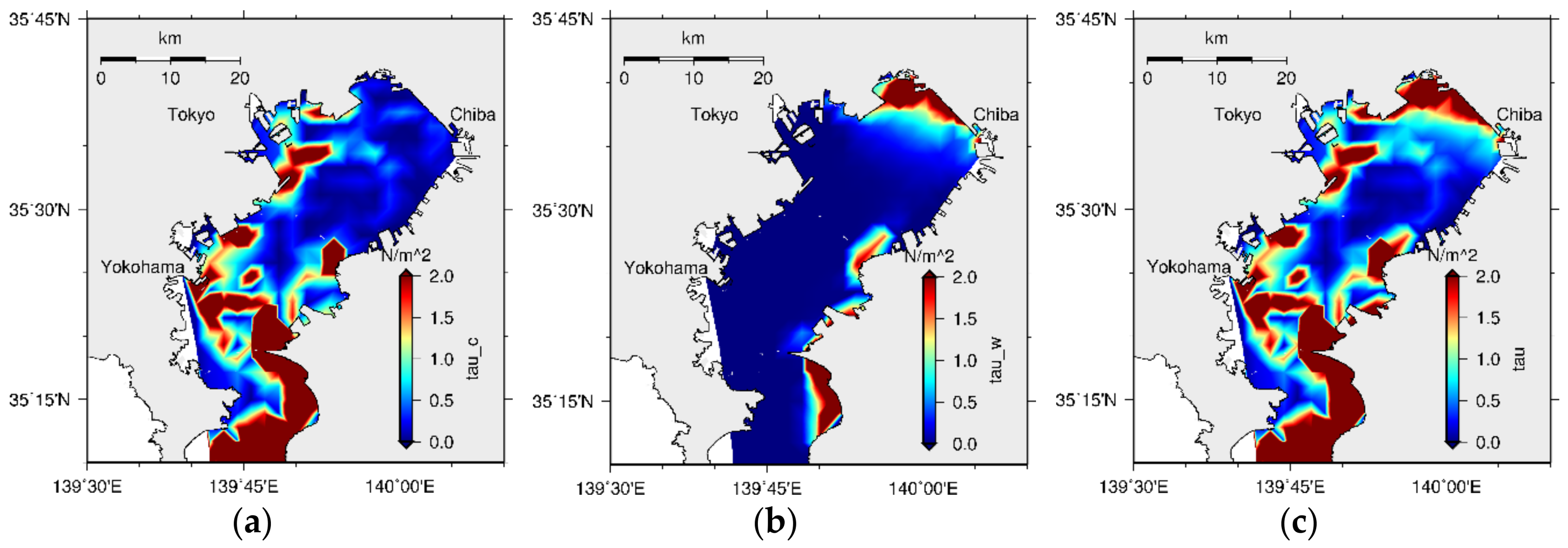
2.4. Bed Shear Stress (BSS) Model
2.5. Pelagic Model
2.6. Pelagic Model Boundary Conditions and Numerical Procedure
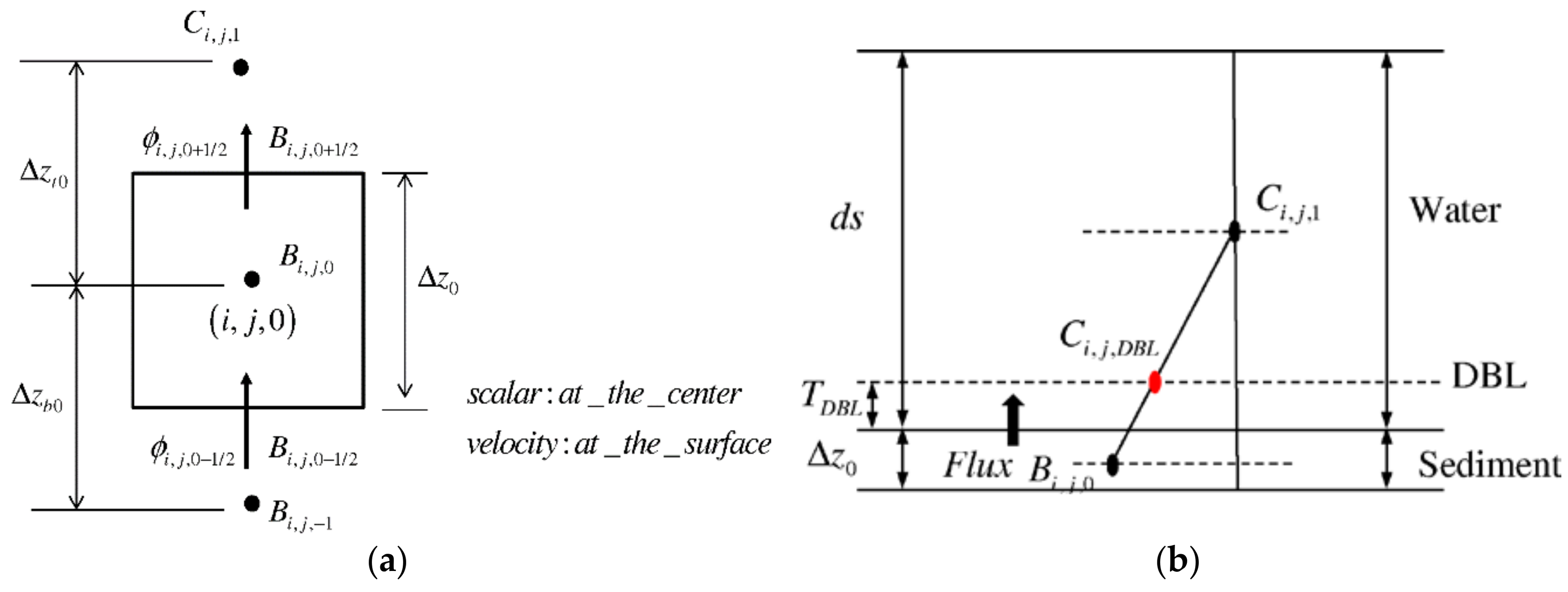
2.7. Benthic Model
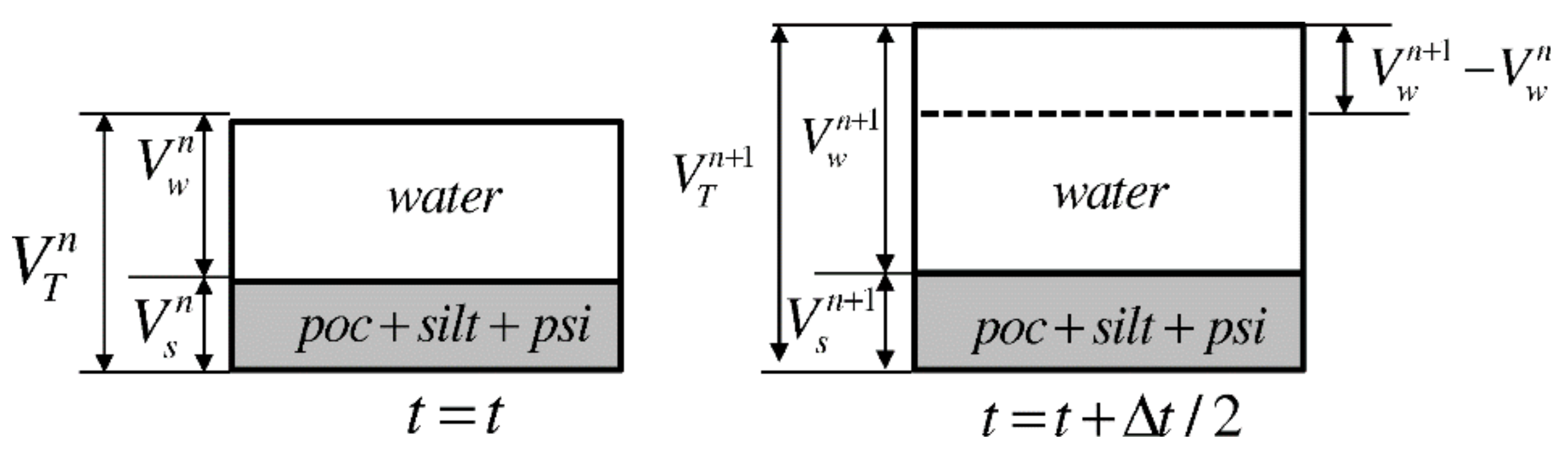
2.8. Benthic Model Boundary Conditions and Numerical Procedure
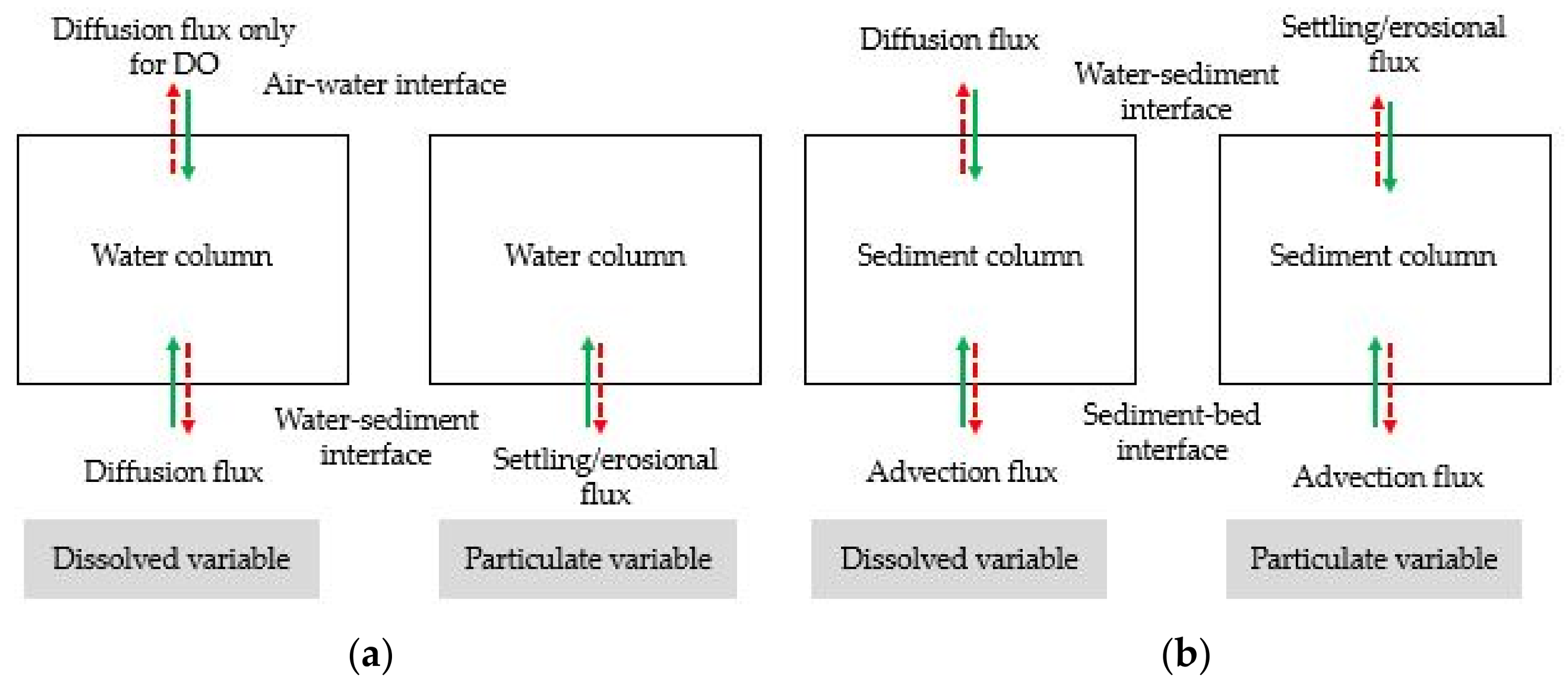
2.9. Benthic and Pelagic Model Coupling
2.9.1. Diffusion Flux at Sediment–Water Interface
2.9.2. Particulate Matter Settling or Resuspension
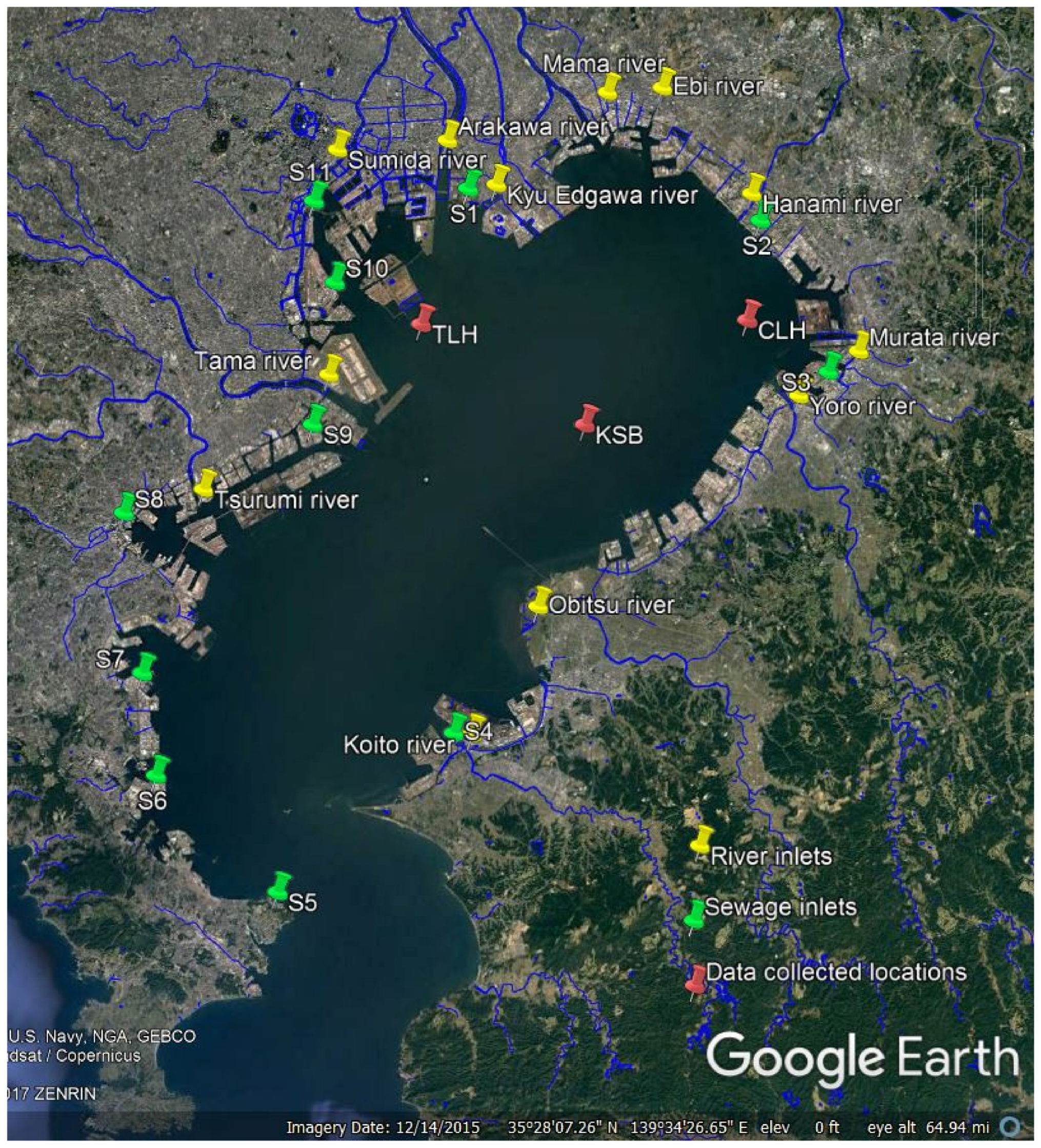
3. Model Application to Tokyo Bay
3.1. Model Grid System
3.2. Initial Conditions
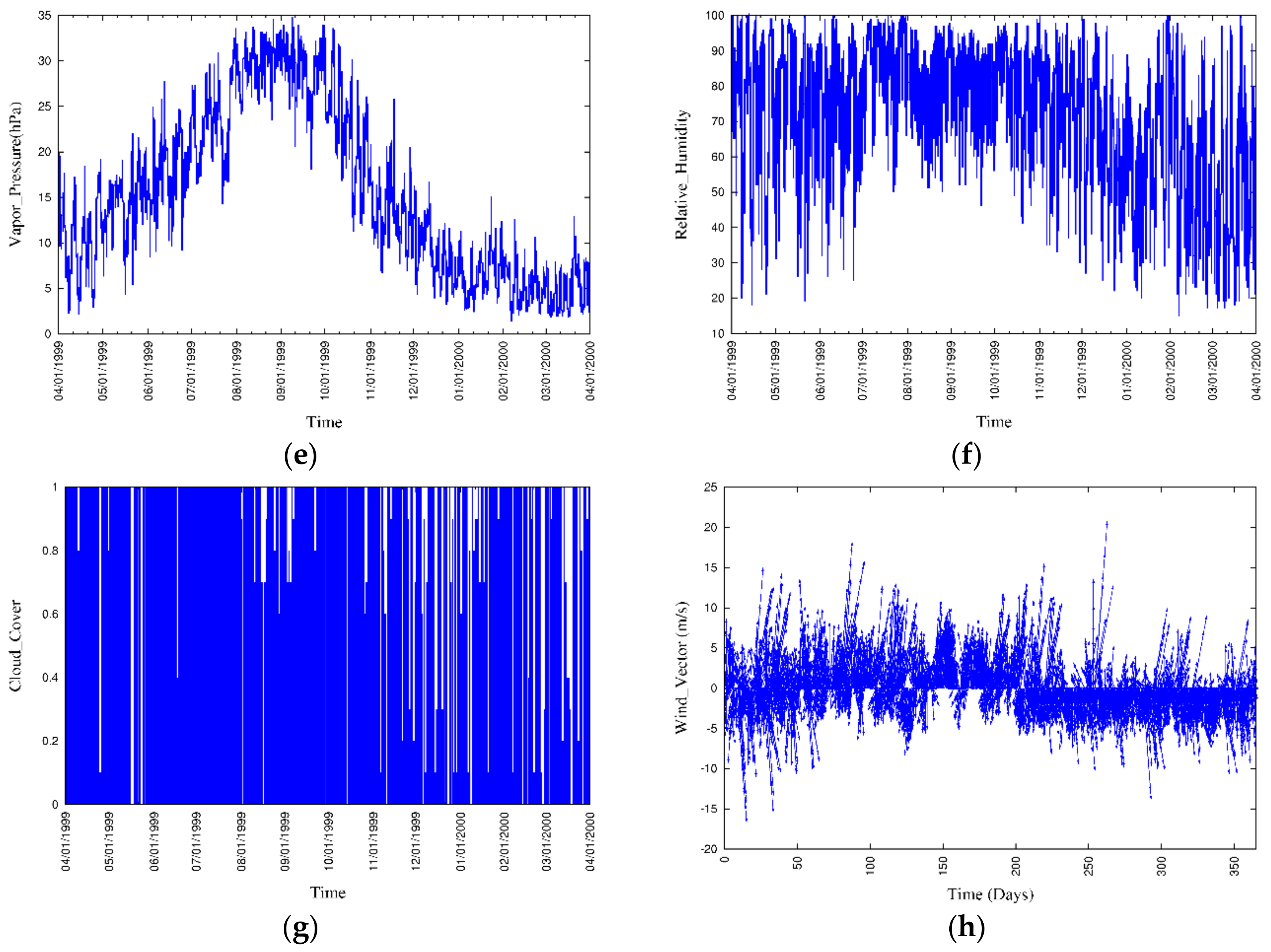
3.3. Boundary Conditions
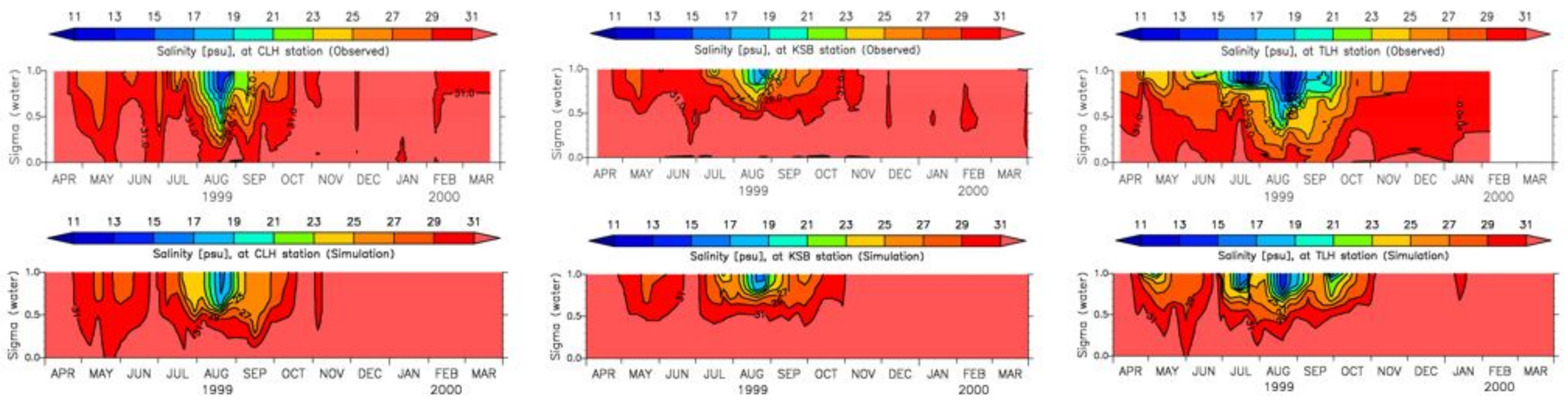
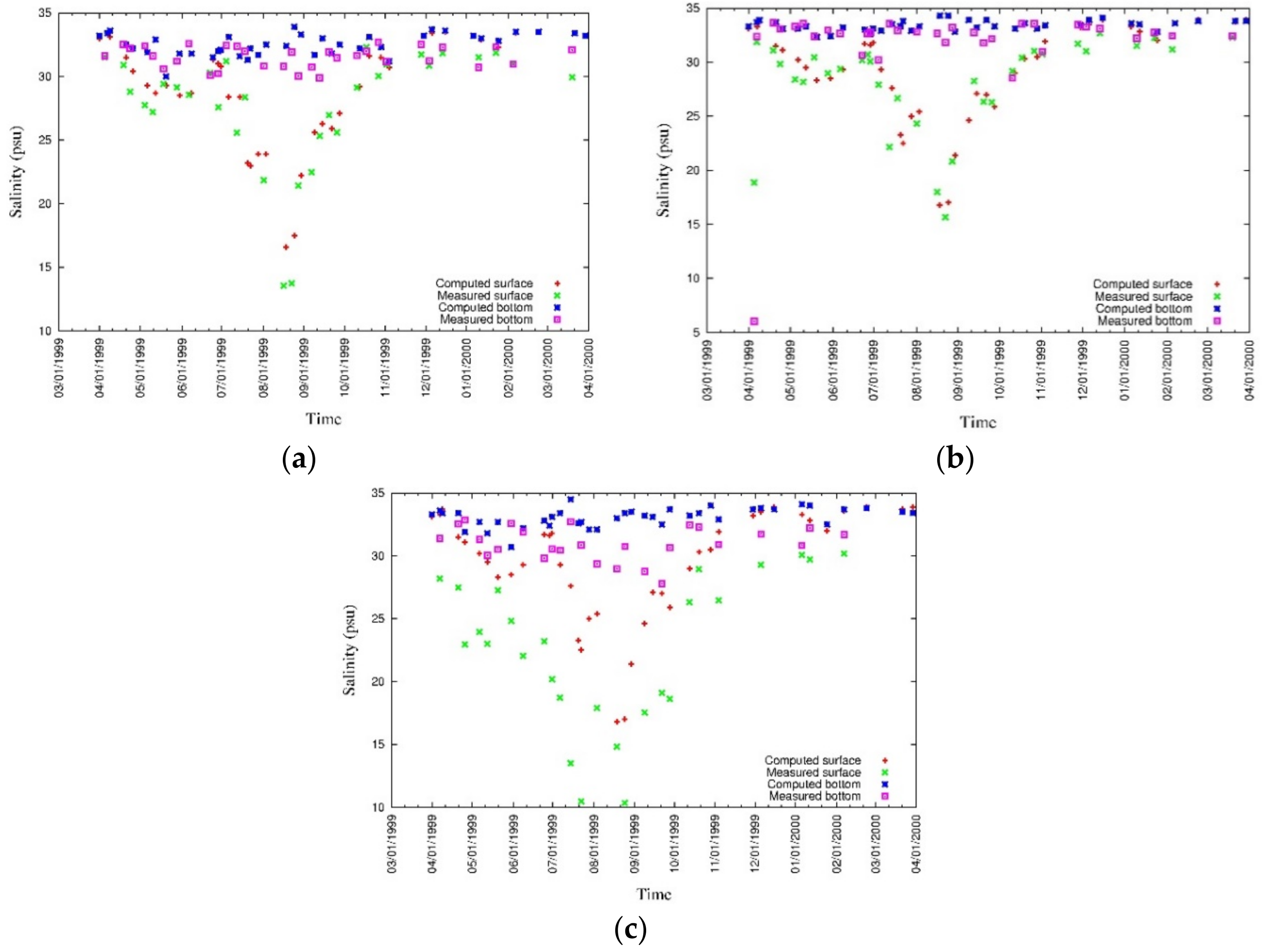
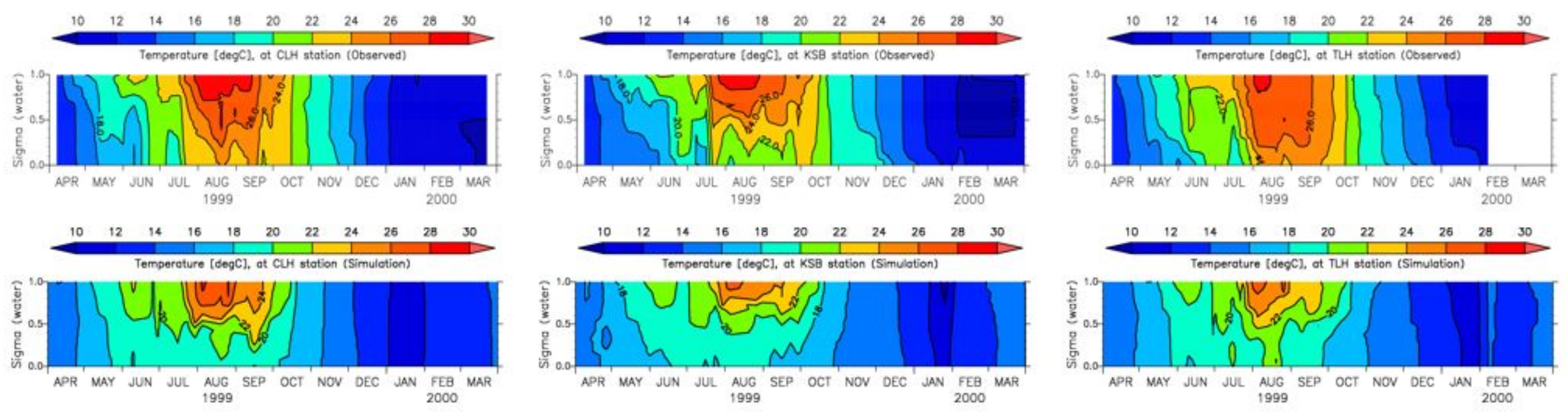
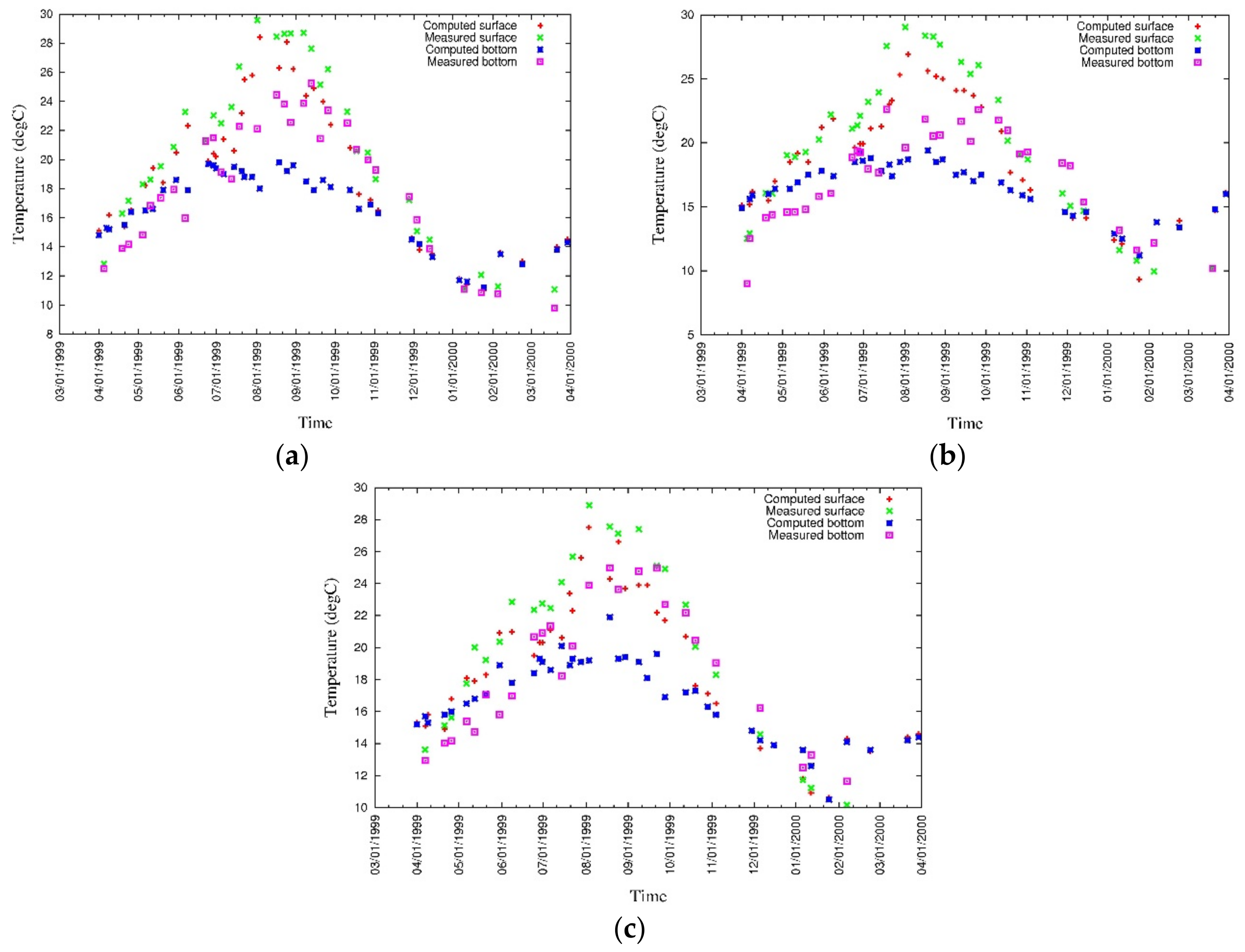
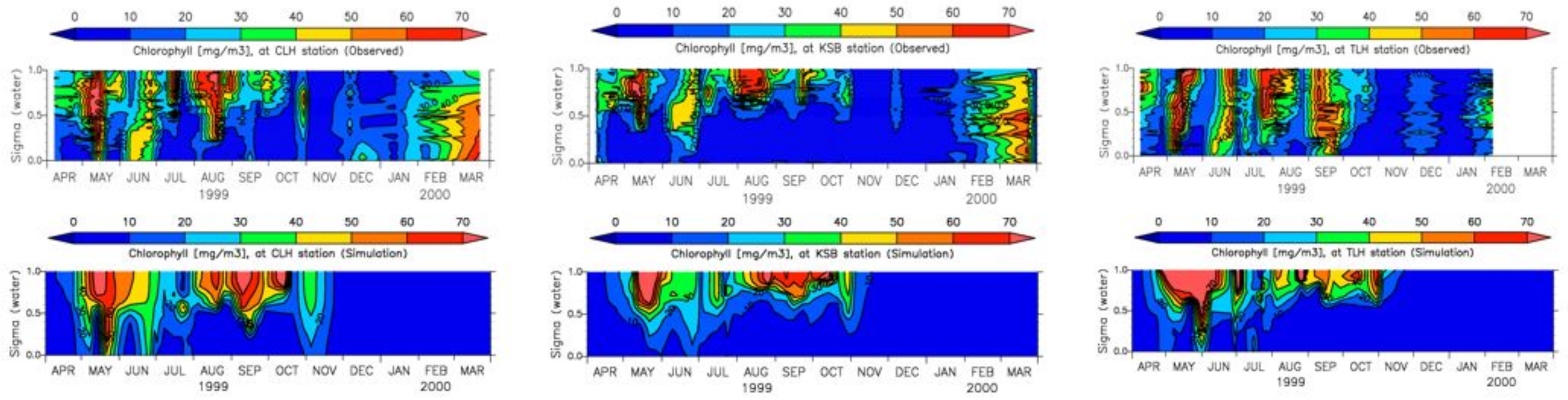
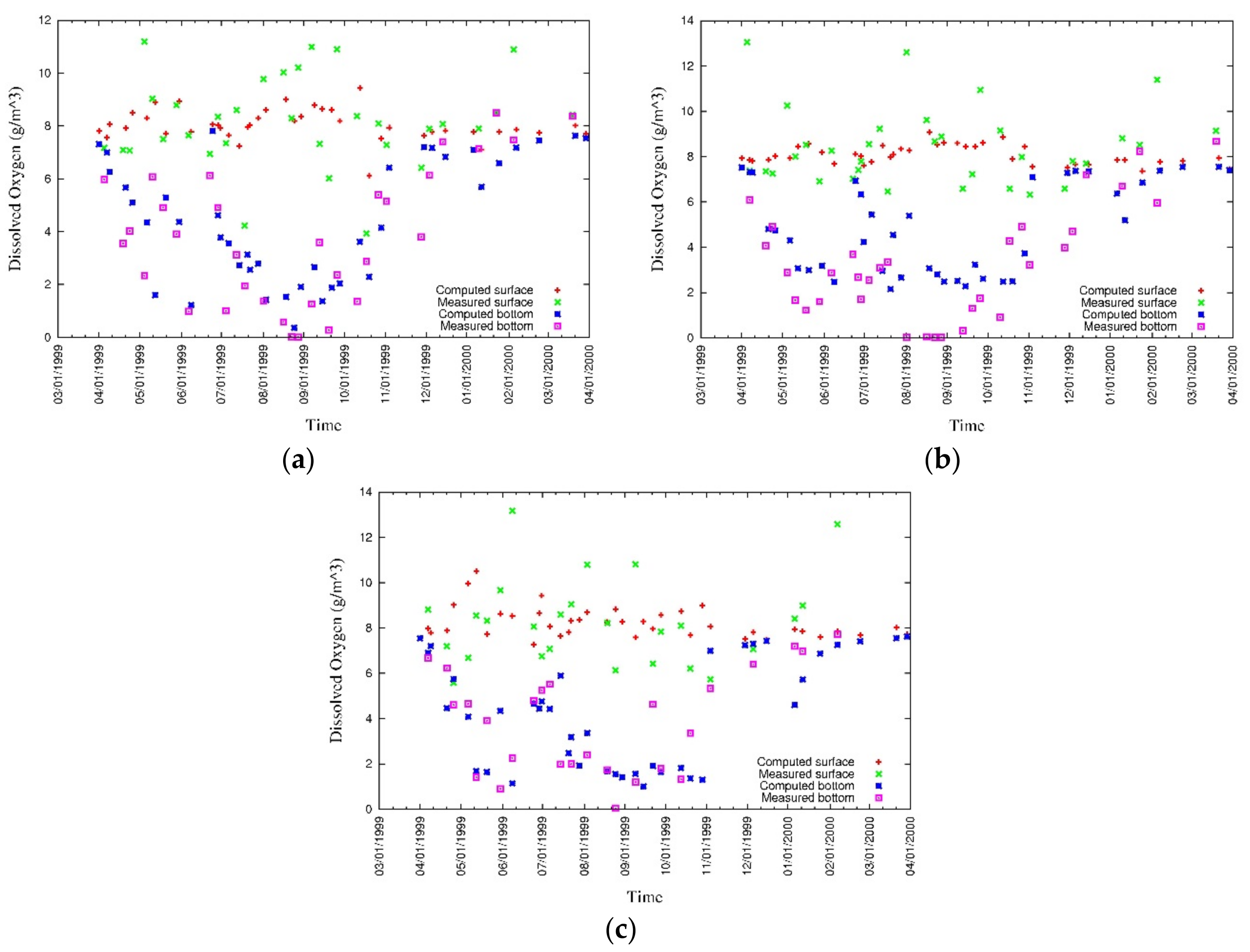
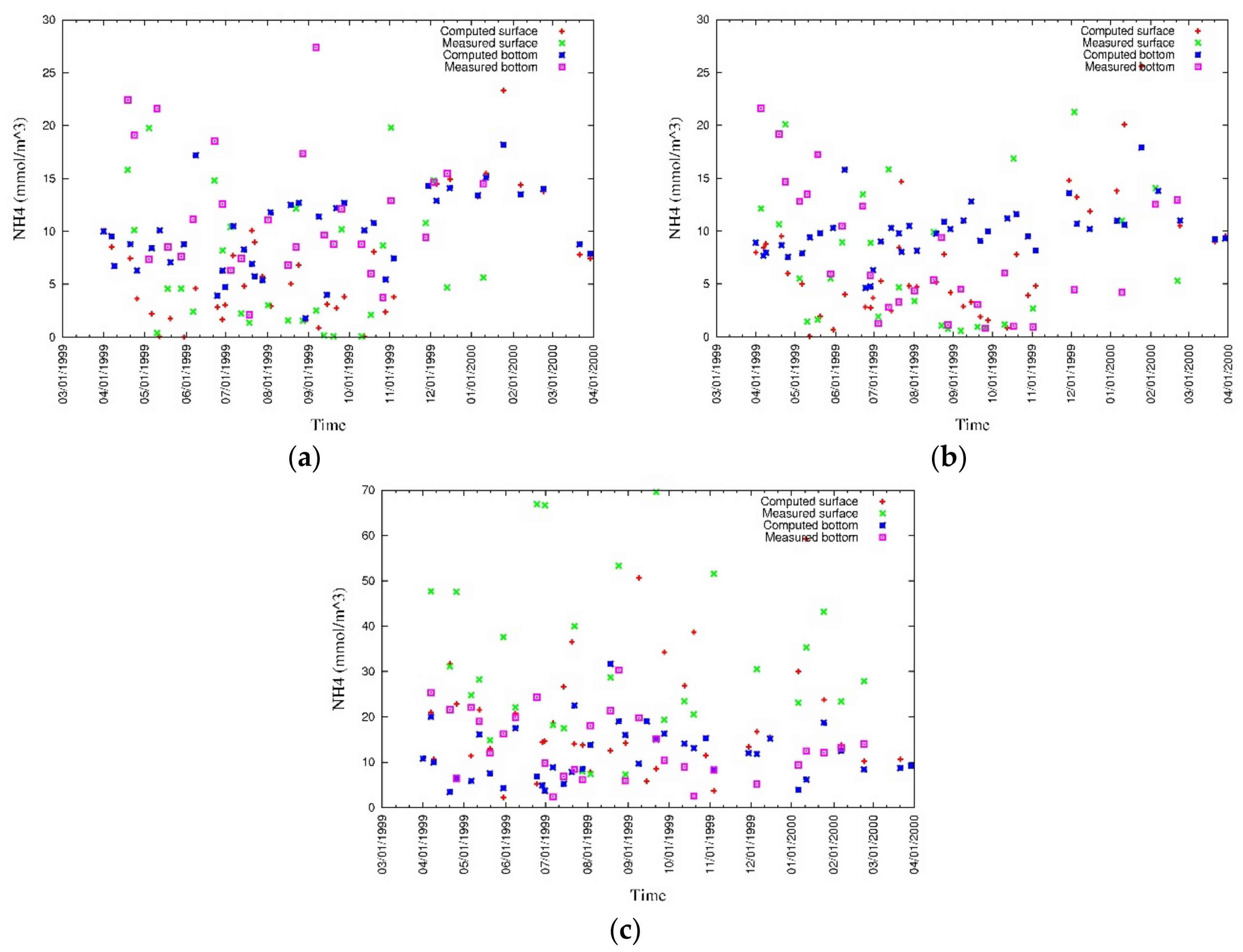
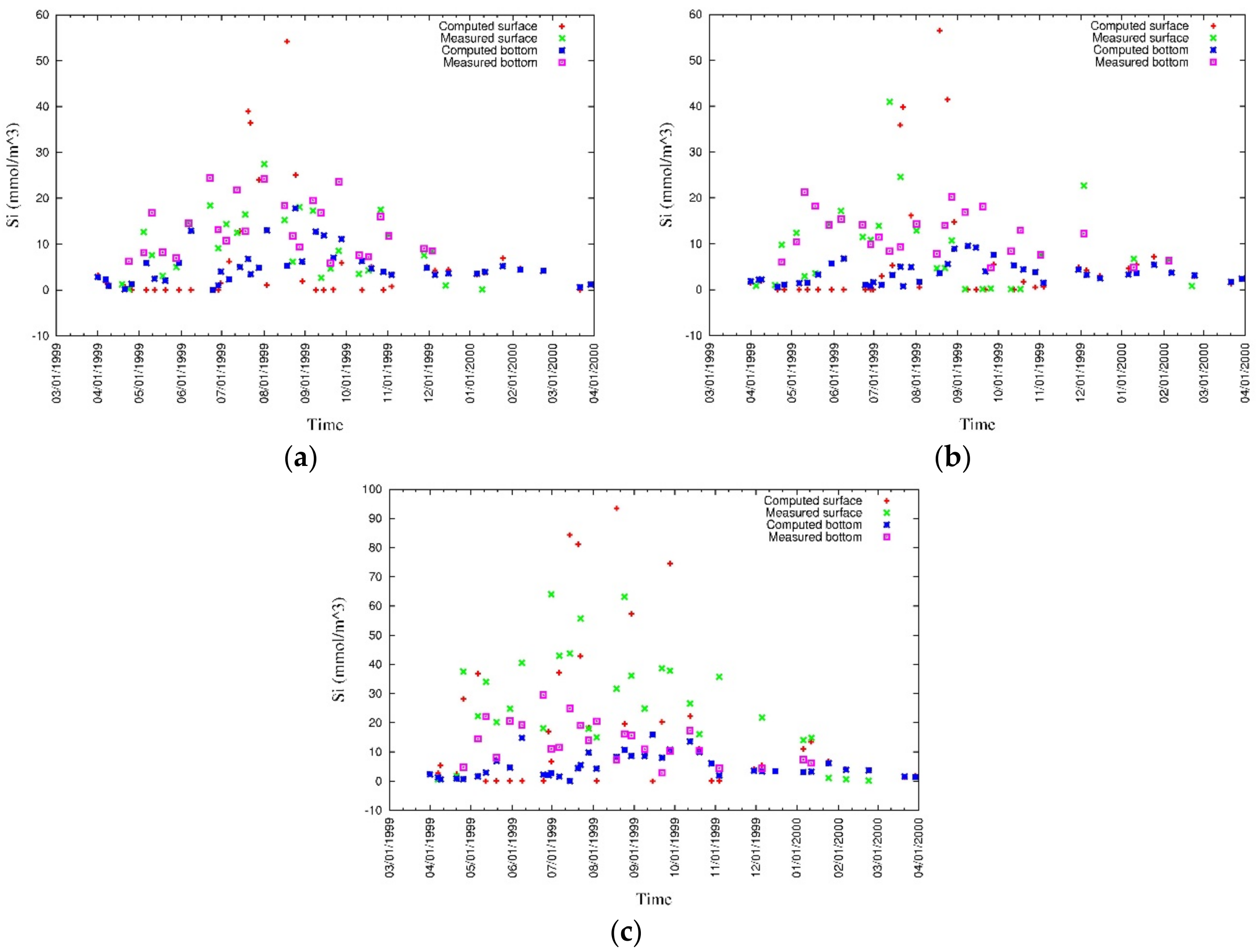
3.4. Model Calibration and Validation
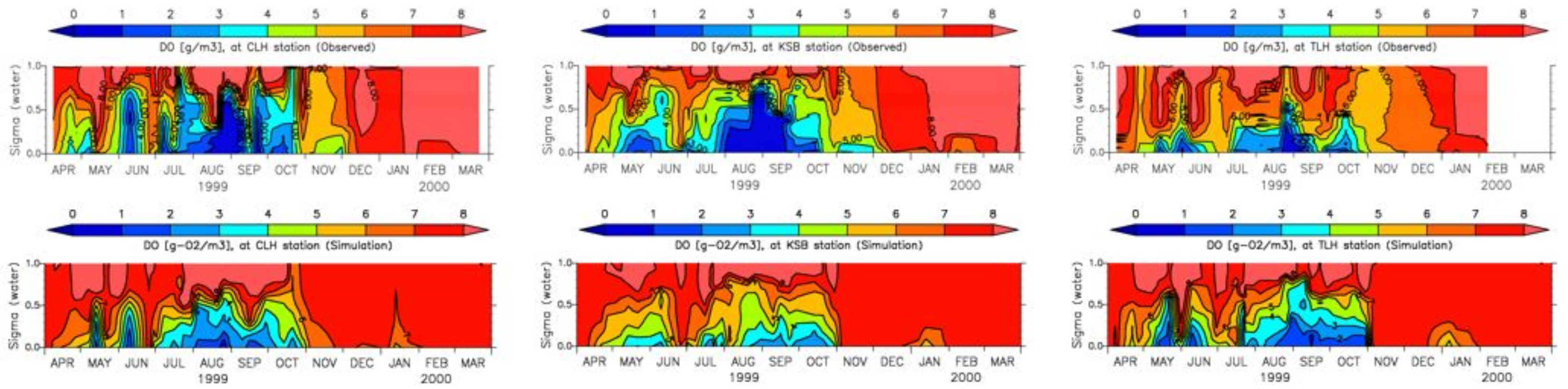
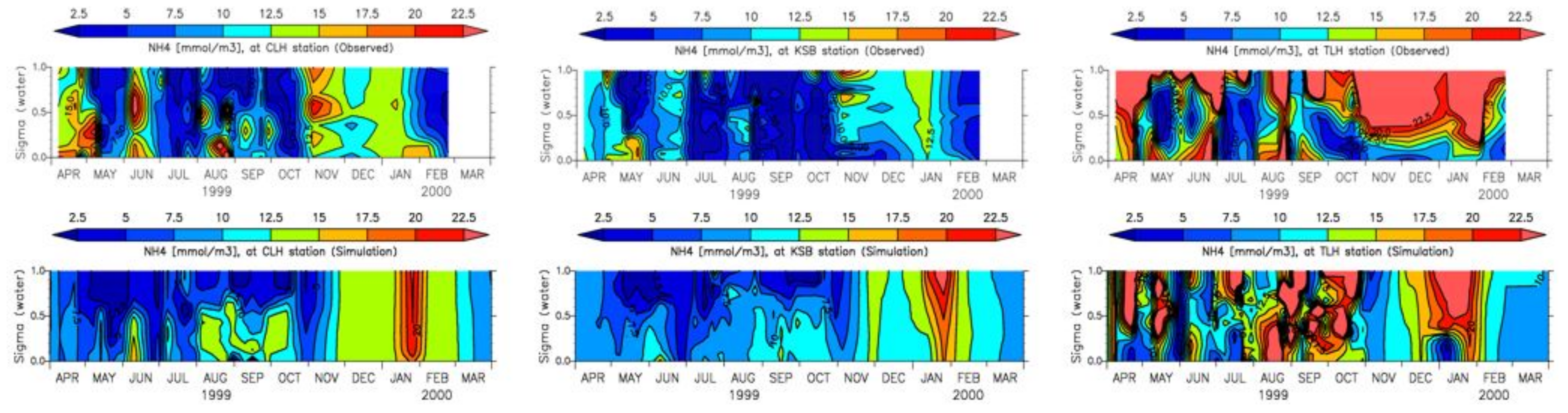
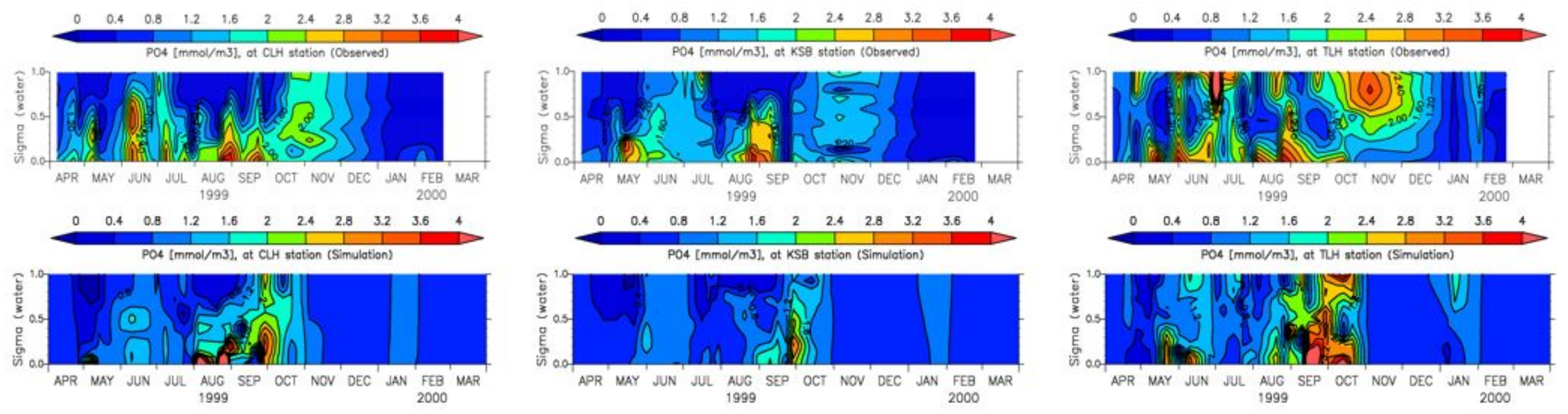
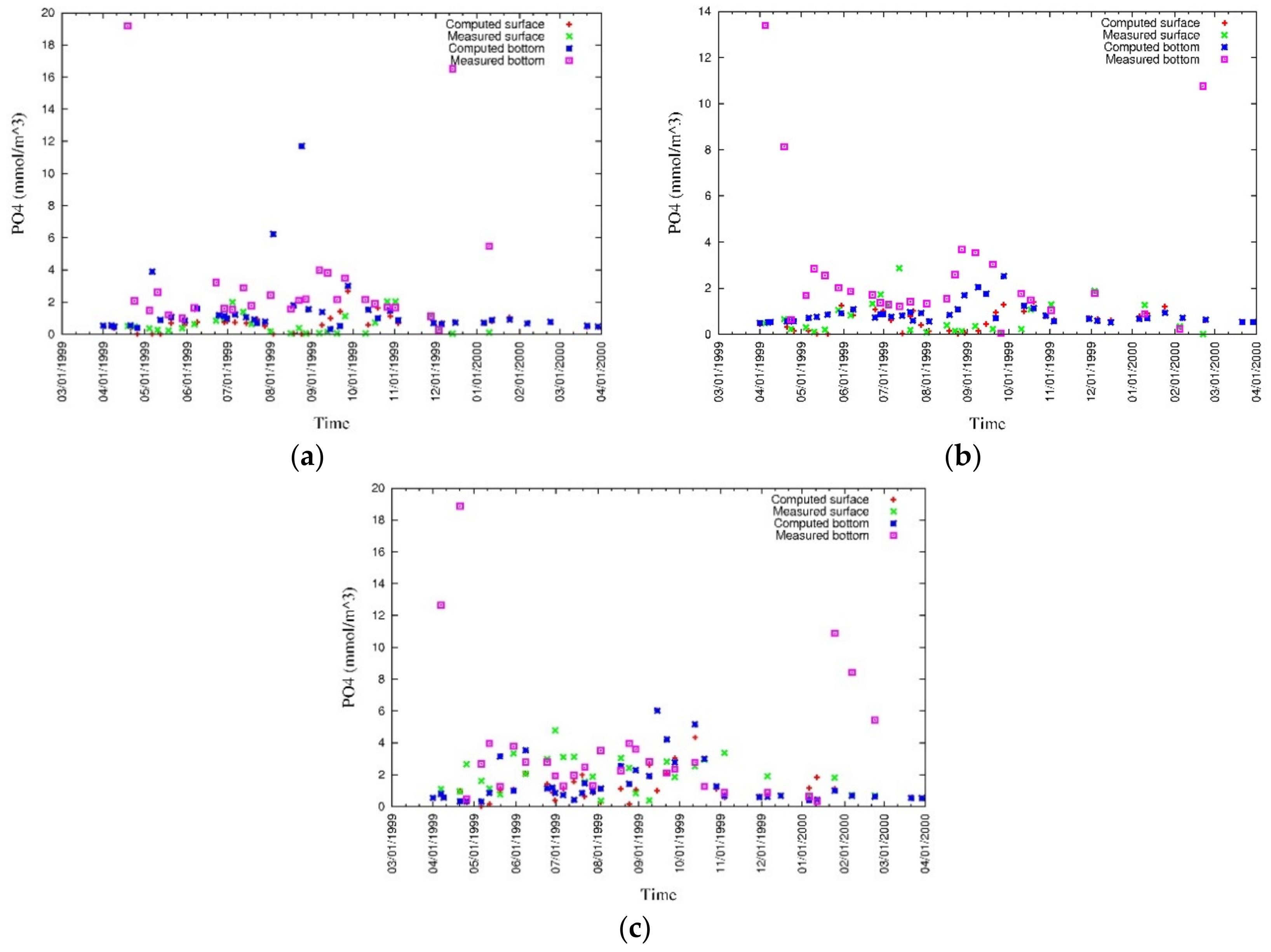
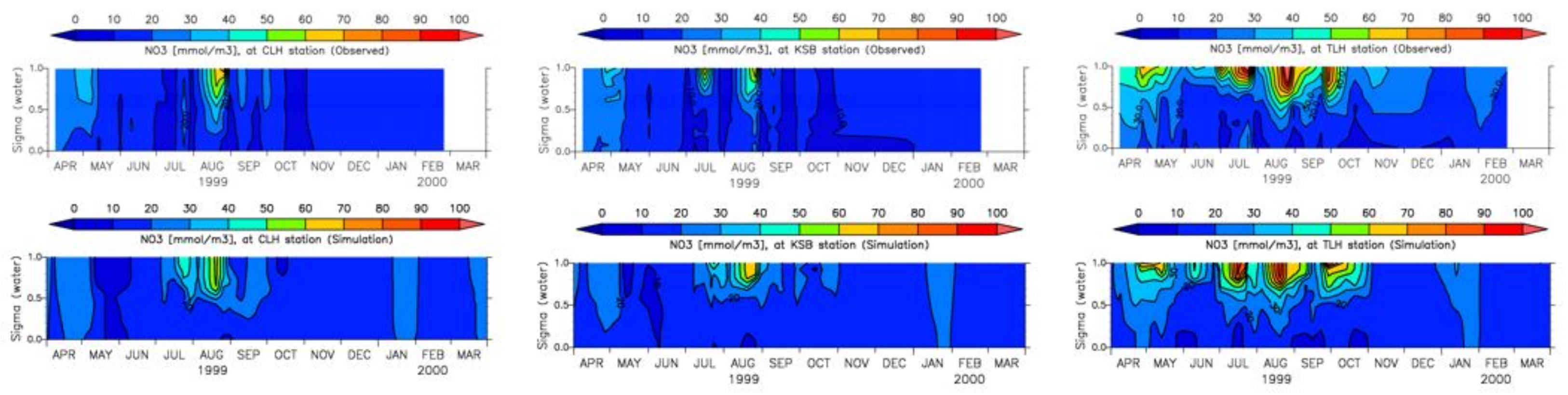
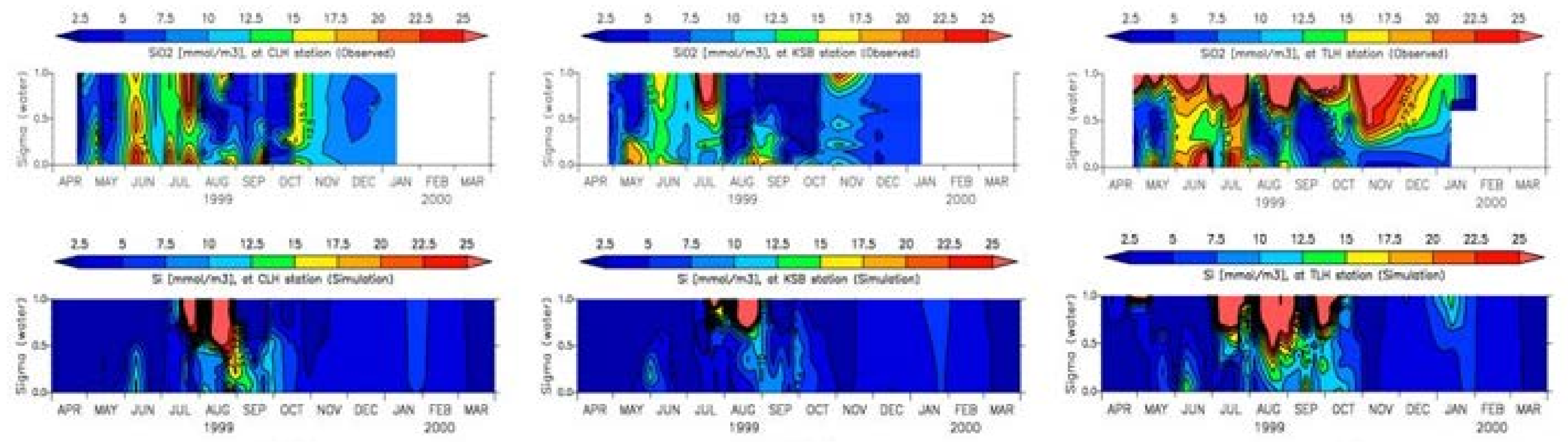
4. Results
4.1. Water Quality
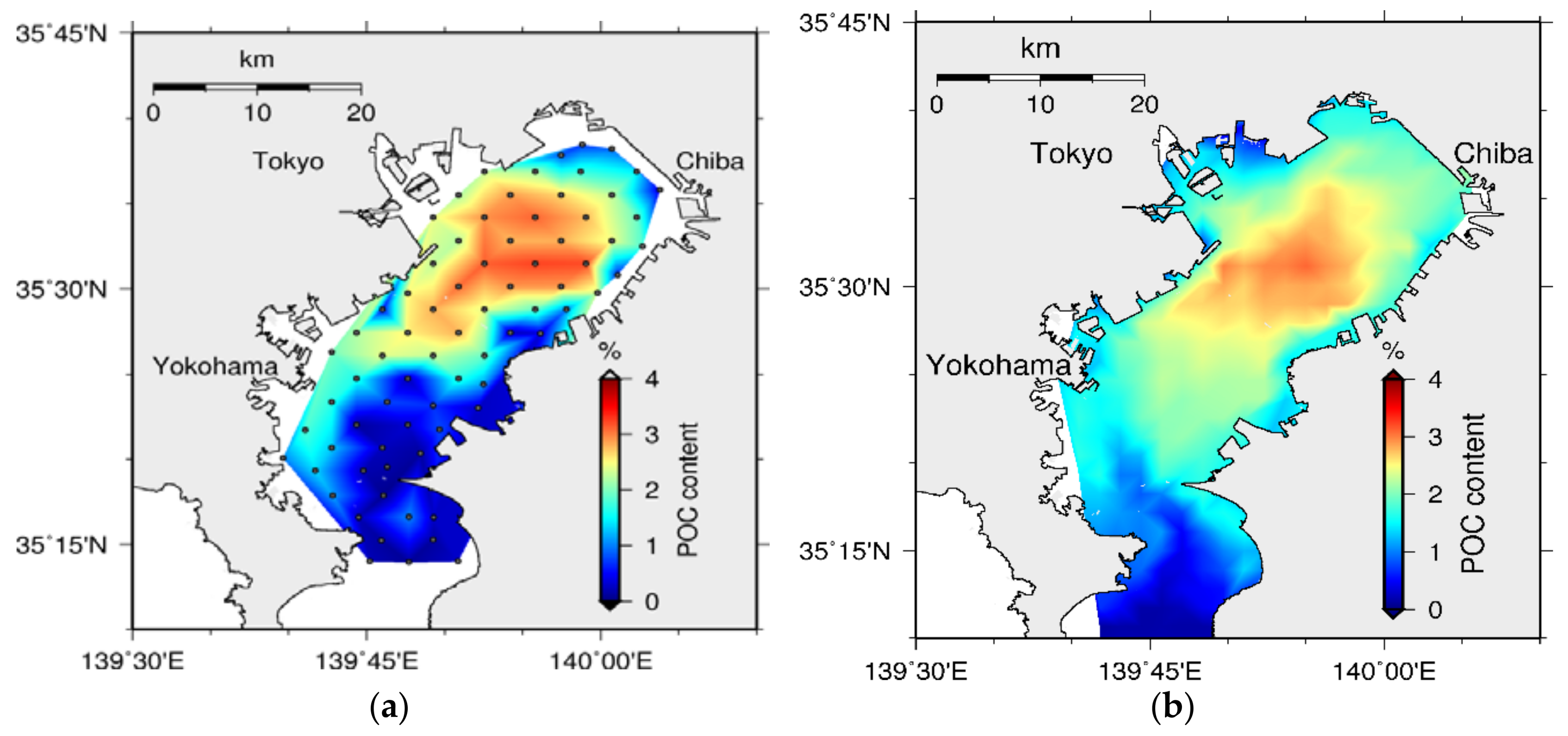
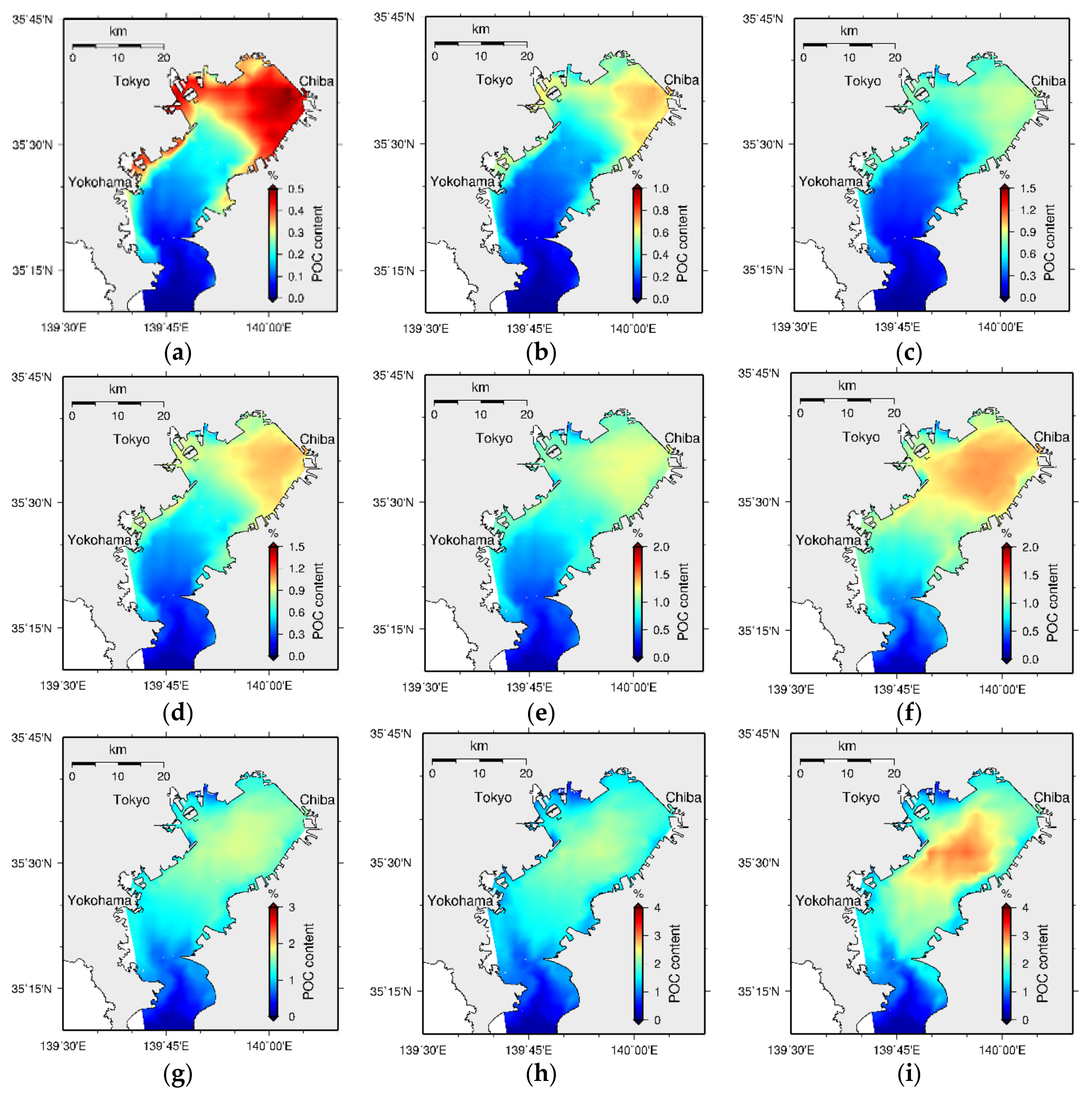
4.2. Sediment Quality
5. Discussion
5.1. Environmental Controls of Water Quality
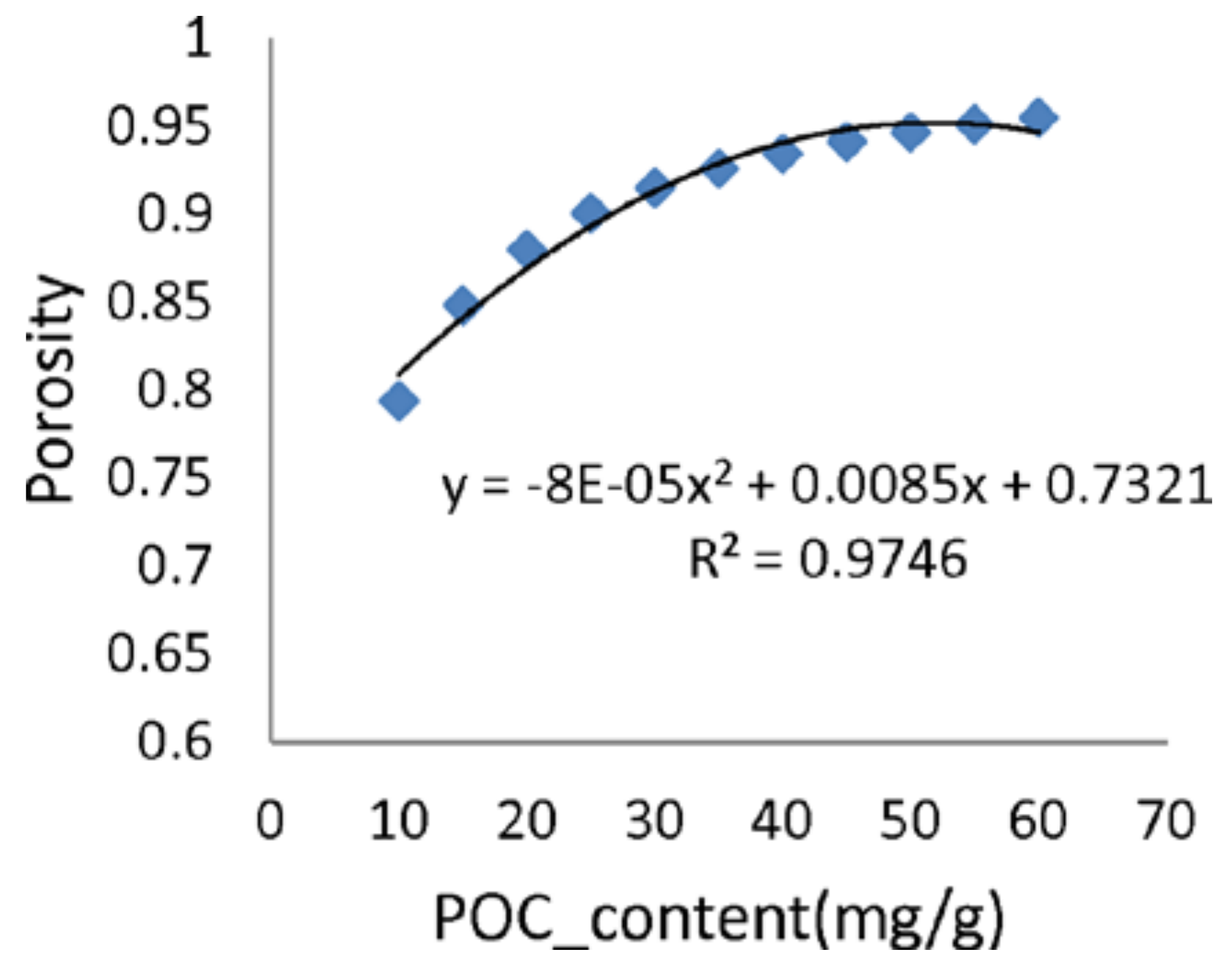
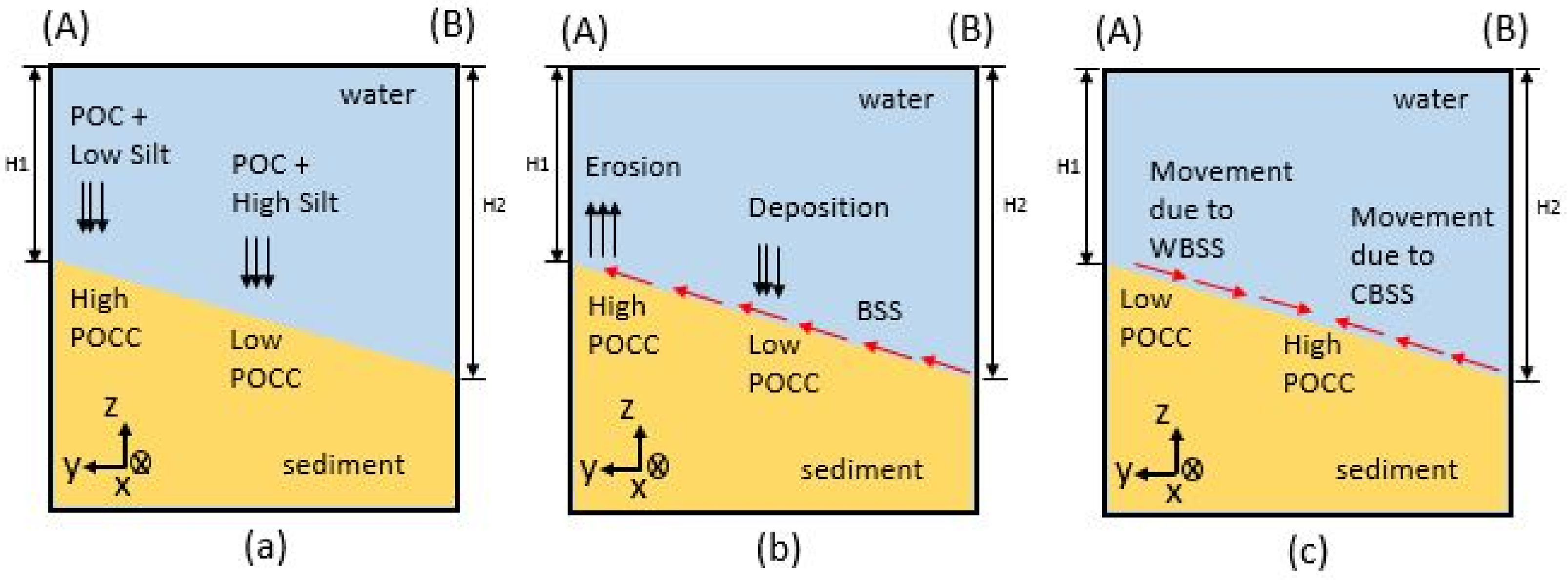
5.2. Bed Formation
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A
| Symbol | Unit | Description | |
|---|---|---|---|
| [Water] | [Sediment] | ||
| Salinity | |||
| [°C] | Temperature | ||
| Phytoplankton concentration | |||
| Zooplankton concentration | |||
| Chlorophyll a concentration | |||
| Particulate organic carbon concentration | |||
| Ammonium nitrogen concentration | |||
| Phosphate phosphorous concentration | |||
| Nitrate nitrogen concentration | |||
| Dissolved silica concentration | |||
| Particulate biogenic silica concentration | |||
| Dissolved oxygen concentration | |||
| Sulfide concentration | |||
| Silt concentration | |||
| Pelagic model | |||
| [Phytoplankton model] | |||
| Rate of primary production | |||
| Rate of metabolism | |||
| Rate of mortality | |||
| Rate of zooplankton grazing on each food | |||
| [1/s] | Maximum rate of phytoplankton growth at 20 °C | ||
| [1/s] | Maximum rate of phytoplankton metabolism at 20 °C | ||
| [1/s] | Maximum rate of phytoplankton mortality at 20 °C | ||
| - | Temperature limit for phytoplankton growth | ||
| - | Light limit for phytoplankton growth | ||
| - | Nutrient limit for phytoplankton growth | ||
| - | Phosphate nutrient limit for phytoplankton growth | ||
| - | Nitrogen nutrient limit for phytoplankton growth | ||
| - | Silica nutrient limit for phytoplankton growth | ||
| [°C] | Lower end of optimal temperature | ||
| [°C] | Upper end of optimal temperature | ||
| Half saturation constant of phosphorous limitation | |||
| Half saturation constant of nitrogen limitation | |||
| Half saturation constant of silica limitation | |||
| Photosynthetically active radiation | |||
| Photosynthetically active radiation at surface | |||
| Optimum of photosynthetically active radiation | |||
| Minimum of photosynthetically active radiation | |||
| - | Light extinction coefficient | ||
| [Zooplankton model] | |||
| Rate of zooplankton growth | |||
| Rate of zooplankton mortality | |||
| Rate of zooplankton absorption | |||
| Rate of availability of minimum food for zooplankton | |||
| [1/s] | Maximum rate of minimum food for zooplankton at 20 °C | ||
| - | Maximum absorb portion of food by zooplankton at 20 °C | ||
| Oxygen half saturation constant for zooplankton metabolism | |||
| Rate of zooplankton grazing at 20 °C | |||
| Rate of zooplankton grazing for each food at 20 °C | |||
| Grazing primitive of zooplankton | |||
| [1/s] | Maximum rate of zooplankton growth at 20 °C | ||
| - | Temperature limit for zooplankton | ||
| - | Food limit for zooplankton | ||
| Threshold food concentration | |||
| Total food proportionality constant for Ivlev formulation | |||
| Rate of zooplankton fecal at 20 °C | |||
| Maximum concentration of zooplankton at 20 °C | |||
| [1/s] | Maximum rate of zooplankton mortality at 20 °C | ||
| [Particulate organic carbon model] | |||
| Rate of aerobic decomposition | |||
| [1/s] | Maximum rate of aerobic decomposition at 20 °C | ||
| - | Temperature coefficient for aerobic decomposition | ||
| Oxygen half saturation constant for aerobic decomposition | |||
| Rate of denitrification as a fraction of POC | |||
| Rate of denitrification | |||
| [1/s] | Maximum rate of denitrification at 20 °C | ||
| - | Temperature coefficient for denitrification | ||
| Half saturation constant for POC in denitrification | |||
| Rate of anaerobic decomposition | |||
| [1/s] | Maximum rate of anaerobic decomposition at 20 °C | ||
| - | Temperature coefficient for anaerobic decomposition | ||
| Half saturation constant for denitrification | |||
| - | Fraction of particulate organic carbon | ||
| [Ammonia model] | |||
| Rate of nitrification | |||
| [1/s] | Maximum rate of nitrification at 20 °C | ||
| - | Temperature coefficient for nitrification | ||
| Oxygen half saturation constant for nitrification | |||
| [Silica model] | |||
| Rate of silica production | |||
| [1/s] | Maximum rate of silica production at 20 °C | ||
| - | Temperature coefficient for particulate silica dissolution | ||
| Half saturation constant for particulate silica dissolution | |||
| Saturated concentration of silica | |||
| [Sulfide model] | |||
| Rate of sulfide oxidization | |||
| [1/s] | Maximum rate of sulfide oxidization at 20 °C | ||
| - | Temperature coefficient for sulfide oxidization | ||
| Oxygen half saturation constant for sulfide oxidation | |||
| Benthic model | |||
| [Particulate organic carbon model] | |||
| Rate of aerobic decomposition | |||
| [1/s] | Maximum rate of aerobic decomposition at 20 °C | ||
| - | Temperature coefficient for aerobic decomposition | ||
| Oxygen half saturation constant for aerobic decomposition | |||
| Rate of denitrification as a fraction of POC | |||
| Rate of denitrification | |||
| [1/s] | Maximum rate of denitrification at 20 °C | ||
| - | Temperature coefficient for denitrification | ||
| Half saturation constant for POC in denitrification | |||
| Rate of anaerobic decomposition | |||
| [1/s] | Maximum rate of anaerobic decomposition at 20 °C | ||
| - | Temperature coefficient for anaerobic decomposition | ||
| Half saturation constant for denitrification | |||
| [Ammonia model] | |||
| Rate of nitrification | |||
| [1/s] | Maximum rate of nitrification at 20 °C | ||
| - | Temperature coefficient for nitrification | ||
| Oxygen half saturation constant for nitrification | |||
| [Silica model] | |||
| Rate of silica production | |||
| [1/s] | Maximum rate of silica production at 20 °C | ||
| - | Temperature coefficient for particulate silica dissolution | ||
| Half saturation constant for particulate silica dissolution | |||
| Saturated concentration of silica | |||
| [Sulfide model] | |||
| Rate of sulfide oxidization | |||
| [1/s] | Maximum rate of sulfide oxidization at 20 °C | ||
| - | Temperature coefficient for sulfide oxidization | ||
| Half saturation constant of oxygen for sulfide oxidization | |||
| [Transfer ratios] | |||
| Ratio of phosphorous to carbon for particulate organic carbon decomposition | |||
| Ratio of nitrogen to carbon for particulate organic carbon decomposition | |||
| Ratio of carbon to nitrogen for denitrification | |||
| Ratio of silica to carbon for particulate organic carbon decomposition | |||
| Ratio of dissolved silica to particulate silica | |||
| Ratio of carbon to sulfur for particulate organic carbon anoxic decomposition | |||
| Ratio of oxygen to carbon for particulate organic carbon decomposition | |||
| Ratio of oxygen to nitrogen for nitrification | |||
| Ratio of oxygen to sulfur for sulfide oxidization | |||
| Benthic–pelagic interaction parameters | |||
| Diffusion flux of phosphate between sediment and water | |||
| Diffusion flux of ammonia between sediment and water | |||
| Diffusion flux of nitrate between sediment and water | |||
| Diffusion flux of Silica between sediment and water | |||
| Diffusion flux of sulfide between sediment and water | |||
| Diffusion flux of oxygen between sediment and water | |||
| Parameter | Units | Value | Main Source |
|---|---|---|---|
| Pelagic model | |||
| Phytoplankton model | |||
| [1/d] | (2.772, 2.772, 2.772) a | [6,75,76] | |
| [1/d] | (0.083, 0.083, 0.083) a | [6,56,76] | |
| [1/d] | (0.1386, 0.1386, 0.1386) a | [6,56,76] | |
| - | (0.8, 0.8, 0.8) a | [20] | |
| - | (0.8, 0.8, 0.8) a | [20] | |
| (1 × 10−8, 0.01, 1 × 10−3) a | [6,56,76] | ||
| (0.5, 0.25, 1 × 10-3) a | [6,56,76] | ||
| (1 × 10−3, 0.02, 1 × 10−3) a | [77] | ||
| (2, 25, 25) a | Tuc | ||
| Zooplankton model | |||
| [1/d] | 0.04 | [78] | |
| - | 0.6 | [76,78,79] | |
| 1.0 | [78] | ||
| [1/d] | 0.2 | [6,56,78] | |
| 0.01 | [76,78,79] | ||
| 50.0 | [78] | ||
| 100.0 | Tuc | ||
| [1/d] | 0.05 | [78] | |
| Particulate organic carbon model | |||
| [1/d] | (0.35, 0.018, 0.0) b | [19] | |
| - | (1.08, 1.08, -) b | [19] | |
| 0.1 | [6] | ||
| [1/d] | (2.0, 0.1, 0.0) b | Tuc | |
| - | 1.086 | [19] | |
| 10.0 | Tuc | ||
| [1/d] | (0.035, 0.0018, 0.0) b | [19] | |
| - | (1.08, 1.15, -) b | [19] | |
| 0.5 | [19] | ||
| - | (0.65, 0.25, 0.1) b | [19] | |
| Ammonium model | |||
| [1/d] | 0.06 | Tuc | |
| - | 1.123 | [19] | |
| 0.37 | [19] | ||
| Silica model | |||
| [1/d] | 1.925 × 10−3 | [19] | |
| - | 1.059 | [19] | |
| 19.8 | [19] | ||
| 26.5 | [19] | ||
| Sulfide model | |||
| [1/d] | 6.0 | Tuc | |
| - | 1.123 | [19] | |
| 2.0 | [19] | ||
| Benthic model | |||
| Particulate organic carbon model | |||
| [1/d] | (0.35, 0.018, 0.0) b | [19] | |
| - | (1.08, 1.08, -) b | [19] | |
| 0.1 | [6] | ||
| [1/d] | (2.0, 0.1, 0.0) b | Tuc | |
| - | 1.086 | [19] | |
| 5000.0 | Tuc | ||
| [1/d] | (0.035, 0.0018, 0.0) b | [19] | |
| - | (1.08, 1.15, -) b | [19] | |
| 0.5 | [19] | ||
| Ammonium model | |||
| [1/d] | 140.63 | Tuc | |
| - | 1.123 | [19] | |
| 0.37 | [19] | ||
| Silica model | |||
| [1/d] | 1.925 × 10−3 | [19] | |
| - | 1.059 | [19] | |
| 19.8 | [19] | ||
| 26.5 | [19] | ||
| Sulfide model | |||
| [1/d] | 6.0 | Tuc | |
| - | 1.123 | [19] | |
| 0.0001 | [19] | ||
| Transfer ratios | |||
| 1.0/(12.0 × 106.0) | [19] | ||
| 16.0/(12.0 × 106.0) | [19] | ||
| (106.0 × 12.0 × 5.0)/424.0 | [18,19] | ||
| 1.0/(12.0 × 8.0) | [19] | ||
| 28.0 | [19] | ||
| (53.0 × 32.0 × 10−3)/(106.0 × 12.0) | [18,19] | ||
| (32.0 × 10−3)/12.0 | [19] | ||
| 4.33 × 14.0 × 10−3 | [18,19] | ||
| 2.0 | [19] | ||
| Biochemical Process | Formulation |
|---|---|
| Phytoplankton | |
| Primary production: | |
| Metabolism: | |
| Mortality: | |
| Zooplankton Grazing: | |
| Zooplankton | |
| Growth: | |
| Mortality: | |
| Fecal: | |
| Metabolism: | |
| Particulate organic carbon | |
| Aerobic carbon diagenesis: | |
| Anaerobic carbon diagenesis with nitrate as electron accepter or denitrification: | |
| Anaerobic carbon diagenesis with sulfate as electron accepter or sulfide production: | |
| Phosphorous | |
| Ammonia | |
| Nitrification: | |
| Nitrate | |
| Particulate silica | |
| Dissolved silica production or particulate silica dissolution: | |
| Dissolved silica | |
| Sulfide | |
| Sulfide oxidation: | |
| Dissolved oxygen | |
| Biochemical Process | Formulation |
|---|---|
| Particulate organic carbon | |
| Aerobic carbon diagenesis: | |
| Anaerobic carbon diagenesis with nitrate as electron accepter or denitrification: | |
| Anaerobic carbon diagenesis with sulfate as electron accepter or sulfide production: | |
| Phosphorous | |
| Ammonia | |
| Nitrification: | |
| Nitrate | |
| Particulate silica | |
| Dissolved silica production or particulate silica dissolution: | |
| Dissolved silica | |
| Sulfide | |
| Sulfide oxidation: | |
| Dissolved oxygen | |
References
- Wild-Allen, K.; Skerratt, J.; Whitehead, J.; Rizwi, F.; Parslow, J. Mechanisms driving estuarine water quality: A 3D biogeochemical model for informed management. Estuar. Coast. Shelf Sci. 2013, 135, 33–45. [Google Scholar] [CrossRef]
- Weinberger, S.; Vetter, M. Using the hydrodynamic model DYRESM based on results of a regional climate model to estimate water temperature changes at Lake Ammersee. Ecol. Model. 2012, 244, 38–48. [Google Scholar] [CrossRef]
- Elhakeem, A.; Elshorbagy, W.; Bleninger, T. Long-term hydrodynamic modeling of the Arabian Gulf. Mar. Pollut. Bull. 2015, 94, 19–36. [Google Scholar] [CrossRef] [PubMed]
- Henderson, A.; Gamito, S.; Karakassis, I.; Pederson, P.; Smaal, A. Use of hydrodynamic and benthic models for managing environmental impacts of marine aquaculture. J. Appl. Ichthyol. 2001, 17, 163–172. [Google Scholar] [CrossRef]
- Rasmeemasmuang, T.; Sasaki, J. Modeling of mud accumulation and bed characteristics in Tokyo Bay. Coast. Eng. J. 2008, 50, 277–307. [Google Scholar] [CrossRef]
- Sohma, A.; Sekiguchi, Y.; Kuwae, T.; Nakamura, Y. A benthic–pelagic coupled ecosystem model to estimate the hypoxic estuary including tidal flat—Model description and validation of seasonal/daily dynamics. Ecol. Model. 2008, 215, 10–39. [Google Scholar] [CrossRef]
- De Mora, L.; Butenschön, M.; Allen, J.I. The assessment of a global marine ecosystem model on the basis of emergent properties and ecosystem function: A case study with ERSEM. Geosci. Model Dev. 2016, 9, 59–76. [Google Scholar] [CrossRef]
- Doney, S.C. Major challenges confronting marine biogeochemical modeling. Glob. Biogeochem. Cycles 1999, 13, 705–714. [Google Scholar] [CrossRef]
- Arhonditsis, G.B.; Brett, M.T. Evaluation of the current state of mechanistic aquatic biogeochemical modeling. Mar. Ecol. Prog. Ser. 2004, 271, 13–26. [Google Scholar] [CrossRef]
- Cerco, C.F.; Thomas, C. Three-dimensional eutrophication model of Chesapeake Bay. J. Environ. Eng. 1993, 119, 1006–1025. [Google Scholar] [CrossRef]
- Proctor, R.; Holt, J.T.; Allen, J.I.; Blackford, J. Nutrient fluxes and budgets for the North West European Shelf from a three-dimensional model. Sci. Total Environ. 2003, 314–316, 769–785. [Google Scholar] [CrossRef]
- Fennel, K.; Wilkin, J.; Levin, J.; Moisan, J.; O’Reilly, J.; Haidvogel, D. Nitrogen cycling in the Middle Atlantic Bight: Results from a three-dimensional model and implications for the North Atlantic nitrogen budget. Glob. Biogeochem. Cycles 2006, 20, GB3007. [Google Scholar] [CrossRef]
- Smits, J.G.C.; van Beek, J.K.L. ECO: A generic eutrophication model including comprehensive sediment–water interaction. PLoS ONE 2013, 8, 25. [Google Scholar] [CrossRef] [PubMed]
- Holstein, J.M.; Wirtz, K.W. Sensitivity analysis of nitrogen and carbon cycling in marine sediments. Estuar. Coast. Shelf Sci. 2009, 82, 632–644. [Google Scholar] [CrossRef]
- Soetaert, K.; Herman, P.M.J.; Middelburg, J.J. A model of early diagenetic processes from the shelf to abyssal depths. Geochim. Cosmochim. Acta 1996, 60, 1019–1040. [Google Scholar] [CrossRef]
- Krumins, V.; Gehlen, M.; Arndt, S.; Van Cappellen, P.; Regnier, P. Dissolved inorganic carbon and alkalinity fluxes from coastal marine sediments: Model estimates for different shelf environments and sensitivity to global change. Biogeosciences 2013, 10, 371–398. [Google Scholar] [CrossRef]
- Capet, A.; Meysman, F.J.R.; Akoumianaki, I.; Soetaert, K.; Grégoire, M. Integrating sediment biogeochemistry into 3D oceanic models: A study of benthic–pelagic coupling in the Black Sea. Ocean Model. 2016, 101, 83–100. [Google Scholar] [CrossRef]
- Mussap, G.; Zavatarelli, M. A numerical study of the benthic–pelagic coupling in a shallow shelf sea (Gulf of Trieste). Reg. Stud. Mar. Sci. 2017, 9, 24–34. [Google Scholar] [CrossRef][Green Version]
- DiToro, D.M. Sediment Flux Modeling, 1st ed.; John Wiley & Sons, Inc.: New York, NY, USA, 2001; 656p, ISBN 978-0-471-13535-7. [Google Scholar]
- Ji, Z.-G. Hydrodynamics and Water Quality: Modeling Rivers, Lakes, and Estuaries; John Wiley & Sons, Inc.: New York, NY, USA, 2007; 704p, ISBN 9780470135433. [Google Scholar]
- Mann, K.H.; Lazier, J.R.N. Dynamics of Marine Ecosystems: Biological-Physical Interactions in the Oceans, 3rd ed.; John Wiley & Sons, Inc.: New York, NY, USA, 2013; 512p, ISBN 978-1-118-68791-8. [Google Scholar]
- Søndergaard, M.; Jensen, J.P.; Jeppesen, E. Role of sediment and internal loading of phosphorus in shallow lakes. Hydrobiologia 2003, 506–509, 135–145. [Google Scholar] [CrossRef]
- Burdige, D.J. 5.09—Estuarine and Coastal Sediments—Coupled Biogeochemical Cycling; Treatise on Estuarine and Coastal Science; Academic Press: Waltham, MA, USA, 2011; Volume 5, pp. 279–316. [Google Scholar]
- Soetaert, K.; Middelburg, J.J.; Herman, P.M.J.; Buis, K. On the coupling of benthic and pelagic biogeochemical models. Earth Sci. Rev. 2000, 51, 173–201. [Google Scholar] [CrossRef]
- Marcus, N.H.; Boero, F. Minireview: The importance of benthic–pelagic coupling and the forgotten role of life cycles in coastal aquatic systems. Limnol. Oceanogr. 1998, 43, 763–768. [Google Scholar] [CrossRef]
- Raffaelli, D.; Bell, E.; Weithoff, G.; Matsumoto, A.; Motta, J.J.C.; Kershaw, P.; Parker, R.; Parry, D.; Jones, M. The ups and downs of benthic ecology: Considerations of scale, heterogeneity and surveillance for benthic–pelagic coupling. J. Exp. Mar. Biol. Ecol. 2003, 285, 191–203. [Google Scholar] [CrossRef]
- Nakane, T.; Nakaka, K.; Bouman, H.; Platt, T. Environmental control of short-term variation in the plankton community of inner Tokyo Bay, Japan. Estuar. Coast. Shelf Sci. 2008, 78, 796–810. [Google Scholar] [CrossRef]
- Koibuchi, Y.; Isobe, M. Phytoplankton bloom mechanism in an area affected by eutrophication: Tokyo bay in spring 1999. Coast. Eng. J. 2007, 49, 461–479. [Google Scholar] [CrossRef]
- Han, M.S.; Furuya, K. Size and species-specific primary productivity and community structure of phytoplankton in Tokyo Bay. J. Plankton Res. 2000, 22, 1221–1235. [Google Scholar] [CrossRef]
- Han, M.S.; Furuya, K. Species-specific productivity of Skeletonema costatum (Bacillariophyceae) in the inner part of Tokyo Bay. Mar. Ecol. Prog. Ser. 1991, 79, 267–273. [Google Scholar] [CrossRef]
- Matsukawa, Y.; Sasaki, K. Nitrogen budget in Tokyo Bay with special reference to the low sedimentation to supply ratio. J. Oceanogr. Soc. Jpn. 1990, 46, 44–54. [Google Scholar] [CrossRef]
- Sasaki, J.; Kanayama, S.; Nakase, K.; Kino, S. Effective application of mechanical circulator for reducing hypoxia in an estuarine trench. Coast. Eng. J. 2009, 51, 309–339. [Google Scholar] [CrossRef]
- Fujiwara, T.; Yamada, Y. Inflow of oceanic water into Tokyo Bay and generation of a subsurface hypoxic water mass. J. Geophys. Res. Oceans 2002, 107, 1–13. [Google Scholar] [CrossRef]
- Heip, C.H.R.; Goosen, N.K.; Herman, P.M.J.; Kromkamp, J.C.; Middelburg, J.J.; Soetaert, K.E.R. Production and consumption of biological particles in temperate tidal estuaries. Oceanogr. Mar. Biol. Ann. Rev. 1995, 33, 1–149. [Google Scholar]
- Sasaki, J.; Isobe, M. Development of a long-term predictive model for baroclinic circulation and its application to blue tide phenomenon in Tokyo Bay. In Proceedings of the 27th International Conference on Coastal Engineering (ICCE), Sydney, Australia, 16–21 July 2000. [Google Scholar]
- Achiari, H.; Sasaki, J. Numerical analysis of wind-wave climate change and spatial distribution of bottom sediment properties in Sanbanze Shallows of Tokyo Bay. J. Coast. Res. 2007, SI50, 343–347. [Google Scholar]
- Orton, T.G.; Lark, R.M.; Sasaki, J. Using Geostatistics to analyze prediction errors from a simulation model of sediment particle sizes across Tokyo Bay. J. Coast. Res. 2013, 29, 141–156. [Google Scholar] [CrossRef]
- Okada, T.; Furukawa, K. Mapping sediment condition and benthos of shoreward area in Tokyo Bay. Annu. Coast. Eng. J. JSCE 2005, 52, 1431–1435. [Google Scholar]
- Okada, T.; Furukawa, K. Mapping sediment conditions of shoreward area in Tokyo Bay using echo features. Proc. Civ. Eng. Ocean 2005, 21, 749–754. [Google Scholar] [CrossRef]
- Attari, M.J.; Sasaki, J. An enhanced numerical model for material cycling and dissolved oxygen dynamics in Tokyo Bay, Japan. In Proceedings of the International Conference on Estuarine and Coastal Modeling, St. Augustine, FL, USA, 7–9 November 2011. [Google Scholar]
- Amunugama, A.A.W.R.R.M.K.; Sasaki, J.; Nakamura, Y.; Suzuki, T. Development of a benthic–pelagic coupled model for reproducing water quality in Tokyo Bay. J. Jpn. Soc. Civ. Eng. Ser. B3 Ocean Eng. 2015, 71, I_886–I_891. [Google Scholar]
- Amunugama, A.A.W.R.R.M.K.; Sasaki, J.; Nakamura, Y.; Suzuki, T. Spatial distribution of sediment quality in Tokyo Bay through benthic–pelagic coupled modeling approach. J. Jpn. Soc. Civ. Eng. Ser. B2 Coast. Eng. 2015, 71, I_1399–I_1404. [Google Scholar] [CrossRef]
- Koibuchi, Y.; Ogura, H.; Ando, H.; Gomyo, M.; Sasaki, J.; Isobe, M. Observation of annual cycle of nutrients in the inner part of Tokyo Bay. Proc. Coastal Eng. JSCE 2000, 47, 1066–1070. [Google Scholar]
- Sasaki, J.; Isobe, M. Development of a long-term predictive model of water quality in Tokyo Bay. In Proceedings of the 6th Conference on Estuarine and Coastal Modeling, ASCE, New Orleans, FL, USA, 1999; pp. 564–580. [Google Scholar]
- Patankar, S.V. Numerical Heat Transfer and Fluid Flow; McGraw-Hill Inc.: New York, NY, USA, 1980; 200p, ISBN 9780070487406. [Google Scholar]
- US Army Corps of Engineers. Shore Protection Manual, 4th ed.; Dept. of the Army, Waterways Experiment Station, Corps of Engineers, Coastal Engineering Research Center, Govt. Printing Office: Washington, DC, USA, 1984; 337p.
- Hasselmann, K.; Sell, W.; Ross, D.B.; Müller, P. A Parametric Wave Prediction Model. J. Phys. Oceanogr. 1976, 6, 200–228. [Google Scholar] [CrossRef]
- Bretschneider, C.L.; Reid, R. Change in wave height due to bottom friction, percolation and refraction. In Proceedings of the 34th Annual Meeting of American Geophisical Union, San Francisco, CA, USA, 14–18 December 2009. [Google Scholar]
- Putnam, J.A. Loss of wave energy due to percolation in a permeable sea bottom. EOS Trans. Am. Geophys. Union 1949, 30, 349–356. [Google Scholar] [CrossRef]
- Putnam, J.A.; Johson, J.W. The dissipation of wave energy by bottom friction. EOS Trans. Am. Geophys. Union 1949, 30, 67–74. [Google Scholar] [CrossRef]
- Van Rijn, L.C. Principles of Sediment Transport in Rivers, Estuaries and Coastal Seas; Aqua Publications: Blokzijl, The Netherland, 1993; Volume 1006, 715p, ISBN 90-800356-2-9. [Google Scholar]
- Gerritsen, H.; Vos, R.J.; van der Kaaij, T.; Lane, A.; Boon, J.G. Suspended sediment modelling in a shelf sea (North Sea). Coast. Eng. 2000, 41, 317–352. [Google Scholar] [CrossRef]
- Muller, A.C.; Muller, D.L. Forecasting future estuarine hypoxia using a wavelet based neural network model. Ocean Model. 2015, 96, 314–323. [Google Scholar] [CrossRef]
- Pan, C.W.; Chuang, Y.L.; Chou, L.S.; Chen, M.H.; Lin, H.J. Factors governing phytoplankton biomass and production in tropical estuaries of western Taiwan. Cont. Shelf Res. 2016, 118, 88–99. [Google Scholar] [CrossRef]
- Chai, C.; Jiang, T.; Cen, J.; Ge, W.; Lu, S. Phytoplankton pigments and functional community structure in relation to environmental factors in the Pearl River Estuary. Oceanologia 2016, 58, 201–211. [Google Scholar] [CrossRef]
- Agawin, N.S.R.; Duarte, C.M.; Agustí, S. Nutrient and temperature control of the contribution of picoplankton to phytoplankton biomass and production. Limnol. Oceanogr. 2000, 45, 591–600. [Google Scholar] [CrossRef]
- Riegman, R.; Kuipers, B.R.; Noordeloos, A.A.M.; Witte, H.J. Size-differential control of phytoplankton and the structure of plankton communities. Neth. J. Sea Res. 1993, 31, 255–265. [Google Scholar] [CrossRef]
- Fennel, W.; Neumann, T. Introduction to the Modelling of Marine Ecosystems; Halpern, D., Ed.; Elsevier: New York, NY, USA, 2004; Volume 72, 297p, ISBN 978-0444517029. [Google Scholar]
- Pennock, J.R. Chlorophyll distributions in the Delaware estuary: Regulation by light-limitation. Estuar. Coast. Shelf Sci. 1985, 21, 711–725. [Google Scholar] [CrossRef]
- Cote, B.; Platt, T. Day-to-day variations in the spring-summer photosynthetic parameters of coastal marine phytoplankton. Limnol. Oceanogr. 1983, 28, 320–344. [Google Scholar] [CrossRef]
- Walsh, J.J.; Whitledg, T.E.; Barvenik, F.W.; Wirick, C.D.; Howe, S.O. Wind events and food chain dynamics within the New York Bight. Limnol. Oceanogr. 1978, 23, 659–683. [Google Scholar] [CrossRef]
- Bouman, H.A.; Nakane, T.; Oka, K.; Nakata, K.; Kunta, K.; Sathyendranath, S.; Platt, T. Environmental controls on phytoplankton production in coastal ecosystems: A case study from Tokyo Bay. Estuar. Coast. Shelf Sci. 2010, 87, 63–72. [Google Scholar] [CrossRef]
- Harrison, W.G.; Platt, T. Photosynthesis-irradiance relationships in polar and temperate phytoplankton populations. Polar Biol. 1986, 5, 153–164. [Google Scholar] [CrossRef]
- Goldman, J.C.; Carpenter, E.J. A kinetic approach to the effect of temperature on algal growth. Limnol. Oceanogr. 1974, 19, 756–766. [Google Scholar] [CrossRef]
- Sasaki, J.; Koibuchi, Y.; Isobe, M. Realistic numerical simulation of water quality and ecosystems in Tokyo Bay. In Proceedings of the Third Joint Meeting of the Coastal Environmental Science and Technology Panel of the United States-Japan Cooperative Program in Natural Resources, Yokohama, Japan, 16–19 July 2002; pp. 143–157. [Google Scholar]
- Otsubo, K.; Harashima, A.; Miyazaki, T.; Yasuoka, Y.; Muraoka, K. Field survey and hydraulic study of ‘Aoshio’ in Tokyo Bay. Mar. Pollut. Bull. 1991, 23, 51–55. [Google Scholar] [CrossRef]
- Yoon, J.S.; Nakatsuji, K.; Muraoka, K. Experimental study on wind-driven stratified flow in enclosed water body. Proc. Hydraul. Eng. 1993, 37, 285–292. [Google Scholar] [CrossRef]
- Matsuyama, M. Numerical experiment in Tokyo Bay: In relating to ‘Aoshio’. Bull. Coast. Oceangr. 1990, 28, 63–74. [Google Scholar]
- Zhu, Z.; Isobe, M. Criteria for the occurrence of wind-driven coastal upwelling associated with ‘Aoshio’ on the southeast shore of Tokyo Bay. J. Oceanogr. 2012, 68, 561–574. [Google Scholar] [CrossRef]
- Fagherazzi, S.; Wiberg, P.L. Importance of wind conditions, fetch, and water levels on wave-generated shear stresses in shallow intertidal basins. J. Geophys. Res. Earth Surf. 2009, 114, F03022. [Google Scholar] [CrossRef]
- Liu, G.; Wu, J.; Wang, Y. Near-bed sediment transport in a heavily modified coastal plain estuary. Int. J. Sediment Res. 2014, 29, 232–245. [Google Scholar] [CrossRef]
- Hunt, S.; Bryan, K.R.; Mullarney, J.C. The influence of wind and waves on the existence of stable intertidal morphology in meso-tidal estuaries. Geomorphology 2015, 228, 158–174. [Google Scholar] [CrossRef]
- Green, M.O. Very small waves and associated sediment resuspension on an estuarine intertidal flat. Estuar. Coast. Shelf Sci. 2011, 93, 449–459. [Google Scholar] [CrossRef]
- Hunt, S.; Bryan, K.R.; Mullarney, J.C. The effect of wind waves on spring-neap variations in sediment transport in two meso-tidal estuarine basins with contrasting fetch. Geomorphology 2017, 280, 76–88. [Google Scholar] [CrossRef]
- Horiguchi, F. Numerical simulations of seasonal cycle of Tokyo Bay using an ecosystem model. J. Adv. Mar. Sci. Technol. Soc. 2001, 12, 1–30. [Google Scholar]
- Rounds, S.; Wood, T.M.; Lynch, D.D. Modeling Discharge, Temperature, and Water Quality in the Tualatin River, Oregon; U.S. Department of the Interior, U.S. Geological Survey: Reston, VA, USA, 1999.
- Paasche, E. Silicon and the ecology of marine plankton diatoms. II. Silicate-uptake kinetics in five diatom species. Mar. Biol. 1973, 19, 262–269. [Google Scholar] [CrossRef]
- Bowie, G.L.; Mills, W.B.; Porcella, D.B.; Campbell, C.L.; Pagenkopf, J.R.; Rupp, G.L.; Johnson, K.M.; Chan, P.W.H.; Gherini, S.A. Rates, Constants, and Kinetics Formulations in Surface Water Quality Modeling, 2nd ed.; United States Environmental Protection Agency, Office of Research and Development, Environmental Research Laboratory: Athens, GA, USA, 1985; 105p.
- Baretta, J.; Ruardij, P. Tidal Flat Estuaries; Springer: Berlin/Heidelberg, Germany, 1988; Volume 71, 281p, ISBN 978-3-642-73753-4. [Google Scholar]


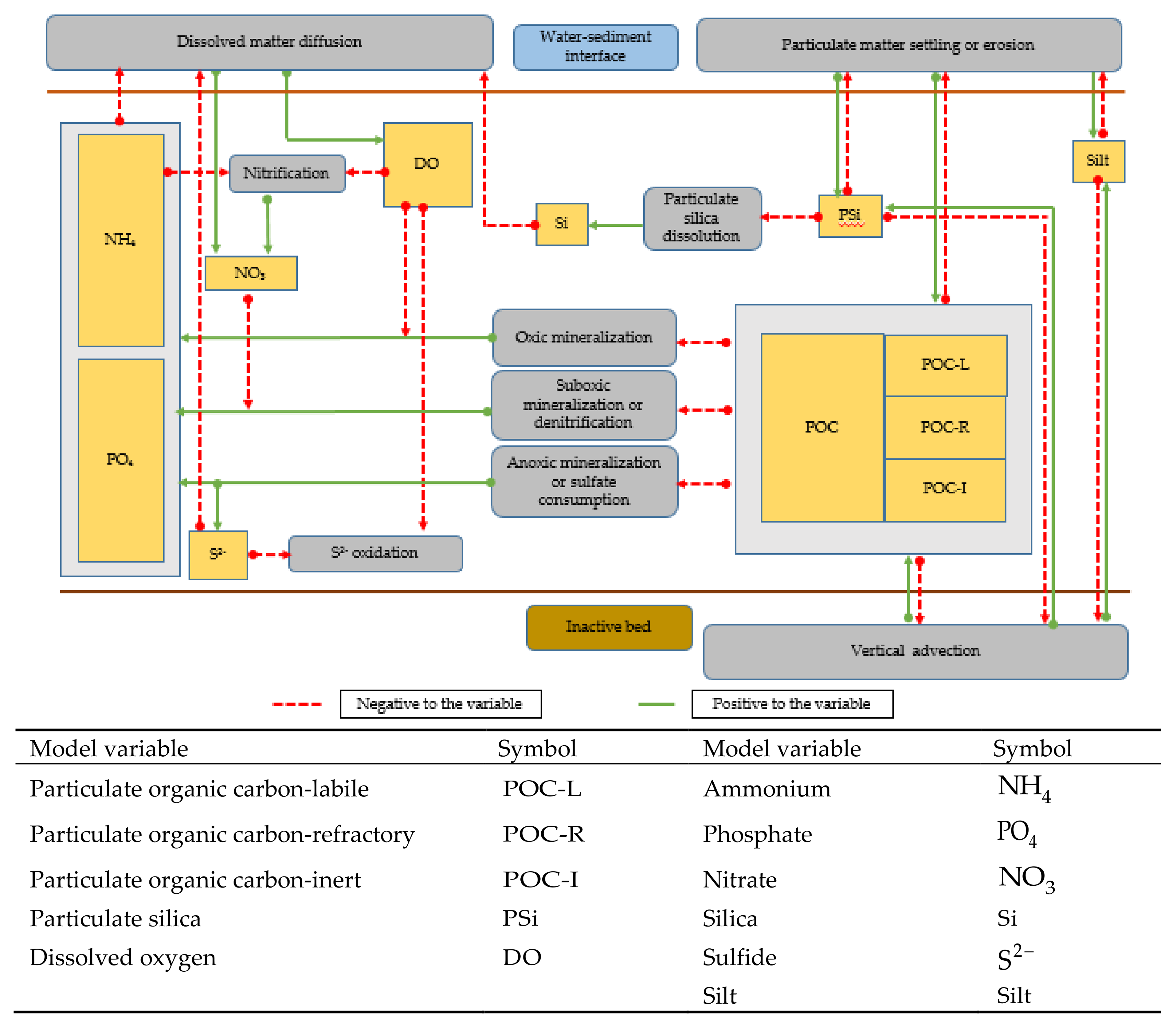






| Photosynthesis—ammonia as electron accepter |
| Photosynthesis—nitrate as electron accepter |
| Oxic mineralization and metabolism |
| Sub-oxic mineralization or denitrification |
| Anoxic mineralization or H2S production |
| Nitrification |
| H2S oxidization |
| Water Variables | Sediment Variables | Unit | |||
|---|---|---|---|---|---|
| Variable | Initial Value | Boundary Value | Variable | Initial Value | |
| 33.5 | 33.5 | ||||
| 13.0 | 13.0 | [°C] | |||
| 5.0, 5.0, 5.0 a | 5.0, 5.0, 5.0 a | ||||
| 37.5 | 37.5 | ||||
| 35.0, 14.0, 5.0 b | 35.0, 14.0, 5.0 b | 0.0, 0.0, 0.0 b | |||
| 10.0 | 10.0 | 0.0 | |||
| 0.5 | 0.5 | 0.0 | |||
| 14.0 | 14.0 | 0.0 | |||
| 2.5 | 2.5 | 0.0 | |||
| 16.0 | 16.0 | 0.0 | |||
| 7.0 | 7.0 | 0.0 | |||
| 0.0 | 0.0 | 0.0 | |||
| 1.0 | 1.0 | 0.0 | |||
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Amunugama, M.; Sasaki, J. Numerical Modeling of Long-Term Biogeochemical Processes and Its Application to Sedimentary Bed Formation in Tokyo Bay. Water 2018, 10, 572. https://doi.org/10.3390/w10050572
Amunugama M, Sasaki J. Numerical Modeling of Long-Term Biogeochemical Processes and Its Application to Sedimentary Bed Formation in Tokyo Bay. Water. 2018; 10(5):572. https://doi.org/10.3390/w10050572
Chicago/Turabian StyleAmunugama, Mangala, and Jun Sasaki. 2018. "Numerical Modeling of Long-Term Biogeochemical Processes and Its Application to Sedimentary Bed Formation in Tokyo Bay" Water 10, no. 5: 572. https://doi.org/10.3390/w10050572
APA StyleAmunugama, M., & Sasaki, J. (2018). Numerical Modeling of Long-Term Biogeochemical Processes and Its Application to Sedimentary Bed Formation in Tokyo Bay. Water, 10(5), 572. https://doi.org/10.3390/w10050572




