Longitudinal Dispersion in Straight Open Channels: Anomalous Breakthrough Curves and First-Order Analytical Solution for the Depth-Averaged Concentration
Abstract
:1. Introduction
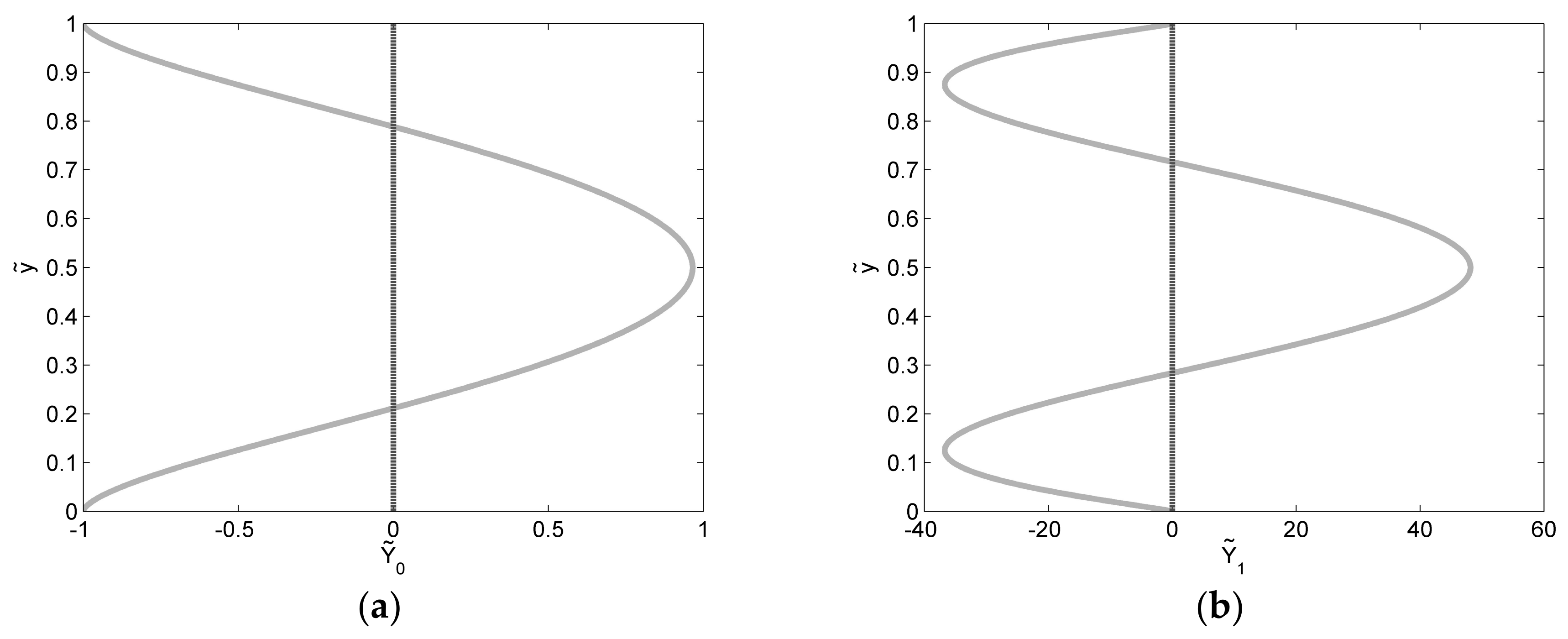
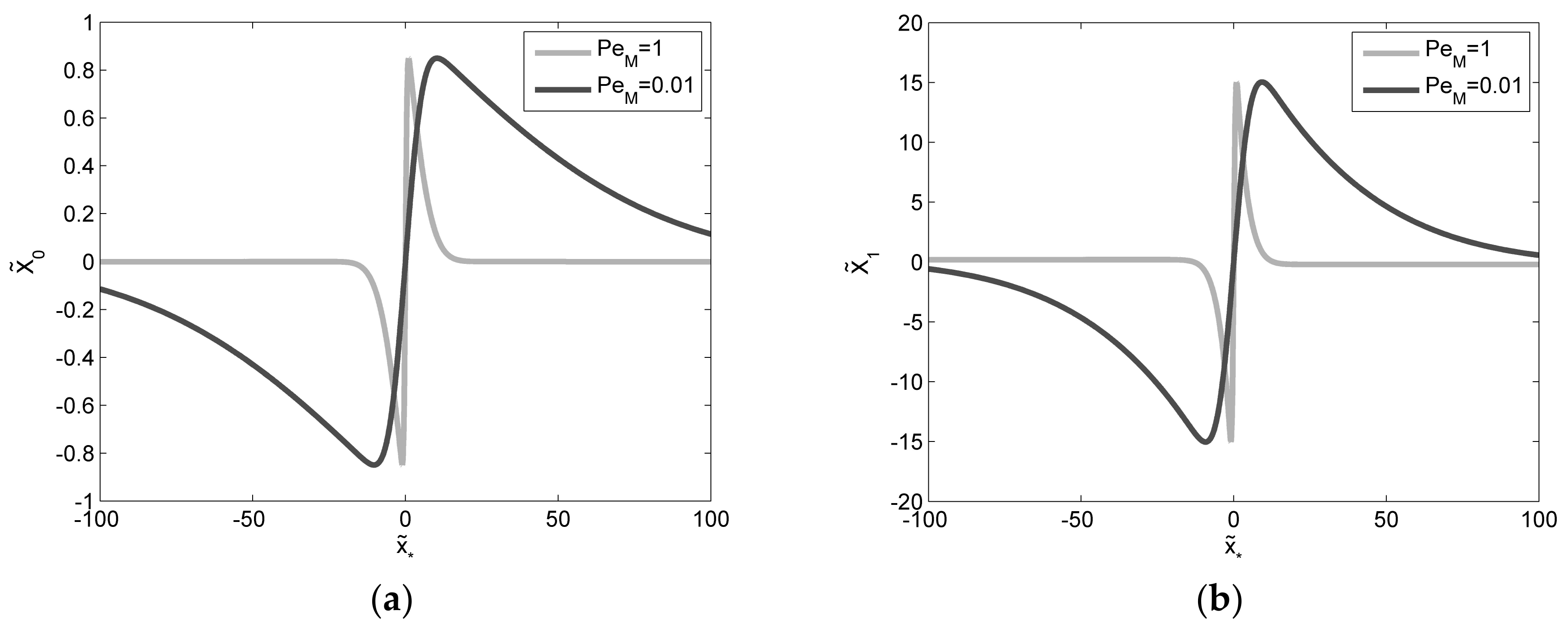
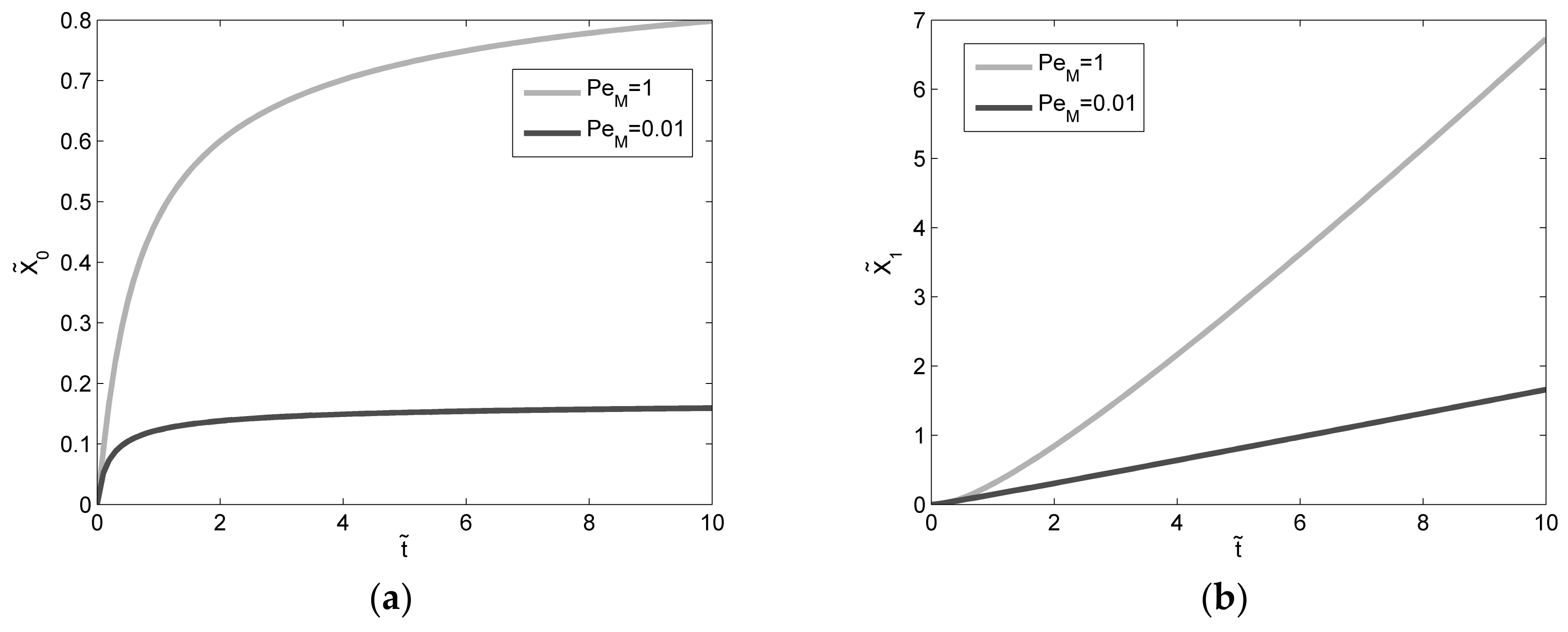
2. Formulation and First Order Analytical Solution
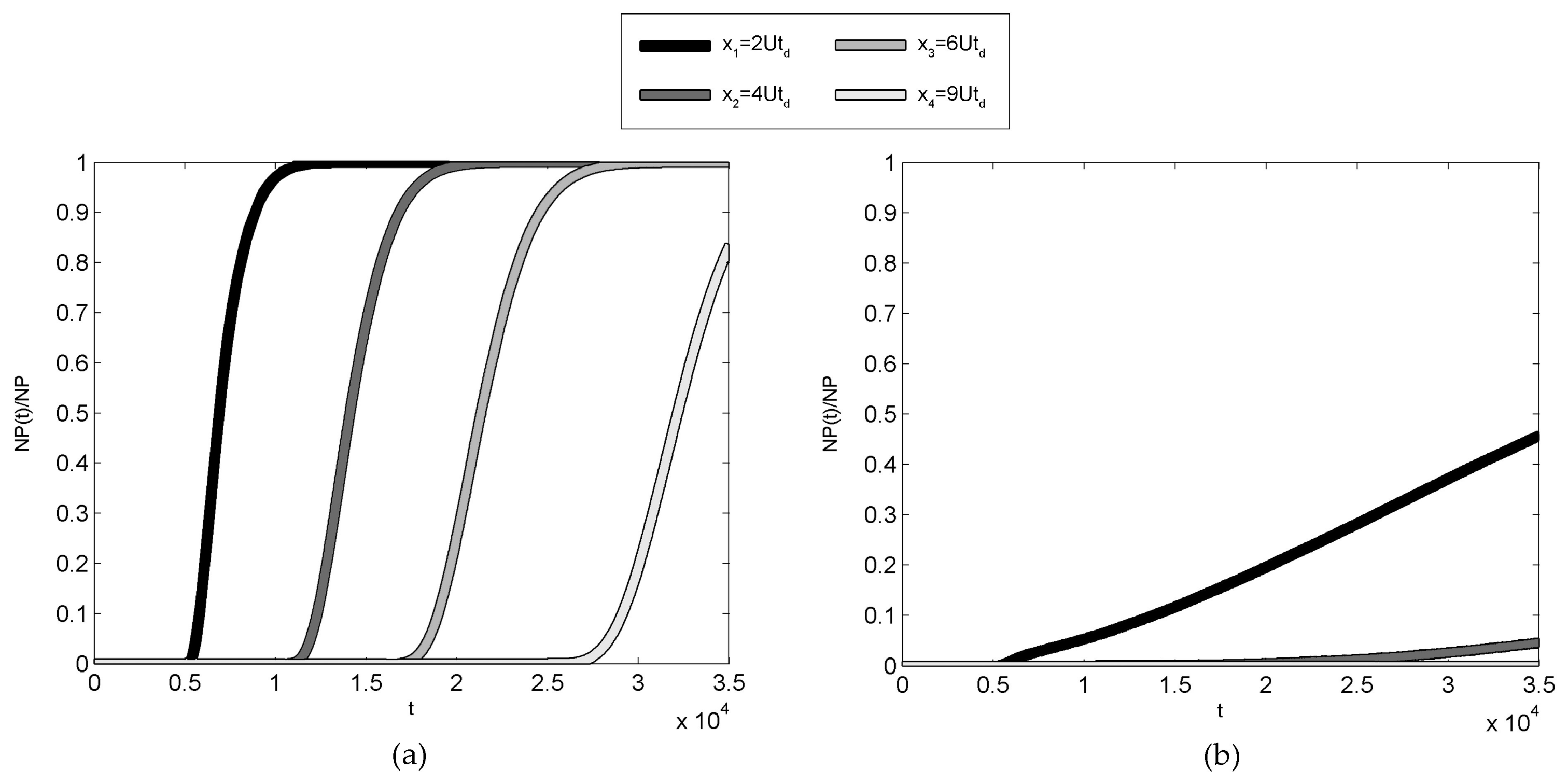
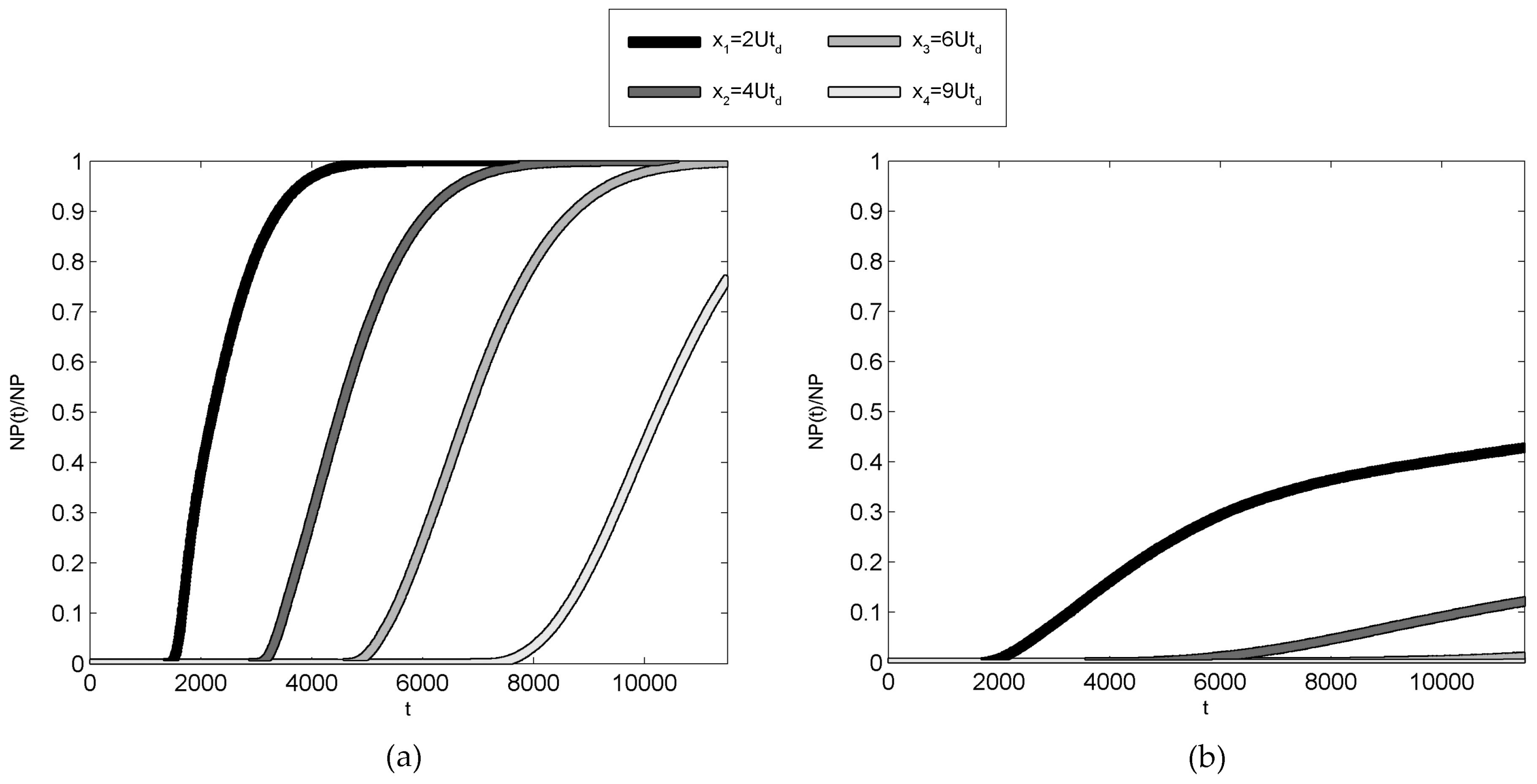
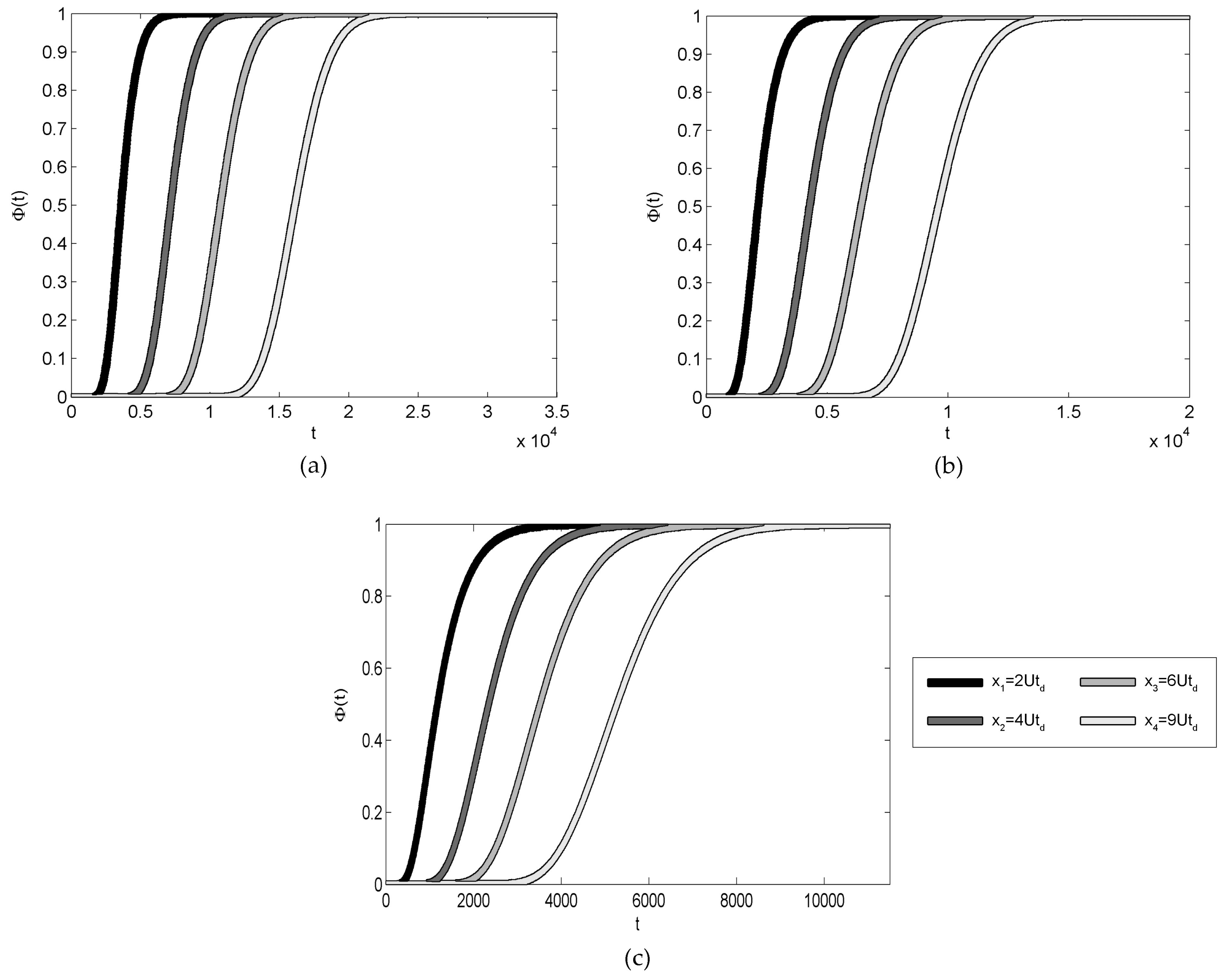
3. Numerical Lagrangian Simulations
4. Conclusions
Author Contributions
Conflicts of Interest
Notation
| A | flow area |
| B | free-surface width |
| c | (time-mean) concentration |
| depth-averaged concentration | |
| deviation of depth-averaged concentration | |
| C | section-averaged concentration |
| C0 | initial section-averaged concentration |
| dimensionless c | |
| dimensionless C | |
| zeroth order solution of perturbation expansion | |
| first order solution of perturbation expansion | |
| dimensionless distance between the two peaks of concentration variance | |
| Dx | longitudinal macro-dispersion coefficient |
| Δt | numerical time step |
| εx | longitudinal mixing coefficient |
| εy | transverse mixing coefficient |
| εz | vertical mixing coefficient |
| depth-averaged transverse mixing coefficient | |
| section-averaged transverse mixing coefficient | |
| Ei | Exponential-Integral Function |
| Erf | Error Function |
| Φxi | Cumulate of concentration at xi |
| g | acceleration due to gravity |
| generic element of a normal distribution | |
| h | local flow depth |
| H | average flow depth |
| Heq | depth of the equivalent rectangular cross-section |
| if | channel bed slope |
| M | total mass |
| NP | total number of particles |
| Pe | Peclet number |
| PeM | macro-Peclet number |
| Ψ | flow section-shape function |
| Sx | cloud longitudinal inertia moment |
| concentration variance | |
| t | time |
| dimensionless t | |
| t0 | longitudinal relaxation time |
| dimensionless longitudinal relaxation time | |
| td | average diffusive time |
| θ | aspect ratio calibration coefficient |
| u | (time-mean) longitudinal velocity |
| depth-averaged longitudinal velocity | |
| deviation of depth-averaged longitudinal velocity | |
| shear velocity | |
| depth-averaged shear velocity | |
| U | section-averaged longitudinal velocity |
| x | longitudinal spatial coordinate |
| y | transverse spatial coordinate |
| z | vertical spatial coordinate |
| dimensionless x | |
| dimensionless y | |
| dimensionless x in a reference frame moving with the section-averaged velocity | |
| X(t) | longitudinal particle position at time t |
| <X(t)> | longitudinal average trajectory |
| Y(t) | transverse particle position at time t |
References
- Weiss, J.B.; Provenzale, A. Transport and Mixing in Geophysical Flows; Springer: Berlin, Germany, 2008. [Google Scholar] [CrossRef]
- De Vincenzo, A.; Molino, B.; Viparelli, R.; Caramuscio, P. A methodological approach for estimating turbidity in a river. Int. J. Sediment Res. 2011, 26, 112–119. [Google Scholar] [CrossRef]
- Pannone, M. On the exact analytical solution for the spatial moments of the cross-sectional average concentration in open channel flows. Water Resour. Res. 2012, 48, W08511. [Google Scholar] [CrossRef]
- Pannone, M. Transient Hydrodynamic Dispersion in Rough Open Channels: Theoretical Analysis of Bed-Form Effects. J. Hydraul. Eng. 2010, 136, 155–164. [Google Scholar] [CrossRef]
- Pannone, M. Longitudinal Dispersion in River Flows Characterized by Random Large-Scale Bed Irregularities: First-Order Analytical Solution. J. Hydraul. Eng. 2012, 138, 400–411. [Google Scholar] [CrossRef]
- Pannone, M.; De Vincenzo, A.; Brancati, F. A Mathematical Model for the Flow Resistance and the Related Hydrodynamic Dispersion Induced by River Dunes. J. Appl. Math. 2013, 2013, 432610. [Google Scholar] [CrossRef]
- Pannone, M. Predictability of tracer dilution in large open channel flows: Analytical solution for the coefficient of variation of the depth-averaged concentration. Water Resour. Res. 2014, 50, 2617–2635. [Google Scholar] [CrossRef]
- Pannone, M.; De Vincenzo, A. Stochastic numerical analysis of anomalous longitudinal dispersion and dilution in shallow decelerating stream flows. Stoch. Environ. Res. Risk Assess. 2015, 29, 2087–2100. [Google Scholar] [CrossRef]
- Pannone, M. Effect of nonlocal transverse mixing on river flows dispersion: A numerical study. Water Resour. Res. 2010, 46, W08534. [Google Scholar] [CrossRef]
- De Barros, F.; Dentz, M.; Koch, J.; Nowak, W. Flow topology and scalar mixing in spatially heterogeneous flow fields. Geophys. Res. Lett. 2012, 39, L08404. [Google Scholar] [CrossRef]
- Shannon, C.E. A Mathematical Theory of Communications. Bell Syst. Tech. J. 1948, 27, 379–423. [Google Scholar] [CrossRef]
- Greco, M.; Mirauda, D.; Plantamura Volpe, A. Manning’s roughness through the entropy parameter for steady open channel flows in low submergence. Procedia Eng. 2014, 70, 773–780. [Google Scholar] [CrossRef]
- Greco, M.; Mirauda, D. An Entropy Based Velocity Profile for Steady Flows with Large-Scale Roughness. In Engineering Geology for Society and Territory, River Basins, Reservoir Sedimentation and Water Resources; Lollino, G., Arattano, M., Rinaldi, M., Giustolisi, O., Marechal, J.C., Grant, G.E., Eds.; Springer: Cham, Switzerland, 2015; Volume 3, pp. 641–645. ISBN 978-3-319-09054-2. [Google Scholar]
- Greco, M.; Mirauda, D. Entropy parameter estimation in large-scale roughness open channel. J. Hydrol. Eng. 2015, 20, 04014047-1–04014047-6. [Google Scholar] [CrossRef]
- Mirauda, D.; De Vincenzo, A.; Pannone, M. Simplified entropic model for the evaluation of suspended load concentration. Water 2018, 10, 378. [Google Scholar] [CrossRef]
- Mirauda, D.; De Vincenzo, A.; Pannone, M. Statistical characterization of flow field structure in evolving braided gravel beds. Spat. Stat. 2017. [Google Scholar] [CrossRef]
- Mirauda, D.; Pannone, M.; De Vincenzo, A. An entropic model for the assessment of stream-wise velocity dip in wide open channels. Entropy 2018, 20, 69. [Google Scholar] [CrossRef]
- Mirauda, D.; Ostoich, M. Surface water vulnerability assessment applying the integrity model as a decision support system for quality improvement. Environ. Impact Assess. Rev. 2011, 31, 161–171. [Google Scholar] [CrossRef]
- Taylor, G.I. The dispersion of matter in turbulent flow through a pipe. Proc. R. Soc. A 1954, 223, 446–468. [Google Scholar] [CrossRef]
- Fischer, H.B. The mechanics of dispersion in natural streams. J. Sanit. Eng. Div. Proc. Am. Soc. Civ. Eng. 1967, 93, 187–216. [Google Scholar]
- Fischer, H.B. Methods for predicting dispersion coefficient in natural streams, with applications to lower reaches of the Green and Duwamish Rivers. U.S. Geol. Surv. Prof. Pap. 1968, 582. Available online: https://pubs.usgs.gov/pp/0582a/report.pdf (accessed on 7 April 2018).
- Rutheford, J.C.; Taylor, M.E.U.; Davies, J.D. Waikato River pollutant flushing rates. J. Environ. Eng. 1980, 106, 1131–1150. [Google Scholar]
- Davis, P.M.; Atkinson, T.C. Longitudinal dispersion in natural channels: 1, Experimental results from the River Severn. Hydrol. Earth Syst. Sci. 2000, 4, 345–353. [Google Scholar]
- Davis, P.M.; Atkinson, T.C. Longitudinal dispersion in natural channels: 2, The roles of shear flow dispersion and dead zones in the River Severn. Hydrol. Earth Syst. Sci. 2000, 4, 355–371. [Google Scholar] [CrossRef]
- Mason, J.R.; Wallis, S.G.; Hope, D. A conservative semi-Lagrangian transport model for rivers with transient storage zones. Water Resour. Res. 2001, 37, 3321–3329. [Google Scholar] [CrossRef]
- Lambertz, P.; Palancar, M.C.; Aragon, L.M.; Gil, R. Determining the dispersion characteristics of rivers from the frequency response of the system. Water Resour. Res. 2006, 42, W09414. [Google Scholar] [CrossRef]
- Marion, A.; Zaramella, M.; Bottacin-Busolin, A. Solute transport in rivers with multiple storage zones: The STIR model. Water Resour. Res. 2008, 44, W10406. [Google Scholar] [CrossRef]
- Ahmad, Z. Mixing length for establishment of longitudinal dispersion in streams. Int. J. Modell. Simul. Acta Press 2004, 29, 127–136. [Google Scholar] [CrossRef]
- Banks, R.B. A mixing cell model for longitudinal dispersion in open channels. Water Resour. Res. 1974, 10, 357–358. [Google Scholar] [CrossRef]
- Fischer, H.B.; List, E.J.; Koh, R.C.Y.; Imberger, J.; Brooks, N.H. Mixing in Inland and Coastal Waters; Academic Press: New York, NY, USA, 1979; p. 483. [Google Scholar]
- Seo, I.W.; Cheong, T.S. Predicting longitudinal dispersion coefficient in natural streams. J. Hydraul. Eng. ASCE 1998, 124, 25–32. [Google Scholar] [CrossRef]
- Sayre, W.W.; Chang, F.M. A laboratory investigation of open-channel dispersion processes for dissolved, suspendend, and floating dispersants. U.S. Geol. Surv. Prof. Pap. 1968, 433. Available online: https://pubs.usgs.gov/pp/0433e/report.pdf (accessed on 7 April 2018).
- Batchelor, G.K. Diffusion in a field of homogeneous turbulence, 2: The relative motion of particles. Proc. Camb. Philos. Soc. 1952, 48, 345–362. [Google Scholar] [CrossRef]
- Deng, Z.; Singh, V.P.; Bengtsson, L. Longitudinal dispersion coefficient in straight rivers. J. Hydraul. Eng. 2001, 127, 919–927. [Google Scholar] [CrossRef]
- Gradshteyn, I.S.; Ryzhik, I.M. Table of Integrals, Series, and Products, 5th ed.; Academic Press Inc.: San Diego, CA, USA, 1994; p. 1204. ISBN 0-12-294755-X. [Google Scholar]
- Kapoor, V.; Gelhar, L.W. Transport in three-dimensionally heterogeneous aquifers: 1. Dynamics of concentration fluctuations. Water Resour. Res. 1994, 30, 1775–1788. [Google Scholar] [CrossRef]
- Kapoor, V.; Gelhar, L.W. Transport in three-dimensionally heterogeneous aquifers: 2. Predictions and observations of concentration fluctuations. Water Resour. Res. 1994, 30, 1789–1801. [Google Scholar] [CrossRef]
- Pannone, M.; Kitanidis, P.K. Large-time behavior of concentration variance and dilution in heterogeneous formations. Water Resour. Res. 1999, 35, 623–634. [Google Scholar] [CrossRef]
- Colosimo, C.; Copertino, V.A.; Veltri, M. La Resistenza Al Moto Nei Corsi D’acqua Naturali a Letto Fisso. Primi Risultati Di Una Ricerca Sperimentale; Atti del XXXIII “Convegno di Idraulica e Costruzioni Idrauliche”; University of Bologna: Bologna, Italy, 1982; pp. 101–115. (In Italian) [Google Scholar]

| River | B (m) | H (m) | U (m/s) | (m/s) | Pe |
|---|---|---|---|---|---|
| R1: Crati (CR) | 26.2 | 0.47 | 0.96 | 0.136 | 656 |
| R2: Lao (BR) | 24 | 0.45 | 1.46 | 0.2 | 649 |
| R3: Follone (T) | 13.5 | 0.38 | 0.92 | 0.136 | 400 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pannone, M.; Mirauda, D.; De Vincenzo, A.; Molino, B. Longitudinal Dispersion in Straight Open Channels: Anomalous Breakthrough Curves and First-Order Analytical Solution for the Depth-Averaged Concentration. Water 2018, 10, 478. https://doi.org/10.3390/w10040478
Pannone M, Mirauda D, De Vincenzo A, Molino B. Longitudinal Dispersion in Straight Open Channels: Anomalous Breakthrough Curves and First-Order Analytical Solution for the Depth-Averaged Concentration. Water. 2018; 10(4):478. https://doi.org/10.3390/w10040478
Chicago/Turabian StylePannone, Marilena, Domenica Mirauda, Annamaria De Vincenzo, and Bruno Molino. 2018. "Longitudinal Dispersion in Straight Open Channels: Anomalous Breakthrough Curves and First-Order Analytical Solution for the Depth-Averaged Concentration" Water 10, no. 4: 478. https://doi.org/10.3390/w10040478
APA StylePannone, M., Mirauda, D., De Vincenzo, A., & Molino, B. (2018). Longitudinal Dispersion in Straight Open Channels: Anomalous Breakthrough Curves and First-Order Analytical Solution for the Depth-Averaged Concentration. Water, 10(4), 478. https://doi.org/10.3390/w10040478





