4.1. Numerical Model
As evidenced by the experimental results, the X-ray fluence, measured at a given location, has a certain distribution caused by both varying luminosity of the photon bursts at the source and also by the possible non-isotropic beaming of photons. The energy spectrum of each spark itself could also vary, and the spectrum parameters are distributed in reality. In this study, because the distribution of photon energy is the primary concern, we have chosen to model only the energy spectrum of photons with unknown distribution parameters. The exclusion of distributions of fluence and detector efficiency from the numerical model makes it possible to conduct a more meaningful comparison of modelled results and experimental data, with fewer unknown parameters. This exclusion is further justified by the fact that, taking the average of the total detected energy of a series of sparks experimentally, the effect of those unknown distributions is implicitly included in the modelled result.
The experimental data provide the net total energy of the X-ray photons, after they penetrate copper shield of varying thickness and finally accumulate in the detector. In order to extract the photon energies from these data, it is necessary to determine the spectrum of X-ray photon energies that will satisfy the experimental data.
In almost all sparks where X-rays were detected, only a single X-ray burst was detected. Therefore, it is reasonable to assume that the energy detected by the detector is the accumulated energy of multiple photons absorbed by the detector within the rise-time of the detection system. If the spectrum and the number of photons are known at the origin of X-rays, then the total energy detected by the NaI(Tl) detector could be calculated for each data point in
Figure 2 by calculating the attenuation of X-ray photons along their path from the source to the detector and integrating the resultant photon intensity curve with respect to energy.
Let us consider the spark to be an isotropic point source of X-ray photons and the total number of photons at the source for a given energy bin
to be denoted by
(the differential photon energy intensity). Then, let the function
be the effective number of photons within the same energy bin at the source confined to a solid angle Ω (calculated using the distance from the detector to the source,
and the surface area of the detector,
). Thus,
can be calculated by multiplying
by the factor
as given in Equation (2). The function
defines both the spectrum and the fluence of X-rays in this case.
Thus, the total number of photons confined to the solid angle Ω having an energy from
to
at the source is given by the integration with respect to energy,
and the total energy of the photons at the source confined to the solid angle Ω is given by the integration,
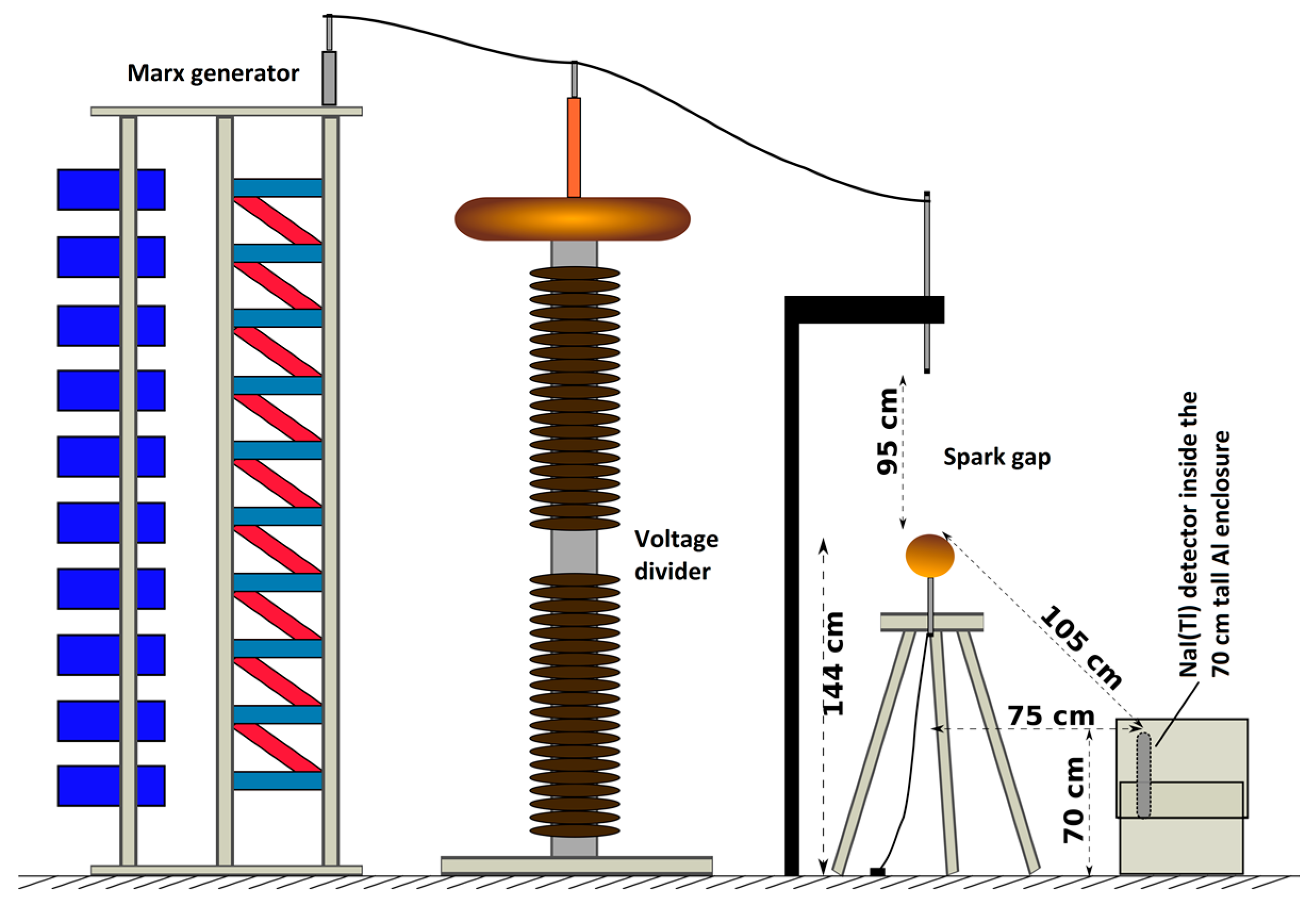
Now, in order to calculate the total detected energy by the NaI(Tl) X-ray detector, the attenuation of X-ray photons defined by function along their path from the origin to the detector surface should be considered. The attenuation from air (roughly 139 cm distance between the middle of the spark gap and aluminium box), aluminium enclosure, which is 3.1 mm thick, and the copper shield of varying thickness are considered in this case.
For a given intensity
, the attenuation of mono energetic photons due to a mass of a certain material with thickness
, density
and attenuation coefficient
is given by,
where
is the intensity after the attenuation, and the term
, which is dependent of the energy, is called the mass attenuation coefficient of the material considered for a given energy
E.
Combining Equations (4) and (5), we can derive the total detected energy by the detector assuming a 100% absorption efficiency,
which is equal to the integration of the attenuated spectrum multiplied by energy, with respect to energy. Additionally the integration of the attenuated result shown in Equation (7) gives the total number of photons detected by the detector.
The NaI(Tl) X-ray detector used in this experiment was equipped with a 3″ × 3″ (i.e., 76 mm diameter, 76 mm thick) solid scintillator crystal. According to the manufacturer, a scintillator having NaI(Tl) crystal of thickness 76 mm has a roughly 80% absorption efficiency for 1 MeV photons and almost 100% absorption efficiency for 500 keV photons. Since we do not expect MeV range photons from a discharge of applied voltage of 950 kV, it is a fair assumption that the detector has almost 100% absorption efficiency for the X-ray photons considered in this experiment. This makes it possible to directly compare the results from Equations (6) and (7) with experimental results.
The mass attenuation coefficients, , for air, copper and aluminium for varying X-ray energies could be obtained from the NIST X-ray mass attenuation coefficient database (NISTIR 5632). Because is energy dependant and given in tabular format, the integrations in Equations (6) and (7) could only be solved numerically (even when an analytical expression for is known).
As can be seen from Equation (7), depending on the solid angle Ω and the initial fluence of photons generated at the source within the energy range to , could also be zero (because in order to get the number of photons from the integration, the result should be rounded off to a whole number). This is also evident experimentally with sparks where no X-rays are detected.
Because the function governing the spectrum and number of photons at the source,
, is unknown, possible analytical expressions for X-ray spectrum for a laboratory spark should be considered. It is also evident that for a given analytical probability density function (or a differential intensity function)
governing the photon spectrum, the function
(which defines the total number of photons emitted at the source for a given energy) is simply the multiplication
by a constant of proportionality
.
In order to find an expression for the distribution of X-ray energy, one has to first find the distribution of electron energies involved in the bremsstrahlung process. Additionally, the bremsstrahlung differential cross-section relevant to the X-ray generation in electrical discharges in air for different ranges of electron energies should also be calculated. Using a combination of theoretical models for the generation of run-away electrons, Monte Carlo simulations of the electron energy and path, and considering the so-called Bethe-Heitler cross section for electron-nuclei bremsstrahlung, the photon fluence has been modelled previously in [
16]. But photon energy spectrum is still arbitrarily assumed to be exponential in this study.
A similar approach is used in this study. A probable energy spectrum for photons is assumed first. Then, using attenuation of the photons, energy deposited at the X-ray detectors is calculated numerically. This result is then compared with experimental data. Two such possible spectrums for photons are considered in this study. The first is a bremsstrahlung spectrum, in its simplest and most crude form of Kramers’ law with an unknown maximum energy, assuming X-rays are primarily produced by the bremsstrahlung process between electrons and nitrogen atoms. The second is an exponential photon distribution with an unknown mean energy.
For both cases, the unknown parameters, the number of photons at the source and at the detector, the total energy produced at the source and the total energy absorbed by the detector could be calculated by defining the arbitrary function with analytical expressions from selected spectrums. In addition to these probable photon distributions, an extreme case where a burst of mono energetic photons is also considered for comparison purposes.
4.2. Simulation and Results with Kramers’ Law
The selection of Kramer’s law as the rule governing the X-ray spectrum is justified not because of any theoretical or experimental evidence of its validity but simply because it provides a rough approximation of a X-ray photon distribution for a bremsstrahlung process of a nearly mono-energetic electron beam hitting a target. In other words, it is reasonable to take this crude approach because the distribution of electrons themselves is not yet known. On the other hand, as can be seen later from Equation (11), the distribution of X-ray energies with this approach tends to follow a distribution of the order which is not significantly different from the assumptions made in previous studies. One can argue that, since electrons themselves have an energy distribution, the superposition of Kramers’ distributions for different electron energies would produce a more accurate photon distribution. But this approach is not considered because as mentioned earlier, Kramers’ rule is used somewhat arbitrarily in this case and superposition is deemed unnecessary.
In this case, we also assume the bremsstrahlung process happens in the spark gap itself but not at the electrodes. Thus, we consider the bremsstrahlung process between electron and nitrogen atoms primarily. The differential intensity I of the bremsstrahlung X-rays photons at any given energy
in the spectrum is given by Kramer’s Law as
where
Z is the mean atomic number (in this case for nitrogen), K is a constant of proportionality,
E is the energy of generated photons and
is the energy of the mono energetic electron beam and also the maximum energy of X-ray photons. The intensity is zero when
(the Duane-Hunt limit) but approaches infinity (
) as
approaches zero.
Now,
for the case of Kramers’ law,
, can be written using Equations (2), (8) and (9) as
By merging all constants together, above expression can be re written as
where the parameter
is the product of the geometric distribution factor
and other two constants
and
. By substituting
for
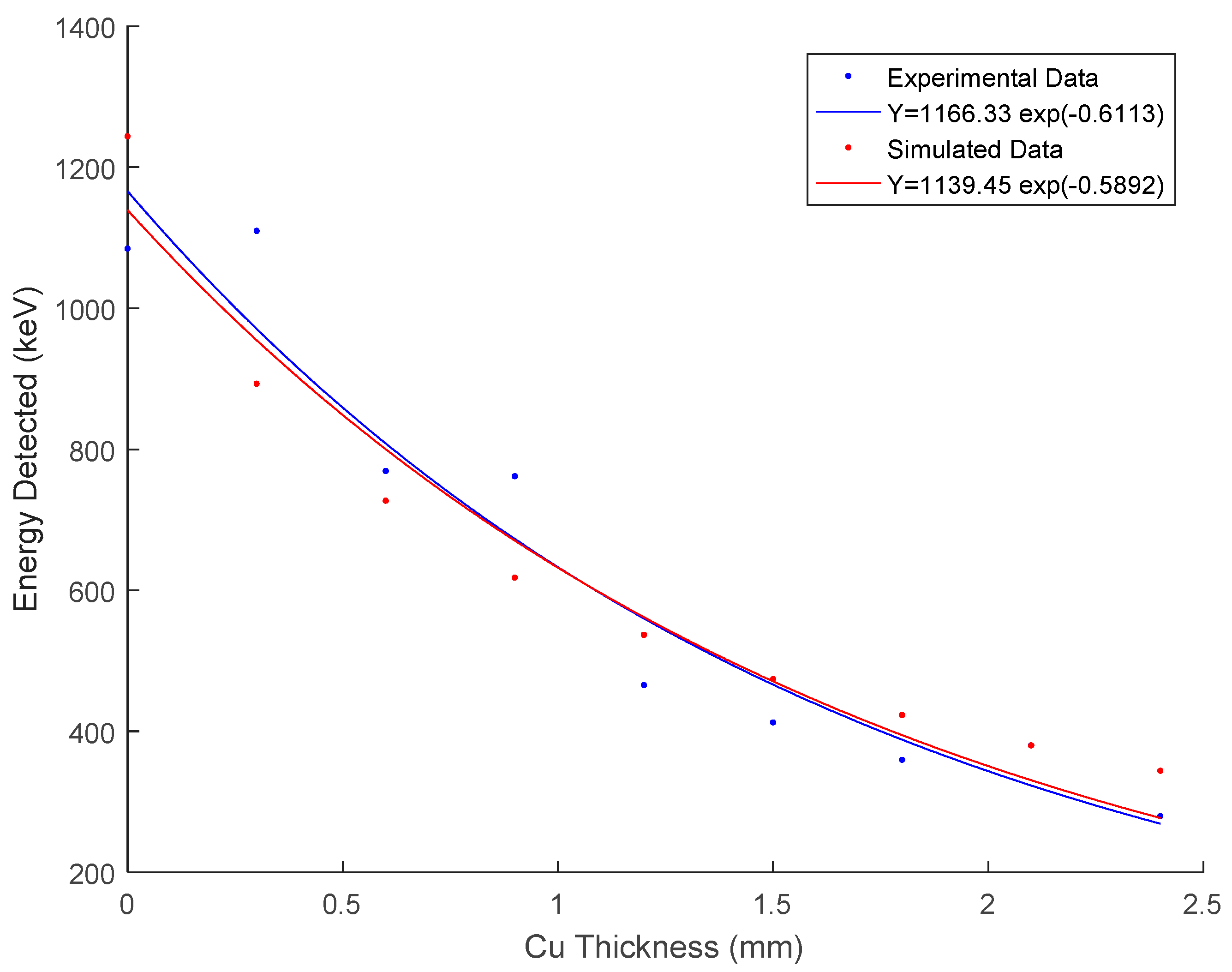
in (6), a simulated best fit curve of detected energy vs the coper thickness similar to the experimental results as shown in
Figure 2 can be generated for two unknown parameters
and
. By comparing this curve with the experimental curve shown in
Figure 2, the best possible combination of
and
is obtained.
During this process all the numerical integrations are calculated using trapezoidal rule with an energy step size of 0.1 keV. The minimum energy necessary to calculate the integration in (6) is considered to be 5 keV, which is derived from the maximum sensitivity of the detector system. But in reality, any value of minimum energy up to about 15 keV would give the same results, because air and aluminium box along the photon path produce a lower cut-off energy around 15 keV. The mass attenuation coefficients , for each energy step are calculated by interpolating the tables obtained from NISTIR 5632 database for different materials.
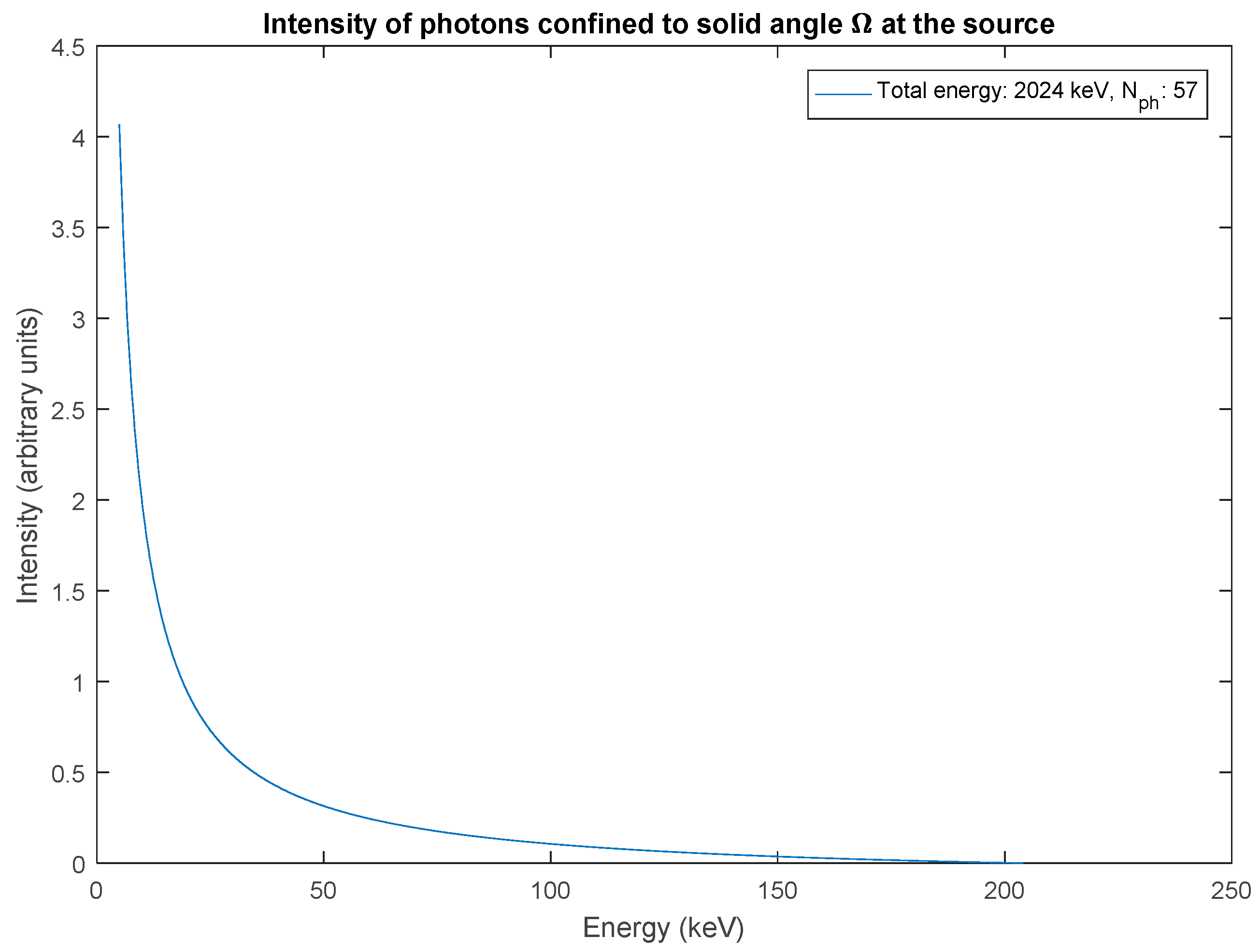
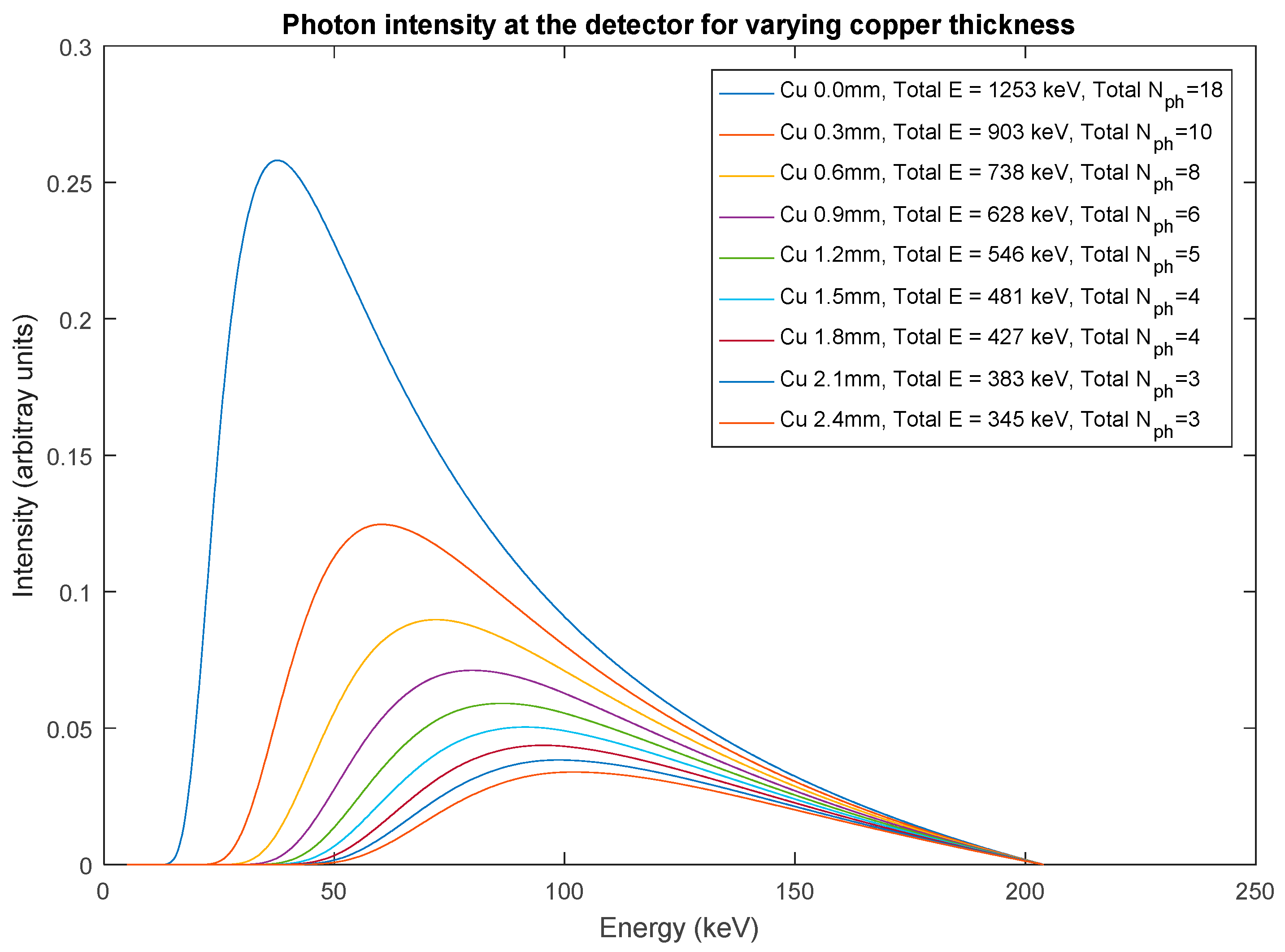
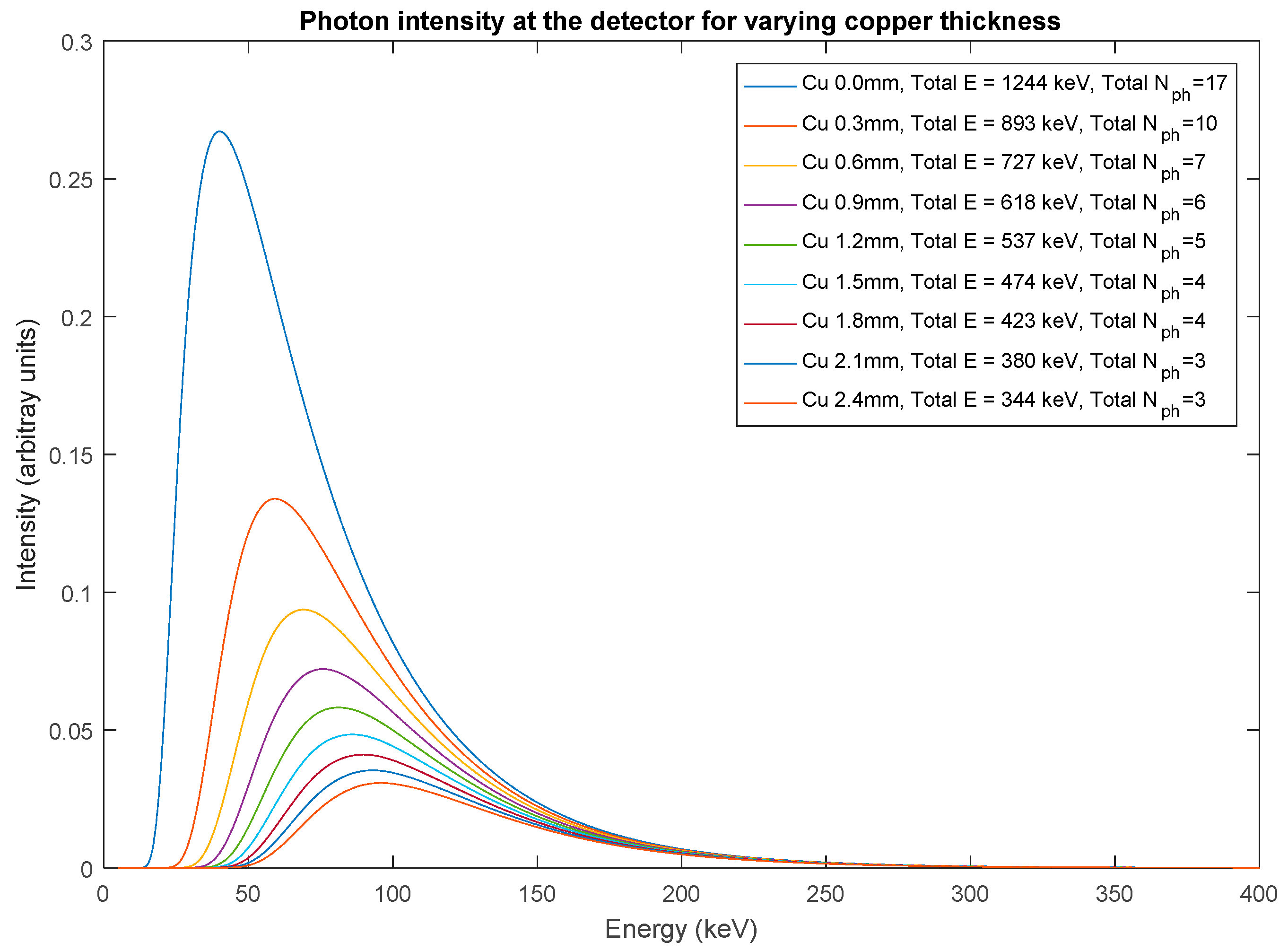
It is interesting to observe the attenuation of the photons along their path from the source to the detector, since the parameters
and
are now known. This gives a picture of how the number of photons confined to Ω decreases along their path from source to the detector. The source photon intensity confined to Ω,
, is shown in
Figure 3. It is also evident from Equation (7) that the term,
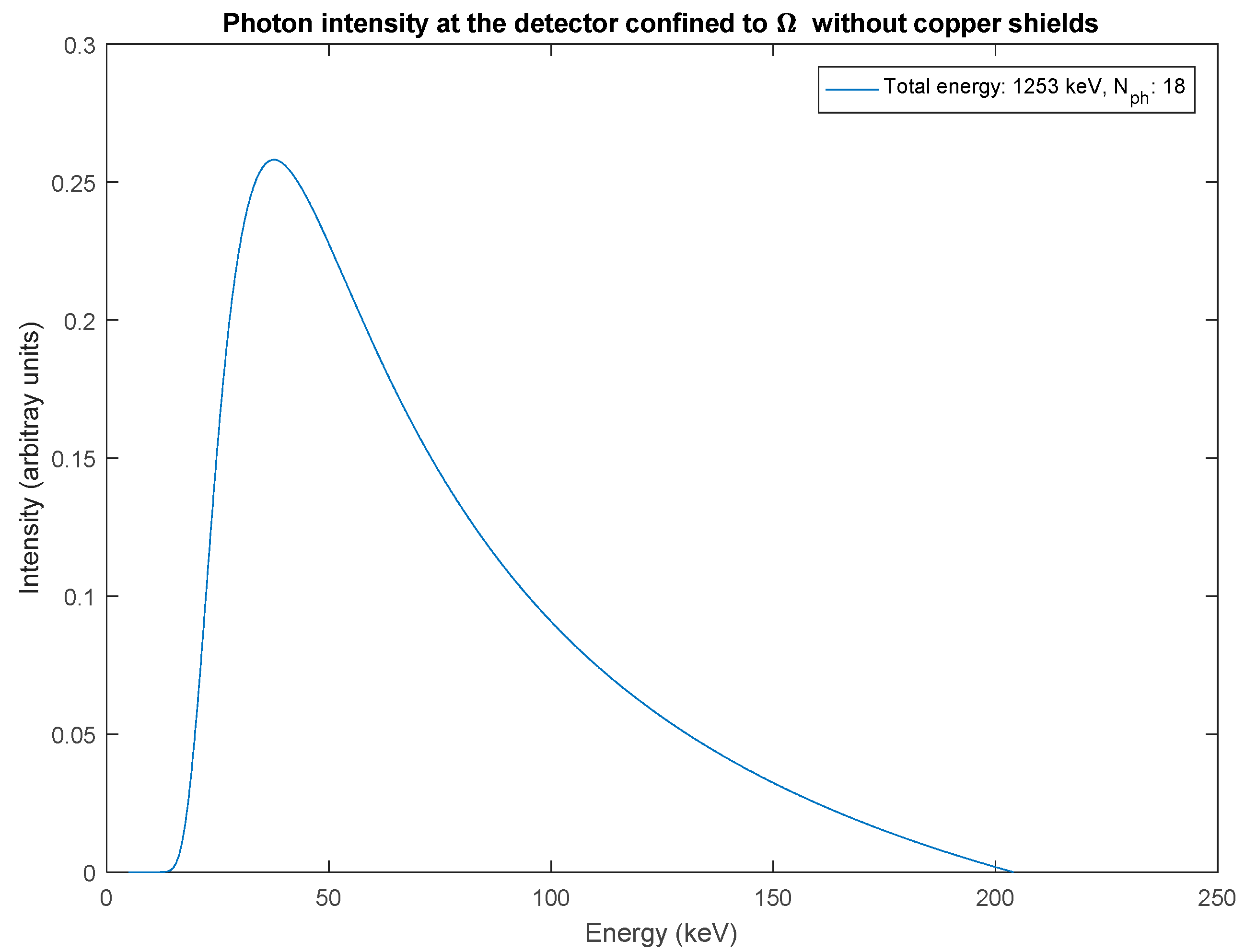
gives the attenuated intensity of photons due to different media along the path of photons. This attenuated intensity of photons along their path is shown in
Figure 4 and
Figure 5 considering the attenuation of air, aluminium and copper. The total number of photons depicted in these figures is calculated by rounding off the integrated results to a whole number. The 15 keV lower cut-off energy due to attenuation is visible in
Figure 4.
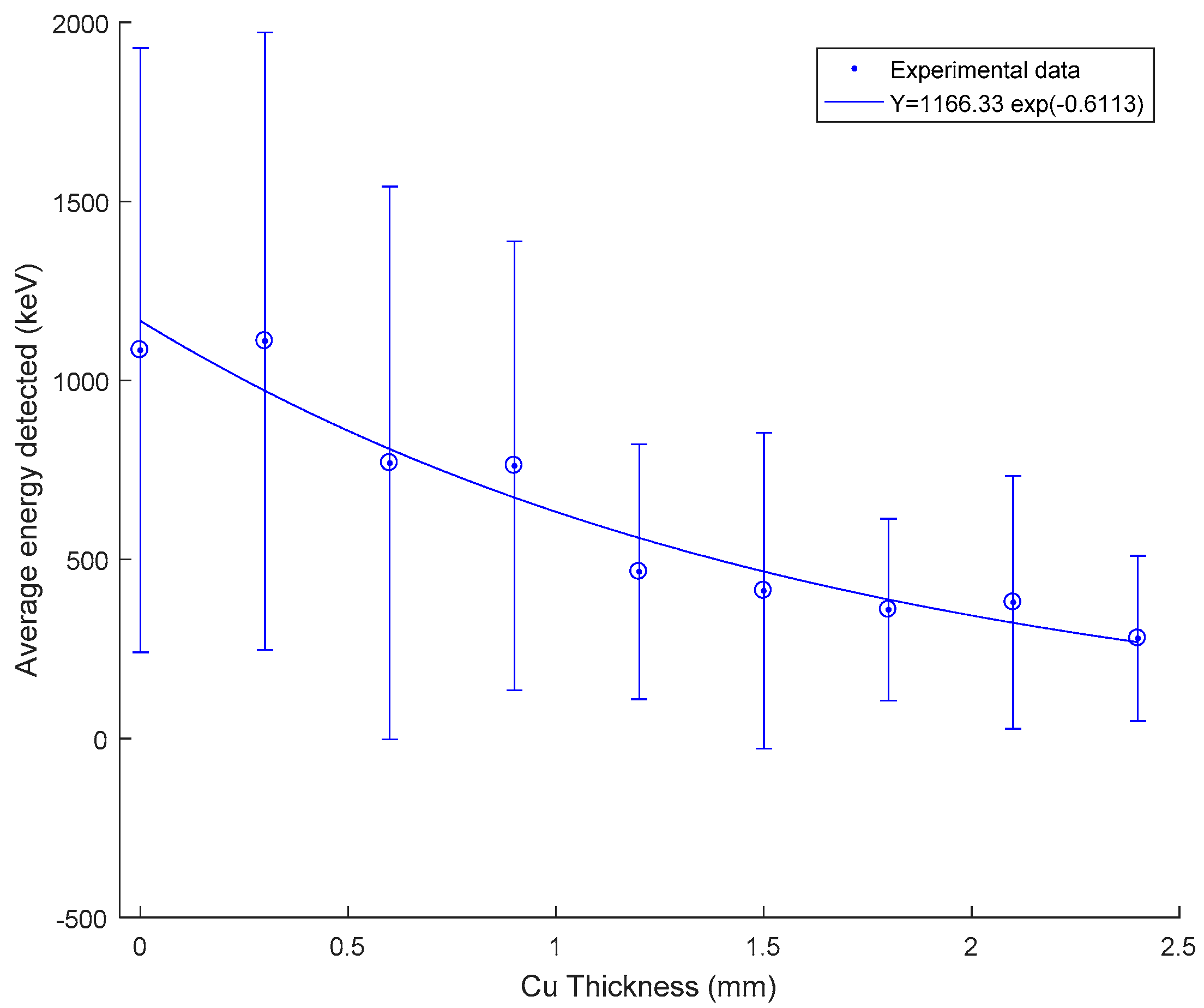
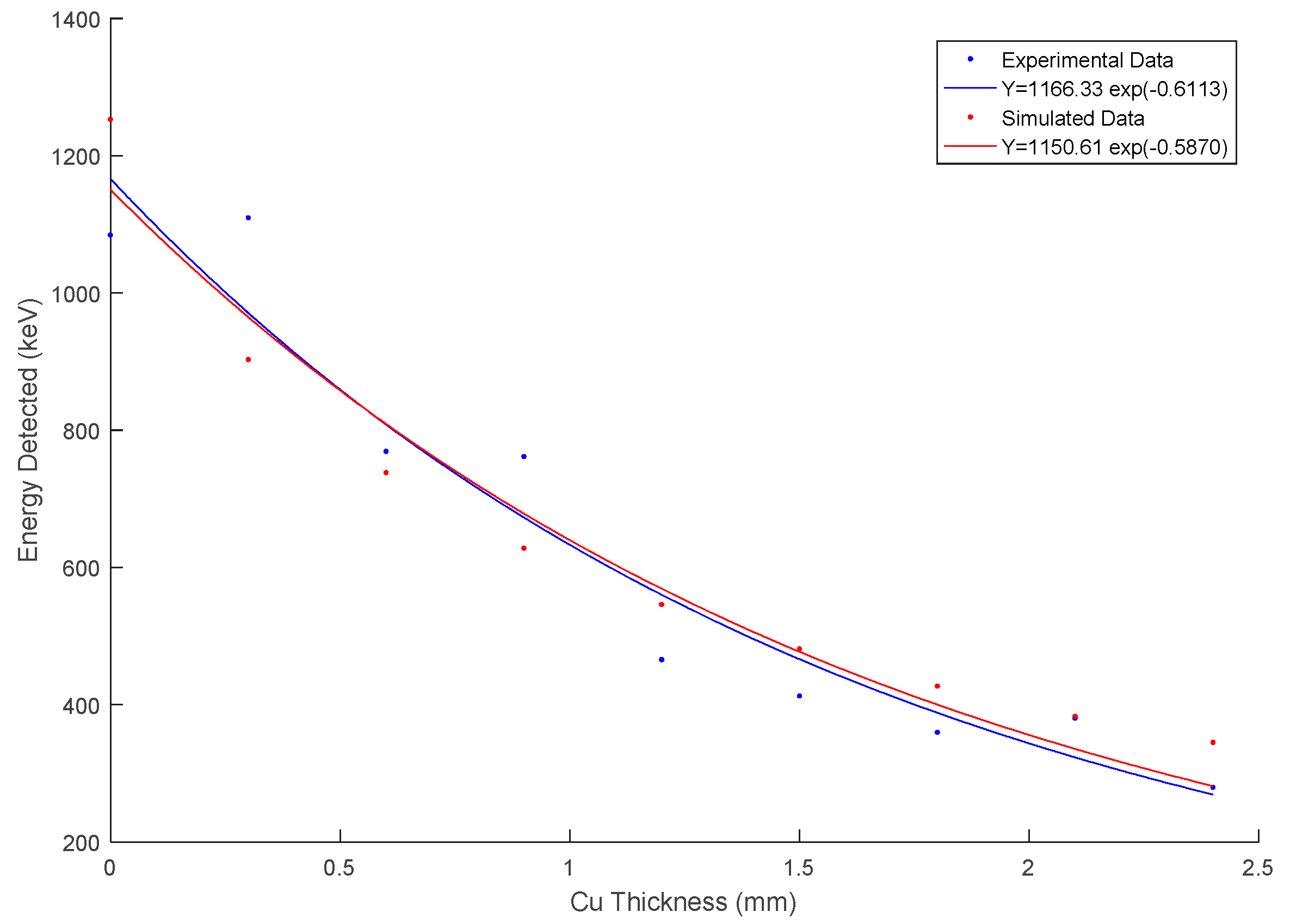
The final simulated results, assuming 100% efficient detection, and the experimental results are shown in
Figure 6. We find a good agreement with the experimental data with
and
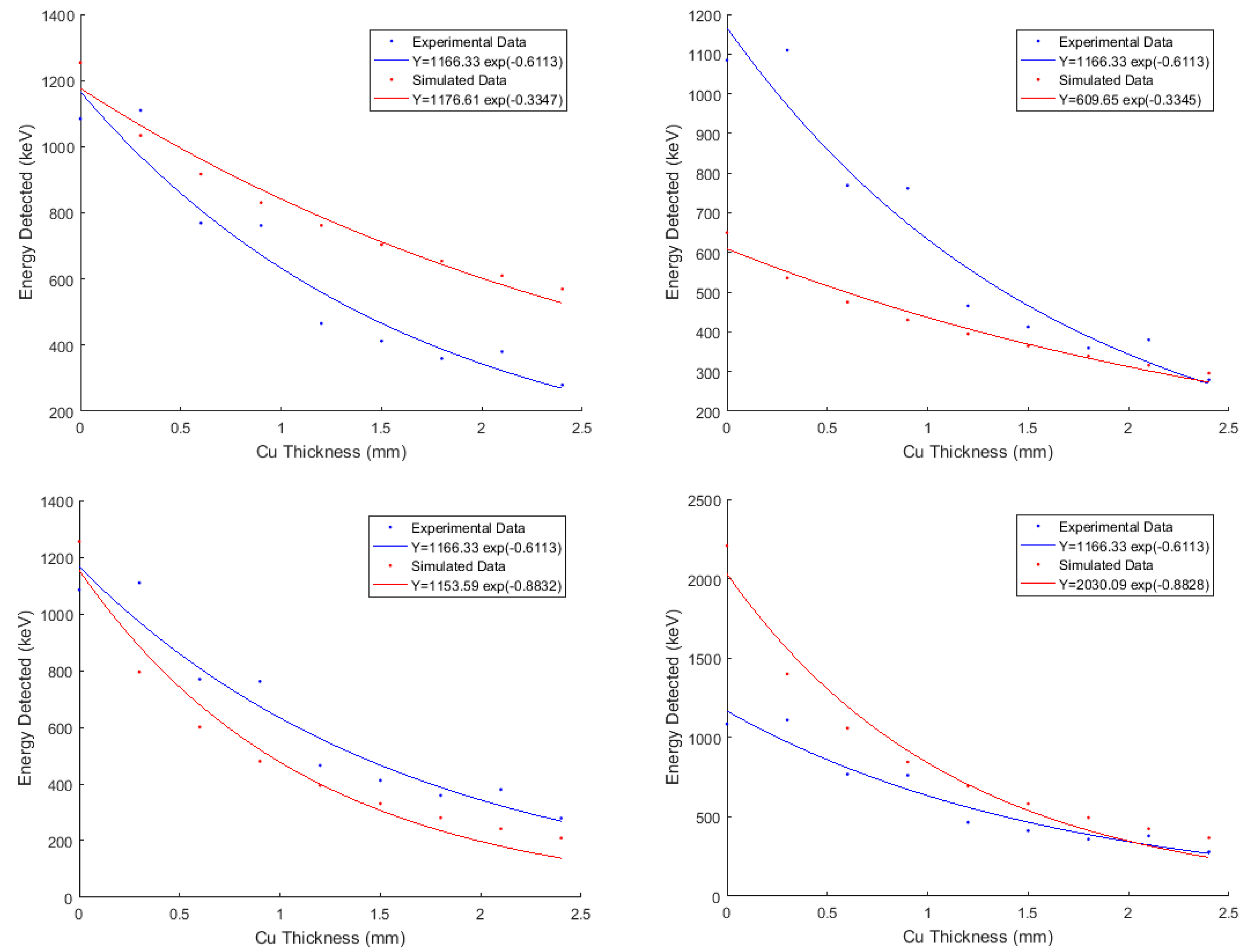
. However, before making any conclusions regarding the X-ray photon energies, it is necessary to determine the sensitivity of the results obtained for the changes to these two parameters. The result of a simple sensitivity analysis of the parameters relative to the simulated results is shown in
Figure 7. Based on this analysis, it is concluded that the fits of the simulated and experimental results match only for the unique set of parameter values
and
.
The parameter
, which is inherently an expression of number of photons, tends to influence the above plot in
Figure 6 linearly, while the maximum energy
has a greater influence on higher coper thickness of the plot. This is understandable because in order to penetrate thicker shields, photons with greater energies are required, and even a large number of low energetic photons cannot penetrate a thicker shield.
On the other hand, this result is also dependent on the shape of the generated spectrum. Even though there are modifications derived to the basic Kramers’ law for electron metal bremsstrahlung based on both experiments and theoretical models yielding accurate spectrums, such expressions for laboratory discharges where bremsstrahlung happens between electrons and air molecules and atoms (nitrogen and oxygen primarily) could not be found. If a bremsstrahlung spectrum, valid for X-ray production from discharges in air, is used instead of the basic Kramer’s law which is applied in the above simulation, the maximum energy would be different from the simulated value of 204 keV.