Long-Range Transport of SO2 from Continental Asia to Northeast Asia and the Northwest Pacific Ocean: Flow Rate Estimation Using OMI Data, Surface in Situ Data, and the HYSPLIT Model
Abstract
:1. Introduction
2. Method
2.1. Data
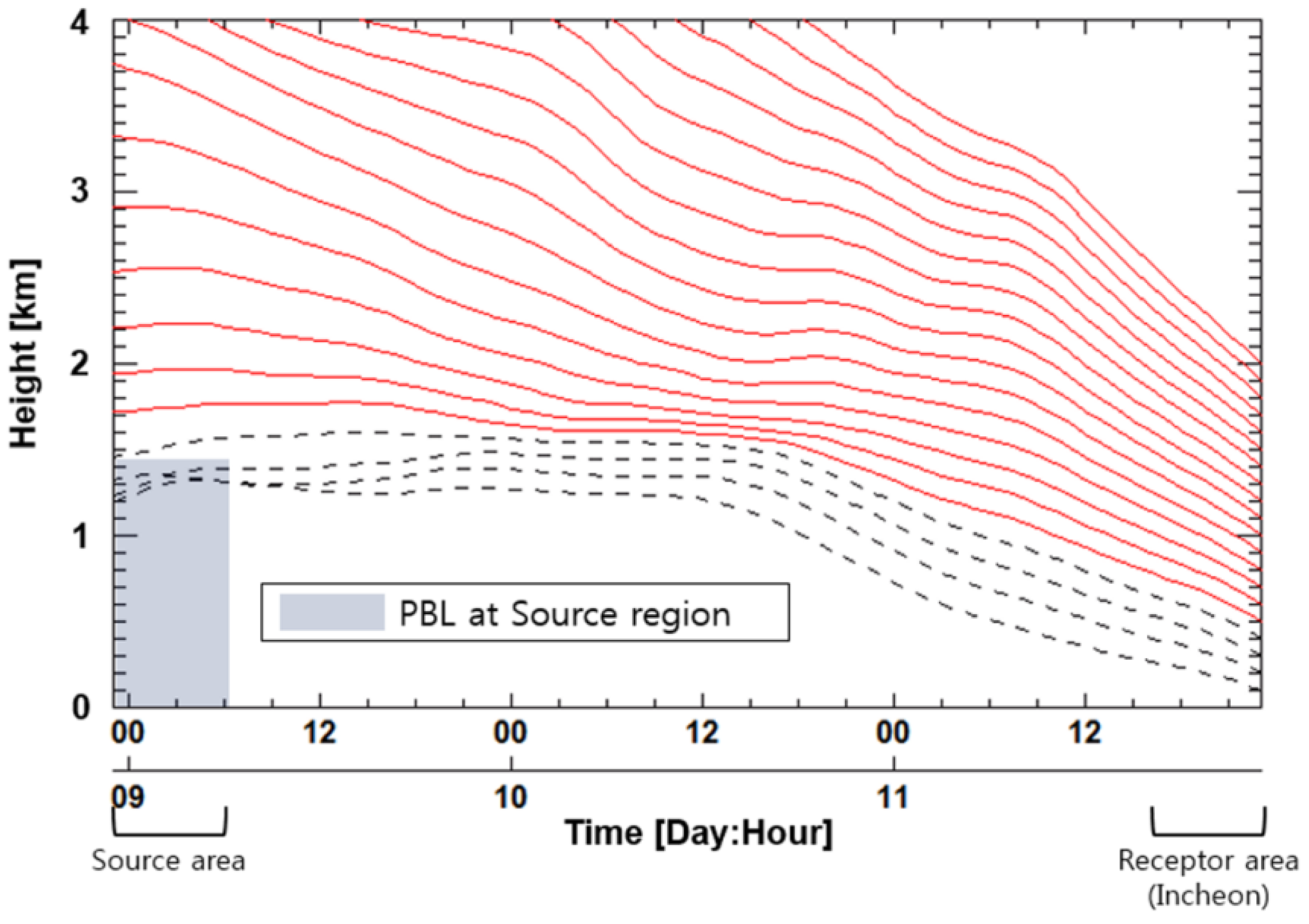
2.2. HYSPLIT Backward Trajectory Simulation
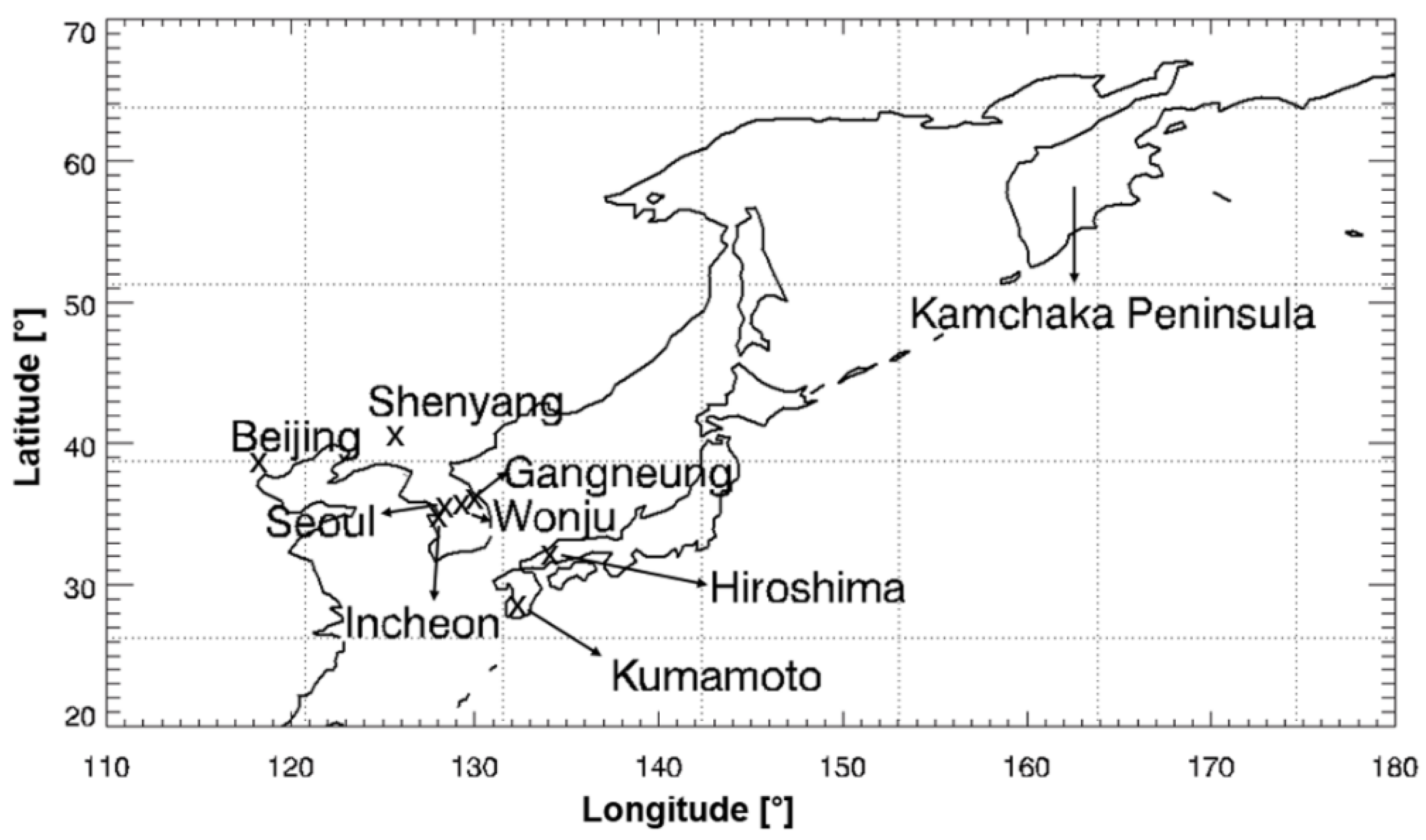
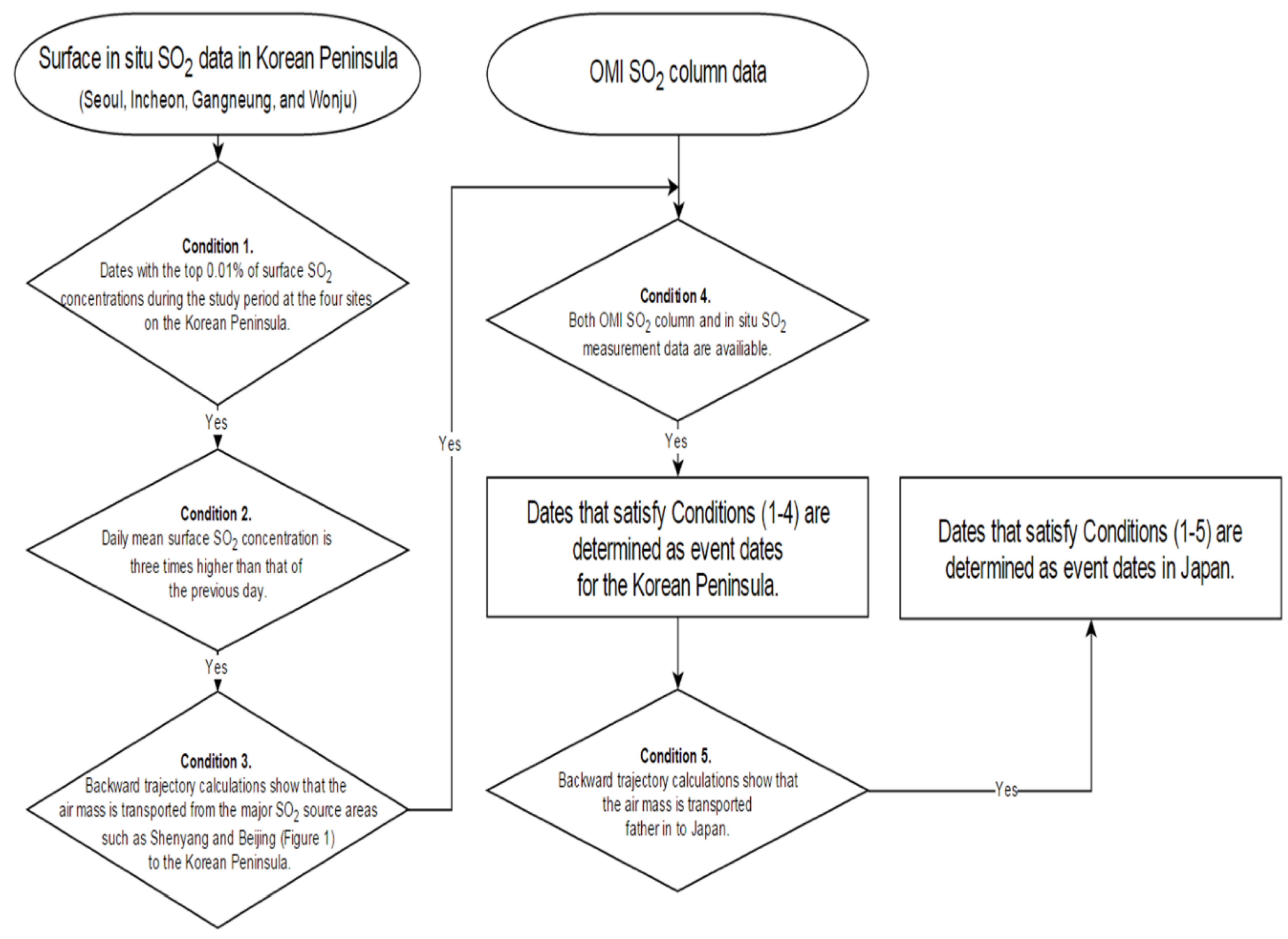
2.3. Study Locations and Dates
- •
- Condition 1. Dates with the top 0.01% of surface SO2 concentrations during the study period at the four sites on the Korean Peninsula.
- •
- Condition 2. Daily mean surface SO2 concentration is three times higher than that of the previous day.
- •
- Condition 3. Backward trajectory calculations show that the air mass is transported from the major SO2 source areas such as Shenyang and Beijing (Figure 1) to the Korean Peninsula.
- •
- Condition 4. Both OMI SO2 column and in situ SO2 measurement data are available. Dates that satisfy Conditions (1–4) are determined as event dates for the Korean Peninsula.
- •
- Condition 5. Backward trajectory calculations show that the air mass is transported farther into Japan. Dates that satisfy Conditions (1–5) are determined as event dates in Japan.
3. Flow Rate Calculation
3.1. Calculation of α
3.2. Calculation of β
4. Results
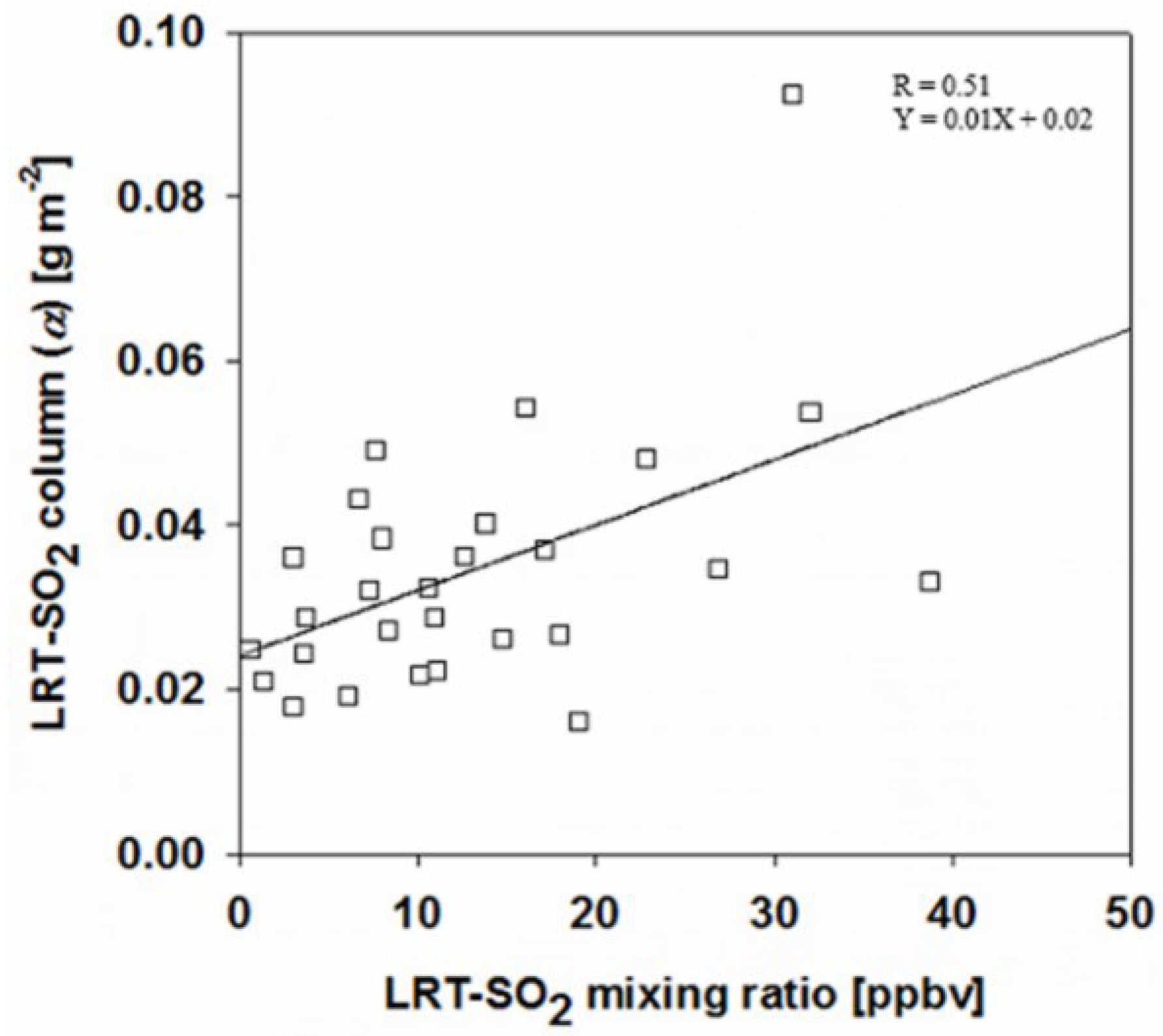
4.1. LRT-SO2 Flow Rates
4.2. Error Estimation
5. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
Abbreviations
| OMI | Ozone Monitoring Instrument |
| MAX-DOAS | Multi AXis Differential Optical Absorption Spectroscopy |
| CPSCF | Conditional Potential Source Contribution Function |
| GEO | GEostationary Orbit |
| LEO | Low Earth Orbit |
| TOA | Top Of the Atmosphere |
| Agl | Above ground level |
| CALIPSO | Cloud-Aerosol Lidar and Infrared Pathfinder Satellite Observations |
| SCIAMACHY | SCanning Imaging Absorption SpectroMeter for Atmospheric CHartographY |
| HYSPLIT | HYbrid Single Particle Lagrangian Integrated Trajectory |
| MODIS | MODerate resolution Imaging Spectroradiometer |
| MISR | Multi-angle Imaging SpectroRadiometer |
References
- Weiss, K.; Gergen, P.J.; Wagener, D.K. Breathing better or wheezing worse? The changing epidemiolgy of asthma morbidity and mortality. Annu. Rev. Public Health 1993, 14, 491–513. [Google Scholar] [CrossRef] [PubMed]
- Dockery, D.W.; Cunningham, J.; Damokosh, A.I.; Neas, L.M.; Spengler, J.D.; Koutrakis, P.; Ware, J.H.; Raizenne, M.; Speizer, F.E. Health effects of acid aerosols on North American children: Respiratory symptoms. Environ. Health Perspect. 1996, 104, 500–505. [Google Scholar] [CrossRef] [PubMed]
- Sunyer, J.; Spix, C.; Quenel, P.; Ponce-de-Leon, A.; Pönka, A.; Barumandzadeh, T.; Touloumi, G.; Bacharova, L.; Wojtyniak, B.; Vonk, J.; et al. Urban air pollution and emergency admissions for asthma in four European cities: The aphea project. Thorax 1997, 52, 760–765. [Google Scholar] [CrossRef] [PubMed]
- Thompson, A.J.; Shields, M.D.; Patterson, C.C. Acute asthma exacerbations and air pollutants in children living in belfast, Northern Ireland. Arch. Environ. Health Int. J. 2001, 56, 234–241. [Google Scholar] [CrossRef] [PubMed]
- Seinfeld, J.H.; Pandis, S.N. Atmospheric Chemistry and Physics; Wiley-Interscience: Hoboken, NJ, USA, 2006. [Google Scholar]
- Whelpdale, D.M.; Watch, G.A.; Kaiser, M. Global Acid Deposition Assessment; World Meteorological Organization, Global Atmosphere Watch: Geneva, Switzerland, 1996. [Google Scholar]
- Vestreng, V.; Myhre, G.; Fagerli, H.; Reis, S.; Tarrasón, L. Twenty-five years of continuous sulphur dioxide emission reduction in Europe. Atmos. Chem. Phys. 2007, 7, 3663–3681. [Google Scholar] [CrossRef]
- Lu, Z.; Streets, D.G.; Zhang, Q.; Wang, S.; Carmichael, G.R.; Cheng, Y.F.; Wei, C.; Chin, M.; Diehl, T.; Tan, Q. Sulfur dioxide emissions in China and sulfur trends in East Asia since 2000. Atmos. Chem. Phys. 2010, 10, 6311–6331. [Google Scholar] [CrossRef]
- Jiang, J.; Zha, Y.; Gao, J.; Jiang, J. Monitoring of SO2 column concentration change over China from Aura OMI data. Int. J. Remote Sens. 2012, 33, 1934–1942. [Google Scholar] [CrossRef]
- Krotkov, N.; McLinden, C.; Li, C.; Lamsal, L.; Celarier, E.; Marchenko, S.; Swartz, W.; Bucsela, E.; Joiner, J.; Duncan, B.; et al. Aura OMI observations of regional SO2 and NO2 pollution changes from 2005 to 2014. Atmos. Chem. Phys. Discuss. 2015, 15, 26555–26607. [Google Scholar] [CrossRef]
- Kurokawa, J.; Ohara, T.; Morikawa, T.; Hanayama, S.; Janssens-Maenhout, G.; Fukui, T.; Kawashima, K.; Akimoto, H. Emissions of air pollutants and greenhouse gases over Asian regions during 2000–2008: Regional emission inventory in Asia (REAS) version 2. Atmos. Chem. Phys. 2013, 13, 11019–11058. [Google Scholar] [CrossRef]
- Park, R.J.; Jacob, D.J.; Field, B.D.; Yantosca, R.M.; Chin, M. Natural and transboundary pollution influences on sulfate-nitrate-ammonium aerosols in the United States: Implications for policy. J. Geophys. Res. Atmos. 2004, 109. [Google Scholar] [CrossRef]
- Heald, C.L.; Jacob, D.J.; Park, R.J.; Alexander, B.; Fairlie, T.D.; Yantosca, R.M.; Chu, D.A. Transpacific transport of Asian anthropogenic aerosols and its impact on surface air quality in the United States. J. Geophys. Res. Atmos. 2006, 111. [Google Scholar] [CrossRef]
- Donkelaar, A.V.; Martin, R.V.; Leaitch, W.R.; Macdonald, A.; Walker, T.; Streets, D.G.; Zhang, Q.; Dunlea, E.J.; Jimenez, J.L.; Dibb, J.E.; et al. Analysis of aircraft and satellite measurements from the intercontinental chemical transport experiment (INTEX-B) to quantify long-range transport of East Asian sulfur to Canada. Atmos. Chem. Phys. 2008, 8, 2999–3014. [Google Scholar] [CrossRef]
- Haywood, J.; Shine, K. The effect of anthropogenic sulfate and soot aerosol on the clear sky planetary radiation budget. Geophys. Res. Lett. 1995, 22, 603–606. [Google Scholar] [CrossRef]
- Lee, C.; Richter, A.; Lee, H.; Kim, Y.J.; Burrows, J.P.; Lee, Y.G.; Choi, B.C. Impact of transport of sulfur dioxide from the Asian continent on the air quality over Korea during may 2005. Atmos. Environ. 2008, 42, 1461–1475. [Google Scholar] [CrossRef]
- Dickerson, R.; Li, C.; Li, Z.; Marufu, L.; Stehr, J.; McClure, B.; Krotkov, N.; Chen, H.; Wang, P.; Xia, X.; et al. Aircraft observations of dust and pollutants over Northeast China: Insight into the meteorological mechanisms of transport. J. Geophys. Res. Atmos. 2007, 112. [Google Scholar] [CrossRef]
- Jeong, U.; Lee, H.; Kim, J.; Kim, W.; Hong, H.; Song, C.K. Determination of the inter-annual and spatial characteristics of the contribution of long-range transport to SO2 levels in Seoul between 2001 and 2010 based on conditional potential source contribution function (CPSCF). Atmos. Environ. 2013, 70, 307–317. [Google Scholar] [CrossRef]
- Hsu, N.C.; Li, C.; Krotkov, N.A.; Liang, Q.; Yang, K.; Tsay, S.C. Rapid transpacific transport in autumn observed by the A-TRAIN satellites. J. Geophys. Res. Atmos. 2012, 117. [Google Scholar] [CrossRef]
- Mallik, C.; Lal, S.; Naja, M.; Chand, D.; Venkataramani, S.; Joshi, H.; Pant, P. Enhanced SO2 concentrations observed over Northern India: Role of long-range transport. Int. J. Remote Sens. 2013, 34, 2749–2762. [Google Scholar] [CrossRef]
- Li, C.; Krotkov, N.A.; Dickerson, R.R.; Li, Z.; Yang, K.; Chin, M. Transport and evolution of a pollution plume from Northern China: A satellite-based case study. J. Geophys. Res. Atmos. 2010, 115. [Google Scholar] [CrossRef]
- Levelt, P.F.; Van den Oord, G.H.; Dobber, M.R.; Malkki, A.; Visser, H.; De Vries, J.; Stammes, P.; Lundell, J.O.; Saari, H. The ozone monitoring instrument. IEEE Trans. Geosci. Remote Sens. 2006, 44, 1093–1101. [Google Scholar] [CrossRef]
- Krotkov, N.; Yang, K.; Carn, S.; Krueger, A. OMSO2 README File Date: 2/26/2008; NASA: Greenbelt, MD, USA, 2006.
- Krotkov, N.A.; Carn, S.A.; Krueger, A.J.; Bhartia, P.K.; Yang, K. Band residual difference algorithm for retrieval of SO2 from the Aura ozone monitoring instrument (OMI). IEEE Trans. Geosci. Remote Sens. 2006, 44, 1259–1266. [Google Scholar] [CrossRef]
- Goddard Earth Sciences Data and Information Services Center (GES-DISC). Available online: http://disc.sci.gsfc.nasa.gov/Aura/data-holdings/OMI/omso2e_v003.html (accessed on 6 April 2016).
- Li, C.; Joiner, J.; Krotkov, N.A.; Bhartia, P.K. A fast and sensitive new satellite SO2 retrieval algorithm based on principal component analysis: Application to the ozone monitoring instrument. Geophys. Res. Lett. 2013, 40, 6314–6318. [Google Scholar] [CrossRef]
- Ozone Monitoring Instrument Data User’s Guide. Available online: http://disc.sci.gsfc.nasa.gov/Aura/additional/documentation/README.OMI_DUG.pdf (accessed on 6 April 2016).
- Goddard Earth Sciences Data and Information Services Center (GES-DISC). Available online: http://disc.sci.gsfc.nasa.gov/Aura/data-holdings/OMI/index.shtml#info (accessed on 6 April 2016).
- Draxler, R.R.; Hess, G. Description of the HYSPLIT4 Modeling System; NOAA Air Resources Laboratory: Silver Spring, MD, USA, 1997.
- Stohl, A. Computation, accuracy and applications of trajectories—A review and bibliography. Atmos. Environ. 1998, 32, 947–966. [Google Scholar] [CrossRef]
- Gebhart, K.A.; Schichtel, B.A.; Barna, M.G. Directional biases in back trajectories caused by model and input data. J. Air Waste Manag. Assoc. 2005, 55, 1649–1662. [Google Scholar] [CrossRef] [PubMed]
- Von Engeln, A.; Teixeira, J. A planetary boundary layer height climatology derived from ECMWF reanalysis data. J. Clim. 2013, 26, 6575–6590. [Google Scholar] [CrossRef]
- Zhang, X.; Geffen, J.; Zhang, P.; Wang, J. Trend spatial & temporal distribution, and sources of the tropospheric SO2 over China based on satellite measurement during 2004–2009. Proc. Dragon 2010, 2, 2008–2010. [Google Scholar]
- Lee, H.-L.; Kim, J.-H.; Ryu, J.-Y.; Kwon, S.-C.; Noh, Y.-M.; Gu, M.-J. 2-Dimensional mapping of sulfur dioxide and bromine oxide at the sakurajima volcano with a ground based scanning imaging spectrograph system. J. Opt. Soc. Korea 2010, 14, 204–208. [Google Scholar] [CrossRef]
- Lee, C.; Kim, Y.J.; Tanimoto, H.; Bobrowski, N.; Platt, U.; Mori, T.; Yamamoto, K.; Hong, C.S. High CLO and ozone depletion observed in the plume of sakurajima volcano, Japan. Geophys. Res. Lett. 2005, 32. [Google Scholar] [CrossRef]
- Bobrowski, N.; Hönninger, G.; Galle, B.; Platt, U. Detection of bromine monoxide in a volcanic plume. Nature 2003, 423, 273–276. [Google Scholar] [CrossRef] [PubMed]
- Bobrowski, N.; Von Glasow, R.; Aiuppa, A.; Inguaggiato, S.; Louban, I.; Ibrahim, O.; Platt, U. Reactive halogen chemistry in volcanic plumes. J. Geophys. Res. Atmos. 2007, 112. [Google Scholar] [CrossRef]
- Clifford, A. Multivariate Error Analysis: A Handbook of Error Propagation and Calculation in Many-Parameter System; Applied Science Publishers Ltd.: London, UK, 1973. [Google Scholar]
- Lee, C.; Martin, R.V.; van Donkelaar, A.; O’Byrne, G.; Krotkov, N.; Richter, A.; Huey, L.G.; Holloway, J.S. Retrieval of vertical columns of sulfur dioxide from SCIAMACHY and OMI: Air mass factor algorithm development, validation, and error analysis. J. Geophys. Res. Atmos. 2009, 114, D22303. [Google Scholar] [CrossRef]



| Event | Date | Receptor Area |
|---|---|---|
| E1 | 23 January 2005 | Seoul, Wonju |
| 24 January 2005 | Seoul, Wonju | |
| E2 | 11 February 2006 | Seoul, Incheon |
| E3 | 22 December 2006 | Seoul, Incheon, Wonju |
| 23 December 2006 | Seoul, Incheon, Wonju | |
| E4 | 13 March 2007 | Seoul, Incheon |
| 14 March 2007 | Seoul, Incheon | |
| 15 March 2007 | Seoul, Incheon | |
| E5 | 7 November 2007 | Seoul, Wonju |
| 8 November 2007 | Seoul, Wonju | |
| E6 | 11 March 2008 | Seoul, Wonju |
| E7 | 6 January 2008 | Incheon, Gangneung |
| 7 January 2008 | Incheon, Gangneung | |
| E8 | 22 December 2006 | Shenyang |
| 23 December 2006 | Gangneung | |
| 24 December 2006 | Hiroshima | |
| E9 | 21 December 2006 | North Yellow Sea |
| 22 December 2006 | Seoul | |
| 23 December 2006 | Kumamoto | |
| E10 | 6 October 2008 | Hokkaido |
| 7 October 2008 | Northwest Pacific Ocean (Aleutian islands) | |
| E11 | 9 October 2006 | Vladivostok |
| 10 October 2006 | Magadan | |
| 11 October 2006 | Northwest Pacific Ocean (Bering Sea) |
| Event | α a, α b, α c, α d (g·m−2) | β a, β b, β c, β d (m·s−1) | Flow Rate (Error) (Mg·h−1 OMI·grid−1) | |
|---|---|---|---|---|
| E1 | 23 | 0.036 a, 0.019 d | 3.0 a, 3.0 d | 9.7 a (±2.5), 5.1 d (±1.4) |
| 24 | 0.038 a, 0.022 d | 5.1 a, 4.7 d | 17.4 a (±4.7), 9.3 d (±2.5) | |
| E2 | 11 | 0.049 a, 0.020 b | 6.3 a, 6.3 b | 27.8 a (±7.5), 11.3 b (±3.1) |
| E3 | 22 | 0.036 a, 0.031 b, 0.054 d | 7.3 a, 7.2 b, 6.8 d | 23.7 a (±6.3), 20.0 b (±5.3), 33.4 d (±8.7) |
| 23 | 0.024 a, 0.024 b,0.092 d | 4.1 a, 4.1 b, 3.8 d | 8.9 a (±2.4), 8.9 b (±2.4), 31.5 d (±8.3) | |
| E4 | 13 | 0.040 a, 0.027 b | 4.3 a, 4.1 b | 15.5 a (±4.1), 10.0 b (±2.7) |
| 14 | 0.048 a, 0.040 b | 1.1 a, 1.1 b | 4.8 a (±1.3), 4.0 b (±1.1) | |
| 15 | 0.034 a, 0.025 b | 4.6 a, 4.8 b | 14.1 a (±3.8), 10.8 b (±3.0) | |
| E5 | 7 | 0.029 a, 0.018 d | 3.3 a, 3.8 d | 8.6 a (±2.2), 6.2 d (±1.5) |
| 8 | 0.027 a, 0.016 d | 1.5 a, 2.0 d | 3.6 a (±1.0), 2.9 d (±0.8) | |
| E6 | 11 | 0.033 a, 0.053 d | 3.7 a, 2.4 d | 11.0 a (±2.9), 11.4 d (±3.0) |
| E7 | 6 | 0.030 b, 0.021 c | 1.5 b, 2.7 c | 4.1 b (±1.1), 5.1 c (±1.4) |
| 7 | 0.026 b, 0.035 c | 7.8 b, 8.6 c | 18.3 b (±4.8), 27.1 c (±7.0) | |
| E8 | 22 | 0.035 | 7.2 | 22.7 (±7.4) |
| 23 | 0.033 | 4.7 | 14.0 (±3.7) | |
| 24 | 0.013 | 3.6 | 4.2 (±1.2) | |
| E9 | 21 | 0.047 | 5.8 | 24.5 (±7.5) |
| 22 | 0.032 | 7.3 | 21.0 (±5.2) | |
| 23 | 0.010 | 5.9 | 5.3 (±1.4) | |
| E10 | 6 | 0.028 | 6.4 | 16.1 (±4.5) |
| 7 | 0.019 | 3.3 | 5.6 (±1.6) | |
| E11 | 9 | 0.034 | 5.3 | 16.2 (±4.4) |
| 10 | 0.020 | 5.0 | 9.0 (±2.4) | |
| 11 | 0.025 | 7.4 | 16.7 (±4.6) | |
© 2016 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons by Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Park, J.; Ryu, J.; Kim, D.; Yeo, J.; Lee, H. Long-Range Transport of SO2 from Continental Asia to Northeast Asia and the Northwest Pacific Ocean: Flow Rate Estimation Using OMI Data, Surface in Situ Data, and the HYSPLIT Model. Atmosphere 2016, 7, 53. https://doi.org/10.3390/atmos7040053
Park J, Ryu J, Kim D, Yeo J, Lee H. Long-Range Transport of SO2 from Continental Asia to Northeast Asia and the Northwest Pacific Ocean: Flow Rate Estimation Using OMI Data, Surface in Situ Data, and the HYSPLIT Model. Atmosphere. 2016; 7(4):53. https://doi.org/10.3390/atmos7040053
Chicago/Turabian StylePark, Junsung, Jaeyong Ryu, Daewon Kim, Jaeho Yeo, and Hanlim Lee. 2016. "Long-Range Transport of SO2 from Continental Asia to Northeast Asia and the Northwest Pacific Ocean: Flow Rate Estimation Using OMI Data, Surface in Situ Data, and the HYSPLIT Model" Atmosphere 7, no. 4: 53. https://doi.org/10.3390/atmos7040053
APA StylePark, J., Ryu, J., Kim, D., Yeo, J., & Lee, H. (2016). Long-Range Transport of SO2 from Continental Asia to Northeast Asia and the Northwest Pacific Ocean: Flow Rate Estimation Using OMI Data, Surface in Situ Data, and the HYSPLIT Model. Atmosphere, 7(4), 53. https://doi.org/10.3390/atmos7040053








