Joint Inversion of Atmospheric Refractivity Profile Based on Ground-Based GPS Phase Delay and Propagation Loss
Abstract
:1. Introduction
2. Forward Model and Parameterization Schemes
2.1. Forward Model-Excess Phase Delay

2.2. Forward Model-Propagation Loss
2.3. Four-Parameter Model
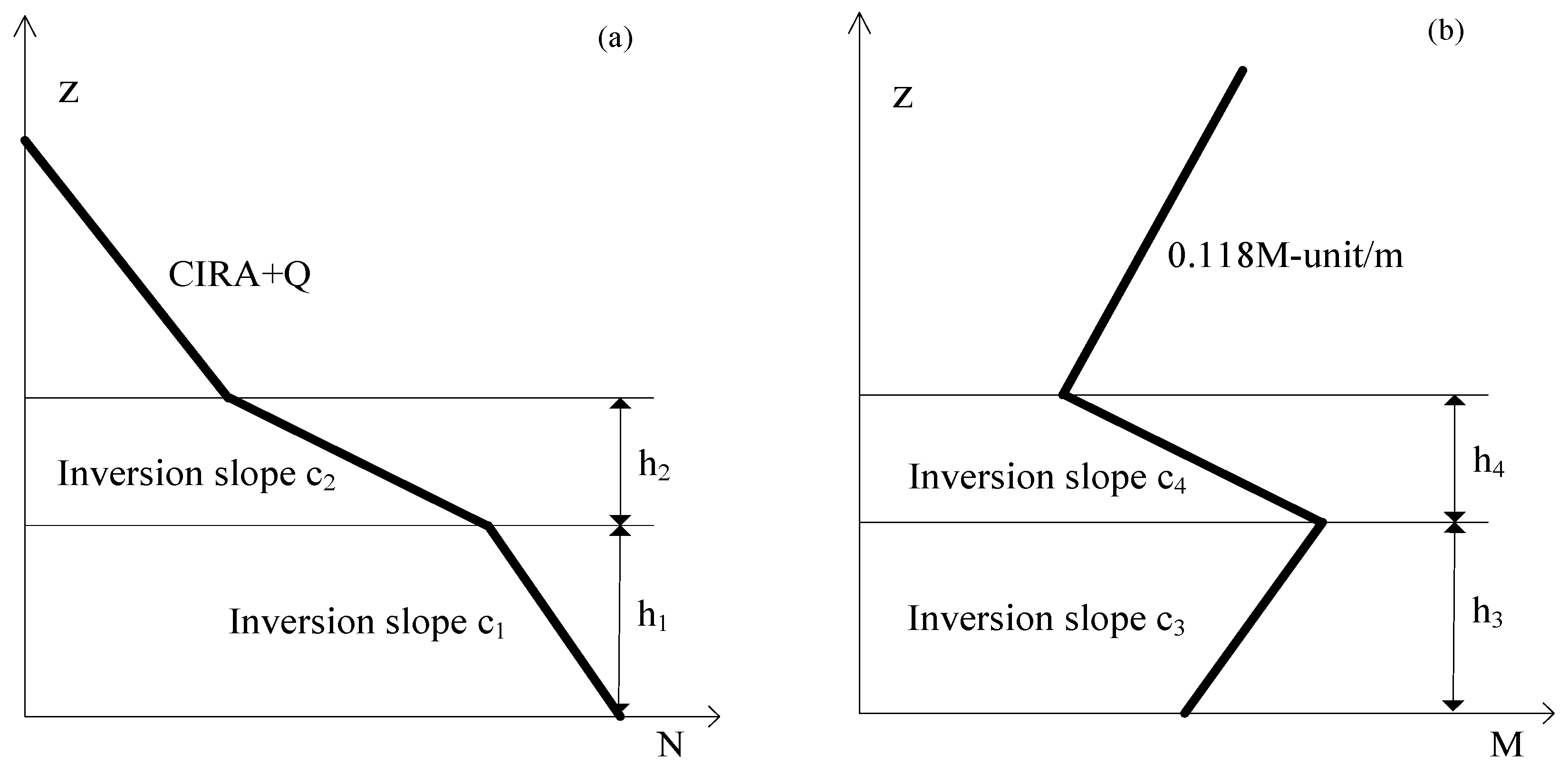
3. Inversion Algorithm
4. Results
4.1. Ideal Condition
| Parameters | Inversion Slope c1 | Height h1 | Inversion Slope c2 | Height h2 | |
|---|---|---|---|---|---|
| Units | N-units/m | m | N-units/m | m | |
| Lower Bound | −0.1 | 50 | −0.4 | 250 | |
| Upper Bound | 0 | 150 | 0 | 350 | |
| True Value | −0.02 | 100 | −0.2 | 300 | |
| NSGA-II | 0% | −0.0227 (13.5%) | 99.8246 (−1.75%) | −0.199 (−0.5%) | 298.7839 (−0.4%) |
| 3% | −0.0155 (−22.5%) | 95.9541 (−4.04%) | −0.1990 (−0.5%) | 306.0764 (2.03%) | |
| 5% | −0.0121 (−39.5%) | 94.7610 (−5.239%) | −0.2008 (0.4%) | 306.0295 (2.01%) | |
| 7% | −0.0254 (27%) | 98.9927 (−1.007%) | −0.2007 (0.35%) | 300.4304 (0.143%) | |
| 10% | −0.0146 (−27%) | 89.1687 (−10.83%) | −0.1959 (−2.05%) | 302.2124 (0.74%) | |
| GA-Delay | 0% | −0.0576 (188%) | 109.5935 (9.59%) | −0.1969 (−1.55%) | 281.8778 (−6.04%) |
| 3% | −0.0519 (159.50%) | 91.2888 (−8.71%) | −0.1723 (−13.85%) | 332.9521 (10.98%) | |
| 5% | −0.0361 (80.50%) | 115.5426 (15.54%) | −0.1865 (−6.75%) | 305.7128 (1.90%) | |
| 7% | −0.0985 (392.50%) | 140.5176 (40.52%) | −0.1665 (−16.75%) | 328.5602 (9.52%) | |
| 10% | −0.0618 (209.00%) | 124.9692 (24.97%) | −0.1693 (−15.35%) | 273.2624 (−8.91%) | |
| GA-Loss | 0% | −0.0208 (4%) | 96.0873 (−3.91%) | −0.1948 (−2.6%) | 309.6933 (3.23%) |
| 3% | −0.0181 (−9.50%) | 102.2168 (2.22%) | −0.2050 (2.50%) | 296.1529 (−1.28%) | |
| 5% | −0.0198 (−1.00%) | 93.3431 (−6.66%) | −0.1897 (−5.15%) | 312.0424 (4.01%) | |
| 7% | −0.01934 (−3.30%) | 97.9463 (−2.054%) | −0.1905 (−4.75%) | 307.5467 (2.52%) | |
| 10% | −0.0293 (46.50%) | 113.9005 (13.90%) | −0.2106 (5.30%) | 262.6215 (−12.46%) | |

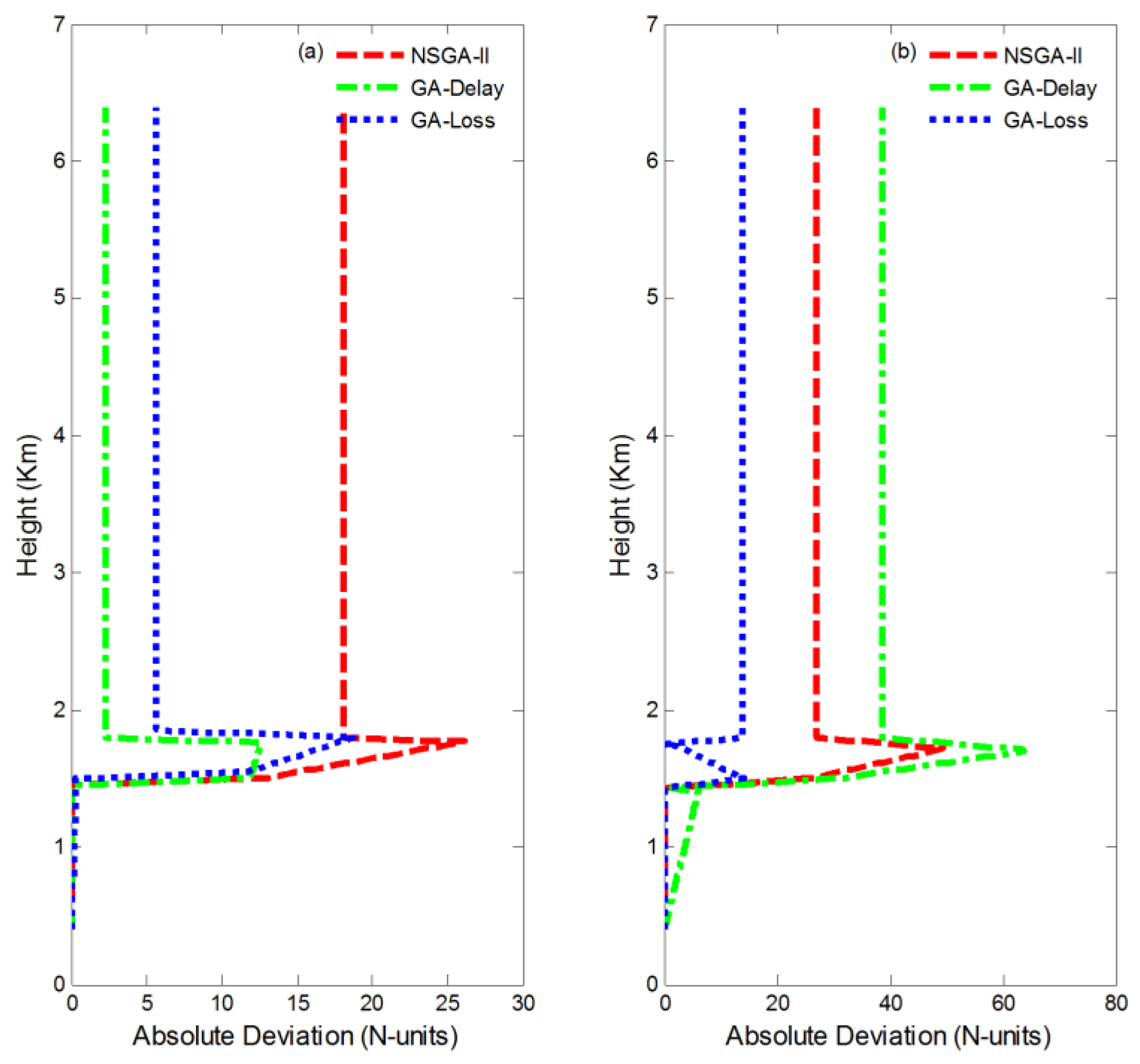
4.2. Adding Gaussian Noise
4.3. Real Data Testing
| Parameters | Inversion Slope c1 | Height h1 | Inversion Slope c2 | Height h2 |
|---|---|---|---|---|
| Units | N-units/m | m | N-units/m | m |
| NSGA-II | −0.0281 | 105.0418 | −0.2013 | 293.8846 |
| GA-Delay | −0.0212 | 102.3759 | −0.2313 | 304.9078 |
| GA-Loss | −0.0374 | 110.5404 | −0.2187 | 298.8363 |

4.4. Feasibility
| Parameters | Inversion Slope c1 | Height h1 | Inversion Slope c2 | Height h2 | |
|---|---|---|---|---|---|
| Units | N-units/m | m | N-units/m | m | |
| True Value | −0.05 | 1100 | −0.3 | 300 | |
| 400 m | NSGA-II | −0.0501 (0.20%) | 1058.2 (−3.80%) | −0.35 (16.67%) | 312.6988 (4.23%) |
| GA-Delay | −0.05 (0.0%) | 1053.1 (−4.26%) | −0.3029 (0.97%) | 309.7630 (3.25%) | |
| GA-Loss | −0.0503 (0.60%) | 1148.4 (4.40%) | −0.2723 (−9.23%) | 304.0592 (1.35%) | |
| 600 m | NSGA-II | −0.05 (0.0%) | 1028.82 (−6.40%) | -0.41 (36.70%) | 289.8588 (−3.38%) |
| GA-Delay | −0.044 (-12.00%) | 1012.1 (−7.99%) | -0.4659 (−55.30%) | 293.7786 (−2.07%) | |
| GA-Loss | −0.05 (0.0%) | 1029.8588 (−6.38%) | -0.2508 (−16.40%) | 311.7714 (3.92%) | |
5. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
Abbreviations
| GA: | The traditional general genetic algorithm |
| GA-Delay: | Retrieval from phase delay by GA |
| GA-Loss: | Retrieval from propagation loss by GA |
References
- Zhao, X. Source localization in the duct environment with the adjoint of the PE propagation model. Atmosphere 2015, 6, 1388–1398. [Google Scholar] [CrossRef]
- Zhang, J.P.; Wu, Z.S.; Zhao, Z.W.; Zhang, Y.S.; Wang, B. Propagation modeling of ocean-scattered low-elevation GPS signal for marine tropospheric duct inversion. Chin. Phys. B 2012, 21. [Google Scholar] [CrossRef]
- Krolik, J.L.; Tabrikian, J. Tropospheric refractivity estimation using radar clutter from the sea surface. SPAWAR Sys. Command. Tech. Rep. 1997, 2989, 635–642. [Google Scholar]
- Gerstoft, P.; Rogers, L.T.; Krolik, J.L.; Hodgkiss, W.S. Inversion for refractivity parameters from radar sea clutter. Radio Sci. 2003, 38. [Google Scholar] [CrossRef]
- Sheng, Z. The estimation of lower refractivity uncertainty from radar sea clutter using the Bayesian-MCMC method. Chin. Phys. B 2013, 22. [Google Scholar] [CrossRef]
- Douvenot, R.; Fabbro, V.; Gerstoft, P.; Bourlier, C.; Saillard, J. A duct mapping method using least squares support vector machines. Radio Sci. 2008, 43. [Google Scholar] [CrossRef]
- Wen, D.B.; Yuan, Y.B.; Ou, J.K.; Zhang, K. Ionospheric response to the geomagnetic storm on 21 Augus 2003 over China using GNSS-based tomographic technique. IEEE Trans. Geosci. Remote Sens. 2010, 48, 3212–3217. [Google Scholar]
- Yardim, C.; Gerstoft, P.; Hodgkiss, W.S. Tracking refractivity from clutter using Kalman and particle filters. IEEE Trans. Antennas Propag. 2008, 56, 1058–1070. [Google Scholar] [CrossRef]
- Sheng, Z.; Wang, J.; Zhou, S.; Zhou, B.H. Parameter estimation for chaotic systems using a hybrid adaptive cuckoo search with simulated annealing algorithm. Chaos 2014, 24. [Google Scholar] [CrossRef] [PubMed]
- Lowry, A.R.; Rocken, C.; Sokolovskiy, S.V.; Anderson, K.D. Vertical profiling of atmospheric refractivity from ground-based GPS. Radio Sci. 2002, 37. [Google Scholar] [CrossRef]
- Sheng, Z.; Fang, H.X. Monitoring of ducting by using a ground-based GPS receiver. Chin. Phys. B 2013, 22, 575–579. [Google Scholar] [CrossRef]
- Wu, X.; Wang, X.; Lü, D. Retrieval of vertical distribution of tropospheric refractivity through ground-based GPS observation. Adv. Atmos. Sci. 2014, 31, 37–47. [Google Scholar] [CrossRef]
- Wu, Y.Y.; Hong, Z.J.; Guo, P.; Zheng, J. Simulation of atmospheric refractive profile retrieving from low-elevation ground-based GPS observation. Chin. J. Geophys. 2010, 53. [Google Scholar] [CrossRef]
- Miller, E.L.; Abriola, L.M.; Aghasi, A. Environmental remediation and restoration: Hydrological and geophysical processing methods. IEEE Signal Process. Mag. 2012, 29, 16–26. [Google Scholar] [CrossRef]
- Liu, J.; Liu, Q.H.; Li, J.; Ma, H.Z.; Yang, L.; Du, H.J. Radiant directionality modeling of joint thermal infrared and microwave for typical crops. J. Remote Sens. 2014, 18. [Google Scholar] [CrossRef]
- Alekseev, A.S. Quantitative statement and general properties of solutions of cooperative inverse problems (integral geophysics). In Proceedings of the 1991SEG Annual Meeting, Houston, TX, USA, 10–14 November 1991.
- Lin, W.; Xiao, P.K.; Houtse, H.; Jian, F.; Cheng, Y.X.; Yong, W. Joint gravity and gravity gradient inversion for subsurface object detection. IEEE Geosci. Remote Sens. Lett. 2013, 10, 865–869. [Google Scholar] [CrossRef]
- Chen, H.G.; Li, J.X.; Wu, J.S.; Yu, P. Study on simulated-annealing MT-gravity joint inversion. Chin. J. Geophys. 2012, 55, 663–670. [Google Scholar]
- Yang, H.; Dai, S.K.; Song, H.B.; Huang, L.P. Overview of joint inversion of integrated. Geophys. Pro. Geophy. 2002, 17, 262–271. [Google Scholar]
- Guo, P.; Kuo, Y.H.; Sokolovskiy, S.V.; Lenschow, D.H. Estimating atmospheric boundary layer depth using COSMIC radio occultation data. J. Atmos. Sci. 2011, 68, 1703–1713. [Google Scholar] [CrossRef]
- Kirchengast, G.; Hafner, J.; Poetzi, W. The CIRA86aQ_UoG Model: An Extension of the CIRA-86 Monthly Tables Including Humidity Tables and a Fortran95 Global Moist Air Climatology Model; IMG/UoG technical report; ESA/ESTEC: Graz, Austria, September 1999. [Google Scholar]
- Deb, K.; Agrawal, S.; Pratap, A.; Meyarivan, T. A fast elitist non-dominated sorting genetic algorithm for multi-objective optimization: NSGA-II. IEEE Trans. Evolut. Comput. 2002, 6, 182–197. [Google Scholar] [CrossRef]
- Deb, K. Multi-objective genetic algorithms: Problem difficulties and construction of test Functions. Evolut. Comput. 1999, 7, 205–230. [Google Scholar] [CrossRef]
- Weber, L.; Wallbaum, S.; Broger, C.; Gubernator, K. Optimization of the biological activity of combinatorial compound libraries by a genetic algorithm. Angew. Chem. Int. Edit. Eng. 1995, 34, 2280–2282. [Google Scholar] [CrossRef]
© 2016 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons by Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liao, Q.; Sheng, Z.; Shi, H. Joint Inversion of Atmospheric Refractivity Profile Based on Ground-Based GPS Phase Delay and Propagation Loss. Atmosphere 2016, 7, 12. https://doi.org/10.3390/atmos7010012
Liao Q, Sheng Z, Shi H. Joint Inversion of Atmospheric Refractivity Profile Based on Ground-Based GPS Phase Delay and Propagation Loss. Atmosphere. 2016; 7(1):12. https://doi.org/10.3390/atmos7010012
Chicago/Turabian StyleLiao, Qixiang, Zheng Sheng, and Hanqing Shi. 2016. "Joint Inversion of Atmospheric Refractivity Profile Based on Ground-Based GPS Phase Delay and Propagation Loss" Atmosphere 7, no. 1: 12. https://doi.org/10.3390/atmos7010012
APA StyleLiao, Q., Sheng, Z., & Shi, H. (2016). Joint Inversion of Atmospheric Refractivity Profile Based on Ground-Based GPS Phase Delay and Propagation Loss. Atmosphere, 7(1), 12. https://doi.org/10.3390/atmos7010012






