Imbalance Term in the TKE Budget over Waves
Abstract
1. Introduction
1.1. Context
1.2. The Surface Boundary Layer
1.3. The Wave-Induced Boundary Layer
1.4. Imbalance Term in the TKE Equation
1.5. Summary and Plan of the Proposed Study
2. Materials and Methods
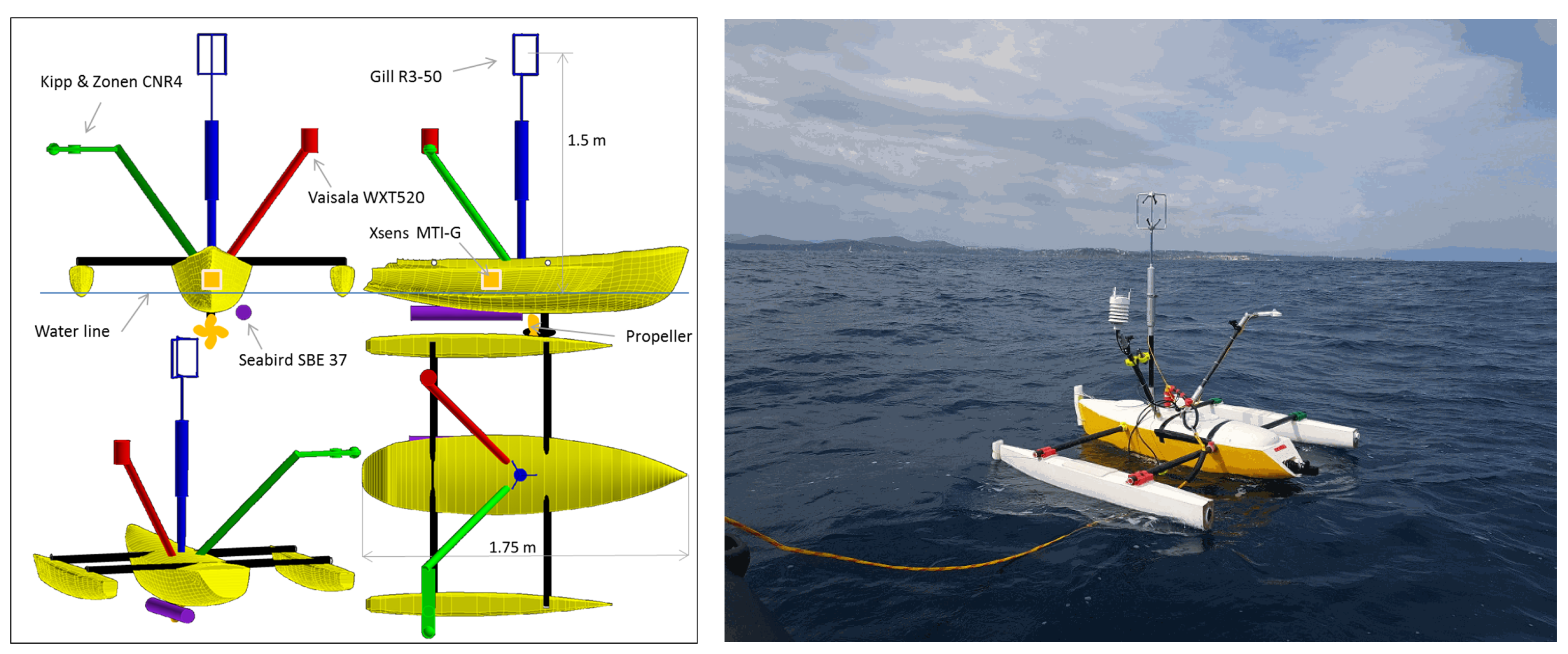
2.1. Open Sea Data
2.2. Wind-Wave Tunnel Experiment
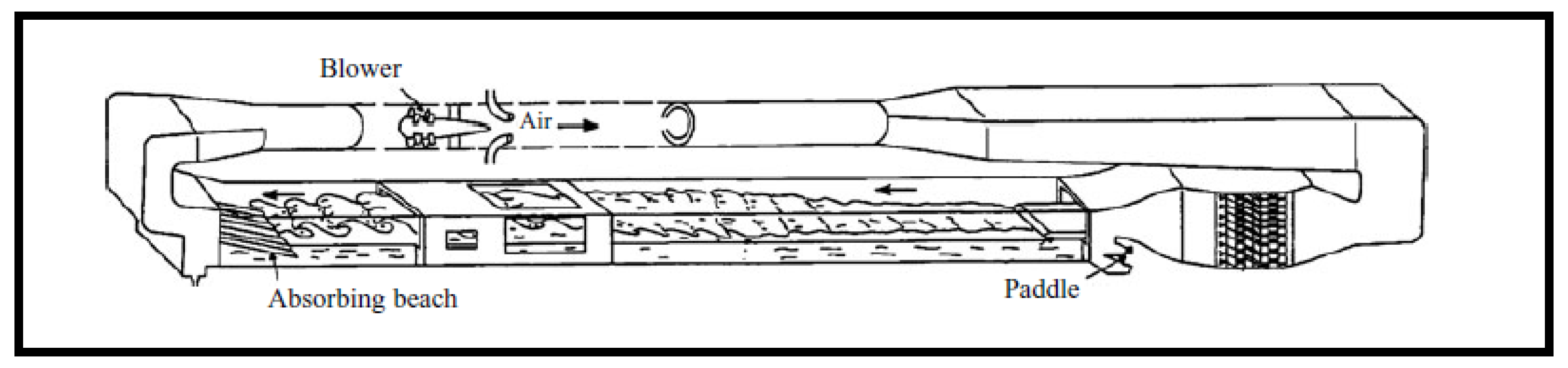
2.2.1. Large Air-Sea Interaction Facility
2.2.2. Experimental Set-Up
3. Results
3.1. Wind and Wave Characteristics
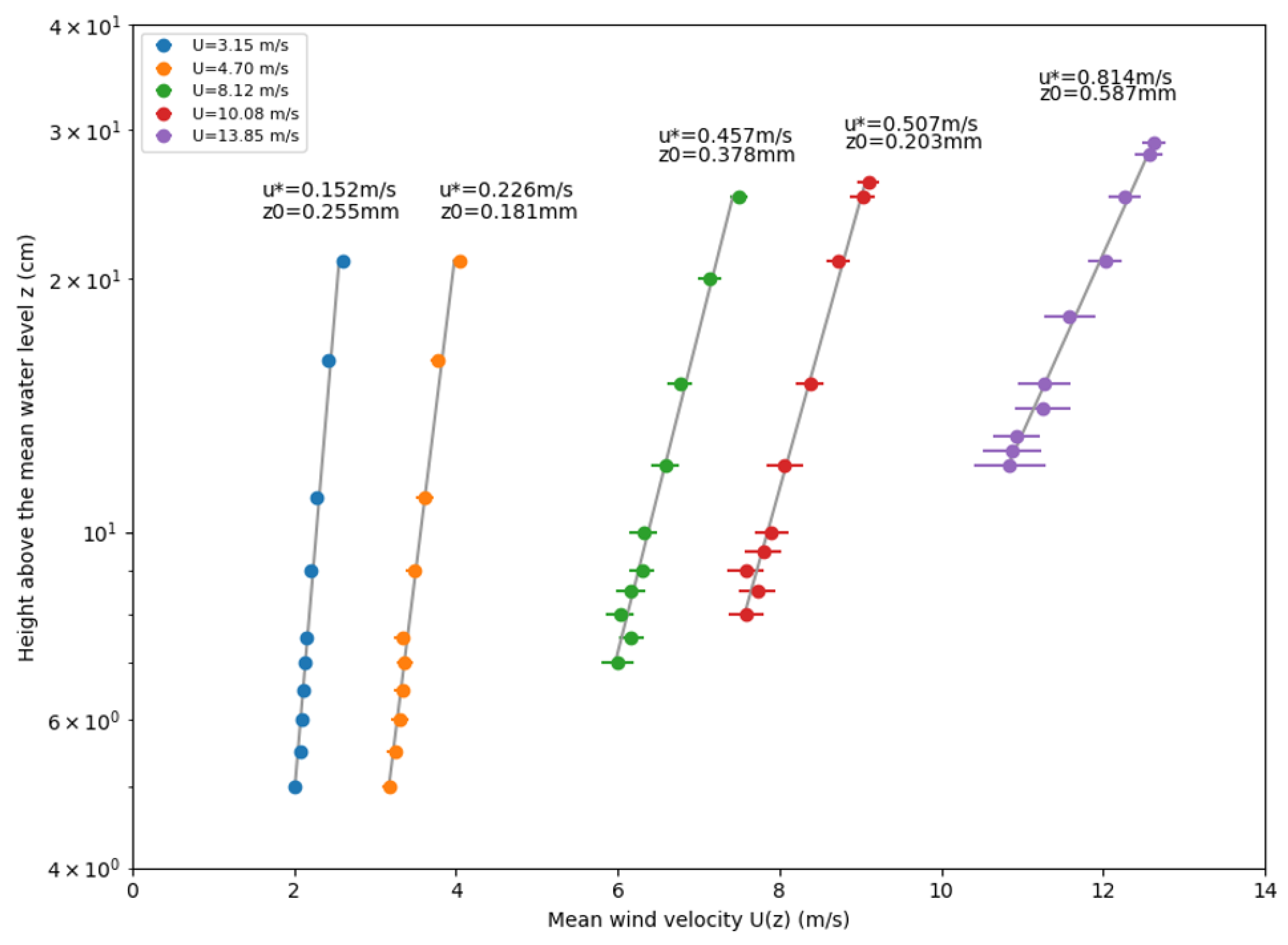
3.1.1. Mean Airflow in the SBL
3.1.2. Wave Characteristics
3.1.3. Spectral Characteristics of Turbulence
3.2. Wind Stress
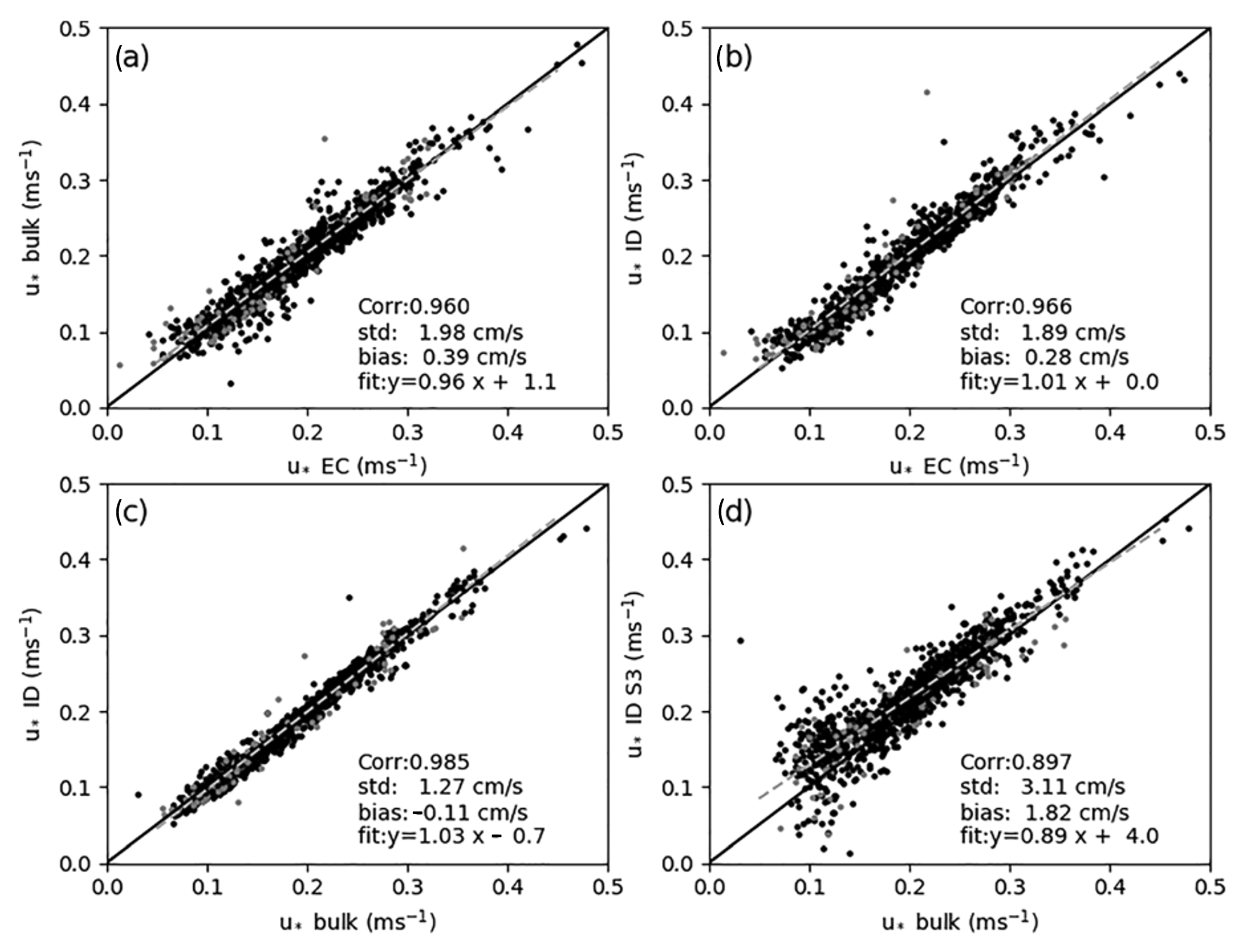
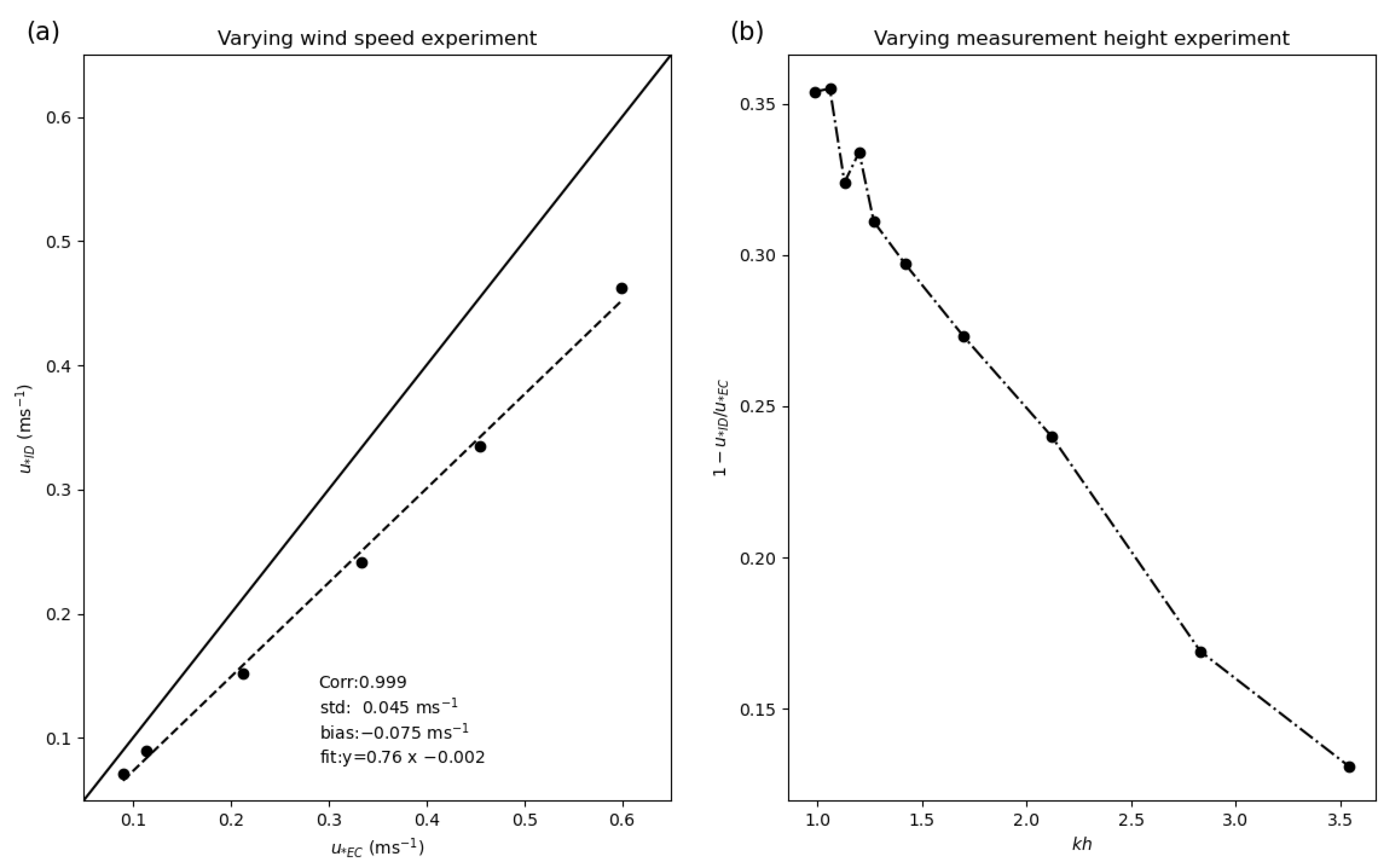
3.2.1. Comparison Between and
3.2.2. Imbalance Term
4. Discussion
5. Summary
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Miles, J.W. On the generation of surface waves by shear flows. J. Fluid Mech. 1957, 3, 185. [Google Scholar] [CrossRef]
- Donelan, M.A.; Haus, B.K.; Reul, N.; Plant, W.J.; Stiassnie, M.; Graber, H.C.; Brown, O.B.; Saltzman, E.S. On the limiting aerodynamic roughness of the ocean in very strong winds. Geophys. Res. Lett. 2004, 31, 2004GL019460. [Google Scholar] [CrossRef]
- Monin, A.; Obukhov, A. Basic Laws of Turbulent Mixing in the Surface Layer of the Atmosphere. Contrib. Geophys. Inst. Acad. Sci. USSR 1954, 24, 163–187. [Google Scholar]
- Obukhov, A.M. Turbulence in an atmosphere with a non-uniform temperature. Bound.-Layer Meteorol. 1971, 2, 7–29. [Google Scholar] [CrossRef]
- Businger, J.A.; Wyngaard, J.C.; Izumi, Y.; Bradley, E.F. Flux-Profile Relationships in the Atmospheric Surface Layer. J. Atmos. Sci. 1971, 28, 181–189. [Google Scholar] [CrossRef]
- Champagne, F.H.; Friehe, C.A.; LaRue, J.C.; Wynagaard, J.C. Flux Measurements, Flux Estimation Techniques, and Fine-Scale Turbulence Measurements in the Unstable Surface Layer Over Land. J. Atmos. Sci. 1977, 34, 515–530. [Google Scholar] [CrossRef]
- Kaimal, J.C.; Wyngaard, J.C. The Kansas and Minnesota experiments. Bound.-Layer Meteorol. 1990, 50, 31–47. [Google Scholar] [CrossRef]
- Fairall, C.W.; Banner, M.L.; Peirson, W.L.; Asher, W.; Morison, R.P. Investigation of the physical scaling of sea spray spume droplet production. J. Geophys. Res. Oceans 2009, 114, 2008JC004918. [Google Scholar] [CrossRef]
- Bruch, W. Experimental and Numerical Study of Sea Spray Generation and Transport, and Their Consequences on the Properties of the Marine Atmospheric Boundary Layer. Ph.D. Thesis, University of Toulon, La Garde, France, 2021. [Google Scholar]
- Kudryavtsev, V.N.; Makin, V.K. Impact of Ocean Spray on the Dynamics of the Marine Atmospheric Boundary Layer. Bound.-Layer Meteorol. 2011, 140, 383–410. [Google Scholar] [CrossRef]
- Geernaert, G.L.; Katsaros, K.B.; Richter, K. Variation of the drag coefficient and its dependence on sea state. J. Geophys. Res. Oceans 1986, 91, 7667–7679. [Google Scholar] [CrossRef]
- Donelan, M.A.; Dobson, F.W.; Smith, S.D.; Anderson, R.J. On the Dependence of Sea Surface Roughness on Wave Development. J. Phys. Oceanogr. 1993, 23, 2143–2149. [Google Scholar] [CrossRef]
- Hsu, C.T.; Hsu, E.Y.; Street, R.L. On the structure of turbulent flow over a progressive water wave: Theory and experiment in a transformed, wave-following co-ordinate system. J. Fluid Mech. 1981, 105, 87. [Google Scholar] [CrossRef]
- Belcher, S.E.; Hunt, J.C.R. Turbulent shear flow over slowly moving waves. J. Fluid Mech. 1993, 251, 109–148. [Google Scholar] [CrossRef]
- Edson, J.B.; Fairall, C.W. Similarity Relationships in the Marine Atmospheric Surface Layer for Terms in the TKE and Scalar Variance Budgets. J. Atmospheric Sci. 1998, 55, 2311–2328. [Google Scholar] [CrossRef]
- Smith, S.D. Coefficients for sea surface wind stress, heat flux, and wind profiles as a function of wind speed and temperature. J. Geophys. Res. Oceans 1988, 93, 15467–15472. [Google Scholar] [CrossRef]
- Charnock, H. Wind stress on a water surface. Q. J. R. Meteorol. Soc. 1955, 81, 639–640. [Google Scholar] [CrossRef]
- Edson, J.B.; Jampana, V.; Weller, R.A.; Bigorre, S.P.; Plueddemann, A.J.; Fairall, C.W.; Miller, S.D.; Mahrt, L.; Vickers, D.; Hersbach, H. On the Exchange of Momentum over the Open Ocean. J. Phys. Oceanogr. 2013, 43, 1589–1610. [Google Scholar] [CrossRef]
- Edson, J.; Crawford, T.; Crescenti, J.; Farrar, T.; Frew, N.; Gerbi, G.; Helmis, C.; Hristov, T.; Khelif, D.; Jessup, A.; et al. The Coupled Boundary Layers and Air–Sea Transfer Experiment in Low Winds. Bull. Am. Meteorol. Soc. 2007, 88, 341–356. [Google Scholar] [CrossRef]
- Soloviev, Y.P.; Kudryavtsev, V.N. Wind-Speed Undulations Over Swell: Field Experiment and Interpretation. Bound.-Layer Meteorol. 2010, 136, 341–363. [Google Scholar] [CrossRef]
- Katsaros, K.B. The aqueous thermal boundary layer. Bound.-Layer Meteorol. 1980, 18, 107–127. [Google Scholar] [CrossRef]
- Bourras, D.; Branger, H.; Reverdin, G.; Marié, L.; Cambra, R.; Baggio, L.; Caudoux, C.; Caudal, G.; Morisset, S.; Geyskens, N.; et al. A New Platform for the Determination of Air–Sea Fluxes (OCARINA): Overview and First Results. J. Atmospheric Ocean. Technol. 2014, 31, 1043–1062. [Google Scholar] [CrossRef]
- Fujitani, T. Direct measurement of turbulent fluxes over the sea during AMTEX. Pap. Meteorol. Geophys. 1981, 32, 119–134. [Google Scholar] [CrossRef]
- Fujitani, T. Method of turbulent flux measurement on a ship by using a stable platform system. Pap. Meteorol. Geophys. 1985, 36, 157–170. [Google Scholar] [CrossRef]
- Tsukamoto, O.; Ohtaki, E.; Ishida, H.; Horiguchi, M.; Mitsuta, Y. On-Board Direct Measurements of Turbulent Fluxes over the Open Sea. J. Meteorol. Soc. Jpn. Ser. II 1990, 68, 203–211. [Google Scholar]
- Bradley, E.F.; Coppin, P.A.; Godfrey, J.S. Measurements of sensible and latent heat flux in the western equatorial Pacific Ocean. J. Geophys. Res. Oceans 1991, 96, 3375–3389. [Google Scholar] [CrossRef]
- Fairall, C.W.; White, A.B.; Edson, J.B.; Hare, J.E. Integrated Shipboard Measurements of the Marine Boundary Layer. J. Atmospheric Ocean. Technol. 1997, 14, 338–359. [Google Scholar] [CrossRef]
- Edson, J.B.; Hinton, A.A.; Prada, K.E.; Hare, J.E.; Fairall, C.W. Direct Covariance Flux Estimates from Mobile Platforms at Sea. J. Atmospheric Ocean. Technol. 1998, 15, 547–562. [Google Scholar] [CrossRef]
- Webster, P.J.; Lukas, R. TOGA COARE: The Coupled Ocean—Atmosphere Response Experiment. Bull. Am. Meteorol. Soc. 1992, 73, 1377–1416. [Google Scholar] [CrossRef]
- Kawamura, H.; Okuda, K.; Kawai, S.; Toba, Y. Structure of Turbulent Boundary Layer over Wind Waves in a Wind Wave Tunnel. Ph.D. Thesis, Tohoku University, Tokyo, Japan, 1981. Volume 28. pp. 69–86. [Google Scholar]
- Lai, R.J.; Shemdin, O.H. Laboratory investigation of air turbulence above simple water waves. J. Geophys. Res. Oceans 1971, 76, 7334–7350. [Google Scholar] [CrossRef]
- Buckley, M.; Veron, F. The turbulent airflow over wind generated surface waves. Eur. J. Mech.-B/Fluids 2019, 73, 132–143. [Google Scholar] [CrossRef]
- Bourras, D.; Cambra, R.; Marié, L.; Bouin, M.; Baggio, L.; Branger, H.; Beghoura, H.; Reverdin, G.; Dewitte, B.; Paulmier, A.; et al. Air-Sea Turbulent Fluxes From a Wave-Following Platform During Six Experiments at Sea. J. Geophys. Res. Oceans 2019, 124, 4290–4321. [Google Scholar] [CrossRef]
- Donelan, M.A.; Drennan, W.M.; Katsaros, K.B. The Air–Sea Momentum Flux in Conditions of Wind Sea and Swell. J. Phys. Oceanogr. 1997, 27, 2087–2099. [Google Scholar] [CrossRef]
- Bruch, W.; Conan, B. Mean Wind Profile Departure from Log-Law in the Lower Marine Atmospheric Boundary Layer for Different Wave-Wind Conditions Using Scanning Wind LiDAR Measurements. Available online: https://hal.science/hal-04984296v1 (accessed on 23 March 2025).
- Elfouhaily, T.; Vandemark, D.; Gourrion, J.; Chapron, B. Estimation of wind stress using dual-frequency TOPEX data. J. Geophys. Res. Oceans 1998, 103, 25101–25108. [Google Scholar] [CrossRef]
- Hara, T.; Sullivan, P.P. Wave Boundary Layer Turbulence over Surface Waves in a Strongly Forced Condition. J. Phys. Oceanogr. 2015, 45, 868–883. [Google Scholar] [CrossRef]
- Pedreros, R.; Dardier, G.; Dupuis, H.; Graber, H.C.; Drennan, W.M.; Weill, A.; Guérin, C.; Nacass, P. Momentum and heat fluxes via the eddy correlation method on the R/V L’Atalante and an ASIS buoy. J. Geophys. Res. Oceans 2003, 108, 2002JC001449. [Google Scholar] [CrossRef]
- Kolmogorov, A.N. Air–Sea Interaction in the Southern Ocean: Exploring the Height of the Wave Boundary Layer at the Air–Sea Interface. Doklady Akademii Nauk SSSR 1941, 30, 301–304. [Google Scholar]
- Dupuis, H.; Taylor, P.K.; Weill, A.; Katsaros, K. Inertial dissipation method applied to derive turbulent fluxes over the ocean during the Surface of the Ocean, Fluxes and Interactions with the Atmosphere/Atlantic Stratocumulus Transition Experiment (SOFIA/ASTEX) and Structure des Echanges Mer-Atmosphere, Proprietes des Heterogeneites Oceaniques: Recherche Experimentale (SEMAPHORE) experiments with low to moderate wind speeds. J. Geophys. Res. Oceans 1997, 102, 21115–21129. [Google Scholar] [CrossRef]
- Taylor, P.K.; Yelland, M.J. The Dependence of Sea Surface Roughness on the Height and Steepness of the Waves. J. Phys. Oceanogr. 2001, 31, 572–590. [Google Scholar] [CrossRef]
- Bourras, D.; Weill, A.; Caniaux, G.; Eymard, L.; Bourlès, B.; Letourneur, S.; Legain, D.; Key, E.; Baudin, F.; Piguet, B.; et al. Turbulent air-sea fluxes in the Gulf of Guinea during the AMMA experiment. J. Geophys. Res. Oceans 2009, 114, 2008JC004951. [Google Scholar] [CrossRef]
- Stull, R.B. An Introduction to Boundary Layer Meteorology; Springer Science & Business Media: Berlin/Heidelberg, Germany, 1988. [Google Scholar] [CrossRef]
- Bourras, D.; Cambra, R.; Marie, L.; Bouin, M.N.; Baggio, L.; Branger, H.; Beghoura, H.; Reverdin, G.; Dewitte, B.; Paulmier, A.; et al. OCARINA (Ocean coupled to the atmosphere: Instrumented research on an auxiliary ship). J. Geophys. Res. Oceans 2019. [Google Scholar] [CrossRef]
- Bourras, D.; Branger, H.; Luneau, C.; Reverdin, G.; Speich, S.; Geykens, N.; Barrois, H.; Clémençon, A. EUREC4A-OA_OCARINA: OCARINA Air-Sea Flux Data. Available online: https://hal.science/hal-03066519/document (accessed on 8 December 2020).
- Cifuentes-Lorenzen, A.; Edson, J.B.; Zappa, C.J. Air–Sea Interaction in the Southern Ocean: Exploring the Height of the Wave Boundary Layer at the Air–Sea Interface. Bound.-Layer Meteorol. 2018, 169, 461–482. [Google Scholar] [CrossRef]
- Villefer, A.; Benoit, M.; Violeau, D.; Luneau, C.; Branger, H. Influence of Following, Regular, and Irregular Long Waves on Wind-Wave Growth with Fetch: An Experimental Study. J. Phys. Oceanogr. 2021, 51, 3435–3448. [Google Scholar] [CrossRef]
- Coantic, M.; Ramamonjiarisoa, A.; Mestayer, P.; Resch, F.; Favre, A. Wind-water tunnel simulation of small-scale ocean-atmosphere interactions. J. Geophys. Res. Oceans 1981, 86, 6607–6626. [Google Scholar] [CrossRef]
- Jørgsen, F.E. How to Measure Turbulence with Hot-Wire Anemometers—A Practical Guide. Available online: https://web.iitd.ac.in/~pmvs/courses/mel705/hotwire2.pdf (accessed on 8 December 2020).
- Grare, L.; Peirson, W.L.; Branger, H.; Walker, J.W.; Giovanangeli, J.P.; Makin, V. Growth and dissipation of wind-forced, deep-water waves. J. Fluid Mech. 2013, 722, 5–50. [Google Scholar] [CrossRef]
- Kaimal, J.C.; Wyngaard, J.C.; Izumi, Y.; Coté, O.R. Spectral characteristics of surface-layer turbulence. Q. J. R. Meteorol. Soc. 1972, 98, 563–589. [Google Scholar] [CrossRef]

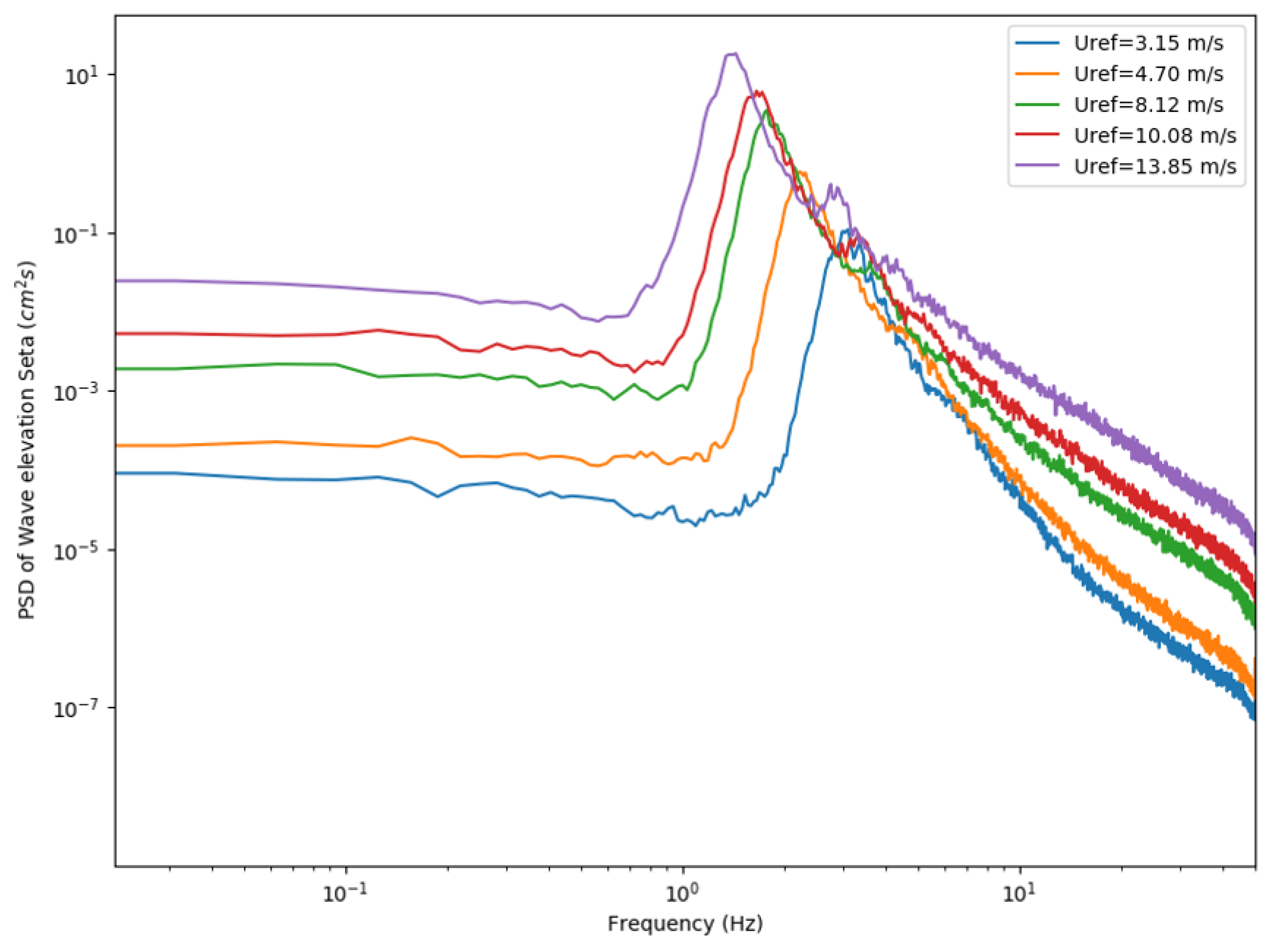
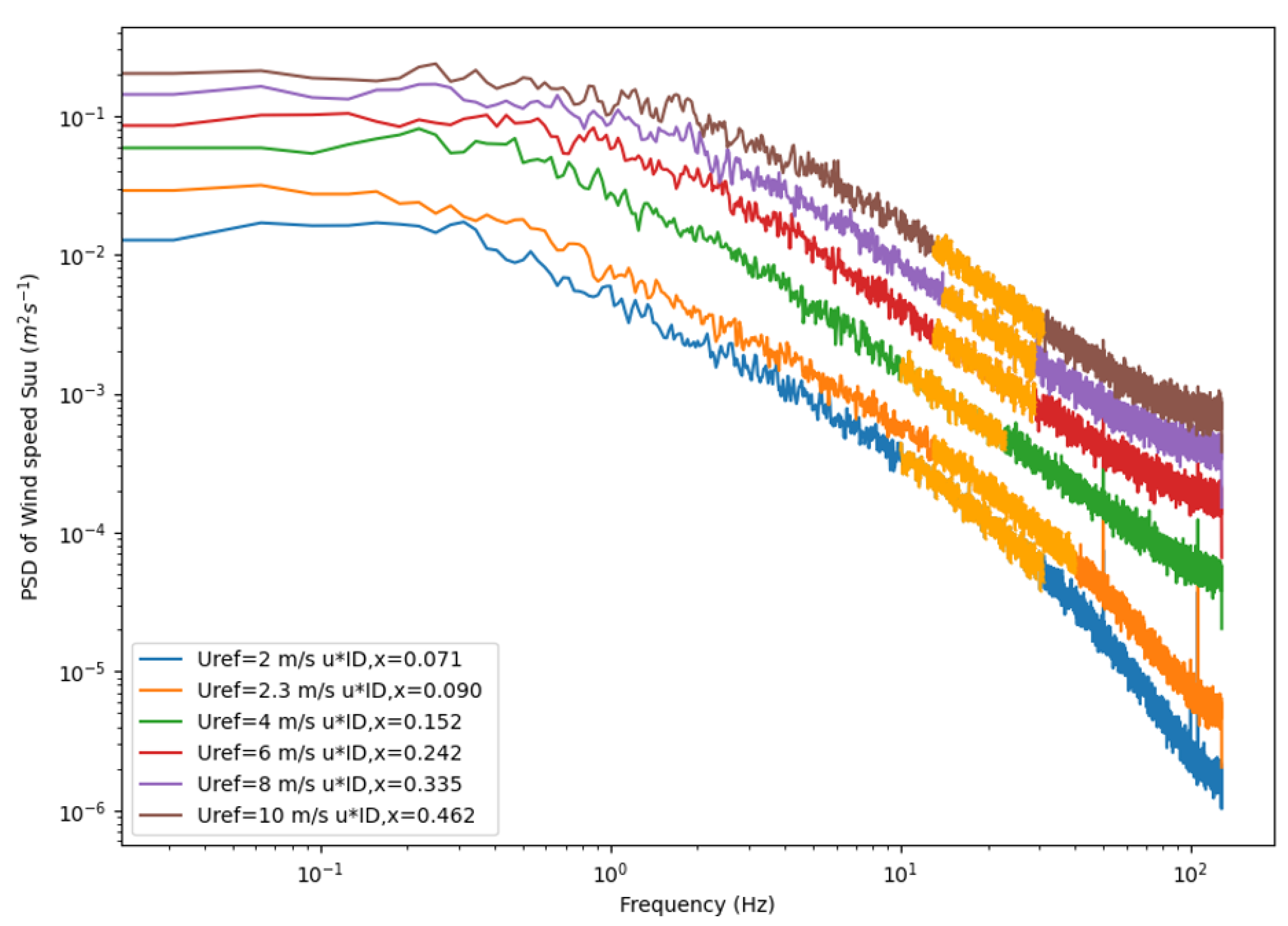
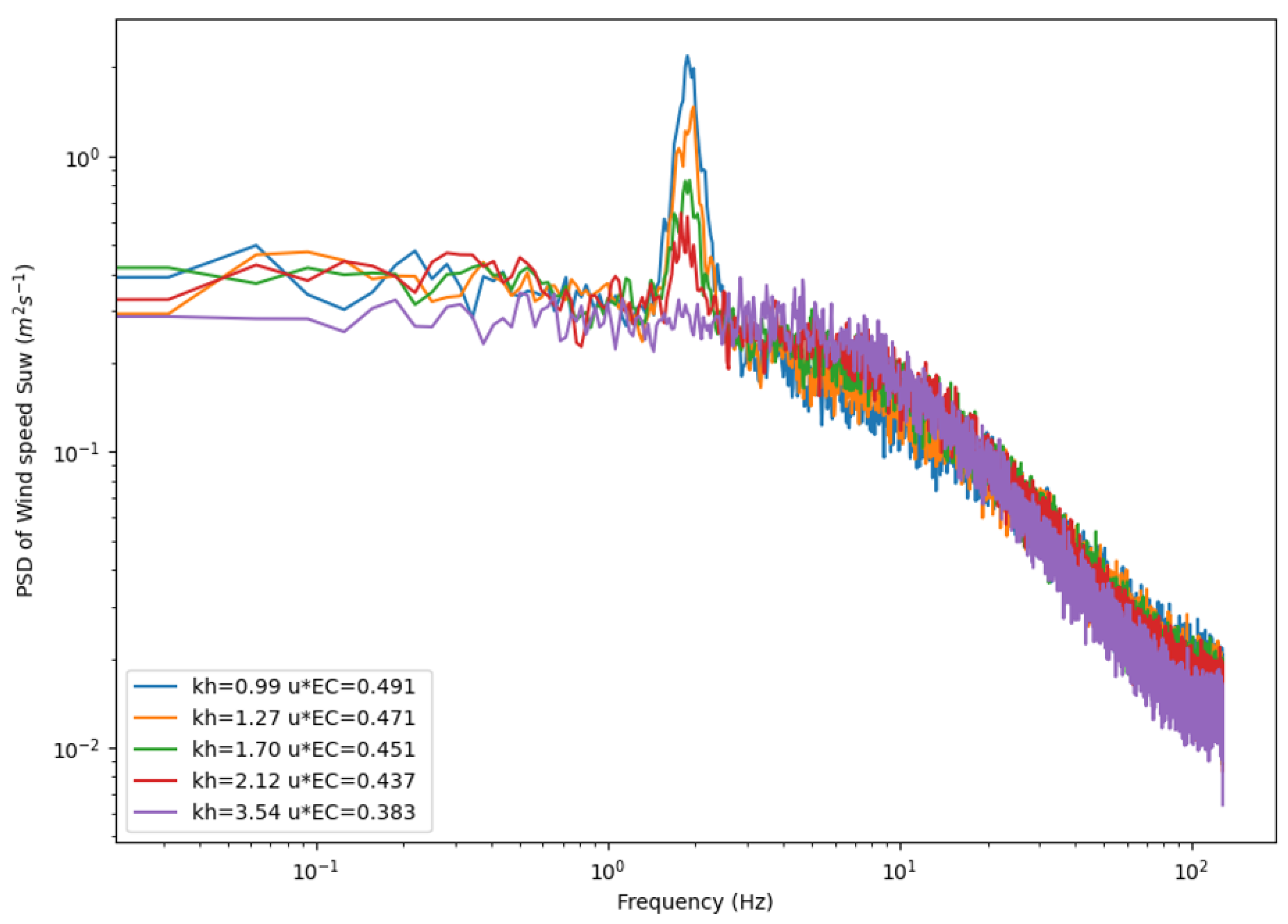

| (m s−1) | (m s−1) | (m s−1) | (mm) |
|---|---|---|---|
| 3.15 | 3.7 | 0.12 | 0.045 |
| 4.70 | 5.8 | 0.20 | 0.083 |
| 8.12 | 11.1 | 0.41 | 0.196 |
| 10.08 | 13.3 | 0.48 | 0.147 |
| 13.85 | 19.8 | 0.81 | 0.559 |
| (m s−1) | (s) | (Hz) | (cm) | (m) | (m s−1) | |
|---|---|---|---|---|---|---|
| 3.15 | 0.323 | 3.10 | 1.14 | 0.165 | 0.51 | 0.13 |
| 4.70 | 0.450 | 2.22 | 2.21 | 0.317 | 0.705 | 0.11 |
| 8.12 | 0.561 | 1.78 | 4.61 | 0.492 | 0.877 | 0.07 |
| 10.08 | 0.603 | 1.66 | 6.28 | 0.568 | 0.942 | 0.07 |
| 13.85 | 0.695 | 1.44 | 10.04 | 0.755 | 1.086 | 0.05 |
| (m s−1) | (m s−1) | (mm) | (m s−1) | (mm) | |
|---|---|---|---|---|---|
| 2 | 3.77 | 0.071 | 0.012 | 0.09 | 0.066 |
| 2.3 | 3.52 | 0.09 | 0.028 | 0.114 | 0.129 |
| 4 | 1.98 | 0.152 | 0.029 | 0.212 | 0.272 |
| 6 | 1.90 | 0.242 | 0.047 | 0.333 | 0.383 |
| 8 | 1.65 | 0.335 | 0.066 | 0.455 | 0.483 |
| 10 | 1.82 | 0.462 | 0.149 | 0.599 | 0.721 |
| (m s−1) | (m s−1) | / | ||
|---|---|---|---|---|
| 0.99 | 0.317 | 0.491 | 0.646 | 0.73 |
| 1.06 | 0.312 | 0.484 | 0.645 | 0.73 |
| 1.13 | 0.236 | 0.482 | 0.676 | 0.69 |
| 1.20 | 0.317 | 0.476 | 0.666 | 0.70 |
| 1.27 | 0.329 | 0.471 | 0.689 | 0.67 |
| 1.42 | 0.327 | 0.465 | 0.703 | 0.65 |
| 1.70 | 0.328 | 0.451 | 0.727 | 0.61 |
| 2.12 | 0.332 | 0.437 | 0.760 | 0.56 |
| 2.83 | 0.340 | 0.409 | 0.831 | 0.43 |
| 3.54 | 0.333 | 0.383 | 0.869 | 0.34 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Vonta, L.; Bourras, D.; Benjeddou, S.; Luneau, C.; Touboul, J.; Fraunié, P.; Sentchev, A.; Villefer, A. Imbalance Term in the TKE Budget over Waves. Atmosphere 2025, 16, 412. https://doi.org/10.3390/atmos16040412
Vonta L, Bourras D, Benjeddou S, Luneau C, Touboul J, Fraunié P, Sentchev A, Villefer A. Imbalance Term in the TKE Budget over Waves. Atmosphere. 2025; 16(4):412. https://doi.org/10.3390/atmos16040412
Chicago/Turabian StyleVonta, Linta, Denis Bourras, Saïd Benjeddou, Christopher Luneau, Julien Touboul, Philippe Fraunié, Alexei Sentchev, and Antoine Villefer. 2025. "Imbalance Term in the TKE Budget over Waves" Atmosphere 16, no. 4: 412. https://doi.org/10.3390/atmos16040412
APA StyleVonta, L., Bourras, D., Benjeddou, S., Luneau, C., Touboul, J., Fraunié, P., Sentchev, A., & Villefer, A. (2025). Imbalance Term in the TKE Budget over Waves. Atmosphere, 16(4), 412. https://doi.org/10.3390/atmos16040412