Effects of Wind Shields on Pedestrian-Level Wind Environment around Outdoor Platforms of a Megatall Building
Abstract
1. Introduction
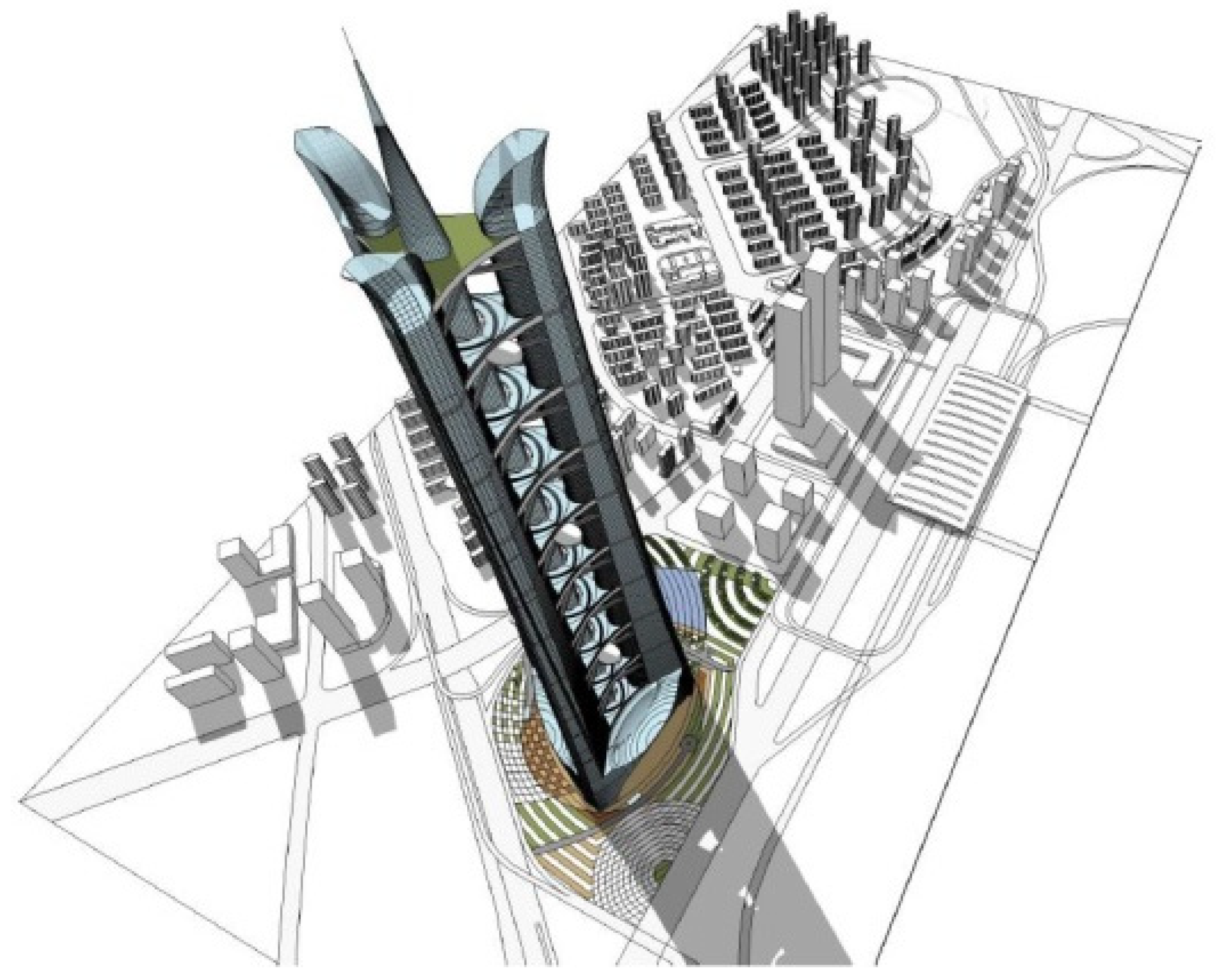
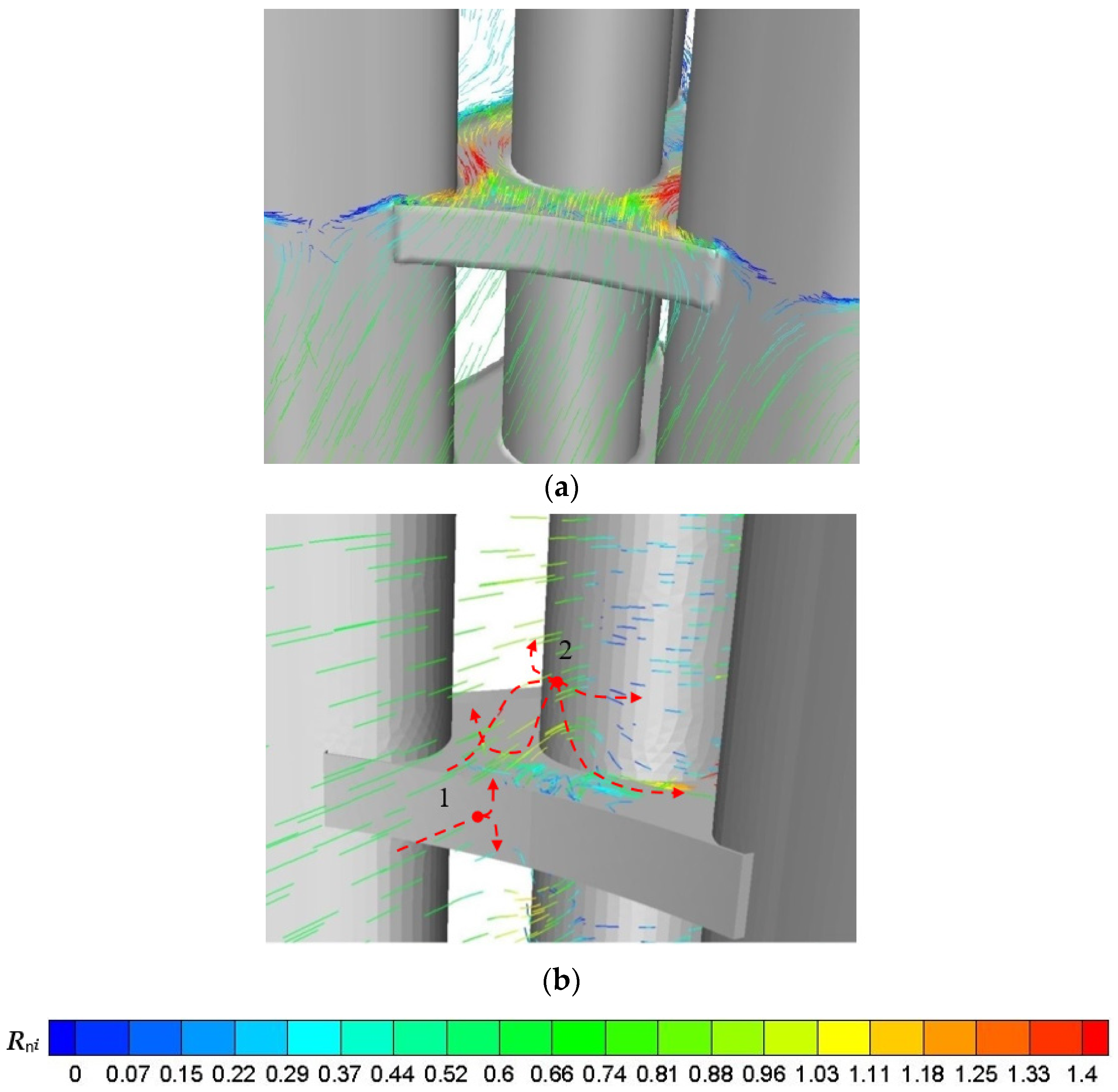
2. Characteristics of Wind Field around the Outdoor Platforms of the Baseline Model
3. Setup of Wind Tunnel Test
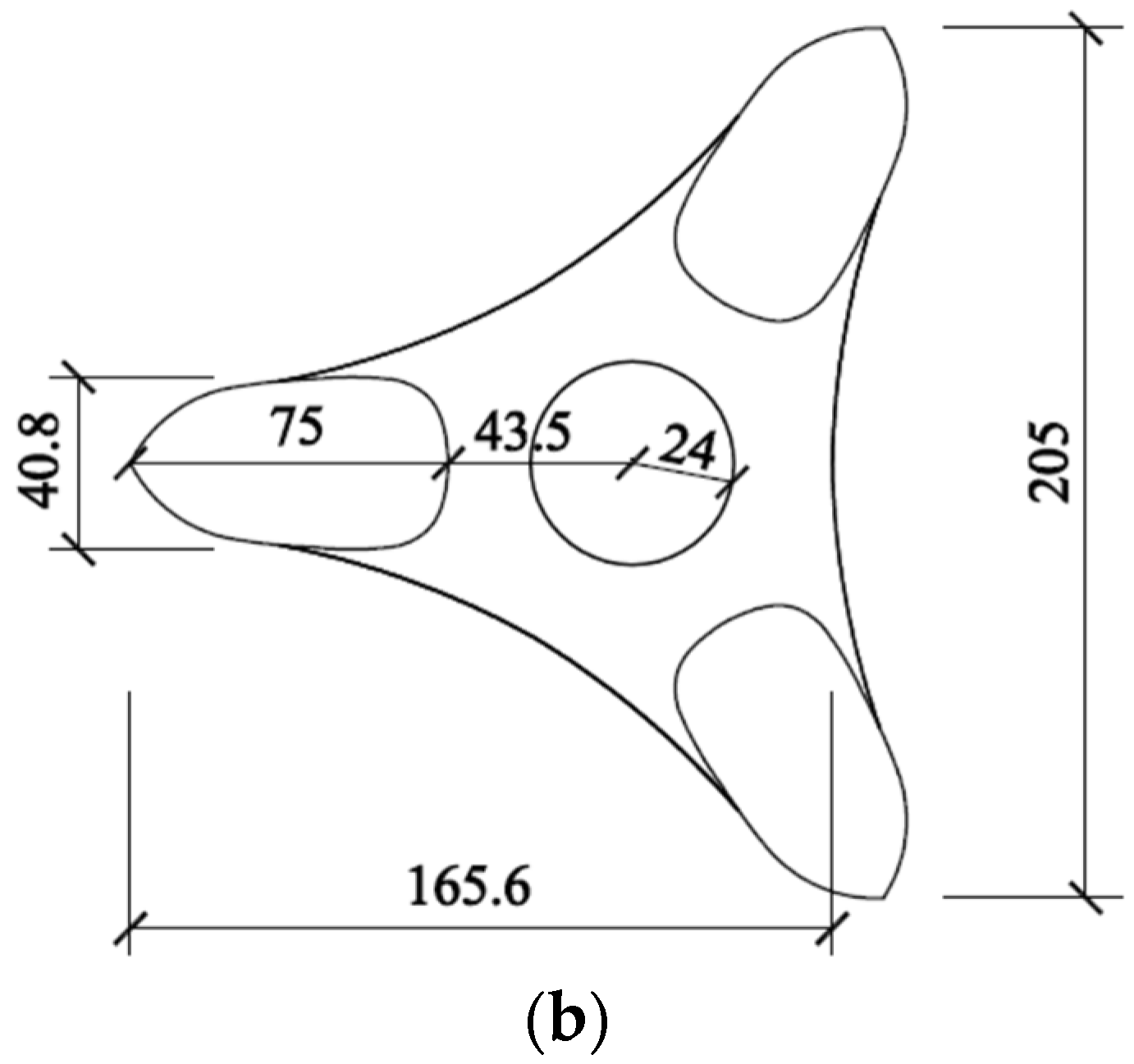
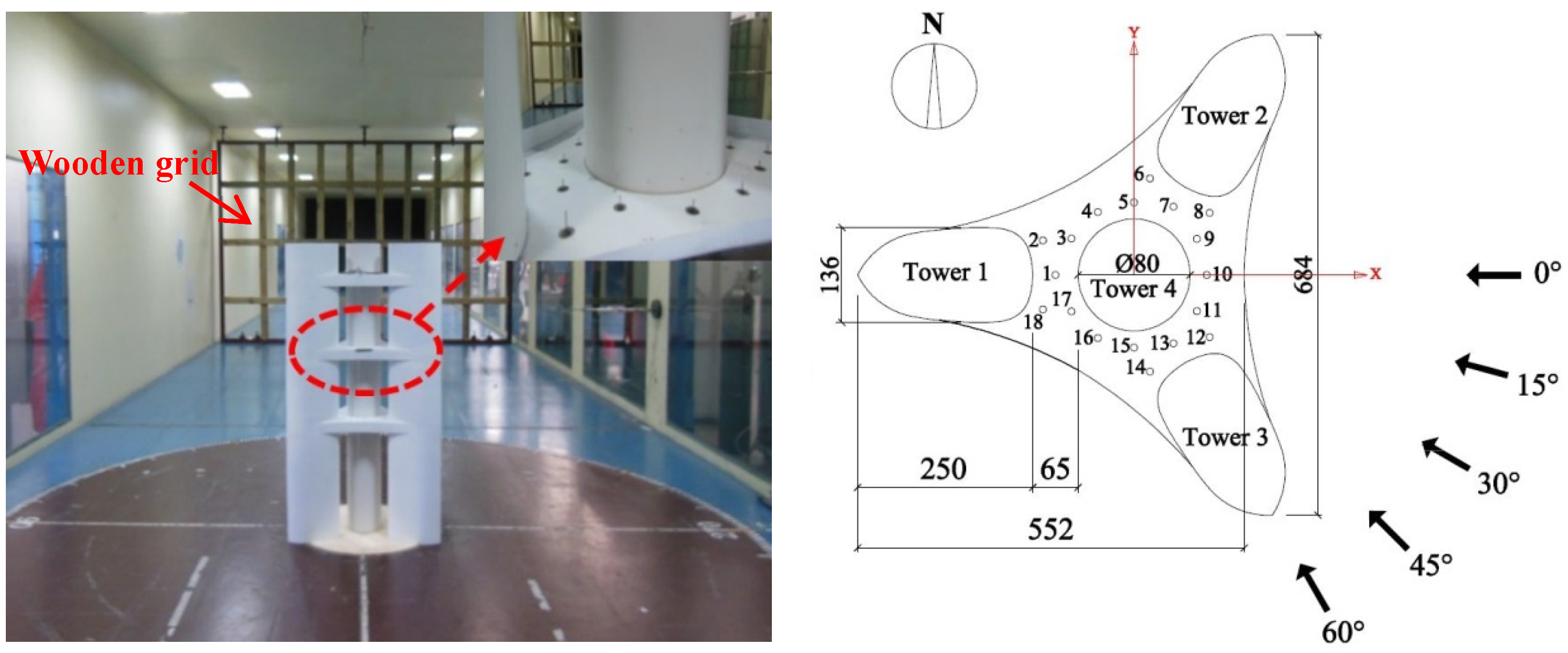
3.1. Test Models
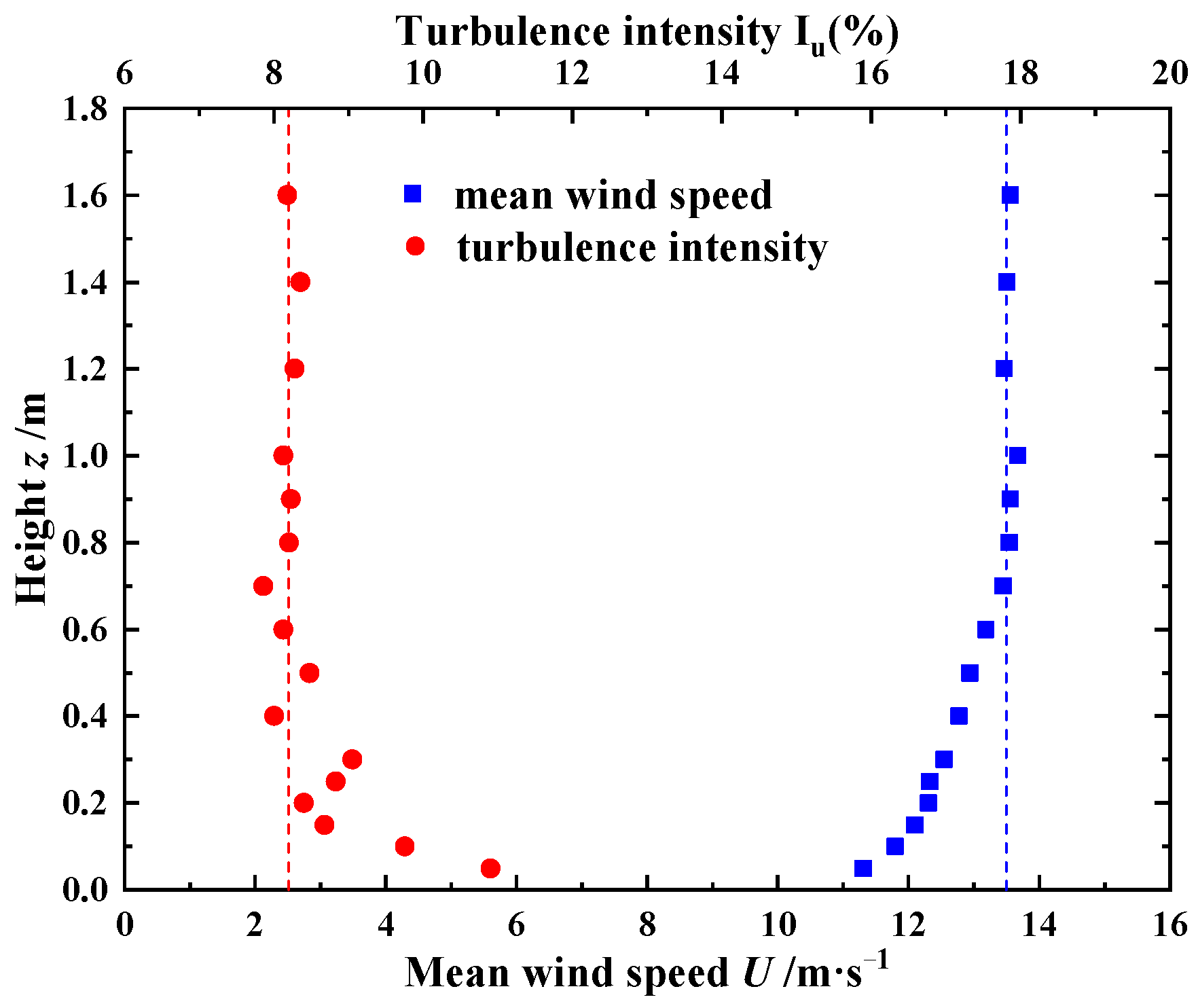
3.2. Generation of the Wind Field
4. Results Analysis and Discussions
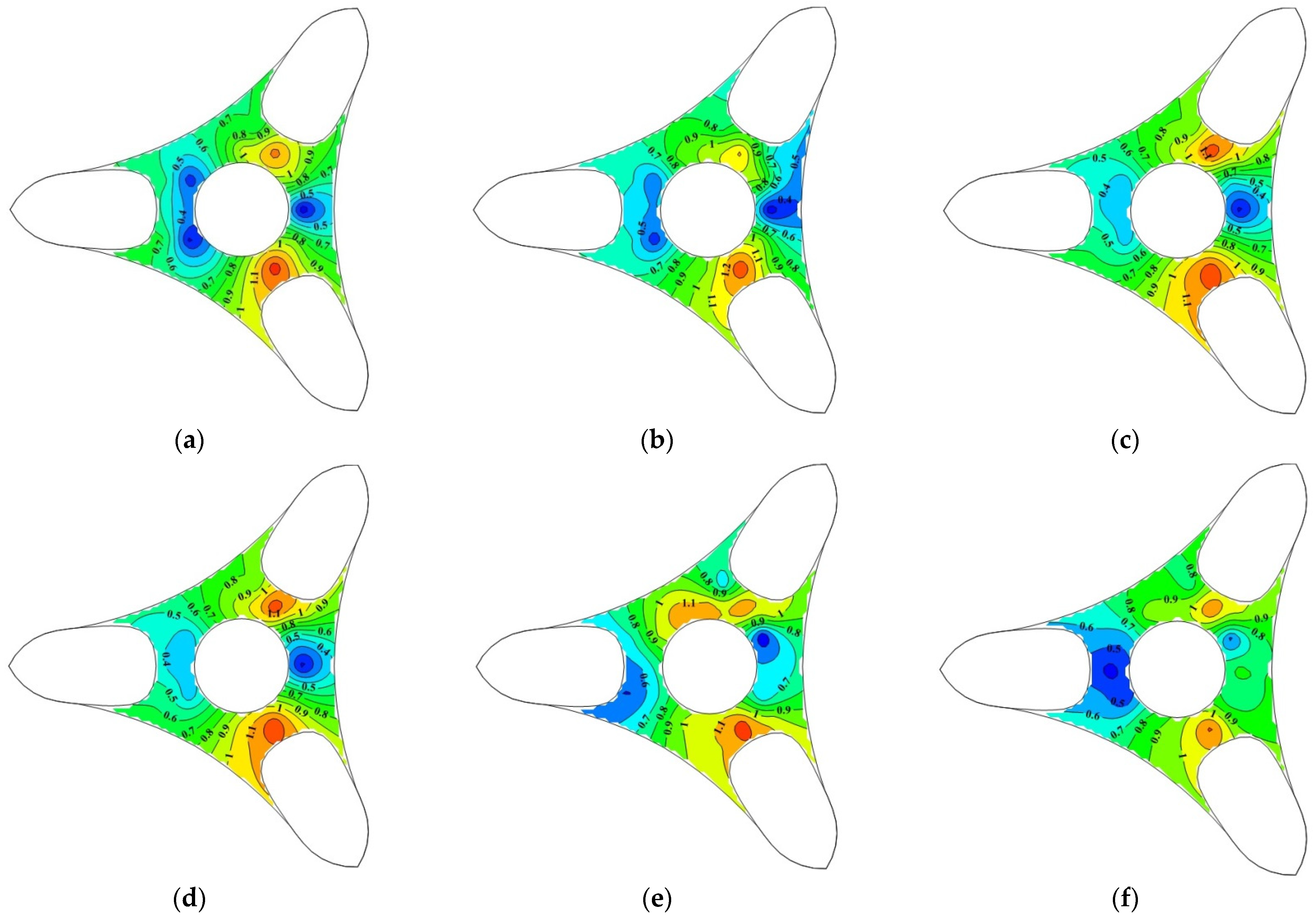
4.1. Characteristics of the Pedestrian-Level Wind Environment
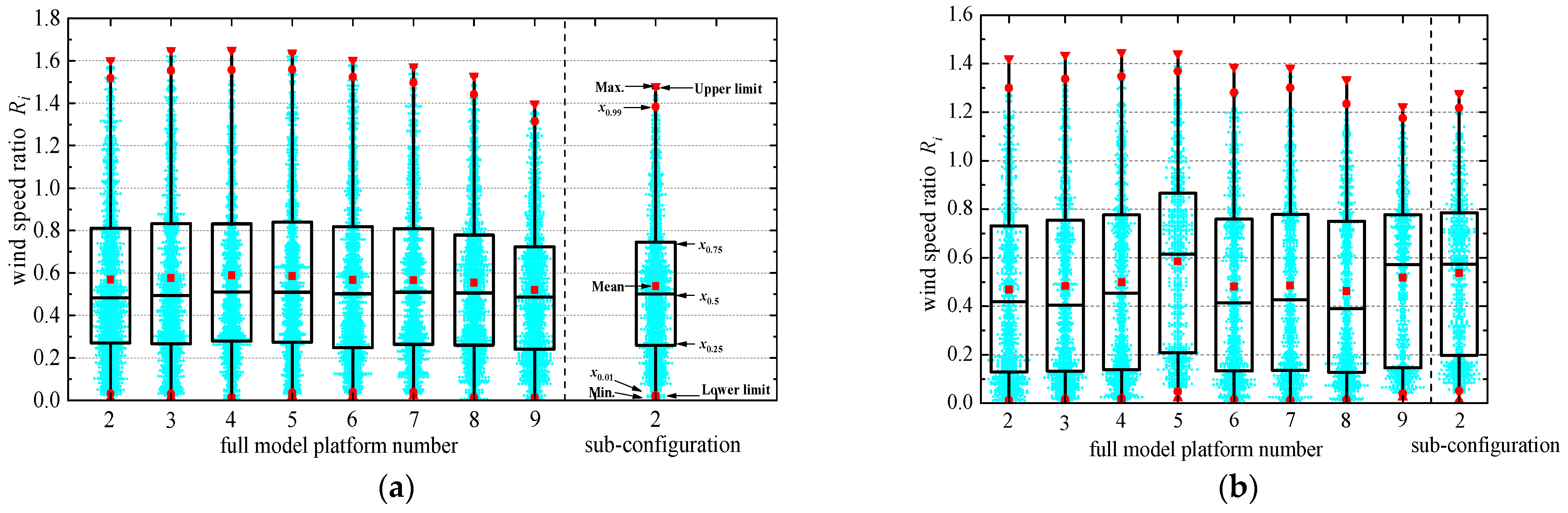
4.2. The Link between the Sub-Configuration Model and the Full Model
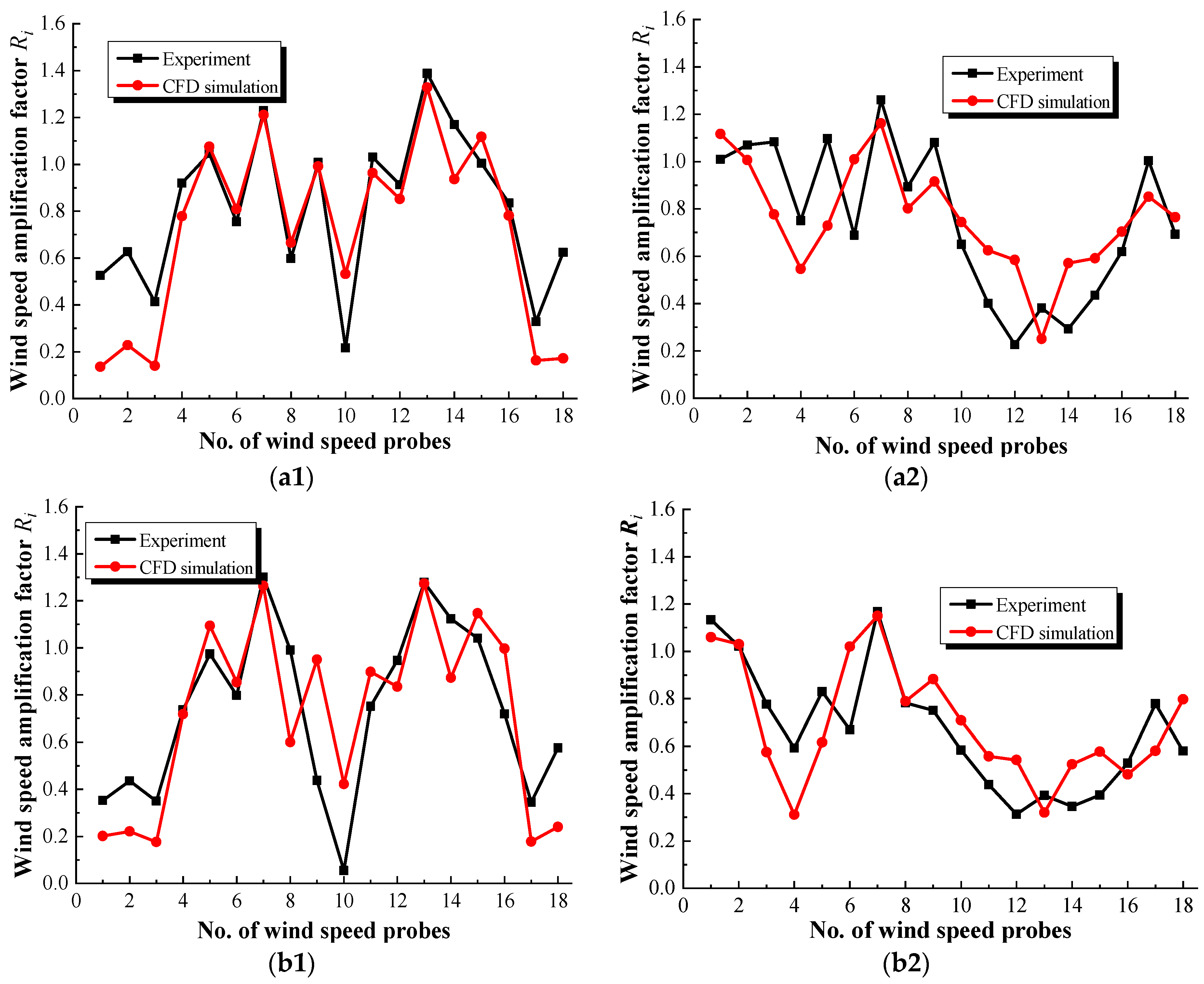
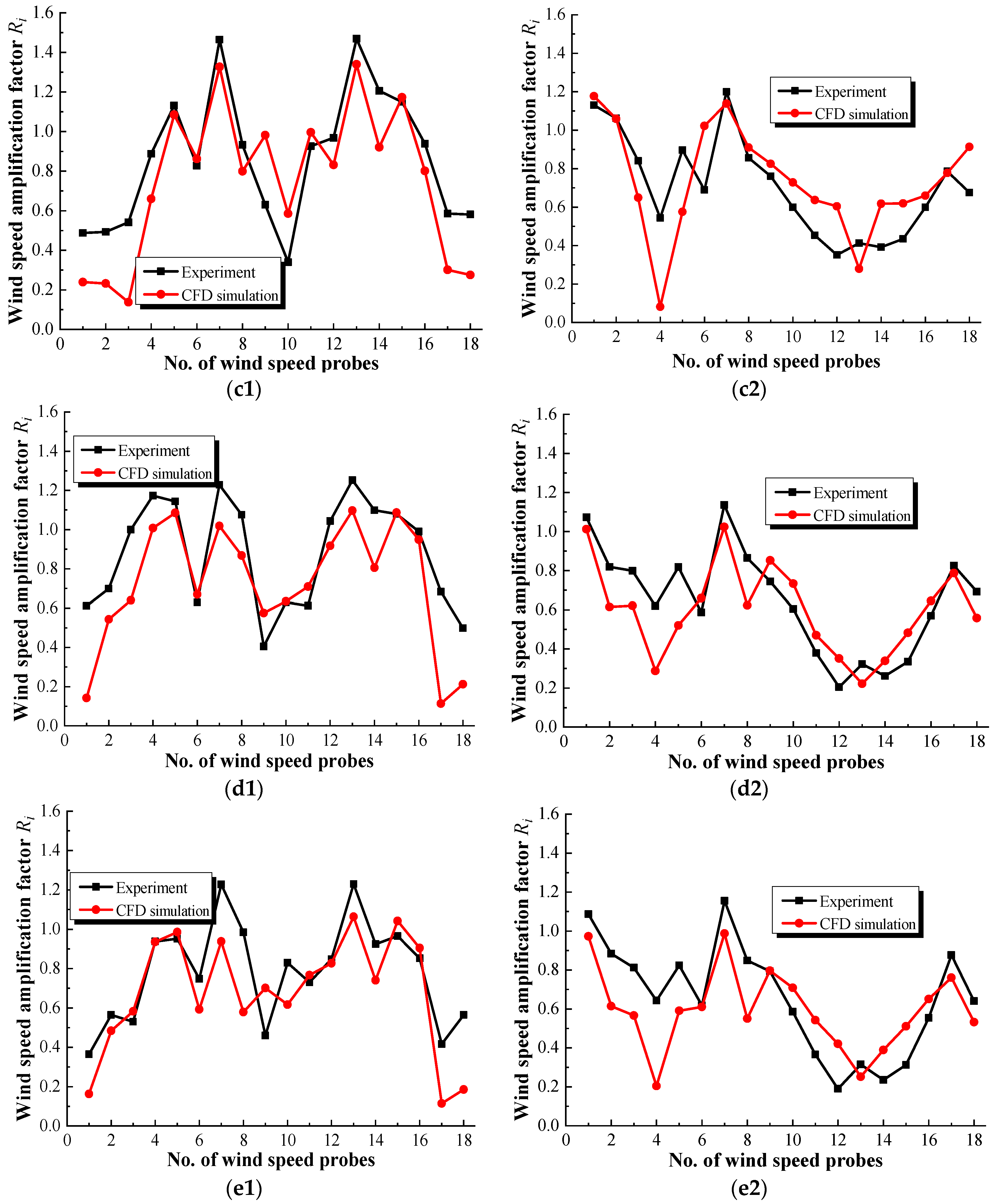
4.2.1. Validation of the CFD Simulation
4.2.2. Link between the Sub-Configuration and Full Model
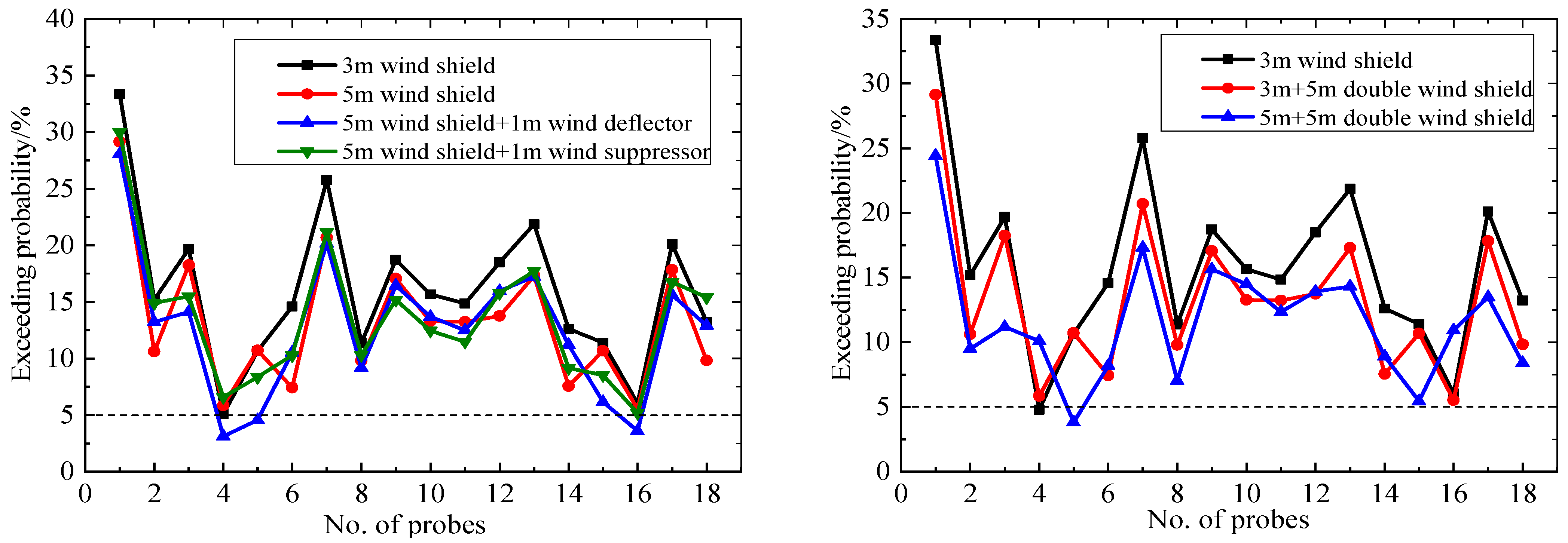
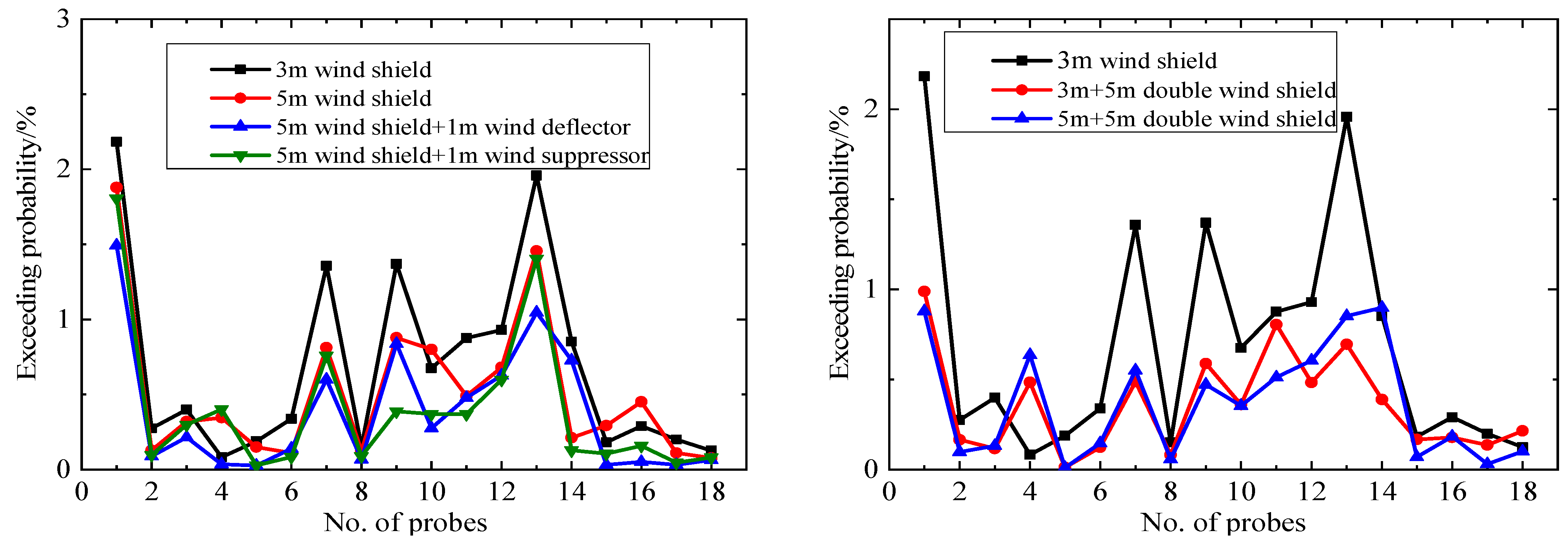
4.3. Assessment of the Pedestrian-Level Wind Environment
5. Conclusions
- The distributions of the pedestrian-level wind-speed ratio, Ri, for the outdoor platform with different aerodynamic measures are similar. Owing to the “funneling effects”, pedestrian-level Ri values in the area between the outer tower and the central tower are significantly higher, and the maximum Ri value is present in this area;
- The pedestrian-level wind-speed distributions of two typical aerodynamic measures (5 m wind shield + 1 m deflector and 5 m + 5 m double wind shields) are similar on the outdoor platform in the full model, and the wind-speed-adjustment coefficients, CR, of the most unfavorable wind environment platform in the full model are 1.15 and 1.1, respectively;
- The evaluation results based on the Lawson criterion show that different aerodynamic measures can effectively reduce the pedestrian-level wind speed and improve the wind environment, but a part of the platform cannot satisfy the requirements of the wind comfort and wind safety. Therefore, more aerodynamic measures, such as roadside trees or high-up shelters, should be further investigated to improve the wind environmental quality in the “wind danger” region;
- This study contributes to the provision of a new research method to study the wind environments of complex building structures by combining wind tunnel tests and CFD simulations with sub-configuration and full models. In addition, it should be noted that the pedestrian-level wind environments around outdoor platforms that are high up on high-rise buildings have been less investigated in previous studies, and this study contributes to the further understanding of the pedestrian-level wind environmental characteristics of outdoor platforms. For poorly performing wind comfort and wind safety on outdoor platforms, the aerodynamic measures proposed in this study can provide important references to improve the pedestrian-level wind environment in future studies and engineering applications.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Wise, A.F.E. Wind effects due to groups of buildings. In Proceedings of the Royal Society Symposium Architectural Aerodynamics Session 3: Effect of Buildings on the Local Wind, London, UK, July 1970; pp. 26–27. Available online: https://www.aivc.org/sites/default/files/members_area/medias/pdf/Airbase/airbase_00136.pdf (accessed on 11 January 2024).
- Lawson, T.V.; Penwarden, A.D. The effects of wind on people in the vicinity of buildings. Proceedings of 4th International Conference on Wind Effects on Buildings and Structures, September 1975; Cambridge University Press: Heathrow, UK, 1975; pp. 605–622. Available online: https://pascal-francis.inist.fr/vibad/index.php?action=getRecordDetail&idt=PASCAL7780305984 (accessed on 11 January 2024).
- Blocken, B.; Carmeliet, J. Pedestrian wind environment around buildings: Literature review and practical examples. Therm. Env. Build. Sci. 2004, 28, 107–159. [Google Scholar] [CrossRef]
- Isyumov, N.; Davenport, A.G. The ground level wind environment in built-up areas. Proceedings of Fourth International Conference on Wind Effects on Buildings and Structures, September 1975; Cambridge University Press: Heathrow, UK, 1975; pp. 403–422. Available online: https://books.google.com/books?hl=hu&lr=&id=pRY9AAAAIAAJ&oi=fnd&pg=RA7-PA43&ots=4kW40o_jDb&sig=sDebM4eWlSfdpi2t196qIlck0Aw (accessed on 11 January 2024).
- Hunt, J.C.R.; Poulton, E.C.; Mumford, J.C. The effects of wind on people; new criteria based on wind tunnel experiments. Build. Environ. 1976, 11, 15–28. [Google Scholar] [CrossRef]
- Lawson, T.V. The wind content of the built environment. J. Wind Eng. Ind. Aerod. 1978, 3, 93–105. [Google Scholar] [CrossRef]
- Melbourne, W.H. Criteria for environmental wind conditions. J. Wind Eng. Ind. Aerod. 1978, 3, 241–249. [Google Scholar] [CrossRef]
- Williams, C.J.; Soligo, M.J.; Cote, J. A discussion of the components for a comprehensive pedestrian level comfort criterion. J. Wind Eng. Ind. Aerod. 1992, 44, 2389–2390. [Google Scholar] [CrossRef]
- Soligo, M.J.; Irwin, P.A.; Williams, C.J.; Schuyler, G.D. A comprehensive assessment of pedestrian comfort including thermal effects. J. Wind Eng. Ind. Aerod. 1998, 77, 753–766. [Google Scholar] [CrossRef]
- Lawson, T.V. The Determination of the Wind Environment of a Building Complex before Construction; Department of Aerospace Engineering, University of Bristol: Bristol, UK, 1990; Report Number TVL9025. [Google Scholar]
- Lawson, T.V. Building Aerodynamics; Imperial College Press: London, UK, 2001. [Google Scholar]
- Koss, H.H. On differences and similarities of applied wind comfort criteria. J. Wind Eng. Ind. Aerod. 2006, 94, 781–797. [Google Scholar] [CrossRef]
- NEN 8100: 2006; Wind Comfort and Wind Danger in the Built Environment. Dutch Standard: Amsterdam, The Netherlands, 2006. (In Dutch)
- NPR 6097: 2006; Application of Mean Hourly Wind Velocity Statistics for the Netherlands. Dutch Practice Guideline: Amsterdam, The Netherlands, 2006. (In Dutch)
- Janssen, W.D.; Blocken, B.; Hooff, T.V. Pedestrian wind comfort around buildings: Comparison of wind comfort criteria based on whole-flow field data for a complex case study. Build. Environ. 2013, 59, 547–562. [Google Scholar] [CrossRef]
- Blocken, B.; Janssen, W.D.; Hooff, T.V. CFD simulation for pedestrian wind comfort and wind safety in urban areas: General decision framework and case study for the Eindhoven University campus. Environ. Modell. Softw. 2012, 30, 15–34. [Google Scholar] [CrossRef]
- Sasaki, R.; Uematsu, Y.; Yamada, M.; Saeki, H. Application of infrared thermography and a knowledge-based system to the evaluation of the pedestrian-level wind environment around buildings. J. Wind Eng. Ind. Aerod. 1997, 67–68, 873–883. [Google Scholar] [CrossRef]
- Tsang, C.W.; Kwok, K.C.S.; Hitchcock, P.A. Wind tunnel study of pedestrian level wind environment around tall buildings: Effects of building dimensions, separation and podium. Build. Environ. 2012, 49, 167–181. [Google Scholar] [CrossRef]
- Youssef, S.W. Experimental Evaluation of Pedestrian-Level Winds; University of Minnesota: Minneapolis, MN, USA, 1998. [Google Scholar]
- Blocken, B.; Roels, S.; Carmeliet, J. Modification of pedestrian wind comfort in the Silvertop Tower passages by an automatic control system. J. Wind Eng. Ind. Aerod. 2004, 92, 849–873. [Google Scholar] [CrossRef]
- Mohan, K.; Flay, R.G.J.; Gairola, A.; Mukherjee, M. Role of landscape elements in ameliorating adverse pedestrian level winds in the vicinity of tall buildings. Bonfring Int. J. Ind. Eng. Manag. Sci. 2012, 2, 41–50. [Google Scholar]
- Kaijima, S.; Bouffanais, R.; Willcox, K. Computational fluid dynamics for architectural design. In Proceedings of the 18th International Conference of Association of Computer-Aided Architectural Design Research in Asia, Hong Kong, China, November 2013; Available online: https://onlinelibrary.wiley.com/doi/10.1002/ad.1566 (accessed on 11 January 2024).
- Moya, R.; Prohasky, D.; Watkins, S.; Ding, Y.; Burry, J.; Burry, M. Aerodynamic strategy applied in an urban shelter design: Simulation and analysis of aerodynamic phenomena in an urban context. In Proceedings of the 32nd eCAADe Conference, Newcastle upon Tyne, UK, 10–12 September 2014; pp. 137–144. [Google Scholar]
- Zheng, C.R.; Li, Y.S.; Wu, Y. Pedestrian-level wind environment on outdoor platforms of a thousand-meter-scale megatall building: Sub-configuration experiment and wind comfort assessment. Build. Environ. 2016, 106, 313–326. [Google Scholar] [CrossRef]
- Revuz, J.; Hargreaves, D.M.; Owen, J.S. On the domain size for the steady-state CFD modelling of a tall building. Wind Struct. 2012, 15, 313–329. [Google Scholar] [CrossRef]
- GB 50009-2012; Load Code for the Design of Building Structures. China Architecture and Building Press: Beijing, China, 2012. (In Chinese)
- Shih, T.H.; Liou, W.W.; Shabbir, A.; Yang, Z.; Zhu, J. A new k-ε eddy-viscosity model for high Reynolds number turbulent flows: Model development and validation. Comput. Fluids 1995, 24, 227–238. [Google Scholar] [CrossRef]
- Irwin, P.A.; Baker, W.F.; Korista, S.; Weismantle, P.A.; Novak, L.C. The BURJ DUBAI TOWER wind tunnel testing of cladding and pedestrian level. Struct. Mag. 2006, 11, 47–51. [Google Scholar]
- Irwin, H. A simple omnidirectional sensor for wind-tunnel studies of pedestrian-level winds. J. Wind Eng. Ind. Aerod. 1981, 7, 219–239. [Google Scholar] [CrossRef]


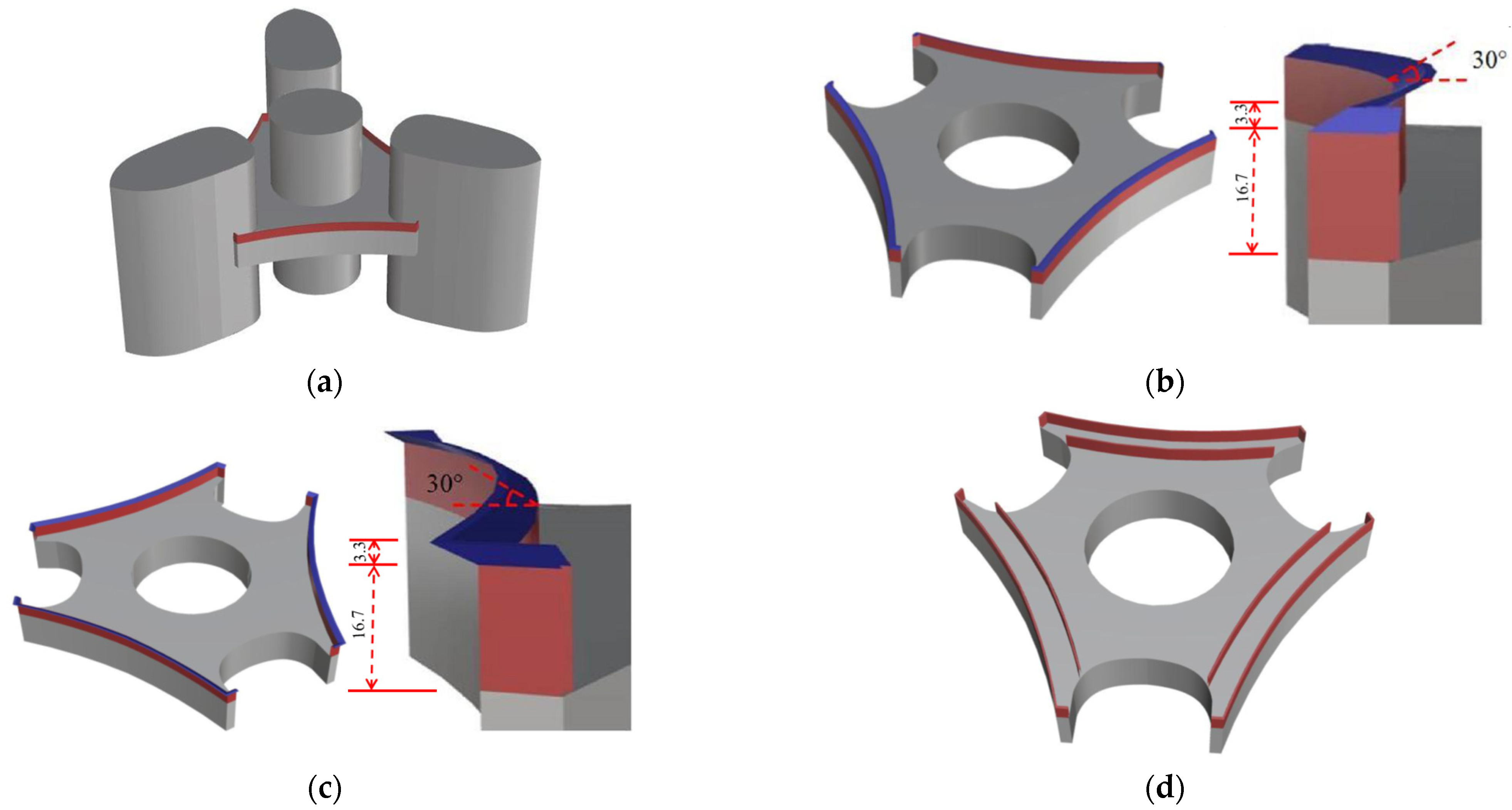




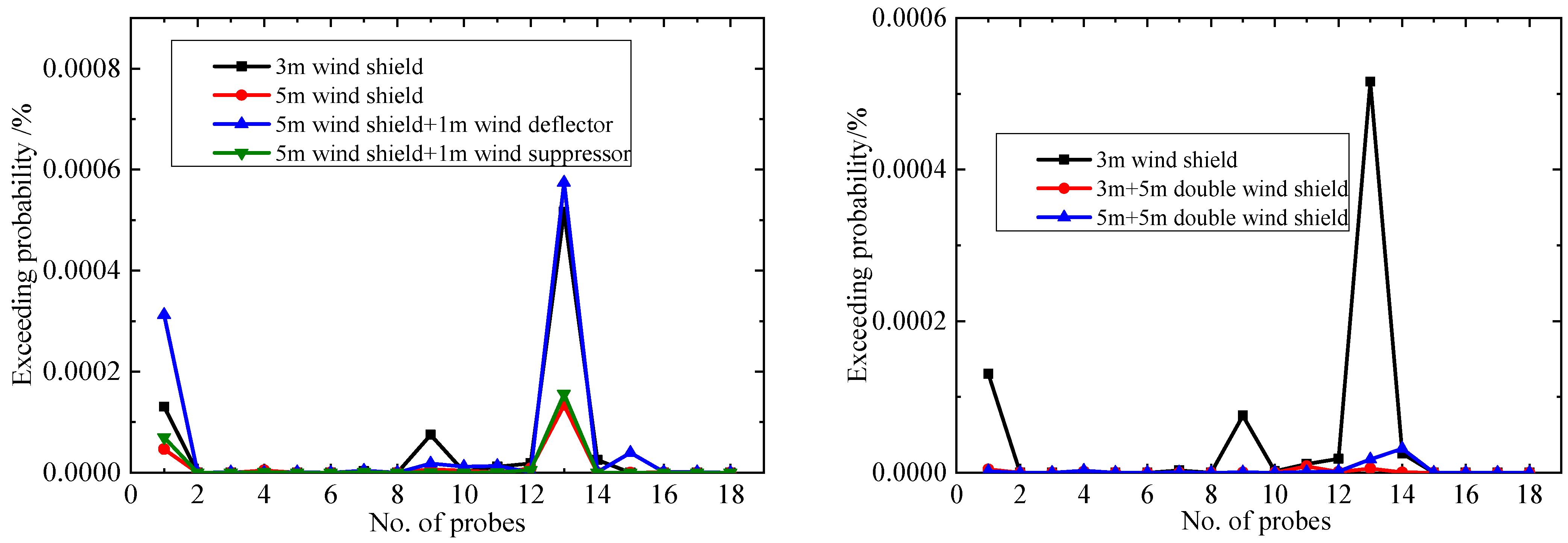
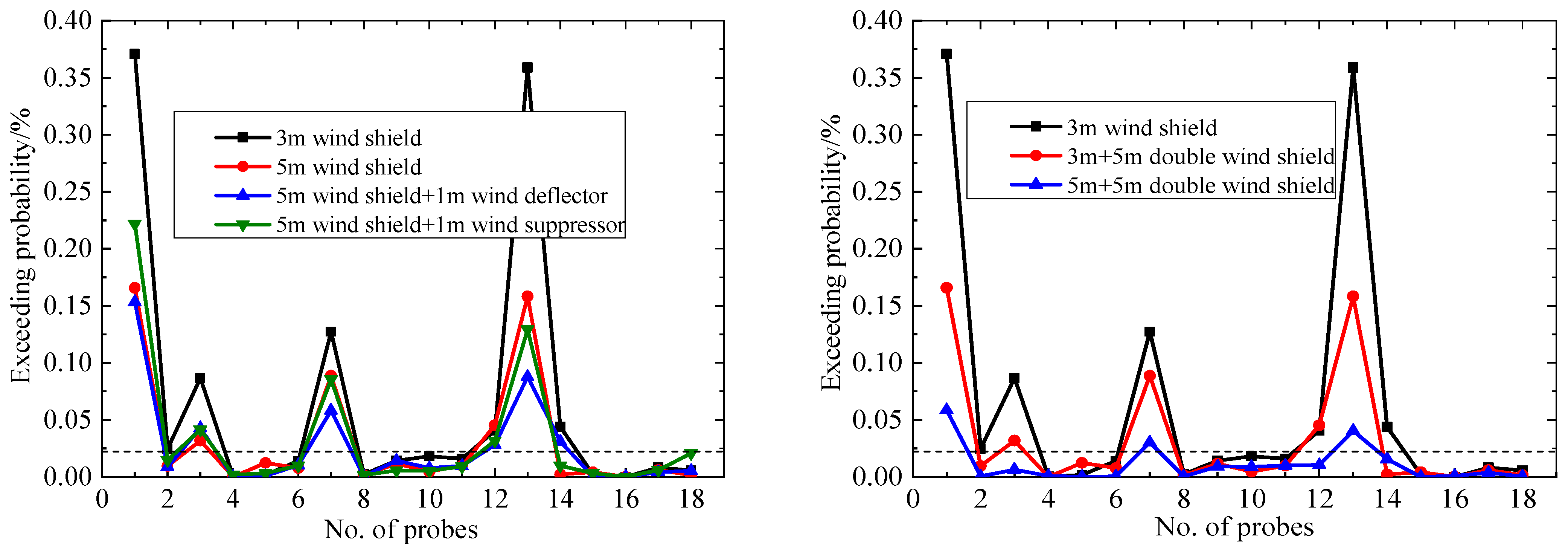

| Case No. | Different Aerodynamic Measures of Wind Shields | Wind Direction θ (°) |
|---|---|---|
| 1 | 5 m high wind shield | 0; 15; 30; 45; 60 |
| 2 | 5 m high wind shield plus 1 m high wind deflector | 0; 15; 30; 45; 60 |
| 3 | 5 m high wind shield plus 1 m high wind suppressor | 0; 15; 30; 45; 60 |
| 4 | 3 m high exterior wind shield plus 5 m high interior wind shield at a spacing of L = 9 m | 0; 15; 30; 45; 60 |
| 5 | 5 m high double wind shields at a spacing of L = 9 m | 0; 15; 30; 45; 60 |
| No. of Test Cases | 0 | 1 | 2 | 3 | 4 | 5 |
|---|---|---|---|---|---|---|
| maximum Rei | 1.49 | 1.40 | 1.26 | 1.28 | 1.25 | 1.29 |
| RFR | — | 6.04% | 15.43% | 14.09% | 16.11% | 13.42% |
| Test Case No. | 0° Wind Direction | 60° Wind Direction | ||||
|---|---|---|---|---|---|---|
| Maximum Error | Minimum Error | Average Error | Maximum Error | Minimum Error | Average Error | |
| 1 | 19.92% | 1.65% | 6.86% | 5.95% | 1.15% | 3.80% |
| 2 | 22.35% | 0.39% | 8.95% | 6.47% | 0.80% | 2.95% |
| 3 | 22.35% | 0.39% | 8.94% | 5.14% | 0.23% | 3.18% |
| 4 | 26.26% | 2.12% | 9.72% | 9.90% | 5.69% | 7.80% |
| 5 | 27.71% | 13.54% | 21.64% | 14.57% | 10.48% | 12.52% |
| Pedestrian Activity | Uthr (m/s) | Pmax (U > Uthr) | |
|---|---|---|---|
| Wind comfort | Sitting long | 4 | 5% |
| Sitting short | 6 | 5% | |
| Strolling | 8 | 5% | |
| Walking fast | 10 | 5% | |
| Wind danger | 20 | 0.022% | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, Z.; Zheng, C.; Lu, D.; Wang, Y.; Chen, Y.; Jin, Z.; Zhang, Z. Effects of Wind Shields on Pedestrian-Level Wind Environment around Outdoor Platforms of a Megatall Building. Atmosphere 2024, 15, 171. https://doi.org/10.3390/atmos15020171
Liu Z, Zheng C, Lu D, Wang Y, Chen Y, Jin Z, Zhang Z. Effects of Wind Shields on Pedestrian-Level Wind Environment around Outdoor Platforms of a Megatall Building. Atmosphere. 2024; 15(2):171. https://doi.org/10.3390/atmos15020171
Chicago/Turabian StyleLiu, Zhao, Chaorong Zheng, Dagang Lu, Yijing Wang, Yong Chen, Zhao Jin, and Zhiming Zhang. 2024. "Effects of Wind Shields on Pedestrian-Level Wind Environment around Outdoor Platforms of a Megatall Building" Atmosphere 15, no. 2: 171. https://doi.org/10.3390/atmos15020171
APA StyleLiu, Z., Zheng, C., Lu, D., Wang, Y., Chen, Y., Jin, Z., & Zhang, Z. (2024). Effects of Wind Shields on Pedestrian-Level Wind Environment around Outdoor Platforms of a Megatall Building. Atmosphere, 15(2), 171. https://doi.org/10.3390/atmos15020171








