Comparison of Atmospheric Ionization for Solar Proton Events of the Last Three Solar Cycles
Abstract
1. Introduction
2. Data and Methods
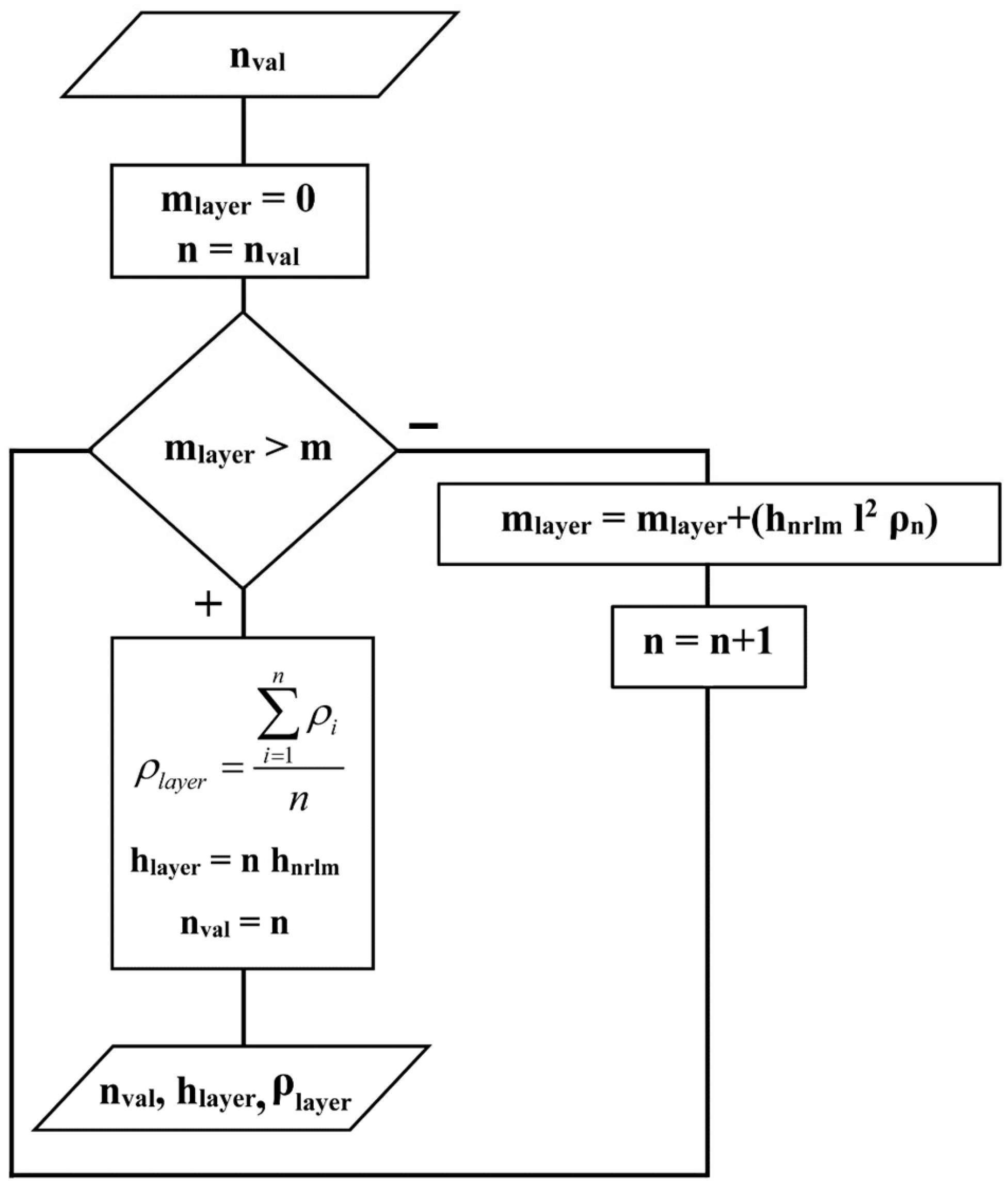
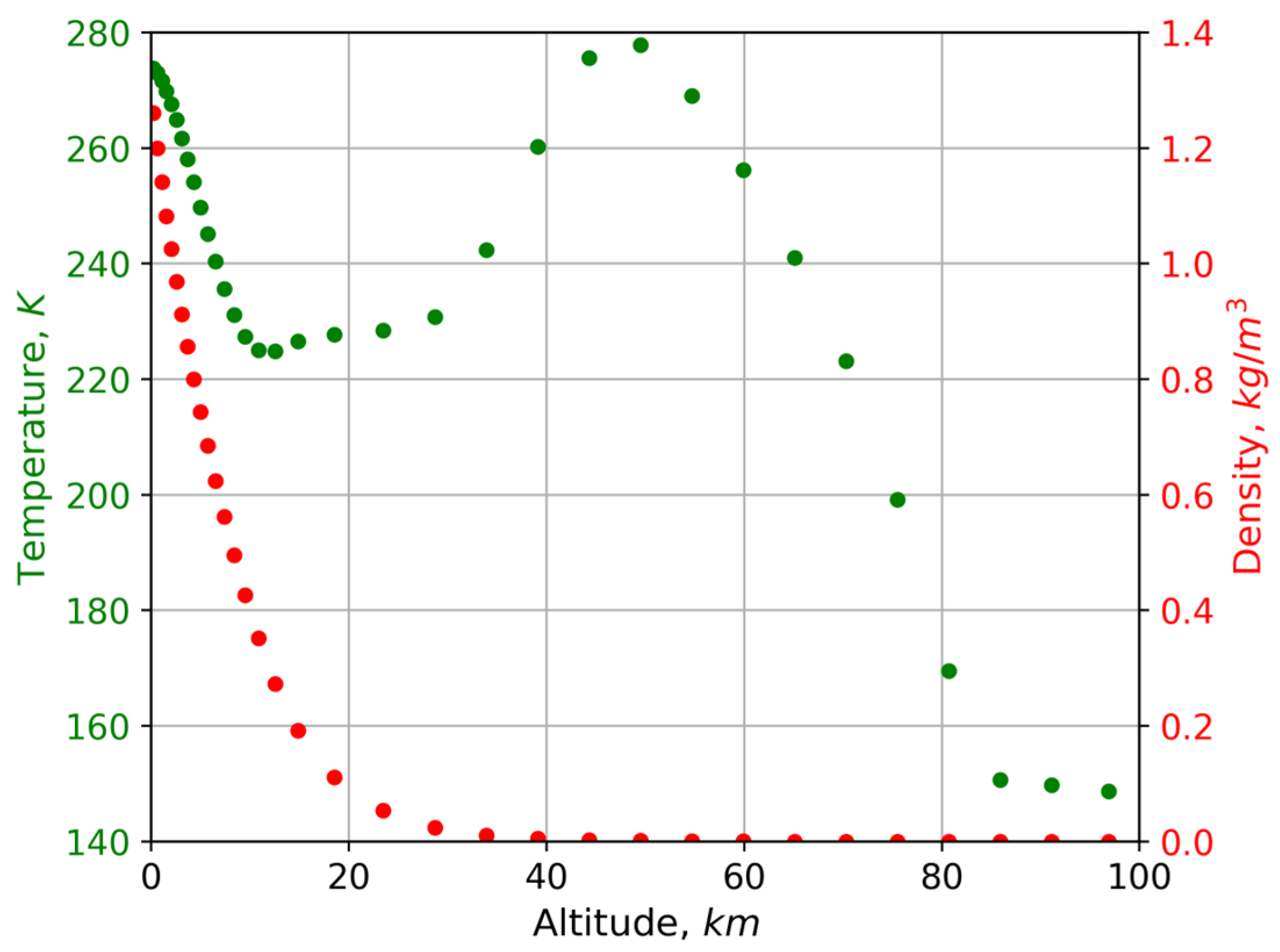
2.1. Geometry Implementation
2.2. Physics Implementation and Ionization Rate Detector Features
2.3. Primary Particle Source
- A random number is generated with a uniform probability distribution density.
- The comparison of and for is conducted until the condition occurs. In the case of negative comparison result, the following operations are carried out next: and .
- The corresponding value for is selected.
2.4. Primary CR Protons’ Spectra
3. Results and Discussion
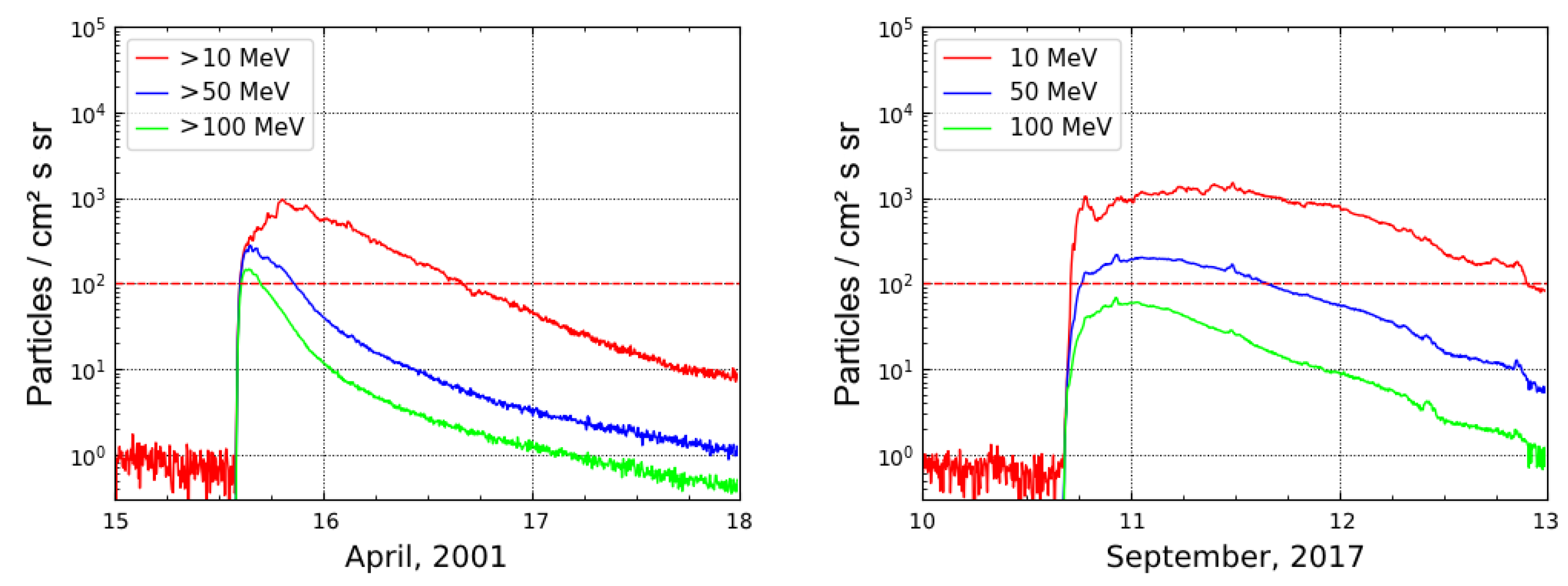
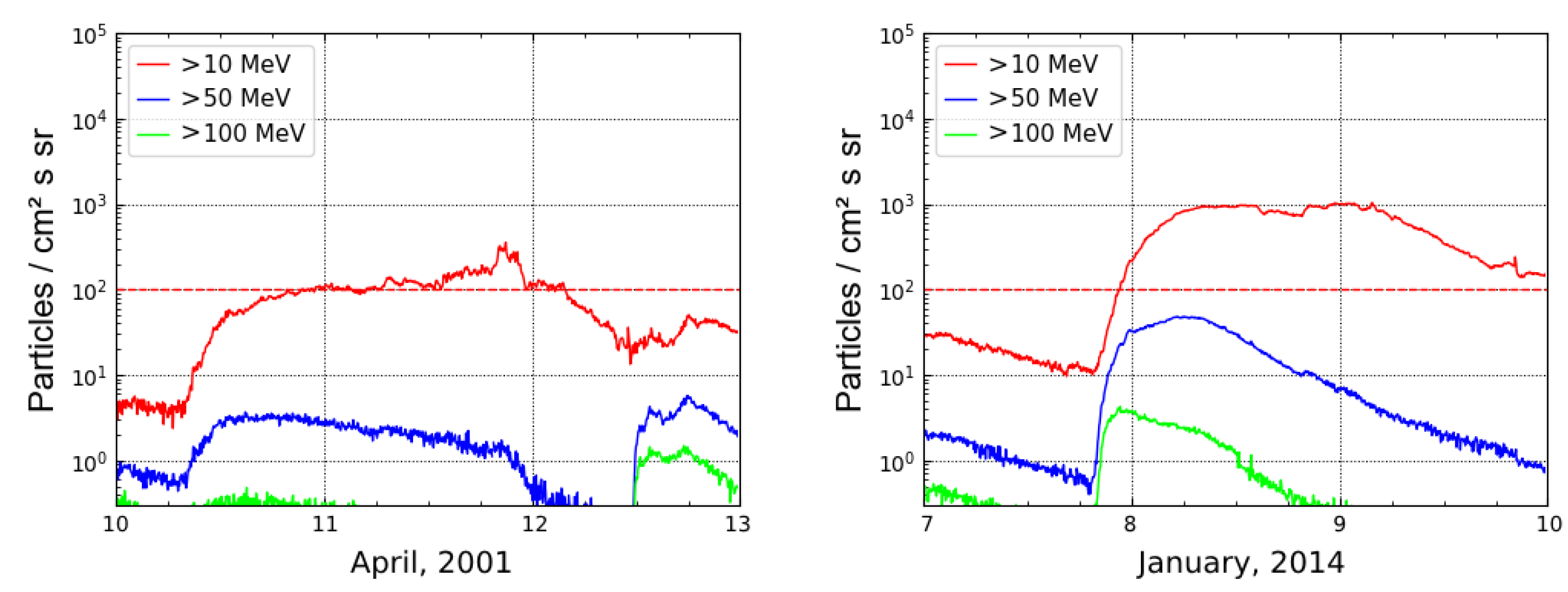
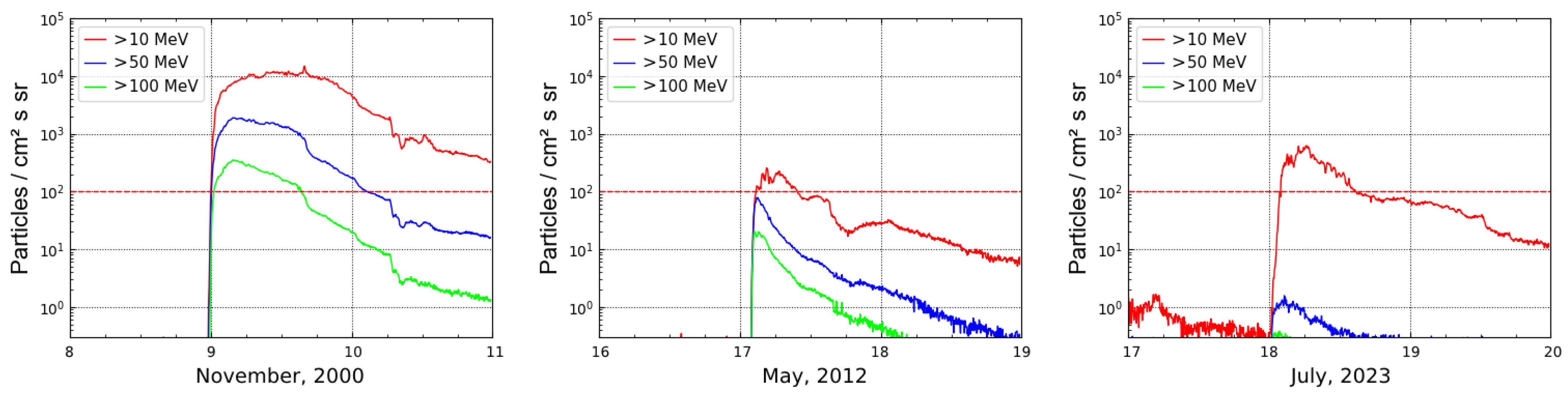
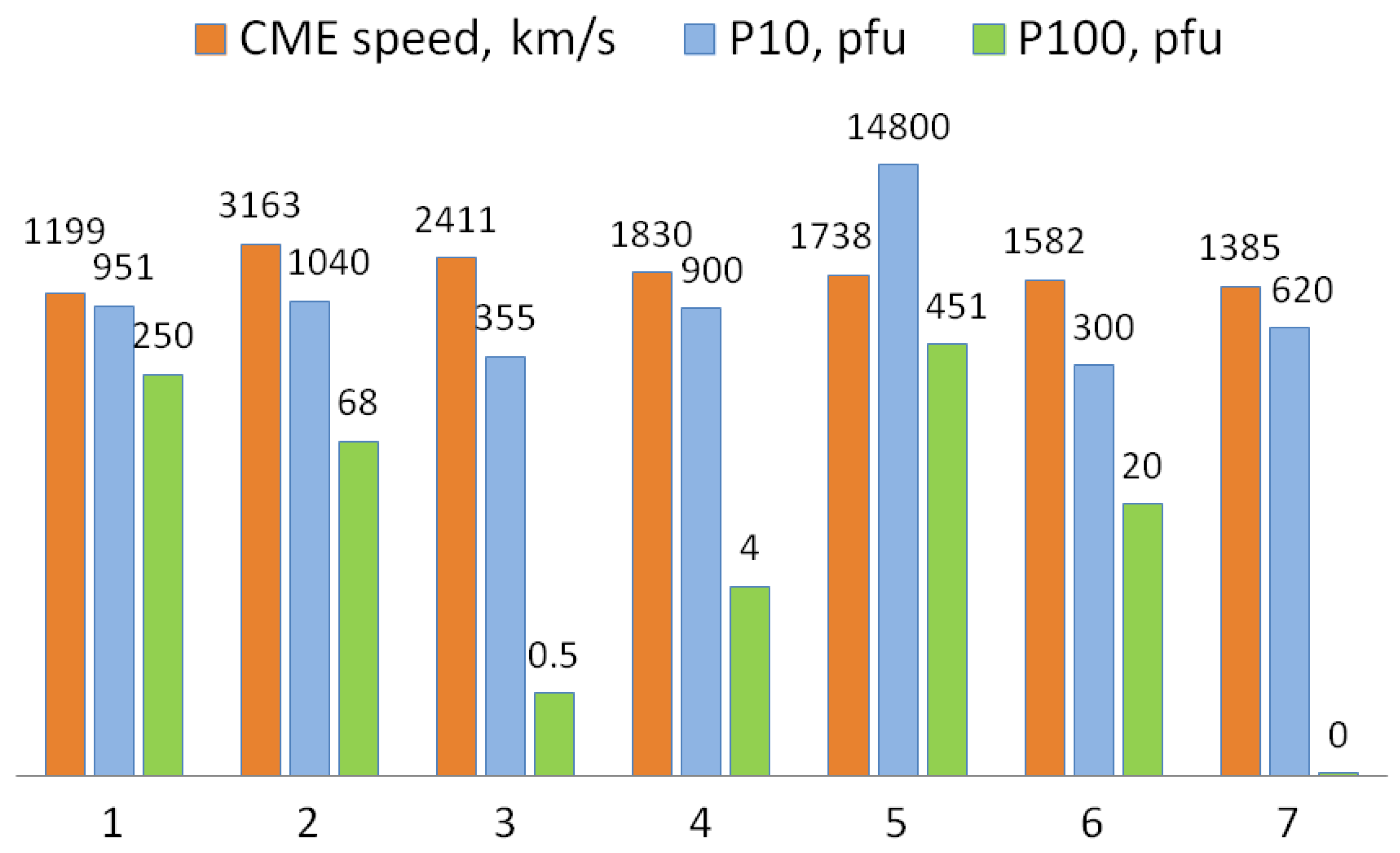
3.1. Description of Events
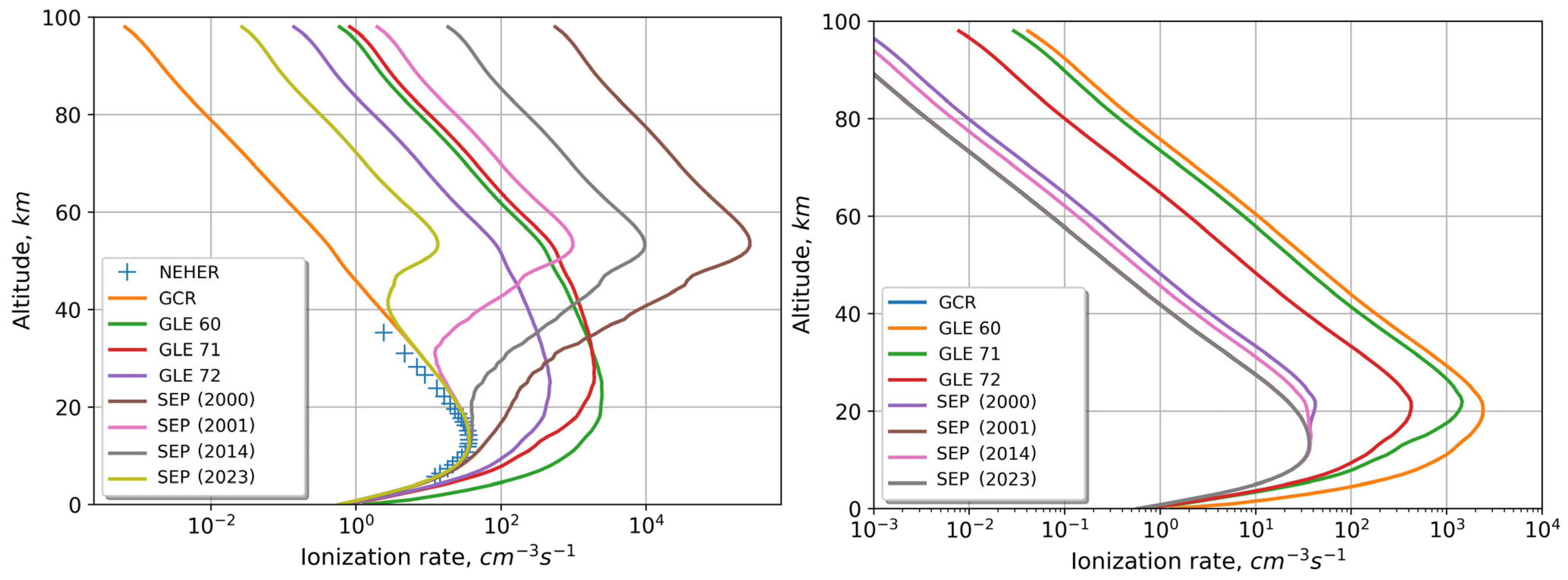
3.2. Calculated Ionization Rate
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Dorman, L. Cosmic Ray Interactions, Propagation, and Acceleration in Space Plasmas; Springer: Dordrecht, The Netherlands, 2006; 847p. [Google Scholar]
- Bazilevskaya, G.A.; Usoskin, I.G.; Flückiger, E.O.; Harrison, R.G.; Desorgher, L.; Bütikofer, R.; Krainev, M.B.; Makhmutov, V.S.; Stozhkov, Y.I.; Svirzhevskaya, A.K.; et al. Cosmic Ray Induced Ion Production in the Atmosphere. Space Sci. Rev. 2008, 137, 149–173. [Google Scholar] [CrossRef]
- Mironova, I.A.; Aplin, K.L.; Arnold, F.; Bazilevskaya, G.A.; Harrison, R.G.; Krivolutsky, A.A.; Nicoll, K.A.; Rozanov, E.V.; Turunen, E.; Usoskin, I.G. Energetic Particle Influence on the Earth’s Atmosphere. Space Sci. Rev. 2015, 194, 1–96. [Google Scholar] [CrossRef]
- Usoskin, I.G.; Kovaltsov, G.A. Cosmic Ray Induced Ionization in the Atmosphere: Full Modeling and Practical Applications. J. Geophys. Res. 2006, 111, D21206. [Google Scholar] [CrossRef]
- Jackman, C.H.; Deland, M.T.; Labow, G.J.; Fleming, E.L.; Weisenstein, D.K.; Ko, M.K.W.; Sinnhuber, M.; Anderson, J.; Russell, J.M. The influence of the several very large solar proton events in years 2000–2003 on the neutral middle atmosphere. Adv. Space Res. 2005, 35, 445–450. [Google Scholar] [CrossRef][Green Version]
- Kirillov, A.S.; Belakhovsky, V.B.; Maurchev, E.A.; Balabin, Y.V.; Germanenko, A.V.; Gvozdevsky, B.B. Vibrational Kinetics of NO and N2 in the Earth’s Middle Atmosphere During GLE69 on 20 January 2005. J. Geophys. Res. Atmos. 2003, 128, e2023JD038600. [Google Scholar] [CrossRef]
- Funke, B.; Baumgaertner, A.; Calisto, M.; Egorova, T.; Jackman, C.H.; Kieser, J.; Krivolutsky, A.; López-Puertas, M.; Marsh, D.R.; Reddmann, T.; et al. Composition changes after the ‘‘Halloween” solar proton event: The High Energy Particle Precipitation in the Atmosphere (HEPPA) model versus MIPAS data intercomparison study. Atmos. Chem. Phys. 2011, 11, 9089–9139. [Google Scholar] [CrossRef]
- Calisto, M.; Usoskin, I.; Rozanov, E. Influence of a Carrington-like event on the atmospheric chemistry, temperature and dynamics: Revised. Environ. Res. Lett. 2013, 8, 045010. [Google Scholar] [CrossRef]
- Dmitriev, A.V.; Jayachandran, P.T.; Tsai, L.-C. Elliptical model of cutoff boundaries for the solar energetic particles measured by POES satellites in December 2006. J. Geophys. Res. 2010, 115, A12244. [Google Scholar] [CrossRef]
- Vashenyuk, E.V.; Balabin, Y.V.; Gvozdevsky, B.B. Features of relativistic solar proton spectra derived from ground level enhancement events (GLE) modeling. Astrophys. Space Sci. Trans. 2011, 7, 459–463. [Google Scholar] [CrossRef]
- Belov, A.V. Flares, ejections, proton events. Geomagn. Aeron. 2017, 57, 727–737. [Google Scholar] [CrossRef]
- Maurchev, E.A.; Balabin, Y.V. RUSCOSMICS—The new software toolbox for detailed analysis of cosmic ray interactions with matter. Solar–Terr. Phys. 2016, 2, 3–10. [Google Scholar]
- Agostinelli, S.; Allison, J.; Amako, K.; Apostolakis, J.; Araujo, H.; Arce, P.; Asai, M.; Axen, D.; Banerjee, S.; Barrand, G.J.N.I.; et al. GEANT4—A simulation toolkit. Nucl. Instrum. Methods Phys. Res. Sec. A Terr. Accel. Spectrometers Detect. Assoc. Equip. 2003, 506, 250–303. [Google Scholar] [CrossRef]
- Allison, J.; Amak, K.; Apostolakis, J.; Arce, P.; Asai, M.; Aso, T.; Bagli, E.; Bagulya, A.; Banerjee, S.; Barrand, G.J.N.I.; et al. Recent developments in Geant4. Nucl. Instrum. Methods Phys. Res. Sec. A Terr. Accel. Spectrometers Detect. Assoc. Equip. 2016, 835, 186–225. [Google Scholar] [CrossRef]
- Picone, J.M.; Hedin, A.E.; Drob, D.P.; Aikin, A.C. NRLMSISE-00 empirical model of the atmosphere: Statistical comparison and scientific issues. J. Geophys. Res. 2002, 107, 1468. [Google Scholar] [CrossRef]
- Heikkinen, A.; Stepanov, N.; Wellish, J.P. Bertini intra–nuclear cascade implementation in Geant4. In Proceedings of the 13th International Conference on Computing in High-Enery and Nuclear Physics (CHEP 2003), La Jolla, CA, USA, 24–28 March 2003. [Google Scholar]
- Amelin, N.S.; Armesto, N. Monte Carlo model for nuclear collisions from SPS to LHC energies. Eur. Phys. J. C—Part. Fields 2001, 22, 149–163. [Google Scholar] [CrossRef]
- Amelin, N.S.; Gudima, K.K.; Toneev, V.D. Quark—Gluon String Model and Ultrarelativistic Heavy Ion Interactions. Sov. J. Nucl. Phys. 1990, 51, 327–333. [Google Scholar]
- Garny, S.; Leuthold, G.; Mares, V.; Paretzke, H.G.; Ruhm, W. GEANT4 Transport Calculations for Neutrons and Photons below 15 MeV. IEEE Trans. Nuclear Sci. 2009, 56, 2392–2396. [Google Scholar] [CrossRef]
- Maurchev, E.A.; Balabin, Y.V.; Germanenko, A.V. Compact Geiger Counters as Additional Tools for Verifying Models of Cosmic Ray Transport through the Earth’s Atmosphere. Bull. Russ. Acad. Sci. Phys. 2021, 85, 1294–1296. [Google Scholar] [CrossRef]
- GOST 25645.122-85; Protons of Galactic Cosmic Rays. USSR State Committee for Standards: Moscow, Russia, 1985; 11p. (In Russian)
- Adriani, O.; Barbarino, G.C.; Bazilevskaya, G.A. PAMELA Measurements of Cosmic–Ray Proton and Helium Spectra. Science 2011, 332, 69–72. [Google Scholar] [CrossRef]
- Adriani, O.; Barbarino, G.C.; Bazilevskaya, G.A. Time dependence of the proton flux measured by PAMELA during 2006 July–2009 December solar minimum. Astrophys. J. 2013, 765, 1–8. [Google Scholar] [CrossRef]
- Maurchev, E.; Shlyk, N.; Abunina, M.; Abunin, A.; Belov, A.; Didenko, K. A Method for the Ambient Equivalent Dose Estimation in a Wide Range of Altitudes during SEP and GLE Events. Atmosphere 2024, 15, 92. [Google Scholar] [CrossRef]
- Balabin, Y.V.; Gvozdevsky, B.B.; Germanenko, A.V.; Maurchev, E.A. GLE events in 24th solar cycle. E3S Web Conf. 2018, 62, 01006. [Google Scholar] [CrossRef]
- Kahler, S.W. The correlation between solar energetic particle peak intensities and speeds of coronal mass ejections: Effects of ambient particle intensities and energy spectra. J. Geophys. Res. Space Phys. 2001, 106, 20947–20956. [Google Scholar] [CrossRef]
- Kurt, V.; Belov, A.; Mavromichalaki, H.; Gerontidou, M. Statistical analysis of solar proton events. Ann. Geophys. 2004, 22, 2255–2271. [Google Scholar] [CrossRef]
- Belov, A.; Garcia, H.; Kurt, V.; Mavromichalaki, H.; Gerontidou, M. Proton Enhancements and Their Relation to the X-ray Flares During the Three Last Solar Cycles. Solar Phys. 2005, 229, 135–159. [Google Scholar] [CrossRef]
- Wang, R.G. Statistical characteristics of solar energetic proton events from January 1997 to June 2005. Astropart. Phys. 2006, 26, 202–208. [Google Scholar] [CrossRef]
- Cliver, E.W.; Ling, A.G.; Belov, A.; Yashiro, S. Size distributions of solar flares and solar energetic particle events. Astrophys. J. Lett. 2012, 756, L29. [Google Scholar] [CrossRef]
- Lario, D.; Aran, A.; Gómez-Herrero, R.; Dresing, N.; Heber, B.; Ho, G.C.; Decker, R.B.; Roelof, E.C. Longitudinal and Radial Dependence of Solar Energetic Particle Peak Intensities: STEREO, ACE, SOHO, GOES, and MESSENGER Observations. Astrophys. J. 2013, 767, 41. [Google Scholar] [CrossRef]
- Dierckxsens, M.; Tziotziou, K.; Dalla, S.; Patsou, I.; Marsh, M.S.; Crosby, N.B.; Malandraki, O.; Tsiropoula, G. Relationship between Solar Energetic Particles and Properties of Flares and CMEs: Statistical Analysis of Solar Cycle 23 Events. Solar Phys. 2015, 290, 841–874. [Google Scholar] [CrossRef]
- Zhang, J.; Temmer, M.; Gopalswamy, N.; Malandraki, O.; Nitta, N.V.; Patsourakos, S.; Shen, F.; Vršnak, B.; Wang, Y.; Webb, D.; et al. Earth-affecting solar transients: A review of progresses in solar cycle 24. Prog. Earth Planet. Sci. 2021, 8, 56. [Google Scholar] [CrossRef]
- Johnson, A.S.; Golightly, M.J.; Lin, T.; Semones, E.J.; Shelfer, T.; Weyland, M.D.; Zapp, E.N. A comparison of measurements and predictions for the April 15 and April 18, 2001 solar proton events. Adv. Space Res. 2006, 37, 1678–1684. [Google Scholar] [CrossRef]
- Plainaki, C.; Mavromichalaki, H.; Belov, A.; Eroshenko, E.; Andriopoulou, M.; Yanke, V. A New Version of the Neutron Monitor Based Anisotropic GLE Model: Application to GLE60. Solar Phys. 2010, 264, 239–254. [Google Scholar] [CrossRef][Green Version]
- Kocharov, L.; Mishev, A.; Riihonen, E.; Vainio, R.; Usoskin, I. A Comparative Study of Ground-level Enhancement Events of Solar Energetic Particles. Astrophys. J. 2023, 958, 122. [Google Scholar] [CrossRef]
- Kurt, V.; Belov, A.; Kudela, K.; Yushkov, B. Some characteristics of GLE on 2017 September 10. Contrib. Astron. Obs. Skalnate Pleso 2018, 48, 329–338. [Google Scholar]
- Cohen, C.M.S.; Mewaldt, R.A. Energetic Particle Events of Cycle 24. Space Weather 2018, 16, 1616–1623. [Google Scholar] [CrossRef]
- de Koning, C.A.; Pizzo, V.J.; Seaton, D.B. The Solar Eruption of 2017 September 10: Wavy with a Chance of Protons. Astrophys. J. 2022, 924, 106. [Google Scholar] [CrossRef]
- Asai, A.; Ishii, T.T.; Kurokawa, H.; Yokoyama, T.; Shimojo, M. Evolution of Conjugate Footpoints inside Flare Ribbons during a Great Two-Ribbon Flare on 2001 April 10. Astrophys. J. 2003, 586, 624–629. [Google Scholar] [CrossRef]
- Wang, H.-M.; Song, H.; Jing, J.; Yurchyshyn, V.; Deng, Y.-Y.; Zhang, H.-Q.; Falconer, D.; Li, J. The Relationship between Magnetic Gradient and Magnetic Shear in Five Super Active Regions Producing Great Flares. Chin. J. Astron. Astrophys. 2006, 6, 477–488. [Google Scholar] [CrossRef]
- Pohjolainen, S.; Al-Hamadani, F.; Valtonen, E. Propagation of Solar Energetic Particles During Multiple Coronal Mass Ejection Events. Solar Phys. 2016, 291, 487–511. [Google Scholar] [CrossRef][Green Version]
- Belov, A.V.; Belova, E.A.; Shlyk, N.S.; Abunina, M.A.; Abunin, A.A. Geoefficiency of Sporadic Phenomena in Solar Cycle 24. Geomagn. Aeron. 2023, 63, 486–496. [Google Scholar] [CrossRef]
- Thakur, N.; Gopalswamy, N.; Mäkelä, P.; Akiyama, S.; Yashiro, S.; Xie, H. Two Exceptions in the Large SEP Events of Solar Cycles 23 and 24. Solar Phys. 2016, 291, 513–530. [Google Scholar] [CrossRef]
- Wang, Y.; Lyu, D.; Qin, G.; Xiao, B. The Effects of Magnetic Boundary on the Uniform Distribution of Energetic Particle Intensities Observed by Multiple Spacecraft. Astrophys. J. 2021, 913, 66. [Google Scholar] [CrossRef]
- Firoz, K.A.; Gan, W.Q.; Li, Y.P.; Rodriguez-Pacheco, J. On the possible mechanism of the first ground level enhancement in cosmic ray intensity of solar cycle 24. Astrophys. Space Sci. 2014, 350, 21–32. [Google Scholar] [CrossRef]
- Neher, H.V. Cosmic rays at high latitudes and altitudes covering four solar maxima. J. Geophys. Res. 1971, 76, 1637–1651. [Google Scholar] [CrossRef]

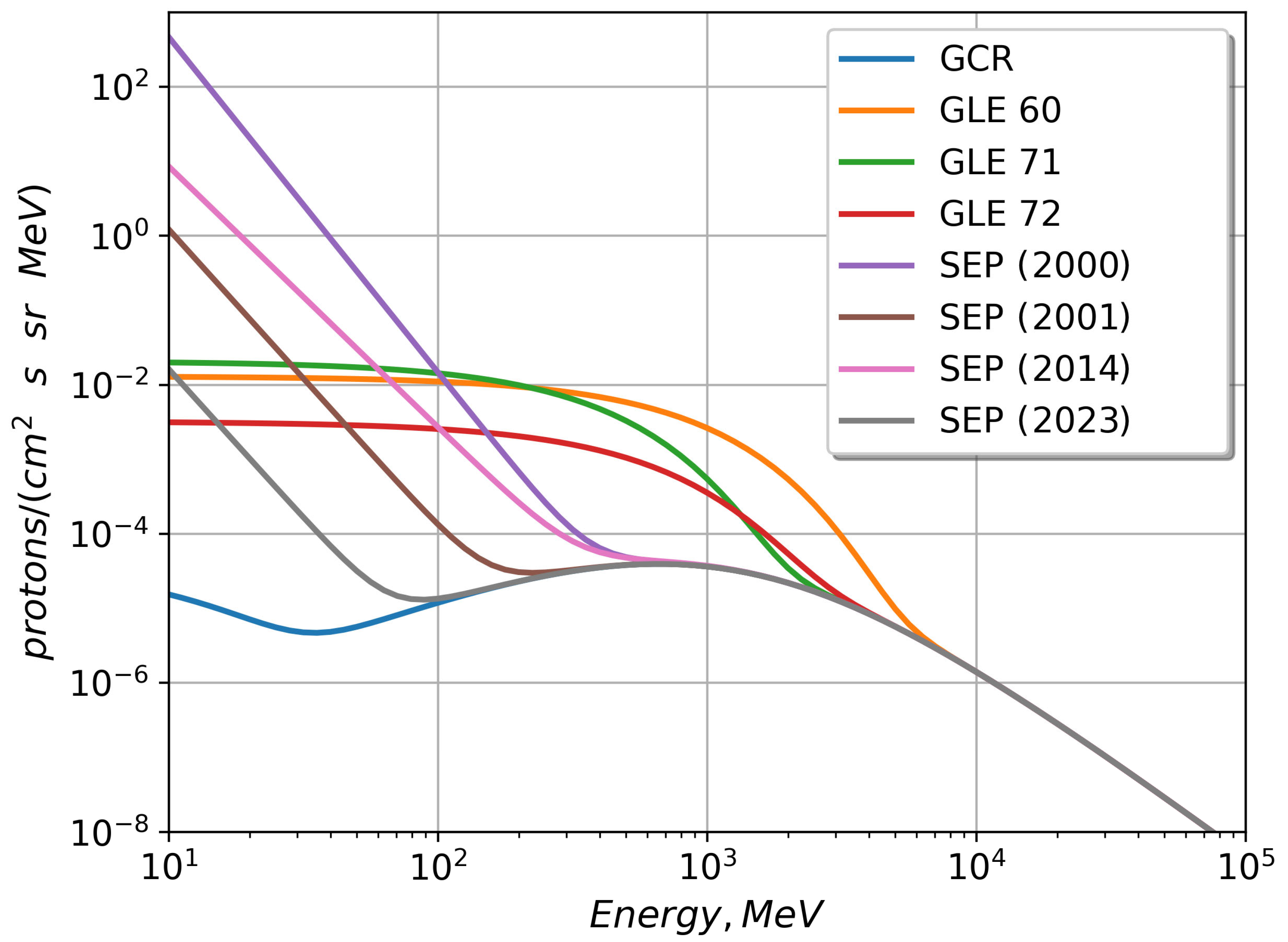
| D | B | C | α | E0 | |
|---|---|---|---|---|---|
| Even cycle minimum | 16 | 8 | 1.1 | 1.3 | 10 |
| Odd cycle minimum | 5.2 | 6.2 | 2 | 1.4 | 20 |
| Cycle maximum | 9.1 | 13 | 0.4 | 1.35 | 10 |
| Group | Event | SC | X-Flare Date and Time | X-Flare Class | Flare Coordinates | CME Date and Time | CME Speed, km/s | P10, pfu | P100, pfu | GLE, % | Remarks |
|---|---|---|---|---|---|---|---|---|---|---|---|
| I | 1 | 23 | 15 April 2001 13:19 | X14.4 | S20W85 | 15 April 2001 14:06 | 1199 | 951 | 250 | 237 | GLE60 |
| 2 | 24 | 10 September 2017 15:35 | X8.2 | S8W88 | 10 September 2017 16:00 | 3163 | 1040 | 68 | 6 | GLE72 | |
| II | 3 | 23 | 10 April 2001 05:06 | X2.3 | S23W09 | 10 April 2001 05:30 | 2411 | 355 | 0.5 | 0 | |
| 4 | 24 | 7 January 2014 18:04 | X1.2 | S15W11 | 7 January 2014 18:24 | 1830 | 900 | 4 | 0 | ||
| III | 5 | 23 | 8 November 2000 22:42 | M7.4 | N10W77 | 8 November 2000 23:06 | 1738 | 14800 | 451 | 0.1 | Sub-GLE |
| 6 | 24 | 17 May 2012 01:25 | M5.1 | N11W76 | 17 May 2012 01:48 | 1582 | 300 | 20 | 15 | GLE71 | |
| 7 | 25 | 17 July 2023 23:37 | M5.7 | S26W87 | 17 July 2023 23:36 | 1385 | 620 | 0 | 0 |
| Altitude, km | GCR | GLE60 | GLE71 | GLE72 | SEP (2000) | SEP (2001) | SEP (2014) | SEP (2023) |
|---|---|---|---|---|---|---|---|---|
| 10 | 31.6 | 793.9 | 177.4 | 114.3 | 41.4 | 31.6 | 32.6 | 31.6 |
| 15 | 35.8 | 1738.4 | 603.8 | 267.6 | 81.9 | 36.0 | 40.0 | 35.8 |
| 20 | 26.5 | 2382.6 | 1324.0 | 416.3 | 135.7 | 27.1 | 39.3 | 26.5 |
| 25 | 16.0 | 2450.2 | 1904.8 | 475.3 | 228.5 | 17.5 | 55.2 | 16.0 |
| 30 | 8.6 | 1977.6 | 1867.6 | 408.7 | 514.0 | 12.8 | 108.5 | 8.6 |
| 35 | 4.4 | 1490.9 | 1623.7 | 322.8 | 1984.7 | 19.0 | 298.0 | 4.6 |
| 40 | 2.2 | 1062.2 | 1278.6 | 237.7 | 7853.4 | 50.6 | 806.7 | 2.9 |
| 45 | 1.1 | 735.5 | 949.8 | 168.5 | 34,041.1 | 167.8 | 2221.1 | 3.3 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Maurchev, E.A.; Shlyk, N.S.; Dmitriev, A.V.; Abunina, M.A.; Didenko, K.A.; Abunin, A.A.; Belov, A.V. Comparison of Atmospheric Ionization for Solar Proton Events of the Last Three Solar Cycles. Atmosphere 2024, 15, 151. https://doi.org/10.3390/atmos15020151
Maurchev EA, Shlyk NS, Dmitriev AV, Abunina MA, Didenko KA, Abunin AA, Belov AV. Comparison of Atmospheric Ionization for Solar Proton Events of the Last Three Solar Cycles. Atmosphere. 2024; 15(2):151. https://doi.org/10.3390/atmos15020151
Chicago/Turabian StyleMaurchev, Eugene A., Nataly S. Shlyk, Alexey V. Dmitriev, Maria A. Abunina, Kseniia A. Didenko, Artem A. Abunin, and Anatoly V. Belov. 2024. "Comparison of Atmospheric Ionization for Solar Proton Events of the Last Three Solar Cycles" Atmosphere 15, no. 2: 151. https://doi.org/10.3390/atmos15020151
APA StyleMaurchev, E. A., Shlyk, N. S., Dmitriev, A. V., Abunina, M. A., Didenko, K. A., Abunin, A. A., & Belov, A. V. (2024). Comparison of Atmospheric Ionization for Solar Proton Events of the Last Three Solar Cycles. Atmosphere, 15(2), 151. https://doi.org/10.3390/atmos15020151







