Large Eddy Simulation of Flow Around Twin Tower Buildings in Tandem Arrangements with Upstream Corner Modification
Abstract
1. Introduction
2. Numerical Simulation Method
2.1. Governing Equations
2.2. Turbulent IBC Generation Method for LES
3. Modeling and Solution Parameter Settings
4. Results and Discussion
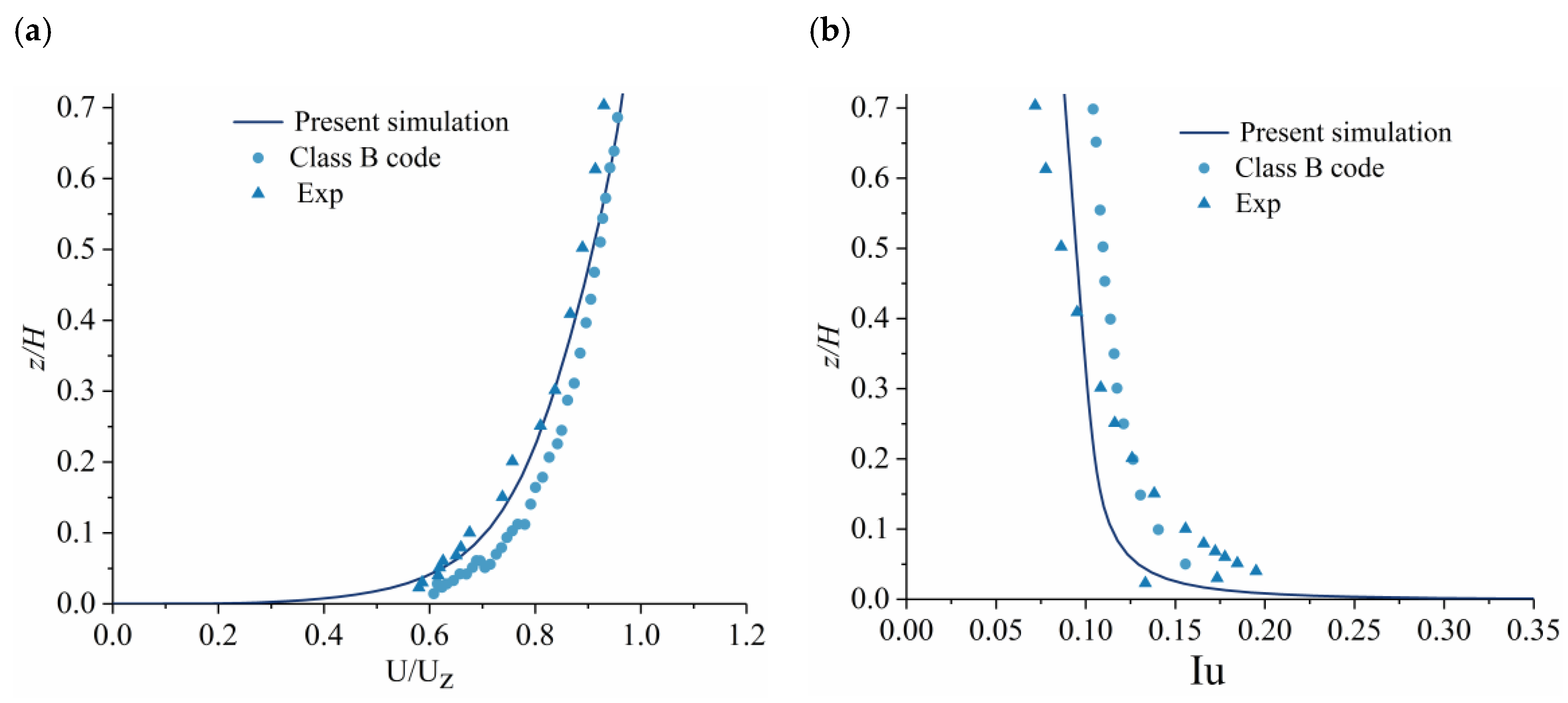
4.1. Validation of the Present Numerical Methods
4.2. Wind Pressure Coefficients
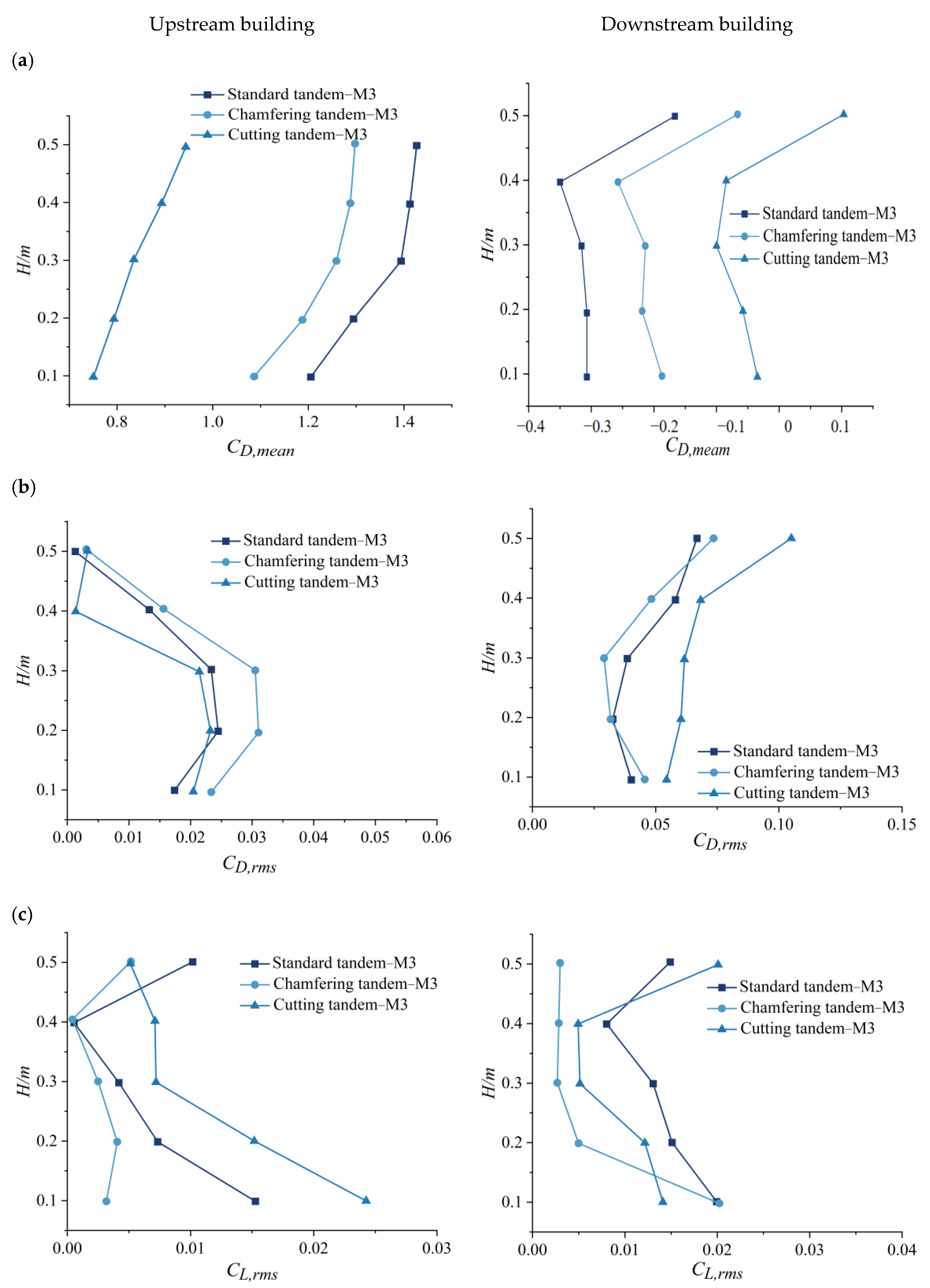
4.3. The Aerodynamic Force Coefficients
4.4. Flow Field Analysis
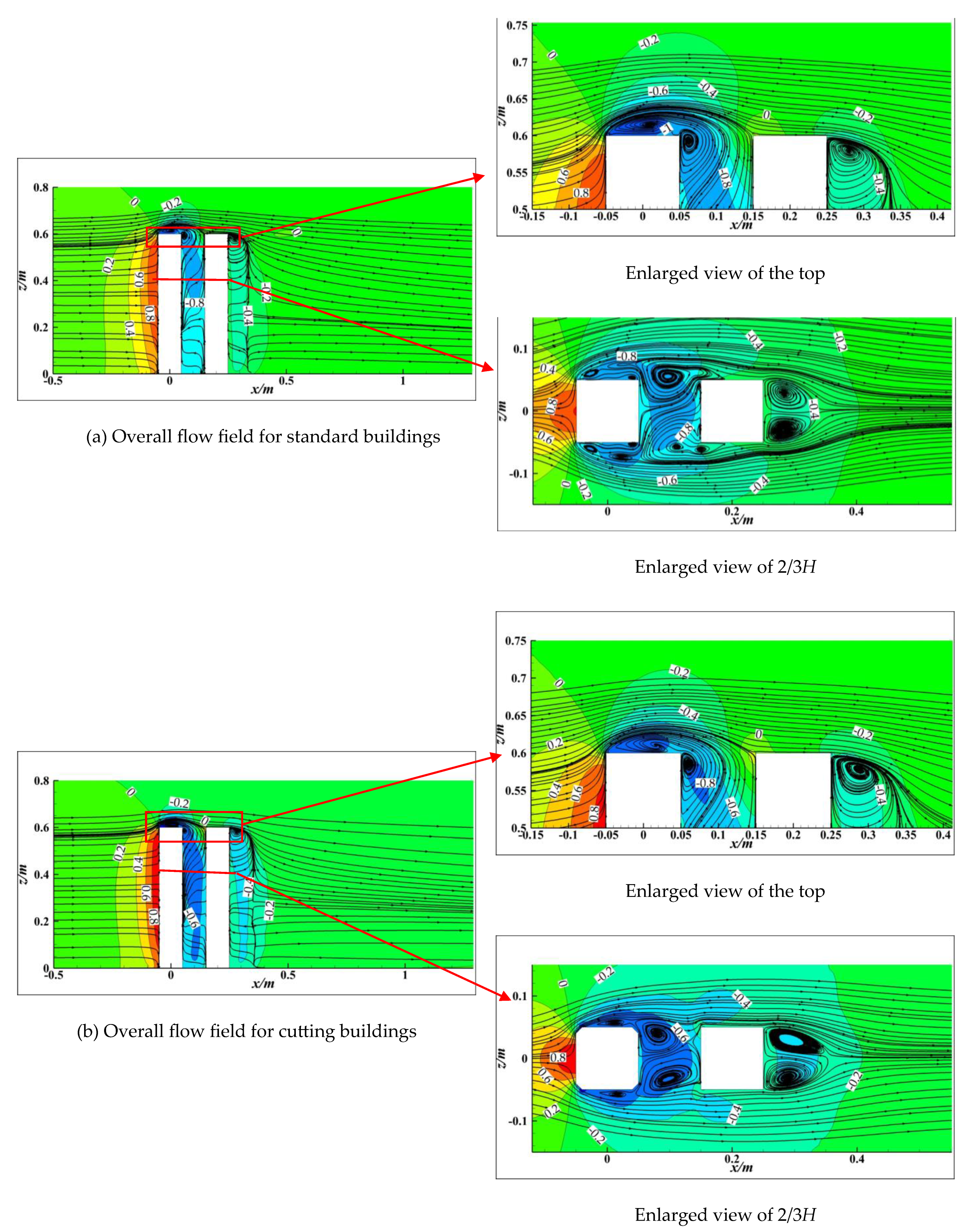
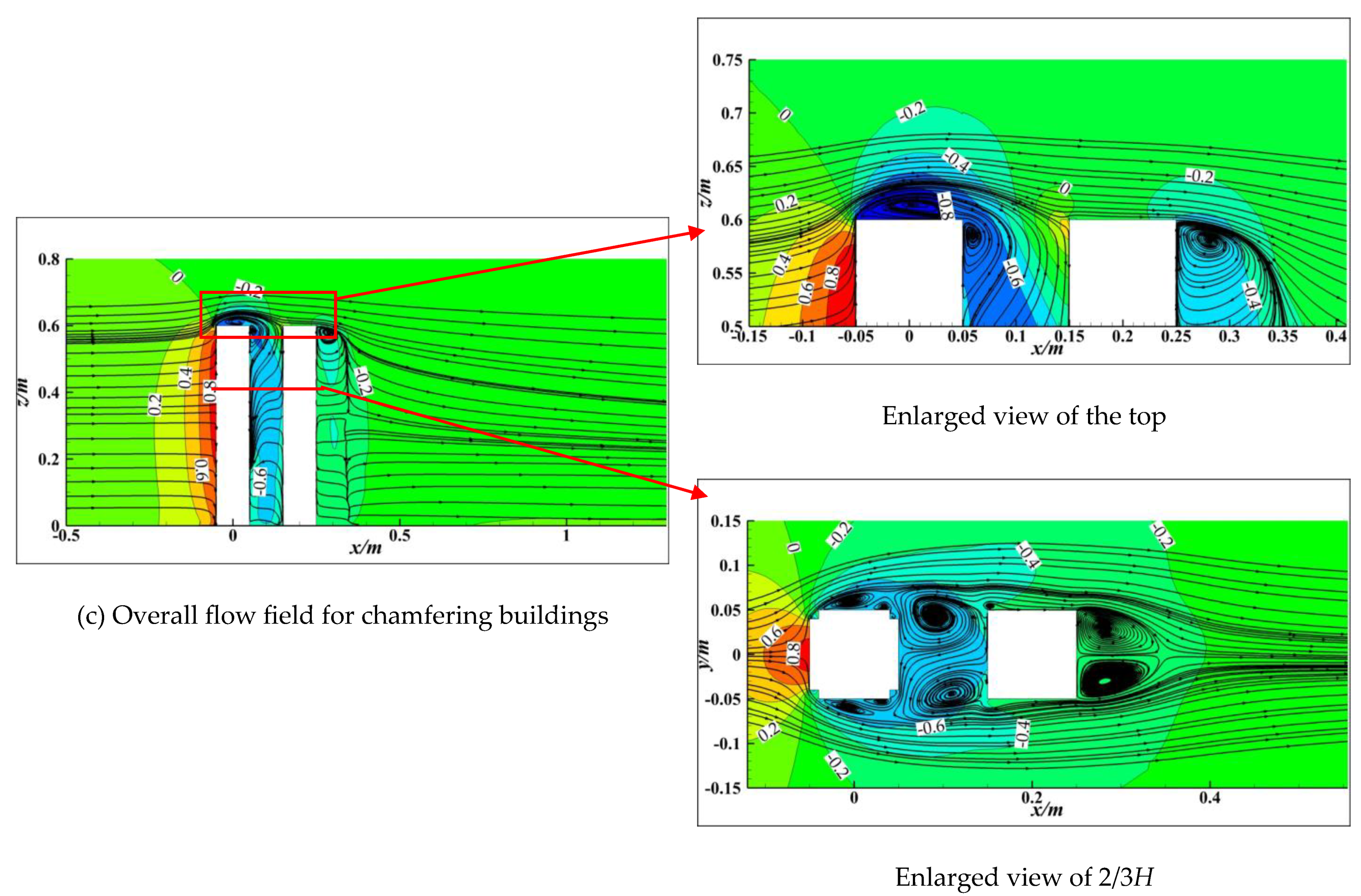
4.4.1. Time-Averaged Flow Field
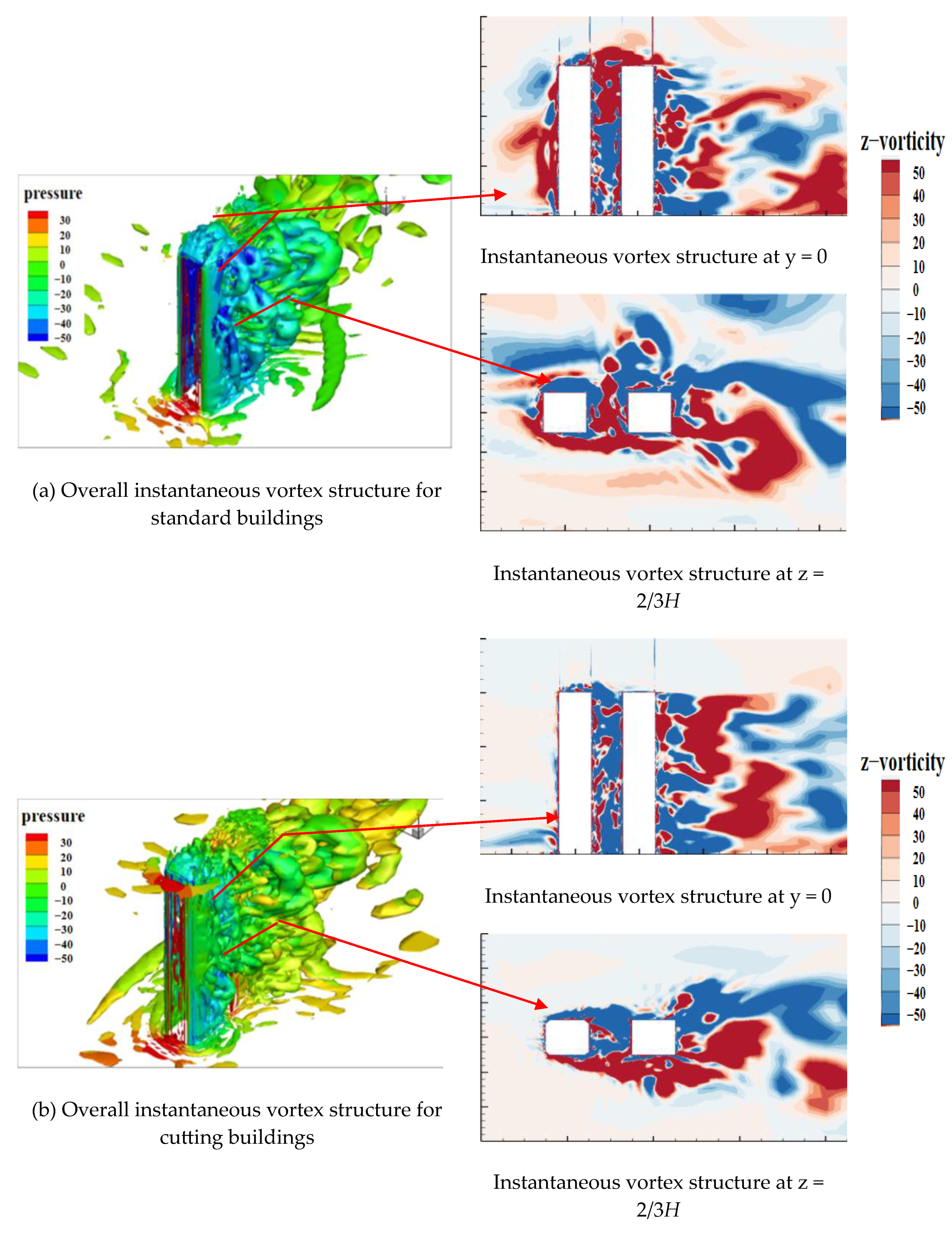
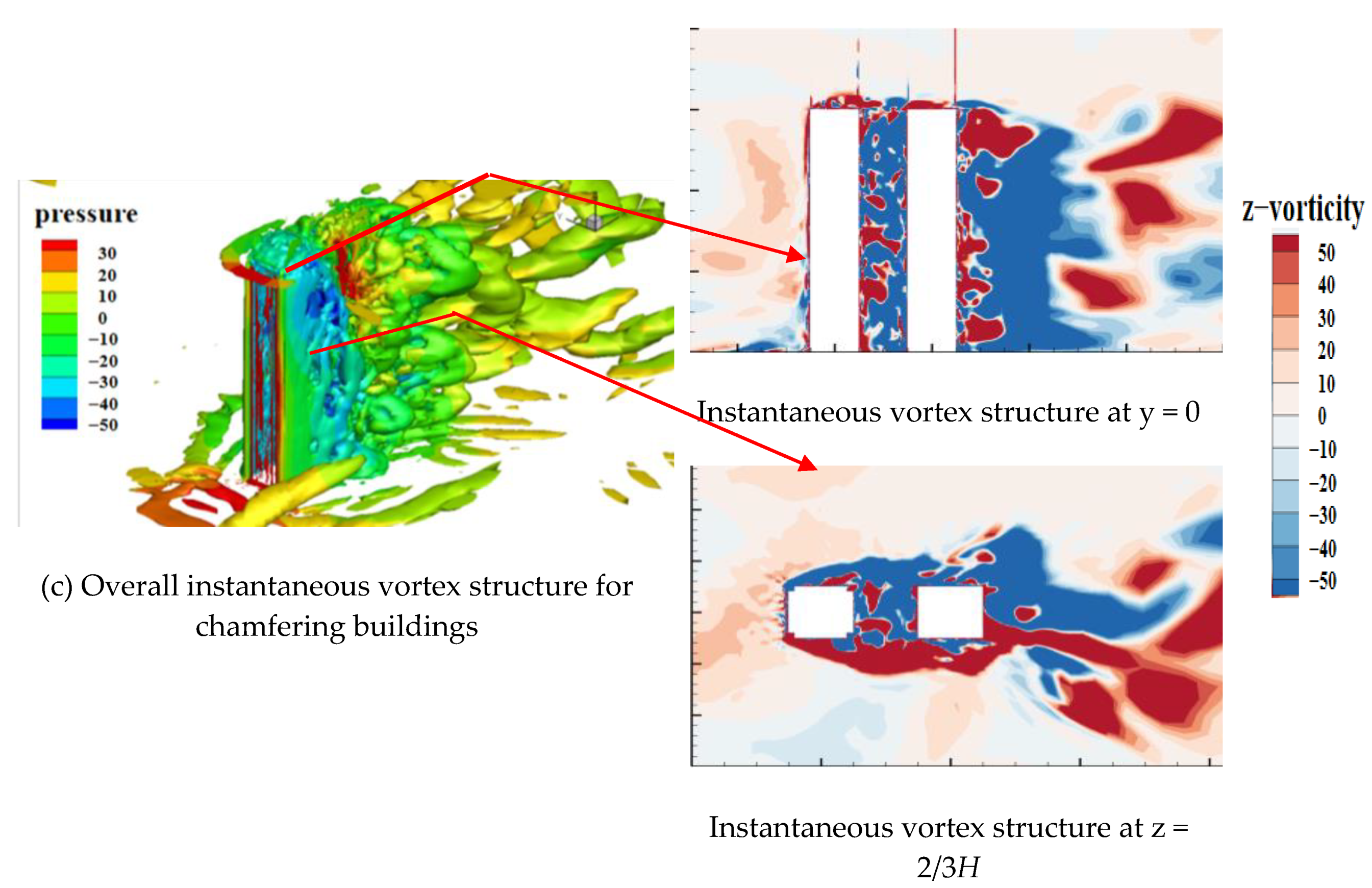
4.4.2. Instantaneous Vortex Structure
5. Conclusions
- (1)
- Large eddy simulation was integrated with equilibrium IBC expressions to generate inflow turbulence models. This approach has been validated for its effectiveness in predicting the mean and fluctuating wind pressure coefficients on three-dimensional tall buildings immersed in the atmospheric boundary layer.
- (2)
- The variations in the wind pressure coefficient for tandem buildings, regardless of whether or not the upstream building has corner modifications, are generally similar. However, notable differences occur in the mean and fluctuating wind pressure coefficients, specifically at the regions where corner modifications are implemented. These corner modification measures are particularly effective at reducing the overall wind pressure around tall buildings, with the most significant impact being observed on the side and leeward surfaces. Among the various modification techniques, corner-cutting proves to be the most effective. Furthermore, corner cutting on the upstream building significantly reduces the drag coefficients for both the upstream and downstream buildings.
- (3)
- In the context of tandem tall buildings, the implementation of upstream corner cutting and chamfering measures plays a crucial role in influencing the shear flow diffusion angle at the upstream leading edges. These modifications significantly impact the separation and reattachment of the flow that has separated upstream, subsequently affecting the downstream tall building. The primary outcome of these influences is a reduction in wind pressure coefficients on the surfaces of the tall buildings, which consequently alters the aerodynamic coefficients. Notably, the upstream corner cutting measure proves to be more effective than chamfering in minimizing flow separation and reattachment, thereby further reducing the surface wind pressure.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Cooper, K.; Nakayama, M.; Sasaki, Y.; Fediw, A.; Resende-Ide, S.; Zan, S.J. Unsteady Aerodynamic force measurements on a super-tall building with a tapered cross section. J. Wind. Eng. Ind. Aerodyn. 1997, 72, 199–212. [Google Scholar] [CrossRef]
- Zhang, W.; Chen, X.; Yang, H.; Liang, H.; Wei, Y. Forced convection for flow across two tandem cylinders with rounded corners in a channel. Int. J. Heat Mass Transf. 2019, 130, 1053–1069. [Google Scholar] [CrossRef]
- Xiaoqing, D.; Xinxin, T.; Wenyong, M.; Erdong, L. Effects of rounded corner on aerodynamics of square cylinders and its flow mechanisms. Chin. J. Theor. Appl. Mech. 2018, 50, 1013–1023. [Google Scholar]
- Xie, J. Aerodynamic optimization of super-tall buildings and its effectiveness assessment. J. Wind. Eng. Ind. Aerodyn. 2014, 130, 88–98. [Google Scholar] [CrossRef]
- Gu, M.; Quan, Y. Across-wind loads of typical tall buildings. J. Wind. Eng. Ind. Aerodyn. 2004, 92, 1147–1165. [Google Scholar] [CrossRef]
- Hui-lan, C.; Yong, Q.; Ming, G. Effect of corner-cut and tapering on across-wind aerodynamic damping of square high-rise buildings. Eng. Mech. 2013, 30, 87–93,100. [Google Scholar]
- Kawai, H. Effect of corner modifications on aeroelastic instabilities of tall buildings. J. Wind. Eng. Ind. Aerodyn. 1998, 74, 719–729. [Google Scholar] [CrossRef]
- Wijesooriya, K.; Mohotti, D.; Lee, C.-K.; Mendis, P. A technical review of computational fluid dynamics (CFD) applications on wind design of tall buildings and structures: Past, present and future. J. Build. Eng. 2023, 74, 106828. [Google Scholar] [CrossRef]
- Feng, C.; Gu, M.; Zheng, D. Numerical simulation of wind effects on super high-rise buildings considering wind veering with height based on CFD. J. Fluids Struct. 2019, 91, 102715. [Google Scholar] [CrossRef]
- Cao, Y.; Tamura, T. Large-eddy simulations of flow past a square cylinder using structured and unstructured grids. Comput. Fluids 2016, 137, 36–54. [Google Scholar] [CrossRef]
- Lu, B.; Li, Q.; Wang, X.; Han, X.; He, J. Large eddy simulation of wind pressures on a 600-m-high skyscraper and comparison with field measurements during super myphoon mangkhut. J. Build. Eng. 2024, 92, 109750. [Google Scholar] [CrossRef]
- Hariprasad, C.; Ajith Kumar, R.; Dahl, J.; Sohn, C.H. Flow Structures around a Square Cylinder: Effect of Corner Chamfering. J. Aerosp. Eng. 2024, 37, 04024021. [Google Scholar] [CrossRef]
- Huang, G.; Fan, Y.; Chen, X.; Yang, X.; Li, Z. Predicting crosswind response of tall buildings: Base isolation and nonlinear aeroelastic effects. Eng. Struct. 2024, 305, 117722. [Google Scholar] [CrossRef]
- Jafari, M.; Alipour, A. Review of approaches, opportunities, and future directions for improving aerodynamics of tall buildings with smart facades. Sustain. Cities Soc. 2021, 72, 102979. [Google Scholar] [CrossRef]
- Qun, Y.; Huitao, Z.; Xiaobin, L. Study on fluctuating pressure distribution and aerodynamic force of flows around two square cylinders in tandem arrangement. Build. Struct. 2020, 50, 140–144. [Google Scholar]
- Liu, T.; Zhou, L.; Huang, D.; Zhang, H.; Wei, C. Aspect ratio and interference effects on flow over two rectangular cylinders in tandem arrangement. Ocean Eng. 2023, 271, 113731. [Google Scholar] [CrossRef]
- Xie, Z.; Gu, M. Wind-induced interference effects between two arbitrarily arranged prisms. Tumu Gongcheng Xuebao 2005, 38, 32–38. [Google Scholar]
- Shao, J.; Shu, C.; Liu, N.; Zhao, X. Numerical investigation of vortex induced rotation of two square cylinders in tandem arrangement. Ocean Eng. 2019, 171, 485–495. [Google Scholar] [CrossRef]
- Wang, H.-F. Three dimension characteristics of finite-length bluff body wake. J. Cent. South Univ. 2011, 42, 495–500. [Google Scholar]
- Wang, H.-F.; Yang, F.; Zou, C. Effects of boundary layer conditions on aerodynamic forces of a finite-length square prism. J. Vib. Shock 2016, 35, 39–46. [Google Scholar] [CrossRef]
- Du, X.; Shi, D.; Dong, H.; Liu, Y. Flow around square-like cylinders with corner and side modifications. J. Wind. Eng. Ind. Aerodyn. 2021, 215, 104686. [Google Scholar] [CrossRef]
- Zheng, D.-Q.; Gu, M.; Zhang, A.-S.; Zhang, J.-G. Large eddy simulation of flow around a single square building model with 1: 1: 6 shape. Zhendong Yu Chongji 2011, 30, 96–100. [Google Scholar]
- Mathey, F.; Cokljat, D.; Bertoglio, J.P.; Sergent, E. Assessment of the vortex method for large eddy simulation inlet conditions. Prog. Comput. Fluid Dyn. Int. J. 2006, 6, 58–67. [Google Scholar] [CrossRef]
- Li, L.; Zheng, D.; Chen, G.; Fang, P.; Ma, W.; Tang, S. Large eddy simulation of flow over a three-dimensional hill with different slope angles. Front. Earth Sci. 2023, 18, 98–111. [Google Scholar] [CrossRef]
- Zheng, D.Q.; Zhang, A.S.; Gu, M. Improvement of inflow boundary condition in large eddy simulation of flow around tall building. Eng. Appl. Comput. Fluid Mech. 2012, 6, 633–647. [Google Scholar] [CrossRef]
- Tominaga, Y.; Mochida, A.; Yoshie, R.; Kataoka, H.; Nozu, T.; Yoshikawa, M.; Shirasawa, T. AIJ guidelines for practical applications of CFD to pedestrian wind environment around buildings. J. Wind. Eng. Ind. Aerodyn. 2008, 96, 1749–1761. [Google Scholar] [CrossRef]
- Franke, J. The European Cost Action 732–quality assurance and improvement of micro-scale meteorological models. In Proceedings of the Seventh Asia-Pacific Conference on Wind Engineering, Taipei, Taiwan, 8–12 November 2009. [Google Scholar]
- GB 50009-2012; Load Code for the Design of Building Structures. China Architecture & Building Press: Beijing, China, 2012.
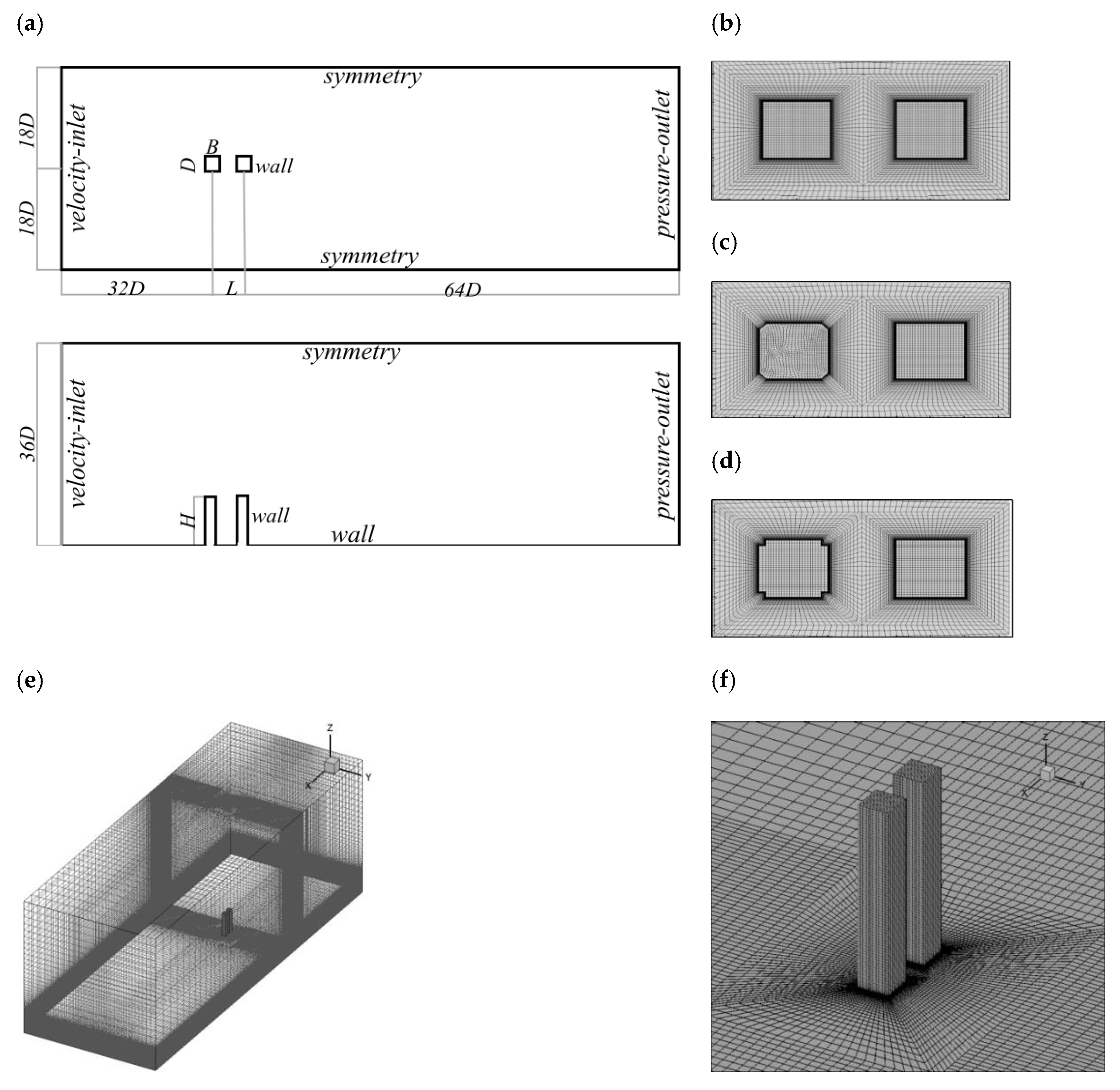


| Case Name | Minimum Grid Size | Total Grid Numbers | y+ |
|---|---|---|---|
| Standard single-M1 | 0.005D | 1,580,000 | <30 |
| Standard single-M2 | 0.001D | 1,680,000 | <15 |
| Standard single-M3 | 0.0005D | 1,830,000 | <5 |
| Standard tandem-M3 | 0.0005D | 2,290,000 | <5 |
| Cutting tandem-M3 | 0.0005D | 2,290,000 | <5 |
| Chamfering tandem-M3 | 0.0005D | 2,290,000 | <5 |
| Parameters | Values |
|---|---|
| Friction velocity u* (m/s) | 0.8 |
| Roughness height z0 (m) | 0.05 |
| Model constant C1 | −0.27 |
| Model constant C2 | 3.16 |
| Abbreviation | Corresponding Physical Quantity |
|---|---|
| Pi | wind pressure |
| Cpi,mean | mean wind pressure coefficient |
| Cpi,rms | fluctuating wind pressure coefficient |
| FD(zi) | layer drag |
| CD(zi) | layer drag coefficient |
| FL(zi) | layer lift |
| CL(zi) | layer lift coefficient |
| Technologies | Explanation | Disadvantages |
|---|---|---|
| DNS | Direct Numerical Simulation: This method does not do anything with the transient N-S equations, but solves them directly, so that the full flow field pulsation information can be obtained. | The method is limited to the study of low Reynolds number flows due to the large workload constraints. |
| RANS | Reynolds Averaged Navier–Stokes: This method performs time-averaging of the transient N-S equation and solves the turbulence model for the unclosed terms appearing in the equations after time-averaging | The method does not yield fluctuating information on the surface of structures. |
| LES | Large Eddy Simulation: Large energy-carrying eddies are solved directly by the instantaneous N-S equation, while small eddies with dissipative effects are solved by appropriate modeling. | The method is costly to compute and requires high mesh quality. |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zheng, D.; Wu, X.; Zhu, Y.; Ma, W.; Fang, P. Large Eddy Simulation of Flow Around Twin Tower Buildings in Tandem Arrangements with Upstream Corner Modification. Atmosphere 2024, 15, 1540. https://doi.org/10.3390/atmos15121540
Zheng D, Wu X, Zhu Y, Ma W, Fang P. Large Eddy Simulation of Flow Around Twin Tower Buildings in Tandem Arrangements with Upstream Corner Modification. Atmosphere. 2024; 15(12):1540. https://doi.org/10.3390/atmos15121540
Chicago/Turabian StyleZheng, Deqian, Xueyuan Wu, Yuzhe Zhu, Wenyong Ma, and Pingzhi Fang. 2024. "Large Eddy Simulation of Flow Around Twin Tower Buildings in Tandem Arrangements with Upstream Corner Modification" Atmosphere 15, no. 12: 1540. https://doi.org/10.3390/atmos15121540
APA StyleZheng, D., Wu, X., Zhu, Y., Ma, W., & Fang, P. (2024). Large Eddy Simulation of Flow Around Twin Tower Buildings in Tandem Arrangements with Upstream Corner Modification. Atmosphere, 15(12), 1540. https://doi.org/10.3390/atmos15121540





