Modeling the Effect of Ionospheric Electron Density Profile and Its Inhomogeneities on Sprite Halos

Abstract

Sprite halos are diffuse glow discharges in the D-region ionosphere triggered by the quasi-electrostatic (QES) fields of lightning discharges. A three-dimensional (3D) QES model is adopted to investigate the effect of ionospheric electron density on sprite halos. The electron density is described by an exponential formula, parameterized by reference height (h’) and sharpness (β), and the local inhomogeneity has a Gaussian density distribution. Simulation results indicate that the reference height and steepness of the nighttime electron density affect the penetration altitudes and amplitudes of normalized electric fields, as well as the altitudes and intensities of the corresponding sprite halos optical emissions. A comparison of the daytime and nighttime conditions demonstrates that the daytime electron density profile is not favorable for generating sprite halos emissions. Furthermore, the pre-existing electron density inhomogeneities lead to enhanced local electric fields and optical emissions, potentially offering a plausible explanation for the horizontal displacement between sprites and their parent lightning, as well as their clustering.

1. Introduction

Transient luminous events (TLEs) are the optical manifestations of the direct coupling between the lower and upper atmosphere through tropospheric lightning discharges. Different types of TLEs have been documented and classified into sprites, sprite halos (or halos), elves, blue starters, blue jets, and gigantic jets [1,2,3]. TLEs have been shown to influence global circuits and climate, upper atmospheric chemistry, and subionospheric radio signal propagation [4,5,6]. Over the past three decades, TLEs have attracted extensive interest from researchers worldwide. Sprites and sprite halos are two types of TLEs occurring in the mesosphere and lower ionosphere; both of them are driven by the quasi-electrostatic (QES) fields produced by intense cloud-to-ground (CG) tropospheric discharges [7,8,9]. Sprites are large-scale mesospheric electrical discharges observed mainly at nighttime in the altitude range of 40–90 km. High-resolution telescopic imaging has confirmed that sprites consist of filamentary streamer discharges (sprite streamers) that extend vertically in altitude [10]. Sprite streamers generally initiate at ∼75 km altitude and sometimes are accompanied by a diffuse glow at higher altitudes, referred to as sprite halos or halos. Sprite halos are short-lived (~2 ms), and descending diffuse pancake-shaped glow occurs at ∼70–85 km altitude with a diameter typically less than 100 km [11,12], which are clearly smaller than elves and are less structured than sprites. Observed halos often precede sprites but could also occur alone.

Previous observational and simulation studies have confirmed that the QES fields produced by CG discharges have a primary role in the initiation of sprites and sprite halos. The widely accepted conventional breakdown model indicates that sprites result from the atmospheric quasi-static electric breakdown at mesospheric altitudes, and these QES fields are nearly linearly proportional to the charge moment change (CMC) in the lightning discharge that produces the sprites. The CMC, defined as the charge amount transfer to the ground multiplied by the vertical cloud-to-ground channel altitude, is an important parameter and has been widely used to assess the capacity of lightning discharges leading to sprite occurrences [7,13,14,15,16]. For example, Hu et al. [13] analyzed numerous sprite-producing lightning strikes over North America and estimated the probability of sprite occurrence. They found that parent lightning with a CMC > 1000 Ckm within 6 ms produces sprites at >90% probability, whereas lightning with a CMC < 600 Ckm only at <10% probability. Sprite-producing strikes from winter thunderstorms in Japan, measured by Hayakawa et al. [14], indicated that even a small CMC of ~200–300 Ckm can initiate sprites. Such a low threshold CMC reported by Hayakawa et al. [14] was supported by the simulation results of Qin et al. [17]. Qin et al. [17] reproduced sprite streamers initiated from pre-existing electron density inhomogeneities for a CMC of as low as 200 Ckm using a 2D plasma fluid model. The ambient conditions in the lower ionosphere, including the electron density profile and its inhomogeneities, also play a key role in the formation of TLEs, especially the initiation of sprite streamers. The electron density profile is one of the most critical and variable parameters of the D-region ionosphere and determines the magnitude of atmospheric conductivity in this altitude region. The standard electron density profile in the lower ionosphere is expressed by a two-parameter exponential formulation derived by Wait and Spies [18] characterized by the reference height parameter (h’) and the ionospheric sharpness parameter (β) and has been extensively applied and validated in various scenarios. The two parameters h’ and β control the altitude and the sharpness of the electron density profile, vary with solar zenith angle, geographic latitude, solar activity, and month [19]. The reference height h’ exhibits diurnal variation, with daytime values h’ < 80 km reaching a minimum at local noon and nighttime values h’ ≥ 80 km. Han and Cummer [20] utilized the very low frequency (VLF) signals propagating in the Earth–ionosphere waveguide to probe the nighttime D region ionosphere and found that the reference height of the ionosphere varies between 82.0 and 87.2 km, with a mean value of 84.9 km. However, there is less consensus regarding the steepness parameter β. Han et al. [21] found that sharpness β was between 0.35 km⁻¹ and 0.45 km⁻¹ and emphasized the general inconsistency between β values obtained from different methods and models. Lay et al. [22] reported that β values reached 2.8 km⁻¹ at night and 0.9 km⁻¹ for daytime. In addition, the local electron density inhomogeneities in the lower ionosphere may arise from a variety of sources, including meteor trails, electrodynamic effects of thunderstorms, and atmospheric gravity wave-breaking [23,24,25].

The influence of electron density profile and its inhomogeneity on TLEs has been much discussed in the literature. For example, Pasko et al. [8] suggested the significance of the conductivity profile and the neutral density in the mesosphere, particularly the role of inhomogeneities in initiating sprite streamers. Stanley et al. [26] detected daytime sprites through the extremely low frequency (ELF) signatures and revealed that daytime sprites’ initiation requires a larger electric field and a lower initiation altitude due to the enhanced ionospheric conductivity during daytime, which is confirmed by the modeling results of Tonev and Velinov [27]. Cummer and Lyons [28] proposed that variations in the mesospheric conductivity profile could explain the differing threshold CMCs observed for sprite initiation. Pasko and Stenbaek-Nielsen [29] noted that the transition altitude from diffuse to streamer region is highly sensitive to the ambient electron density profile. Stenbaek-Nielsen et al. [30] indicated that mesospheric conductivity may significantly influence the types of sprites observed. Lay et al. [31] reported that the steepness of the electron density profile may facilitate sprite streamers’ initiation, which was further discussed by Qin et al. [32]. Qin et al. [33] demonstrated that lower mesospheric ambient conductivity leads to a lower threshold CMC for the production of carrot sprites. Marshall et al. [34] simulated elves modulated by gravity waves using a 3D electromagnetic pulse (EMP) model, in which both neutral and electron densities were considered. Zhang et al. [35] utilized similar atmospheric density perturbations in a 3D QES model to simulate the impacts of atmospheric gravity waves on the initiation and optical emissions of sprite halos. Wang et al. [36] discussed the modulation effects of concentric gravity waves on elves and sprite halos using a 2D EMP model.

Most existing related studies focus on the influence on sprites, especially on the initiation and evolution of sprite streamers, while there is a relative dearth of discussion concerning the effects of electron density profile on sprite halos. Although sprite halos may appear more frequently than sprites [37], the observation results of sprite halos are relatively less than sprites. The numerical simulation model remains an effective method for studying sprite halos. Sprite halos can be numerically simulated using a 2D QES model previously introduced by Pasko et al. [7,8]. In this paper, a 3D QES model is adopted to further investigate the effect of ionospheric electron density profile and its inhomogeneities on the sprite halos. It is noteworthy that the 3D QES model is a typical large-scale electrostatic coupling model. Although it cannot simulate the dynamics of sprite streamers, the results are useful for the study of sprites. The simulations of sprite streamers require the plasma fluid model with higher resolution, which has been reported in [38,39,40].

The rest of this paper is organized as follows. In Section 2, the 3D QES heating model adopted here is briefly introduced, which includes the ionospheric nonlinear effect, such as heating, breakdown ionization, and optical emissions. The ionospheric electron density is described using ionospheric density distribution according to Wait and Spies [18], and the inhomogeneity has a Gaussian density distribution. Section 3 presents the simulation results and discussion on the effects of electron density. The impacts of the vertical profile of electron density and the local inhomogeneity are presented in Section 3.1 and Section 3.2, respectively. Finally, conclusions are presented in Section 4.

2. Methods

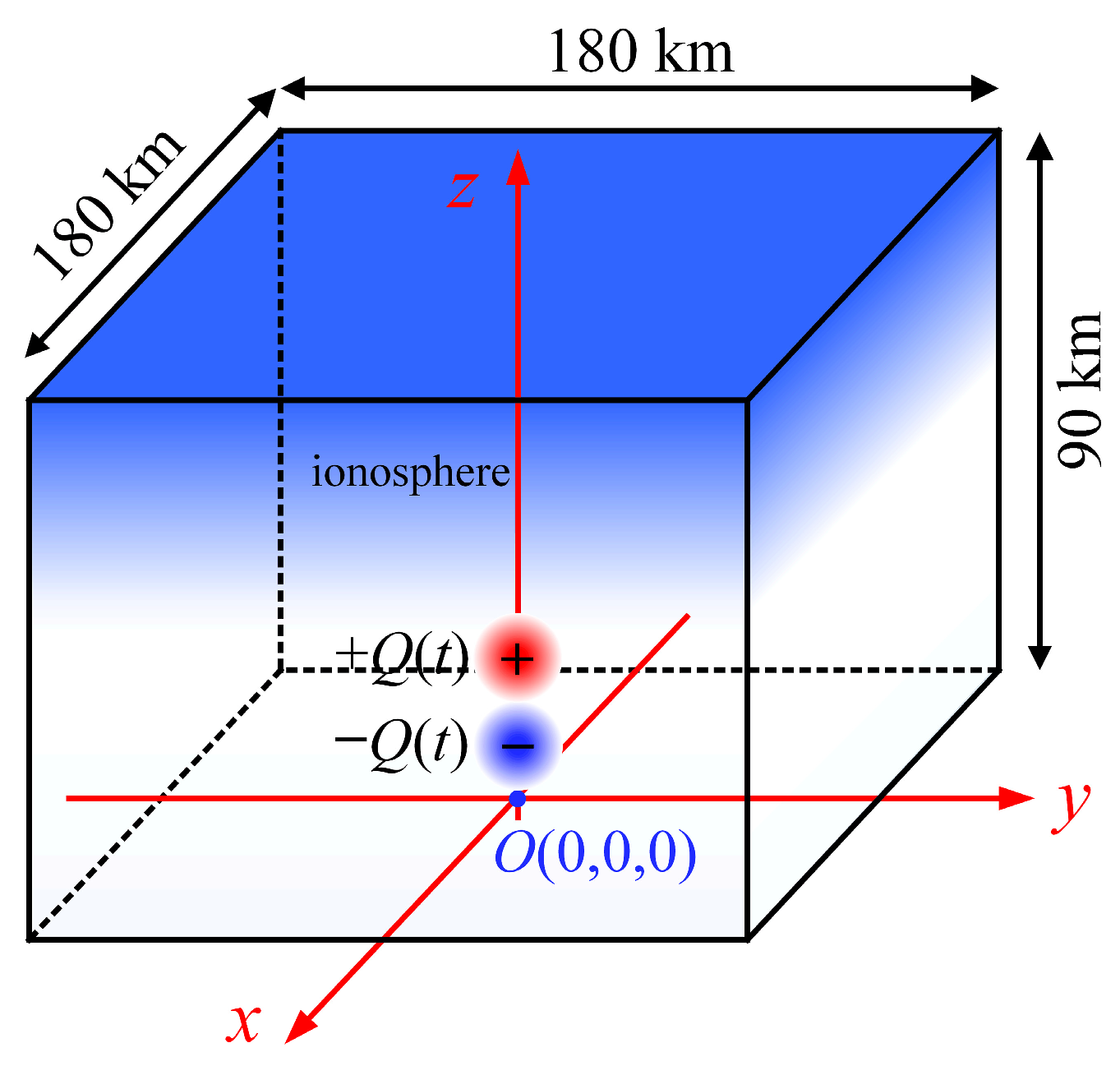
2.1. The 3D QES Heating Model

The 3D QES heating model developed by Zhang et al. [35] was adopted to simulate the thundercloud–ionosphere electrostatic interaction and the resulting sprite halos emissions. This model extends the 2D axisymmetric model proposed by Pasko [7] into 3D Cartesian coordinates (x, y, z), with the z-axis representing altitude (in km). As illustrated in Figure 1, the simulation domain is 180 × 180 × 90 km³ and is divided into 1 (in x) × 1 (in y) × 2/3 (in z) km³ grid cells, surrounded by perfectly conducting boundary conditions (φ = 0). Within the computational domain, the electrostatic field E, charge density ρ, and electrostatic potential φ satisfy the charge conservation equation and Poisson’s equation [7]:

$$\left\{\begin{array}{c}{\displaystyle \frac{\partial \rho }{\partial t}}-\nabla \sigma \cdot \nabla \phi +{\rho }_s\sigma /{\epsilon }_0=0\\ \hspace{0.33em}\nabla \cdot E=-{\nabla }^2\phi =(\rho +{\rho }_s)/{\epsilon }_0\end{array}\right.,$$

(1)

where ε₀ and σ are the permittivity and atmospheric conductivity, respectively. ρ_s is the thundercloud source charge density. The electric field E in the simulation domain is calculated by solving Poisson’s equation with a finite difference iterative relaxation scheme. A typical thundercloud with dipole structure is adopted to simulate +CG discharge, and the thundercloud charge density has a Gaussian spherically symmetric distribution of ρ_s(x,y,z,t) = ρ₀(t)exp{–[(x – x_±)²/a² + (y – y_±)²/b² + (z – z_±)²/c²]}, where ρ₀(t) = Q(t)/V, the sizes of the charge distribution a = b = c = 3 km, (x_±, y_±, z_±) is the location of the positive and negative charge center. By default, the positive charge center is centered at (0 km, 0 km, 10 km), and the negative charge center is centered at (0 km, 0 km, 5 km), respectively. The total source charge is Q(t) = ∫ρ_s(x,y,z,t)dV, is expressed by

$$Q(t)=\left\{\begin{array}{c}\hspace{1em}\hspace{1em}{Q}_0{\displaystyle \frac{\tan \mathrm{h}(t/{\tau }_f)}{\tan \mathrm{h}(1)}}\hspace{1em}\hspace{1em},0<t<{\tau }_f\\ Q_0\left[1-{\displaystyle \frac{\tan \mathrm{h}((t-{\tau }_f)/{\tau }_s)}{\tan \mathrm{h}(1)}}\right],{\tau }_f<t<{\tau }_f+{\tau }_s\end{array}\right.,$$

(2)

where τ_f = 0.5 s and τ_s = 1 ms are the duration of accumulation and release of positive charge Q₀, respectively [7]. In this paper, the thundercloud positive charge with the magnitude of Q₀ = 150 C is removed within 1 ms for a +CG discharge [35].

In this model, self-consistent evaluation of the conductivity σ in the above Equation (1) is crucial in the simulation of thundercloud electrodynamic upward coupling with the lower ionosphere. The total conductivity σ = σ_i + σ_e is the sum of ion conductivity σ_i and electron conductivity σ_e, as shown in Figure 2a. Below 60 km, the ion conductivity is dominant and a function of altitude z (in km), expressed by σ_i = 5 × 10⁻¹⁴e^{z/6 km} S/m, which is taken from Dejnakarintra and Park [41]. Above 60 km, the electron conductivity dominates the total conductivity, taken by σ_e = q_eN_eμ_e [42], where q_e is the electron charge, μ_e is the electron mobility, and N_e is the electron density. The electron mobility μ_e is a nonlinear function of the reduced electric field (E/N), where N is the neutral density taken from the NRLMSISE-00 model [43]. The neutral density is proportional to the breakdown field E_k = 3.2 × 10⁶N/N₀ and decreases exponentially with increasing altitude; the neutral density on the ground is N₀ = 2.688 × 10²⁵ m⁻³. The analytical form of μ_e formulated by Pasko et al. [8] based on the data of an electron swarm experiment is used in this model, as shown in Figure 2b. The initial electron density profile and its possible inhomogeneity are the focus of this paper, and the related parameter set is introduced in the following Section 2.2. The variation in the electron density N_e follows the continuity equation dN_e/dt = (ν_i – ν_a)N_e, where ν_i and ν_a are ionization and attachment rates, both of them depend on the reduced electric field, as shown in Figure 2c. The conventional breakdown field E_k = 3.2 × 10⁶N/N₀ is also shown in Figure 2c (dashed line). The ionization rate ν_i calculated by the analytical expression given by Papadopoulos et al. [44], and the analytical form of attachment rate ν_a is presented in Pasko [7]. Due to only 1 ms timescale of lightning discharges used in our simulations, the main chemical reactions are considered to include the electron impact ionization of N₂ and O₂, as well as the electron dissociative attachment to O₂, while many slow chemical reactions are ignored, such as three-body detachment, electron–ion, and ion–ion recombination [45,46,47]. The influence of the geomagnetic field is also neglected due to electron collisions dominating in the lower ionospheric D region [8].

Video observations with optical equipment are one of the primary means in most studies of sprite dynamics. Thus, our 3D model further simulates the optical emission of sprite halos. As shown in Figure 2d, like the parameterization scheme of Pasko [7], five bands are calculated. Previous studies have indicated that the red color of sprites is primarily due to the excitation of the first positive band of N₂ (${\mathrm{N}}_2 1\mathrm{P}$). Therefore, only the red band (${\mathrm{N}}_2 1\mathrm{P}$) is introduced in this article. The optical excitation rate ν_k, a nonlinear function of the electric field, is calculated by the analytical expression [48]:

$$\log ({\displaystyle \frac{v_k{N}_0}{N}})={\sum }_{i=0}^3{a}_i{x}^i,$$

(3)

where x = log₁₀(EN₀/N), and the approximation coefficients of ${\mathrm{N}}_2 1\mathrm{P}$ are $a_0=-1301.0$, $a_1=563.03$, $a_2=-80.715$, $a_3=3.8647$ [49,50]. The number density of N₂ in different bands during the excited state of k are calculated by the continuity equation ${\displaystyle \frac{\partial n_k}{\partial t}}=-{\displaystyle \frac{n_k}{{\tau }_k}}+v_k{N}_e$, where ${\tau }_k={\left[A_k+{\alpha }_1{N}_{N_2}+{\alpha }_2{N}_{o_2}\right]}^{-1}$ is the lifetime of state k, $A_k=1.7\times {10}^5{s}^{-1}$ is the transition coefficient, ${\alpha }_1={10}^{-17} {\mathrm{m}}^3{\mathrm{s}}^{-1}$, and ${\alpha }_2=0 {\mathrm{m}}^3{\mathrm{s}}^{-1}$ are the quenching rates for N₂ colliding with N₂ and O₂, $N_{{\mathrm{N}}_2}$ and $N_{{\mathrm{O}}_2}$ are the number densities of N₂ and O₂, respectively [51] (p. 119). ν_kN_e denotes the excitation progress of electrons. Finally, based on the calculated $n_k$, the optical emission intensities I_k (in Rayleighs) are calculated by $I_k={10}^{-6}{\int }_L{A}_k{n}_{k}dl$, where the integral is taken along L, representing the horizontal line of sight. Details on the model formulation, parameter set, and model accuracy verification of the 3D QES model introduced above can be found in [35].

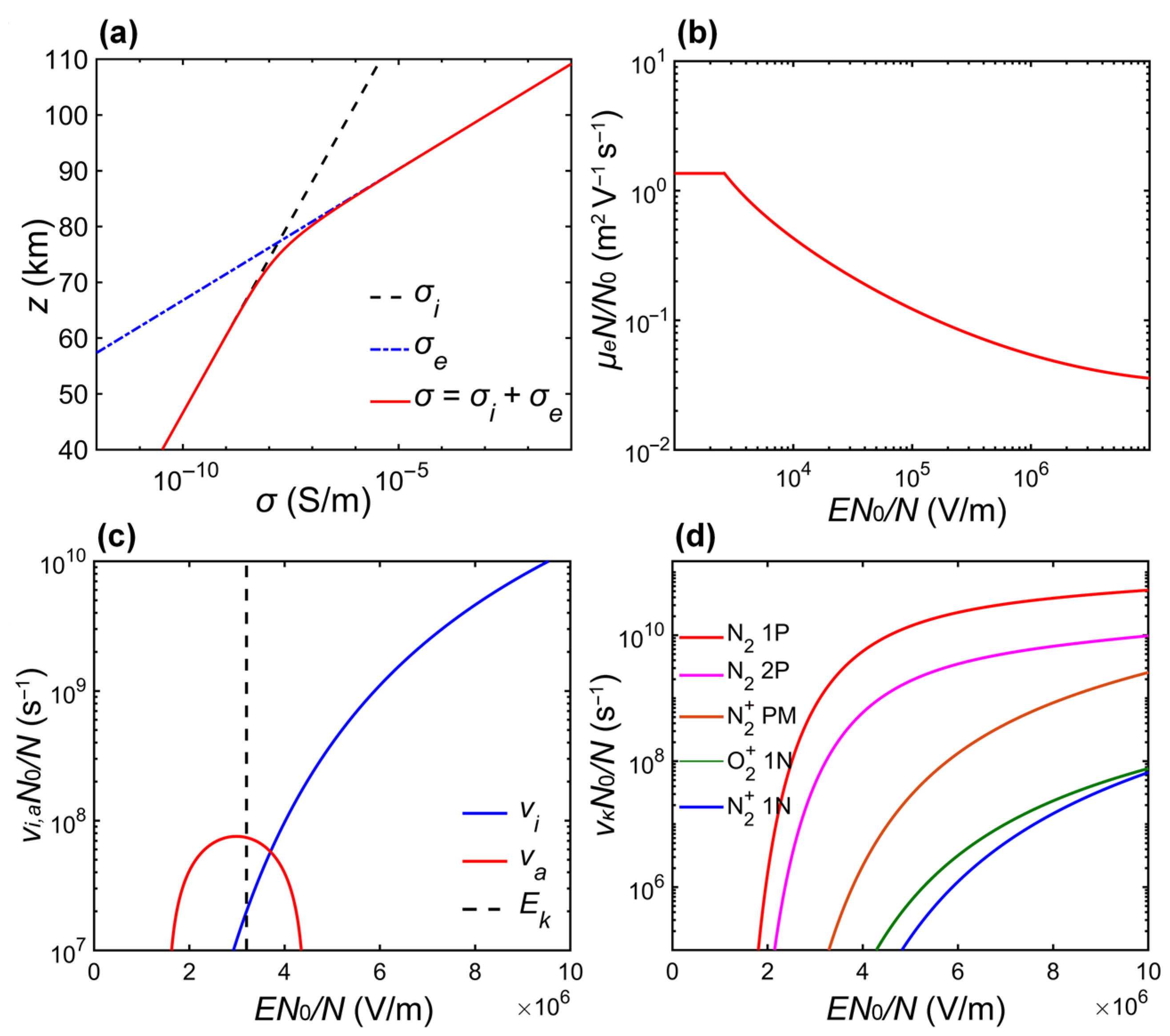
2.2. Ionospheric D-Region Electron Density

The 3D QES model can accommodate any realistic background electron density profile. To assess the impact of electron density on sprite halos, we mainly consider two cases of the electron density distribution, including the large-scale vertical profile of ionospheric electron density and the local ionospheric electron density inhomogeneity.

For the case of a large-scale vertical profile of ionospheric electron density, the standard D region electron density N_e at an altitude h (in km) is expressed by a two-parameter exponential profile as follows:

$$N_e\left(h\right)=1.43\times 10^{13}\exp (-0.15h^{\prime }\left)\exp [\right(\beta -0.15\left)\right(h-h^{\prime }\left)\right],$$

(4)

where N_e (in cm⁻³), h’ (in km), and β (in km⁻¹) are the ionospheric sharpness parameter and reference height parameter, respectively. Note that h’ is sometimes denoted as the virtual reflection height of the ionosphere for VLF waves. This exponential profile in the standard form for the electron density originates from the theoretical work of Wait and Spies [18], which has been widely used in studies of VLF propagation [13,19,21] and in TLEs studies [8,32,35] and agrees well with directly observed profiles in the D region [52]. Of course, the actual ionosphere is not exactly like this, but it offers a reasonable approximation. By default, β = 0.5 km⁻¹, h’ = 85 km are used as the typical electron density profile of the nighttime ionosphere, while for the daytime ionosphere, the typical profile is characterized by β = 0.3 km⁻¹, h’ = 72 km [20].

For the case of local ionospheric electron density inhomogeneity, based on the D-region electron density profile described above, pre-existing electron inhomogeneities are placed in the lower ionosphere. The morphological scales of electron inhomogeneities could be complicated in the actual situation. For convenience, we refer to the parameterization scheme proposed by Qin et al. [32] but adopt a larger scale due to the lower resolution of the 3D model. Consequently, the inhomogeneity in the 3D model has a Gaussian density distribution as follows:

$$N_{\mathrm{inhomo}}\left(x,y,z\right)=N_{\mathrm{peak}}\exp \left[-\left({\displaystyle \frac{(x-x_n{)}^2}{x_0^2}}+{\displaystyle \frac{(y-y_n{)}^2}{y_0^2}}+{\displaystyle \frac{(z-z_n{)}^2}{z_0^2}}\right)\right]$$

(5)

where N_peak = 1 × 10⁹ m⁻³ is the peak density of the initial inhomogeneity in this study, (x_n, y_n, z_n) are the coordinates of the inhomogeneity center. x₀, y₀ and z₀ are the sizes of the inhomogeneity along x, y, and z directions, respectively. Previous studies indicate that the scale of ionospheric disturbances can range from tens of meters to tens of kilometers due to different causes, such as meteors and gravity waves [25]. In this paper, the inhomogeneity distribution has a length of 5 km (z₀ = 2.5 km) in the vertical direction and 2 km (x₀ = y₀ = 1 km) in the horizontal direction; the center height is set to z_n = 75 km. The coordinates (x_n, y_n) govern the horizontal placement of the inhomogeneity. For example, when x_n = y_n = 0 km, the inhomogeneity is centered directly above the thundercloud charge center. Our 3D model offers flexibility, allowing the inhomogeneity to be positioned anywhere and to account for multiple inhomogeneities.

3. Results and Discussion

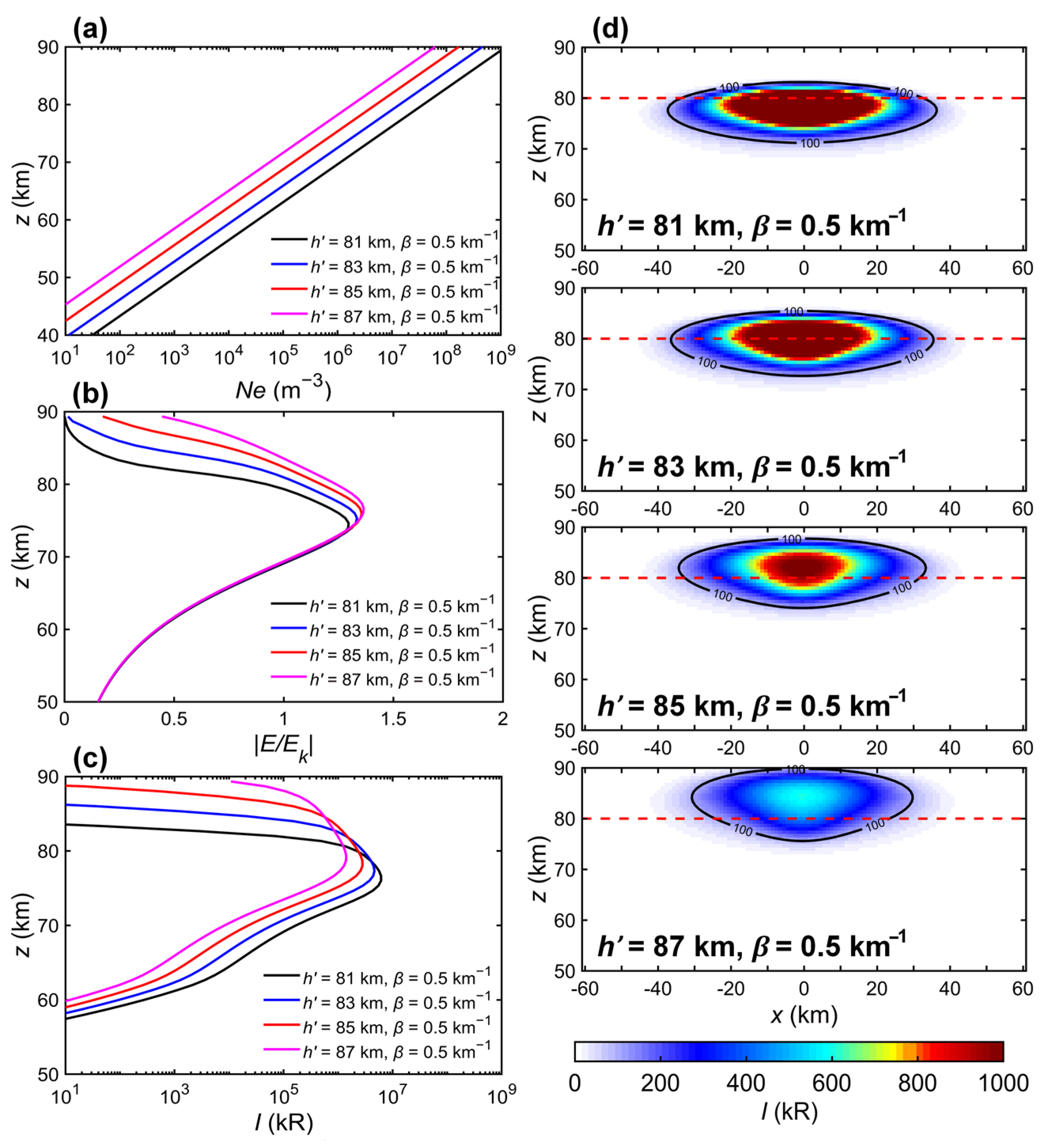
3.1. Effect of the Vertical Profile of Electron Density

The ionospheric electron density is modeled by a two-parameter exponential profile, as described in Equation (4) of Section 2.2 above. This paper primarily focuses on the electron density profile in the nighttime lower ionosphere. The effect of the vertical profile of electron density will be evaluated by varying the two variables, namely the ionospheric height parameter h’ and the ionospheric sharpness parameter β. In all simulation cases, a positive charge Q = 150 C is removed by a +CG discharge from 10 km altitude within 1 ms.

For the nighttime ionospheric electron density profile, the typical nighttime values of h’ range from 82.0 to 87.2 km [20]. For comparison, the height parameter h’ ranges from 81 to 87 km, which was used to produce the modeled results presented in Figure 3, and β is set to 0.5 km⁻¹. The height parameter h’ enables vertical shifting of the electron density profile, as shown in Figure 3a. For the +CG lightning discharge considered here, the maximum normalized electric field E/E_k and maximum optical intensity generated in the low ionosphere appear at the end of the discharge (marked as 1 ms) [7,35]. Thus, the altitude profiles of the normalized electric field E/E_k and optical emission intensities (N₂ 1P) directly above the charge center at t = 1 ms are shown in Figure 3b and 3c, respectively. The sprite halos captured are optical images that have been time-averaged due to the exposure time of the instruments. Therefore, the optical emissions shown in Figure 3d, observed in the horizontal line of sight, are averaged over the main duration of the sprite halos (2 ms), reflecting the overall spatial distribution of the sprite halos [7,35]. The simulated sprite halos are pancake-shaped and have horizontal scales less than 100 km, which are in good agreement with those documented in Pasko et al. [8] and are also consistent with observations [9,11]. As the reference height h’ increases, the penetration height of the normalized electric field E/E_k above ~72 km becomes greater due to the lower electric field relaxation at higher altitudes. The increase in the normalized electric field E/E_k amplitude is primarily because the neutral density decreases exponentially with increasing altitude, reducing the electric field breakdown threshold E_k = 3.2 × 10⁶N/N₀. The corresponding optical emissions occur at higher altitudes but with reduced intensities due to the lower electron densities at higher altitudes being less conducive to the generation of excited number density (n_k in Section 2.1) of N₂. For higher height parameter h’, the intensities of sprite halos obviously weaken. It is possible that in some sprite events, only sprite streamers are observed; sprite halos may indeed exist, but they are not observed because their diffuse emissions are too weak to be detected.

Same as Figure 3 but for the same height parameter h’ = 85 km, Figure 4 shows the simulation results for different ionospheric sharpness parameters β ranging from 0.3 to 0.9 km⁻¹. The corresponding electron density profiles, altitude profiles of the normalized electric field E/E_k, optical emission intensities directly above the charge center, and the time-averaged optical emission intensity of N₂ 1P over a time of 2 ms are shown in Figure 4b,c,d, respectively. The normalized electric field E/E_k above the reference height h’ = 85 km decreases with increasing β due to the enhanced electric field relaxation caused by the increased electron density. However, within the altitude range of ~72 to 85 km, the normalized electric field values exhibit an opposite trend, as the decreased electron density leads to reduced electric field relaxation. As the sharpness parameter β increases, the optical emission intensities (N₂ 1P) are reduced at the higher altitudes, similar to the results shown in Figure 3, and the vertical distribution of the optical radiation becomes more confined. The corresponding time-averaged optical emission of N₂ 1P occurs at relatively higher altitudes but with a lower intensity, and it also has a reduced vertical scale.

Figure 5 further compares the results of daytime and nighttime ionospheric electron density profiles. Altitude electron density profiles are shown in Figure 5a; for a typical nighttime ionosphere condition, h’ is set to 85 km and β is set to 0.5 km⁻¹, while for a typical daytime ionosphere condition h’ is set to 72 km and β is set to 0.3 km⁻¹ [20]. The normalized electric field E/E_k cross-section at x = 0 km at t = 1 ms and the normalized electric field altitude profiles directly above the charge center over a 2 ms period after lightning discharges for both daytime and nighttime are shown in Figure 5b,c, respectively. The cross-section of normalized electric field E/E_k is pancake-shaped; altitude profiles of E/E_k appear at high altitude and exhibit a downward developing trend. Compared to the nighttime ionosphere condition, the daytime ionospheric electron density profile has a lower reference height and a lower sharpness parameter, which means that the daytime electron density is greater than the nighttime condition at the same altitude. The increase in electron density leads to more shielding and relaxation of the electric field; thus, the normalized electric field generated by the same +CG discharge is concentrated at the lower altitude of 60~70 km for daytime. In addition to electric field relaxation, the amplitude of the normalized electric field E/E_k for daytime is reduced due to the higher neutral density N at lower altitudes, which leads to a higher breakdown threshold E_k = 3.2 × 10⁶N/N₀. The corresponding time-averaged optical emission of N₂ 1P over a time of 2 ms is shown in Figure 5d; under the same lightning discharge conditions, the optical emission intensities (N₂ 1P) are reduced for daytime at the lower altitudes, and the horizontal distribution range of the optical emission is smaller than that at night. The results indicate that daytime ionospheric conditions are not conducive to the generation of sprite halos optical emissions considering the same lightning discharges. Sprite halos’ optical emission in the daytime requires a larger lightning charge moment change, and the altitude of the sprite halos is lower than that at night. A similar conclusion was obtained by Tonev and Velinov [27], but for the formation of sprites.

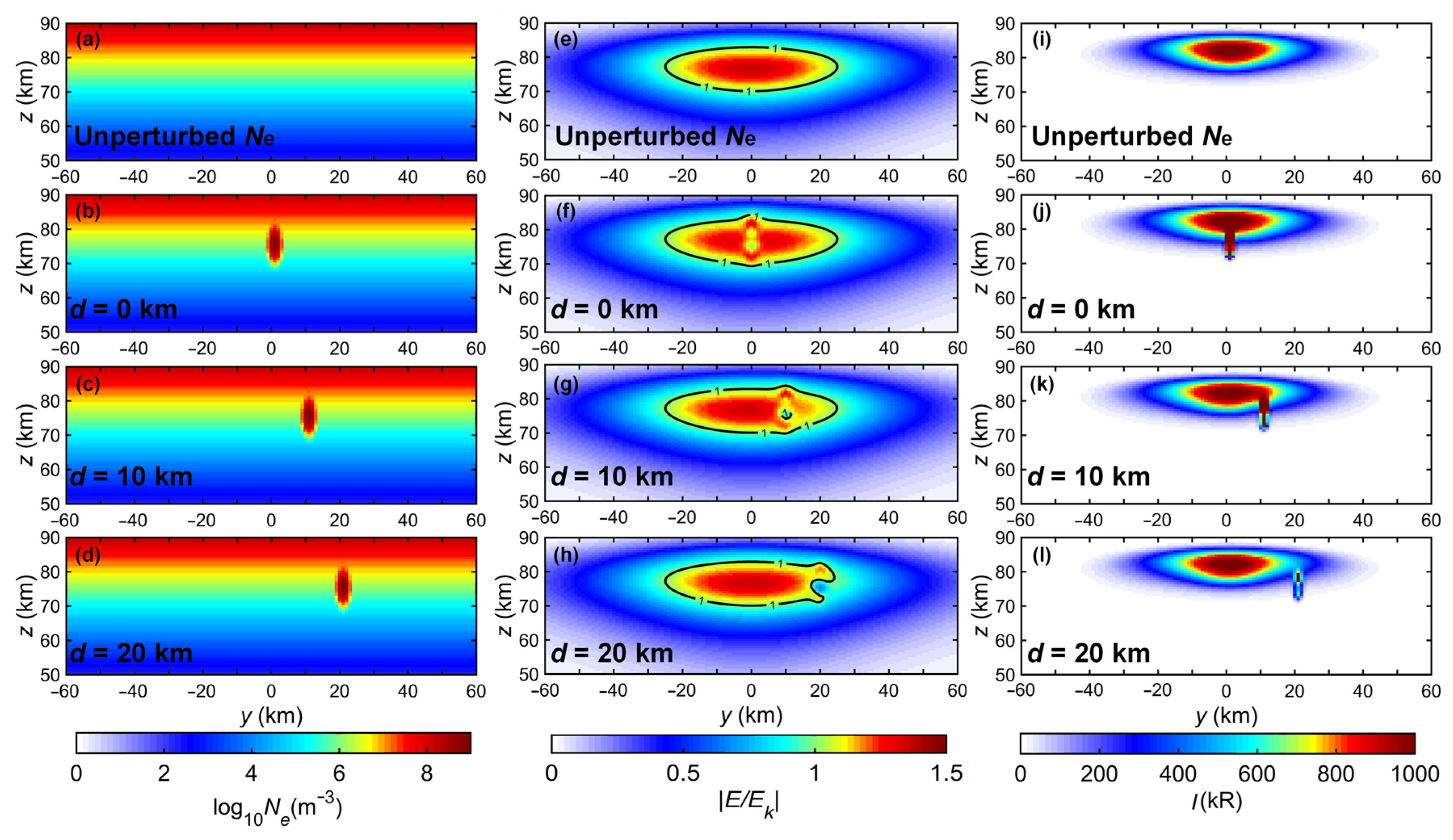
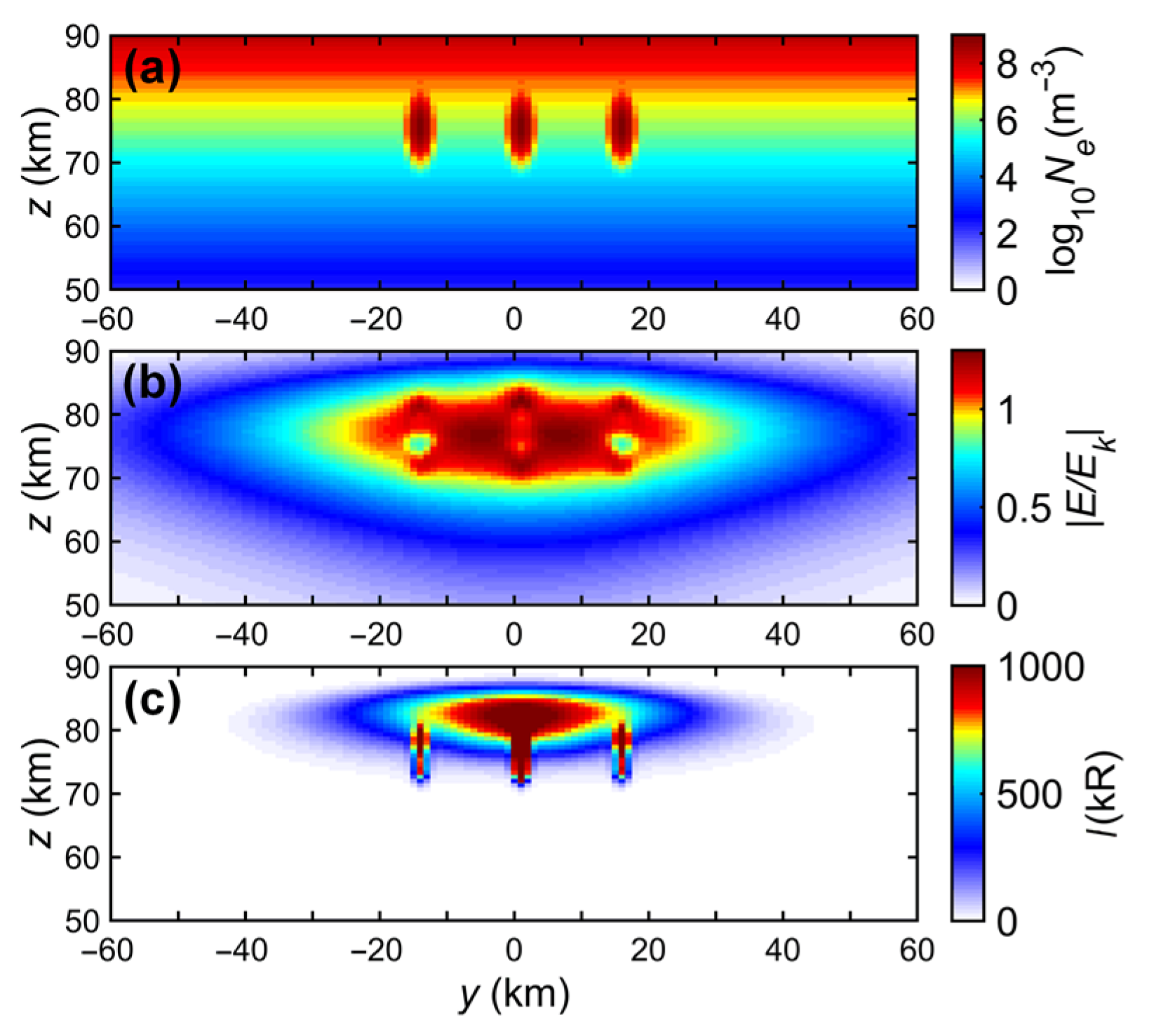
3.2. Effect of the Local Electron Density Inhomogeneities

Based on the nighttime ionospheric electron density profile with h’ = 85 km and β = 0.5 km⁻¹, a local electron density inhomogeneity characterized by N_peak = 1 × 10⁹ m⁻³ is placed at 75 km altitude, the horizontal distance between the inhomogeneity and the thundercloud charge center is represented by d along the y-axis in the 3D model, and the other parameter set is introduced in Section 2.2. For comparative analysis, the case of the nighttime electron density profile without the inhomogeneity (namely unperturbed N_e) is also shown in the simulation results.

Figure 6 illustrates the effects of a local electron density inhomogeneity. Figure 6a–d show the altitude distribution of the electron density without and with ionospheric inhomogeneity, considering different horizontal shifts from the thundercloud charge center (d = 0~20 km). Figure 6e–h display the corresponding vertical distribution of the normalized electric field E/E_k in a cross-sectional view at x = 0 km, at t = 1 ms. It is obvious from Figure 6f–h that the normalized electric field E/E_k around the inhomogeneous region of electron density exceeds that of other regions at the same height. However, the amplitude of the normalized electric field E/E_k inside the local region is lower, which can be explained by the electrical screening effect of the surrounding rapid increase in electron density on the internal electric field. In other words, the electron density inside the inhomogeneous region is very high, leading to rapid relaxation of the electric field. Although our 3D QES model cannot simulate streamer discharges, the simulation results can also provide a reference for qualitative studies of sprites. In brief, the existence of local electron density inhomogeneity alters the atmospheric conductivity, affecting the amplitude and morphological distribution of local electric fields and making certain positions more prone to electrical breakdown, which may facilitate the possible generation of sprite streamers. The key role of local electron density inhomogeneity in producing sprite streamers has been confirmed by Qin et al. [40].

Figure 6i–l further gives the simulated time-averaged optical emission intensity of N₂ 1P over a 2 ms period. It can be clearly seen that the optical emission intensity at the position of local inhomogeneity is obviously enhanced, while the main portion of the sprite halos still maintains a pancake-like outline. It is reasonable to speculate that when the electron density inhomogeneity has different morphological scales, the deformations of sprite halos emissions will be different, such as the electron density perturbed by a sinusoidal gravity wave [35]. In previous observations of Moudry et al. [53], some sprite halos are structured to some extent or have a large-scale lobe, which may be caused by electron density inhomogeneities. In our 3D model, the electron density inhomogeneity at any position can be simulated. When the local electron density inhomogeneity is displaced from the tropospheric thundercloud charge center, the position of local electrical breakdown also shifts, which may offer a possible explanation for the horizontal displacement between sprites and their parent lightning discharges, as observed in many studies [54,55,56].

Figure 7 further demonstrates the effects of multiple inhomogeneities; three electron density inhomogeneities are assumed to be distributed in the lower ionosphere at 15 km intervals. The altitude distribution of the electron density is depicted in Figure 7a. Figure 7b illustrates the cross-sectional view of the normalized electric field at x = 0 km at the end of the discharge. Similar to the results of Figure 6, the local normalized electric fields are enhanced, corresponding to the position of the electron density inhomogeneities, which is highly favorable for the possible generation of multiple sprite streamers. Figure 7c displays the time-averaged optical emission intensity of N₂ 1P over a 2 ms period. The simulation results can be utilized to explain the emergence of a sprite cluster, where multiple sprite streamers appear simultaneously. As Pasko et al. [8] suggested, the inhomogeneities of the upper atmosphere may contribute to the spatial and temporal structure observed in sprites.

4. Conclusions

In this study, the impacts of the ionospheric electron density on the sprite halos were modeled using the 3D QES heating model, including both the large-scale vertical profile of electron density and local electron density inhomogeneity. The ionospheric electron density was modeled by a two-parameter exponential profile using ionospheric density distribution according to Wait and Spies [18], and the electron density inhomogeneity in the lower ionosphere has a Gaussian density distribution. The main conclusions can be summarized as follows:

(1)

For the effect of nighttime ionospheric electron density with the same sharpness parameter β = 0.5 km⁻¹ and different height parameters h’ = 81~87 km, as the reference height h’ increases, the normalized electric field E/E_k above ~72 km penetrates to higher altitudes with increased amplitude. The corresponding sprite halos optical emissions occur at higher altitudes but with lower intensities.

(2)

For the effect of nighttime ionospheric electron density with the same height parameter h’ = 85 km and varying sharpness parameters β = 0.3~0.9 km⁻¹, as the sharpness parameter β increases, the corresponding optical emissions occur at higher altitudes but with reduced intensity and a smaller vertical extent.

(3)

Comparison results of ionospheric electron density between daytime and nighttime show that the normalized electric field E/E_k produced by the same +CG discharge penetrates to lower altitudes and has a reduced amplitude in the daytime, which is not favorable for initiating sprite halos optical emissions.

(4)

The presence of local electron density inhomogeneity leads to localized enhancements of the normalized electric field E/E_k and optical emission intensities. The horizontal shifts of these inhomogeneities offer a plausible explanation for the horizontal displacement between sprites and their parent lightning discharges. Furthermore, the effects of multiple inhomogeneities provide a possible cause for the formation of sprite clusters.

This paper focuses on the influence of electron density. The modulation of neutral density can also impact the generation of TLEs due to the strong dependence of the air breakdown threshold on the neutral density. Furthermore, for a +CG discharge considered in the present study, the positive charge Q = 150 C is removed from 10 km altitude within 1 ms, which is fixed in order to limit the number of variables in the parametric study. When considering lower charge or longer discharge time, the atmospheric parameterization scheme would need to be further updated; more species and more reactions need to be considered, especially the detachment process [47].