Calculation of Resonance Fluorescence Scattering Cross Sections of Metal Particles in the Middle and Upper Atmosphere and Comparison of Their Detectability
Abstract
1. Introduction
2. Resonance Fluorescence Scattering Cross Section
2.1. Calculation Method of Resonance Fluorescence Scattering Cross Section
2.2. Calculation of Scattering Cross Sections of Metal Atoms and Ions
2.3. Variation in Effective Backscattering Cross Section with Laser Linewidth
3. Discussion
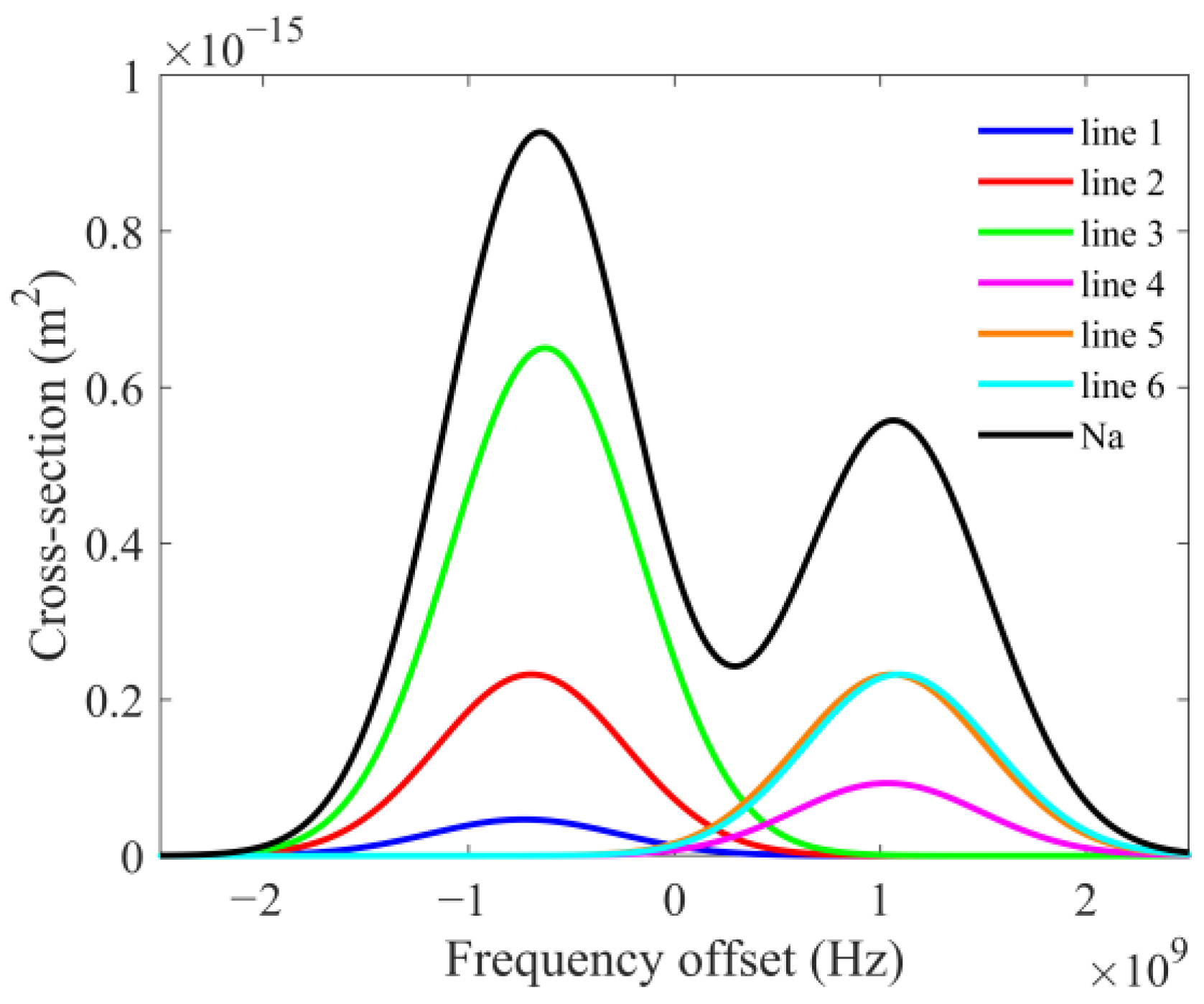
3.1. Variation in Effective Backscattering Cross Section of Sodium Atom with Laser Linewidth
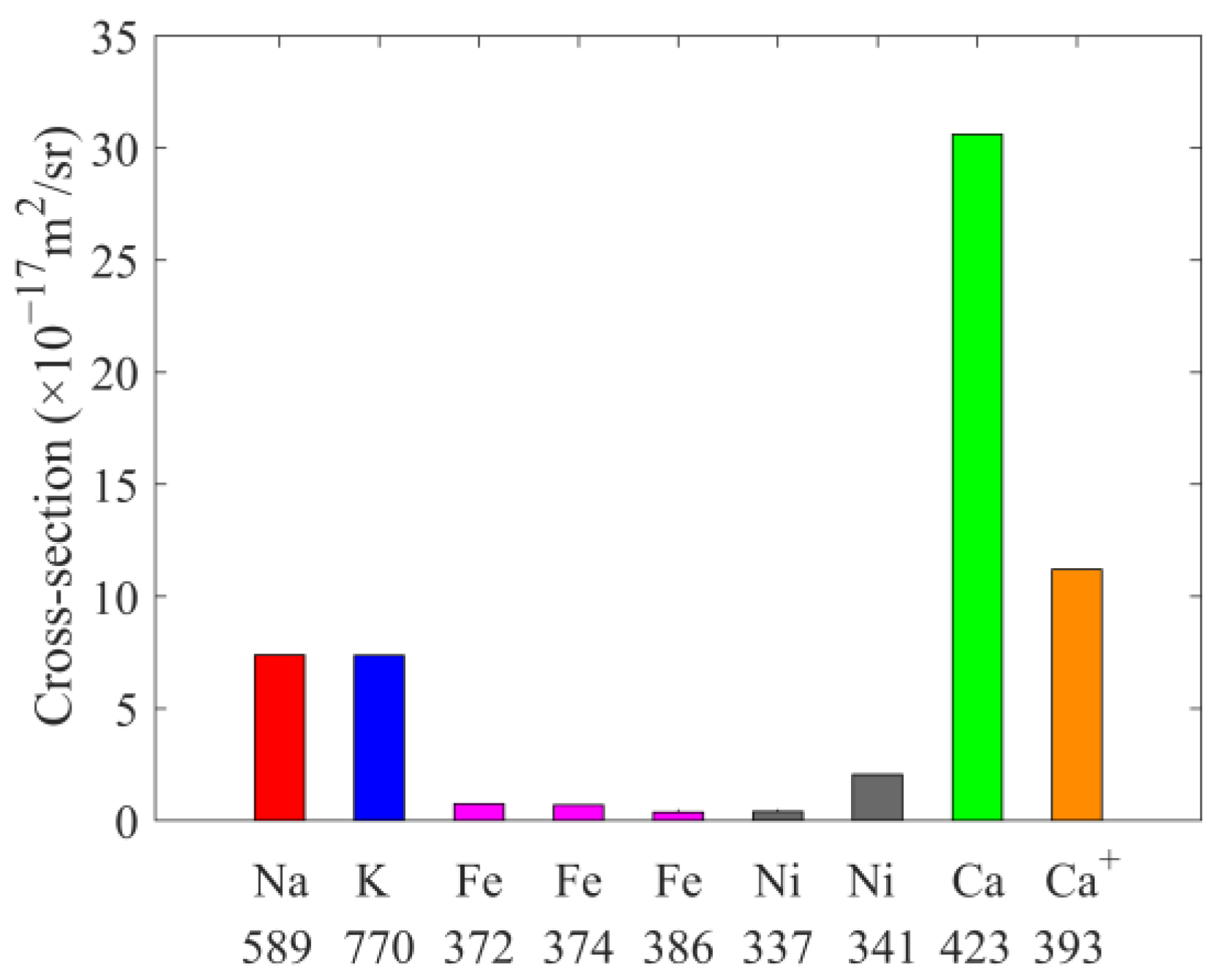
3.2. Comparison of Detectability of Metal Atoms and Ions
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Megie, G. Laser measurements of atmospheric trace constituents. Laser Remote Chem. Anal. 1988, 333, 437–443. [Google Scholar]
- Astafiev, O.; Zagoskin, A.M.; Abdumalikov Jr, A.; Pashkin, Y.A.; Yamamoto, T.; Inomata, K.; Nakamura, Y.; Tsai, J.S. Resonance fluorescence of a single artificial atom. Science 2010, 327, 840–843. [Google Scholar] [CrossRef] [PubMed]
- Gardner, C.S. Sodium resonance fluorescence lidar applications in atmospheric science and astronomy. Proc. IEEE 1989, 77, 408–418. [Google Scholar] [CrossRef]
- She, C.; Yu, J.; Latifi, H.; Bills, R. High-spectral-resolution fluorescence light detection and ranging for mesospheric sodium temperature measurements. Appl. Opt. 1992, 31, 2095–2106. [Google Scholar] [CrossRef] [PubMed]
- von Zahn, U.; Höffner, J. Mesopause temperature profiling by potassium lidar. Geophys. Res. Lett. 1996, 23, 141–144. [Google Scholar] [CrossRef]
- Fuhr, J.; Martin, G.; Wlese, W.; Younger, S. Atomic transition probabilities for iron, cobalt, and nickel (a critical data compilation of allowed lines). J. Phys. Chem. Ref. Data 1981, 10, 305–566. [Google Scholar] [CrossRef]
- Gardner, C.S.; Papen, G.C.; Chu, X.; Pan, W. First lidar observations of middle atmosphere temperatures, Fe densities, and polar mesospheric clouds over the North and South Poles. Geophys. Res. Lett. 2001, 28, 1199–1202. [Google Scholar] [CrossRef]
- Gerding, M.; Daly, S.; Plane, J. Lidar soundings of the mesospheric nickel layer using Ni (3F) and Ni (3D) transitions. Geophys. Res. Lett. 2019, 46, 408–415. [Google Scholar] [CrossRef]
- Wu, F.; Chu, X.; Du, L.; Jiao, J.; Zheng, H.; Xun, Y.; Feng, W.; Plane, J.M.; Yang, G. First Simultaneous Lidar Observations of Thermosphere-Ionosphere Sporadic Ni and Na (TISNi and TISNa) Layers (~105–120 km) Over Beijing (40.42° N, 116.02° E). Geophys. Res. Lett. 2022, 49, e2022GL100397. [Google Scholar] [CrossRef]
- Smith, G.; Raggett, D. Oscillator strengths and collisional damping parameters for lines of neutral calcium. J. Phys. B At. Mol. Phys. 1981, 14, 4015. [Google Scholar] [CrossRef]
- Granier, G.; Jégou, J.-P.; Mégie, G. Resonant lidar detection of Ca and Ca+ in the upper atmosphere. Geophys. Res. Lett. 1985, 12, 655–658. [Google Scholar] [CrossRef]
- Alpers, M.; Höfffner, J.; von Zahn, U. Upper atmosphere Ca and Ca+ at mid-latitudes: First simultaneous and common-volume lidar observations. Geophys. Res. Lett. 1996, 23, 567–570. [Google Scholar] [CrossRef]
- Abreu, V.J. Wind measurements from an orbital platform using a lidar system with incoherent detection: An analysis. Appl. Opt. 1979, 18, 2992–2997. [Google Scholar] [CrossRef] [PubMed]
- Yeh, S.-D.; Browell, E.V. Shuttle lidar resonance fluorescence investigations. 2: Analysis of thermospheric Mg+ measurements. Appl. Opt. 1982, 21, 2373–2380. [Google Scholar] [CrossRef]
- Raizada, S.; Smith, J.; Lautenbach, J.; Aponte, N.; Perillat, P.; Sulzer, M.; Mathews, J. New lidar observations of Ca+ in the mesosphere and lower thermosphere over arecibo. Geophys. Res. Lett. 2020, 47, e2020GL087113. [Google Scholar] [CrossRef]
- Gerding, M.; Alpers, M.; Höffner, J.; Von Zahn, U. Sporadic Ca and Ca+ layers at mid-latitudes: Simultaneous observations and implications for their formation. In Annales Geophysicae; Springer: Berlin/Heidelberg, Germany, 2001; pp. 47–58. [Google Scholar]
- Fujii, T.; Fukuchi, T. Resonance fluorescence lidar for measurements of the middle and upper atmosphere. In Laser Remote Sensing; CRC Press: Boca Raton, FL, USA, 2005; pp. 197–450. [Google Scholar]
- Yi, F.; Zhang, S.; Zeng, H.; He, Y.; Yue, X.; Liu, J.; Lv, H.; Xiong, D. Lidar observations of sporadic Na layers over Wuhan (30.5° N, 114.4° E). Geophys. Res. Lett. 2002, 29, 1–4. [Google Scholar] [CrossRef]
- Bowman, M.; Gibson, A.; Sandford, M. Atmospheric sodium measured by a tuned laser radar. Nature 1969, 221, 456–457. [Google Scholar] [CrossRef]
- Gelbwachs, J.A. Iron Boltzmann factor LIDAR: Proposed new remote-sensing technique for mesospheric temperature. Appl. Opt. 1994, 33, 7151–7156. [Google Scholar] [CrossRef]
- Collins, R.; Li, J.; Martus, C. First lidar observation of the mesospheric nickel layer. Geophys. Res. Lett. 2015, 42, 665–671. [Google Scholar] [CrossRef]
- Kane, T.J.; Gardner, C.S. Lidar observations of the meteoric deposition of mesospheric metals. Science 1993, 259, 1297–1300. [Google Scholar] [CrossRef]
- Siegman, A.E. Lasers; University Science Books: Dulles, VA, USA, 1986. [Google Scholar]
- Bills, R.E.; Gardner, C.S.; She, C.Y. Narrowband lidar technique for sodium temperature and Doppler wind observations of the upper atmosphere. Opt. Eng. 1991, 30, 13–21. [Google Scholar] [CrossRef]
- Wu, F.; Zheng, H.; Yang, Y.; Cheng, X.; Li, F.; Du, L.; Wang, J.; Jiao, J.; Plane, J.M.; Feng, W. Lidar observations of the upper atmospheric nickel layer at Beijing (40° N, 116° E). J. Quant. Spectrosc. Radiat. Transf. 2021, 260, 107468. [Google Scholar] [CrossRef]
- Lautenbach, J.; Höffner, J. Scanning iron temperature lidar for mesopause temperature observation. Appl. Opt. 2004, 43, 4559–4563. [Google Scholar] [CrossRef] [PubMed]
- Hasinoff, S.W. Photon, Poisson Noise. Comput. Vis. A Ref. Guide 2014, 4, 16. [Google Scholar]
- Krueger, D.A.; She, C.-Y.; Yuan, T. Retrieving mesopause temperature and line-of-sight wind from full-diurnal-cycle Na lidar observations. Appl. Opt. 2015, 54, 9469–9489. [Google Scholar] [CrossRef]
- Meyer, V.R. Measurement uncertainty. J. Chromatogr. A 2007, 1158, 15–24. [Google Scholar] [CrossRef] [PubMed]
- Gardner, C.S.; Kane, T.J.; Senft, D.C.; Qian, J.; Papen, G.C. Simultaneous observations of sporadic E, Na, Fe, and Ca+ layers at Urbana, Illinois: Three case studies. J. Geophys. Res. Atmos. 1993, 98, 16865–16873. [Google Scholar] [CrossRef]



| Wavelength (nm) | Atomic or Ionic Mass (×10−26 kg) | Oscillator Strength (f) | References | |
|---|---|---|---|---|
| Na | 589.158 | 3.705 | 0.6408 | Megie (1988) [1] |
| 39K | 769.898 | 6.468 | 0.3327 | von Zahn (1996) [5] |
| 41K | 769.898 | 6.780 | 0.3327 | von Zahn (1996) [5] |
| Fe | 372.099 | 9.288 | 0.0414 | Fuhr (1981) [6] |
| 373.819 | 9.288 | 0.0382 | Fuhr (1981) [6] | |
| 385.991 | 9.288 | 0.0217 | Fuhr (1981) [6] | |
| Ni | 336.956 | 9.761 | 0.024 | Gerding (2019) [8] |
| 341.476 | 9.761 | 0.12 | Gerding (2019) [8] | |
| Ca | 422.673 | 6.665 | 1.75 | Granier (1985) [11] |
| Ca+ | 393.366 | 6.665 | 0.69 | Granier (1985) [11] |
| Hyperfine Structure Line | 2S1/2 | 2P3/2 | Frequency Offset (GHz) | Relative Line Strength (fi) | |
|---|---|---|---|---|---|
| D2a | 1 | F=2 | F=1 | −0.7150 | 1/32 |
| 2 | F=2 | F=2 | −0.6806 | 5/32 | |
| 3 | F=2 | F=3 | −0.6216 | 14/32 | |
| D2b | 4 | F=1 | F=0 | 1.0408 | 2/32 |
| 5 | F=1 | F=1 | 1.0566 | 5/32 | |
| 6 | F=1 | F=2 | 1.0911 | 5/32 |
| 2S1/2 | 2P1/2 | Offset (GHz) | Relative Line Strength (fi) | |
|---|---|---|---|---|
| 39K | 41K | |||
| F=1 | F=2 | 0.310 | 0.405 | 5/16 |
| F=1 | F=1 | 0.254 | 0.375 | 1/16 |
| F=2 | F=2 | −0.152 | 0.151 | 5/16 |
| F=2 | F=1 | −0.208 | 0.121 | 5/16 |
| Wavelength (nm) | Peak Backscattering Cross-Section (m2/sr) | Peak Absorption Cross-Section (m2) | Other’s Value of Peak Absorption Cross-Section (m2) | References | |
|---|---|---|---|---|---|
| Na | 589.158 | 7.38 × 10−17 | 9.27 × 10−16 | 1.50 × 10−15 | Gardner (1989) [3] |
| ~9.25 × 10−16 | Bills (1991) [24] | ||||
| K | 769.898 | 7.37 × 10−17 | 9.26 × 10−16 | 1.34 × 10−15 | Gardner (1989) [3] |
| ~9.25 × 10−16 | von Zahn (1996) [5] | ||||
| Fe | 372.099 | 7.53 × 10−18 | 9.46 × 10−17 | 9.43 × 10−17 | Gardner (1989) [3] |
| 373.819 | 6.98 × 10−18 | 8.77 × 10−17 | 8.80 × 10−17 | Gelbwachs (1994) [20] | |
| 385.991 | 3.75 × 10−18 | 4.72 × 10−17 | 4.88 × 10−17 | Lautenbach (2004) [26] | |
| Ni | 336.956 | 4.05 × 10−18 | 5.09 × 10−17 | - | - |
| 341.476 | 2.05 × 10−17 | 2.58 × 10−16 | - | - | |
| Ca | 422.673 | 3.06 × 10−16 | 3.85 × 10−15 | 3.85 × 10−15 | Gardner (1989) [3] |
| Ca+ | 393.366 | 1.12 × 10−16 | 1.41 × 10−15 | 1.41 × 10−15 | Gardner (1989) [3] |
| Wave Length (nm) | Effective Backscattering Cross-Section (m2/sr) | Density (cm−3) | References of Density | Ratio of Relative Uncertainty to Na | |
|---|---|---|---|---|---|
| Na | 589.158 | 4.07 × 10−17 | ~5000 | Gardner (1993) [30] | 1 |
| K | 769.898 | 3.83 × 10−17 | ~60 | von Zahn (1996) [5] | 8.23 |
| Fe | 372.099 | 4.43 × 10−18 | ~20,000 | Gelbwachs (1994) [20] | 1.91 |
| 373.819 | 4.09 × 10−18 | ~780 | Gelbwachs (1994) [20] | 10.03 | |
| 385.991 | 2.16 × 10−18 | ~20,000 | Lautenbach (2004) [26] | 2.68 | |
| Ni | 336.956 | 2.50 × 10−18 | ~300 | Wu (2021) [25] | 21.79 |
| 341.476 | 1.26 × 10−17 | ~300 | Wu (2021) [25] | 9.65 | |
| Ca | 422.673 | 1.85 × 10−16 | ~30 | Alpers (1996) [12] | 7.15 |
| Ca+ | 393.366 | 7.08 × 10−17 | ~200 | Alpers (1996) [12] | 4.64 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, K.; Wang, Z.; Wu, Y.; Xia, Y.; Xun, Y.; Wu, F.; Jiao, J.; Du, L. Calculation of Resonance Fluorescence Scattering Cross Sections of Metal Particles in the Middle and Upper Atmosphere and Comparison of Their Detectability. Atmosphere 2023, 14, 1283. https://doi.org/10.3390/atmos14081283
Wang K, Wang Z, Wu Y, Xia Y, Xun Y, Wu F, Jiao J, Du L. Calculation of Resonance Fluorescence Scattering Cross Sections of Metal Particles in the Middle and Upper Atmosphere and Comparison of Their Detectability. Atmosphere. 2023; 14(8):1283. https://doi.org/10.3390/atmos14081283
Chicago/Turabian StyleWang, Kexin, Zelong Wang, Yuxuan Wu, Yuan Xia, Yuchang Xun, Fuju Wu, Jing Jiao, and Lifang Du. 2023. "Calculation of Resonance Fluorescence Scattering Cross Sections of Metal Particles in the Middle and Upper Atmosphere and Comparison of Their Detectability" Atmosphere 14, no. 8: 1283. https://doi.org/10.3390/atmos14081283
APA StyleWang, K., Wang, Z., Wu, Y., Xia, Y., Xun, Y., Wu, F., Jiao, J., & Du, L. (2023). Calculation of Resonance Fluorescence Scattering Cross Sections of Metal Particles in the Middle and Upper Atmosphere and Comparison of Their Detectability. Atmosphere, 14(8), 1283. https://doi.org/10.3390/atmos14081283








