A Study of the Pulse Cleaning Process for Metal Fiber Filter Bags Based on a Discrete Phase Particle Deposition Model
Abstract
:1. Introduction
2. Materials and Methods
2.1. Model Assumptions and Simplifications
- (1)
- The presence of solid particles (type of solid particles and forces applied to them) is assumed not to affect flow patterns;
- (2)
- The filter bag is represented as a cylindrical surface, and its deformation in operation is not considered;
- (3)
- Air parameters at room temperature are used for the gas parameters;
- (4)
- A uniform inlet air velocity distribution is assumed;
- (5)
- The fluid is assumed to be isothermal and incompressible for constant flow.
2.2. Airflow Phase Model
2.3. Discrete Phase Model
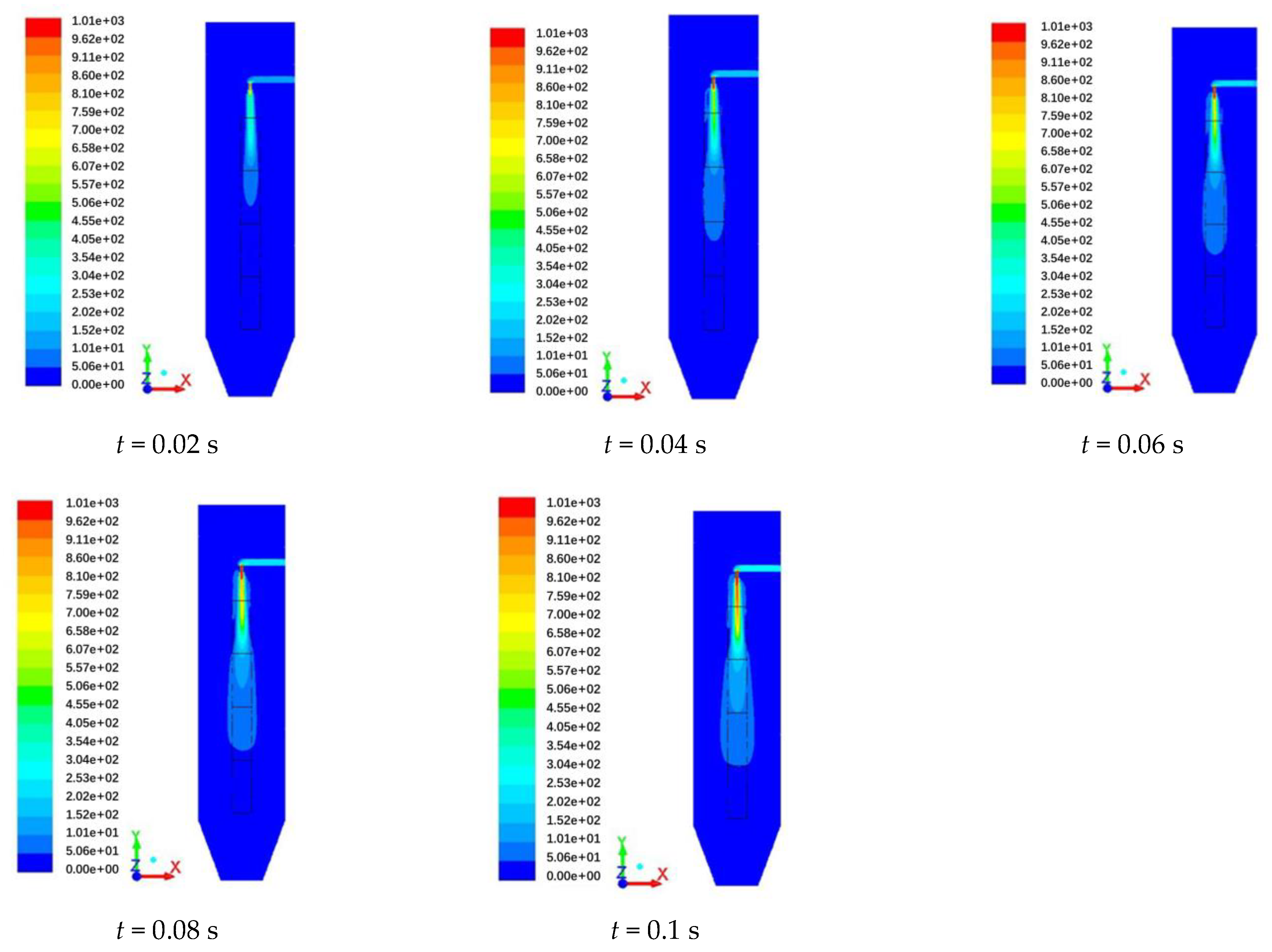
2.4. Geometric Models
2.5. Boundary Conditions
- (1)
- Inlet and outlet boundary conditions: the speed of the gas was less than 68 m/s; the compressibility of the gas could be ignored [17]. Due to the pulse blowing cleaning, its blowing airflow was released from the nozzle within a very short time at a speed greater than 68 m/s, so the gas compressibility could not be ignored. The inlet boundary condition was, therefore, set as a pressure inlet. The outlet boundary conditions were set to pressure outlet boundary conditions.
- (2)
- Wall boundary conditions: the model of the entire box wall, flower plate, the bottom of the filter bag, blowing tube, and nozzle outer wall were set as the wall boundary conditions; each wall was set to no slip.
- (3)
- The boundary conditions of the filter bag: using a porous jump media model, under the different particle deposition distributions, in the simulation of the dust collector pulse cleaning process, the 1400 mm-long filter bag was divided into four parts.
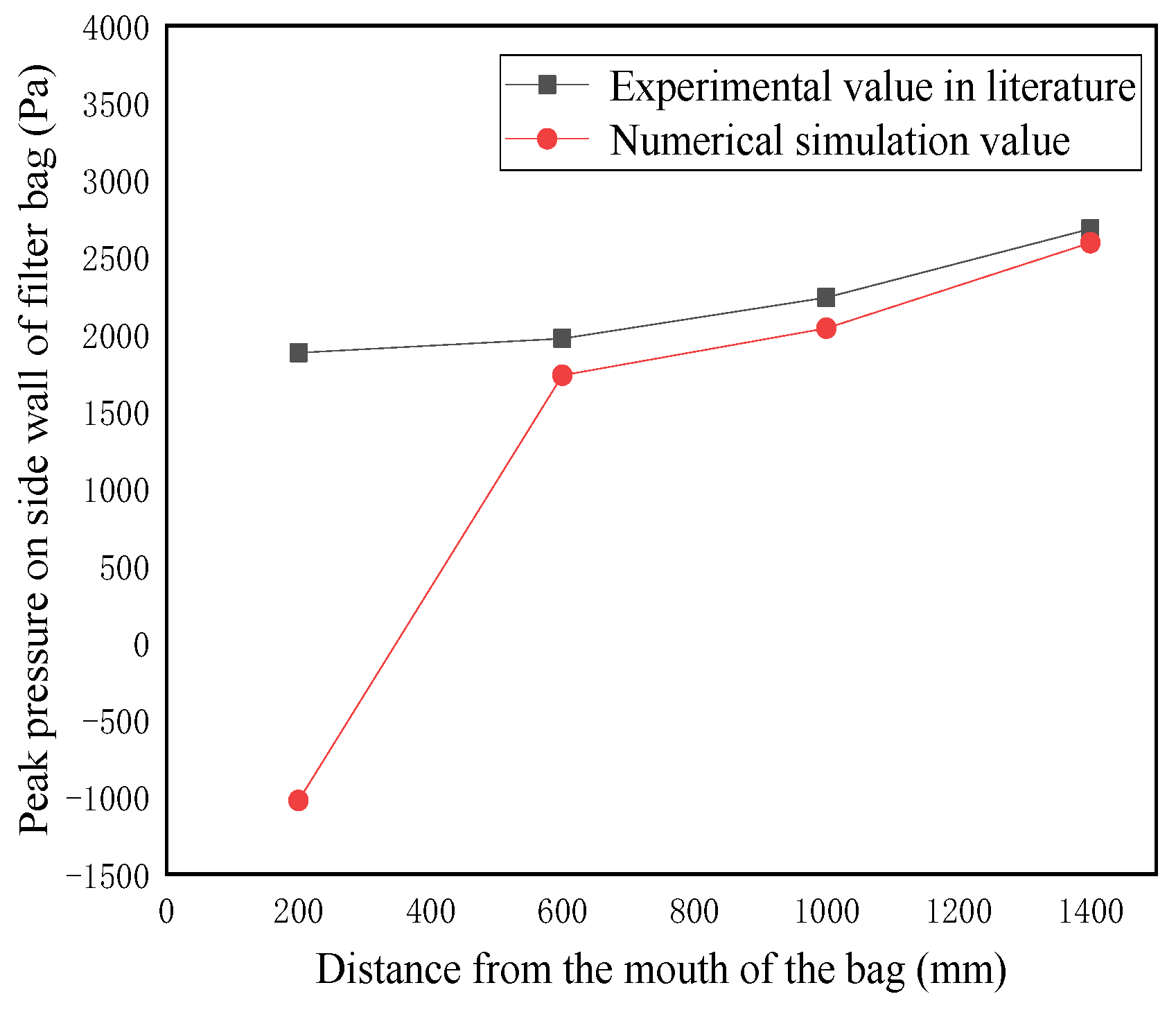
2.6. Validation of Numerical Calculation Models
3. Results and Discussion
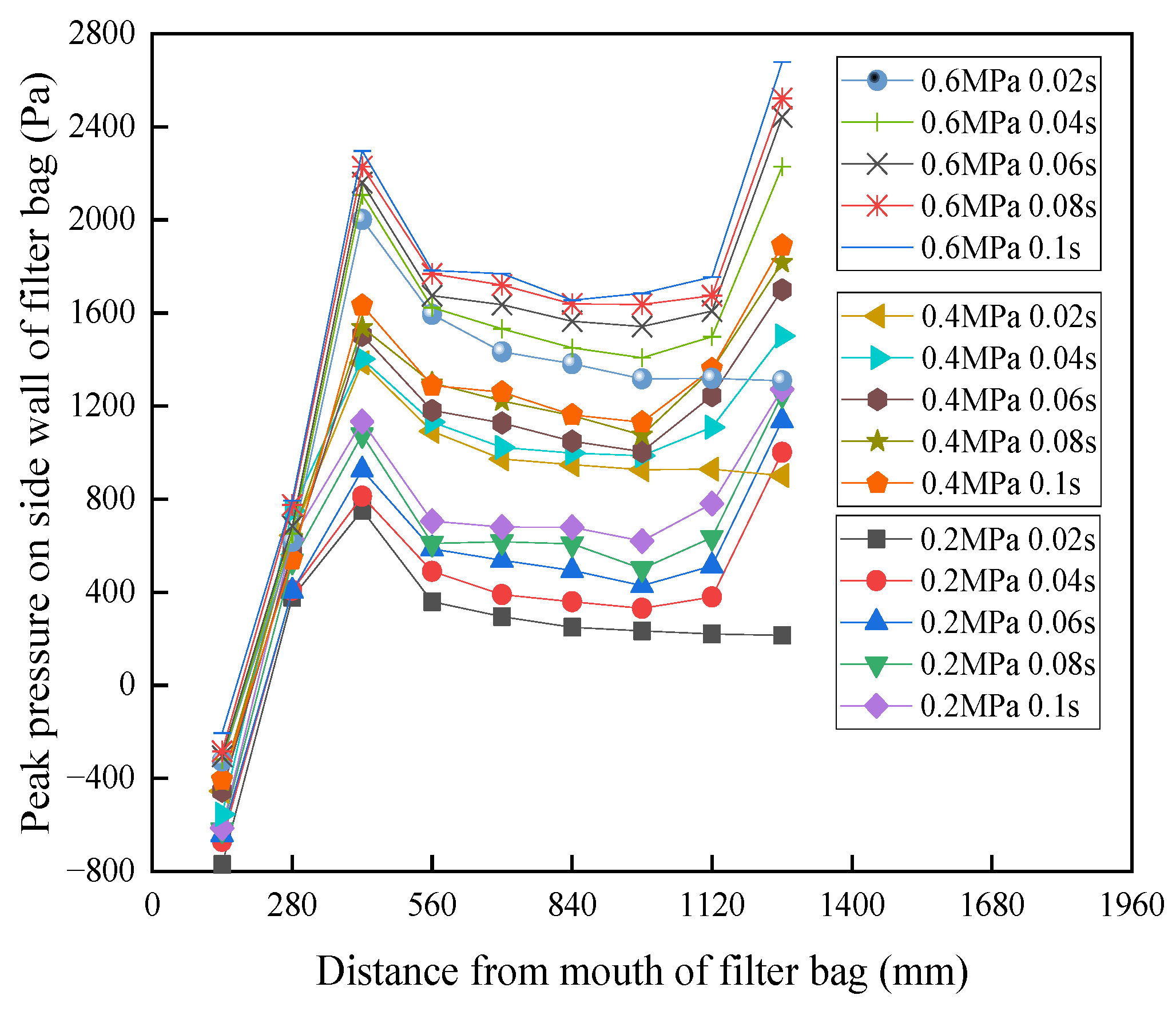
3.1. Influence of Blowing Pressure on the Effect of Ash Cleaning
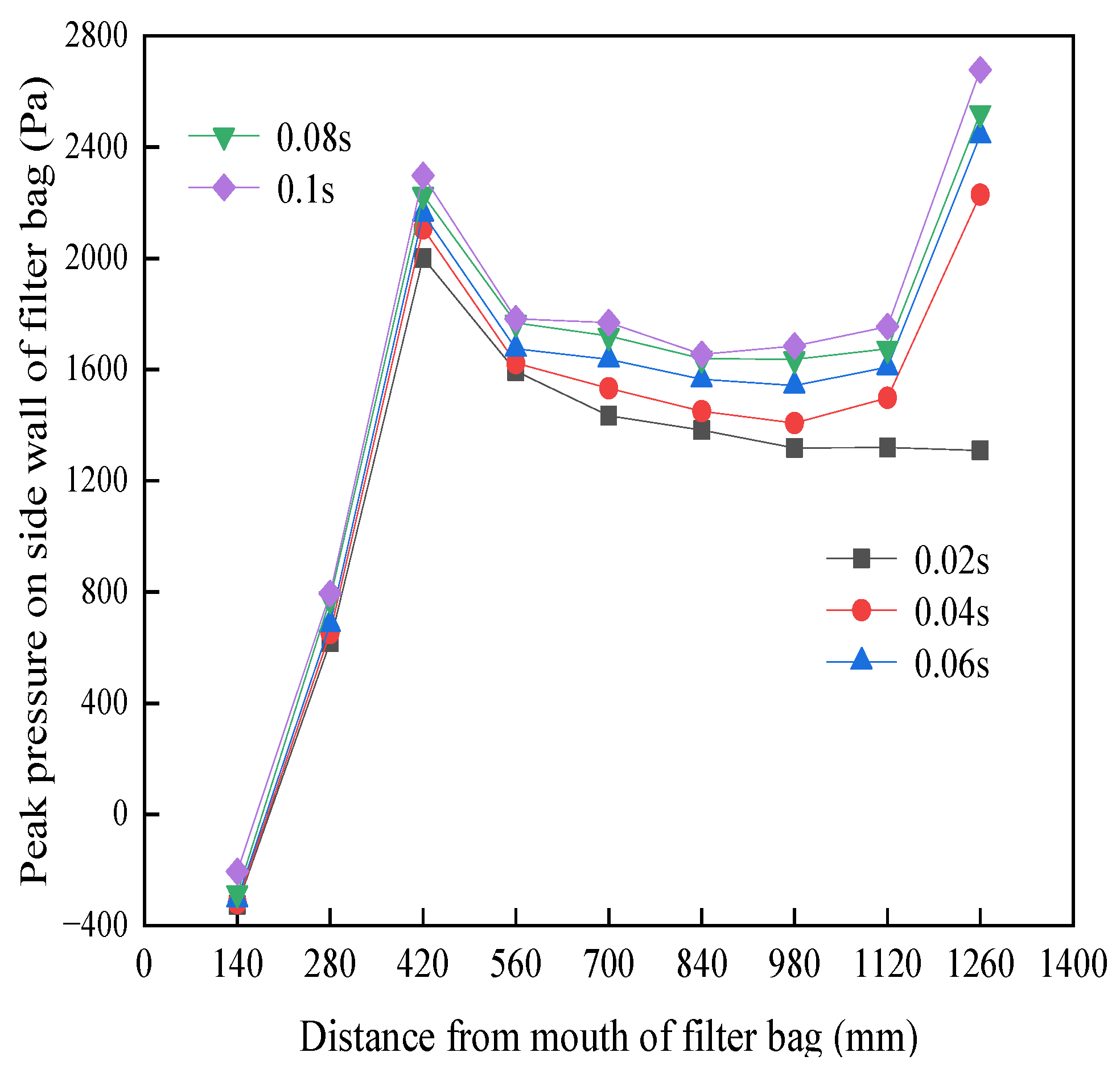
3.2. Influence of Pulse width on Ash Cleaning Effect
3.3. Optimum Operating Parameters for Ash Cleaning
4. Conclusions
- (1)
- The peak pressure on the side wall of the filter bag increases with the increase in the pulse width, but the blowing time should be determined within a certain range, according to the length of the filter bag and the dust content on the surface of the filter bag.
- (2)
- The effect of blowing pressure on the peak pressure on the side wall of the filter bag is more obvious compared to the pulse width. In actual production, we must choose the dust cleaning operation parameters by considering the dust adhesion on the surface of the filter bag and the length of the filter bag; when the filter bag is longer, the pulse width should also be increased to a certain extent.
- (3)
- When the dust load on the surface of the filter bag is small, 0.6 MPa and 0.08 s are selected as the best operating parameters. These operation parameters guarantee an excellent dust cleaning effect to the maximum extent, while avoiding excessive dust cleaning. At the same time, in actual production, the dust cleaning operation parameters should be flexibly adjusted, taking into account the dust adhesion on the surface of the filter bag and the length of the filter bag, which has a guiding meaning for the regulation of operating parameters.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Zhang, M.; Qin, W.; Ma, X.; Liu, A.; Yan, C.; Li, P.; Huang, M.; He, C. An Environmentally Friendly Technology of Metal Fiber Bag Filter to Purify Dust-Laden Airflow. Atmosphere 2022, 13, 13030485. [Google Scholar]
- Wang, P.; Cao, Y.; Luo, H.; Li, T.; Yang, B.; Li, H.; Liang, T.; Yu, J.; Wang, L.; Ma, F.; et al. Remarkable enrichment of heavy metals in baghouse filter dust during direct-fired thermal desorption of contaminated soil. J. Hazard. Mater. 2022, 430, 128301. [Google Scholar]
- Lv, Z.Y.; Yu, Y.; Ren, M.; Dang, T.; Wu, S.; Zhou, H.; Gao, L.; Yue, J.; Zhang, H.; Chen, J. Spraying polyacrylamide solution to improve the removal of particle-phase dioxins by bag filter in a full-scale municipal solid waste incineration system. Chemosphere 2021, 285, 131392. [Google Scholar] [CrossRef] [PubMed]
- Liu, X.; Shen, H. Effect of Venturi Structures on the Cleaning Performance of a Pulse Jet Baghouse. Appl. Sci. 2019, 9, 3687. [Google Scholar]
- Thomas, D.; Penicot, P.; Contal, P.; Leclerc, D.; Vendel, J. Clogging of fibrous filters by solid aerosol particles experimental and modelling study. Chem. Eng. Sci. 2001, 56, 3549–3561. [Google Scholar] [CrossRef]
- Jeon, K.J.; Jung, Y.-W. A simulation study on the compression behavior of dust cakes. Powder Technol. 2004, 141, 1–11. [Google Scholar] [CrossRef]
- Simon, X.; Chazelet, S.; Thomas, D.; Bémer, D.; Régnier, R. Experimental study of pulse-jet cleaning of bag filters supported by rigid rings. Powder Technol. 2007, 172, 67–81. [Google Scholar] [CrossRef]
- Wilis Cândido Pereira, T.; Bezerra Marques, F.; Fábio de Assis Ressel, P.; Cunha Ribeiro, D.; Mara Santana Rocha, S. The influence of the fabric filter layout of in a flow mass filtrate. J. Clean. Prod. 2016, 111, 117–124. [Google Scholar] [CrossRef]
- Lo, L.M.; Hu, S.C.; Chen, D.R.; Pui, D.Y. Numerical study of pleated fabric cartridges during pulse-jet cleaning. Powder Technol. 2009, 198, 75–81. [Google Scholar] [CrossRef]
- Rothwell, E. Pulse-driven injectors for fabric dust filters III: Comparative performance of model and commercial assemblies. Filtr. Sep. 1990, 27, 345–349. [Google Scholar] [CrossRef]
- Danyin, Z.; Chun, W. Handbook of Pulsed Baghouse Dust Collectors, 1st ed.; Chemical Industry Press: Beijing, China, 2011; p. 125. [Google Scholar]
- Rongrong, A.; Xuedong, L.; Wenming, L.; Xinyue, D.; Tao, P. Analysis of airflow field uniformity in metal fiber bag dust collector. China Powder Technol. 2020, 26, 22–30. [Google Scholar]
- Yanzhen, Y.; Yuanhui, Y.; Jiangyuan, L.; Liangjun, L. Research on the uniformity of airflow in the internal flow field of baghouse dust collectors. Mach. Manag. Dev. 2013, 5, 45–46. [Google Scholar]
- Ling, P.; Yanzhen, Y. Numerical simulation of the internal flow field of baghouse dust collectors. J. Environ. Eng. 2012, 8, 2750–2754. [Google Scholar]
- Jingxia, Z.; Henggen, S.; Aimin, F.; Jin, L. Numerical simulation analysis of airflow in the blowing tube of baghouse dust collector. Power Environ. Prot. 2008, 3, 30–32. [Google Scholar]
- Rocha, S.; Marques, F.; Pereira, F. Applications of CFD techniques in the design of fabric filters. Chem. Eng. Trans. 2014, 39, 1369–1374. [Google Scholar]
- Zengji, C.; Tianyu, L. Fluid Mechanics Pumps and Fans, 5th ed.; China Construction Industry Press: Beijing, China, 1999; p. 154. [Google Scholar]
- Liu, H.C.; Tsai, C.J. Influence of Design and Operation Parameters on Bag-Cleaning Performance of Pulse-Jet Baghouse. J. Environ. Eng. 1999, 125, 583–891. [Google Scholar]
- Cirqueira, S.S.R.; Tanabe, E.H.; Aguiar, M.L. Experimental investigation of particle deposition in filter media during filtration cycles with regeneration by pulse jet cleaning. Process Saf. Environ. Prot. 2019, 127, 288–298. [Google Scholar] [CrossRef]
- He, C.; Yan, C.; Tang, C.; Huang, M.; Ren, L.; Zhang, M. Nitrogen pulse jet cleaning of the pleated filter cartridge clogged with adhesive hygroscopic dusts. Process Saf. Environ. Prot. 2021, 147, 430–438. [Google Scholar]
- Piao, C.X.; Ha, H.C.; Kim, S.J. Experimental Study on Optimum Pulse Jet Cleaning Conditions of a Cartridge Filter System. J. Korean Soc. Occup. Environ. Hyg. 2015, 25, 542–553. [Google Scholar]
- Chen, L.; Liu, Z.; Sun, Y.; Qian, F.; Han, Y.; Lu, J. Experimental Study on the Dust-Cleaning Performance of New Structure Microporous Membrane Filter Plate. Atmosphere 2022, 13, 817. [Google Scholar]
- Shi, D.; Li, J.; Du, Y.; Wu, Q.; Huang, S.; Huang, H.; Wu, D. Influence of Relative Humidity on the Characteristics of Filter Cake Using Particle Flow Code Simulation. Atmosphere 2022, 13, 770. [Google Scholar]




Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, W.; Xu, Z.; Liu, X. A Study of the Pulse Cleaning Process for Metal Fiber Filter Bags Based on a Discrete Phase Particle Deposition Model. Atmosphere 2023, 14, 156. https://doi.org/10.3390/atmos14010156
Liu W, Xu Z, Liu X. A Study of the Pulse Cleaning Process for Metal Fiber Filter Bags Based on a Discrete Phase Particle Deposition Model. Atmosphere. 2023; 14(1):156. https://doi.org/10.3390/atmos14010156
Chicago/Turabian StyleLiu, Wenming, Zhiqiang Xu, and Xuedong Liu. 2023. "A Study of the Pulse Cleaning Process for Metal Fiber Filter Bags Based on a Discrete Phase Particle Deposition Model" Atmosphere 14, no. 1: 156. https://doi.org/10.3390/atmos14010156
APA StyleLiu, W., Xu, Z., & Liu, X. (2023). A Study of the Pulse Cleaning Process for Metal Fiber Filter Bags Based on a Discrete Phase Particle Deposition Model. Atmosphere, 14(1), 156. https://doi.org/10.3390/atmos14010156




