Modelling Methane Adsorption Isotherms on Shale at Different Temperatures
Abstract
:1. Introduction
2. Basic Theory
2.1. Polanyi Adsorption Potential
2.2. Virtual Saturated Vapor Pressure
2.3. Adsorption Volume
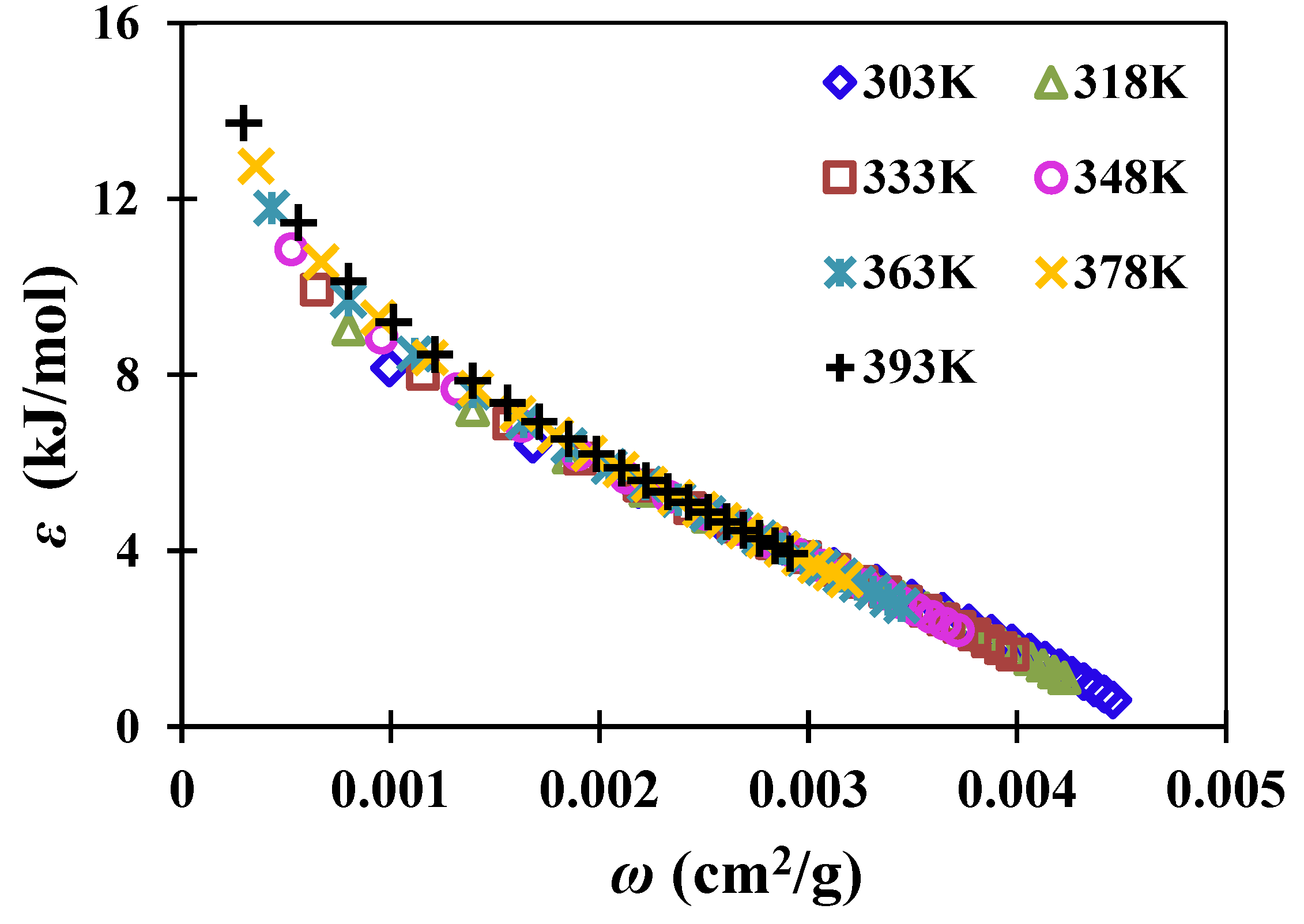
2.4. Characteristic Curve
3. Method and Validation
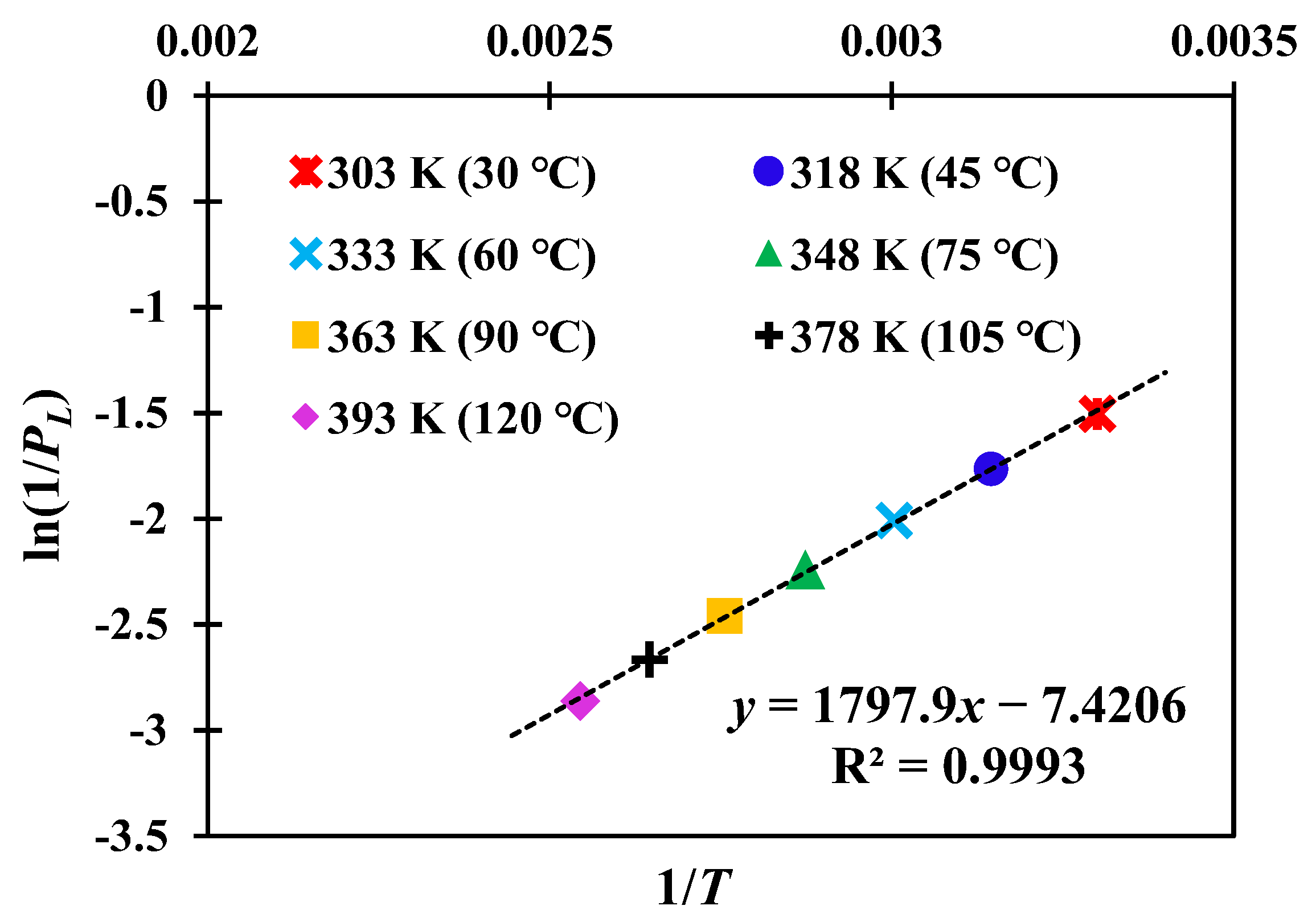
3.1. Relationship between Langmuir Pressure at Different Temperatures
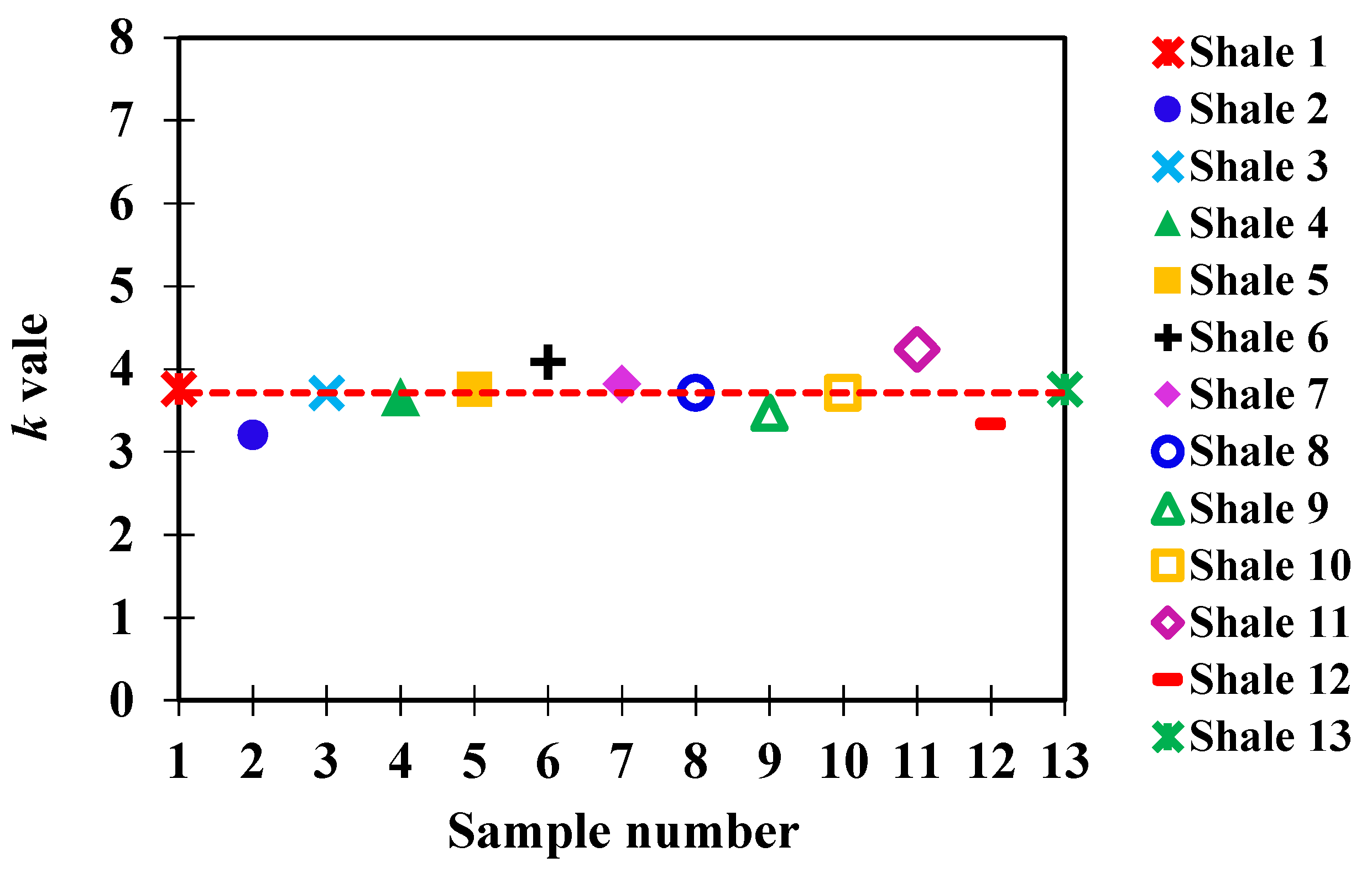
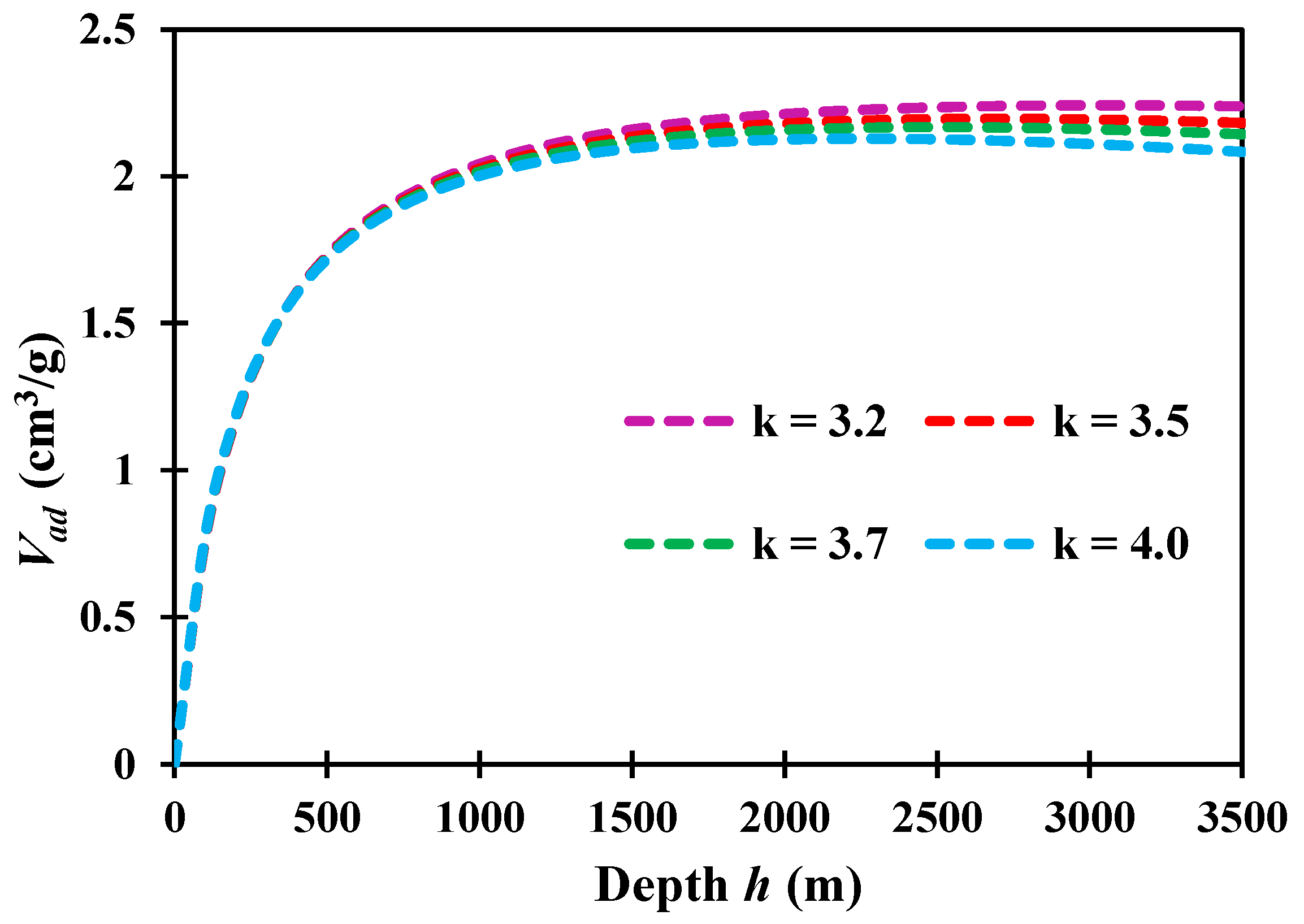
3.2. Estimating k Value for Methane Adsorption on Shale
3.3. Model Validation
4. Results and Discussions
4.1. Basic Data for Shale Formation
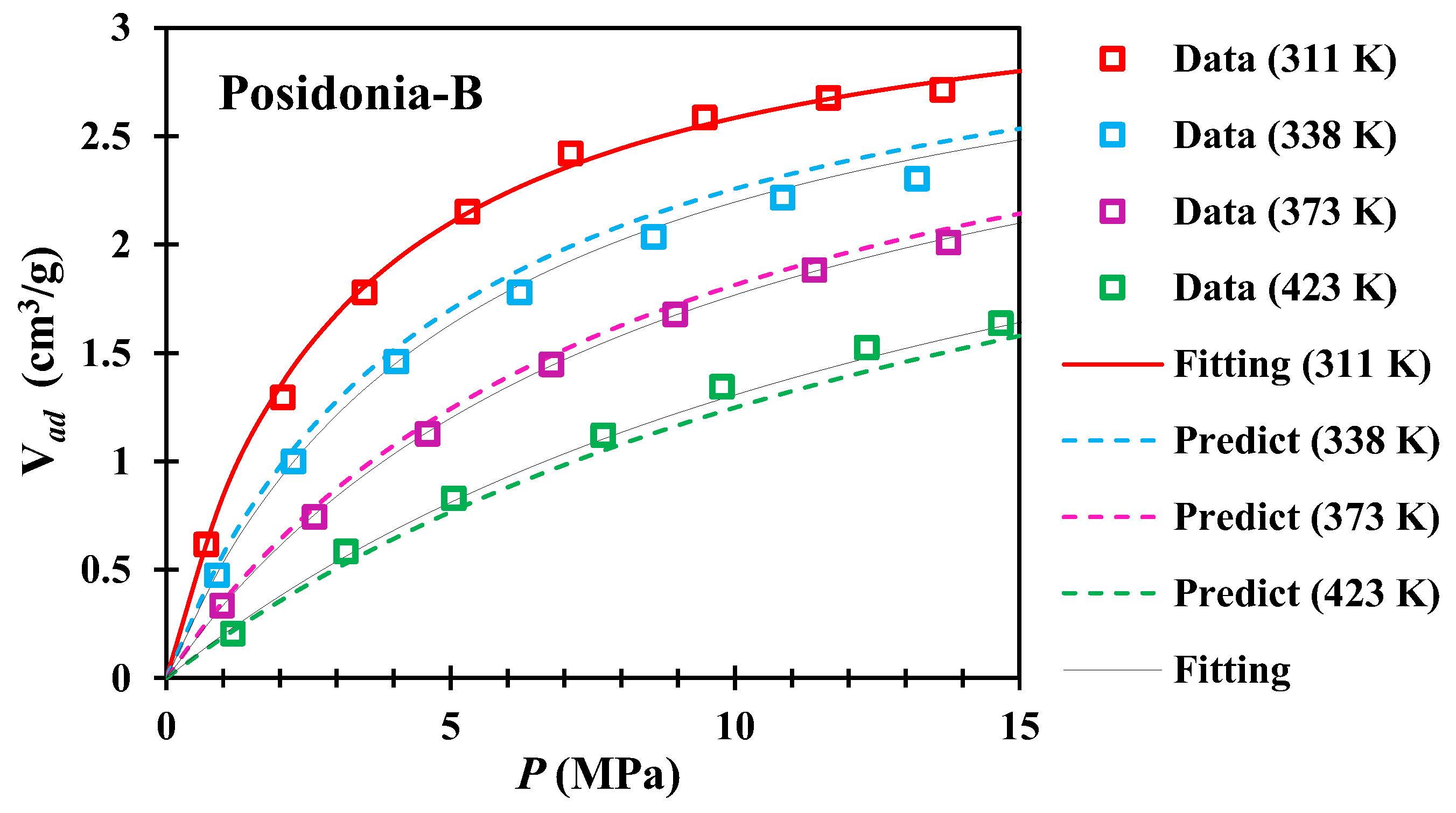
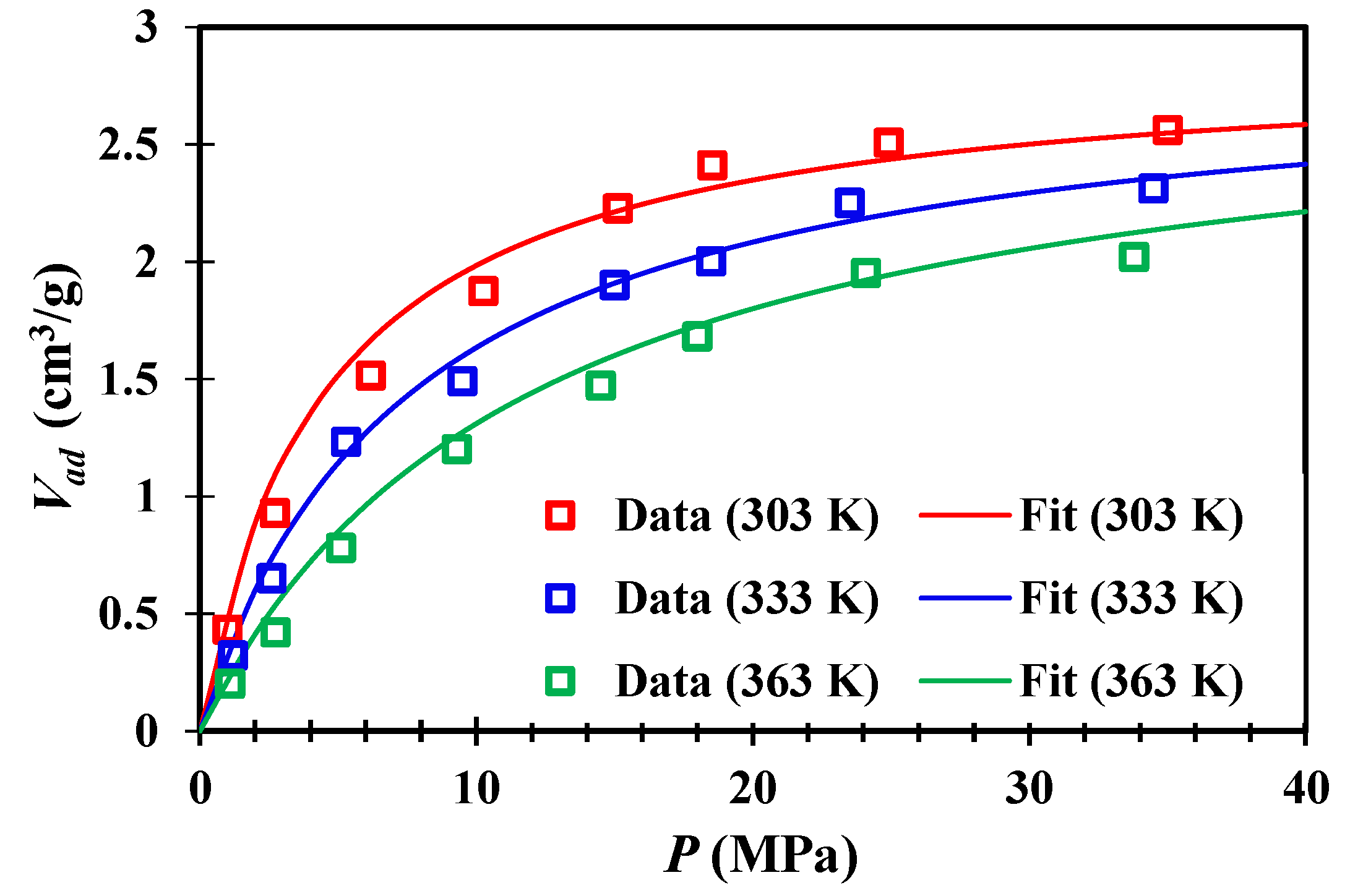
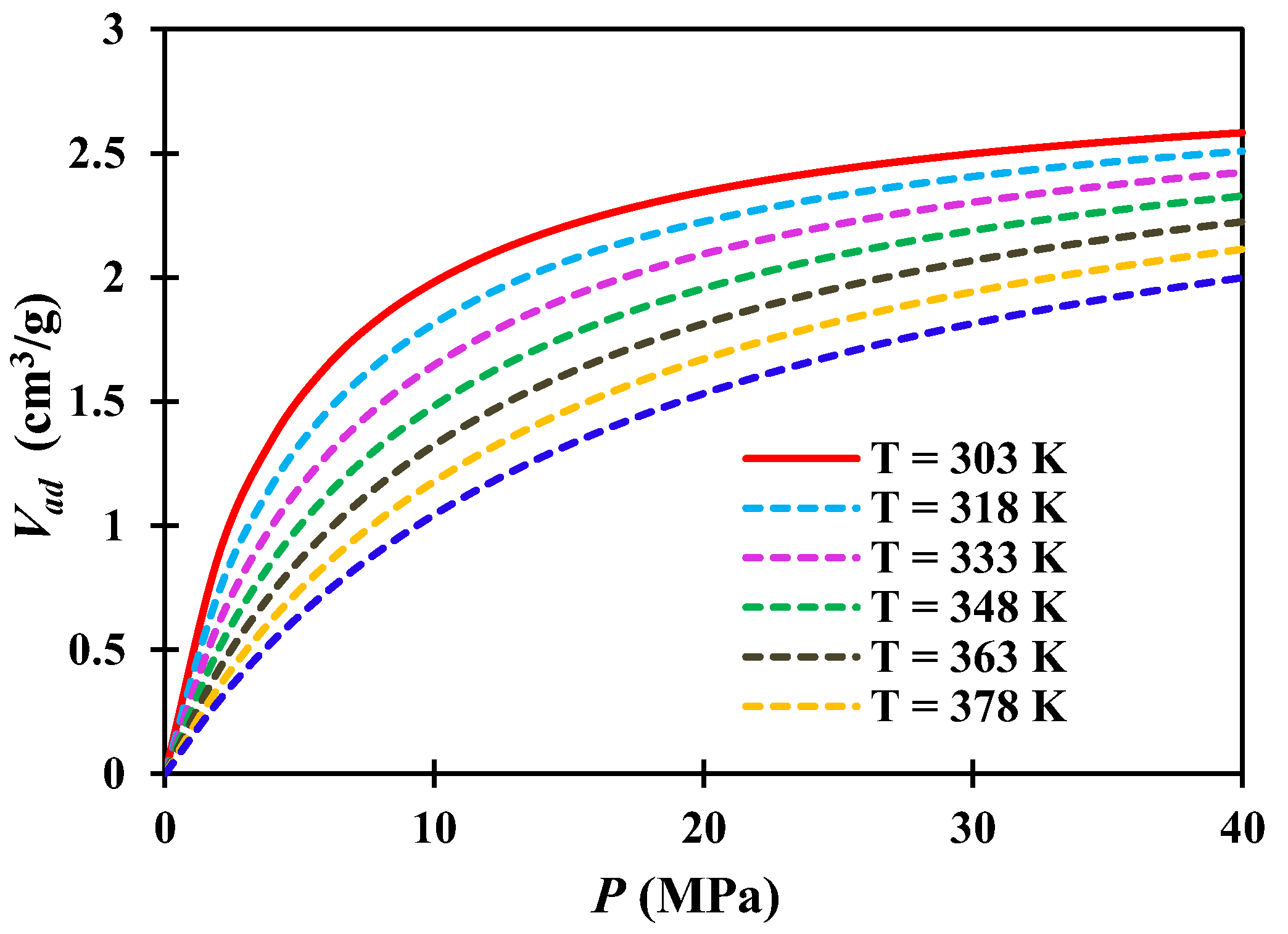
4.2. Prediction of Adsorption Isotherms
4.3. Adsorption Characteristic Curve
4.4. Isosteric Heat of Adsorption
5. Applications
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A
| Sample | T (K) | 1/T | PL (MPa) | lnPL | X | Y | k |
|---|---|---|---|---|---|---|---|
| WIC-149 (Shale 1) | 311.53 | 0.00321 | 7.58 | 2.03 | 152.89 | −160.04 | 3.7632 |
| 337.84 | 0.00296 | 12.86 | 2.55 | 193.20 | −351.94 | ||
| 373.13 | 0.00268 | 18.86 | 2.94 | 250.46 | −531.62 | ||
| HAR-038 (Shale 2) | 311.53 | 0.00321 | 8.34 | 2.12 | 152.89 | −189.64 | 3.2078 |
| 337.84 | 0.00296 | 12.60 | 2.53 | 193.20 | −345.18 | ||
| 373.13 | 0.00268 | 17.58 | 2.87 | 250.46 | −505.50 | ||
| HAR-060 (Shale 3) | 311.53 | 0.00321 | 7.51 | 2.02 | 152.89 | −156.93 | 3.7154 |
| 337.84 | 0.00296 | 11.91 | 2.48 | 193.20 | −325.92 | ||
| 373.13 | 0.00268 | 18.36 | 2.91 | 250.46 | −521.54 | ||
| HAD-119 (Shale 4) | 311.53 | 0.00321 | 5.92 | 1.78 | 152.89 | −82.78 | 3.6668 |
| 337.84 | 0.00296 | 9.84 | 2.29 | 193.20 | −261.40 | ||
| 373.13 | 0.00268 | 14.91 | 2.70 | 250.46 | −443.93 | ||
| Haynesville (Shale 5) | 311.53 | 0.00321 | 3.05 | 1.12 | 152.89 | 123.45 | 3.7594 |
| 337.84 | 0.00296 | 5.71 | 1.74 | 193.20 | −77.61 | ||
| 373.13 | 0.00268 | 8.84 | 2.18 | 250.46 | −248.78 | ||
| Alum_D (Shale 6) | 311.53 | 0.00321 | 4.24 | 1.44 | 152.89 | 21.27 | 4.0876 |
| 337.84 | 0.00296 | 6.90 | 1.93 | 193.20 | −141.46 | ||
| 358.42 | 0.00279 | 9.87 | 2.29 | 226.17 | −278.40 | ||
| Alum_O (Shale 7) | 311.53 | 0.00321 | 3.73 | 1.32 | 152.89 | 60.83 | 3.8243 |
| 337.84 | 0.00296 | 6.67 | 1.90 | 193.20 | −129.98 | ||
| 373.13 | 0.00268 | 10.59 | 2.36 | 250.46 | −316.32 | ||
| Alum_G (Shale 8) | 311.53 | 0.00321 | 2.82 | 1.04 | 152.89 | 148.06 | 3.7144 |
| 337.84 | 0.00296 | 5.32 | 1.67 | 193.20 | −53.63 | ||
| 373.13 | 0.00268 | 8.18 | 2.10 | 250.46 | −220.05 | ||
| Alum_S2-16 (Shale 9) | 311.53 | 0.00321 | 2.36 | 0.86 | 152.89 | 204.13 | 3.4737 |
| 337.84 | 0.00296 | 4.05 | 1.40 | 193.20 | 38.60 | ||
| 373.13 | 0.00268 | 6.56 | 1.88 | 250.46 | −137.59 | ||
| Alum_S2-18 (Shale 10) | 311.53 | 0.00321 | 2.14 | 0.76 | 152.89 | 233.42 | 3.7144 |
| 337.84 | 0.00296 | 3.64 | 1.29 | 193.20 | 74.41 | ||
| 373.13 | 0.00268 | 6.25 | 1.83 | 250.46 | −119.30 | ||
| 423.73 | 0.00236 | 11.63 | 2.45 | 338.30 | −399.04 | ||
| Barnett_M1-576 (Shale 11) | 311.53 | 0.00321 | 5.66 | 1.73 | 152.89 | −68.76 | 4.239 |
| 337.84 | 0.00296 | 9.70 | 2.27 | 193.20 | −256.67 | ||
| 373.13 | 0.00268 | 16.61 | 2.81 | 250.46 | −484.23 | ||
| Barnett_M1-586a (Shale 12) | 311.53 | 0.00321 | 7.07 | 1.96 | 152.89 | −138.23 | 3.3383 |
| 337.84 | 0.00296 | 9.93 | 2.30 | 193.20 | −264.77 | ||
| 373.13 | 0.00268 | 15.89 | 2.77 | 250.46 | −467.81 | ||
| Barnett_B1 (Shale 13) | 311.53 | 0.00321 | 3.26 | 1.18 | 152.89 | 103.20 | 3.7591 |
| 337.84 | 0.00296 | 6.12 | 1.81 | 193.20 | −101.26 | ||
| 373.13 | 0.00268 | 11.13 | 2.41 | 250.46 | −334.98 | ||
| 423.73 | 0.00236 | 18.82 | 2.94 | 338.30 | −602.85 |
References
- Li, X.; Wang, X.; Li, Y.; Wu, K.; Shi, J.; Yang, L.; Feng, D.; Zhang, T.; Yu, P. Water distribution characteristic and effect on methane adsorption capacity in shale clay. Int. J. Coal Geol. 2016, 159, 135–154. [Google Scholar] [CrossRef]
- Li, J.; Li, X.; Wu, K.; Wang, X.; Shi, J.; Yang, L.; Zhang, H.; Sun, Z.; Wang, R.; Feng, D. Water sorption and distribution characteristics in clay and shale: Effect of surface force. Energy Fuels 2016, 30, 8863–8874. [Google Scholar] [CrossRef]
- Li, J.; Li, X.; Wu, K.; Feng, D.; Zhang, T.; Zhang, Y. Thickness and stability of water film confined inside nanoslits and nanocapillaries of shale and clay. Int. J. Coal Geol. 2017, 179, 253–268. [Google Scholar] [CrossRef]
- Guo, W.; Gao, S.; Hu, Z.; Liu, H.; Yu, R. Impact of temperature on the isothermal adsorption/desorption of shale gas. Pet. Explor. Dev. 2013, 40, 514–519. [Google Scholar] [CrossRef]
- Tang, X.; Ripepi, N.; Stadie, N.P.; Yu, L. Thermodynamic analysis of methane adsorption on gas shale. J. Cent. South Univ. 2014, 45, 2871–2877. [Google Scholar]
- Ross, D.J.K.; Bustin, M.R. Characterizing the shale gas resource potential of Devonian–Mississippian strata in the Western Canada sedimentary basin: Application of an integrated formation evaluation. AAPG Bull. 2008, 92, 87–125. [Google Scholar] [CrossRef]
- Gasparik, M.; Bertier, P.; Gensterblum, Y.; Ghanizadeh, A.; Krooss, B.M.; Littke, R. Geological controls on the methane storage capacity in organic-rich shales. Int. J. Coal Geol. 2014, 123, 34–51. [Google Scholar] [CrossRef]
- Rexer, T.F.T.; Benham, M.J.; Aplin, A.C.; Thomas, K.M. Methane Adsorption on Shale under Simulated Geological Temperature and Pressure Conditions. Energy Fuels 2013, 27, 3099–3109. [Google Scholar] [CrossRef] [Green Version]
- Gasparik, M.; Ghanizadeh, A.; Gensterblum, Y.; Kroossa, B.M. “Multi-temperature” method for high-pressure sorption measurements on moist shales. Rev. Sci. Instrum. 2013, 84, 1–9. [Google Scholar] [CrossRef]
- Polanyi, M. The Potential Theory of Adsorption. Science 1963, 141, 1010–1013. [Google Scholar] [CrossRef]
- Su, X.B.; Chen, R.; Lin, X.Y.; Song, Y. Application of adsorption potential theory in the fractionation of coalbed gas during the process of adsorption/desorption. Acta Geol. Sin. 2008, 82, 1382–1389. [Google Scholar]
- Du, X.; Wu, E. Application of the adsorption potential theory to hydrogen adsorption on zeolites above critical temperature. Acta Phys. Chim. Sin. 2007, 23, 813–819. [Google Scholar] [CrossRef]
- Thommes, M.; Kaneko, K.; Neimark, A.V.; Olivier, J.P.; Rodriguez-Reinoso, F.; Rouquerol, J.; Sing, K.S.W. Physisorption of gases, with special reference to the evaluation of surface area and pore size distribution (IUPAC Technical Report). Pure Appl. Chem. 2015, 87, 1051–1069. [Google Scholar] [CrossRef] [Green Version]
- Ramirez-Pastor, A.J.; Bulnes, F. Differential heat of adsorption in the presence of an order-disorder phase transition. Phys. A 2000, 283, 198–203. [Google Scholar] [CrossRef]
- Pang, Y.; Wang, S.; Yao, X.; Hu, X.; Chen, S. Evaluation of Gas Adsorption in Nanoporous Shale by Simplified Local Density Model Integrated with Pore Structure and Pore Size Distribution. Langmuir 2022, 38, 3641–3655. [Google Scholar] [CrossRef] [PubMed]
- Fu, J.; Tian, Y.; Wu, J. Classical density functional theory for methane adsorption in metal-organic framework materials. AIChE J. 2015, 61, 3012–3021. [Google Scholar] [CrossRef]
- Yu, X.; Li, J.; Chen, Z.; Wu, K.; Zhang, L.; Yang, S. Effects of helium adsorption in carbon nanopores on apparent void volumes and excess methane adsorption isotherms. Fuel 2020, 270, 117499. [Google Scholar] [CrossRef]
- Yu, X.; Li, J.; Chen, Z.; Wu, K.; Zhang, L. Effects of an adsorbent accessible volume on methane adsorption on shale. Comput. Methods Appl. Mech. Eng. 2020, 370, 113222. [Google Scholar] [CrossRef]
- Huang, L.; Ning, Z.; Wang, Q.; Qi, R.; Zeng, Y.; Qin, H.; Ye, H.; Zhang, W. Molecular simulation of adsorption behaviors of methane, carbon dioxide and their mixtures on kerogen: Effect of kerogen maturity and moisture content. Fuel 2018, 211, 159–172. [Google Scholar] [CrossRef]
- Yu, X.; Li, J.; Chen, Z.; Wu, K.; Zhang, L.; Yang, S.; Hui, G.; Yang, M. Determination of CH4, C2H6 and CO2 adsorption in shale kerogens coupling sorption-induced swelling. Chem. Eng. J. 2021, 410, 127690. [Google Scholar] [CrossRef]
- Getman, R.B.; Bae, Y.S.; Wilmer, C.E.; Snurr, R.Q. Review and analysis of molecular simulations of methane, hydrogen, and acetylene storage in metal–organic frameworks. Chem. Rev. 2012, 112, 703–723. [Google Scholar] [CrossRef] [PubMed]
- Li, J.; Wu, K.; Chen, Z.; Wang, K.; Luo, J.; Xu, J.; Li, R.; Yu, R.; Li, X. On the negative excess isotherms for methane adsorption at high pressure: Modeling and experiment. SPE J. 2019, 24, 2504–2525. [Google Scholar] [CrossRef]
- Ambrose, R.J.; Hartman, R.C.; Diaz-Campos, M.; Akkutlu, I.Y.; Sondergeld, C.H. Shale gas-in-place calculations part I: New pore-scale considerations. Spe J. 2012, 17, 219–229. [Google Scholar] [CrossRef]
- Langmuir, I. The evaporation, condensation and reflection of molecules and the mechanism of adsorption. Phys. Rev. 1916, 8, 149. [Google Scholar] [CrossRef]
- Langmuir, I. The adsorption of gases on plane surfaces of glass, mica and platinum. J. Am. Chem. Soc. 1918, 40, 1361–1403. [Google Scholar] [CrossRef] [Green Version]
- Li, J.; Li, X.; Shi, J.; Zhang, H.; Wu, K.; Chen, Z. Mechanism of liquid-phase adsorption and desorption in coalbed methane systems: A new insight into an old problem. SPE Reserv. Eval. Eng. 2017, 20, 639–653. [Google Scholar] [CrossRef]
- Li, J.; Chen, Z.; Wu, K.; Wang, K.; Luo, J.; Feng, D.; Qu, S.; Li, X. A multi-site model to determine supercritical methane adsorption in energetically heterogeneous shales. Chem. Eng. J. 2018, 349, 438–455. [Google Scholar] [CrossRef]
- Li, J.; Wu, K.; Chen, Z.; Wang, W.; Yang, B.; Wang, K.; Luo, J.; Yu, R. Effects of energetic heterogeneity on gas adsorption and gas storage in geologic shale systems. Appl. Energy 2019, 251, 113368. [Google Scholar] [CrossRef]
- Amankwah, K.A.G.; Schwarz, J.A. A modified approach for estimating pseudo-vapor pressures in the application of the Dubinin-Astakhov equation. Carbon 1995, 33, 1313–1319. [Google Scholar] [CrossRef]
- Dubinin, M.M. The Potential Theory of Adsorption of Gases and Vapors for Adsorbents with Energetically Nonuniform Surfaces. Chem. Rev. 1960, 60, 235–241. [Google Scholar] [CrossRef]
- Haydel, J.J.; Kobayashi, R. Adsorption Equilibria in Methane-Propane-Silica Gel System at High Pressures. Ind. Eng. Chem. Fundam. 1967, 6, 546–554. [Google Scholar] [CrossRef]
- Mavor, M.J.; Hartman, C.; Pratt, T.J. Uncertainty in sorption isotherm measurements. In Proceedings of the International Coalbed Methane Symposium, Tuscaloosa, AL, USA, 11 April 2004. [Google Scholar]
- Ji, L.; Zhang, T.; Milliken, K.L.; Qu, J.; Zhang, X. Experimental investigation of main controls to methane adsorption in clay-rich rocks. Appl. Geochem. 2012, 27, 2533–2545. [Google Scholar] [CrossRef]
- Liu, D.; Yuan, P.; Liu, H.; Li, T.; Tan, D.; Yuan, W.; He, H. High-pressure adsorption of methane on montmorillonite, kaolinite and illite. Appl. Clay Sci. 2013, 85, 25–30. [Google Scholar] [CrossRef]
- Zhang, T.; Ellis, G.; Ruppel, S.C.; Milliken, K.; Yang, R. Effect of organic matter type and thermal maturity on methane adsorption in shale-gas systems. Org. Geochem. 2012, 47, 120–131. [Google Scholar] [CrossRef]
- Xia, X.; Tang, Y. Isotope fractionation of methane during natural gas flow with coupled diffusion and adsorption/desorption. Geochim. Cosmochim. Acta 2012, 77, 489–503. [Google Scholar] [CrossRef]


| Sample | T (K) | Experiment Data | Model Prediction | |||
|---|---|---|---|---|---|---|
| Vm (cm3/g) | PL (MPa) | Fitting Accuracy | PL (MPa) | Prediction Accuracy | ||
| Posidonia-A Shale | 311 K | 2.24 | 4.12 | 99.1% | Use | |
| 338 K | 6.81 | 99.5% | 6.35 | 97.2% | ||
| 373 K | 11.92 | 99.8% | 10.83 | 96.1% | ||
| Posidonia-B Shale | 311 K | 3.36 | 3.01 | 99.3% | Use | |
| 338 K | 5.32 | 99.4% | 4.88 | 95.8% | ||
| 373 K | 9.06 | 99.7% | 8.52 | 97.5% | ||
| 423 K | 15.74 | 99.3% | 16.92 | 95.4% | ||
| Vm (cm3/g) | T (K) | PL (MPa) | Accuracy |
|---|---|---|---|
| 2.875 | 303 | 4.496 | 99.05% |
| 333 | 7.589 | 99.27% | |
| 363 | 11.938 | 99.46% |
| Vm (cm3/g) | T (K) | Predicted PL (MPa) | Experiment PL (MPa) | 1/T | ln(1/P) |
|---|---|---|---|---|---|
| 2.875 | 303 | 4.496 (use) | 4.496 (Table 2) | 0.0033 | −1.5040 |
| 318 | 5.839 | 0.0031 | −1.7646 | ||
| 333 | 7.459 | 7.589 (Table 2) | 0.0030 | −2.0095 | |
| 348 | 9.398 | 0.0028 | −2.2405 | ||
| 363 | 11.693 | 11.938 (Table 2) | 0.0027 | −2.4590 | |
| 378 | 14.385 | 0.0026 | −2.6662 | ||
| 393 | 17.518 | 0.0025 | −2.8632 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, D. Modelling Methane Adsorption Isotherms on Shale at Different Temperatures. Atmosphere 2022, 13, 865. https://doi.org/10.3390/atmos13060865
Zhang D. Modelling Methane Adsorption Isotherms on Shale at Different Temperatures. Atmosphere. 2022; 13(6):865. https://doi.org/10.3390/atmos13060865
Chicago/Turabian StyleZhang, Dongqing. 2022. "Modelling Methane Adsorption Isotherms on Shale at Different Temperatures" Atmosphere 13, no. 6: 865. https://doi.org/10.3390/atmos13060865
APA StyleZhang, D. (2022). Modelling Methane Adsorption Isotherms on Shale at Different Temperatures. Atmosphere, 13(6), 865. https://doi.org/10.3390/atmos13060865





