Evaluation of the Wind Environment around Multiple Urban Canyons Using Numerical Modeling
Abstract
:1. Introduction
2. Numerical Description
2.1. Computational Fluid Dynamics Model
2.2. Simulation Set-Up
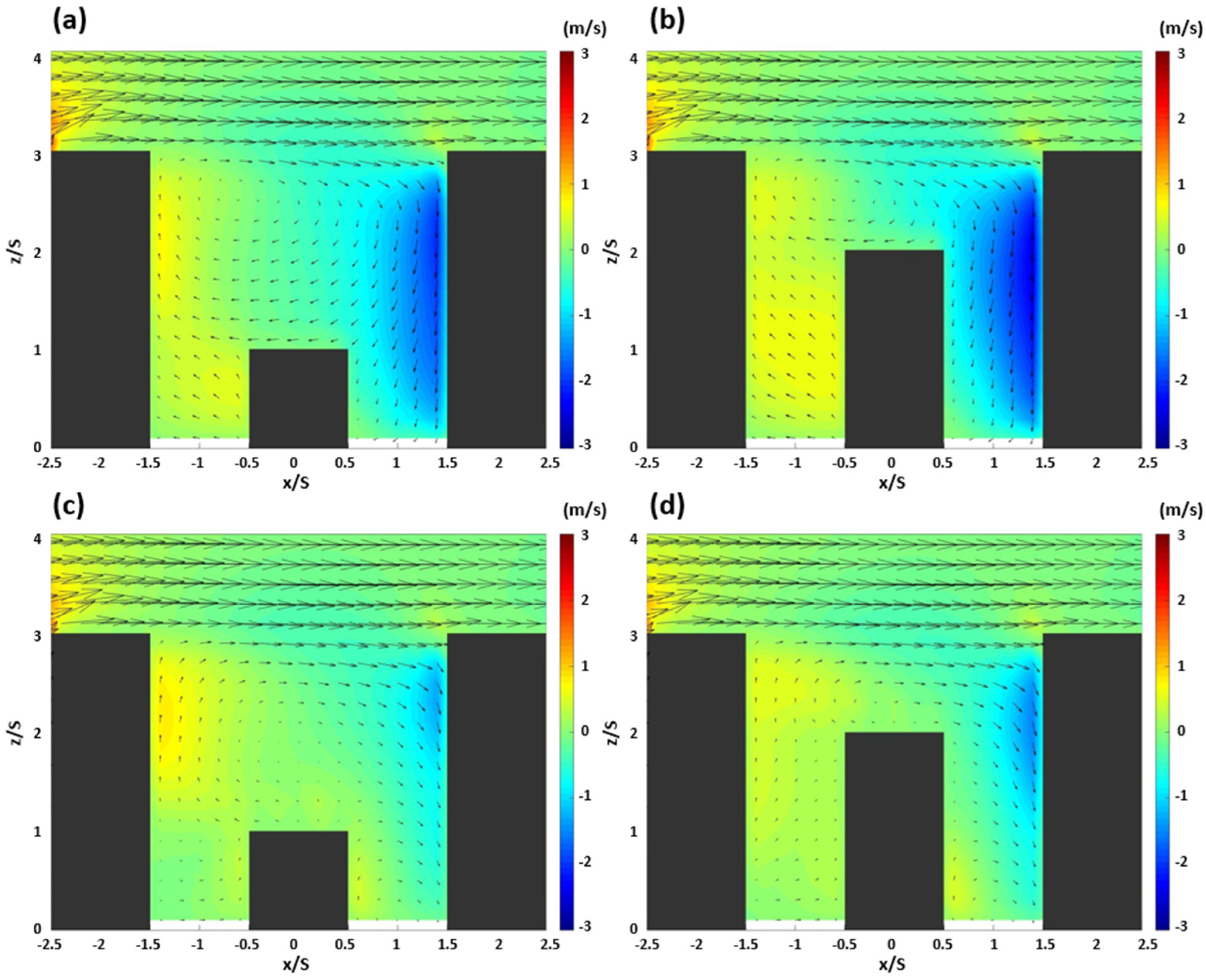
3. Step-Up to Step-Down Canyons
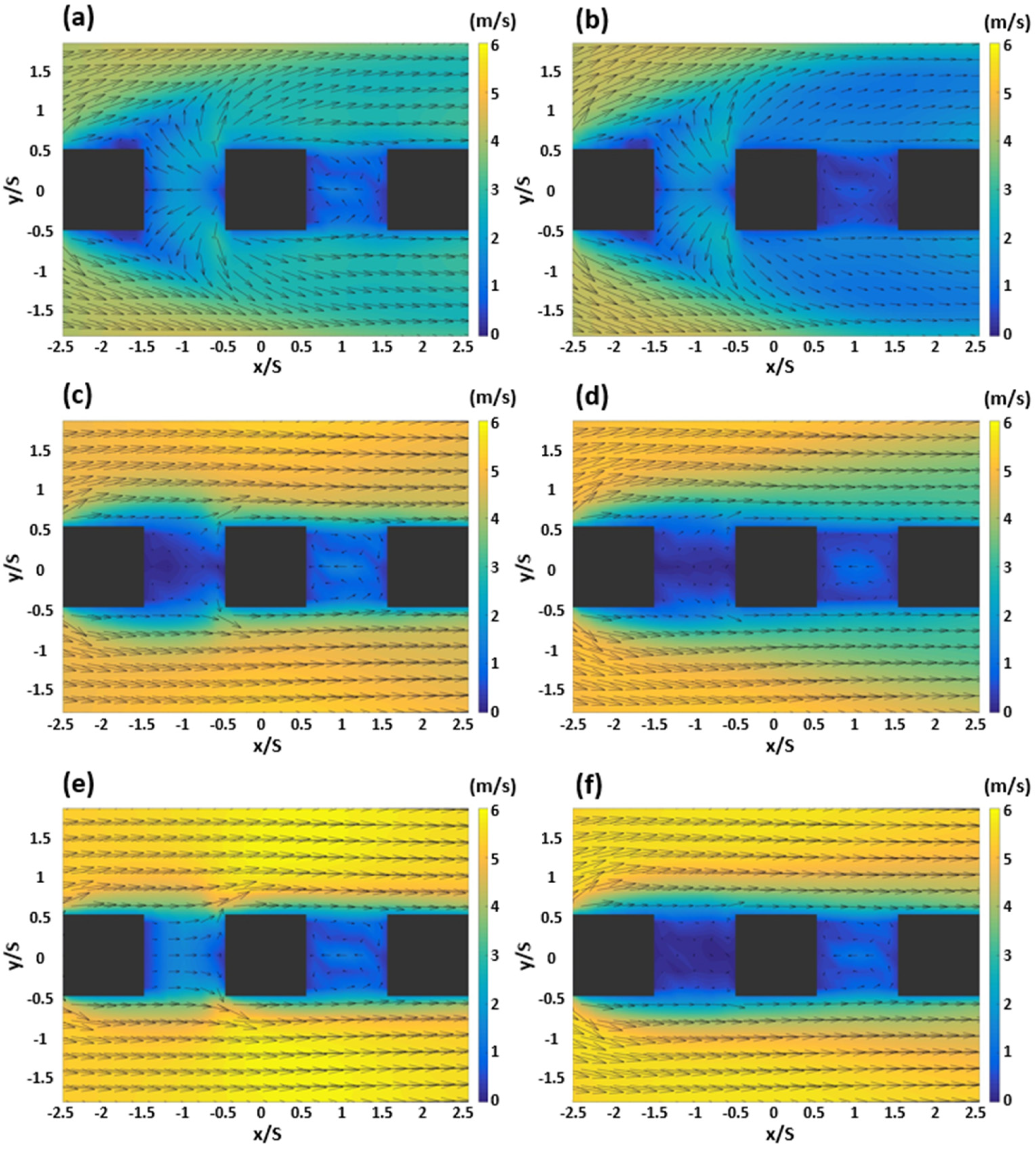
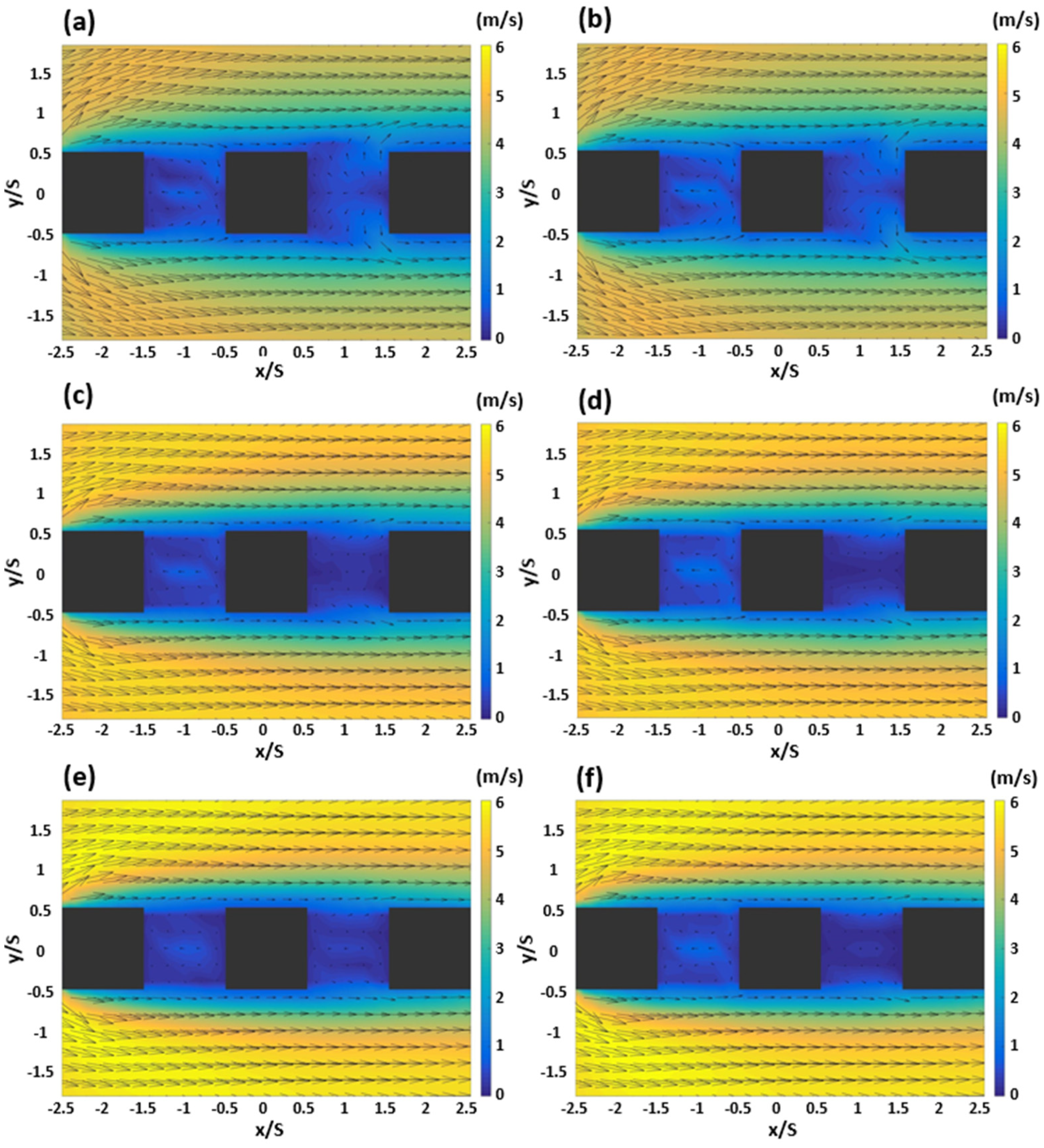
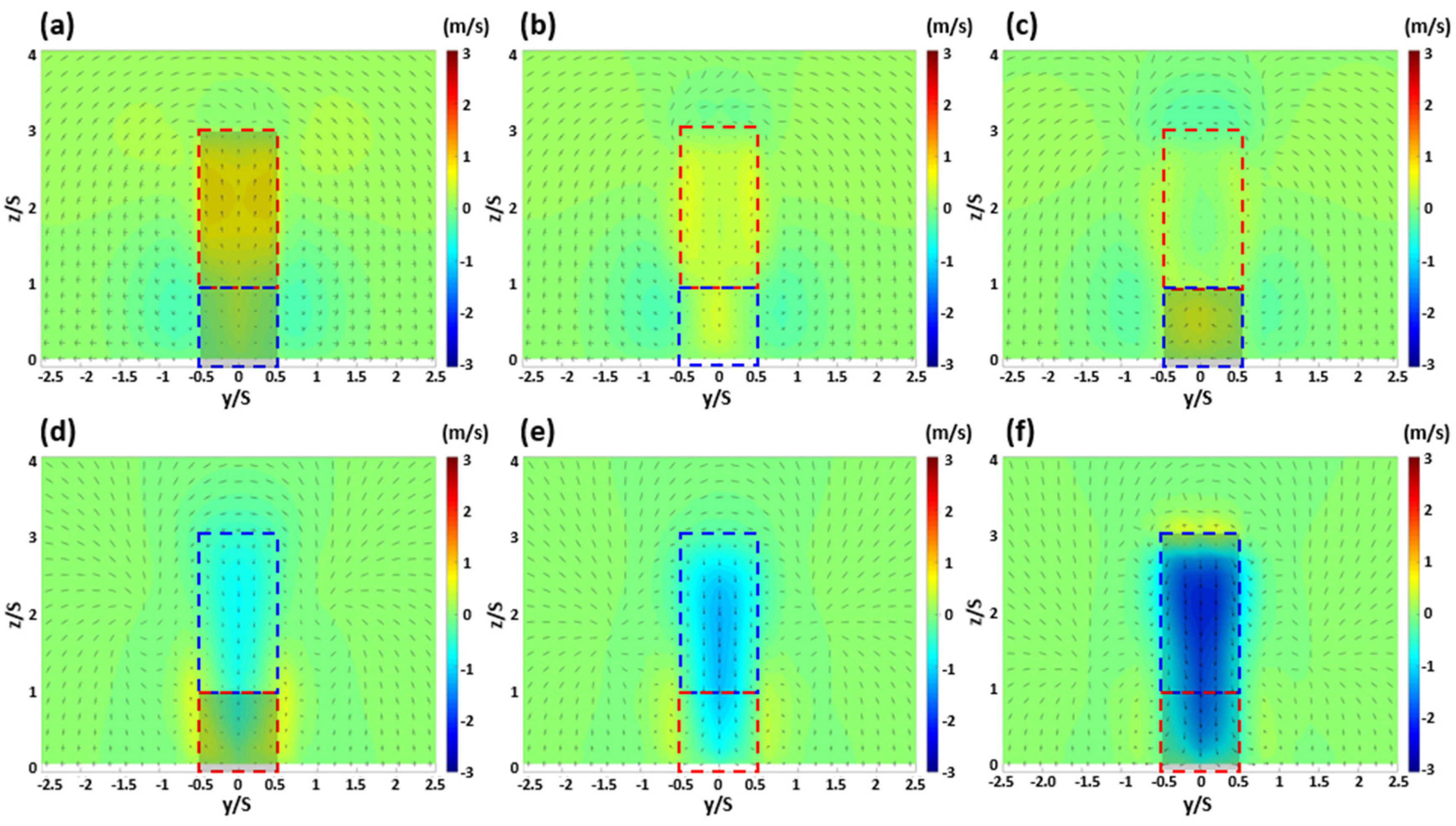
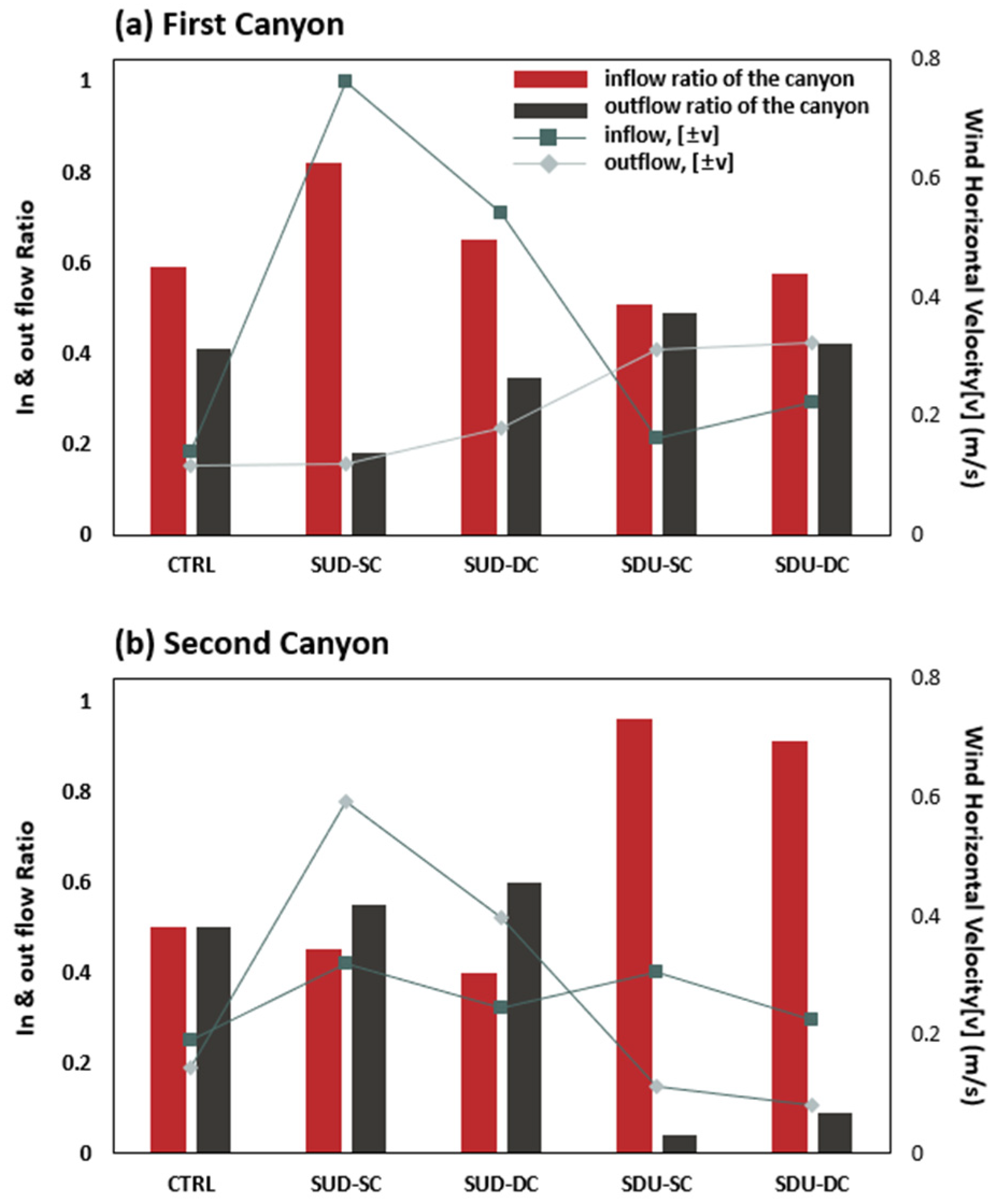
3.1. Horizontal Wind Field and Wind Speed
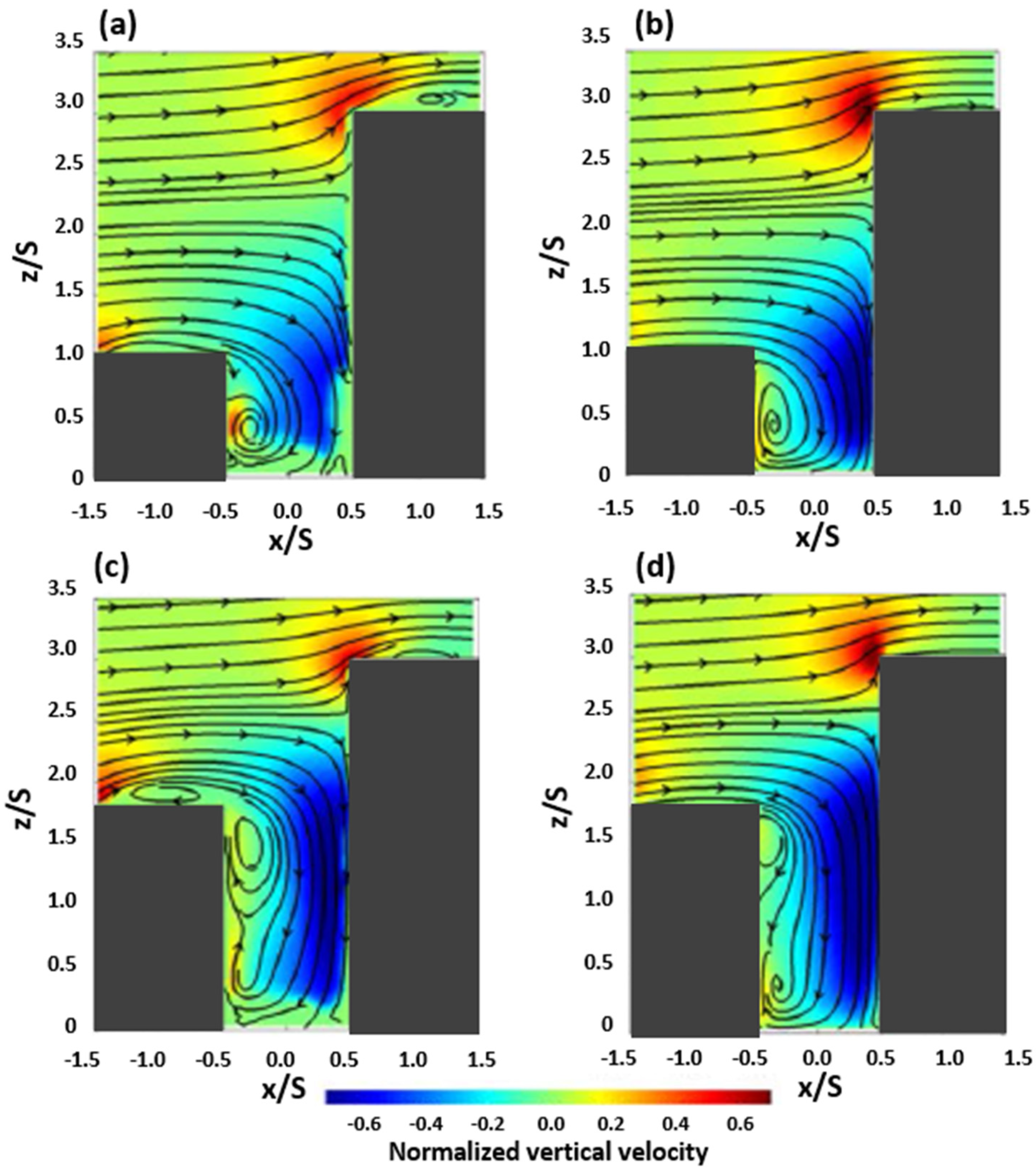
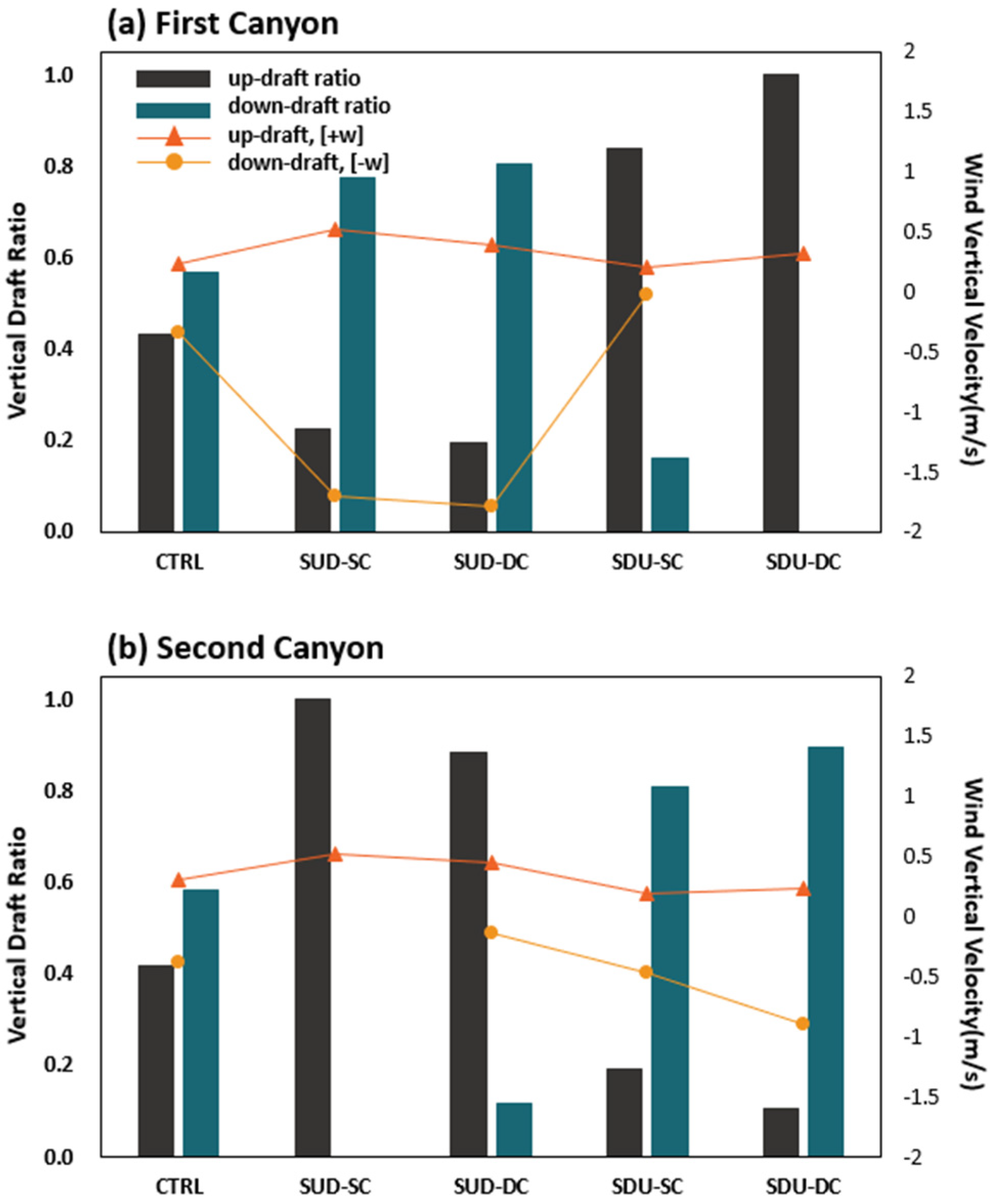
3.2. Vertical Wind Field and Velocity Component
4. Step-Down to Step-Up Canyons
4.1. Horizontal Wind Field and Wind Speed
4.2. Vertical Wind Field and Velocity Component
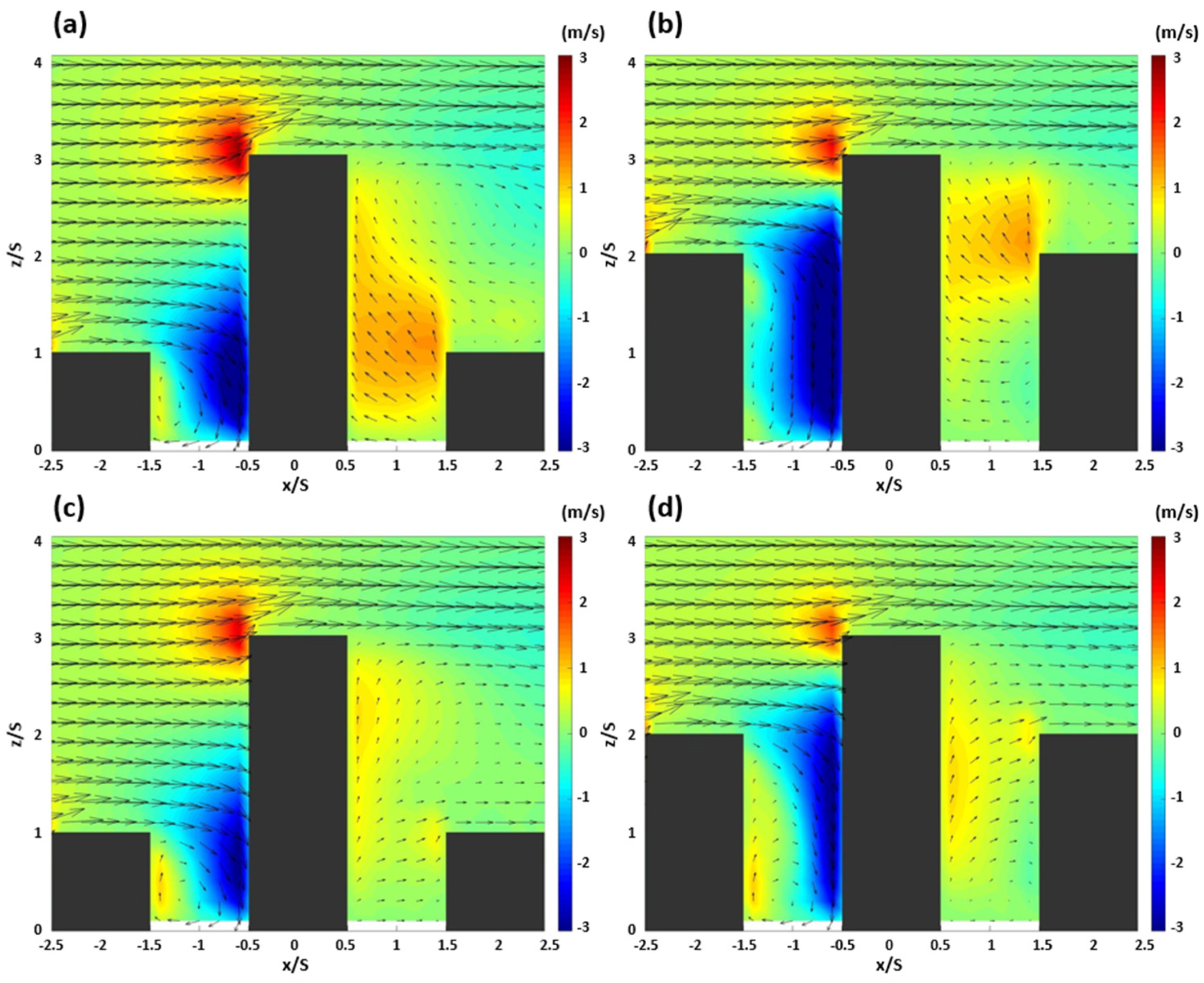
5. Wind Velocity Component in Multiple Canyons
6. Summary and Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Bibri, S.E.; Krogstie, J.; Kärrholm, M. Compact city planning and development: Emerging practices and strategies for achieving the goals of sustainability. Dev. Built Environ. 2020, 4, 100021. [Google Scholar] [CrossRef]
- Van Der Waals, J. The compact city and the environment: A review. J. Econmic Hum. Geogr. 2000, 91, 111–121. [Google Scholar] [CrossRef]
- Park, K.; Choi, M.J.; Cho, H.-S. The Effects of Urban Compactness on Temperature. J. Environ. Policy Adm. 2017, 25, 1–19. [Google Scholar] [CrossRef]
- Kang, J.E.; Yoon, D.; Bae, H.-J. Evaluating the effect of compact urban form on air quality in Korea. Environ. Plan. B Urban Anal. City Sci. 2017, 46, 179–200. [Google Scholar] [CrossRef]
- Kim, J.S.; Kang, J.E. Effects of Compact Spatial Characteristics on the Urban Thermal Environment. J. Urban Des. Inst. Korea Urban Des. 2018, 19, 21–36. [Google Scholar] [CrossRef]
- Kang, I.H. The strategy of compact city in Japan. Korean Policy Stud. Rev. 2018, 27, 221–244. [Google Scholar]
- Mochida, A.; Lun, I.Y. Prediction of wind environment and thermal comfort at pedestrian level in urban area. J. Wind Eng. Ind. Aerodyn. 2008, 96, 1498–1527. [Google Scholar] [CrossRef]
- Cheong, C.H.; Ryu, S.R. An analysis on the building wind variation in the residential community redestrian area by the planting method. J. Archit. Inst. Korea-Plan. Des. 2013, 29, 253–262. [Google Scholar] [CrossRef]
- Fan, Y.; Wang, Q.; Yin, S.; Li, Y. Effect of city shape on urban wind patterns and convective heat transfer in calm and stable background conditions. Build. Environ. 2019, 162, 106288. [Google Scholar] [CrossRef]
- Zhao, Y.; Li, H.; Kubilay, A.; Carmeliet, J. Buoyancy effects on the flows around flat and steep street canyons in simplified urban settings subject to a neutral approaching boundary layer: Wind tunnel PIV measurements. Sci. Total Environ. 2021, 797, 149067. [Google Scholar] [CrossRef]
- Pancholy, P.P.; Clemens, K.; Geoghegan, P.; Jermy, M.; Moyers-Gonzalez, M.; Wilson, P.L. Numerical study of flow structure and pedestrian-level wind comfort inside urban street canyons. J. R. Soc. N. Z. 2021, 51, 307–332. [Google Scholar] [CrossRef]
- Mittal, H.; Sharma, A.; Gairola, A. A review on the study of urban wind at the pedestrian level around buildings. J. Build. Eng. 2018, 18, 154–163. [Google Scholar] [CrossRef]
- Ku, C.-A.; Tsai, H.-K. Evaluating the Influence of Urban Morphology on Urban Wind Environment Based on Computational Fluid Dynamics Simulation. ISPRS Int. J. Geo-Inf. 2020, 9, 399. [Google Scholar] [CrossRef]
- Oke, T.R. Street design and urban canopy layer climate. Energy Build. 1988, 11, 103–113. [Google Scholar] [CrossRef]
- Son, M.; Kim, D.-Y. Flow characteristics in building canyon by surface flow regimes. J. Korean Soc. Urban Environ. 2020, 20, 9–16. [Google Scholar] [CrossRef]
- Kim, J.J. The effects of obstacle aspect ratio on surrounding flows. Atmos. Korean Meteorol. Soc. 2007, 17, 381–391. [Google Scholar]
- Kim, E.-R.; Park, R.J.; Lee, D.-G.; Kim, J.-J. A Study on the Characteristics of Flow and Reactive Pollutants’ Dispersion in Step-up Street Canyons Using a CFD Model. Atmosphere 2015, 25, 473–482. [Google Scholar] [CrossRef] [Green Version]
- Son, M.; Kim, D.-Y. Numerical analysis of wind velocity components in vertical development vortex. J. Korean Soc. Urban Environ. 2019, 19, 209–216. [Google Scholar] [CrossRef]
- Zheng, H.-Y.; Jin, W.-C.; Lee, S.-H.; Lee, K.-S. Wind Characteristics of Urban Street Canyon at High Rise Building Area. J. Korea Soc. Environ. Restor. Technol. 2012, 15, 9–18. [Google Scholar] [CrossRef]
- Li, Z.; Shi, T.; Wu, Y.; Zhang, H.; Juan, Y.-H.; Ming, T.; Zhou, N. Effect of traffic tidal flow on pollutant dispersion in various street canyons and corresponding mitigation strategies. Energy Built Environ. 2020, 1, 242–253. [Google Scholar] [CrossRef]
- Li, Z.; Zhang, H.; Wen, C.-Y.; Yang, A.-S.; Juan, Y.-H. Effects of height-asymmetric street canyon configurations on outdoor air temperature and air quality. Build. Environ. 2020, 183, 107195. [Google Scholar] [CrossRef]
- Baik, J.-J.; Park, R.-S.; Chun, H.-Y.; Kim, J.-J. A Laboratory Model of Urban Street-Canyon Flows. J. Appl. Meteorol. 2000, 39, 1592–1600. [Google Scholar] [CrossRef]
- Cui, D.; Li, X.; Liu, J.; Yuan, L.; Mak, C.M.; Fan, Y.; Kwok, K. Effects of building layouts and envelope features on wind flow and pollutant exposure in height-asymmetric street canyons. Build. Environ. 2021, 205, 108177. [Google Scholar] [CrossRef]
- Addepalli, B.; Pardyjak, E.R. Investigation of the Flow Structure in Step-Up Street Canyons—Mean Flow and Turbulence Statistics. Boundary-Layer Meteorol. 2013, 148, 133–155. [Google Scholar] [CrossRef]
- Park, S.-J.; Kim, J.-J.; Choi, W.; Kim, E.-R.; Song, C.-K.; Pardyjak, E.R. Flow Characteristics Around Step-Up Street Canyons with Various Building Aspect Ratios. Bound.-Layer Meteorol. 2019, 174, 411–431. [Google Scholar] [CrossRef] [Green Version]
- Addepalli, B.; Pardyjak, E.R. A study of flow felds in step-down street canyons. Envrion. Fluid. Mech. 2015, 15, 439–481. [Google Scholar] [CrossRef]
- Park, S.-J.; Choi, W.; Kim, J.-J.; Kim, M.J.; Park, R.J.; Han, K.-S.; Kang, G. Effects of building–roof cooling on the flow and dispersion of reactive pollutants in an idealized urban street canyon. Build. Environ. 2016, 109, 175–189. [Google Scholar] [CrossRef] [Green Version]
- Voordeckers, D.; Lauriks, T.; Denys, S.; Billen, P.; Tytgat, T.; Van Acker, M. Guidelines for passive control of traffic-related air pollution in street canyons: An overview for urban planning. Landsc. Urban Plan. 2020, 207, 103980. [Google Scholar] [CrossRef]
- Kim, J.-J. A numerical study of the effects of ambient wind direction on flow and dispersion in urban street canyons using the RNG k-ε turbulence model. Atmos. Environ. 2004, 38, 3039–3048. [Google Scholar] [CrossRef]
- Versteeg, H.K.; Malalasekera, W. An Introduction to Computational Fluid Dynamics: The Finite Volume Method, 2nd ed.; Prearson Education Limited: London, UK, 2007; pp. 66–97. [Google Scholar]
- Khawaja, H.; Moatamedi, M. Semi-Implicit Method for Pressure-Linked Equations (SIMPLE)—Solution in MATLAB®. Int. J. Multiphysics 2018, 12, 313–326. [Google Scholar] [CrossRef] [Green Version]
- Patankar, S.V. Numerical Heat Transfer and Fluid Flow, 1st ed.; McGraw-Hill: New York, NY, USA, 1980; pp. 126–131. [Google Scholar] [CrossRef]
- Yakhot, V.; Orszag, S.A.; Thangam, S.; Gatski, T.B.; Speziable, C.G. Development of turbulence models for shear flow by a double expansion technique. Phys. Fluids 1992, 4, 1510–1520. [Google Scholar] [CrossRef] [Green Version]
- Tutar, M.; Oguz, G. Large eddy simulation of wind flow around parallel buildings with varying configurations. Fluid Dyn. Res. 2002, 31, 289–315. [Google Scholar] [CrossRef]
- Hayati, A.N.; Stoll, R.; Pardyjak, E.R.; Harman, T.; Kim, J. Comparative metrics for computational approaches in non-uniform street-canyon flows. Build. Environ. 2019, 158, 16–27. [Google Scholar] [CrossRef]
- Brown, M.J.; Lawson, R.E., Jr.; DeCroix, D.S.; Lee, R.L. Mean flow and turbulence measurements around a 2-D array of buildings in a wind tunnel. In Proceedings of the 11th Joint Confernce on the Applications of Air Pollution Meteorology with the A&WMA, Long Beach, CA, USA, 9 January 2000; pp. 35–40. [Google Scholar]
- Castro, I.P.; Apsley, D.D. Flow and dispersion over topography: A comparison between numerical and laboratory data for two-dimensional flows. Atmos. Environ. 1997, 31, 839–850. [Google Scholar] [CrossRef]
- Razak, A.A.; Hagishima, A.; Ikegaya, N.; Tanimoto, J. Analysis of airflow over building arrays for assessment of urban wind environment. Build. Environ. 2013, 59, 56–65. [Google Scholar] [CrossRef]
- Tominaga, Y.; Shirzadi, M. Wind tunnel measurement of three-dimensional turbulent flow structures around a building group: Impact of high-rise buildings on pedestrian wind environment. Build. Environ. 2021, 206, 108389. [Google Scholar] [CrossRef]
- H’Ng, Y.M.; Ikegaya, N.; Zaki, S.A.; Hagishima, A.; Mohammad, A.F. Wind-tunnel estimation of mean and turbulent wind speeds within canopy layer for urban campus. Urban Clim. 2022, 41, 101064. [Google Scholar] [CrossRef]
- Furtak-Cole, E.; Ngan, K. Predicting mean velocity profiles inside urban canyons. J. Wind Eng. Ind. Aerodyn. 2020, 207, 104280. [Google Scholar] [CrossRef]
- Anup, K.C.; Whale, J.; Urmee, T. Urban wind conditions and small wind turbines in the built environment: A review. Renew. Energy 2019, 131, 268–283. [Google Scholar] [CrossRef]
- Fan, M.; Li, W.; Luo, X.; Shui, Q.; Jing, L.; Gu, Z.; Yu, C. Parameterised drag model for the underlying surface roughness of buildings in urban wind environment simulation. Build. Environ. 2021, 209, 108651. [Google Scholar] [CrossRef]





| Experiments | z/S | Appellation (Classification) | ||
|---|---|---|---|---|
| Bu | Bc | Bd | ||
| CTRL | 1.0 | 1.0 | 1.0 | Identical Urban Canyons |
| Step-up to step-down Canyons (Case 1) | 1.0 | 3.0 | 1.0 | Shallow Urban Canyons |
| 2.0 | 3.0 | 2.0 | Deep Urban Canyons | |
| Step-down to step-up Canyons (Case 2) | 3.0 | 1.0 | 3.0 | Shallow Urban Canyons |
| 3.0 | 2.0 | 3.0 | Deep Urban Canyons | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Son, M.; Lee, J.-I.; Kim, J.-J.; Park, S.-J.; Kim, D.; Kim, D.-Y. Evaluation of the Wind Environment around Multiple Urban Canyons Using Numerical Modeling. Atmosphere 2022, 13, 834. https://doi.org/10.3390/atmos13050834
Son M, Lee J-I, Kim J-J, Park S-J, Kim D, Kim D-Y. Evaluation of the Wind Environment around Multiple Urban Canyons Using Numerical Modeling. Atmosphere. 2022; 13(5):834. https://doi.org/10.3390/atmos13050834
Chicago/Turabian StyleSon, Minu, Jeong-In Lee, Jae-Jin Kim, Soo-Jin Park, Daegi Kim, and Do-Yong Kim. 2022. "Evaluation of the Wind Environment around Multiple Urban Canyons Using Numerical Modeling" Atmosphere 13, no. 5: 834. https://doi.org/10.3390/atmos13050834
APA StyleSon, M., Lee, J.-I., Kim, J.-J., Park, S.-J., Kim, D., & Kim, D.-Y. (2022). Evaluation of the Wind Environment around Multiple Urban Canyons Using Numerical Modeling. Atmosphere, 13(5), 834. https://doi.org/10.3390/atmos13050834








