The Influence of Pore Distribution of Coal Char in the Char Fragmentation and Included Minerals Partitioning: A Percolation Modeling
Abstract
:1. Introduction
2. Model Formulation
2.1. Model Hypothesis
- The char fragmentation is neglected, and the char swelling ratio is supposed to be finite during coal pyrolysis, therefore generating little cenospheric char. The largest initial porosity in the reaction is 0.5. Therefore, the char conversion is reviewed in the range of φ = 10–50%, which belongs to dense char and porous char.
- Only considering the influence of macropores (>0.05 µm) during char combustion in the external diffusion-controlled regime, and the char oxidation reaction could proceed inside and outside the particle simultaneously.
- It is assumed that all the minerals exist in the form of fine spherical particles, and their densities are constants, neglecting the influence of excluded minerals in the model because it can be removed through a physical method. Wang et al. [27] made a similar assumption in their model.
- During char combustion, minerals do not vaporize, crash and take place chemical reaction; two particles gather their volumes as they contact each other and find a new size supposing the new particle is still a sphere. Generally, the mass of inorganic mineral vaporization is less than 5% of total mineral mass [28], so it would not have a significant effect on the final size distribution of PM1–10.
- No hollow ash particles are formed. The aggregate volume of the two minerals is the sum of the previous two, and the mass of the new particulate is the sum of the previous two.
2.2. Initial Model
2.3. The Input of Initial Mineral Size
- Measuring more than 4000 particles by CCSEM, an N × N matrix is constituted by these data, then the order of all data will be changed through a program of “random” accompanied by Matlab (MATLAB 2017a, The MathWorks, Inc., Natick, MA, USA) software. Table 1 shows the number and detailed size distribution of included minerals measured by CCSEM.
- We should find all pore sites in the char matrix and let the data of these sites in the mineral matrix be ‘0’ to ensure that the porosities of these two matrices are identical.
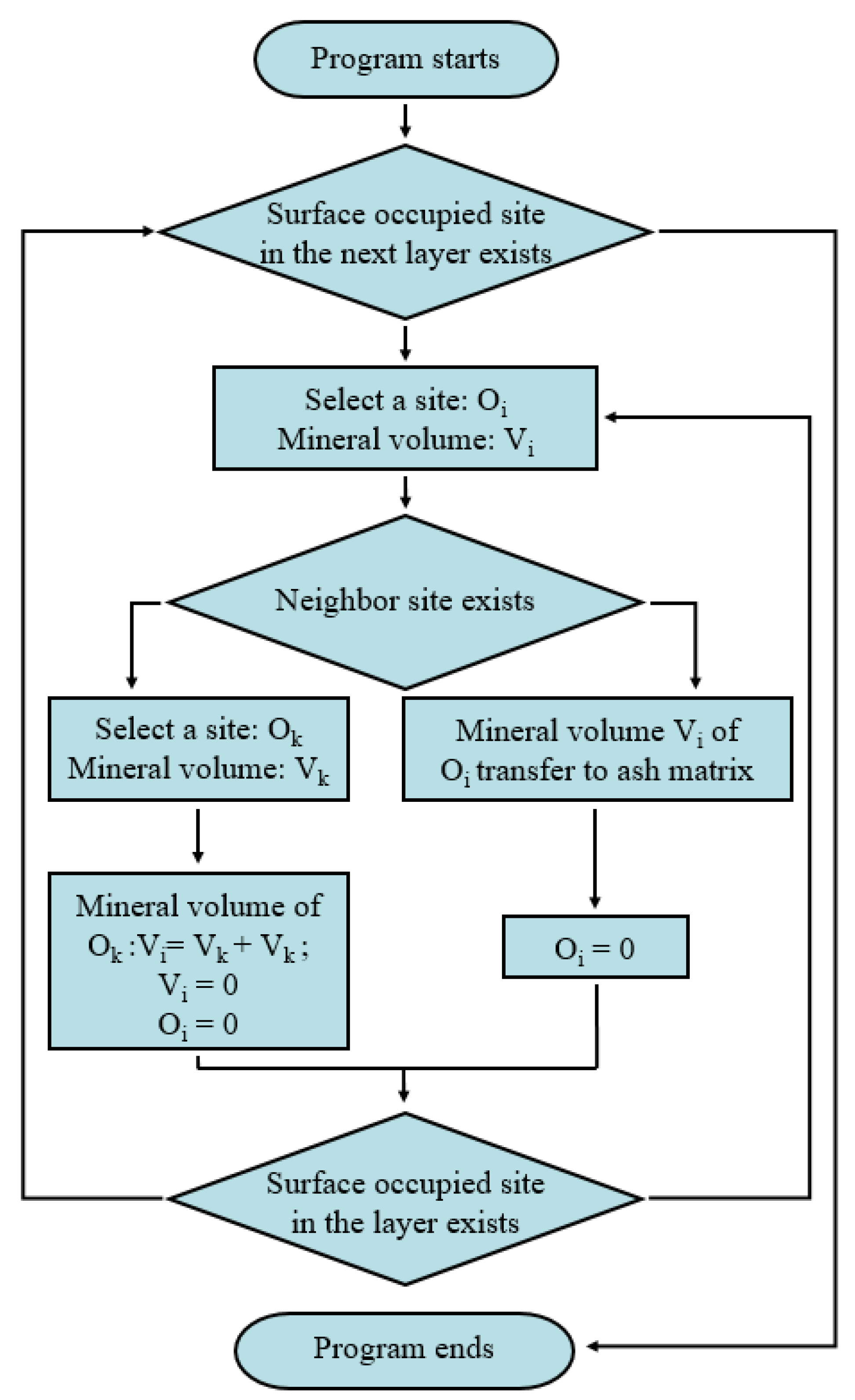
2.4. The Program of Char Conversion and Mineral Partitioning
- Firstly, we need to obtain surface occupied sites from the char matrix, then choose an occupied site Oi which has a mineral volume of Vi as the first reactive site.
- According to the 8-connection rule, if Oi has adjacent occupied sites, one of these, such as Ok with volume a of Vk, will be chosen to obtain the mineral from Oi, then mineral in Oi is 0, and the site Oi in char matrix is 0. The mineral volume of site Ok becomes Vi + Vk now.
- If Oi has no adjacent occupied sites, the mineral in site Oi will be regarded as ash which no longer connects with other minerals and is stored in the mineral matrix, then the mineral in Oi is 0, and the site Oi in the char matrix is 0, too.
- Repeat steps 1–3 until the reaction of the surface occupied sites of this layer is complete.
- Carrying out the reaction of the next layer through continuing steps 1–4, the program finishes as the reaction of all layers is complete. At last, it is all ‘0’ in the char matrix, the final mineral matrix is the ash matrix, and the data should be transferred from ash minerals volume to these sizes. The procedure is described in Figure 2.
3. Results and Discussion
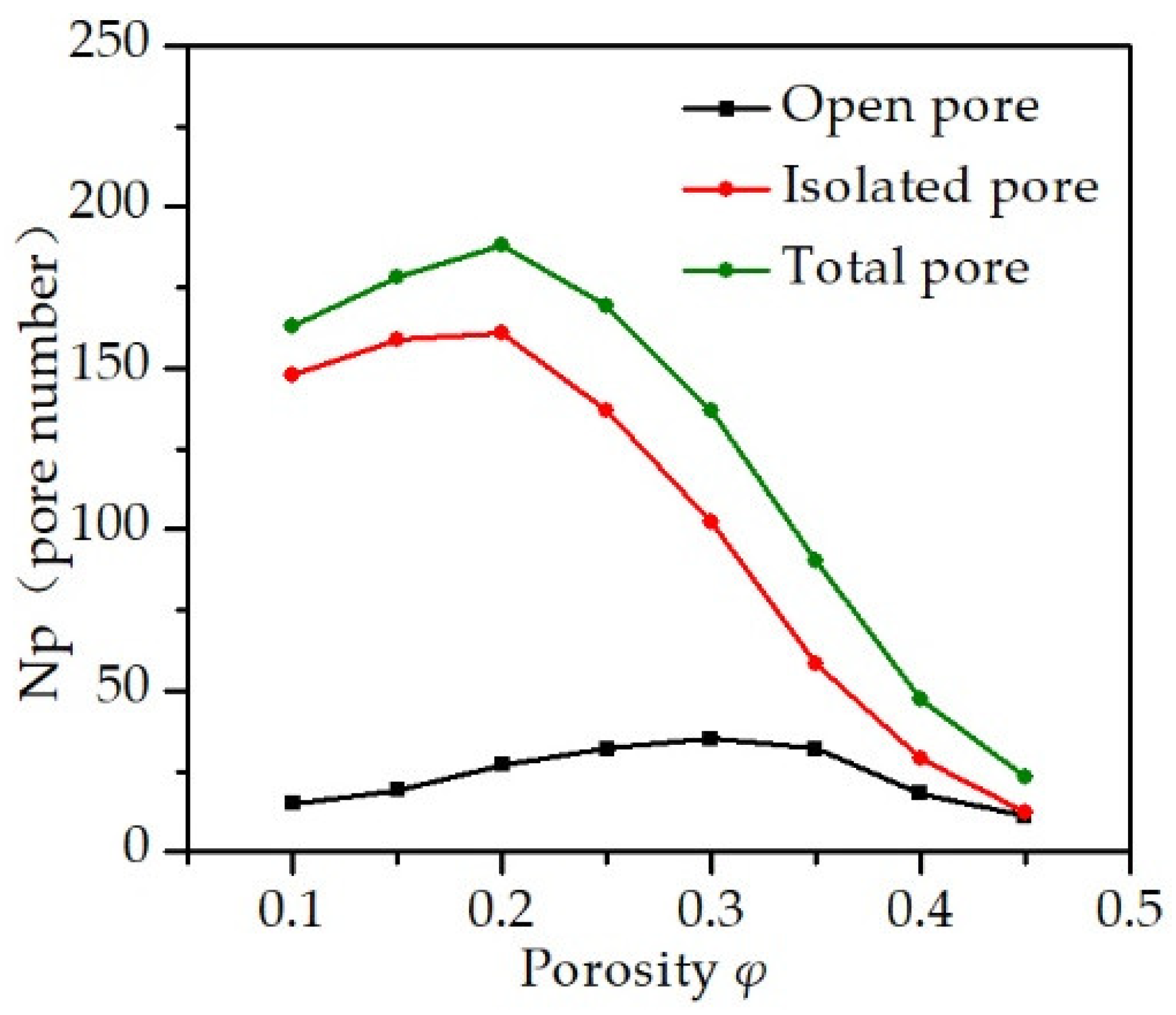
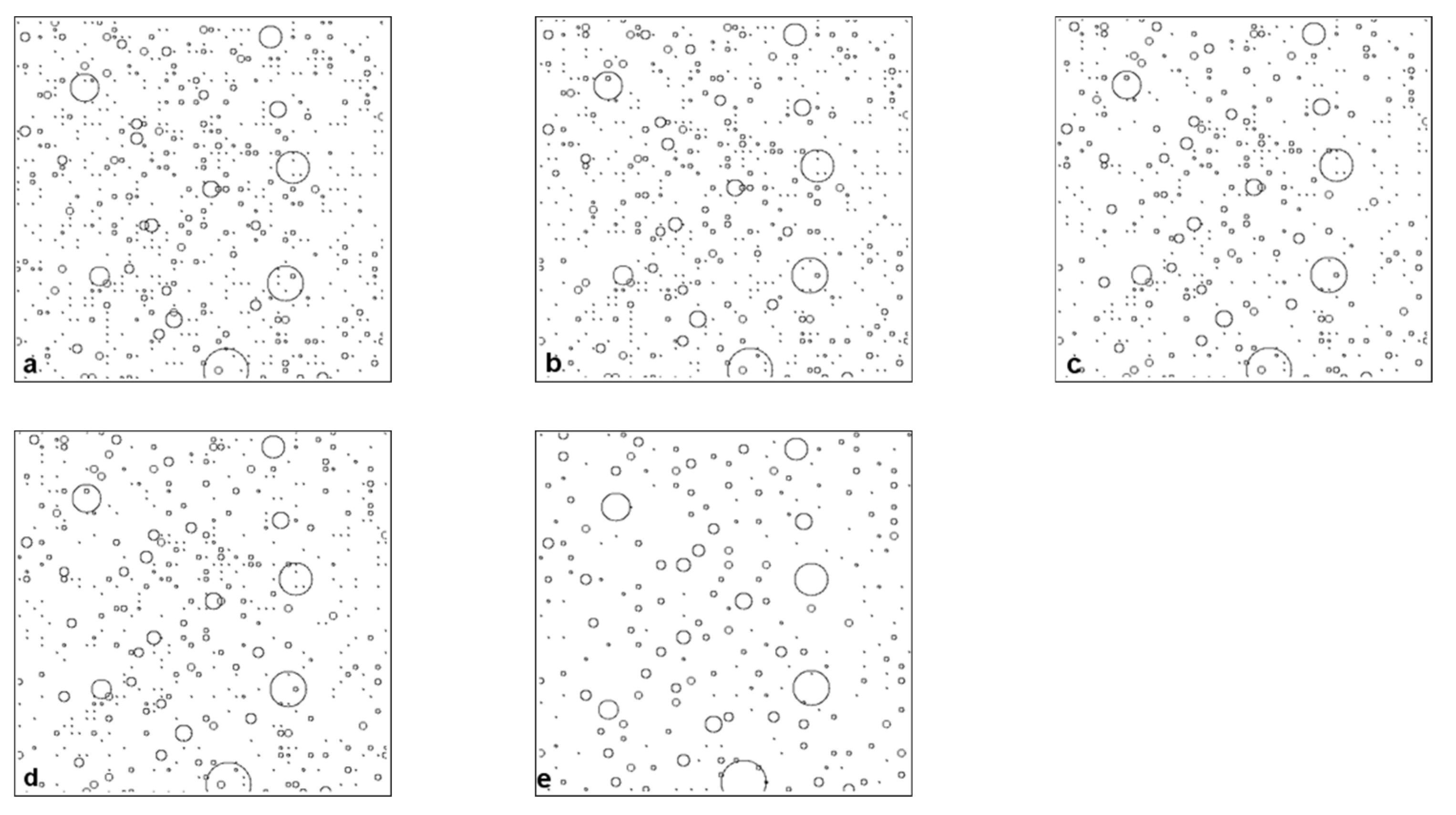
3.1. Initial Pore Distribution
3.2. Impact of Initial Porosity on Char Fragmentation
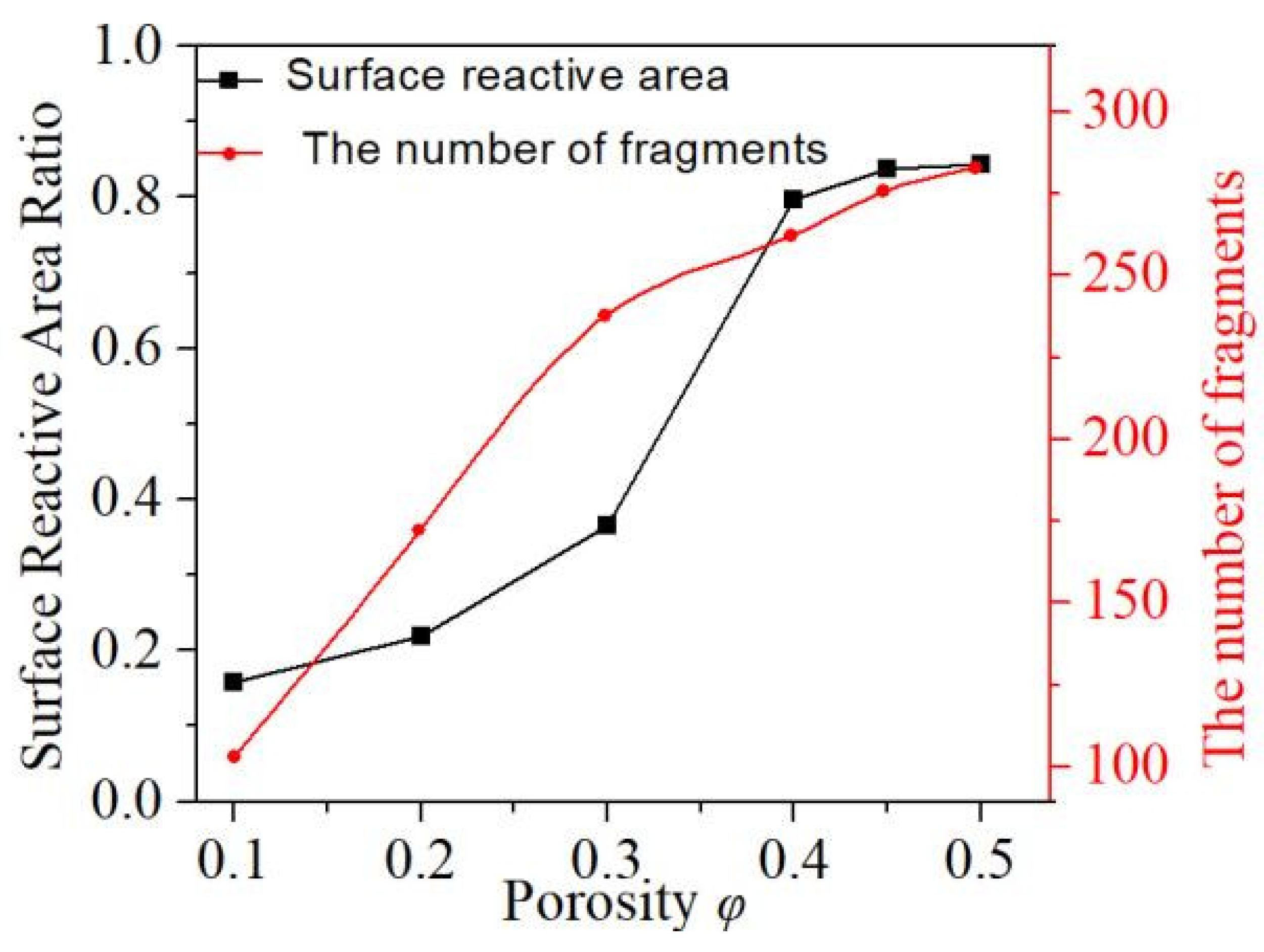
3.3. Impact of Char Conversion on Char Fragmentation
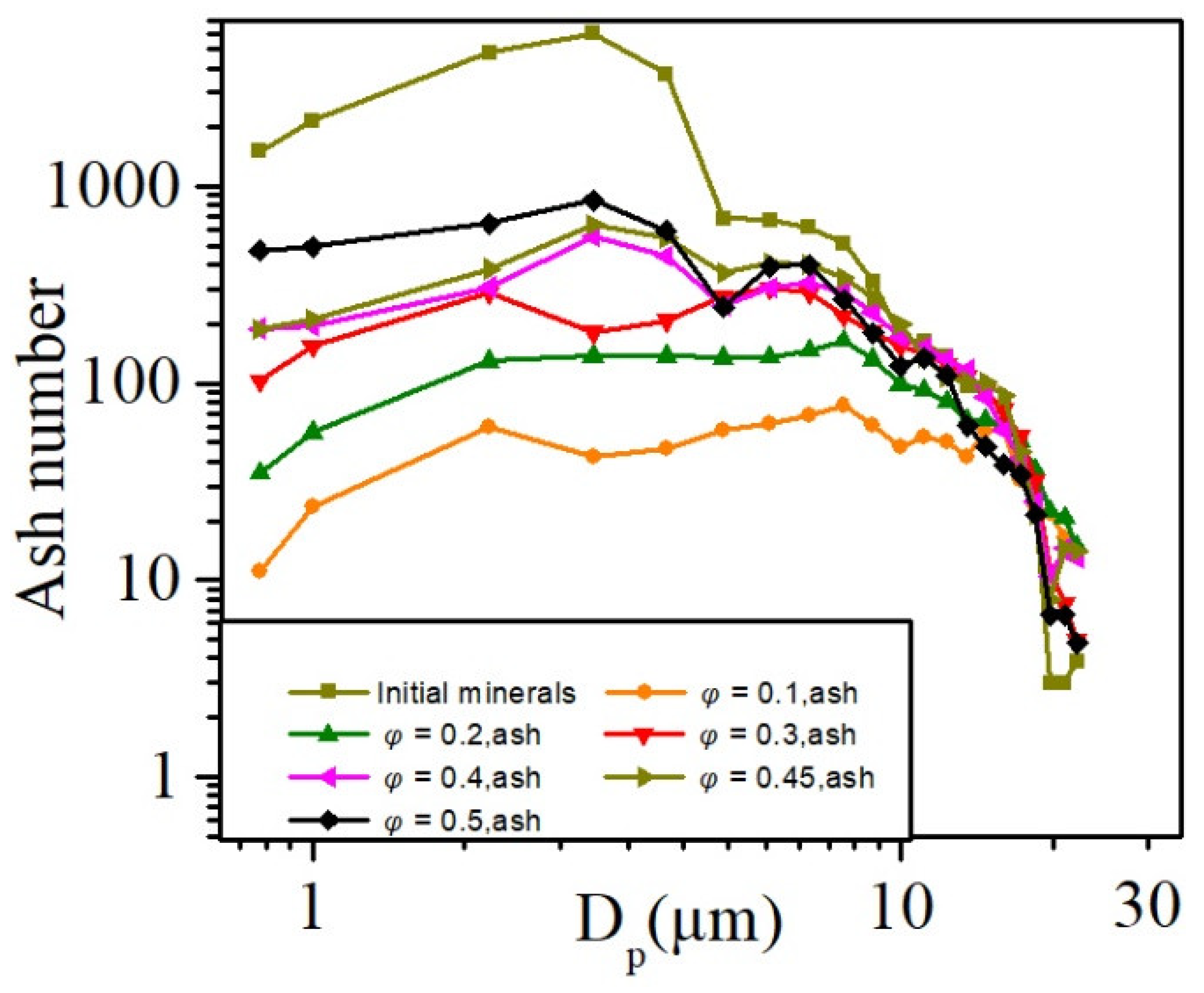
3.4. The Change of Ash Distributions with the Char Conversion
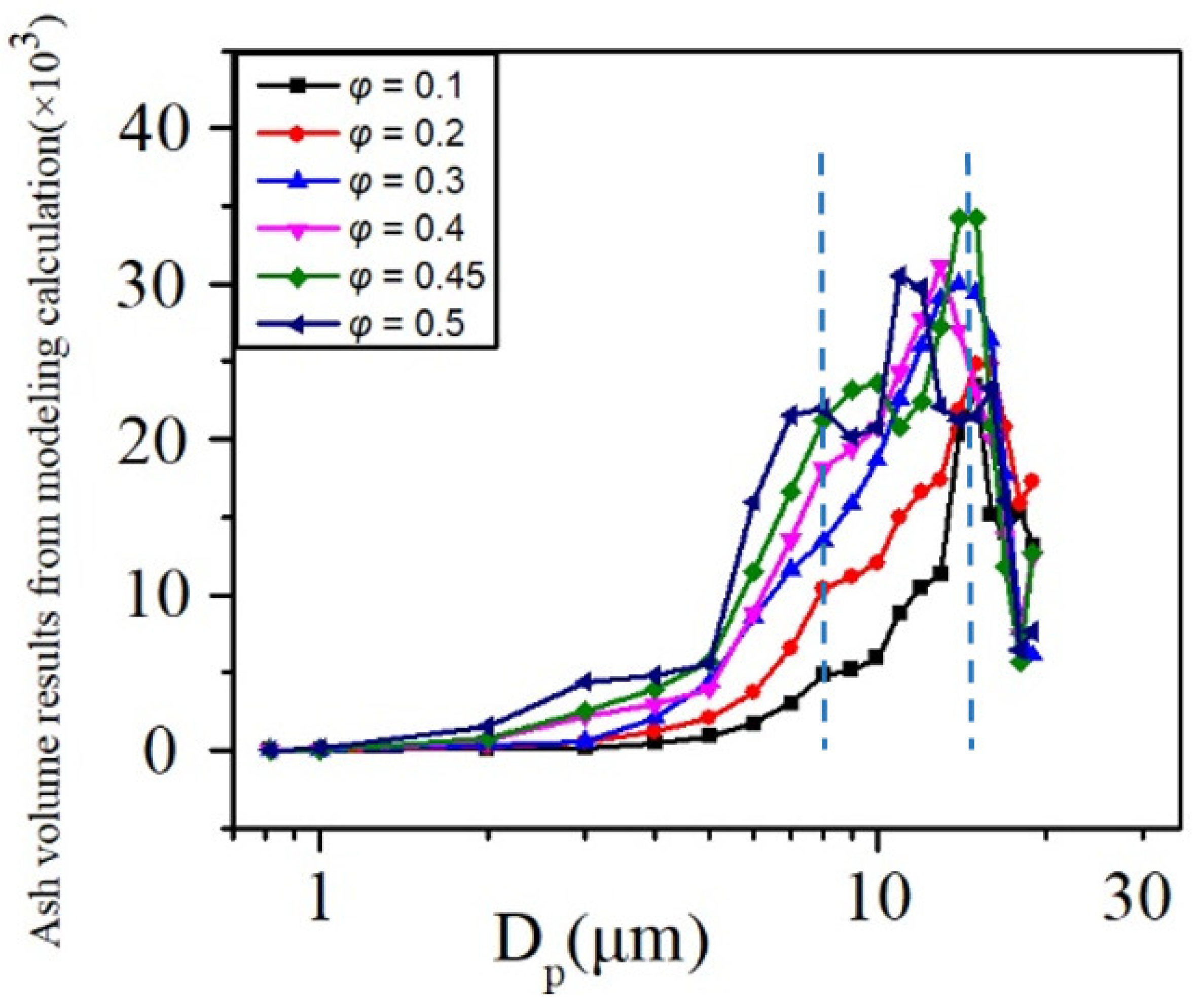
3.5. The Final Particle Size Distribution
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Xu, M.; Qiao, Y.; Zheng, C.; Li, L.; Liu, J. Modeling of homogeneous mercury speciation using detailed chemical kinetics. Combust. Flame 2003, 132, 208–218. [Google Scholar] [CrossRef]
- Sheng, C.; Li, Y.; Liu, X.; Yao, H.; Xu, M. Ash particle formation during O2/CO2 combustion of pulverized coals. Fuel Process. Technol. 2007, 88, 1021–1028. [Google Scholar] [CrossRef]
- Luo, G.; Yao, H.; Xu, M.; Gupta, R.; Xu, Z. Identifying modes of occurrence of mercury in coal by temperature programmed pyrolysis. Proc. Combust. Inst. 2011, 33, 2763–2769. [Google Scholar] [CrossRef]
- Zhu, Y.; Chen, Y.; Cheng, W.; Zhang, W.; Hu, J.; Zeng, K.; Yang, H.; Shao, J.; Chen, H. Reduction of fine particulate matter emissions from cornstalk combustion by calcium phosphates additives. Fuel 2021, 283, 119303. [Google Scholar] [CrossRef]
- Sarofim, A.F.; Howard, J.B.; Padia, A.S. The physical transformation of the mineral matter in pulverized coal under simulated combustion conditions. Combust. Sci. Technol. 1977, 16, 187–204. [Google Scholar] [CrossRef]
- Flagan, R.C.; Friedlander, S.K. Recent Developments in Aerosol Science; Shaw, D.T., Ed.; John Wiley & Sons: Hoboken, NJ, USA, 1978; pp. 25–59. [Google Scholar]
- Linak, W.P.; Miller, C.A.; Seames, W.S.; Wendt, J.O.; Ishinomori, T.; Endo, Y.; Miyamae, S. On trimodal particle size distributions in fly ash from pulverized-coal combustion. Proc. Combust. Inst. 2002, 29, 441–447. [Google Scholar] [CrossRef] [Green Version]
- Seames, W.S. An initial study of the fine fragmentation fly ash particle mode generated during pulverized coal combustion. Fuel Process. Technol. 2003, 81, 109–125. [Google Scholar] [CrossRef]
- Yu, D.; Xu, M.; Yao, H.; Sui, J.; Liu, X.; Yu, Y.; Cao, Q. Use of elemental size distributions in identifying particle formation modes. Proc. Combust. Inst. 2007, 31, 1921–1928. [Google Scholar] [CrossRef]
- Yu, D.; Xu, M.; Yao, H.; Liu, X.; Zhou, K. A new method for identifying the modes of particulate matter from pulverized coal combustion. Powder Technol. 2008, 183, 105–114. [Google Scholar] [CrossRef]
- Liu, S.; Zhu, G.; Niu, Y.; Wen, L.; Lei, Y.; Wang, D. Characteristics of particulate emissions from coal char combustion: Char fragmentation and ash coalescence behaviors. Fuel 2022, 310, 122283. [Google Scholar] [CrossRef]
- Gao, Q.; Li, S.; Zhao, Y.; Yao, Q. Mechanism on the contribution of coal/char fragmentation to fly ash formation during pulverized coal combustion. Proc. Combust. Inst. 2019, 37, 2831–2839. [Google Scholar] [CrossRef]
- Mitchell, R.E.; Akanetuk, A.J. The impact of fragmentation on char conversion during pulverized coal combustion. Symp. Combust. 1996, 26, 3137–3144. [Google Scholar] [CrossRef]
- Feng, B.; Bhatia, S.K. Percolative fragmentation of char particles during gasification. Energy Fuels 2000, 14, 297–307. [Google Scholar] [CrossRef]
- Miccio, F. Modeling percolative fragmentation during conversion of entrained char particles. Korean J. Chem. Eng. 2004, 21, 404–411. [Google Scholar] [CrossRef]
- Helble, J.J.; Sarofim, A.F. Influence of char fragmentation on ash particle size distributions. Combust. Flame 1989, 76, 183–196. [Google Scholar] [CrossRef]
- Baxter, L.L. Char fragmentation and fly ash formation during pulverized-coal combustion. Combust. Flame 1992, 90, 174–184. [Google Scholar] [CrossRef]
- Liu, G.; Wu, H.; Gupta, R.P.; Lucas, J.A.; Tate, A.G.; Wall, T.F. Modeling the fragmentation of non-uniform porous char particles during pulverized coal combustion. Fuel 2000, 79, 627–633. [Google Scholar] [CrossRef]
- Kang, S.G.; Sarofim, A.F.; Beér, J.M. Effect of char structure on residual ash formation during pulverized coal combustion. Symp. (Int.) Combust. 1992, 24, 1153–1159. [Google Scholar] [CrossRef]
- Salatino, P.; Miccio, F.; Massimilla, L. Combustion and percolative fragmentation of carbons. Combust. Flame 1993, 95, 342–350. [Google Scholar] [CrossRef]
- Liu, X.; Xu, M.; Yao, H.; Yu, D.; Gao, X.; Cao, Q.; Cai, Y. Effect of combustion parameters on the emission and chemical composition of particulate matter during coal combustion. Energy Fuels 2007, 21, 157–162. [Google Scholar] [CrossRef]
- Kang, S.G.; Kerstein, A.R.; Helble, J.J.; Sarofim, A.F. Simulation of residual ash formation during pulverized coal combustion: Bimodal ash particle size distribution. Aerosol Sci. Technol. 1990, 13, 401–412. [Google Scholar] [CrossRef]
- Yu, D.; Xu, M. A Computer simulation study of char fragmentation. Proc. CSEE 2005, 25, 90–93. (In Chinese) [Google Scholar]
- Yu, D.; Xu, M.; Huang, J.; Li, G.; Liu, X.; Yun, Y. A model study on char fragmentation and ash formation. J. Eng. Thermophys. 2005, 26, 1041–1044. (In Chinese) [Google Scholar]
- Yu, D.; Xu, M.; Zhang, L.; Yao, H.; Wang, Q.; Ninomiya, Y. Computer-controlled scanning electron microscopy (CCSEM) investigation on the heterogeneous nature of mineral matter in six typical Chinese coals. Energy Fuels 2007, 21, 468–476. [Google Scholar] [CrossRef]
- Tang, L.G.; Gupta, R.P.; Sheng, C.D.; Wall, T.F. A mechanistic approach to characterize coal heterogeneity in predicting high-temperature volatile matter yields. Energy Fuels 2004, 18, 1716–1722. [Google Scholar] [CrossRef]
- Wang, H.; Li, H.; Chen, Q.; Gao, J.; Wang, C. Modified three-dimensional percolation simulation of char fragmentation and particulate formation. AIP Adv. 2017, 7, 025016. [Google Scholar] [CrossRef] [Green Version]
- Quann, R.J. Ash Vaporization under Simulated Pulverized Coal Combustion Conditions. Ph.D. Thesis, Massachusetts Institute of Technology, Cambridge, MA, USA, 1982. [Google Scholar]
- Gupta, R.P.; Wall, T.F.; Kajigaya, I.; Miyamae, S.; Tsumita, Y. Computer-controlled scanning electron microscopy of minerals in coal—implications for ash deposition. Prog. Energy Combust. Sci. 1998, 24, 523–543. [Google Scholar] [CrossRef]
- Wen, C.; Gao, X.; Xu, M. A CCSEM study on the transformation of included and excluded minerals during coal devolatilization and char combustion. Fuel 2016, 172, 96–104. [Google Scholar] [CrossRef]
- Wen, C.; Xu, M.; Zhou, K.; Yu, D.; Zhan, Z.; Mo, X. The melting potential of various ash components generated from coal combustion: Indicated by the circularity of individual particles using CCSEM technology. Fuel Process. Technol. 2015, 133, 128–136. [Google Scholar] [CrossRef]
- Benfell, K.E.; Liu, G.S.; Roberts, D.G.; Harris, D.J.; Lucas, J.A.; Bailey, J.G.; Wall, T.F. Modeling char combustion: The influence of parent coal petrography and pyrolysis pressure on the structure and intrinsic reactivity of its char. Proc. Combust. Inst. 2000, 28, 2233–2241. [Google Scholar] [CrossRef]
- Edwards, D.A.; Hanes, J.; Caponetti, G.; Hrkach, J.; Ben-Jebria, A.; Eskew, M.L.; Mintzes, J.; Deaver, D.; Lotan, N.; Langer, R. Large porous particles for pulmonary drug delivery. Science 1997, 276, 1868–1872. [Google Scholar] [CrossRef] [Green Version]
- Wen, C.; Gao, X.; Yu, Y.; Wu, J.; Xu, M.; Wu, H. Emission of inorganic PM10 from included mineral matter during the combustion of pulverized coals of various ranks. Fuel 2015, 140, 526–530. [Google Scholar] [CrossRef]





| Geometrical Diameter D (µm) | 0.5–1 | 1–2 | 2–3 | 3–4 | 4–5 |
|---|---|---|---|---|---|
| Number | 504 | 1464 | 2096 | 230 | 223 |
| Geometrical Diameter D (µm) | 5–6 | 6–7 | 7–8 | 8–9 | 9–10 |
| Number | 167 | 58 | 53 | 32 | 28 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, R.; Jing, Z.; Ma, J.; Qin, L.; Yan, K.; Wen, C. The Influence of Pore Distribution of Coal Char in the Char Fragmentation and Included Minerals Partitioning: A Percolation Modeling. Atmosphere 2022, 13, 628. https://doi.org/10.3390/atmos13040628
Li R, Jing Z, Ma J, Qin L, Yan K, Wen C. The Influence of Pore Distribution of Coal Char in the Char Fragmentation and Included Minerals Partitioning: A Percolation Modeling. Atmosphere. 2022; 13(4):628. https://doi.org/10.3390/atmos13040628
Chicago/Turabian StyleLi, Rui, Zhenqi Jing, Jingjing Ma, Long Qin, Kai Yan, and Chang Wen. 2022. "The Influence of Pore Distribution of Coal Char in the Char Fragmentation and Included Minerals Partitioning: A Percolation Modeling" Atmosphere 13, no. 4: 628. https://doi.org/10.3390/atmos13040628
APA StyleLi, R., Jing, Z., Ma, J., Qin, L., Yan, K., & Wen, C. (2022). The Influence of Pore Distribution of Coal Char in the Char Fragmentation and Included Minerals Partitioning: A Percolation Modeling. Atmosphere, 13(4), 628. https://doi.org/10.3390/atmos13040628





