An Empirical Equation for Wet-Bulb Temperature Using Air Temperature and Relative Humidity
Abstract
1. Introduction
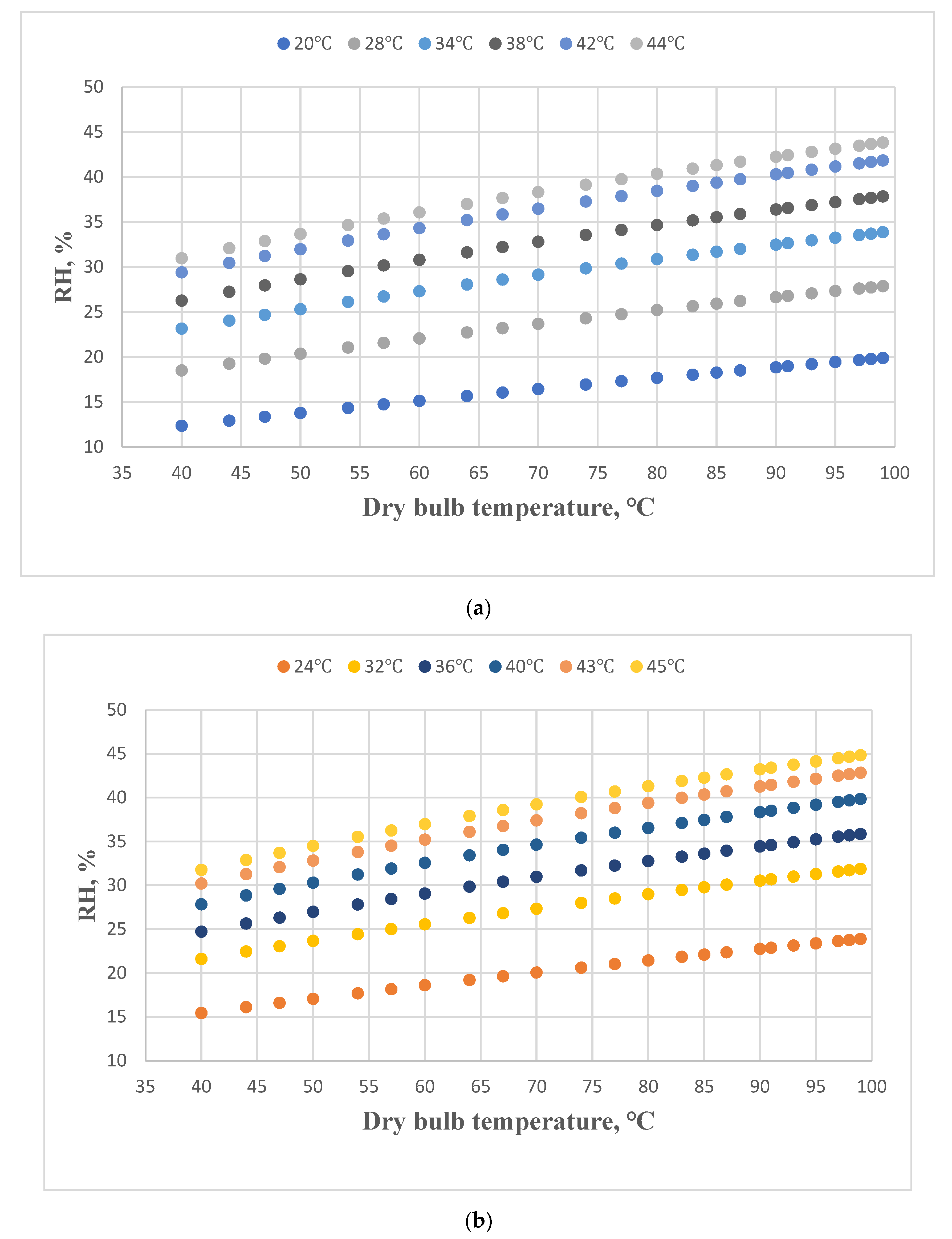
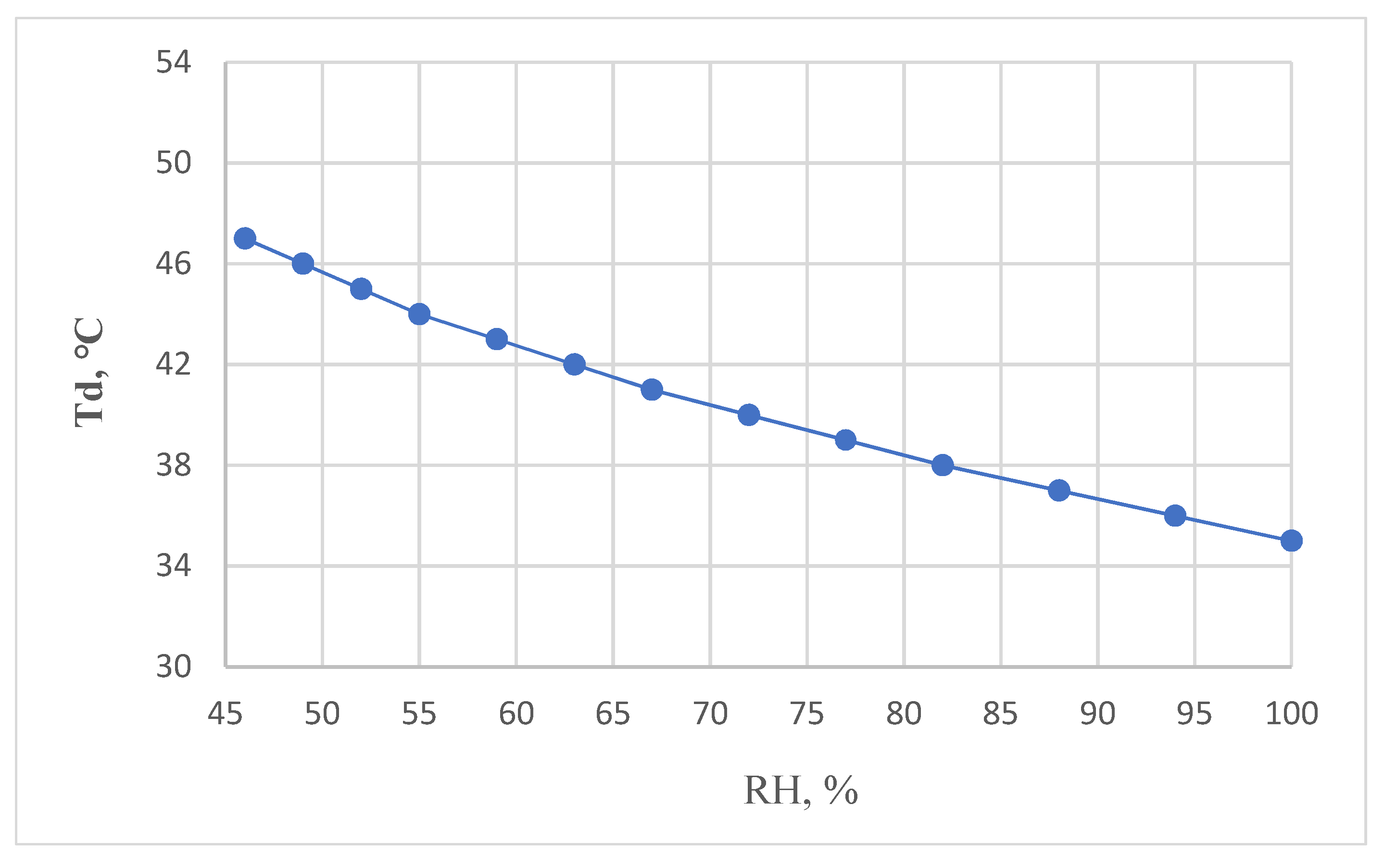
2. Methodology
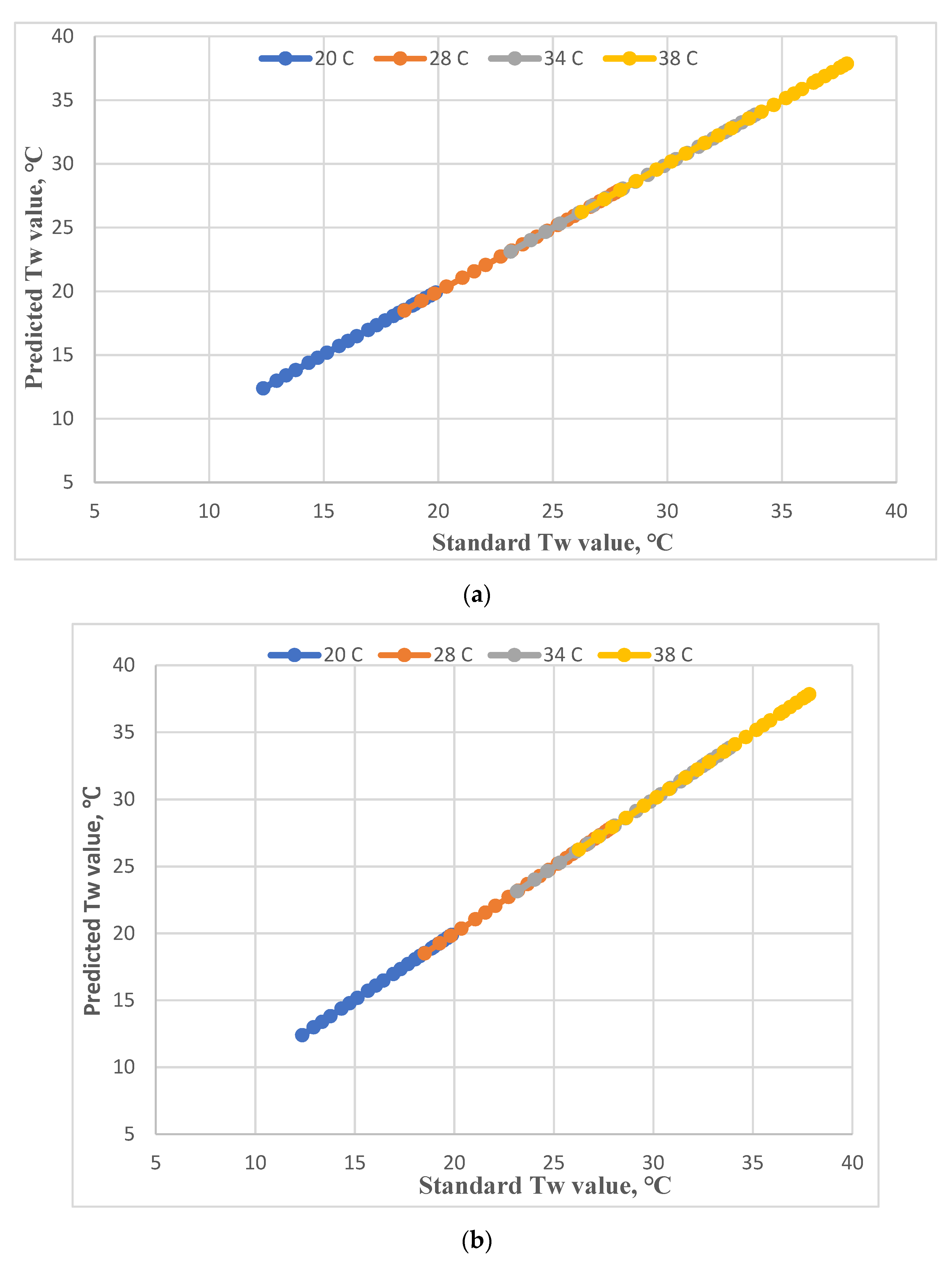
3. Development of the Tw Empirical Equation
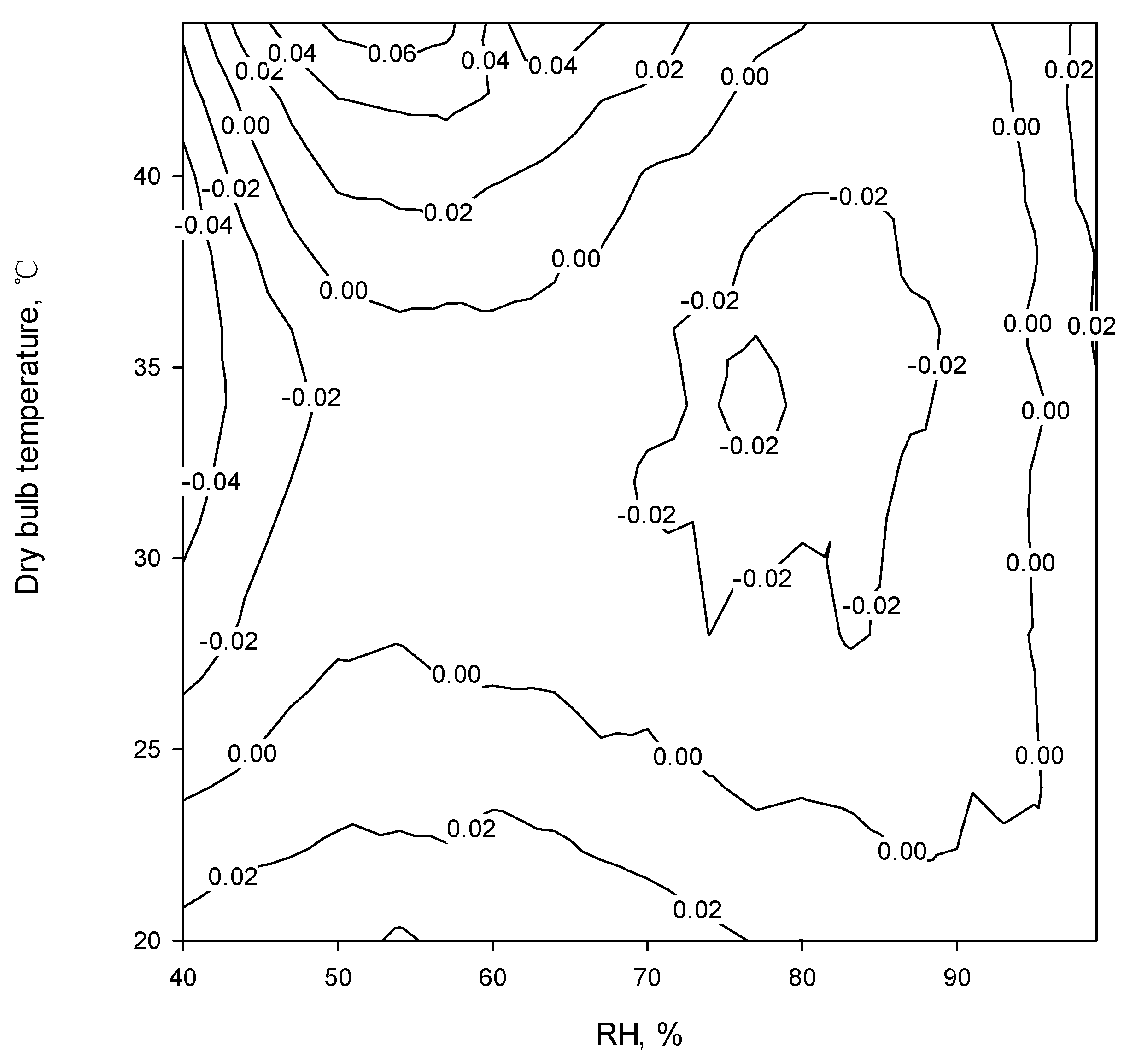
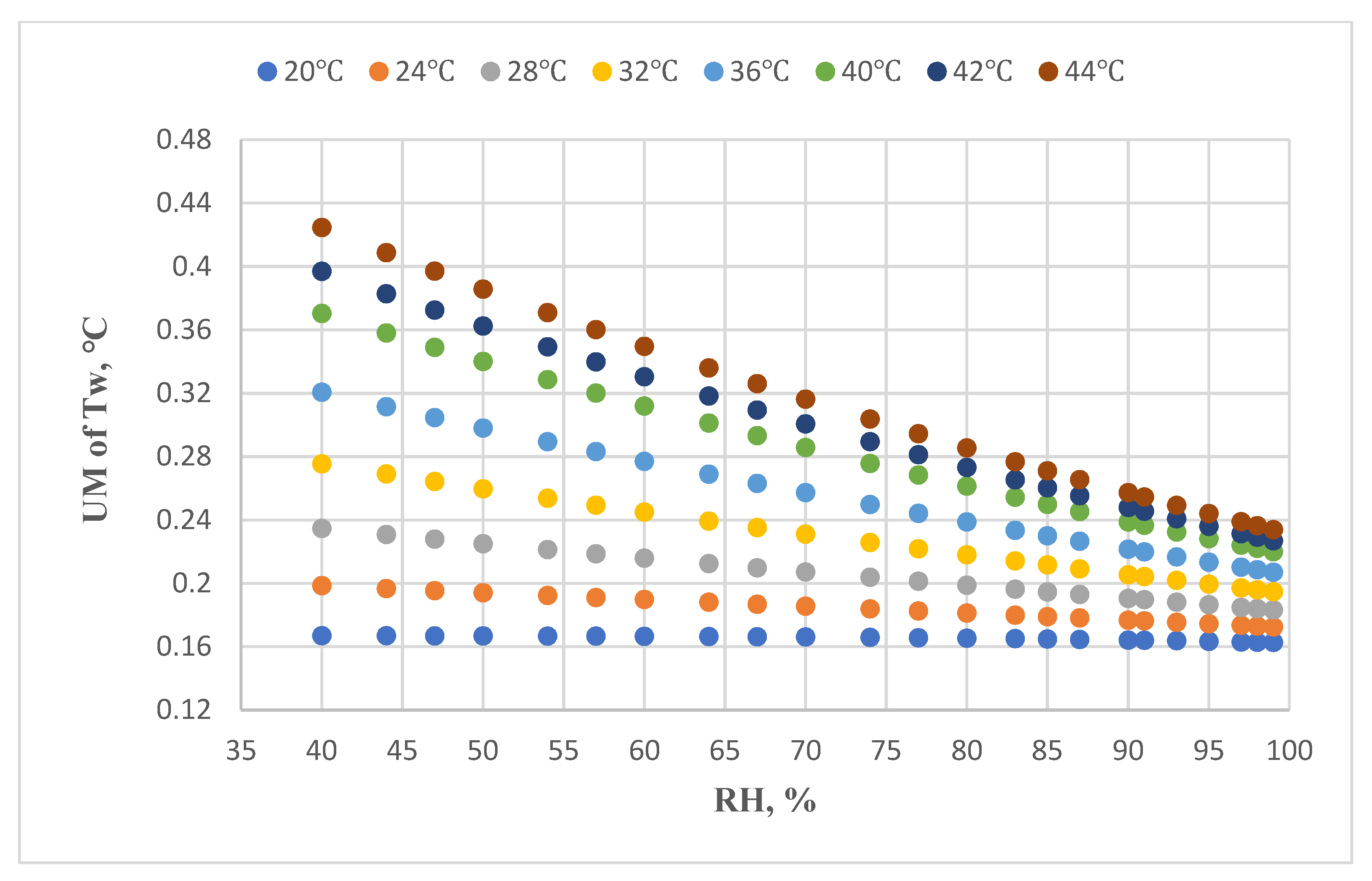
4. The Measurement Uncertainty for the Tw Equation
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Dunne, J.P.; Stouffer, R.J.; John, J.G. Reductions in labour capacity from heat stress under climate warming. Nat. Clim. Chang. 2018, 3, 563–566. [Google Scholar] [CrossRef]
- Ghani, S.; Bialy, E.M.; Bakochristou, F.; Gamaledin, S.M.A.; Rashwan, M.M.; Hughes, B. Thermal comfort investigation of an outdoor air-conditioned area in a hot and arid environment. Sci. Technol. Built Environ. 2017, 23, 1113–1131. [Google Scholar] [CrossRef]
- Sherwood, S.C.; Huber, M. An adaptability limit to climate change due to heat stress. Proc. Natl. Acad. Sci. USA 2010, 107, 9552–9555. [Google Scholar] [CrossRef] [PubMed]
- ASHRAE. Thermal Comfort Conditions; ASHRAE Standard 55.66; ASHRAE: New York, NY, USA, 1966. [Google Scholar]
- Epstein, Y.; Moran, D.S. Thermal comfort and the heat stress indices. Ind. Health 2006, 44, 388–398. [Google Scholar] [CrossRef] [PubMed]
- Haldane, J.S. The influence of high air temperatures No. I. Epidemiol. Infect. 1905, 5, 494–513. [Google Scholar] [CrossRef]
- Abdel-Ghany, A.M.; Al-Helal, I.M.; Shady, M.R. Human thermal comfort and heat stress in an outdoor urban arid environment: A case study. Adv. Meteorol. 2013, 2013, 693541. [Google Scholar] [CrossRef]
- Abdel-Ghany, A.M.; Al-Helal, I.M.; Shady, M.R. Effect of the evaporative cooling on the human thermal comfort and heat stress in a greenhouse under arid conditions. Adv. Meteorol. 2013, 2013, 361471. [Google Scholar] [CrossRef]
- Abdel-Ghany, A.M.; Al-Helal, I.M.; Shady, M.R. Evaluation of human thermal comfort and heat stress in an outdoor urban setting in summer under arid climatic conditions. Environ. Prot. Eng. 2014, 40, 3. [Google Scholar] [CrossRef]
- Anuja, N.; Priya Amutha, N. Assessment of thermal comfort in institutional building based on theoretical and experimental analysis. Asian Rev. Civ. Eng. 2016, 5, 7–12. [Google Scholar]
- Ali, S.B.; Patnaik, S. Thermal comfort in urban open spaces: Objective assessment and subjective perception study in the tropical city of Bhopal, India. Urban Clim. 2018, 24, 954–967. [Google Scholar] [CrossRef]
- Aghamohammadi, N.; Fong, C.S.; Idrus, M.H.M.; Ramakreshnan, L.; Haque, U. Outdoor thermal comfort and somatic symptoms among students in a tropical city. Sustain. Cities Soc. 2021, 72, 103015. [Google Scholar] [CrossRef]
- Fairey, P.W. Passive Cooling and Human Comfort; Florida Solar Energy Center: Cocoa, FL, USA, 1981. [Google Scholar]
- Budd, G.M. Wet-bulb globe temperature (WBGT)—Its history and its limitations. J. Sci. Med. Sport 2008, 11, 20–32. [Google Scholar] [CrossRef]
- Sherwood, S.C. How important is humidity in heat stress? J. Geophys. Res. Atmos. 2018, 123, 808–810. [Google Scholar] [CrossRef]
- Pal, J.; Eltahir, E. Future temperature in southwest Asia is projected to exceed a threshold for human adaptability. Nat. Clin. Chang. 2016, 6, 197–200. [Google Scholar] [CrossRef]
- Raymond, C.; Singh, D.; Horton, R.M. Spatiotemporal patterns and synoptics of extreme wet-bulb temperature in the contiguous United States. J. Geophys. Res. Atmos. 2017, 122, 13–108. [Google Scholar] [CrossRef]
- Raymond, C.; Matthews, T.; Horton, R.M. The emergence of heat and humidity is too severe for human tolerance. Sci. Adv. 2020, 6, eaaw1838. [Google Scholar] [CrossRef]
- Zhang, Y.; Held, I.; Fueglistaler, S. Projections of tropical heat stress constrained by atmospheric dynamics. Nat. Geosci. 2021, 14, 133–137. [Google Scholar] [CrossRef]
- Vecellio, D.J.; Wolf, S.T.; Cottle, R.M.; Kenney, W.L. Evaluating the 35 °C wet-bulb temperature adaptability threshold for young, healthy subjects (PSU HEAT Project). J. Appl. Physiol. 2021, 132, 340–345. [Google Scholar] [CrossRef]
- Vecellio, D.J.; Wolf, S.T.; Cottle, R.M.; Kenney, W.L. Suitability of thermal indices in describing heat stress compensability. FASEB J. 2022, 36, R5194. [Google Scholar] [CrossRef]
- Anders, C. Wet-Bulb Temperature Is Important, Climate Experts Say. So What Is It? 2021. Available online: https://www.washingtonpost.com/weather/2021/07/24/wet-bulb-temperature-extreme-heat/ (accessed on 24 July 2021).
- Reiners, P.W. Wet Bulb Temperature. Available online: https://www.salon.com/2021/07/18/wet-bulb-temperature-climate-change/ (accessed on 26 July 2021).
- Prevost-Manuel, J. How Hot Is Too Hot for Humans? Understanding Wet-Bulb Temperatures. Available online: https://www.cbc.ca/news/science/how-hot-is-too-hot-for-humans-understanding-wet-bulb-temperatures-1.6088415 (accessed on 30 May 2022).
- Chow, D. Deadly ‘Wet-Bulb Temperatures’ Are Being Stoked by Climate Change and Heat Waves. NBC News. Available online: https://www.nbcnews.com/science/science-news/wet-bulb-temperature-weather-average-climate-human-heat-wave-rcna27478 (accessed on 7 July 2022).
- Sirur, S. What Are Wet Bulb Temperatures, and Why They Probably Won’t Cross 35°C Long Enough to Be Lethal? Available online: https://theprint.in/environment/what-are-wet-bulb-temperatures-and-why-they-probably-wont-cross-35c-long-enough-to-be-lethal/951637/ (accessed on 24 May 2022).
- Buis, A. Too Hot to Handle: How Climate Change May Make Some Places Too Hot to Live. NASA. Available online: https://climate.nasa.gov/ask-nasa-climate/3151/too-hot-to-handle-how-climate-change-may-make-some-places-too-hot-to-live/ (accessed on 9 July 2022).
- Ganaie, A. Explained! How Harmful Is Rising Incidence of Fatal Wet-Bulb Temperature for Humans? Available online: https://www.digpu.com/health/surge-in-measles-cases-mandates-catch-up-immunization-campaigns (accessed on 30 July 2022).
- The Economist. The Increasing Frequency of Fatal Wet-Bulb Temperatures. Available online: https://www.economist.com/the-economist-explains/2022/05/13/the-increasing-frequency-of-fatal-wet-bulb-temperatures? (accessed on 13 July 2022).
- Alfano, F.R.D.A.; Palella, B.I.; Riccio, G. On the problems related to natural wet bulb temperature indirect evaluation for the assessment of hot thermal environments by means of WBGT. Ann. Occup. Hyg. 2012, 56, 1063–1079. [Google Scholar]
- Golbabaei, F.; Asour, A.A.; Kolahdoozi, M.; Mohammadiyan, M. The limitations of WBGT index for application in industries: A systematic review. J. Occup. Hyg. Eng. 2021, 13, 365–381. [Google Scholar] [CrossRef]
- Wernecke, R.; Wernecke, J. Industrial Moisture and Humidity Measurement: A Practical Guide; Wiley: Hoboken, NJ, USA, 2014. [Google Scholar]
- Wiederhold, P.R. Water Vapor Measurement: Methods and Instrumentation; CRC Press: Boca Raton, FL, USA, 2012. [Google Scholar]
- Lu, H.; Chen, C. Uncertainty evaluation of humidity sensors calibrated by saturated salt solutions. Measurement 2007, 40, 591–599. [Google Scholar] [CrossRef]
- Stull, R. Wet-bulb temperature from relative humidity and air temperature. J. Appl. Meteorol. Climatol. 2011, 50, 2267–2269. [Google Scholar] [CrossRef]
- Raymond, M. Classical and Modern Regression with Applications, 2nd ed.; Duxbury: Pacific Grove, CA, USA, 2000. [Google Scholar]
- Ellison, S.L.; Williams, A. EURACHEM/CITAC Guide CG4: Quantifying Uncertainty in Analytical Measurement, 3rd ed.; EURACHEM: Teddington, UK, 2012. [Google Scholar]
- Iso, I.; Oiml, B. BIMP, IEC, IFCC, ISO, IUPAC, IUPAP, OIML. In Guide to the Expression of Uncertainty in Measurement; ISO: Geneva, Switzerland, 1993. [Google Scholar]
- ISO/IEC 98-3; Uncertainty of Measurement—Part 3: Guide to the Expression of Uncertainty in Measurement. ISO Edition: Geneva, Switzerland, 2010.
- Chen, C. Evaluation of measurement uncertainty for thermometers with calibration equations. Accredit. Qual. Assur. 2006, 11, 75–82. [Google Scholar] [CrossRef]



| Criteria | Model I | Model II | Stull Eq. |
|---|---|---|---|
| 0.07212 | 0.04706 | 0.86078 | |
| −0.05860 | −0.029848 | −0.15988 | |
| 0.01683 | 0.01195 | 0.21357 | |
| 0.02145 | 0.01576 | 0.2860 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, H.-Y.; Chen, C.-C. An Empirical Equation for Wet-Bulb Temperature Using Air Temperature and Relative Humidity. Atmosphere 2022, 13, 1765. https://doi.org/10.3390/atmos13111765
Chen H-Y, Chen C-C. An Empirical Equation for Wet-Bulb Temperature Using Air Temperature and Relative Humidity. Atmosphere. 2022; 13(11):1765. https://doi.org/10.3390/atmos13111765
Chicago/Turabian StyleChen, Hsuan-Yu, and Chia-Chung Chen. 2022. "An Empirical Equation for Wet-Bulb Temperature Using Air Temperature and Relative Humidity" Atmosphere 13, no. 11: 1765. https://doi.org/10.3390/atmos13111765
APA StyleChen, H.-Y., & Chen, C.-C. (2022). An Empirical Equation for Wet-Bulb Temperature Using Air Temperature and Relative Humidity. Atmosphere, 13(11), 1765. https://doi.org/10.3390/atmos13111765






